Unstable Leader Inception Criteria of Atmospheric Discharges
Abstract
:1. Introduction
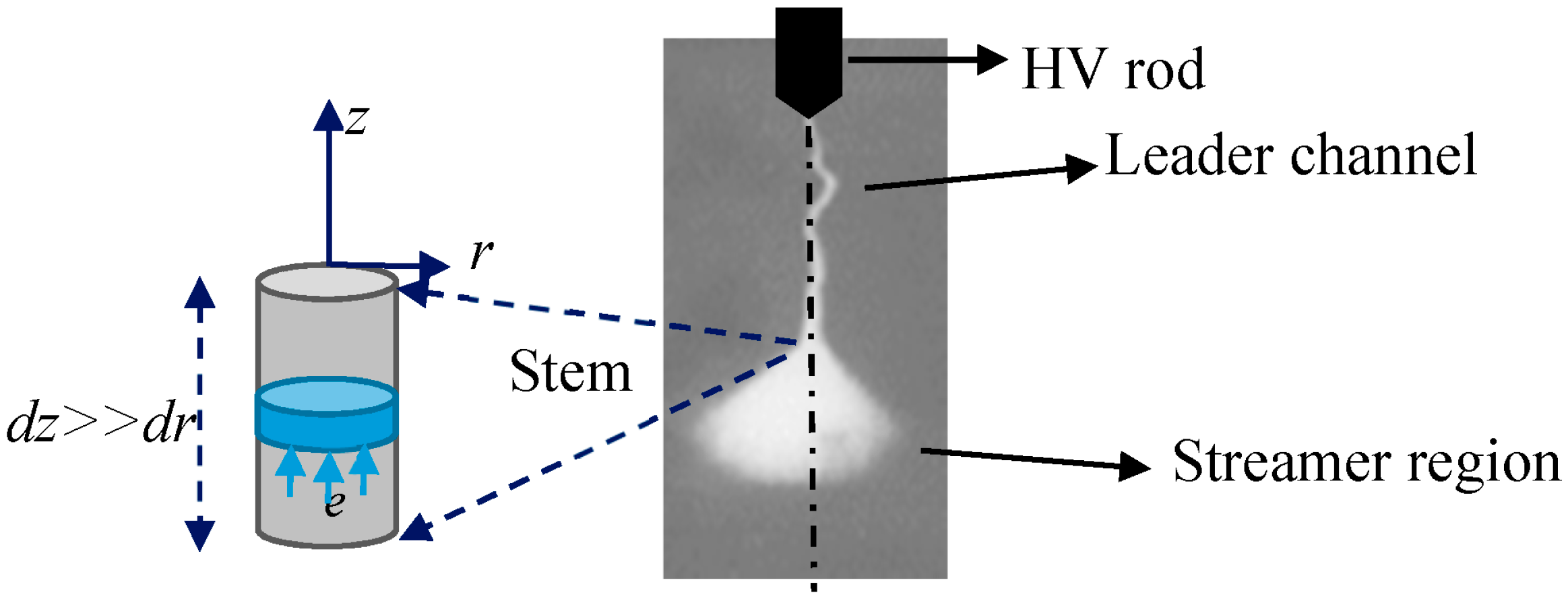
2. Model Formulation
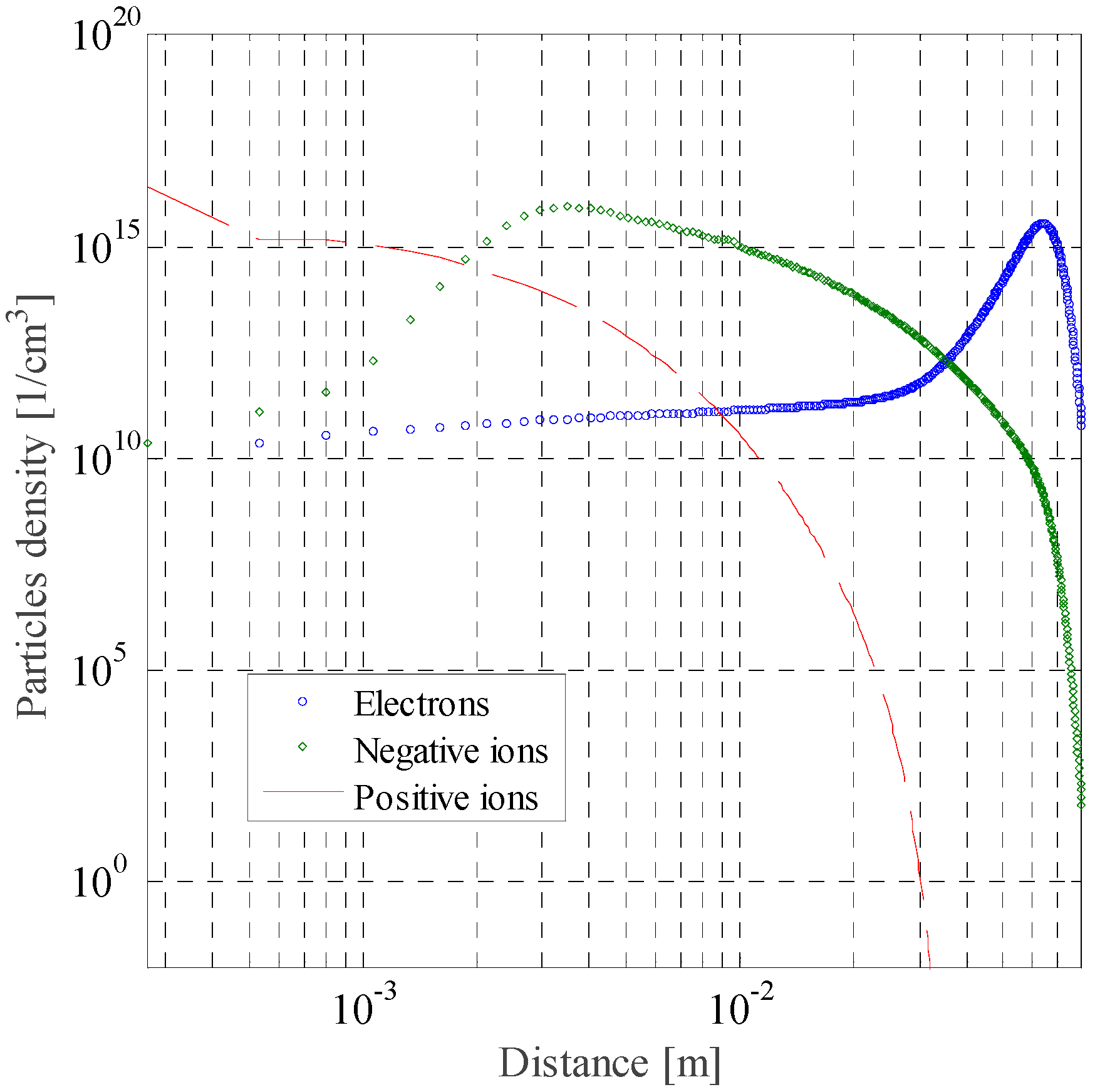
2.1. Continuity Equations of Particles
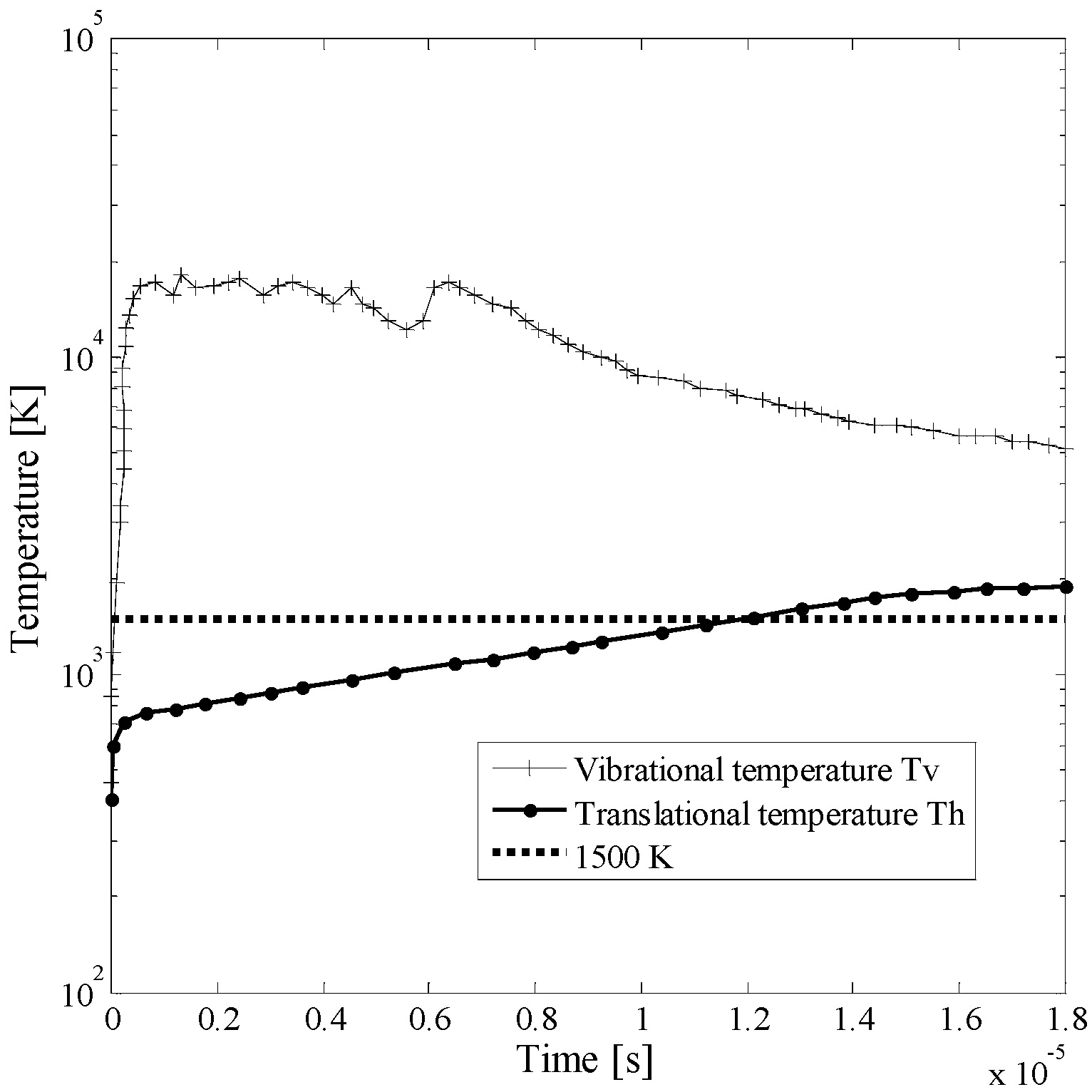
2.2. Gas Heating
3. Validation of the Model
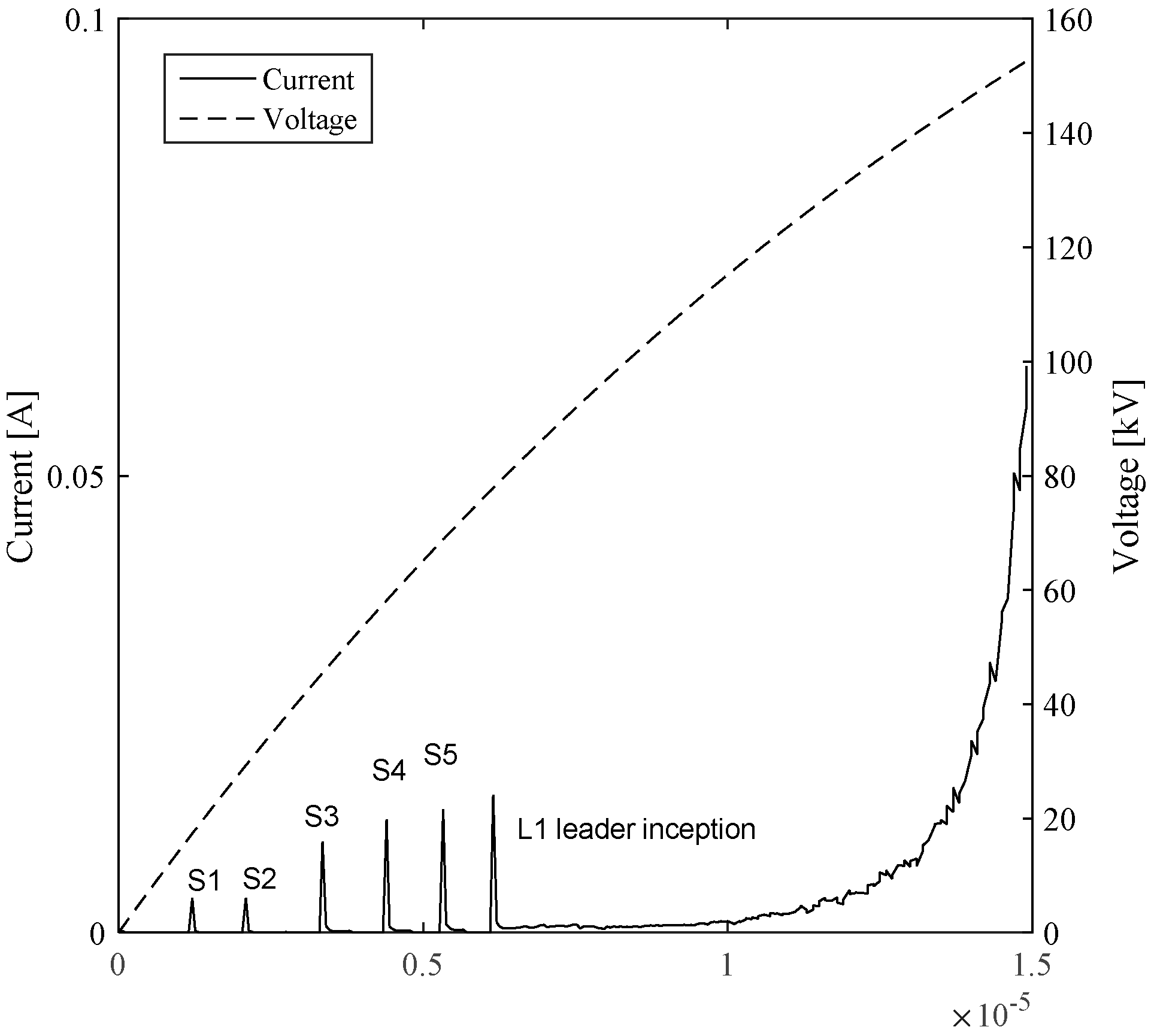
3.1. Discharge Characteristics
3.2. Temperature Change—Leader Inception
4. Role of the Vibrational Energy and Relation to Minimum Charge Required for Leader Inception
Charge Required to Leader Inception
5. Conclusions
- Photoionization is essential for the development and propagation of positive streamers. It enables the seed electrons in the high electric field region at the head of the streamer.
- The most important mechanisms to increase the electron density, and consequently incept a leader, were the fast electron detachment from negative ions caused by oxygen atoms and the acceleration of the electron impact ionization due to NO molecules. These processes lead to an increase of electron density and a decrease of the local electric field.
- The rise of temperature on the channel directly depended on the energy available at the streamer channel, the electric field reduction and the vibrational energy relaxation, and the recombination of particles, which were the principal processes to limit the energy input into the leader. Consequently, it is incorrect to assume that all streamer energy is directly used for heating and a unique amount of charge is required to heat the channel and incept a leader.
- Calculations indicate that sharp tips allow more charge to flow into the channel before leader inception takes place than blunt tips. Therefore, the amount of electrical charge required to achieve leader inception conditions varies and depends on the electric field distribution of the arrangement, i.e., geometry, applied voltage, the space charge, and environmental conditions, among others.
- The study shows that the criterion of a constant minimum electrical charge of 1 μC to incept a leader channel, used in lightning attachment and long gap discharge models, is not well-founded and it disregards part of the physics of the discharge. Leader inception depends on the amount of energy injected into the channel used for heating processes.
Author Contributions
Conflicts of Interest
Appendix A
| Reaction | Rate Coefficient (cm3/s or cm6/s) | |
|---|---|---|
| Ionization | ||
| Direct ionization α | ||
| (A1) | 4.9 × 10−9 exp (–657/(E/N))F | |
| (A2) | 8.1 × 10−9 exp (–925/(E/N))F | |
| Stepwise ionization α′ | ||
| (A3) | 5.0 × 10−9 exp (–460/(E/N))F | |
| (A4) | 4.0 × 10−9 exp (–713/(E/N))F | |
| Associative ionization α″ | ||
| (A5) | 1.9 × 10−9 | |
| (A6) | 1.4 × 10−10 | |
| (A7) | 2.6 × 10−17 T1.43 exp (–31140/T) | |
| Electron-ion recombination β | ||
| (A8) | 2.0 × 10−7 (300/Te)0.7 | |
| (A9) | 2.8 × 10−7 (300/Te)0.5 | |
| (A10) | 2.3 × 10−6 Te–0.45 | |
| Electron attachment η | ||
| (A11) | ||
| Electron detachment D and D* | ||
| (A12) | 5 × 10−10 | |
| (A13) | ||
| Electron impact dissociation | ||
| (A14) | k(E/N)F | |
| (A15) | 4.4 × 10−11 k(E/N)F | |
| (A16) | 6.4 × 10−10 | |
| Electron impact excitation | ||
| (A17) | 1.1 × 10−10 | |
| (A18) | 3.6 × 10−10 | |
| Chemical reactions | ||
| (A19) | 2.54 × 10−12 | |
| (A20) | 3.0 × 10−10 | |
| (A21) | 2.1 × 10−11 | |
| (A22) | 7.0 × 10−12 | |
| (A23) | 10−12T−0.5 | |
| (A24) | ||
| (A25) | ||
| (A26) | ||
| Reaction | εj* [eV] | |
|---|---|---|
| (A27) | 12.08 | |
| (A28) | 15.58 | |
| (A29) | 9.26 | |
| (A30) | 13.62 | |
| (A31) | 6.17 | |
| (A32) | 7.35 | |
| (A33) | 5.9 |
References
- Baldo, G.; Gallimberti, I.; Garcia, H.N.; Hutzler, B.; Jouaire, J.; Simon, M.F. Breakdown phenomena of long gaps under switching impulse conditions influence of distance and voltage level. IEEE Trans. Power Appar. Syst. 1975, 94, 1131–1140. [Google Scholar] [CrossRef]
- Jones, B. Switching Surges and Air Insulation. Philos. Trans. R. Soc. Lond. Ser. A Math. Phys. Sci. 1973, 275, 165–180. [Google Scholar] [CrossRef]
- Carrara, G.; Thione, L. Switching surge strength of large air gaps: A physical approach. IEEE Trans. Power Appar. Syst. 1976, 95, 512–524. [Google Scholar] [CrossRef]
- Rizk, F.A.M. Switehing impulse strength of air insulation: Leader inception criterion. IEEE Power Eng. Rev. 1989, 9, 60–61. [Google Scholar] [CrossRef]
- Goelian, N.; Lalande, P.; Bacchiega, G.L.; Gazzani, A.; Gallimberti, I. A simplified model for the simulation of positive-spark development in long air gaps. Appl. Phys. 1997, 30, 2441–2452. [Google Scholar] [CrossRef]
- Hutzler, B.; Hutzler-Barre, D. Leader Propagation Model for Predetermination of Switching Surge Flashover Voltage of Large Air Gaps. IEEE Trans. Power Appar. Syst. 1978, PAS-97, 1087–1096. [Google Scholar] [CrossRef]
- Gallimberti, I. The mechanism of the long spark formation. J. Phys. Colloq. 1979, 40, 193–250. [Google Scholar] [CrossRef]
- Vidal, F.; Gallimberti, I.; Rizk, F.A.M.; Johnston, T.W.; Bondiou-Clergerie, A.; Comtois, D.; Kieffer, J.C.; La Fontaine, B.; Mercure, H.P.; Pépin, H. Modeling of the air plasma near the tip of the positive leader. IEEE Trans. Plasma Sci. 2002, 30, 1339–1349. [Google Scholar] [CrossRef]
- Popov, N.A. Formation and development of a leader channel in air. Plasma Phys. Rep. 2003, 29, 695–708. [Google Scholar] [CrossRef]
- Bazelyan, E.M.; Yu, P.R.; Aleksandrov, N.L. The effect of reduced air density on streamer-to-leader transition and on properties of long positive leader. J. Phys. D Appl. Phys. 2007, 40, 4133. [Google Scholar] [CrossRef]
- Marode, E.; Bastien, F.; Bakker, M. A model of the streamer-induced spark formation based on neutral dynamics. J. Appl. Phys. 1979, 50, 140–146. [Google Scholar] [CrossRef]
- Aleksandrov, N.L.; Bazelyan, E.M.; Raizer, Y.P. Initiation and development of first lightning leader : The effects of coronae and position of lightning origin. Atmos. Res. 2005, 76, 307–329. [Google Scholar] [CrossRef]
- Bondiou, A.; Gallimberti, I. Theoretical modelling of the development of the positive spark in long gaps. J. Phys. D Appl. Phys. 1994, 27, 1252–1266. [Google Scholar] [CrossRef]
- Beccerra, M.; Cooray, V. A simplified physical model to determine the lightning upward connecting leader inception. IEEE Trans. Power Deliv. 2006, 21, 897–908. [Google Scholar] [CrossRef]
- Naidis, G.V. Dynamics of streamer breakdown of short non-uniform air gaps. J. Phys. D Appl. Phys. 2005, 38, 3889–3893. [Google Scholar] [CrossRef]
- Naidis, G.V. Simulation of streamer-to-spark transition in short non-uniform air gaps. J. Phys. D Appl. Phys. 1999, 32, 2649–2654. [Google Scholar] [CrossRef]
- Popov, N.A. Study of the formation and propagation of a leader channel in air. Plasma Phys. Rep. 2009, 35, 785–793. [Google Scholar] [CrossRef]
- Lalande, P.; Bondiou-Clergerie, A.; Bacchiega, G.; Gallimberti, I. Observations and modeling of lightning leaders. Comptes Rendus Phys. 2002, 3, 1375–1392. [Google Scholar] [CrossRef]
- Arevalo, L.; Wu, D.; Jacobson, B. A consistent approach to estimate the breakdown voltage of high voltage electrodes under positive switching impulses. J. Appl. Phys. 2013, 114, 83301. [Google Scholar] [CrossRef]
- Arevalo, L. Numerical Simulations of Long Spark and Lightning Attachment; Uppsala University: Uppsala, Sweden, 2011. [Google Scholar]
- Becerra, M.; Cooray, V.; Hartono, Z.A. Identification of lightning vulnerability points on complex grounded structures. J. Electrost. 2007, 65, 562–570. [Google Scholar] [CrossRef]
- Arevalo, L.; Cooray, V. Streamer to leader transition criteria for propagation of long sparks and lightning leaders. In Proceedings of the 2014 International Conference on Lightning Protection (ICLP), Shanghai, China, 11–18 October 2014; Institute of Electrical and Electronics Engineers Inc.: Piscataway, NJ, USA, 2014; pp. 480–483. [Google Scholar]
- Arevalo, L.; Cooray, V. Upward leader inception caused by a sudden change of cloud electric field. In Proceedings of the 2014 International Conference on Lightning Protection (ICLP), Shanghai, China, 11–18 October 2014; Institute of Electrical and Electronics Engineers Inc.: Piscataway, NJ, USA, 2014; pp. 484–487. [Google Scholar]
- Arevalo, L.; Cooray, V. Corona charge produced by thundercloud fields in grounded rods. In Proceedings of the 2012 International Conference on Lightning Protection (ICLP), Vienna, Austria, 2–7 September 2012; pp. 1–6. [Google Scholar]
- Arévalo, L.; Becerra, M.; Román, F. Positive corona current simulation in a non-uniform coaxial arrangement. WSEAS Trans. Inf. Sci. Appl. 2005, 2, 1595–1606. [Google Scholar]
- Bazelyan, É.M.; Raizer, Y.P. Spark Discharge; CRC Press: Boca Raton, FL, USA, 1997; ISBN 9780849328688. [Google Scholar]
- Aleksandrov, N.; Bazyelan, E.; Kochetov, I.; Dyatko, N. The ionization kinetics and electric field in the leader channel in long air gaps. J. Phys. D Appl. Phys. 1997, 30, 1616–1624. [Google Scholar] [CrossRef]
- Dhali, S.K.; Williams, P.F. Two-dimensional studies of streamers in gases. J. Appl. Phys. 1987, 62, 4696–4707. [Google Scholar] [CrossRef]
- Luque, A.; Ratushnaya, V.; Ebert, U. Positive and negative streamers in ambient air: Modelling evolution and velocities. J. Phys. D Appl. Phys. 2008, 41, 234005. [Google Scholar] [CrossRef]
- Group, L.R. Research on long gap discharges at Les Renardières. Electra 1972, 23, 53–157. [Google Scholar]
- Popov, N.A. Investigation of the mechanism for rapid heating of nitrogen and air in gas discharges. Plasma Phys. Rep. 2001, 27, 886–896. [Google Scholar] [CrossRef]
- Legler, W. Anregung von UV-Strahlung in Stickstoff und Wasserstoff durch einen Elektronenschwarm. Z. Phys. 1963, 173, 169–183. [Google Scholar] [CrossRef]
- Davies, A.J.; Evans, C.J.; Townsend, P.; Woodison, P.M. Computation of axial and radial development of discharges between plane parallel electrodes. Proc. Inst. Electr. Eng. 1977, 124, 179. [Google Scholar] [CrossRef]
- Dresvin, S.; Donskoi, A. Physics and Technology of Low-Temperature Plasmas; Iowa State University Press: Ames, IA, USA, 1977; ISBN 0813819504. [Google Scholar]
- Popov, N.A. Fast gas heating in a nitrogen—Oxygen discharge plasma: I. Kinetic mechanism. J. Phys. D Appl. Phys. 2011, 44, 285201. [Google Scholar] [CrossRef]
- Flitti, A.; Pancheshnyi, S.V. Gas heating in fast pulsed discharges in N–O mixtures. Eur. Phys. J. Appl. Phys. 2009, 45, 21001. [Google Scholar] [CrossRef]
- Kennedy, J.T. Study of the Avalanche to Streamer Transition in Insulating Gases; Eindhoven University of Technology: Eindhoven, The Netherlands, 1995. [Google Scholar]
- Steven, Z. Fully dimensional fluz corrected transport algorithms for fluids. J. Comput. 1979, 31, 335–362. [Google Scholar]
- Morrow, R.; Lowke, J.J. Streamer propagation in air. J. Phys. D Appl. Phys. 1999, 30, 614–627. [Google Scholar] [CrossRef]
- Bastien, F.; Marode, E. Breakdown simulation of electronegative gases in non-uniform field. J. Phys. D Appl. Phys. 1985, 18, 377–393. [Google Scholar] [CrossRef]
- Wu, C.; Xie, S.; Qi, F.; Li, B.; Wan, J.; He, J. Effect of corona discharges on the inception of positive upward leader-streamer system. Int. J. Mod. Phys. B 2013, 27, 1350165. [Google Scholar] [CrossRef]
- Kossyi, I.A.; Kostinsky, A.Y.; Matveyev, A.A.; Silakov, V.P. Kinetic scheme of the non-equilibrium discharge in nitrogen-oxygen mixtures. Plasma Sources Sci. Technol. 1992, 1, 207–220. [Google Scholar] [CrossRef]
- Benilov, M.S.; Naidis, G.V. Modelling of low-current discharges in atmospheric-pressure air taking account of non-equilibrium effects. J. Phys. D Appl. Phys. 2003, 36, 1834–1841. [Google Scholar] [CrossRef]
- Capitelli, M.; Ferreira, C.M.; Gordiets, B.F.; Osipov, A.I. Plasma Kinetics in Atmospheric Gases; Springer: New York, NY, USA, 2000; ISBN 978-3-662-04158-1. [Google Scholar]
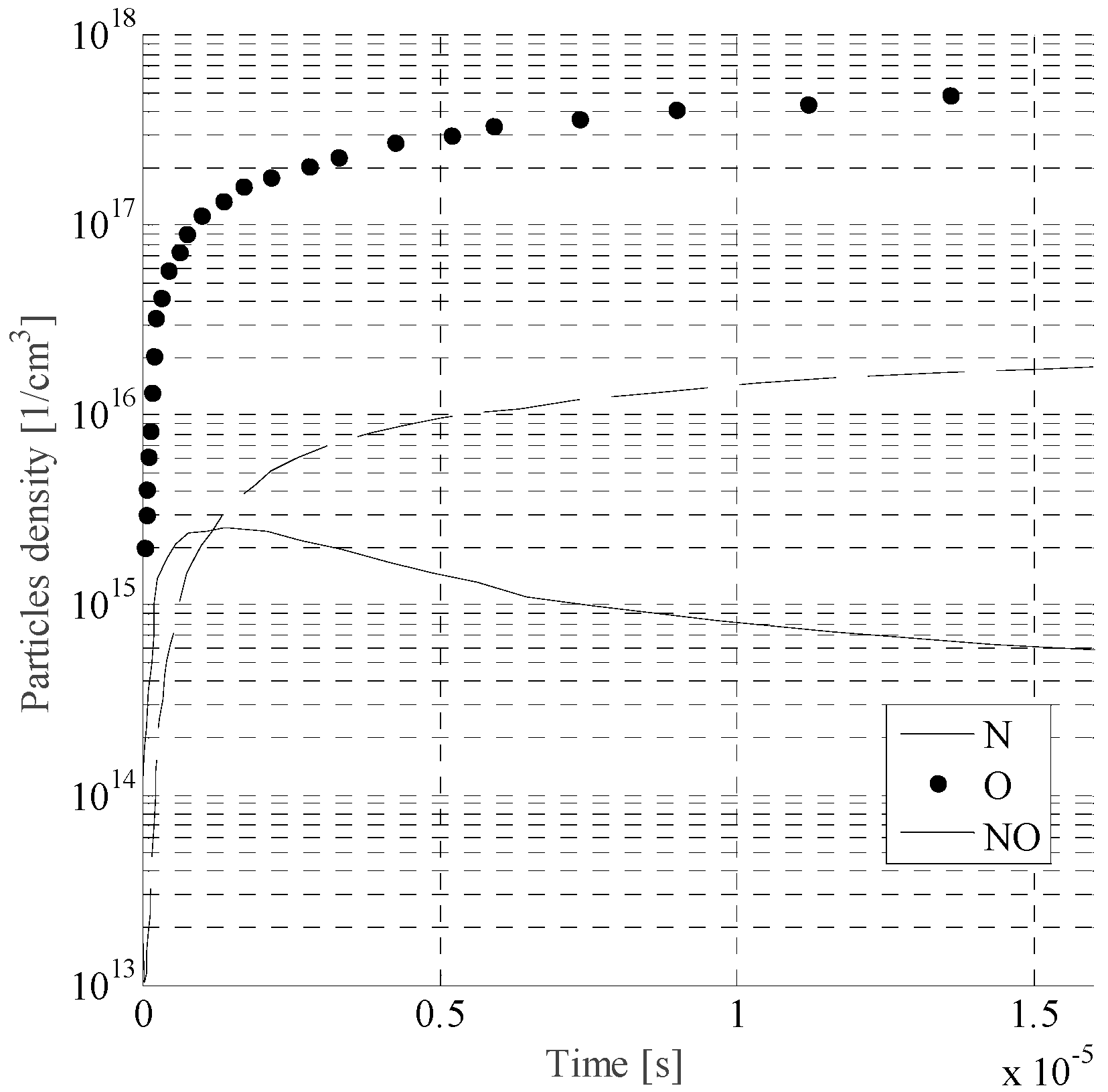
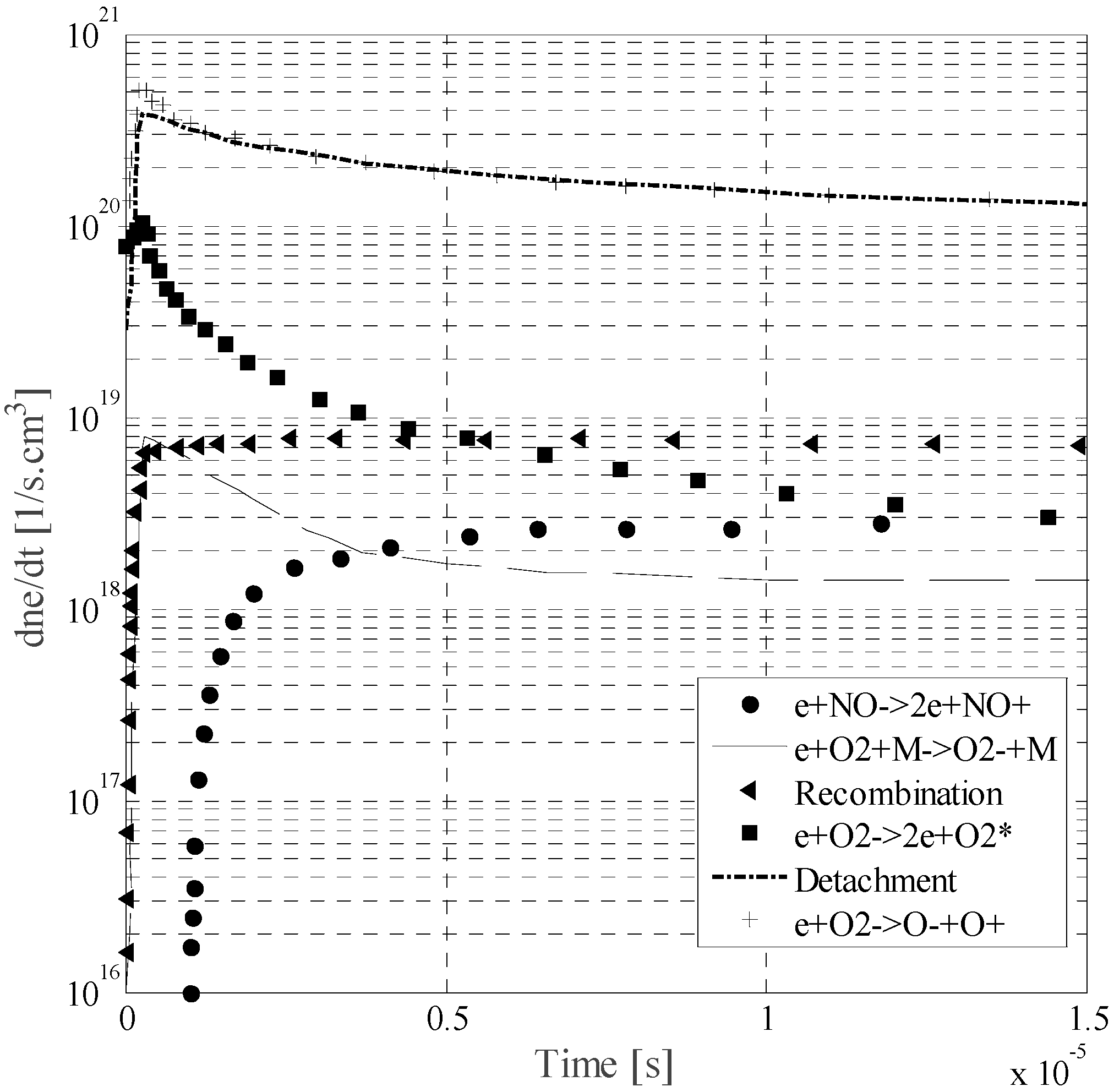
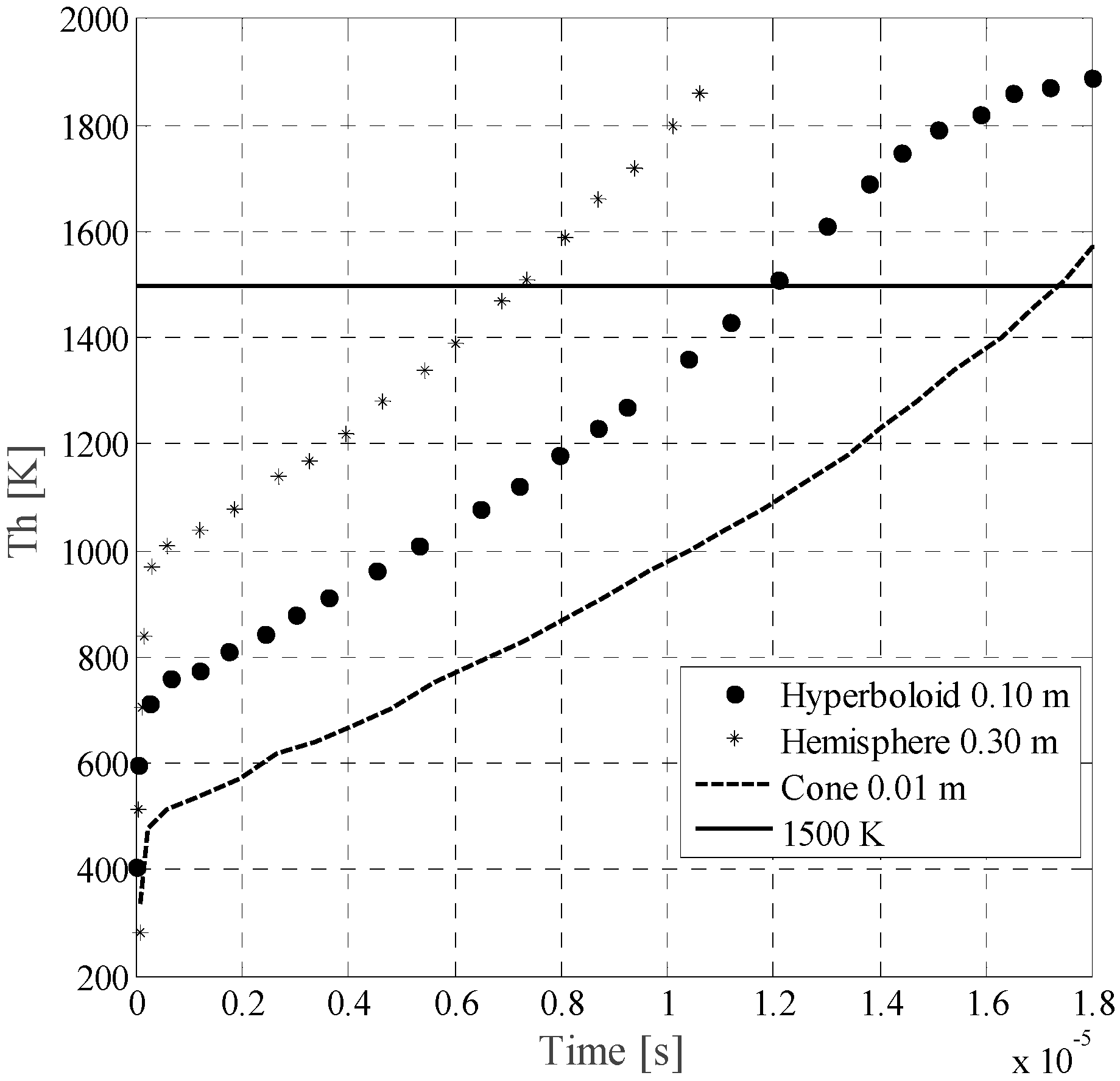
| Tip Radius (m) | 0.01 | 0.10 | 0.30 |
|---|---|---|---|
| 0.98 | 0.86 | 0.72 |
| Tip Radius (m) | 0.30 | 0.10 | 0.01 |
|---|---|---|---|
| Injected at leader inception (μC) | 0.25 | 0.45 | 0.67 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Arevalo, L.; Cooray, V. Unstable Leader Inception Criteria of Atmospheric Discharges. Atmosphere 2017, 8, 156. https://doi.org/10.3390/atmos8090156
Arevalo L, Cooray V. Unstable Leader Inception Criteria of Atmospheric Discharges. Atmosphere. 2017; 8(9):156. https://doi.org/10.3390/atmos8090156
Chicago/Turabian StyleArevalo, Liliana, and Vernon Cooray. 2017. "Unstable Leader Inception Criteria of Atmospheric Discharges" Atmosphere 8, no. 9: 156. https://doi.org/10.3390/atmos8090156
APA StyleArevalo, L., & Cooray, V. (2017). Unstable Leader Inception Criteria of Atmospheric Discharges. Atmosphere, 8(9), 156. https://doi.org/10.3390/atmos8090156






