Dissipation Scaling with a Variable Cϵ Coefficient in the Stable Atmospheric Boundary Layer
Abstract
1. Introduction
2. Theory
2.1. Equilibrium Scaling
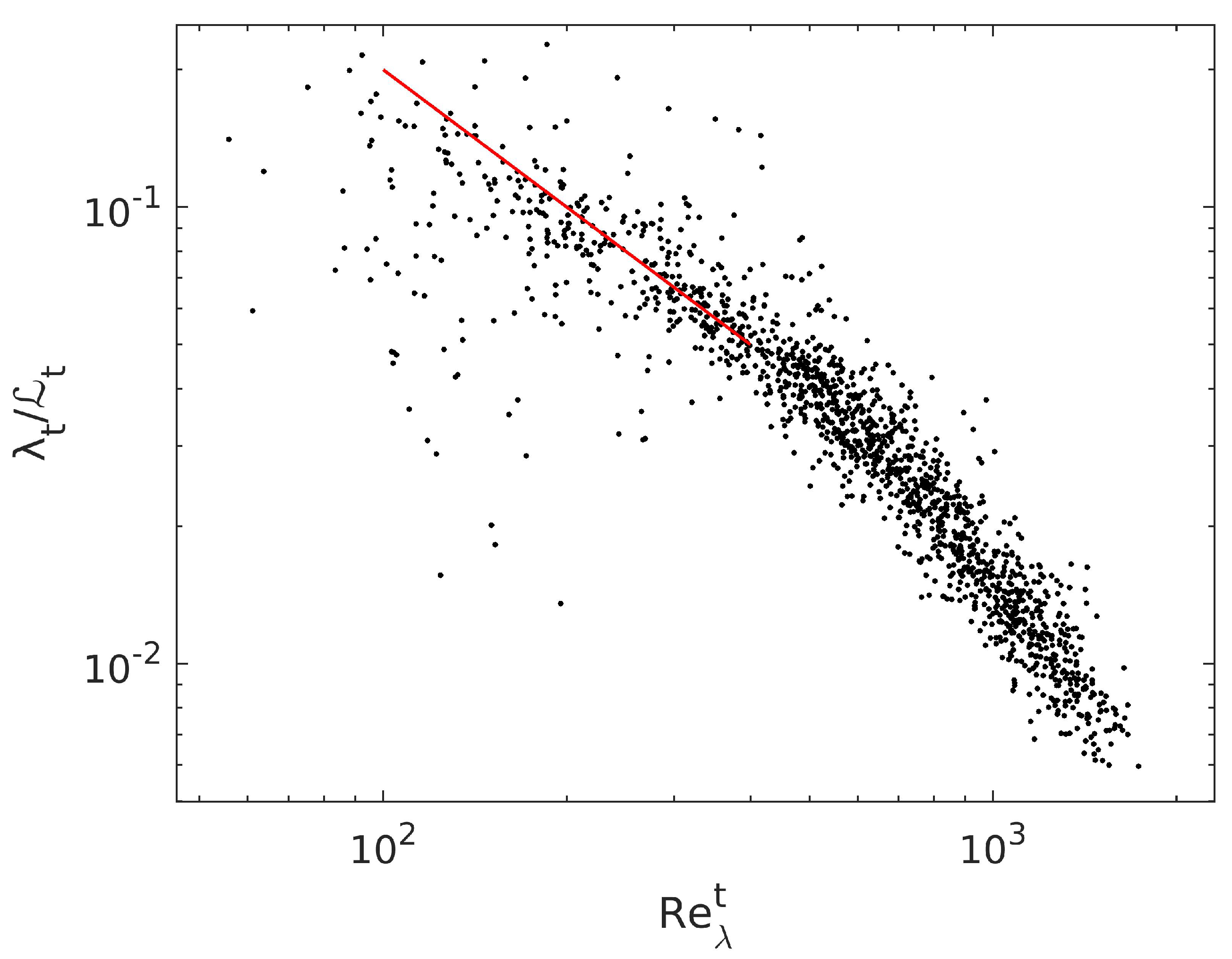
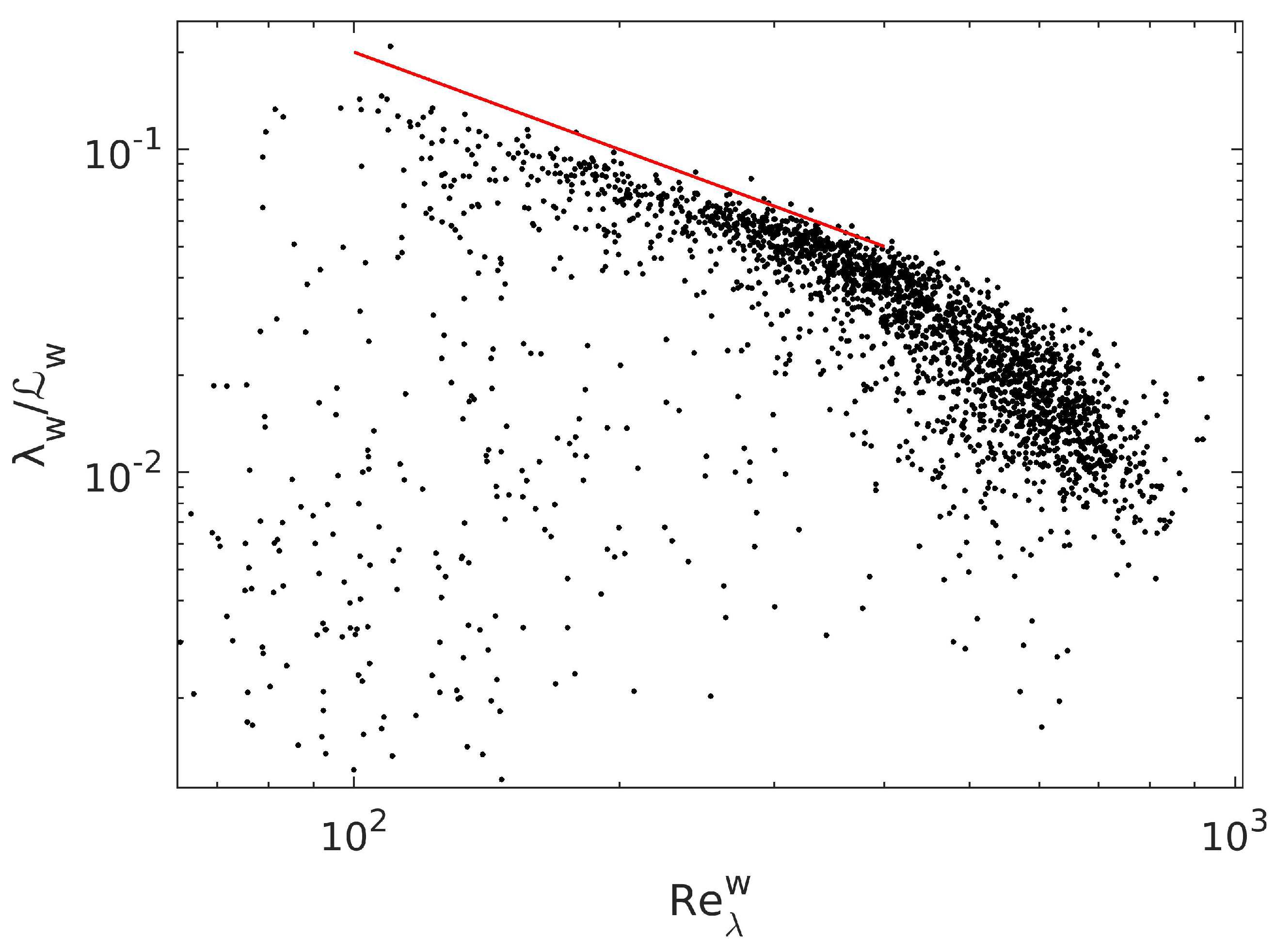
2.2. Non-Equilibrium Scaling
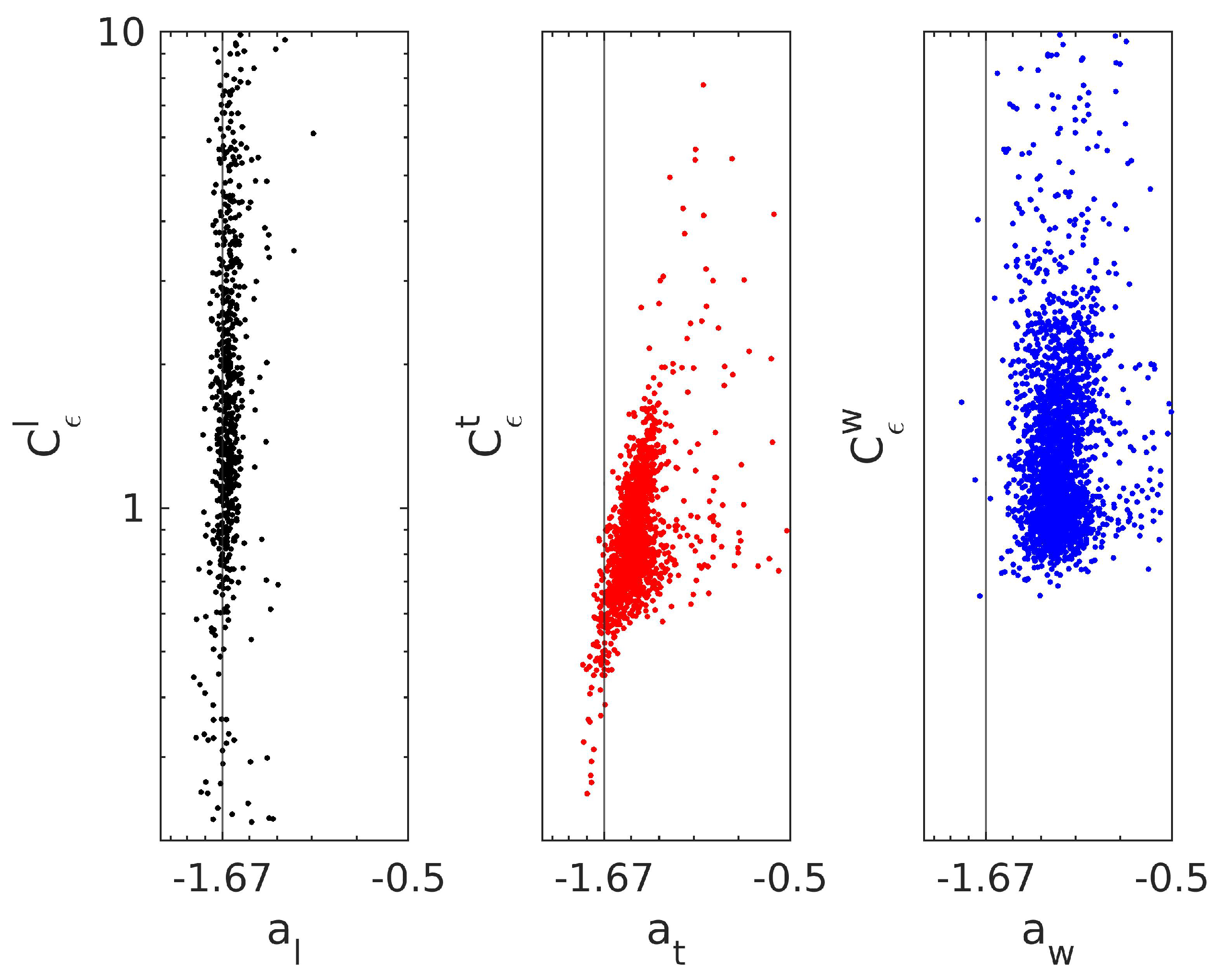
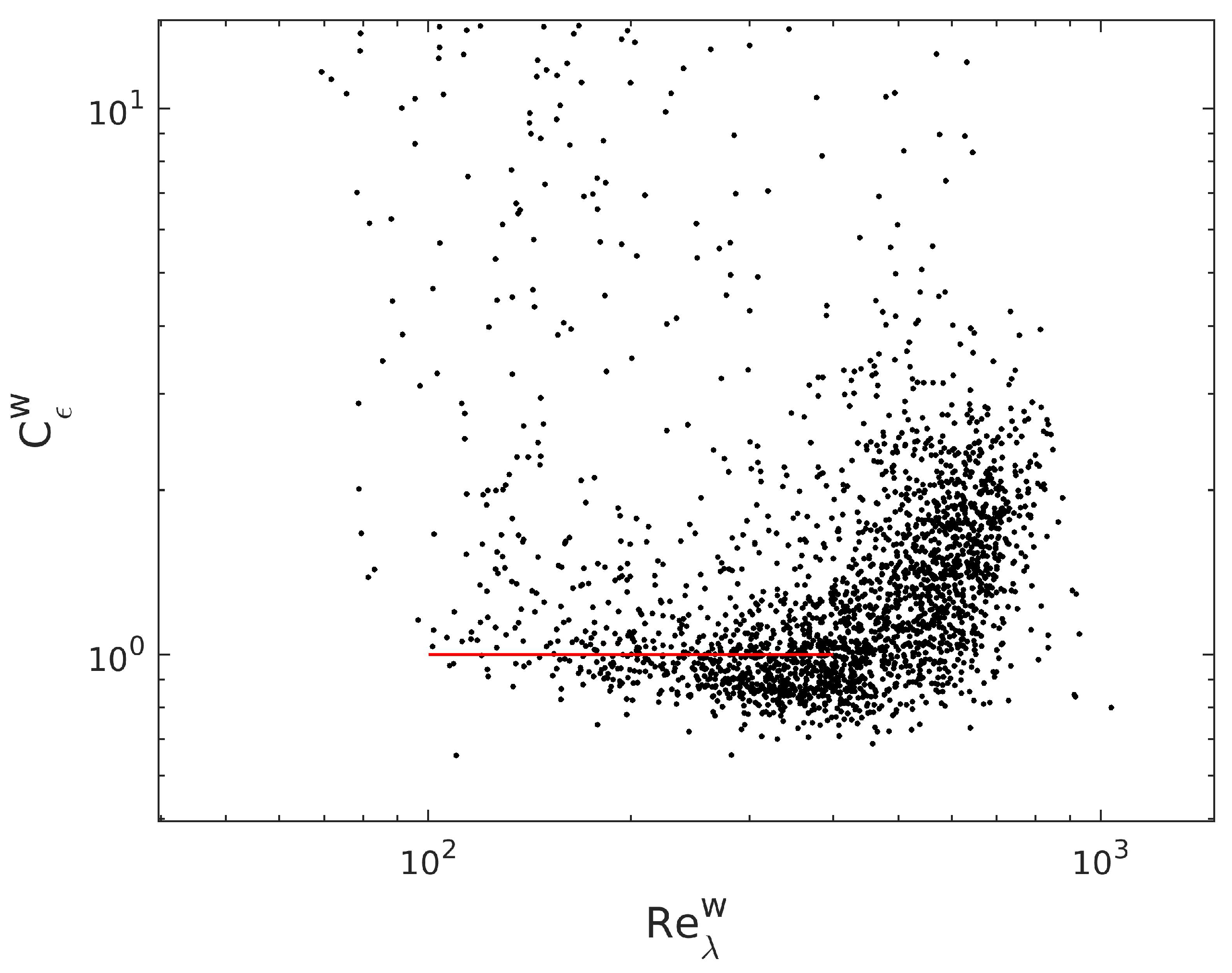
2.3. Dependence of on
3. Data and Methods
3.1. MOSAiC Observations
3.2. Turbulence Statistics
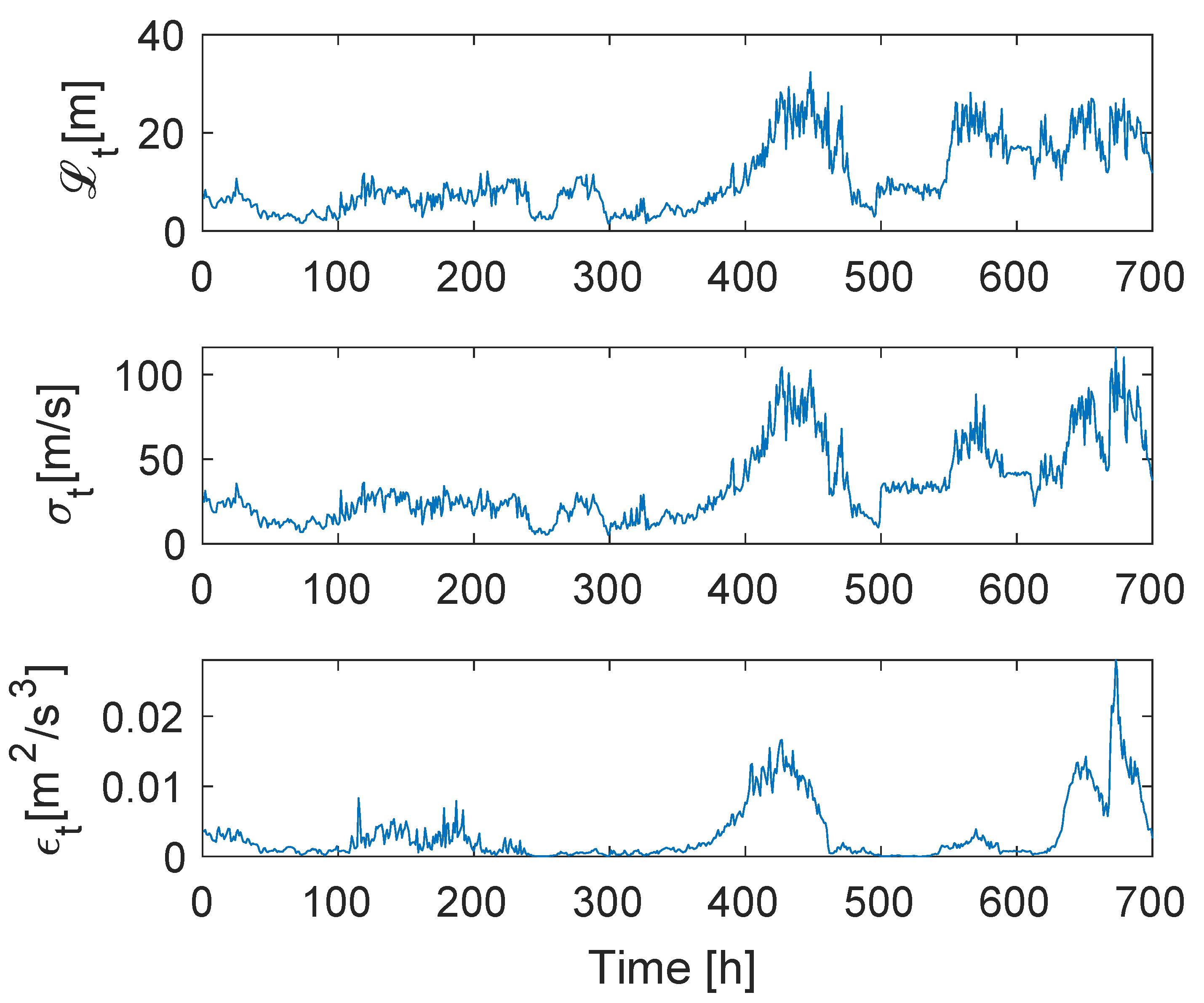
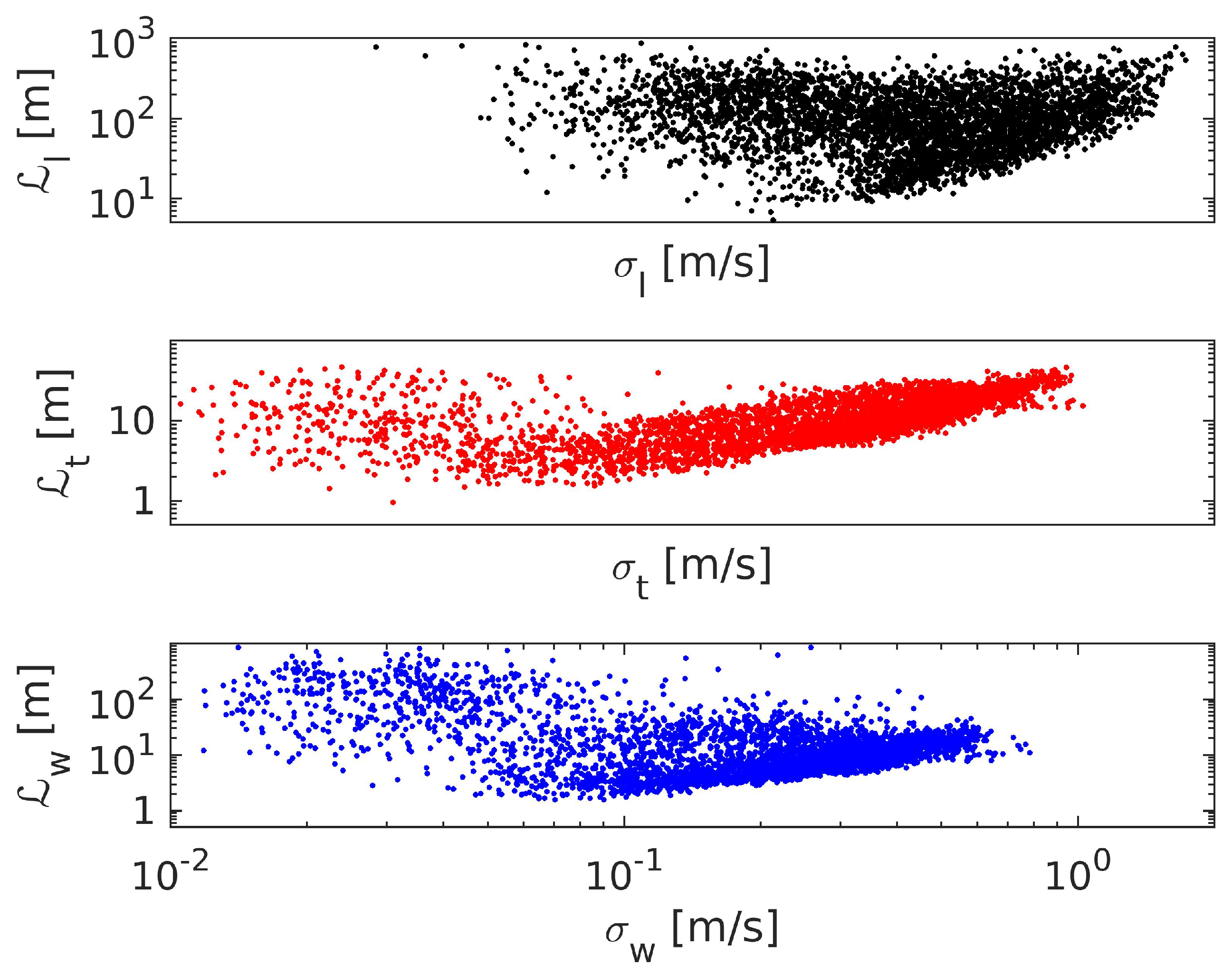
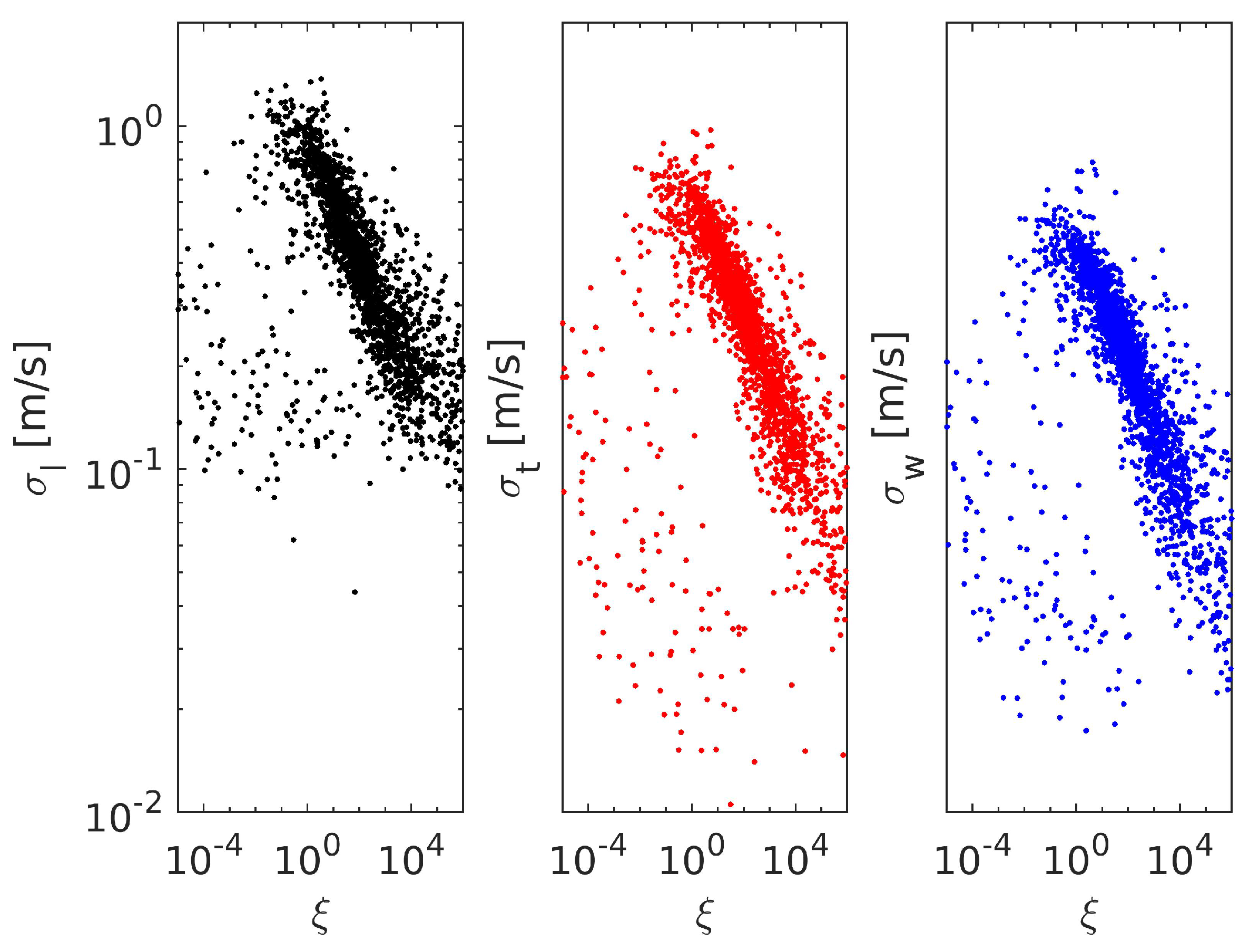
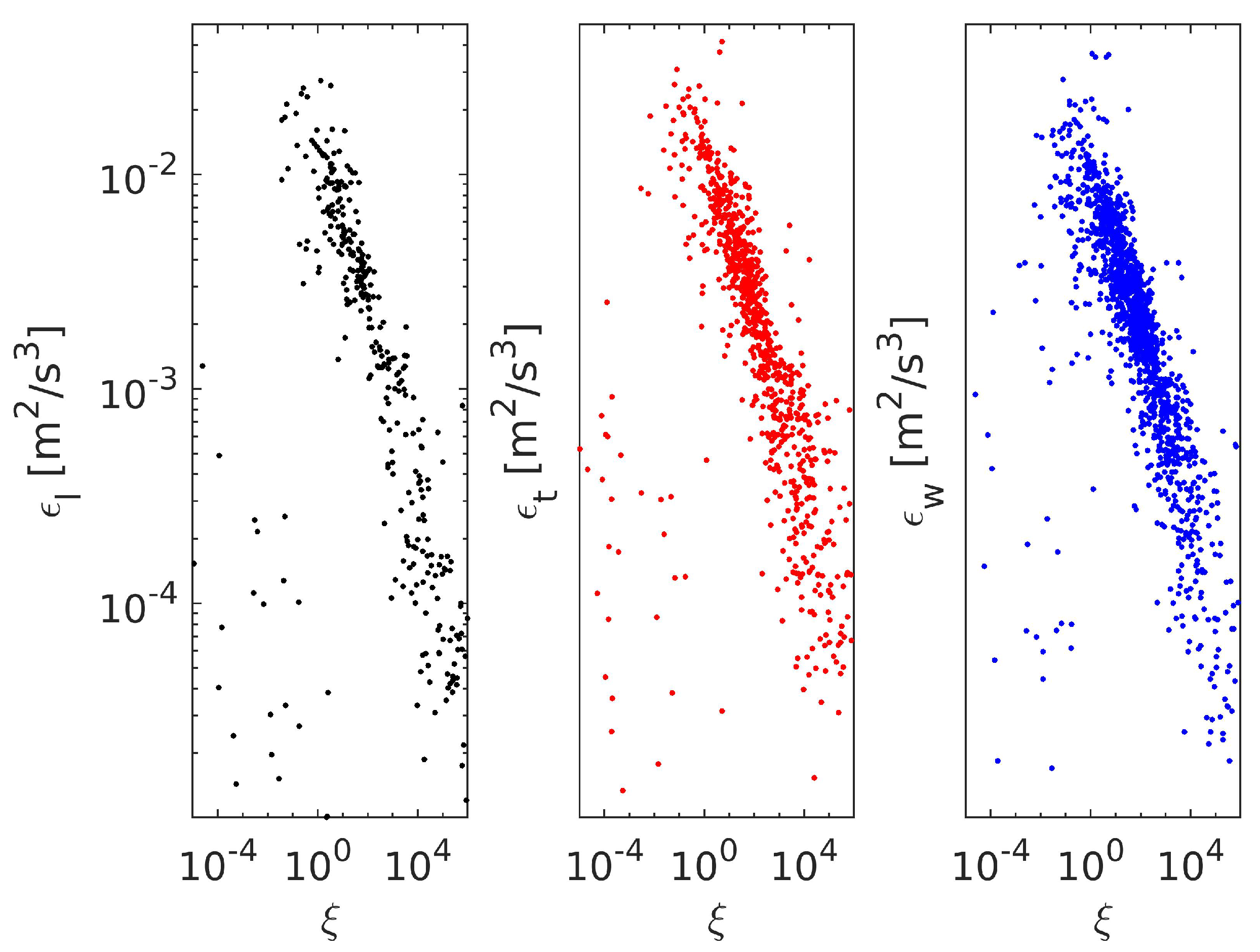
4. Results
5. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| ABL | Atmospheric boundary layer |
| MOSAiC | Multidisciplinary drifting Observatory for the Study of Arctic Climate |
References
- Taylor, G.I. Statistical theory of turbulence. Proc. Roy. Soc. London 1935, A151, 421–444. [Google Scholar] [CrossRef]
- Lv, Y.; Muñoz-Esparza, D.; Chen, X.; Zhang, C.; Luo, M.; Wang, R.; Zhou, B. Stability dependence of the turbulent dissipation rate in the convective atmospheric boundary layer. Geophys. Res. Lett. 2023, 50, e2023GL103326. [Google Scholar] [CrossRef]
- Monin, A.S.; Obukhov, A.M. Basic laws of turbulent mixing in the surface layer of the atmosphere. Tr. Nauk. SSSR Geophiz. Inst. 1954, 24, 163–187. [Google Scholar]
- Blackadar, A.K. The vertical distribution of wind and turbulent exchange in a neutral atmosphere. J. Geophys. Res. 1962, 67, 3095–3102. [Google Scholar] [CrossRef]
- Mellor, G.; Yamada, T. Hierarchy of turbulence closure models for planetary boundary-layers. J. Atmos. Sci. 1974, 31, 1791–1806. [Google Scholar] [CrossRef]
- Valente, P.; Vassilicos, J.C. Universal dissipation scaling for non-equilibrium turbulence. Phys. Rev. Lett. 2012, 108, 214503. [Google Scholar] [CrossRef]
- Vassilicos, J.C. Dissipation in turbulent flows. Annu. Rev. Fluid Mech. 2015, 47, 95–114. [Google Scholar] [CrossRef]
- Dairay, T.; Obligado, M.; Vassilicos, J.C. Non-equilibrium scaling laws in axisymmetric turbulent wakes. J. Fluid Mech. 2015, 781, 166–195. [Google Scholar] [CrossRef]
- Goto, S.; Vassilicos, J.C. Local equilibrium hypothesis and Taylor’s dissipation law. Fluid Dyn. Res. 2016, 48, 021402. [Google Scholar] [CrossRef]
- Goto, S.; Vassilicos, J.C. Unsteady turbulence cascades. Phys. Rev. E 2016, 94, 053108. [Google Scholar] [CrossRef]
- Bos, W.J.T.; Rubinstein, R. Dissipation in unsteady turbulence. Phys. Rev. Fluids 2017, 2, 022601. [Google Scholar] [CrossRef]
- Nedić, J.; Tavoularis, S. Energy dissipation scaling in uniformly sheared turbulence. Phys. Rev. E 2016, 93, 033115. [Google Scholar] [CrossRef] [PubMed]
- Nedić, J.; Tavoularis, S.; Marusic, I. Dissipation scaling in constant–pressure turbulent boundary layers. Phys. Rev. Fluids 2017, 2, 032601. [Google Scholar] [CrossRef]
- Wacławczyk, M.; Nowak, J.L.; Siebert, H.; Malinowski, S.P. Detecting Nonequilibrium States in Atmospheric Turbulence. J. Atmos. Sci. 2022, 79, 2757–2772. [Google Scholar] [CrossRef]
- Obligado, M.; Brun, C.; Silvestrini, J.H.; Schettini, E. Dissipation Scalings in the Turbulent Boundary Layer at Moderate Reθ. Flow Turb. Comb. 2022, 108, 105–122. [Google Scholar] [CrossRef]
- Chowdhuri, S.; Banerjee, T. Quantifying small-scale anisotropy in turbulent flows. Phys. Rev. Fluids 2024, 9, 074604. [Google Scholar] [CrossRef]
- Sorbjan, Z. Local Structure of Turbulence in Stably Stratified Boundary Layers. J. Atmos. Sci. 2006, 63, 1526–1537. [Google Scholar] [CrossRef]
- Mahrt, L. Stably Stratified Atmospheric Boundary Layers. Annu. Rev. Fluid Mech. 2014, 46, 23–45. [Google Scholar] [CrossRef]
- Heisel, M.; Sullivan, P.P.; Katul, G.G.; Chamecki, M. Turbulence Organization and Mean Profile Shapes in the Stably Stratified Boundary Layer: Zones of Uniform Momentum and Air Temperature. Bound.-Layer Meteorol. 2023, 186, 533–565. [Google Scholar] [CrossRef]
- Cox, C.; Gallagher, M.; Shupe, M.; Persson, O.; Blomquist, B.; Grachev, A.; Riihimaki, L.; Kutchenreiter, M.; Morris, V.; Solomon, A. Met City Meteorological and Surface Flux Measurements (Level 3 Final), Multidisciplinary Drifting Observatory for the Study of Arctic Climate (MOSAiC), Central Artic, October 2019–September 2020 [Dataset]. NSF Arctic Data Center. 2023. Available online: https://arcticdata.io/catalog/view/doi:10.18739/A2TM7227K (accessed on 28 December 2024). [CrossRef]
- Schiavon, M.; Barbano, F.; Brogno, L.; Leo, L.S.; Tampieri, F.; Di Sabatino, S. On the parametrizations for the dissipation rate of the turbulence kinetic energy in stable conditions. Bull. Atmos. Sci. Technol. 2023, 4, 3. [Google Scholar] [CrossRef]
- Pope, S.B. Turbulent Flows; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar]
- Du, S.; Sawford, B.L.; Wilson, J.D.; Wilson, D.J. Estimation of the Kolmogorov constant (C0) for the Lagrangian structure function, using a second-order Lagrangian model of grid turbulence. Phys. Fluids 1995, 7, 3083–3090. [Google Scholar] [CrossRef]
- Nakanishi, M. Improvement of the Mellor–Yamada turbulence closure model based on large-eddy simulation data. Bound.-Layer Meteorol. 2001, 99, 349–378. [Google Scholar] [CrossRef]
- Basu, S.; He, P.; DeMarco, A.W. Parametrizing the Energy Dissipation Rate in Stably Stratified Flows. Bound.-Layer Meteorol. 2021, 178, 167–184. [Google Scholar] [CrossRef]
- Mellor, G.L.; Yamada, T. Development of a turbulence closure model for geophysical fluid problems. Rev. Geophys. Space 1982, 20, 851–875. [Google Scholar] [CrossRef]
- Zilitinkevich, S.; Druzhinin, O.; Glazunov, A.; Kadantsev, E.; Mortikov, E.; Repina, I.; Troitskay, Y. Dissipation rate of turbulent kinetic energy in stably stratified sheared flows. Atmos. Chem. Phys. 2019, 19, 2489–2496. [Google Scholar] [CrossRef]
- Sorbjan, Z. Similarity scaling systems for stably stratified turbulent flows. Q. J. R. Meteorol. Soc. 2016, 142, 805–810. [Google Scholar] [CrossRef]
- Łobocki, L.; Porretta-Tomaszewska, P. Prediction of gradient-based similarity functions from the Mellor–Yamada model. Q. J. R. Meteorol. Soc. 2021, 147, 3922–3939. [Google Scholar] [CrossRef]
- Wacławczyk, M.; Yano, J.I.; Florczyk, G.M. Local Similarity Theory as the Invariant Solution of the Governing Equations. Bound.-Layer Meteorol. 2024, 190, 23. [Google Scholar] [CrossRef]
- Yoshizawa, A. Nonequilibrium effect of the turbulent-energy-production process on the inertial-range energy spectrum. Phys. Rev. E 1994, 49, 4065–4071. [Google Scholar] [CrossRef]
- Apostolidis, A.; Laval, J.P.; Vassilicos, J.C. Scalings of turbulence dissipation in space and time for turbulent channel flow. J. Fluid Mech. 2022, 946, A41. [Google Scholar] [CrossRef]
- Steiros, K. Turbulence near initial conditions. Phys. Rev. Fluids 2022, 7, 104607. [Google Scholar] [CrossRef]
- Karasewicz, M.; Wacławczyk, M.; Ortiz-Amezcua, P.; Janicka, Ł.; Poczta, P.; Kassar Borges, C.; Stachlewska, I.S. Investigation of non-equilibrium turbulence decay in the atmospheric boundary layer using Doppler lidar measurements. Atmos. Chem. Phys. 2024, 24, 13231–13251. [Google Scholar] [CrossRef]
- Cox, C.J.; Gallagher, M.R.; Shupe, M.D.; Persson, P.O.G.; Solomon, A.; Fairall, C.W.; Ayers, T.; Blomquist, B.; Brooks, I.M.; Costa, D.; et al. Continuous observations of the surface energy budget and meteorology over the Arctic sea ice during MOSAiC. Sci. Data 2023, 10, 519. [Google Scholar] [CrossRef] [PubMed]
- Cummins, D.P.; Guemas, V.; Cox, C.J.; Gallagher, M.R.; Shupe, M.D. Surface turbulent fluxes from the MOSAiC campaign predicted by machine learning. Geophys. Res. Lett. 2023, 50, e2023GL105698. [Google Scholar] [CrossRef]
- Stiperski, I.; Calaf, M. Generalizing Monin-Obukhov Similarity Theory (1954) for Complex Atmospheric Turbulence. Phys. Rev. Lett. 2023, 130, 124001. [Google Scholar] [CrossRef]
- Peng, S.; Yang, Q.; Shupe, M.D.; Han, B.; Chen, D.; Liu, C. The vertical structure of turbulence kinetic energy near the Arctic sea-ice surface. Geophys. Res. Lett. 2024, 51, e2024GL110792. [Google Scholar] [CrossRef]
- Schröder, M.; Bätge, T.; Bodenschatz, E.; Wilczek, M.; Bagheri, G. Estimating the turbulent kinetic energy dissipation rate from one-dimensional velocity measurements in time. Atmos. Meas. Tech. 2024, 17, 627–657. [Google Scholar] [CrossRef]
- Nowak, J.L.; Lothon, M.; Lenschow, D.H.; Malinowski, S.P. The ratio of transverse to longitudinal turbulent velocity statistics for aircraft measurements. Atmos. Meas. Tech. 2025, 18, 93–114. [Google Scholar] [CrossRef]
- Wacławczyk, M.; Gozingan, A.S.; Nzotungishaka, J.; Mohammadi, M.; P. Malinowski, S.P. Comparison of Different Techniques to Calculate Properties of Atmospheric Turbulence from Low-Resolution Data. Atmosphere 2020, 11, 199. [Google Scholar] [CrossRef]
- Obligado, M.; Vassilicos, J.C. The non-equilibrium part of the inertial range in decaying homogeneous turbulence. Europhys. Lett. 2019, 127, 64004. [Google Scholar] [CrossRef]
- Nixdorf, U.; Dethloff, K.; Rex, M.; Shupe, M.; Sommerfeld, A.; Perovich, D.; Nicolaus, M.; Heuze, C.; Rabe, B.; Loose, B.; et al. MOSAiC Extended Acknowledgement. Zenodo. 2021. Available online: https://zenodo.org/records/5541624 (accessed on 28 December 2024). [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wacławczyk, M.; Nzotungishaka, J.; Jędrejko, P.; Sarkar, J.; Malinowski, S.P. Dissipation Scaling with a Variable Cϵ Coefficient in the Stable Atmospheric Boundary Layer. Atmosphere 2025, 16, 188. https://doi.org/10.3390/atmos16020188
Wacławczyk M, Nzotungishaka J, Jędrejko P, Sarkar J, Malinowski SP. Dissipation Scaling with a Variable Cϵ Coefficient in the Stable Atmospheric Boundary Layer. Atmosphere. 2025; 16(2):188. https://doi.org/10.3390/atmos16020188
Chicago/Turabian StyleWacławczyk, Marta, Jackson Nzotungishaka, Paweł Jędrejko, Joydeep Sarkar, and Szymon P. Malinowski. 2025. "Dissipation Scaling with a Variable Cϵ Coefficient in the Stable Atmospheric Boundary Layer" Atmosphere 16, no. 2: 188. https://doi.org/10.3390/atmos16020188
APA StyleWacławczyk, M., Nzotungishaka, J., Jędrejko, P., Sarkar, J., & Malinowski, S. P. (2025). Dissipation Scaling with a Variable Cϵ Coefficient in the Stable Atmospheric Boundary Layer. Atmosphere, 16(2), 188. https://doi.org/10.3390/atmos16020188






