Abstract
We present a statistical study of some physical properties of medium-scale traveling ionospheric disturbances (MSTIDs) registered over the Mexican territory during 2018 and 2019 (solar minimum). The analysis is based on total electron content () approximations using data from the ground-based Global Navigation Satellite System (GNSS) receivers at different locations, divided into three regions according to geographic longitudes: west, center, and east. The MSTIDs were classified into day and night events, and only geomagnetically quiet days were considered to reduce the solar influence. We explored fundamental aspects of the MSTIDs, such as differences between day and night events, occurrence patterns, and geographical differences. Our results show some similarities with the occurrence periods of gravity waves, exhibiting high activity during summer and winter. For this period, however, most events occurred between 20:00 and 04:00 AM UT. The most energetic events, i.e., large amplitudes and power, occurred around the sunset terminator. This suggests that the density gradient generated when the sunlight falls benefits MSTID formation.
1. Introduction
Medium-scale traveling ionospheric disturbances (MSTIDs) are electron density fluctuations (wave-like) in the ionosphere plasma [,,]. The study of these disturbances is relevant because they are involved in the dynamic processes linked to lower–upper atmosphere coupling. Thus, disturbances may travel from the lower to the upper atmosphere, causing plasma irregularities [,,]. Moreover, research on MSTIDs is also crucial for space weather since they can interfere with radio wave communications [,,]. In this regard, multiple efforts have been made to understand their origin and evolution. Measurements from different instruments have shown that MSTIDs typically have periods from 15 to 60 min, with wavelengths of around 100 to 500 km and a phase velocity of 50 to 400 m/s [,,,,]. Note that these physical parameters coincide with the properties of internal gravity waves in the lower atmosphere (here referred to as atmospheric gravity waves (GWs)). Therefore, the term ”TID” is usually associated with them [,].
The properties of these disturbances (amplitude, propagation direction, and velocity) may depend on latitude, longitude, local time, and season [,,,,,]. Although these disturbances are observed day and night throughout the year, it is essential to distinguish between them since they have different generation mechanisms. During the daytime, atmospheric gravity waves (GW) are proposed as the primary mechanism for generating MSTIDs. There are many possible generation sources of GWs, such as topographic, convective, shear, geostrophic adjustment, and wave–wave interactions. According to simulation studies, tropospheric convection is the mechanism capable of generating secondary GWs traveling up to the thermosphere [,,].
Nighttime MSTIDs are presumably associated with electrodynamical processes. One of these electrodynamical processes is the Perkins instability (PI) [,,]. However, according to different studies, this instability alone cannot generate nighttime MSTIDs [,]. Thus, Perkins instability must be supplemented by other irregularities, such as a polarized electric field with sporadic E-layers (Es) or GWs, to produce MSTIDs at night [,,,,].
A correlation between the occurrence of MSTIDs and the solar cycle has been suggested. For nighttime MSTIDs, some analyses reported positive and negative correlations with the solar cycle [,,,,]. For that reason, this correlation is still unclear. In our case, the study period corresponds with the solar minimum, and only geomagnetically undisturbed days are considered to avoid solar influence.
We present the characterization of MSTIDs based on fluctuations over the Mexican territory during 2018–2019, a period of minimum solar activity. This study aims to analyze the ionospheric disturbances generated by processes in the lower atmosphere and establish a methodology for future studies in Mexico. The questions to be addressed in this research are: What is the pattern of occurrence for MSTIDs appearing over Mexico? Is there a geographical influence on the occurrence of TIDs? What is the relevance of occurrence time in determining the origin of the disturbance?
2. Materials and Methods
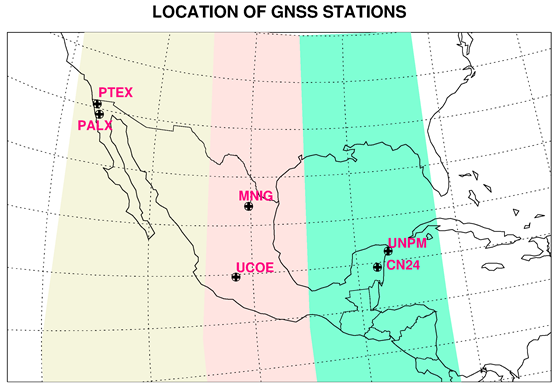
This study uses GNSS data from receivers across Mexico, which are part of the National Seismological Service and Transboundary, Land, and Atmosphere Long-term Observational and Collaborative Network (SSN - TLALOCNet) []. We focus on six GNSS stations located at different latitudes and longitudes to compare MSTID characteristics in three regions: the west (PALX, PTEX), center (MNIG, UCOE), and east (UNPM, CN24). Table 1 indicates the geographic coordinates of all the receiver stations according to the following: columns corresponding to the receiver station name, geographic latitude, and longitude. This table also includes a map of the station’s location, highlighting the three regions in different colors: yellow (west), red (center), and green (east). These stations cover critical locations in Mexico, such as the northeast, northwest, center, and southeast, to study the geographical influence in the development of TIDs. Moreover, our study was conducted during solar minimum activity for two years, 2018–2019, taking geomagnetically quiet days only.

Table 1.
List of GNSS receivers across Mexican territory used to identify TID events. Columns correspond to the receiver station name, geographic latitude, and geographical longitude. The map on the top shows the three regions for this study in different colors: west in yellow, center in red, and east in green.
2.1. TEC Estimation
There are two processes for approximating values along the line of sight between the GNSS satellite and the receiver. The first one is based on the pseudoranges or pseudo-distances (distance between the satellite and the receiver), calculated simply by a linear approximation: light velocity multiplied by the time difference between the emission and reception of the signal. The second method uses phase differences between two signals (carrier phases). In this study, we used Gopi Seemala’s software (program version 3) based on both approximations [].
In the case of GPS, the receivers use a dual-frequency ( MHz and MHz) to remove ionospheric effects. Thus, the general approximation to estimate is given by Equation (1):
where and are the carrier phases, and are the wavelengths, and are the number of cycles, and and are the receiver and satellite errors, respectively. The approximation is given in unit(s) (TECu), where 1 TECu = electrons/m2. This method only considers those satellites with an elevation angle above 20° to reduce the leveling errors. The general method can be consulted in Rama Rao et al. [].
2.2. Estimation of Detrended TEC (dTEC) and Identification of TID Events
According to the literature, TIDs are characterized by falls or diminished values with some specific periodicities depending on the nature of the disturbance [,,]. Thus, a detrending technique applied to data is required to identify them. In this case, the data behavior was fitted by a high-order polynomial function established using the Savitzky-Golay filter []. This filter employs the convolution function over a data window evaluated at an n-order polynomial (see Equation (2)). Finally, this polynomial function was subtracted from data. The mathematical expression to remove the tendency of data is represented by Equation (3). Here, “” is referred to as the detrended . It is important to mention that we use one-hour-centered data windows, i.e., we took ±30 min around each point in the data array. It guarantees that all possible wave patterns with periods similar to MSTIDs (15 to 60 min) are being included in the spectral analysis [,,].
By analyzing the behavior, wave-like patterns related to the signatures of TIDs can be distinguished in the signal. However, we follow two extra criteria to select TID events: (1) the wave-like patterns must exhibit at least two wave cycles, and (2) all the maximums in this wave-like pattern are above 0.1 TECu. This threshold value of the TID events was established by analyzing the standard deviation of all wave-like patterns identified in the MNIG station. Other authors have used threshold values between 0.07 and 1 TECu [,,], which agree with the value used in this work. It is important to mention that the amplitude was calculated using the mean value approximation between the upper and lower points of the disturbance, referred to as the average absolute amplitude. Also, the TID events were fitted by a polynomial curve to extract the occurrence time and the interval.
A spectral analysis based on the continuous wavelet transform (CWT) was used to determine the period of TIDs observed. The CWT of the data series was calculated following Torrence and Compo’s methodology []. Figure 1 shows an example of the TID identification process. The upper panel shows the variation at one satellite, and the lower panel shows the CWT for the same time series. In the CWT, the horizontal axis represents the hours along the day, the vertical axis represents the period, and the signal’s power (intensity) is represented with colors. For a better understanding, we can visualize the CWT as a matrix array, where each row corresponds to one period value, the columns represent the time of the signal recording, and every point in the array is weighted according to a power value represented by the color distribution. Thus, the highest disturbances appear in red, while blue is associated with low activity. The pink square delimitates the TID event. The same process was applied to each satellite registered by the receiver station. Different parameters were extracted from this analysis, such as observational time, date, power or disturbance intensity, and amplitude.

Figure 1.
The detrended signal from one satellite (up) and its continuous wavelet transform (down). The color code symbolizes the intensity of each period.
2.3. Identification of TIDs Periodicities
The TIDs were classified into day and night events using the solar terminator position throughout the year. After this classification, a probability density function was applied to all TID periodicities falling in each group (daytime or nighttime). Using the Scott rule, we constructed a histogram for the TID periodicity distribution to establish the probability density function []. Under this rule, the bar width of the histogram was determined using Equations (4) and (5). Here, “n” is the total number of TID periods, “” is the standard deviation without skewness, X represents the TID periods, and “” is the data average. Finally, the probability density distribution for TID periods () was calculated using the approximations of Equations (6) and (7), where means the total data for each period of the distribution in an interval and M is the total available data.
After obtaining all the probability density distributions, a normal distribution was fitted to obtain the representative periodicity for each dataset. Figure 2 shows the periodicities of each region’s TID events identified during 2018 and 2019. The blue and red colors indicate nighttime and daytime events, respectively. The dashed line represents the probability density function in the daytime (red) and nighttime (blue) of the TID events, where is the mean periodicity, and is the value of one standard deviation.
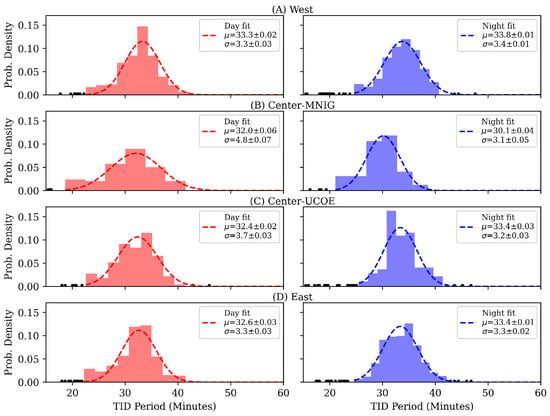
Figure 2.
Distribution of MSTID periodicities observed in the three regions of the Mexican territory: (A) west, (B) center-MNIG, (C) center-UCOE, and (D) east. The dashed line is the probability density function for daytime (red) and nighttime (blue) MSTID events.
3. Results and Discussion
We will present the main results concerning TID events that occurred over three regions of the Mexican territory, which were identified using GNSS data. In order to simplify the discussion, this section is divided into subsections: (1) periodicity and occurrence time, (2) disturbance energy, and (3) possible mechanism for generation.
3.1. Periodicity and Occurrence Time
According to the literature, periods between 15 min and 1 h are expected for the MSTIDs, which agrees with the results of the periodicity analysis shown in Figure 2. For daytime MSTIDs, the average periodicity was around 32.6 ± 3.9 min, while for nighttime TIDs, the average periodicity was 32.7 ± 3.5 min. Comparing these average values and considering their standard deviations, we found that both periods are similar. Thus, using the periodicity parameter, we cannot distinguish between day and night MSTIDs.
Table 2 summarizes each region’s periodicities and number of events. Here, we can see that the west and east stations registered more events, while the center stations exhibited a low MSTID activity. However, it is important to note that there was just one year of data for the MNIG receiver, which is why the number of events was lower than for the other receivers.

Table 2.
Periodicity and number of TID events identified for each region.
By analyzing the number of identified MSTIDs, we can anticipate that the nighttime events were predominant in most stations for this period. Figure 3 shows the monthly occurrence of MSTID events. Red and blue bars indicate the daytime and nighttime events, respectively. As we can observe in these plots, the number of events shows similarities between the three regions. For instance, most of the MSTID events occurred at night, exhibiting a predominance around mid-year (May, June, and July), especially in the west and east stations. This result suggests a high MSTID activity during summer. Also, the UCOE station (central region) registered many nighttime events during January, November, and December, suggesting a high MSTID activity during winter in this region. A similar result was found by Huang et al. [], who indicated that this result is unique compared to previous studies where nighttime MSTIDs were reported in the summer.
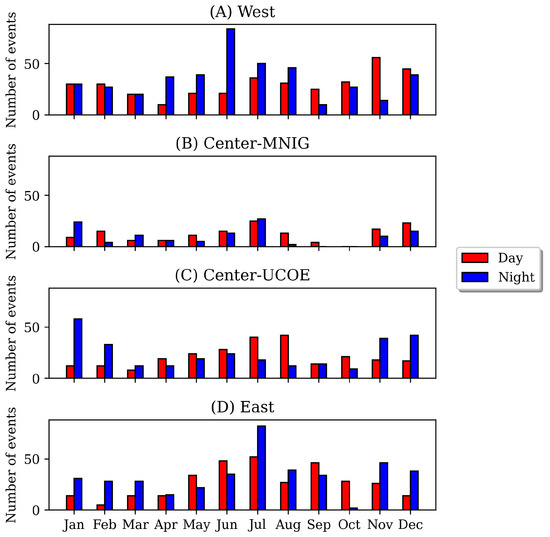
Figure 3.
Monthly occurrence of MSTID events observed in the three regions: (A) west, (B) center-MNIG, (C) center-UCOE, and (D) east. Blue and red colors indicate nighttime and daytime events, respectively.
At this point, we want to explore if there is a seasonal pattern to determine the disturbance’s origin. Thus, it is crucial to remember that the period of our study corresponds to a solar minimum, and only geomagnetically quiet days were considered. This means that electron density fluctuations related to MSTIDs were caused by physical processes in the lower atmosphere [,,,]. Moreover, as some authors have indicated, the occurrence time is also required to speculate on the origin of the disturbances. In this sense, to analyze the distribution of the occurrence time, we recorded the total number of MSTID events registered in a given month, which was taken as 100%, and the number of events registered per hour. Based on these quantities, we established the occurrence percentage of MSTID events every month and every hour. Figure 4 displays a map showing the hours and occurrence months to visualize the main MSTID activity for the three regions. Colors indicate the occurrence percentage of MSTID events, with dark red being the highest occurrence. The color scale reaches up to 53.33%, indicating that this percentage is the highest in the distribution of events throughout the months and hours. Also, it is important to highlight that just a few zones exhibited a high MSTID activity, located around the sunset terminator. In the west region, there was high MSTID activity from August to September; in the east region, this activity was more intense from September to October. In the central region, the MNIG station also exhibited high activity from August to September, and the UCOE station mainly did during August. In addition, the MSTID activity remained significant during April, May, June, and July. Despite most events occurring at night in the west and east stations, a significant percentage were registered around mid-day during January, November, and December.

Figure 4.
Percentage distribution of occurrence of MSTID events by hours and months. The color scale indicates the occurrence percentage, with dark red denoting the highest occurrence. Dashed lines represent solar terminators.
A detailed inspection of Figure 4 shows that many MSTID events occurred around the sunset terminator for this period. Different studies have indicated that terminators are sources of MSTIDs because these regions aid tropospheric convective motions that, at the same time, are involved in the generation of secondary GW traveling up to the thermosphere [,,]. However, in this case, the MSTIDs were registered only during the sunset terminator. Similar results were obtained by Figueiredo et al. [] using low-latitude stations, questioning why MSTID events are not generated at the sunrise terminator. This indicates that low density benefits the MSTIDs. This anti-correlation between MSTID occurrence and electron density has been recently reported by Katamzi-Joseph et al. [].
Additionally, a vertical temperature gradient is necessary to generate atmospheric instability, which is one of the primary triggers for the convective motions in the troposphere []. This condition is consistent with the high MSTID occurrence during summer when the temperature gradient at solar terminators could be more significant. However, a data analysis of the temperature gradient is required to corroborate.
3.2. Disturbance Energy
Considering TIDs as mechanical waves, we can assume that their power is proportional to the wave’s energy, which is in turn related to the squared amplitude. Following this assumption, we first analyzed the amplitude variability of MSTIDs to investigate the disturbance energy. Thus, a statistical analysis based on the median and quartiles of the MSTID amplitudes was developed. Figure 5 shows the amplitude variability as a function of the local time (left panels) and throughout the year (center and right panels) for day and nighttime (same color scheme as Figure 3). On the left panels, the solid line corresponds to the median value, where the shadowed region symbolizes the first and third quartiles for the amplitude variation of MSTID with the local time, encompassing 50% of the events. As we can see, the local time analysis shows that the values of the amplitude median are very close (low variation), with values between 0.1 and 0.2 TECu.

Figure 5.
Amplitude variation of for both local time (LT) and months through the year. The plots in each row are referred to as (A) west region, (B) center-MNIG, (C) center-UCOE, and (D) east region. Solid lines represent the median, while the colored region indicates the variation between the first and third quartiles (IQR).
Nonetheless, the shadowed region is very wide, i.e., there is a high dispersion of the amplitudes, mainly at nighttime. This high dispersion also suggests that the largest amplitudes of MSTIDs occurred at night. The east stations (CN24 and UNPM) mainly registered the highest amplitude dispersion between 20:00 and 04:00 AM LT.
In Figure 5, we also included the annual amplitude variability of MSTID events (center and right panels) to investigate if they were more frequent in some months than others. These plots were constructed by estimating the amplitude median (AMA) for each month and its respective first and third quartiles (IQR) to indicate the amplitude dispersion. This process was repeated for each time interval, daytime (red) and nighttime (blue). In this case, we did not identify a particular pattern, but, as observed in the local time plots, the amplitude dispersion at night was higher than during the daytime.
Similar behavior was identified using the MSTID power variation, in which nighttime events presented a higher power than daytime events. Figure 6 summarizes the variability range between the first and third quartiles of the TID power. Here, we can visualize that the highest variability occurred near the solar terminators. As mentioned before, solar terminators are common sources of TIDs because, to a first approximation, the transition between day and night contributes to generating atmospheric instabilities. Notably, our results suggest that the most energetic MSTIDs occurred around the sunset terminator for this period. This means that the energy transfer from the lower atmosphere is more efficient at night due to the reduction in electron density during sunset, which reduces wave damping (e.g., Kelley [], Katamzi-Joseph et al. []). This fact could also explain why MSTID events were not generated at the sunrise terminator.
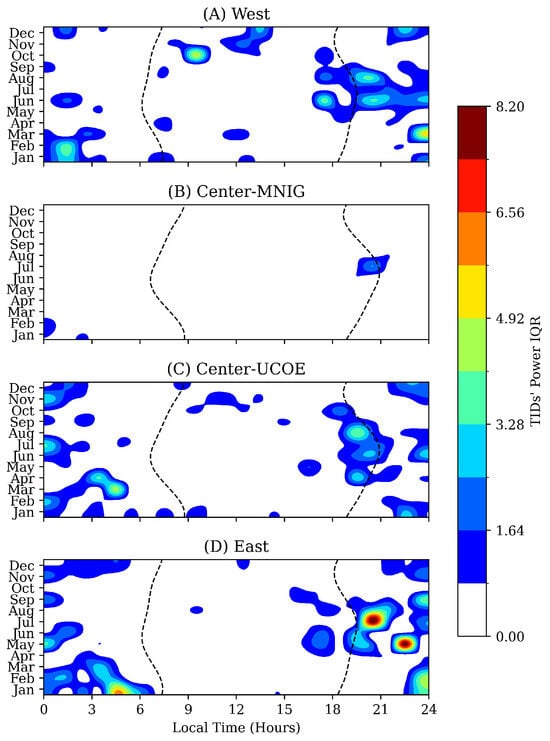
Figure 6.
Distribution of the IQR’s power variability throughout the months and hours. Dark red indicates the maximum power variation, and dashed lines represent the solar terminators.
By exploring the relationship between the power and average amplitude of MSTIDs, we can also corroborate that the strongest MSTIDs occurred at night. Figure 7 shows the correlation between the power and average amplitude of MSTID events. Red and blue colors symbolize the day and night events, respectively. Each region has a different symbol: west with triangles, center-MNIG with stars, center-UCOE with circles, and east with squares. The dashed line represents the curve fitted by a power-law , where and R is the coefficient of determination appearing in the legend. As expected, there is a strong correlation between power and average amplitude (), except UCOE, which presents . In this plot, it can be noted that the wave-like patterns did not exhibit amplitudes higher than 0.7 TECu during this period. According to previous studies, the average electron density over this region is around ≈12.9 ± 5 TECu at midnight, and density reductions represent about 5.4% [,].
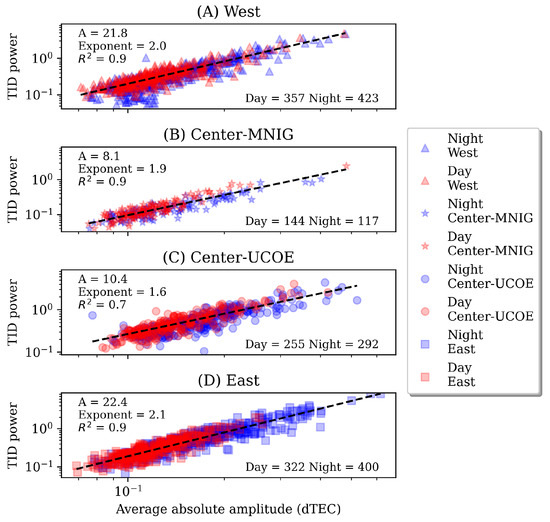
Figure 7.
Power variation versus the amplitude of MSTID events. The symbols indicate each region: (A) west (triangles), (B) center-MNIG (stars), (C) center-UCOE (circles), and (D) east (squares). Red and blue colors refer to daytime and nighttime events.
3.3. Possible Mechanism for Generation
In general, ionospheric irregularities (plasma bubbles, TIDs) arise from complex dynamical processes seeded by plasma instabilities plus the physical conditions of the lower–upper atmosphere system. Thus, the development of MSTIDs combines different factors such as atmospheric conditions, wind patterns, geographical position, local time, year’s season, and solar activity. Here, we have explored some of the fundamental aspects of the MSTIDs, such as differences between day and night, occurrence patterns, geographical contrast, and disturbance energy. To a first approximation, our results reveal that the MSTID occurrence pattern is congruent with gravity wave observations, whose activity has been reported to be at its maximum in summer and winter [,]. Furthermore, most MSTID events occurred at the sunset terminator, considered a potential source of GWs [].
As mentioned before, the information of gravity waves is typically associated with convective motions in the lower atmosphere that can originate from atmospheric instabilities, e.g., temperature differences between the lower atmosphere, orography, and earthquakes. In this regard, Borchevkina et al. [] suggested that temperature differences between the troposphere and thermosphere are the key to the convective motions, highlighting the importance of lower–upper atmospheric coupling.
To explore the generation mechanism in more detail, we analyzed the neutral wind (orientation and velocity) using data from the Prediction Of Worldwide Energy Resources (POWER) project, a compilation of datasets from new satellite systems []. In this case, data were taken at the troposphere (≈50 m above mean sea level). Following the structure in Figure 5, the wind median for the orientation and velocity were calculated for every hour and month. Figure 8a shows the orientation median of neutral wind for hours (left) and months (right), while Figure 8b corresponds to the median velocity of neutral wind for hours (left) and months (right). Blue and red circles symbolize the monthly median for nighttime and daytime events. The wind variability of orientation and velocity do not exhibit a clear pattern to explain the generation of disturbances in the thermosphere. Neutral wind orientation shows a considerable change during the morning (6 AM to 12 PM) in the west and central regions. However, the average occurrence time for MSTIDs in the three regions was around the sunset terminator (17:00 to 20:00 LT), which means that changes in wind orientation are not correlated with the MSTIDs occurrence.
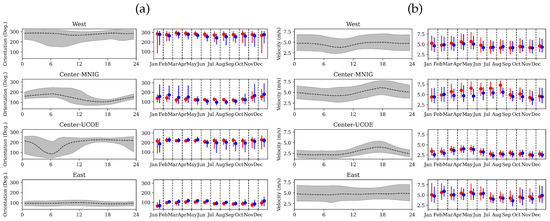
Figure 8.
(a) Variation in wind orientation throughout the hours (left) and months (right) for the three regions. (b) Variation in wind velocity throughout the hours (left) and months (right) for the three regions. The gray zone corresponds to the IQR variation, and blue and red circles represent the monthly median values for day and night events, respectively.
On the other hand, the wind velocity pattern presented an increment during the night. Although the velocity range was small (from 2.5 to 7.5 km/h), the median velocity enhanced at night. Particularly at geographical positions such as the UCOE station, this enhancement remained from April to June (spring). A similar behavior was observed in the western region. Besides, the wind velocity pattern presented the highest values around MNIG station from April to August.
It is important to highlight that velocity increments occurred during the spring and summer when Mexico’s highest temperatures were registered. At night, this variability in the troposphere could amplify the convective motions and disturbance development in the mesosphere (GW) and thermosphere (MSTIDs). Moreover, considering the annual profile (e.g., Romero-Hernandez et al. []), the minimum electron density occurs in the summer, which should reduce the wave damping and, at the same time, increment the MSTID generation. However, further studies are necessary.
4. Conclusions
The MSTIDs are ionospheric irregularities seeded by plasma instabilities and physical conditions in the lower–upper atmosphere. Their development combines different factors such as atmospheric conditions, wind patterns, geographical position, local time, the year’s season, and solar activity. We presented the analysis of MSTID events over Mexican territory during 2018 and 2019, a period of minimal activity in Solar Cycle 24. Based on GNSS data from the SSN-TLALOCNet network, we explored some fundamental aspects of MSTIDs, such as differences between day and night events, seasonal variability, geographical contrast, and disturbance energy. The main results can be summarized as follows:
- The MSTID events were predominant at night for this period.
- The maximum MSTID activity occurred during the summer for the west and east regions, while most events were registered in the winter for the central region. Both periods coincide with gravity wave observations.
- A high percentage of the events was registered near the sunset terminator.
- Nighttime events exhibited the highest amplitude and energy variations. This suggests that density reduction benefits MSTID formation (reduction in wave damping).
- According to the POWER data analysis, high variability in wind velocity patterns was registered during the spring and summer, mainly at night, which coincides with the MSTID occurrence (May–July). However, it is necessary to analyze the wind variation in the mesosphere and lower thermosphere to corroborate this possible source for MSTID generation.
Author Contributions
Conceptualization, E.R.-H., F.S.-S. and O.F.J.; methodology, E.R.-H., F.S.-S., E.A.-R. and M.R.-M.; software, F.S.-S. and R.A.-G.; validation, O.F.J., G.A.d.S.P., C.M.D., M.A.S. and J.A.G.-E.; formal analysis, E.R.-H. and F.S.-S.; investigation, E.R.-H. and O.F.J.; resources; data curation, F.S.-S.; writing—original draft preparation, E.R.-H.; writing—review and editing, O.F.J., E.A.-R., C.A.G.-P., F.A.G.-C., S.A. and E.P.-T.; funding acquisition, E.P.-T. All authors have read and agreed to the published version of the manuscript.
Funding
This work was carried out with financial support from Universidad Autónoma de Nuevo León (UANL), PAICYT project 273-CE-2022 and the CONAHCYT project CF-2023-G-364.
Institutional Review Board Statement
Not applicable.
Informed Consent Statement
Not applicable.
Data Availability Statement
The data presented in this study are openly available in TLALOCNet-SSN (http://tlalocnet.udg.mx/).
Acknowledgments
E.R.-H acknowledges support from the PAICYT project 273-CE-2022 and the CONAHCYT project CF-2023-G-364. F.S.-S. thanks CONAHCYT for the master’s fellowship. E.A.-R. acknowledges support from the DGAPA/PAPIIT project IN106224, and the CONAHCYT project CF-2023-G-364. M.R.-M acknowledges support from the DGAPA project (IN115423). This article is based on GPS data provided by TLALOCNet, SSN-TLALOCNet and SSN GPS/GNSS networks operated by Servicio de Geodesia Satelital (SGS), and Servicio Sismológico Nacional (SSN) [] at the Instituto de Geofísica-Universidad Nacional Autónoma de México (UNAM) and the GAGE facility operated by UNAVCO Inc. We gratefully acknowledge all personnel from SGS, SSN and UNAVCO Inc. for station maintenance, data acquisition, IT support and data curation and distribution for these networks. TLALOCNet, SSN-TLALOCNet and other GPS related operations from SGS and SSN are supported by CONAHCyT projects 253760, 256012 and 2017-01-5955, UNAM-Programa de Apoyo a Proyectos de Investigación e Innovación Tecnológica (PAPIIT), projects IN104213, IN111509, IN109315-3, IN104818-3 and PAPIIT project IN111509 to R. Pérez, National Science Foundation grant 2025104 and supplemental support from UNAM-Instituto de Geofísica. UNAVCO’s initial support for TLALOCNet was supported by the National Science Foundation and the National Aeronautics and Space Adminsitration under NSF Cooperative Agreement EAR-1338091. The authors thank the reviewers for their constructive comments and helpful suggestions, which improved an earlier version of the manuscript.
Conflicts of Interest
The authors declare no conflicts of interest.
References
- Hocke, K.; Schlegel, K.A. Review of atmospheric gravity waves and travelling ionospheric disturbances: 1982–1995. Ann. Geophys. 1996, 14, 917–940. [Google Scholar]
- Kotake, N.; Otsuka, Y.; Tsugawa, T.; Ogawa, T.; Saito, A. Climatological study of GPS total electron content variations caused by medium-scale traveling ionospheric disturbances. J. Geophys. Res. Space Phys. 2006, 111, A04306. [Google Scholar] [CrossRef]
- Tsugawa, T.; Kotake, N.; Otsuka, Y.; Saito, A. Medium-scale traveling ionospheric disturbances observed by GPS receiver network in Japan: A short review. GPS Solut. 2007, 11, 139–144. [Google Scholar] [CrossRef]
- Borchevkina, O.P.; Adamson, S.O.; Dyakov, Y.A.; Karpov, I.V.; Golubkov, G.V.; Wang, P.-K.; Golubkov, M.G. The Influence of Tropospheric Processes on Disturbances in the D and E Ionospheric Layers. Atmosphere 2021, 12, 1116. [Google Scholar] [CrossRef]
- Figueiredo, C.A.O.B.; Takahashi, H.; Wrasse, C.M.; Otsuka, Y.; Shiokawa, K.; Barros, D. Medium-scle traveling ionospheric disturbances observed by detrended total electron content maps over Brazil. J. Geophys. Res. Space Phys. 2018, 123, 2215–2227. [Google Scholar] [CrossRef]
- Fritts, D.C.; Alexander, M.J. Gravity wave dynamics and effects in the middle atmosphere. Rev. Geophys. 2003, 41, 1003:1–1003:64. [Google Scholar] [CrossRef]
- Kotake, N.; Otsuka, Y.; Tsugawa, T.; Ogawa, T.; Saito, A. A Statistical study of medium-scale traveling ionospheric disturbances observed with the GPS networks in Southern California. Earth Planets Space 2007, 59, 95–102. [Google Scholar] [CrossRef]
- Liu, Y.; Zhou, C.; Xu, T.; Tang, Q.; Deng, Z.X.; Chen, G.Y.; Wang, Z.K. Review of ionospheric irregularities and ionospheric electrodynamic coupling in the middle latitude region. Earth Planet. Phys. 2021, 5, 462–482. [Google Scholar] [CrossRef]
- Jonah, O.F.; Kherani, E.A.; De Paula, E.R. Observation of TEC perturbation associated with medium-scale traveling ionospheric disturbance and possible seeding mechanism of atmospheric gravity wave at a Brazilian sector. J. Geophys. Res. Space Phys. 2016, 121, 2531–2546. [Google Scholar] [CrossRef]
- Otsuka, Y.; Kotake, N.; Shiokawa, K.; Ogawa, T.; Tsugawa, T.; Saito, A. Statistical study of medium-scale traveling ionospheric disturbances observed with a GPS receoiver network in Japan. In Aeronomy of the Earth’s Atmosphere and Ionosphere; IAGA Special Sopron Book Series; Springer: Dordrecht, The Netherlands, 2011; pp. 291–299. [Google Scholar]
- Sivakandan, M.; Otsuka, Y.; Ghosh, P.; Shinagawa, H.; Shinbori, A.; Miyoshi, Y. Comparison of seasonal and longitudinal variation of daytime MSTID activity using GPS observation and GAIA simulations. Earth Planets Space 2021, 73, 35. [Google Scholar] [CrossRef]
- Kelley, M.C. On the origin of mesoscale TIDs at midlatitudes. Ann. Geophys. 2011, 29, 361–366. [Google Scholar] [CrossRef]
- Makela, J.J.; Otsuka, Y. Overview of Nighttime Ionospheric Instabilities at Low- and Mid-Latitudes: Coupling Aspects Resulting in Structuring at the Mesoscale. Space Sci. Rev. 2012, 168, 419–440. [Google Scholar] [CrossRef]
- Oluwadare, T.S.; Jakowski, N.; Valladares, C.E.; Akala, A.O.O.; Abe, O.E.; Alizadeh, M.M.; Schuh, H. Climatology of Medium-Scale Traveling Ionospheric Disturbances (MSTIDs) Observed with GPS Networks in the North African Region. Pure Appl. Geophys. 2022, 179, 2617. [Google Scholar] [CrossRef]
- Otsuka, Y.; Suzuki, K.; Nakagawa, S.; Nishioka, M.; Shiokawa, K.; Tsugawa, T. GPS observations of medium-scale traveling ionospheric disturbances over Europe. Ann. Geophys. 2013, 31, 163–172. [Google Scholar] [CrossRef]
- Zhou, Q.; Mathews, J.D. On the physical explanation of the Perkins instability. J. Geophys. Res. Space Phys. 2006, 111, A12309. [Google Scholar] [CrossRef]
- Chang, L.C.; Hsieh, Y.C.; Chao, C.K.; Duann, Y.; Salinas, C.C.J.H.; Liu, J.Y.; Lin, C.C. Variability and distribution of nighttime equatorial to mid latitude ionospheric irregularities and vertical plasma drift observed by FORMOSAT-5 Advanced Ionospheric Probe in-situ measurements from 2017–2020. Adv. Space Res. 2023, 73, 3536–3549. [Google Scholar] [CrossRef]
- Cosgrove, R.B.; Tsunoda, R.T.; Fukao, S.; Yamamoto, M. Coupling of the Perkins instability and the sporadic E layer instability derived from physical arguments. J. Geophys. Res. Space Phys. 2004, 100, 1–11. [Google Scholar] [CrossRef]
- Huang, F.; Lei, J.; Dou, X.; Luan, X.; Zhong, J. Nighttime medium-scale traveling ionospheric disturbances from airglow imager and Global Navigation Satellite Systems observations. Geophys. Res. Lett. 2018, 45, 31–38. [Google Scholar] [CrossRef]
- Lee, W.K.; Kil, H.; Paxton, L.J. Global distribution of nighttime MSTIDs and its association with E region irregularities seen by CHAMP satellite. J. Geophys. Res. Space Phys. 2021, 126, e2020JA028836. [Google Scholar] [CrossRef]
- Hernández-Pajares, M.; Juan, J.M.; Sanz, J.; Aragón-Ángel, A. Propagation of medium scale traveling ionospheric disturbances at different latitudes and solar cycle conditions. Radio Sci. 2012, 47, RS0K05. [Google Scholar] [CrossRef]
- Otsuka, Y.; Shinbori, A.; Tsugawa, T.; Nishioka, M. Solar activity dependence of medium-scale traveling ionospheric disturbances using GPS receivers in Japan. Earth Planets Space 2021, 73, 22. [Google Scholar] [CrossRef]
- Terra, P.; Vargas, F.; Brum, C.G.M.; Miller, E.S. Geomagnetic and solar dependency of MSTIDs occurrence rate: A climatology based on airglow observations from the Arecibo observatory ROF. J. Geophys. Res. Space Phys. 2020, 125, e2019JA027770. [Google Scholar] [CrossRef]
- Cabral-Cano, E.; Pérez-Campos, X.; Márquez-Azúa, B.; Sergeeva, M.A.; Salazar-Tlaczani, L.; DeMets, C.; Adams, D.; Galetzka, J.; Hodgkinson, K.; Feaux, K.; et al. TLALOCNet: A Continuous GPS-Met Backbone in Mexico for Seismotectonic and Atmospheric Research. Seismol. Res. Lett. 2018, 80, 373–381. [Google Scholar] [CrossRef]
- Seemala, G.K.; Valladares, C.E. Statistics of total electron content depletions observed over the South American continent for the year 2008. Radio Sci. 2011, 46, RS5019. [Google Scholar] [CrossRef]
- Rama Rao, P.V.S.; Gopi Krishna, S.; Niranjan, K.; Prasad, D.S.V.V.D. Temporal and spatial variations in TEC using simultaneous measurements from the Indian GPS network of receivers during the low solar activity period of 2004–2005. Ann. Geophys. 2008, 24, 3279–3292. [Google Scholar] [CrossRef]
- Savitzky, A.; Golay, M.J.E. Smoothing and differentiation of data by simplified least squares procedures. Anal. Chem. 1964, 36, 1627–1639. [Google Scholar] [CrossRef]
- Torrence, C.; Compo, G.P. A practical guide to wavelet analysis. Bull. Am. Meteorol. Soc. 1998, 79, 61–78. [Google Scholar] [CrossRef]
- Scott David, W. On optimal and data-based histograms. Biometrika 1979, 66, 605–610. [Google Scholar] [CrossRef]
- MacDougall, J.; Abdu, M.; Batista, I.; Fagundes, P.R.; Sahai, Y.; Jayachandran, P.T. On the production of traveling ionospheric disturbances by atmospheric gravity waves. J. Atmos. Sol. Terr. Phys. 2009, 71, 2013–2016. [Google Scholar] [CrossRef]
- Katamzi-Joseph, Z.T.; Grawe, M.A.; Makela, J.J.; Habar, J.B.; Martinis, C.; Baumgardner, J. First results on characteristics of nighttime MSTIDs observed over South Africa: Influence of thermospheric wind and sporadic E. J. Geophys. Res. Space Phys. 2022, 127, e2022JA030375. [Google Scholar] [CrossRef]
- Romero-Hernandez, E.; Denardini, C.M.; Jonah, O.F.; Essien, P.; Picanço, G.A.S.; Nogueira, P.A.B.; Rodriguez-Martinez, M.; Resende, L.C.A.; De La Luz, V.; Aguilar-Rodriguez, E.; et al. Nighttime ionospheric TEC study over Latin America during moderate and high solar activity. J. Geophys. Res. Space Phys. 2020, 125, e2020JA028210. [Google Scholar] [CrossRef]
- Sergeeva, M.A.; Maltseva, O.A.; Gonzalez-Esparza, J.A.; Mejia-Ambriz, J.C.; De La Luz, V.; Corona-Romero, P.; Gonzalez, L.X.; Gatica-Acevedo, V.J.; Romero-Hernandez, E.; Rodriguez-Martinez, M.; et al. TEC behavior over the Mexican region. Ann. Geophys. 2018, 61, GM104. [Google Scholar] [CrossRef]
- Liu, H.; Pedatella, N.; Hocke, K. Medium-scale gravity wave activity in the bottomside F region in tropical regions. Geophys. Res. Lett. 2017, 44, 7099–7105. [Google Scholar] [CrossRef]
- NASA Prediction of Worldwide Energy Resources Project. Available online: https://power.larc.nasa.gov/ (accessed on 18 July 2023).
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).