Numerical Simulation of the Plant Shelterbelt Configuration Based on Porous Media Model
Abstract
1. Introduction
2. Materials and Methods
2.1. Modeling of Porous Media in Plant Canopies
2.2. Governing Equations
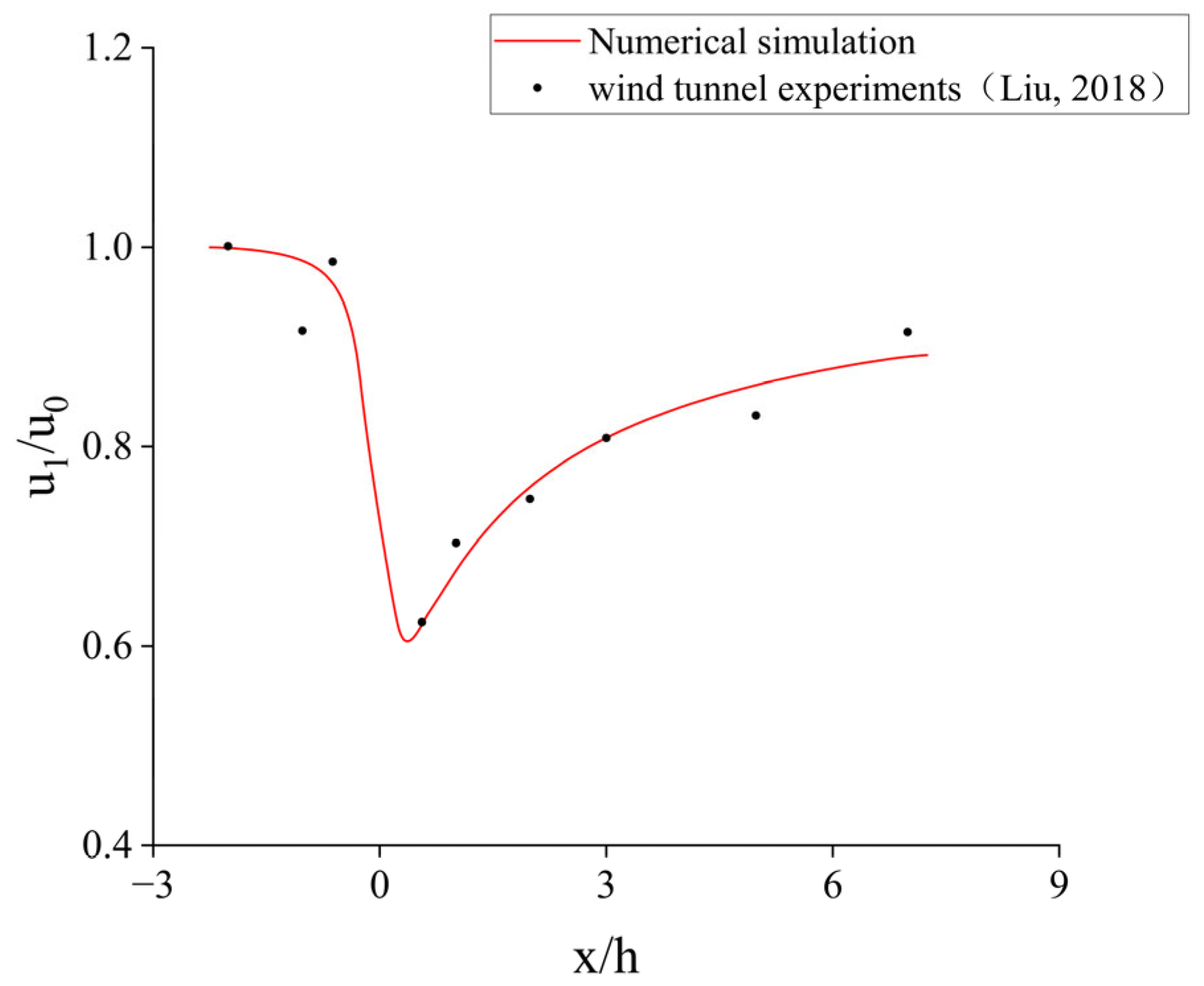
2.3. Model Validation
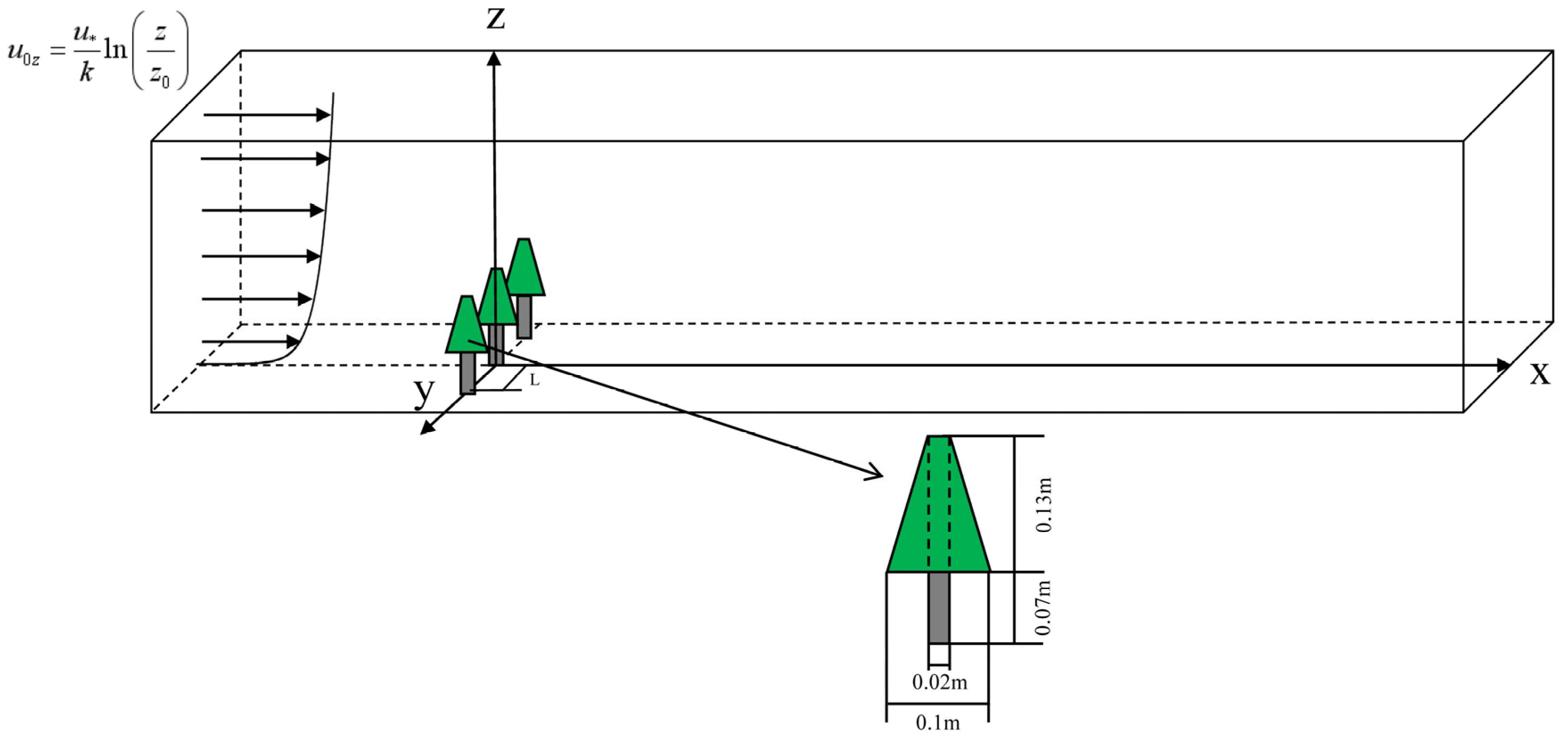
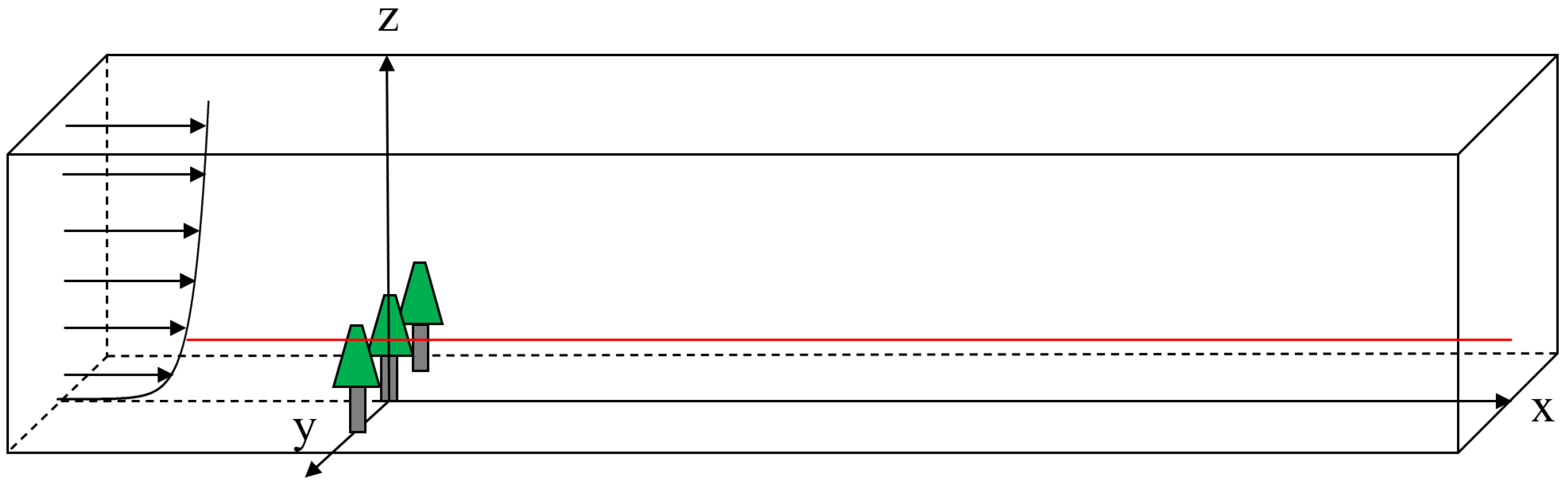
2.4. Computational Modeling and Boundary Conditions
3. Results and Discussion
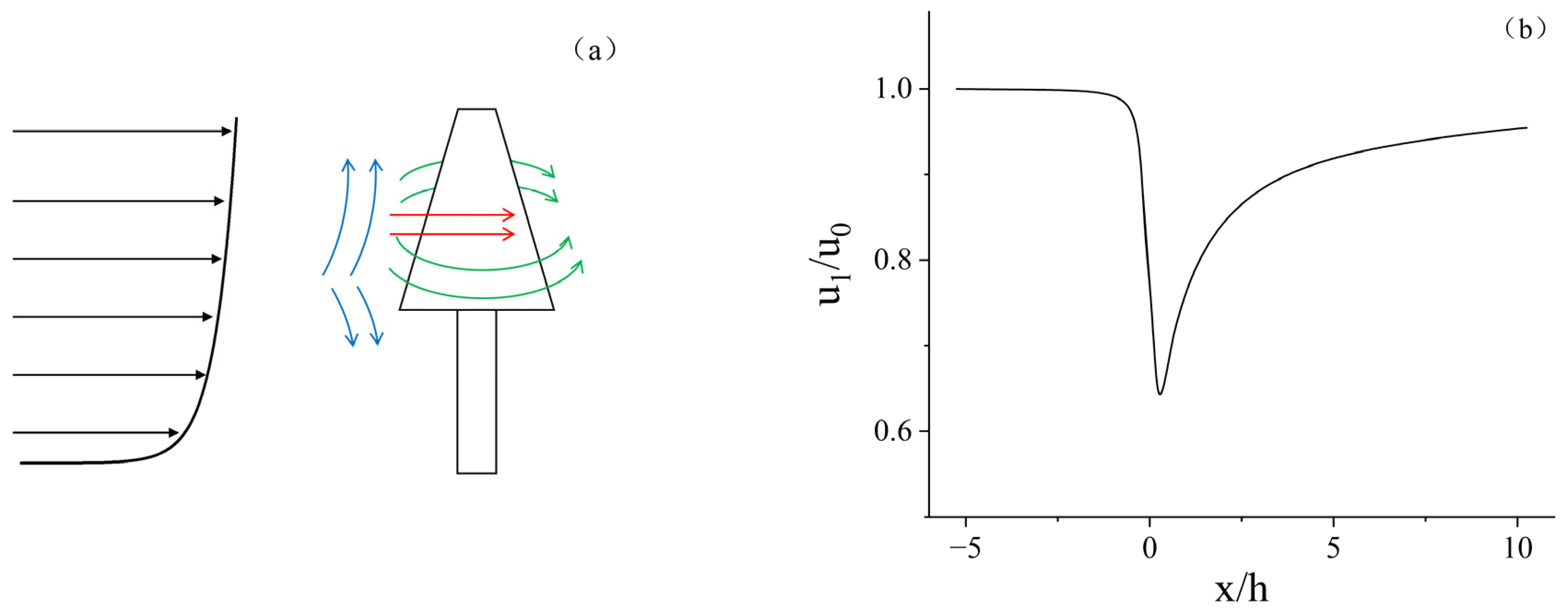
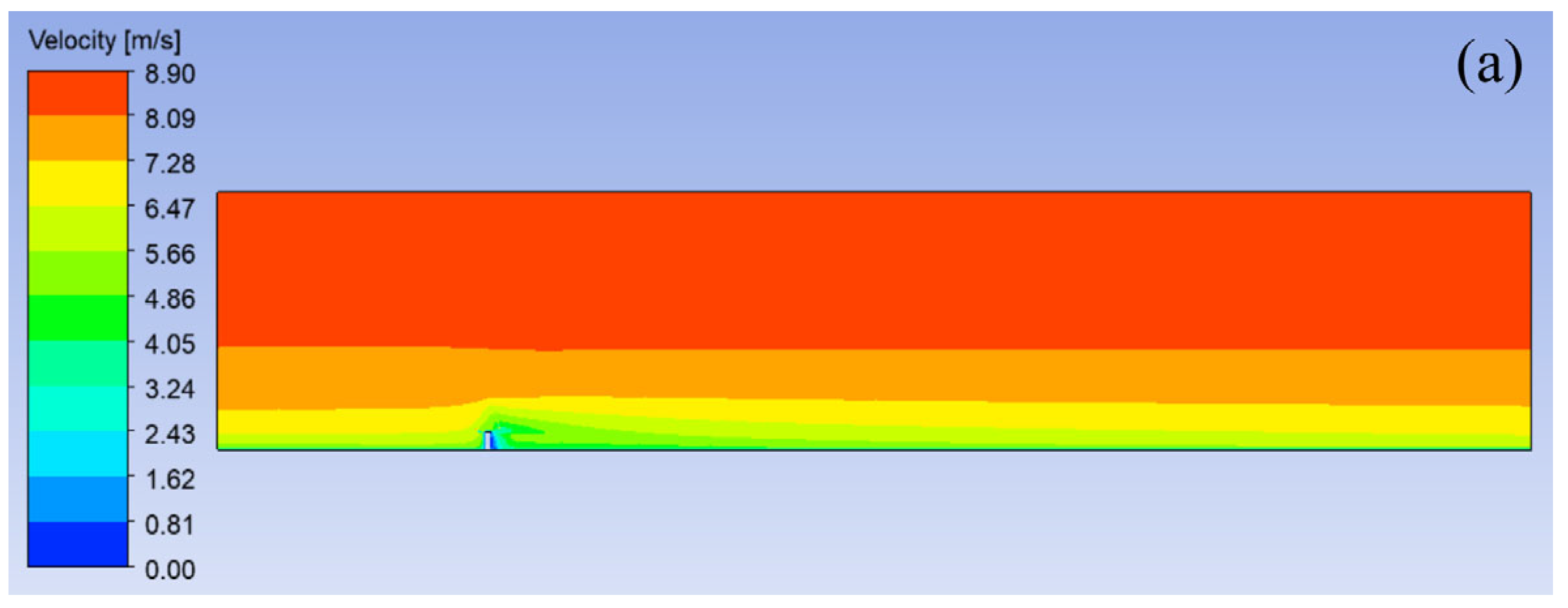
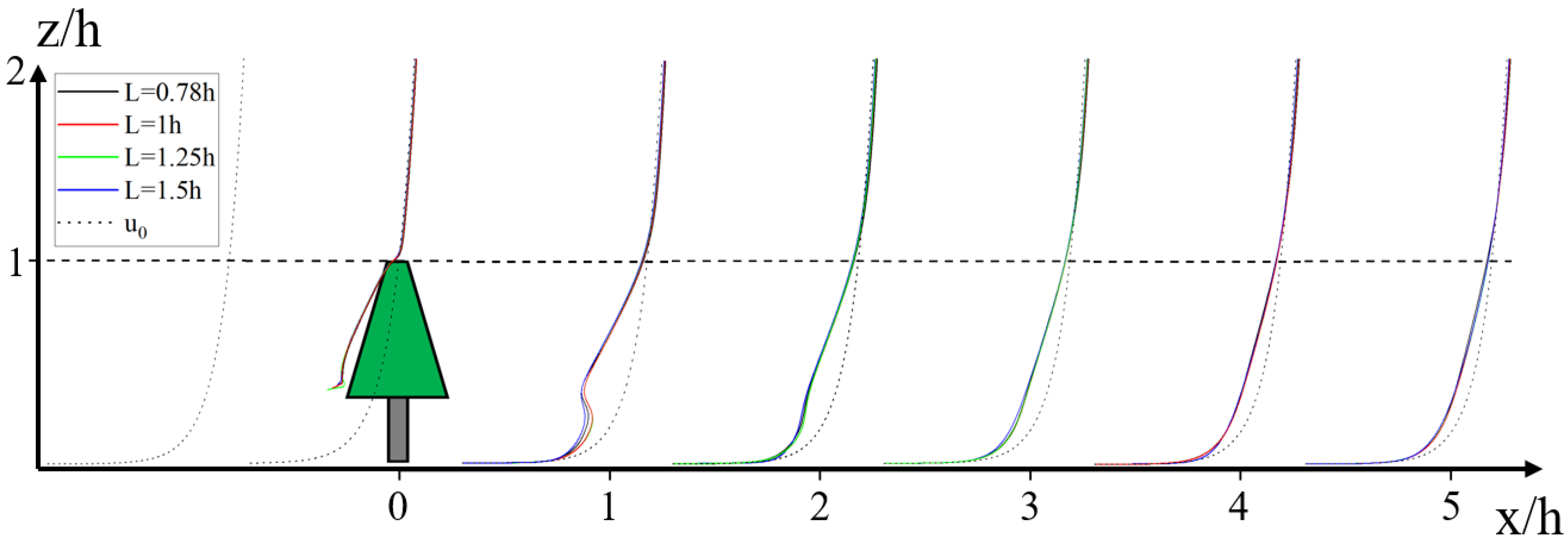
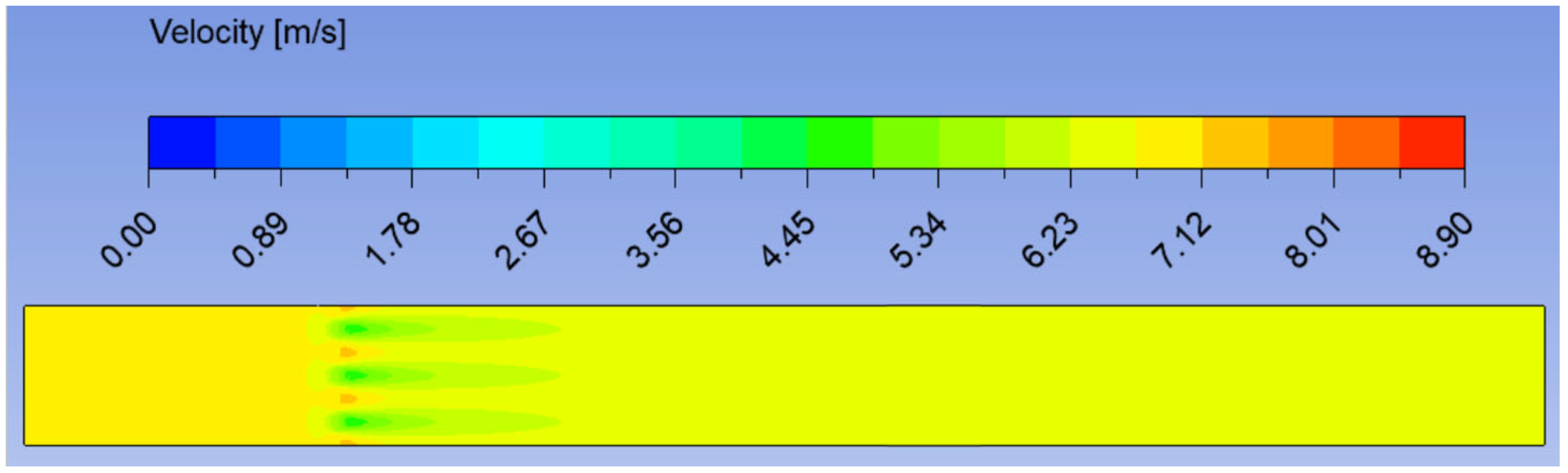
3.1. Flow Field Analysis of a Single Plant
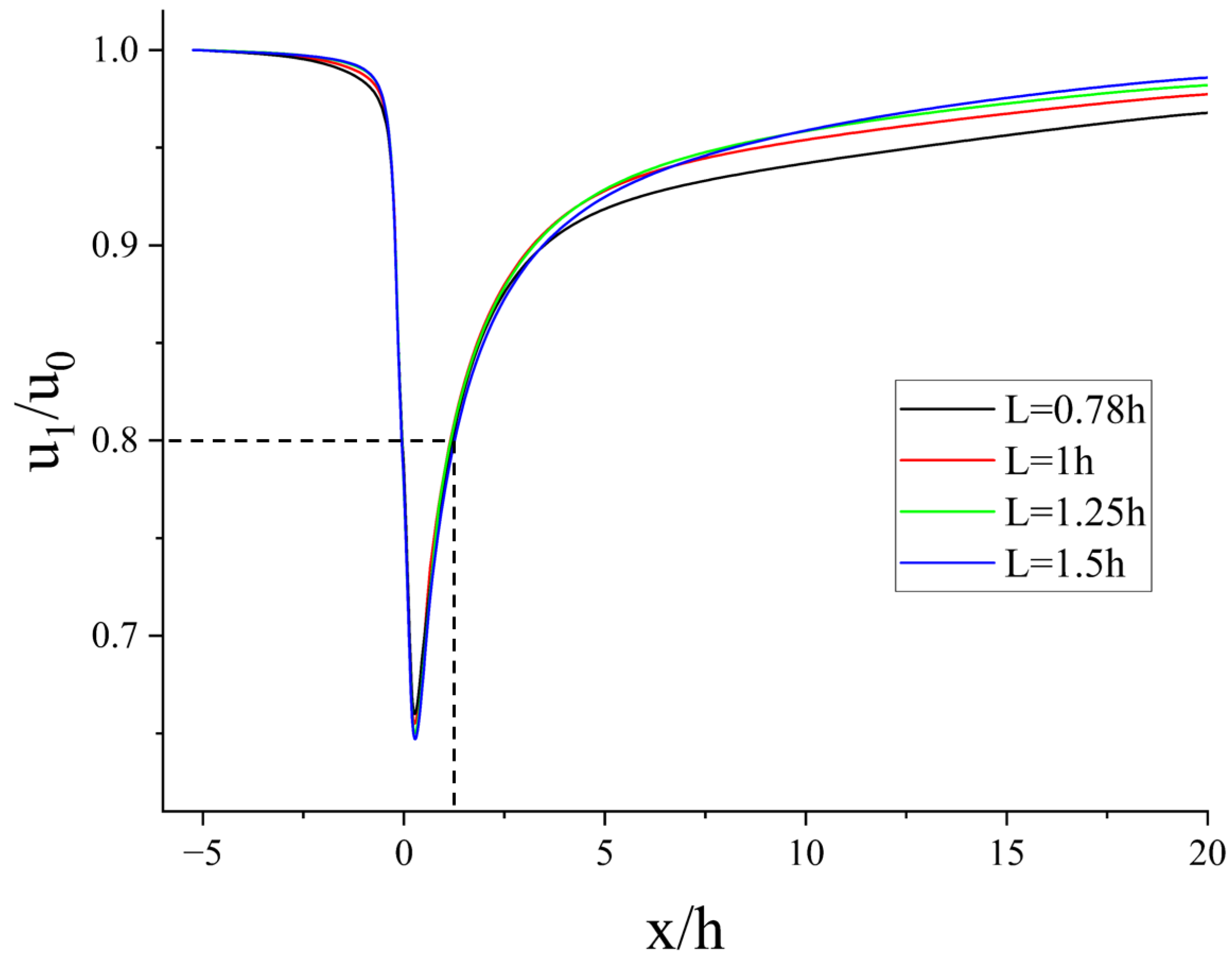
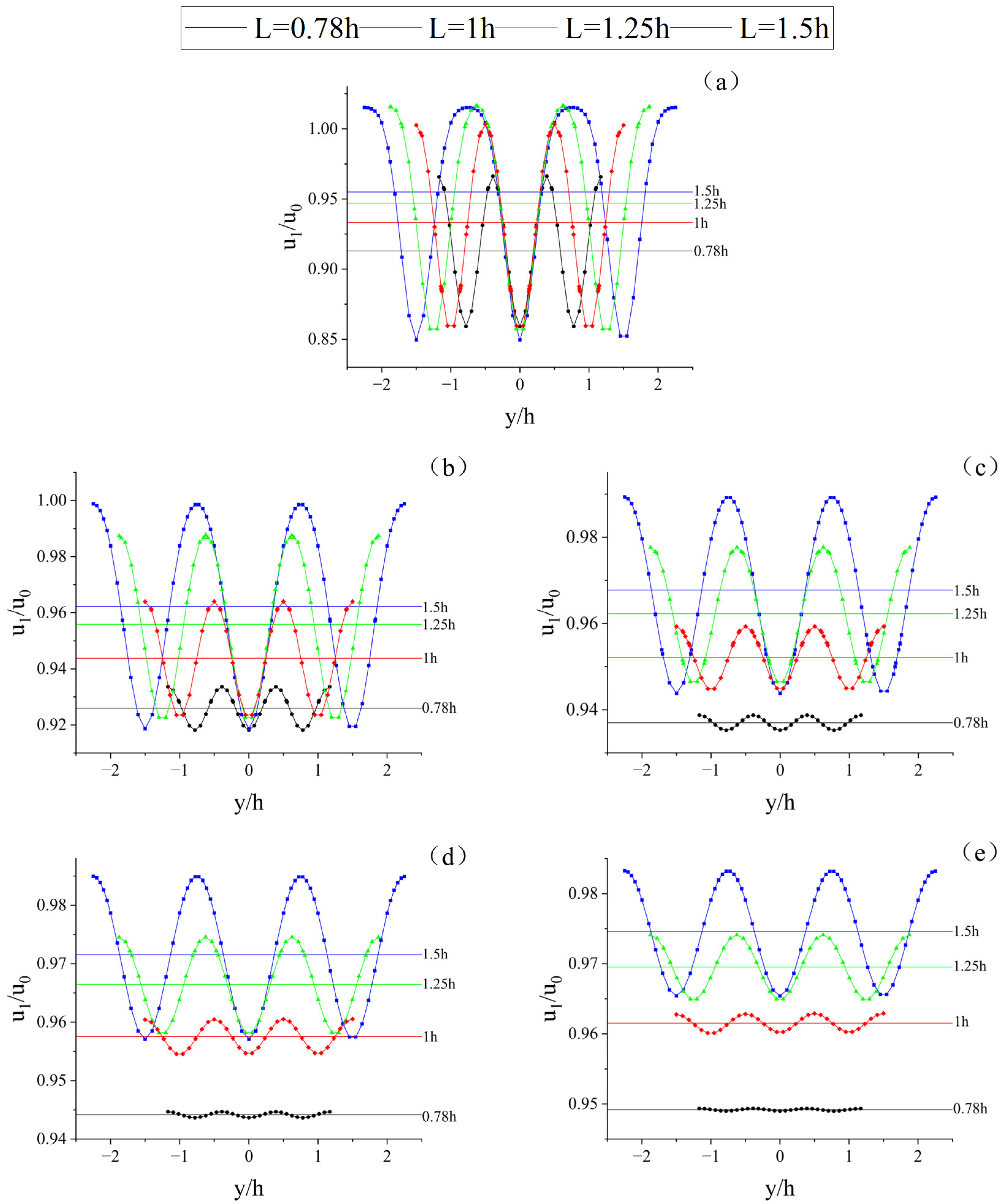
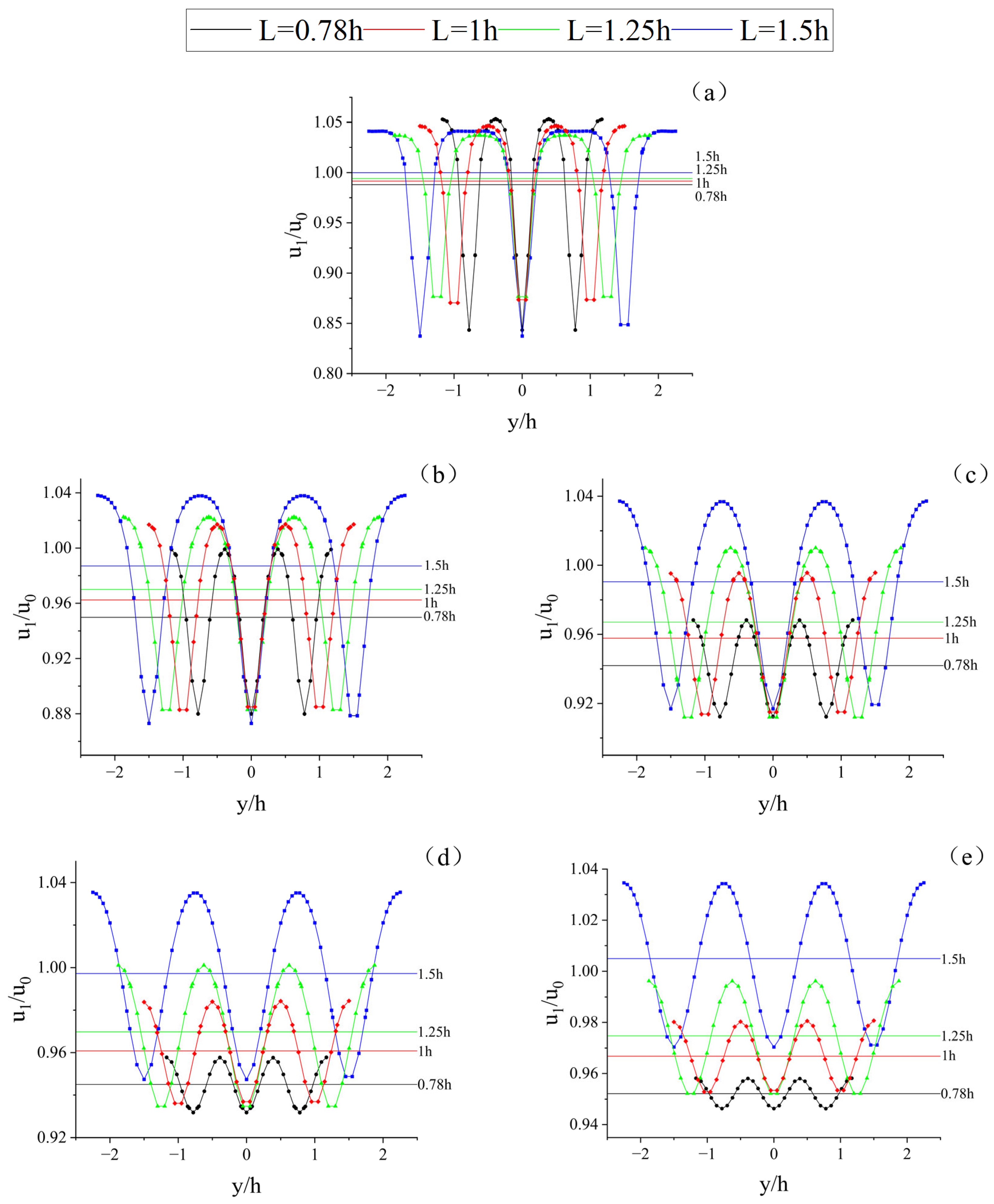
3.2. Sensitivity Analysis of the Plant Spacing Variation
3.2.1. Vertical Velocity Profiles
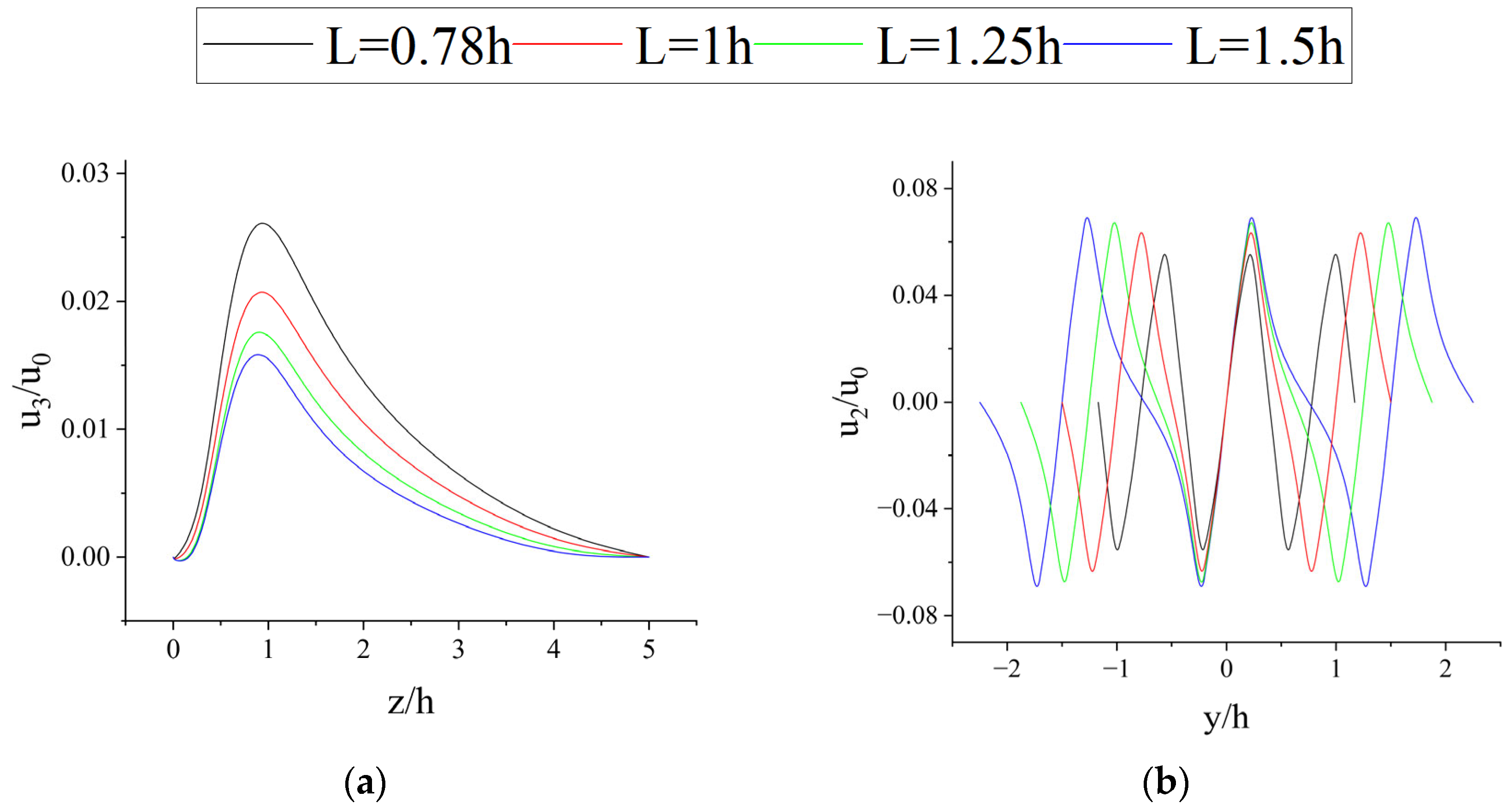
3.2.2. Influence of Canopy on the Flow Field
3.2.3. Influence of Solid Trunk on the Flow Field
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Li, S.Y.; Tang, Q.L.; Lei, J.Q.; Xu, X.W.; Jiang, J.; Wang, Y.D. An overview of non-conventional water resource utilization technologies for biological sand control in Xinjiang, northwest China. Environ. Earth Sci. 2015, 73, 873–885. [Google Scholar] [CrossRef]
- Li, H.L.; Wang, Y.D.; Li, S.Y.; Askar, A.; Wang, H.F. Shelter Efficiency of Various Shelterbelt Configurations: A Wind Tunnel Study. Atmosphere 2022, 13, 1022. [Google Scholar] [CrossRef]
- Yang, W.B.; Li, W.; Dang, H.Z.; Feng, W.; Lu, Q.; Jiang, L.N.; Yang, H.Y.; Wu, X.Q. Desertification Control with Low Coverage Vegetation—Principles, Models and Effects; Science Press: Beijing, China, 2016; pp. 316–320. [Google Scholar]
- Cornelis, W.M.; Gabriels, D. Optimal windbreak design for wind-erosion control. J. Arid. Environ. 2005, 61, 315–332. [Google Scholar] [CrossRef]
- Cheng, H.; He, W.W.; Liu, C.C.; Zou, X.Y.; Kang, L.Q.; Chen, T.; Zhang, K. Transition model for airflow fields from single plants to multiple plants. Agric. For. Meteorol. 2019, 266, 29–42. [Google Scholar] [CrossRef]
- Mayaud, J.R.; Wiggs, G.F.; Bailey, R.M. Characterizing turbulent wind flow around dryland vegetation. Earth Surf. Process. Landf. 2016, 41, 1421–1436. [Google Scholar] [CrossRef]
- Okin, G.S. A new model of wind erosion in the presence of vegetation. J. Geophys. Res. Earth Surf. 2008, 113, 758. [Google Scholar] [CrossRef]
- Cheng, H.; Liu, C.; Kang, L. Experimental study on the effect of plant spacing, number of rows and arrangement on the airflow field of forest belt in a wind tunnel. J. Arid. Environ. 2020, 178, 104169. [Google Scholar] [CrossRef]
- Dong, Z.B.; Luo, W.Y.; Qian, G.Q.; Wang, H.T. A wind tunnel simulation of the mean velocity fields behind upright porous fences. Agric. For. Meteorol. 2007, 146, 82–93. [Google Scholar] [CrossRef]
- Svensson, U.; Häggkvist, K. A two-equation turbulence model for canopy flows. J. Wind. Eng. Ind. Aerodyn. 1990, 35, 201–211. [Google Scholar] [CrossRef]
- Gash, J.H.C. Observations of turbulence downwind of a forest-heath interface. Boundary-Layer Meteorol. 1986, 36, 227–237. [Google Scholar] [CrossRef]
- Wu, T.G.; Yu, M.K.; Wang, G.; Wang, Z.X.; Duan, X.; Dong, Y.; Cheng, X.Y. Effects of stand structure on wind speed reduction in a Metasequoia glyptostroboides shelterbelt. Agrofor. Syst. 2013, 87, 251–257. [Google Scholar] [CrossRef]
- Wu, X.X.; Zou, X.Y.; Zhou, N.; Zhang, C.L.; Shi, S. Deceleration efficiencies of shrub windbreaks in a wind tunnel. Aeolian Res. 2015, 16, 11–23. [Google Scholar] [CrossRef]
- Rosenfeld, M.; Marom, G.; Bitan, A. Numerical simulation of the airflow across trees in a windbreak. Boundary-Layer Meteorol. 2010, 135, 89–107. [Google Scholar] [CrossRef]
- Sogachev, A.; Panferov, O. Modification of two-equation models to account for plant drag. Boundary-Layer Meteorol. 2006, 121, 229–266. [Google Scholar] [CrossRef]
- Santiago, J.L.; Martin, F.; Cuerva, A.; Bezdenejnykh, N.; Sanz-Andrés, A. Experimental and numerical study of wind flow behind windbreaks. Atmos. Environ. 2007, 41, 6406–6420. [Google Scholar] [CrossRef]
- Wilson, J.D.; Yee, E. Calculation of winds disturbed by an array of fences. Agric. For. Meteorol. 2003, 115, 31–50. [Google Scholar] [CrossRef]
- Packwood, A.R. Flow through porous fences in thick boundary layers: Comparisons between laboratory and numerical experiments. J. Wind. Eng. Ind. Aerodyn. 2000, 88, 75–90. [Google Scholar] [CrossRef]
- Mochida, A.; Tabata, Y.; Iwata, T.; Yoshino, H. Examining tree canopy models for CFD prediction of wind environment at pedestrian level. J. Wind. Eng. Ind. Aerodyn. 2008, 96, 1667–1677. [Google Scholar] [CrossRef]
- Endalew, A.M.; Hertog, M.; Delele, M.A.; Baetens, K.; Persoons, T.; Baelmans, M.; Verboven, P. CFD modelling and wind tunnel validation of airflow through plant canopies using 3D canopy architecture. Int. J. Heat Fluid Flow 2009, 30, 356–368. [Google Scholar] [CrossRef]
- Heisler, G.M.; Dewalle, D.R. Effects of windbreak structure on wind flow. Agric. Ecosyst. Environ. 1988, 22, 41–69. [Google Scholar] [CrossRef]
- Perera, M.D.A.E.S. Shelter behind two-dimensional solid and porous fences. J. Wind. Eng. Ind. Aerodyn. 1981, 8, 93–104. [Google Scholar] [CrossRef]
- Skidmore, E.L.; Hagen, L.J. Evapotranspiration and the Aerial Environment as Influenced by Windbreaks; Springer: Berlin/Heidelberg, Germany, 1970. [Google Scholar]
- Launder, B.E.; Spalding, D.B. The numerical computation of turbulent flows. In Numerical Prediction of Flow, Heat Transfer, Turbulence and Combustion; Pergamon: Berlin, Germany, 1983; pp. 96–116. [Google Scholar]
- Liu, C.C.; Zheng, Z.Q.; Cheng, H.; Zou, X.Y. Airflow around single and multiple plants. Agric. For. Meteorol. 2018, 252, 27–38. [Google Scholar] [CrossRef]
- Blocken, B.; Carmeliet, J.; Stathopoulos, T. CFD evaluation of wind speed conditions in passages between parallel buildings—Effect of wall-function roughness modifications for the atmospheric boundary layer flow. J. Wind. Eng. Ind. Aerodyn. 2007, 95, 941–962. [Google Scholar] [CrossRef]
- Leenders, J.K.; Boxel, J.H.; Sterk, G. The effect of single vegetation elements on wind speed and sediment transport in the Sahelian zone of Burkina Faso. Earth Surf. Process. Landf. J. Br. Geomorphol. Res. Group 2007, 32, 1454–1474. [Google Scholar] [CrossRef]
- Ma, R.; Wang, J.; Qu, J.; Liu, H. Effectiveness of shelterbelt with a non-uniform density distribution. J. Wind. Eng. Ind. Aerodyn. 2010, 98, 767–771. [Google Scholar] [CrossRef]
- Judd, M.J.; Raupach, M.R.; Finnigan, J.J. A wind tunnel study of turbulent flow around single and multiple windbreaks, part I: Velocity fields. Boundary-Layer Meteorol. 1996, 80, 127–165. [Google Scholar] [CrossRef]
- Raupach, M.R.; Finnigan, J.J.; Brunet, Y. Coherent eddies and turbulence in vegetation canopies: The mixing-layer analogy. Boundary-Layer Meteorology 25th Anniversary Volume, 1970–1995: Invited Reviews and Selected Contributions to Recognise Ted Munn’s Contribution as Editor over the Past 25 Years; Springer: Berlin/Heidelberg, Germany, 1996; pp. 351–382. [Google Scholar]
- Van, E.J.; Karschon, R.; Razumova, L.A.; Robertson, G.W. Windbreaks and shelterbelts. World Meteorological Orgabization, Commission for Agricultural Meteorology; World Meteorological Organization: Geneva, Switzerland, 1964; p. 188. [Google Scholar]





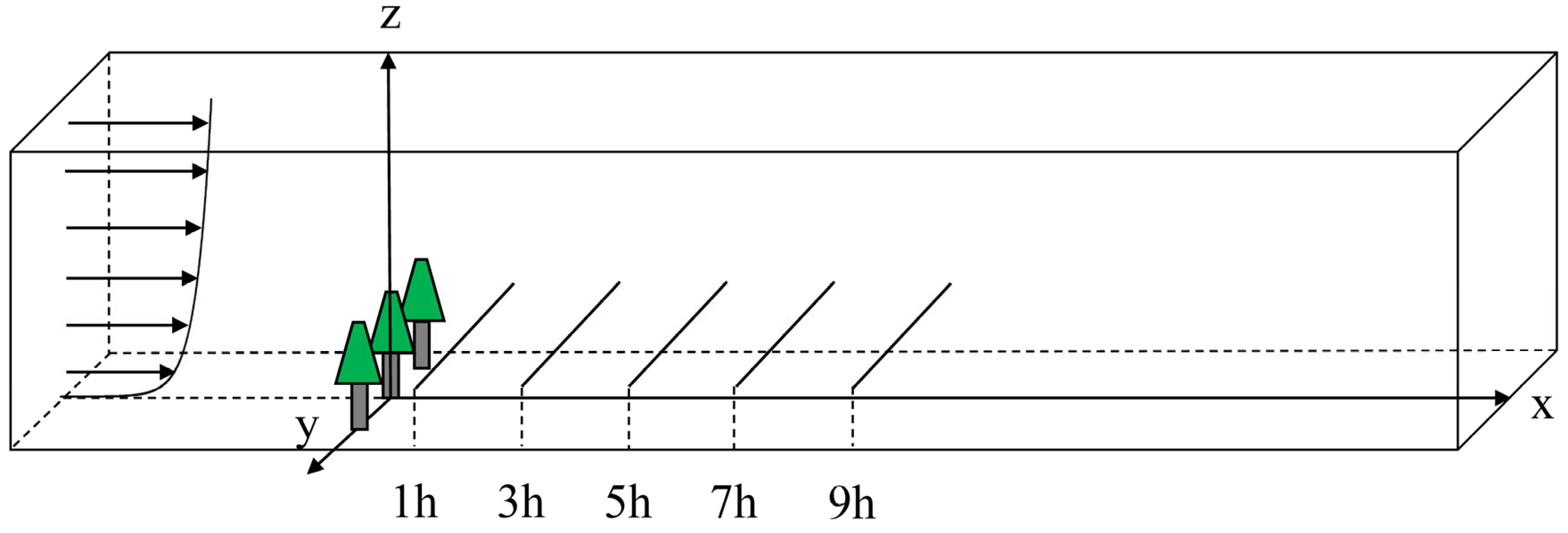

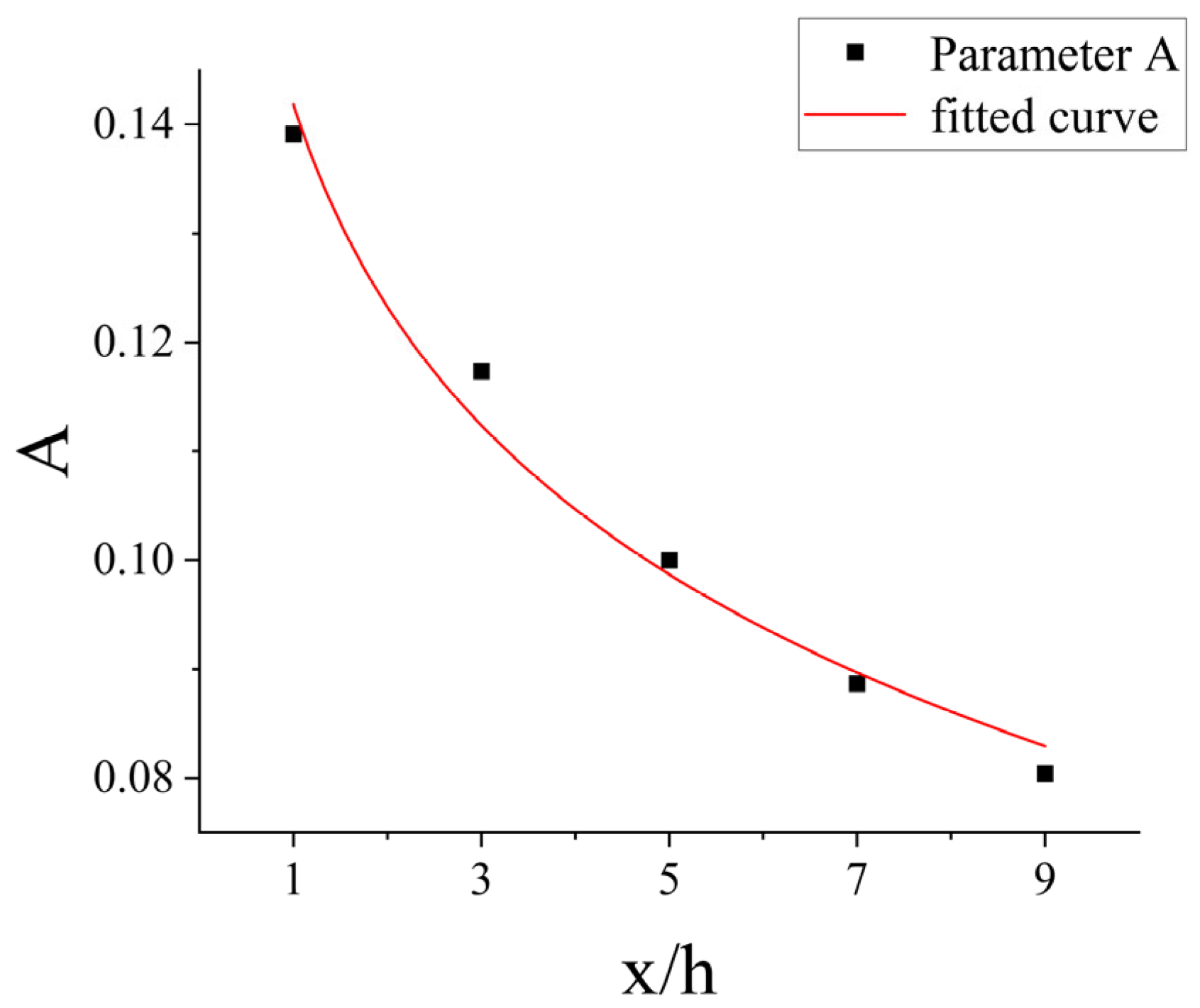

| Leeward Distance | Parameter A |
|---|---|
| 1 h | 0.13912 |
| 3 h | 0.11737 |
| 5 h | 0.10001 |
| 7 h | 0.08868 |
| 9 h | 0.0804 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, Y.; Huang, N.; Sun, J.; Zhan, K.; Li, X.; Han, B.; Zhang, J. Numerical Simulation of the Plant Shelterbelt Configuration Based on Porous Media Model. Atmosphere 2024, 15, 602. https://doi.org/10.3390/atmos15050602
Zhao Y, Huang N, Sun J, Zhan K, Li X, Han B, Zhang J. Numerical Simulation of the Plant Shelterbelt Configuration Based on Porous Media Model. Atmosphere. 2024; 15(5):602. https://doi.org/10.3390/atmos15050602
Chicago/Turabian StyleZhao, Yuhao, Ning Huang, Jialiang Sun, Kejie Zhan, Xuanmin Li, Bin Han, and Jie Zhang. 2024. "Numerical Simulation of the Plant Shelterbelt Configuration Based on Porous Media Model" Atmosphere 15, no. 5: 602. https://doi.org/10.3390/atmos15050602
APA StyleZhao, Y., Huang, N., Sun, J., Zhan, K., Li, X., Han, B., & Zhang, J. (2024). Numerical Simulation of the Plant Shelterbelt Configuration Based on Porous Media Model. Atmosphere, 15(5), 602. https://doi.org/10.3390/atmos15050602








