1. Introduction
Accidental releases of hazardous materials can have severe consequences for human health and the environment. The dispersion of these materials can be significantly influenced by complex terrain and environmental conditions. In urban areas, the presence of buildings, streets, and other structures can create obstacles and alter wind patterns. Several real-world accidents have demonstrated the importance of considering the complex terrain and environmental factors in urban areas when assessing the dispersion of accidental releases. For instance, the Bhopal gas tragedy in India in 1984, which caused the release of toxic gas from a pesticide plant, was exacerbated by the local topography that trapped the gas in low-lying areas [
1]. Another example is the Buncefield oil depot explosion in the UK in 2005 which caused a large release of hydrocarbons and was complicated by the presence of nearby buildings that affected the dispersion of the material [
2].
The development of computing capacity makes it possible to use three-dimensional (3D) models to simulate the dispersion of a hazardous material in a complex environment. The use of these models raises a number of issues, the most significant of which are mesh management, how to introduce and maintain the atmospheric wind profile, and turbulence. Work has been initiated to support the proper use of these, whether for general use [
3] or for a particular application such as hydrogen [
4] or LNG safety [
5]. A key aspect is the validation of the tool, which consists of comparing its predicted results to experimental data, to assess its accuracy and reliability.
Unfortunately, there are little good-quality experimental data on dispersion in complex environments and urban areas. When they exist, they are obtained with regular obstacles.
The Mock Urban Setting Test (MUST) [
6] was a field experiment conducted by the Defense Threat Reduction Agency (DTRA) in 2001 at the U.S. Army Dugway Proving Ground (DPG) Horizontal Grid test site. The MUST design consisted of a regular 12 by 10 array of shipping containers. The tests consisted of releases of a tracer gas (propylene) in a range of different meteorological and urban scenarios.
The Jack Rabbit project was focused on studying the effects of chlorine releases in outdoor environments [
7]. The project was led by the Department of Homeland Security (DHS) Science and Technology Directorate (S&T) Chemical Security Analysis Center (CSAC). The Jack Rabbit project involved the controlled release of chlorine gas at various quantities and rates in outdoor environments. Shipping containers were positioned in various configurations to simulate urban environments, and the release of chlorine gas was studied to better understand its dispersion characteristics in such environments.
To fill this gap, Ineris launched an experimental campaign intended to study the atmospheric dispersion of massive carbon dioxide (CO2) releases on the CENZUB site (action training center) in an urban area located in Sissonne, France.
CO2 was chosen for the experiments not only for the practical aspects of studying the dispersion phenomenon but also for its relevance. Indeed, given the expansion of the carbon capture, utilization, and storage (CCUS) economy, the primary concerns revolve around the potential unintended hazards linked to CO2 leaks. CCUS is a process that captures carbon dioxide (CO2) emissions from industrial processes and power generation, and then either utilizes them for various purposes or stores them underground to prevent their release into the atmosphere. The development of CCUS technology has gained momentum in recent years as a potential solution to combat climate change. According to the Global CCS Institute, there are currently 65 large-scale CCUS facilities in operation or under construction worldwide, with a combined capture capacity of over 127 million tons per year. Europe is leading the way in CCUS development, with over half of the current operational or under-construction facilities located in the region. The United Kingdom, Norway, and the Netherlands are among the top countries in Europe with the highest number of CCUS projects. These figures demonstrate the increasing interest and investment in CCUS technology as a means of reducing greenhouse gas emissions and meeting climate goals.
The need to consider the complex environment and topography was highlighted by the Satartia incident. On 22 February 2020, a carbon dioxide (CO
2) pipeline operated by Denbury Gulf Coast Pipelines LLC (Denbury) ruptured in proximity to the community of Satartia, Mississippi. It resulted in local evacuations and caused almost 50 people to seek medical attention. The influence of topography in the dispersion was highlighted in the PHMSA failure investigation report [
8]: “
The weather conditions and unique topography of the accident site prevented the CO2 vapor from rapidly dispersing and allowing a plume to form that migrated toward Satartia”.
The main objective of this study was, therefore, to produce high-quality dispersion data replicating a large-scale accidental release of hazardous material in an urban area. A secondary objective was to test and qualify new sensor technologies. Ineris was once part of the Interreg SAFESIDE project [
9], the objective of which was the development of new analysis equipment based on infrared spectroscopy to allow for the measurement of the types of gases and their concentrations that are released during accidents. The SAFESIDE consortium was invited during the campaign to test their prototypes.
The paper describes in detail the experimental setup and the testing campaign. The main results are then presented and discussed.
2. Description of the Experimental Setup
2.1. Experiment Site
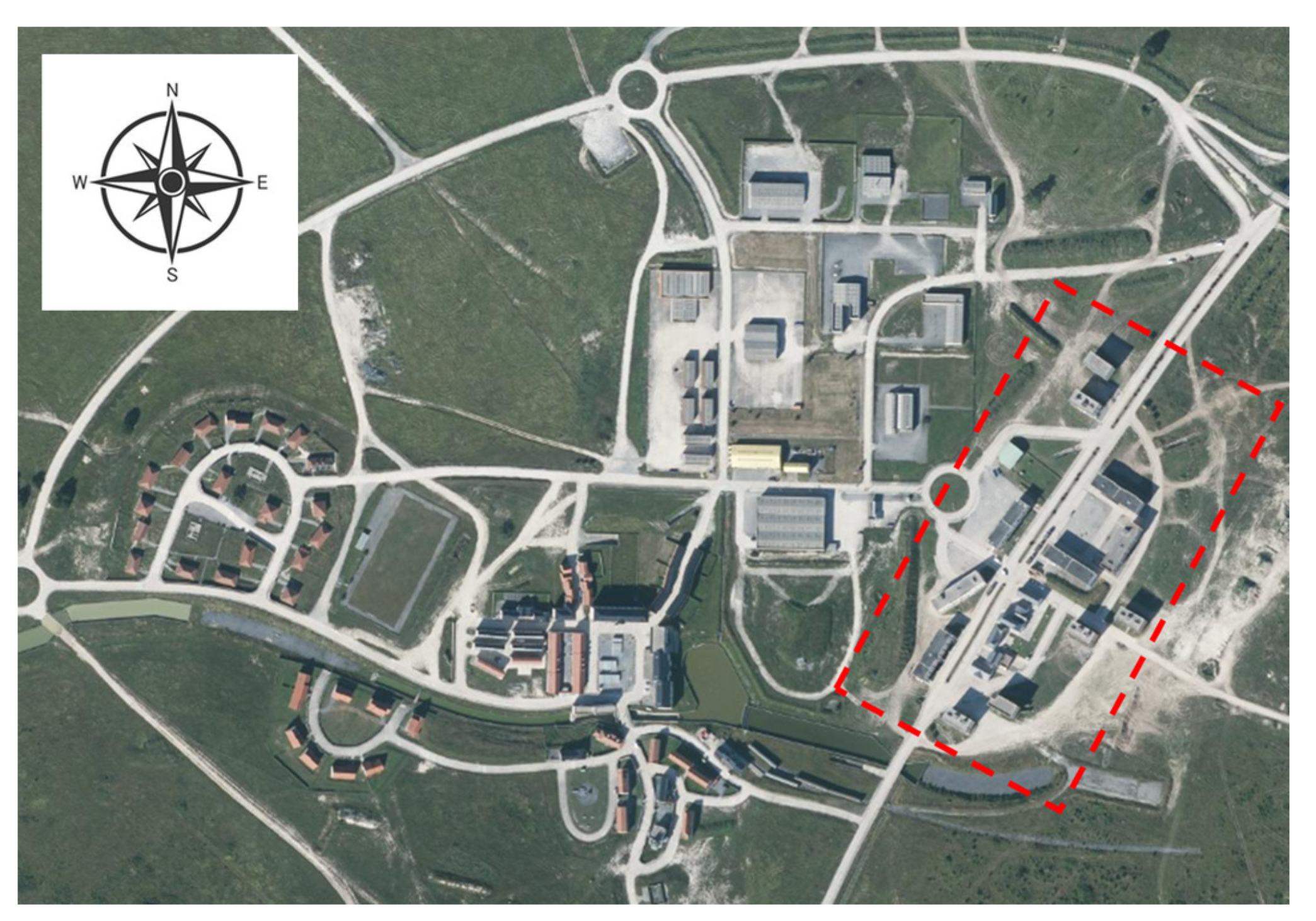
The
Centre d’entrainement aux actions en zone urbaine (CENZUB) is a purpose-built facility for training French armed forces in urban warfare skills. This otherwise uninhabited site is located at Sissonne in north-eastern France. It is the largest training area of its type in Europe. The site contains two constructed districts—Beauséjour and Jeoffrécourt. A satellite view of Jeoffrécourt, where the campaign was carried out, is presented in
Figure 1. In this figure, the red frame represents the experimental area.
We can distinguish four zones in the site: residential, city center, industrial, and modern. The experiments were carried out in the latter one, which is presented in
Figure 2. This zone is where the tallest buildings are located. Their height ranges from 7 to 17 m.
CENZUB and Ineris have initiated a collaboration; CENZUB is interested in improving its training scenarios and procedures to take into account industrial risk management in an urban environment. The experimental campaign in this paper was part of that objective.
2.2. Why CO2?
The main objective of the experimental campaign was to study dense gas dispersion in an urban area. To do so we chose CO2 which has a lot of advantages that facilitate its use for such a campaign:
Moreover, CO
2 and the risk associated with it are of first interest in the context of CO
2 economic growth. As presented in the introduction, the need for a validated dispersion tool that takes into account complex terrain was highlighted by the Satartia CO
2 pipeline incident. Ineris has a long experience in CO
2 experiments and has been involved in several projects related to the assessment of hazardous phenomena linked to CO
2 massive leakages (CO2Pipehaz, CO2Quest, and C4U). The fundamentally important issue is the accurate prediction of the fluid phase, discharge rate, and subsequent atmospheric dispersion during accidental releases from pressurized CO
2 pipelines to quantify all the hazard consequences associated with CO
2 pipeline failure, thus forming the basis for emergency response planning and determining minimum safe distances from populated areas. Several experimental discharge configurations with large storage conditions [
10,
11,
12,
13] have allowed a better understanding and evaluation of the dispersion of a CO
2 cloud. The blowdown of a highly instrumented pipeline has also been deeply studied considering the potential presence of impurities mixed in the CO
2 and their influence on the thermodynamic properties and formation of solids [
14], which could lead to clogging and would be a cause of leakage. Ineris has a good knowledge of the source term calculation and dedicated tools which allowed us to focus on dispersion. Moreover, we developed a method to calculate the concentration from the temperature measurement [
15]. During the experiments, temperature sensors were used as well as gas sensors.
2.3. Release System
The releases were made directly from a truck. To do so, a release device was connected to the filling line of the truck as shown in
Figure 3. It consisted of a 1.5 m long and 2-inch diameter pipe. It was directed horizontally at a height of 1 m. It is worth noting that after preliminary experiments, cables were added to help stabilize the system and avoid vibrations.
One truck was used for three experiments that were conducted on the same day. The CO2 inside the truck was at saturation at a pressure of about 15 bar. The quantity, pressure, and temperature inside the truck were monitored using the embedded sensors. The pressure and mass were manually noted at regular intervals during the releases. This is how the mass flow rates presented below were obtained.
2.4. Instrumentation
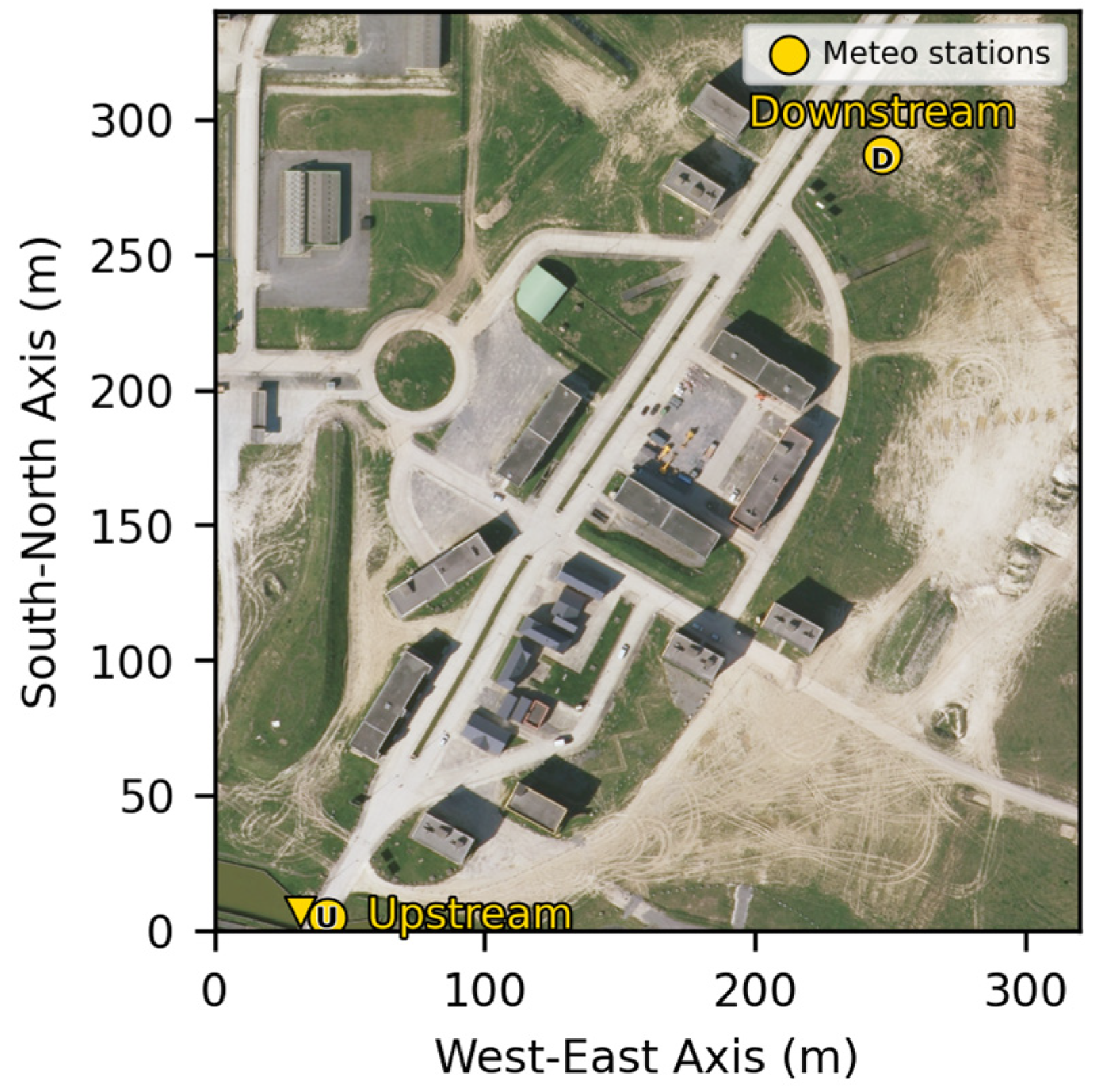
2.4.1. Weather Masts
To ensure that the wind field maintained a preferential direction over the entire test area, two weather masts were positioned, one upstream of the discharge point and the other downstream. The positions of the two weather masts used for the experiments are presented in
Figure 4.
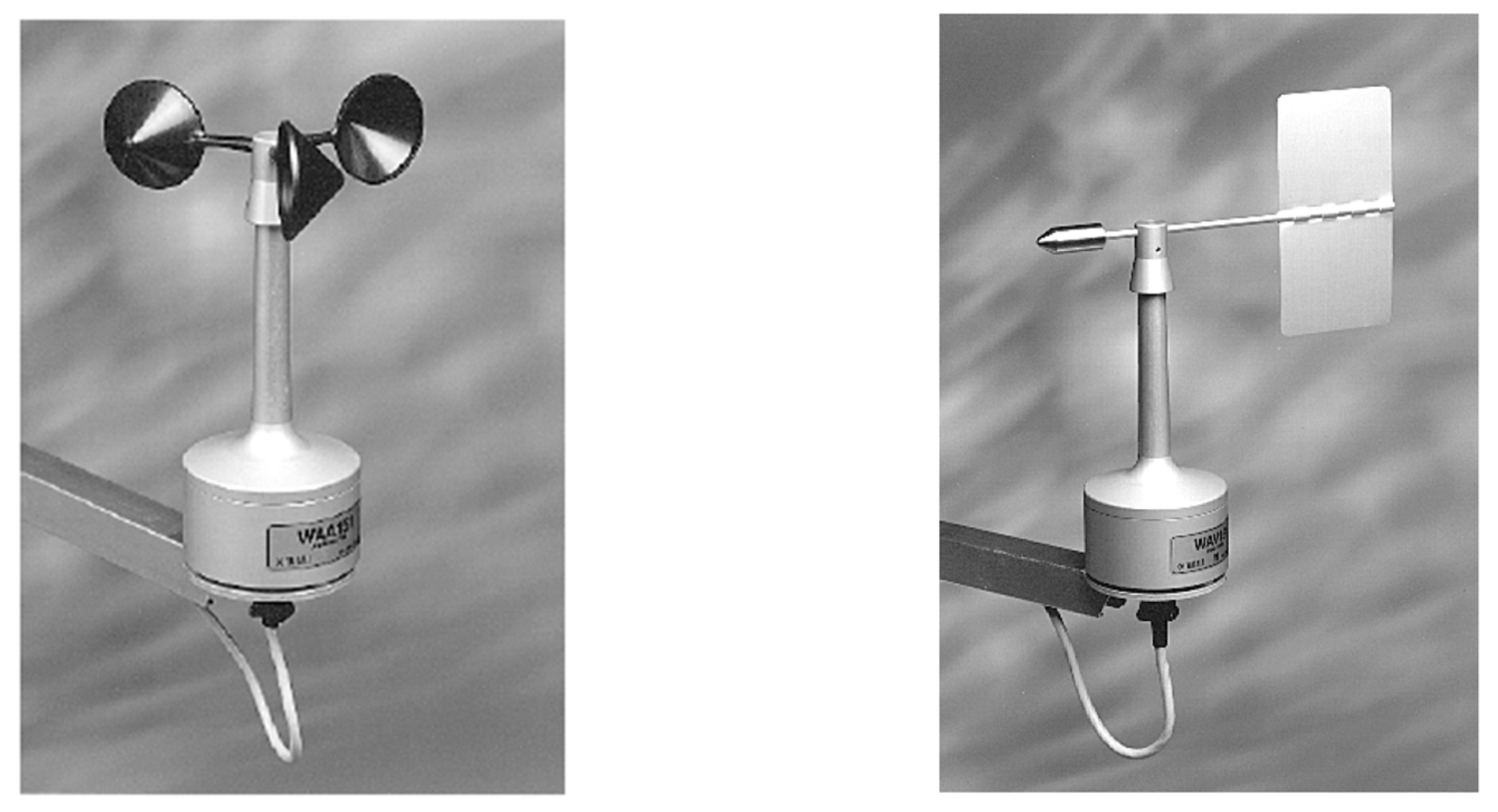
The upstream station sensor (
Figure 5) is an ultrasonic wind sensor that has three identical transducers that generate ultrasonic signals. Wind speed and direction are determined by measuring the time the ultrasonic signal takes to move from one transducer to the other transducers. The vertical component of the velocity is not measured. The acquisition frequency is 0.1 Hz. The accuracy of the measure is ±3° for the wind direction and 3% for the wind speed. The response time of this sensor is 0.25 s.
The downstream station sensor (
Figure 6) uses mechanical technology. It is a device consisting of a wind vane and an anemometer to measure the direction and speed of the wind, respectively. The vertical component of the velocity is not measured. The acquisition frequency is 1 Hz. The accuracy of the measurement is ±2.8° for the wind direction and ±0.17 m/s for the wind speed.
Both stations also measure precipitation, ambient temperature, pressure, and relative humidity.
2.4.2. Sensors (IR, Catharometer, Tk)
Three technologies of sensors have been used during this experimental campaign. The technologies are described below.
IR
An infrared gas concentration sensor operates based on the principle of absorption of infrared (IR) radiation by gas molecules. It utilizes the specific absorption characteristics of gases in the IR spectrum to measure their concentrations accurately. The sensor consists of an IR source that emits a beam of IR radiation at specific wavelengths, typically in the mid-infrared range. The IR beam passes through a chamber containing the gas to be measured. On the other side of the chamber, there is an IR detector that detects the intensity of the transmitted radiation. Gas molecules in the chamber absorb specific wavelengths of IR radiation according to their molecular properties. The amount of absorption depends on the concentration of the gas in the chamber, i.e., the more concentrated the gas, the greater the absorption of specific IR wavelengths. The IR detector measures the intensity of the transmitted radiation after it has passed through the gas sample. By comparing this intensity with the intensity of the initial IR beam emitted by the source, the sensor can determine the absorption caused by the gas in the chamber. This absorption is directly related to the concentration of the gas. To ensure accurate measurements, the sensors have been calibrated in a laboratory to establish a calibration curve. This curve relates the detected absorption to the CO2 gas concentrations. During the experiment, the sensor uses this calibration curve to convert the measured absorption into a gas concentration value. Two types of IR sensors were used for the experiments with two different operating ranges, denoted IR1 et IR2.
Catharometer
A catharometer operates based on the principle of thermal conductivity. The sensor consists of two parallel, heated wire elements made of different materials. When a gas sample flows over the sensor, the thermal conductivity of the gas affects the temperature of the wires differently. The wire with a higher thermal conductivity will be cooler compared to the other wire. This temperature difference between the two wires is directly proportional to the concentration of the gas being measured. To measure the gas concentration, a constant current is passed through the wire elements to maintain their temperature. A Wheatstone bridge circuit is used to measure the temperature difference between the two wires. The bridge circuit compares the resistance of the two wires and produces an output voltage proportional to the temperature difference. The output voltage is then calibrated to correspond to the concentration of the target gas. This calibration is performed by comparing the sensor’s response to known concentrations of the gas under controlled conditions. The calibration curve is typically established by using a reference gas mixture with known concentrations.
Tk
A methodology developed by Jamois et al. [
15] allows for estimating the CO
2 concentration by means of the measure of local temperature, considering that in the far field the local temperature is much larger than −80 °C:
where
,
and
are the specific heat for the respective gases,
is the ambient temperature,
is the temperature measured at the sampling point in the CO
2 plume,
is the temperature of the CO
2 at the end of the jet expansion zone,
is the mass fraction of air humidity which should be condensed at
(equilibrium),
is the latent heat of condensation for water,
is the latent heat of condensation (vapor to solid) for CO
2, and
is the mass fraction of condensed CO
2 at the end of the jet expansion zone. Good results were obtained with
.
The main sensor characteristics are summarized in
Table 1.
3. Experimental Campaign Overview
3.1. Experimental Configuration
Three CO
2 releases were performed the same day with one truckload in three different configurations: one axial street release (experiment n°2) and two impacting releases (against a small (experiment n°3) and high-rise building (experiment n°1)). These configurations are presented in
Figure 7.
The three experiments were conducted on the same day. Between each experiment, the truck and the sensors were moved to prepare for the following experiment. The schedule, duration, and mass flow rates of the experiments are presented in
Table 2. The first experiment was stopped after 2 min because of a significant accumulation of gas and the return of the gas to the point of discharge generating risk for operators. Due to the short duration, the flow rate could not be accurately calculated. It was surely of the same order of magnitude as the two other experiments that lasted about 15 min. The amount of CO
2 released during the first experiment was around 900 kg and around 6500 kg was released during experiments 2 and 3.
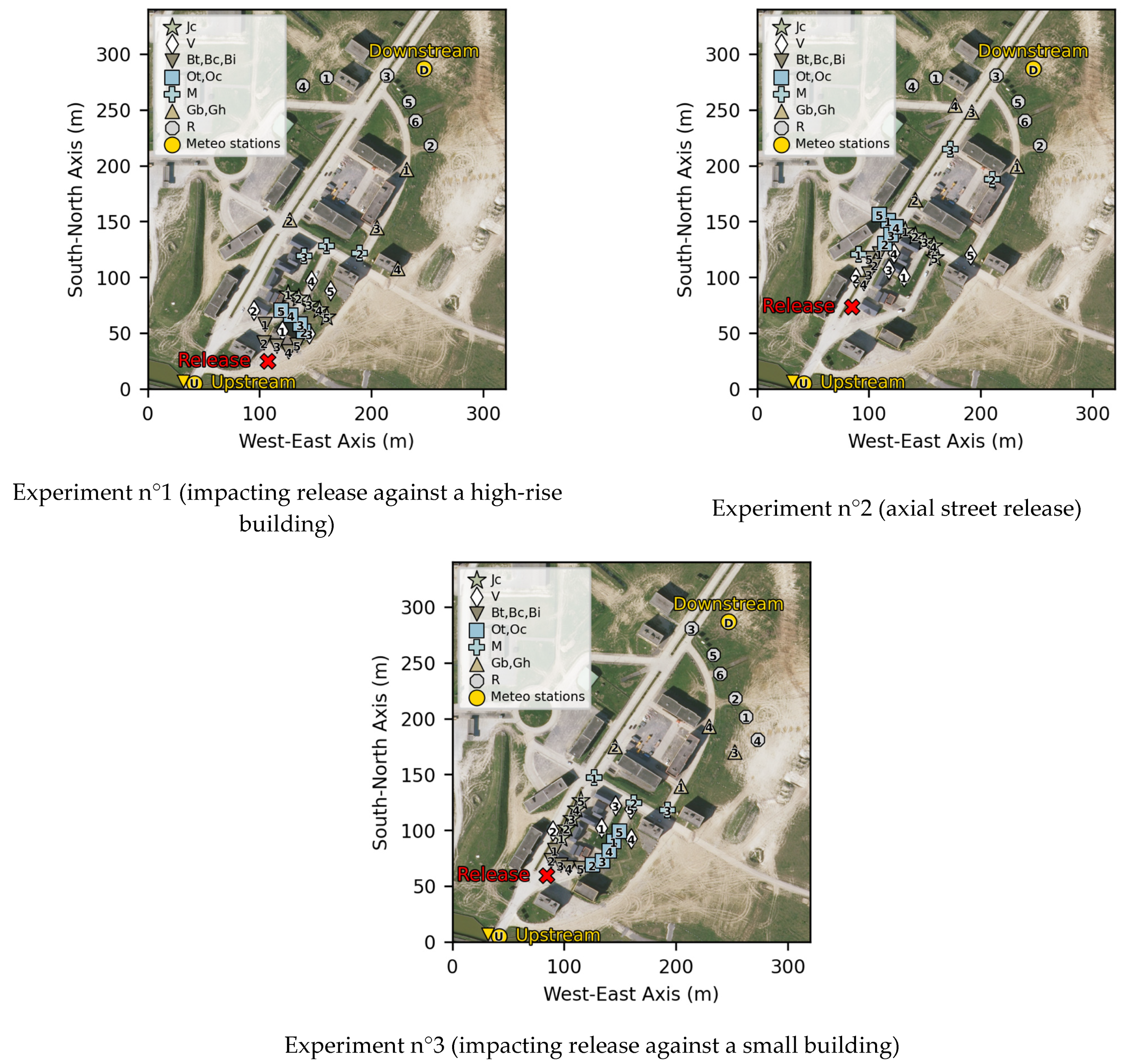
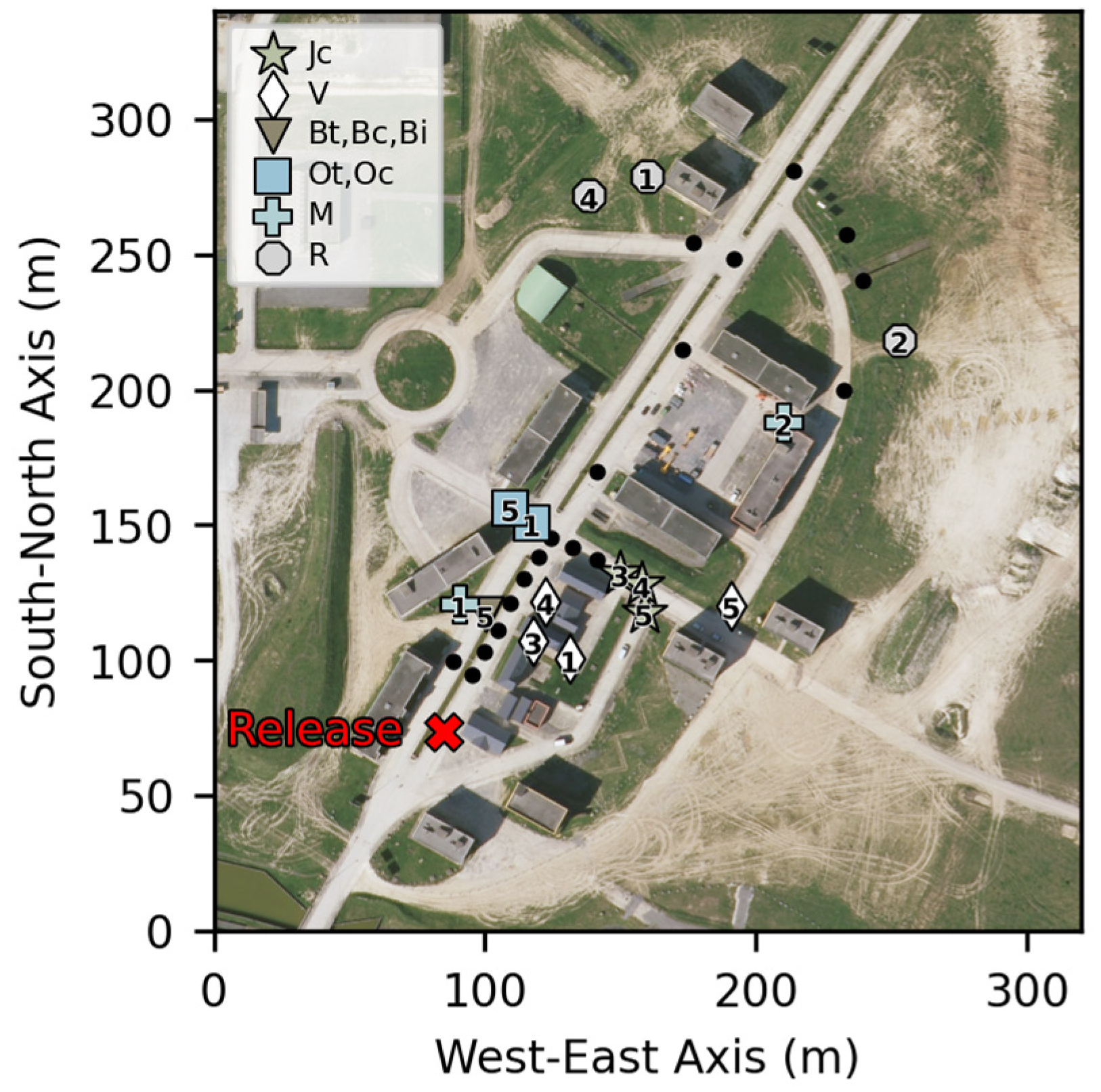
3.2. Sensor Map
Three technologies of sensors were used to monitor the CO
2 concentration. Their characteristics are described in
Section 2.4.2. The nomenclature used is presented in
Table 3 and the sensor map for each experiment is shown in
Figure 8.
In line with their measurement range, the catharometers were preferentially placed in the near field (typically < 50 m), and the infrared sensors in the more distant field. Different technologies of sensors were placed together at some points to be able to compare their measurements.
All sensors were located at a 1 m height except for the four IR1 that were placed at a 3 m height. Four IR1 were placed at a 1 m height at the same location to assess the impact of height on the measured concentrations. The 1 m high sensor position was adapted to evaluate the behavior of heavy gases. The 3 m high sensor position provides an overview of the vertical concentration gradient.
Depending on the experiment configuration, the sensors were positioned in places of strategic interest. For experiments 1 and 3, sensors were placed in front of the impacted building and behind or on the side of it to measure the impact of recirculation on gas concentration. Sensors were positioned in cross streets or between buildings to assess the influence of the built environment on dispersion. In the far field, an arc of sensors was positioned to assess the influence of the experiment configuration beyond the building zone.
The indices
c,
t, and
i refer to the catharometer, thermocouple, and infrared technologies, respectively. The first letters refer to the group of sensors (see
Figure 8).
3.3. Weather Conditions
Meteorological data play a crucial role in dispersion trial experiments as it provides essential information about the atmospheric conditions that influence the dispersion phenomenon. Their precise measurement is therefore essential.
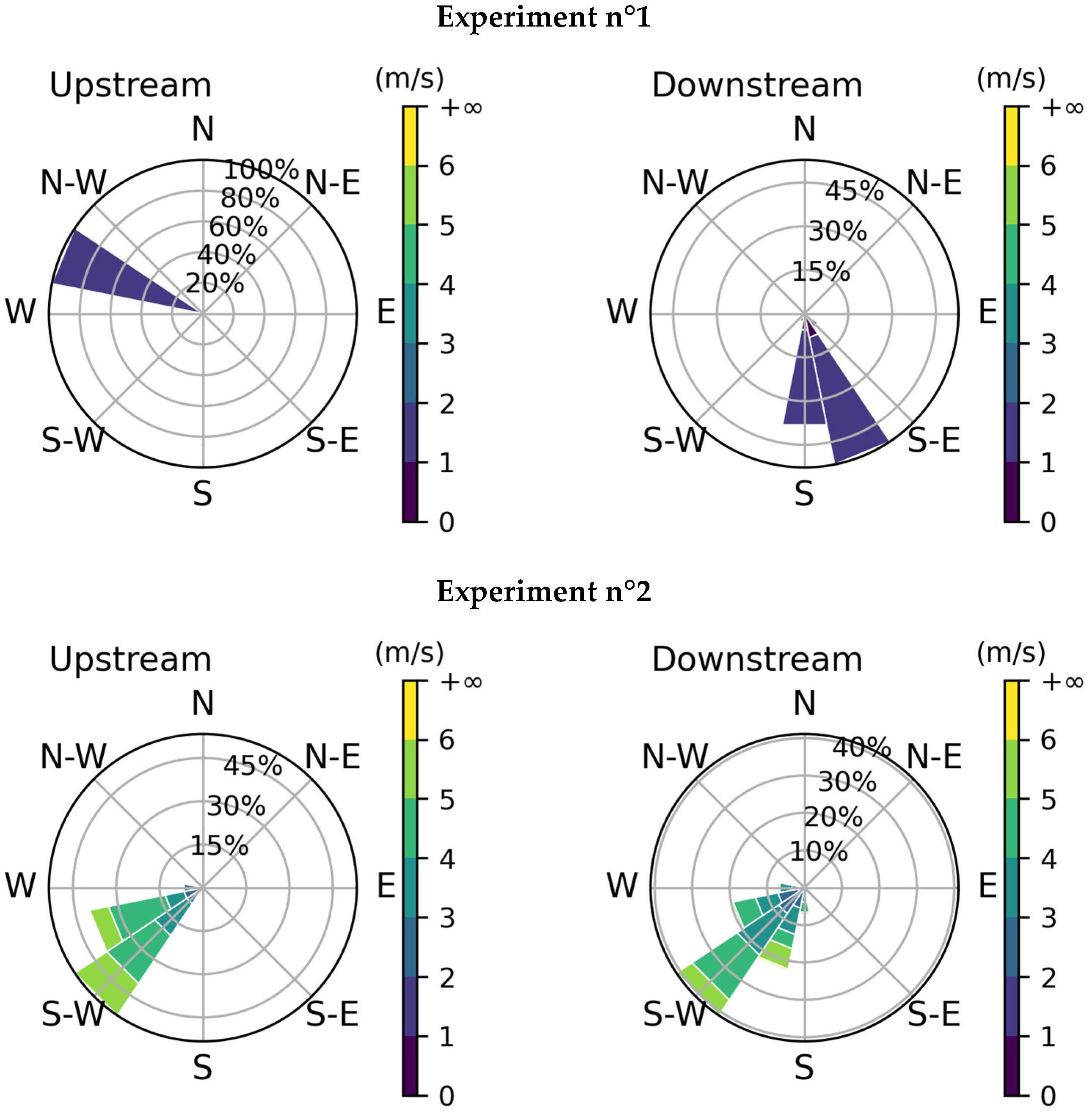
Two weather stations were used, one upstream and one downstream. The raw data collected from the stations include wind velocity and direction, temperature, and humidity. The acquisition rate was 1 s for the downstream station and 60 s for the upstream station. The wind changes obtained for both stations and during each experiment are shown in
Figure 9 and the mean conditions are in
Table 4. By convention, the wind direction indicates where it comes from; the 0° corresponds to the north, and the angle increases clockwise.
The results show good consistency between the two stations except for the first experiment. There are two reasons for this. The first one is that the wind velocity was very low so the direction was much more variable. The second one is that the release was short so we do not have a lot of measurements for the upstream station. The average wind velocity and direction of the first experiment will not be analyzed hereafter.
The mean conditions are not sufficient to represent weather conditions. It is also interesting to look at fluctuations. To do so, we need to rotate the reference frame so that one of the new unit vectors (denoted
a) is parallel to the mean wind direction. By definition, the mean wind direction projected onto the unit vector
b (orthogonal to
a) is zero. The mean wind velocity and variances of its two components are given in
Table 5. Fluctuations increase throughout the day, which is consistent with the fact that it was a sunny day, i.e., as the ground heats up, turbulence increases.
4. Results Analysis
4.1. Data Processing
For each sensor technology, the data acquired were post-processed to obtain the evolution of concentration. The behavior was similar for each sensor and experiment. Before the cloud, there was a plateau that represented the background concentration of CO
2 in the air. As the cloud passes the concentration fluctuates. After the discharge, the concentration returns to a level close to that prior to the discharge, except for some catharometers for which some saturation effects were observed (
Figure 10).
These data were then processed:
Negative values were set to 0.
A background concentration was calculated for each sensor. The average concentration over an interval of 1 min prior to the release was used. Then, the background concentration was subtracted from all the data to obtain the relative concentration.
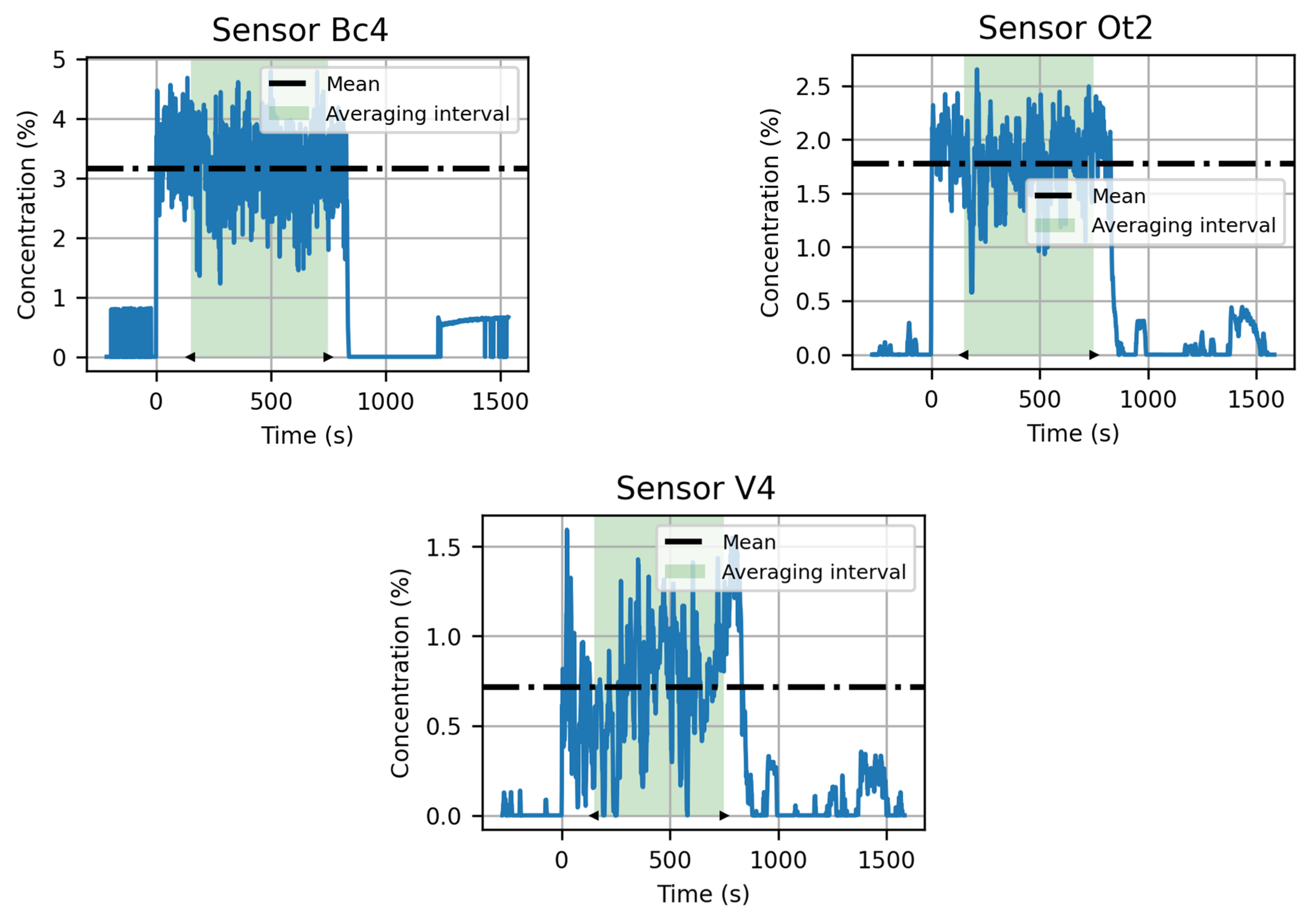
To find an average concentration for each sensor, the following method was used. We calculated the average concentration over a period of 10 min during the releases (which lasted approximately 15 min). This way, we ensured that the cloud was established at every measuring point and that the method was the same for all sensors. For the first experiment that lasted only 2 min, the duration was insufficient to carry out such a study. The method is illustrated in
Figure 11.
In
Figure 11, the blue lines show the evolution of the concentration at selected sensors, the green area displays the 10-minute period in which the mean was computed, and the dashed lines show the average concentrations. Concentrations measured during experiment 1 were not subsequently analyzed in the present article, as the short duration of the release did not allow a steady state to be reached.
4.2. Sensors Technology Analysis
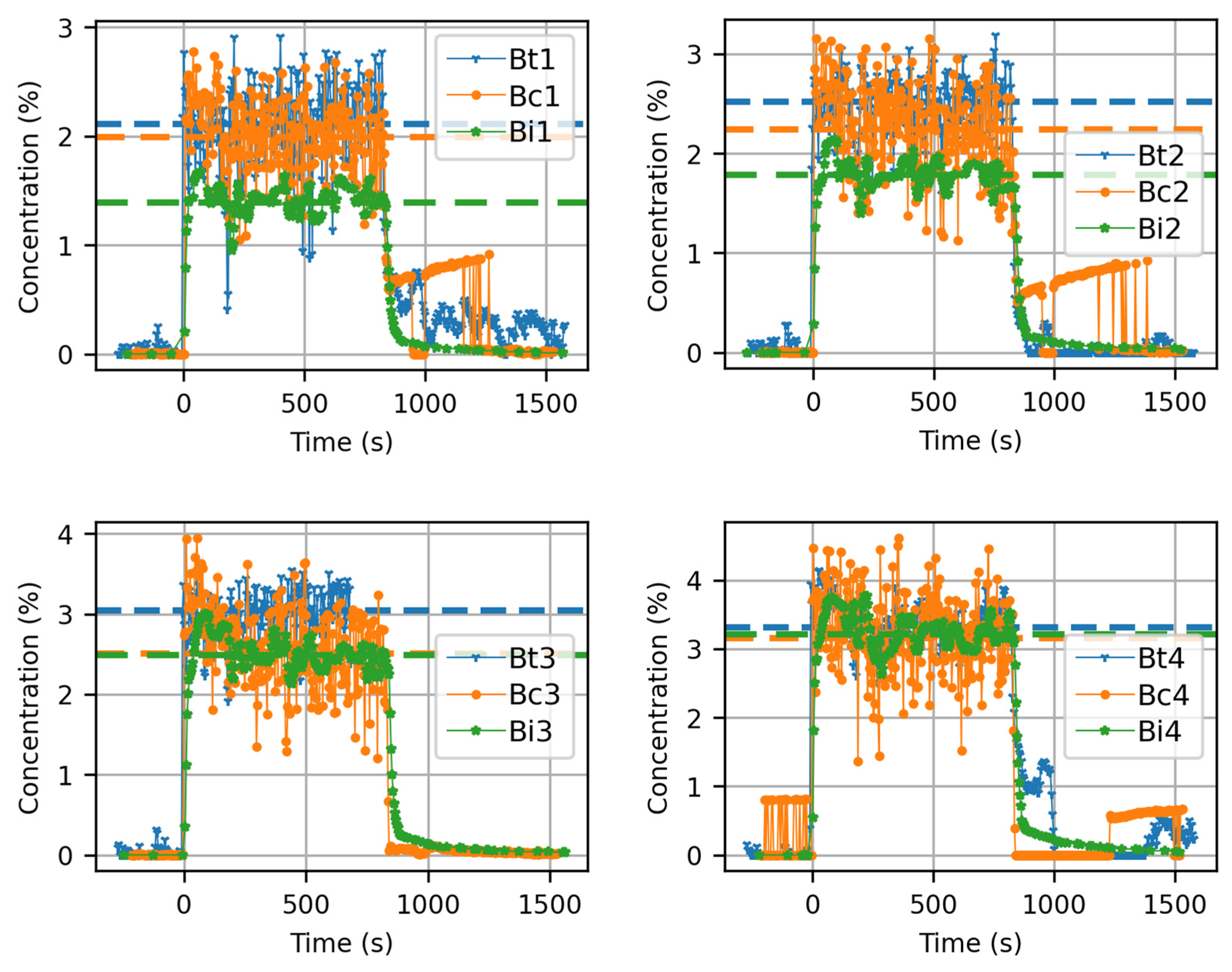
Figure 12 displays the treated data for the three sensor technologies located in four near-field positions for experiment 2. These three technologies were compared at the B position. As shown in
Figure 8, sensors B1 to B4 were located very close to the source release and arranged, on its axis, in descending order (B4 was the closest to the release and B1 was the furthest away).
Catharometer and thermocouple technologies present larger fluctuations than the IR1 technology due to their smaller response time (respectively, <1 s against 30 s). The dashed lines show the average concentrations in
Figure 12. These mean concentrations calculated for the three technologies are in good agreement. The differences observed can be explained by the measurement uncertainties associated with the sensors themselves and their use. Catharometers and IR1 concentration measurements are sensitive to temperature. Although a correction is applied, differences between the measurement points can also be explained by temperature differences. Finally, the presence of dry ice particles in the near field could interfere with the measurement.
4.3. Influence of the Built Environment on Dispersion
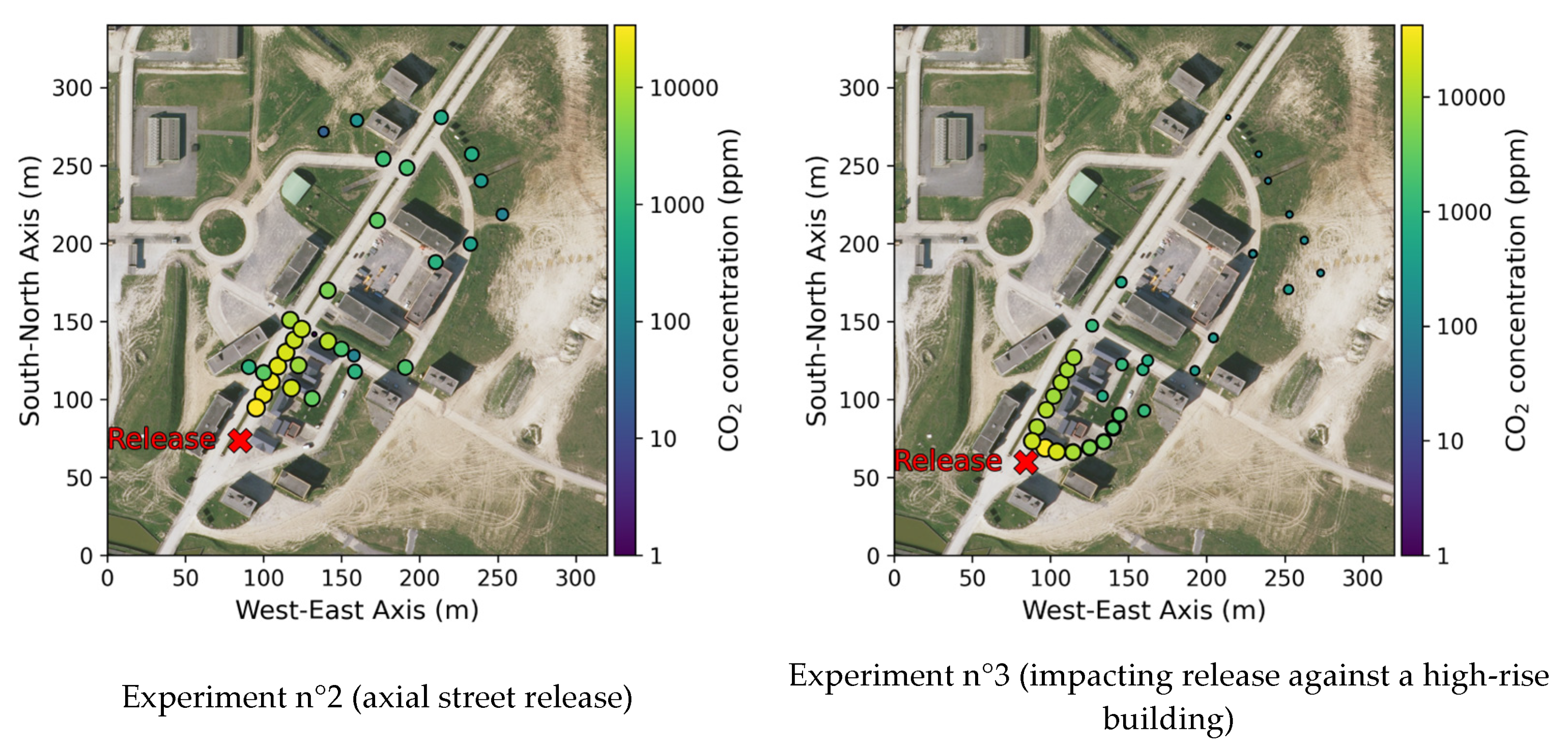
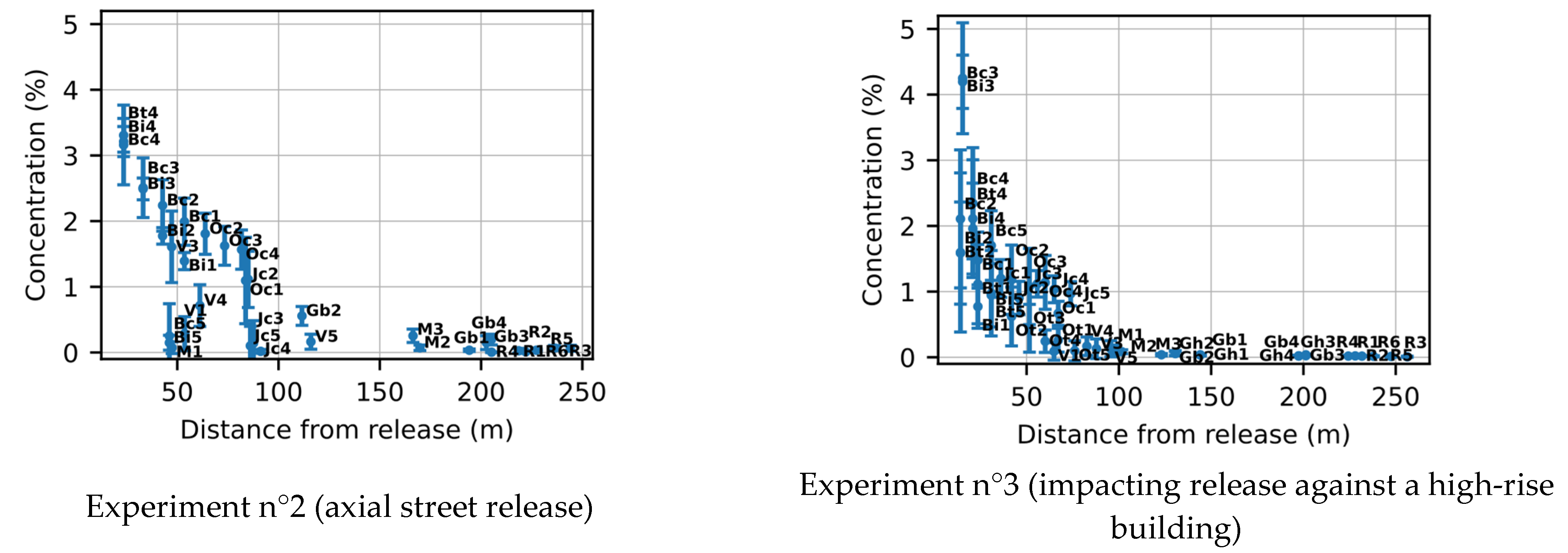
The cartography of the mean concentrations obtained for experiments n°2 and 3 are presented in
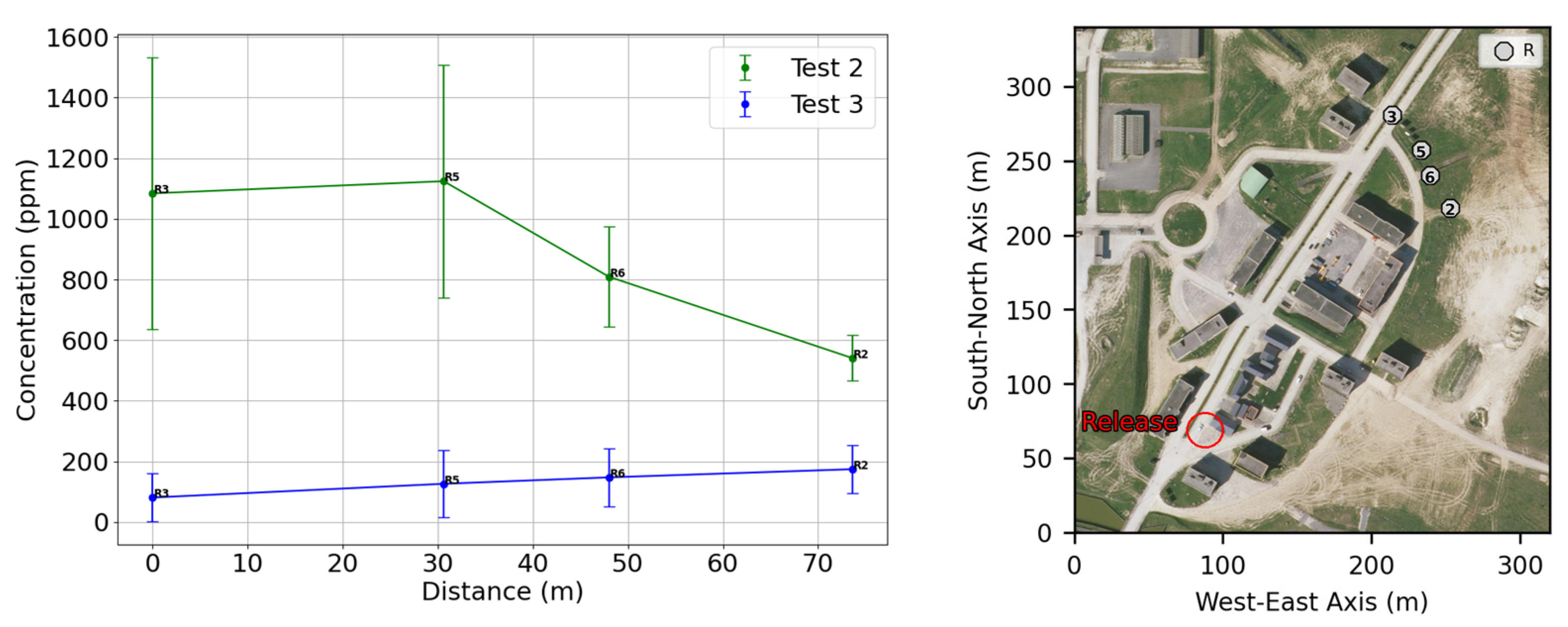
Figure 13. We can already see the influence of the buildings and how the gas goes through the streets, even the cross streets. In the far field, we can clearly see that the average concentrations are lower for experiment 3, where the release impacts a building in the near field, increasing the plume dilution. This difference is highlighted in
Figure 14, with the mean concentrations measured in the far field for sensors that were at the same position for the two experiments.
The mean and standard deviation of all the sensors in experiments 2 and 3 are presented in
Figure 15. The concentration of the closest sensor is higher for experiment 3 because of the accumulation of gas in front of the impacted building. Once again, we can see that the decrease in concentration is faster for experiment 3 due to the impact on the building which leads to greater dilution.
The mean concentration in the axial street for experiment 2 is presented in
Figure 16. The graph rupture observed at 100 m is caused by the specific airflow conditions induced by the crossed street where part of the cloud rushes in. This shows the importance of considering buildings in the dispersion modeling.
4.4. Statistical Analysis of the Concentrations
The influence of the built environment on the dispersion and mean concentrations was studied. It was also interesting to look at the fluctuations of concentrations.
Table 6 displays various statistical parameters related to concentration measurements (cf. [
16]) for experiment n°2:
- -
The average concentration (C) and standard deviation (σ);
- -
The conditional average (Cp) and standard deviation () based on non-zero concentrations;
- -
The intensity of fluctuation ;
- -
The conditional intensity ;
- -
The intermittency factor .
Sensors are listed in descending order of their intermittency factor. This factor represents the fraction of time the released gas is present at the sensor’s position.
Figure 17 represents a cartography of the sensors for experiment n°2.
Sensors with an intermittence factor of less than 100% are shown in
Figure 17. These sensors are those that were not in the axis of the release or of the wind and were subject to the meandering effect or sheltered by buildings. Sensors with an intermittence factor of 100% experienced a relatively consistent concentration profile due to their direct exposure to the plume. The observation of intermittency values below 100% in regions obstructed by obstacles suggests the presence of localized flow patterns and reduced mixing. When there are obstacles such as buildings in the path, the airflow becomes more complicated. It forms swirling patterns of air behind the obstacles, creating localized recirculation. These patterns disrupt the mixing and dilution of pollutants, causing less variation in pollution levels. These findings highlight the importance of considering the impact of obstacles on dispersion, particularly in urban areas.
5. Conclusions
To overcome the lack of experimental data on dispersion in complex urban areas, Ineris conducted an experimental campaign at the CENZUB site in Sissonne, France. The main objective was to study the atmospheric dispersion of massive carbon dioxide (CO2) releases in a realistic urban area. CO2 was chosen for its practicality and relevance in the context of the carbon capture, utilization, and storage (CCUS) economy. The development of CCUS technology has gained momentum as a potential solution to combat climate change, making it crucial to understand the dispersion risks associated with CO2 leaks.
The experimental setup involved releasing CO2 from a truck and monitoring its dispersion using various sensor technologies, including infrared gas concentration sensors, catharometers, and temperature sensors. The weather conditions, wind velocity and direction, were also measured using weather stations positioned at strategic locations. The data collected from the sensors were post-processed to analyze concentration levels and fluctuations. The results demonstrated the influence of the built environment on dispersion patterns.
Overall, this experimental campaign provided valuable high-quality data on CO2 dispersion in a realistic urban area. The findings emphasize the importance of considering complex terrain and environmental factors when assessing the risks associated with hazardous material releases in urban environments. The study contributes to the creation of data for the development of validated dispersion models and enhances our understanding of the potential consequences of accidental releases, particularly in the context of CCUS technology and carbon management.
A comparison of CFD tools predictions and these experimental results is currently underway. This raises the question of introducing the wind and turbulence atmospheric profile and maintaining it in the calculation domain for such tools.
Author Contributions
Conceptualization, L.J. and G.L.; methodology, L.J. and G.L.; software, L.J., G.L., T.C. and O.R.; validation, L.J., G.L. and T.C.; formal analysis, L.J., G.L. and O.R.; investigation, L.J. and G.L.; resources, L.J., G.L. and T.C.; data curation, L.J., G.L., T.C. and O.R.; writing—original draft preparation, L.J., G.L. and O.R.; writing—review and editing, L.J., G.L., T.C. and O.R.; visualization, L.J., G.L., T.C. and O.R.; supervision, L.J. and G.L.; project administration, L.J. and G.L.; funding acquisition, L.J. and G.L. All authors have read and agreed to the published version of the manuscript.
Funding
This research received no external funding.
Informed Consent Statement
Not applicable.
Data Availability Statement
On reasonable request, the corresponding author will provide the data that support the conclusions of this study.
Conflicts of Interest
The authors declare no conflict of interest.
References
- Singh, M.P.; Ghosh, S. Bhopal gas tragedy: Model simulation of the dispersion scenario. J. Hazard. Mater. 1987, 17, 1–22. [Google Scholar] [CrossRef]
- Atkinson, G.; Coldrick, S.; Gant, S.; Cusco, L. Flammable vapor cloud generation from overfilling tanks: Learning the lessons from Buncefield. J. Loss Prev. Process Ind. 2015, 35, 329–338. [Google Scholar] [CrossRef]
- INERIS. Guide de Bonnes Pratiques pour la Réalisation de Modélisations 3D pour des Scénarios de Dispersion Atmosphérique en Situation Accidentelle; Rapport de Synthèse des Travaux du Groupe de Travail National; INERIS: Verneuil-en-Halatte, France, 2015. [Google Scholar]
- Baraldi, D.; Melideo, D.; Kotchourko, A.; Ren, K.; Yanez, J.; Jedicke, O.; Giannissi, S.G.; Tolias, I.C.; Venetsanos, A.G.; Keenan, J.; et al. Development of a model evaluation protocol for CFD analysis of hydrogen safety issues the SUSANA project. Int. J. Hydrogen Energy 2017, 42, 7633–7643. [Google Scholar] [CrossRef]
- Ivings, M.J.; Lea, C.J.; Webber, D.M.; Jagger, S.F.; Coldrick, S. A protocol for the evaluation of LNG vapour dispersion models. J. Loss Prev. Process Ind. 2013, 26, 153–163. [Google Scholar] [CrossRef]
- Biltoft, C.A. Customer Report for Mock Urban Setting Test; DPG Document 8-CO (2001): 160-000; Defense Threat Reduction Agency: Alexandria, VA, USA, 2001. [Google Scholar]
- Fox, S.; Hanna, S.; Mazzola, T.; Spicer, T.; Chang, J.; Gant, S. Overview of the Jack Rabbit II (JR II) field experiments and summary of the methods used in the dispersion model comparisons. Atmos. Environ. 2022, 269, 118783. [Google Scholar] [CrossRef]
- PHMSA. Pipeline and Hazardous Materials Safety Administration Failure Investigation Report—Denbury Gulf Coast Pipelines LLC—Pipeline Rupture/Natural Force Damage, 22 February 2020; PHMSA: Washington, DC, USA, 2022.
- Défossez, F.; Hernandez, Y.; Lecourt, J.B.; Gognau, A.; Boivinet, S.; Vallon, R.; Parvitte, B.; Zeninari, V.; Brohez, S.; Dewaele, D.; et al. Real-time and on-field CO2 sensing based on a fast frequency modulation OPO system. Opt. Sens. Detect. SPIE 2022, 12139, 243–251. [Google Scholar]
- Mahgerefteh, H.; Fairweather, M.; Wareing, C.J.; Falle, S.A.E.G.; Mahgerefteh, H.; Martynov, S.; Brown, S.; Narasimhamurthy, V.D.; Storvik, I.E.; Sælen, E.; et al. CO2pipehaz: Quantitative hazard assessment for next generation CO2 pipelines. In Proceedings of the 22nd Hazards Symposium on Process Safety and Environmental Protection, Liverpool, UK, 11–14 April 2011. No. 156. ICHEME. NC. [Google Scholar]
- Woolley, R.M.; Proust, C.; Fairweather, M.; Falle, S.A.E.G.; Hebrard, J.; Jamois, D.; Wareing, C.J. Experimental measurement and RANS modelling of multiphase CO2 jet releases. In THMT-12: Proceedings of the Seventh International Symposium on Turbulence Heat and Mass Transfer, Palermo, Italy, 24–27 September 2012; Begel House Inc.: Danbury, CT, USA, 2012. [Google Scholar]
- Woolley, R.M.; Fairweather, M.; Wareing, C.J.; Falle, S.A.E.G.; Proust, C.; Hebrard, J.; Jamois, D. Experimental measurement and Reynolds-averaged Navier–Stokes modelling of the near-field structure of multi-phase CO2 jet releases. Int. J. Greenh. Gas Control. 2013, 18, 139–149. [Google Scholar] [CrossRef]
- Hébrard, J.; Jamois, D.; Proust, C.; Spruijt, M.; Hulsbosch-Dam, C.E.C.; Molag, M.; Messina, E. Medium scale CO2 releases. Energy Procedia 2016, 86, 479–488. [Google Scholar] [CrossRef]
- Martynov, S.; Zheng, W.; Mahgerefteh, H.; Brown, S.; Hebrard, J.; Jamois, D.; Proust, C. Computational and experimental study of solid-phase formation during the decompression of high-pressure CO2 pipelines. Ind. Eng. Chem. Res. 2018, 57, 7054–7063. [Google Scholar] [CrossRef]
- Jamois, D.; Proust, C.; Hebrard, J. Hardware and instrumentation to investigate massive releases of dense phase CO2. Can. J. Chem. Eng. 2015, 93, 234–240. [Google Scholar] [CrossRef]
- Wilson, D.J. Concentration Fluctuations and Averaging Time in Vapor Clouds; John Wiley & Sons: Hoboken, NJ, USA, 2010. [Google Scholar]
Figure 1.
Satellite view of Jeoffrécourt in France in the CENZUB site (coordinates in decimal degrees: N 49.546163, E 3.965208). The red frame represents the experimental area.
Figure 1.
Satellite view of Jeoffrécourt in France in the CENZUB site (coordinates in decimal degrees: N 49.546163, E 3.965208). The red frame represents the experimental area.
Figure 2.
Photograph of the Jeoffrécourt modern zone.
Figure 2.
Photograph of the Jeoffrécourt modern zone.
Figure 3.
Photograph of the release system installed on the truck (left) and cables (right).
Figure 3.
Photograph of the release system installed on the truck (left) and cables (right).
Figure 4.
Positions of the weather station masts.
Figure 4.
Positions of the weather station masts.
Figure 5.
Upstream station sensor.
Figure 5.
Upstream station sensor.
Figure 6.
Downstream station sensor.
Figure 6.
Downstream station sensor.
Figure 7.
Pictures of the release configuration for the three experiments.
Figure 7.
Pictures of the release configuration for the three experiments.
Figure 8.
Cartography of sensor positions for all three experiments.
Figure 8.
Cartography of sensor positions for all three experiments.
Figure 9.
Wind changes for the three experiments: upstream (on the left) and downstream (on the right). By convention, the direction indicates where the wind is coming from.
Figure 9.
Wind changes for the three experiments: upstream (on the left) and downstream (on the right). By convention, the direction indicates where the wind is coming from.
Figure 10.
Raw data measured by Bc1 catharometer during experiment n°2.
Figure 10.
Raw data measured by Bc1 catharometer during experiment n°2.
Figure 11.
Average calculation method illustrated on concentrations measured by sensors Bc4, Ot2, and V4 during experiment n°2. Concentrations are in blue lines, average concentrations are represented by dashed lines and green area displays the averaging interval.
Figure 11.
Average calculation method illustrated on concentrations measured by sensors Bc4, Ot2, and V4 during experiment n°2. Concentrations are in blue lines, average concentrations are represented by dashed lines and green area displays the averaging interval.
Figure 12.
Concentrations measured by the three sensor technologies during experiment n°2: t is for the thermometer, c is for the catharometer, and i is for the infrared sensor. Average concentrations during CO2 release are represented by dashed lines for each sensor.
Figure 12.
Concentrations measured by the three sensor technologies during experiment n°2: t is for the thermometer, c is for the catharometer, and i is for the infrared sensor. Average concentrations during CO2 release are represented by dashed lines for each sensor.
Figure 13.
Mean concentrations cartography in log color scale.
Figure 13.
Mean concentrations cartography in log color scale.
Figure 14.
On the left: far-field mean concentrations and standard deviation comparison between experiments 2 and 3. On the right: position of the selected sensors.
Figure 14.
On the left: far-field mean concentrations and standard deviation comparison between experiments 2 and 3. On the right: position of the selected sensors.
Figure 15.
Mean concentration and standard deviation with distance to the release.
Figure 15.
Mean concentration and standard deviation with distance to the release.
Figure 16.
On the left: mean values and standard deviations of concentration in the axial street for experiment n°2. On the right: positions of the selected sensors.
Figure 16.
On the left: mean values and standard deviations of concentration in the axial street for experiment n°2. On the right: positions of the selected sensors.
Figure 17.
Cartography of sensors with an intermittence factor of less than 100% for experiment n°2. The positions of the other sensors are represented by black dots.
Figure 17.
Cartography of sensors with an intermittence factor of less than 100% for experiment n°2. The positions of the other sensors are represented by black dots.
Table 1.
Main sensor characteristics.
Table 1.
Main sensor characteristics.
| | IR1 | IR2 | Catharometer | Tk |
|---|
| Name | Honeywell BW Solo | Class’Air | XEN-5320 ALU | K-Thermocouple |
| Range | 0–50,000 ppm | 0–5000 ppm | 100 ppm–100% | −40 °C–375 °C |
| T90 response time | 30 s | >1 min | <1 s | <1 s |
| Acquisition frequency | 1 s | 1 min | 1 s | 1 s |
Table 2.
Duration and mass flow rate.
Table 2.
Duration and mass flow rate.
| Experiment | Duration | Mean Flowrate |
|---|
| 1 | 09:18:36 to 09:20:43 (127 s) | Not available |
| 2 | 13:03:34 to 13:17:27 (893 s) | 7.0 kg/s |
| 3 | 15:04:19 to 15:19:31 (912 s) | 7.4 kg/s |
Table 3.
Sensor’s nomenclature.
Table 3.
Sensor’s nomenclature.
| Sensors References | Technology | Height (m) |
|---|
| Bc, Jc, and Oc | Catharometers | 1 |
| Bt, Ot, and M | Thermocouple | 1 |
| Bi, V, and Gb | IR1 | 1 |
| Gh | IR1 | 3 |
| R | IR2 | 1 |
Table 4.
Mean weather conditions (downstream station).
Table 4.
Mean weather conditions (downstream station).
| Experiment | 1 | 2 | 3 |
|---|
| Mean wind velocity (m/s) | - | 3.68 | 3.70 |
| Mean wind direction (deg°) | - | 223.9 | 235.6 |
| Temperature (°C) | 7.7 | 16.0 | 16.4 |
| Humidity (%) | 91.4 | 54.0 | 47.2 |
Table 5.
Velocity fluctuations (downstream station).
Table 5.
Velocity fluctuations (downstream station).
| Experiment | 2 | 3 |
|---|
| Mean wind velocity (m/s) | 3.42 | 3.34 |
| σa2 (m2/s2) | 1.3 | 2.6 |
| σb2 (m2/s2) | 1.7 | 2.0 |
Table 6.
Statistical results for Experiment n°2.
Table 6.
Statistical results for Experiment n°2.
| Sensor | (ppm) | (ppm) | | (ppm) | (ppm) | | |
|---|
| Bt4 | 33,069 | 2568 | 0.08 | 33,069 | 2568 | 0.08 | 100 |
| Bi4 | 32,116 | 2286 | 0.07 | 32,116 | 2286 | 0.07 | 100 |
| Bc4 | 31,599 | 6070 | 0.19 | 31,599 | 6070 | 0.19 | 100 |
| Bt2 | 25,158 | 2836 | 0.11 | 25,158 | 2836 | 0.11 | 100 |
| Bc3 | 25,086 | 4543 | 0.18 | 25,086 | 4543 | 0.18 | 100 |
| Bi3 | 24,903 | 1657 | 0.07 | 24,903 | 1657 | 0.07 | 100 |
| Bc2 | 22,388 | 3912 | 0.17 | 22,388 | 3912 | 0.17 | 100 |
| Bt1 | 21,085 | 4243 | 0.20 | 21,085 | 4243 | 0.20 | 100 |
| Bc1 | 19,911 | 3591 | 0.18 | 19,911 | 3591 | 0.18 | 100 |
| Oc2 | 18,073 | 3127 | 0.17 | 18,073 | 3127 | 0.17 | 100 |
| Ot2 | 17,796 | 3245 | 0.18 | 17,796 | 3245 | 0.18 | 100 |
| Bi2 | 17,753 | 1255 | 0.07 | 17,753 | 1255 | 0.07 | 100 |
| Oc3 | 16,238 | 2955 | 0.18 | 16,238 | 2955 | 0.18 | 100 |
| Oc4 | 15,660 | 2986 | 0.19 | 15,660 | 2986 | 0.19 | 100 |
| Bi1 | 13,915 | 1304 | 0.09 | 13,915 | 1304 | 0.09 | 100 |
| Ot3 | 13,596 | 3156 | 0.23 | 13,596 | 3156 | 0.23 | 100 |
| Ot4 | 13,101 | 3685 | 0.28 | 13,101 | 3685 | 0.28 | 100 |
| Jc2 | 11,129 | 4289 | 0.39 | 11,129 | 4289 | 0.39 | 100 |
| Oc1 | 10,954 | 6578 | 0.60 | 10,954 | 6578 | 0.60 | 100 |
| Gb2 | 5563 | 1437 | 0.26 | 5563 | 1437 | 0.26 | 100 |
| Gh2 | 4141 | 1127 | 0.27 | 4141 | 1127 | 0.27 | 100 |
| M3 | 2536 | 1014 | 0.40 | 2536 | 1014 | 0.40 | 100 |
| Gb3 | 1962 | 810 | 0.41 | 1962 | 810 | 0.41 | 100 |
| Gh3 | 1833 | 661 | 0.36 | 1833 | 661 | 0.36 | 100 |
| Gb4 | 1498 | 1054 | 0.70 | 1498 | 1054 | 0.70 | 100 |
| Bi5 | 1482 | 1129 | 0.76 | 1482 | 1129 | 0.76 | 100 |
| Gh4 | 1322 | 794 | 0.60 | 1322 | 794 | 0.60 | 100 |
| R5 | 676 | 383 | 0.57 | 676 | 383 | 0.57 | 100 |
| R3 | 643 | 448 | 0.70 | 643 | 448 | 0.70 | 100 |
| Gb1 | 343 | 189 | 0.55 | 343 | 189 | 0.55 | 100 |
| Gh1 | 339 | 158 | 0.46 | 339 | 158 | 0.46 | 100 |
| R6 | 338 | 165 | 0.49 | 338 | 165 | 0.49 | 100 |
| V3 | 16,086 | 5371 | 0.34 | 16,140 | 5443 | 0.33 | 99.7 |
| Ot1 | 7471 | 5173 | 0.70 | 7572 | 5211 | 0.68 | 98.7 |
| M2 | 712 | 410 | 0.59 | 725 | 418 | 0.57 | 98.1 |
| V4 | 7138 | 2987 | 0.44 | 7333 | 3176 | 0.41 | 97.3 |
| Ot5 | 3957 | 3568 | 0.92 | 4341 | 3623 | 0.82 | 91.2 |
| R1 | 258 | 363 | 1.38 | 287 | 355 | 1.27 | 90.0 |
| R2 | 95 | 69 | 0.77 | 105 | 73 | 0.66 | 90.0 |
| V5 | 1633 | 1054 | 0.70 | 1832 | 1147 | 0.58 | 89.1 |
| V1 | 2878 | 2511 | 0.90 | 3235 | 2576 | 0.78 | 89.0 |
| Bt5 | 2314 | 2454 | 1.07 | 2806 | 2471 | 0.87 | 82.5 |
| R4 | 39 | 52 | 1.28 | 49 | 50 | 1.06 | 80.0 |
| M1 | 738 | 911 | 1.21 | 934 | 894 | 0.98 | 79.0 |
| Jc3 | 1417 | 3896 | 2.41 | 1969 | 3421 | 1.98 | 72.0 |
| Bc5 | 2505 | 5675 | 1.96 | 3917 | 4913 | 1.45 | 63.9 |
| Jc4 | 171 | 328 | 1.68 | 348 | 289 | 0.94 | 49.2 |
| Jc5 | 1015 | 4255 | 2.92 | 2524 | 2969 | 1.69 | 40.2 |
| Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).