Dispersion and Radiation Modelling in ESTE System Using Urban LPM
Abstract
1. Introduction
2. Modelling Approach
2.1. Modelling of Dispersion in Urban Atmosphere
- (i)
- Particles with radii smaller than 5 μm and gases: Gravitational settling can be neglected due to their small particle size; thus, the flow in the mean wind field and the dispersion are dominant and considered in the calculation, while gravitational settling is not.
- (ii)
- Particles with radii between 5 μm and 80 μm: Gravitational settling is non-negligible; thus, it is considered in the flow in the mean wind field and in the dispersion. Gravitational settling is represented by the terminal velocity vt, given as (Stoke’s law):
- (iii)
- Particles with radii greater than 80 μm: The main effect determining the vertical motion is gravitational settling. The gravitational fall begins at zero velocity and is described by the equation (Stoke’s law for a small sphere):
- (i)
- Particles with radii smaller than 80 μm: Each particle has its settling velocity. For particles larger than 5 μm, the settling velocity is approximated using their terminal velocity. For particles smaller than 5 μm, the settling velocity is approximated with a curve expressing the dependence between the particle radius and settling velocity (according to [3]). When the bottom face of the particle’s cell is a ground surface, the particle leaves one part of its activity on the surface. This part of the activity is expressed using the settling velocity as:
- (ii)
- Particles with radii greater than 80 μm: When crossing a horizontal surface, the particle is deposited totally because its contact with the surface is due to gravitational fall. When crossing a vertical surface, it is reflected by a specified probability. Otherwise is deposited totally. The probability of reflection is set to 85% (based on [17]).
2.2. Applied Computational Domain
2.3. Modelling of Urban Conditions Using Empirical Briggs Formulas
2.4. JU2003 Experiment
2.5. Modelling of Radiological Effects
3. Results
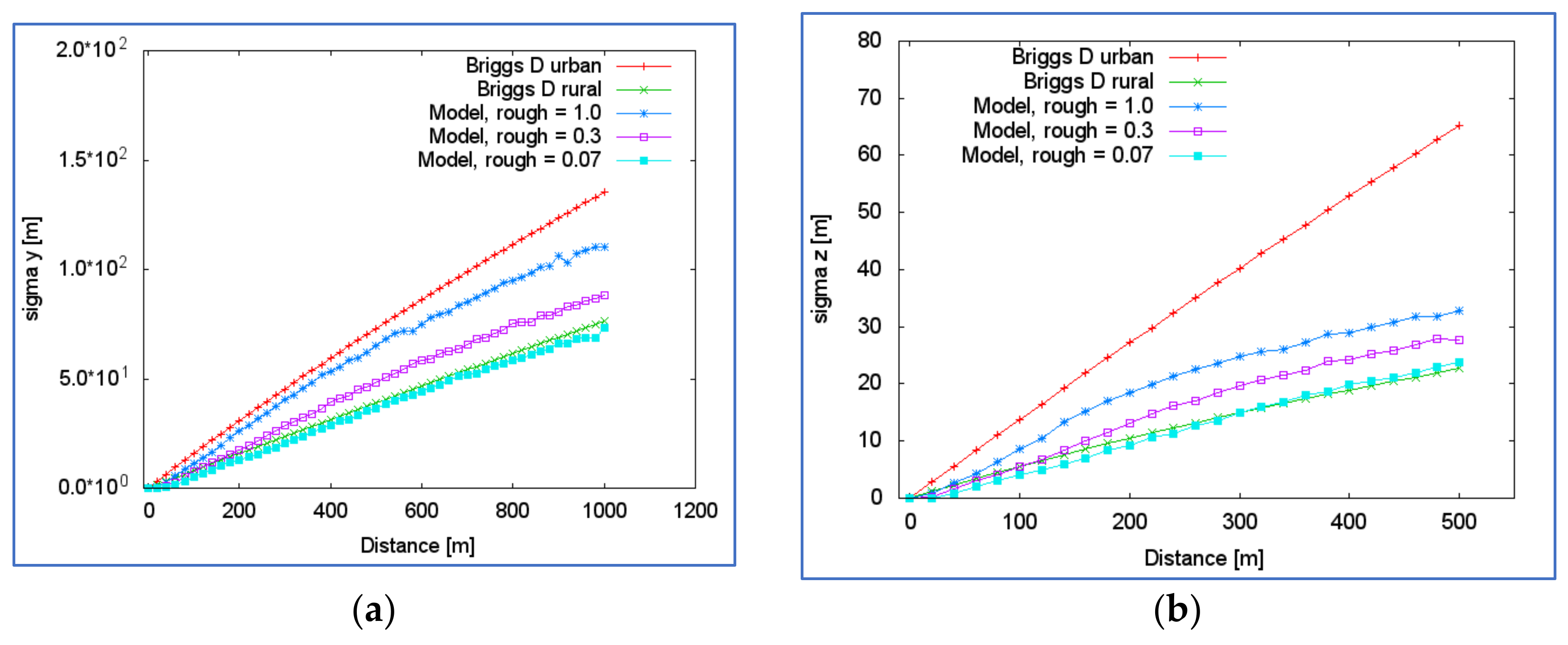
3.1. Model Comparison to Briggs Formulas
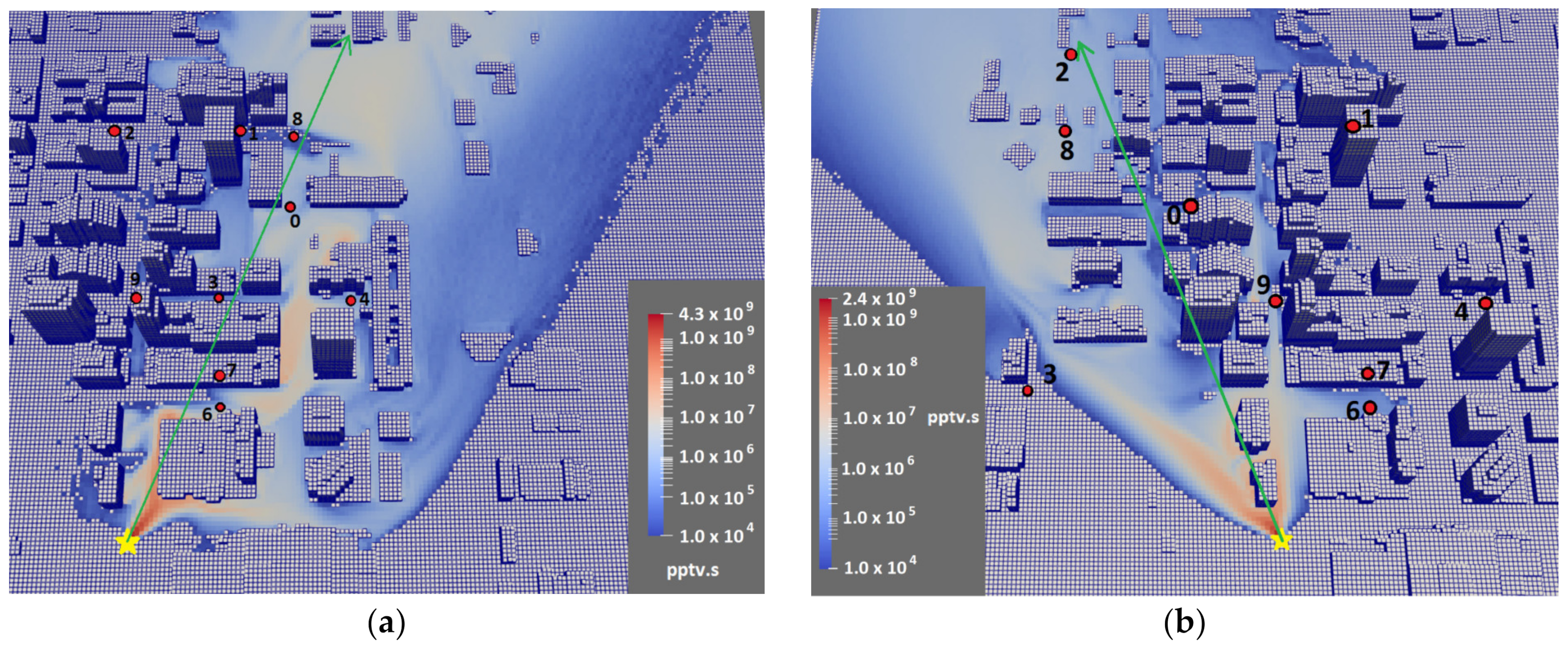
3.2. Model Comparison to JU2003 Experiment
3.3. Computational Time Analysis
4. Conclusions and Summary
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Sykes, R.I.; Parker, S.F.; Henn, D.S.; Cerasoli, C.P.; Santos, L.P. PC-SCIPUFF Version 1.3—Technical Documentation; ARAP Report No. 725; Titan Corporation: San Diego, CA, USA, 2000. [Google Scholar]
- Briggs, G.A. Diffuion Estimation for Small Emissions, ATDL Contribution File No. 79; Atmospheric Turbulence and Diffusion Laboratory: Oak Ridge, TN, USA, 1973. [Google Scholar]
- Hanna, S.R.; Briggs, G.A.; Hosker, R.P. Handbook on Atmospheric Diffusion; Technical Report DOE-TIC-11223; Office of Energy Research, US Department of Energy: Washington, DC, USA, 1982.
- Liptak, L.; Fojcíková, E.; Carny, P. Comparison of the ESTE CBRN Model with the Joint Urban 2003 Experiment. Bound. Layer Meteorol. 2019, 171, 439–464. [Google Scholar] [CrossRef]
- Hernández-Ceballos, M.A.; Hanna, S.; Bianconi, R.; Bellasio, R.; Mazzola, T.; Chang, J.; Andronopoulos, S.; Armand, P.; Benbouta, N.; Čarný, P.; et al. UDINEE: Evaluation of Multiple Models with Data from the JU2003 Puff Releases in Oklahoma City. Part I: Comparison of Observed and Predicted Concentrations. Bound. Layer Meteorol. 2019, 171, 323–349. [Google Scholar] [CrossRef]
- Hernández-Ceballos, M.A.; Hanna, S.; Bianconi, R.; Bellasio, R.; Chang, J.; Mazzola, T.; Andronopoulos, S.; Armand, P.; Benbouta, N.; Čarný, P.; et al. UDINEE: Evaluation of Multiple Models with Data from the JU2003 Puff Releases in Oklahoma City. Part II: Simulation of Puff Parameters. Bound. Layer Meteorol. 2019, 171, 351–376. [Google Scholar] [CrossRef]
- Rodean, H.C. Stochastic Lagrangian Models of Turbulent Diffusion; The American Meteorological Society: Boston, MA, USA, 1996; 82p. [Google Scholar]
- Williams, M.D.; Brown, M.J.; Boswell, D.; Singh, B.; Pardyjak, E.M. Testing of the QUIC-Plume Model with Wind-Tunnel Measurements for a High-Rise Building. In Proceedings of the 26th Agricultural and Forest Meteorology/13th Air Pollution/5th Urban Environment/16th Biometeorology and Aerobiology, January 2004. Available online: https://ams.confex.com/ams/AFAPURBBIO/webprogram/Paper78636.html (accessed on 2 May 2023).
- Benbouta, N. Canadian Urban Flow and Dispersion Model (CUDM): Developing Canadian Capabilities for CBRN Hazard Dispersion in Urban Areas; CMOE Dorval TR 2007-001 Technical Report; Environment Canada: Toronto, ON, Canada, 2007. [Google Scholar]
- Hogue, R.; Benbouta, N.; Mailhot, J.; Belair, S.; Lemonsu, A.; Yee, E.; Lien, F.-S.; Wilson, J.D. The development of a prototype of Canadian urban flow and dispersion modeling system. In Proceedings of the AMS 89 Annual Conference, Joint Session 19 Urban Transport and Dispersion Modeling, Phoenix, AZ, USA, 14 January 2009. [Google Scholar]
- Oldrini, O.; Armand, P.; Duchenne, C.; Olry, C.; Moussafir, J.; Tinarelli, G. Description and preliminary validation of the PMSS fast response parallel atmospheric flow and dispersion solver in complex built-up areas. Environ. Fluid Mech. 2017, 17, 997–1014. [Google Scholar] [CrossRef]
- Oldrini, O.; Armand, P.; Duchenne, C.; Perdriel, S. Parallelization Performances of PMSS Flow and Dispersion Modeling System over a Huge Urban Area. Atmosphere 2019, 10, 404. [Google Scholar] [CrossRef]
- Röckle, R. Bestimmung der Strömungsverhältnisse im Bereich komplexer Bebauungsstrukturen. Ph.D. Thesis, Techn. Hochschule Darmstadt, Darmstadt, Germany, 1990. [Google Scholar]
- Ek, N.; Benbouta, N.; Bourgouin, P.; Gauthier, J.-P.; Gauthier, N.; Hogue, R.; Holly, M.; Trudel, S.; Zaganescu, C. The Canadian urban dispersion modelling (CUDM) system: An operational prototype. In Proceedings of the 34th AMOP Technical Seminar on Environmental Contamination and Response, Banff, AB, Canada, 4–6 October 2011; pp. 603–623. [Google Scholar]
- Thomson, D.J. Criteria for the selection of stochastic models of particle trajectories in turbulent flows. J. Fluid Mech. 1987, 180, 529–556. [Google Scholar] [CrossRef]
- Patankar, S.V.; Spalding, D.B. A calculation procedure for heat, mass and momentum transfer in three-dimensional parabolic flows. Int. J. Heat Mass Transf. 1972, 15, 1787–1806. [Google Scholar] [CrossRef]
- Roed, J.; Andersson, K.G. Early Measurements in Scandinavia following the Chernobyl Accident (IAEA-TECDOC—1131); International Atomic Energy Agency (IAEA): Vienna, Austria, 2000. [Google Scholar]
- Lipták, Ľ.; Fojcíková, E.; Krpelanová, M.; Fabová, V.; Čarný, P. The ESTE Decision Support System for Nuclear and Radiological Emergencies: Atmospheric Dispersion Models. Atmosphere 2021, 12, 204. [Google Scholar] [CrossRef]
- ParaView—Open Source Post-Processing Visualization Engine. Available online: https://www.paraview.org/ (accessed on 25 June 2023).
- Clawson, K.L.; Carter, R.G.; Lacroix, D.J.; Biltoft, C.A.; Hukari, N.F.; Johnson, R.C.; Rich, J.D. Joint Urban 2003 (JU03) SF6 Atmospheric Tracer Field Tests; NOAA Technical Memorandum OARARL-254; Air Resources Lab.: College Park, MD, USA, 2005. [Google Scholar]
- Allwine, K.J.; Leach, M.J.; Stockham, L.W.; Shinn, J.S.; Hosker, R.P.; Bowers, J.F.; Pace, J.C. Overview of Joint Urban 2003—An atmospheric Dispersion Study in Oklahoma City. Preprints, Symposium on Planning, Nowcasting and Forecasting in the Urban Zone, American Meteorological Society. 2004. Available online: www.researchgate.net (accessed on 23 June 2023).
- Wesson, G.; Katul, G.; Lai, C.-T. Sensible heat flux estimation by flux variance and half-order time derivative methods. Water Resour. Res. 2001, 37, 2333–2343. [Google Scholar] [CrossRef]
- Möller, T.; Trumbore, B. Fast, Minimum Storage Ray-Triangle Intersection. J. Graph. Tools 1997, 2, 21–28. [Google Scholar] [CrossRef]
- Los Alamos National Laboratory. MCNP—A General Monte Carlo N-Particle Transport Code, Version 5, Software Product Produced by Los Alamos National Laboratory. Available online: https://mcnp.lanl.gov/ (accessed on 2 February 2021).

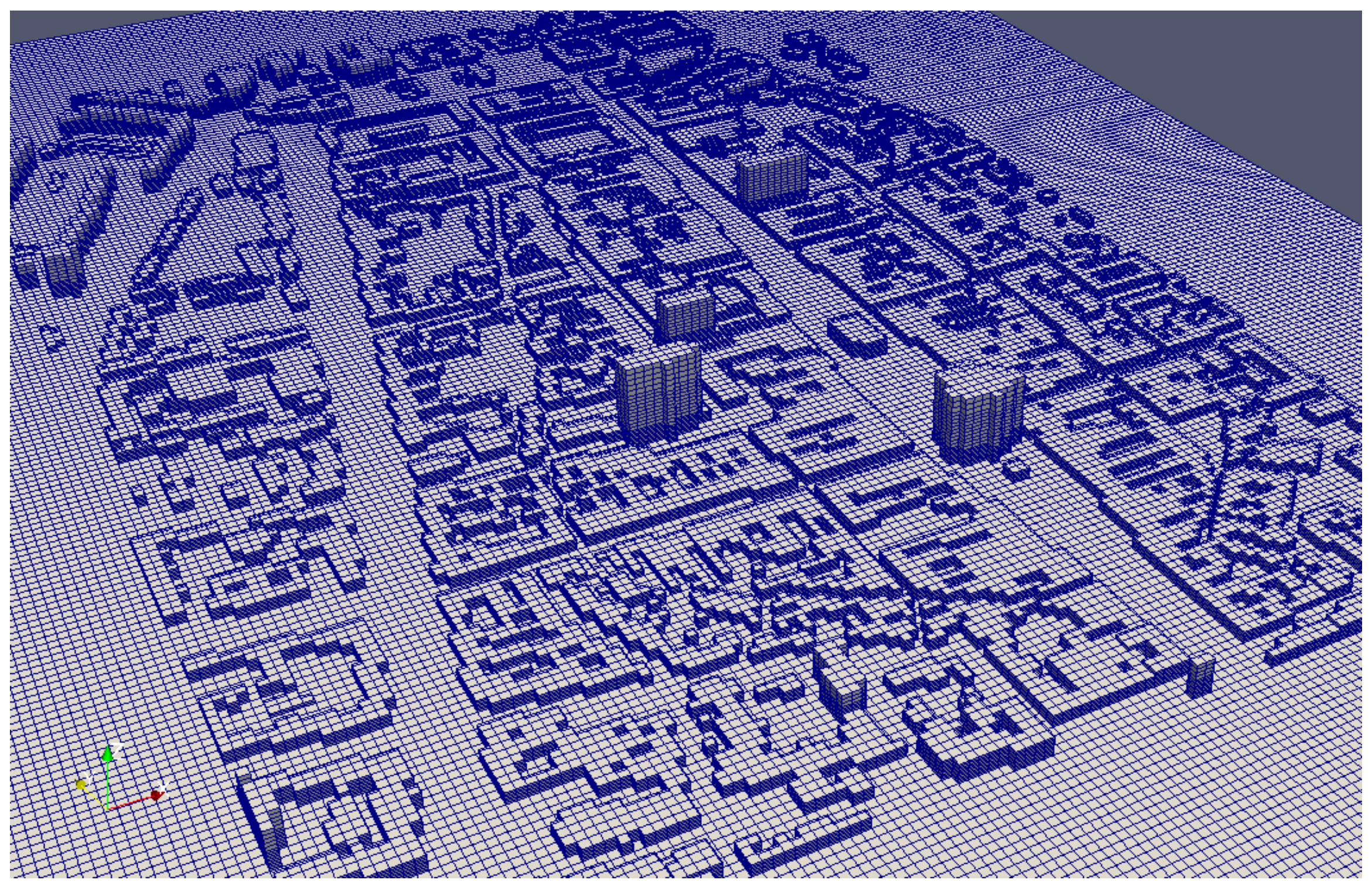


| Parameter | NPP Mochovce | Košice | Oklahoma City |
|---|---|---|---|
| Discretization | 70 × 140 × 39 | 185 × 242 × 39 | 275 × 330 × 46 |
| Total number of cells | 377,474 | 1,684,925 | 4,029,620 |
| Horizontal resolution | 20 m × 20 m | 7 m × 7 m | 5 m × 5 m |
| Maximum height above ground [m] | 1000 m | 189 m | 194 m |
| Height resolution [m] | 4.0 to 50 m | 1.5 to 12 m | 1.0 to 16 m |
| Pasquill Stability | Urban σy (m) | Urban σz (m) | Rural σy (m) | Rural σz (m) |
|---|---|---|---|---|
| A (unstable) | 0.32 × (1 + 0.0004x)−0.5 | 0.24 × (1 + 0.001x)−0.5 | 0.22 × (1 + 0.0001x)−0.5 | 0.20x |
| D (neutral) | 0.16 × (1 + 0.0004x)−0.5 | 0.14 × (1 + 0.0003x)−0.5 | 0.08 × (1 + 0.0001x)−0.5 | 0.06 × (1 + 0.0015x)−0.5 |
| F (stable) | 0.11 × (1 + 0.0004x)−0.5 | 0.08 × (1 + 0.00015x)−0.5 | 0.04 × (1 + 0.0001x)−0.5 | 0.016 × (1 + 0.0003x)−1 |
| Case | Pasquill Stability | Wind Speed [m/s] | Surface Roughness | Monin–Obukhov [m] | Friction Velocity | Interpolated Parameters for y | Interpolated Parameters for z |
|---|---|---|---|---|---|---|---|
| 1 | D | 2.4 | 1.0 | - | 0.4169 | A = 0.147 B = 0.0005 | A = 0.113 B = −0.0018 |
| 2 | D | 4.8 | 1.0 | - | 0.8338 | A = 0.147 B = 0.0005 | A = 0.116 B = −0.0019 |
| 3 | D | 2.4 | 0.3 | - | 0.2736 | A = 0.097 B = 0.0001 | A = 0.066 B = −0.0003 |
| 4 | D | 4.8 | 0.3 | - | 0.5472 | A = 0.095 B = 0.0000 | A = 0.064 B = −0.0001 |
| 5 | D | 2.4 | 0.07 | - | 0.1935 | A = 0.063 B = −0.0006 | A = 0.042 B = 0.0011 |
| 6 | D | 4.8 | 0.07 | - | 0.3870 | A = 0.064 B = −0.0005 | A = 0.041 B = 0.0011 |
| 7 | F | 1.9 | 1.0 | 610 | 0.32 | A = 0.953 B = 0.0009 | A = 0.067 B = −0.0013 |
| 8 | F | 1.9 | 0.07 | 610 | 0.15 | A = 0.059 B = 0.0007 | A = 0.032 B = −0.0001 |
| IOP 5—TGA No. | Puff 1- Concentration [pptv] | Puff 2- Concentration [pptv] | Puff 3- Concentration [pptv] | Mean- Concentration [pptv] | Modeled- Concentration [pptv] |
|---|---|---|---|---|---|
| 0 | 9890 | 5810 | 5800 | 7166 | 1060 +/− 100 |
| 1 | - | - | 663 | 221 | 132 +/− 26 |
| 2 | 13,500 | 4570 | 1870 | 6646 | 5310 +/− 510 |
| 4 | >25,300 | 4380 | 2250 | ≈10,643 | 0 +/− 0 |
| 6 | 48 | 6710 | >23,100 | ≈9952 | 7440 +/− 26 |
| 7 | 0 | 8870 | >24,500 | ≈11,123 | 4320 +/− 1500 |
| 8 | 12,100 | 4290 | 2020 | 6137 | 10,300 +/− 800 |
| 9 | 9210 | >12,100 | >12,200 | ≈>11,170 | 110,700 +/− 6400 |
| IOP 3—TGA No. | Puff 1- Concentration [pptv] | Puff 2- Concentration [pptv] | Puff 3- Concentration [pptv] | Mean- Concentration [pptv] | Modeled- Concentration [pptv] |
| 0 | 12,600 | 12,300 | 19,600 | 14,833 | 12,700 +/− 2100 |
| 1 | 3130 | 0 | 4180 | 2437 | 0 +/− 20 |
| 2 | 0 | 0 | 202 | 67 | 0 +/− 0 |
| 3 | 10,100 | 1710 | 12,500 | 8100 | 2920 +/− 100 |
| 4 | >13,000 | >12,800 | >25,800 | >12,800 | 45,700 +/− 5300 |
| 6 | >11,900 | 21,600 | >23,700 | ≈21,600 | 35,900 +/− 200 |
| 7 | >12,200 | >12,100 | >11,900 | >11,900 | 390,000 +/− 98,000 |
| 8 | 4808 | 163 | 10,700 | 5224 | 530 +/− 0 |
| 9 | 0 | 329 | 11,700 | 4010 | 530 +/− 200 |
| Oklahoma City | City of Košice | NPP Mochovce | |
|---|---|---|---|
| Total cell number | 4,029,620 | 1,684,925 | 377,474 |
| Number of particles | Time [min] CPU/GPGPU | Time [min] CPU/GPGPU | Time [min] CPU/GPGPU |
| 10,000 | 0.9/0.1 | 0.4/0.1 | 0.1/0.1 |
| 100,000 | 1.4/0.3 | 0.8/0.2 | 0.5/0.2 |
| 250,000 | 2.2/0.4 | 1.6/0.4 | 1.3/0.4 |
| 500,000 | 3.9/0.7 | 2.9/0.7 | 2.4/0.7 |
| 1,000,000 | 6.8/1.3 | 5.8/1.2 | 5.0/1.2 |
| GPGPU Time [s] | CPU Time [s] | |
|---|---|---|
| Inhalation | <1 | <1 |
| Groundshine | 3 | 4 |
| Cloudshine | 11 | 13 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lipták, Ľ.; Čarný, P.; Marčišovský, M.; Marčišovská, M.; Chylý, M.; Fojciková, E. Dispersion and Radiation Modelling in ESTE System Using Urban LPM. Atmosphere 2023, 14, 1077. https://doi.org/10.3390/atmos14071077
Lipták Ľ, Čarný P, Marčišovský M, Marčišovská M, Chylý M, Fojciková E. Dispersion and Radiation Modelling in ESTE System Using Urban LPM. Atmosphere. 2023; 14(7):1077. https://doi.org/10.3390/atmos14071077
Chicago/Turabian StyleLipták, Ľudovít, Peter Čarný, Michal Marčišovský, Mária Marčišovská, Miroslav Chylý, and Eva Fojciková. 2023. "Dispersion and Radiation Modelling in ESTE System Using Urban LPM" Atmosphere 14, no. 7: 1077. https://doi.org/10.3390/atmos14071077
APA StyleLipták, Ľ., Čarný, P., Marčišovský, M., Marčišovská, M., Chylý, M., & Fojciková, E. (2023). Dispersion and Radiation Modelling in ESTE System Using Urban LPM. Atmosphere, 14(7), 1077. https://doi.org/10.3390/atmos14071077





