Analysis of Flow Structures and Global Parameters across a Heated Square Cylinder in Forced and Mixed Convection
Abstract
1. Introduction
- (i)
- Re (Reynolds number) = ,
- (ii)
- Ri (Richardson number) = ,
- (iii)
- Pr (Prandtl number) = ,
- (iv)
- α = Free-stream orientation (with respect to gravity) and
- (v)
- ϕ = Bluff-body orientation (with respect to the X-axis).
- Combined effects of fluid inertia, buoyancy forces and free-stream orientations on Strouhal number and on global parameters are not investigated in detail for mixed convective flow past a square cylinder.
- The associated effects of Reynolds number, buoyancy forces and free-stream orientations on surface pressure, surface vorticity, and local or time mean heat transfer have not been studied in detail in previous studies.
- The characteristic maps of mean or steady drag coefficient and Nusselt number are not produced to date for a wide range of Re, Ri and α in mixed convective flow past an isolated canonical bluff body of square shape.
2. Mathematical Formulation
- Length scale ≡ ‘’ = the edge of square cylinder.
- Velocity scale ≡ ‘’ = the free-stream velocity magnitude.
- Time scale ≡ ‘’ = the residence ≡ time spent in the vicinity of the cylinder by the fluid particles.
v = V/U∞ and
τ = tU∞/d.
Boundary Conditions
3. Numerical Scheme and Various Aspects
3.1. Grid Structure
3.2. Numerical Scheme
3.3. Sensitivity to Numerical Parameters
- Lift coefficient, CL = ,
- Drag coefficient, CD = ,
- Moment coefficient
- Nusselt number, Nu = and
- Strouhal number, St =
3.4. Validation Studies
4. Results and Discussion
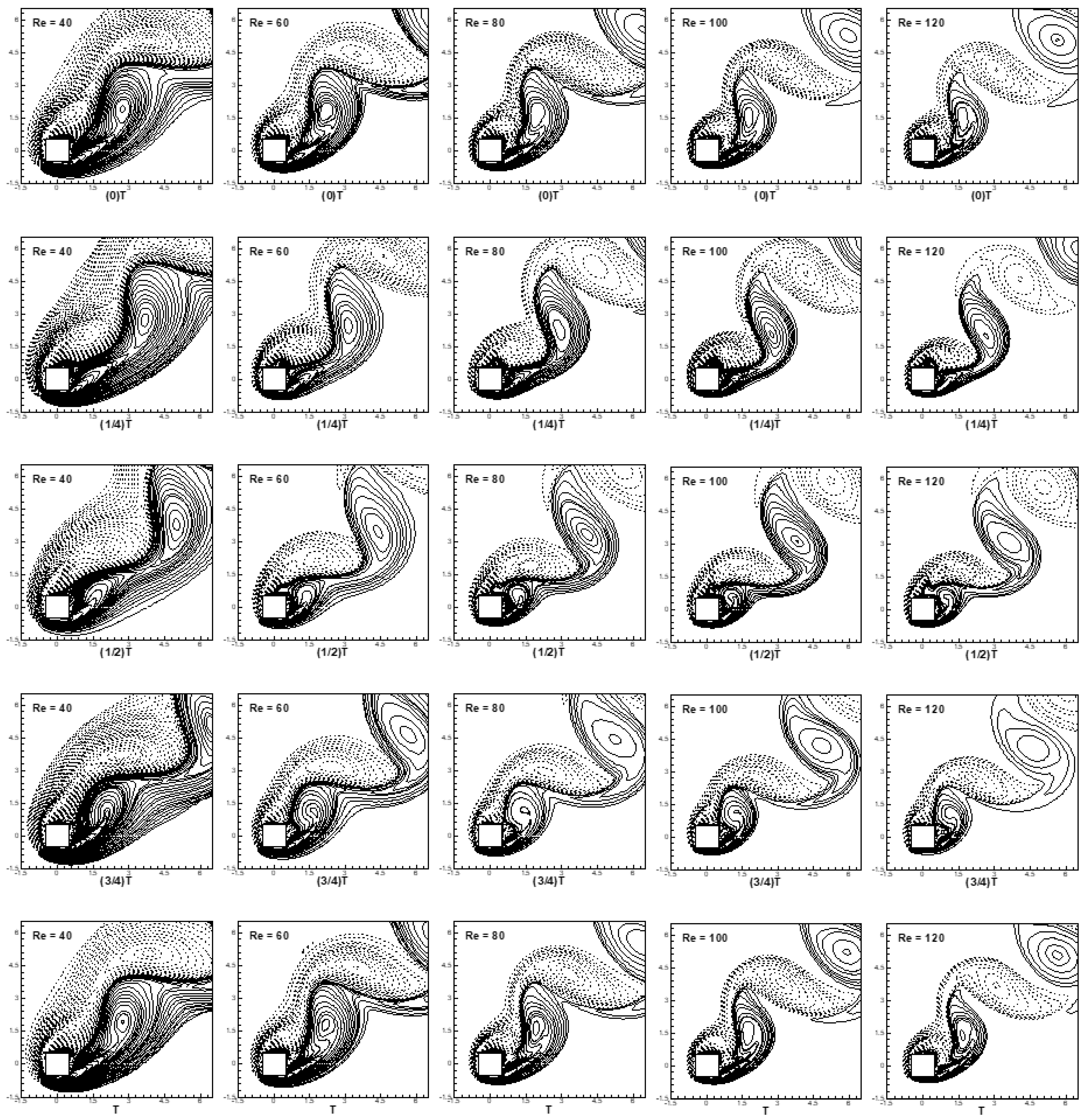
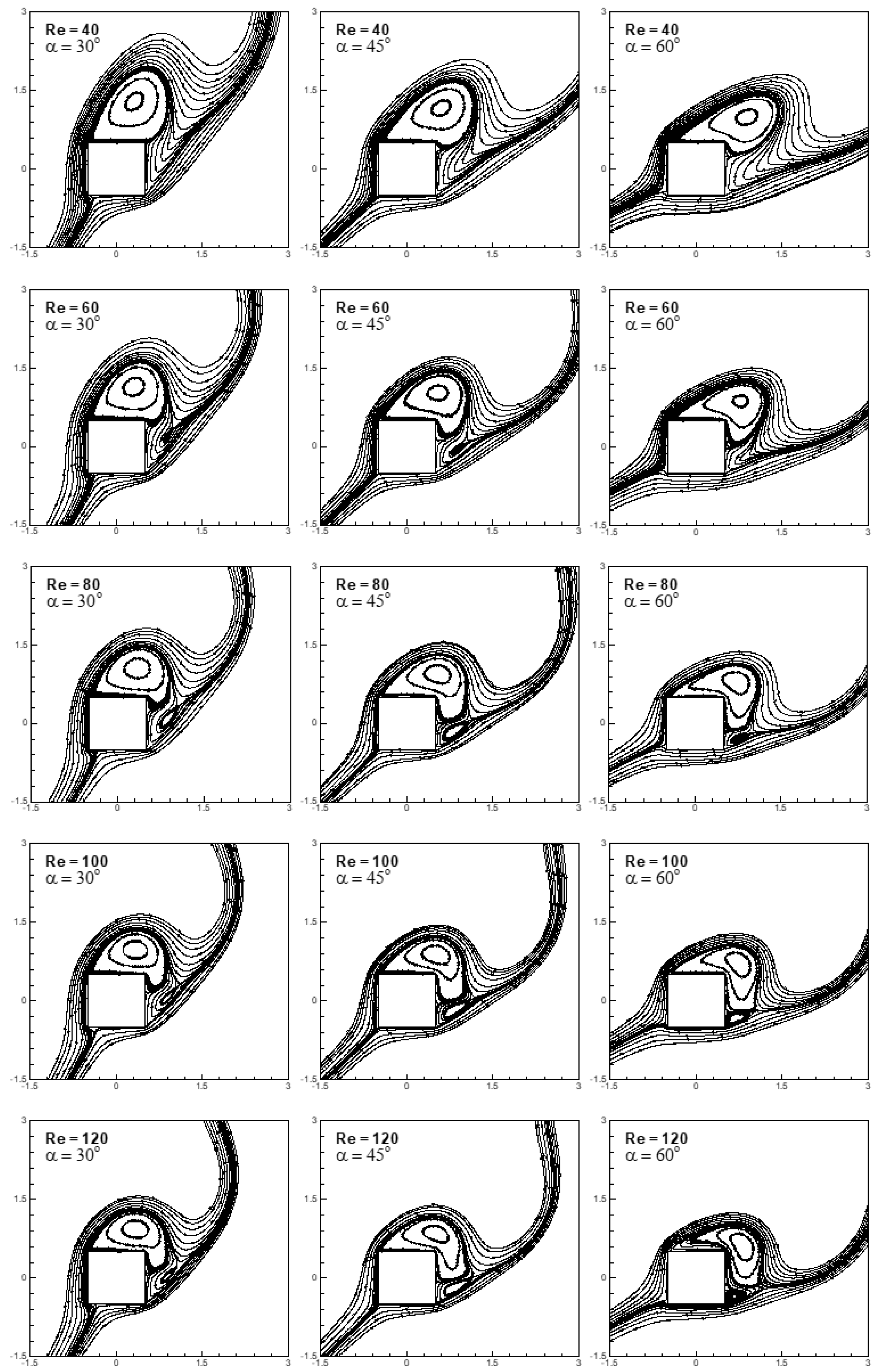
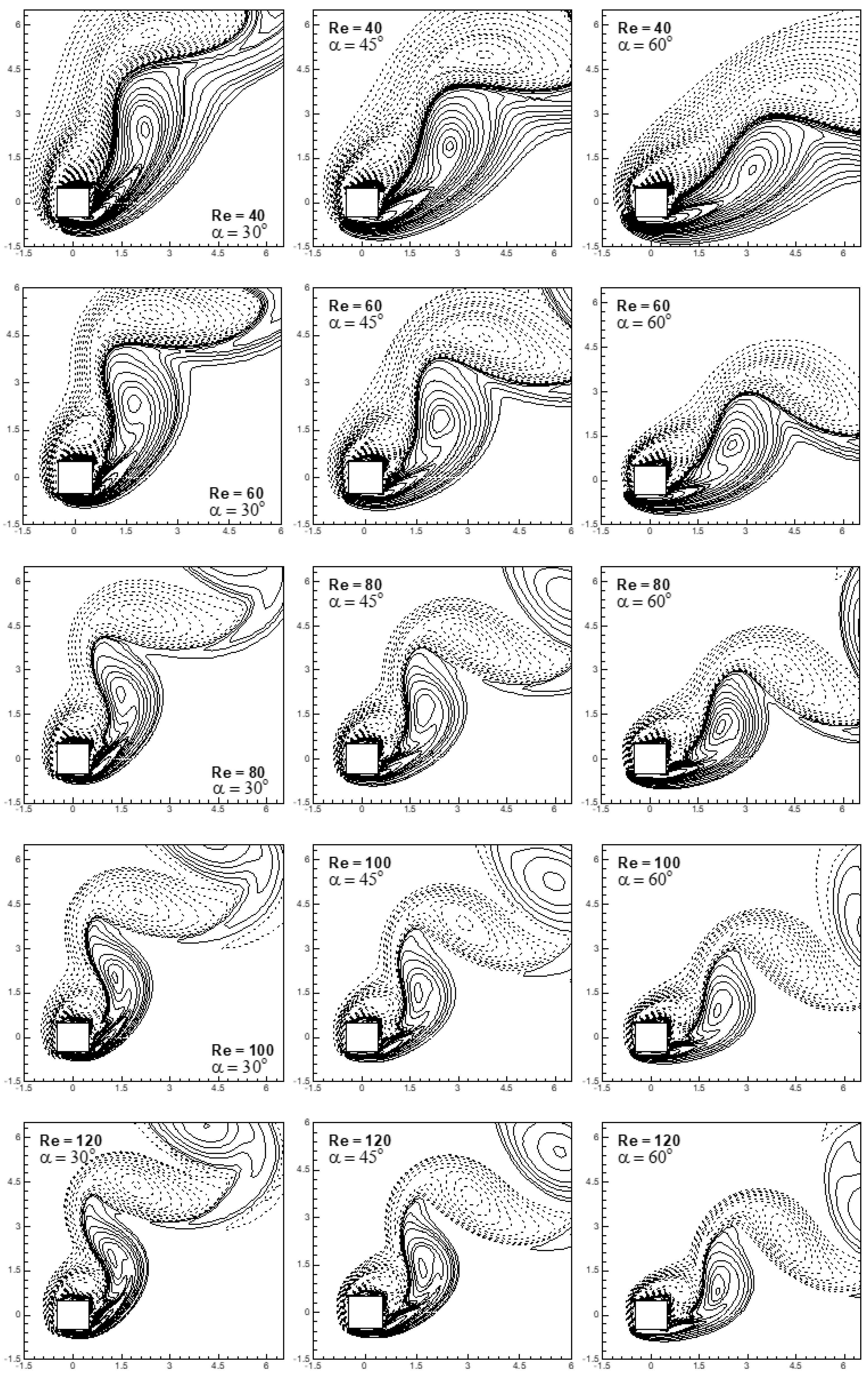
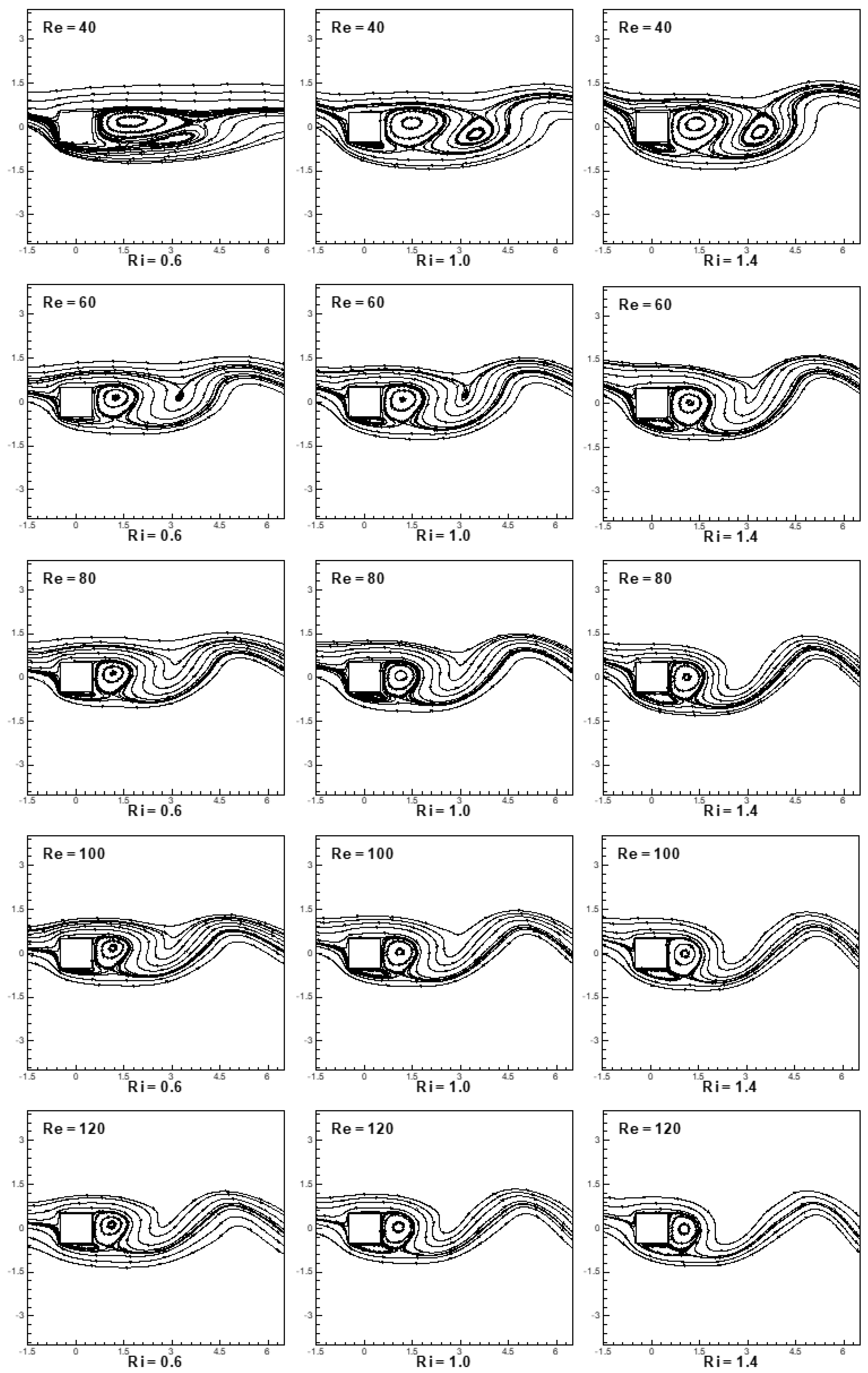
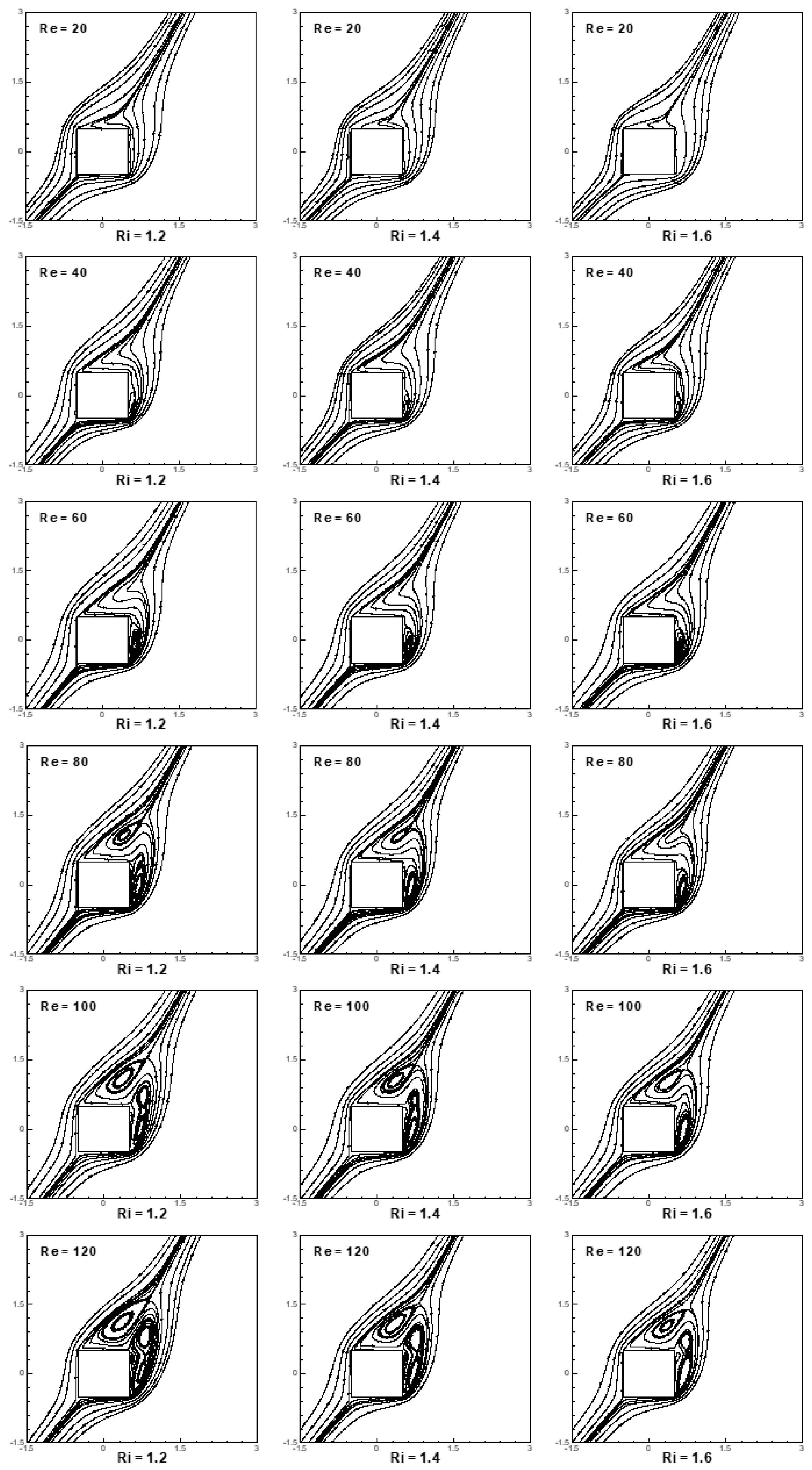
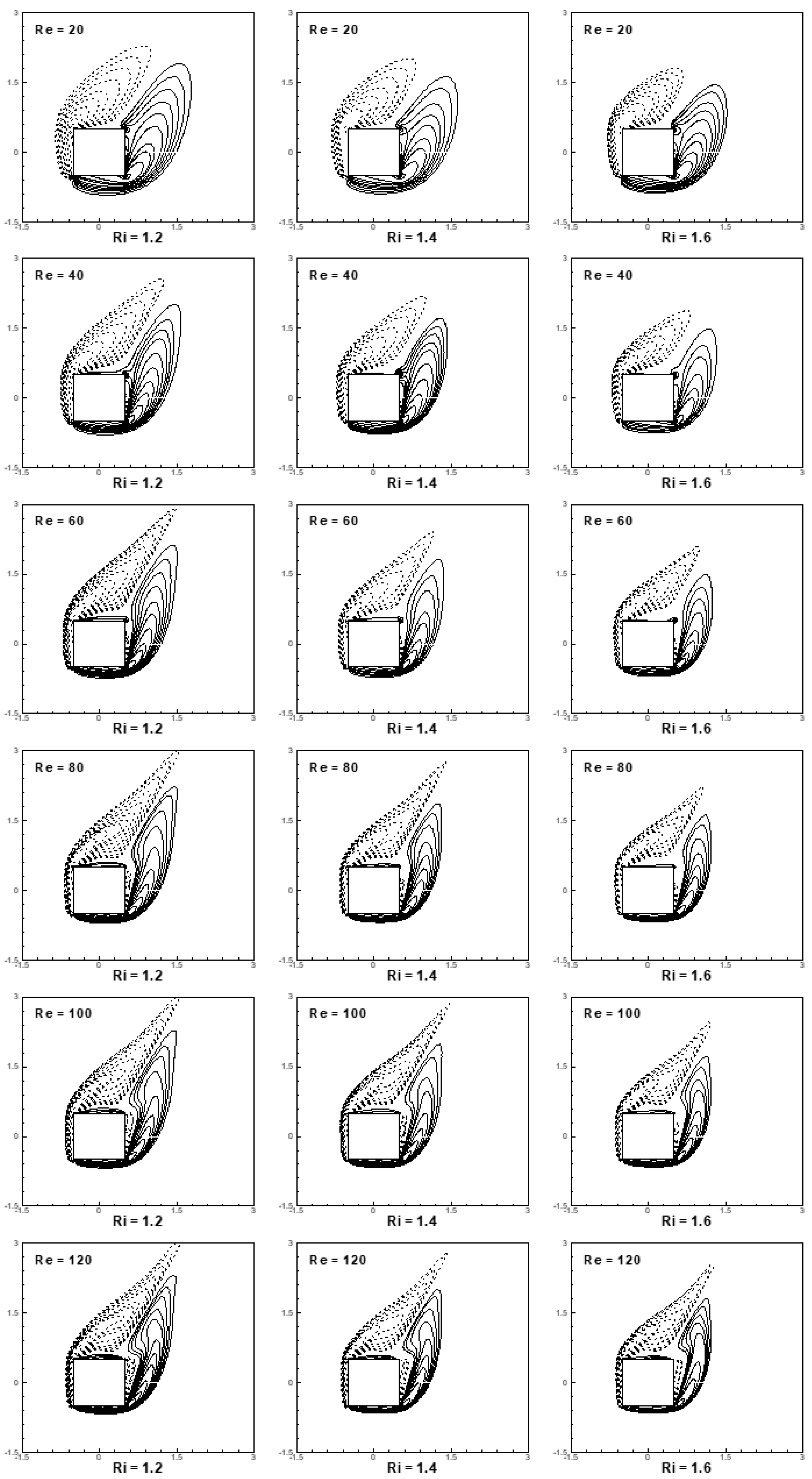
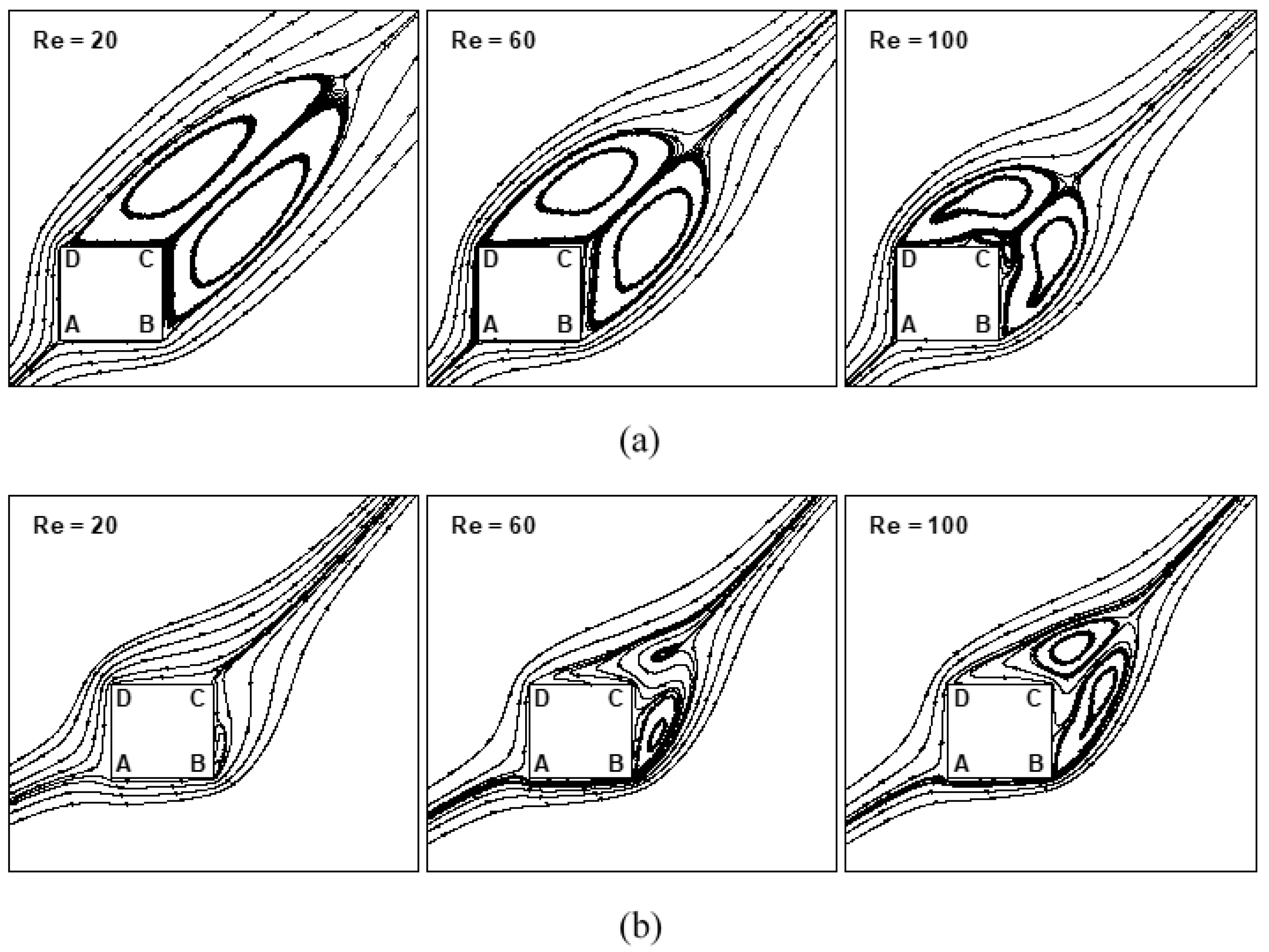
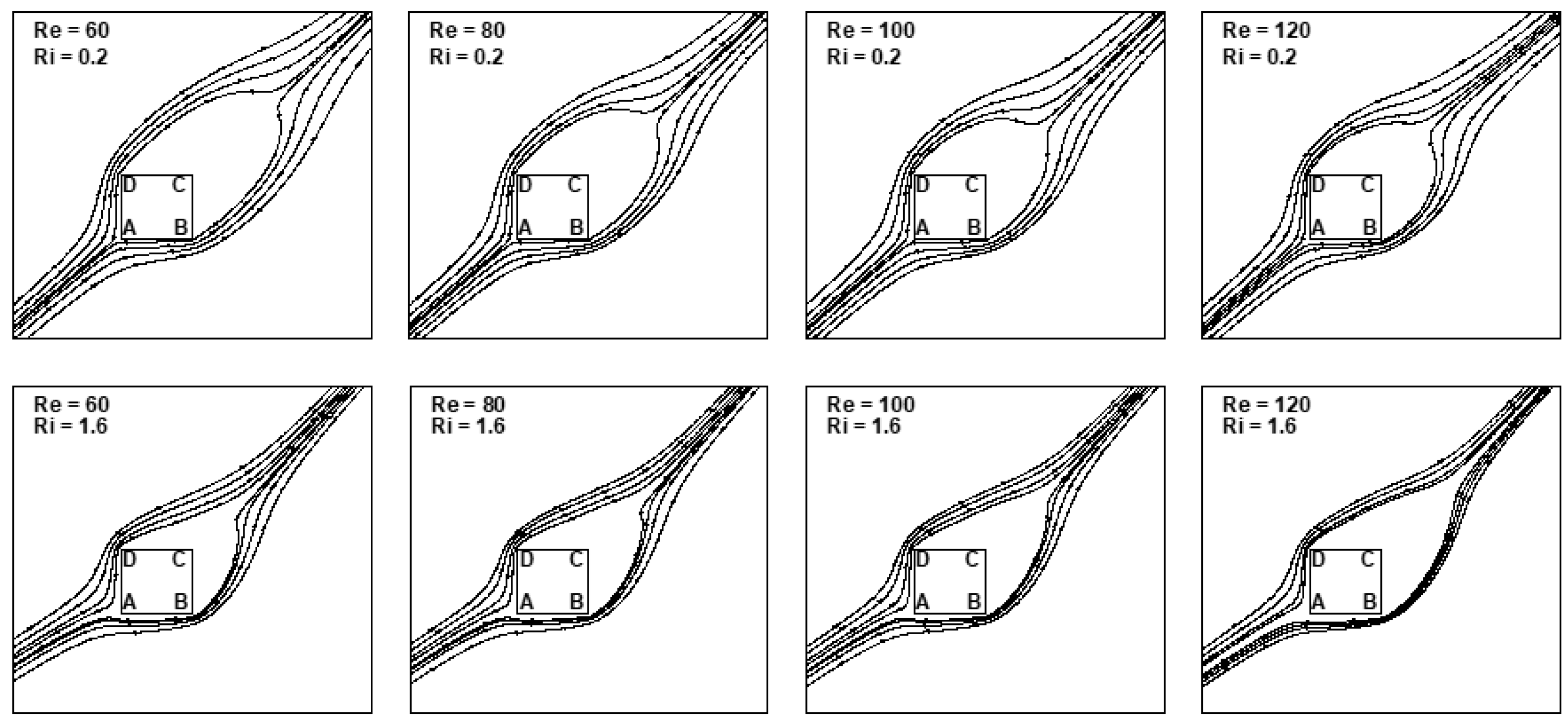
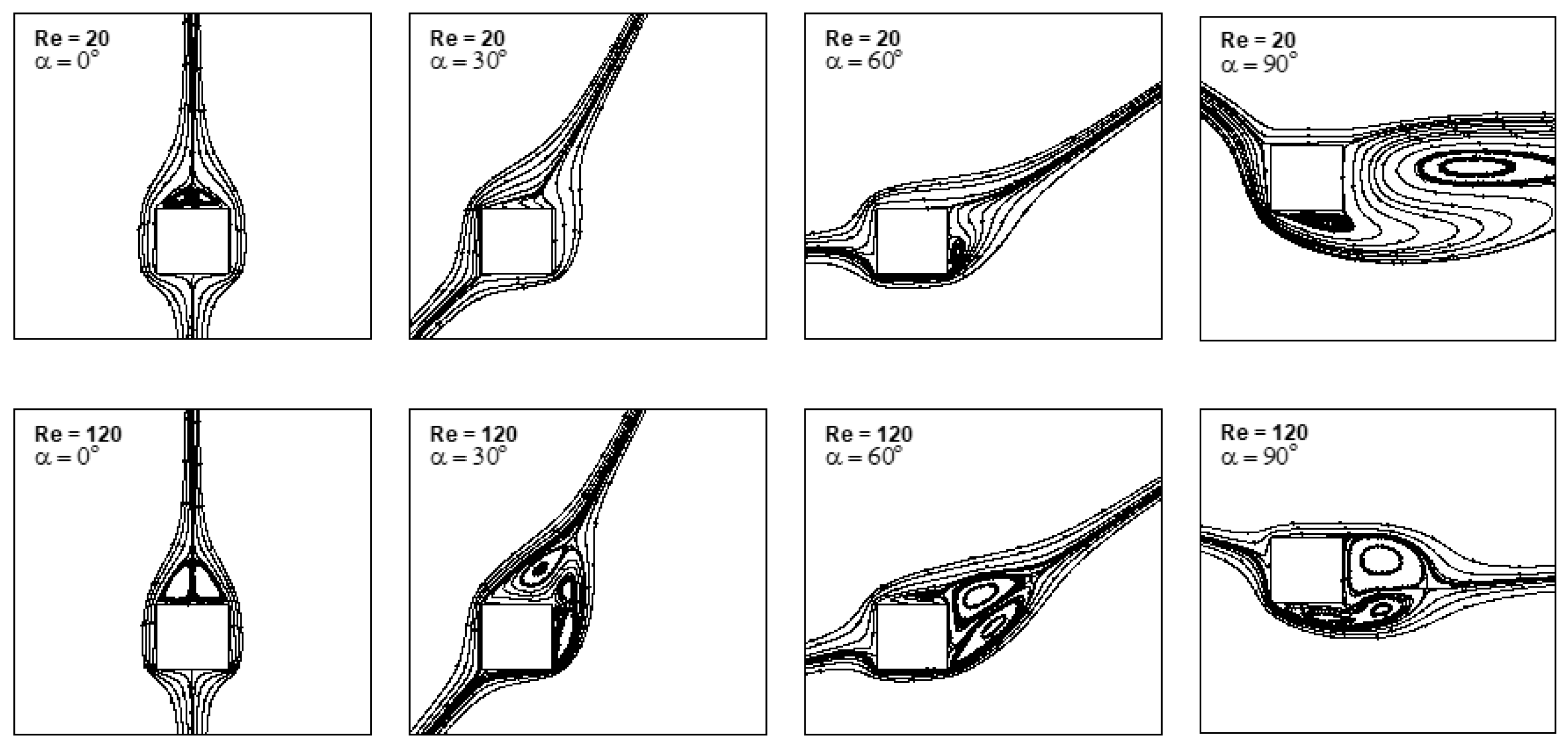
4.1. Streamline Patterns and Contours of Vorticity
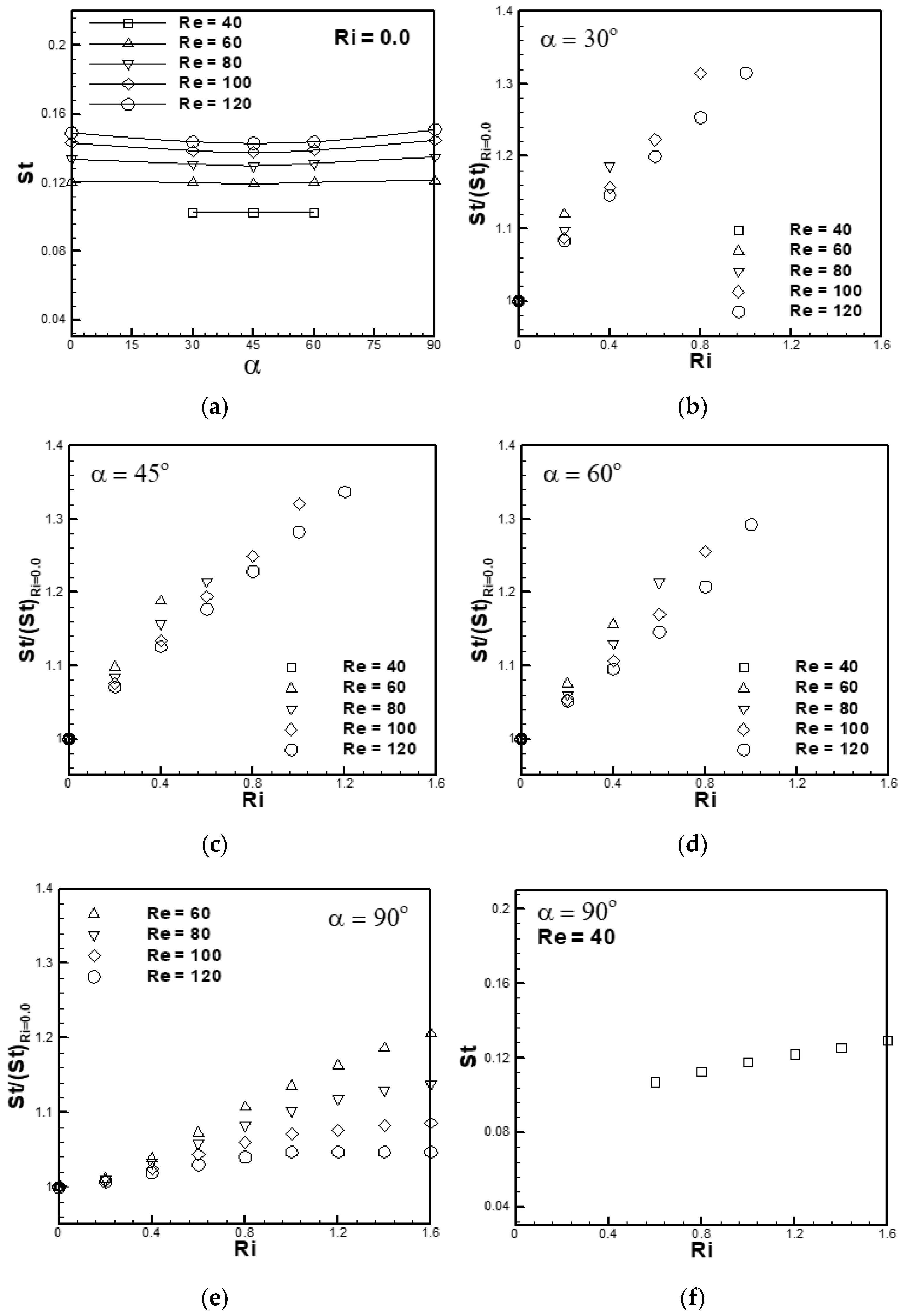
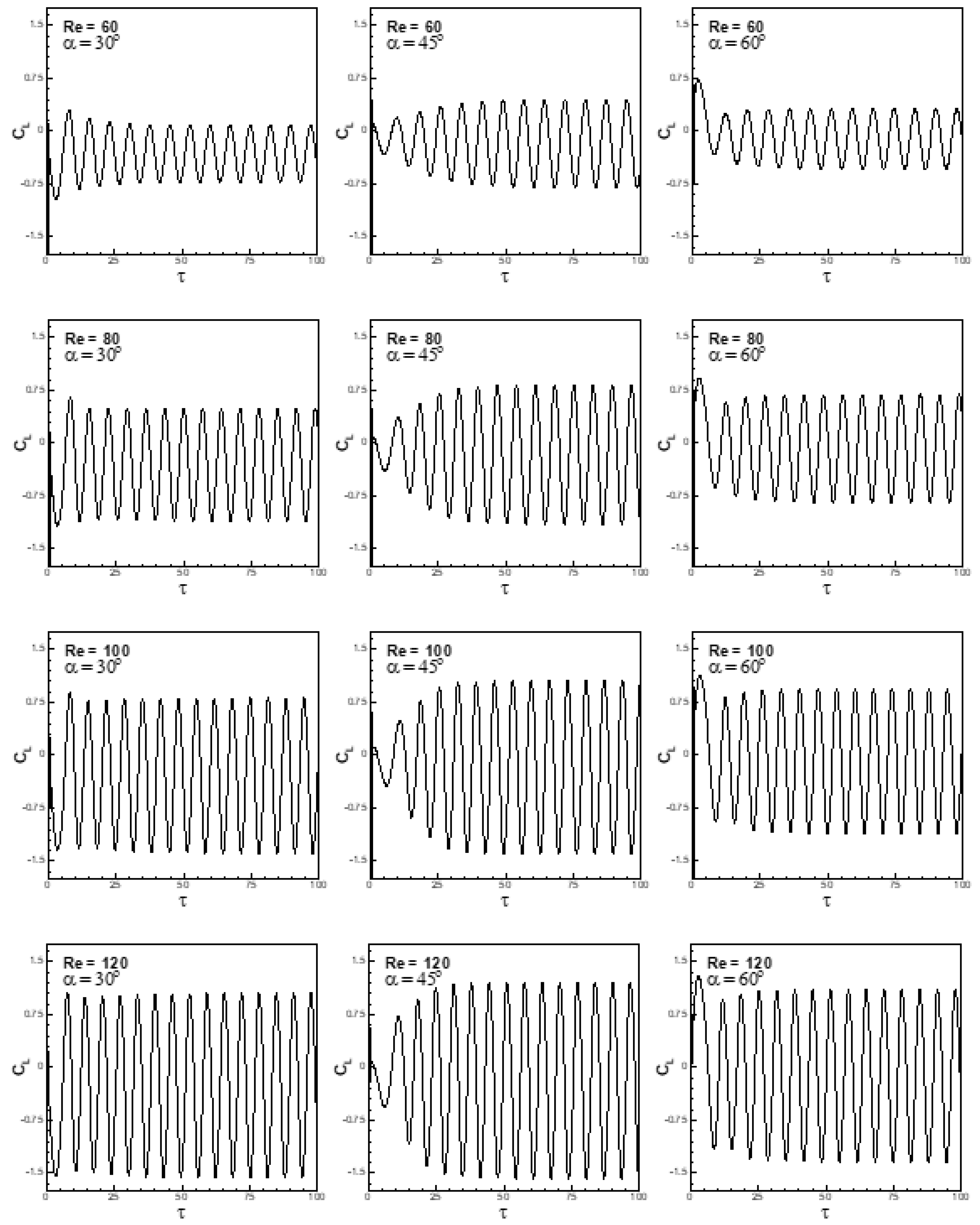
4.2. Characteristics of the Strouhal Number
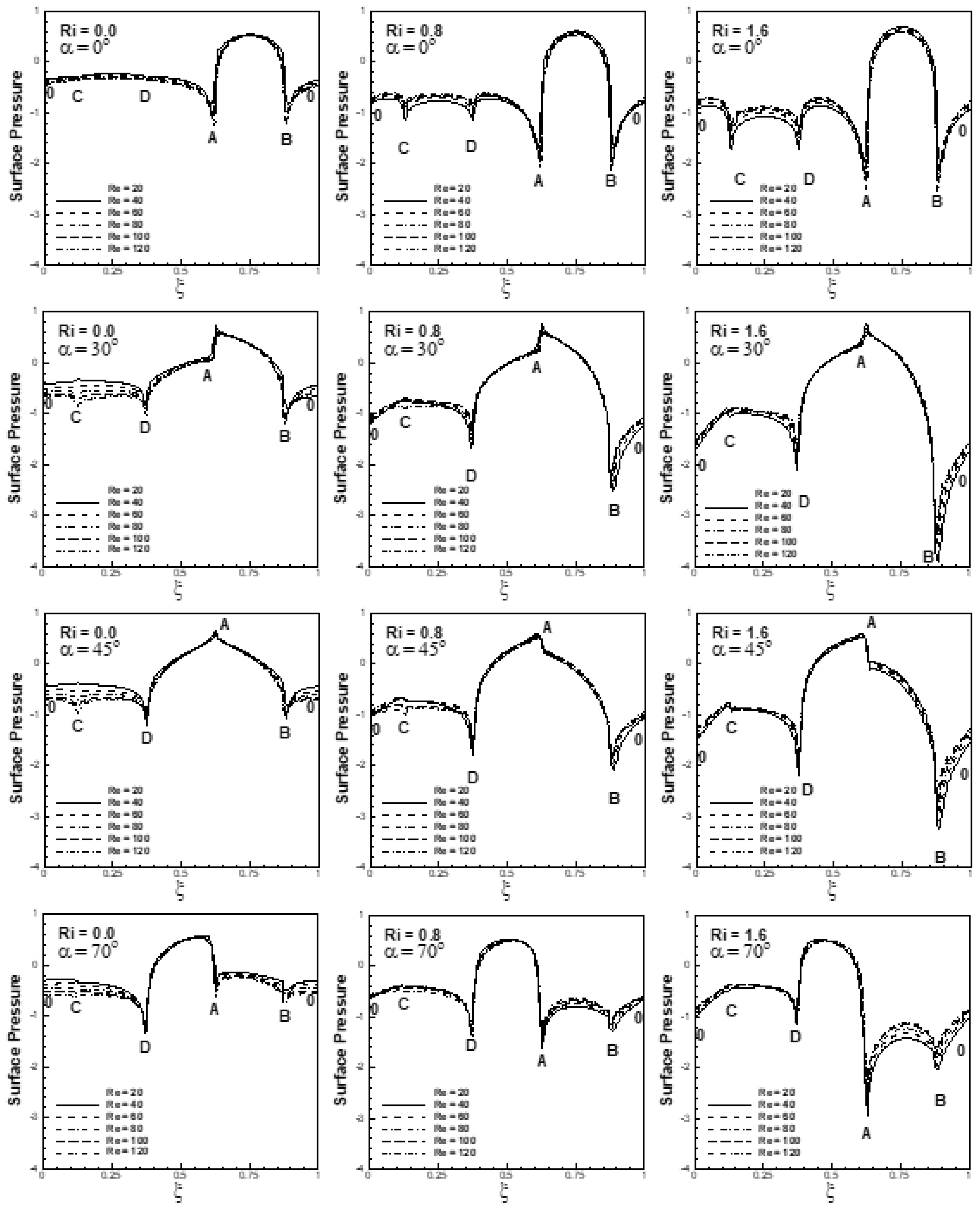
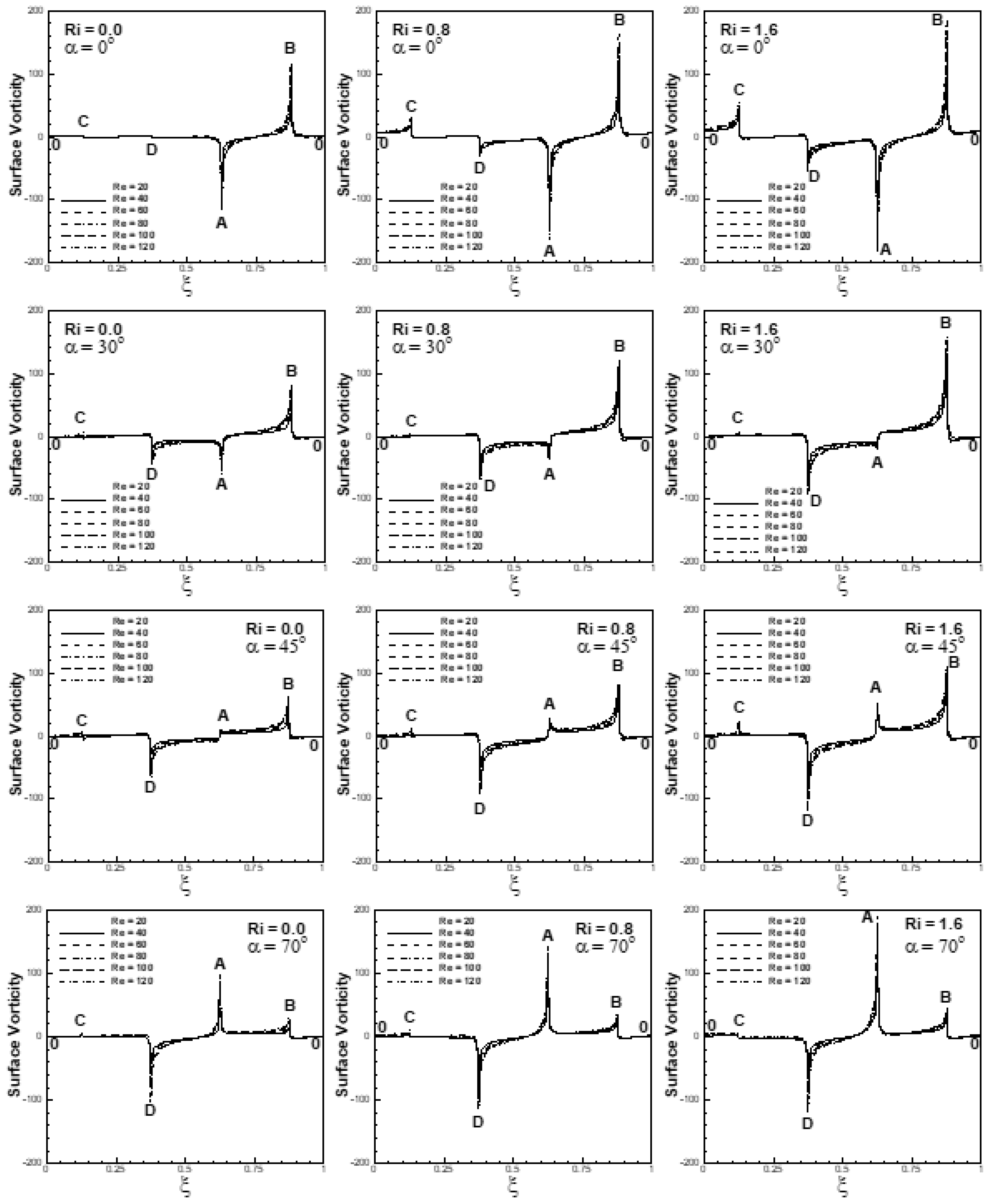
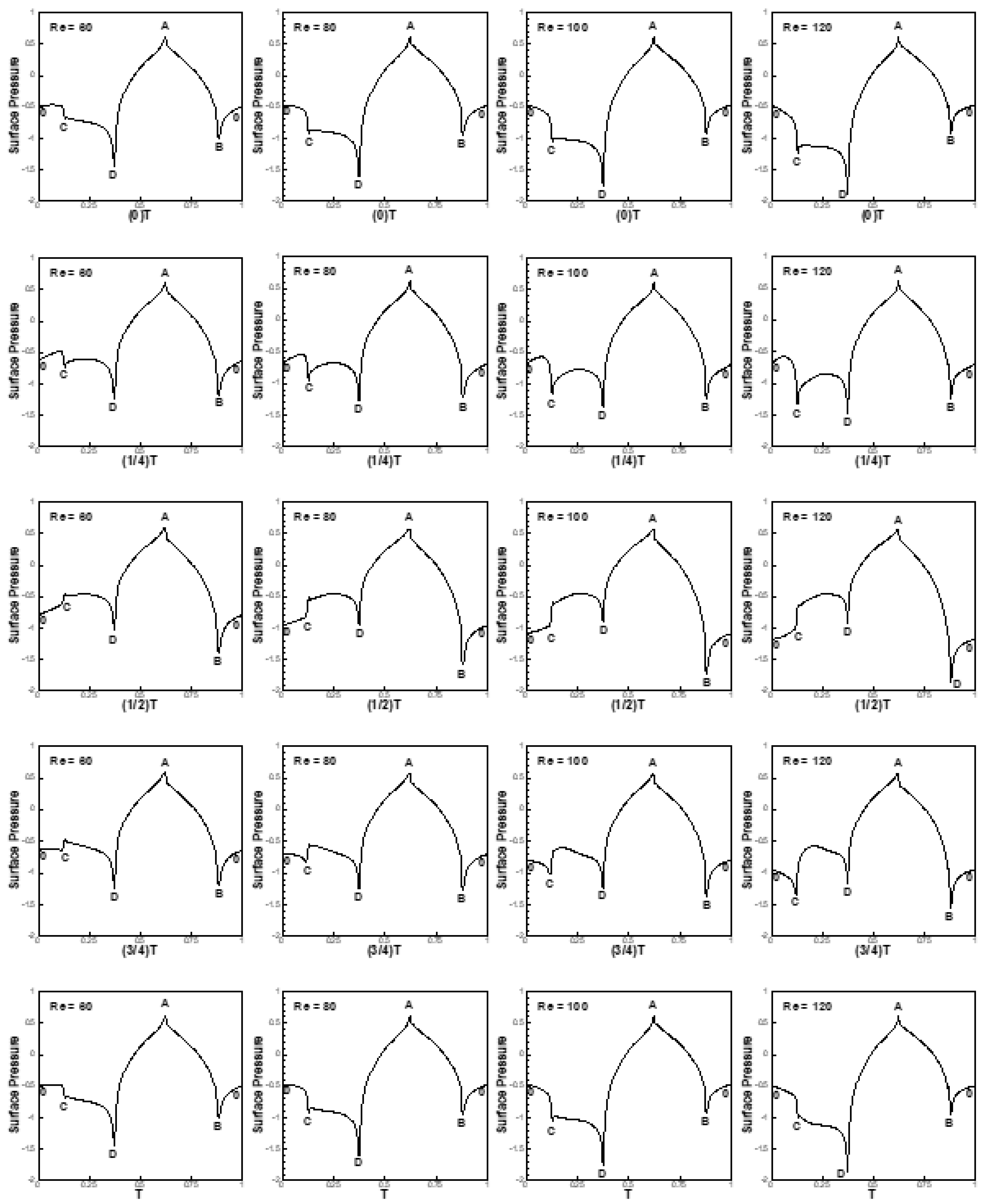
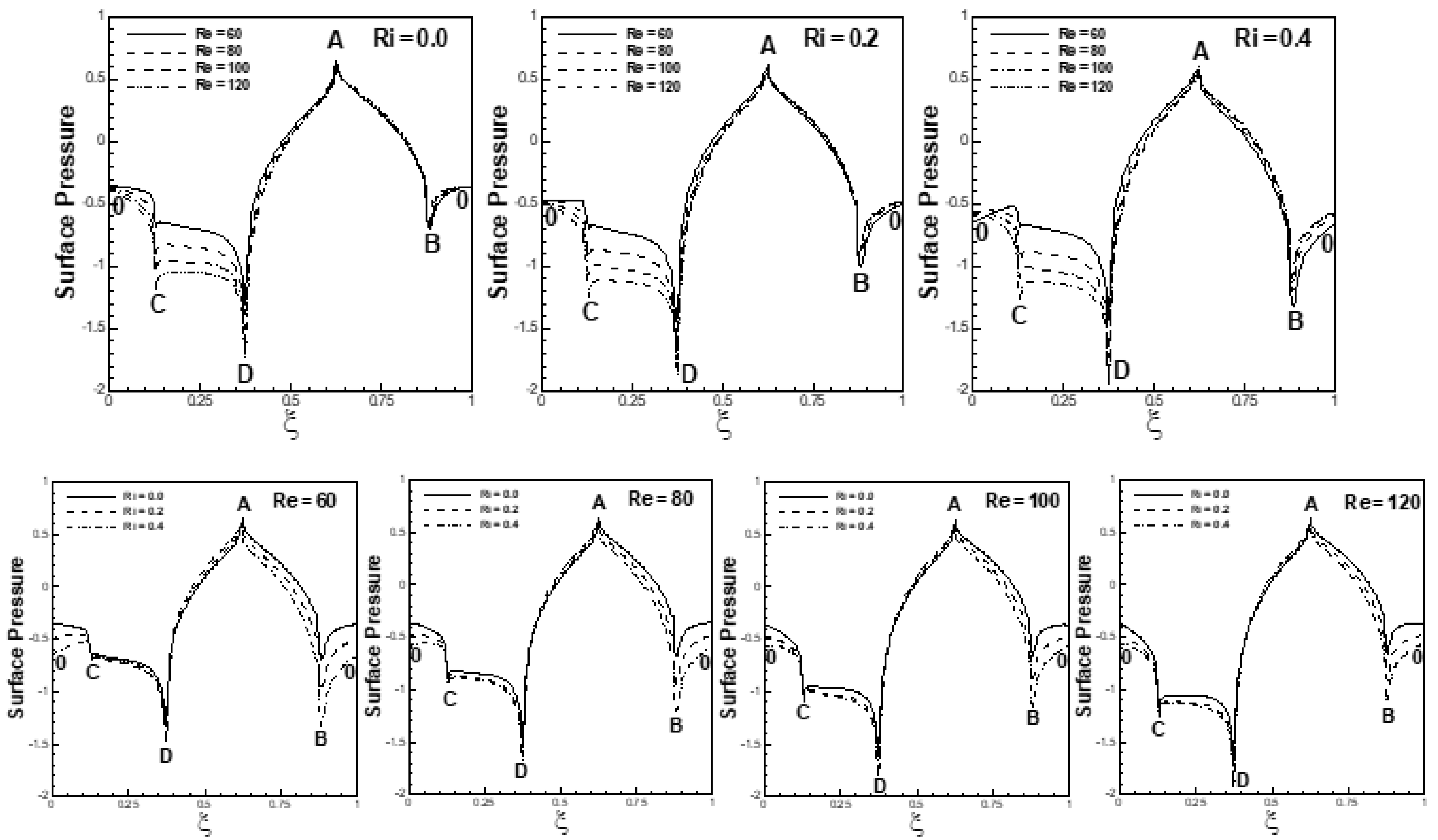
4.3. Mean Surface Pressure and Surface Vorticity
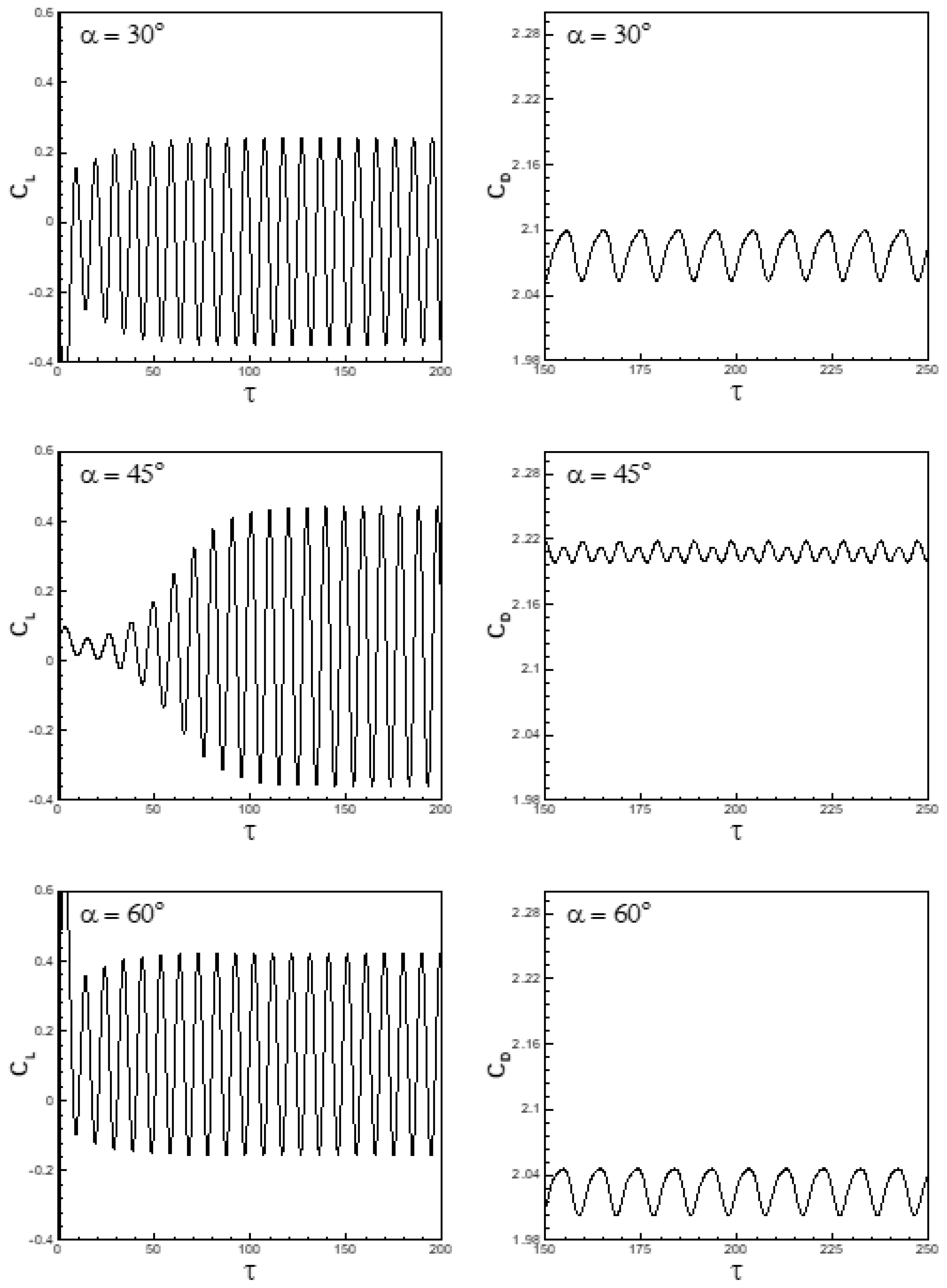
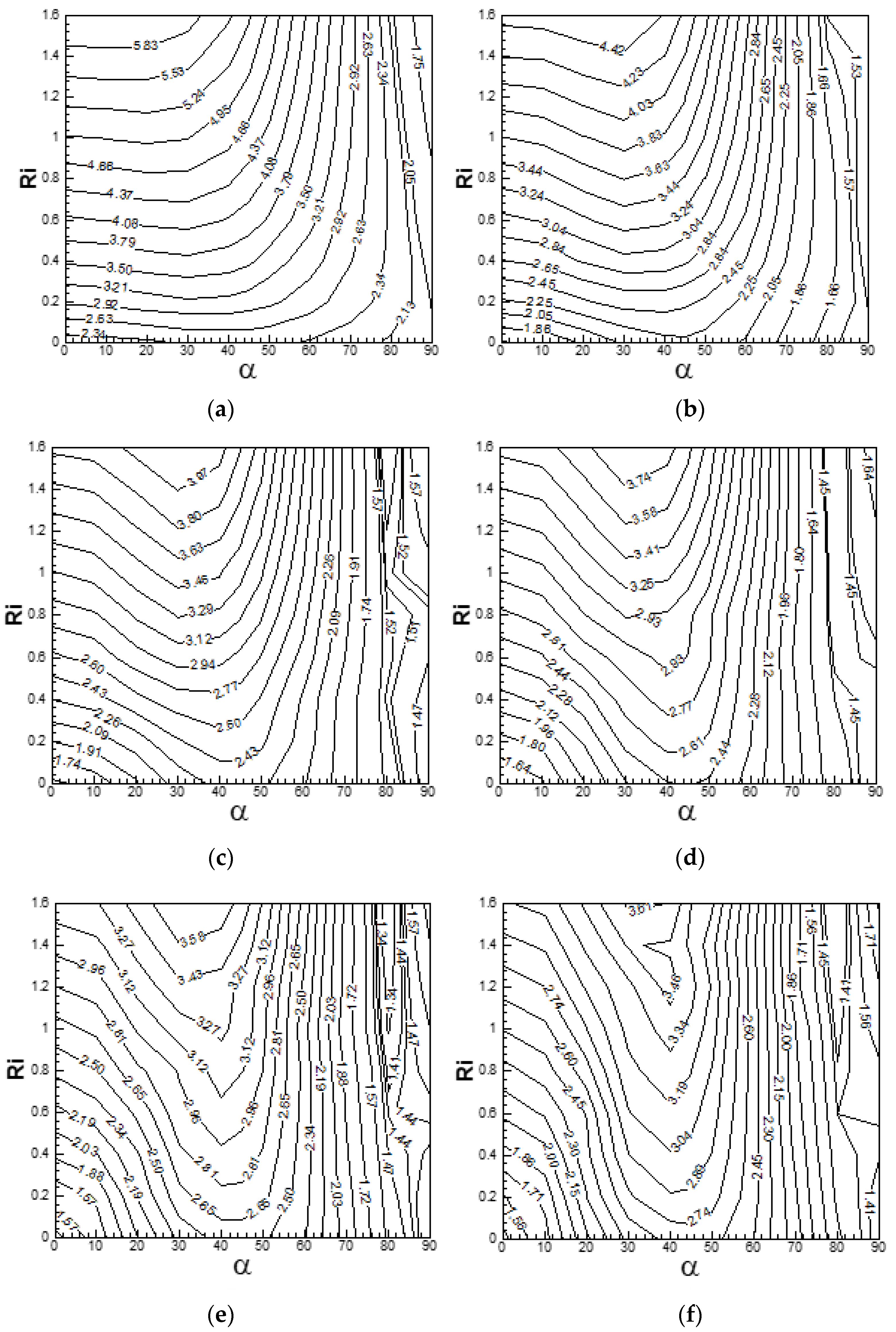
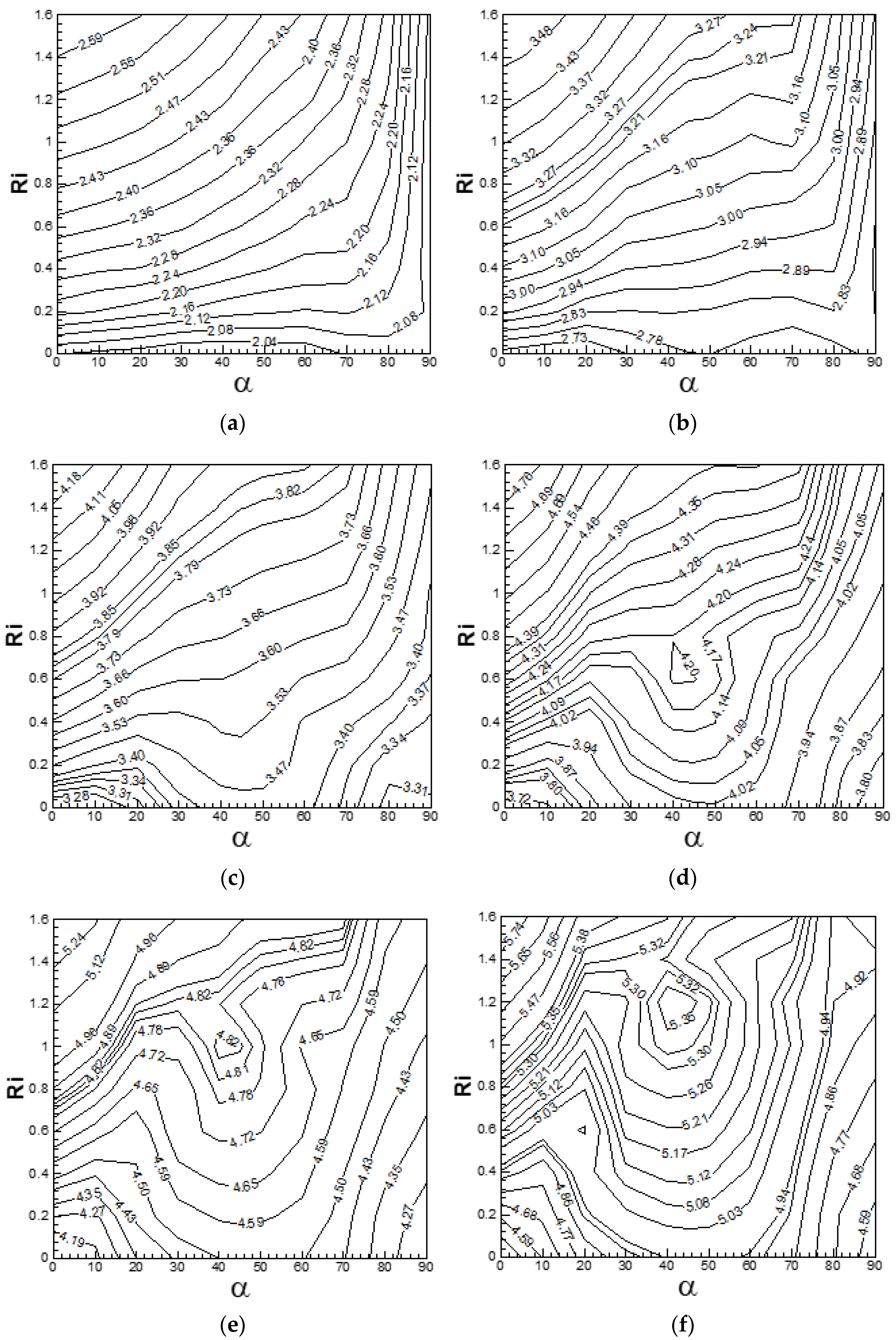
4.4. Global Parameters
4.4.1. Aerodynamic Parameters
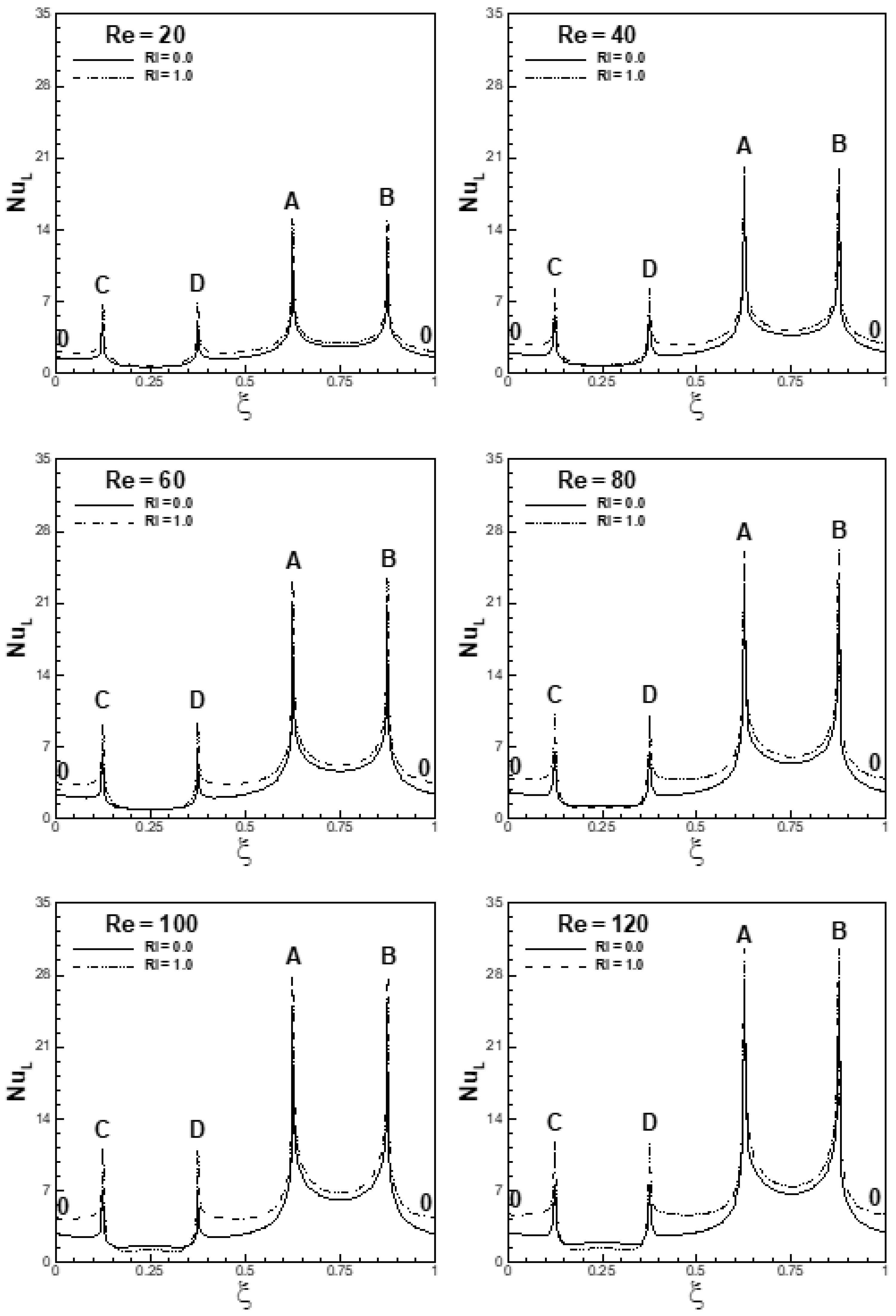
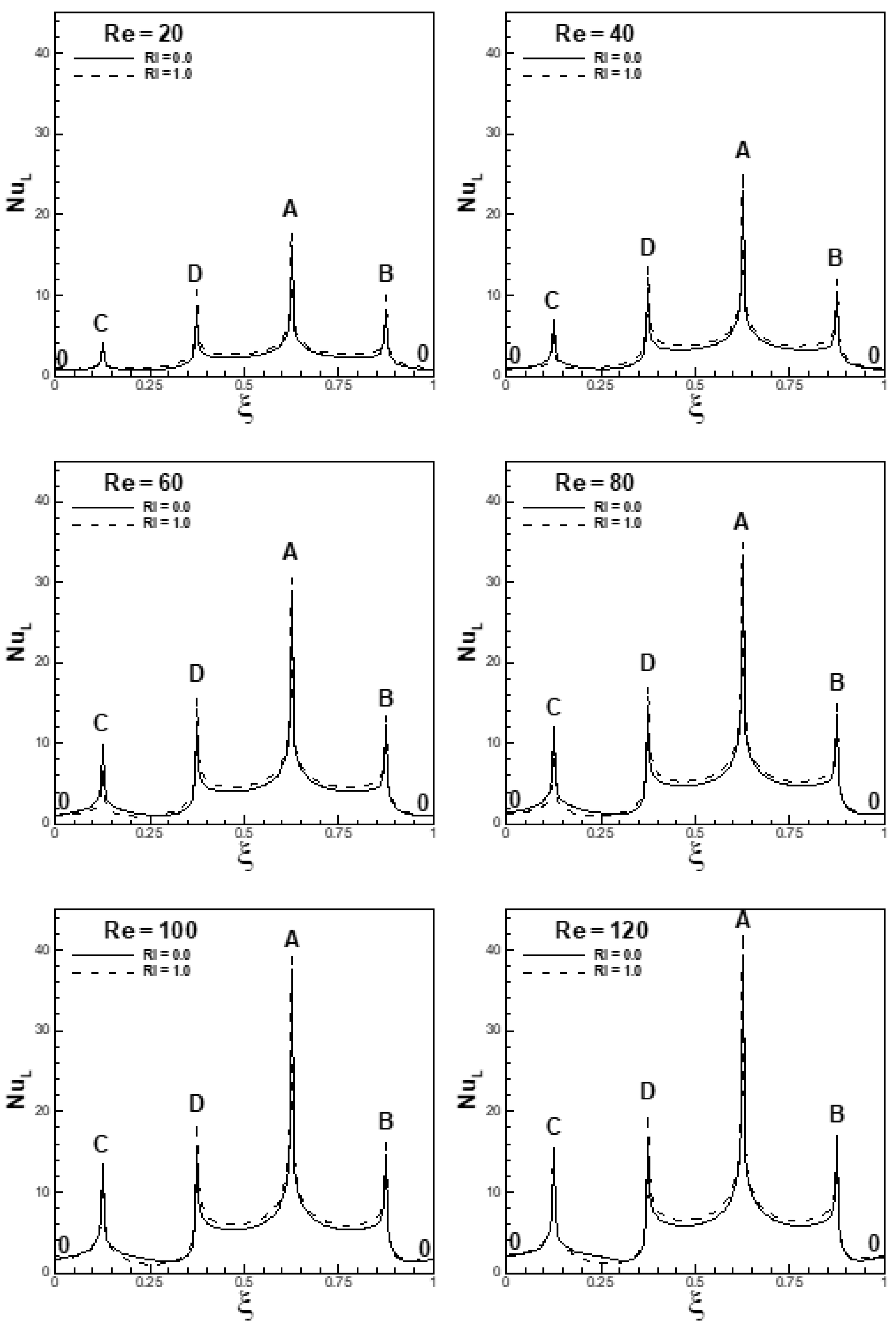
4.4.2. Heat Transfer Characteristics
5. Conclusions
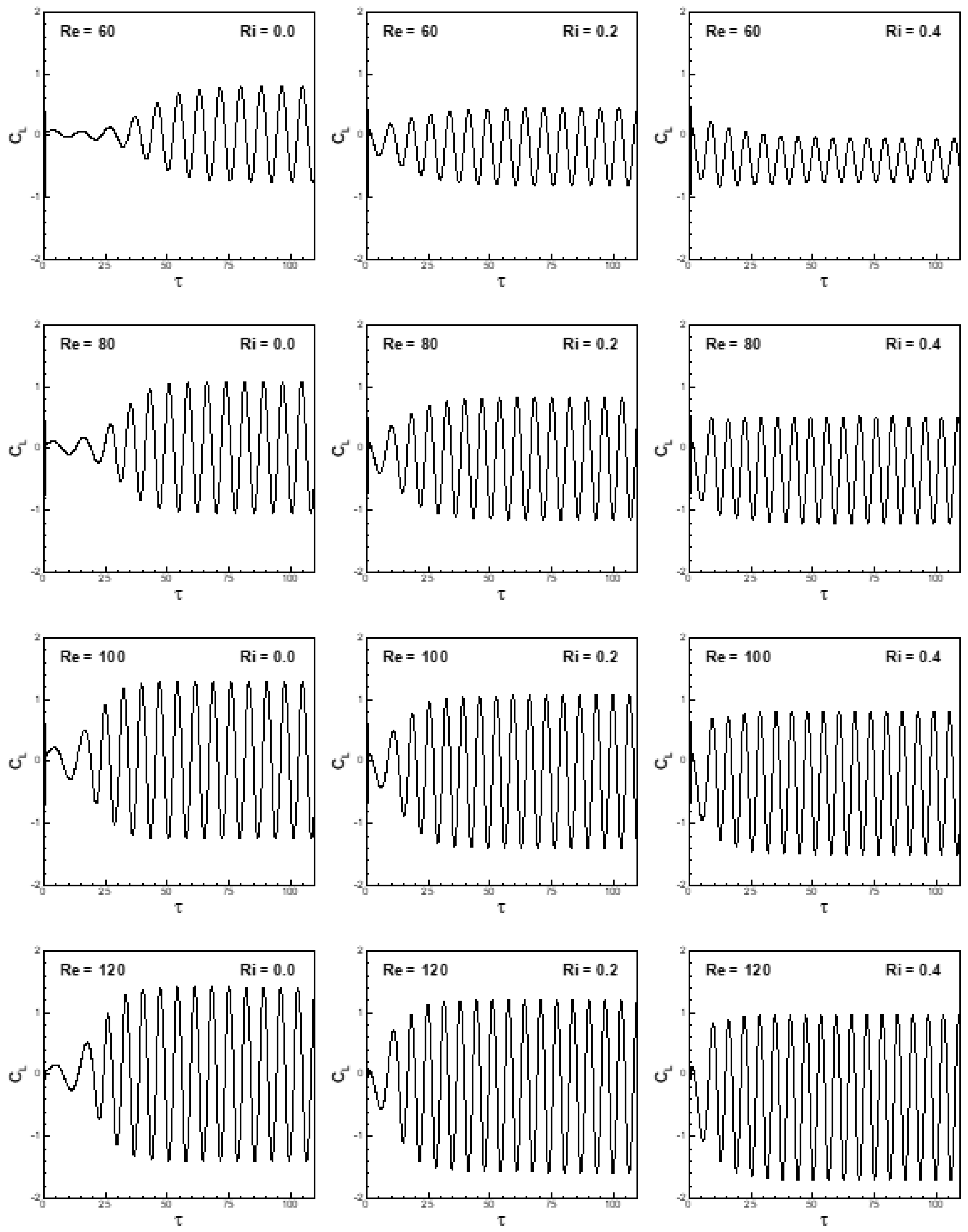
- It is observed that the vortices are reduced in size with increasing Re and are shed more frequently. The frequency of vortex-shedding increases either with an increase in Re and/or Ri for the entire range of α. The Strouhal number is found to be maximal for Re = 120 at (α = 45°, Ri = 1.2), which is approximately 34% over and above the forced flow value at the same time (Re, α);
- In the forced flow regime, mean pressure on the faces AB, BC, CD and DA of the cylinder is found to decrease with an increase in Re; an increase in Re also decreases the pressure at the corners of the cylinder. The mean surface pressure in the mixed convective flow regime changes significantly due to buoyancy forces. The effect of buoyancy forces on surface pressure reduces with an increase in α and is found to be the least at α = 90°. Mean vorticity along the surface and vertices of the cylinder is found to increase with an increase in Re;
- In the mixed convective flow regime, the amplitudes of lift coefficient are observed largest at α = 45° and increase with an increase in Re for the entire range of α. For free-stream orientations α ≠ 0°, the mean coefficient of lift increases with an increase in Ri, while it decreases with an increase in Re. Sensitivity of the mean lift coefficient to Ri is observed more at lower Re than at higher Re. The mean (or steady) coefficient of lift is found to be maximal at (Re = 20, Ri = 1.6) for any α ≠ 0°. The mean coefficient of moment () follows the same trend as followed by the mean coefficient of lift;
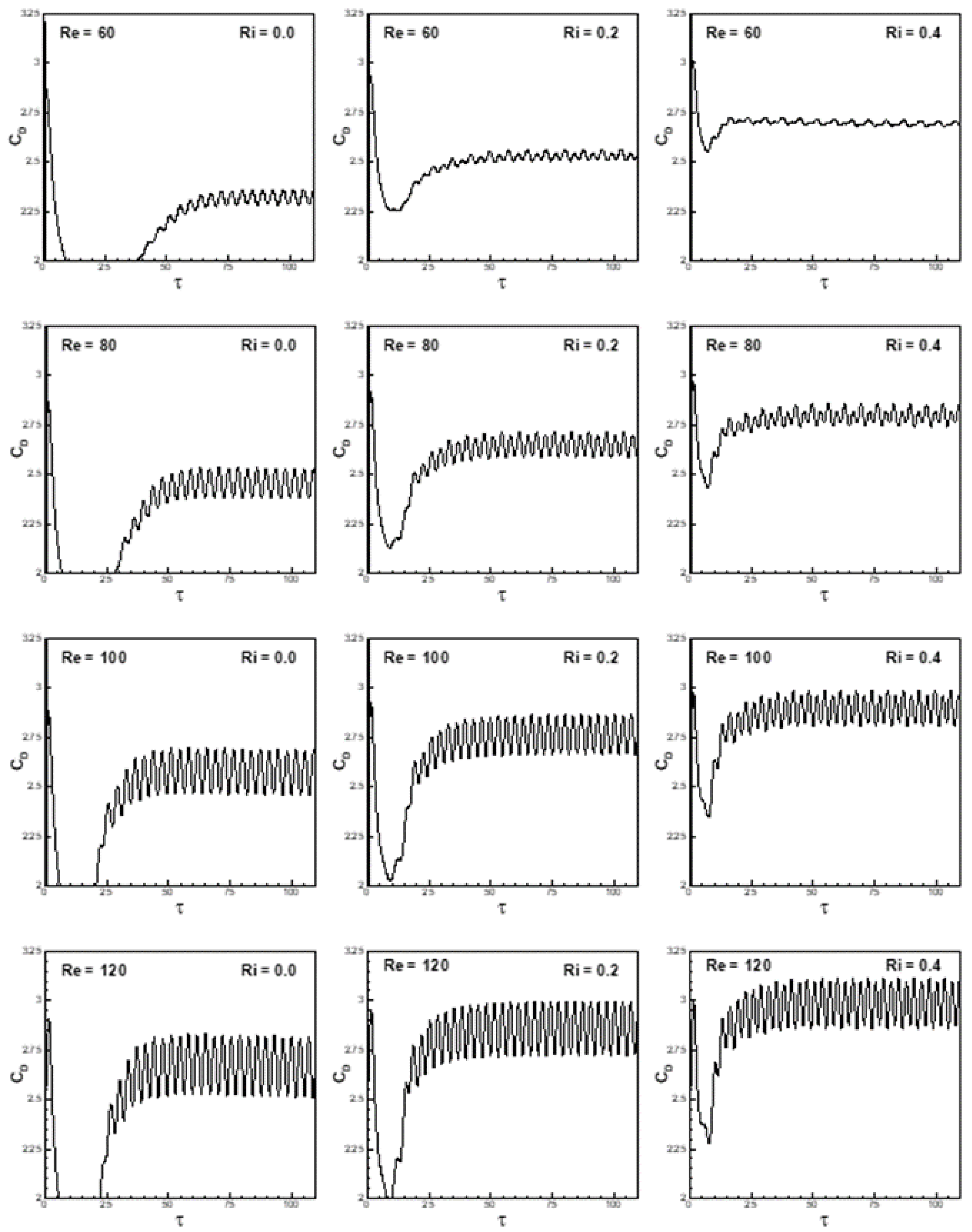
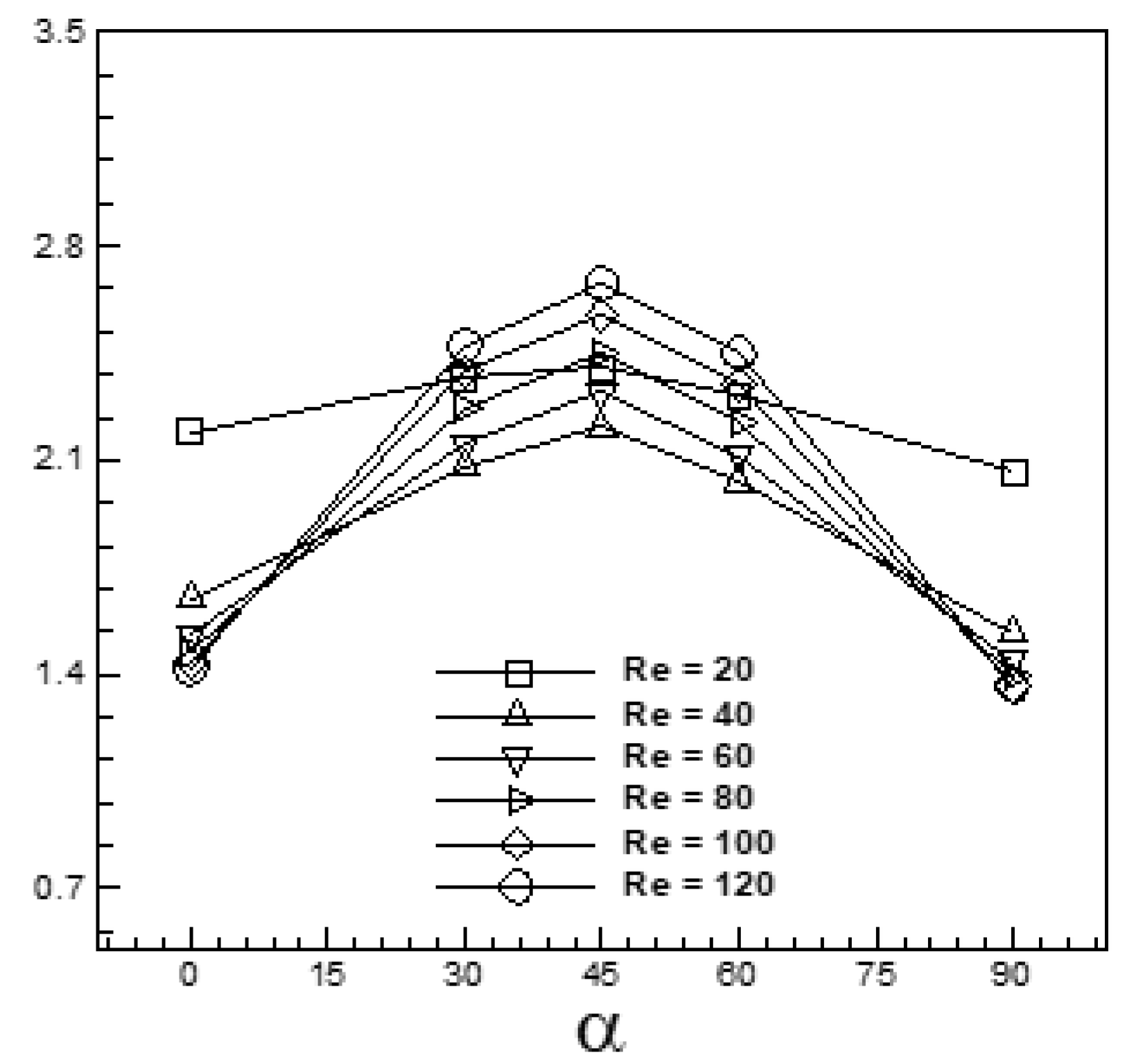
- For the entire range of Re, the amplitudes of the drag coefficient are found to be smallest at α = 45°. It is observed that the amplitudes and the mean values of the drag coefficient increase with an increase in Re. Mean drag coefficient , increases with an increase in α, reaches its maximum value at α = 45° and then decreases with an increase in α for the entire range of Re. Sensitivity of to α is observed minimum for Re = 20 and maximum for Re = 120;
- The ratio increases with an increase in Ri for the entire range of Re. Sensitivity of to Re is observed to be lower for unsteady flows than for steady flows. It is also observed that at a fixed value of Ri, the ratio decreases with an increase in Re. The sensitivity of to Ri is the observed maximum at α = 0° for the entire range of Re, and it decreases with an increase in α and is found to be theleast at α = 90°;
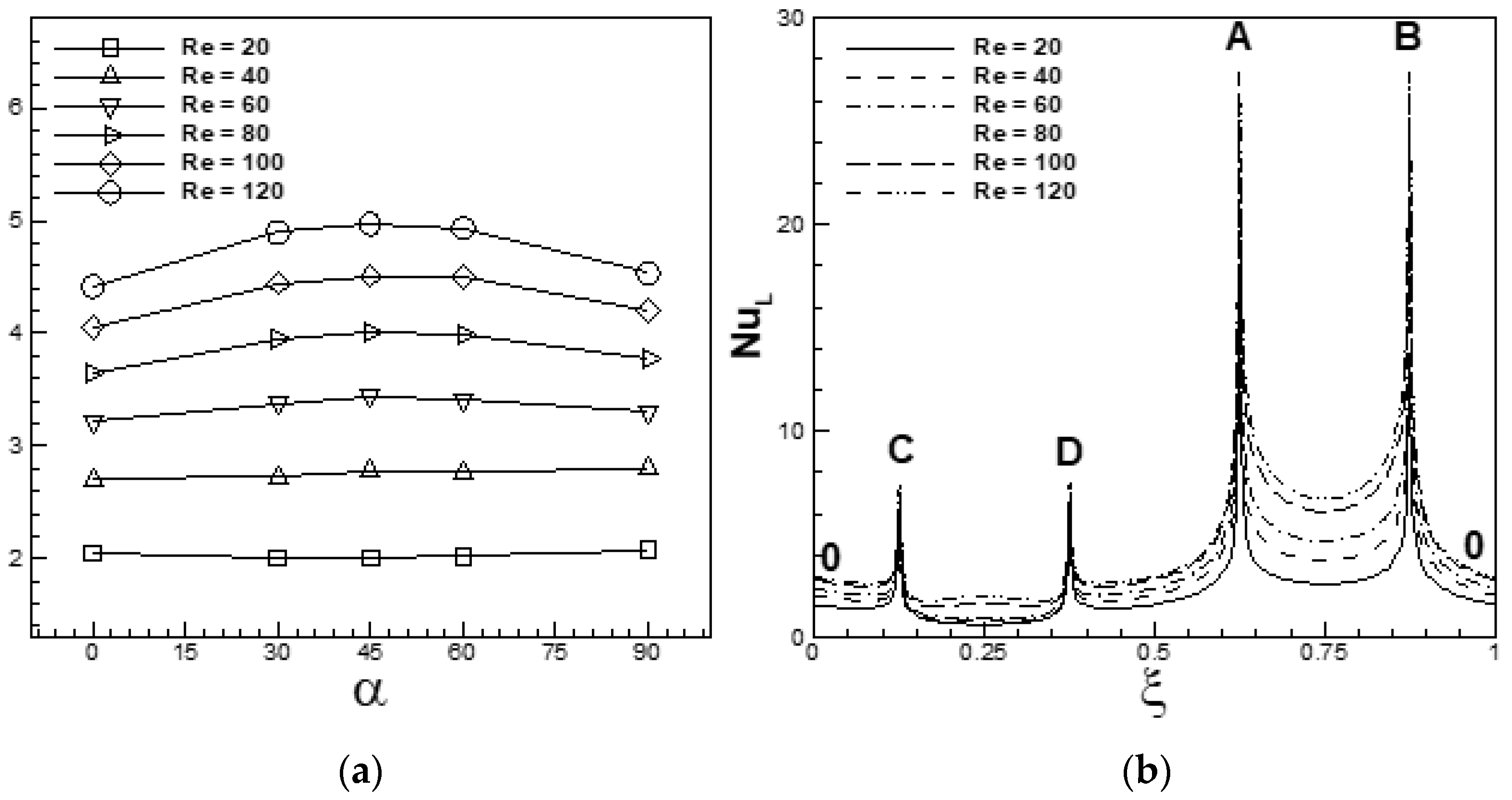
- At a fixed α, the mean Nusselt number in the forced flow regime increases significantly with an increase in Re. The sensitivity of to α increases with an increase in Re for a fixed α. The sensitivity of the Nusselt number to Ri is found to decrease with an increase in α and is found to be least sensitive to Ri at α = 90°. Additionally, the Nusselt number is found to be more sensitive to Ri for steady flows than for unsteady flows;
- In the mixed-flow regime, the ratio for a given Re is found to increase with an increase in Ri at α = 0°. For Re = 100, the ratio increases to 31.94%, 11.31%, 08.38%, 08.30% and 08.23% at α = 0°, 30°, 45°, 60° and 90°, respectively, as Ri is increased to 1.6. The percentage change in the ratio for the entire range of Re is found to be 14.07%, 14.13%, 11.74% and 10.62% at α = 30°, 45°, 60° and 90°, respectively, at a fixed value of Ri = 1.6;
- From the contour maps of mean/steady drag coefficient and Nusselt number it is possible to identify the ranges of parameters (α, Ri) that can yield a relatively high mean/steady heat transfer rate accompanied by relatively low values of mean/steady drag coefficient. Such a scenario is possible for [70° ≤ α ≤ 90°, 0 ≤ Ri ≤ 1.6] and for any .
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Turki, S.; Abbassi, H.; Nasrallah, S.B. Two-dimensional laminar fluid flow and heat transfer in a channel with a built-in heated square cylinder. Int. J. Ther. Sci. 2003, 42, 1105–1113. [Google Scholar] [CrossRef]
- Sharma, A.; Eswaran, V. Effect of aiding and opposing buoyancy on the heat and fluid flow across a square cylinder at Re = 100. Nume. Heat Trans. Part A 2004, 45, 601–624. [Google Scholar] [CrossRef]
- Bhattacharyya, S.; Mahapatra, S. Vortex shedding around a heated square cylinder under the influence of buoyancy. Heat Mass Trans. 2005, 41, 824–833. [Google Scholar] [CrossRef]
- Sharma, A.; Eswaran, V. Effect of channel-confinement and aiding/opposing buoyancy on the two-dimensional laminar flow and heat transfer across a square cylinder. Int. J. Heat Mass Trans. 2005, 48, 5310–5322. [Google Scholar] [CrossRef]
- Singh, S.K.; Panigrahi, P.K.; Muralidhar, K. Effect of buoyancy on the wakes of circular and square cylinders: A schlieren-interferometric study. Exp. Fluids 2007, 43, 101–123. [Google Scholar] [CrossRef]
- Perng, S.-W.; Wu, H.-W. Buoyancy-aided/opposed convection heat transfer for unsteady turbulent flow across a square cylinder in a vertical channel. Int. J. Heat Mass Trans. 2007, 50, 3701–3717. [Google Scholar] [CrossRef]
- Dhiman, A.K.; Chhabra, R.P.; Eswaran, V. Steady mixed convection across a confined square cylinder. Int. Comm. Heat Mass Trans. 2008, 35, 47–55. [Google Scholar] [CrossRef]
- Kakade, A.A.; Singh, S.K.; Panigrahi, P.K.; Muralidhar, K. Schlieren investigation of the square cylinder wake: Joint influence of buoyancy and orientation. Phys. Fluids 2010, 22, 054107. [Google Scholar] [CrossRef]
- Chatterjee, D. Triggering vortex-shedding by superimposed thermal buoyancy around bluff obstacles in cross-flow at low Reynolds numbers. Nume. Heat Trans. Part A 2012, 61, 800–806. [Google Scholar] [CrossRef]
- Chatterjee, D.; Mondal, B. Effect of thermal buoyancy on the two-dimensional upward flow and heat transfer around a square cylinder. Heat Trans. Eng. 2012, 33, 1063–1074. [Google Scholar] [CrossRef]
- Dhiman, A.; Sharma, N.; Kumar, S. Buoyancy-aided momentum and heat transfer in a vertical channel with a built-in square cylinder. Int. J. Sust. Energy 2013, 33, 963–984. [Google Scholar] [CrossRef]
- Yang, G.; Wu, J. Effect of side ratio and aiding/opposing buoyancy on the aerodynamic and heat transfer characteristics around a rectangular cylinder at low Reynolds numbers. Nume. Heat Trans. Part A 2013, 64, 1016–1037. [Google Scholar] [CrossRef]
- Sivakumar, S.; Nirmalkar, N.; Chhabra, R.P. Effect of orientation on mixed convection from a heated square bar in Newtonian and power-law fluids. Nume. Heat Trans. Part A 2014, 65, 435–460. [Google Scholar] [CrossRef]
- Islam, S.U.; Rahman, H.; Abbasi, W.S.; Shahina, T. Lattice boltzmann study of wake structure and force statistics for various gap spacings between a square cylinder with a detached flat plate. Arabian J. Sci. and Eng. 2015, 40, 2169–2182. [Google Scholar] [CrossRef]
- Rastan, M.R.; Sohankar, A.; Alam, M.M. Flow control over a square cylinder using attached rigid and flexible splitter plate at intermediate flow regime. Phys. Fluids 2017, 29, 103601. [Google Scholar] [CrossRef]
- Dey, P.; Das, A.K. Analysis of fluid flow and heat transfer characteristics over a square cylinder: Effect of corner radius and nanofluid volume fraction. Arabian J. Sci. and Eng. 2017, 42, 1687–1698. [Google Scholar] [CrossRef]
- Bensedira, S.; El-hadj, A.A.; Semmar, D.; Messaoudene, N.A. Dynamic analysis of flow around two side-by-side cylinders near a wall. Arabian J. Sci. and Eng. 2018, 43, 4531–4540. [Google Scholar] [CrossRef]
- Wang, Y.Q. Effects of Reynolds number on vortex structure behind a surface-mounted finite square cylinder with AR = 7. Phys. Fluids 2019, 31, 115103. [Google Scholar] [CrossRef]
- Islam, S.U.; Ullah, N.; Zhou, C.Y. Fluid dynamics of flow around side-by-side arranged cylinders. Arabian J. Sci. and Eng. 2020, 45, 5907–5923. [Google Scholar] [CrossRef]
- Abdelhamid, M.M.A.T.; Sohankar, A. Effect of cylinder corner radius and attack angle on heat transfer and flow topology. Int. J. Mech. Sci. 2020, 175, 105566. [Google Scholar]
- Rashid, A.; Hasan, N. Steady and unsteady flow regimes in two-dimensional mixed convective flow of air past a heated square cylinder. Int. J. Mech. Sci. 2020, 175, 105533. [Google Scholar]
- Zafar, F.; Alam, M.M. Mixed convection heat transfer from a circular cylinder submerged in wake. Int. J. Mech. Sci. 2000, 183, 105733. [Google Scholar] [CrossRef]
- Rashid, A.; Anshumaan, S. Numerical study of fluid dynamics and heat transfer characteristics for the flow past a heated square cylinder. Jordan J. Mech. Ind. Eng. 2021, 15, 357–376. [Google Scholar]
- Abbasi, W.S.; Naheed, A.; Islam, S.U.; Rahman, H. Investigation of Optimum Conditions for Flow Control Around Two Inline Square Cylinders. Arabian J. Sci. Eng. 2021, 46, 2845–2864. [Google Scholar] [CrossRef]
- Sohankar, A.M.A.; Alam, M.M. Flow over rectangular cylinder: Effects of cylinder aspect ratio and Reynolds number. Int. J. Mech. Sci. 2021, 195, 106264. [Google Scholar]
- Arif, M.R.; Hasan, N. Effect of free-stream inclination and buoyancy on flow past a square cylinder in large-scale heating regime. Phys. Fluids 2021, 33, 073601. [Google Scholar] [CrossRef]
- Tritton, D.J. Physical fluid dynamics. ELBS Edi. Chap. 1979, 13, 127–130. [Google Scholar]
- Rhie, C.M.; Chow, W.L. Numerical study of the turbulent flow past an airfoil with trailing edge separation. AIAA J. 1983, 21, 1525–1532. [Google Scholar] [CrossRef]
- Amsden, A.A.; Harlow, F.H. The SMAC Method: A Numerical Technique for Calculating Incompressible Fluid Flows; Los Alamos Scientific Report; Los Alamos Scientific Lab.: Los Alamos, NM, USA, 1970; p. LA 4370. [Google Scholar]
- Cheng, L.; Armfield, S. A simplified marker and cell method for unsteady flows on non-staggered grids. Int. J. Nume. Meth. Fluids 1995, 21, 15–34. [Google Scholar] [CrossRef]
- Kim, S.W.; Benson, T.J. Comparison of the SMAC, PISO and iterative time advancing schemes for unsteady flows. Compt. Fluids 1992, 21, 435–454. [Google Scholar] [CrossRef]
- Kuwahara, K. Unsteady flow simulation and its visualization. In Proceedings of the 30th AIAA Fluid Dynamics Conference AIAA, Norfolk, VA, USA, 28 June–1 July 1999. [Google Scholar] [CrossRef]
- Hasan, N.; Sanghi, S. The dynamics of two-dimensional buoyancy driven convection in a horizontal rotating cylinder. J. Heat Trans. 2004, 126, 963–984. [Google Scholar] [CrossRef]
- Hasan, N.; Anwer, S.F.; Sanghi, S. On the outflow boundary condition for external incompressible flows: A new approach. J. Compu. Phys. 2005, 206, 661–683. [Google Scholar] [CrossRef]
- Gresho, P.M. Incompressible fluid dynamics: Some fundamental formulation issue. Annu. Rev. Fluid Mech. 1991, 23, 413–454. [Google Scholar] [CrossRef]
- Hasan, N.; Rashid, A. Vortex-shedding suppression in two-dimensional mixed convective flows past circular and square cylinders. Phys. Fluids 2013, 22, 053603. [Google Scholar] [CrossRef]
- Sohankar, A.; Norberg, C.; Davidson, L. Low-Reynolds-number flow around a cylinder at incidence: Study of blockage, onset of vortex shedding and outlet boundary condition. Int. J. Nume. Meth. Fluids 1998, 26, 39–56. [Google Scholar] [CrossRef]
- Ranjan, R.; Dalal, A.; Biswas, G. A numerical study of fluid flow and heat transfer around a square cylinder at incidence using unstructured grids. Nume. Heat Trans. Part A 2008, 54, 890–913. [Google Scholar] [CrossRef]






 unsteady flow).
unsteady flow).
 unsteady flow).
unsteady flow).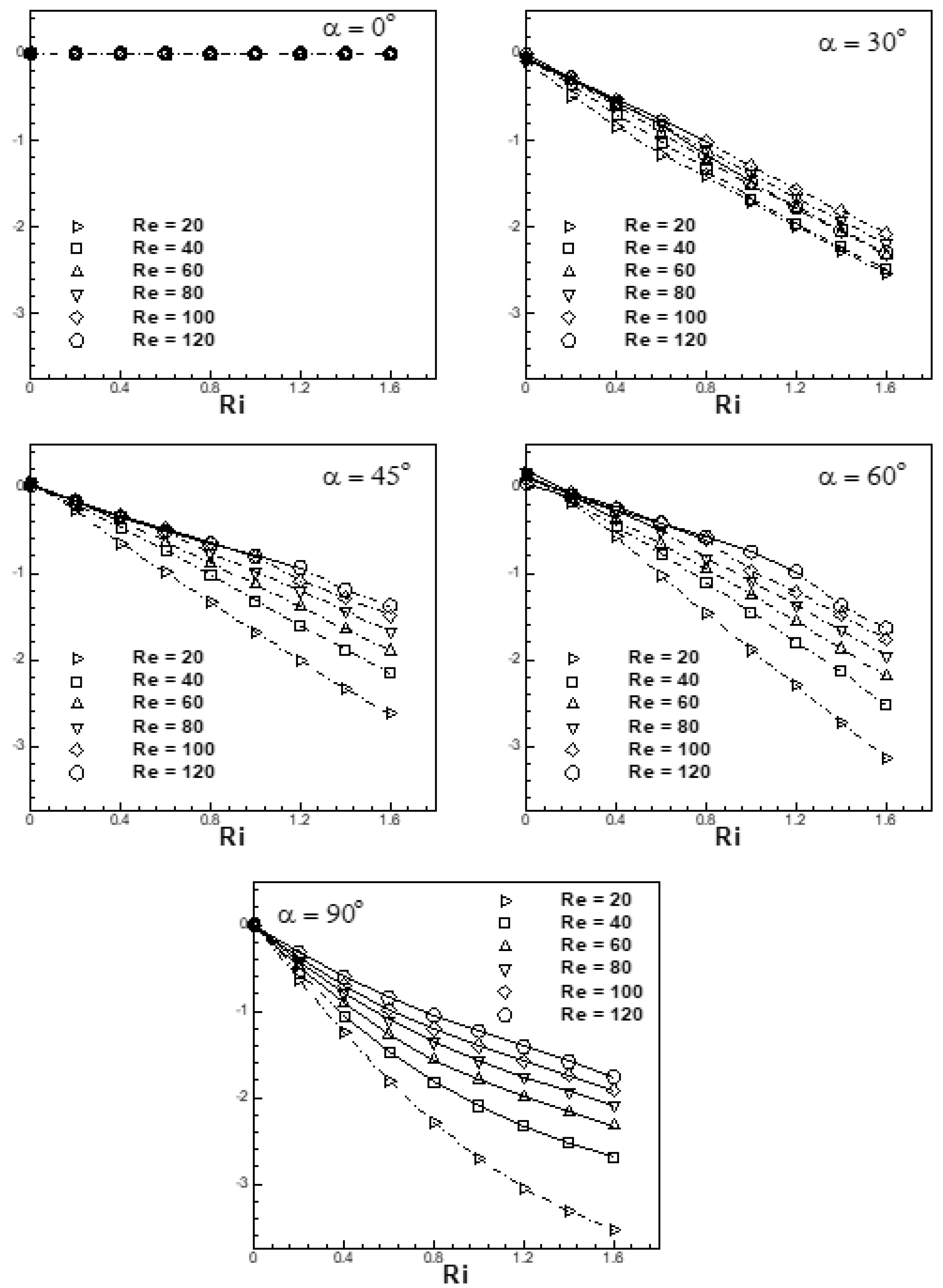
 unsteady flow).
unsteady flow).
 unsteady flow).
unsteady flow).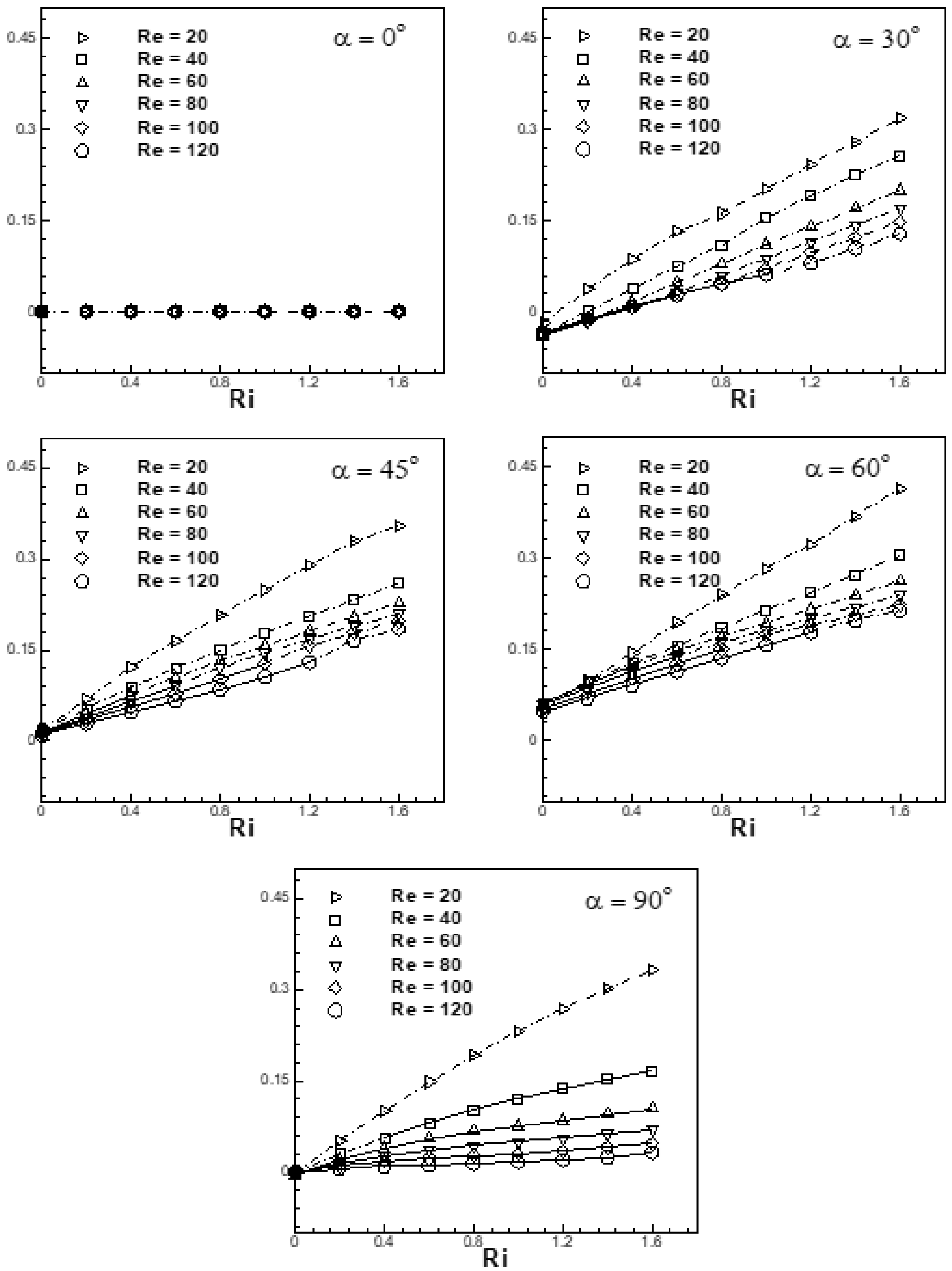
 unsteady flow).
unsteady flow).
 unsteady flow).
unsteady flow).

 unsteady flow).
unsteady flow).
 unsteady flow).
unsteady flow).
| Parameters | Sharma and Eswaran [2] | Sohankar et al. [37] | Ranjan et al. [38] | Present |
|---|---|---|---|---|
| CL,rms | 0.183 | 0.139 | 0.190 | 0.175 |
| CD (time mean) | 1.559 | 1.460 | 1.449 | 1.438 |
| Nu (time mean) | 4.070 | - | 4.124 | 4.051 |
| St | 0.148 | 0.146 | 0.145 | 0.143 |
| Reynolds Number (Re) | Free-Stream Orientation (α) | Richardson Number (Ri) | St | |||
|---|---|---|---|---|---|---|
| 30° | 0 | −5.5705 × 10−2 | 2.0795 | 0.1026 | 2.7245 | |
| 40 | 45° | 0 | 4.2002 × 10−2 | 2.2072 | 0.1028 | 2.7782 |
| 60° | 0 | 0.1355 | 2.0274 | 0.1028 | 2.7640 | |
| 0° | 0 | −1.4203 × 10−3 | 1.5275 | 0.1206 | 3.2128 | |
| 60 | 30° | 0.2 | −0.3193 | 2.4101 | 0.1345 | 3.4517 |
| 45° | 0 | 2.7197 × 10−2 | 2.3219 | 0.1195 | 3.4411 | |
| 45° | 0.2 | −0.1722 | 2.5314 | 0.1312 | 3.5082 | |
| 45° | 0.4 | −0.3919 | 2.6915 | 0.1420 | 3.5460 | |
| 60° | 0.2 | −0.1064 | 2.1886 | 0.1293 | 3.4491 | |
| 0° | 0 | −1.3655 × 10−3 | 1.4712 | 0.1338 | 3.6471 | |
| 80 | 30° | 0.2 | −0.3061 | 2.4965 | 0.1438 | 4.0371 |
| 45° | 0 | 2.2897 × 10−2 | 2.4524 | 0.1300 | 4.0069 | |
| 45° | 0.2 | −0.1558 | 2.6495 | 0.1409 | 4.0888 | |
| 45° | 0.4 | −0.3341 | 2.7957 | 0.1504 | 4.1508 | |
| 60° | 0.2 | −6.7151 × 10−2 | 2.2865 | 0.1394 | 4.0381 | |
| 0° | 0 | −1.0942 × 10−3 | 1.4380 | 0.1432 | 4.0511 | |
| 100 | 30° | 0.2 | −0.2841 | 2.6090 | 0.1508 | 4.5526 |
| 45° | 0 | 1.9272 × 10−2 | 2.5763 | 0.1377 | 4.5124 | |
| 45° | 0.2 | −0.1570 | 2.7651 | 0.1481 | 4.6026 | |
| 45° | 0.4 | −0.3285 | 2.8961 | 0.1562 | 4.6722 | |
| 60° | 0.2 | −6.7827 × 10−2 | 2.3987 | 0.1464 | 4.5625 | |
| 0° | 0 | −4.1067 × 10−4 | 1.4212 | 0.1492 | 4.4162 | |
| 120 | 30° | 0.2 | −0.2418 | 2.7184 | 0.1560 | 5.0199 |
| 45° | 0 | 9.6314 × 10−3 | 2.6740 | 0.1430 | 4.9684 | |
| 45° | 0.2 | −0.1721 | 2.8663 | 0.1531 | 5.0658 | |
| 45° | 0.4 | −0.3489 | 2.9888 | 0.1610 | 5.1373 | |
| 60° | 0.2 | −0.1142 | 2.5118 | 0.1515 | 5.0137 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ali, R.; Hasan, N. Analysis of Flow Structures and Global Parameters across a Heated Square Cylinder in Forced and Mixed Convection. Atmosphere 2023, 14, 22. https://doi.org/10.3390/atmos14010022
Ali R, Hasan N. Analysis of Flow Structures and Global Parameters across a Heated Square Cylinder in Forced and Mixed Convection. Atmosphere. 2023; 14(1):22. https://doi.org/10.3390/atmos14010022
Chicago/Turabian StyleAli, Rashid, and Nadeem Hasan. 2023. "Analysis of Flow Structures and Global Parameters across a Heated Square Cylinder in Forced and Mixed Convection" Atmosphere 14, no. 1: 22. https://doi.org/10.3390/atmos14010022
APA StyleAli, R., & Hasan, N. (2023). Analysis of Flow Structures and Global Parameters across a Heated Square Cylinder in Forced and Mixed Convection. Atmosphere, 14(1), 22. https://doi.org/10.3390/atmos14010022





