Convection Initiation Associated with the Merger of an Immature Sea-Breeze Front and a Gust Front in Bohai Bay Region, North China: A Case Study
Abstract
1. Introduction
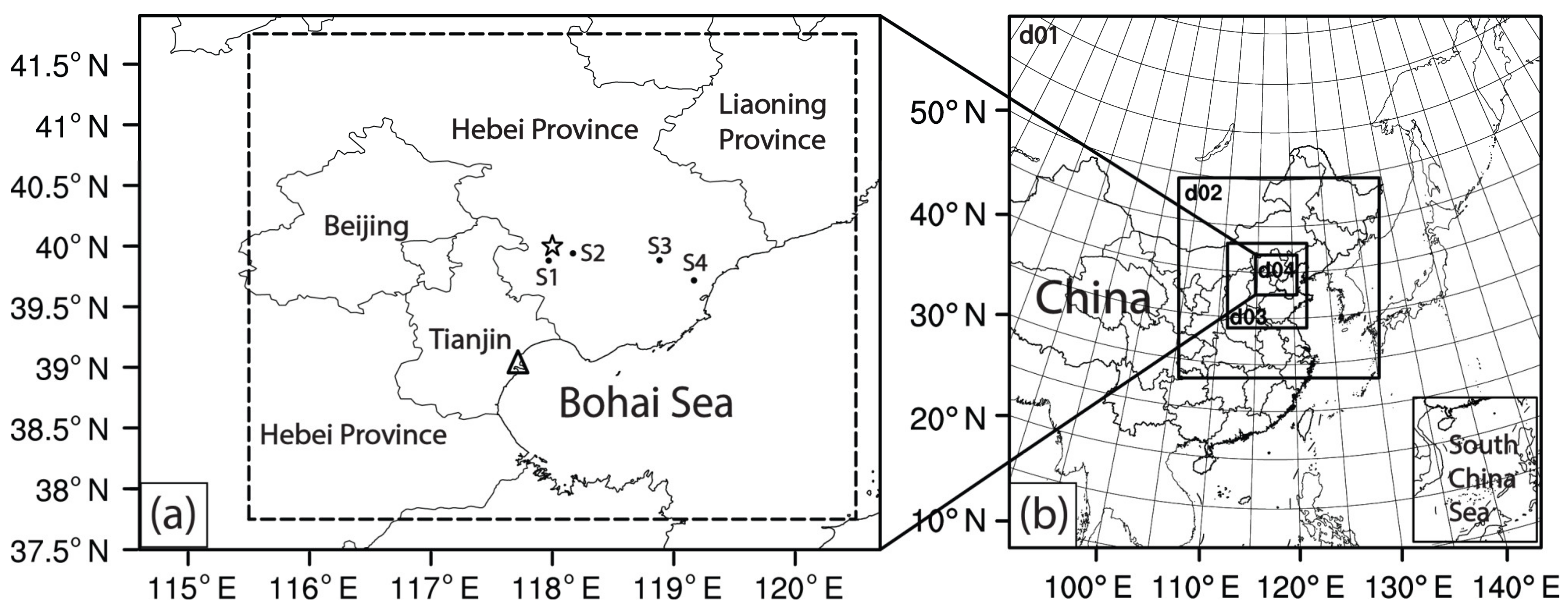
2. Case Overview
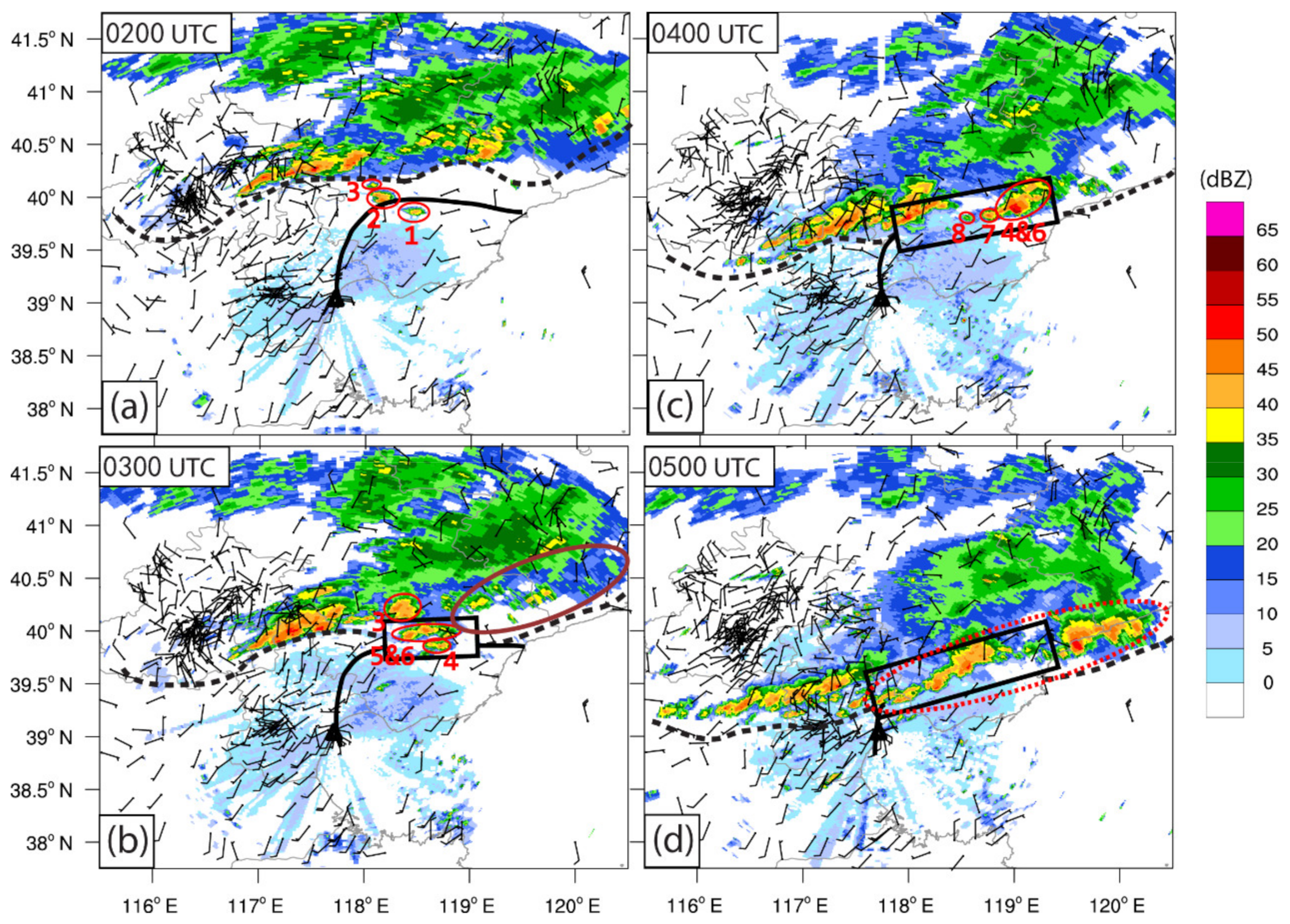
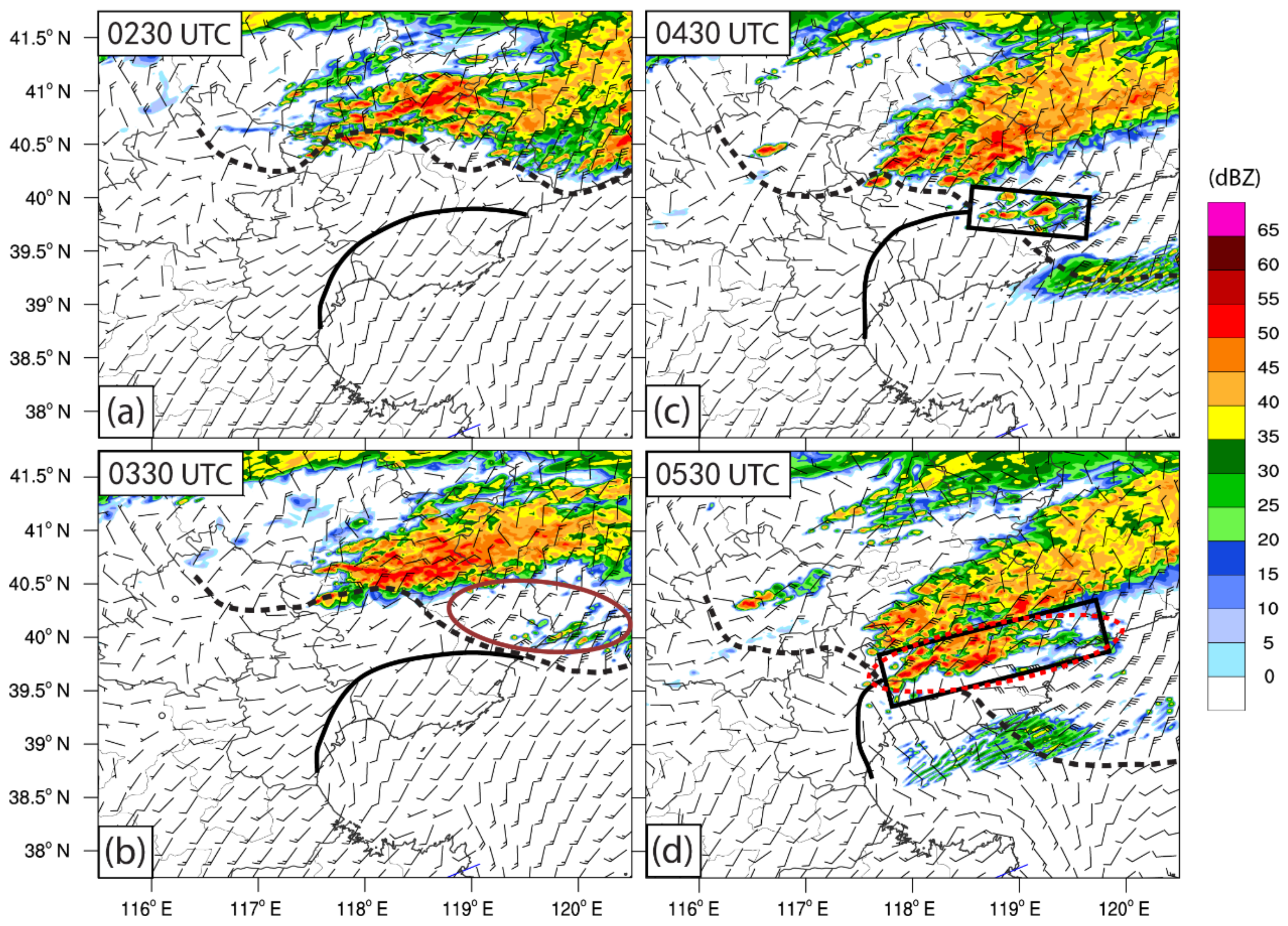
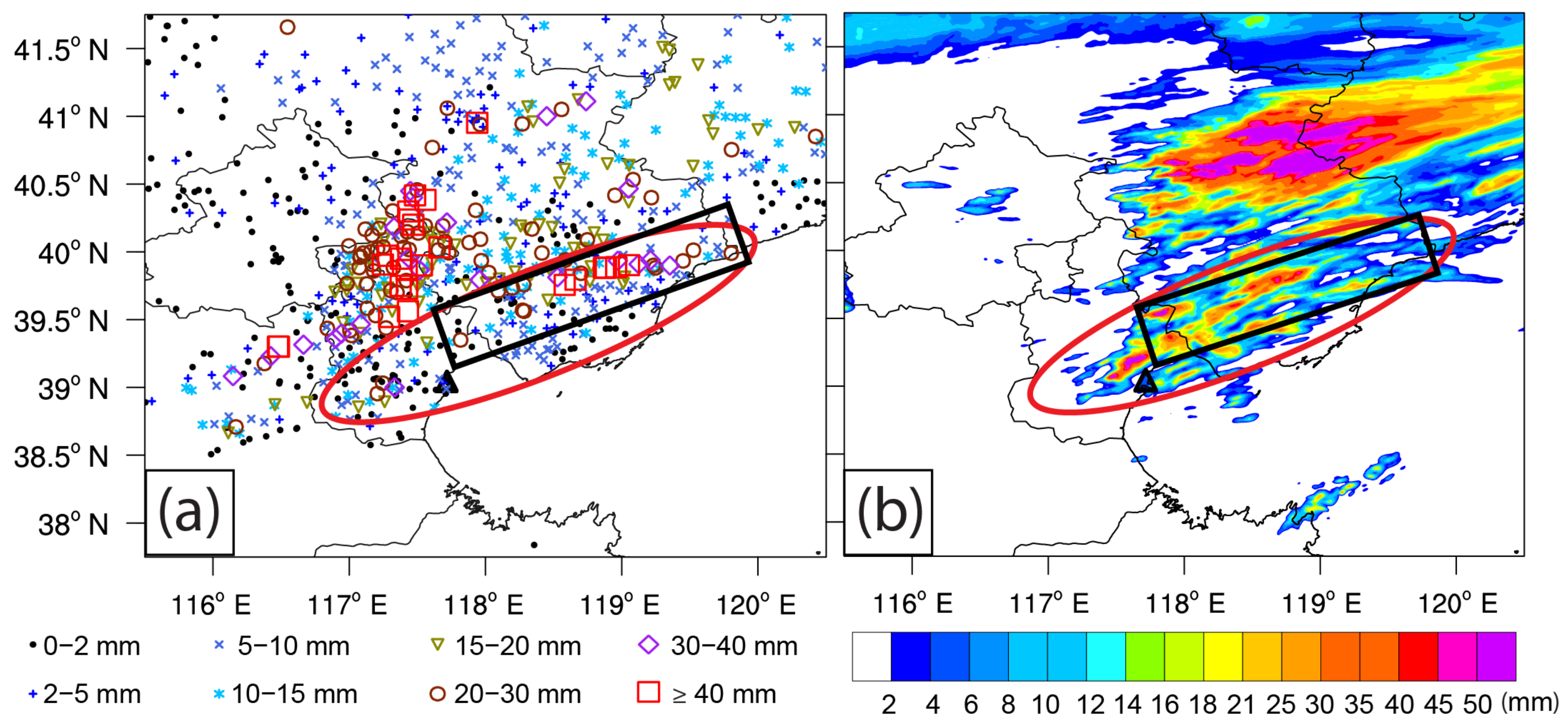
2.1. System Evolution
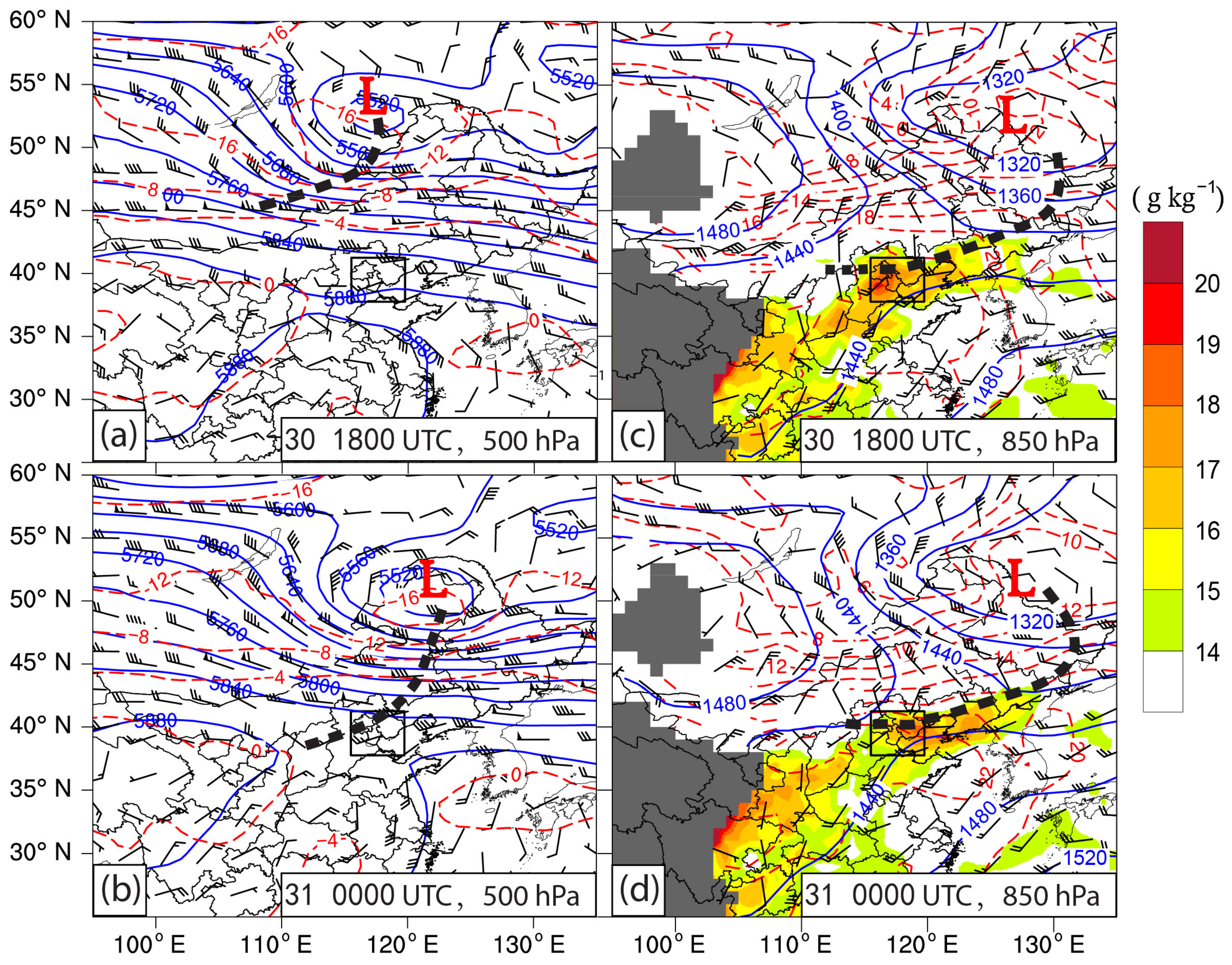
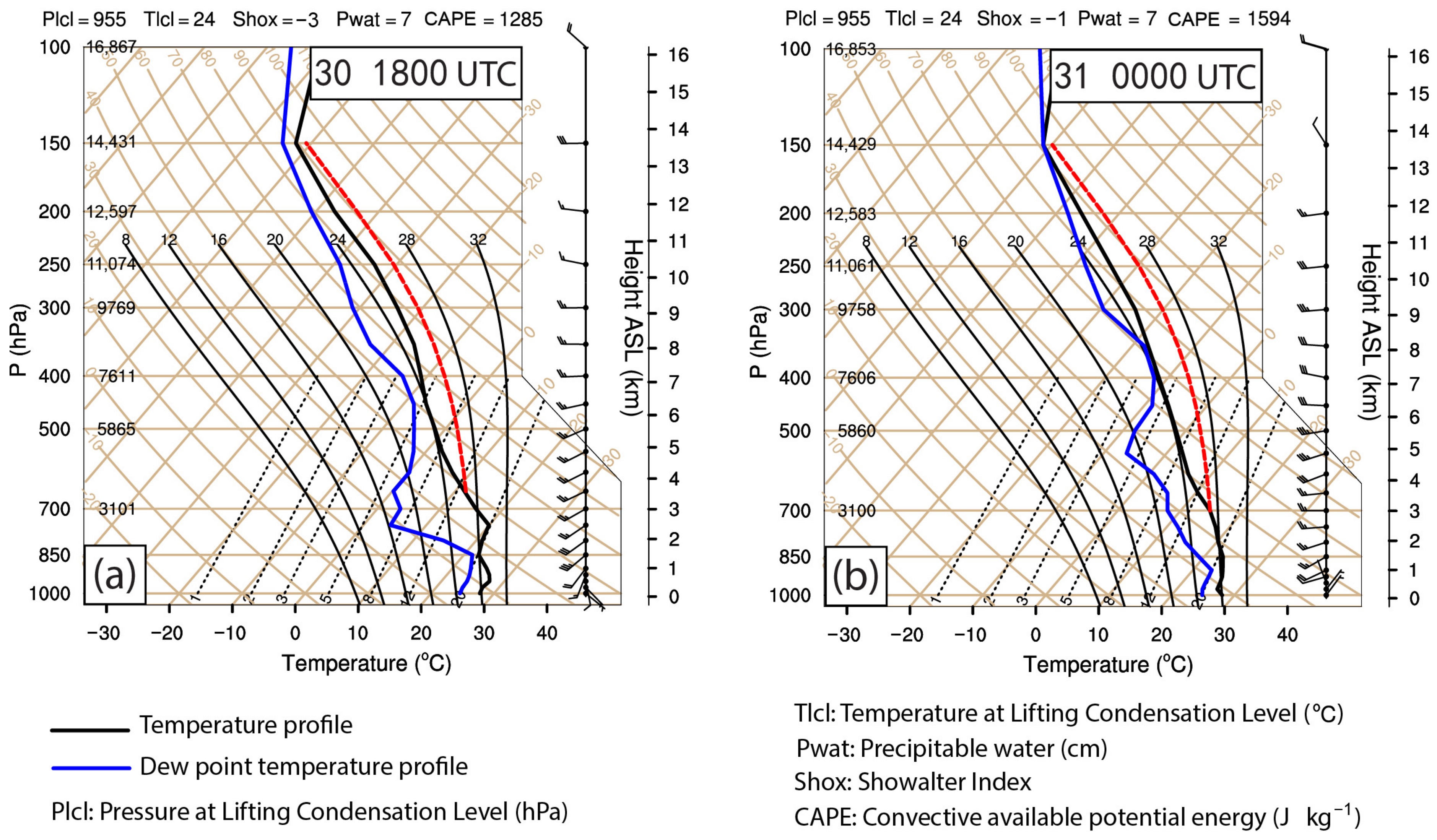
2.2. Environmental Conditions
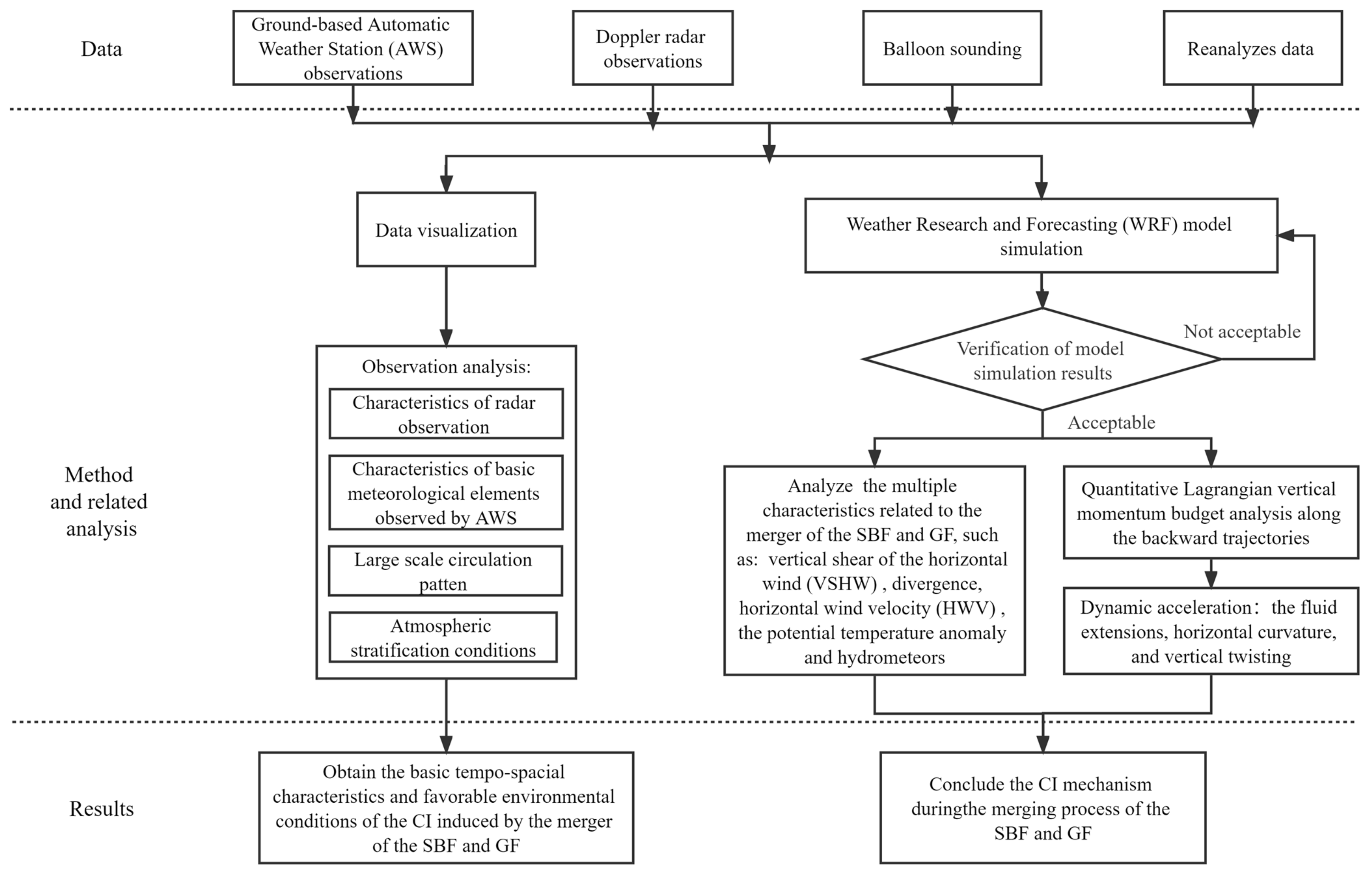
3. Numerical Simulation
3.1. Setup of Numerical Experiment
3.2. Evaluation of Simulation
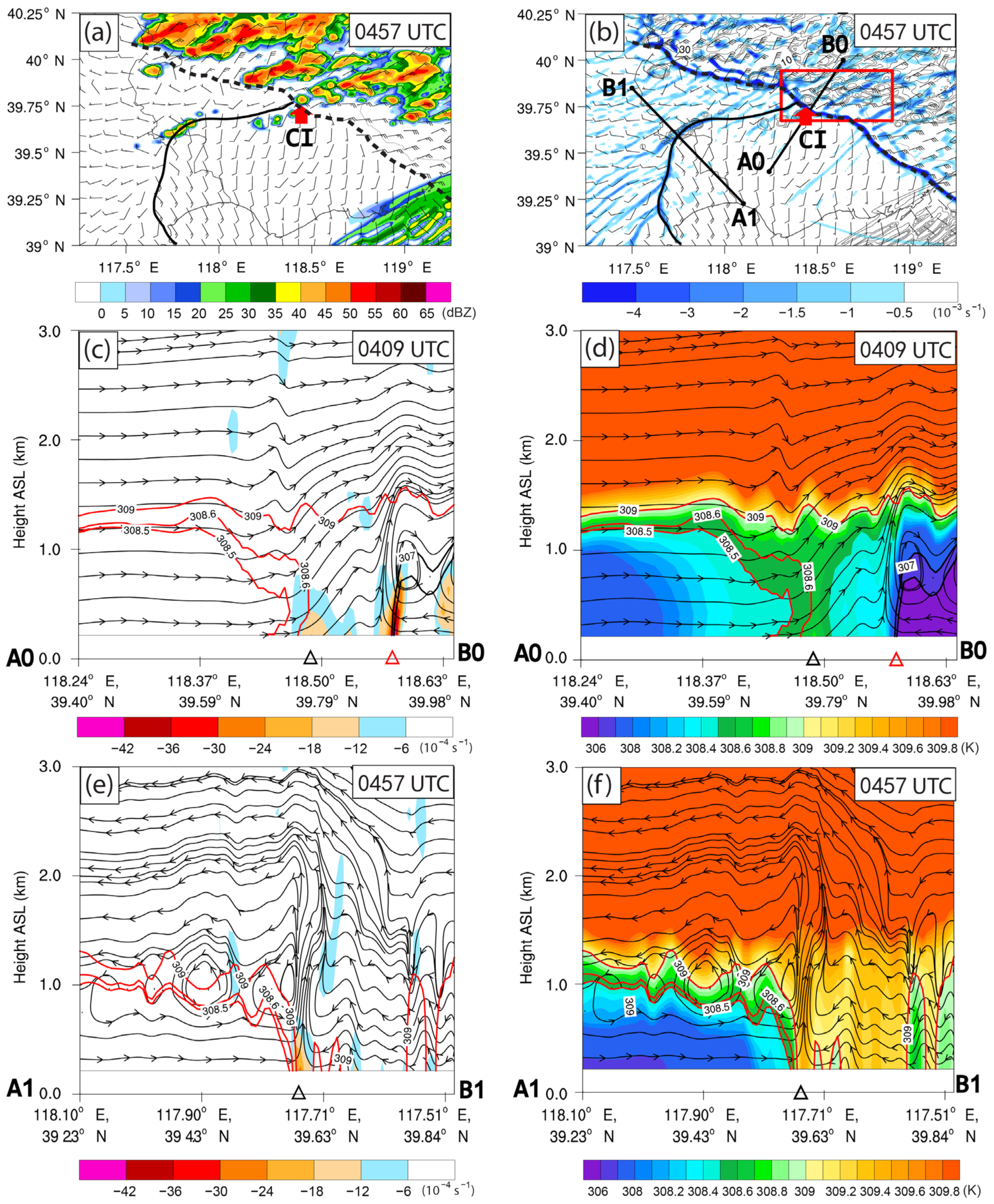
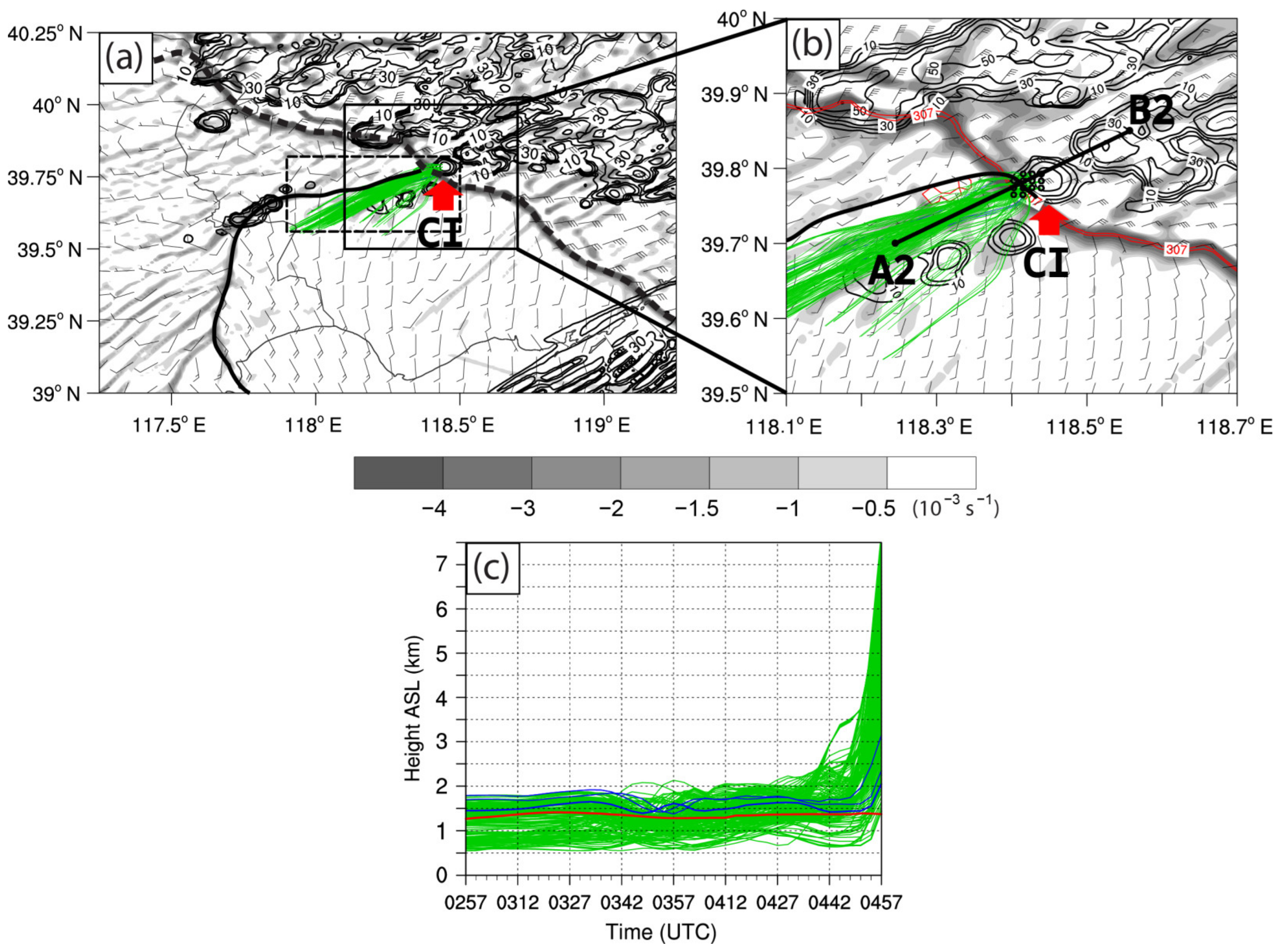
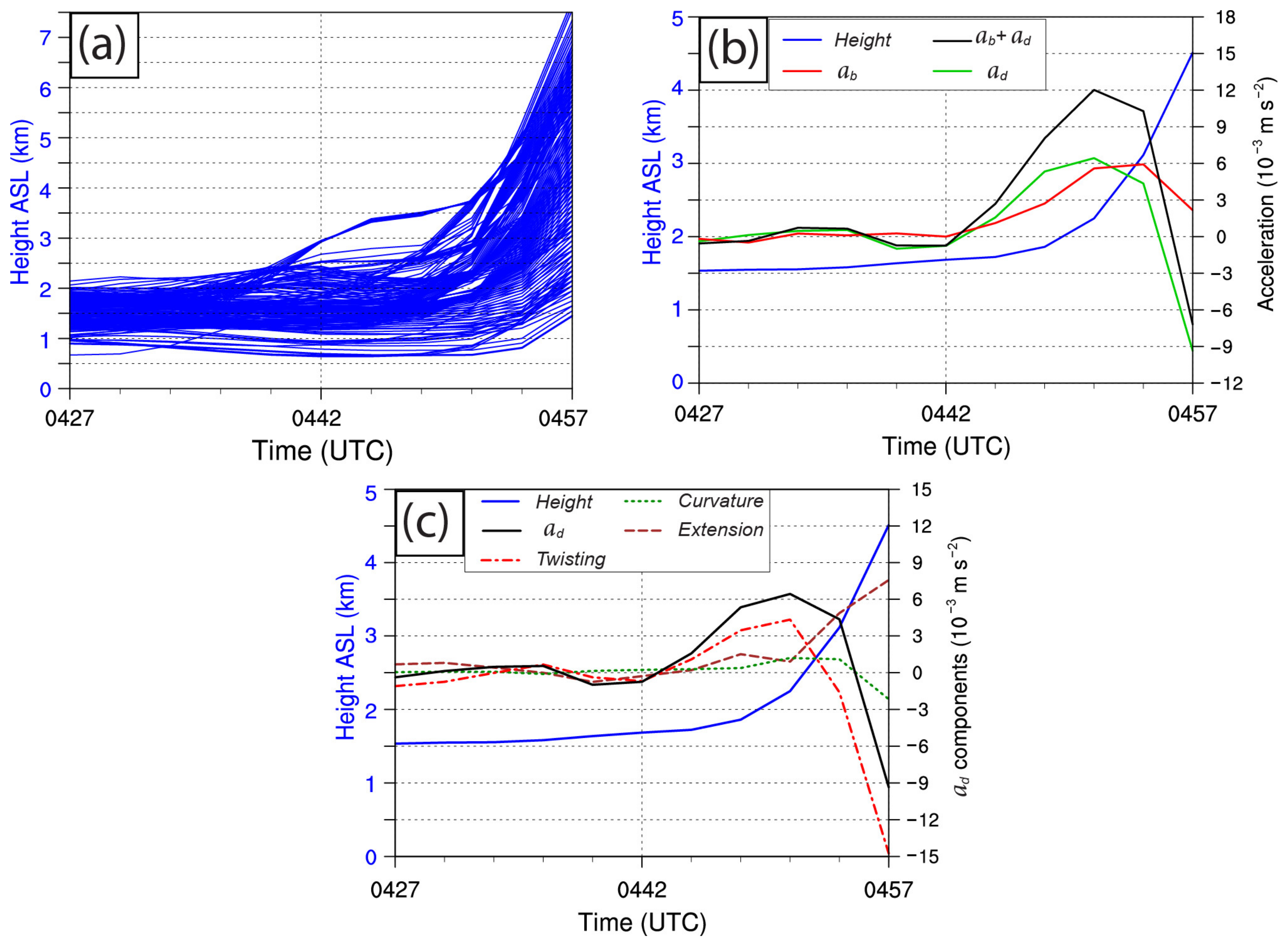
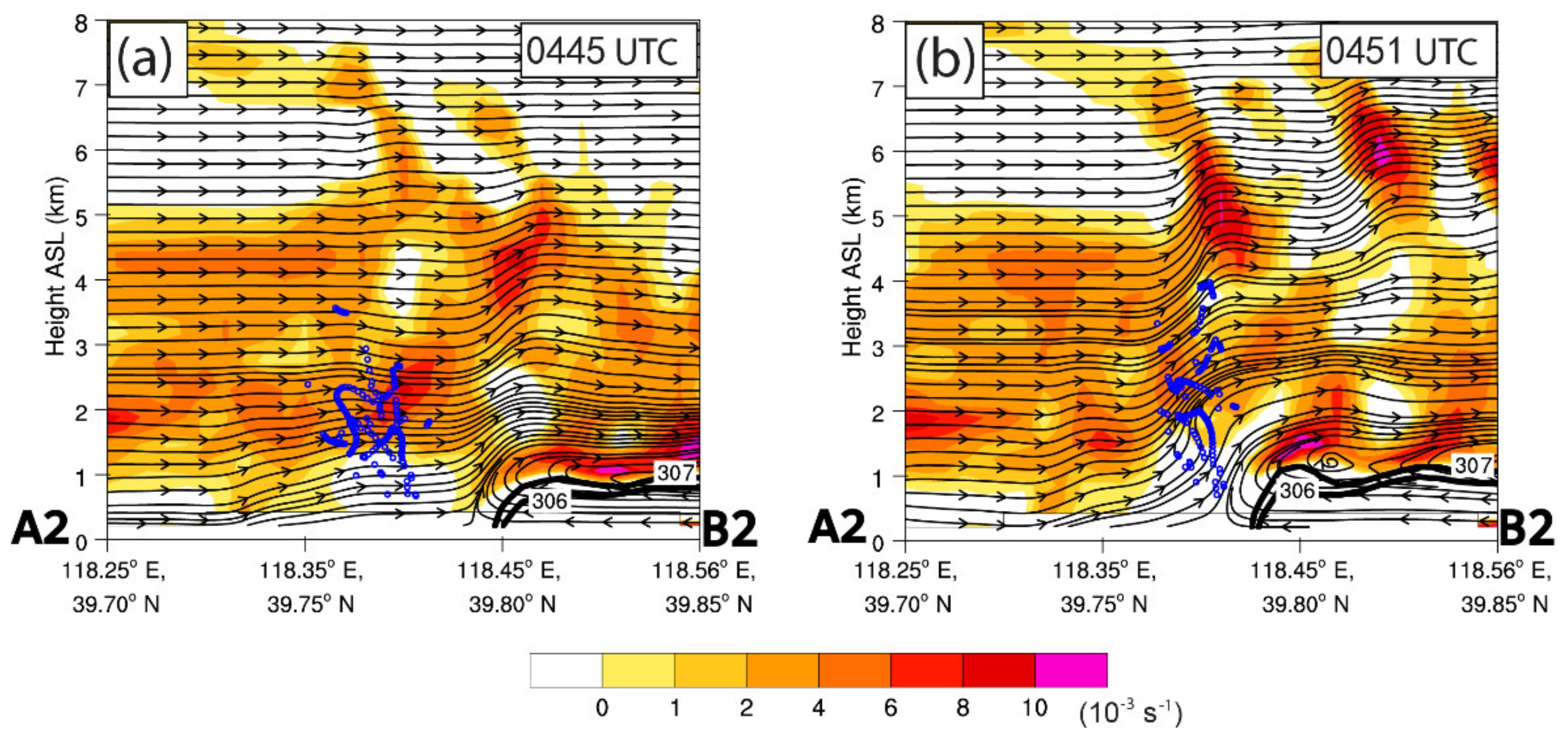
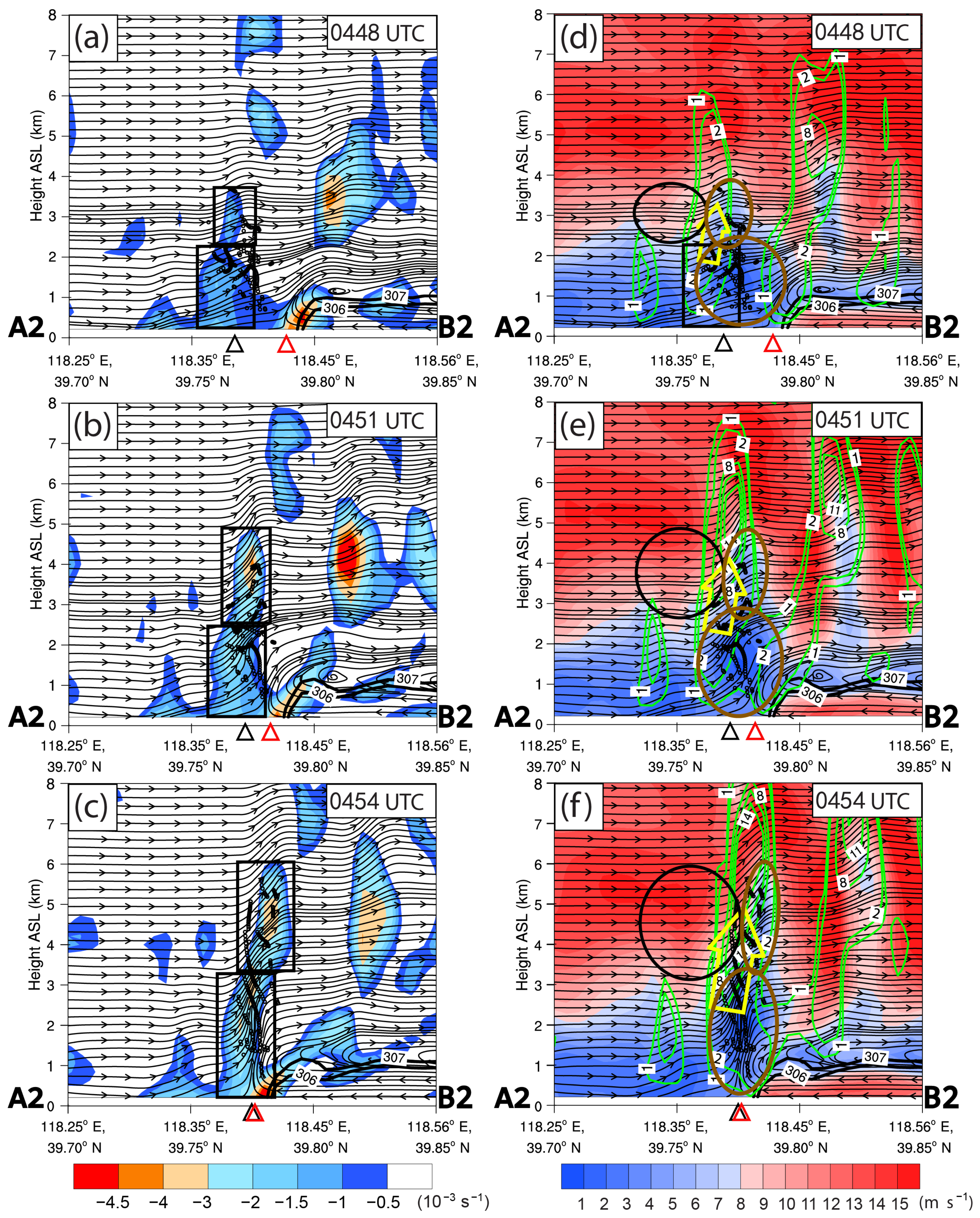
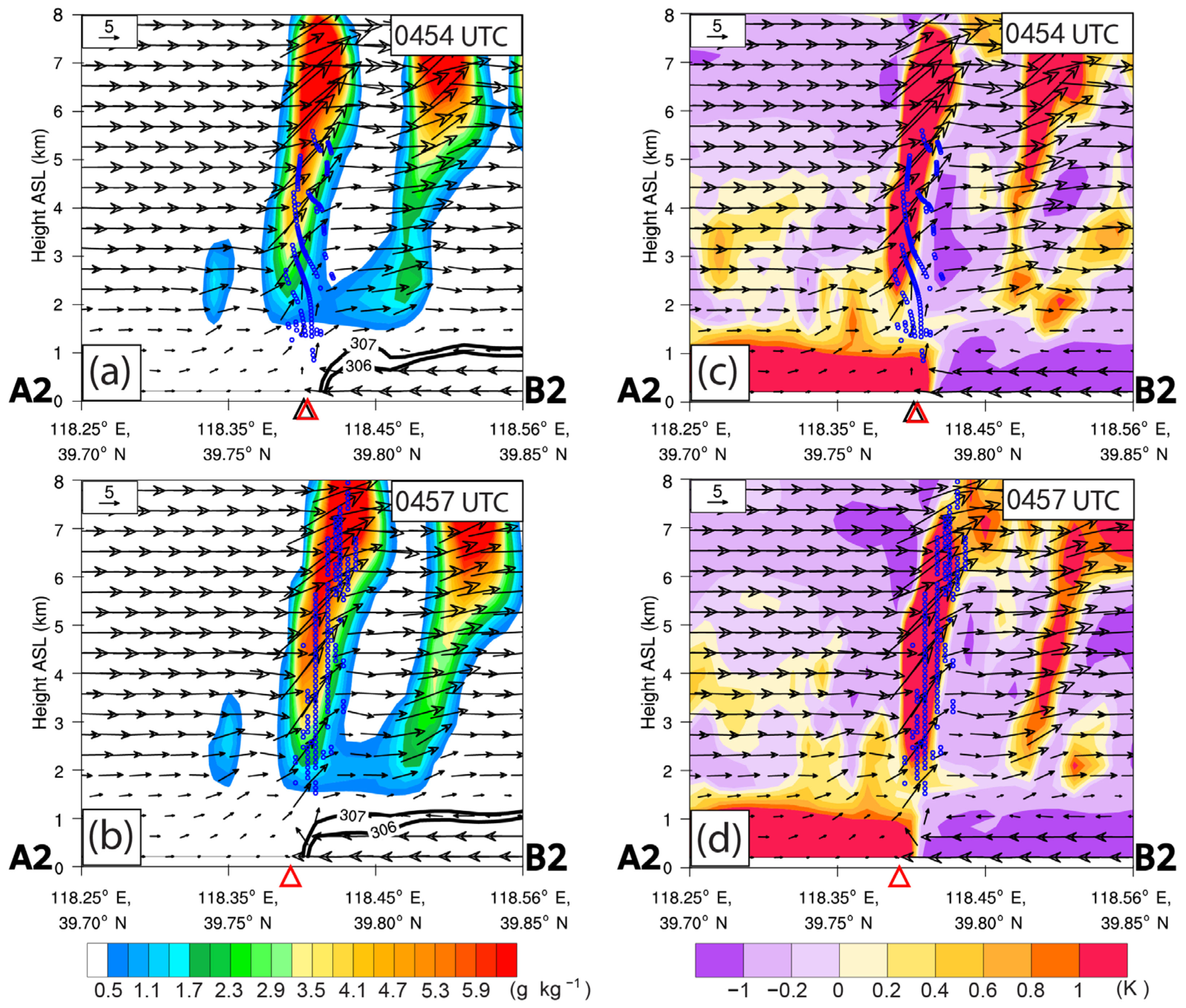
4. Convection Initiation
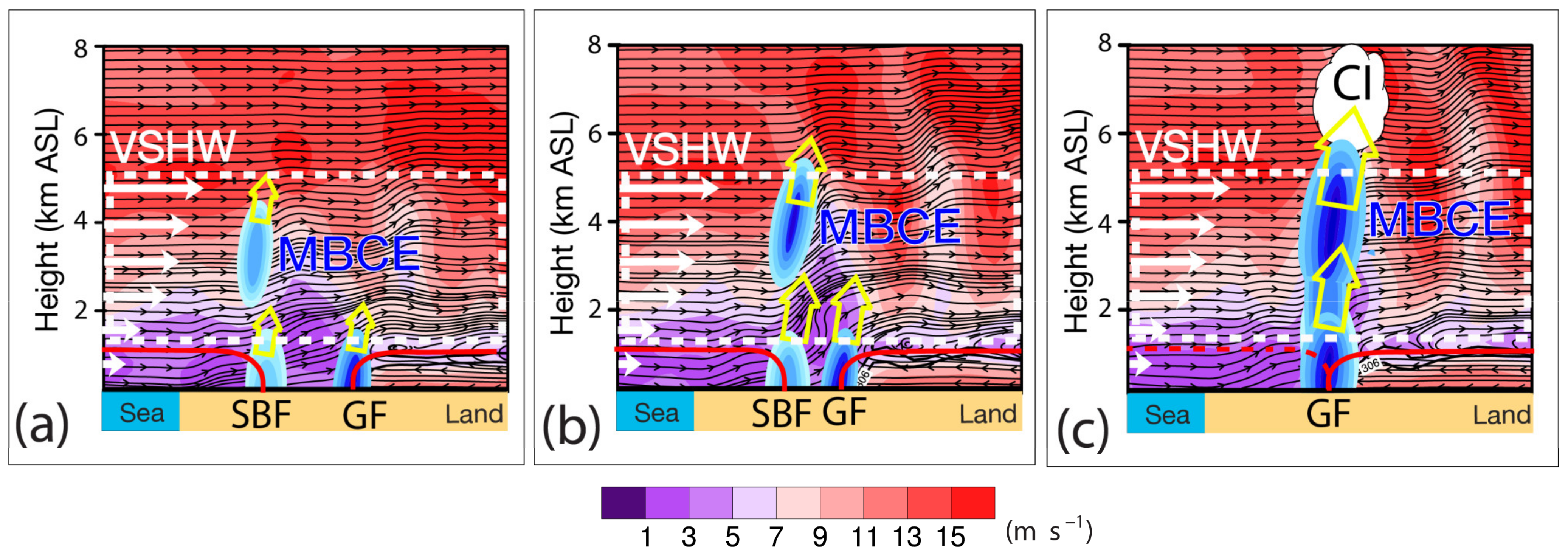
5. Summary and Discussion
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lock, N.A.; Houston, A.L. Empirical examination of the factors regulating thunderstorm initiation. Mon. Weather Rev. 2014, 142, 240–258. [Google Scholar] [CrossRef]
- Ukkonen, P.; Manzato, A.; Makela, A. Evaluation of thunderstorm predictors for Finland using reanalyses and neural networks. J. Appl. Meteorol. Climatol. 2017, 56, 2335–2352. [Google Scholar] [CrossRef]
- Du, Y.; Chen, G. Heavy rainfall associated with double low-level jets over Southern China. Part II: Convection initiation. Mon. Weather Rev. 2019, 147, 543–565. [Google Scholar] [CrossRef]
- Abulikemu, A.; Ming, J.; Xu, X.; Zhuge, X.; Wang, Y.; Zhang, Y.; Zhang, S.; Yu, B.; Aireti, M. Mechanisms of Convection Initiation in the Southwestern Xinjiang, Northwest China: A Case Study. Atmosphere 2020, 11, 1335. [Google Scholar] [CrossRef]
- Lin, G.; Grasmick, C.; Geerts, B.; Wang, Z.; Deng, M. Convection initiation and bore formation following the collision of mesoscale boundaries over a developing stable boundary layer: A case study from PECAN. Mont. Weather Rev. 2021, 149, 2351–2367. [Google Scholar] [CrossRef]
- Kong, M.; Abulikemu, A.; Zheng, J.; Aireti, M.; An, D. A Case Study on Convection Initiation Associated with Horizontal Convective Rolls over Ili River Valley in Xinjiang, Northwest China. Water 2022, 14, 1017. [Google Scholar] [CrossRef]
- Markowski, P.; Convective Storm Initiation and Organization. Atmospheric Convection: Research and Operational Forecasting Aspects; CISM International Centre for Mechanical Sciences, 475; Giaiotti, D.B., Steinacker, R., Stel, F., Eds.; Springer: Vienna, Austria, 2007. [Google Scholar] [CrossRef]
- Wilhelmson, R.B.; Chen, C.S. A simulation of the development of successive cells along a cold outflow boundary. J. Atmos. Sci. 1982, 39, 1466–1483. [Google Scholar] [CrossRef]
- Wilson, J.W.; Schreiber, W.E. Initiation of convective storms at radar-observed boundary-layer convergence lines. Mon. Weather Rev. 1986, 114, 2516–2536. [Google Scholar] [CrossRef]
- Harrison, S.J.; Mecikalski, J.R.; Knupp, K.R. Analysis of outflow boundary collisions in North-Central Alabama. Weather Forecast 2009, 24, 1680–1690. [Google Scholar] [CrossRef]
- Lompar, M.; Curic, M.; Romanic, D. Implementation of a gust front head collapse scheme in the WRF numerical model. Atmos. Res. 2018, 203, 231–245. [Google Scholar] [CrossRef]
- Grasmick, C.; Geerts, B.; Turner, D.D.; Wang, Z.; Weckwerth, T.M. The Relation between Nocturnal MCS Evolution and Its Outflow Boundaries in the Stable Boundary Layer: An Observational Study of the 15 July 2015 MCS in PECAN. Mon. Weather Rev. 2018, 146, 3203–3226. [Google Scholar] [CrossRef]
- Bai, L.; Meng, Z.; Huang, Y.; Zhang, Y.; Niu, S.; Su, T. Convection Initiation Resulting From the Interaction Between a Quasi-Stationary Dryline and Intersecting Gust Fronts: A Case Study. J. Geophys. Res. Atmos. 2019, 124, 2379–2396. [Google Scholar] [CrossRef]
- Abulikemu, A.; Wang, Y.; Gao, R.; Wang, Y.; Xu, X. A numerical study of convection initiation associated with a gust front in Bohai Bay region, North China. J. Geophys. Res. Atmos. 2019, 124, 843–860. [Google Scholar] [CrossRef]
- Hirt, M.; Craig, G.C.; Schäfer, S.A.K.; Savre, J.; Heinze, R. Cold-pool-driven convective initiation: Using causal graph analysis to determine what convection-permitting models are missing. Q. J. R. Meteorol. Soc. 2020, 146, 2205–2227. [Google Scholar] [CrossRef]
- Simpson, J.E. Sea Breeze and Local Wind; Cambridge University Press: Cambridge, UK, 1994; p. 234. [Google Scholar]
- Xian, Z.J.; Pielke, R.A. The Effects of Width of Landmasses on the Development of Sea Breezes. J. Appl. Meteorol. 1991, 30, 1280–1304. [Google Scholar] [CrossRef]
- Lee, O.; Shun, C.M. Observation of sea breeze interactions at and near Hong Kong International Airport. Meteorol. Appl. 2003, 10, 1–9. [Google Scholar] [CrossRef]
- Birch, C.E.; Roberts, M.J.; Garcia-Carreras, L.; Ackerley, D.; Reeder, M.J.; Lock, A.P.; Schiemann, R. Sea-Breeze Dynamics and Convection Initiation: The Influence of Convective Parameterization in Weather and Climate Model Biases. J. Clim. 2015, 28, 8093–8108. [Google Scholar] [CrossRef]
- Wu, F.; Lombardo, K. Precipitation Enhancement in Squall Lines Moving Over Mountainous Coastal Regions. J. Atmos. Sci. 2021, 78, 3089–3113. [Google Scholar] [CrossRef]
- Bergemann, M.; Jakob, C.; Lane, T.P. 2015: Global detection and analysis of coastline-associated rainfall using an objective pattern recognition technique. J. Clim. 2015, 28, 7225–7236. [Google Scholar] [CrossRef]
- Plant, R.S.; Keith, G.J. Occurrence of Kelvin-Helmholtz Billows in Sea-breeze Circulations. Bound.-Layer Meteorol. 2007, 122, 1–15. [Google Scholar] [CrossRef]
- Browand, F.K.; Troutt, T.R. A note on spanwise structure in the two-dimensional mixing layer. J. Fluid Mech. 1980, 97, 771–781. [Google Scholar] [CrossRef]
- Lasheras, J.C.; Choi, H. Three-dimensional instability of a plane free shear layer: An experimental study of the formation and evolution of streamwise vortices. J. Fluid Mech. 1988, 189, 53–86. [Google Scholar] [CrossRef]
- Schowalter, D.G.; Van Van Atta, C.W.; Lasheras, J.C. A study of streamwise vortex structure in a stratified shear layer. J. Fluid Mech. 1994, 281, 247–291. [Google Scholar] [CrossRef]
- Simpson, J.E. Gravity Currents in the Environment and the Laboratory; Cambridge University Press: New York, NY, USA, 1997; p. 244. [Google Scholar]
- Buckley, R.L.; Kurzeja, R.J. An Observational and Numerical Study of the Nocturnal Sea Breeze. Part I: Structure and Circulation. J. Appl. Meteorol. 1997, 36, 1577–1598. [Google Scholar] [CrossRef]
- Miller, S.; Keim, B.; Talbot, R.; Mao, H. Sea breeze: Structure, forecasting, and impacts. Rev. Geophys. 2003, 41, 1011. [Google Scholar] [CrossRef]
- Crosman, E.T.; Horel, J.D. Sea and lake breezes: A review of numerical studies. Bound.-Layer Meteorol. 2010, 137, 1–29. [Google Scholar] [CrossRef]
- Chen, G.; Iwai, H.; Ishii, S.; Saito, K.; Seko, H.; Sha, W.; Iwasaki, T. Structures of the sea-breeze front in dual-Doppler lidar observation and coupled mesoscale-to-LES modeling. J. Geophys. Res. Atmos. 2019, 124, 2397–2413. [Google Scholar] [CrossRef]
- Purdom, J.F.W.; Marcus, K. Thunderstorm trigger mechanisms over the southeast U.S. preprints. In Proceedings of the 12th Conference on Severe Local Storms, San Antonio, TX, USA, 12–15 January 1982; pp. 487–488. [Google Scholar]
- Nicholls, M.E.; Pielke, R.A.; Cotton, W.R. A Two-Dimensional Numerical Investigation of the interaction between Sea Breezes and Deep Convection over the Florida Peninsula. Mon. Weather Rev. 1991, 119, 298–323. [Google Scholar] [CrossRef][Green Version]
- Fankhauser, J.C.; Crook, N.A.; Tuttle, J.; Miller, L.J.; Wade, C.G. Initiation of Deep Convection Along Boundary-Layer Convergence Lines in a Semitropical Environment. Mon. Weather Rev. 1995, 123, 291–313. [Google Scholar] [CrossRef]
- Kingsmill, D.E.; Crook, N.A. An observational study of atmospheric bore formation from colliding density currents. Mon. Weather Rev. 2003, 131, 2985–3002. [Google Scholar] [CrossRef]
- Carbone, R.E.; Wilson, J.W.; Keenan, T.D.; Hacker, J.M. Tropical island convection in the absence of significant topography. Part I: Life cycle of diurnally forced convection. Mon. Weather Rev. 2000, 128, 3459–3480. [Google Scholar] [CrossRef]
- Kingsmill, D.E. Convection Initiation Associated with a Sea-Breeze Front, a Gust Front, and Their Collision. Mon. Weather Rev. 1995, 123, 2913–2933. [Google Scholar] [CrossRef]
- Abulikemu, A.; Xu, X.; Wang, Y.; Ding, J.; Wang, Y. Atypical Occlusion Process Caused by the Merger of a Sea-breeze Front and Gust Front. Adv. Atmos. Sci. 2015, 32, 1431–1443. [Google Scholar] [CrossRef]
- Abulikemu, A.; Xu, X.; Wang, Y.; Ding, J.; Zhang, S.; Shen, W. A modeling study of convection initiation prior to the merger of a sea-breeze front and a gust front. Atmos. Res. 2016, 182, 10–19. [Google Scholar] [CrossRef]
- Mahoney, W.P. Gust front characteristics and the kinematics associated with interacting thunderstorm outflows. Mon. Weather Rev. 1988, 116, 1474–1491. [Google Scholar] [CrossRef]
- Weckwerth, T.M.; Parsons, D.B. A review of convection initiation and motivation for IHOP_2002. Mon. Weather Rev. 2006, 134, 5–22. [Google Scholar] [CrossRef]
- Schultz, D.M. Review of cold fronts with prefrontal troughs and wind shifts. Mon. Weather Rev. 2005, 133, 2449–2472. [Google Scholar] [CrossRef]
- Rotunno, R.; Klemp, J.B.; Weisman, M.L. A theory for strong, long-lived squall lines. J. Atmos. Sci. 1988, 46, 463–485. [Google Scholar] [CrossRef]
- Skamarock, W.C.; Klemp, J.B.; Dudhia, J.; Gill, D.O.; Liu, Z.; Berner, J.; Wang, W.; Powers, J.G.; Duda, M.G.; Barker, D.M.; et al. A Description of the Advanced Research WRF Model Version 4.1 (No. NCAR/TN-556+STR); National Center for Atmospheric Research: Boulder, CO, USA, 2019. [Google Scholar] [CrossRef]
- Xu, X.; Xue, M.; Wang, Y. The genesis of mesovortices within a real-data simulation of a bow echo system. J. Atmos. Sci. 2015, 72, 1963–1986. [Google Scholar] [CrossRef]
- Lim, K.-S.S.; Hong, S.-Y. Development of an effective double-moment cloud microphysics scheme with prognostic cloud condensation nuclei (CCN) for weather and climate models. Mon. Weather Rev. 2010, 138, 1587–1612. [Google Scholar] [CrossRef]
- Hong, S.Y.; Lim, J.O. The WRF single-moment 6-class microphysics scheme (WSM6). J. Korean Meteorol. Soc. 2006, 42, 129–151. Available online: https://www2.mmm.ucar.edu/wrf/users/workshops/WS2006/abstracts/PSession05/P5_4_Hong.pdf (accessed on 5 April 2020).
- Dudhia, J. Numerical study of convection observed during the winter monsoon experiment using a mesoscale two-dimensional model. J. Atmos. Sci. 1989, 46, 3077–3107. [Google Scholar] [CrossRef]
- Mlawer, E.J.; Taubman, S.J.; Brown, P.D.; Iacono, M.J.; Clough, S.A. Radiative transfer for inhomogeneous atmospheres:RRTM, a validated correlated-k model for the longwave. J. Geophys. Res. Atmos. 1997, 102, 16663–16682. [Google Scholar] [CrossRef]
- Tewari, M.; Chen, F.; Wang, W.; Dudhia, J.; LeMone, M.A.; Mitchell, K.; Ek, M.; Gayno, G.; Wegiel, J.; Cuenca, R.H. Implementation and verification of the unified NOAH land surface model in the WRF model. In Proceedings of the 20th Conference on Weather Analysis and Forecasting/16th Conference on Numerical Weather Prediction, Seattle, WA, USA, 12–16 January 2004; Available online: https://ams.confex.com/ams/84Annual/techprogram/paper_69061.htm. (accessed on 5 April 2020).
- Kain, J.S. The Kain–Fritsch convective parameterization: An update. J. Appl. Meteorol. 2004, 43, 170–181. [Google Scholar] [CrossRef]
- Xu, X.; Xue, M.; Wang, Y.; Huang, H. Mechanisms of secondary convection within a Mei-Yu frontal mesoscale convective system in eastern China. J. Geophys. Res. Atmos. 2017, 122, 47–64. [Google Scholar] [CrossRef]
- Jeevanjee, N.; Romps, D.M. Effective buoyancy, inertial pressure, and the mechanical generation of boundary layer mass flux by cold pools. J. Atmos. Sci. 2015, 72, 3199–3213. [Google Scholar] [CrossRef][Green Version]
- Davies-Jones, R. An expression for effective buoyancy in surroundings with horizontal density gradients. J. Atmos. Sci. 2003, 60, 2922–2925. [Google Scholar] [CrossRef]
- Torri, G.; Kuang, Z.M.; Tian, Y. Mechanisms for convection triggering by cold pools. Geophys. Res. Lett. 2015, 42, 1943–1950. [Google Scholar] [CrossRef]
- Adams, J.C. MUDPACK: Multigrid portable Fortran software for the efficient solution of linear elliptic partial differential equations. Appl. Math. Comput. 1989, 34, 113–146. [Google Scholar] [CrossRef]
- Schenkman, A.D.; Xue, M.; Dawson, D.T., II. The cause of internal outflow surges in a high-resolution simulation of the 8 May 2003 Oklahoma City tornadic supercell. J. Atmos. Sci. 2016, 73, 353–370. [Google Scholar] [CrossRef]
- Dawson, D.T.; Xue, M.; Shapiro, A.; Milbrandt, J.A.; Schenkman, A.D. Sensitivity of real-data simulations of the 3 May 1999 Oklahoma City tornadic supercell and associated tornadoes to multimoment microphysics. Part II: Analysis of buoyancy and dynamic pressure forces in simulated tornado-like vortices. J. Atmos. Sci. 2016, 73, 1039–1061. [Google Scholar] [CrossRef]
- Klemp, J.B.; Rotunno, R. A study of the tornadic region within a supercell thunderstorm. J. Atmos. Sci. 1983, 40, 359–377. [Google Scholar] [CrossRef]
- Wakimoto, R.M.; Atkins, N.T. Observations of the sea-breeze front during Cape. 1. Single-Doppler, satellite, and cloud photogrammetry analysis. Mon. Weather Rev. 1994, 122, 1092–1114. [Google Scholar] [CrossRef][Green Version]
- Dailey, P.S.; Fovell, R.G. Numerical simulation of the interaction between the sea-breeze front and horizontal convective rolls.Part I: Offshore ambient flow. Mon. Weather Rev. 1999, 127, 858–878. [Google Scholar] [CrossRef]
- Fovell, R.G.; Dailey, P.S. Numerical simulation of the interaction between the sea-breeze front and horizontal convective rolls.Part II: Alongshore ambient flow. Mon. Weather Rev. 2001, 129, 2057–2072. [Google Scholar] [CrossRef]
- Fovell, R.G. Upstream influence of numerically simulated squall-line storms. Q. J. R. Meteorol. Soc. 2002, 128, 893–912. [Google Scholar] [CrossRef]
- Fovell, R.G.; Mullendore, G.L.; Kim, S.H. Discrete propagation in numerically simulated nocturnal squall lines. Mon. Weather Rev. 2006, 134, 3735–3752. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zheng, J.; Abulikemu, A.; Wang, Y.; Kong, M.; Liu, Y. Convection Initiation Associated with the Merger of an Immature Sea-Breeze Front and a Gust Front in Bohai Bay Region, North China: A Case Study. Atmosphere 2022, 13, 750. https://doi.org/10.3390/atmos13050750
Zheng J, Abulikemu A, Wang Y, Kong M, Liu Y. Convection Initiation Associated with the Merger of an Immature Sea-Breeze Front and a Gust Front in Bohai Bay Region, North China: A Case Study. Atmosphere. 2022; 13(5):750. https://doi.org/10.3390/atmos13050750
Chicago/Turabian StyleZheng, Jingjing, Abuduwaili Abulikemu, Yan Wang, Meini Kong, and Yiwei Liu. 2022. "Convection Initiation Associated with the Merger of an Immature Sea-Breeze Front and a Gust Front in Bohai Bay Region, North China: A Case Study" Atmosphere 13, no. 5: 750. https://doi.org/10.3390/atmos13050750
APA StyleZheng, J., Abulikemu, A., Wang, Y., Kong, M., & Liu, Y. (2022). Convection Initiation Associated with the Merger of an Immature Sea-Breeze Front and a Gust Front in Bohai Bay Region, North China: A Case Study. Atmosphere, 13(5), 750. https://doi.org/10.3390/atmos13050750





