Numerical Study on Thermal-Hydraulic Performance of Printed Circuit Heat Exchangers during Natural Gas Trans-Critical Liquefaction
Abstract
:1. Introduction
2. Numerical Model
2.1. Computational Domain
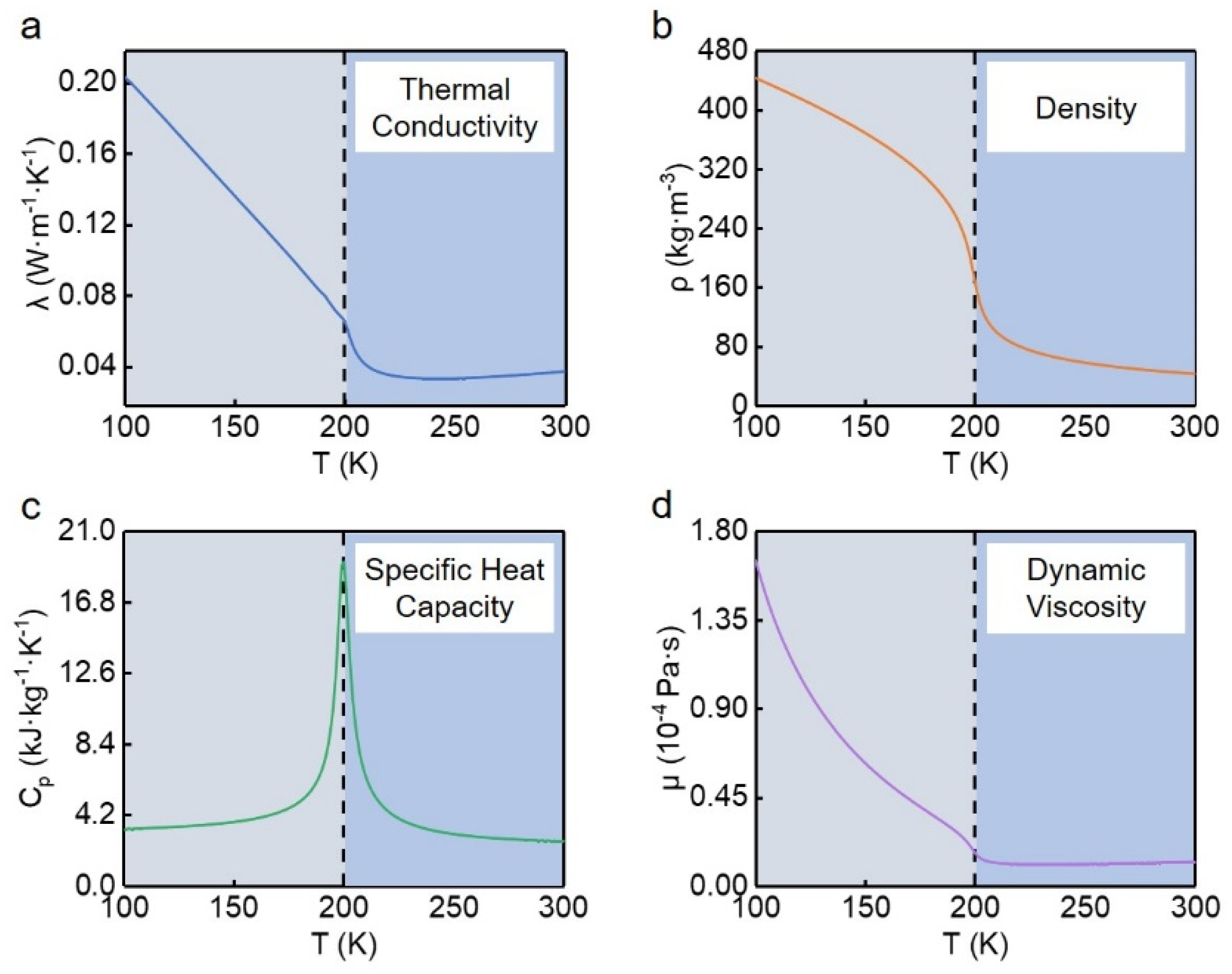
2.2. Computational Model
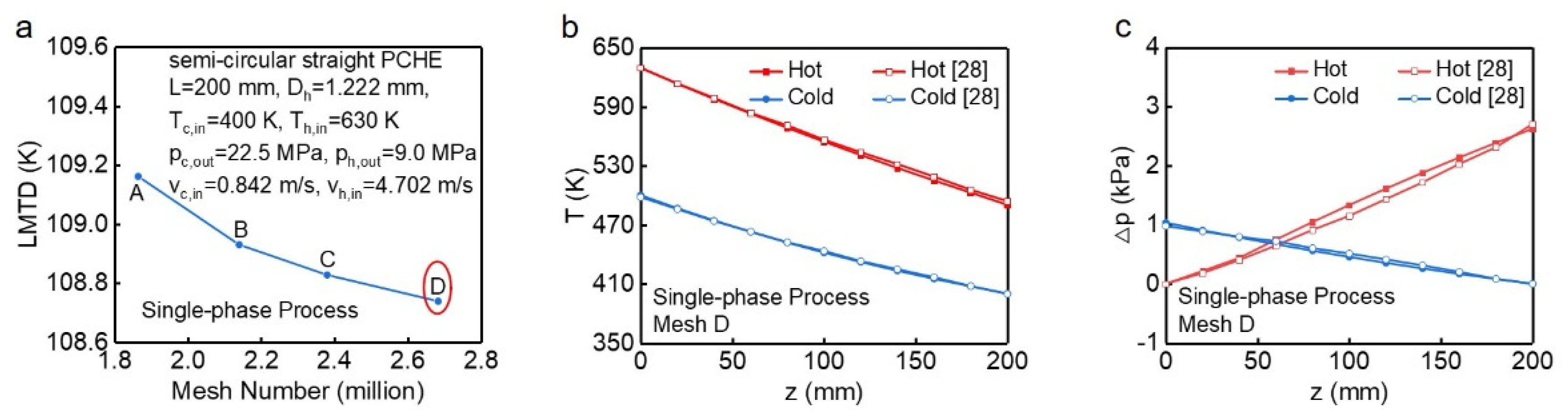
2.3. Validation
2.4. Mesh Independence Test
3. Results and Discussion
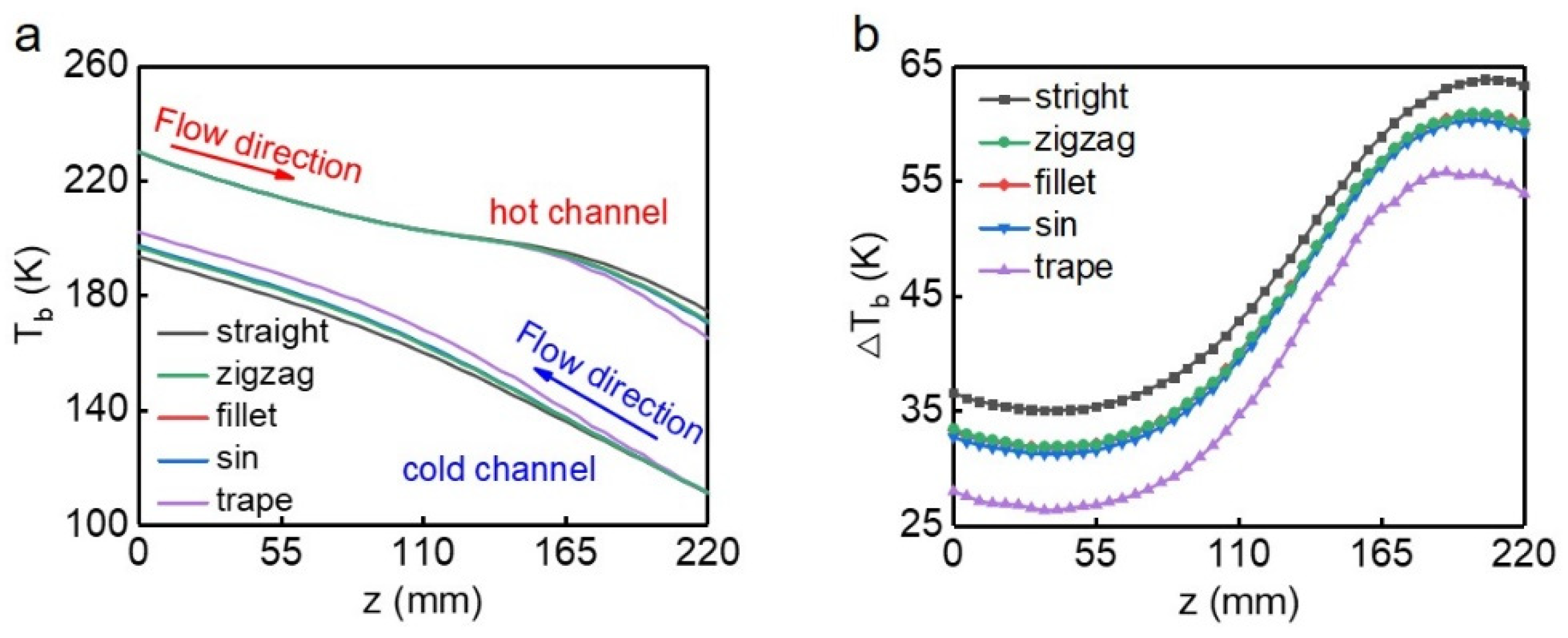
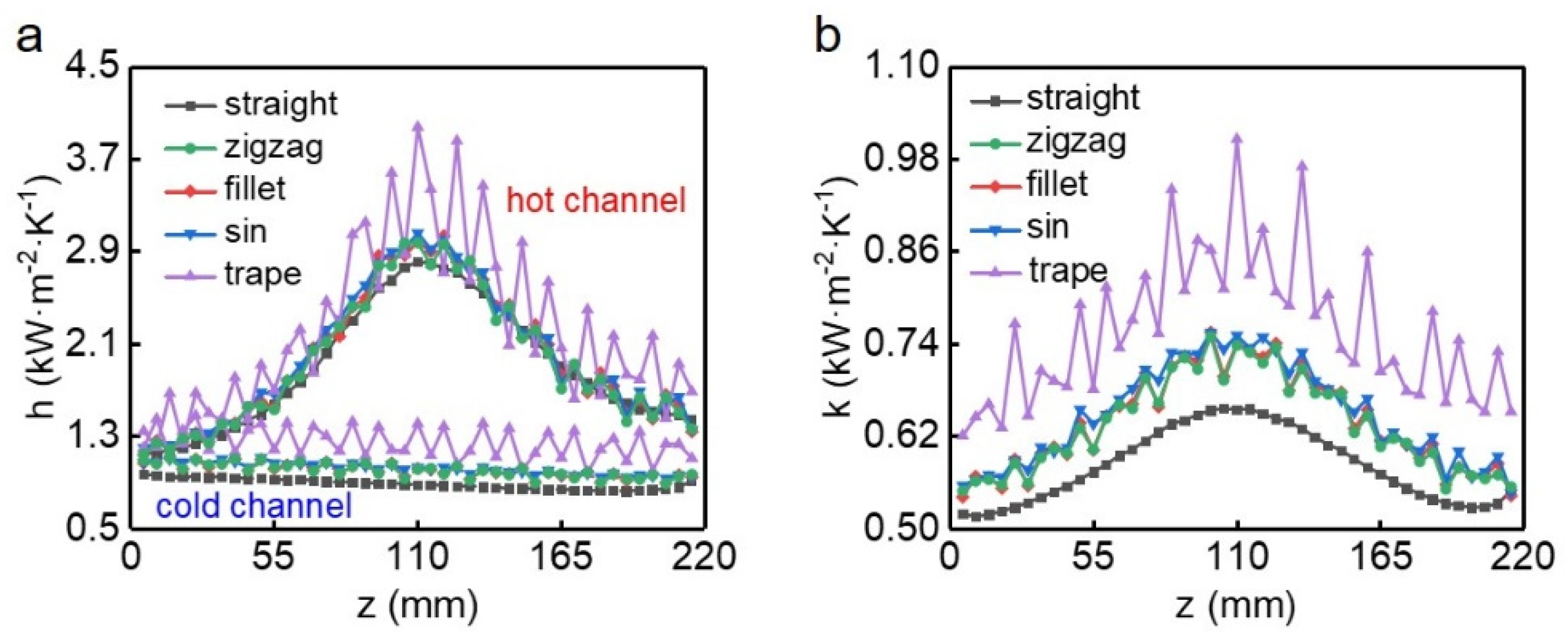
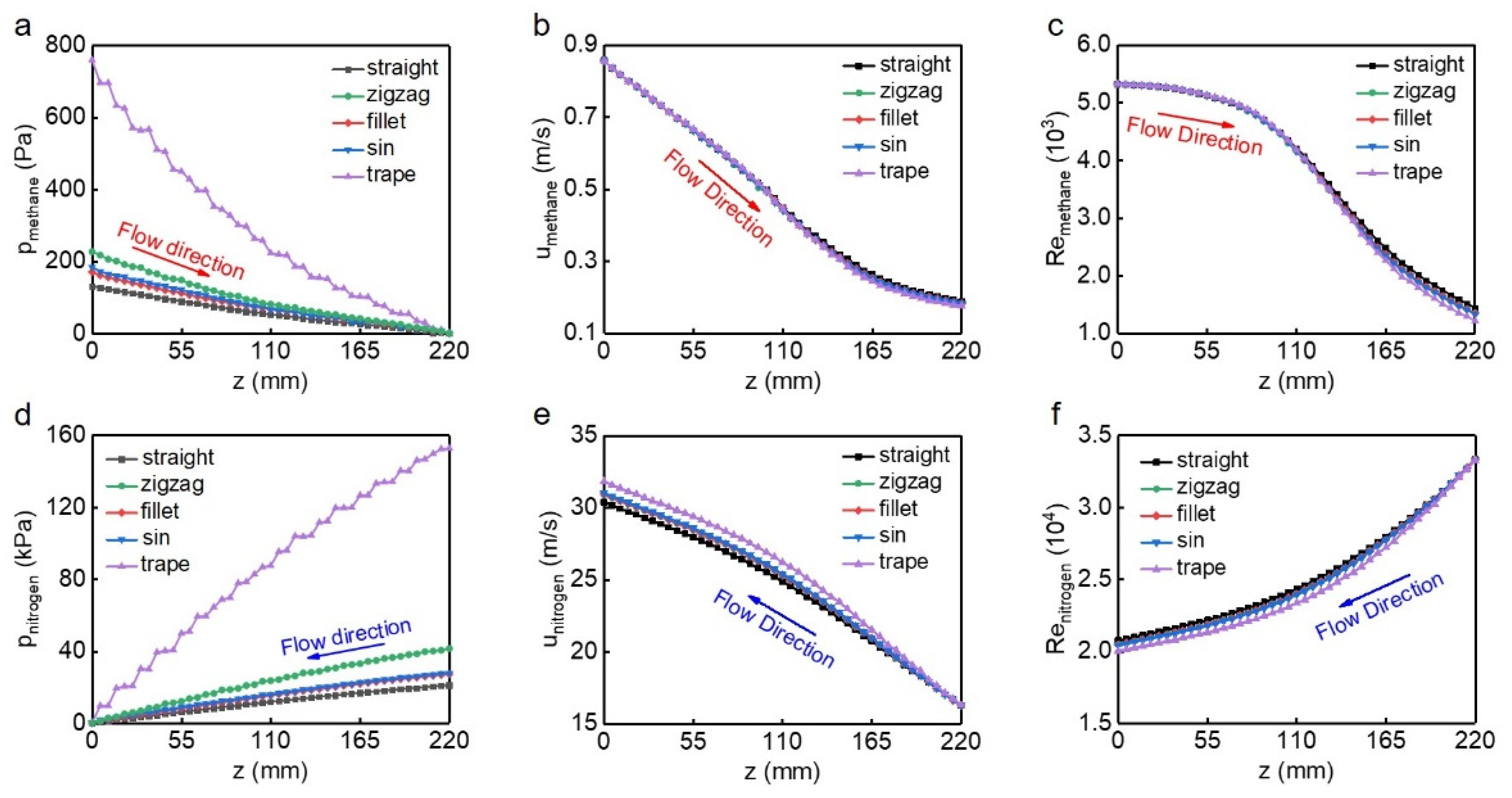
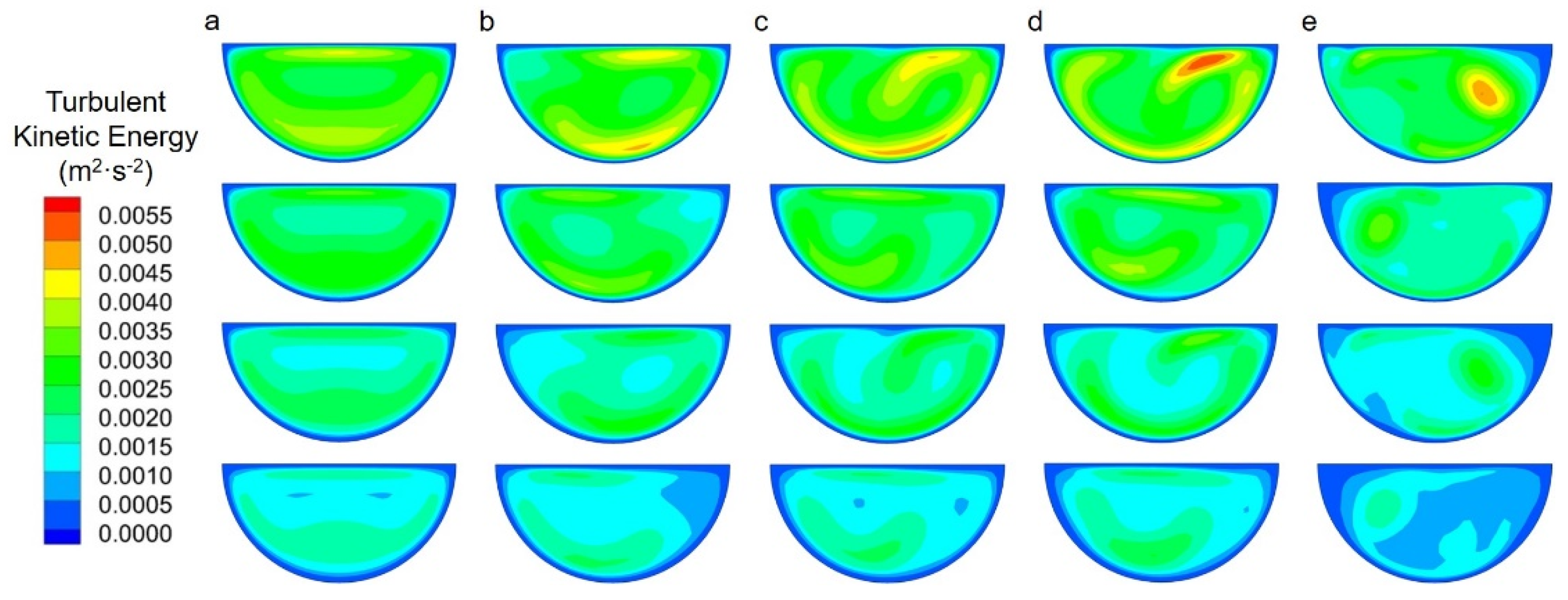
3.1. Heat Transfer Characteristics
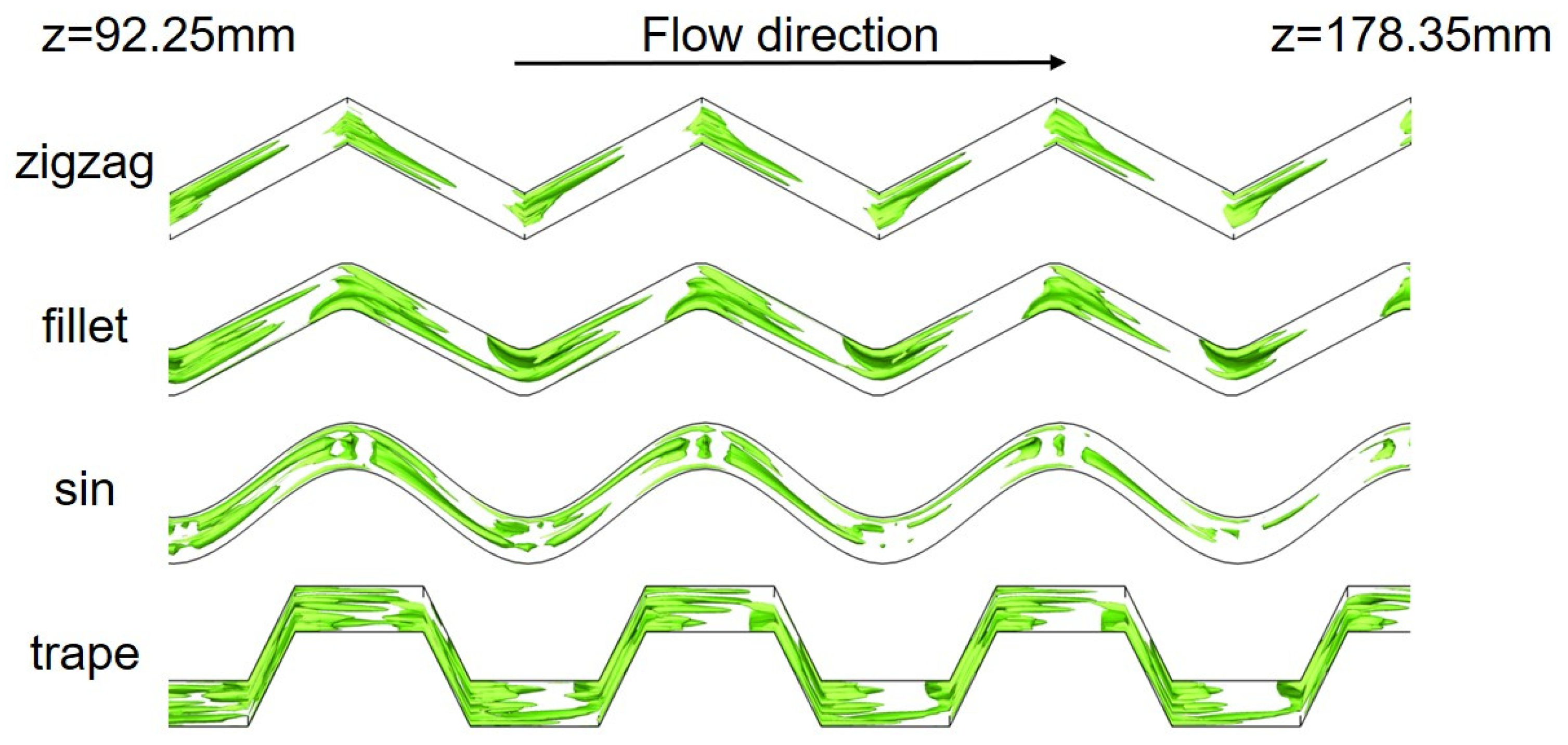
3.2. Flow Characteristics
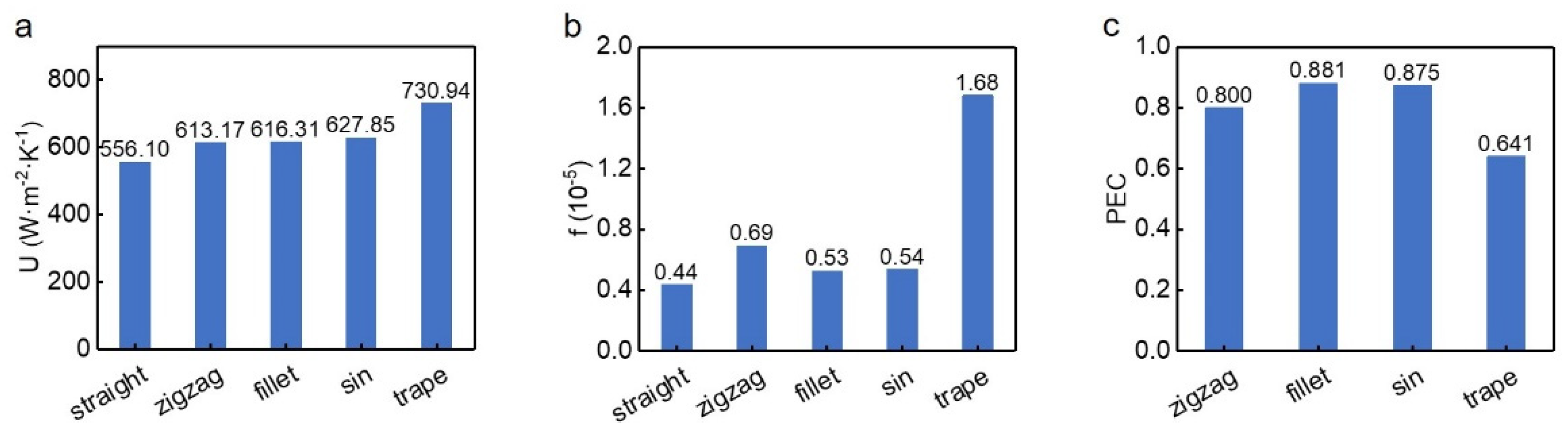
3.3. Comprehensive Performance
4. Conclusions
- (1)
- The local heat transfer coefficients peak near the pseudo-critical point of NG among all the channels. Additionally, all the wavy channels can improve the heat transfer performance of PCHEs, where the largest enhancement is achieved by the trapezoidal channel. Compared with the straight channel, the local heat transfer coefficient will be increased by up to 53% in the trapezoidal channel.
- (2)
- The vortex will greatly increase the local pressure drop gradient in the wavy channels. The trapezoidal channel has the largest pressure loss which is 3.0 times larger than that of the remaining three wavy channels and nearly 4.5 times larger than that of the straight channel.
- (3)
- All the wavy channels can improve the performance of heat transfer by 10.26%, 10.83%, 12.90% and 31.44%, respectively; however, the benefit of the improvement cannot offset the penalty of flow deterioration.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| A | amplitude (mm) |
| cp | specific heat (J/kg K) |
| f | Fanning friction factor |
| FLNG | floating liquefied natural gas |
| h | local convective heat transfer coefficient (W/m2 K) |
| k | local heat transfer coefficient (W/m2 K) |
| Lflow | channel length (mm) |
| Lsw | sweep length (mm) |
| LMTD | logarithmic mean temperature difference |
| LNG | liquefied natural gas |
| NG | natural gas |
| p | pressure (Pa) |
| PCHEs | printed circuit heat exchangers |
| PEC | performance evaluation criteria |
| r | chamfer radius (mm) |
| SST | SST k–ω model |
| T | bulk temperature (°C) |
| u | velocity (m/s) |
| U | average surface heat transfer coefficient (W/m2 K) |
| Greek | |
| λ | half wavelength (mm) |
| μ | dynamic viscosity (kg/m s) |
| ρ | density (kg/m3) |
References
- Basak, M.; Perrons, R.K.; Coffey, V. Schedule overruns as a barrier for liquefied natural gas projects: A review of the literature and research agenda. Energy Rep. 2019, 5, 210–220. [Google Scholar] [CrossRef]
- Zhang, J.; Tan, Y.; Zhang, T.; Yu, K.; Wang, X.; Zhao, Q. Natural gas market and underground gas storage development in China. J. Energy Storage 2020, 29, 101338. [Google Scholar] [CrossRef]
- Gowid, S.; Dixon, R.; Ghani, S. Profitability, reliability and condition based monitoring of LNG floating platforms: A review. J. Nat. Gas Sci. Eng. 2015, 27, 1495–1511. [Google Scholar] [CrossRef] [Green Version]
- Peng, Z.-R.; Zheng, Q.-Y.; Chen, J.; Yu, S.-C.; Zhang, X.-R. Numerical investigation on heat transfer and pressure drop characteristics of coupling transcritical flow and two-phase flow in a printed circuit heat exchanger. Int. J. Heat Mass Transf. 2020, 153, 119557. [Google Scholar] [CrossRef]
- Zhu, J.; Sun, C.; Li, Y.; Shan, W. Experiment on adaptability of feed gas flow rate and sea conditions on FLNG spiral wound heat exchanger. Int. J. Heat Mass Transf. 2019, 138, 659–666. [Google Scholar] [CrossRef]
- Lee, S.; Long, N.V.D.; Lee, M. Design and optimization of natural gas liquefaction and recovery processes for offshore floating liquefied natural gas plants. Ind. Eng. Chem. Res. 2012, 51, 10021–10030. [Google Scholar] [CrossRef]
- Wang, K.; Ren, T.; Ding, G. Progress of research and application of printed circuit heat exchanger for offshore LNG platform. J. Refrig. 2016, 37, 70–77. [Google Scholar]
- McCormack, D. In the application of printed circuit heat exchanger technology in the pebble bed modular reactor demonstration plant. In Proceedings of the Turbo Expo: Power for Land, Sea, and Air, American Society of Mechanical Engineers, New Orleans, LA, USA, 4–7 June 2001; p. V003T01A086. [Google Scholar]
- Figley, J.T. Numerical Modeling and Performance Analysis of Printed Circuit Heat Exchanger for Very High-Temperature Reactors. Master’s Thesis, The Ohio State University, Columbus, OH, USA, 2009. [Google Scholar]
- Huang, C.; Cai, W.; Wang, Y.; Liu, Y.; Li, Q.; Li, B. Review on the characteristics of flow and heat transfer in printed circuit heat exchangers. Appl. Therm. Eng. 2019, 153, 190–205. [Google Scholar] [CrossRef]
- Reay, D. Compact heat exchangers: A review of current equipment and R&D in the field. Heat Recovery Syst. CHP 1994, 14, 459–474. [Google Scholar]
- Kim, W.; Baik, Y.-J.; Jeon, S.; Jeon, D.; Byon, C. A mathematical correlation for predicting the thermal performance of cross, parallel, and counterflow PCHEs. Int. J. Heat Mass Transf. 2017, 106, 1294–1302. [Google Scholar] [CrossRef]
- Ma, T.; Li, M.-J.; Xu, J.-L.; Cao, F. Thermodynamic analysis and performance prediction on dynamic response characteristic of PCHE in 1000 MW S-CO2 coal fired power plant. Energy 2019, 175, 123–138. [Google Scholar] [CrossRef]
- Shin, C.W.; No, H.C. Experimental study for pressure drop and flow instability of two-phase flow in the PCHE-type steam generator for SMRs. Nucl. Eng. Des. 2017, 318, 109–118. [Google Scholar] [CrossRef]
- Palko, D.; Anglart, H. Theoretical and numerical study of heat transfer deterioration in high performance light water reactor. Sci. Technol. Nucl. Install. 2008, 2008, 405072. [Google Scholar] [CrossRef] [Green Version]
- Aneesh, A.; Sharma, A.; Srivastava, A.; Chaudhury, P. Effects of wavy channel configurations on thermal-hydraulic characteristics of Printed Circuit Heat Exchanger (PCHE). Int. J. Heat Mass Transf. 2018, 118, 304–315. [Google Scholar] [CrossRef]
- Kwon, D.; Jin, L.; Jung, W.; Jeong, S. Experimental investigation of heat transfer coefficient of mini-channel PCHE (printed circuit heat exchanger). Cryogenics 2018, 92, 41–49. [Google Scholar] [CrossRef]
- Zhao, Z.; Zhao, K.; Jia, D.; Jiang, P.; Shen, R. Numerical investigation on the flow and heat transfer characteristics of supercritical liquefied natural gas in an airfoil fin printed circuit heat exchanger. Energies 2017, 10, 1828. [Google Scholar] [CrossRef] [Green Version]
- Zhang, P.; Ma, T.; Ke, H.; Wang, W.; Lin, Y.; Wang, Q. Numerical investigation on local thermal characteristics of printed circuit heat exchanger for natural gas liquefication. Energy Procedia 2019, 158, 5408–5413. [Google Scholar] [CrossRef]
- Ma, T.; Zhang, P.; Lian, J.; Ke, H.; Wang, W.; Lin, Y.; Wang, Q. Numerical Study on Flow and Heat Transfer Performance of Natural Gas in a Printed Circuit Heat Exchanger During Transcritical Liquefaction. J. Fluids Eng. 2021, 143, 040901. [Google Scholar] [CrossRef]
- Smith, L.; Craig, B. Properties of metallic materials for LNG service. Stainl. Steel World 2001, 13, 27–32. [Google Scholar]
- Li, H.; Kruizenga, A.; Anderson, M.; Corradini, M.; Luo, Y.; Wang, H.; Li, H. Development of a new forced convection heat transfer correlation for CO2 in both heating and cooling modes at supercritical pressures. Int. J. Therm. Sci. 2011, 50, 2430–2442. [Google Scholar] [CrossRef]
- Li, H.; Zhang, Y.; Zhang, L.; Yao, M.; Kruizenga, A.; Anderson, M. PDF-based modeling on the turbulent convection heat transfer of supercritical CO2 in the printed circuit heat exchangers for the supercritical CO2 Brayton cycle. Int. J. Heat Mass Transf. 2016, 98, 204–218. [Google Scholar] [CrossRef]
- Xiang, M.; Guo, J.; Huai, X.; Cui, X. Thermal analysis of supercritical pressure CO2 in horizontal tubes under cooling condition. J. Supercrit. Fluids 2017, 130, 389–398. [Google Scholar] [CrossRef]
- Ren, Z.; Zhao, C.-R.; Jiang, P.-X.; Bo, H.-L. Investigation on local convection heat transfer of supercritical CO2 during cooling in horizontal semicircular channels of printed circuit heat exchanger. Appl. Therm. Eng. 2019, 157, 113697. [Google Scholar] [CrossRef]
- Zhang, H.; Guo, J.; Huai, X.; Cui, X.; Cheng, K. Buoyancy effects on coupled heat transfer of supercritical pressure CO2 in horizontal semicircular channels. Int. J. Heat Mass Transf. 2019, 134, 437–449. [Google Scholar] [CrossRef]
- Xu, X.; Wang, Q.; Li, L.; Chen, Y.; Ma, T. Study on thermal resistance distribution and local heat transfer enhancement method for SCO2–water heat exchange process near pseudo-critical temperature. Int. J. Heat Mass Transf. 2015, 82, 179–188. [Google Scholar] [CrossRef]
- Meshram, A.; Jaiswal, A.K.; Khivsara, S.D.; Ortega, J.D.; Ho, C.; Bapat, R.; Dutta, P. Modeling and analysis of a printed circuit heat exchanger for supercritical CO2 power cycle applications. Appl. Therm. Eng. 2016, 109, 861–870. [Google Scholar] [CrossRef] [Green Version]
- Webb, R.L. Performance evaluation criteria for use of enhanced heat transfer surfaces in heat exchanger design. Int. J. Heat Mass Transf. 1981, 24, 715–726. [Google Scholar] [CrossRef]
- Yang, S.; Zhao, Z.; Zhang, Y.; Chen, Z.; Yang, M. Effects of Fin Arrangements on Thermal Hydraulic Performance of Supercritical Nitrogen in Printed Circuit Heat Exchanger. Processes 2021, 9, 861. [Google Scholar] [CrossRef]



| Geometry Parameter | Zigzag | Fillet | Sin | Trape |
|---|---|---|---|---|
| Half wavelength λ | 12.3 | 12.3 | 12.3 | 12.3 |
| Amplitude A | 1.648 | 1.507 | 1.648 | 1.648 |
| Chamfer radius r (or Sweep length Lsw) | - | 4 | - | 9.004 |
| Channel length Lflow | 229.212 | 228.312 | 230.662 | 245.970 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, L.; Yan, A.; Shi, Q.; Xiao, X.; Zhu, C.; Yang, X. Numerical Study on Thermal-Hydraulic Performance of Printed Circuit Heat Exchangers during Natural Gas Trans-Critical Liquefaction. Atmosphere 2022, 13, 730. https://doi.org/10.3390/atmos13050730
Liu L, Yan A, Shi Q, Xiao X, Zhu C, Yang X. Numerical Study on Thermal-Hydraulic Performance of Printed Circuit Heat Exchangers during Natural Gas Trans-Critical Liquefaction. Atmosphere. 2022; 13(5):730. https://doi.org/10.3390/atmos13050730
Chicago/Turabian StyleLiu, Lei, Aihua Yan, Qiuxiang Shi, Xi Xiao, Chenbing Zhu, and Xuelong Yang. 2022. "Numerical Study on Thermal-Hydraulic Performance of Printed Circuit Heat Exchangers during Natural Gas Trans-Critical Liquefaction" Atmosphere 13, no. 5: 730. https://doi.org/10.3390/atmos13050730
APA StyleLiu, L., Yan, A., Shi, Q., Xiao, X., Zhu, C., & Yang, X. (2022). Numerical Study on Thermal-Hydraulic Performance of Printed Circuit Heat Exchangers during Natural Gas Trans-Critical Liquefaction. Atmosphere, 13(5), 730. https://doi.org/10.3390/atmos13050730







