Determination of Volatility Parameters of Secondary Organic Aerosol Components via Thermal Analysis
Abstract
1. Introduction
2. Materials and Methods
2.1. Material
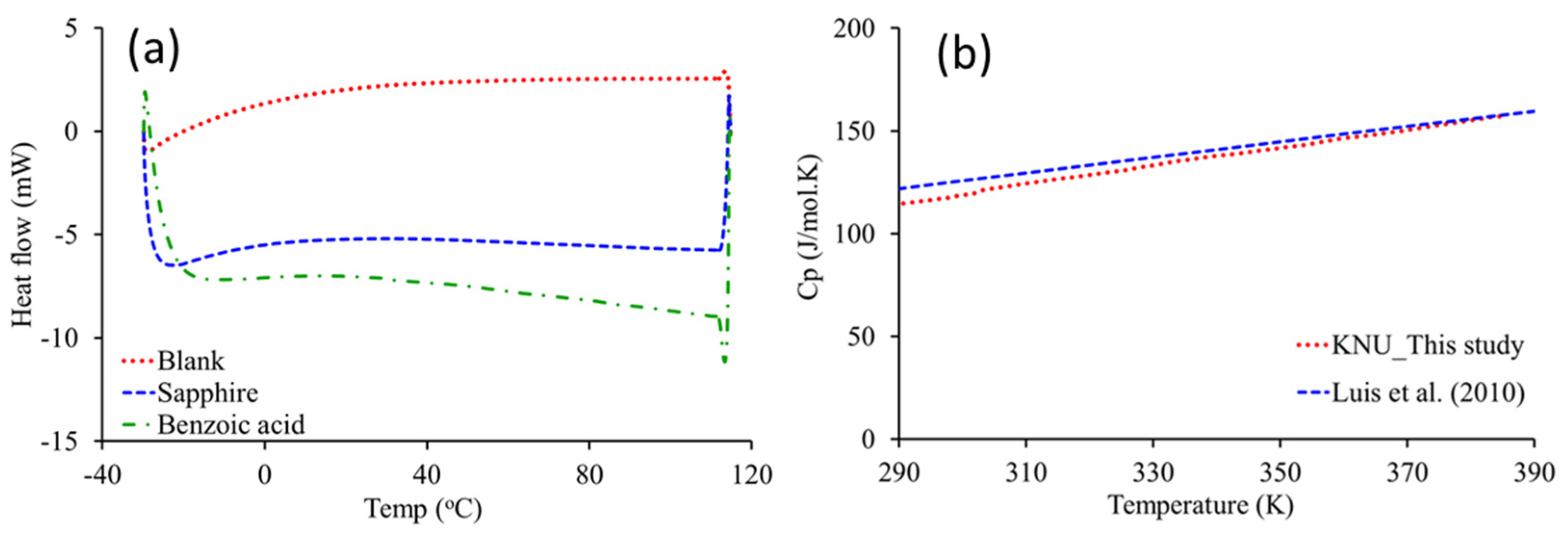
2.2. Heat Capacity Analysis
2.3. TGA/DSC Analysis
2.4. Estimation of Specific Heat Capacity
2.5. Estimation of the Melting Point and Enthalpy
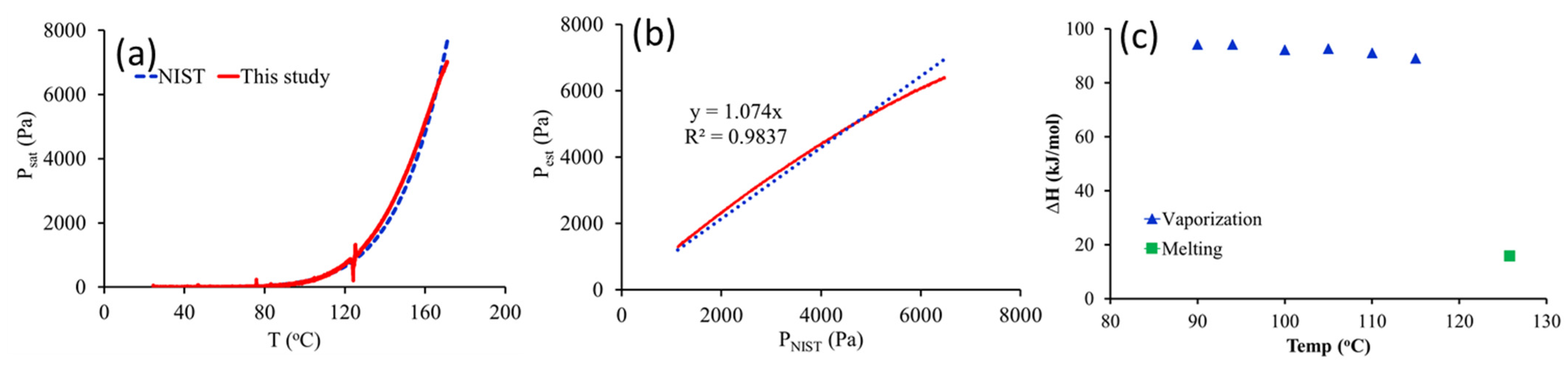
2.6. Estimation of Saturation Vapor Pressure and Vaporization Enthalpy
3. Results and Discussion
3.1. Specific Heat Capacity
3.2. Melting Point
3.3. Vaporization Enthalpy
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Anderson, J.O.; Thundiyil, J.G.; Stolbach, A. Clearing the Air: A Review of the Effects of Particulate Matter Air Pollution on Human Health. J. Med. Toxicol. 2012, 8, 166–175. [Google Scholar] [CrossRef] [PubMed]
- Rao, X.; Zhong, J.; Brook, R.D.; Rajagopalan, S. Effect of Particulate Matter Air Pollution on Cardiovascular Oxidative Stress Pathways. Antioxid. Redox Signal. 2018, 28, 797–818. [Google Scholar] [CrossRef] [PubMed]
- Pöschl, U. Atmospheric aerosols: Composition, transformation, climate and health effects. Angew. Chem. Int. Ed. 2005, 44, 7520–7540. [Google Scholar] [CrossRef] [PubMed]
- Hallquist, M.; Wenger, J.C.; Baltensperger, U.; Rudich, Y.; Simpson, D.; Claeys, M.; Dommen, J.; Donahue, N.M.; George, C.; Goldstein, A.H.; et al. The formation, properties and impact of secondary organic aerosol: Current and emerging issues. Atmos. Chem. Phys. 2009, 9, 5155–5236. [Google Scholar] [CrossRef]
- Zhang, Q.; Jimenez, J.L.; Canagaratna, M.R.; Ulbrich, I.M.; Ng, N.L.; Worsnop, D.R.; Sun, Y. Understanding atmospheric organic aerosols via factor analysis of aerosol mass spectrometry: A review. Anal. Bioanal. Chem. 2011, 401, 3045–3067. [Google Scholar] [CrossRef]
- D’Ambro, E.L.; Schobesberger, S.; Gaston, C.J.; Lopez-Hilfiker, F.D.; Lee, B.H.; Liu, J.; Zelenyuk, A.; Bell, D.; Cappa, C.D.; Helgestad, T.; et al. Chamber-based insights into the factors controlling epoxydiol (IEPOX) secondary organic aerosol (SOA) yield, composition, and volatility. Atmos. Chem. Phys. 2019, 19, 11253–11265. [Google Scholar] [CrossRef]
- Shilling, J.E.; Zawadowicz, M.A.; Liu, J.; Zaveri, R.A.; Zelenyuk, A. Photochemical Aging Alters Secondary Organic Aerosol Partitioning Behavior. ACS Earth Space Chem. 2019, 3, 2704–2716. [Google Scholar] [CrossRef]
- Saleh, R.; Khlystov, A.; Shihadeh, A. Effect of aerosol generation method on measured saturation pressure and enthalpy of vaporization for dicarboxylic acid aerosols. Aerosol Sci. Technol. 2010, 44, 302–307. [Google Scholar] [CrossRef]
- Salo, K.; Jonsson, Å.M.; Andersson, P.U.; Hallquist, M. Aerosol volatility and enthalpy of sublimation of carboxylic acids. J. Phys. Chem. A 2010, 114, 4586–4594. [Google Scholar] [CrossRef]
- Lamb, B.; Grosjean, D.; Pun, B.; Seigneur, C. Review of the Emissions, Atmospheric Chemistry, and Gas/Particle Partition of Biogenic Volatile Organic Compounds and Reaction Products. Final Rep. 1999, NTIS PB2000, 192875. [Google Scholar]
- Babar, Z.B.; Ashraf, F.; Park, J.-H.; Duy, P.; Dao, Q.; Cho, C.S.; Lim, H.-J.; Quang Dao, P.D.; Cho, C.S.; Lim, H.-J. Exploring Volatility Properties of Discrete Secondary Organic Aerosol Constituents of α-Pinene and Polycyclic Aromatic Hydrocarbons. ACS Earth Space Chem. 2020, 4, 2299–2311. [Google Scholar] [CrossRef]
- Kołodziejczyk, A.; Pyrcz, P.; Pobudkowska, A.; Błaziak, K.; Szmigielski, R. Physicochemical Properties of Pinic, Pinonic, Norpinic, and Norpinonic Acids as Relevant α-Pinene Oxidation Products. J. Phys. Chem. B 2019, 123, 8261–8267. [Google Scholar] [CrossRef] [PubMed]
- Park, J.H.; Babar, Z.B.; Baek, S.J.; Kim, H.S.; Lim, H.J. Effects of NOx on the molecular composition of secondary organic aerosol formed by the ozonolysis and photooxidation of A-pinene. Atmos. Environ. 2017, 166, 263–275. [Google Scholar] [CrossRef]
- Fick, J.; Pommer, L.; Nilsson, C.; Andersson, B. Effect of OH radicals, relative humidity, and time on the composition of the products formed in the ozonolysis of α-pinene. Atmos. Environ. 2003, 37, 4087–4096. [Google Scholar] [CrossRef]
- Grayson, J.W.; Zhang, Y.; Mutzel, A.; Renbaum-Wolff, L.; Böge, O.; Kamal, S.; Herrmann, H.; Martin, S.T.; Bertram, A.K. Effect of varying experimental conditions on the viscosity of α-pinene derived secondary organic material. Atmos. Chem. Phys. Discuss. 2015, 15, 32967–33002. [Google Scholar] [CrossRef]
- Mutzel, A.; Rodigast, M.; Iinuma, Y.; Böge, O.; Herrmann, H. Monoterpene SOA–contribution of first-generation oxidation products to formation and chemical composition. Atmos. Environ. 2016, 130, 136–144. [Google Scholar] [CrossRef]
- Emanuelsson, E.U.; Hallquist, M.; Kristensen, K.; Glasius, M.; Bohn, B.; Fuchs, H.; Kammer, B.; Kiendler-Scharr, A.; Nehr, S.; Rubach, F.; et al. Formation of anthropogenic secondary organic aerosol (SOA) and its influence on biogenic SOA properties. Atmos. Chem. Phys. 2013, 13, 2837–2855. [Google Scholar] [CrossRef]
- Jaoui, M.; Corse, E.; Kleindienst, T.E.; Offenberg, J.H.; Lewandowski, M.; Edney, E.O. Analysis of secondary organic aerosol compounds from the photooxidation of d-limonene in the presence of NOx and their detection in ambient PM2.5. Environ. Sci. Technol. 2006, 40, 3819–3828. [Google Scholar] [CrossRef]
- Yasmeen, F.; Szmigielski, R.; Vermeylen, R.; Gõmez-González, Y.; Surratt, J.D.; Chan, A.W.H.; Seinfeld, J.H.; Maenhaut, W.; Claeys, M. Mass spectrometric characterization of isomeric terpenoic acids from the oxidation of α-pinene, β-pinene, d-limonene, and Î" 3-carene in fine forest aerosol. J. Mass Spectrom. 2011, 46, 425–442. [Google Scholar] [CrossRef]
- Tang, G.; Liu, M.; Zhou, Q.; He, H.; Chen, K.; Zhang, H.; Hu, J.; Huang, Q.; Luo, Y.; Ke, H. Microplastics and polycyclic aromatic hydrocarbons (PAHs) in Xiamen coastal areas: Implications for anthropogenic impacts. Sci. Total Environ. 2018, 634, 811–820. [Google Scholar] [CrossRef]
- Srivastava, D.; Daellenbach, K.R.; Zhang, Y.; Bonnaire, N.; Chazeau, B.; Perraudin, E.; Gros, V.; Lucarelli, F.; Villenave, E.; Prévôt, A.S.H.; et al. Comparison of five methodologies to apportion organic aerosol sources during a PM pollution event. Sci. Total Environ. 2021, 757, 143168. [Google Scholar] [CrossRef] [PubMed]
- Ho, K.F.; Ho, S.S.H.; Lee, S.C.; Kawamura, K.; Zou, S.C.; Cao, J.J.; Xu, H.M. Summer and winter variations of dicarboxylic acids, fatty acids and benzoic acid in PM2.5 in Pearl Delta River Region, China. Atmos. Chem. Phys. 2011, 11, 2197–2208. [Google Scholar] [CrossRef]
- Chhabra, P.S.; Lambe, A.T.; Canagaratna, M.R.; Stark, H.; Jayne, J.T.; Onasch, T.B.; Davidovits, P.; Kimmel, J.R.; Worsnop, D.R. Application of high-resolution time-of-flight chemical ionization mass spectrometry measurements to estimate volatility distributions of α-pinene and naphthalene oxidation products. Atmos. Meas. Tech. 2015, 8, 1–18. [Google Scholar] [CrossRef]
- Michoud, V.; Hallemans, E.; Chiappini, L.; Leoz-Garziandia, E.; Colomb, A.; Dusanter, S.; Fronval, I.; Gheusi, F.; Jaffrezo, J.L.; Léonardis, T.; et al. Molecular characterization of gaseous and particulate oxygenated compounds at a remote site in Cape Corsica in the western Mediterranean Basin. Atmos. Chem. Phys. 2021, 21, 8067–8088. [Google Scholar] [CrossRef]
- Gao, X.; Zhang, Y.; Liu, Y. Temperature-dependent hygroscopic behaviors of atmospherically relevant water-soluble carboxylic acid salts studied by ATR-FTIR spectroscopy. Atmos. Environ. 2018, 191, 312–319. [Google Scholar] [CrossRef]
- Jia, L.; Xu, Y. Effects of relative humidity on ozone and secondary organic aerosol formation from the photooxidation of benzene and ethylbenzene. Aerosol. Sci. Technol. 2014, 48, 1–12. [Google Scholar] [CrossRef]
- Ciarelli, G.; Haddad, I.E.; Bruns, E.; Aksoyoglu, S.; Möhler, O.; Baltensperger, U.; Prévôt, A.S.H. Constraining a hybrid volatility basis-set model for aging of wood-burning emissions using smog chamber experiments: A box-model study based on the VBS scheme of the CAMx model (v5.40). Geosci. Model Dev. 2017, 10, 2303–2320. [Google Scholar] [CrossRef]
- Zhang, J.; He, X.; Ding, X.; Yu, J.Z.; Ying, Q. Modeling Secondary Organic Aerosol Tracers and Tracer-to-SOA Ratios for Monoterpenes and Sesquiterpenes Using a Chemical Transport Model. Environ. Sci. Technol. 2022, 56, 804–813. [Google Scholar] [CrossRef]
- Pennington, E.A.; Seltzer, K.M.; Murphy, B.N.; Qin, M.; Seinfeld, J.H.; Pye, H.O.T. Modeling secondary organic aerosol formation from volatile chemical products. Atmos. Chem. Phys. 2021, 21, 18247–18261. [Google Scholar] [CrossRef]
- Lane, T.E.; Donahue, N.M.; Pandis, S.N. Effect of NOx on secondary organic aerosol concentrations. Environ. Sci. Technol. 2008, 42, 6022–6027. [Google Scholar] [CrossRef]
- Weagle, C.L.; Snider, G.; Li, C.; Van Donkelaar, A.; Philip, S.; Bissonnette, P.; Burke, J.; Jackson, J.; Latimer, R.; Stone, E.; et al. Global Sources of Fine Particulate Matter: Interpretation of PM2.5 Chemical Composition Observed by SPARTAN using a Global Chemical Transport Model. Environ. Sci. Technol. 2018, 52, 11670–11681. [Google Scholar] [CrossRef] [PubMed]
- Bilde, M.; Barsanti, K.; Booth, M.; Cappa, C.D.; Donahue, N.M.; Emanuelsson, E.U.; McFiggans, G.; Krieger, U.K.; Marcolli, C.; Topping, D.; et al. Saturation Vapor Pressures and Transition Enthalpies of Low-Volatility Organic Molecules of Atmospheric Relevance: From Dicarboxylic Acids to Complex Mixtures. Chem. Rev. 2015, 115, 4115–4156. [Google Scholar] [CrossRef] [PubMed]
- Saleh, R.; Walker, J.; Khlystov, A. Determination of saturation pressure and enthalpy of vaporization of semi-volatile aerosols: The integrated volume method. J. Aerosol Sci. 2008, 39, 876–887. [Google Scholar] [CrossRef]
- Alibakhshi, A. Enthalpy of vaporization, its temperature dependence and correlation with surface tension: A theoretical approach. Fluid Phase Equilib. 2017, 432, 62–69. [Google Scholar] [CrossRef]
- Donahue, N.M.; Robinson, A.L.; Stanier, C.O.; Pandis, S.N. Coupled partitioning, dilution, and chemical aging of semivolatile organics. Environ. Sci. Technol. 2006, 40, 2635–2643. [Google Scholar] [CrossRef]
- Donahue, N.M.; Kroll, J.H.; Pandis, S.N.; Robinson, A.L. A two-dimensional volatility basis set-Part 2: Diagnostics of organic-aerosol evolution. Atmos. Chem. Phys. 2012, 12, 615–634. [Google Scholar] [CrossRef]
- Pankow, J.F.; Asher, W.E. SIMPOL.1: A simple group contribution method for predicting vapor pressures and enthalpies of vaporization of multifunctional organic compounds. Atmos. Chem. Phys. 2008, 8, 2773–2796. [Google Scholar] [CrossRef]
- Chickos, J.S.; Acree, W.E. Enthalpies of vaporization of organic and organometallic compounds, 1880-2002. J. Phys. Chem. Ref. Data 2003, 32, 519–878. [Google Scholar] [CrossRef]
- Ribeiro Da Silva, M.A.V.; Monte, M.J.S.; Ribeiro, J.R. Vapour pressures and the enthalpies and entropies of sublimation of five dicarboxylic acids. J. Chem. Thermodyn. 1999, 31, 1093–1107. [Google Scholar] [CrossRef]
- Bernardes, C.E.S.; Joseph, A.; da Piedade, M.E.M. Some practical aspects of heat capacity determination by differential scanning calorimetry. Thermochim. Acta 2020, 687, 178574. [Google Scholar] [CrossRef]
- Giani, S.; Riesen, R.; Schawe, J.E.K. An Indirect Method for Vapor Pressure and Phase Change Enthalpy Determination by Thermogravimetry. Int. J. Thermophys. 2018, 39, 84. [Google Scholar] [CrossRef]
- Ramos, F.; Ledo, J.M.; Flores, H.; Camarillo, E.A.; Carvente, J.; Amador, M.P. Evaluation of sublimation enthalpy by thermogravimetry: Analysis of the diffusion effects in the case of methyl and phenyl substituted hydantoins. Thermochim. Acta 2017, 655, 181–193. [Google Scholar] [CrossRef]
- Booth, A.M.; Montague, W.J.; Barley, M.H.; Topping, D.O.; McFiggans, G.; Garforth, A.; Percival, C.J. Solid state and sub-cooled liquid vapour pressures of cyclic aliphatic dicarboxylic acids. Atmos. Chem. Phys. 2011, 11, 655–665. [Google Scholar] [CrossRef]
- ASTM E1269-11; Standard Test Method for Determining Specific Heat Capacity by Differential Scanning Calorimetry. ASTM International: West Conshohocken, PA, USA, 2018.
- Mourad, A.H.I.; Akkad, R.O.; Soliman, A.A.; Madkour, T.M. Characterisation of thermally treated and untreated polyethylene- polypropylene blends using DSC, TGA and IR techniques. Plast. Rubber Compos. 2009, 38, 265–278. [Google Scholar] [CrossRef]
- Chickos, J.S.; Hosseini, S.; Hesse, D.G.; Liebman, J.F. Heat capacity corrections to a standard state: A comparison of new and some literature methods for organic liquids and solids. Struct. Chem. 1993, 4, 271–278. [Google Scholar] [CrossRef]
- Wright, S.F.; Phang, P.; Dollimore, D.; Alexander, K.S. An overview of calibration materials used in thermal analysis—Benzoic acid. Thermochim. Acta 2002, 392–393, 251–257. [Google Scholar] [CrossRef]
- Santos, L.M.N.B.F.; Rocha, M.A.A.; Gomes, L.R.; Schröder, B.; Coutinho, J.A.P. Gaseous phase heat capacity of benzoic acid. J. Chem. Eng. Data 2010, 55, 2799–2808. [Google Scholar] [CrossRef]
- Fukai, M.; Matsuo, T.; Suga, H. Thermodynamic properties of phase transitions in malonic acid and its deuterated analogue. Thermochim. Acta 1991, 183, 215–243. [Google Scholar] [CrossRef]
- Parks, G.S.; West, T.J.; Moore, G.E. Thermal Data on Organic Compounds. XXI. Some Heat Capacity, Entropy and Free Energy Data for the Four Methylnonanes. J. Am. Chem. Soc. 1941, 63, 1133–1135. [Google Scholar] [CrossRef]
- Bret-Dibat, P.; Lichanot, A. Proprietes thermodynamiques des isomeres de position de benzenes disubstitues en phase condensee. Thermochim. Acta 1989, 147, 261–271. [Google Scholar] [CrossRef]
- Iten, M.; Liu, S.; Shukla, A.; Silva, P.D. Investigating the impact of Cp-T values determined by DSC on the PCM-CFD model. Appl. Therm. Eng. 2017, 117, 65–75. [Google Scholar] [CrossRef]
- Booth, A.M.; Barley, M.H.; Topping, D.O.; McFiggans, G.; Garforth, A.; Percival, C.J. Solid state and sub-cooled liquid vapour pressures of substituted dicarboxylic acids using Knudsen Effusion Mass Spectrometry (KEMS) and Differential Scanning Calorimetry. Atmos. Chem. Phys. 2010, 10, 4879–4892. [Google Scholar] [CrossRef]
- Bilde, M.; Svenningsson, B.; Mønster, J.; Rosenørn, T. Even-odd alternation of evaporation rates and vapor pressures of C3-C9 dicarboxylic acid aerosols. Environ. Sci. Technol. 2003, 37, 1371–1378. [Google Scholar] [CrossRef]
- Acree, W.E. Thermodynamic properties of organic compounds: Enthalpy of fusion and melting point temperature compilation. Thermochim. Acta 1991, 189, 37–56. [Google Scholar] [CrossRef]
- Roux, M.V.; Temprado, M.; Chickos, J.S. Vaporization, fusion and sublimation enthalpies of the dicarboxylic acids from C4 to C14 and C16. J. Chem. Thermodyn. 2005, 37, 941–953. [Google Scholar] [CrossRef]
- Sharma, B.L.; Kant, R.; Sharma, R.; Tandon, S. Deviations of binary organic eutectic melt systems. Mater. Chem. Phys. 2003, 82, 216–224. [Google Scholar] [CrossRef]
- Brittain, H.G. Vibrational spectroscopic studies of cocrystals and salts. 2. The benzylamine-benzoic acid system. Cryst. Growth Des. 2009, 9, 3497–3503. [Google Scholar] [CrossRef]
- Gracin, S.; Rasmuson, Å.C. Solubility of phenylacetic acid, p-hydroxyphenylacetic acid, p-aminophenylacetic acid, p-hydroxybenzoic acid, and ibuprofen in pure solvents. J. Chem. Eng. Data 2002, 47, 1379–1383. [Google Scholar] [CrossRef]
- Nordström, F.L.; Rasmuson, Å.C. Phase equilibria and thermodynamics of p-hydroxybenzoic acid. J. Pharm. Sci. 2006, 95, 748–760. [Google Scholar] [CrossRef]
- Sabbah, R.; Perez, L. Thermodynamic study of phthalic, isophthalic, and terephthalic acids. Can. J. Chem. Can. Chim. 1999, 77, 1508–1513. [Google Scholar] [CrossRef]
- Lee, M.J.; Chang, Y.K.; Lin, H.M.; Chen, C.H. Solid-liquid equilibria for 4-methoxyphenol with catechol, ethylenediamine, or piperazine. J. Chem. Eng. Data 1997, 42, 349–352. [Google Scholar] [CrossRef]
- Verevkin, S.P.; Kozlova, S.A. Di-hydroxybenzenes: Catechol, resorcinol, and hydroquinone. Enthalpies of phase transitions revisited. Thermochim. Acta 2008, 471, 33–42. [Google Scholar] [CrossRef]
- Kostenidou, E.; Karnezi, E.; Kolodziejczyk, A.; Szmigielski, R.; Pandis, S.N. Physical and Chemical Properties of 3-Methyl-1,2,3-butanetricarboxylic Acid (MBTCA) Aerosol. Environ. Sci. Technol. 2018, 52, 1150–1155. [Google Scholar] [CrossRef] [PubMed]
- Murray, J.P.; Cavell, K.J.; Hill, J.O. A DSC study of benzoic acid: A suggested calibrant compound. Thermochim. Acta 1980, 36, 97–101. [Google Scholar] [CrossRef]
- Chatterjee, K.; Dollimore, D.; Alexander, K.S. Calculation of vapor pressure curves for hydroxy benzoic acid derivatives using thermogravimetry. Thermochim. Acta 2002, 392, 107–117. [Google Scholar] [CrossRef]
- Chatterjee, K.; Hazra, A.; Dollimore, D.; Alexander, K.S. Estimating vapor pressure curves by thermogravimetry: A rapid and convenient method for characterization of pharmaceuticals. Eur. J. Pharm. Biopharm. 2002, 54, 171–180. [Google Scholar] [CrossRef]
- Roy, S.; Riga, A.T.; Alexander, K.S. Experimental design aids the development of a differential scanning calorimetry standard test procedure for pharmaceuticals. Thermochim. Acta 2002, 392–393, 399–404. [Google Scholar] [CrossRef]
- National Institute of Standards and Technology (2021) Benzoic acid. Available online: https://webbook.nist.gov/cgi/cbook.cgi?ID=C65850&Mask=4&Type=ANTOINE&Plot=on (accessed on 3 April 2021).
- Cappa, C.D.; Lovejoy, E.R.; Ravishankara, A.R. Evidence for liquid-like and nonideal behavior of a mixture of organic aerosol components. Proc. Natl. Acad. Sci. USA 2008, 105, 18687–18691. [Google Scholar] [CrossRef]
- Soonsin, V.; Zardini, A.A.; Marcolli, C.; Zuend, A.; Krieger, U.K. The vapor pressures and activities of dicarboxylic acids reconsidered: The impact of the physical state of the aerosol. Atmos. Chem. Phys. 2010, 10, 11753–11767. [Google Scholar] [CrossRef]
- Chattopadhyay, S.; Ziemann, P.J. Vapor pressures of substituted and unsubstituted monocarboxylic and dicarboxylic acids measured using an improved thermal desorption particle beam mass spectrometry method. Aerosol Sci. Technol. 2005, 39, 1085–1100. [Google Scholar] [CrossRef]
- Tao, Y.; Mcmurry, P.H. Vapor Pressures and Surface Free Energies of C14-C18 Monocarboxylic Acids and C5 and C6 Dicarboxylic Acids. Environ. Sci. Technol. 1989, 23, 1519–1523. [Google Scholar] [CrossRef]
- Gao, G.-Y.; Lin, S.-Y. Thermodynamic investigations of nitroxoline sublimation by simultaneous DSC-FTIR method and isothermal TG analysis. J. Pharm. Sci. 2010, 99, 255–261. [Google Scholar] [CrossRef] [PubMed]
- Heath, E.A.; Singh, P.; Ebisuzaki, Y. Structure of p-hydroxybenzoic acid and p-hydroxybenzoic acid-acetone complex (2/1). Acta Crystallogr. Sect. C Cryst. Struct. Commun. 1992, 48, 1960–1965. [Google Scholar] [CrossRef]
- Babar, Z.B.; Ashraf, F.; Park, J.-H.H.; Lim, H.-J.J. Volatility parameters of secondary organic aerosol components determined using a thermal denuder. Atmos. Environ. 2020, 226, 117405. [Google Scholar] [CrossRef]
- Chen, X.; Oja, V.; Chan, W.G.; Hajaligol, M.R. Vapor pressure characterization of several phenolics and polyhydric compounds by Knudsen effusion method. J. Chem. Eng. Data 2006, 51, 386–391. [Google Scholar] [CrossRef]
- Ortiz-Montalvo, D.L.; Lim, Y.B.; Perri, M.J.; Seitzinger, S.P.; Turpin, B.J. Volatility and yield of glycolaldehyde SOA formed through aqueous photochemistry and droplet evaporation. Aerosol Sci. Technol. 2012, 46, 1002–1014. [Google Scholar] [CrossRef]
- Saha, P.K.; Grieshop, A.P. Exploring Divergent Volatility Properties from Yield and Thermodenuder Measurements of Secondary Organic Aerosol from α-Pinene Ozonolysis. Environ. Sci. Technol. 2016, 50, 5740–5749. [Google Scholar] [CrossRef]
- Sato, K.; Fujitani, Y.; Inomata, S.; Morino, Y.; Tanabe, K.; Hikida, T.; Shimono, A.; Takami, A.; Fushimi, A.; Kondo, Y.; et al. A study of volatility by composition, heating, and dilution measurements of secondary organic aerosol from 1,3,5-trimethylbenzene. Atmos. Chem. Phys. 2019, 19, 14901–14915. [Google Scholar] [CrossRef]
- Carlton, A.G.; Bhave, P.V.; Napelenok, S.L.; Edney, E.O.; Sarwar, G.; Pinder, R.W.; Pouliot, G.A.; Houyoux, M.; Agency, U.S.E.P.; Carolina, N.; et al. Model representation of secondary organic aerosol in CMAQv4.7. Environ. Sci. Technol. 2010, 44, 8553–8560. [Google Scholar] [CrossRef]
- Sakulyanontvittaya, T.; Guenther, A.; Helmig, D.; Milford, J.; Wiedinmyer, C. Secondary organic aerosol from sesquiterpene and monoterpene emissions in the United States. Environ. Sci. Technol. 2008, 42, 8784–8790. [Google Scholar] [CrossRef][Green Version]
- Bian, F.; Bowman, F.M. A lumping model for composition- and temperature-dependent partitioning of secondary organic aerosols. Atmos. Environ. 2005, 39, 1263–1274. [Google Scholar] [CrossRef]
- Boyd, C.M.; Nah, T.; Xu, L.; Berkemeier, T.; Ng, N.L. Secondary Organic Aerosol (SOA) from Nitrate Radical Oxidation of Monoterpenes: Effects of Temperature, Dilution, and Humidity on Aerosol Formation, Mixing, and Evaporation. Environ. Sci. Technol. 2017, 51, 7831–7841. [Google Scholar] [CrossRef] [PubMed]
- Tsigaridis, K.; Daskalakis, N.; Kanakidou, M.; Adams, P.J.; Artaxo, P.; Bahadur, R.; Balkanski, Y.; Bauer, S.E.; Bellouin, N.; Benedetti, A. The AeroCom evaluation and intercomparison of organic aerosol in global models. Atmos. Chem. Phys. 2014, 14, 10845–10895. [Google Scholar] [CrossRef]
| SOA Species | Tm (°C) | CP, 298 K (J mol−1 K−1) | Cp(T) = A + BT + CT2 + DT3 + ET−2 | References | ||||
|---|---|---|---|---|---|---|---|---|
| A | B | C | D | E | ||||
| Malonic acid | 132.8 | 137.1 | −1.91 × 105 | 1.17 × 103 | −2.69 × 100 | 2.20 × 10−3 | 2.02 × 109 | This study |
| -- | 128.1 | Fukai et al. (1991) [49] | ||||||
| Suberic acid | 141.1 | 221.5 | −1.33 × 105 | 8.02 × 102 | −1.80 × 100 | 1.44 × 10−3 | 1.45 × 109 | This study |
| Benzoic acid | 122.7 | 118.1 | 7.01 × 102 | −1.98 × 100 | 1.56 × 10−3 | 1.62 × 10−6 | −1.53 × 107 | This study |
| 121.3 | 125.1 | Santos et al. (2010) [48] | ||||||
| Phthalic acid | 193.9 | 169.4 | −1.16 × 104 | 6.60 × 101 | −1.37 × 10−1 | 1.01 × 10−4 | 1.46 × 108 | This study |
| 187.1 | Parks et al. (1941) [50] | |||||||
| Catechol | 108.1 | 135.9 | 1.51 × 104 | −7.72 × 101 | 1.45 × 10−1 | −9.37 × 10−5 | −2.15 × 108 | This study |
| 140.2 | 1.32 × 102 | 2.80 × 10−1 | 5.40 × 10−4 | 2.05 × 10−3 | Bret-Dibat et al. (1989) [51] | |||
| Pinic acid | 94.9 | 189.9 | −1.30 × 104 | 7.4 × 101 | −1.53× 10−1 | 1.14 × 10−4 | 1.64 × 108 | This study |
| Ketopinic acid | 103.1 | 223.9 | −1.94 × 105 | 1.19 × 103 | −2.74 × 100 | 2.23 × 10−3 | 2.06 × 109 | This study |
| cis-Pinonic acid | 107.1 | 246.1 | −7.53 × 105 | 4.64 × 103 | −1.07 × 101 | 8.84 × 10−3 | 7.94 × 109 | This study |
| Terpenylic acid | 81.5 | 223.2 | −3.87 × 105 | 2.74 × 103 | −7.35 × 100 | 7.04 × 10−3 | 3.17 × 109 | This study |
| DTAA * | 130.2 | 524.1 | −1.08 × 105 | 6.24 × 102 | −1.34 × 100 | 1.03 × 10−3 | 1.31 × 109 | This study |
| SOA Species | Tm (°C) | ΔHfus, Tm (kJ mol−1) | ΔHfus,298 K (kJ mol−1) | Method * | References |
|---|---|---|---|---|---|
| Malonic acid | 132.8 | 22.7 | 16.8 | TGA–DSC a | This study |
| 132.9 | 18.7 | KME d | Booth et al. (2010) [53] | ||
| Succinic acid | 183.8 | 63.5 | 54.9 | TGA–DSC a | This study |
| 187.8 | TDMA b | Bilde et al. (2003) [54] | |||
| Adipic acid | 151.4 | 35.0 | 28.1 | TGA–DSC a | This study |
| 152.9 | TDMA b | Bilde et al. (2003) [54] | |||
| 153.2 | 34.85 | Acree et al. (1991) [55] | |||
| Suberic acid | 141.1 | 28.2 | 21.9 | TGA–DSC a | This study |
| 142.2 | 28.2 | TGA–DSC a | Acree et al. (1991) [55] | ||
| 140.1 | 30.7 | TGA–DSC a | Roux et al. (2005) [56] | ||
| Benzoic acid | 122.7 | 14.2 ± 1.5 | 8.6 ± 1.5 | TGA–DSC a | This study |
| 121.4 | 17.3 | TGA–DSC a | Sharma et al. (2003) [57] | ||
| 122.4 | 17.7 | TGA–DSC a | Ramos et al. (2017) [42] | ||
| 123.7 | 16.9 | TGA–DSC a | Brittain et al. (2009) [58] | ||
| 4-Hydroxybenzoic acid | 215.5 | 30.6 | 20.2 | TGA–DSC a | This study |
| 214.8 | 32 | TGA–DSCa | Gracin et al. (2002) [59] | ||
| 214 | 31.4 | TGA–DSC a | Nordström et al. (2006) [60] | ||
| Phthalic acid | 193.9 | 102.4 | 93.2 | TGA–DSC a | This study |
| 190.35 | 36.5 | Calorimetric | Sabbah et al. (1999) [61] | ||
| Catechol | 108.1 | 22.1 ± 0.8 | 17.6 ± 0.8 | TGA–DSC a | This study |
| 104.5 | 22.5 | SLE c | Lee et al. (1997) [62] | ||
| 103.5 | 22.8 | TGA–DSC a | Verevkin et al. (2008) [63] | ||
| Pinic acid | 94.9 | 7.3 | 3.5 | TGA–DSC a | This study |
| 91.1 | 16.6 | TGA–DSC a | Kołodziejczyk et al. (2019c) [12] | ||
| Ketopinic acid | 103.1 | 13.9 | 9.7 | TGA–DSC a | This study |
| cis-Pinonic acid | 107.1 | 29.7 | 25.5 | TGA–DSC a | This study |
| 103.2 | 30.4 | TGA–DSC a | Kołodziejczyk et al. (2019c) [12] | ||
| Terpenylic acid | 81.5 | 7.9 | 4.8 | TGA–DSC a | This study |
| DTAA e | 147.1 | 20.5 | 12.8 | TGA–DSC a | This study |
| 121.1 | 7.12 | TGA–DSC a | Kołodziejczyk et al. (2020) [64] | ||
| 3-MBTCA f | 136.4 | 130.0 | 123.9 | TGA–DSC a | This study |
| 149.6 | 85.2 | TGA–DSC a | Kołodziejczyk et al. (2020) [64] |
| SOA Species | ΔHvap (kJ mol−1) | Method * | References |
|---|---|---|---|
| Malonic acid | 132.7 | TGA–DSC a | This study |
| 132.1 ± 5 | TPD f | Cappa et al. (2008) [70] | |
| 107 ± 4 | EDB b | Soonsin et al. (2010) [71] | |
| 111.4 | KME i | Ribeiro et al. (1999) [39] | |
| Succinic acid | 116.1 | TGA–DSC a | This study |
| 112 ± 12 | VTDMA e | Salo et al. (2010) [9] | |
| 125 ± 8 | EDB b | Soonsin et al. (2010) [71] | |
| 119.5 | TPTD g | Chattopadhyay et al. (2005) [72] | |
| Adipic acid | 99.0 | TGA–DSC a | This study |
| 119 ± 18 | KME i | Booth et al. (2010) [53] | |
| 97± 8 | VTDMA e | Salo et al. (2010) [9] | |
| 118 | TDMA d | Tao et al. (1989) [73] | |
| Suberic acid | 94.1 | TGA–DSC a | This study |
| 101 ± 10 | VTDMA e | Salo et al. (2010) [9] | |
| 130 | TPD f | Cappa et al. (2008) [70] | |
| Benzoic acid | 93.2 ± 0.4 | TGA–DSC a | This study |
| 89.7 ± 3 | TGA–DSC a | Murray et al. (1980) [65] | |
| 91.4 | TGA–DSC a | Gau-Yi et al. (2010) [74] | |
| 4-Hydroxybenzoic acid | 119.5 | TGA–DSC a | This study |
| 120 | TGA–DSC a | Heath et al. (1992) [75] | |
| Phthalic acid | 131.6 | TGA–DSC a | This study |
| 129.8 | Calorimetric | Sabbah et al. (1999) [61] | |
| 135.9 ± 3.1 | IVM c | Babar et al. (2020a) [76] | |
| Catechol | 93.0 ± 2.5 | TGA–DSC a | This study |
| 80.0 | KME i | Chen et al. (2006) [77] | |
| Ketopinic acid | 113.8 | TGA–DSC a | This study |
| 136.6 ± 3.5 | IVM c | Babar et al. (2020a) [76] | |
| DTAA j | 130.2 | TGA–DSC a | This study |
| 135.4 ± 3.7 | IVM c | Babar et al. (2020b) [11] | |
| 3-MBTCA k | 124.4 | TGA–DSC a | This study |
| 128.4 ± 4.7 | IVM c | Babar et al. (2020b) [11] | |
| 150 ± 15 | DMT h | Kostenidou et al. (2018) [64] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ashraf, F.; Babar, Z.B.; Park, J.-H.; Dao, P.D.Q.; Cho, C.S.; Lim, H.-J. Determination of Volatility Parameters of Secondary Organic Aerosol Components via Thermal Analysis. Atmosphere 2022, 13, 709. https://doi.org/10.3390/atmos13050709
Ashraf F, Babar ZB, Park J-H, Dao PDQ, Cho CS, Lim H-J. Determination of Volatility Parameters of Secondary Organic Aerosol Components via Thermal Analysis. Atmosphere. 2022; 13(5):709. https://doi.org/10.3390/atmos13050709
Chicago/Turabian StyleAshraf, Fawad, Zaeem Bin Babar, Jun-Hyun Park, Pham Duy Quang Dao, Chan Sik Cho, and Ho-Jin Lim. 2022. "Determination of Volatility Parameters of Secondary Organic Aerosol Components via Thermal Analysis" Atmosphere 13, no. 5: 709. https://doi.org/10.3390/atmos13050709
APA StyleAshraf, F., Babar, Z. B., Park, J.-H., Dao, P. D. Q., Cho, C. S., & Lim, H.-J. (2022). Determination of Volatility Parameters of Secondary Organic Aerosol Components via Thermal Analysis. Atmosphere, 13(5), 709. https://doi.org/10.3390/atmos13050709






