Investigating Neutral and Stable Atmospheric Surface Layers Using Computational Fluid Dynamics
Abstract
1. Introduction
2. Materials and Methods
2.1. CFD Equations and Numerical Methods
2.2. CASES-99 Data
2.3. Monin–Obukhov Similarity Theory and the SBL Profiles
2.4. Approach to the CFD Simulations
3. Results
4. Discussion
5. Conclusions
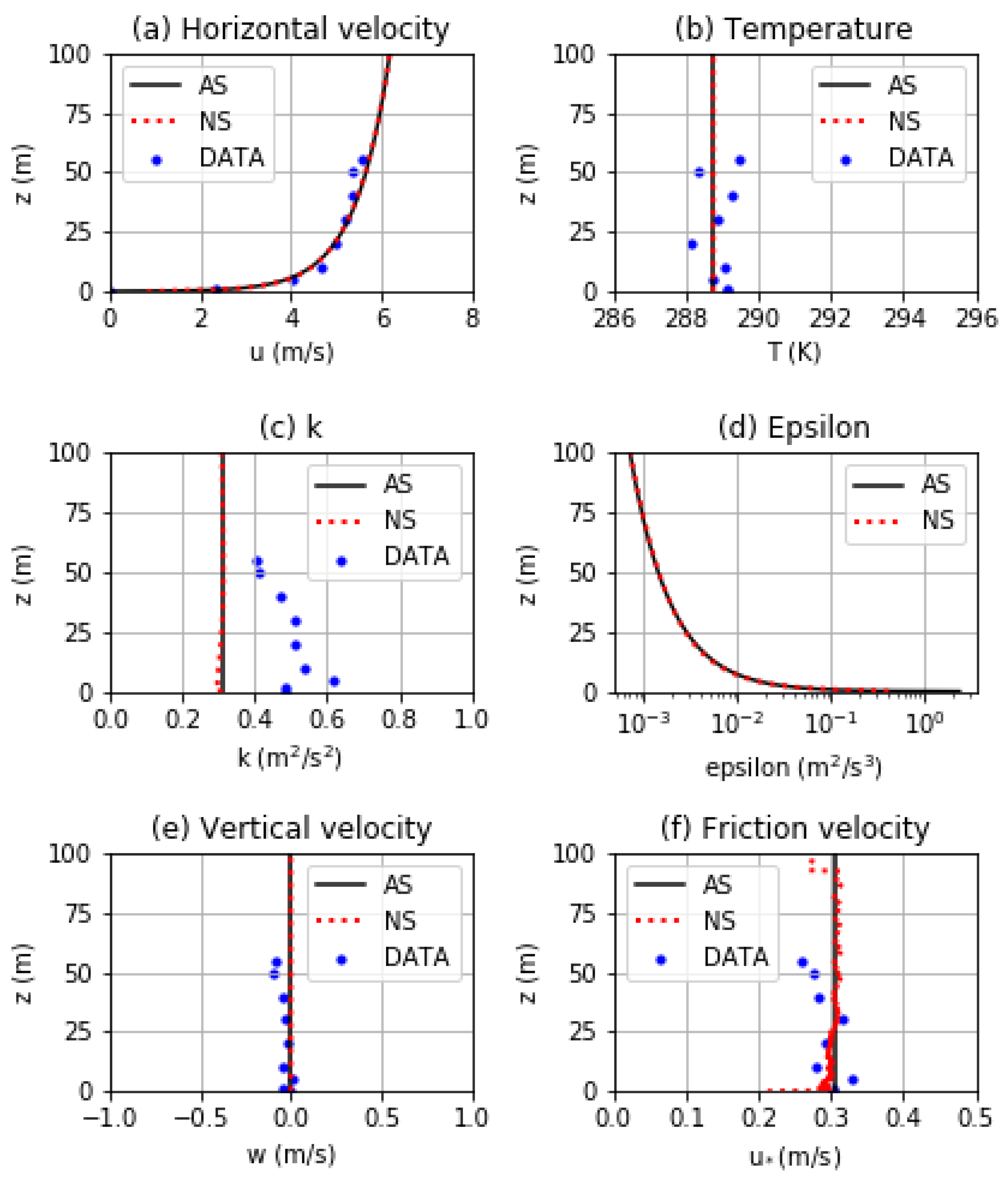
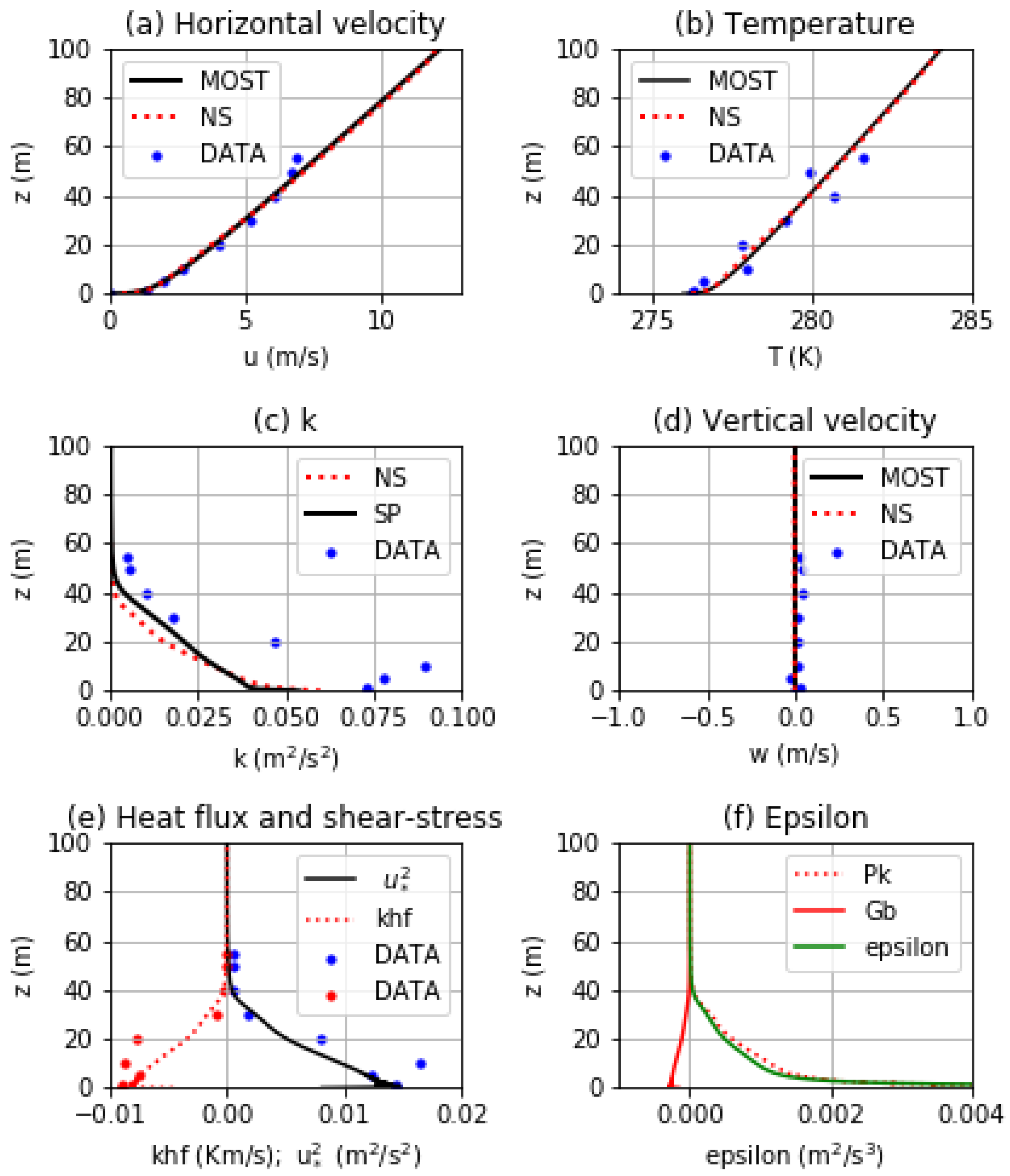
- The theoretical reference profiles for u, θ, and k, which were applied as upwind boundary conditions, were successfully reproduced by using CFD for the NBL. For the SBL, the reference MOST profiles were successfully reproduced for u and θ, as was the reference profile obtained by experiment in the case of k.
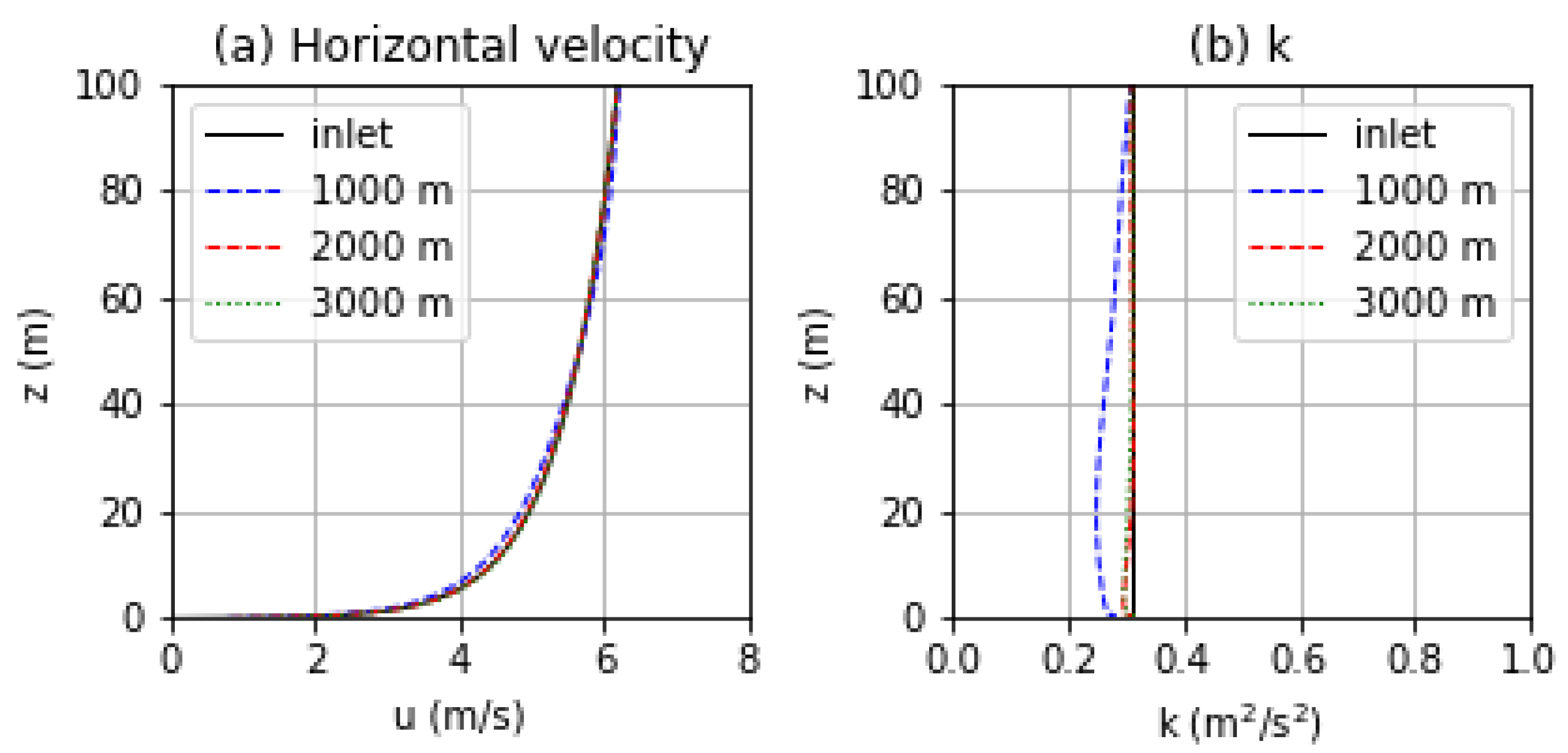
- Reference profiles were maintained throughout the computational domain for both u and θ. It took some distance for k to fully reach the equilibrium situation, especially for the NBL; but, thereafter, k was sustained in the domain and matched the respective upwind reference profiles. Small errors in the velocities were thought to affect k.
- The CASES-99 observations of u and θ can be adequately reproduced with CFD for the test cases; k showed less agreement with the observations, and possible reasons for this were considered.
- Reasonable agreement of the numerical results with the observed surface layer depth, and the profiles of shear stress and heat flux were obtained for the SBL.
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Stull, R. An Introduction to Boundary Layer Meteorology; Springer: New York, NY, USA, 2009. [Google Scholar]
- Richards, P.J.; Hoxey, R.P. Appropriate boundary conditions for computational wind engineering models using the k-ε turbulence model. J. Wind. Eng. Ind. Aerodyn. 1993, 46–47, 145–153. [Google Scholar] [CrossRef]
- Richards, P.J.; Norris, S.E. Appropriate boundary conditions for computational wind engineering models revisited. J. Wind. Eng. Ind. Aerodyn. 2011, 99, 257–266. [Google Scholar] [CrossRef]
- Yang, Y.; Gu, G.; Chen, S.; Jin, X. New inflow boundary conditions for modelling the neutral equilibrium atmospheric boundary layer in computational wind engineering. J. Wind. Eng. Ind. Aerodyn. 2009, 97, 88–95. [Google Scholar] [CrossRef]
- Hargreaves, D.M.; Wright, N.G. On the use of the k-ε model in commercial CFD software to model the neutral atmospheric boundary layer. J. Wind. Eng. Ind. Aerodyn. 2007, 95, 355–369. [Google Scholar] [CrossRef]
- Parente, A.; Gorle, C.; van Beeck, J.; Bennoci, C. Improved k-ε model and wall function formulation for the RANS simulation of ABL flows. J. Wind. Eng. Ind. Aerodyn. 2011, 99, 267–278. [Google Scholar] [CrossRef]
- Alinot, C.; Masson, C. k-epsilon model for the atmospheric boundary layer under various thermal stratifications. J. Sol. Energy Eng. 2005, 127, 438–443. [Google Scholar] [CrossRef]
- Chang, C.-Y.; Schmidt, J.; Dorenkamper, M.; Stoevesandt, B. A consistent steady state simulation method for stratified atmospheric boundary layer flows. J. Wind. Eng. Ind. Aerodyn. 2018, 172, 55–67. [Google Scholar] [CrossRef]
- Optis, M.; Monahan, A.; Bosveld, F.C. Moving beyond Monin-Obuhkov similarity theory in modelling wind speed profiles in the lower atmospheric boundary layer under stable stratification. Bound.-Layer Meteorol. 2014, 153, 497–514. [Google Scholar] [CrossRef]
- Launder, B.E.; Spalding, D.B. The numerical computation of turbulent flows. Comput. Method Appl. Mech. 1974, 3, 269–289. [Google Scholar] [CrossRef]
- OpenFOAM. Available online: https://www.openfoam.com/ (accessed on 7 June 2021).
- Nozaki, F. Available online: http://caefn.com/openfoam/solvers-buoyantpimplefoam/ (accessed on 7 June 2021).
- Versteeg, H.; Malalasekera, W. An Introduction to Computational Fluid Dynamics: The Finite Volume Method; Pearson Education Limited: London, UK, 2007. [Google Scholar]
- Li, D. Turbulent Prandtl number in the atmospheric boundary layer—where are we now? Atmos. Res. 2019, 216, 86–105. [Google Scholar] [CrossRef]
- CHAM. Available online: http://www.cham.co.uk/phoenics/d_polis/d_enc/turmod/enc_t341.htm/ (accessed on 7 June 2021).
- Zeli, V.; Brethouwer, G.; Wallin, S.; Johansson, A.V. Consistent boundary-condition treatment for computation of the atmospheric boundary layer using the explicit algebraic Reynolds-stress model. Bound.-Layer Meteorol. 2019, 171, 53–77. [Google Scholar] [CrossRef]
- Poulos, G.S.; Blumen, W.; Fritts, D.C.; Lundquist, J.K.; Sun, J.; Burns, S.P.; Nappo, C.; Banta, R.; Newsom, R.; Cuxart, J.; et al. CASES-99: A Comprehensive Investigation of the Stable Nocturnal Boundary Layer. Bull. Am. Meteorol. Soc. 2002, 83, 555–581. [Google Scholar] [CrossRef]
- 5 Minute Statistics of ISFF Data during CASES-99 Version 1.0. 2016, UCAR/NCAR—Earth Observing Laboratory. Available online: https://www.eol.ucar.edu/field_projects/cases-99/ (accessed on 7 June 2021).
- Cheng, Y.; Oarlange, M.B.; Brutsaert, W. Pathology of Monin-Obukhov similarity in the stable boundary layer. J. Geophys. Res. 2005, 110, 1–10. [Google Scholar] [CrossRef]
- Vickers, D.; Mahrt, L. The Cospectral Gap and Turbulent Flux Calculations. J. Atmos. Ocean Technol. 2003, 20, 660–672. [Google Scholar] [CrossRef]
- Yague, C.; Viana, S.; Maqueda, G.; Redondo, J.M. Influence of stability on the flux-profile relationships for wind speed, φm, and temperature, φh, for the stable atmospheric boundary layer. Nonlinear Processes Geophys. 2006, 13, 185–203. [Google Scholar] [CrossRef][Green Version]
- Taylor, P.; Laroche, D.; Wang, Z.-Q.; McLaren, R. Obukhov Length Computation Using Simple Measurements from Weather Stations and AXYS Wind Sentinel Buoys; WWOSC: Montreal, QC, Canada, 2014. [Google Scholar]
- Emeis, S. Wind Energy Meteorology: Atmospheric Physics for Wind Power Generation; Springer Science & Business Media: New York, NY, USA, 2018. [Google Scholar]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gemmell, F. Investigating Neutral and Stable Atmospheric Surface Layers Using Computational Fluid Dynamics. Atmosphere 2022, 13, 221. https://doi.org/10.3390/atmos13020221
Gemmell F. Investigating Neutral and Stable Atmospheric Surface Layers Using Computational Fluid Dynamics. Atmosphere. 2022; 13(2):221. https://doi.org/10.3390/atmos13020221
Chicago/Turabian StyleGemmell, Fraser. 2022. "Investigating Neutral and Stable Atmospheric Surface Layers Using Computational Fluid Dynamics" Atmosphere 13, no. 2: 221. https://doi.org/10.3390/atmos13020221
APA StyleGemmell, F. (2022). Investigating Neutral and Stable Atmospheric Surface Layers Using Computational Fluid Dynamics. Atmosphere, 13(2), 221. https://doi.org/10.3390/atmos13020221




