Effect of SST in the Northwest Indian Ocean on Synoptic Eddies over the South China Sea-Philippine Sea in June
Abstract
:1. Introduction
2. Data and Methods
3. Results
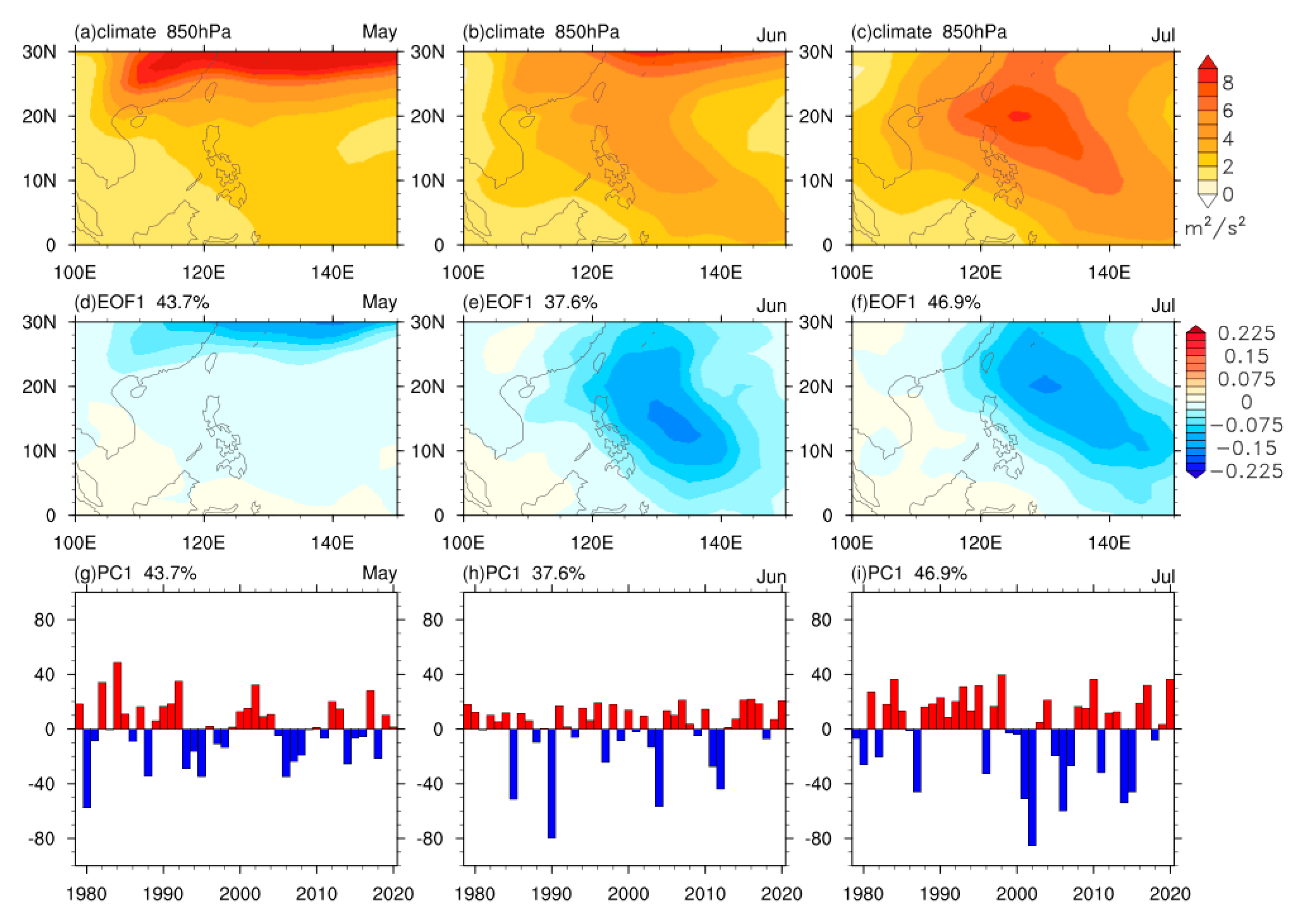
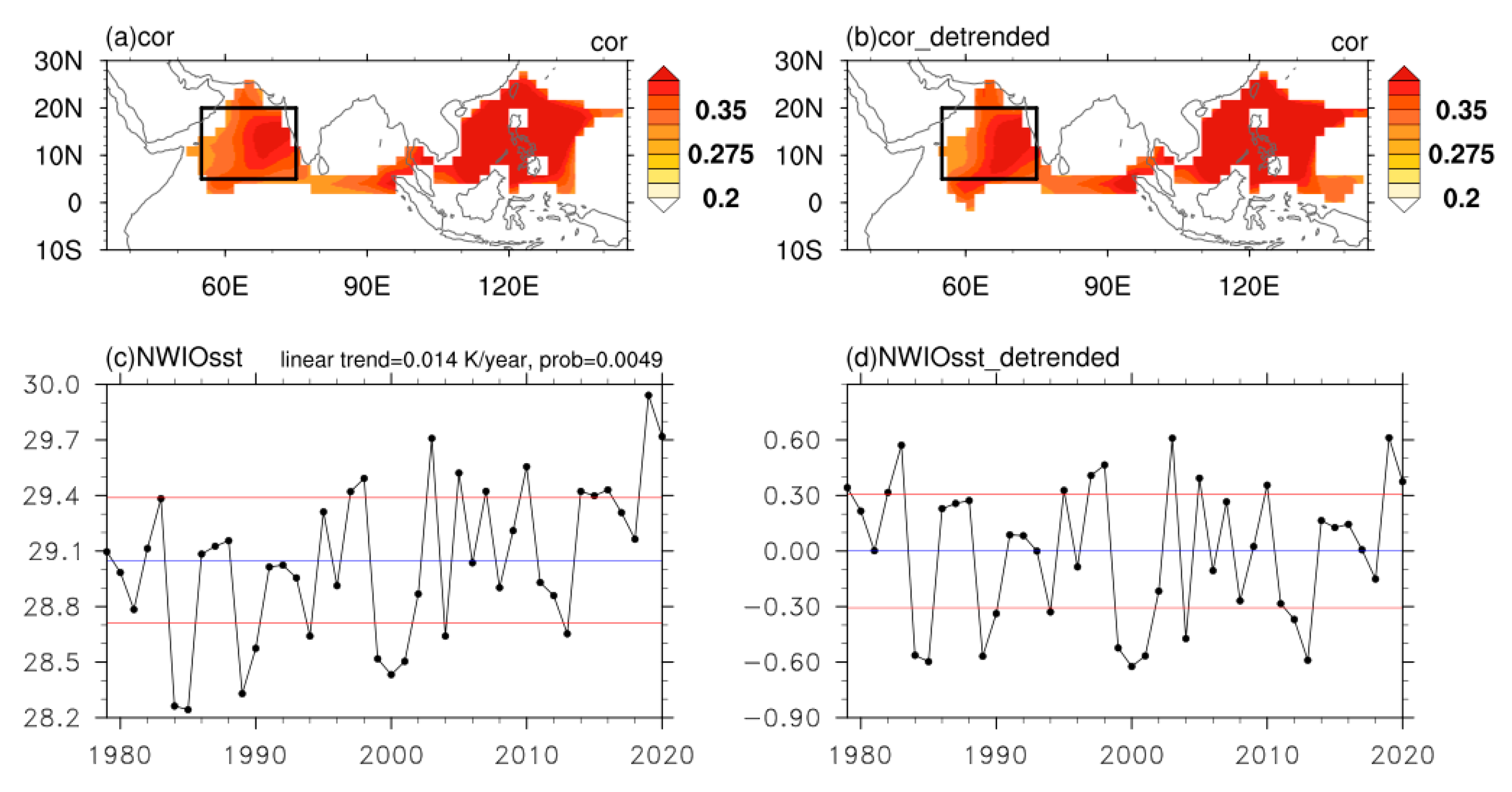
3.1. Link between Synoptic Eddies in the SCS-PS and Northwest Indian Ocean SST
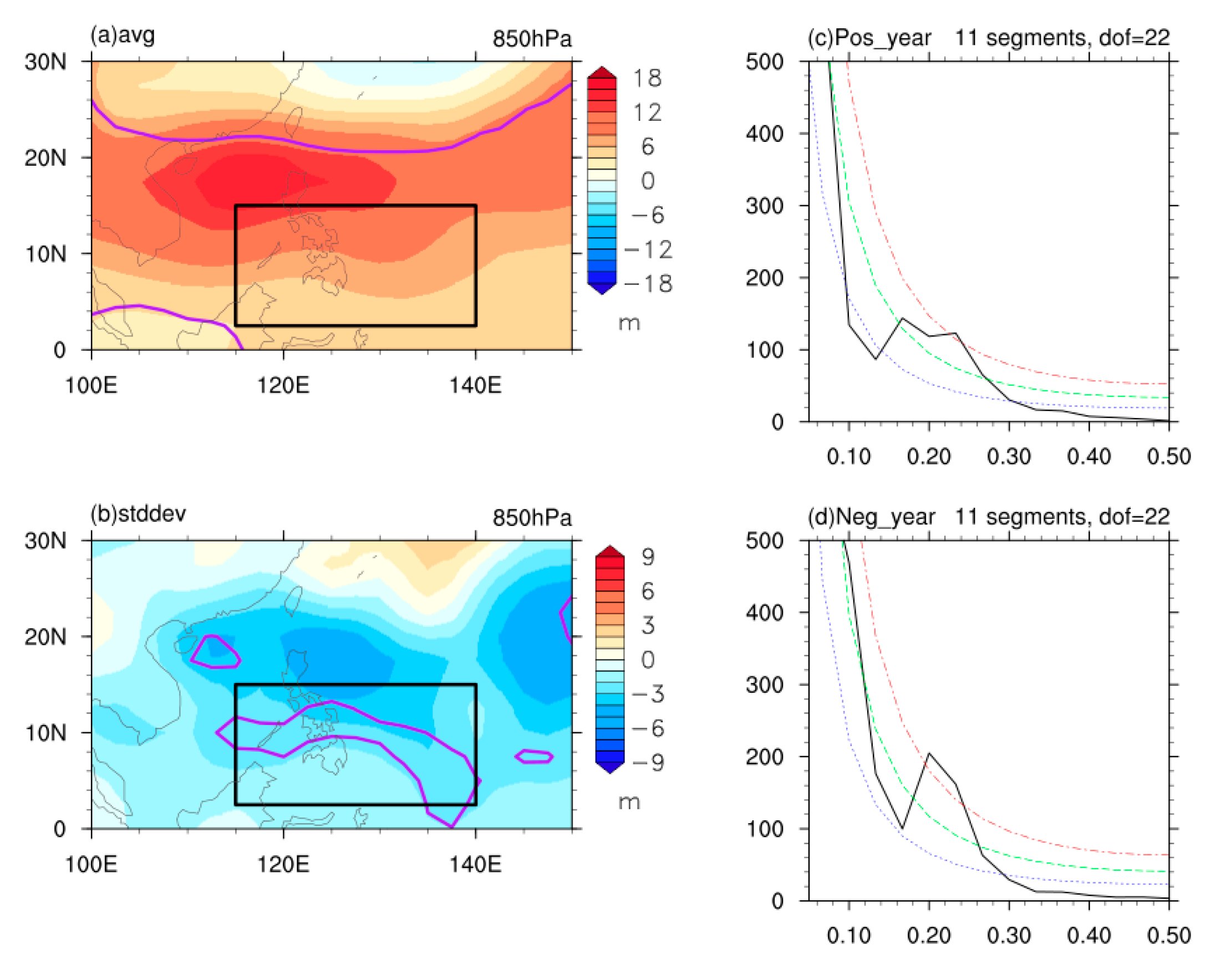
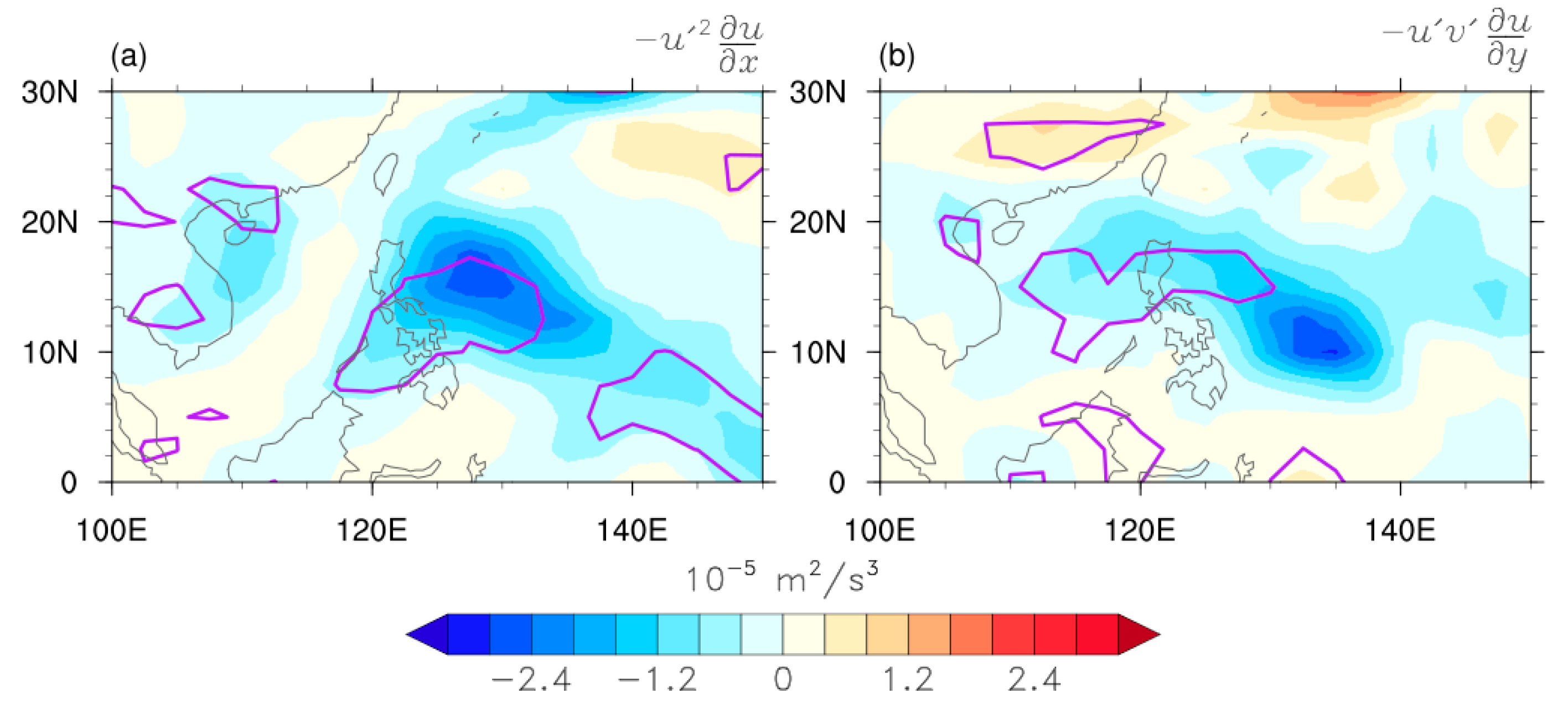
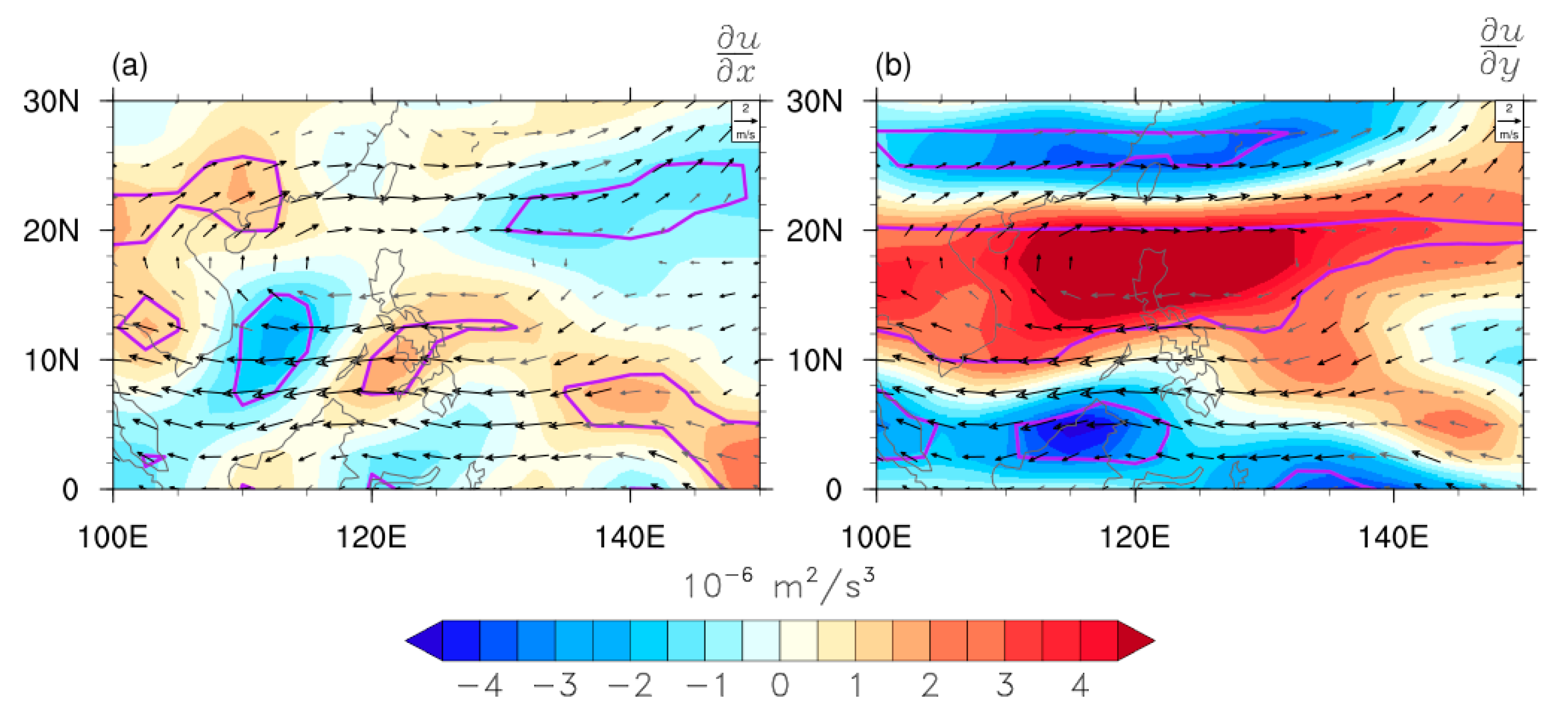
3.2. Change in the Energy Conversion of EKE over the SCS-PS in Response to Anomalous Northwest Indian Ocean SST
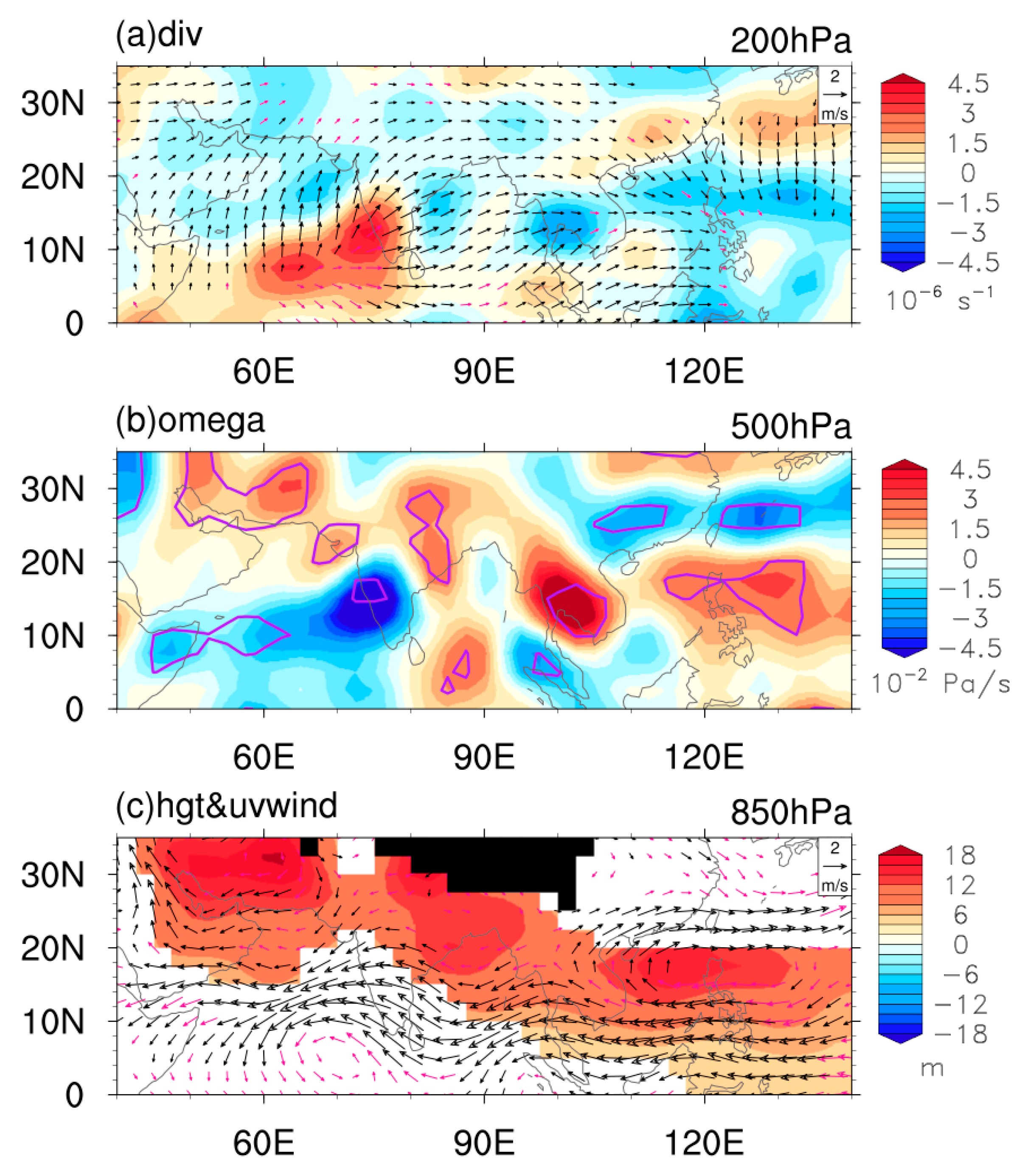
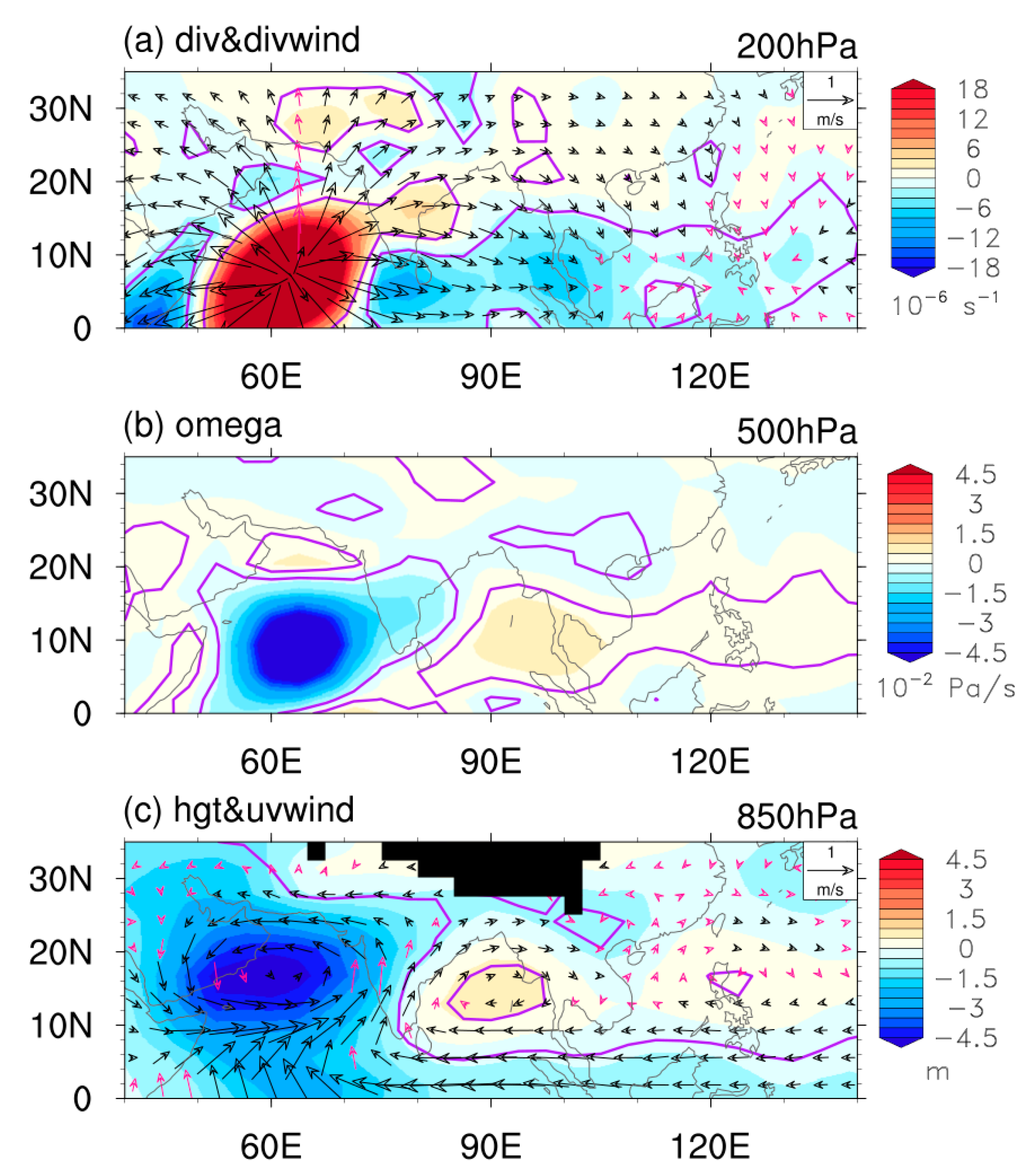
3.3. Atmospheric Circulation Anomaly Driven by Anomalous Northwest Indian Ocean SST
4. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Lau, K.; Lau, N. Observed structure and propagation characteristics of tropical summertime synoptic scale disturbances. Mon. Weather Rev. 1990, 118, 1888–1913. [Google Scholar] [CrossRef]
- Hsu, P.-C.; Li, T.; Tsou, C.-H. Interactions between Boreal Summer Intraseasonal Oscillations and Synoptic-Scale Disturbances over the Western North Pacific. Part I: Energetics Diagnosis. J. Clim. 2011, 24, 927–994. [Google Scholar] [CrossRef]
- Li, T. Origin of the Summertime Synoptic-Scale Wave Train in the Western North Pacific. J. Atmos. Sci. 2006, 63, 1093–1102. [Google Scholar] [CrossRef] [Green Version]
- Fu, B.; Li, T.; Peng, M.S.; Weng, F. Analysis of tropical cyclogenesis in the western North Pacific for 2000 and 2001. Weather Forecast 2007, 22, 763–780. [Google Scholar] [CrossRef] [Green Version]
- Yuan, J.; Li, T.; Wang, D. Precursor synoptic-scale disturbances associated with tropical cyclogenesis in the South China Sea during 2000–2011. Int. J. Climatol. 2015, 35, 3454–3470. [Google Scholar] [CrossRef]
- Xu, Y.; Li, T.; Peng, M. Roles of the synoptic-scale wave train, the intraseasonal oscillation, and high-frequency eddies in the genesis of Typhoon Manyi (2001). J Atmos Sci. 2014, 71, 3706–3722. [Google Scholar] [CrossRef]
- Zhou, H.; Hsu, P.-C.; Qian, Y. Close linkage between quasi-biweekly oscillation and tropical cyclone intensification over the western North Pacific. Atmos. Sci. Lett. 2018, 19, e826. [Google Scholar] [CrossRef]
- Liu, H.B.; Yan, R.J.; Wang, B.; Chen, G.H.; Ling, J.; Fu, S.M. Multiscale combined action and disturbance characteristics of pre-summer extreme precipitation events over South China. Adv. Atmos. Sci. 2022, in press. [Google Scholar] [CrossRef]
- Hu, P.; Chen, W.; Li, Z.; Chen, S.; Wang, L.; Liu, Y. Close Linkage of the South China Sea Summer Monsoon Onset and Extreme Rainfall in May over Southeast Asia: Role of the Synoptic-Scale Systems. J. Clim. 2022, 35, 4347–4362. [Google Scholar] [CrossRef]
- Wang, W.; Zhou, W.; Li, X.; Wang, X.; Wang, D. Synoptic-scale characteristics and atmospheric controls of summer heat waves in China. Clim. Dyn. 2016, 46, 2923–2941. [Google Scholar] [CrossRef]
- Leung, M.Y.-T.; Zhou, W.; Cheung, K.Y.; Gong, H.N.; Zhang, Y. Enhancement of lower tropospheric winter synoptic temperature variations in Southwest China and the northern Indochina Peninsula after 2010. Clim. Dyn. 2019, 53, 2281–2294. [Google Scholar] [CrossRef]
- Guo, M.; Zhang, M.; Wang, H.; Wang, L.; Liu, S.; Zong, L.; Zhang, Y.; Li, Y. Dual effects of synoptic weather patterns and urbanization on summer diurnal temperature range in an urban agglomeration of East China. Front. Environ. Sci. 2021, 9, 672295. [Google Scholar] [CrossRef]
- Hsu, P.-C.; Tsou, C.-H.; Hsu, H.-H.; Chen, J.-H. Eddy energy along the tropical storm track in association with ENSO. J. Meteorol. Soc. Jpn. 2009, 87, 687–704. [Google Scholar] [CrossRef] [Green Version]
- Zeng, L.; Li, X.; Du, Y.; Shi, R.; Yao, J.; Wang, D.; Sui, D. Synoptic-scale disturbances over the northern South China Sea and their responses to El Niño. Acta Oceanol. Sin. 2012, 31, 69–78. [Google Scholar] [CrossRef] [Green Version]
- Yuan, Y.; Zhou, W.; Chan, J.C.L.; Li, C. Impacts of the basin-wide Indian Ocean SSTA on the South China Sea summer monsoon onset. Int. J. Climatol. 2008, 28, 1579–1587. [Google Scholar] [CrossRef]
- Leung, M.Y.T.; Zhou, W.; Wang, D.; Chan, P.W.; Lee, S.M.; Tong, H.W. Remote tropical Western Indian ocean forcing on changes in June precipitation in South China and the Indochina Peninsula. J. Clim. 2020, 33, 7553–7566. [Google Scholar] [CrossRef]
- Lorenz, E.N. Available Potential Energy and the Maintenance of the General Circulation. Tellus 1955, 7, 157–167. [Google Scholar] [CrossRef] [Green Version]
- Ulbrich, U.; Speth, P. The Global Energy Cycle of Stationary and Transient Atmospheric Waves: Results from ECMWF Analyses. Meteorol. Atmos. Phys. 1991, 45, 125–138. [Google Scholar] [CrossRef]
- Leung, M.Y.-T.; Cheung, H.H.N.; Zhou, W. Energetics and dynamics associated with two typical mobile trough pathways over East Asia in boreal winter. Clim. Dyn. 2015, 44, 1611–1626. [Google Scholar] [CrossRef]
- Hu, P.; Huangfu, J.; Chen, W.; Huang, R. South China Sea summer monsoon withdrawal and the synoptic-scale wave train over the western North Pacific. Int. J. Climatol. 2020, 40, 5599–5611. [Google Scholar] [CrossRef]
- Huangfu, J.; Cao, X.; Wu, R.; Chen, G.; Chen, W. Influences of central Pacific warming on synoptic-scale wave intensity over the northwest Pacific. Clim. Dyn. 2022, 58, 555–567. [Google Scholar] [CrossRef]
- Yang, M.; Li, C.; Li, X.; Chen, X.; Li, L. The linkage between midwinter suppression of the North Pacific storm track and atmospheric circulation features in the Northern Hemisphere. Adv. Atmos. Sci. 2022, 39, 502–518. [Google Scholar] [CrossRef]
- Kanamitsu, M.; Ebisuzaki, W.; Woollen, J.; Yang, S.-K.; Hnilo, J.J.; Fiorino, M.; Potter, G.L. NCEP-DOE AMIP-II Reanalysis (R-2). Bull. Am. Meteorol. Soc. 2002, 83, 1631–1643. [Google Scholar] [CrossRef] [Green Version]
- Huang, B.; Thorne, P.W.; Banzon, V.F.; Boyer, T.; Chepurin, G.; Lawrimore, J.H.; Menne, M.J.; Smith, T.M.; Vose, R.S.; Zhang, H.-M. Extended Reconstructed Sea Surface Temperature, Version 5 (ERSSTv5): Upgrades, Validations, and Intercomparisons. J. Clim. 2017, 30, 8179–8205. [Google Scholar] [CrossRef]
- Lau, K.; Lau, N. The energetics and propagation dynamics of tropical summertime synoptic-scale disturbances. Mon. Weather Rev. 1992, 120, 2523–2539. [Google Scholar] [CrossRef]
- Fukutomi, Y.; Kodama, C.; Yamada, Y.; Noda, A.T.; Satoh, M. Tropical synoptic-scale wave disturbances over the western Pacific simulated by a global cloud-system resolving model. Theor. Appl. Climatol. 2016, 124, 737–755. [Google Scholar] [CrossRef]
- Ha, Y.; Zhong, Z.; Zhao, H.; Zhu, Y.; Hu, Y. A Climatological Perspective on Extratropical Synoptic-Scale Transient Eddy Activity Response to Western Pacific Tropical Cyclones. Adv. Atmos. Sci. 2022, 39, 333–343. [Google Scholar] [CrossRef]
- Duchon, C.E. Lanczos Filtering in One and Two Dimensions. J. Appl. Meteorol. 1979, 18, 1016–1022. [Google Scholar] [CrossRef]
- Belu, R.; Koracin, D. Statistical and Spectral Analysis of Wind Characteristics Relevant to Wind Energy Assessment Using Tower Measurements in Complex Terrain. J. Wind Energy 2013, 2013, 739162. [Google Scholar] [CrossRef]
- Kaya, E.; Barutcu, B.; Mentes, S.S. A method based on the Van der Hoven spectrum for performance evaluation in prediction of wind speed. Turk. J. Earth Sci. 2013, 22, 12. [Google Scholar] [CrossRef]
- Shikhovtsev, A.Y.; Pavel, G.K.; Evgeniy, A.K.; Mansur, A.I.; Shuhrat, A.E.; Yusufjon, A.T. Energy Spectra of Atmospheric Turbulence for Calculating C2n Parameter. I. Maidanak and Suffa Observatories in Uzbekistan. Atmosphere 2021, 12, 1614. [Google Scholar] [CrossRef]
- Molteni, F. Atmospheric simulations using a GCM with simplified physical parametrizations. I: Model climatology and variability in multi-decadal experiments. Clim. Dyn. 2003, 20, 175–191. [Google Scholar] [CrossRef]
- Kucharski, F.; Molteni, F.; Bracco, A. Decadal interactions between the western tropical Pacific and the North Atlantic Oscillation. Clim. Dyn. 2006, 26, 79–91. [Google Scholar] [CrossRef]
- Kucharski, F.; Molteni, F.; King, M.P.; Farneti, R.; Kang, I.S.; Feudale, L. On the need of intermediate complexity general circulation models: A “SPEEDY’’ example. Bull. Am. Meteorol. Soc. 2013, 94, 25–30. [Google Scholar] [CrossRef]
- Jian, Y.; Lin, X.; Zhou, W.; Jian, M.; Leung, M.Y.-T.; Cheung, P.K.Y. Analysis of record-high temperature over southeast coastal China in winter 2018/19: The combined effect of mid- to high-latitude circulation systems and SST forcing over the North Atlantic and tropical western Pacific. J. Clim. 2020, 33, 8813–8831. [Google Scholar] [CrossRef]
- Jian, Y.; Leung, M.Y.-T.; Zhou, W.; Jian, M.; Yang, S.; Lin, X. Interdecadal shift of the relationship between ENSO and winter synoptic temperature variability over the Asian–Pacific–American region in the 1980s. J. Clim. 2021, 34, 5321–5335. [Google Scholar] [CrossRef]
- Leung, M.Y.-T.; Wang, D.; Zhou, W.; Cheung, P.K.Y.; Jian, Y.; Xiao, F. Joint effect of West Pacific warming and the Arctic Oscillation on the bidecadal variation and trend of the East Asian trough. J. Clim. 2022, 35, 2491–2501. [Google Scholar] [CrossRef]
- Leung, M.Y.-T.; Wang, D.; Zhou, W.; Zhang, Y.; Wang, L. Interdecadal variation in available potential energy of stationary eddies in the midlatitude Northern Hemisphere in response to the North Pacific Gyre Oscillation. Geophys. Res. Let. 2022, 49, e2022GL098297. [Google Scholar] [CrossRef]
- Feng, W.; Leung, M.Y.-T.; Wang, D.; Zhou, W.; Zhang, O.Y.W. An extreme drought over South China in 2020/21 concurrent with an unprecedented warm Northwest Pacific and La Niña. Adv. Atmos. Sci. 2022, 39, 1637–1649. [Google Scholar] [CrossRef]
- North, G.R.; Bell, T.L.; Cahalan, R.F.; Moeng, F.J. Sampling Errors in the Estimation of Empirical Orthogonal Functions. Mon. Weather Rev. 1982, 110, 699–706. [Google Scholar] [CrossRef]
- Bader, J.; Latif, M. The impact of decadal-scale Indian Ocean sea surface temperature anomalies on Sahelian rainfall and the North Atlantic Oscillation. Geophys. Res. Lett. 2003, 30, 2169. [Google Scholar] [CrossRef] [Green Version]
- Sharma, S.; Ha, K.J.; Cai, W.; Chung, E.S.; Bódai, T. Local meridional circulation changes contribute to a projected slowdown of the Indian Ocean Walker circulation. NPJ Clim. Atmos. Sci. 2022, 5, 15. [Google Scholar] [CrossRef]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lan, M.; Leung, M.Y.-T.; Wang, D.; Feng, W.; Yang, W. Effect of SST in the Northwest Indian Ocean on Synoptic Eddies over the South China Sea-Philippine Sea in June. Atmosphere 2022, 13, 2073. https://doi.org/10.3390/atmos13122073
Lan M, Leung MY-T, Wang D, Feng W, Yang W. Effect of SST in the Northwest Indian Ocean on Synoptic Eddies over the South China Sea-Philippine Sea in June. Atmosphere. 2022; 13(12):2073. https://doi.org/10.3390/atmos13122073
Chicago/Turabian StyleLan, Ming, Marco Y.-T. Leung, Dongxiao Wang, Weijie Feng, and Wei Yang. 2022. "Effect of SST in the Northwest Indian Ocean on Synoptic Eddies over the South China Sea-Philippine Sea in June" Atmosphere 13, no. 12: 2073. https://doi.org/10.3390/atmos13122073
APA StyleLan, M., Leung, M. Y.-T., Wang, D., Feng, W., & Yang, W. (2022). Effect of SST in the Northwest Indian Ocean on Synoptic Eddies over the South China Sea-Philippine Sea in June. Atmosphere, 13(12), 2073. https://doi.org/10.3390/atmos13122073






