Numerical Simulation of the Dispersion of Exhaled Aerosols from a Manikin with a Realistic Upper Airway
Abstract
1. Introduction
2. Materials and Methods
2.1. Geometry Model
2.2. Governing Equations
2.2.1. Fluid Phase
2.2.2. Discrete Phase
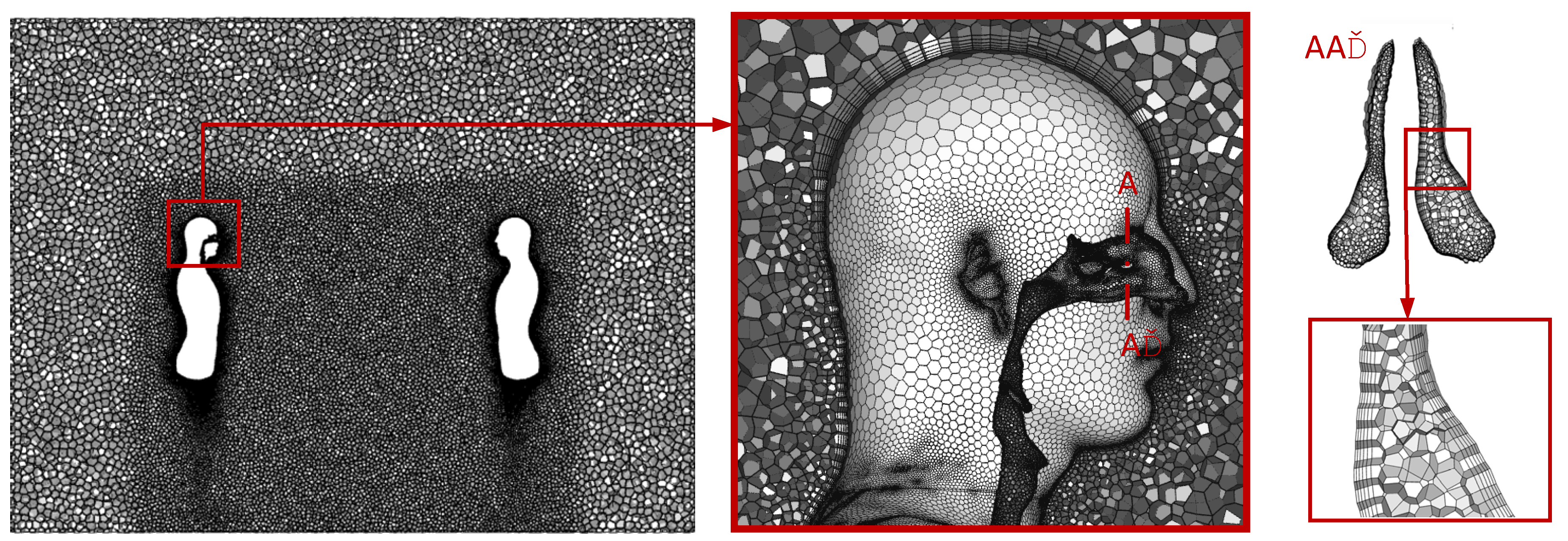
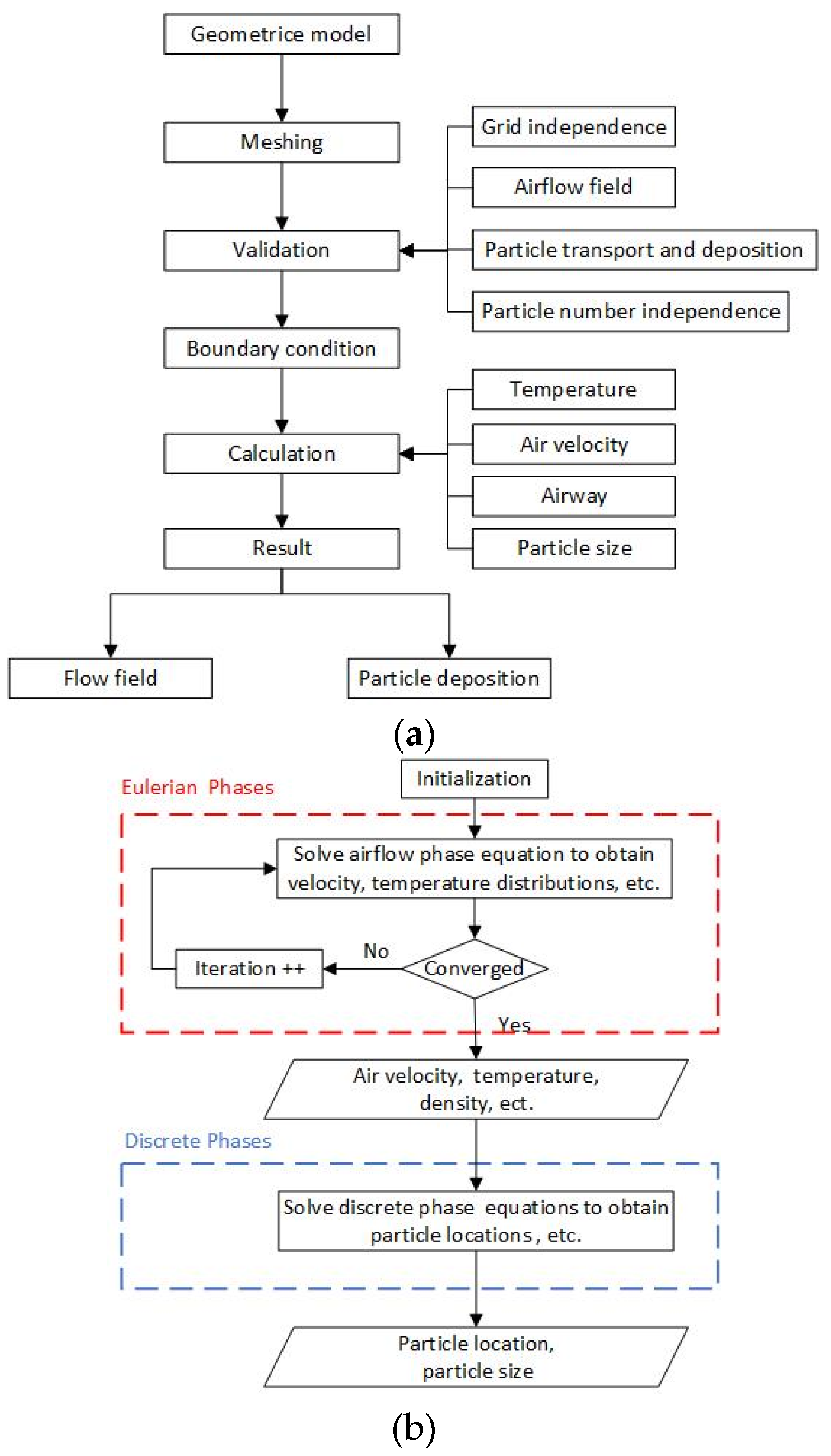
2.3. Grids and Boundary Conditions
2.4. Grid Independence and Model Validation
- The wind speed under different grid numbers was compared, and 7.1 million grids were selected for simulation.
- The results of the validation of the respiratory flow field model and the particle transport and deposition model showed that the transitional SST model can well predict the flow field outside the respiratory tract, and the DPM model can be used to predict the indoor particle deposition.
- The results of particle independence verification showed that when the number of particles was more than 110,000, the absolute value of the difference between the particle deposition rate and the maximum particle deposition was less than 0.02%. Therefore, 110,000 particles were also used in the following simulation.
2.5. Simulation Setup
3. Results and Discussion
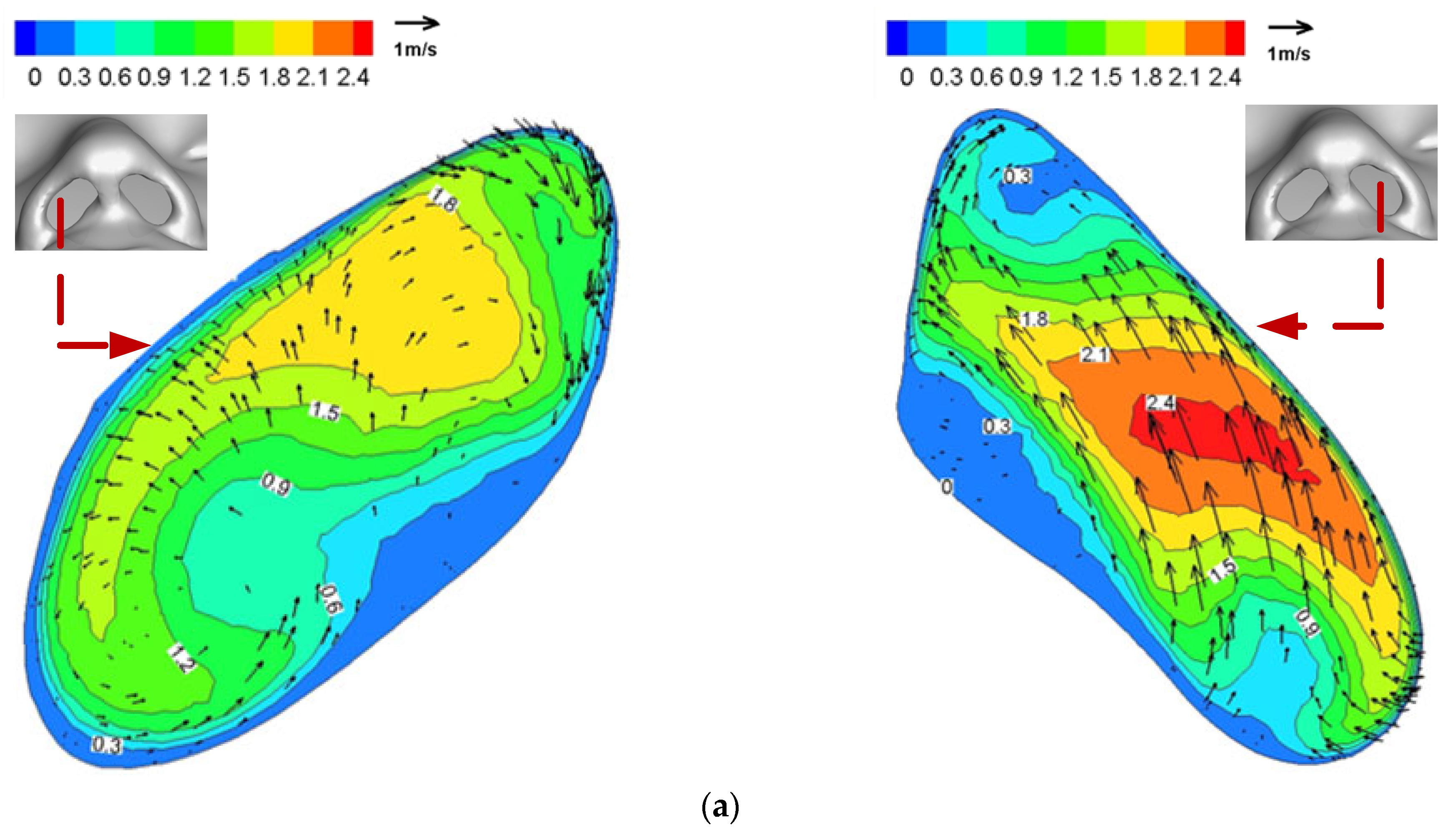
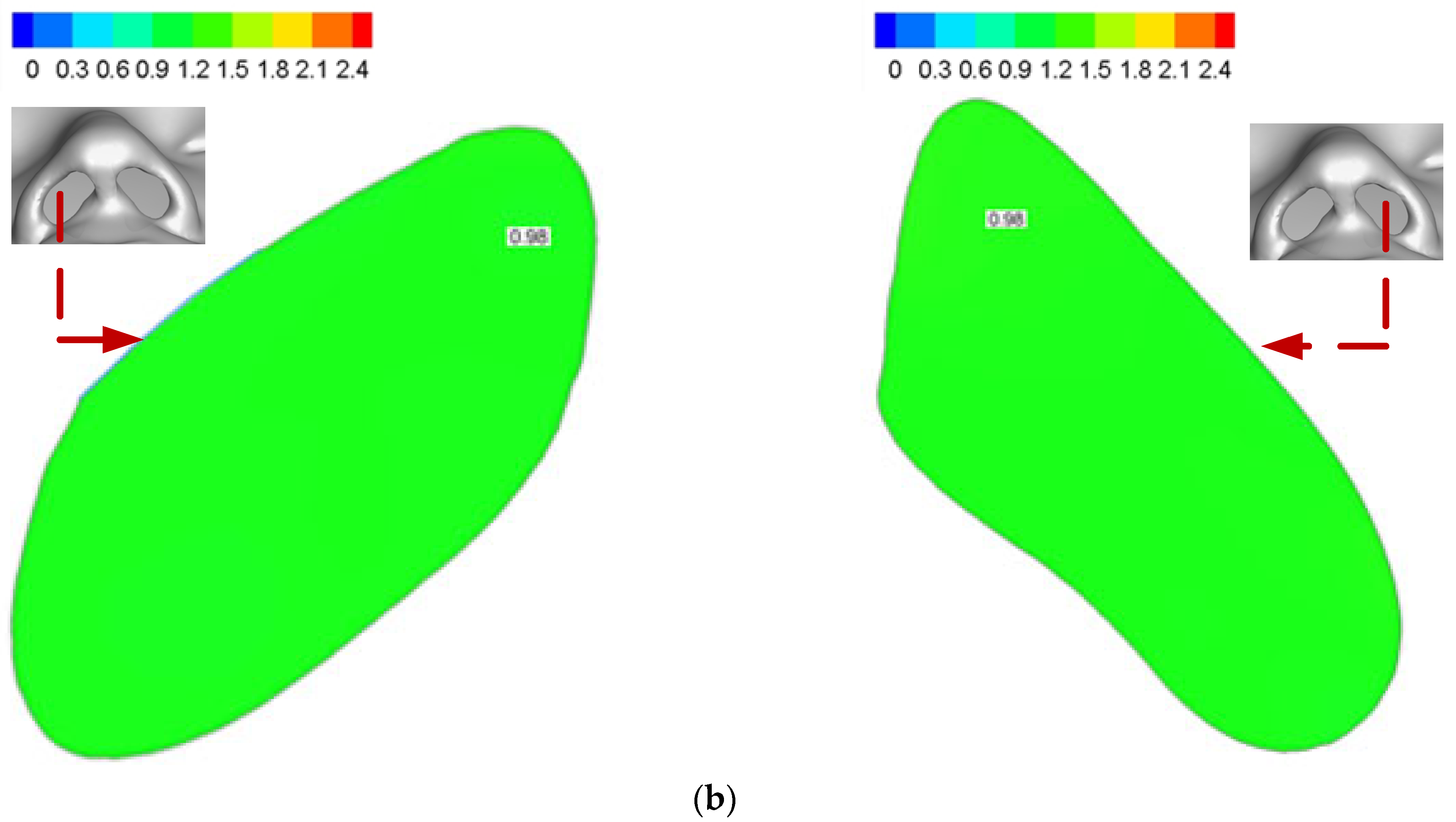
3.1. Airflow Field
3.2. Particle Dispersion and Fate
4. Conclusions
- Feasibility of the method
- 2.
- Influence of particulate matter based on real nasal respiratory tract model
- 3.
- Effect of environmental factors on particulate matter
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Xu, C.; Nielsen, P.V.; Gong, G. Measuring the exhaled breath of a manikin and human subjects. Indoor Air 2015, 25, 188–197. [Google Scholar] [CrossRef]
- Córdova, A.; Latasa, I. Respiratory Flows As A Method For Safely Preventing The Coronavirus Transmission (COVID-19). Apunts Sports Med. 2020, 55, 81–85. [Google Scholar] [CrossRef]
- Morawska, L.; Johnson, G.R.; Ristovski, Z.D.; Hargreaves, M.; Mengersen, K.; Corbett, S.; Chao, C.Y.H.; Katoshevski, D. Size distribution, and sites of origin of droplets expelled from the human respiratory tract during expiratory activities. J. Aerosol. Sci. 2008, 40, 256–269. [Google Scholar] [CrossRef]
- Chen, W.Z.; Zhang, N.; Wei, J.J.; Yen, H.L.; Li, Y.G. Short-range airborne route dominates exposure of respiratory infection during close contact. Build Environ. 2020, 176, 106859. [Google Scholar] [CrossRef]
- Fabian, P.; Mcdevitt, J.J.; Dehaan, W.H.; Fung, R.O.; Cowling, B.J.; Chan, K.H.; Leung, G.M.; Milton, D.K. Influenza virus in human exhaled breath: An observational study. PLoS ONE 2008, 3, e2691. [Google Scholar] [CrossRef] [PubMed]
- Edwards, D.A.; Man, J.C.; Brand, P.; Katstra, J.P.; Sommerer, K.; Stone, H.A.; Nardell, E.; Scheuch, G. Inhaling to mitigate exhaled bioaerosols. Proc. Natl. Acad. Sci. USA 2004, 101, 17383–17388. [Google Scholar] [CrossRef]
- Haghnegahdar, A.; Zhao, J.; Feng, Y. Lung aerosol dynamics of airborne influenza A virus-laden droplets and the resultant immune system responses: An in silico study. J. Aerosol. Sci. 2019, 134, 34–55. [Google Scholar] [CrossRef]
- Zhang, Z.; Kleinstreuer, C.; Chong, S.K.; Cheng, Y.S. Vaporizing Microdroplet Inhalation, Transport, and Deposition in a Human Upper Airway Model. J. Aerosol. Sci. 2004, 38, 36–49. [Google Scholar] [CrossRef]
- Kiasadegh, M.; Emdad, H.; Ahmadi, G.; Abouali, O. Transient numerical simulation of airflow and fibrous particles in a human upper airway model. J. Aerosol. Sci. 2020, 140, 105480. [Google Scholar] [CrossRef]
- Li, X.P.; Niu, J.L.; Gao, N. Spatial distribution of human respiratory droplet residuals and exposure risk for the co-occupant under different ventilation methods. HVAC&R RES. 2011, 17, 432–445. [Google Scholar]
- Gupta, J.K.; Lin, C.H.; Chen, Q. Characterizing exhaled airflow from breathing and talking. Indoor Air 2010, 20, 31–39. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.X.; Feng, G.H.; Bi, Y.; Cai, Y.L.; Zhang, Z.; Cao, G.Y. Distribution of droplet aerosols generated by mouth coughing and nose breathing in an air-conditioned room. Sustain. Cities Soc. 2019, 51, 101721–101730. [Google Scholar] [CrossRef]
- Rencken, G.K.; Rutherford, E.K.; Ghanta, N.; Kongoletos, J.; Glicksman, L. Patterns of SARS-CoV-2 Aerosol Spread in Typical Classrooms. Build. Environ. 2021, 204, 108167–108188. [Google Scholar] [CrossRef] [PubMed]
- Feng, G.H.; Bi, Y.; Zhang, Y.X.; Cai, Y.L.; Huang, K.L. Study on the motion law of aerosols produced by human respiration under the action of a thermal plume of different intensities—ScienceDirect. Sustain. Cities Soc. 2022, 54, 101935–101947. [Google Scholar] [CrossRef] [PubMed]
- Ivanov, M.; Mijorski, S. Assessment of Transient CFD Techniques for Virtual Thermal Manikins’ Breathing Simulations. Environ. Sci.-Proc. Imp. 2019, 6, 241–251. [Google Scholar]
- Feng, Y.; Marchal, T.; Sperry, T.; Yi, H. Influence of wind and relative humidity on the social distancing effectiveness to prevent COVID-19 airborne transmission: A numerical study. J. Aerosol. Sci. 2020, 147, 105585–105594. [Google Scholar] [CrossRef]
- Wang, J.Y.; Tang, K.; Feng, K.; Lin, X.; Lv, W.F.; Chen, K.; Wang, F. Impact of temperature and relative humidity on the transmission of COVID-19: A modeling study in China and the United States. BMJ Open 2021, 11, 043863. [Google Scholar] [CrossRef]
- Jahangiri, M.; Jahangiri, M.; Najafgholipour, M. The sensitivity and specificity analyses of ambient temperature and population size on the transmission rate of the novel coronavirus (COVID-19) in different provinces of Iran. Sci. Total. Environ. 2020, 728, 138872. [Google Scholar] [CrossRef]
- Usmani, M.; Jamal, Y.; Gangwar, M.; Magers, B.; Chaves, G.J.; Wu, C.Y.; Colwell, R.; Jutla, A. Asymmetric Relationship between Ambient Air Temperature and Incidence of COVID-19 in the Human Population. Am. J. Trop. Med. Hyg. 2022, 106, 877–885. [Google Scholar] [CrossRef]
- Centers for Disease Control and Prevention. Available online: https://blogs.cdc.gov/publichealthmatters/2020/04/essential-errands (accessed on 20 April 2020).
- Xie, X.J.; Li, Y.; Chwang, A.T.Y.; Ho, P.L.; Seto, W.H. How far droplets can move in indoor environments--revisiting the Wells evaporation-falling curve. Indoor Air 2007, 17, 211–225. [Google Scholar] [CrossRef]
- Menter, F.R.; Langtry, R.V.; Lker, S. Transition modeling for general purpose CFD codes. Flow Turbul. Combust. 2006, 77, 277–303. [Google Scholar] [CrossRef]
- Zhang, Z.; Kleinstreuer, C. Laminar-to-turbulent fluid–nanoparticle dynamics simulations: Model comparisons and nanoparticle-deposition applications. Int. J. Numer. Methods Eng. 2011, 27, 1930–1950. [Google Scholar] [CrossRef]
- Menter, F.R.; Langtry, R.B.; Völker, S. Transition Modelling for General Purpose CFD Codes. Engineer. Eng. Turbul. Model. Exp. 2005, 77, 31–48. [Google Scholar]
- Menter, F.R.; Langtry, R.B.; Likki, S.R.; Suzen, Y.B.; Huang, P.G.; Völker, S. A Correlation-Based Transition Model Using Local Variables—Part I: Model Formulation. J. Turbomach. 2004, 128, 413–422. [Google Scholar] [CrossRef]
- Chen, F.; Yu, S.; Lai, A. Modeling particle distribution and deposition in indoor environments with a new drift-flux model. Atmos. Environ. 2006, 40, 357–367. [Google Scholar] [CrossRef]
- Gralton, J.; Tovey, E.; McLaws, M.L.; Rawlinson, W.D. The role of particle size in aerosolized pathogen transmission: A review. J. Infect. 2011, 62, 1–13. [Google Scholar] [CrossRef] [PubMed]
- Fairchild, C.I.; Stampfer, J.F. Particle Concentration in Exhaled Breath. AIHAJ 1987, 48, 948–950. [Google Scholar] [CrossRef] [PubMed]
- Gao, N.P.; Niu, J.L.; Morawska, L. Distribution characteristics of respiratory aerosols in enclosed environments. J. Southeast Univ. 2010, 2, 811–818. [Google Scholar]
- Kavousi, F.; Behjat, Y.; Shahhosseini, S. Optimal design of drainage channel geometry parameters in vane demister liquid–gas separators. Chem. Eng. Res. Des. 2013, 91, 1212. [Google Scholar] [CrossRef]
- Chao, C.; Wan, M.P. A study of the dispersion of expiratory aerosols in unidirectional downward and ceiling-return type airflows using a multiphase approach. Indoor Air 2006, 16, 296–312. [Google Scholar] [CrossRef]
- Zhao, B.; Yang, C.; Yang, X.; Liu, S. Particle dispersion and deposition in ventilated rooms: Testing and evaluation of different Eulerian and Lagrangian models. Build. Environ. 2008, 43, 388–397. [Google Scholar] [CrossRef]
- Walsh, J.K.; Weimer, A.W.; Hrenya, C.M. Thermophoretic deposition of aerosol particles in laminar tube flow with mixed convection. JAS 2006, 37, 715–734. [Google Scholar] [CrossRef]
- Gao, N.; He, Q.; Niu, J. Numerical study of the lock-up phenomenon of human exhaled droplets under a displacement ventilated room. Build. Simul. China 2012, 5, 51–60. [Google Scholar] [CrossRef]
- Yu, L.; Wei, G.; Wang, D.Z.; Wang, C. Direct Numerical Simulation on Turbulent Transportation and Thermophoretic Deposition of Micron-Sized Particles in Rectangle Channel. In Proceedings of the 2016 International Conference on Environment, Climate Change, and Sustainable Development (ECCSD 2016), Beijing, China, 28 May 2016. [Google Scholar]
- Park, A.W.; Reitz, R.D. A gas jet superposition model for CFD modeling of group-hole nozzle sprays. Int. J. Heat Fluid Flow 2009, 30, 1193–1201. [Google Scholar] [CrossRef]
- Pulat, W.P.; Beyazoglu, E. Computational investigation of confined wall inclination effects on impinging jet fluid flow and heat transfer. Int. J. Therm. Sci. 2021, 163, 106749. [Google Scholar] [CrossRef]
- Chen, X.; Feng, Y.; Zhong, W.; Kleinstreuer, C. Numerical investigation of the interaction, transport, and deposition of multicomponent droplets in a simple mouth-throat model. JAS 2017, 105, 108–127. [Google Scholar] [CrossRef]
- Settles, G.S.; Hargather, M.J. A review of recent developments in schlieren and shadowgraph techniques. Meas. Sci. Technol. 2017, 28, 042001. [Google Scholar] [CrossRef]
- Bivolarova, M.; Ondráček, J.; Melikov, A.; Ždímal, V. A comparison between tracer gas and aerosol particles distribution indoors: The impact of ventilation rate, interaction of airflows, and presence of objects. Indoor Air 2017, 27, 1201–1212. [Google Scholar] [CrossRef]


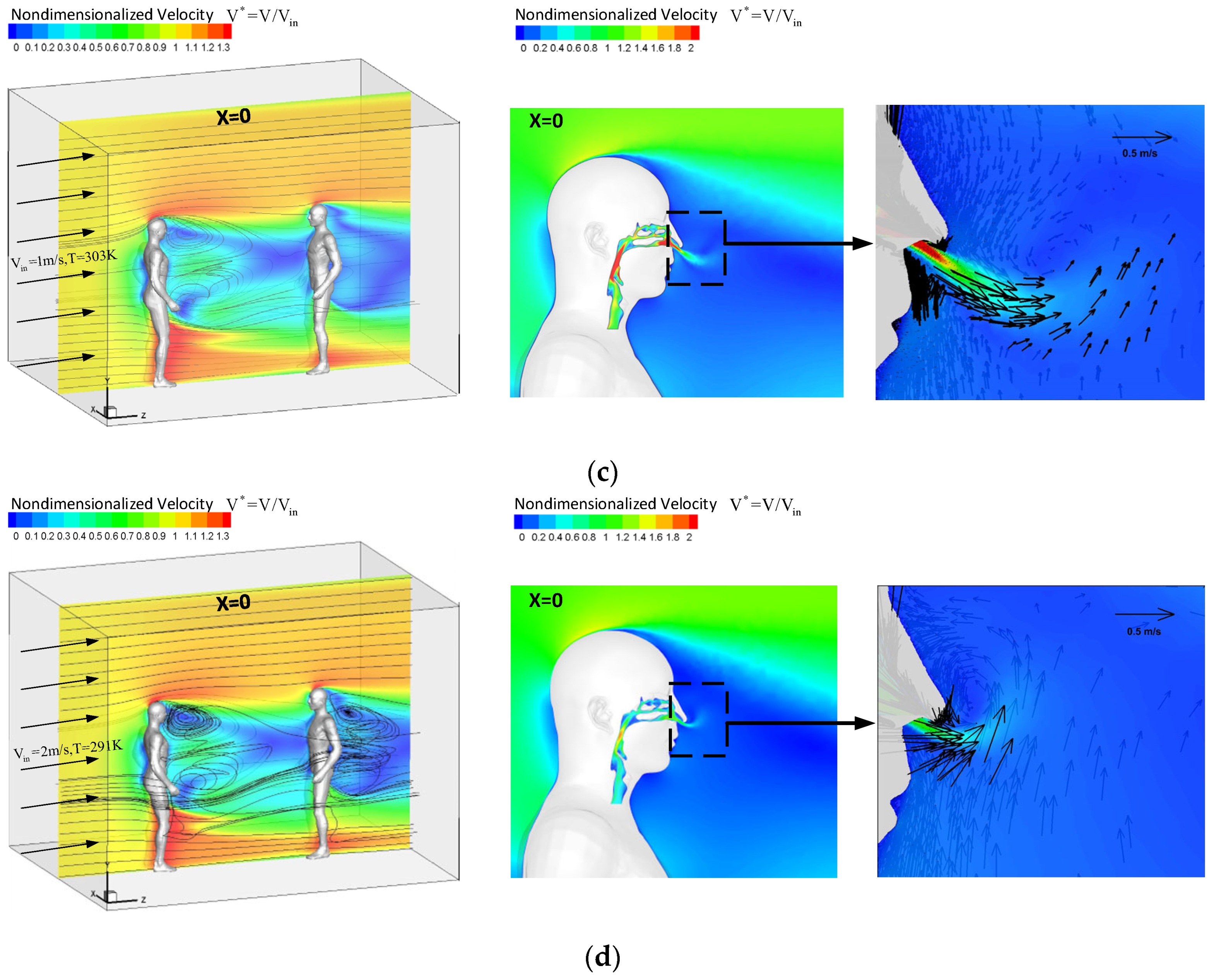
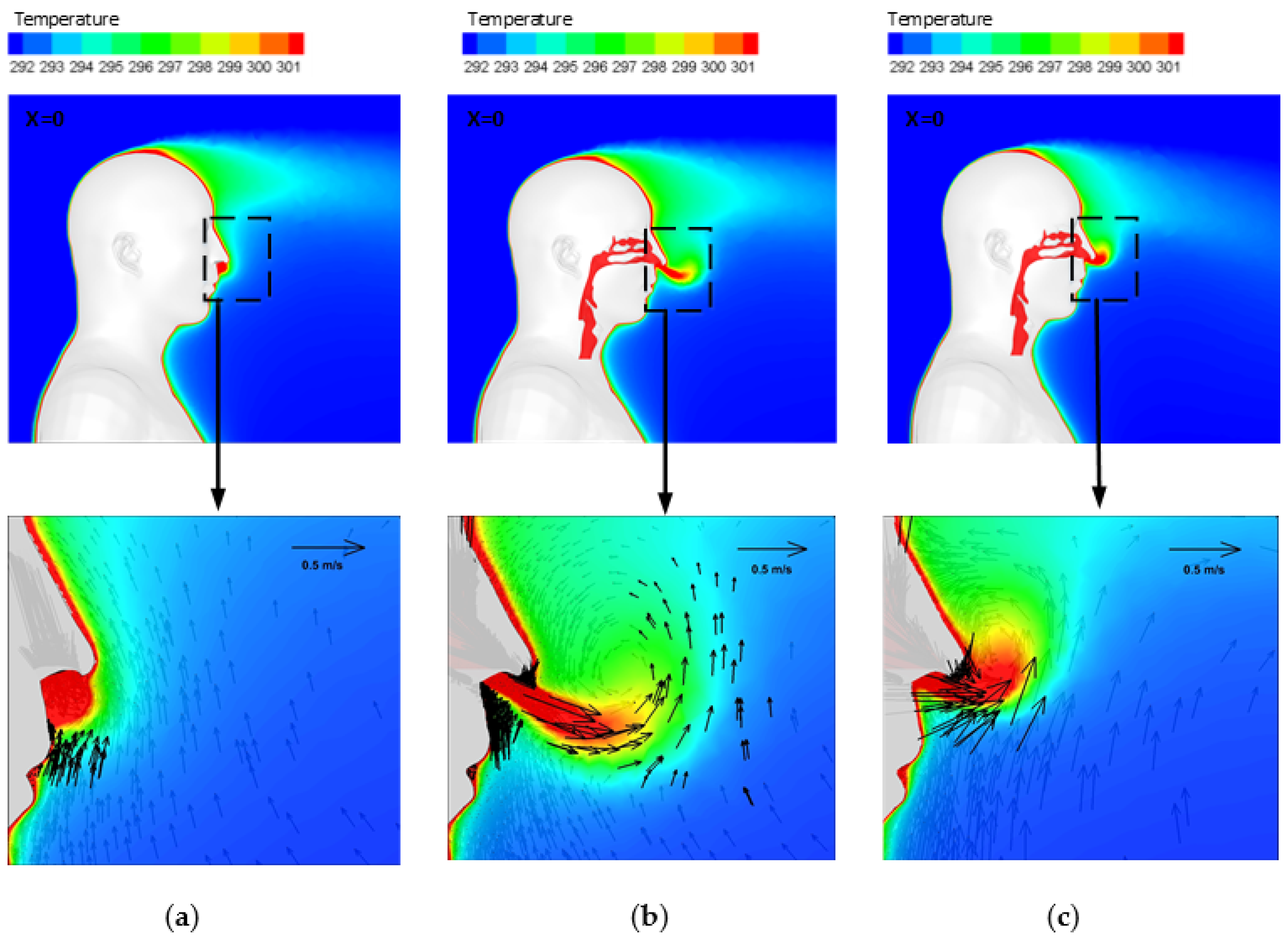
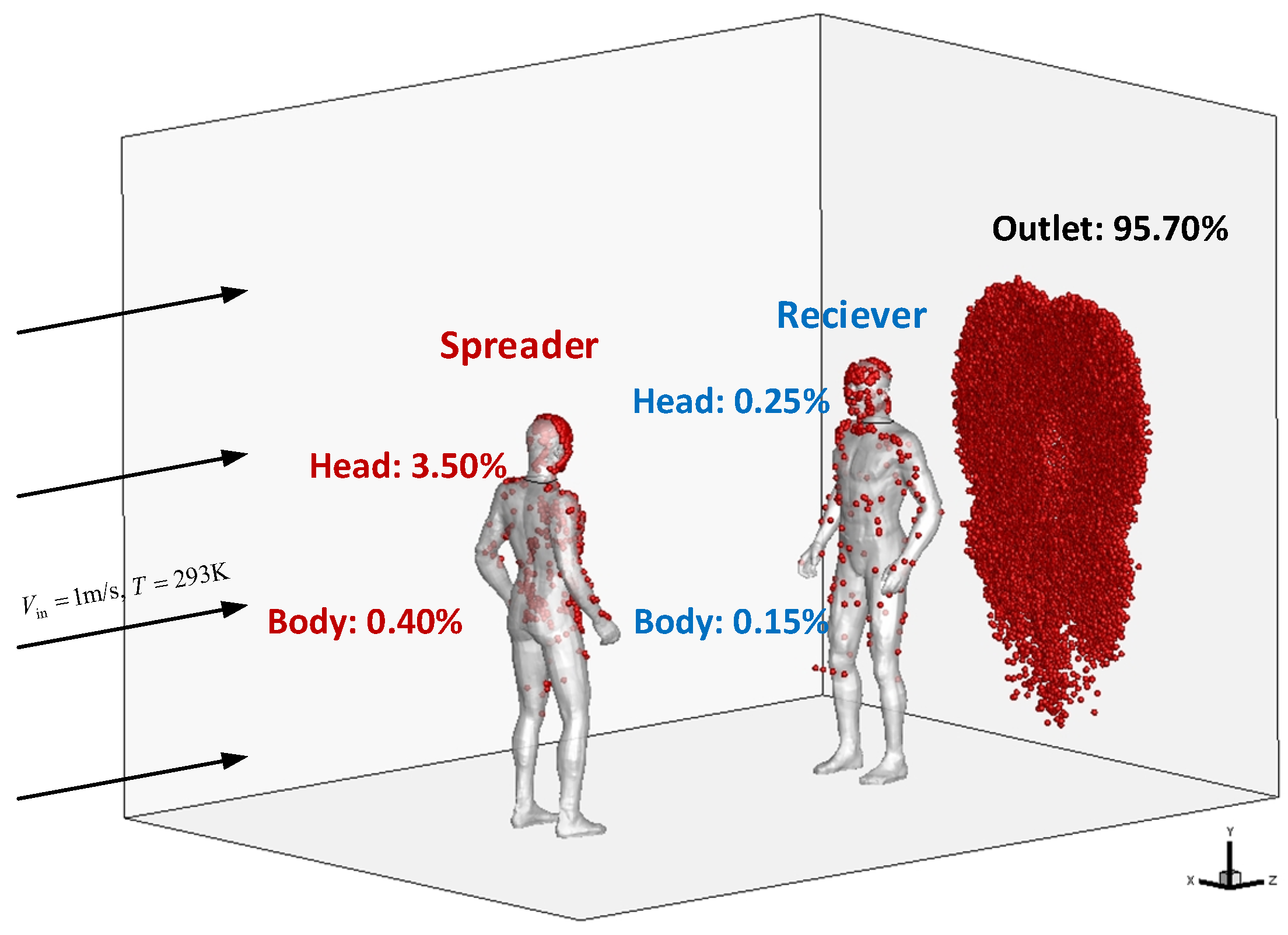
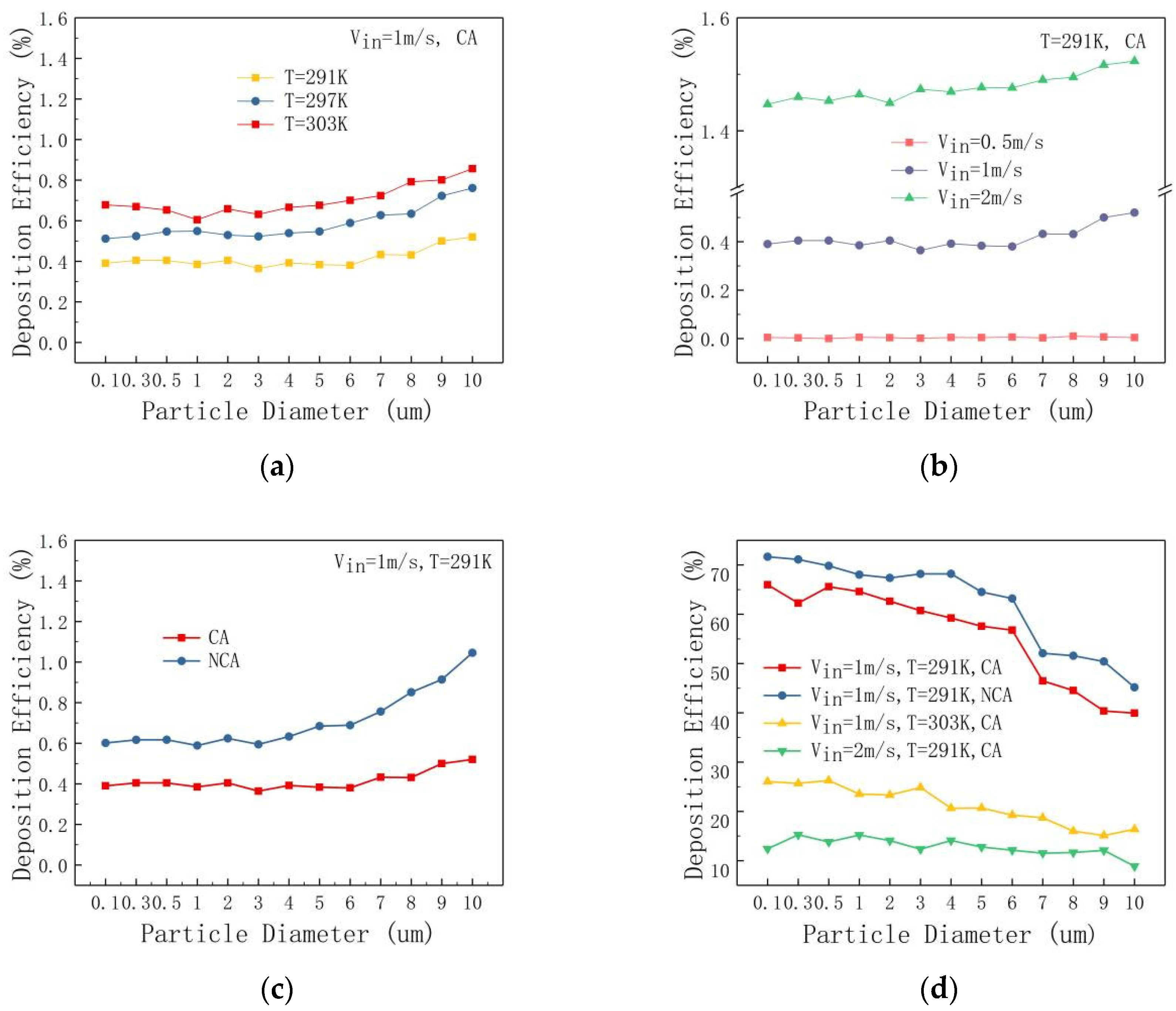
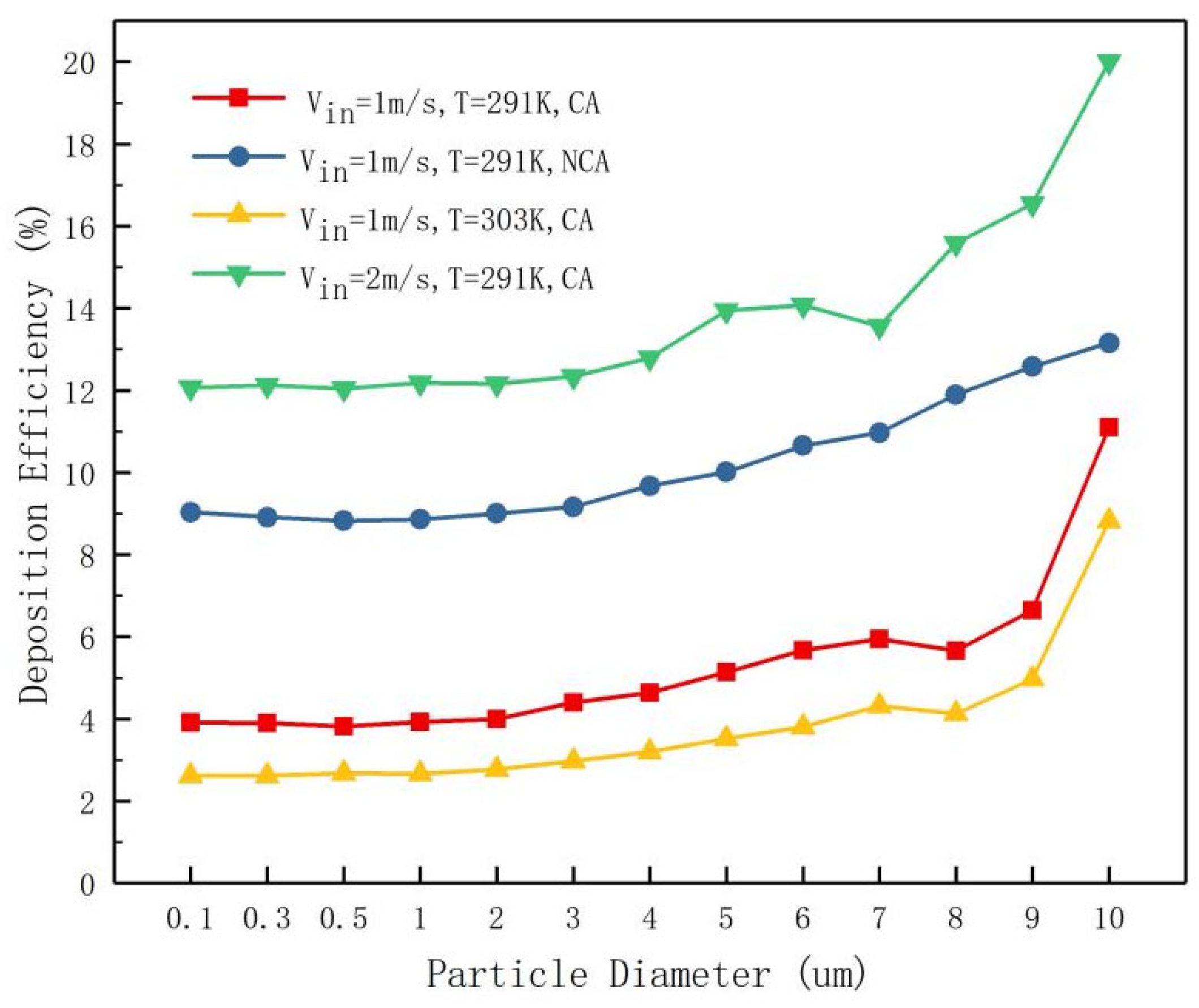
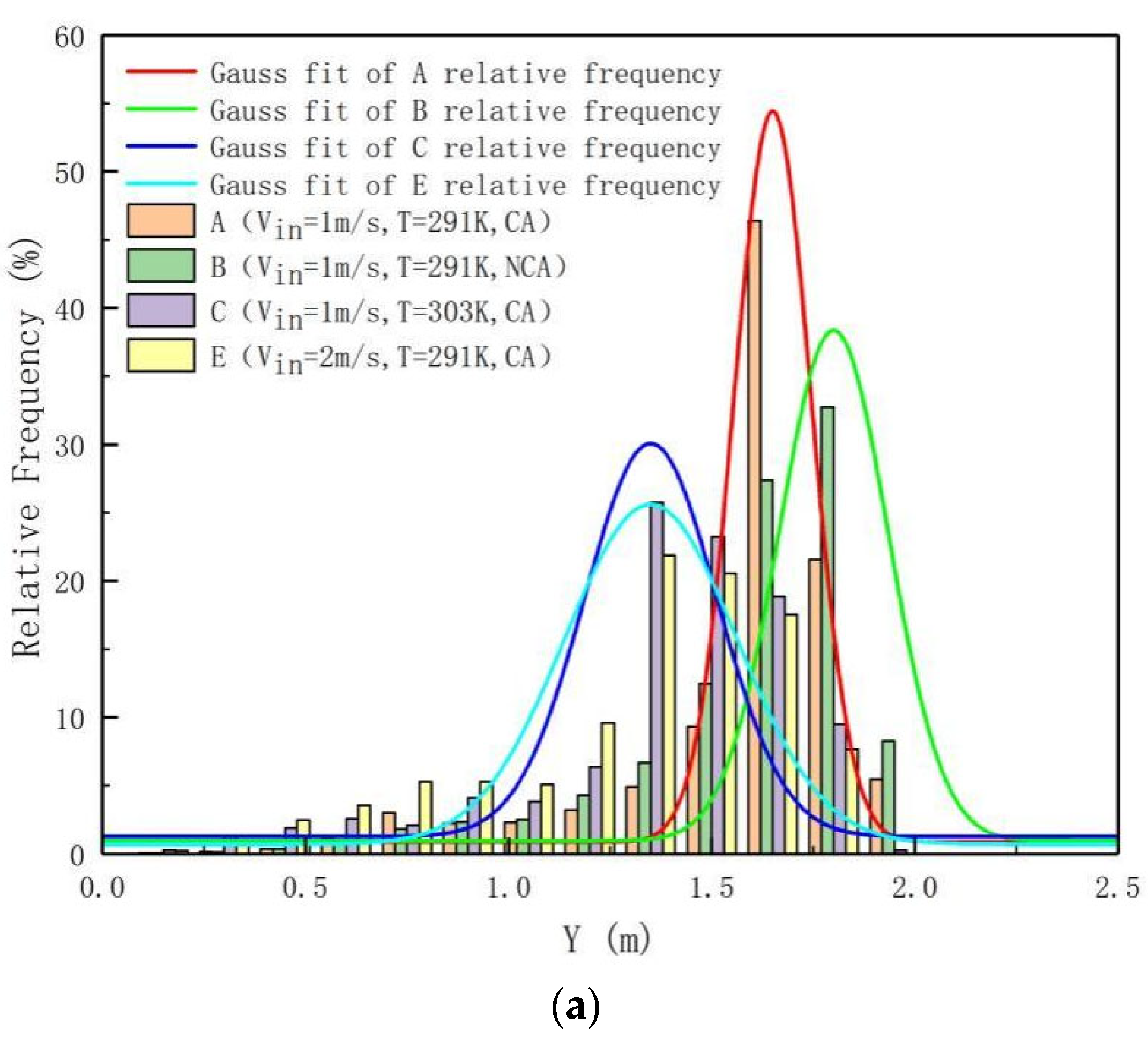
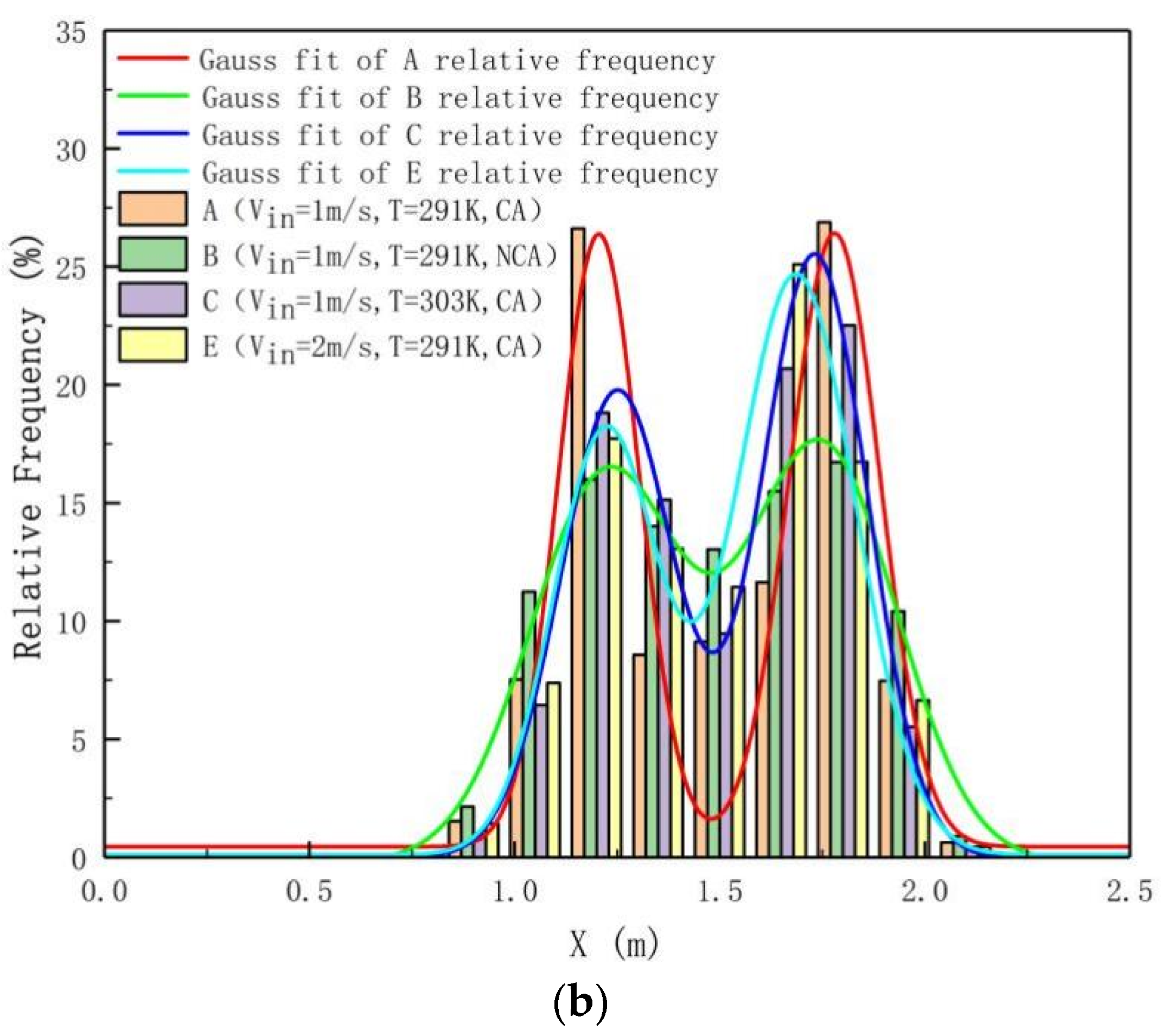
| Condition | Airway | Temperature (K) | Velocity (m/s) |
|---|---|---|---|
| A | existence | 291 | 1 |
| B | non-existent | 291 | 1 |
| C | existence | 303 | 1 |
| D | existence | 291 | 0.5 |
| E | existence | 291 | 2 |
| F | existence | 297 | 1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wei, J.; Xie, H.; Chen, X.; Quan, X.; Zhang, Z.; Xie, X.; Shi, J.; Zeng, G. Numerical Simulation of the Dispersion of Exhaled Aerosols from a Manikin with a Realistic Upper Airway. Atmosphere 2022, 13, 2050. https://doi.org/10.3390/atmos13122050
Wei J, Xie H, Chen X, Quan X, Zhang Z, Xie X, Shi J, Zeng G. Numerical Simulation of the Dispersion of Exhaled Aerosols from a Manikin with a Realistic Upper Airway. Atmosphere. 2022; 13(12):2050. https://doi.org/10.3390/atmos13122050
Chicago/Turabian StyleWei, Jiayu, Hao Xie, Xiaole Chen, Xibin Quan, Zhicong Zhang, Xiaojian Xie, Jianping Shi, and Guanghui Zeng. 2022. "Numerical Simulation of the Dispersion of Exhaled Aerosols from a Manikin with a Realistic Upper Airway" Atmosphere 13, no. 12: 2050. https://doi.org/10.3390/atmos13122050
APA StyleWei, J., Xie, H., Chen, X., Quan, X., Zhang, Z., Xie, X., Shi, J., & Zeng, G. (2022). Numerical Simulation of the Dispersion of Exhaled Aerosols from a Manikin with a Realistic Upper Airway. Atmosphere, 13(12), 2050. https://doi.org/10.3390/atmos13122050







