Equivalent Current Systems of Quiet Ionosphere during the 24th Solar Cycle Derived from the Geomagnetic Records in China
Abstract
1. Introduction
2. Materials and Methods
3. Results
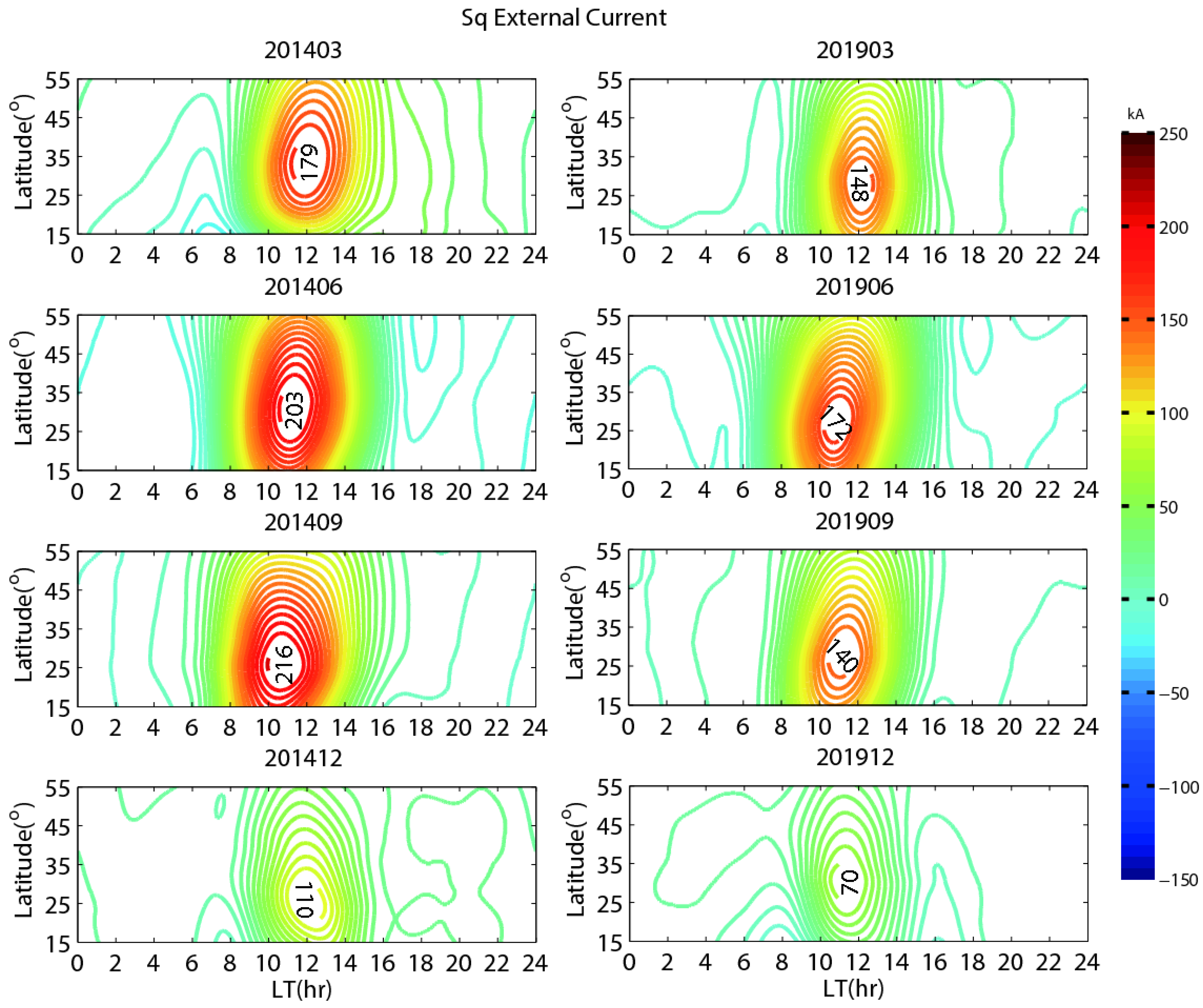
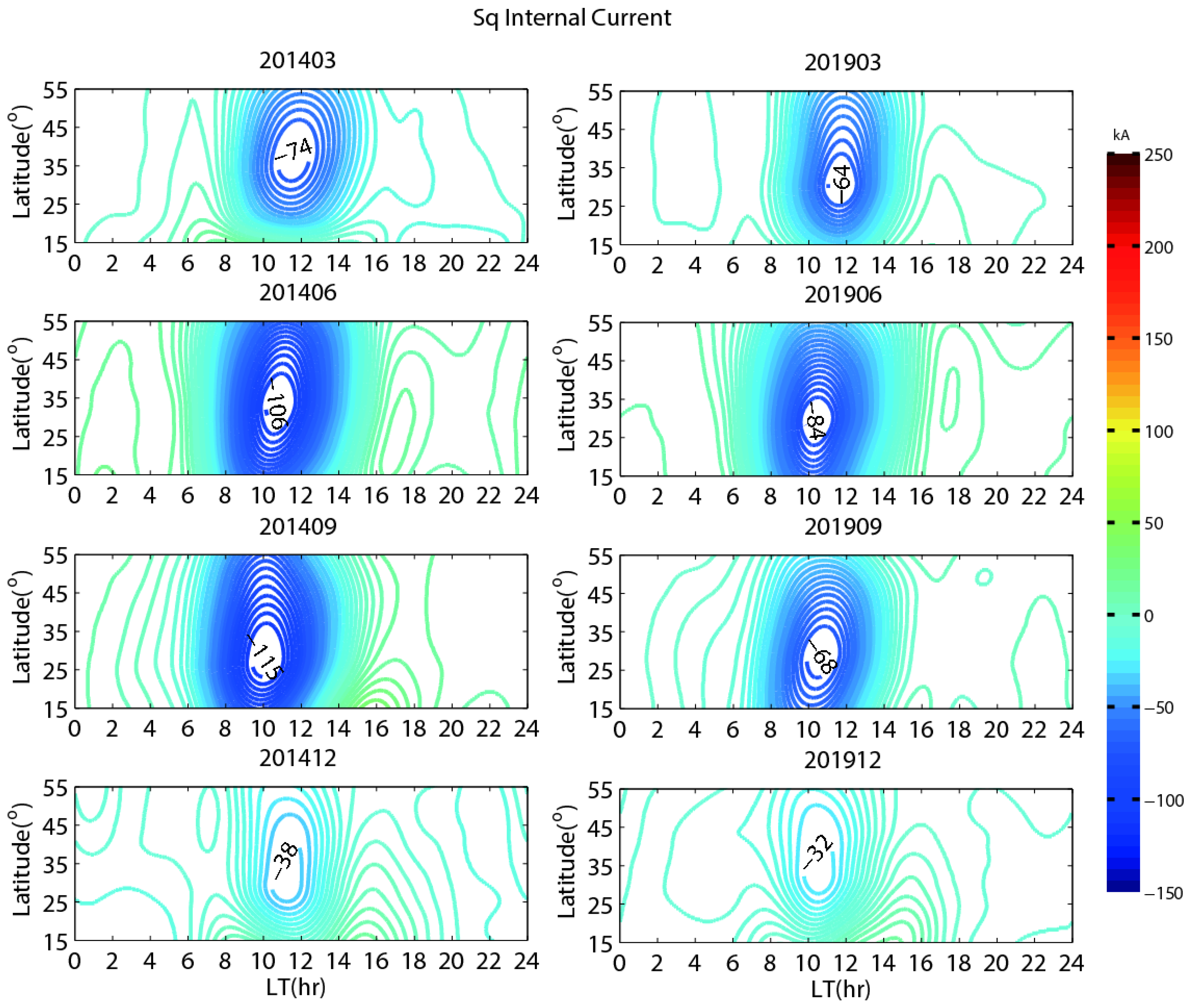
3.1. Sq Currents in Solar Maximum and Minimum Years
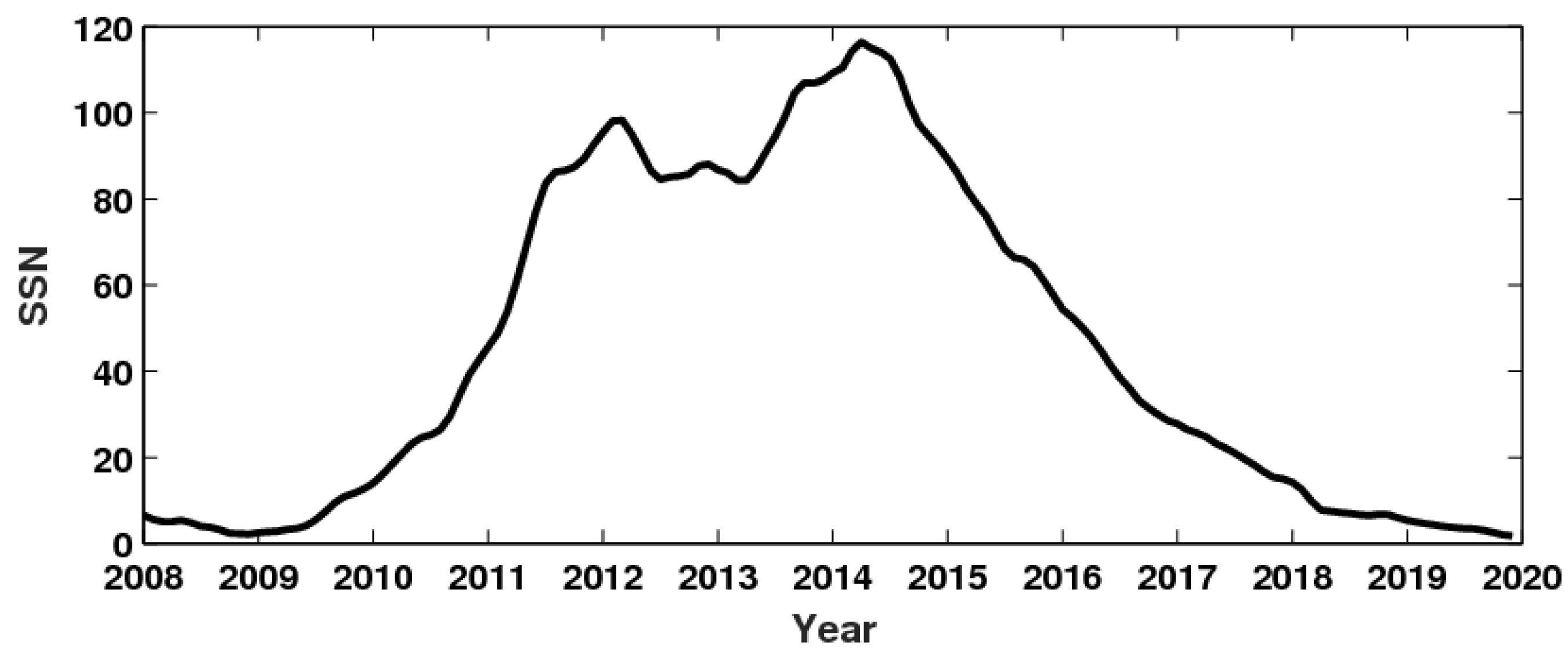
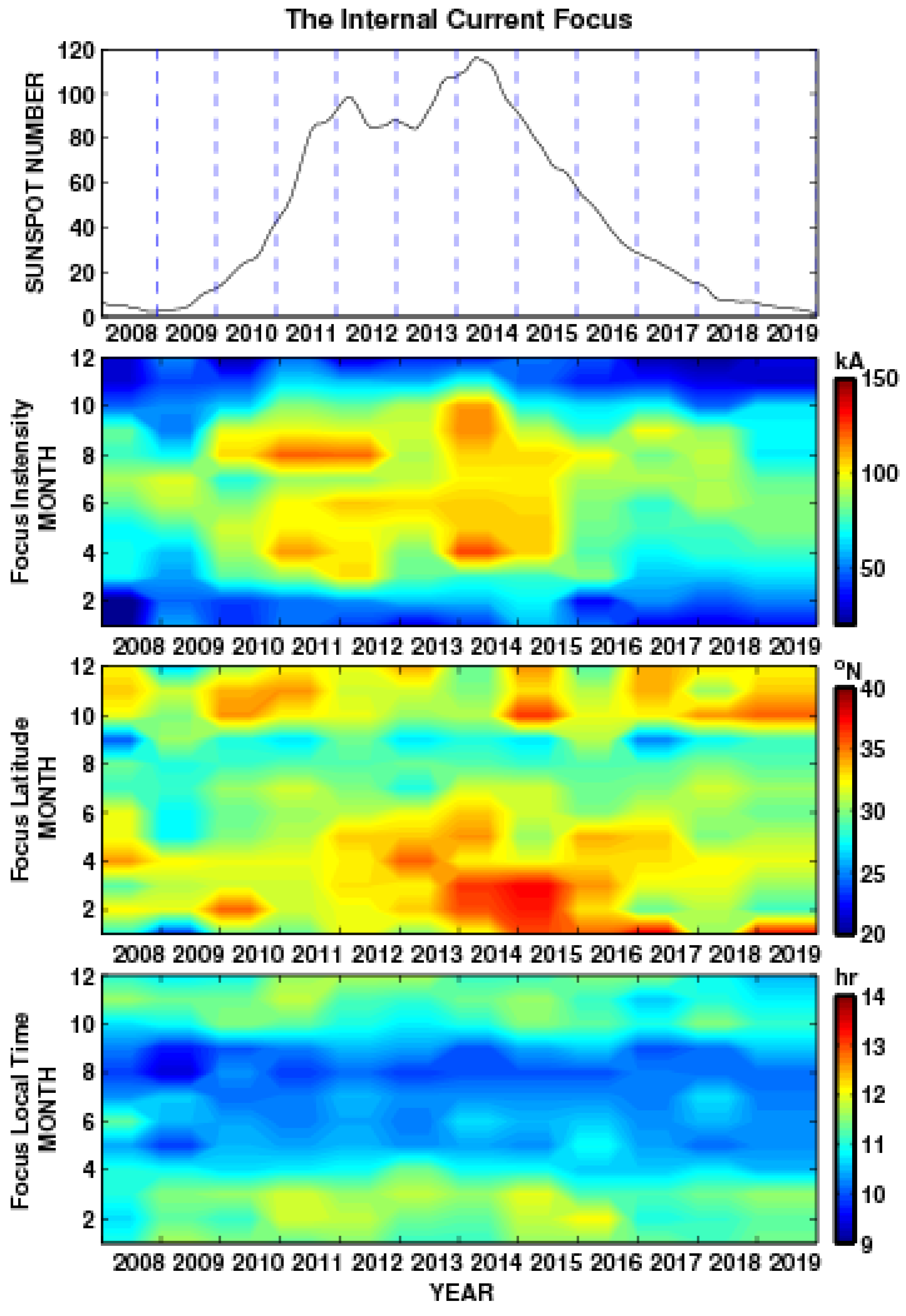
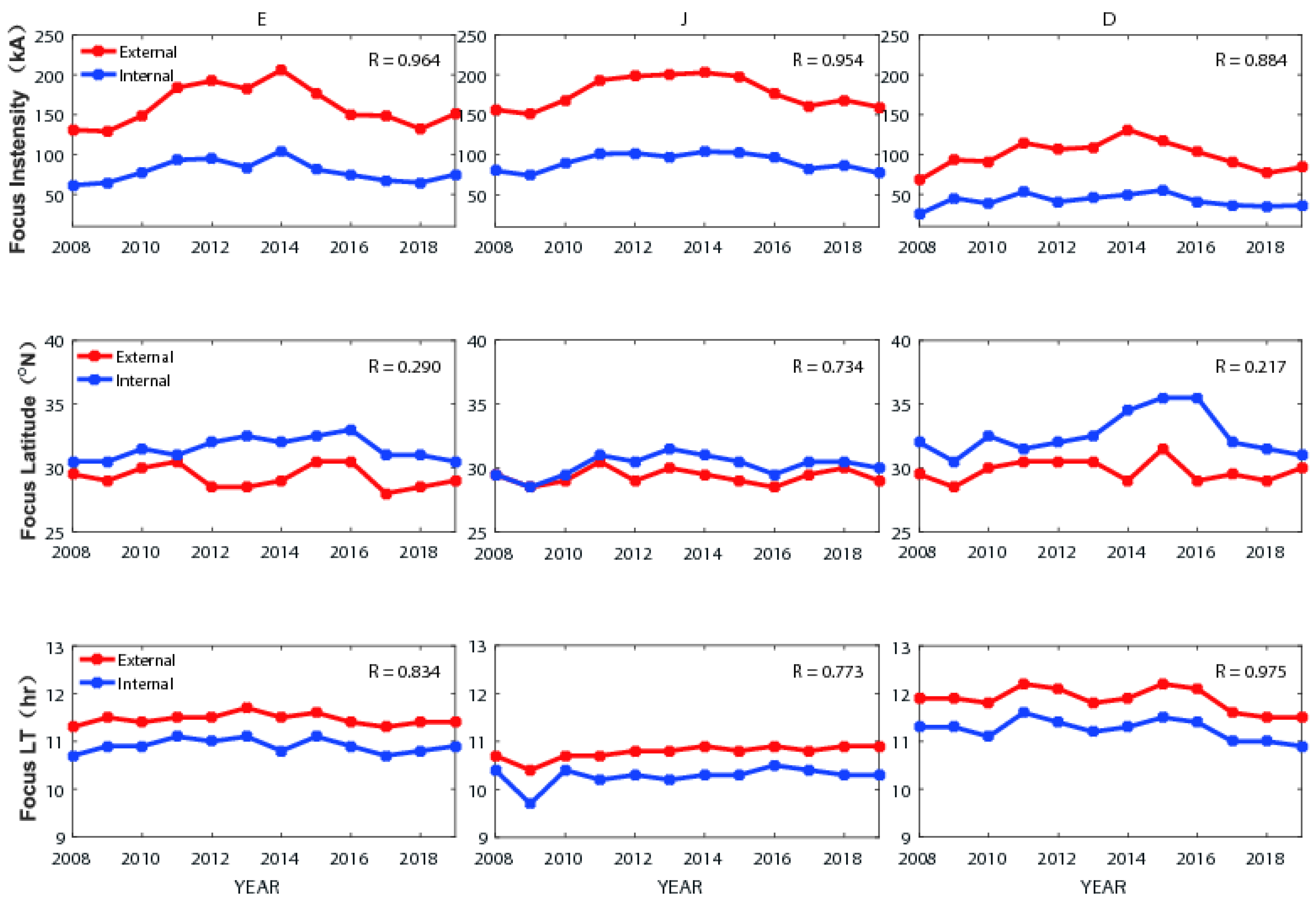
3.2. Variation of Sq Currents in the Solar Cycle
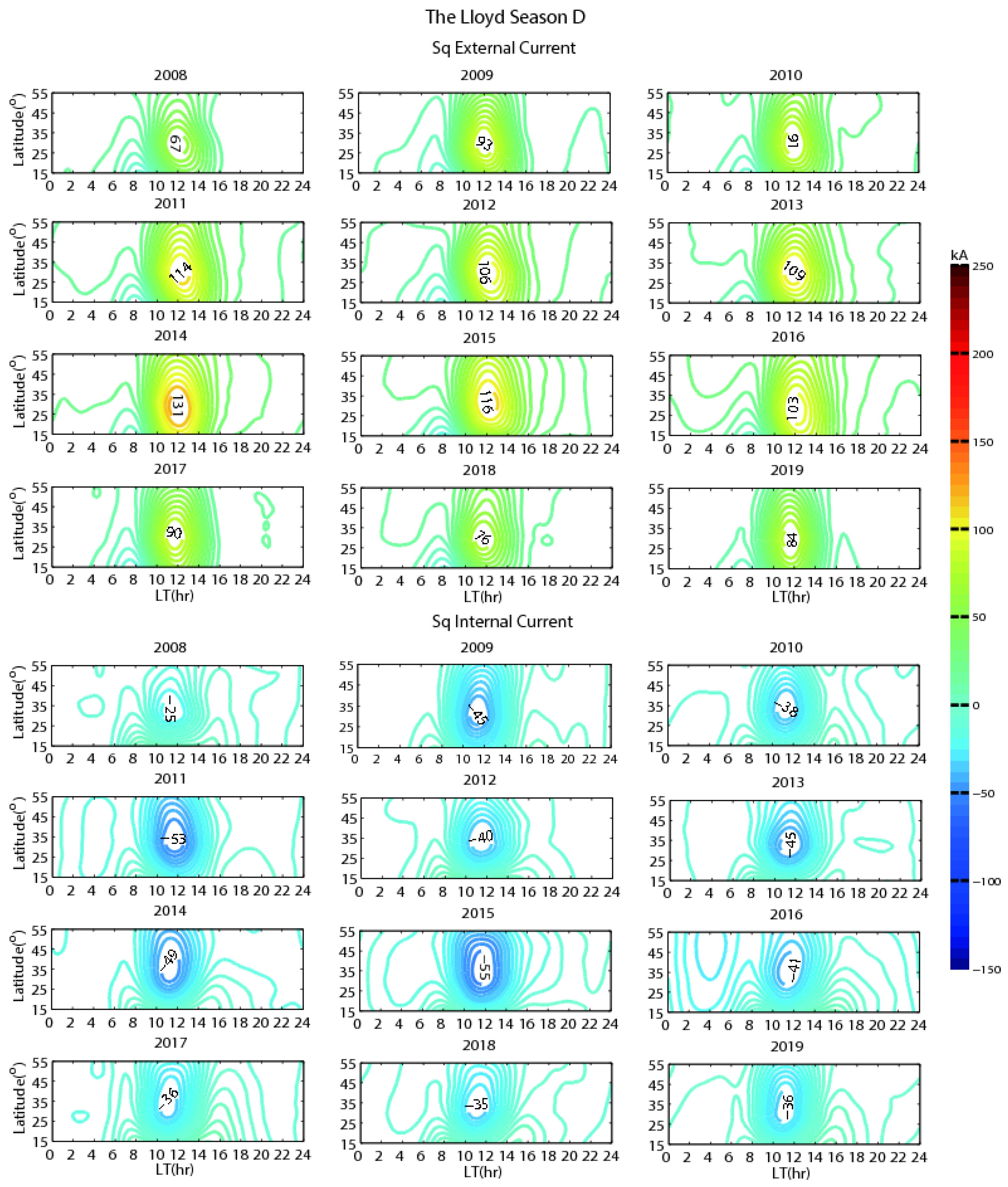
3.3. Variations of Sq currents in Lloyd seasons
4. Discussion
5. Conclusions
- The intensities of both Sq external and internal currents are consistent with solar activity, and are stronger in years with higher solar activity than in years with lower solar activity for the same month or season. However, the positions of the current foci are evidently unaffected by solar activity.
- The intensities of the Sq currents exhibit primary semiannual variation during periods of higher solar activity and obvious annual variation during periods of lower solar activity.
- The latitude of the internal current vortex shows evident seasonal variation in the Lloyd seasons, while the external one presents no clear seasonal variation.
- The strongest correlation exists in the D season for the local time between the external and internal foci in the China region. The local time of the internal current foci is usually 20–40 min earlier than that of the external current foci.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Stening, R.; Reztsova, T.; Ivers, D.; Turner, J.; Winch, D. A critique of methods of determining the position of the focus of the Sq current system. J. Geophys. Res. Space Phys. 2005, 110, A04305110. [Google Scholar] [CrossRef]
- Stening, R.; Reztsova, T.; Minh, L.H. Day-to-day changes in the latitudes of the foci of the Sq current system and their relation to equatorial electrojet strength. J. Geophys. Res. Space Phys. 2005, 110, A10308. [Google Scholar] [CrossRef]
- Xu, W. Geomagnetism; Seismological Press: Beijing, China, 2003. (In Chinese) [Google Scholar]
- Matsushita, S. Solar quiet and lunar daily variation fields. In Physics of Geomagnetic Phenomena; Matsushita, S., Campbell, W.H., Eds.; Academic: New York, NY, USA, 1967; pp. 302–424. [Google Scholar]
- Matsushita, S. Morphology of slowly-varying geomagnetic external fields—A review. Phys. Earth Planet. Inter. 1975, 10, 299–312. [Google Scholar] [CrossRef]
- Richmond, A.D.; Matsushita, S.; Tarpley, J.D. On the production mechanism of electric currents and fields in the ionosphere. J. Geophys. Res. Space Phys. 1976, 81, 547–555. [Google Scholar] [CrossRef]
- Richmond, A.D. Ionospheric wind dynamo theory: A review. J. Geomagtn. Geoelec. 1979, 31, 287–310. [Google Scholar] [CrossRef]
- Forbes, J.M.; Lindzen, R.S. Atmospheric solar tides and their electrodynamic effects, I: The global Sq current system. J. Atmos. Terr. Phys. 1976, 38, 897–910. [Google Scholar] [CrossRef]
- Malin, S.R.C.; Gupta, J.C. The Sq current system during the International Geophysical Year. Geophys. J. R. Astron. Soc. 1977, 49, 515–529. [Google Scholar] [CrossRef]
- Rees, D. Rocket Measurements of Annual Mean Prevailing, Diurnal and Semi-Diurnal Winds in the Lower Thermosphere at Mid-Latitudes. J. Geomagn. Geoelec. 1979, 31, 253–265. [Google Scholar] [CrossRef][Green Version]
- Takeda, M. Day-to-day variation of equivalent Sq current system during March 11–26, 1970. J. Geomagn. Geoelectr. 1984, 36, 215–338. [Google Scholar] [CrossRef]
- Campbell, W.H.; Schiffmacher, E.R. Quiet ionospheric currents of the northern hemisphere derived from geomagnetic field records. J. Geophys. Res. 1985, 90, 6475–6486. [Google Scholar] [CrossRef]
- Campbell, W.H.; Schiffmacher, E.R. Quiet Ionospheric Currents and Earth Conductivity Profile Computed from Quiet-time Geomagnetic Field Changes in the Region of Australia. Aust. J. Phys. 1987, 40, 73–87. [Google Scholar] [CrossRef]
- Takeda, M. Contribution of wind, conductivity, and geomagnetic main field to the variation in the geomagnetic Sq field. J. Geophys. Res. Space Phys. 2013, 118, 4516–4522. [Google Scholar] [CrossRef]
- Pedatella, N.M.; Forbes, J.M.; Richmond, A.D. Seasonal and longitudinal variations of the solar quiet (Sq) current system during solar minimum determined by CHAMP satellite magnetic field observations. J. Geophys. Res. 2011, 116, A04317. [Google Scholar] [CrossRef]
- Yamazaki, Y.; Yumoto, K.; Cardinal, M.G.; Fraser, B.J.; Hattori, P.; Kakinami, Y.; Liu, J.Y.; Lynn, K.J.W.; Marshall, R.; McNamara, D.; et al. An empirical model of the quiet daily geomagnetic field variation. J. Geophys. Res. 2011, 116, A10312. [Google Scholar]
- Hibberd, F.H. Day-to-day variability of the Sq geomagnetic field variation. Aust. J. Phys. 1981, 34, 81–90. [Google Scholar] [CrossRef]
- Xu, W. Physics of Electromagnetic Phenomena of the Earth; Press of University of Science and Technology of China: Hefei, China, 2009. (In Chinese) [Google Scholar]
- Price, A.T. Electromagnetic induction within the Earth. In Physics of Geomagnetic Phenomena; Matsushita, S., Campbell, W.H., Eds.; Academic: New York, NY, USA, 1967; pp. 235–295. [Google Scholar]
- Gauss, C.F. Allgemeine Theorie des Erdmagnetismus, Resultate aus den Beobachtungen des Magnetischen Vereins im Jahre 1838. In Scientific Memoirs Selected from the Transactions of Foreign Academies and Learned Societies and from Foreign Journals; Gauss, C.F., Weber, W.W., Eds.; Sabine, E.; Taylor, R., Translators; Taylor: London, UK, 1841; pp. 184–251. [Google Scholar]
- Schuster, A. The diurnal variation of terrestrial magnetism. Philos. Trans. R. Soc. Lond. Ser. A. 1889, 180, 467–518. [Google Scholar]
- Chapman, S.; Bartels, J. Geomagnetism; Oxford University Press: Oxford, UK, 1940. [Google Scholar]
- Winch, D.E. Spherical harmonic analysis of geomagnetic tides, 1964–1965. Philos. Trans. R. Soc. 1981, A303, 1–104. [Google Scholar] [CrossRef]
- Takeda, M. Time variation of global geomagnetic Sq field in 1964 and 1980. J. Atmos. Sol.-Terr. Phys. 1999, 61, 765–774. [Google Scholar] [CrossRef]
- Takeda, M. Features of global geomagnetic Sq field from 1980 to 1990. J. Geophys. Res. 2002, 107, 1252. [Google Scholar] [CrossRef]
- Zhao, X.; Yang, D.; He, Y.; Yu, P.; Liu, X.; Zhang, S.; Luo, K.; Hu, X. The study of Sq equivalent current during the solar cycle. Chin. J. Geophys. 2014, 57, 3777–3788. [Google Scholar]
- Stening, R.J.; Winch, D.E. The ionospheric Sq current system obtained by spherical harmonic analysis. J. Geophys. Res. Space Phys. 2013, 118, 1288–1297. [Google Scholar] [CrossRef]
- Yamazaki, Y.; Yumoto, K.; Uozumi, T.; Yoshikawa, A.; Cardinal, M.G. Equivalent current systems for the annual and semiannual Sq variations observed along the 210-MM CPMN stations. J. Geophys. Res. 2009, 114, A12320. [Google Scholar]
- Liu, X.; Han, P.; Hattori, K.; Chen, H.; Yoshino, C.; Zhao, X.; Jiao, L.; Ma, X.; Lei, Y. Seasonal variation characteristics of geomagnetic Sq external and internal equivalent current systems in East-Asia and Oceania regions. J. Geophys. Res. Space Phys. 2021, 126, e2021JA029113. [Google Scholar] [CrossRef]
- Campbell, W.H.; Arora, B.R.; Schiffmacher, E.R. External Sq currents in the India-Siberia region. J. Geophys. Res. 1993, 98, 3741–3752. [Google Scholar] [CrossRef]
- Campbell, W.H.; Schiffmacher, E.R. Quiet ionospheric currents of the southern hemisphere derived from geomagnetic records. J. Geophys. Res. 1988, 93, 933–944. [Google Scholar] [CrossRef]
- Sutcliffe, P.R. The development of a regional geomagnetic daily variation model using neural networks. Ann. Geophys. 2000, 18, 120–128. [Google Scholar] [CrossRef]
- Xu, W.; Kamide, Y. Decomposition of daily geomagnetic variations by using method of natural orthogonal component. J. Geophys. Res. 2004, 109, A05218. [Google Scholar] [CrossRef]
- Chen, G.; Xu, W.; Du, A.; Wu, Y.; Chen, B.; Liu, X. Statistical characteristics of the day-to-day variability in the geomagnetic Sq field. J. Geophys. Res. 2007, 112, A06320. [Google Scholar] [CrossRef]
- Zhao, X.; Du, A.; Xu, W.; Hong, M.; Liu, L.; Wei, Y.; Wang, C. The origin of the prenoon-postnoon asymmetry for Sq current system. Chin. J. Geophys. 2008, 51, 643–649. [Google Scholar] [CrossRef]
- Matsushita, S.; Maeda, H. On the geomagnetic solar quiet daily variation field during the IGY. J. Geophys. Res. 1965, 70, 2535–2558. [Google Scholar] [CrossRef]
- Campbell, W.H.; Matsushita, S. Sq currents: A comparison of quiet and active year behavior. J. Geophys. Res. 1982, 87, 5305–5308. [Google Scholar] [CrossRef]
- Stening, R.J. Variations in the strength of the Sq current system. Ann. Geophys. 1995, 13, 627–632. [Google Scholar] [CrossRef]
- Campbell, W.H. A description of the external and internal quiet daily variation currents at North American locations for a quiet-Sun year. Geophys. J. R. Astron. Soc. 1983, 73, 51–64. [Google Scholar] [CrossRef]
- Zhang, S.; Fu, C.; He, Y.; Yang, D.; Li, Q.; Zhao, X.; Wang, J. Quality control of observation data by the geomagnetic network of China. Data Sci. J. 2016, 15, 15. [Google Scholar] [CrossRef]
- Xin, C.; Zhang, S. The analysis of baselines for different fluxgate theodolites of geomagnetic observatories. Data Sci. J. 2011, 10, IAGA159–IAGA168. [Google Scholar]
- Zhang, S.; Yang, D. Study on the stability and accuracy of baseline values measured during the calibrating time intervals. Data Sci. J. 2011, 10, IAGA19–IAGA24. [Google Scholar] [CrossRef]
- Matsushita, S.; Campbell, W.H. Lunar semidiurnal variations of geomagnetic field determined from the 2.5-min data scalings. J. Atmos. Terr. Phys. 1972, 34, 1187–1200. [Google Scholar] [CrossRef]
- Campbell, W.H. Annual and semiannual changes of the quiet daily variations (Sq) in the geomagnetic field at North American locations. J. Geophys. Res. 1982, 87, 785–796. [Google Scholar] [CrossRef]
- Yamazaki, Y.; Maute, A. Sq and EEJ—A Review on the Daily Variation of the Geomagnetic Field Caused by Ionospheric Dynamo Currents. Space Sci. Rev. 2017, 206, 299–405. [Google Scholar] [CrossRef]
- Kuvshinov, A.V.; Manoj, C.; Olsen, N.; Sabaka, T. On induction effects of geomagnetic daily variations from equatorial electrojet and solar quiet sources at low and middle latitudes. J. Geophys. Res. 2007, 112, B10102. [Google Scholar] [CrossRef]
- Zhao, X.; He, Y.; Li, Q.; Liu, X. Analysis of the geomagnetic component Z daily variation amplitude based on the Geomagnetic Network of China during solar quiet days. Chin. J. Geophys. 2022, 65, 3728–3742. [Google Scholar]
- Kuvshinov, A.V.; Avdeev, D.B.; Pankratov, O.V. Global induction by Sq and Dst sources in the presence of oceans: Bimodal solutions for non-uniform spherical surface shells above radially symmetric earth models in comparison to observations. Geophys. J. Int. 1999, 137, 630–650. [Google Scholar] [CrossRef]
- Kuvshinov, A.V.; Utada, H. Anomaly of the geomagnetic Sq variation in Japan: Effect from 3-D subterranean structure or the ocean effect? Geophys. J. Int. 2010, 183, 1239–1247. [Google Scholar] [CrossRef]
- Olsen, N. The solar cycle variability of lunar and solar daily geomagnetic variations. Ann. Geophys. 1993, 11, 254–262. [Google Scholar]
- Maeda, H. Horizontal wind systems in the ionospheric E region deduced from the dynamo theory of the geomagnetic Sq variation, I: Non-rotating earth. J. Geomagn. Geoelectr. 1955, 7, 121–132. [Google Scholar] [CrossRef]
- Maeda, H. Horizontal wind systems in the ionospheric E region deduced from the dynamo theory of the geomagnetic Sq variation, III. J. Geomagn. Geoelectr. 1957, 9, 86–93. [Google Scholar] [CrossRef][Green Version]
- Kato, S. Horizontal wind systems in the ionospheric E region deduced from the dynamo theory of the geomagnetic Sq variation, II, Rotating earth. J. Geomagn. Geoelectr. 1956, 8, 24–37. [Google Scholar] [CrossRef]
- Kato, S. Horizontal wind systems in the ionospheric E region deduced from the dynamo theory of the geomagnetic Sq variation, IV. J. Geomagn. Geoelectr. 1957, 9, 107–115. [Google Scholar] [CrossRef]
- Yamazaki, Y.; Richmond, A.D. A theory of ionospheric response to upward-propagating tides: Electrodynamic effects and tidal mixing effects. J. Geophys. Res. Space Phys. 2013, 118, 5891–5905. [Google Scholar] [CrossRef]
- Vincent, R.A.; Tsuda, T.; Kato, S. A comparative study of mesospheric solar tides observed at Adelaide and Kyoto. J. Geophys. Res. 1988, 93, 699–708. [Google Scholar] [CrossRef]
- Hays, P.B.; Wu, D.L.; The HRDI Science Team. Observations of the diurnal tide from space. J. Atmos. Sci. 1994, 51, 3077–3093. [Google Scholar] [CrossRef]
- Burrage, M.D.; Hagan, M.E.; Skinner, W.R.; Wu, D.L.; Hays, P.B. Long-term variability in the solar diurnal tide observed by HRDI and simulated by the GSWM. Geophys. Res. Lett. 1995, 22, 2641–2644. [Google Scholar] [CrossRef]
- McLandress, C.; Shepherd, G.G.; Solheim, B.H. Satellite observations of thermospheric tides: Results from the Wind Imaging Interferometer on UARS. J. Geophys. Res. 1996, 101, 4093–4114. [Google Scholar] [CrossRef]
- Amayenc, P. Tidal oscillations of the meridional neutral wind at midlatitudes. Radio Sci. 1974, 9, 281–293. [Google Scholar] [CrossRef]
- Matsushita, S.; Xu, W. Equivalent ionospheric current systems representing solar daily variations of the polar geomagnetic field. J. Geophys. Res. 1982, 87, 8241–8254. [Google Scholar] [CrossRef]

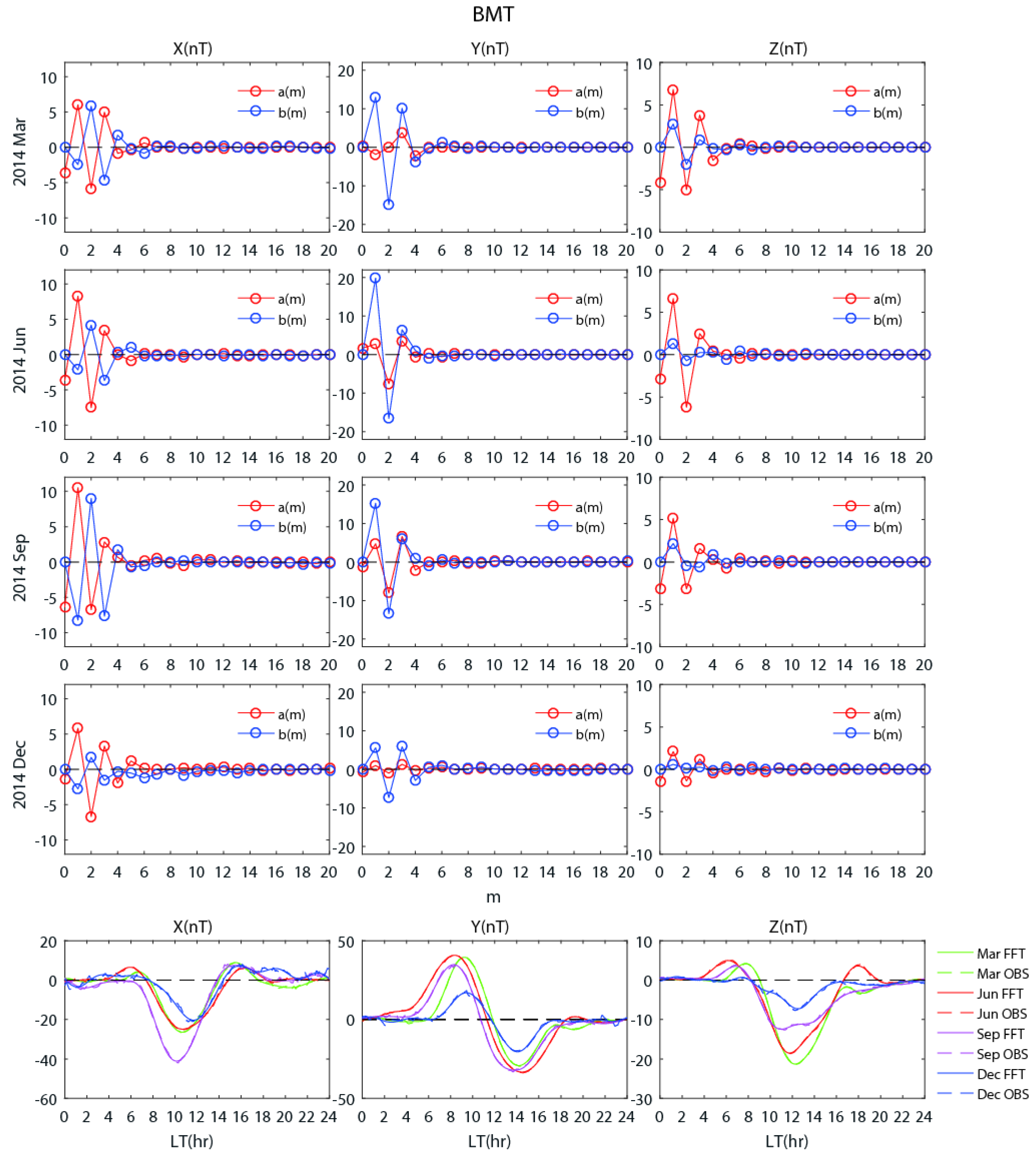
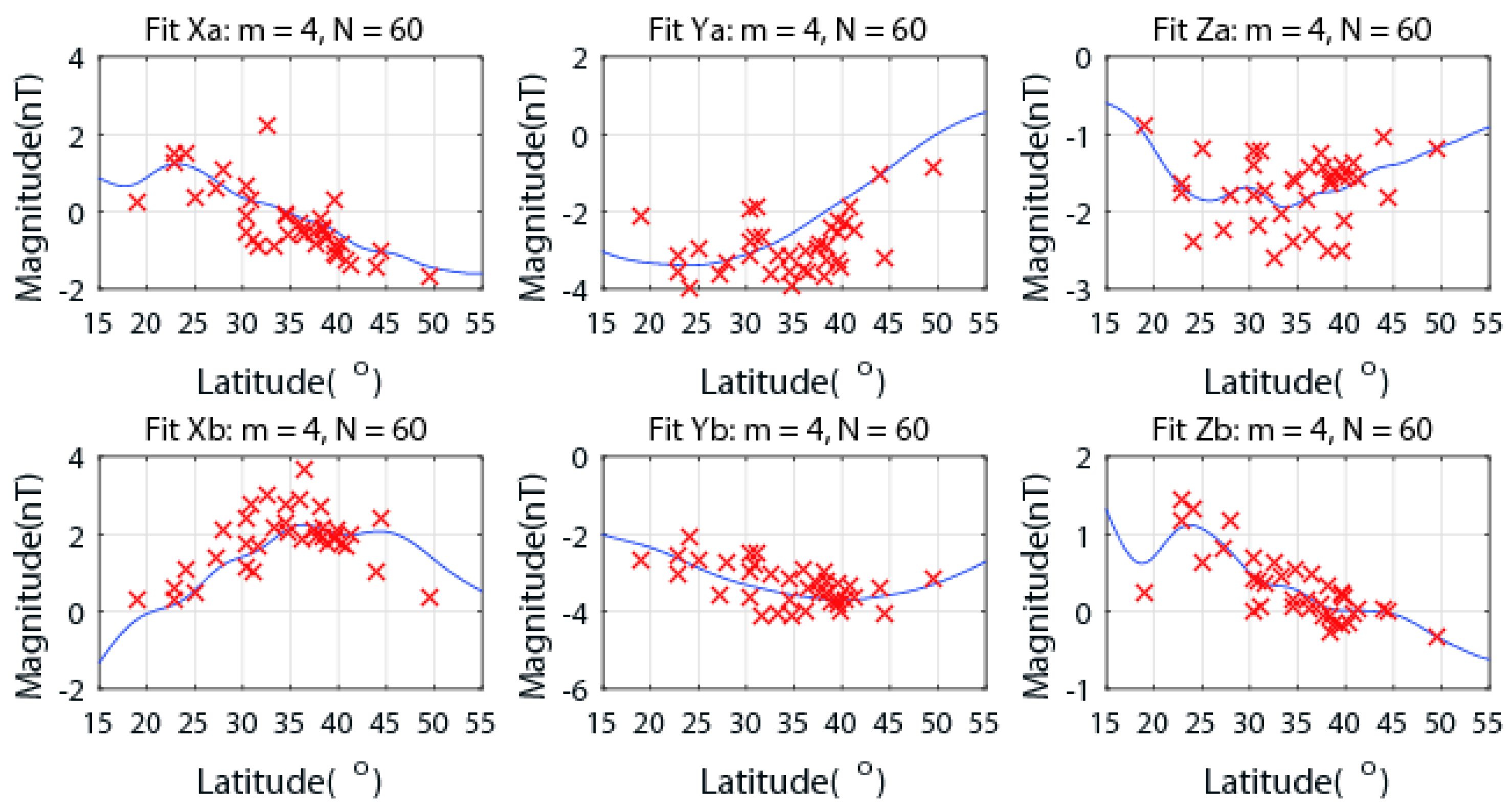
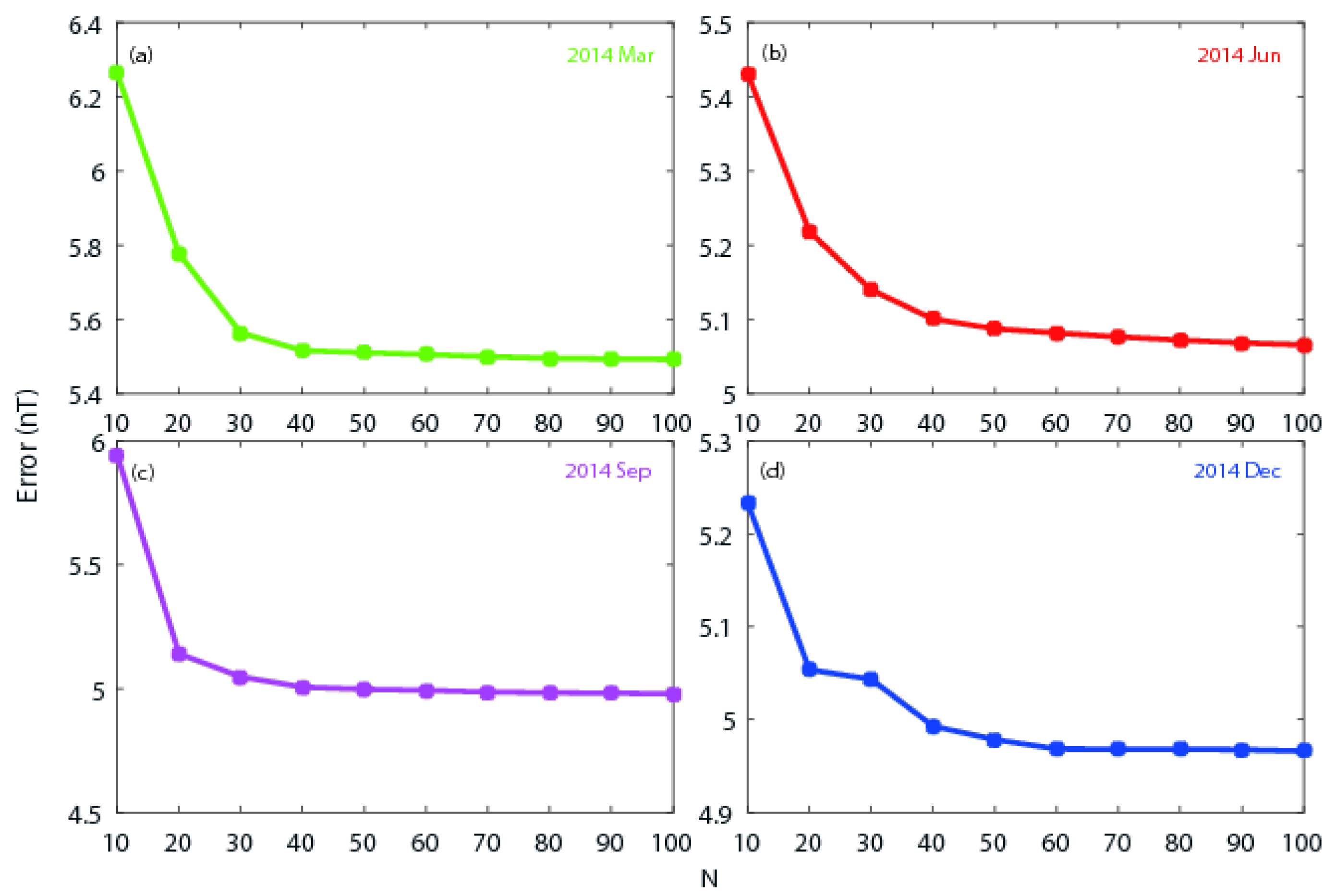





| 2014 | Mar. | Jun. | Sep. | Dec. | ||||
|---|---|---|---|---|---|---|---|---|
| me | rms | me | rms | me | rms | me | rms | |
| X | 3.24 | 3.29 | 2.49 | 2.57 | 3.34 | 3.37 | 2.93 | 2.99 |
| Y | 3.17 | 3.25 | 3.42 | 3.51 | 1.91 | 2.07 | 2.30 | 2.34 |
| Z | 0.66 | 0.73 | 1.00 | 1.31 | 0.83 | 0.96 | 0.61 | 0.70 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, X.; He, Y.; Wu, Y.; Li, Q. Equivalent Current Systems of Quiet Ionosphere during the 24th Solar Cycle Derived from the Geomagnetic Records in China. Atmosphere 2022, 13, 1843. https://doi.org/10.3390/atmos13111843
Zhao X, He Y, Wu Y, Li Q. Equivalent Current Systems of Quiet Ionosphere during the 24th Solar Cycle Derived from the Geomagnetic Records in China. Atmosphere. 2022; 13(11):1843. https://doi.org/10.3390/atmos13111843
Chicago/Turabian StyleZhao, Xudong, Yufei He, Yingyan Wu, and Qi Li. 2022. "Equivalent Current Systems of Quiet Ionosphere during the 24th Solar Cycle Derived from the Geomagnetic Records in China" Atmosphere 13, no. 11: 1843. https://doi.org/10.3390/atmos13111843
APA StyleZhao, X., He, Y., Wu, Y., & Li, Q. (2022). Equivalent Current Systems of Quiet Ionosphere during the 24th Solar Cycle Derived from the Geomagnetic Records in China. Atmosphere, 13(11), 1843. https://doi.org/10.3390/atmos13111843





