CFD-Based Numerical Simulation on the Combined Spraying Dust Suppression Device
Abstract
:1. Introduction
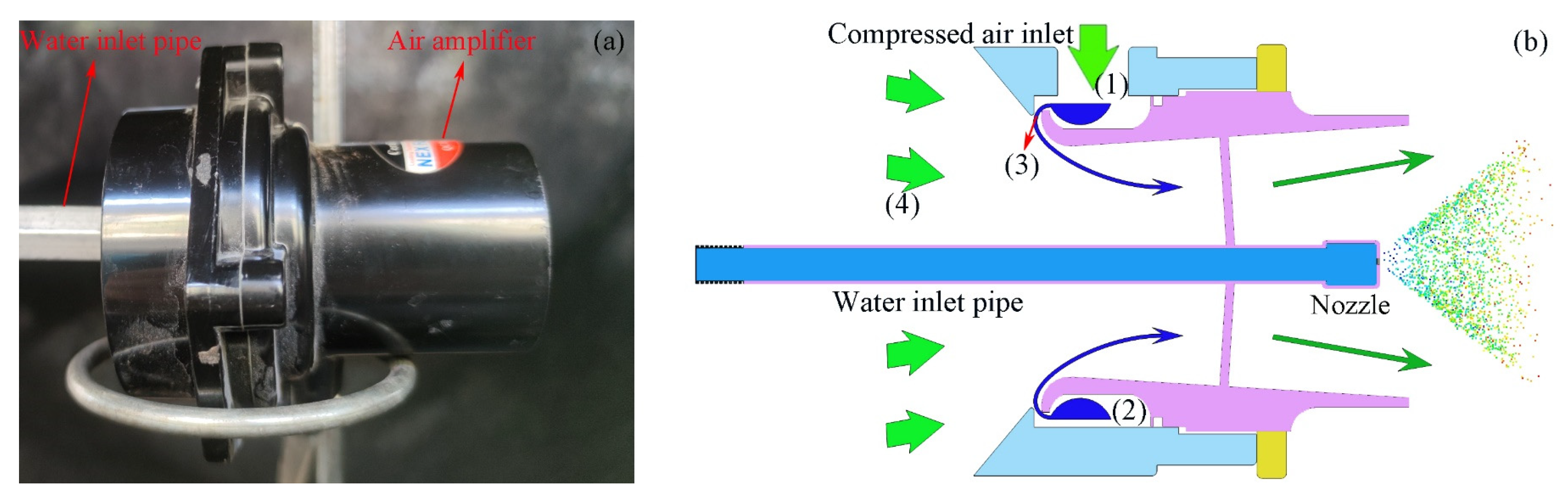
2. Principle of the Combined Spraying Dust Suppression Device
3. Physical Model and Boundary Conditions
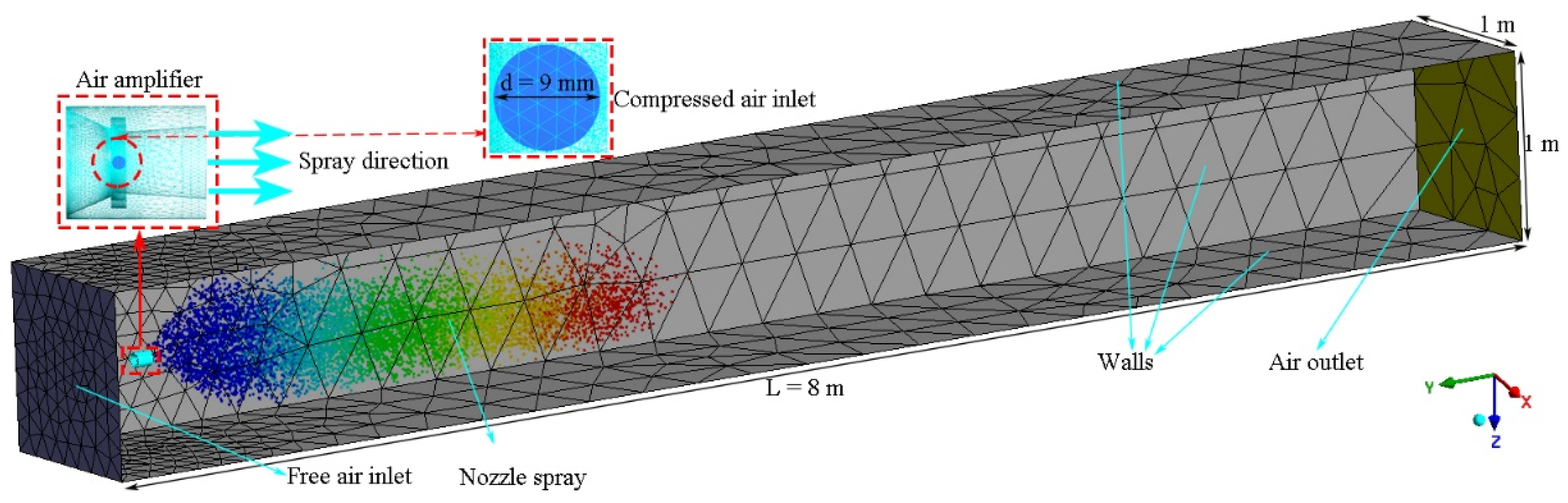
3.1. Physical Model
3.2. Mathematical Model
- (1)
- Airflow motion equation
- (2)
- Droplet motion equation
3.3. Boundary Conditions
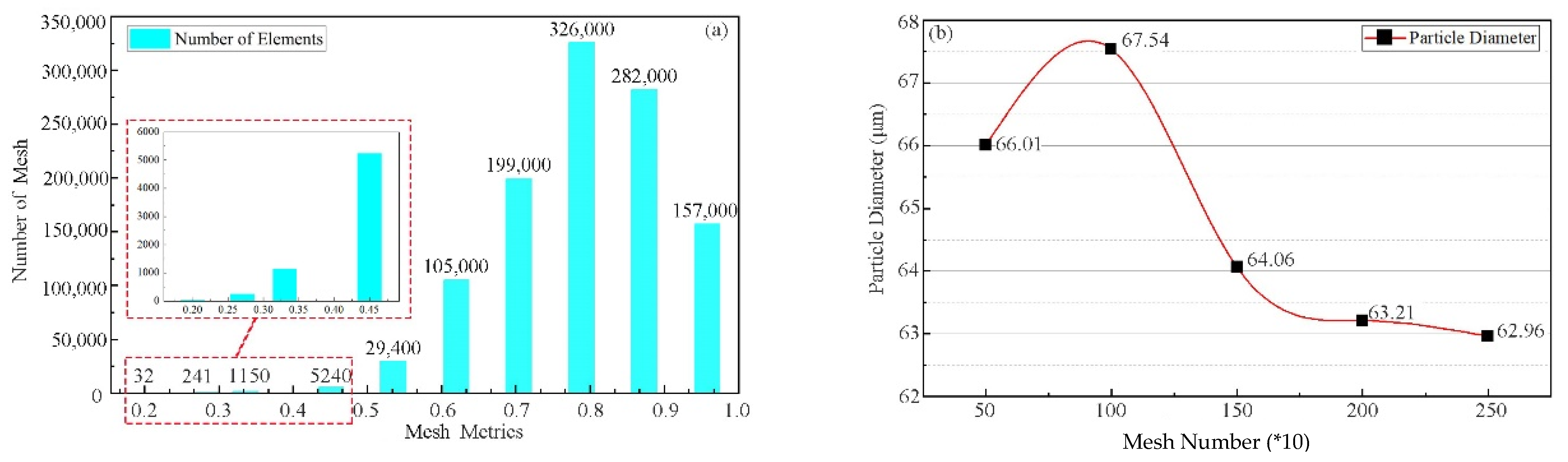
3.4. Mesh Generation and Independence Validation
4. Model Effectiveness Validation
4.1. Experimental System and Scheme
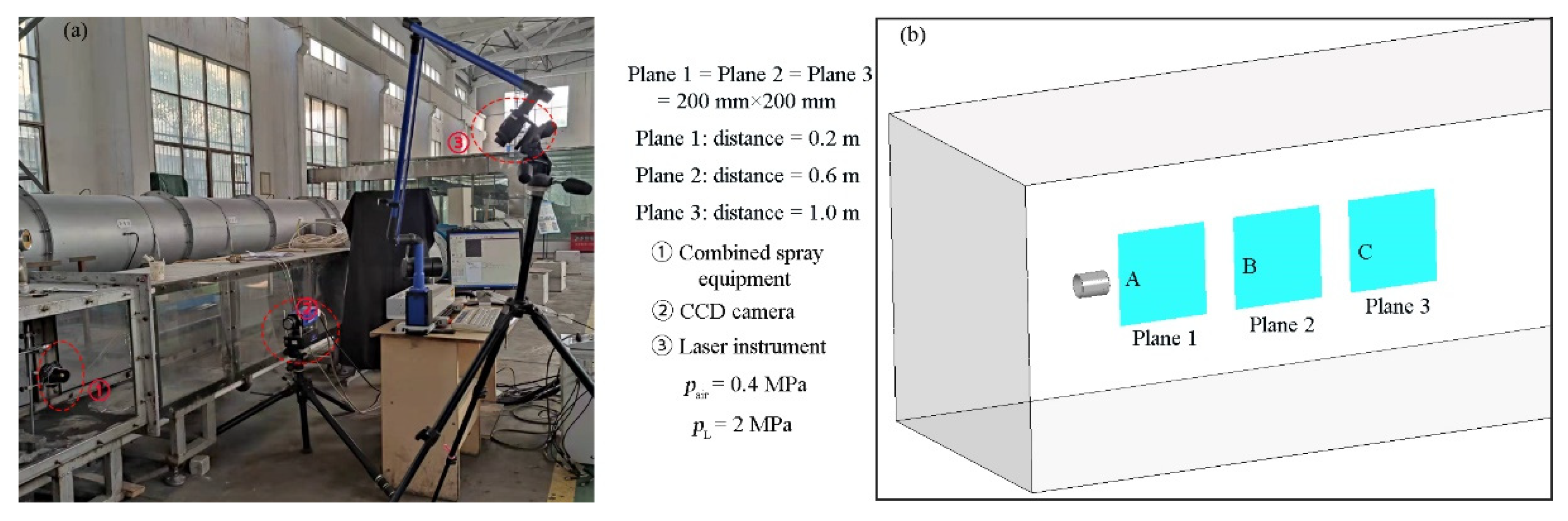
4.1.1. Experimental System
4.1.2. Airflow Field Test Scheme
4.1.3. Measurement Scheme of the Droplet Size
4.2. Experimental Results and Analysis
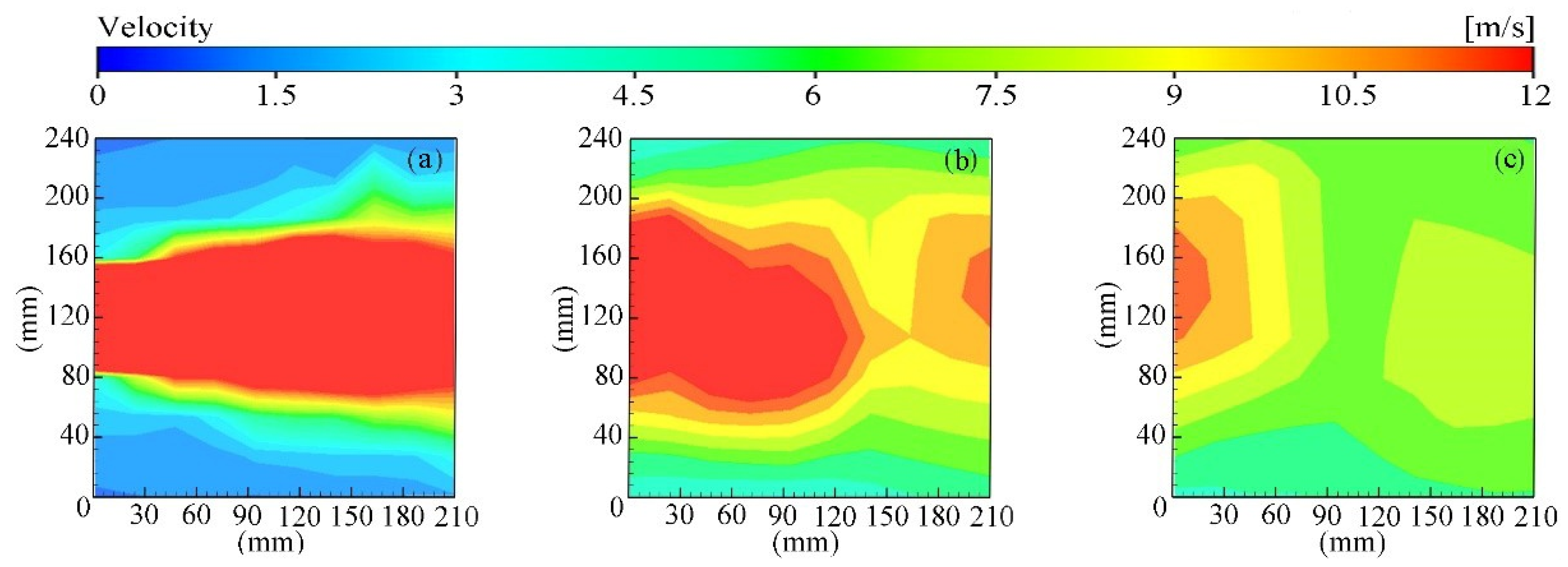
4.2.1. Airflow Field
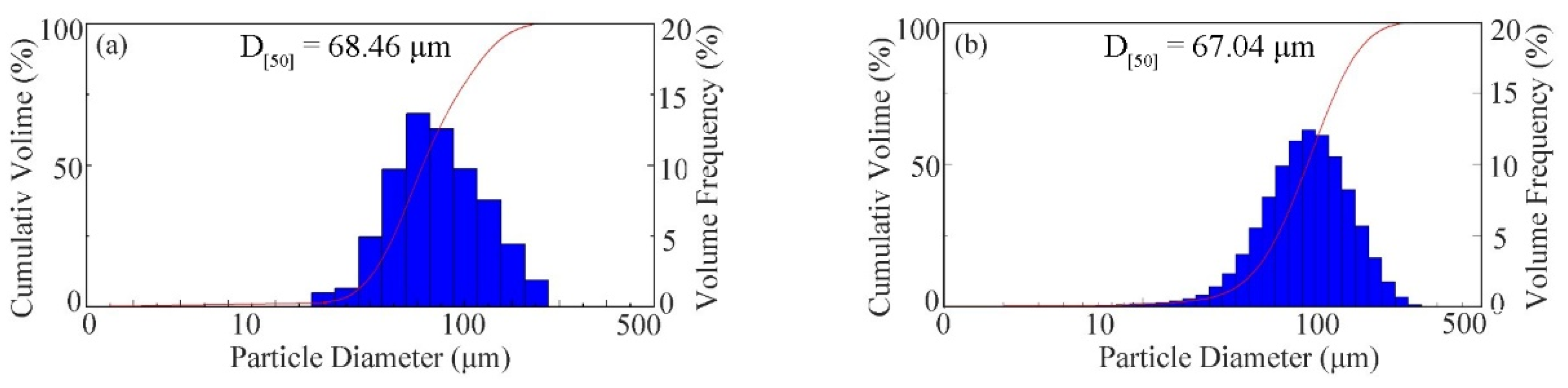
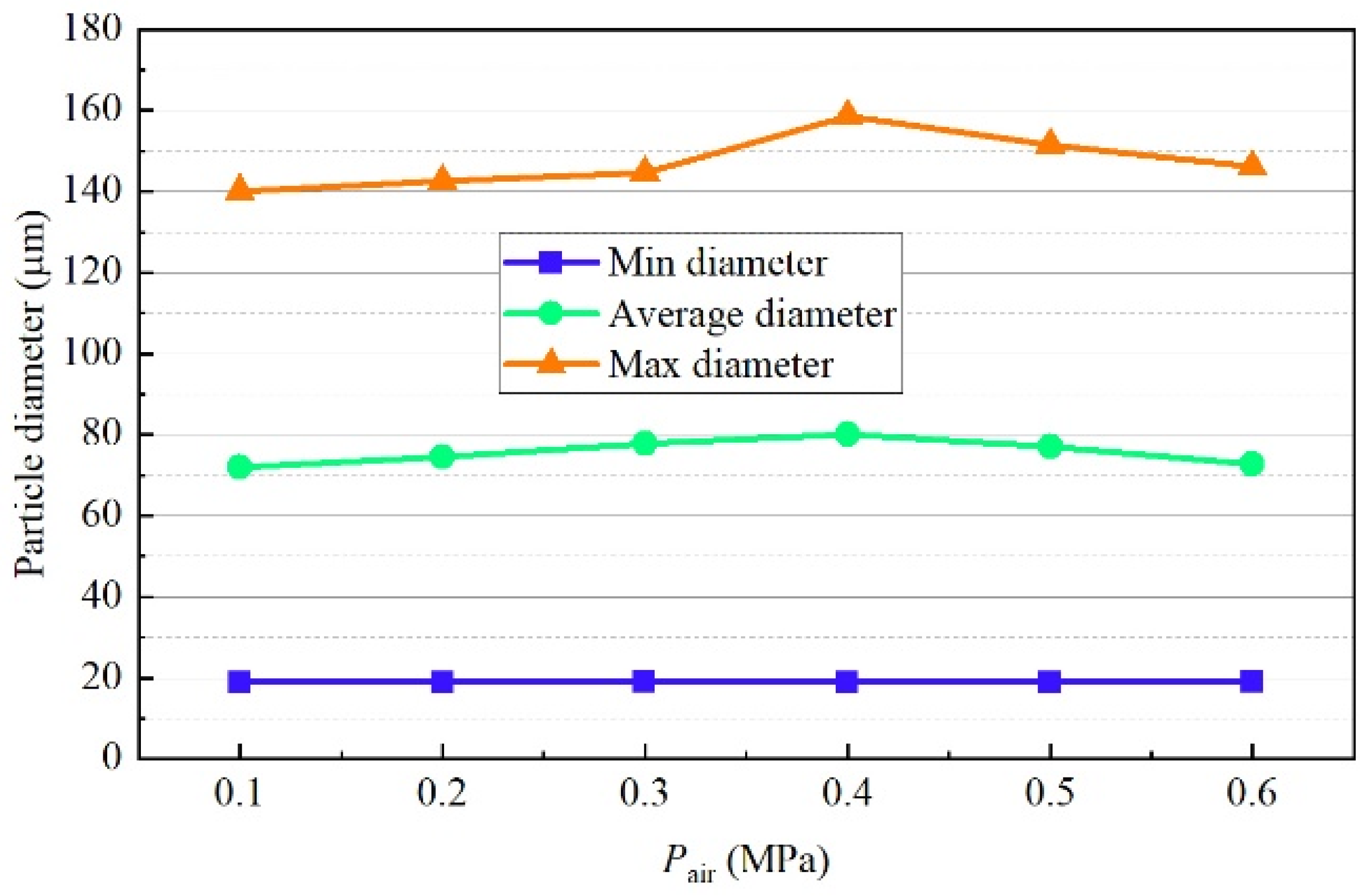
4.2.2. Droplet Size
5. Numerical Simulation Results and Analysis
5.1. Basic Characteristics
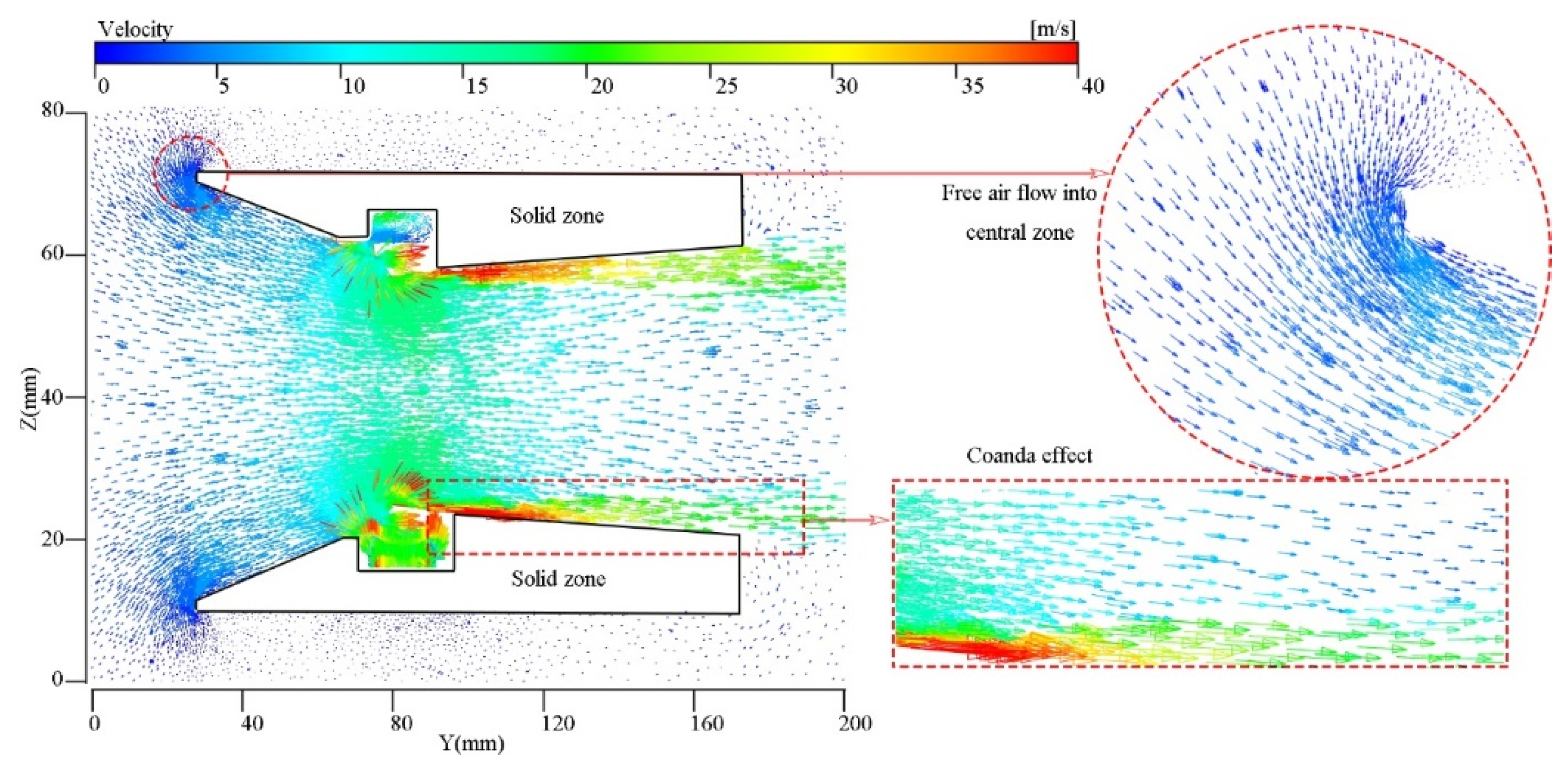
5.1.1. Airflow Field Distribution
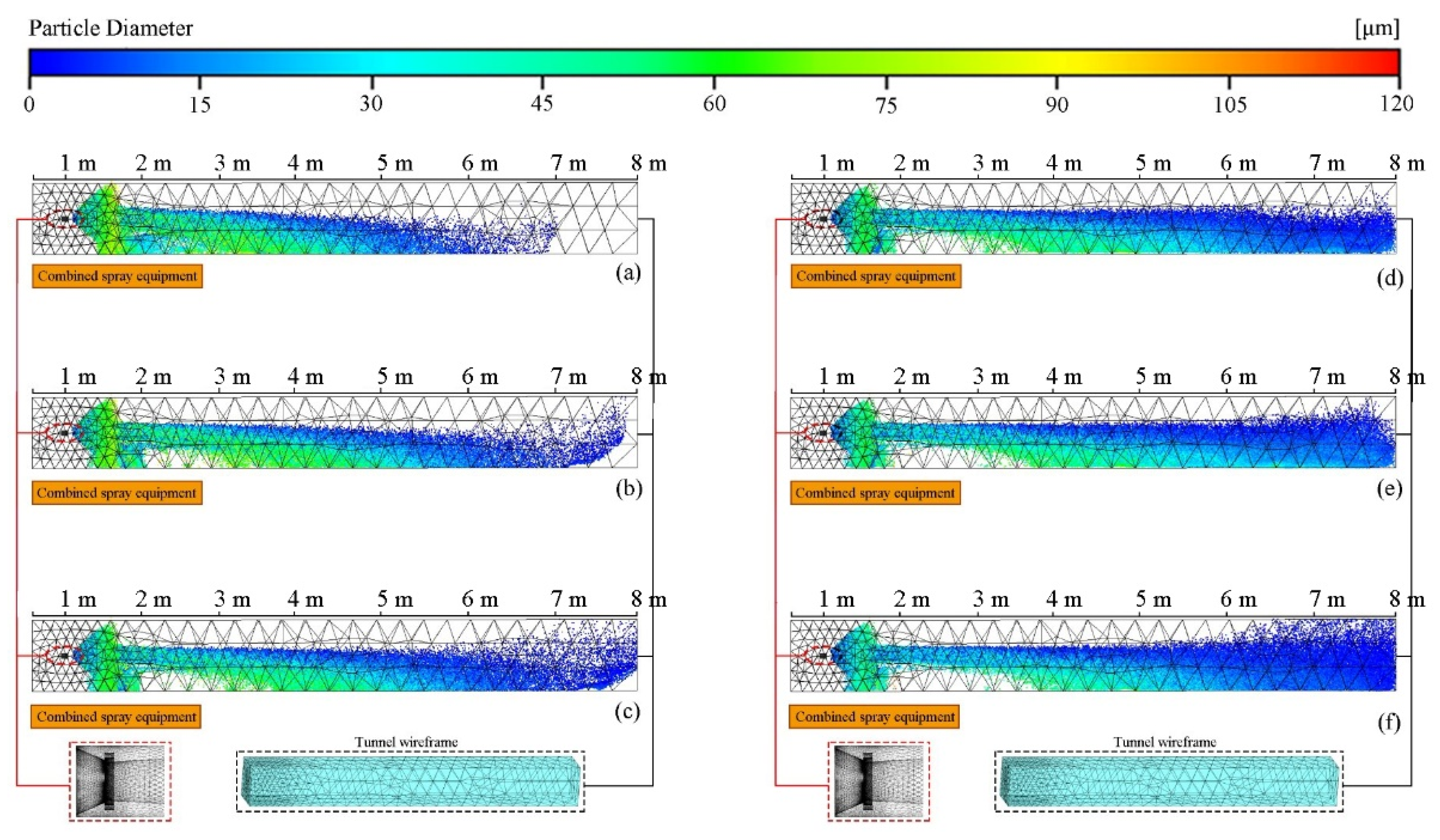
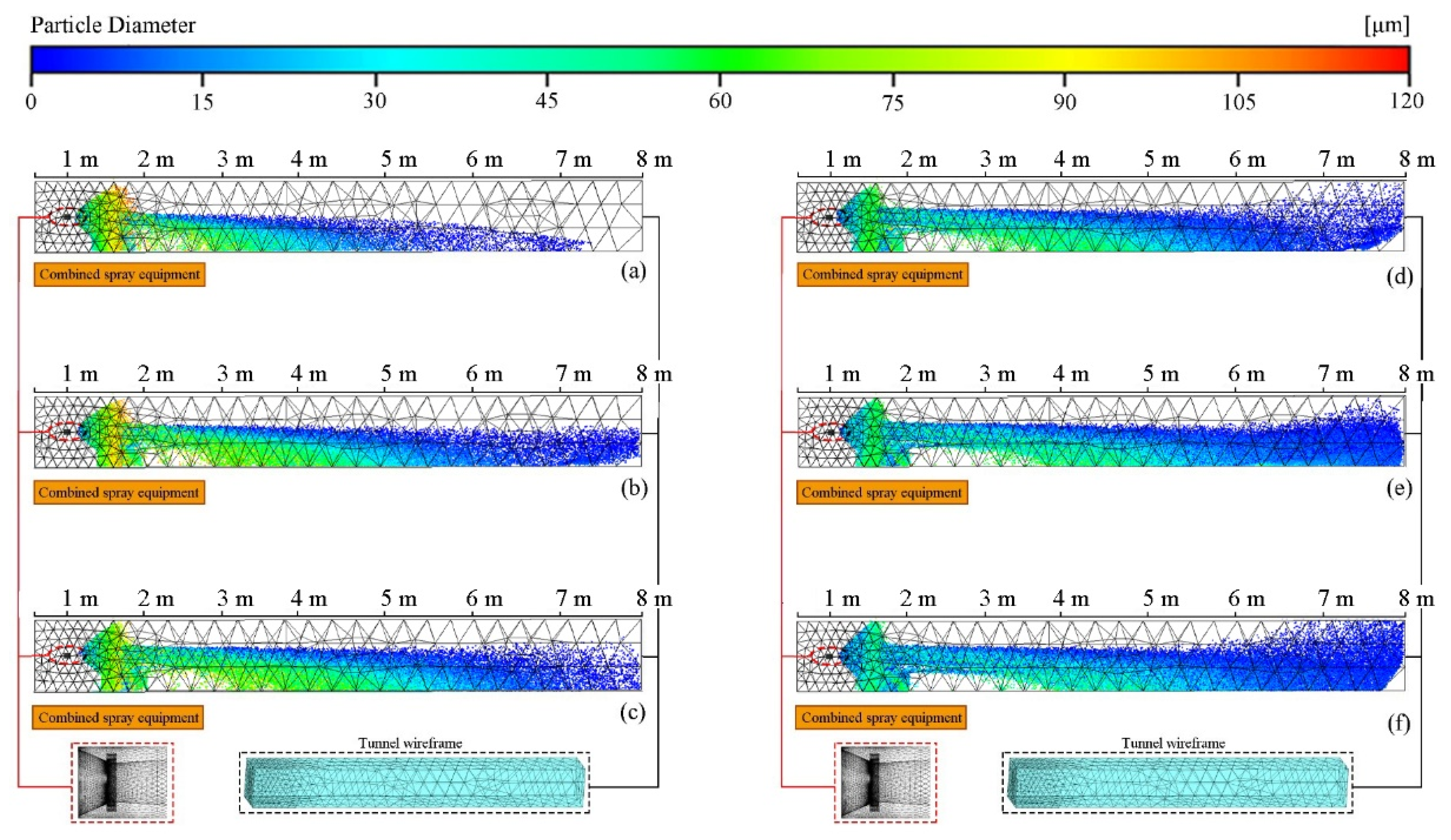
5.1.2. Droplet Size Distribution
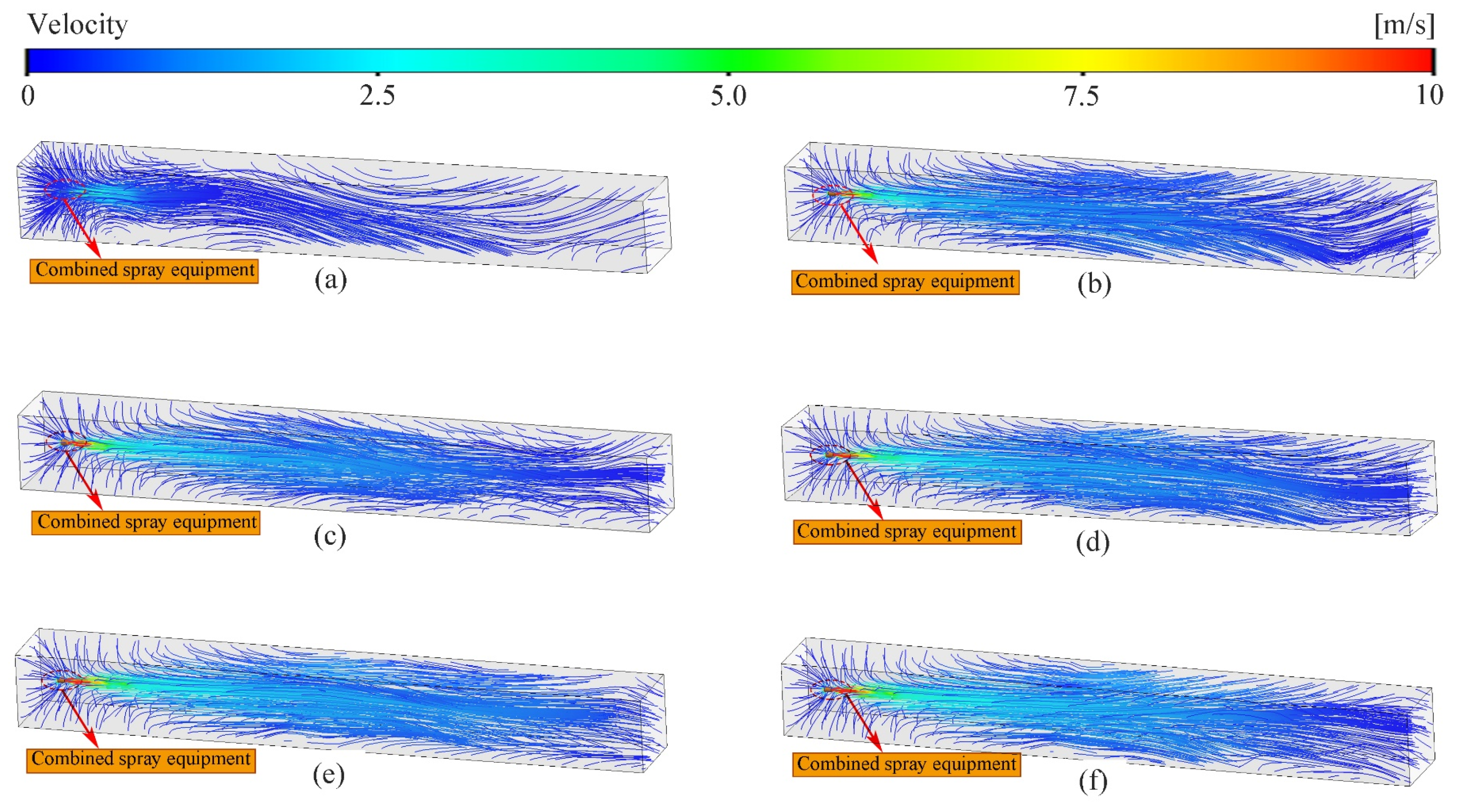
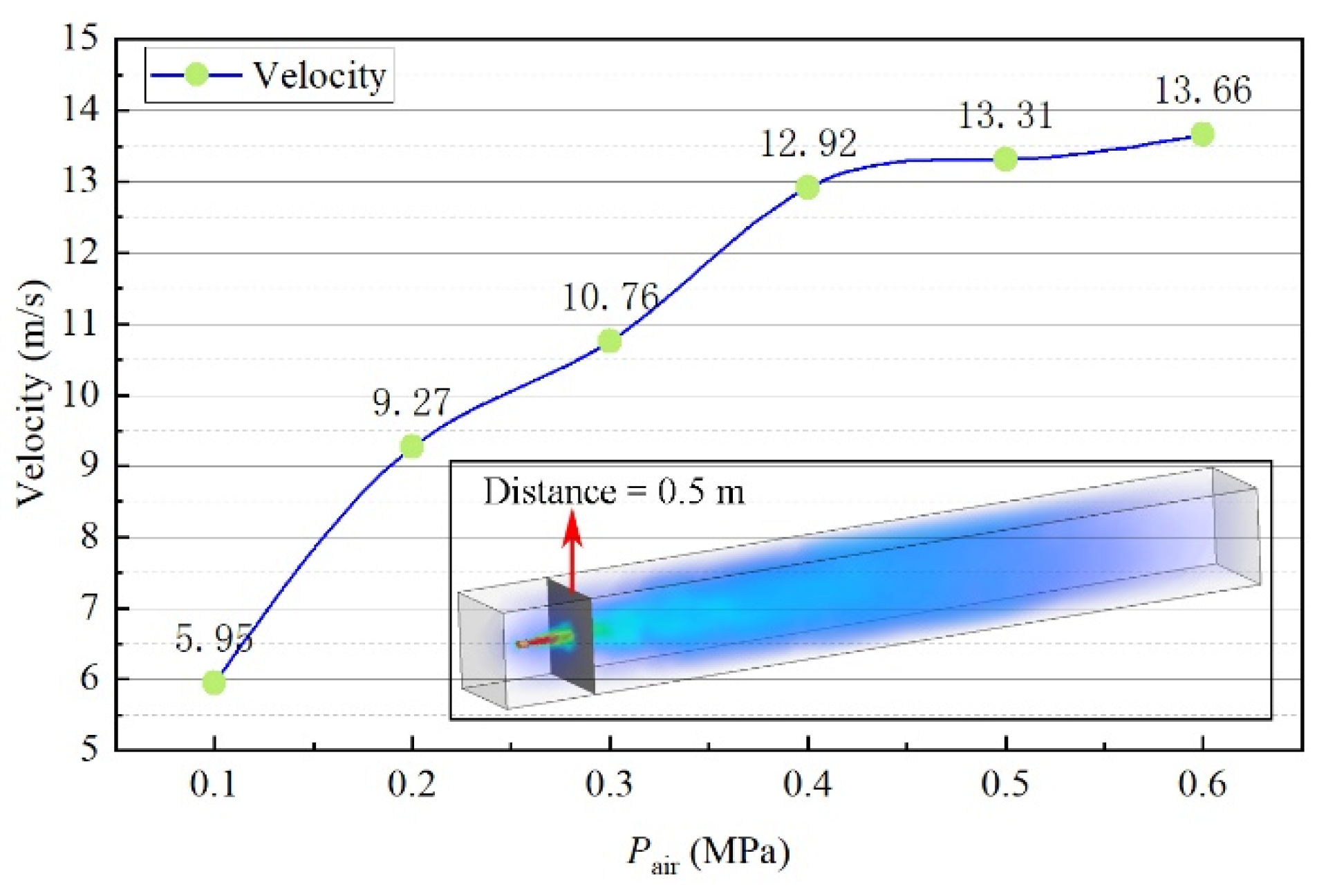
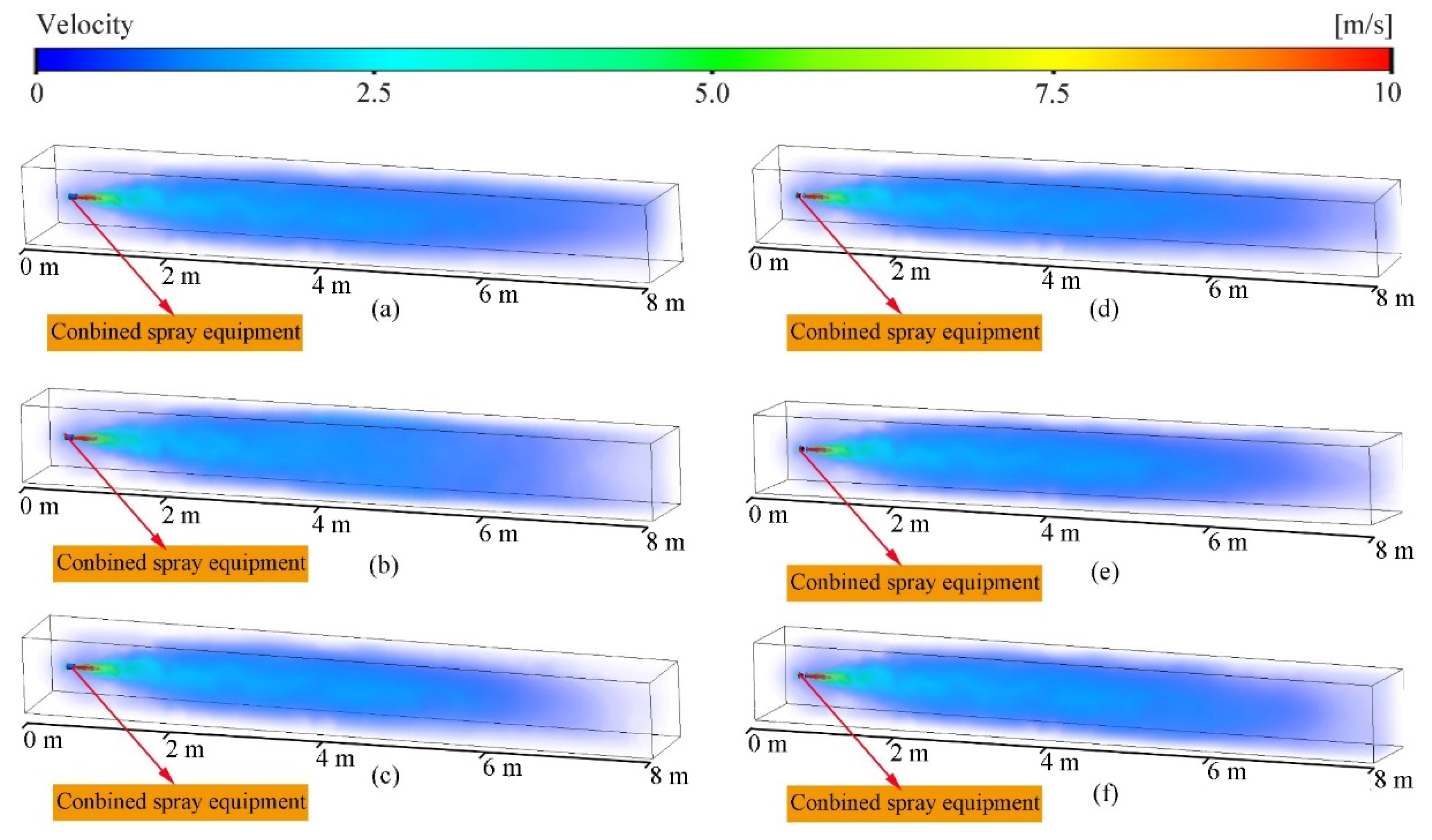
5.2. Effects of the Air Supply Pressure on the Airflow Field and Spray Field
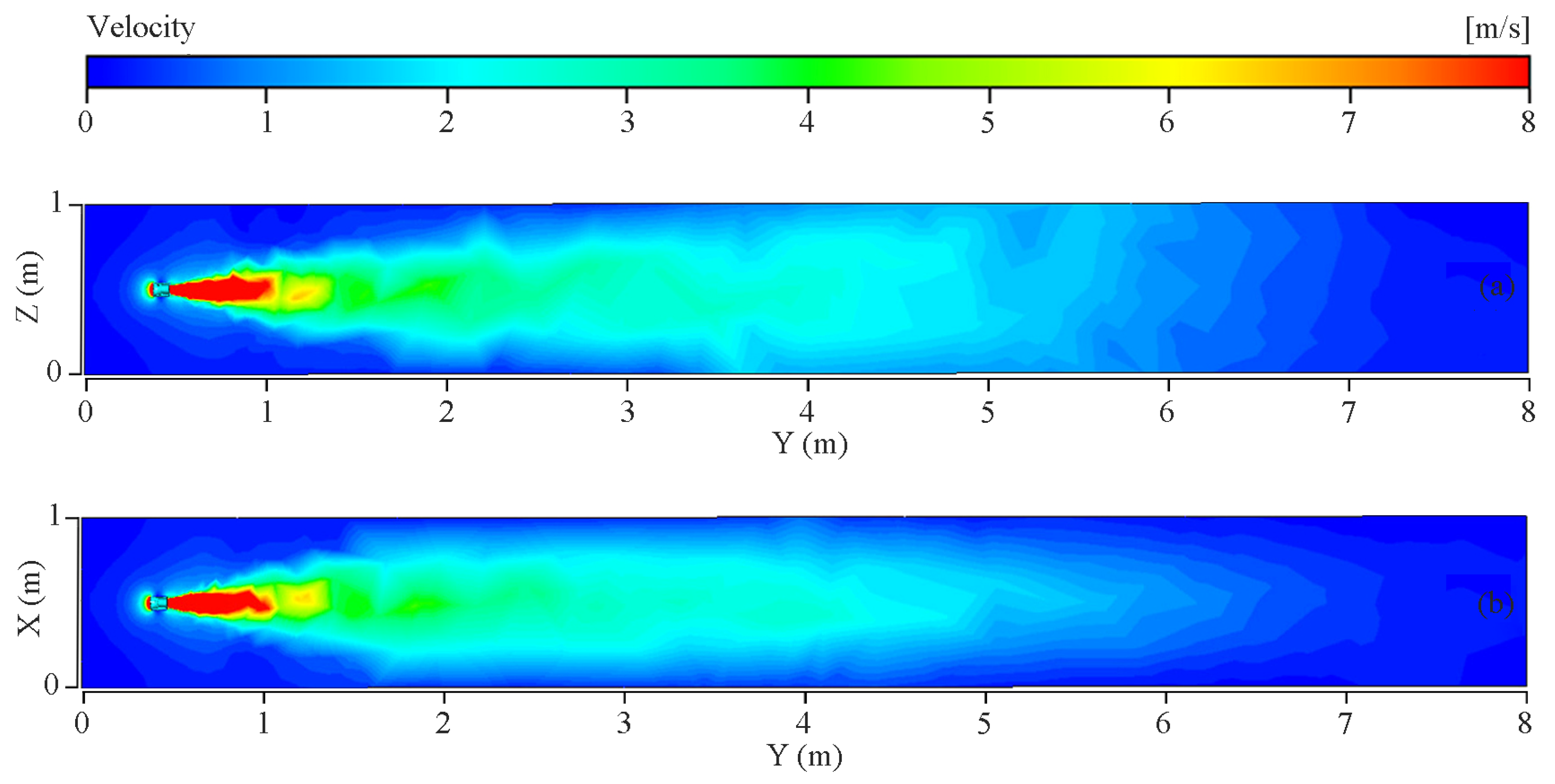
5.2.1. Airflow Field
5.2.2. Pressure Field
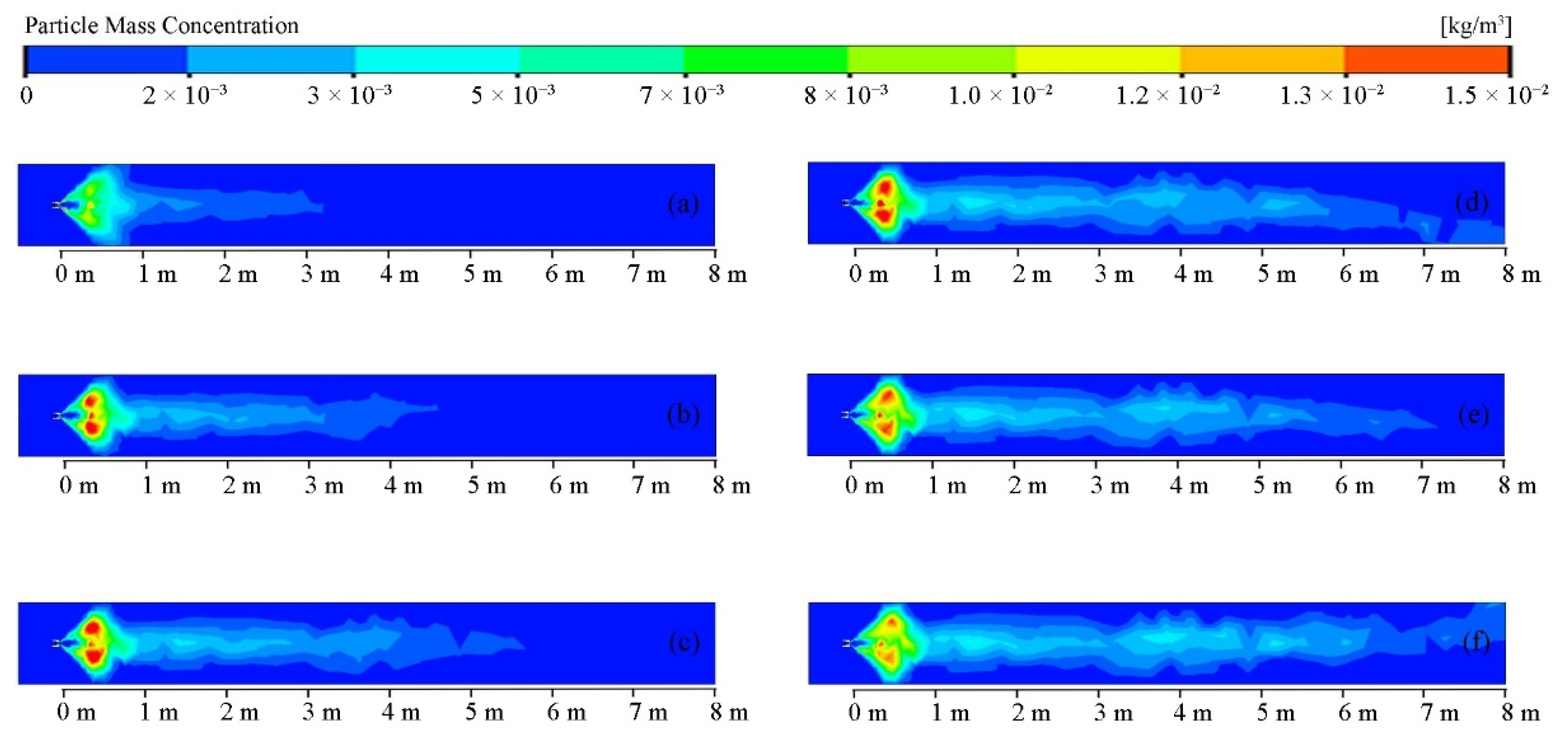
5.2.3. Spray Field
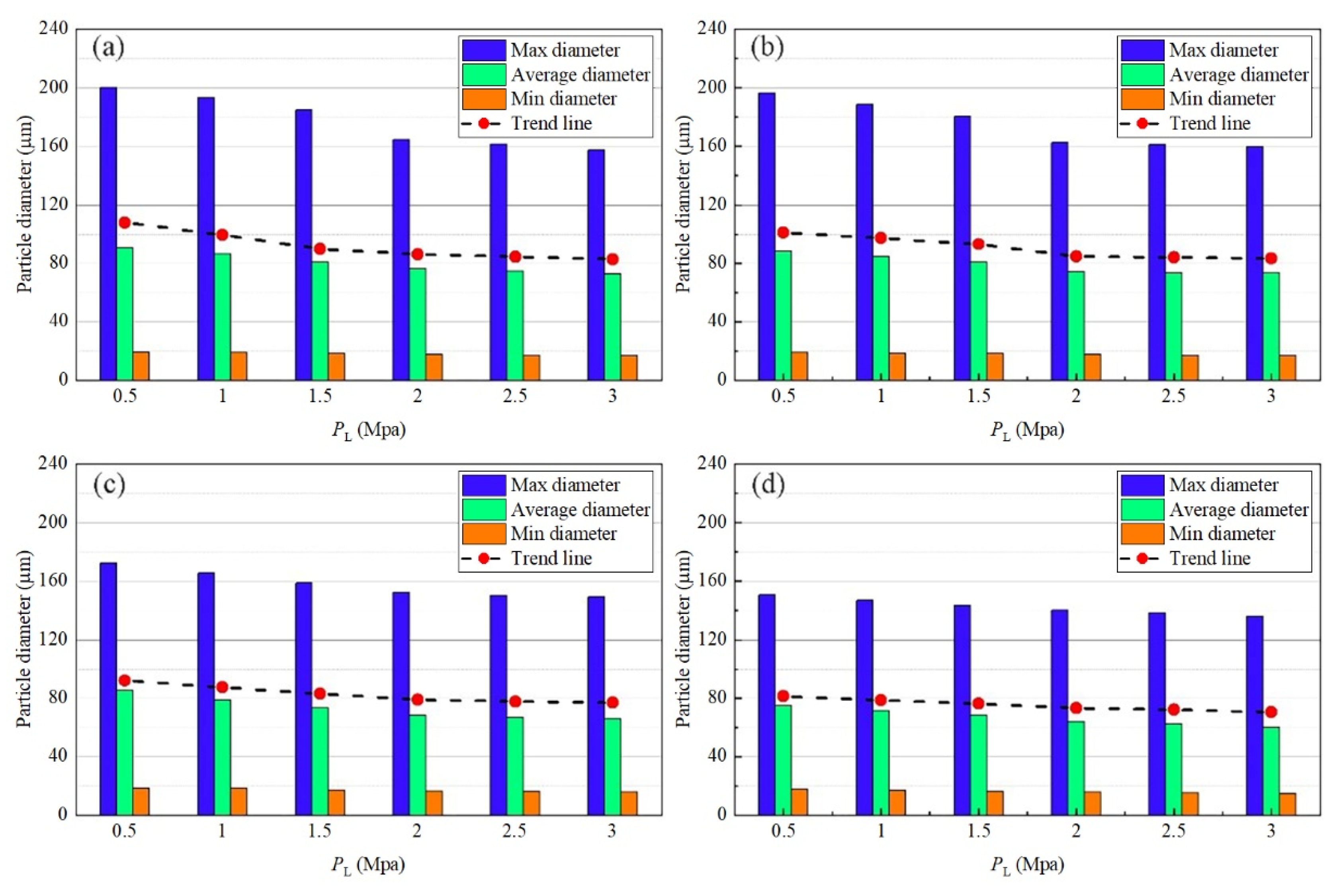
5.2.4. Spraying Range
5.3. Effects of the Water Supply Pressure on the Airflow Field and Spray Field
5.3.1. Airflow Field
5.3.2. Spray Field
5.3.3. Spraying Range
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zhang, C.; Yuan, S.; Zhang, N.N.; Li, C.; Li, H.; Yang, W.; En, Y. Dust-suppression and cooling effects of spray system installed between hydraulic supports in fully mechanized coal-mining face. Build. Environ. 2021, 204, 108106. [Google Scholar] [CrossRef]
- Wang, Y.P.; Jiang, Z.G.; Zhang, F.; Lu, Y.; Bao, Y.W. Study on dust diffusion characteristics of continuous dust sources and spray dust control technology in fully mechanized working face. Powder Technol. 2022, 396, 718–730. [Google Scholar] [CrossRef]
- Zillgitt, M.; Schmidt, E. Quantification of particle separation using electrostatically charged spray mist in a dust chamber. Particuology 2021, 58, 92–98. [Google Scholar] [CrossRef]
- Ma, Q.X.; Nie, W.; Yang, S.B.; Xu, C.W.; Peng, H.T.; Liu, Z.Q.; Guo, C.; Cai, X.J. Effect of spraying on coal dust diffusion in a coal mine based on a numerical simulation. Environ. Pollut. 2020, 264, 114717. [Google Scholar] [CrossRef] [PubMed]
- Nie, W.; Ma, Q.X.; Cai, X.J.; Peng, H.T.; Xu, C.W.; Guo, C.; Zhang, S.B.; Cheng, L. A multi-indicator orthogonal investigation into the dust suppression effect of a shearer-mounted negative-pressure spraying device. Powder Technol. 2022, 399, 117135. [Google Scholar] [CrossRef]
- Bałaga, D.; Siegmund, M.; Kalita, M.; Williamson, B.J.; Walentek, A.; Małachowski, M. Selection of operational parameters for a smart spraying system to control airborne PM10 and PM2.5 dusts in underground coal mines. Process Saf. Environ. Prot. 2021, 148, 482–494. [Google Scholar] [CrossRef]
- Zhang, S.B.; Nie, W.; Guo, C.; Peng, H.T.; Ma, Q.X.; Xu, C.W.; Zhang, H.; Liu, Q.Y. Optimization of spray dust suppression device in return air tunnel of a coal mine based on CFD technology. Build. Environ. 2021, 203, 108059. [Google Scholar] [CrossRef]
- Wang, Y.P.; Jiang, Z.G. Research on mine cleaner production based on high wettability spray control dust pollution. Case Stud. Therm. Eng. 2021, 25, 100896. [Google Scholar] [CrossRef]
- Xu, C.W.; Nie, W.; Liu, Z.Q.; Peng, H.T.; Yang, S.B.; Liu, Q. Multi-factor numerical simulation study on spray dust suppression device in coal mining process. Energy 2019, 182, 544–558. [Google Scholar] [CrossRef]
- Wang, P.F.; Zhang, K.; Liu, R.H. Influence of air supply pressure on atomization characteristics and dust-suppression efficiency of internal-mixing air-assisted atomizing nozzle. Powder Technol. 2019, 355, 393–407. [Google Scholar] [CrossRef]
- Liu, Z.Q.; Nie, W.; Peng, H.T.; Yang, S.B.; Chen, D.W.; Liu, Q. The effects of the spraying pressure and nozzle orifice diameter on the atomizing rules and dust suppression performances of an external spraying system in a fully-mechanized excavation face. Powder Technol. 2019, 350, 62–80. [Google Scholar] [CrossRef]
- Han, H.; Wang, P.F.; Li, Y.J.; Liu, R.H.; Tian, C. Effect of water supply pressure on atomization characteristics and dust-reduction efficiency of internal mixing air atomizing nozzle. Adv. Powder Technol. 2020, 31, 252–268. [Google Scholar] [CrossRef]
- Wang, P.F.; Shi, Y.J.; Zhang, L.Y.; Li, Y.J. Effect of structural parameters on atomization characteristics and dust reduction performance of internal-mixing air-assisted atomizer nozzle. Process Saf. Environ. Prot. 2019, 128, 316–328. [Google Scholar] [CrossRef]
- Salcedo, R.; Zhu, H.P.; Jeon, H.; Ozkan, E.; Wei, Z.M.; Gil, E. Characterisation of activation pressure, flowrate and spray angle for hollow-cone nozzles controlled by pulse width modulation. Biosyst. Eng. 2022, 218, 139–152. [Google Scholar] [CrossRef]
- Yang, S.B.; Nie, W.; Lv, S.S.; Liu, Z.Q.; Peng, H.T.; Ma, X.; Cai, P.; Xu, C.W. Effects of spraying pressure and installation angle of nozzles on atomization characteristics of external spraying system at a fully-mechanized mining face. Powder Technol. 2019, 343, 754–764. [Google Scholar] [CrossRef]
- Wang, P.F.; Han, H.; Liu, R.H.; Gao, R.Z.; Wu, G.G. Effect of outlet diameter on atomization characteristics and dust reduction performance of X-swirl pressure nozzle. Process Saf. Environ. Prot. 2020, 137, 340–351. [Google Scholar] [CrossRef]
- Xia, J.; Zhang, Q.K.; Huang, Z.; Ju, D.H.; Lu, X.C. Experimental study of injection characteristics under diesel’s sub/trans/supercritical conditions with various nozzle diameters and injection pressures. Energy Convers. Manag. 2020, 215, 112949. [Google Scholar] [CrossRef]
- Jiang, Y.; Liu, J.L.; Li, H.; Hua, L.; Yong, Y.C. Droplet distribution characteristics of impact sprinklers with circular and noncircular nozzles: Effect of nozzle aspect ratios and equivalent diameters. Biosyst. Eng. 2021, 212, 200–214. [Google Scholar] [CrossRef]
- Robichaud, G.; Dixon, R.B.; Potturi, A.S.; Cassidy, D.; Edwards, J.R.; Sohn, A.; Dow, T.A.; Muddiman, D.C. Design, modeling, fabrication, and evaluation of the air amplifier for improved detection of biomolecules by electrospray ionization mass spectrometry. Int. J. Mass Spectrom. 2011, 300, 99–107. [Google Scholar] [CrossRef]
- Eltawil, M.A.; Alamri, A.M.; Azam, M.M. Design a novel air to water pressure amplifier powered by PV system for reverse osmosis desalination. Renew. Sustain. Energy Rev. 2022, 160, 112295. [Google Scholar] [CrossRef]
- Du, F.; Zhu, D.H.; Jing, M.C.; Xue, Y.B.; Song, S.L. The application of air amplifiers in reducing the amount of dry tail tobacco in SH313 type wire dryer. Tob. Technol. 2012, 5, 24–26. [Google Scholar]
- Chen, X.Y.; Zheng, Y.H. Performance study of battery pack cooling system based on air amplifier. J. Qingdao Univ. (Eng. Ed.) 2015, 30, 58–72. [Google Scholar]
- Yao, C.; Cheng, F.Y.; Chen, B.W.; Huang, J.; Yi, F.; Yang, W.S.; Zhou, C.H. Automotive cooling device based on air amplifier. China Sci. Technol. Inf. 2019, 7, 83–85. [Google Scholar]
- Wang, Z.X.; Wang, C.L.; Shan, Z.Y. Discussion of the application of air amplifiers in air purification and humidification systems. Metall. Manag. 2020, 7, 57–58. [Google Scholar]
- Dixon, R.B.; Muddiman, D.C.; Hawkridge, A.M.; Fedorov, A.G. Probing the Mechanisms of an Air Amplifier Using a LTQ-FT-ICR-MS and Fluorescence Spectroscopy. J. Am. Soc. Mass Spectrom. 2007, 18, 1909–1913. [Google Scholar] [CrossRef] [PubMed]
- Ren, W.X.; Shi, J.T.; Zhu, J.T.; Guo, Q. An innovative dust suppression device used in underground tunneling. Tunn. Undergr. Space Technol. 2020, 99, 103337. [Google Scholar] [CrossRef]
- Sun, B.; Cheng, W.M.; Wang, J.Y.; Wang, H.; Ma, Y.Y. Development of Venturi negative-pressure secondary dedust device and application of local spray closure technique. Adv. Powder Technol. 2019, 30, 42–54. [Google Scholar] [CrossRef]
- Shi, N.; Lu, J.X.; Liu, Q. Numerical simulation of the internal flow field of an air amplifier. Mech. Electron. 2015, 4, 8–10. [Google Scholar]
- Huang, P.; Dai, C.X.; Huang, C.; Wang, J.J. Discussion on the application of air amplifier in oil mist treatment of cold heading machine. Metrol. Test. Technol. 2016, 43, 71–73. [Google Scholar]
- Yu, H.M.; Cheng, W.M.; Peng, H.T.; Xie, Y. An investigation of the nozzle’s atomization dust suppression rules in a fully-mechanized excavation face based on the airflow-droplet-dust three-phase coupling model. Adv. Powder Technol. 2018, 29, 941–956. [Google Scholar] [CrossRef]
- Li, Y.J.; Wang, P.F.; Liu, R.H.; Gao, R.Z. Optimization of structural parameters and installation position of the wall-mounted air cylinder in the fully mechanized excavation face based on CFD and orthogonal design. Process Saf. Environ. Prot. 2019, 130, 344–358. [Google Scholar] [CrossRef]







| Model | Define |
|---|---|
| Solver | Segregated |
| Viscous Model | Realizable k-ε Model |
| Discrete Phase Model | On |
| Injection Type | Pressure-Swirl-Atomizer |
| Material | Water-Liquid |
| Injector Inner Diameter | 0.0005 m |
| Spray Half Angle | 37.058° |
| Upstream Pressure | 0.5~3 MPa |
| Flow Rate | 0.00325 kg/s |
| Inlet Boundary Type | Pressure inlet, 0.1~0.6 MPa |
| Outlet Boundary Type | Pressure outlet, 0 Pa |
| Parameter | Experimental Value | Simulation Value | Relative Error |
|---|---|---|---|
| VA | 27.85 (m/s) | 25.69 (m/s) | 7.76% |
| VB | 12.77 (m/s) | 12.96 (m/s) | 1.49% |
| VC | 8.66 (m/s) | 8.02 (m/s) | 7.39% |
| Negative pressure | −293.1 Pa | −312.4 Pa | 6.58% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mo, S.; Wang, P.; Gao, R.; Chen, S.; Li, S. CFD-Based Numerical Simulation on the Combined Spraying Dust Suppression Device. Atmosphere 2022, 13, 1543. https://doi.org/10.3390/atmos13101543
Mo S, Wang P, Gao R, Chen S, Li S. CFD-Based Numerical Simulation on the Combined Spraying Dust Suppression Device. Atmosphere. 2022; 13(10):1543. https://doi.org/10.3390/atmos13101543
Chicago/Turabian StyleMo, Shitu, Pengfei Wang, Runze Gao, Shiqiang Chen, and Shilin Li. 2022. "CFD-Based Numerical Simulation on the Combined Spraying Dust Suppression Device" Atmosphere 13, no. 10: 1543. https://doi.org/10.3390/atmos13101543
APA StyleMo, S., Wang, P., Gao, R., Chen, S., & Li, S. (2022). CFD-Based Numerical Simulation on the Combined Spraying Dust Suppression Device. Atmosphere, 13(10), 1543. https://doi.org/10.3390/atmos13101543






