Radon Spatial Variations in University’s Buildings Located in an Italian Karst Region
Abstract
:1. Introduction
- underground working activities,
- workplaces at ground floor and basement if located in radon priority areas,
- specific types of workplaces identified by the National Radon Action Plan, and
- spa facilities.
2. Materials and Methods
2.1. The Sample
2.2. Measure of Indoor Radon Average Activity Concentration
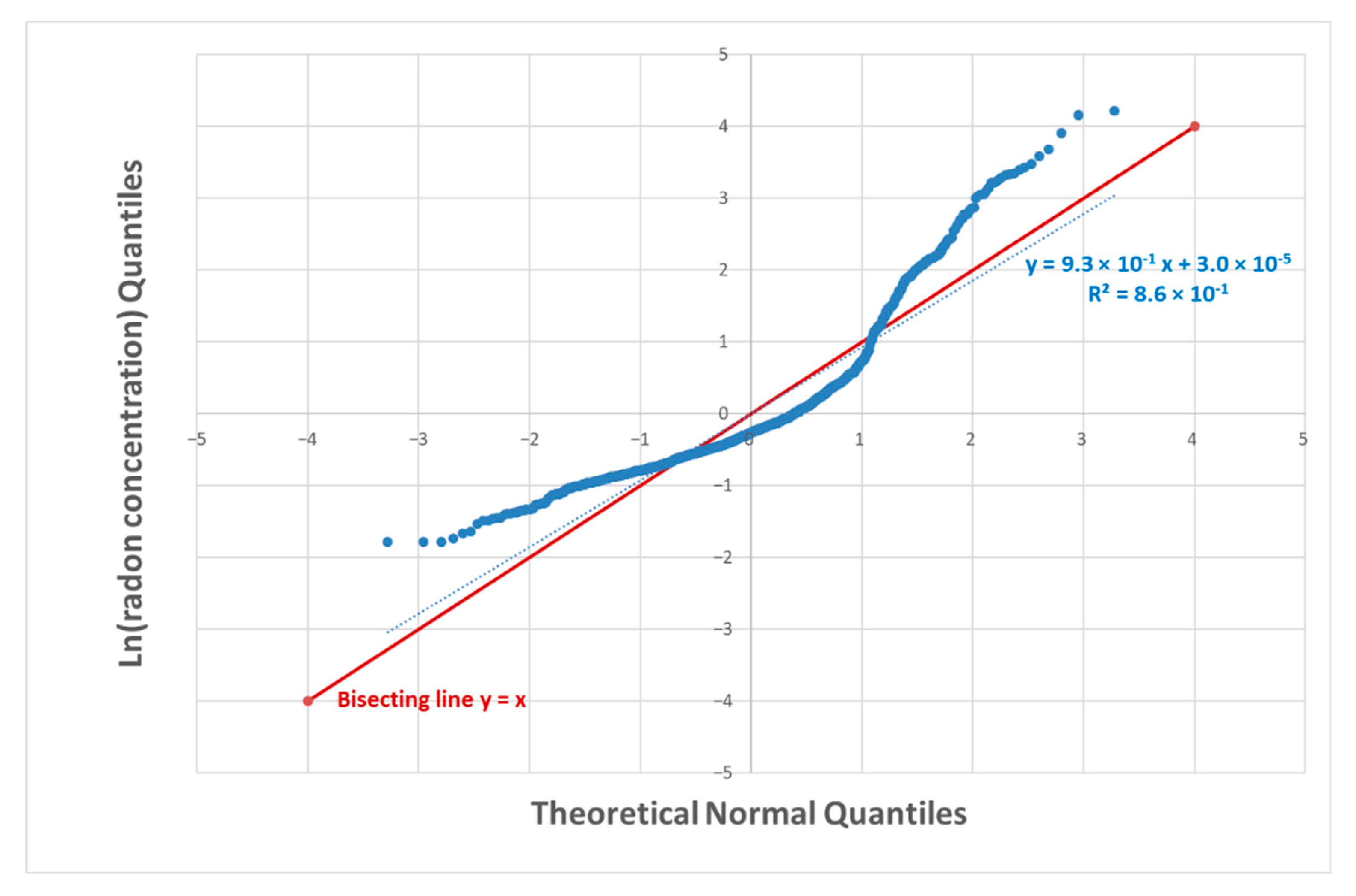
2.3. Statistical Data Analysis
3. Results and Discussion
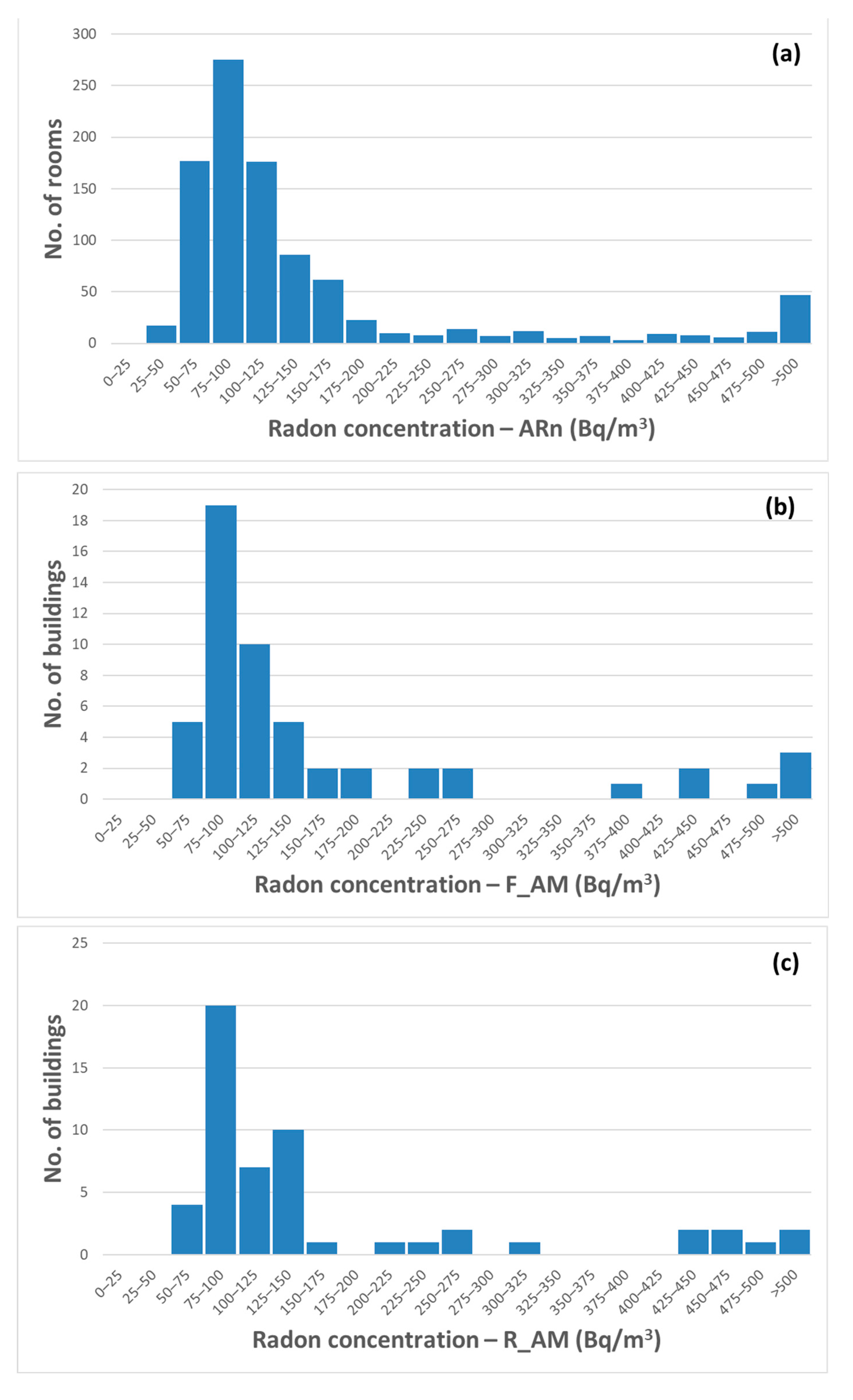
3.1. Distribution of Annual Radon Activity Concentration in Rooms and Buildings
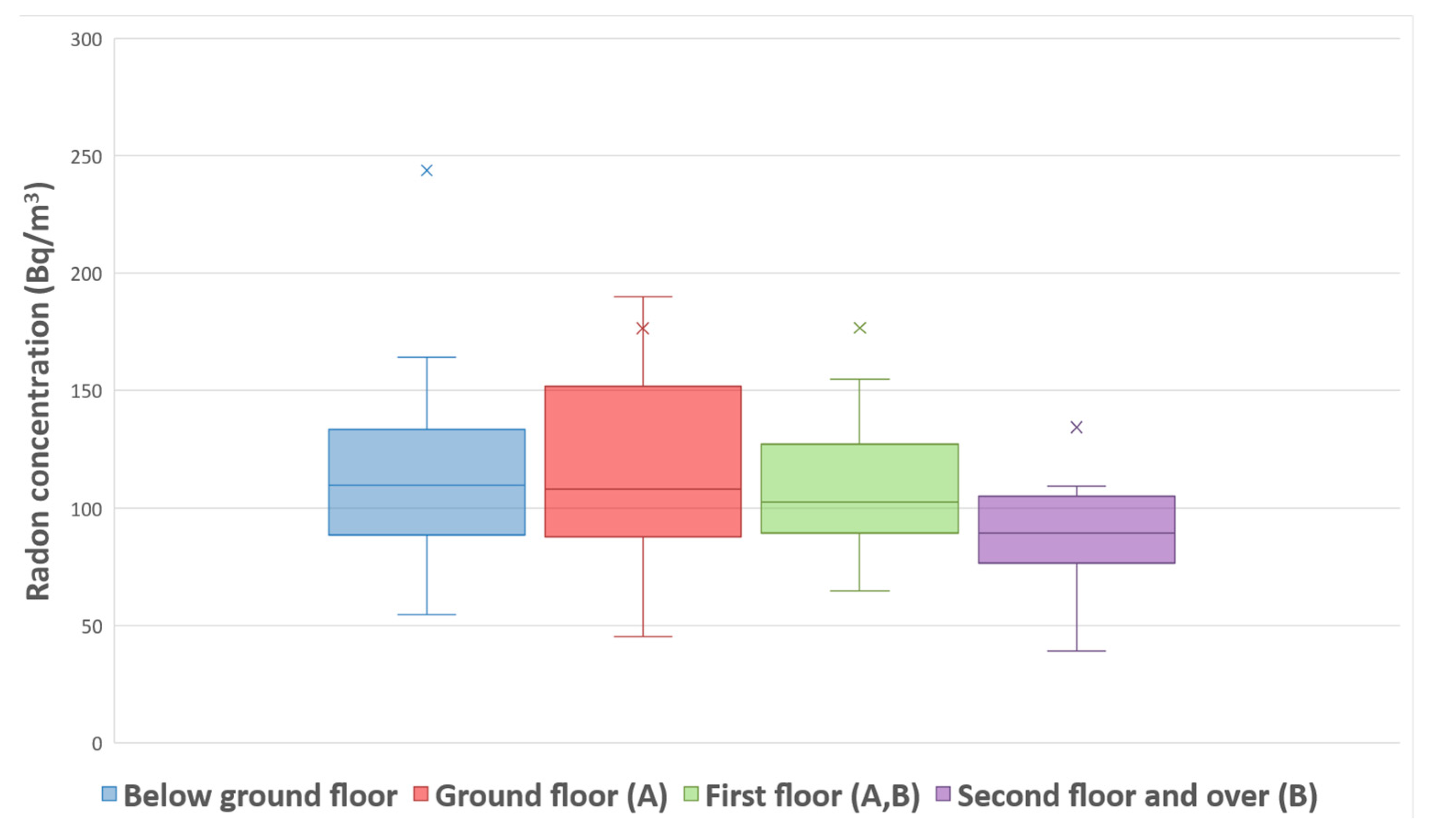
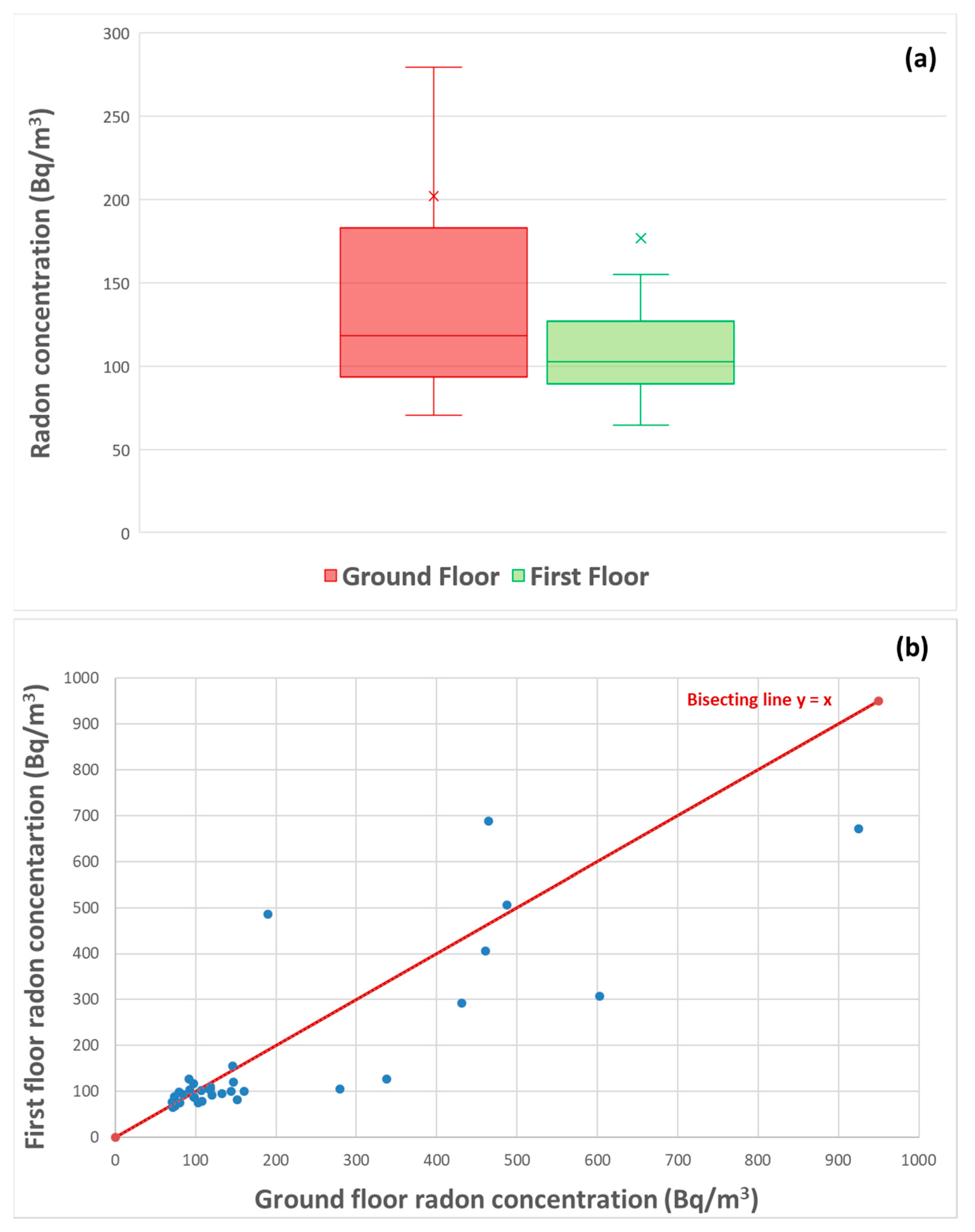
3.2. Annual Radon Activity Concentrations as Function of Floor Level
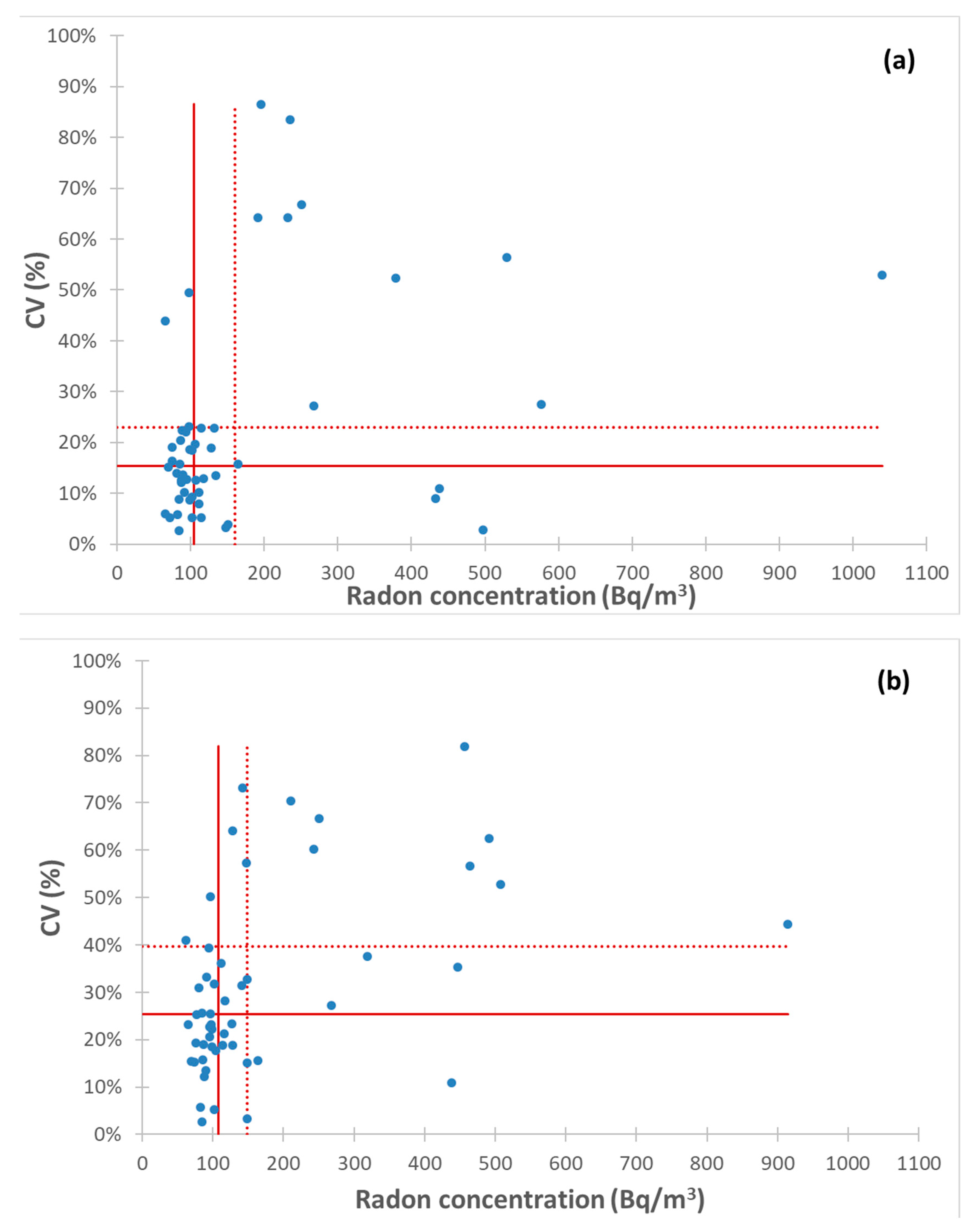
3.3. Distribution of CVs Per Building and Floor Level
- 1st dataset (F_AM): for each building, the CV value is calculated as the ratio between SD and AM computed from radon averages of each floor of the building.
- 2nd dataset (R_AM): for each building, the CV value is calculated by AM and SD computed from all rooms ARns of the building.
- 3rd dataset (BGF): for each building, the value of CV refers to all rooms ARn values at BGF.
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Zeeb, H.; Shannoun, F.; WHO. WHO Handbook on Indoor Radon: A Public Health Perspective; World Health Organization: Geneva, Switzerland, 2009. [Google Scholar]
- UNSCEAR Sources and Effects of Ionizing Radiation. Report to the General Assembly, with Scientific Annexes; UNSCEAR: Vienna, Austria, 2000. [Google Scholar]
- Nazaroff, W.W. Radon transport from soil to air. Rev. Geophys. 1992, 30, 137–160. [Google Scholar] [CrossRef]
- Dai, D.; Neal, F.B.; Diem, J.; Deocampo, D.M.; Stauber, C.; Dignam, T. Confluent impact of housing and geology on indoor radon concentrations in Atlanta, Georgia, United States. Sci. Total Environ. 2019, 668, 500–511. [Google Scholar] [CrossRef] [PubMed]
- European Commission Council Directive 2013/59/Euratom of 5 December 2013. Off. J. Eur. Union 2014, L13-1, 1–73.
- Online resources: European Commission Radiation Protection 193 Radon in workplaces. In Implementing the Requirements in Council Directive 2013/59/Euratom; 2020; Available online: https://op.europa.eu/s/pDS7 (accessed on 11 November 2019).
- Online Resources: Decreto Legislativo 31 Luglio 2020, n. 101. 2020. Available online: https://www.gazzettaufficiale.it/eli/id/2020/08/12/20G00121/sg (accessed on 12 August 2020).
- Borgoni, R.; De Francesco, D.; De Bartolo, D.; Tzavidis, N. Hierarchical modeling of indoor radon concentration: How much do geology and building factors matter? J. Environ. Radioact. 2014, 138, 227–237. [Google Scholar] [CrossRef] [PubMed]
- Atik, S.; Yetis, H.; Denizli, H.; Evrendilek, F. How Do Different Locations, Floors and Aspects Influence Indoor Radon Concentrations? An Empirical Study Using Neural Networks for a University Campus in Northwestern Turkey. Indoor Built Environ. 2013, 22, 650–658. [Google Scholar] [CrossRef]
- Madureira, J.; Paciência, I.; Rufo, J.; Moreira, A.; de Oliveira Fernandes, E.; Pereira, A. Radon in indoor air of primary schools: Determinant factors, their variability and effective dose. Environ. Geochem. Health 2016, 38, 523–533. [Google Scholar] [CrossRef] [PubMed]
- Curguz, Z.; Venoso, G.; Zunic, Z.S.; Mirjanic, D.; Ampollini, M.; Carpentieri, C.; Di Carlo, C.; Caprio, M.; Alavantic, D.; Kolarz, P.; et al. Spatial Variability of Indoor Radon Concentration in Schools: Implications on Radon Measurement Protocols. Radiat. Prot. Dosimetry 2020, 191, 133–137. [Google Scholar] [CrossRef] [PubMed]
- Antignani, S.; Bochicchio, F.; Ampollini, M.; Venoso, G.; Bruni, B.; Innamorati, S.; Malaguti, L.; Stefano, A. Radon concentration variations between and within buildings of a research institute. Radiat. Meas. 2009, 44, 1040–1044. [Google Scholar] [CrossRef]
- Bochicchio, F.; Žunić, Z.S.; Carpentieri, C.; Antignani, S.; Venoso, G.; Carelli, V.; Cordedda, C.; Veselinović, N.; Tollefsen, T.; Bossew, P. Radon in indoor air of primary schools: A systematic survey to evaluate factors affecting radon concentration levels and their variability. Indoor Air 2014, 24, 315–326. [Google Scholar] [CrossRef] [PubMed]
- Ferri, G.M.; Intranuovo, G.; Cavone, D.; Corrado, V.; Birtolo, F.; Tricase, P.; Fuso, R.; Vilardi, V.; Sumerano, M.; L’Abbate, N.; et al. Estimates of the lung cancer cases attributable to radon in municipalities of two Apulia provinces (Italy) and assessment of main exposure determinants. Int. J. Environ. Res. Public Health 2018, 15, 1294. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Trevisi, R.; Caricato, A.; D’Alessandro, M.; Fernández, M.; Leonardi, F.; Luches, A.; Tonnarini, S.; Veschetti, M. A pilot study on natural radioactivity in schools of south-east Italy. Environ. Int. 2010, 36, 276–280. [Google Scholar] [CrossRef] [PubMed]
- Trevisi, R.; Leonardi, F.; Simeoni, C.; Tonnarini, S.; Veschetti, M. Indoor radon levels in schools of South-East Italy. J. Environ. Radioact. 2012, 112, 160–164. [Google Scholar] [CrossRef] [PubMed]
- Tunno, T.; Caricato, A.P.; Fernandez, M.; Leonardi, F.; Tonnarini, S.; Veschetti, M.; Zannoni, G.; Trevisi, R. Critical aspects of radon remediation in karst limestone areas: Some experiences in schools of South Italy. J. Radiol. Prot. 2017, 37, 160–175. [Google Scholar] [CrossRef] [PubMed]
- Leonardi, F.; Veschetti, M.; Tonnarini, S.; Cardellini, F.; Trevisi, R. A step towards accreditation: A robustness test of etching process. Appl. Radiat. Isot. 2015, 102, 93–97. [Google Scholar] [CrossRef] [PubMed]
- D’Alessandro, M.; Leonardi, F.; Tonnarini, S.; Trevisi, R.; Veschetti, M. Development of a framework of quality assurance practices for a radon passive dosemeter service. J. Radiol. Prot. 2010, 30, 149. [Google Scholar] [CrossRef] [PubMed]
- Cinelli, G.; Tondeur, F. Log-normality of indoor radon data in the Walloon region of Belgium. J. Environ. Radioact. 2015, 143, 100–109. [Google Scholar] [CrossRef] [PubMed]
- Alharbi, S.H.; Akber, R.A. Radon and thoron concentrations in public workplaces in Brisbane, Australia. J. Environ. Radioact. 2015, 144, 69–76. [Google Scholar] [CrossRef] [PubMed]
- Bochicchio, F.; Campos-Venuti, G.; Piermattei, S.; Nuccetelli, C.; Risica, S.; Tommasino, L.; Torri, G.; Magnoni, M.; Agnesod, G.; Sgorbati, G.; et al. Annual average and seasonal variations of residential radon concentration for all the Italian Regions. Radiat. Meas. 2005, 40, 686–694. [Google Scholar] [CrossRef]
- Charlet, J.M.; Zhu, H.C.; Poffijn, A. The radon anomaly of Porcheresse (Ardennes, Belgium). A case study. Nuovo Cim. C 1999, 22, 491–496. [Google Scholar]



| Floor | No. of Rooms Per Floor | No. of Buildings Per Floor |
|---|---|---|
| Below ground | 116 (12%) | 21 |
| Ground | 642 (67%) | 53 |
| First | 116 (12%) | 34 |
| Second | 56 (6%) | 13 |
| Third | 19 (2%) | 4 |
| Fourth | 14 (1%) | 2 |
| Total | 963 | |
| Story | No. of buildings per story | |
| 1 | 16 (29.6%) | |
| 2 | 20 (37.0%) | |
| 3 | 6 (11.1%) | |
| 4 | 8 (14.8%) | |
| 5 | 3 (5.6%) | |
| 6 | 1 (1.9%) | |
| Total | 54 |
| Dataset | Range (Bq/m3) | Q1–Q3 (Bq/m3) | Median (Bq/m3) | AM (Bq/m3) | SD (Bq/m3) | GM (Bq/m3) | GSD | CV (%) |
|---|---|---|---|---|---|---|---|---|
| ARn | 38–1849 | 80–145 | 101 | 161 | 189 | 120 | 1.9 | 117 |
| F_AM 3 | 65–1040 | 88–161 | 104 | 174 | 173 | 134 | 1.9 | 100 |
| R_AM 4 | 61–915 | 90–149 | 108 | 174 | 159 | 136 | 1.9 | 92 |
| Rn < 200 Bq/m3 | 200 < Rn < 300 Bq/m3 | 300 < Rn < 500 Bq/m3 | Rn > 500 Bq/m3 | |||||
| ARn | 816 (85%) | 39 (4%) | 61 (6%) | 47 (5%) | ||||
| F_AM 3 | 43 (80%) | 4 (7%) | 4 (7%) | 3 (6%) | ||||
| R_AM 4 | 42 (78%) | 4 (7%) | 6 (11%) | 2 (4%) |
| Floor | N | Range (Bq/m3) | Q1–Q3 (Bq/m3) | Median (Bq/m3) | AM (Bq/m3) | SD (Bq/m3) | CV (%) |
|---|---|---|---|---|---|---|---|
| Below ground | 21 | 54–1849 | 88–134 | 110 | 244 | 410 | 168 |
| Ground A | 53 | 45–925 | 88–152 | 108 | 176 | 166 | 94 |
| First A,B | 34 | 65–688 | 89–127 | 102 | 177 | 172 | 97 |
| Second and upper B | 13 | 39–714 | 76–105 | 89 | 134 | 175 | 130 |
| Below ground | 20 | 54–1849 | 88–130 | 108 | 248 | 421 | 170 |
| Ground | 20 | 45–925 | 78–154 | 102 | 186 | 220 | 118 |
| Ground A | 34 | 70–925 | 94–183 | 118 | 202 | 192 | 95 |
| First A | 34 | 65–688 | 89–127 | 102 | 177 | 172 | 97 |
| Dataset | N | Range (%) | Q1–Q3 (%) | Median (%) | AM (%) | SD (%) |
|---|---|---|---|---|---|---|
| F_AM 2,A | 52 | 3–87 | 9–23 | 15 | 23 | 21 |
| R_AM 3,A | 52 | 3–82 | 18–40 | 25 | 31 | 20 |
| BGF | 16 | 8–107 | 16–34 | 26 | 30 | 24 |
| GF B,C | 51 | 3–72 | 17–41 | 24 | 29 | 19 |
| FF B | 31 | 0.5–113 | 11–24 | 18 | 23 | 23 |
| SUF C | 12 | 7–38 | 8–21 | 13 | 16 | 11 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Leonardi, F.; Botti, T.; Buresti, G.; Caricato, A.P.; Chezzi, A.; Pepe, C.; Spagnolo, S.; Tonnarini, S.; Veschetti, M.; Trevisi, R. Radon Spatial Variations in University’s Buildings Located in an Italian Karst Region. Atmosphere 2021, 12, 1048. https://doi.org/10.3390/atmos12081048
Leonardi F, Botti T, Buresti G, Caricato AP, Chezzi A, Pepe C, Spagnolo S, Tonnarini S, Veschetti M, Trevisi R. Radon Spatial Variations in University’s Buildings Located in an Italian Karst Region. Atmosphere. 2021; 12(8):1048. https://doi.org/10.3390/atmos12081048
Chicago/Turabian StyleLeonardi, Federica, Teresa Botti, Giuliana Buresti, Anna Paola Caricato, Alberto Chezzi, Carlo Pepe, Sabina Spagnolo, Sabrina Tonnarini, Miriam Veschetti, and Rosabianca Trevisi. 2021. "Radon Spatial Variations in University’s Buildings Located in an Italian Karst Region" Atmosphere 12, no. 8: 1048. https://doi.org/10.3390/atmos12081048
APA StyleLeonardi, F., Botti, T., Buresti, G., Caricato, A. P., Chezzi, A., Pepe, C., Spagnolo, S., Tonnarini, S., Veschetti, M., & Trevisi, R. (2021). Radon Spatial Variations in University’s Buildings Located in an Italian Karst Region. Atmosphere, 12(8), 1048. https://doi.org/10.3390/atmos12081048








