Anabatic Flow along a Uniformly Heated Slope Studied through Large-Eddy Simulation
Abstract
1. Introduction
2. Numerical Methodology
2.1. Governing Equations
2.2. Non-Dimensional Numbers and Parameters
2.3. Large-Eddy Simulation Technique
2.4. Algorithm and Numerical Settings
3. Simulation Approach Validation
3.1. Validation Case Setup
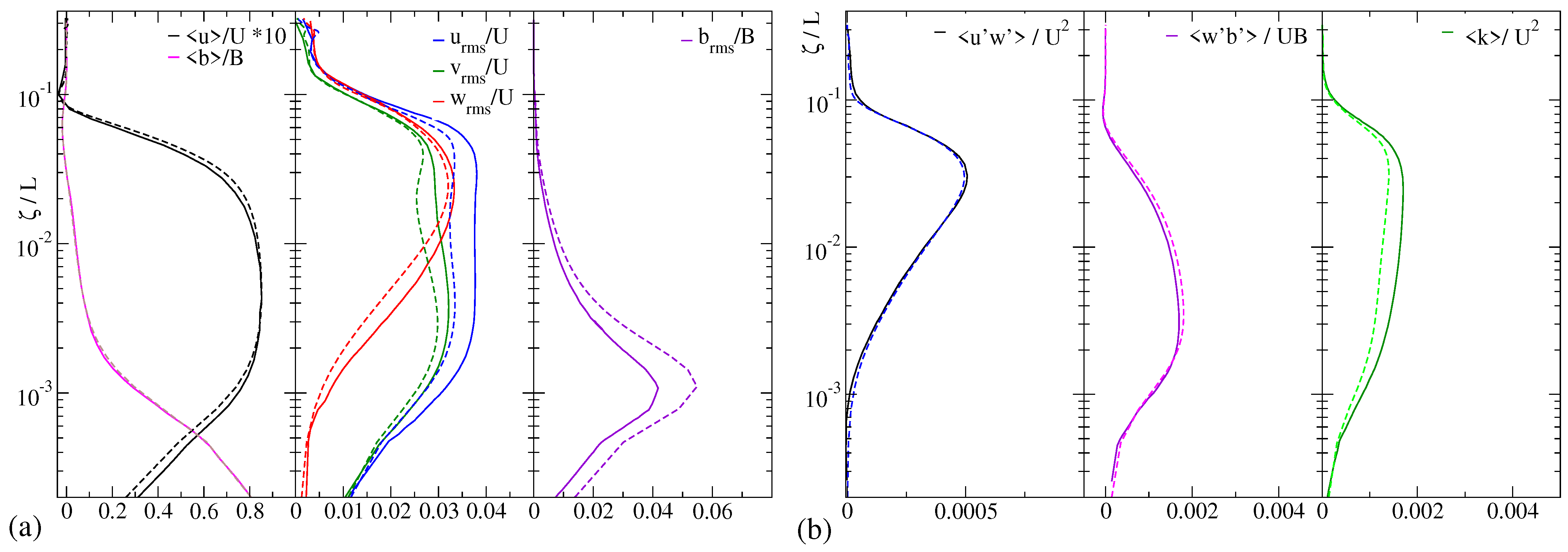
3.2. Validation Results
4. Case Study Setup
4.1. Computational Grid, Initial and Boundary Conditions
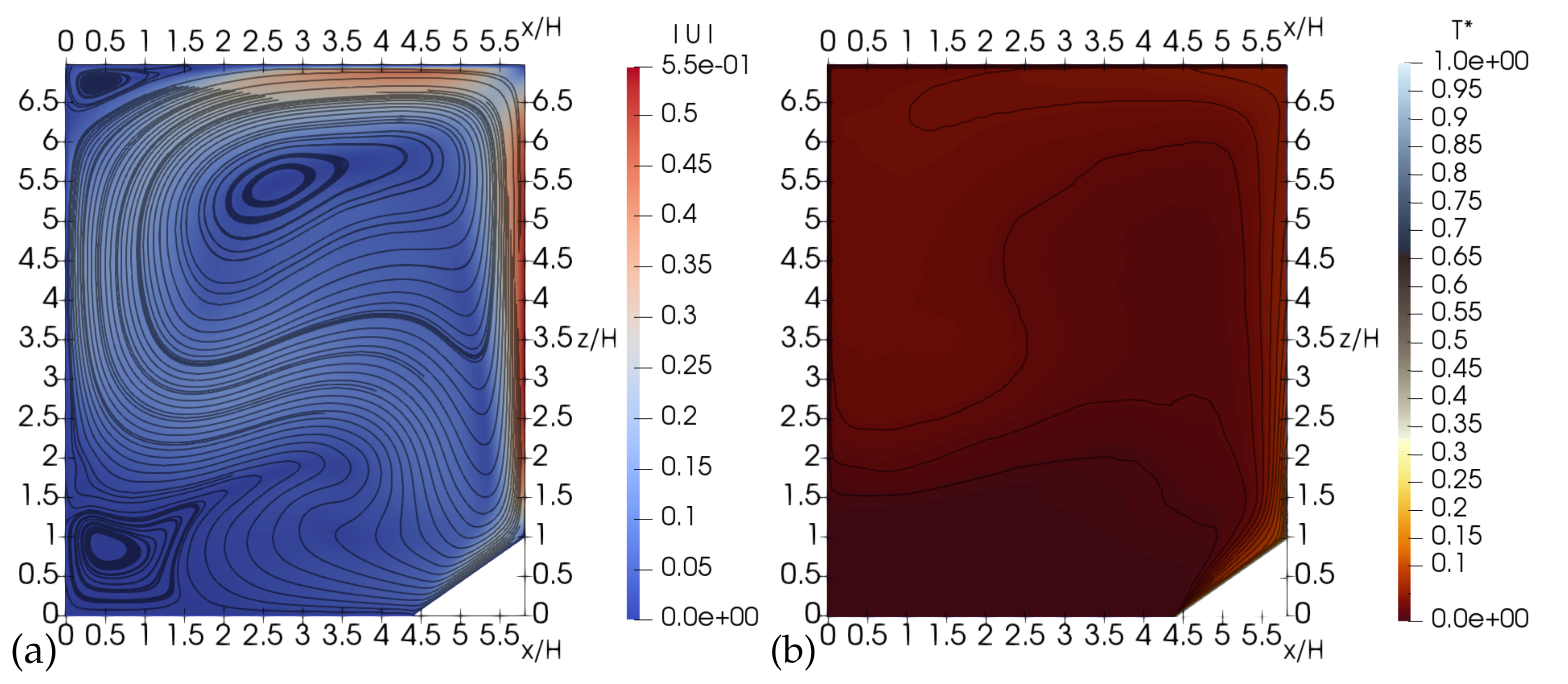
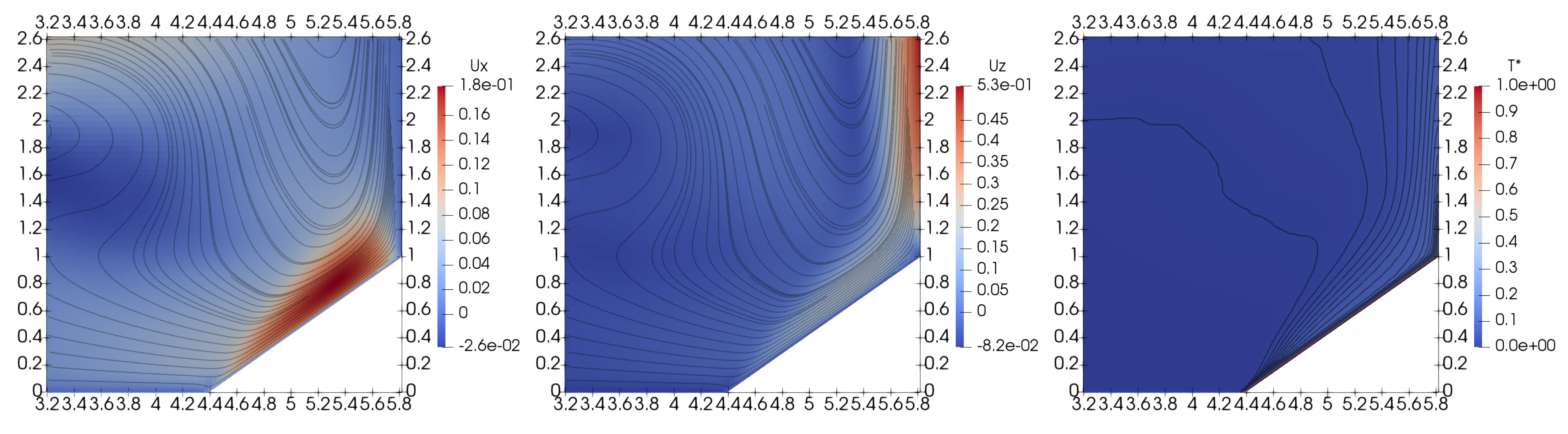
4.2. Overall Circulation
5. Results and Discussion
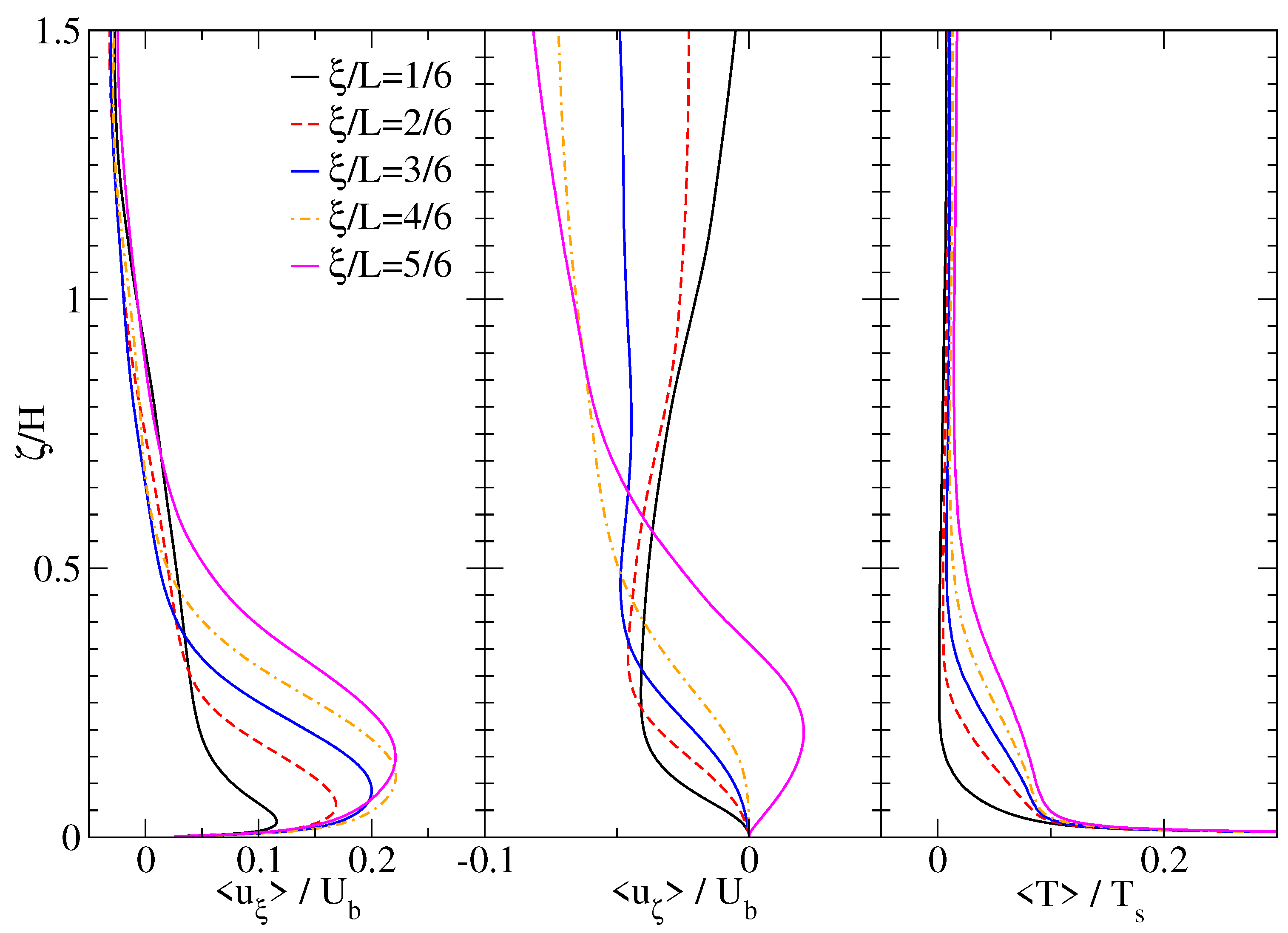
5.1. First-Order Statistics
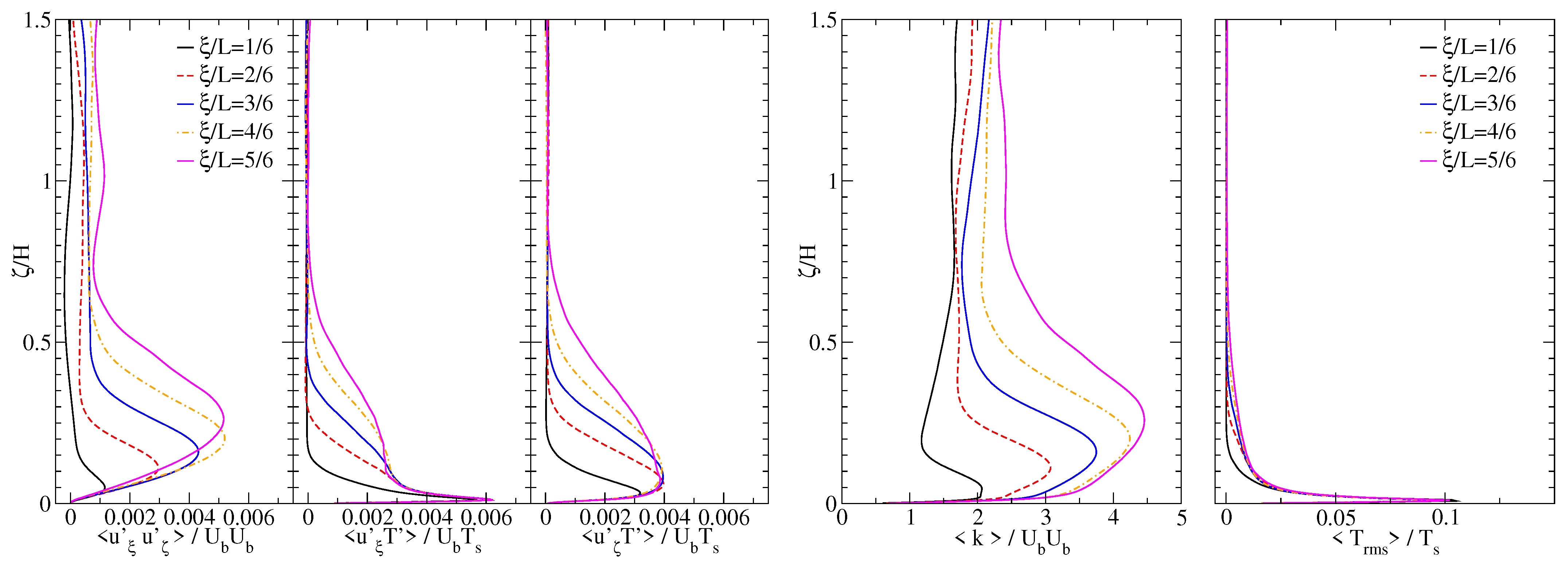
5.2. Second-Order Statistics
- Conductive layer:
- The thin region close to the slope surface () where the slope-parallel velocity rapidly increases, temperatures following an exponential decay, and slope-parallel heat fluxes turbulent flows reach their maximum. Slope-normal heat transfer is dominated by conduction.
- Convective layer:
- The layer above the slope where the anabatic flow mainly develops, corresponding to the region of largest slope-parallel velocity component and most energetic turbulent fluxes. Temperature profiles display an almost linear decrease, and the layer thickness increases along the slope before being destroyed by the ascending flow.
- Outer region:
- Relatively far from the slope (). The velocity loses most of the energy generated by the buoyancy force at the slope, the temperature perturbation is almost negligible, and turbulent fluxes decay to low values. This region is almost unperturbed by the slope anabatic flow.
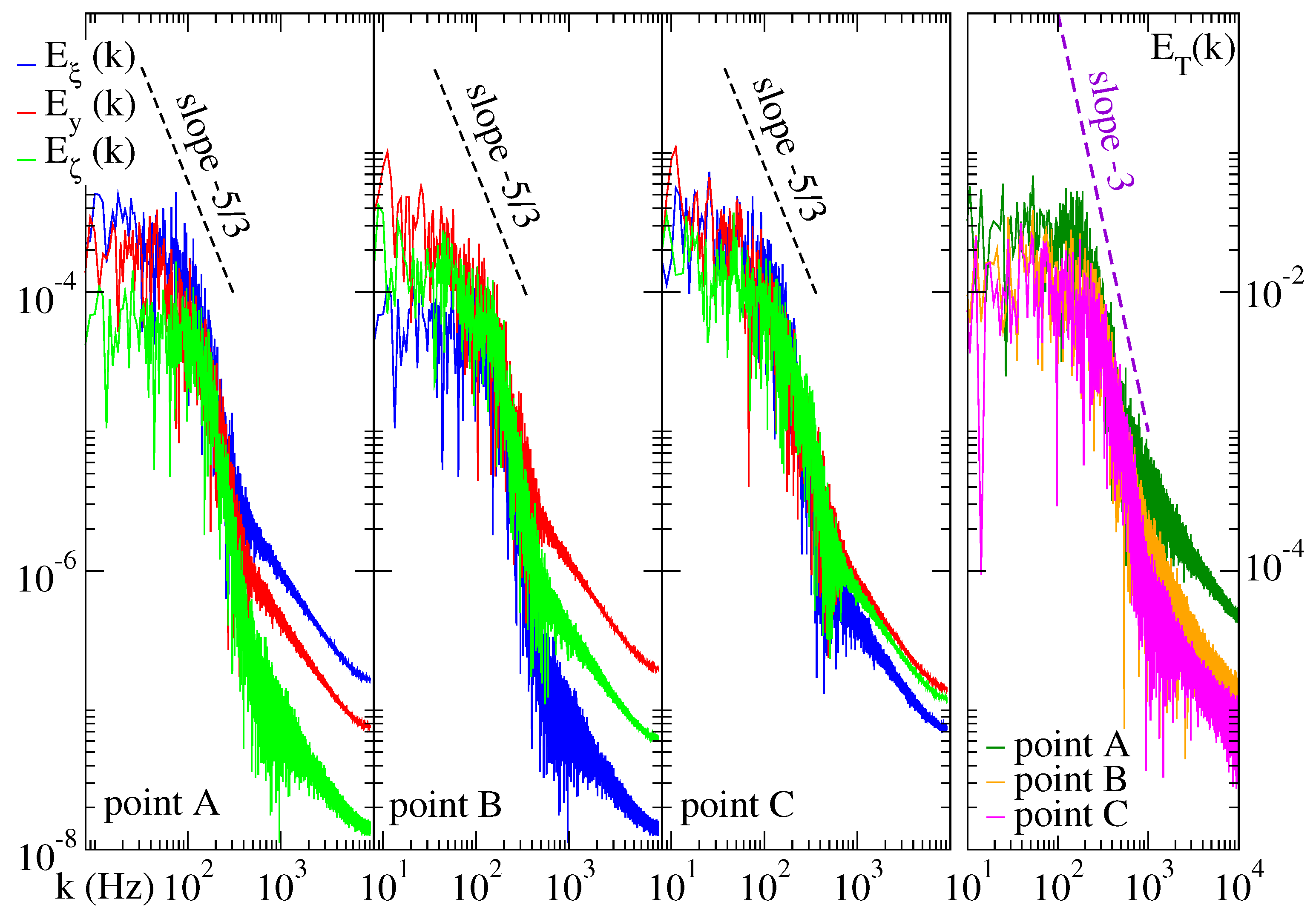
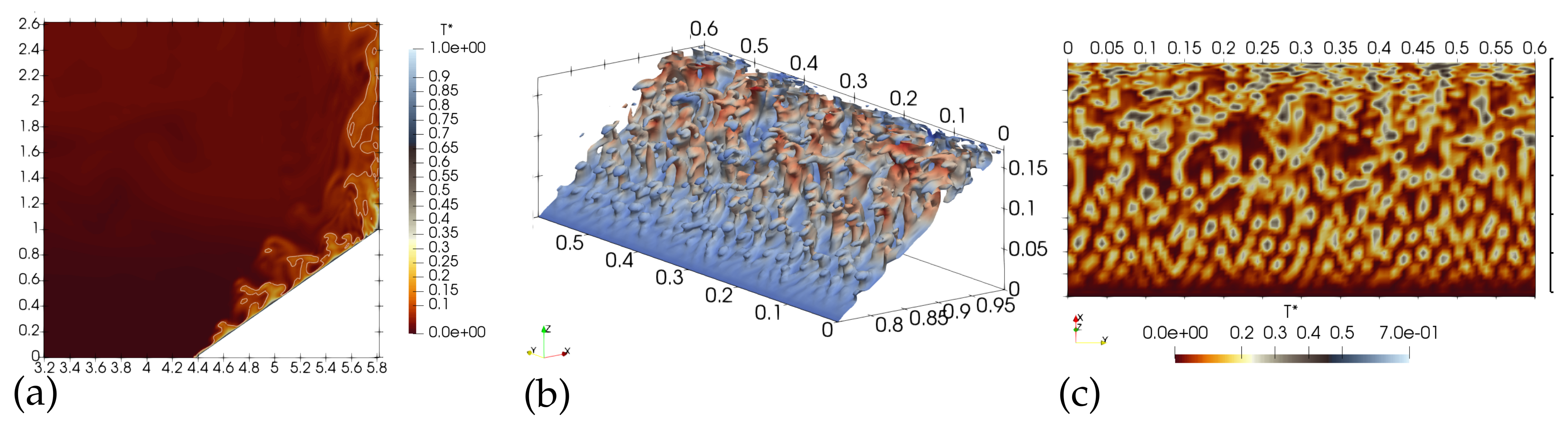
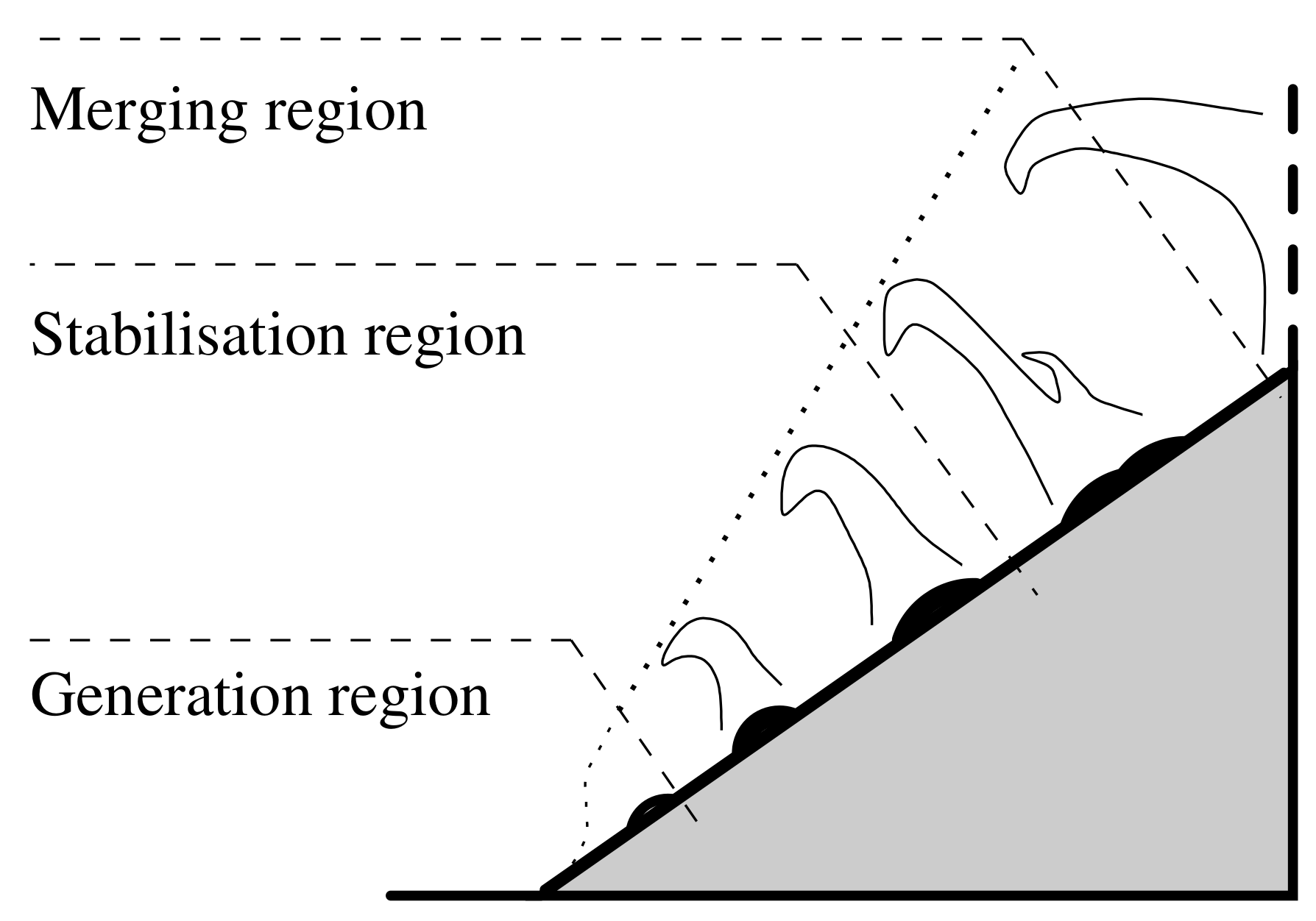
5.3. Turbulent Structures
- Generation region (): Spots are almost absent at the bottom edge of the slope. The weak thermal instabilities are generated, but plumes are almost not detectable.
- Stabilisation region (): Spots assume an almost circular shape and the central temperature increases. Plumes emerge and develop in the convective layer, assuming their hook-line shape.
- Merging region (): Spots merge together while they are transported near the apex, where they appear elongated spanwise and are destroyed. Plumes detached from the surface and their axis is rotated horizontally. They are transported by the main flow and eventually destroyed by the highly energetic ascending flow.
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Whiteman, D.C. Observations of Thermally Developed Wind Systems in Mountainous Terrain. In Atmospheric Processes over Complex Terrain; Blumen, W., Ed.; American Meteorological Society: Boston, MA, USA, 1990; pp. 5–42._2. [Google Scholar] [CrossRef]
- Monti, P.; Fernando, H.; Princevac, M.; Chan, W.; Kowalewski, T.; Pardyjak, E. Observations of Flow and Turbulence in the Nocturnal Boundary Layer over a Slope. J. Atmos. Sci. 2002, 59. [Google Scholar] [CrossRef]
- Rampanelli, G.; Zardi, D.; Rotunno, R. Mechanisms of Up-Valley Winds. J. Atmos. Sci. 2004, 61, 3097–3111. [Google Scholar] [CrossRef]
- Rotach, M.; Zardi, D. On the Boundary Layer Structure over Highly Complex Terrain: Key Findings from MAP and Related Projects; Hrvatski Meteoroloski Casopis: Zagreb, Croatia, 2005; pp. 124–127. [Google Scholar]
- Fernando, H.J.S. Fluid dynamics of urban atmospheres in complex terrain. Annu. Rev. Fluid Mech. 2008, 42, 365–389. [Google Scholar] [CrossRef]
- Zardi, D.; Whiteman, C. Diurnal Mountain Wind Systems. Mt. Weather. Res. Forecast. 2013, 35–119._2. [Google Scholar] [CrossRef]
- Jensen, D.; Nadeau, D.; Hoch, S.; Pardyjak, E. Observations of Near-Surface Heat-Flux and Temperature Profiles Through the Early Evening Transition over Contrasting Surfaces. Bound. Layer Meteorol. 2015, 159. [Google Scholar] [CrossRef]
- Princevac, M.; Fernando, H. A criterion for the generation of turbulent anabatic flows. Phys. Fluids 2007, 19, 105102. [Google Scholar] [CrossRef]
- Hunt, J.; Fernando, H.; Princevac, M. Unsteady thermally driven flows on gentle slopes. J. Atmos. Sci. 2003, 60, 2169–2182. [Google Scholar] [CrossRef]
- Serafin, S.; Adler, B.; Cuxart, J.; De Wekker, S.F.J.; Gohm, A.; Grisogono, B.; Kalthoff, N.; Kirshbaum, D.J.; Rotach, M.W.; Schmidli, J.; et al. Exchange Processes in the Atmospheric Boundary Layer Over Mountainous Terrain. Atmosphere 2018, 9, 102. [Google Scholar] [CrossRef]
- Princevac, M.; Fernando, H. Morning breakup of cold pools in complex terrain. J. Fluid Mech. 2008, 616, 99. [Google Scholar] [CrossRef]
- Nadeau, D.F.; Oldroyd, H.J.; Pardyjak, E.R.; Sommer, N.; Hoch, S.W.; Parlange, M.B. Field observations of the morning transition over a steep slope in a narrow alpine valley. Environ. Fluid Mech. 2018, 20, 1–22. [Google Scholar] [CrossRef]
- Grubišić, V.; Doyle, J.; Kuettner, J.; Mobbs, S.; Smith, R.; Whiteman, C.; Dirks, R.; Czyzyk, S.; Cohn, S.; Vosper, S.; et al. The Terrain-Induced Rotor Experiment: An overview of the field campaign and some highlights of special observations. Bull. Amer. Meteor. Soc. 2008, 89, 1513–1533. [Google Scholar] [CrossRef]
- Serafin, S.; Zardi, D. Structure of the Atmospheric Boundary Layer in the Vicinity of a Developing Upslope Flow System: A Numerical Model Study. J. Atmos. Sci. 2010, 67, 1171–1185. [Google Scholar] [CrossRef]
- De Wekker, S.F.J. Observational and Numerical Evidence of Depressed Convective Boundary Layer Heights near a Mountain Base. J. Appl. Meteorol. Climatol. 2008, 47, 1017–1026. [Google Scholar] [CrossRef]
- Serafin, S.; Zardi, D. Daytime Heat Transfer Processes Related to Slope Flows and Turbulent Convection in an Idealized Mountain Valley. J. Atmos. Sci. 2010, 67, 3739–3756. [Google Scholar] [CrossRef]
- De Wekker, S.F.; Kossmann, M. Convective boundary layer heights over mountainous terrain—A review of concepts. Front. Earth Sci. 2015, 3, 77. [Google Scholar] [CrossRef]
- Finnigan, J.; Ayotte, K.; Harman, I.; Katul, G.; Oldroyd, H.; Patton, E.; Poggi, D.; Ross, A.; Taylor, P. Boundary-Layer Flow over complex topography. Bound.-Layer Meteorol. 2020, 177, 247–313. [Google Scholar] [CrossRef]
- Prandtl, L. Fuhrer Durch Die Stromungslehre; Veweg und Sohn: Wiesbaden, Germany, 1942; pp. 373–375. [Google Scholar]
- Defant, F. Local Winds. In Compendium of Meteorology; Malone, T.F., Ed.; American Meteorological Society: Boston, MA, USA, 1951. [Google Scholar] [CrossRef]
- Egger, J. Linear two-dimensional theory of thermally induced slope winds. Contrib. Atmos. Phys. 1981, 54, 465–481. [Google Scholar]
- Ye, Z.J.; Segal, M.; Pielke, R.A. Effects of Atmospheric Thermal Stability and Slope Steepness on the Development of Daytime Thermally Induced Upslope Flow. J. Atmos. Sci. 1987, 44, 3341–3354. [Google Scholar] [CrossRef][Green Version]
- Kavčič, I.; Grisogono, B. Katabatic flow with Coriolis effect and gradually varying eddy diffusivity. In Atmospheric Boundary Layers: Nature, Theory and Applications to Environmental Modelling and Security; Baklanov, A., Grisogono, B., Eds.; Springer: New York, NY, USA, 2007; pp. 221–231. [Google Scholar] [CrossRef]
- Zardi, D.; Serafin, S. An analytic solution for time-periodic thermally driven slope flows. Q. J. R. Meteorol. Soc. 2015, 141, 1968–1974. [Google Scholar] [CrossRef]
- Guttler, I.; Marinovic, I.; Večenaj, Ž.; Grisogono, B. Energetics of Slope Flows: Linear and Weakly Nonlinear Solutions of the Extended Prandtl Model. Front. Earth Sci. 2016, 4. [Google Scholar] [CrossRef]
- Whiteman, C.D. Breakup of temperature inversions in deep mountain valleys: Part I. Observations. J. Appl. Meteorol. Climatol. 1982, 21, 270–289. [Google Scholar] [CrossRef]
- Kader, B.; Yaglom, A. Mean fields and fluctuation moments in unstably stratified turbulent boundary layers. J. Fluid Mech. 1990, 212, 637–662. [Google Scholar] [CrossRef]
- Moroni, M.; Cenedese, A. Laboratory Simulations of Local Winds in the Atmospheric Boundary Layer via Image Analysis. Adv. Meteorol. 2015, 2015, 1–34. [Google Scholar] [CrossRef]
- Perry, S.; Snyder, W. Laboratory simulations of the atmospheric mixed-layer in flow over complex topography. Phys. Fluids 2017, 29, 020702. [Google Scholar] [CrossRef]
- Reuten, C.; Steyn, D.G.; Allen, S.E. Water tank studies of atmospheric boundary layer structure and air pollution transport in upslope flow systems. J. Geophys. Res. (Atmos.) 2007, 112. [Google Scholar] [CrossRef]
- Crook, N.A.; Tucker, D.F. Flow over Heated Terrain. Part I: Linear Theory and Idealized Numerical Simulations. Mon. Weather Rev. 2005, 133, 2552–2564. [Google Scholar] [CrossRef][Green Version]
- Hocut, C.; Liberzon, D.; Fernando, H. Separation of upslope flow over a uniform slope. J. Fluid Mech. 2015, 775, 266–287. [Google Scholar] [CrossRef]
- Goldshmid, R.H.; Bardoel, S.L.; Hocut, C.M.; Zhong, Q.; Liberzon, D.; Fernando, H.J. Separation of upslope flow over a plateau. Atmosphere 2018, 9, 165. [Google Scholar] [CrossRef]
- Skyllingstad, E.D. Large-eddy simulation of katabatic flows. Bound. Layer Meteorol. 2003, 106, 217–243. [Google Scholar] [CrossRef]
- Grisogono, B.; Axelsen, S. A Note on the Pure Katabatic Wind Maximum over Gentle Slopes. Bound. Layer Meteorol. 2012, 142, 527–538. [Google Scholar] [CrossRef]
- Axelsen, S.L.; van Dop, H. Large-eddy simulation of katabatic winds. Part 1: Comparison with observations. Acta Geophys. 2009, 57, 803–836. [Google Scholar] [CrossRef]
- Axelsen, S.L.; van Dop, H. Large-eddy simulation of katabatic winds. Part 2: Sensitivity study and comparison with analytical models. Acta Geophys. 2009, 57, 837–856. [Google Scholar] [CrossRef]
- Schumann, U. Large-eddy simulation of the up-slope boundary layer. Q. J. R. Meteorol. Soc. 1990, 116, 637–670. [Google Scholar] [CrossRef]
- Dörnbrack, A.; Schumann, U. Numerical simulation of turbulent convective flow over wavy terrain. Bound.-Layer Meteorol. 1993, 65, 323–355. [Google Scholar] [CrossRef]
- Anquetin, S.; Guilbaud, C.; Chollet, J.P. The formation and destruction of inversion layers within a deep valley. J. Appl. Meteorol. 1998, 37, 1547–1560. [Google Scholar] [CrossRef]
- Luo, Z.; Li, Y. Passive urban ventilation by combined buoyancy-driven slope flow and wall flow: Parametric CFD studies on idealized city models. Atmos. Environ. 2011, 45, 5946–5956. [Google Scholar] [CrossRef]
- Fedorovich, E.; Shapiro, A. Structure of numerically simulated katabatic and anabatic flows along steep slopes. Acta Geophys. 2009, 57, 981–1010. [Google Scholar] [CrossRef]
- Giometto, M.G.; Katul, G.G.; Fang, J.; Parlange, M.B. Direct numerical simulation of turbulent slope flows up to Grashof number Gr = 2.1×1011. J. Fluid Mech. 2017, 829, 589–620. [Google Scholar] [CrossRef]
- Talbot, C.; Bou-Zeid, E.; Smith, J. Nested Mesoscale Large-Eddy Simulations with WRF: Performance in Real Test Cases. J. Hydrometeorol. 2012, 13, 1421–1441. [Google Scholar] [CrossRef]
- Catalano, F.; Cenedese, A. High-resolution numerical modeling of thermally driven slope winds in a valley with strong capping. J. Appl. Meteorol. Climatol. 2010, 49, 1859–1880. [Google Scholar] [CrossRef]
- Chow, F.K.; Weigel, A.P.; Street, R.L.; Rotach, M.W.; Xue, M. High-resolution large-eddy simulations of flow in a steep Alpine valley. Part I: Methodology, verification, and sensitivity experiments. J. Appl. Meteorol. Climatol. 2006, 45, 63–86. [Google Scholar] [CrossRef]
- Golaz, J.C.; Doyle, J.D.; Wang, S. One-way nested large-eddy simulation over the Askervein Hill. J. Adv. Model. Earth Syst. 2009, 1. [Google Scholar] [CrossRef]
- Weigel, A.P.; Chow, F.K.; Rotach, M.W.; Street, R.L.; Xue, M. High-resolution large-eddy simulations of flow in a steep Alpine valley. Part II: Flow structure and heat budgets. J. Appl. Meteorol. Climatol. 2006, 45, 87–107. [Google Scholar] [CrossRef]
- Châtelain, A.; Ducros, F.; Métais, O. LES of turbulent heat transfer: Proper convection numerical schemes for temperature transport. Int. J. Numer. Methods Fluids 2004, 44, 1017–1044. [Google Scholar] [CrossRef]
- Fedorovic, E.; Shapiro, A. Turbulent natural convection along a vertical plate immersed in a stably stratified fluid. J. Fluid Mech. 2009, 636, 41–57. [Google Scholar] [CrossRef]
- Sagaut, P. Large Eddy Simulation for Incompressible Flows. An Introduction; Springer: Berlin/Heidelberg, Germany, 2000. [Google Scholar]
- Pope, S. Turbulent Flows; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar]
- Smagorinsky, J. general circulation experiments with the primitive equations: I. The basic experiment. Mon. Weather Rev. 1963, 91, 99. [Google Scholar] [CrossRef]
- Van Driest, E. On turbulent flow near a wall. J. Aeronaut. Sci. 1956, 23, 1007–1011. [Google Scholar] [CrossRef]
- Cintolesi, C.; Petronio, A.; Armenio, V. Turbulent structures of buoyant jet in cross-flow studied through large-eddy simulation. Environ. Fluid Mech. 2019, 19, 401–433. [Google Scholar] [CrossRef]
- Cintolesi, C.; Pulvirenti, B.; Di Sabatino, S. Large-Eddy Simulations of Pollutant Removal Enhancement from Urban Canyons. Bound. Layer Meteorol. 2021. [Google Scholar] [CrossRef]
- OpenFOAM. The OpenFOAM Foundation. Version 6.0. Available online: https://openfoam.org/ (accessed on 1 May 2019).
- Jasak, H.; Weller, H.; Gosman, A. High resolution NVD differencing scheme for arbitrarily unstructured meshes. Int. J. Numer. Meth. Fluids 1999, 31, 431–449. [Google Scholar] [CrossRef]
- Trivellato, F.; Raciti Castelli, M. On the Courant–Friedrichs–Lewy criterion of rotating grids in 2D vertical-axis wind turbine analysis. Renew. Energy 2014, 62, 53–62. [Google Scholar] [CrossRef]
- Cintolesi, C.; Petronio, A.; Armenio, V. Large eddy simulation of turbulent buoyant flow in a confined cavity with conjugate heat transfer. Phys. Fluids 2015, 27. [Google Scholar] [CrossRef]
- Smith, C.M.; Skyllingstad, E.D. Numerical Simulation of Katabatic Flow with Changing Slope Angle. Mon. Weather Rev. 2005, 133, 3065–3080. [Google Scholar] [CrossRef]
- Piomelli, U.; Balaras, E. Wall-Layer Models for Large-Eddy Simulations. Annu. Rev. Fluid Mech. 2002, 34, 349–374. [Google Scholar] [CrossRef]
- De Wit, L.; van Rhee, C.; Keetels, G. Turbulent Interaction of a Buoyant Jet with Cross-Flow. J. Hydraul. Eng. 2014, 140, 04014060. [Google Scholar] [CrossRef]
- Cavar, D.; Meyer, K.E. LES of turbulent jet in cross-flow: Part 1 – A numerical validation study. Int. J. Heat Fluid Flow 2012, 36, 18–34. [Google Scholar] [CrossRef]
- Launder, B.; Spalding, D. The numerical computation of turbulent flows. Comput. Methods Appl. Mech. Eng. 1974, 2, 263–289. [Google Scholar] [CrossRef]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cintolesi, C.; Di Santo, D.; Barbano, F.; Di Sabatino, S. Anabatic Flow along a Uniformly Heated Slope Studied through Large-Eddy Simulation. Atmosphere 2021, 12, 850. https://doi.org/10.3390/atmos12070850
Cintolesi C, Di Santo D, Barbano F, Di Sabatino S. Anabatic Flow along a Uniformly Heated Slope Studied through Large-Eddy Simulation. Atmosphere. 2021; 12(7):850. https://doi.org/10.3390/atmos12070850
Chicago/Turabian StyleCintolesi, Carlo, Dario Di Santo, Francesco Barbano, and Silvana Di Sabatino. 2021. "Anabatic Flow along a Uniformly Heated Slope Studied through Large-Eddy Simulation" Atmosphere 12, no. 7: 850. https://doi.org/10.3390/atmos12070850
APA StyleCintolesi, C., Di Santo, D., Barbano, F., & Di Sabatino, S. (2021). Anabatic Flow along a Uniformly Heated Slope Studied through Large-Eddy Simulation. Atmosphere, 12(7), 850. https://doi.org/10.3390/atmos12070850





