Teak Tree-Ring Cellulose δ13C, δ18O, and Tree-Ring Width from Northwestern Thailand Capture Different Aspects of Asian Monsoon Variability
Abstract
1. Introduction
2. Materials and Methods
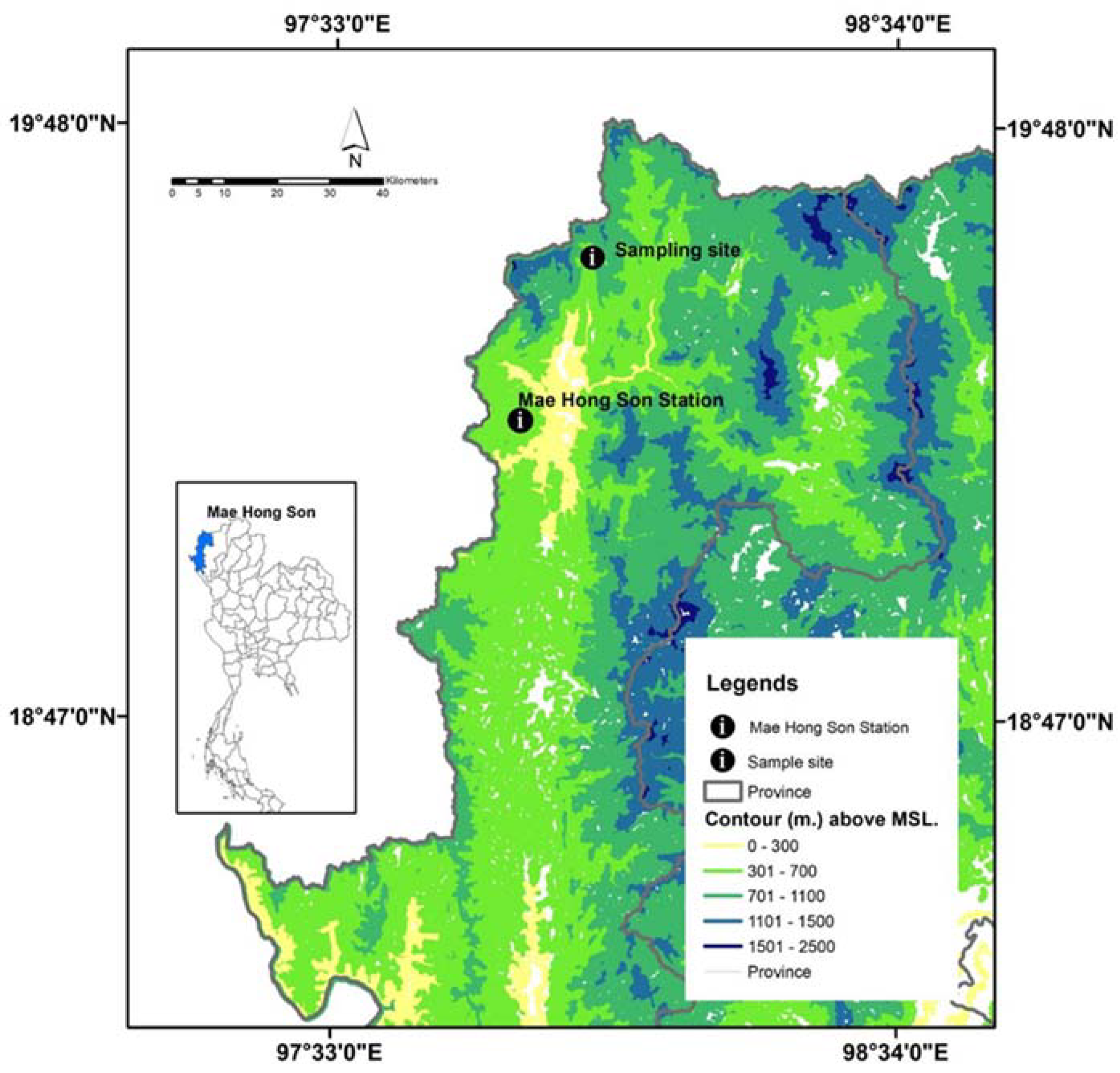
2.1. Study Area and Sampling Process
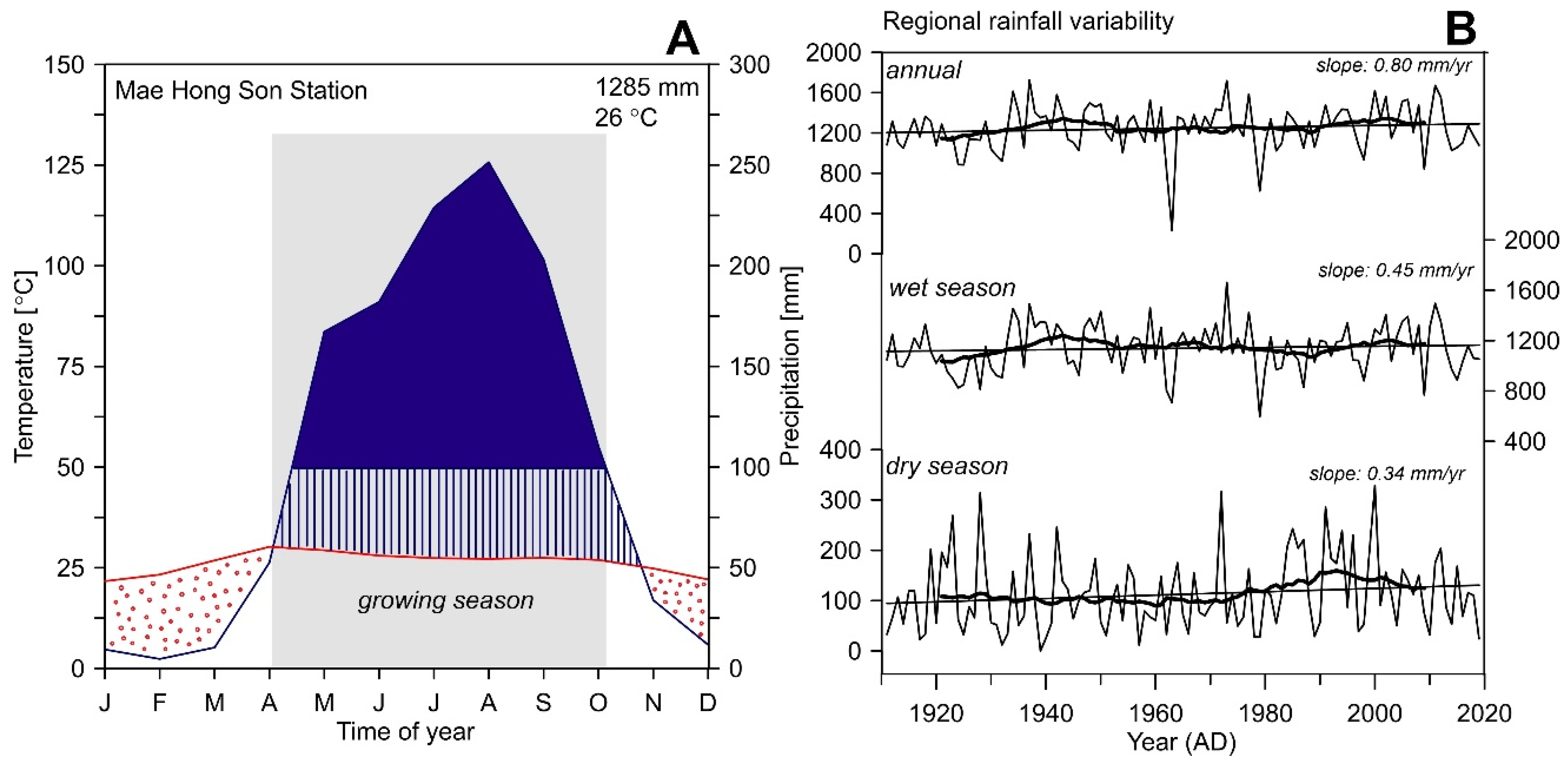
2.2. Climate Conditions in Northwestern Thailand
2.3. Cellulose Extraction
2.4. Statistical Analysis
3. Results
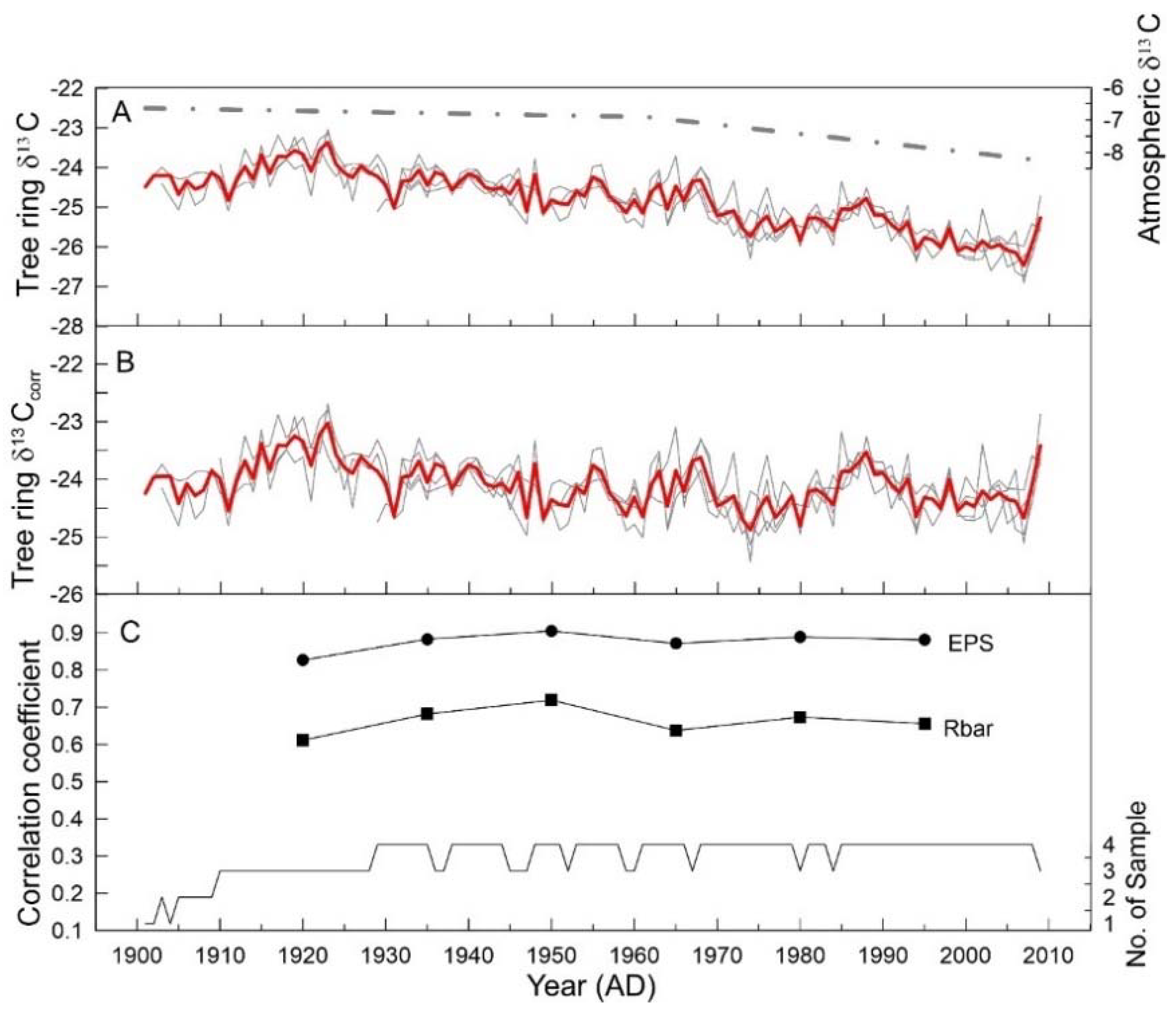
3.1. The Collection from the Effects of Changing Atmospheric δ13C
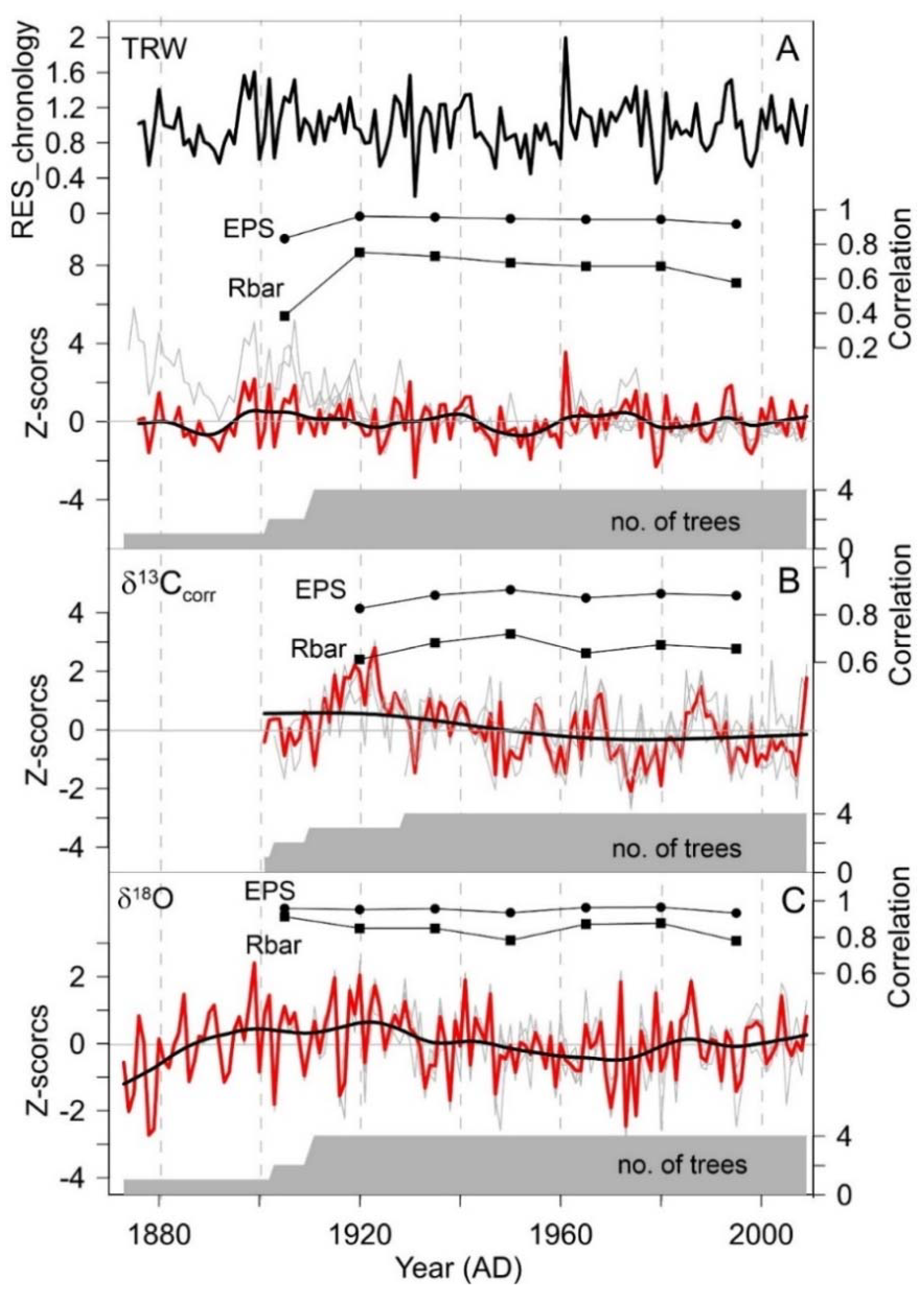
3.2. Chronologies Characteristics of Tree-Ring Parameters
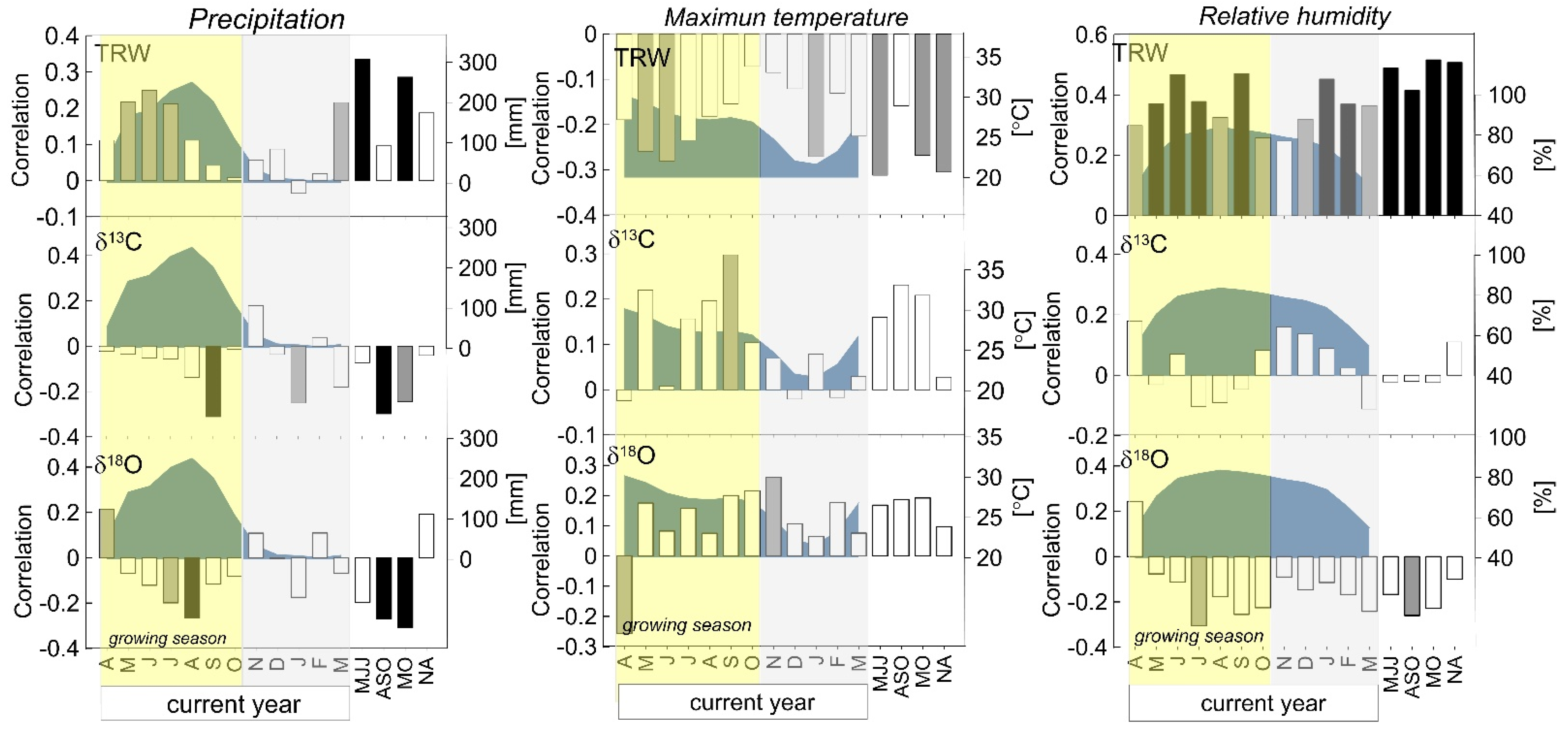
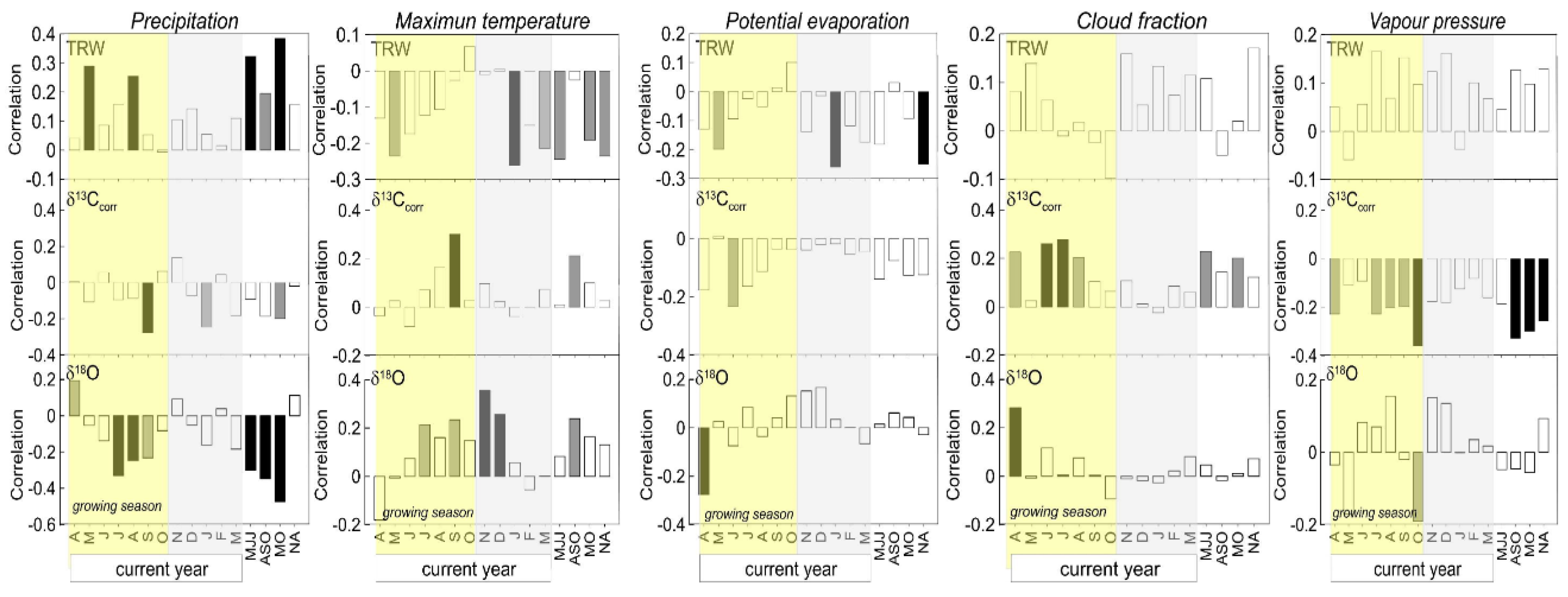
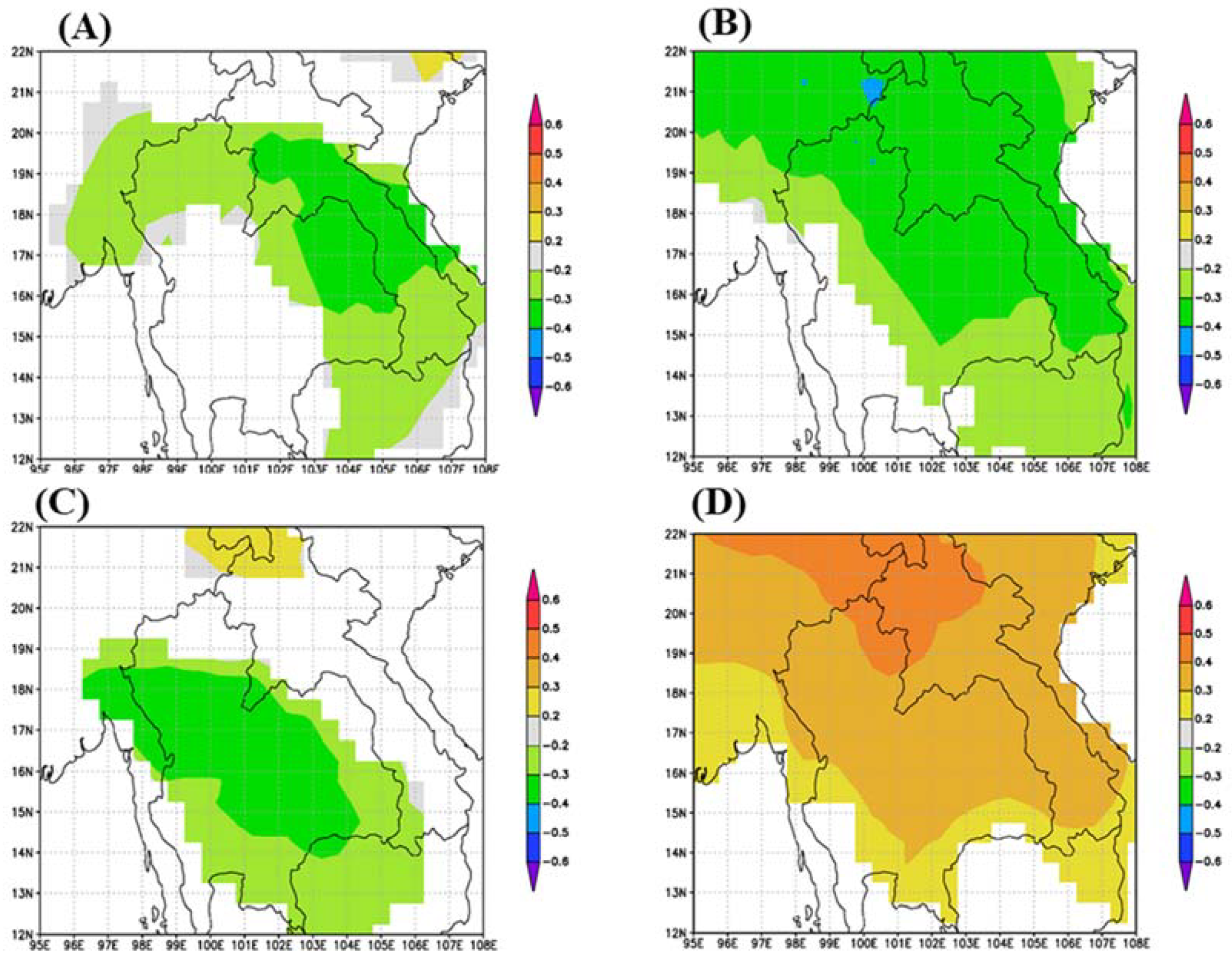
3.3. Climate Correlation of Tree-Ring Parameters
4. Discussion
4.1. Chronology Characteristics of All Tree-Ring Parameters
4.2. Climate Control of the Teak Tree-Ring Parameters
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Pumijumnong, N. Dendrochrnologie mit Teak (Tectona grandis L.) in Nord-Thailand. Jahrringbildung—Chronologiennetz—Klimasignal. Diss. Univ. Hamburg 1995, 104. [Google Scholar]
- Ram, S.; Borgaonkar, H.; Sikder, A. Tree-ring analysis of teak (Tectona grandis LF) in central India and its relationship with rainfall and moisture index. J. Earth Syst. Sci. 2008, 117, 637. [Google Scholar] [CrossRef]
- Buckley, B.M.; Palakit, K.; Duangsathaporn, K.; Sanguantham, P.; Prasomsin, P. Decadal scale droughts over northwestern Thailand over the past 448 years: Links to the tropical Pacific and Indian Ocean sectors. Clim. Dyn. 2007, 29, 63–71. [Google Scholar] [CrossRef]
- Pumijumnong, N. Dendrochronology in Southeast Asia. Trees 2013, 27, 343–358. [Google Scholar] [CrossRef]
- Jacoby, G.C.; Krusic, P.J. Progress in dendroclimatic studies in Indonesia. Terr. Atmos. Ocean. Sci. 1994, 5, 349–363. [Google Scholar]
- Kaosa-ard, A. Teak (Tectona grandis Linn. f) its natural distribution and related factors. Nat. His. Bull. Siam. Soc. 1981, 29, 55–74. [Google Scholar]
- Zaw, Z.; Fan, Z.-X.; Bräuning, A.; Liu, W.; Gaire, N.P.; Than, K.Z.; Panthi, S. Monsoon precipitation variations in Myanmar since AD 1770: Linkage to tropical ocean-atmospheric circulations. Clim. Dyn. 2021, 56, 3337–3352. [Google Scholar] [CrossRef]
- Pumijumnong, N. Teak tree ring widths: Ecology and climatology research in Northwest Thailand. Sci. Technol. Dev. 2012, 31, 165–174. [Google Scholar]
- D’Arrigo, R.; Palmer, J.; Ummenhofer, C.; Kyaw, N.; Krusic, P. Myanmar monsoon drought variability inferred by tree rings over the past 300 years: Linkages to ENSO. PAGES News 2013, 21, 50–51. [Google Scholar] [CrossRef]
- D’Arrigo, R.; Ummenhofer, C.C. The climate of Myanmar: Evidence for effects of the Pacific Decadal Oscillation. Int. J. Climatol. 2015, 35, 634–640. [Google Scholar] [CrossRef]
- Xu, C.; Sano, M.; Nakatsuka, T. Tree ring cellulose δ18O of Fokienia hodginsii in northern Laos: A promising proxy to reconstruct ENSO? J. Geophys. Res. Atmos. 2011, 116. [Google Scholar] [CrossRef]
- Schollaen, K.; Heinrich, I.; Neuwirth, B.; Krusic, P.J.; D’Arrigo, R.D.; Karyanto, O.; Helle, G. Multiple tree-ring chronologies (ring width, δ13C and δ18O) reveal dry and rainy season signals of rainfall in Indonesia. Quat. Sci. Rev. 2013, 73, 170–181. [Google Scholar] [CrossRef]
- Poussart, P.F.; Evans, M.N.; Schrag, D.P. Resolving seasonality in tropical trees: Multi-decade, high-resolution oxygen and carbon isotope records from Indonesia and Thailand. Earth Planet. Sci. Lett. 2004, 218, 301–316. [Google Scholar] [CrossRef]
- Poussart, P.F.; Schrag, D.P. Seasonally resolved stable isotope chronologies from northern Thailand deciduous trees. Earth Planet. Sci. Lett. 2005, 235, 752–765. [Google Scholar] [CrossRef]
- Muangsong, C.; Cai, B.; Pumijumnong, N.; Hu, C.; Lei, G. Intra-seasonal variability of teak tree-ring cellulose δ18O from northwestern Thailand: A potential proxy of Thailand summer monsoon rainfall. Holocene 2016, 26, 1397–1405. [Google Scholar] [CrossRef]
- Muangsong, C.; Pumijumnong, N.; Cai, B.; Buajan, S.; Lei, G.; Wang, F.; Li, M.; Payomrat, P. Effect of changes in precipitation amounts and moisture sources on inter-and intra-annual stable oxygen isotope ratios (δ18O) of teak trees from northern Thailand. Agric. For. Meteorol. 2020, 281, 107820. [Google Scholar] [CrossRef]
- Buajan, S.; Pumijumnong, N.; Li, Q.; Liu, Y. Oxygen isotope (δ¹⁸O) of teak tree-rings in north-west Thailand. J. Trop. For. Sci. 2016, 28, 396–405. [Google Scholar]
- Xu, C.; Pumijumnong, N.; Nakatsuka, T.; Sano, M.; Guo, Z. Inter-annual and multi-decadal variability of monsoon season rainfall in central Thailand during the period 1804–1999, as inferred from tree ring oxygen isotopes. Int. J. Climatol. 2018, 38, 5766–5776. [Google Scholar] [CrossRef]
- Xu, C.; Pumijumnong, N.; Nakatsuka, T.; Sano, M.; Li, Z. A tree-ring cellulose δ18O-based July–October precipitation reconstruction since AD 1828, northwest Thailand. J. Hydrol. 2015, 529, 433–441. [Google Scholar] [CrossRef]
- Payomrat, P.; Liu, Y.; Pumijumnong, N.; Li, Q.; Song, H. Tree-ring stable carbon isotope-based June–September maximum temperature reconstruction since AD 1788, north-west Thailand. Tellus B Chem. Phys. Meteorol. 2018, 70, 1443655. [Google Scholar] [CrossRef]
- McCarroll, D.; Loader, N.J. Stable isotopes in tree rings. Quat. Sci. Rev. 2004, 23, 771–801. [Google Scholar] [CrossRef]
- Gebrekirstos, A.; Worbes, M.; Teketay, D.; Fetene, M.; Mitlöhner, R. Stable carbon isotope ratios in tree rings of co-occurring species from semi-arid tropics in Africa: Patterns and climatic signals. Global Planet. Chang. 2009, 66, 253–260. [Google Scholar] [CrossRef]
- Rinntech. TSAP-WinTM: Time Series Analysis and Presentation for Dendrochronology and Related Applications. Version 4.64 for Microsoft Windows User Reference; Rinn, F., Ed.; Rinntech: Heidelberg, Germany, 2011. [Google Scholar]
- Holmes, R. Program COFECHA User’s Manual; Laboratory of Tree-Ring Research, The University of Arizona: Tucson, AZ, USA, 1983. [Google Scholar]
- Grissino-Mayer, H.D. Evaluating crossdating accuracy: A manual and tutorial for the computer program COFECHA. Tree Ring Res. 2001, 57, 205–221. [Google Scholar]
- Cook, E.R. A Time Series Analysis Approach to Tree Ring Standardization (Dendrochronology, Forestry, Dendroclimatology, Autoregressive Process). Ph.D. Thesis, The University of Arizona, Tucson, AZ, USA, 1985. [Google Scholar]
- Thai Meteorological Department. Average Rainfall and Temperature Data from Mae Hong Son Station. Available online: http://www.tmd.go.th (accessed on 25 February 2021).
- Wieloch, T.; Helle, G.; Heinrich, I.; Voigt, M.; Schyma, P. A novel device for batch-wise isolation of α-cellulose from small-amount wholewood samples. Dendrochronologia 2011, 29, 115–117. [Google Scholar] [CrossRef]
- Pumijumnong, N.; Bräuning, A.; Sano, M.; Nakatsuka, T.; Muangsong, C.; Buajan, S. A 338-year tree-ring oxygen isotope record from Thai teak captures the variations in the Asian summer monsoon system. Sci. Rep. 2020, 10, 1–11. [Google Scholar] [CrossRef] [PubMed]
- Wigley, T.M.; Briffa, K.R.; Jones, P.D. On the average value of correlated time series, with applications in dendroclimatology and hydrometeorology. J. Clim. Appl. Meteorol. 1984, 23, 201–213. [Google Scholar] [CrossRef]
- Leavitt, S.W.; Danzer, S.R. Method for batch processing small wood samples to holocellulose for stable-carbon isotope analysis. Anal. Chem. 1993, 65, 87–89. [Google Scholar] [CrossRef]
- McCarroll, D.; Gagen, M.H.; Loader, N.J.; Robertson, I.; Anchukaitis, K.J.; Los, S.; Young, G.H.; Jalkanen, R.; Kirchhefer, A.; Waterhouse, J.S. Correction of tree ring stable carbon isotope chronologies for changes in the carbon dioxide content of the atmosphere. Geochim. Cosmochim. Acta 2009, 73, 1539–1547. [Google Scholar] [CrossRef]
- Freyer, H.; Belacy, N. 13C/12C records in northern hemispheric trees during the past 500 years—anthropogenic impact and climatic superpositions. J. Geophys. Res. Ocean 1983, 88, 6844–6852. [Google Scholar] [CrossRef]
- Robertson, I.; Switsur, V.; Carter, A.; Barker, A.; Waterhouse, J.; Briffa, K.; Jones, P. Signal strength and climate relationships in 13C/12C ratios of tree ring cellulose from oak in east England. J. Geophys. Res. Atmos. 1997, 102, 19507–19516. [Google Scholar] [CrossRef]
- Anderson, W.; Bernasconi, S.; McKenzie, J.; Saurer, M. Oxygen and carbon isotopic record of climatic variability in tree ring cellulose (Picea abies): An example from central Switzerland (1913–1995). J. Geophys. Res. Atmos. 1998, 103, 31625–31636. [Google Scholar] [CrossRef]
- Duquesnay, A.; Bréda, N.; Stievenard, M.; Dupouey, J.-L. Changes of tree-ring δ13C and water-use efficiency of beech (Fagus sylvatica L.) in north-eastern France during the past century. Plant Cell Environ. 1998, 21, 565–572. [Google Scholar] [CrossRef]
- Saurer, M.; Borella, S.; Leuenberger, M. δ18O of tree rings of beech (Fagus silvatica) as a record of δ18O of the growing season precipitation. Tellus B Chem. Phys. Meteorol. 1997, 49, 80–92. [Google Scholar] [CrossRef]
- Helle, G.; Schleser, G.H. Beyond CO2 13C/12C-fixation by Rubisco—An interpretation of C variations in tree rings from novel intra-seasonalstudies on broad-leaf trees. Plant Cell Environ. 2004, 27, 367–380. [Google Scholar] [CrossRef]
- Leavitt, S.W. Tree-ring C–H–O isotope variability and sampling. Sci. Total Environ. 2010, 408, 5244–5253. [Google Scholar] [CrossRef] [PubMed]
- Ferrio, J.; Florit, A.; Vega, A.; Serrano, L.; Voltas, J. Δ 13 C and tree-ring width reflect different drought responses in Quercus ilex and Pinus halepensis. Oecologia 2003, 137, 512–518. [Google Scholar] [CrossRef] [PubMed]
- Pumijumnong, N.; Muangsong, C.; Buajan, S.; Sano, M.; Nakatsuka, T. Climate variability over the past 100 years in Myanmar derived from tree-ring stable oxygen isotope variations in Teak. Theor. Appl. Climatol. 2020, 139, 1401–1414. [Google Scholar] [CrossRef]
- Sano, M.; Xu, C.; Nakatsuka, T. A 300-year Vietnam hydroclimate and ENSO variability record reconstructed from tree ringδ18O. J. Geophys. Res. Atmos. 2012, 117. [Google Scholar] [CrossRef]
- Preechamart, S.; Pumijumnong, N.; Payomrat, P.; Buajan, S. Variation in climate signals in teak tree-ring chronologies in two different growth areas. Forests 2018, 9, 772. [Google Scholar] [CrossRef]
- Pumijumnong, N.; Palakit, K. Effects of climate variability on the annual and intra-annual ring formation of Pinus merkusii growing in Central Thailand. Environ. Nat. Resour. J. 2020, 18, 234–248. [Google Scholar] [CrossRef]
- Ohashi, S.; Okada, N.; Nobuchi, T.; Siripatanadilok, S.; Veenin, T. Detecting invisible growth rings of trees in seasonally dry forests in Thailand: Isotopic and wood anatomical approaches. Trees 2009, 23, 813–822. [Google Scholar] [CrossRef]
- Managave, S.; Jani, R.; Rao, T.N.; Sunilkumar, K.; Satheeshkumar, S.; Ramesh, R. Intra-event isotope and raindrop size data of tropical rain reveal effects concealed by event averaged data. Clim. Dyn. 2016, 47, 981–987. [Google Scholar] [CrossRef]
- Galeano, E.; Vasconcelos, T.S.; Novais de Oliveira, P.; Carrer, H. Physiological and molecular responses to drought stress in teak (Tectona grandis Lf). PLoS ONE 2019, 14, e0221571. [Google Scholar] [CrossRef]
- Cai, B.; Pumijumnong, N.; Tan, M.; Muangsong, C.; Kong, X.; Jiang, X.; Nan, S. Effects of intraseasonal variation of summer monsoon rainfall on stable isotope and growth rate of a stalagmite from northwestern Thailand. J. Geophys. Res. Atmos. 2010, 115. [Google Scholar] [CrossRef]
- Wei, Z.; Lee, X.; Liu, Z.; Seeboonruang, U.; Koike, M.; Yoshimura, K. Influences of large-scale convection and moisture source on monthly precipitation isotope ratios observed in Thailand, Southeast Asia. Earth Planet. Sci. Lett. 2018, 488, 181–192. [Google Scholar] [CrossRef]
- Xu, C.; Sano, M.; Nakatsuka, T. A 400-year record of hydroclimate variability and local ENSO history in northern Southeast Asia inferred from tree-ring δ18O. Palaeogeogr. Palaeoclim. Palaeoecol. 2013, 386, 588–598. [Google Scholar] [CrossRef]
- Brienen, R.J.; Helle, G.; Pons, T.L.; Guyot, J.-L.; Gloor, M. Oxygen isotopes in tree rings are a good proxy for Amazon precipitation and El Niño-Southern Oscillation variability. Proc. Natl. Acad. Sci. USA 2012, 109, 16957–16962. [Google Scholar] [CrossRef]
- Scheidegger, Y.; Saurer, M.; Bahn, M.; Siegwolf, R. Linking stable oxygen and carbon isotopes with stomatal conductance and photosynthetic capacity: A conceptual model. Oecologia 2000, 125, 350–357. [Google Scholar] [CrossRef] [PubMed]
| Samples | Tree-Ring Width | Tree-Ring δ13C | Tree-Ring δ18O | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 13a | 19a | 21b | 02b | 13a | 19a | 21b | 02b | 13a | 19a | 21b | 02b | |
| TKNK3_13a | 1 | 1 | 1 | |||||||||
| TKNK3_19a | 0.459 | 1 | 0.764 | 1 | 0.708 | 1 | ||||||
| TKNK3_21b | 0.627 | 0.339 | 1 | 0.828 | 0.794 | 1 | 0.764 | 0.673 | 1 | |||
| TKNK3_02b | 0.767 | 0.265 | 0.678 | 1 | 0.836 | 0.799 | 0.845 | 1 | 0.777 | 0.701 | 0.720 | 1 |
| Chronology | δ13CTR | TRW | δ18OTR |
|---|---|---|---|
| δ13CTR | 1 | ||
| TRW | 0.060 | 1 | |
| δ18OTR | 0.400 ** | 0.022 | 1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pumijumnong, N.; Payomrat, P.; Buajan, S.; Bräuning, A.; Muangsong, C.; Chareonwong, U.; Songtrirat, P.; Palakit, K.; Liu, Y.; Li, Q. Teak Tree-Ring Cellulose δ13C, δ18O, and Tree-Ring Width from Northwestern Thailand Capture Different Aspects of Asian Monsoon Variability. Atmosphere 2021, 12, 778. https://doi.org/10.3390/atmos12060778
Pumijumnong N, Payomrat P, Buajan S, Bräuning A, Muangsong C, Chareonwong U, Songtrirat P, Palakit K, Liu Y, Li Q. Teak Tree-Ring Cellulose δ13C, δ18O, and Tree-Ring Width from Northwestern Thailand Capture Different Aspects of Asian Monsoon Variability. Atmosphere. 2021; 12(6):778. https://doi.org/10.3390/atmos12060778
Chicago/Turabian StylePumijumnong, Nathsuda, Paramate Payomrat, Supaporn Buajan, Achim Bräuning, Chotika Muangsong, Uthai Chareonwong, Piyarat Songtrirat, Kritsadapan Palakit, Yu Liu, and Qiang Li. 2021. "Teak Tree-Ring Cellulose δ13C, δ18O, and Tree-Ring Width from Northwestern Thailand Capture Different Aspects of Asian Monsoon Variability" Atmosphere 12, no. 6: 778. https://doi.org/10.3390/atmos12060778
APA StylePumijumnong, N., Payomrat, P., Buajan, S., Bräuning, A., Muangsong, C., Chareonwong, U., Songtrirat, P., Palakit, K., Liu, Y., & Li, Q. (2021). Teak Tree-Ring Cellulose δ13C, δ18O, and Tree-Ring Width from Northwestern Thailand Capture Different Aspects of Asian Monsoon Variability. Atmosphere, 12(6), 778. https://doi.org/10.3390/atmos12060778









