Atmospheric Effects on the Isotopic Composition of Ozone
Abstract
:1. Introduction
2. Pressure Dependence
3. Temperature Variation
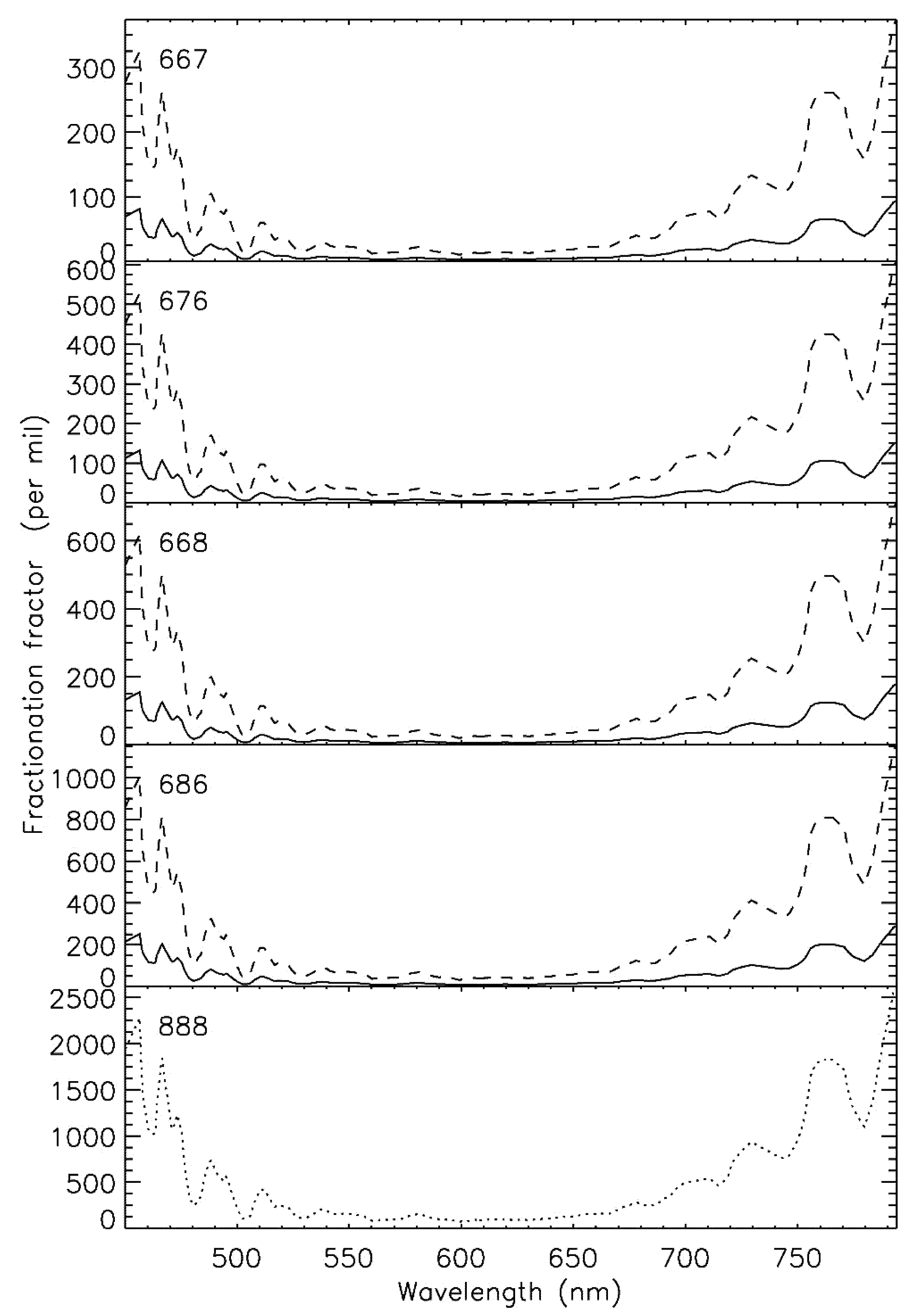
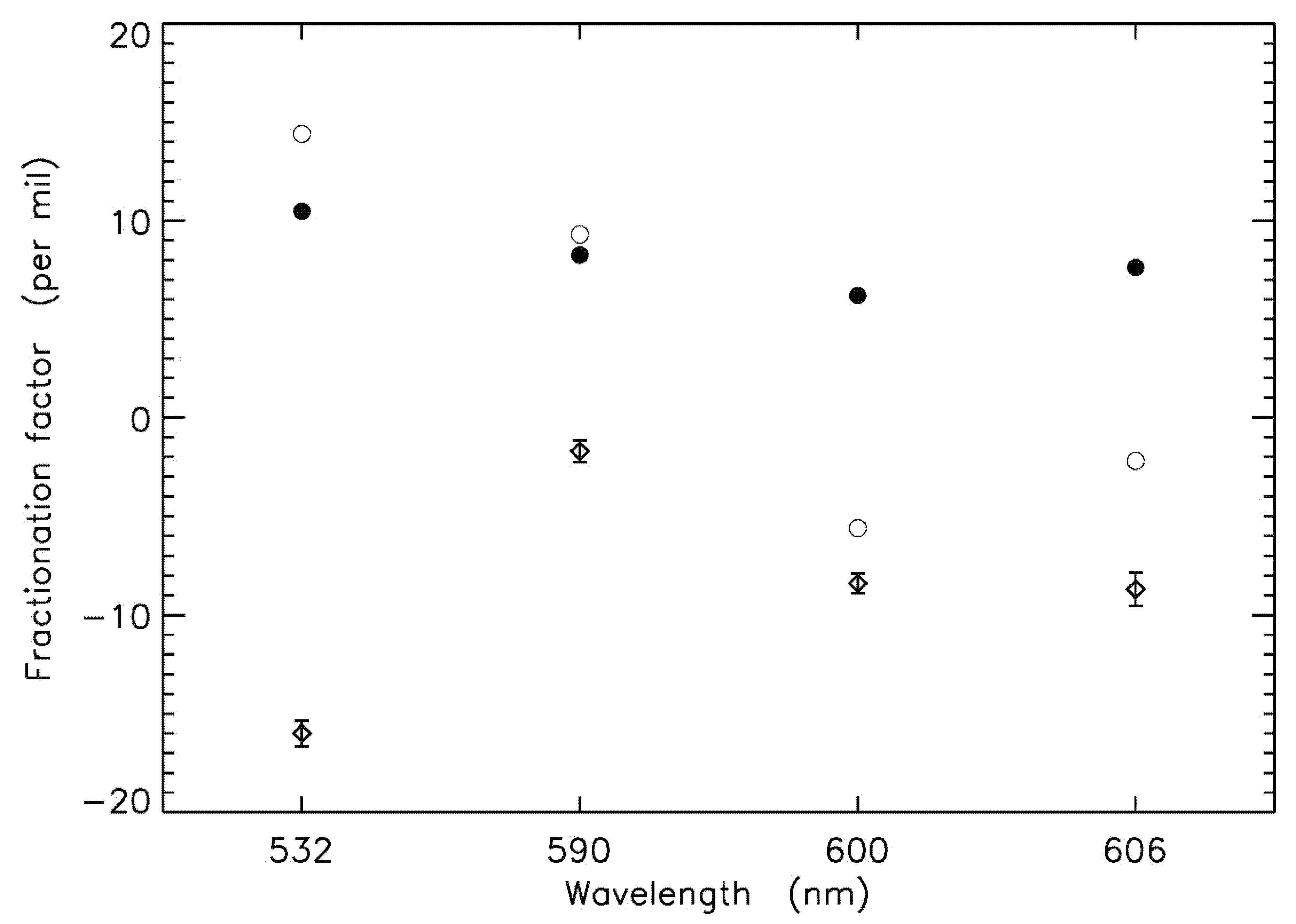
4. Chappuis Band Photolysis
5. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Irion, F.W.; Gunson, M.R.; Rinsland, C.P.; Yung, Y.L.; Abrams, M.C.; Chang, A.Y.; Goldman, A. Heavy ozone enrichments from ATMOS infrared solar spectra. Geophys. Res. Lett. 1996, 23, 2377–2380. [Google Scholar] [CrossRef] [Green Version]
- Krankowsky, D.; Bartecki, F.; Klees, G.G.; Mauersberger, K.; Schellenbach, K.; Stehr, J. Measurement of Heavy Isotope Enrichment in Tropospheric Ozone. Geophys. Res. Lett. 1995, 22, 1713–1716. [Google Scholar] [CrossRef]
- Krankowsky, D.; Lammerzahl, P.; Mauersberger, K.; Janssen, C.; Tuzson, B.; Rockmann, T. Stratospheric ozone isotope fractionations derived from collected samples. J. Geophys. Res. Atmos. 2007, 112, D08301. [Google Scholar] [CrossRef] [Green Version]
- Mauersberger, K. Measurement of Heavy Ozone in the Stratosphere. Geophys. Res. Lett. 1981, 8, 935–937. [Google Scholar] [CrossRef]
- Liang, M.C.; Irion, F.W.; Weibel, J.D.; Miller, C.E.; Blake, G.A.; Yung, Y.L. Isotopic composition of stratospheric ozone. J. Geophys. Res. Atmos. 2006, 111, D02302. [Google Scholar] [CrossRef] [Green Version]
- Liang, M.C.; Yung, Y.L. Sources of the oxygen isotopic anomaly in atmospheric N2O. J. Geophys. Res. Atmos. 2007, 112, D13307. [Google Scholar] [CrossRef] [Green Version]
- Thiemens, M.H. History and applications of mass-independent isotope effects. Annu. Rev. Earth Planet. Sci. 2006, 34, 217–262. [Google Scholar] [CrossRef] [Green Version]
- Johnston, J.C.; Thiemens, M.H. The isotopic composition of tropospheric ozone in three environments. J. Geophys. Res. Atmos. 1997, 102, 25395–25404. [Google Scholar] [CrossRef]
- Haverd, V.; Toon, G.C.; Griffith, D.W. Evidence for altitude–dependent photolysis–induced 18O isotopic fractionation in stratospheric ozone. Geophys. Res. Lett. 2005, 32, L22808. [Google Scholar] [CrossRef] [Green Version]
- Jonkheid, B.; Röckmann, T.; Glatthor, N.; Janssen, C.; Stiller, G.; Clarmann, T.V. Retrievals of heavy ozone with MIPAS. Atmos. Meas. Tech. 2016, 9, 6069–6079. [Google Scholar] [CrossRef]
- Kasai, Y.J.; Urban, J.; Takahashi, C.; Hoshino, S.; Takahashi, K.; Inatani, J.; Shiotani, M.; Masuko, H. Stratospheric ozone isotope enrichment studied by submillimeter wave heterodyne radiometry: The observation capabilities of SMILES. IEEE Trans. Geosci. Remote Sens. 2006, 44, 676–693. [Google Scholar] [CrossRef]
- Fernando, A.M.; Bernath, P.F.; Boone, C.D. Ozone isotopologue measurements from the Atmospheric Chemistry Experiment (ACE). J. Quant. Spectrosc. Radiat. Transf. 2019, 238, 106547. [Google Scholar] [CrossRef]
- Bao, H.; Thiemens, M.H.; Farquhar, J.; Campbell, D.A.; Lee, C.C.; Heine, K.; Loope, D.B. Anomalous 17O compositions in massive sulphate deposits on the Earth. Nature 2000, 406, 176–178. [Google Scholar] [CrossRef]
- Boering, K.A.; Jackson, T.; Hoag, K.J.; Cole, A.S.; Perri, M.J.; Thiemens, M.; Atlas, E. Observations of the anomalous oxygen isotopic composition of carbon dioxide in the lower stratosphere and the flux of the anomaly to the troposphere. Geophys. Res. Lett. 2004, 31, L03109. [Google Scholar] [CrossRef] [Green Version]
- Cliff, S.S.; Thiemens, M.H. The 18O/16O and 17O/16O ratios in atmospheric nitrous oxide: A mass-independent anomaly. Science 1997, 278, 1774–1776. [Google Scholar] [CrossRef]
- Lammerzahl, P.; Rockmann, T.; Brenninkmeijer, C.A.M.; Krankowsky, D.; Mauersberger, K. Oxygen isotope composition of stratospheric carbon dioxide. Geophys. Res. Lett. 2002, 29, 1582. [Google Scholar] [CrossRef]
- Liang, M.C.; Blake, G.A.; Lewis, B.R.; Yung, Y.L. Oxygen isotopic composition of carbon dioxide in the middle atmosphere. Proc. Natl. Acad. Sci. USA 2007, 104, 21–25. [Google Scholar] [CrossRef] [Green Version]
- Michalski, G.; Scott, Z.; Kabiling, M.; Thiemens, M.H. First measurements and modeling of Delta O-17 in atmospheric nitrate. Geophys. Res. Lett. 2003, 30, 1870. [Google Scholar] [CrossRef]
- Morin, S.; Savarino, J.; Frey, M.M.; Yan, N.; Bekki, S.; Bottenheim, J.W.; Martins, J.M. Tracing the origin and fate of NOx in the Arctic atmosphere using stable isotopes in nitrate. Science 2008, 322, 730–732. [Google Scholar] [CrossRef]
- Thiemens, M.H.; Jackson, T.; Zipf, E.C.; Erdman, P.W.; Vanegmond, C. Carbon-Dioxide and Oxygen-Isotope Anomalies in the Mesosphere and Stratosphere. Science 1995, 270, 969–972. [Google Scholar] [CrossRef]
- Lyons, J.R. Transfer of mass-independent fractionation in ozone to other oxygen-containing radicals in the atmosphere. Geophys. Res. Lett. 2001, 28, 3231–3234. [Google Scholar] [CrossRef]
- Liang, M.C.; Blake, G.A.; Yung, Y.L. Seasonal cycle of C16O16O, C16O17O, and C16O18O in the middle atmosphere: Implications for mesospheric dynamics and biogeochemical sources and sinks of CO2. J. Geophys. Res. Atmos. 2008, 113, D12305. [Google Scholar] [CrossRef] [Green Version]
- Wiegel, A.A.; Cole, A.S.; Hoag, K.J.; Atlas, E.L.; Schauffler, S.M.; Boering, K.A. Unexpected variations in the triple oxygen isotope composition of stratospheric carbon dioxide. Proc. Natl. Acad. Sci. USA 2013, 110, 17680–17685. [Google Scholar] [CrossRef] [Green Version]
- Liang, M.C.; Mahata, S.; Laskar, A.H.; Thiemens, M.H.; Newman, S. Oxygen isotope anomaly in tropospheric CO2 and implications for CO2 residence time in the atmosphere and gross primary productivity. Sci. Rep. 2017, 7, 13180. [Google Scholar] [CrossRef]
- Koren, G.; Schneider, L.; van der Velde, I.R.; van Schaik, E.; Gromov, S.S.; Adnew, G.A.; Mrozek, D.J.; Hofmann, M.E.; Liang, M.C.; Mahata, S. Global 3D Simulations of the Triple Oxygen Isotope Signature Δ17O in Atmospheric CO2. J. Geophys. Res. Atmos. 2018, 145, 8808–8836. [Google Scholar]
- Guha, T.; Lin, C.T.; Bhattacharya, S.K.; Mahajan, A.S.; Ou-Yang, C.F.; Lan, Y.P.; Hsu, S.C.; Liang, M.C. Isotopic ratios of nitrate in aerosol samples from Mt. Lulin, a high-altitude station in Central Taiwan. Atmos. Environ. 2017, 154, 53–69. [Google Scholar] [CrossRef]
- Liang, M.C.; Blake, G.A.; Yung, Y.L. A semianalytic model for photo-induced isotopic fractionation in simple molecules. J. Geophys. Res. Atmos. 2004, 109, D10308. [Google Scholar] [CrossRef]
- Blake, G.A.; Liang, M.C.; Morgan, C.G.; Yung, Y.L. A Born-Oppenheimer photolysis model of N2O fractionation. Geophys. Res. Lett. 2003, 30, 1656. [Google Scholar] [CrossRef] [Green Version]
- Yung, Y.L.; Miller, C.E. Isotopic fractionation of stratospheric nitrous oxide. Science 1997, 278, 1778–1780. [Google Scholar] [CrossRef]
- Früchtl, M.; Janssen, C.; Röckmann, T. Experimental study on isotope fractionation effects in visible photolysis of O3 and in the O+O3 odd oxygen sink reaction. J. Geophys. Res. Atmos. 2015, 120, 4398–4416. [Google Scholar] [CrossRef]
- Früchtl, M.; Janssen, C.; Taraborrelli, D.; Gromov, S.; Röckmann, T. Wavelength–dependent isotope fractionation in visible light O3 photolysis and atmospheric implications. Geophys. Res. Lett. 2015, 42, 8711–8718. [Google Scholar] [CrossRef]
- Huang, C.H.; Bhattacharya, S.K.; Hsieh, Z.M.; Chen, Y.J.; Yih, T.S.; Liang, M.C. Isotopic Fractionation in Photolysis of Ozone in the Hartley and Chappuis Bands. Earth Space Sci. 2019, 6, 752–773. [Google Scholar] [CrossRef] [Green Version]
- Guenther, J.; Erbacher, B.; Krankowsky, D.; Mauersberger, K. Pressure dependence of two relative ozone formation rate coefficients. Chem. Phys. Lett. 1999, 306, 209–213. [Google Scholar] [CrossRef]
- Gao, Y.Q.; Marcus, R.A. An approximate theory of the ozone isotopic effects: Rate constant ratios and pressure dependence. J. Chem. Phys. 2007, 127, 244316. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gao, Y.Q.; Marcus, R.A. Strange and unconventional isotope effects in ozone formation. Science 2001, 293, 259–263. [Google Scholar] [CrossRef] [Green Version]
- Gao, Y.Q.; Marcus, R.A. On the theory of the strange and unconventional isotopic effects in ozone formation. J. Chem. Phys. 2002, 116, 137–154. [Google Scholar] [CrossRef] [Green Version]
- Marcus, R.A.; Gao, Y.Q. Pressure effects on bimolecular recombination and unimolecular dissociation reactions. J. Chem. Phys. 2001, 114, 9807–9812. [Google Scholar] [CrossRef] [Green Version]
- Kaiser, J.; Rockmann, T.; Brenninkmeijer, C.A.M. Temperature dependence of isotope fractionation in N2O photolysis. Phys. Chem. Chem. Phys. 2002, 4, 4420–4430. [Google Scholar] [CrossRef]
- Morton, J.; Barnes, J.; Schueler, B.; Mauersberger, K. Laboratory Studies of Heavy Ozone. J. Geophys. Res. Atmos. 1990, 95, 901–907. [Google Scholar] [CrossRef]
- Kistler, R.; Kalnay, E.; Collins, W.; Saha, S.; White, G.; Woollen, J.; Chelliah, M.; Ebisuzaki, W.; Kanamitsu, M.; Kousky, V.; et al. The NCEP-NCAR 50-year reanalysis: Monthly means CD-ROM and documentation. Bull. Am. Meteorol. Soc. 2001, 82, 247–267. [Google Scholar] [CrossRef]
- Miller, C.E.; Onorato, R.M.; Liang, M.C.; Yung, Y.L. Extraordinary isotopic fractionation in ozone photolysis. Geophys. Res. Lett. 2005, 32, L14814. [Google Scholar] [CrossRef] [Green Version]
- Anderson, S.M.; Maeder, J.; Mauersberger, K. Effect of Isotopic-Substitution on the Visible Absorption-Spectrum of Ozone. J. Chem. Phys. 1991, 94, 6351–6357. [Google Scholar] [CrossRef]
- Anderson, S.M.; Mauersberger, K. Ozone Absorption-Spectroscopy in Search of Low-Lying Electronic States. J. Geophys. Res. Atmos. 1995, 100, 3033–3048. [Google Scholar] [CrossRef]
- Wen, J.; Thiemens, M.H. Experimental and Theoretical-Study of Isotope Effects on Ozone Decomposition. J. Geophys. Res. Atmos. 1991, 96, 10911–10921. [Google Scholar] [CrossRef]
- Chakraborty, S.; Chakraborty, S. Isotopic fractionation of the O3-nitric oxide reaction. Curr. Sci. 2003, 85, 1210–1212. [Google Scholar]
- Liao, H.; Yung, Y.L.; Seinfeld, J.H. Effects of aerosols on tropospheric photolysis rates in clear and cloudy atmospheres. J. Geophys. Res. Atmos. 1999, 104, 23697–23707. [Google Scholar] [CrossRef] [Green Version]
- Stamnes, K.; Tsay, S.C.; Wiscombe, W.; Jayaweera, K. Numerically stable algorithm for discrete-ordinate-method radiative transfer in multiple scattering and emitting layered media. Appl. Opt. 1988, 27, 2502–2509. [Google Scholar] [CrossRef]
- Berhanu, T.A.; Savarino, J.; Bhattacharya, S.K.; Vicars, W.C. 17O excess transfer during the NO2 + O3 --> NO3 + O2 reaction. J. Chem. Phys. 2012, 136, 044311. [Google Scholar] [CrossRef]
- Liu, S.C.; Kley, D.; Mcfarland, M.; Mahlman, J.D.; Levy, H. On the Origin of Tropospheric Ozone. J. Geophys. Res. Ocean. 1980, 85, 7546–7552. [Google Scholar] [CrossRef]
- Liu, S.C.; Trainer, M.; Fehsenfeld, F.C.; Parrish, D.D.; Williams, E.J.; Fahey, D.W.; Hubler, G.; Murphy, P.C. Ozone Production in the Rural Troposphere and the Implications for Regional and Global Ozone Distributions. J. Geophys. Res. Atmos. 1987, 92, 4191–4207. [Google Scholar] [CrossRef]
- Bao, H.; Lyons, J.R.; Zhou, C. Triple oxygen isotope evidence for elevated CO2 levels after a Neoproterozoic glaciation. Nature 2008, 453, 504–506. [Google Scholar] [CrossRef]
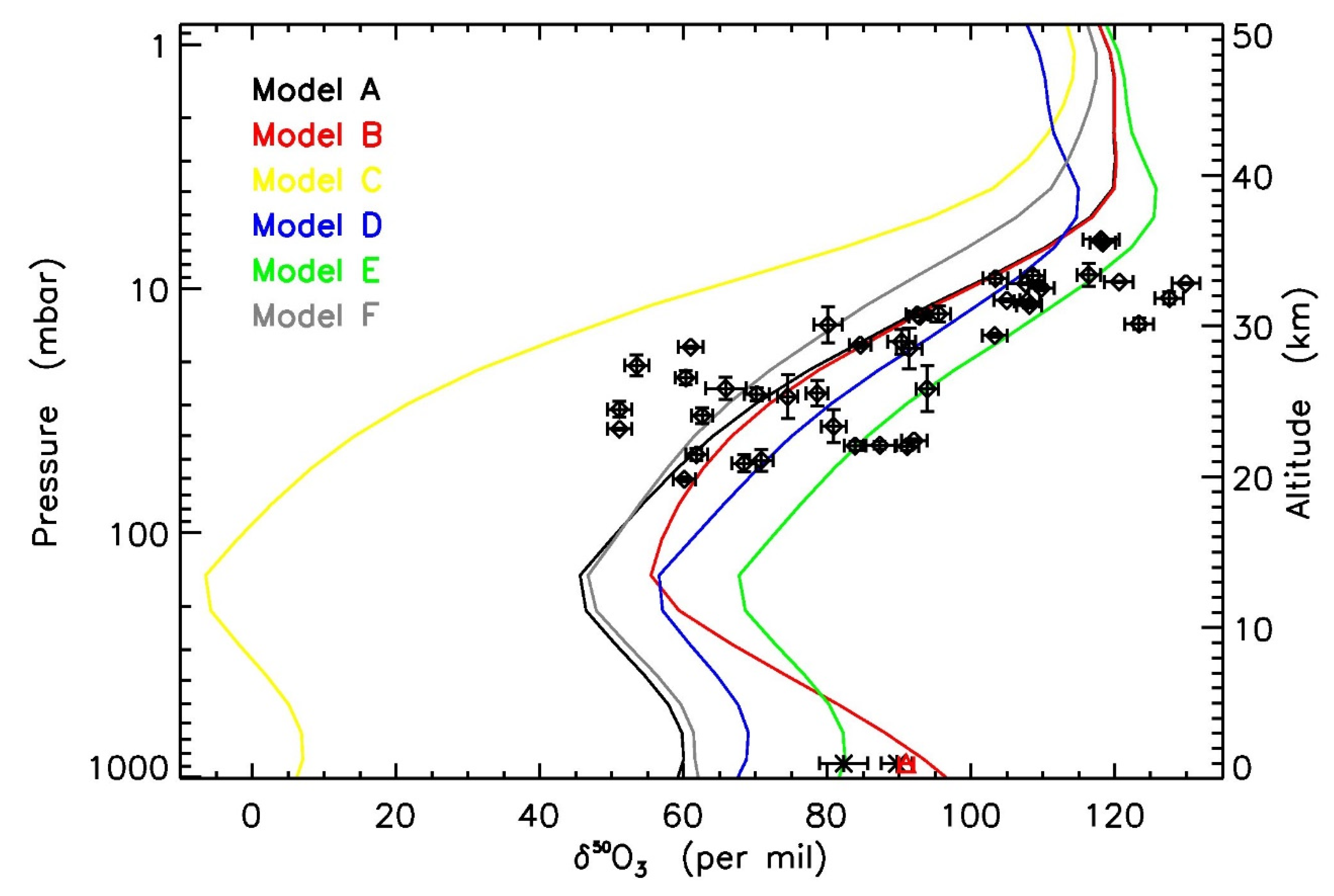
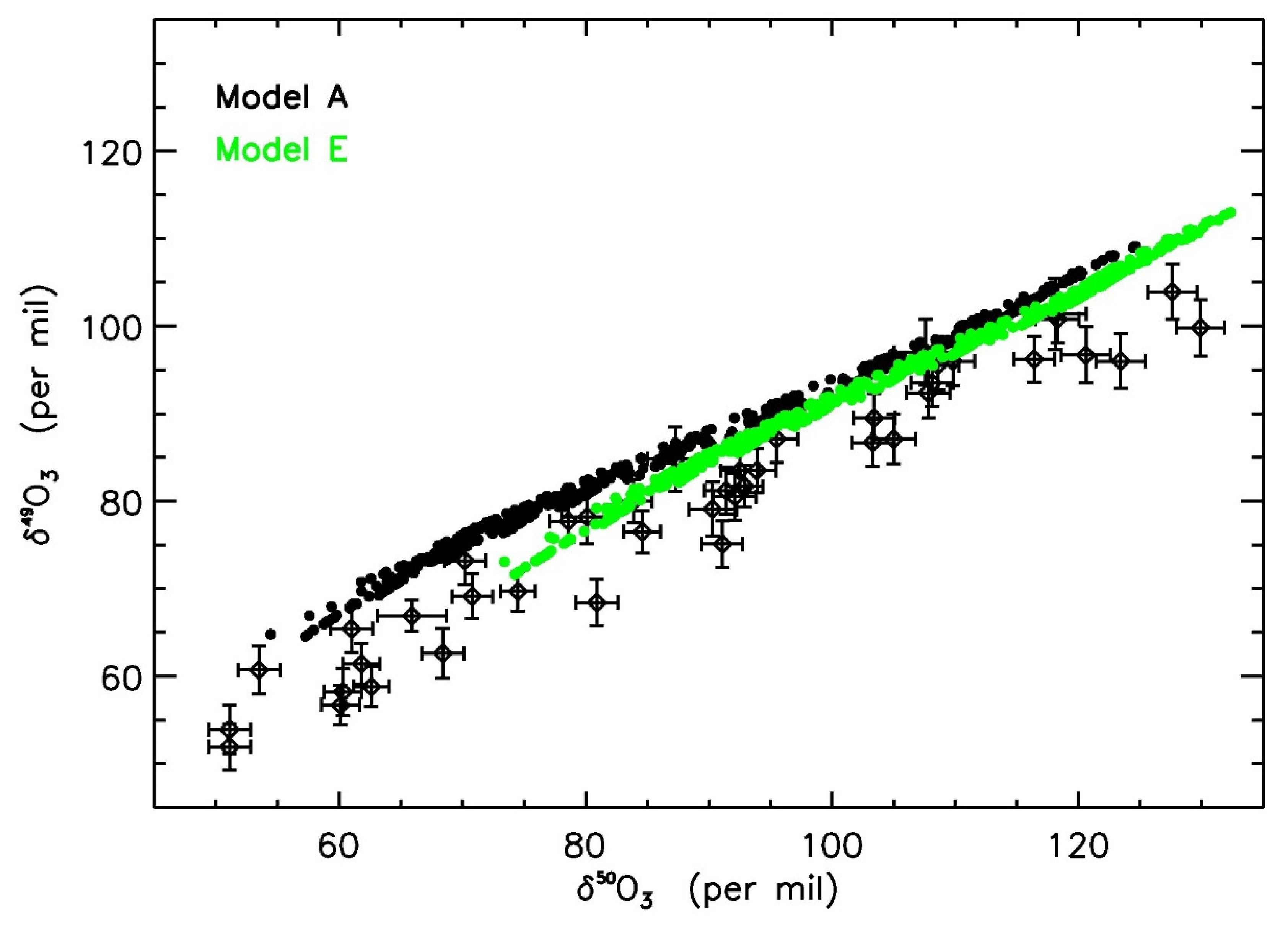
| Temperature | Pressure | Photolysis | Cloud | |
|---|---|---|---|---|
| Model A | Nominal | Y | Modified | N |
| Model B | Nominal | N | Modified | N |
| Model C | Nominal | Y | Scaled | N |
| Model D | Reduced | Y | ZPE | N |
| Model E | Nominal | Y | ZPE | N |
| Model F | Nominal | Y | Modified | Y |
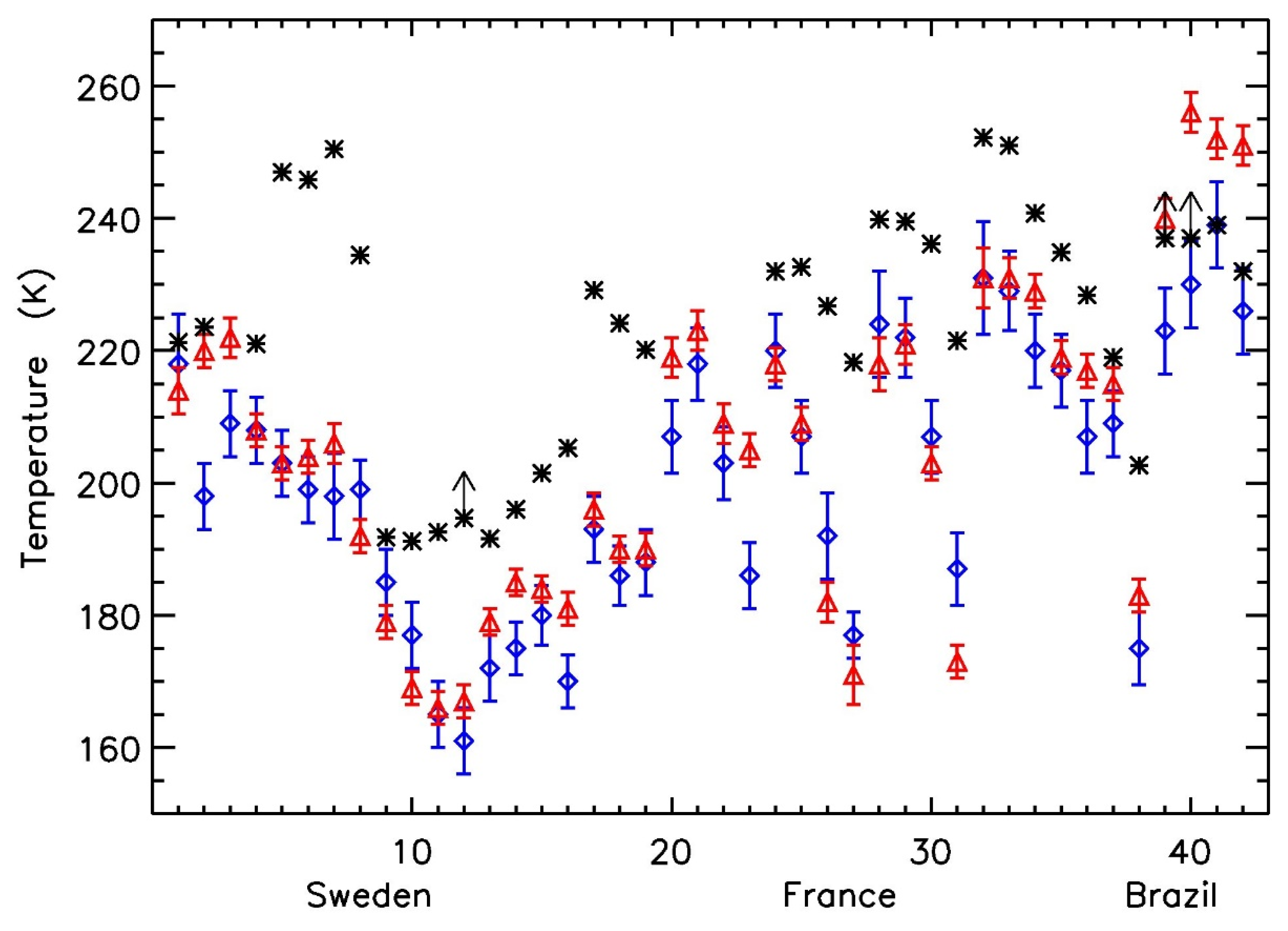
| Number | Date | Height | T(δ49O3) | T(δ50O3) | Tclim | Tin situ | TNCEP |
|---|---|---|---|---|---|---|---|
| Sweden | |||||||
| 1 | 10 May 1998 | 22.1 | 218 ± 15 | 214 ± 7 | 218 | 221.3 | 222 |
| 2 | 10 May 1998 | 22 | 198 ± 10 | 220 ± 5 | 218 | 223.59 | 222 |
| 3 | 10 May 1998 | 22.4 | 209 ± 10 | 222 ± 6 | 218 | N/A | 222.1 |
| 4 | 10 May 1998 | 22.1 | 208 ± 10 | 208 ± 5 | 218 | 221.08 | 222 |
| 5 | 3 May 1999 | 30.8 | 203 ± 10 | 203 ± 5 | 229 | 247 | 230 |
| 6 | 3 May 1999 | 30.6 | 199 ± 10 | 204 ± 5 | 229 | 245.85 | 229.8 |
| 7 | 3 May 1999 | 29 | 198 ± 13 | 206 ± 6 | 226 | 250.5 | 226.5 |
| 8 | 3 May 1999 | 25.5 | 199 ± 9 | 192 ± 5 | 221 | 234.45 | 220.7 |
| 9 | 3 December 2001 | 28.6 | 185 ± 10 | 179 ± 5 | 201 | 191.8 | 205.8 |
| 10 | 3 December 2001 | 27.4 | 177 ± 10 | 169 ± 5 | 201 | 191.2 | 205.7 |
| 11 | 3 December 2001 | 24.5 | 165 ± 10 | 166 ± 5 | 201 | 192.55 | 206.3 |
| 12 | 3 December 2001 | 23.2 | 161 ± 10 | 167 ± 5 | 202 | >194.7 | 207 |
| 13 | 28 November 2002 | 26.6 | 172 ± 10 | 179 ± 4 | 198 | 191.6 | 192.4 |
| 14 | 28 November 2002 | 24.1 | 175 ± 8 | 185 ± 4 | 199 | 196 | 195 |
| 15 | 28 November 2002 | 21.5 | 180 ± 9 | 184 ± 4 | 204 | 201.5 | 199.7 |
| 16 | 28 November 2002 | 19.8 | 170 ± 8 | 181 ± 5 | 208 | 205.3 | 203.2 |
| France | |||||||
| 17 | 3 October 1998 | 28.7 | 193 ± 10 | 196 ± 5 | 222 | 229.15 | 223.1 |
| 18 | 3 October 1998 | 25.3 | 186 ± 9 | 190 ± 4 | 216 | 224.15 | 218.7 |
| 19 | 3 October 1998 | 21.1 | 188 ± 10 | 190 ± 5 | 214 | 220.15 | 215.2 |
| 20 | 11 October 1999 | 31.7 | 207 ± 11 | 219 ± 6 | 223 | N/A | 225.4 |
| 21 | 11 October 1999 | 31.6 | 218 ± 11 | 223 ± 6 | 223 | N/A | 225.3 |
| 22 | 11 October 1999 | 28.5 | 203 ± 11 | 209 ± 6 | 221 | N/A | 219.9 |
| 23 | 11 October 1999 | 23.4 | 186 ± 10 | 205 ± 5 | 216 | N/A | 212.8 |
| 24 | 4 October 2000 | 33.4 | 220 ± 11 | 218 ± 5 | 226 | 232 | 230.4 |
| 25 | 4 October 2000 | 33.1 | 207 ± 11 | 209 ± 5 | 226 | 232.63 | 230 |
| 26 | 4 October 2000 | 30.1 | 192 ± 13 | 182 ± 6 | 222 | 226.73 | 223.7 |
| 27 | 4 October 2000 | 25.9 | 177 ± 7 | 171 ± 9 | 217 | 218.25 | 215.8 |
| 28 | 11 May 2001 | 32.8 | 224 ± 16 | 218 ± 8 | 231 | 239.86 | 238.7 |
| 29 | 11 May 2001 | 32.5 | 222 ± 12 | 221 ± 6 | 231 | 239.55 | 238 |
| 30 | 11 May 2001 | 30.8 | 207 ± 11 | 203 ± 5 | 229 | 236.15 | 233.5 |
| 31 | 11 May 2001 | 25.5 | 187 ± 11 | 173 ± 5 | 220 | 221.5 | 220.4 |
| 32 | 25 April 2002 | 35.8 | 231 ± 17 | 231 ± 9 | 233 | 252.25 | 242.8 |
| 33 | 25 April 2002 | 35.5 | 229 ± 12 | 231 ± 6 | 233 | 251 | 242.2 |
| 34 | 25 April 2002 | 33.4 | 220 ± 11 | 229 ± 5 | 233 | 240.81 | 237.2 |
| 35 | 25 April 2002 | 31.4 | 217 ± 11 | 219 ± 5 | 230 | 234.89 | 232.4 |
| Brazil | |||||||
| 36 | 29–30 November 2004 | 29.4 | 207 ± 11 | 217 ± 5 | 224 | 228.4 | 227.5 |
| 37 | 29–30 November 2004 | 25.8 | 209 ± 10 | 215 ± 5 | 216 | 219 | 220 |
| 38 | 29–30 November 2004 | 20.9 | 175 ± 11 | 183 ± 5 | 207 | 202.7 | 206.6 |
| 39 | 4 June 2005 | 32.9 | 223 ± 13 | 240 ± 6 | 230 | >237 | 232.4 |
| 40 | 4 June 2005 | 32.8 | 230 ± 13 | 256 ± 6 | 230 | >237 | 232.3 |
| 41 | 4 June 2005 | 31.9 | 239 ± 13 | 252 ± 6 | 229 | 239 | 230.8 |
| 42 | 4 June 2005 | 30.1 | 226 ± 13 | 251 ± 6 | 227 | 232 | 228.2 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liang, M.-C.; Chen, Y.-C.; Gao, Y.-Q.; Zhang, X.; Yung, Y.L. Atmospheric Effects on the Isotopic Composition of Ozone. Atmosphere 2021, 12, 1673. https://doi.org/10.3390/atmos12121673
Liang M-C, Chen Y-C, Gao Y-Q, Zhang X, Yung YL. Atmospheric Effects on the Isotopic Composition of Ozone. Atmosphere. 2021; 12(12):1673. https://doi.org/10.3390/atmos12121673
Chicago/Turabian StyleLiang, Mao-Chang, Yi-Chun Chen, Yi-Qin Gao, Xi Zhang, and Yuk L. Yung. 2021. "Atmospheric Effects on the Isotopic Composition of Ozone" Atmosphere 12, no. 12: 1673. https://doi.org/10.3390/atmos12121673
APA StyleLiang, M.-C., Chen, Y.-C., Gao, Y.-Q., Zhang, X., & Yung, Y. L. (2021). Atmospheric Effects on the Isotopic Composition of Ozone. Atmosphere, 12(12), 1673. https://doi.org/10.3390/atmos12121673







