Gravity Wave Investigations over Comandante Ferraz Antarctic Station in 2017: General Characteristics, Wind Filtering and Case Study
Abstract
1. Introduction
2. Instrumentation and Methodology
2.1. Airglow All-Sky Imager and Data Analysis
2.2. Meteor Radars and Wind Models
2.3. Blocking Diagram
3. Results and Discussions
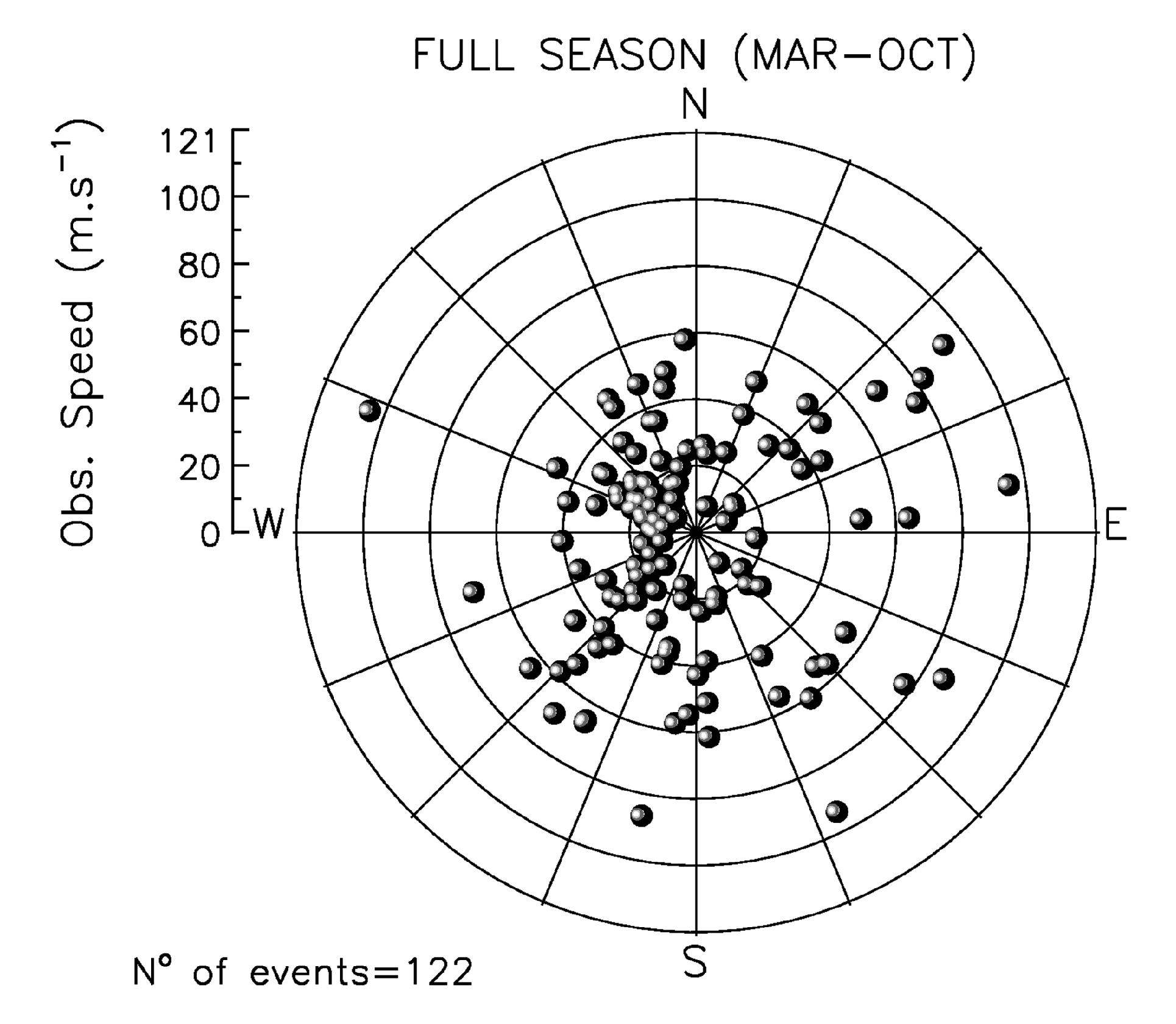
3.1. Airglow Observations
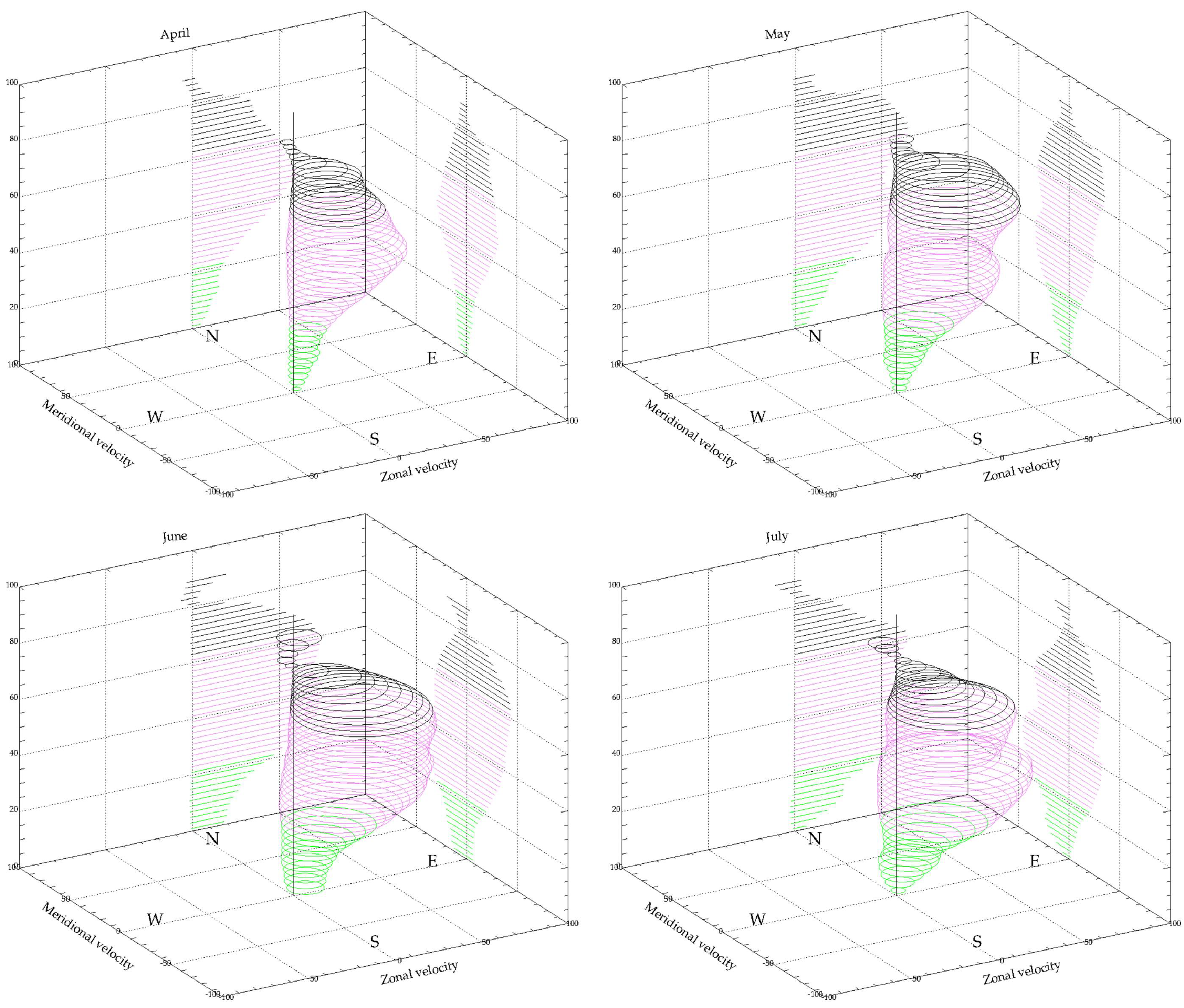
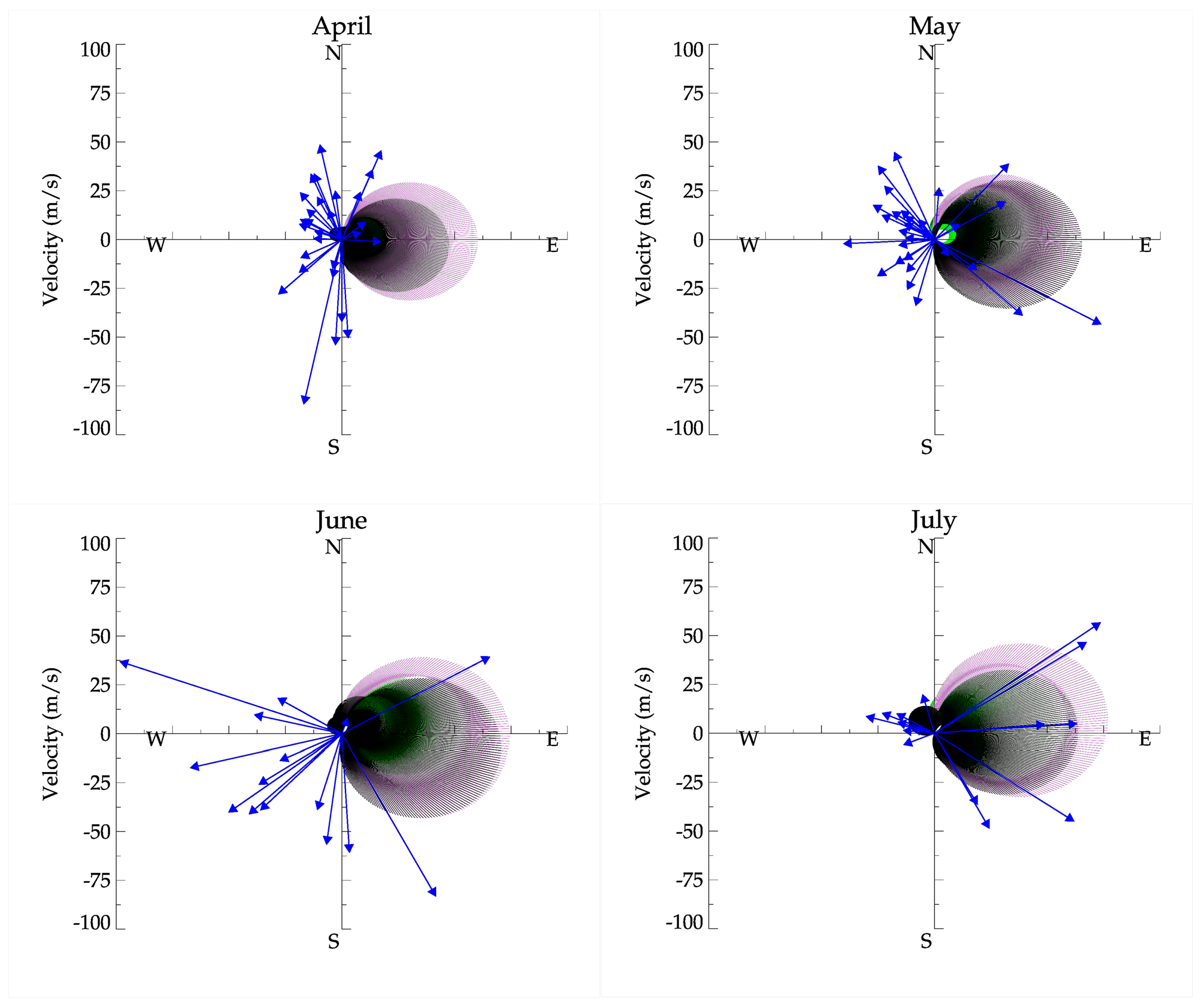
3.2. Blocking Diagrams
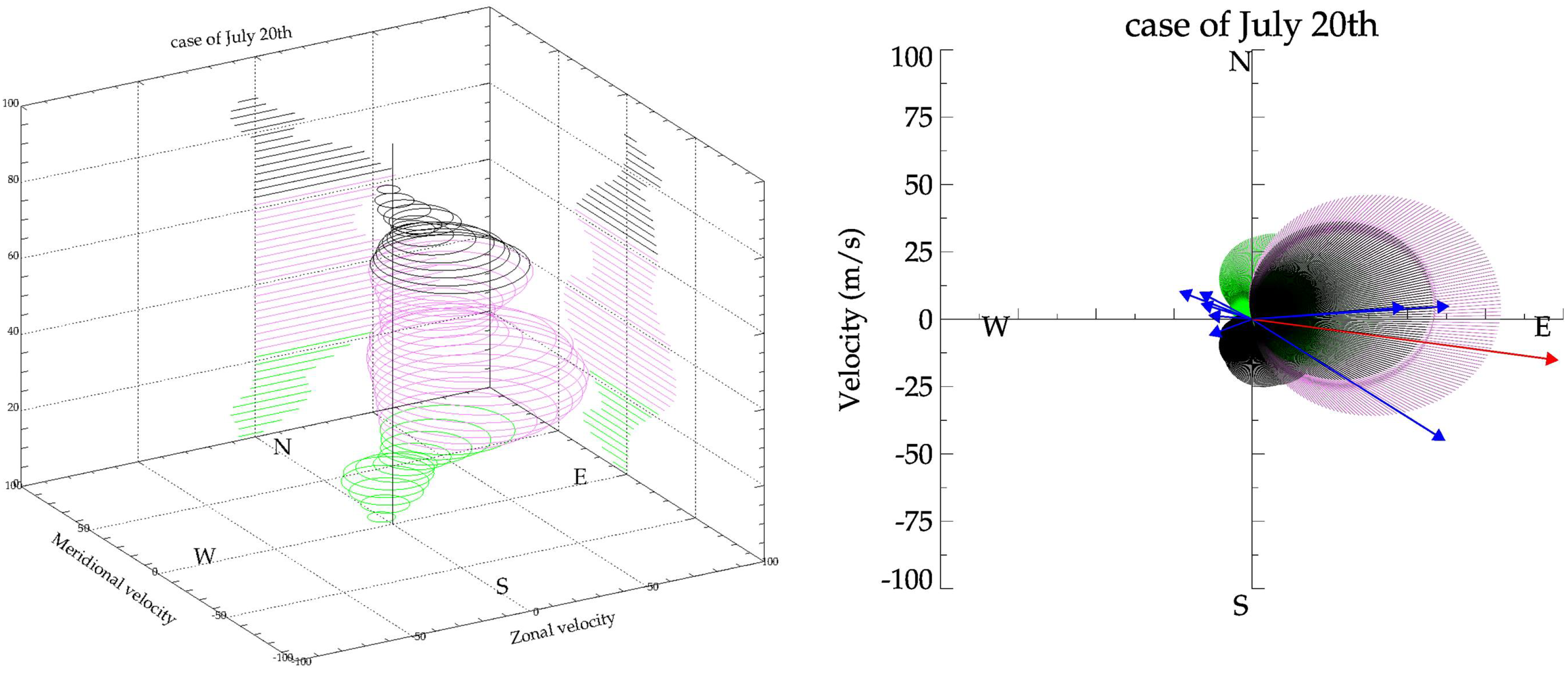
4. Case Study of July 20–21
4.1. Blocking Diagram Analysis
4.2. Reverse Ray Tracing
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Nappo, C. An Introduction to Atmospheric Gravity Waves; International Geophysics; Elsevier Science: San Diego, CA, USA, 2002. [Google Scholar]
- Holton, J.R.; Alexander, M.J. The Role of Waves in the Transport Circulation of the Middle Atmosphere. In Atmospheric Science Across the Stratopause; American Geophysical Union (AGU): Washington, DC, USA, 2013; pp. 21–35. [Google Scholar] [CrossRef]
- Fritts, D.C.; Alexander, M.J. Gravity wave dynamics and effects in the middle atmosphere. Rev. Geophys. 2003, 41. [Google Scholar] [CrossRef]
- Krisch, I.; Preusse, P.; Ungermann, J.; Dörnbrack, A.; Eckermann, S.D.; Ern, M.; Friedl-Vallon, F.; Kaufmann, M.; Oelhaf, H.; Rapp, M.; et al. First tomographic observations of gravity waves by the infrared limb imager GLORIA. Atmos. Chem. Phys. 2017, 17, 14937–14953. [Google Scholar] [CrossRef]
- Pautet, P.D.; Taylor, M.J.; Eckermann, S.D.; Criddle, N. Regional Distribution of Mesospheric Small-Scale Gravity Waves During DEEPWAVE. J. Geophys. Res. Atmos. 2019, 124, 7069–7081. [Google Scholar] [CrossRef]
- Matsuda, T.S.; Nakamura, T.; Ejiri, M.K.; Tsutsumi, M.; Tomikawa, Y.; Taylor, M.J.; Zhao, Y.; Pautet, P.D.; Murphy, D.J.; Moffat-Griffin, T. Characteristics of mesospheric gravity waves over Antarctica observed by Antarctic Gravity Wave Instrument Network imagers using 3-D spectral analyses. J. Geophys. Res. Atmos. 2017, 122, 8969–8981. [Google Scholar] [CrossRef]
- Suzuki, S.; Shiokawa, K.; Otsuka, Y.; Ogawa, T.; Nakamura, K.; Nakamura, T. A concentric gravity wave structure in the mesospheric airglow images. J. Geophys. Res. Atmos. 2007, 112. [Google Scholar] [CrossRef]
- Lai, C.; Yue, J.; Xu, J.; Straka, W.C.; Miller, S.D.; Liu, X. Suomi NPP VIIRS/DNB imagery of nightglow gravity waves from various sources over China. Adv. Space Res. 2017, 59, 1951–1961. [Google Scholar] [CrossRef]
- Demissie, T.D.; Espy, P.J.; Kleinknecht, N.H.; Hatlen, M.; Kaifler, N.; Baumgarten, G. Characteristics and sources of gravity waves observed in noctilucent cloud over Norway. Atmos. Chem. Phys. 2014, 14, 12133–12142. [Google Scholar] [CrossRef]
- Kim, Y.; Eckermann, S.D.; Chun, H. An overview of the past, present and future of gravity-wave drag parametrization for numerical climate and weather prediction models. Atmos. -Ocean 2003, 41, 65–98. [Google Scholar] [CrossRef]
- Medvedev, A.S.; Yiğit, E. Gravity Waves in Planetary Atmospheres: Their Effects and Parameterization in Global Circulation Models. Atmosphere 2019, 10, 531. [Google Scholar] [CrossRef]
- Xu, X.; Xue, M.; Teixeira, M.A.C.; Tang, J.; Wang, Y. Parameterization of Directional Absorption of Orographic Gravity Waves and Its Impact on the Atmospheric General Circulation Simulated by the Weather Research and Forecasting Model. J. Atmos. Sci. 2019, 76, 3435–3453. [Google Scholar] [CrossRef]
- Hindley, N.P.; Wright, C.J.; Smith, N.D.; Mitchell, N.J. The southern stratospheric gravity wave hot spot: Individual waves and their momentum fluxes measured by COSMIC GPS-RO. Atmos. Chem. Phys. 2015, 15, 7797–7818. [Google Scholar] [CrossRef]
- Bageston, J.V.; Wrasse, C.M.; Gobbi, D.; Takahashi, H.; Souza, P.B. Observation of mesospheric gravity waves at Comandante Ferraz Antarctica Station (62° S). Ann. Geophys. 2009, 27, 2593–2598. [Google Scholar] [CrossRef]
- Nielsen, K.; Taylor, M.; Hibbins, R.; Jarvis, M. Climatology of short-period mesospheric gravity waves over Halley, Antarctica (76° S, 27° W). J. Atmos. Sol. -Terr. Phys. 2009, 71, 991–1000. [Google Scholar] [CrossRef]
- Moffat-Griffin, T.; Hibbins, R.E.; Jarvis, M.J.; Colwell, S.R. Seasonal variations of gravity wave activity in the lower stratosphere over an Antarctic Peninsula station. J. Geophys. Res. Atmos. 2011, 116. [Google Scholar] [CrossRef]
- de la Torre, A.; Alexander, P.; Hierro, R.; Llamedo, P.; Rolla, A.; Schmidt, T.; Wickert, J. Large-amplitude gravity waves above the southern Andes, the Drake Passage, and the Antarctic Peninsula. J. Geophys. Res. Atmos. 2012, 117. [Google Scholar] [CrossRef]
- Fritts, D.C.; Janches, D.; Iimura, H.; Hocking, W.K.; Bageston, J.V.; Leme, N.M.P. Drake Antarctic Agile Meteor Radar first results: Configuration and comparison of mean and tidal wind and gravity wave momentum flux measurements with Southern Argentina Agile Meteor Radar. J. Geophys. Res. Atmos. 2012, 117. [Google Scholar] [CrossRef]
- Baumgarten, K.; Gerding, M.; Baumgarten, G.; Lübken, F.J. Temporal variability of tidal and gravity waves during a record long 10-day continuous lidar sounding. Atmos. Chem. Phys. 2018, 18, 371–384. [Google Scholar] [CrossRef]
- Beldon, C.L.; Mitchell, N.J. Gravity wave–tidal interactions in the mesosphere and lower thermosphere over Rothera, Antarctica (68° S, 68° W). J. Geophys. Res. Atmos. 2010, 115. [Google Scholar] [CrossRef]
- Suzuki, S.; Nakamura, T.; Ejiri, M.K.; Tsutsumi, M.; Shiokawa, K.; Kawahara, T.D. Simultaneous airglow, lidar, and radar measurements of mesospheric gravity waves over Japan. J. Geophys. Res. Atmos. 2010, 115. [Google Scholar] [CrossRef]
- Vincent, R.A.; Kovalam, S.; Reid, I.M.; Younger, J.P. Gravity wave flux retrievals using meteor radars. Geophys. Res. Lett. 2010, 37. [Google Scholar] [CrossRef]
- Nyassor, P.K.; Buriti, R.A.; Paulino, I.; Medeiros, A.F.; Takahashi, H.; Wrasse, C.M.; Gobbi, D. Determination of gravity wave parameters in the airglow combining photometer and imager data. Ann. Geophys. 2018, 36, 705–715. [Google Scholar] [CrossRef]
- Garcia, F.J.; Taylor, M.J.; Kelley, M.C. Two-dimensional spectral analysis of mesospheric airglow image data. Appl. Opt. 1997, 36, 7374–7385. [Google Scholar] [CrossRef] [PubMed]
- Bageston, J.V.; Wrasse, C.M.; Batista, P.P.; Hibbins, R.E.; C Fritts, D.; Gobbi, D.; Andrioli, V.F. Observation of a mesospheric front in a thermal-doppler duct over King George Island, Antarctica. Atmos. Chem. Phys. 2011, 11, 12137–12147. [Google Scholar] [CrossRef]
- Wrasse, C.M.; Takahashi, H.; de Medeiros, A.F.; e Michael John Taylor, L.M.L.; Gobbi, D.; Fechine, J. Determinação dos parâmetros de ondas de gravidade através da análise espectral de imagens de aeroluminescência. Braz. J. Geophys. 2007, 25. [Google Scholar] [CrossRef][Green Version]
- Bevington, P.; Robinson, D. Data Reduction and Error Analysis for the Physical Sciences; McGraw-Hill Higher Education, McGraw-Hill Education: New York, NY, USA, 2002. [Google Scholar]
- Jee, G.; Kim, J.H.; Lee, C.; Kim, Y. Ground-based Observations for the Upper Atmosphere at King Sejong Station, Antarctica. J. Astron. Space Sci. 2014, 31, 169–176. [Google Scholar] [CrossRef]
- Hersbach, H.; de Rosnay, P.; Bell, B.; Schepers, D.; Simmons, A.; Soci, C.; Abdalla, S.; Alonso-Balmaseda, M.; Balsamo, G.; Bechtold, P.; et al. Operational global reanalysis: Progress, future directions and synergies with NWP. ECMWF 2018. [Google Scholar] [CrossRef]
- Drob, D.P.; Emmert, J.T.; Crowley, G.; Picone, J.M.; Shepherd, G.G.; Skinner, W.; Hays, P.; Niciejewski, R.J.; Larsen, M.; She, C.Y.; et al. An empirical model of the Earth’s horizontal wind fields: HWM07. J. Geophys. Res. Space Phys. 2008, 113. [Google Scholar] [CrossRef]
- Drob, D.P.; Emmert, J.T.; Meriwether, J.W.; Makela, J.J.; Doornbos, E.; Conde, M.; Hernandez, G.; Noto, J.; Zawdie, K.A.; McDonald, S.E.; et al. An update to the Horizontal Wind Model (HWM): The quiet time thermosphere. Earth Space Sci. 2015, 2, 301–319. [Google Scholar] [CrossRef]
- Gelaro, R.; McCarty, W.; Suárez, M.J.; Todling, R.; Molod, A.; Takacs, L.; Randles, C.A.; Darmenov, A.; Bosilovich, M.G.; Reichle, R.; et al. The Modern-Era Retrospective Analysis for Research and Applications, Version 2 (MERRA-2). J. Clim. 2017, 30, 5419–5454. [Google Scholar] [CrossRef]
- Gossard, E.; Hooke, W. Waves in the Atmosphere: Atmospheric Infrasound and Gravity Waves: Their Generation and Propagation; Developments in atmospheric science; Elsevier Scientific Pub. Co.: Amsterdam, The Netherlands, 1975. [Google Scholar]
- Medeiros, A.F.; Taylor, M.J.; Takahashi, H.; Batista, P.P.; Gobbi, D. An investigation of gravity wave activity in the low-latitude upper mesosphere: Propagation direction and wind filtering. J. Geophys. Res. Atmos. 2003, 108. [Google Scholar] [CrossRef]
- Wrasse, C.; Nakamura, T.; Tsuda, T.; Takahashi, H.; Medeiros, A.; Taylor, M.; Gobbi, D.; Salatun, A.; Suratno; Achmad, E.; et al. Reverse ray tracing of the mesospheric gravity waves observed at 23° S (Brazil) and 7° S (Indonesia) in airglow imagers. J. Atmos. Sol. -Terr. Phys. 2006, 68, 163–181. [Google Scholar] [CrossRef]
- Wrasse, C.M.; Nakamura, T.; Takahashi, H.; Medeiros, A.F.; Taylor, M.J.; Gobbi, D.; Denardini, C.M.; Fechine, J.; Buriti, R.A.; Salatun, A.; et al. Mesospheric gravity waves observed near equatorial and low-middle latitude stations: Wave characteristics and reverse ray tracing results. Ann. Geophys. 2006, 24, 3229–3240. [Google Scholar] [CrossRef]
- Taylor, M.; Hapgood, M. On the origin of ripple-type wave structure in the OH nightglow emission. Planet. Space Sci. 1990, 38, 1421–1430. [Google Scholar] [CrossRef]
- Hecht, J.H. Instability layers and airglow imaging. Rev. Geophys. 2004, 42. [Google Scholar] [CrossRef]
- Walterscheid, R.; Hecht, J.; Vincent, R.; Reid, I.; Woithe, J.; Hickey, M. Analysis and interpretation of airglow and radar observations of quasi-monochromatic gravity waves in the upper mesosphere and lower thermosphere over Adelaide, Australia (35° S, 138° E). J. Atmos. Sol. -Terr. Phys. 1999, 61, 461–478. [Google Scholar] [CrossRef]
- Hecht, J.H.; Walterscheid, R.L.; Hickey, M.P.; Franke, S.J. Climatology and modeling of quasi-monochromatic atmospheric gravity waves observed over Urbana Illinois. J. Geophys. Res. Atmos. 2001, 106, 5181–5195. [Google Scholar] [CrossRef]
- Perwitasari, S.; Nakamura, T.; Kogure, M.; Tomikawa, Y.; Ejiri, M.K.; Shiokawa, K. Comparison of gravity wave propagation directions observed by mesospheric airglow imaging at three different latitudes using the M-transform. Ann. Geophys. 2018, 36, 1597–1605. [Google Scholar] [CrossRef]
- Figueiredo, C.A.O.B.; Takahashi, H.; Wrasse, C.M.; Otsuka, Y.; Shiokawa, K.; Barros, D. Investigation of Nighttime MSTIDS Observed by Optical Thermosphere Imagers at Low Latitudes: Morphology, Propagation Direction, and Wind Filtering. J. Geophys. Res. Space Phys. 2018, 123, 7843–7857. [Google Scholar] [CrossRef]
- Paulino, I.; Moraes, J.F.; Maranhão, G.L.; Wrasse, C.M.; Buriti, R.A.; Medeiros, A.F.; Paulino, A.R.; Takahashi, H.; Makela, J.J.; Meriwether, J.W.; et al. Intrinsic parameters of periodic waves observed in the OI6300 airglow layer over the Brazilian equatorial region. Ann. Geophys. 2018, 36, 265–273. [Google Scholar] [CrossRef]
- Campos, J.; Paulino, I.; Wrasse, C.; Medeiros, A.; Paulino, A.; Buriti, R. Observations of Small-Scale Gravity Waves in the Equatorial Upper Mesosphere. Braz. J. Geophys. 2016, 34, 469–477. [Google Scholar] [CrossRef][Green Version]
- Tomikawa, Y. Gravity wave transmission diagram. Ann. Geophys. 2015, 33, 1479–1484. [Google Scholar] [CrossRef]
- Figueiredo, C.A.O.B.; Takahashi, H.; Wrasse, C.M.; Otsuka, Y.; Shiokawa, K.; Barros, D. Medium-Scale Traveling Ionospheric Disturbances Observed by Detrended Total Electron Content Maps Over Brazil. J. Geophys. Res. Space Phys. 2018, 123, 2215–2227. [Google Scholar] [CrossRef]
- Lighthill, M. Waves in Fluids; Cambridge University Press: Cambridge, UK, 1978. [Google Scholar]
- Marks, C.J.; Eckermann, S.D. A Three-Dimensional Nonhydrostatic Ray-Tracing Model for Gravity Waves: Formulation and Preliminary Results for the Middle Atmosphere. J. Atmos. Sci. 1995, 52, 1959–1984. [Google Scholar] [CrossRef]
- Trinh, Q.T.; Ern, M.; Doornbos, E.; Preusse, P.; Riese, M. Satellite observations of middle atmosphere–thermosphere vertical coupling by gravity waves. Ann. Geophys. 2018, 36, 425–444. [Google Scholar] [CrossRef]
- Vadas, S.L.; Liu, H.L. Numerical modeling of the large-scale neutral and plasma responses to the body forces created by the dissipation of gravity waves from 6 h of deep convection in Brazil. J. Geophys. Res. Space Phys. 2013, 118, 2593–2617. [Google Scholar] [CrossRef]
- Vadas, S.L.; Liu, H.L.; Lieberman, R.S. Numerical modeling of the global changes to the thermosphere and ionosphere from the dissipation of gravity waves from deep convection. J. Geophys. Res. Space Phys. 2014, 119, 7762–7793. [Google Scholar] [CrossRef]
- Rourke, S.; Mulligan, F.J.; French, W.J.R.; Murphy, D.J. A Climatological Study of Short-Period Gravity Waves and Ripples at Davis Station, Antarctica (68S, 78E), During the (Austral Winter February–October) Period 1999–2013. J. Geophys. Res. Atmos. 2017, 122, 11388–11404. [Google Scholar] [CrossRef]
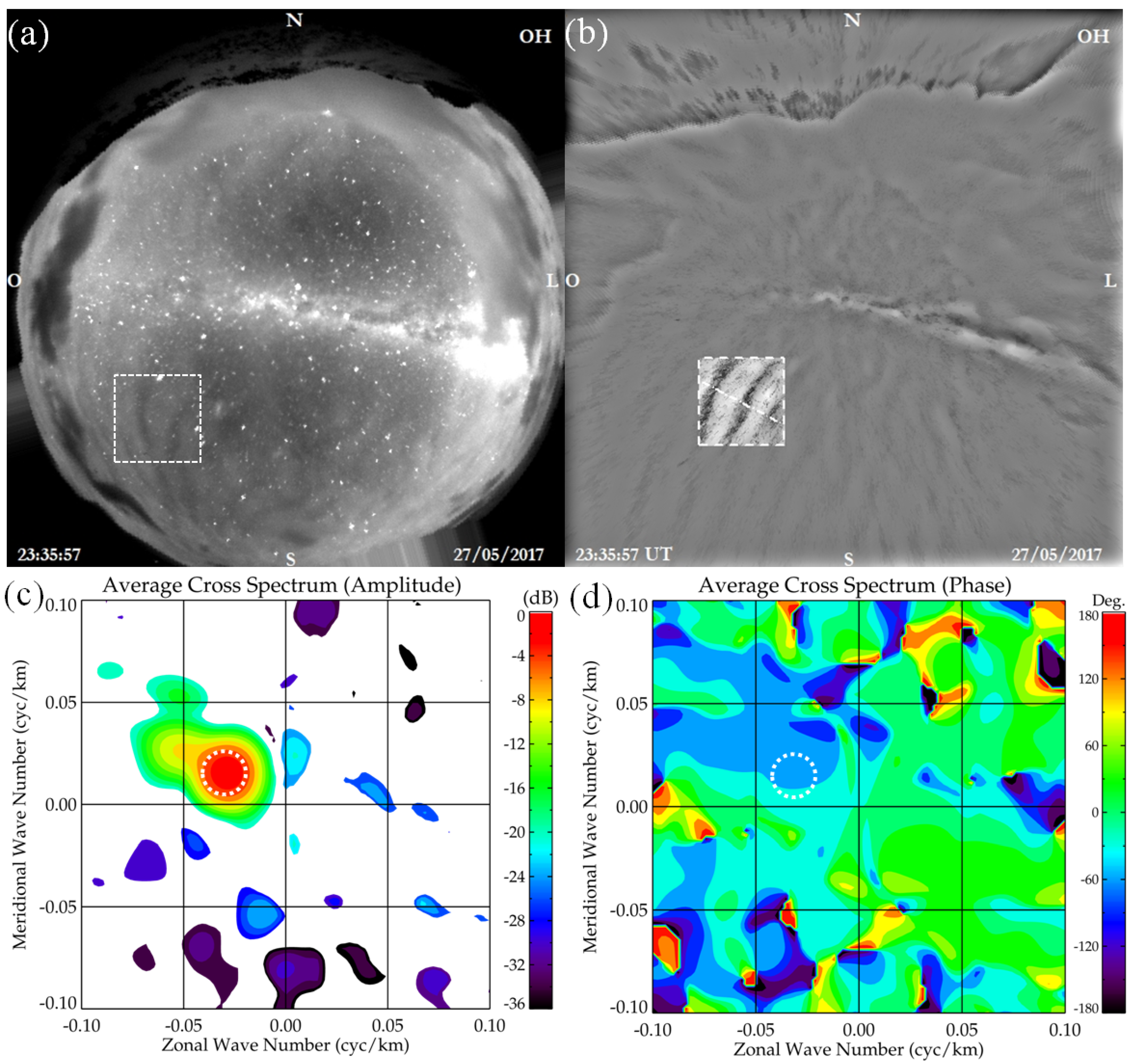
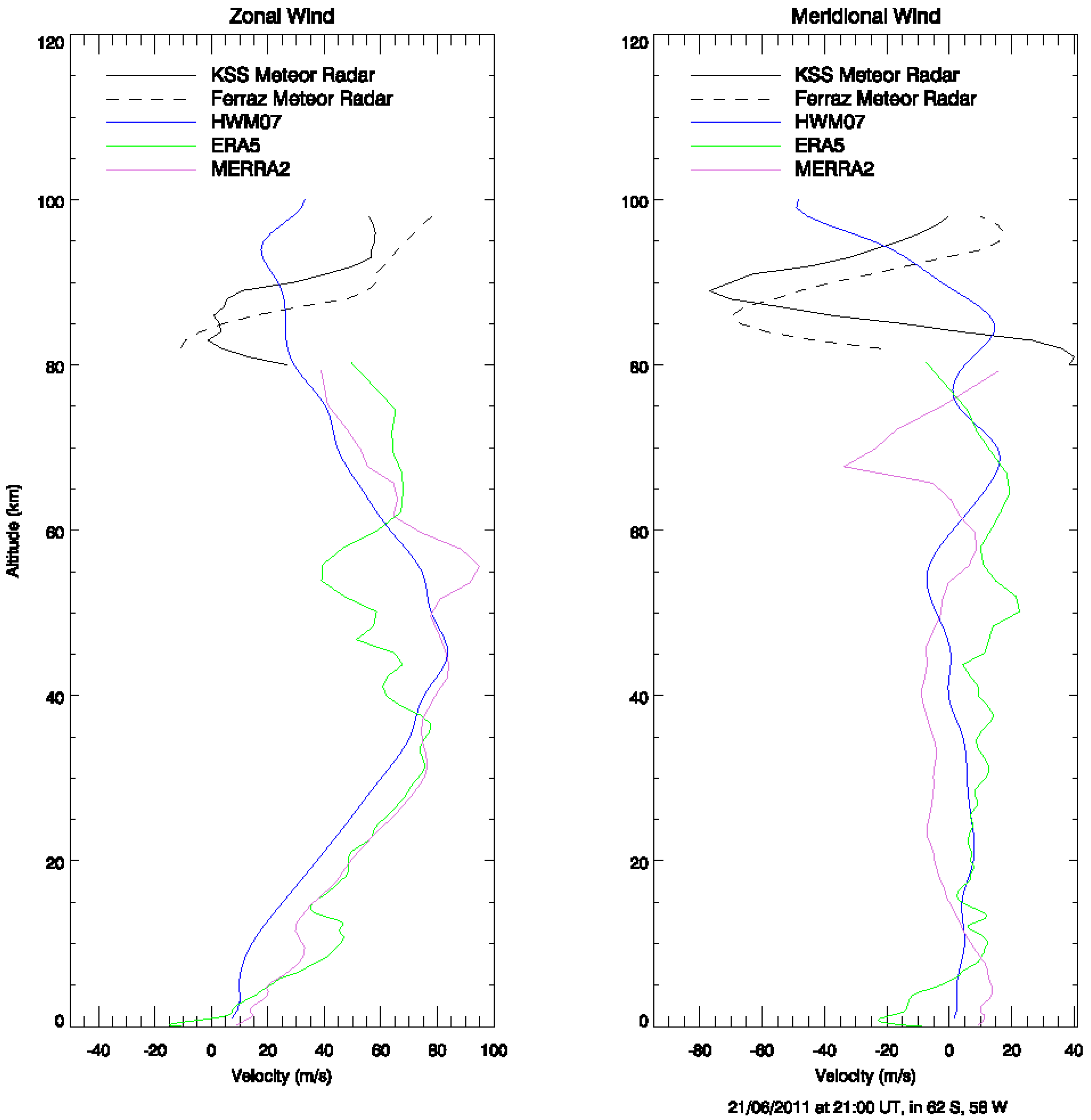

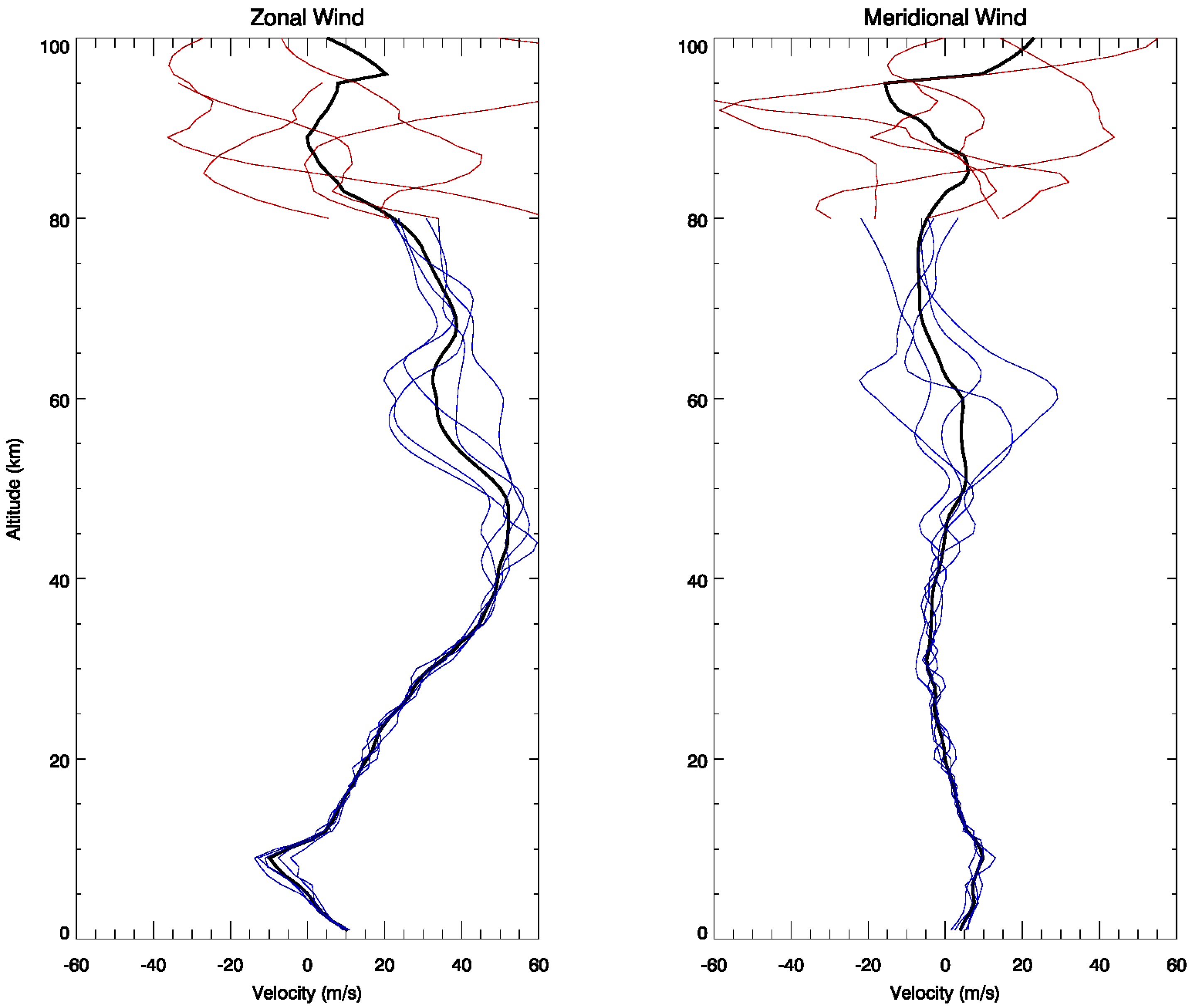
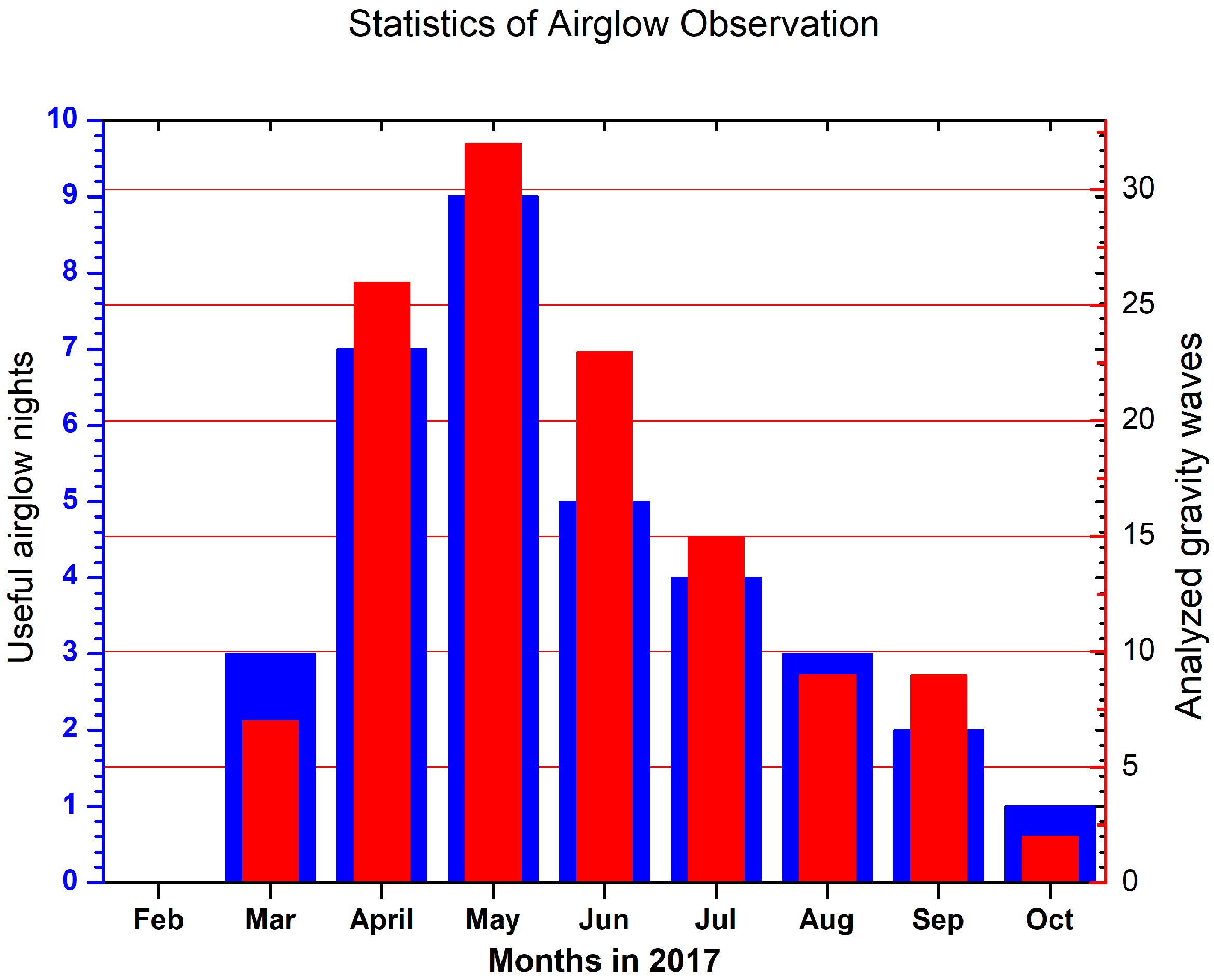

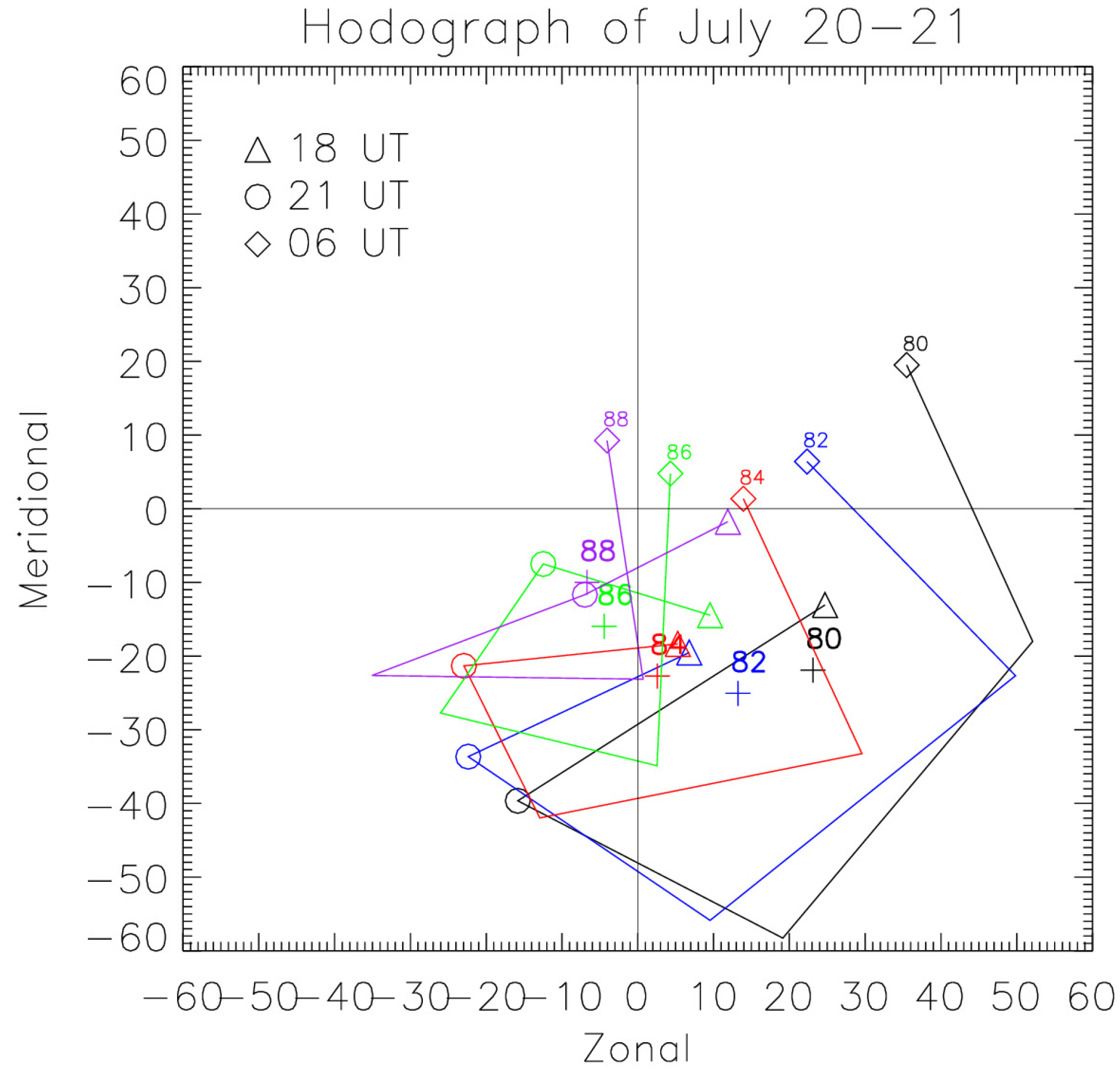
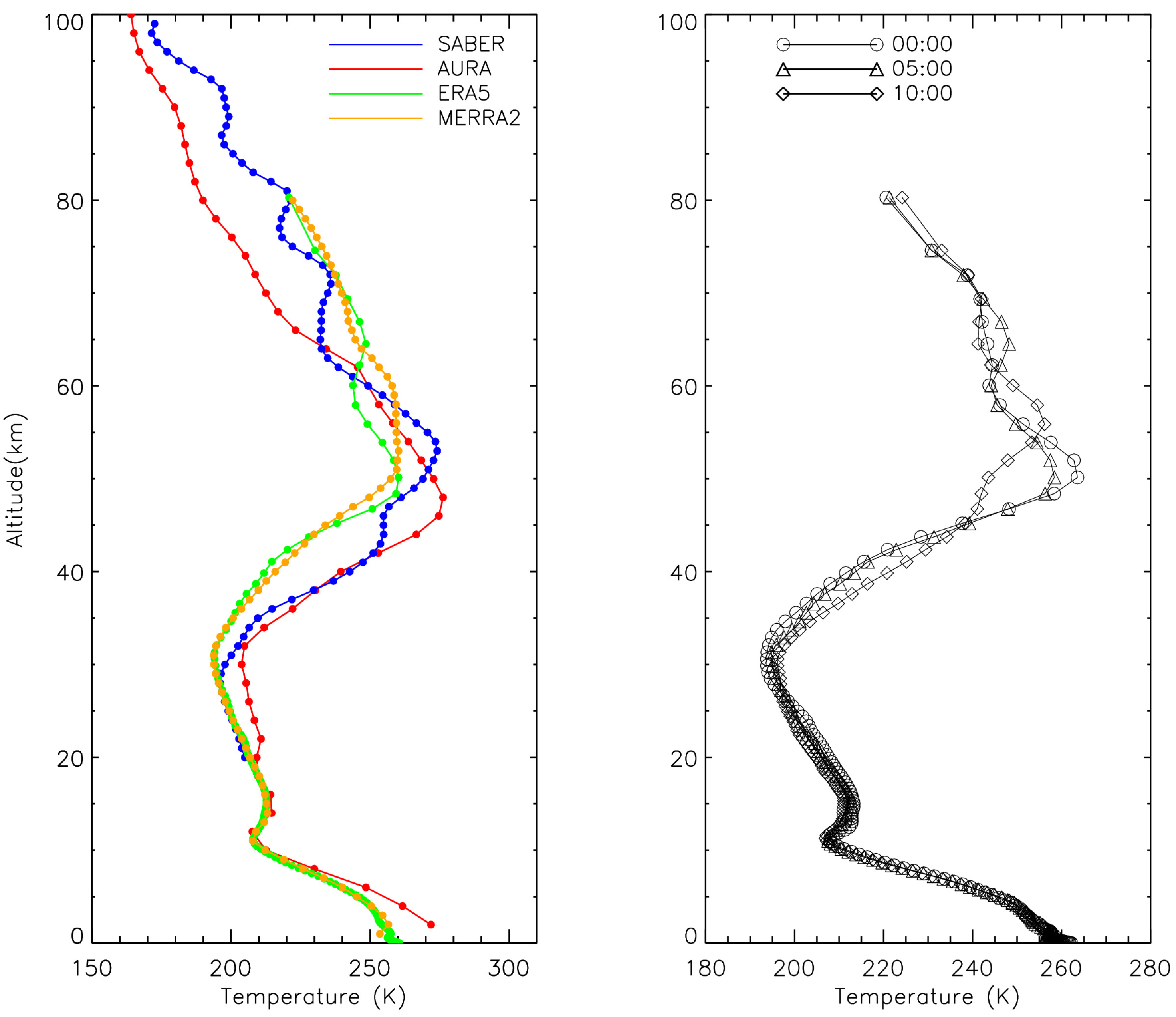

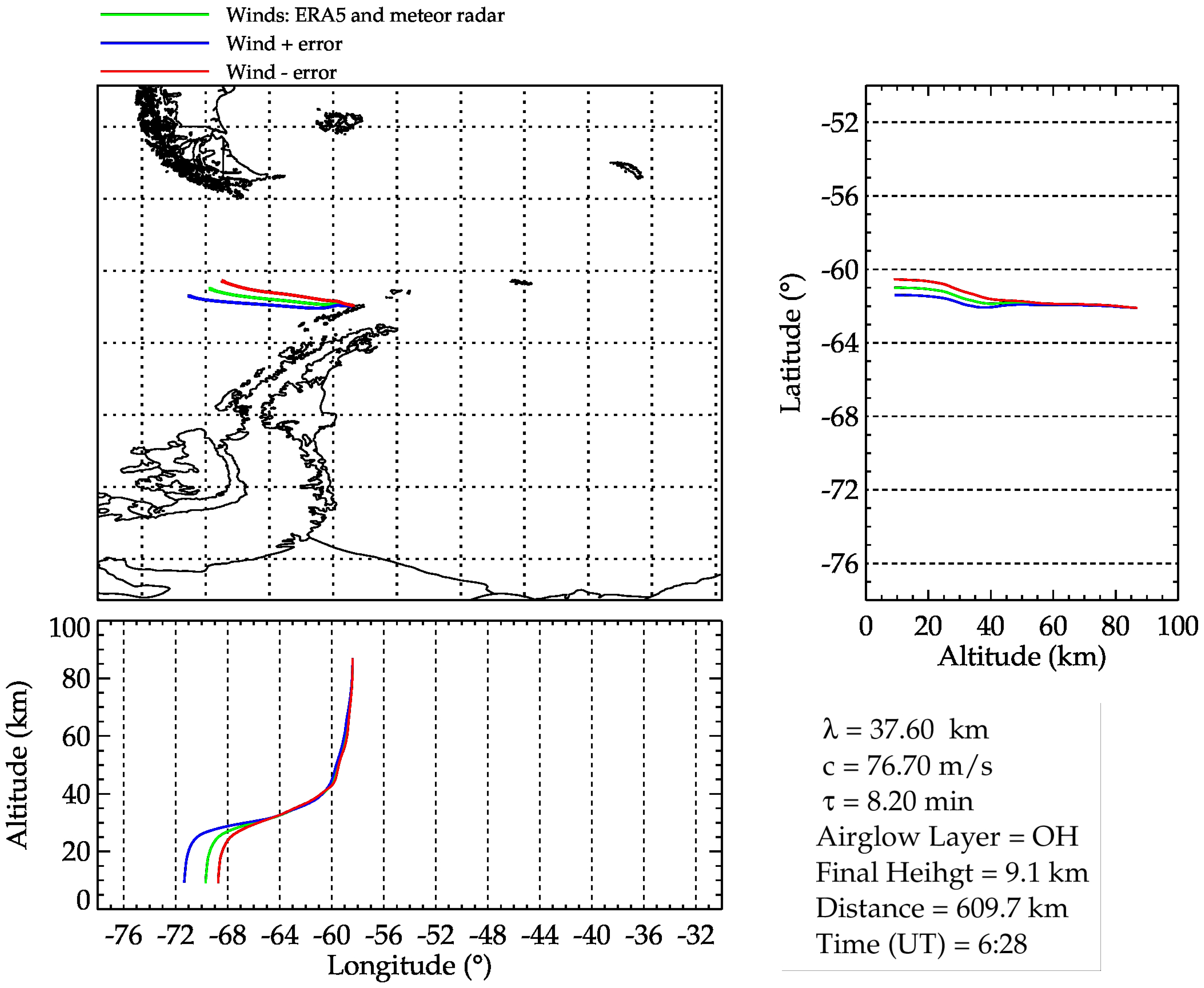
| Wave # | Observed | Horizontal | Period | Phase | Propagation |
|---|---|---|---|---|---|
| Time (UT) | Wavelength (km) | (min) | Speed (m/s) | Direction (°) | |
| 1 | 21:49 | 23.2 | 7.9 | 49.2 | 84.8 (E) |
| 2 | 22:07 | 26.3 | 24.9 | 19.6 | 301.0 (NW) |
| 3 | 02:55 | 26.8 | 31.9 | 14;0 | 276.0 (W) |
| 4 | 03:11 | 52.0 | 34.0 | 25.5 | 294.0 (NW) |
| 5 | 03:23 | 15.6 | 14.9 | 17.4 | 289.5 (W) |
| 6 | 05:48 | 29.3 | 32.6 | 15.0 | 246.4 (W) |
| 7 | 08:29 | 39.3 | 10.3 | 63.5 | 85.6 (E) |
| 8 | 08:30 | 181.0 | 30.0 | 99.0 | 98.8 (E) |
| 9 | 10:32 | 37.6 | 8.2 | 76.7 | 126 (SE) |
| Wave # | Observed | Generated | Final Height | Horizontal | Propagating | Direction |
|---|---|---|---|---|---|---|
| Time (UT) | Time (UT) | (km) | Distance (km) | Direction (°) | Stopped | |
| 1 | 21:49 | 20:11 | 72.3 | 129.6 | 84.8 (E) | W |
| 2 | 22:07 | 21:23 | 86.9 | 30.6 | 301.0 (NW) | E |
| 3 | 02:55 | 02:11 | 83.2 | 136 | 276.0 (W) | - |
| 4 | 03:11 | 2:52 | 84.4 | 33.9 | 294.0 (NW) | - |
| 5 | 03:23 | - | - | - | 289.5 (W) | - |
| 6 | 05:48 | 03:54 | 4.3 | 118.8 | 246.4 (W) | SW |
| 7 | 08:29 | 05:16 | 63.6 | 397.4 | 85.6 (E) | SW |
| 8 | 08:30 | 02:50 | 33.7 | 907.1 | 98.8 (E) | W |
| 9 | 10:32 | 06:28 | 9.1 | 609.7 | 126 (SE) | W |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Giongo, G.A.; Bageston, J.V.; Figueiredo, C.A.O.B.; Wrasse, C.M.; Kam, H.; Kim, Y.H.; Schuch, N.J. Gravity Wave Investigations over Comandante Ferraz Antarctic Station in 2017: General Characteristics, Wind Filtering and Case Study. Atmosphere 2020, 11, 880. https://doi.org/10.3390/atmos11080880
Giongo GA, Bageston JV, Figueiredo CAOB, Wrasse CM, Kam H, Kim YH, Schuch NJ. Gravity Wave Investigations over Comandante Ferraz Antarctic Station in 2017: General Characteristics, Wind Filtering and Case Study. Atmosphere. 2020; 11(8):880. https://doi.org/10.3390/atmos11080880
Chicago/Turabian StyleGiongo, Gabriel Augusto, José Valentin Bageston, Cosme Alexandre Oliveira Barros Figueiredo, Cristiano Max Wrasse, Hosik Kam, Yong Ha Kim, and Nelson Jorge Schuch. 2020. "Gravity Wave Investigations over Comandante Ferraz Antarctic Station in 2017: General Characteristics, Wind Filtering and Case Study" Atmosphere 11, no. 8: 880. https://doi.org/10.3390/atmos11080880
APA StyleGiongo, G. A., Bageston, J. V., Figueiredo, C. A. O. B., Wrasse, C. M., Kam, H., Kim, Y. H., & Schuch, N. J. (2020). Gravity Wave Investigations over Comandante Ferraz Antarctic Station in 2017: General Characteristics, Wind Filtering and Case Study. Atmosphere, 11(8), 880. https://doi.org/10.3390/atmos11080880





