Elliptical Structures of Gravity Waves Produced by Typhoon Soudelor in 2015 near Taiwan
Abstract
1. Introduction
2. Description of the Model and Methods of Analysis
3. Overview of TC Soudelor and Model Validation
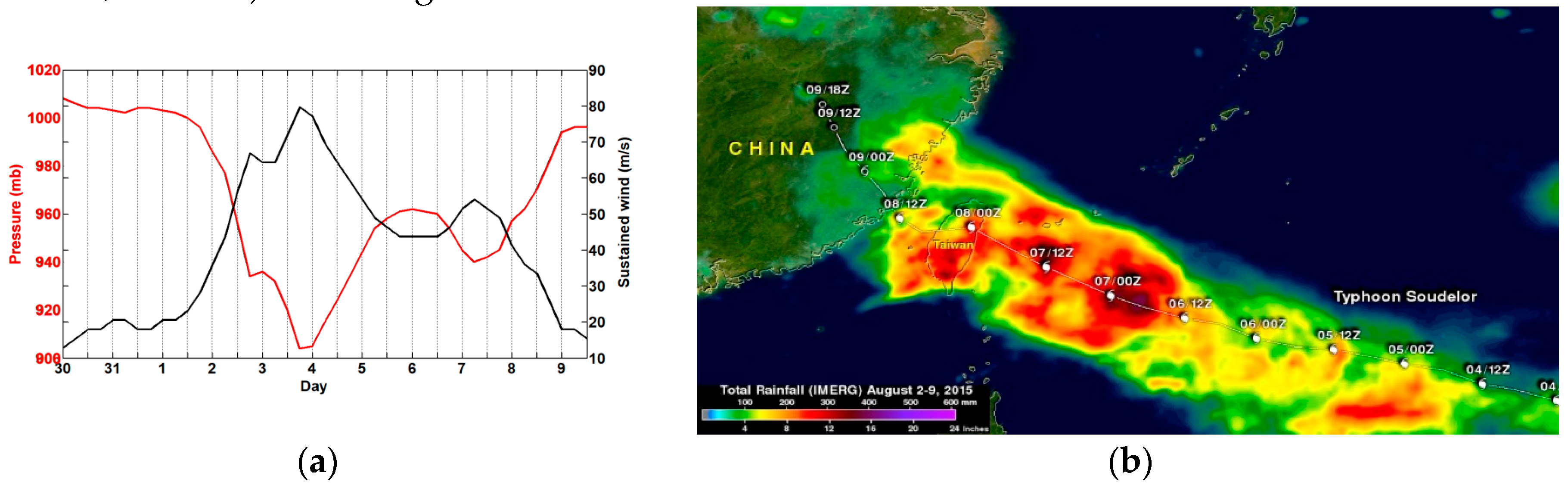
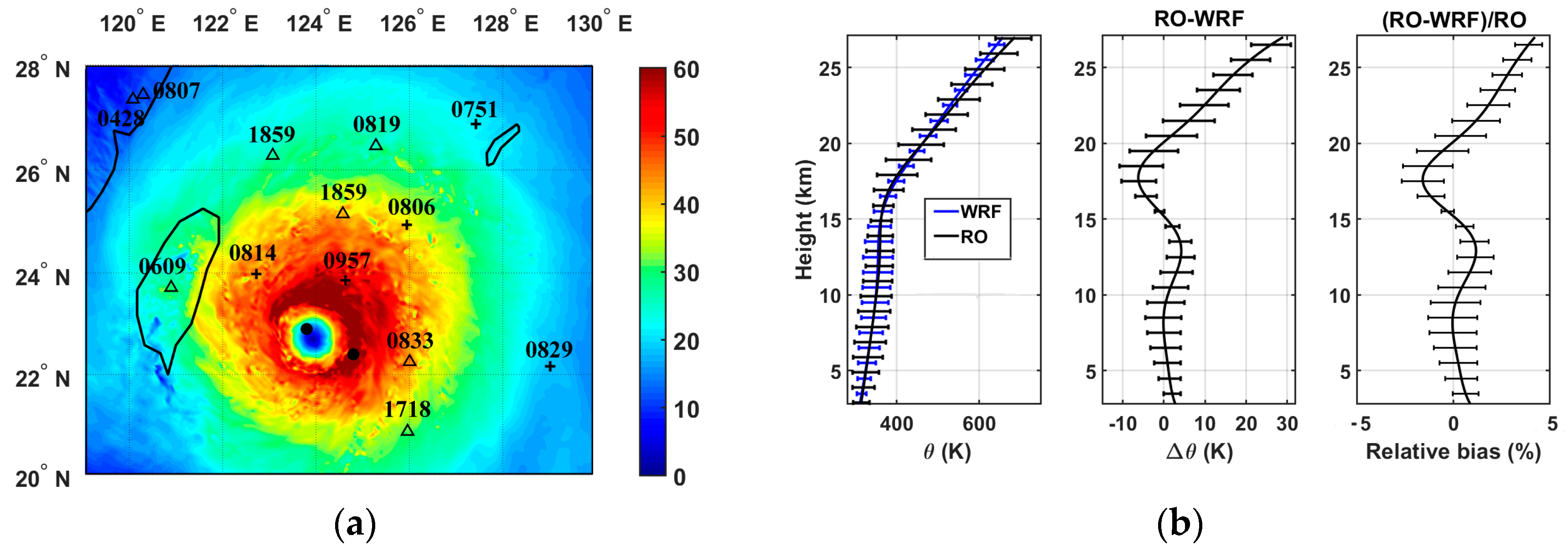
3.1. Tropical Cyclone Soudelor (2015)
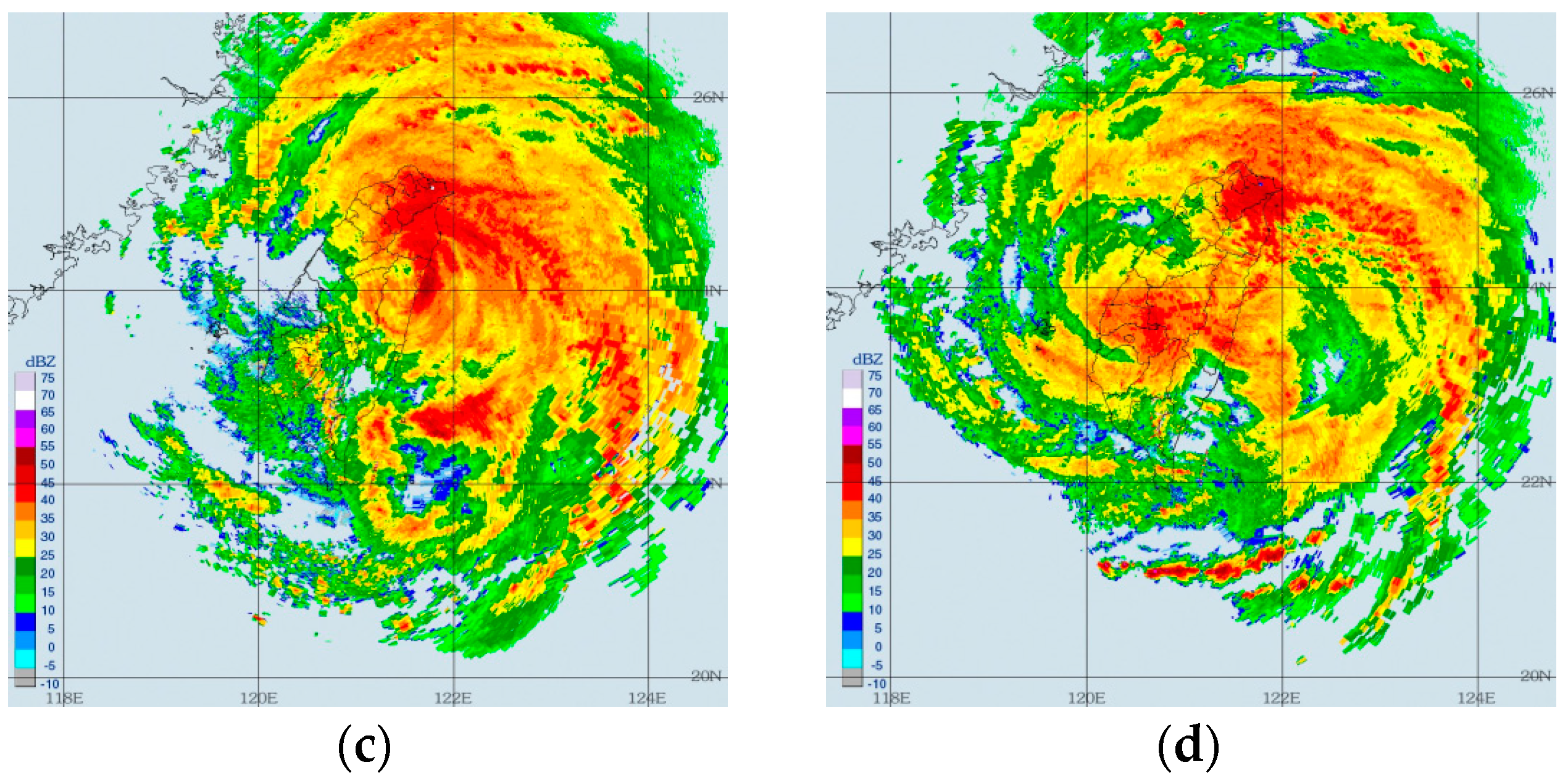
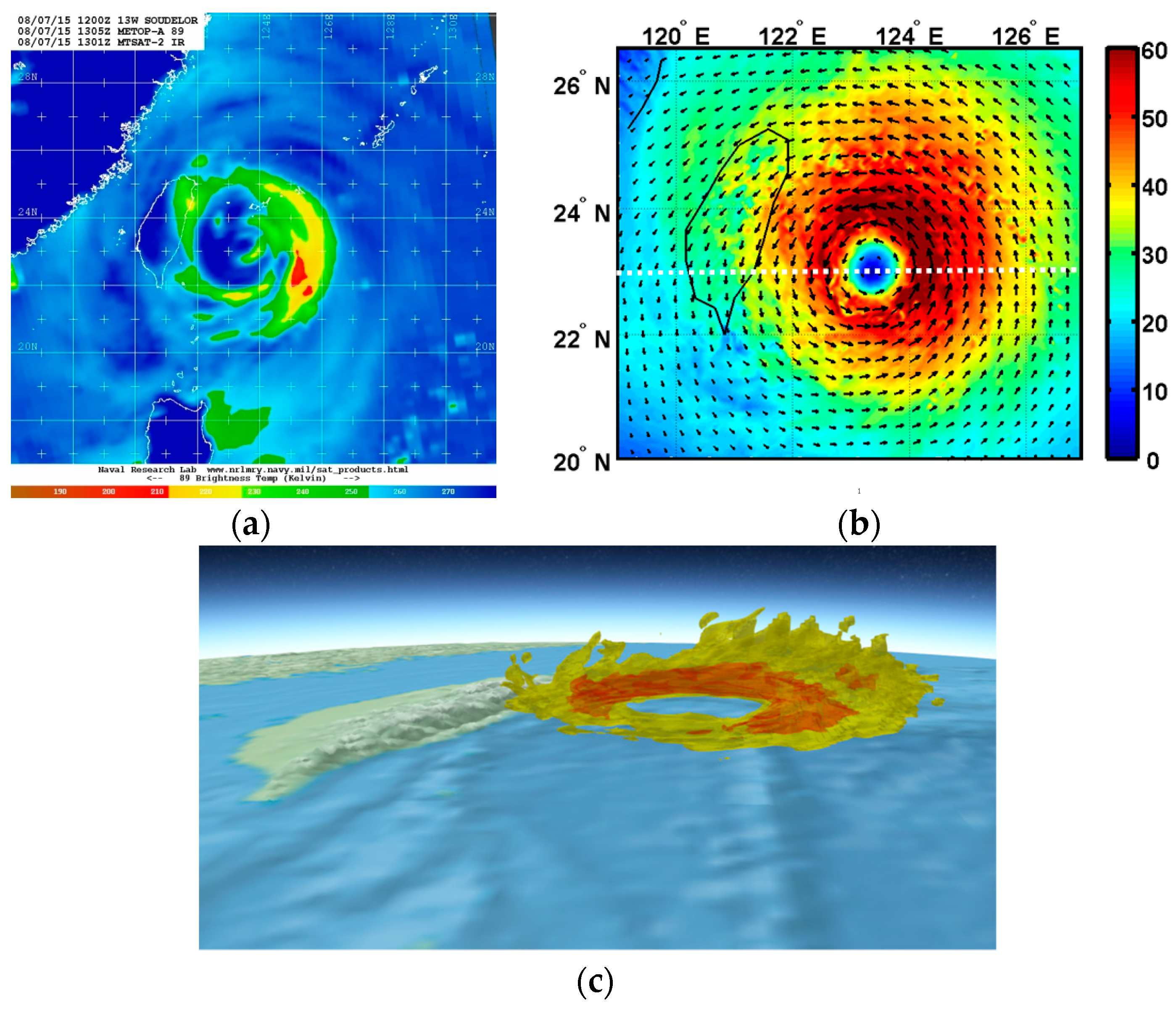
3.2. Model Validation
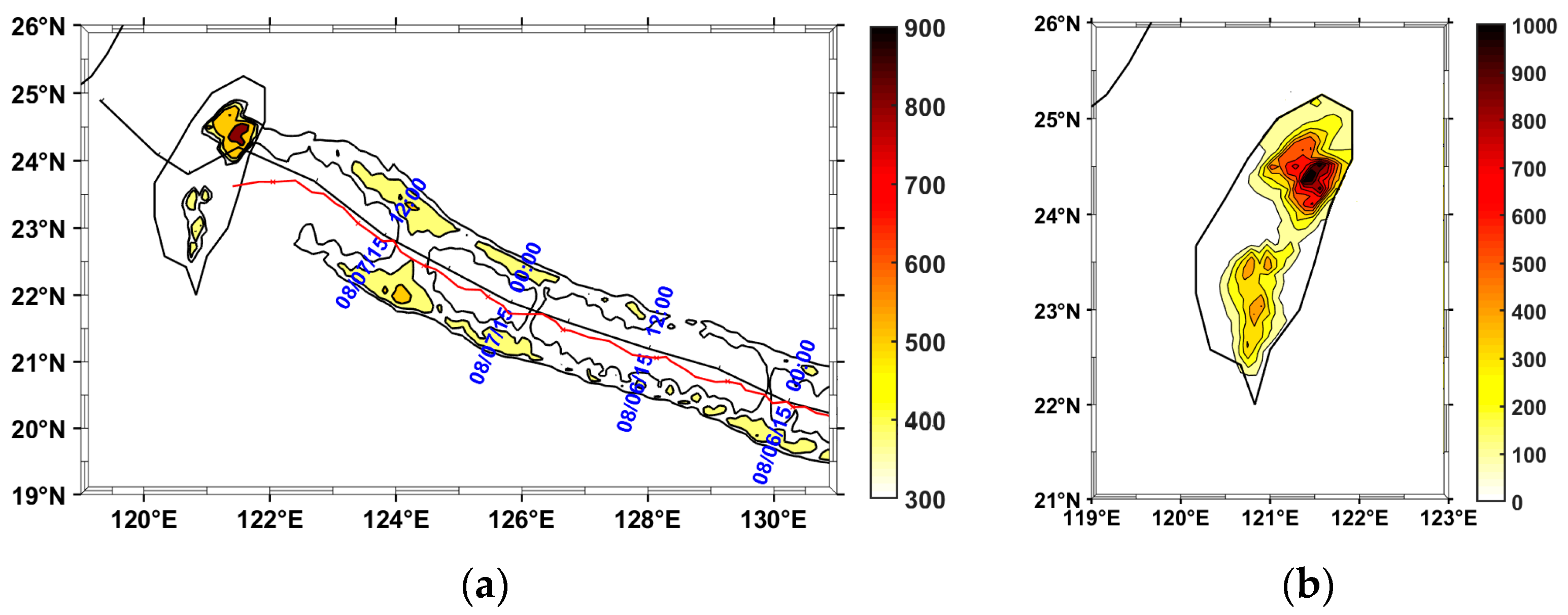
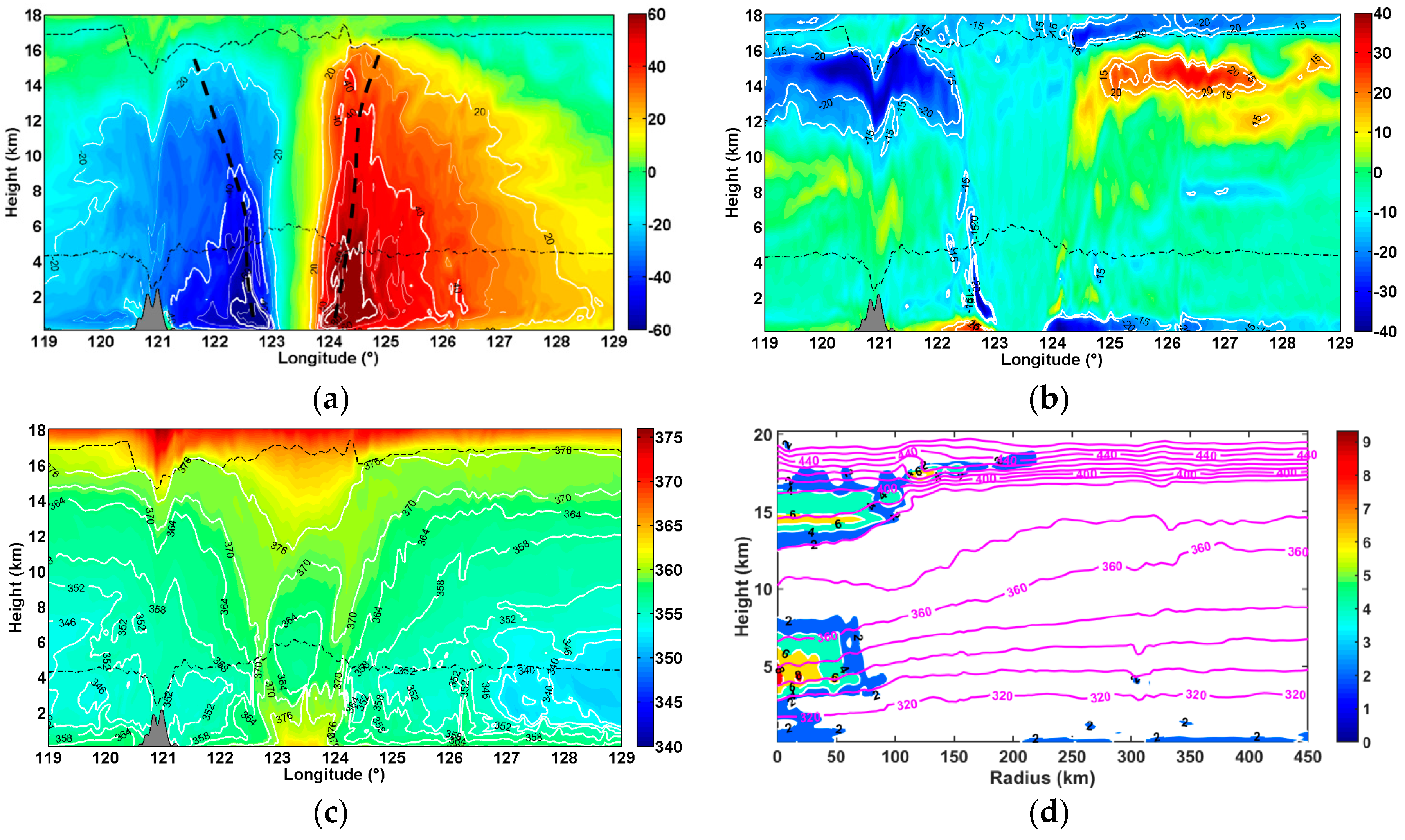
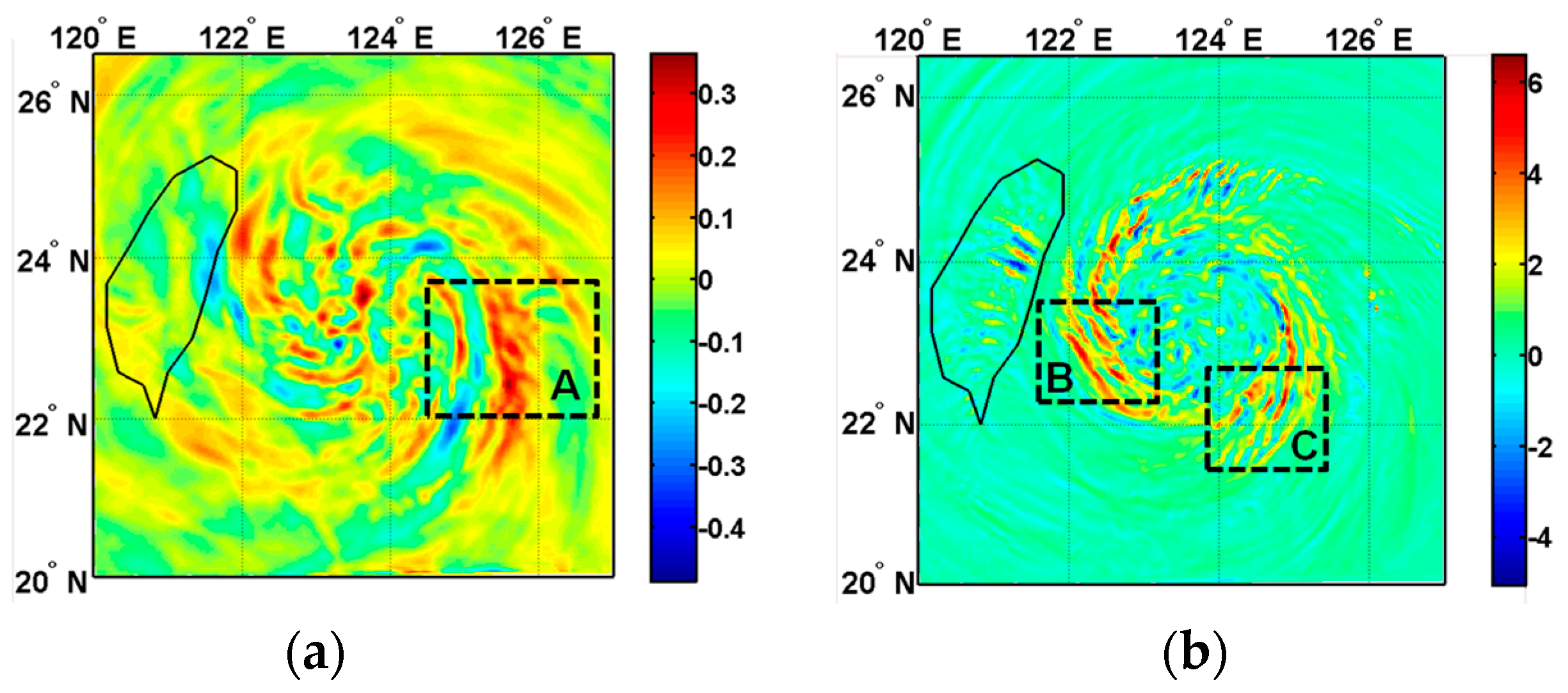
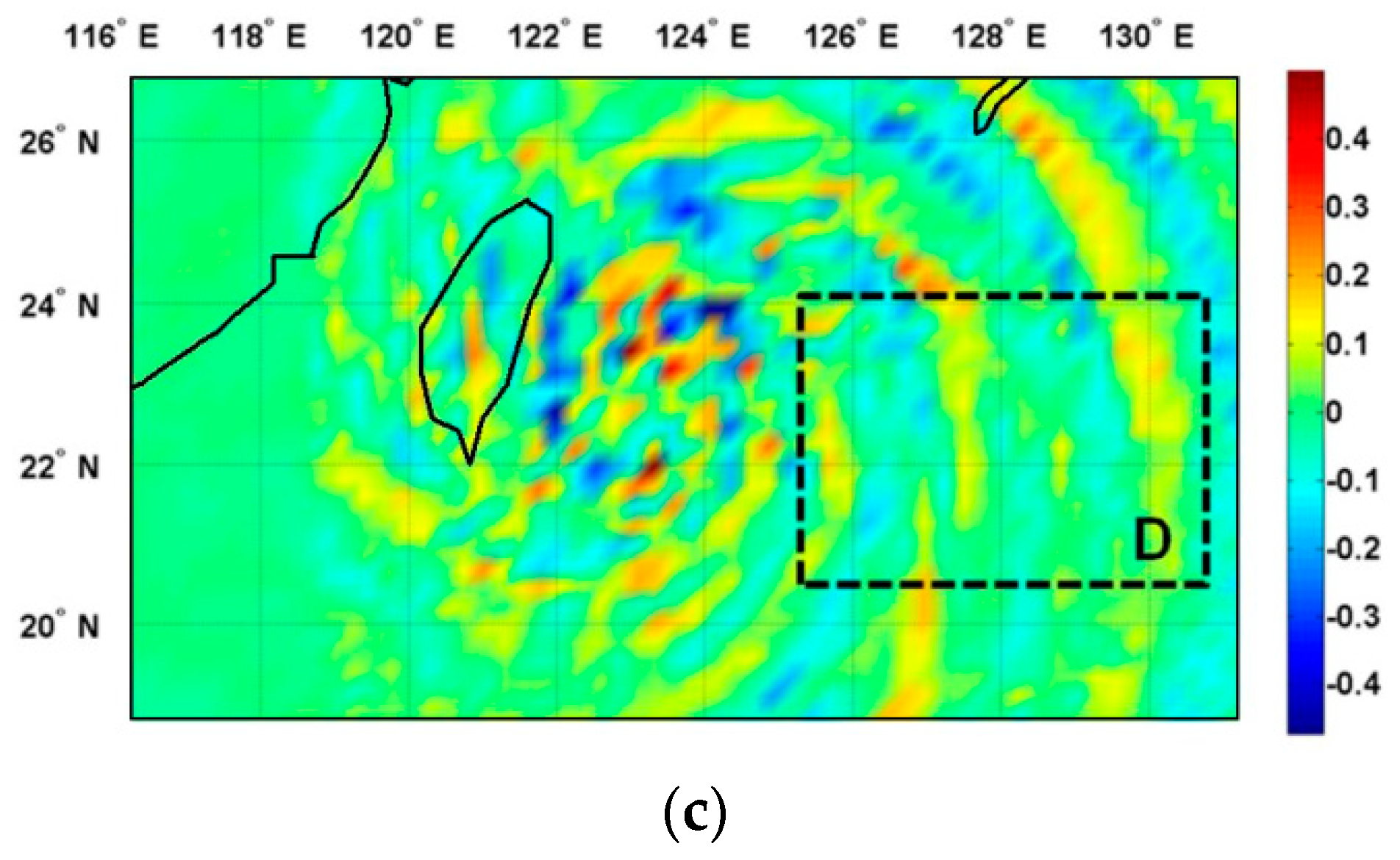
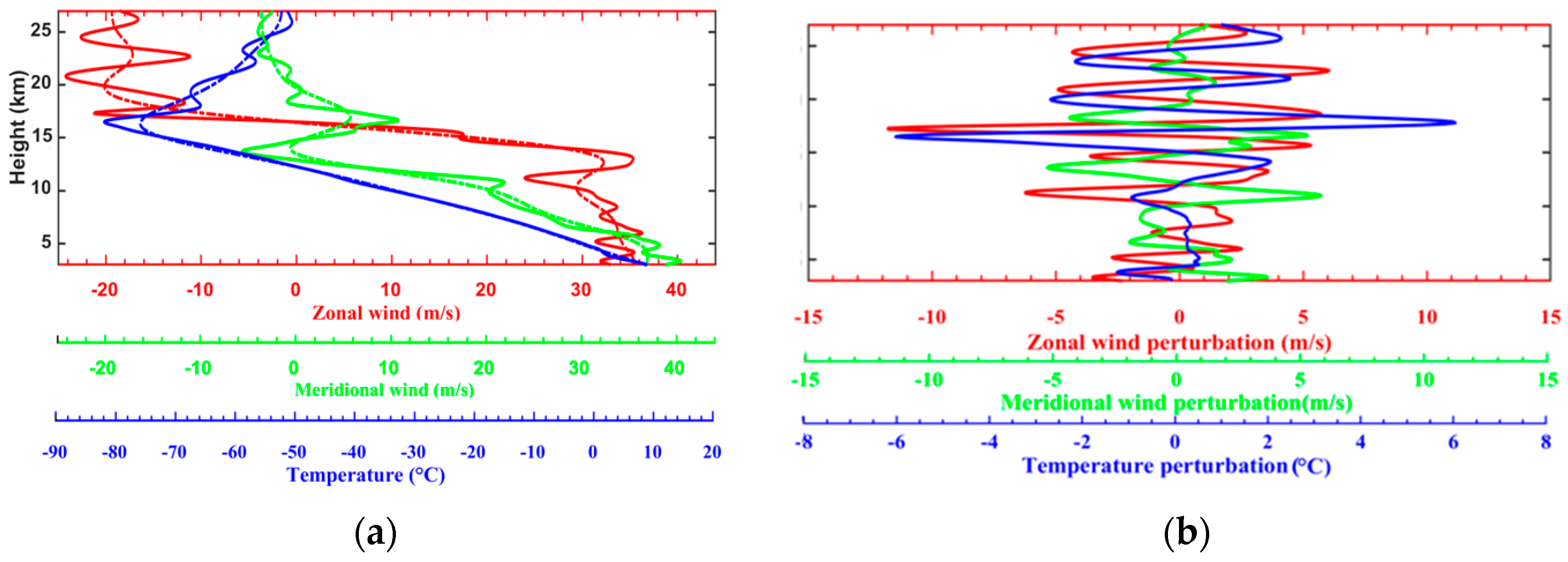
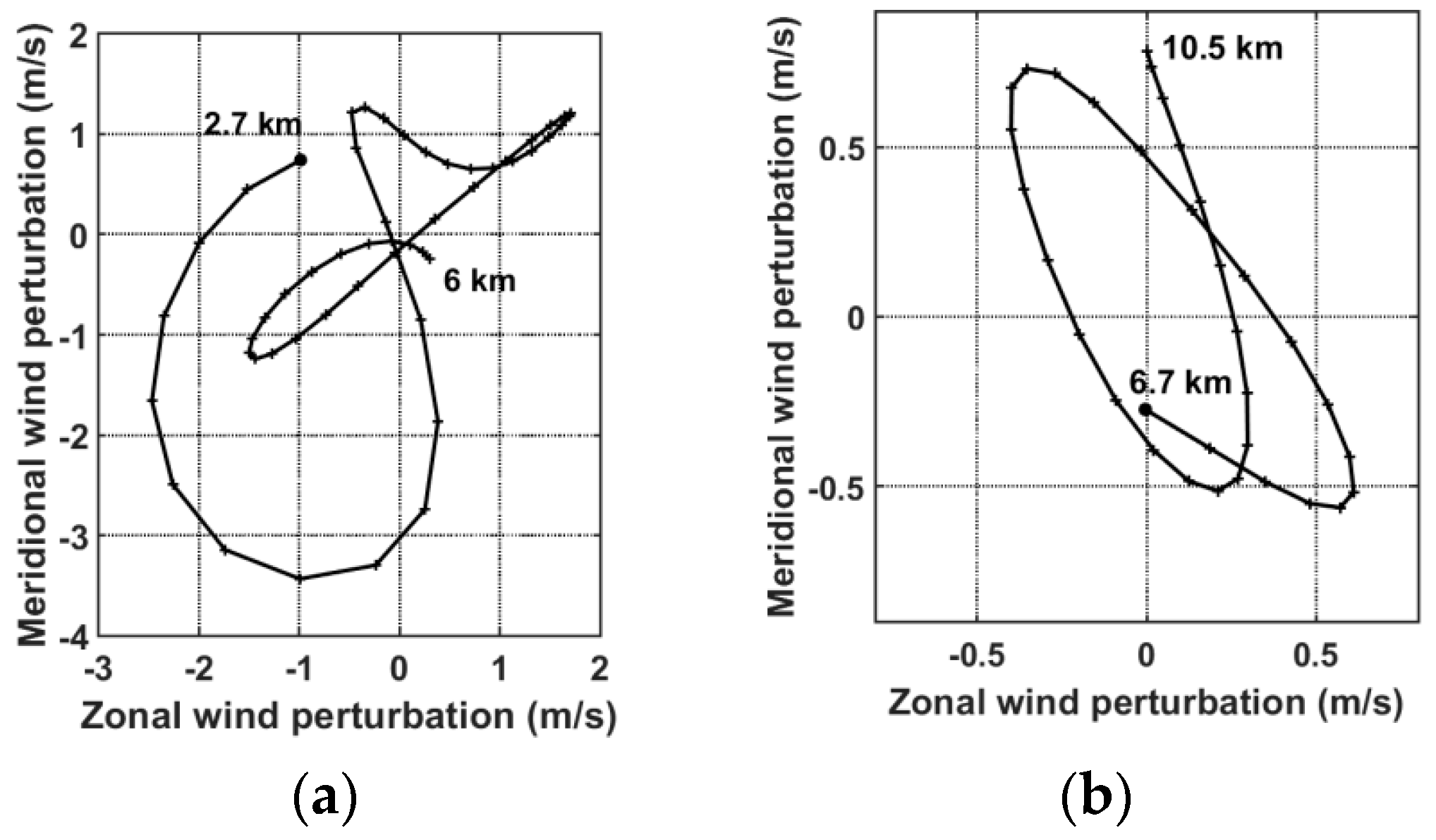
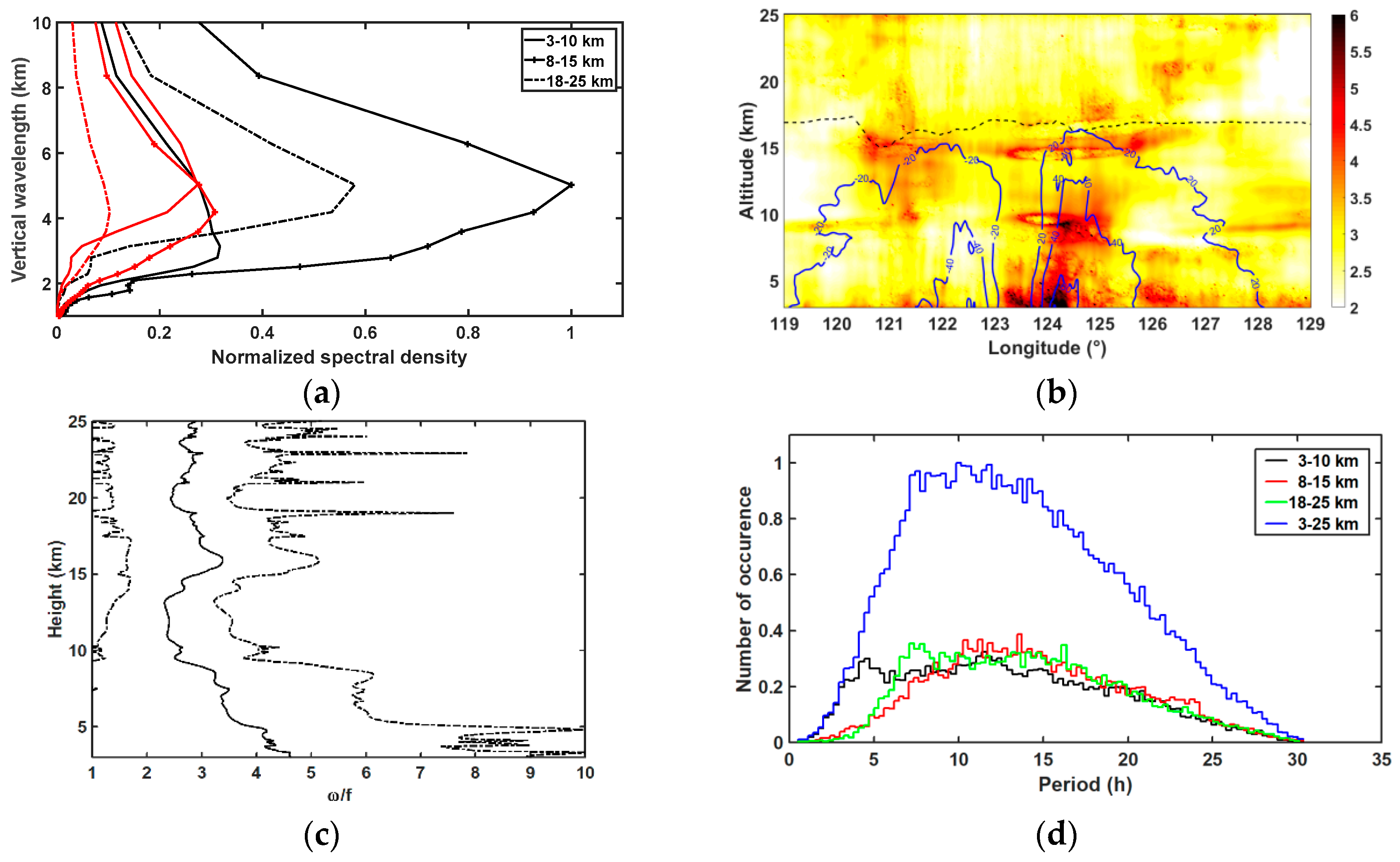
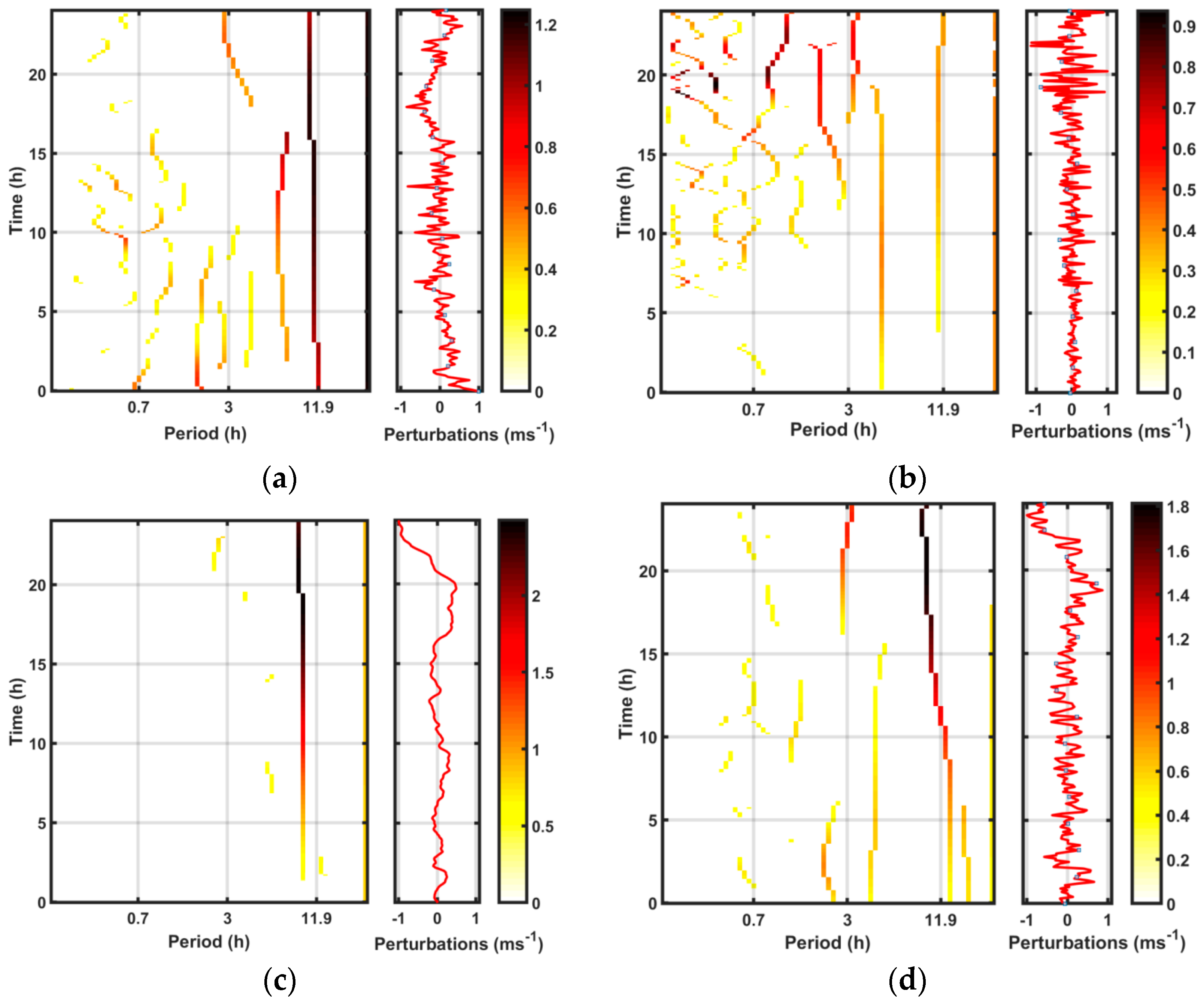
4. Results
5. Summary and Conclusions
Supplementary Materials
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Smalley, K.M.; Dessler, A.E.; Bekki, S.; Deushi, M.; Marchand, M.; Morgenstern, O.; Plummer, D.A.; Shibata, K.; Yamashita, Y.; Zeng, G. Contribution of different processes to changes in tropical lower-stratospheric water vapor in chemistry-climate models. Atmos. Chem. Phys. 2017, 17, 8031–8044. [Google Scholar] [CrossRef]
- Podglajen, A.; Hertzog, A.; Plougonven, R.; Žagar, N. Assessment of the accuracy of (re)analyses in the equatorial lower stratosphere. J. Geophys. Res. 2014, 119, 166–188. [Google Scholar] [CrossRef]
- Butchart, N.; Cionni, I.; Eyring, V.; Shepherd, T.G.; Waugh, D.W.; Akiyoshi, H.; Tian, W. Chemistry-climate model simulations of twenty-first century stratospheric climate and circulation changes. J. Clim. 2010, 23, 5349–5374. [Google Scholar] [CrossRef]
- Garcia, R.R.; Smith, A.K.; Kinnison, D.E.; de la Cámara, Á.; Murphy, D.J. Modification of the gravity wave parameterization in the Whole Atmosphere Community Climate Model: Motivation and results. J. Atmos. Sci. 2017, 74, 275–291. [Google Scholar] [CrossRef]
- Yiğit, E.; Knížová, P.K.; Georgieva, K.; Ward, W. A review of vertical coupling in the Atmosphere–Ionosphere system: Effects of waves, sudden stratospheric warmings, space weather, and of solar activity. J. Atmos. Sol. Terr. Phys. 2016, 141, 1–12. [Google Scholar] [CrossRef]
- Liu, H.L.; McInerney, J.M.; Santos, S.; Lauritzen, P.H.; Taylor, M.A.; Pedatella, N.M. Gravity waves simulated by high-resolution Whole Atmosphere Community Climate Model. Geophys. Res. Lett. 2014, 41, 9106–9112. [Google Scholar] [CrossRef]
- De la Camara, A.; Lott, F.; Jewtoukoff, V.; Plougonven, R.; Hertzog, A. On the gravity wave forcing during the southern stratospheric final warming in LMDZ. J. Atmos. Sci. 2016, 73, 3213–3226. [Google Scholar] [CrossRef]
- Scinocca, J.F. An accurate spectral nonorographic gravity wave drag parameterization for general circulation models. J. Atmos. Sci. 2003, 60, 667–682. [Google Scholar] [CrossRef]
- McCormac, J.P.; Eckermann, S.D.; Hogan, T.F. Generation of a quasi-biennial oscillation in an NWP model using a stochastic gravity wave drag parameterization. Mon. Wea. Rev. 2015, 143, 2121–2147. [Google Scholar] [CrossRef]
- Fritts, D.C.; Alexander, M.J. Gravity wave dynamics and effects in the middle atmosphere. Rev. Geophys. 2003, 41, 1003. [Google Scholar] [CrossRef]
- Tsuda, T.; Nishida, M.; Rocken, C.; Ware, R.H. A global morphology of gravity wave activity in the stratosphere revealed by the GPS occultation data (GPS/MET). J. Geophys. Res. 2000, 105, 7257–7273. [Google Scholar] [CrossRef]
- Chane Ming, F.; Roff, G.; Robert, L.; Leveau, J. Gravity waves characteristic over Tromelin island during the passage of cyclone Hudah. Geophys. Res. Lett. 2002, 29, 1–4. [Google Scholar] [CrossRef]
- Liou, Y.A.; Pavelyev, A.G.; Wicker, J.; Liu, S.F.; Pavelyev, A.A.; Schmidt, T.; Igarashi, K. Application of GPS radio occultation method for observation of the internal waves in the atmosphere. J. Geophys. Res. 2006, 111, D06104. [Google Scholar] [CrossRef]
- Preusse, P.; Schroeder, S.; Hoffmann, L.; Ern, M.; Friedl-Vallon, F.; Ungermann, J.; Riese, M. New perspectives on gravity wave remote sensing by spaceborne infrared limb imaging. Atmos. Meas. Tech. 2009, 2, 299–311. [Google Scholar] [CrossRef]
- Meyer, C.I.; Ern, M.; Hoffmann, L.; Trinh, Q.T.; Alexander, M.J. Intercomparison of AIRS and HIRDLS stratospheric gravity wave observations. Atmos. Meas. Tech. 2018, 11, 215–232. [Google Scholar] [CrossRef]
- Kuester, M.A.; Alexander, M.J.; Ray, E.A. A model study of gravity waves over Hurricane Humberto (2001). J. Atmos. Sci. 2008, 65, 3231–3246. [Google Scholar] [CrossRef]
- Kim, S.Y.; Chun, H.Y. Stratospheric gravity waves generated by Typhoon Saomai (2006): Numerical modeling in a moving frame following the typhoon. J. Atmos. Sci. 2010, 67, 3617–3636. [Google Scholar] [CrossRef]
- Chen, D.; Chen, Z.Y.; Lü, D.R. Spatiotemporal spectrum and momentum flux of the stratospheric gravity waves generated by a typhoon. Sci. China: Earth Sci. 2012, 56, 54–62. [Google Scholar] [CrossRef]
- Preusse, P.; Ern, M.; Bechtold, P.; Eckermann, S.D.; Kalisch, S.; Trinh, Q.T.; Riese, M. Characteristics of gravity waves resolved by ECMWF. Atmos. Chem. Phys. 2014, 14, 10483–10508. [Google Scholar] [CrossRef]
- Chane Ming, F.; Ibrahim, C.; Barthe, C.; Jolivet, S.; Keckhut, P.; Liou, Y.A.; Kuleshov, Y. Observation and a numerical study of gravity waves during tropical cyclone Ivan (2008). Atmos. Chem. Phys. 2014, 14, 641–658. [Google Scholar] [CrossRef]
- Liou, Y.A.; Pavelyev, A.G.; Liu, S.F.; Pavelyev, A.A.; Yen, N.; Huang, C.Y.; Fong, C.J. FORMOSAT-3/COSMIC GPS radio occultation mission: Preliminary results. IEEE Trans. Geosci. Remote Sens. 2007, 45, 3813–3826. [Google Scholar] [CrossRef]
- Yu, D.; Xu, X.; Luo, J.; Li, J. On the relationship between gravity waves and tropopause height and temperature over the globe revealed by COSMIC radio occultation measurements. Atmosphere 2019, 10, 75. [Google Scholar] [CrossRef]
- Emanuel, K. 100 Years of Progress in Tropical Cyclone Research. Meteorol. Monogr. 2018, 59, 15.11–15.68. [Google Scholar] [CrossRef]
- Chane Ming, F.; Chen, Z.; Roux, F. Analysis of gravity waves produced by intense tropical cyclones. Ann. Geophys. 2010, 28, 531–547. [Google Scholar] [CrossRef]
- Ibrahim, C.; Chane-Ming, F.; Barthe, C.; Kuleshov, Y. Diagnosis of tropical cyclone activity through gravity wave energy density in the South West Indian Ocean. Geophys. Res. Lett. 2010, 37, L09807. [Google Scholar] [CrossRef]
- Hoffmann, L.; Wu, X.; Alexander, M.J. Satellite observations of stratospheric gravity waves associated with the intensification of tropical cyclones. Geophys. Res. Lett. 2018, 45, 1692–1700. [Google Scholar] [CrossRef]
- Nolan, D.S.; Zhang, J.A. Spiral gravity waves radiating from tropical cyclones. Geophys. Res. Lett. 2017, 44, 3924–3931. [Google Scholar] [CrossRef]
- Liu, Y.; Zhang, D.L.; Yau, M.K. A Multiscale numerical study of hurricane Andrew (1992). Part II: kinematics and inner-core Structures. Mon. Wea. Rev. 1999, 127, 2597–2616. [Google Scholar] [CrossRef]
- Chen, S.; Lu, Y.; Li, W.; Wen, Z. Identification and analysis of high-frequency oscillations in the eyewalls of tropical cyclones. Adv. Atmos. Sci. 2015, 32, 624–634. [Google Scholar] [CrossRef]
- Wu, L.; Braun, S.A. Effects of environmentally induced asymmetries on hurricane intensity: A numerical study. J. Atmos. Sci. 2004, 61, 3065–3081. [Google Scholar] [CrossRef]
- Kepert, D.J. Tropical Cyclone Structure and Dynamics. In Global Perspectives on Tropical Cyclones: From Science to Mitigation; Chan, J.C.L., Kepert, D.J., Eds.; World Scientific Publishing Company: Singapore, Singapore, 2010; pp. 3–53. [Google Scholar]
- Skamarock, W.C.; Klemp, J.B.; Dudhia, J.; Gill, D.O.; Barker, D.M.; Duda, M.G.; Powers, J.G. A description of the advanced research WRF version 3. In NCAR Technical Note; NCAR: Boulder, CO, USA, 2008. [Google Scholar]
- Kain, J.S. The Kain Fritsch Convective Parameterization: An Update. J. App. Meteor. 2004, 43, 170–181. [Google Scholar] [CrossRef]
- Hong, S.Y.; Noh, Y.; Dudhia, J. A new vertical diffusion package with an explicit treatment of entrainment processes. Mon. Wea. Rev. 2006, 134, 1318–1341. [Google Scholar] [CrossRef]
- Thompson, G.; Field, P.R.; Rasmussen, R.M.; Hall, W.D. Explicit forecasts of winter precipitation using an improved bulk microphysics scheme. Part II: Implementation of a new snow parameterization. Mon. Wea. Rev. 2008, 136, 5095–5115. [Google Scholar] [CrossRef]
- Mlawer, E.J.; Taubman, S.J.; Brown, P.D.; Iacono, M.J.; Clough, S.A. Radiative transfer for inhomogeneous atmospheres: RRTM, a validated correlated-k model for the longwave. J. Geophys. Res. 1997, 102, 16663–16682. [Google Scholar] [CrossRef]
- Dudhia, J. Numerical study of convection observed during the Winter Monsoon Experiment using a mesoscale two-dimensional model. J. Atmos. Sci. 1989, 46, 3077–3107. [Google Scholar] [CrossRef]
- Ma, L.M.; Bao, X.W. Parametrization of planetary boundary-layer height with helicity and verification with tropical cyclone prediction. Boundary Layer Meteorol. 2016, 160, 569–593. [Google Scholar] [CrossRef]
- Choudhury, D.; Das, S. The sensitivity to the microphysical schemes on the skill of forecasting the track and intensity of tropical cyclones using WRF-ARW model. J. Earth Syst. Sci. 2017, 126, 1–10. [Google Scholar] [CrossRef]
- Tao, W.K.; Shi, J.J.; Chen, S.; Lang, S.; Lin, P.L.; Hong, S.Y.; Hou, A. The impact of microphysical schemes on intensity and track of hurricane. Asia-Pacific J. Atmos. Sci. 2011, 47, 1–16. [Google Scholar] [CrossRef]
- Gopalakrishnan, S.G.; Goldenberg, S.; Quirino, T.; Zhang, X.; Marks, F.; Yeh, K.S.; Tallapragada, V. Toward improving high-resolution numerical hurricane forecasting: Influence of model horizontal grid resolution, initialization, and physics. Wea. Forecast. 2012, 27, 647–666. [Google Scholar] [CrossRef]
- Zhang, D.L.; Wang, X. Dependence of hurricane intensity and structures on vertical resolution and time-step size. Adv. Atmos. Sci. 2003, 20, 711–725. [Google Scholar] [CrossRef]
- Ma, Z.; Fei, J.; Huang, X.; Cheng, X. Sensitivity of tropical cyclone intensity and structure to vertical resolution in WRF. Asia-Pacific J. Atmos. Sci. 2012, 48, 67–81. [Google Scholar] [CrossRef]
- Fierro, A.O.; Rogers, R.F.; Marks, F.D.; Nolan, D.S. The Impact of horizontal grid spacing on the microphysical and kinematic structures of strong tropical cyclones simulated with the WRF-ARW model. Mon. Wea. Rev. 2009, 137, 3717–3743. [Google Scholar] [CrossRef]
- Vincent, R.A.; Allen, S.J.; Eckermann, S.D. Gravity wave parameters in the lower stratosphere. In Gravity Wave Processes: Their Parameterization in Global Climate Models; Hamilton, K., Ed.; Springer: New York, NY, USA, 1997; pp. 7–25. [Google Scholar]
- Sato, K.; Tateno, S.; Watanabe, S.; Kawatani, Y. Gravity wave characteristics in the Southern Hemisphere revealed by a high-resolution middle-atmosphere general circulation model. J. Atmos. Sci. 2012, 69, 1378–1396. [Google Scholar] [CrossRef]
- Gill, A.E. Atmosphere-Ocean Dynamics; Academic Press: London, UK, 1982; p. 259. [Google Scholar]
- Chane Ming, F.; Molinaro, F.; Leveau, J.; Keckhut, P.; Hauchecorne, A.; Godin, S. Vertical short-scale structures in the upper tropospheric-lower stratospheric temperature and ozone at la Réunion island (20.8° S, 55.3° E). J. Geophys. Res. 2000, 105, 26857–26870. [Google Scholar] [CrossRef]
- Lu, C.; Koch, S.E.; Wang, N. Stokes parameter analysis of a packet of turbulence-generating gravity waves. J. Geophys. Res. 2005, 110, D20105. [Google Scholar] [CrossRef]
- Chane Ming, F.; Vignelles, D.; Jegou, F.; Berthet, G.; Renard, J.B.; Gheusi, F.; Kuleshov, Y. Gravity-wave effects on tracer gases and stratospheric aerosol concentrations during the 2013 ChArMEx campaign. Atmos. Chem. Phys. 2016, 16, 8023–8042. [Google Scholar] [CrossRef]
- Lin, Y.L.; Witcraft, N.C.; Kuo, Y.H. Dynamics of track deflection associated with the passage of tropical cyclones over a mesoscale mountain. Mon. Wea. Rev. 2006, 13, 3509–3538. [Google Scholar] [CrossRef]
- Liu, L.; Lin, Y.L.; Chen, S.H. Effects of landfall location and approach angle of an idealized tropical cyclone over a long mountain range. Front. Earth Sci. 2016. [Google Scholar] [CrossRef]
- Wu, J.F.; Xue, X.H.; Hoffmann, L.; Dou, X.K.; Li, H.M.; Chen, T.D. A case study of typhoon-induced gravity waves and the orographic impacts related to Typhoon Mindulle (2004) over Taiwan. J. Geophys. Res. Atmos. 2015, 120, 9193–9207. [Google Scholar] [CrossRef]
- Chang, C.T.; Vadeboncoeur, M.A.; Lin, T.C. Resistance and resilience of social–ecological systems to recurrent typhoon disturbance on a subtropical island: Taiwan. Ecosphere 2018, 9, e02071. [Google Scholar] [CrossRef]
- Wu, M.C.; Hong, J.S.; Hsiao, L.F.; Hsu, L.H.; Wang, C.J. Effective use of ensemble numerical weather predictions in Taiwan by means of a SOM-based cluster analysis technique. Water 2017, 9, 836. [Google Scholar] [CrossRef]
- Mallik, M.A.K.; Ahasan, M.N.; Chowdhury, M.A.M. Simulation of track and landfall of tropical cyclone Viyaru and its associated strom surges using NWP models. Am. J. Mar. Sci. 2015, 3, 11–21. [Google Scholar]
- Mehra, A.; Tallapragada, V.; Zhang, Z.; Liu, B.; Zhu, L.; Wang, W.; Kim, H.S. Advancing the state of the art in operational tropical cyclone forecasting at NCEP. Trop. Cyclone Res. Rev. 2018, 7, 51–56. [Google Scholar]
- Mohapatra, M.; Nayak, D.P.; Sharma, M.; Sharma, R.P.; Bandyopadhyay, B.K. Evaluation of landfall forecast over North Indian Ocean issued by India Meteorological Department. J. Earth Syst. Sci. 2015, 124, 861–874. [Google Scholar] [CrossRef]
- Omranian, E.; Sharif, H.O.; Tavakoly, A.A. How well can global precipitation measurement (GPM) capture hurricanes? Case study: Hurricane Harvey. Remote Sens. 2018, 10, 1150. [Google Scholar] [CrossRef]
- Liu, Y.; Zhang, D.L.; Yau, M.K. A multiscale numerical study of Hurricane Andrew (1992). Part I: Explicit simulation and verification. Mon. Wea. Rev. 1997, 125, 3073–3093. [Google Scholar] [CrossRef]
- Jaiswal, R.S.; Fredrick, S.R.; Rasheed, M.; Neela, V.S.; Zaveri, L. Study of TRMM estimated freezing level height in the 36 N-36 S region. Indian J. Geo-Mar. Sci. 2015, 44, 1071–1095. [Google Scholar]
- Zhang, D.L.; Liu, Y.; Yau, M.K. A multiscale numerical study of Hurricane Andrew (1992). Part V: Inner-core thermodynamics. Mon. Wea. Rev. 2002, 130, 2745–2763. [Google Scholar] [CrossRef]
- Stern, D.P.; Nolan, D.S. On the height of the warm core in tropical cyclones. J. Atmos. Sci. 2012, 69, 1657–1680. [Google Scholar] [CrossRef]
- Kieu, C.Q.; Tallapragada, V.; Zhang, D.L.; Moon, Z. On the development of double warm-core structures in intense tropical cyclones. J. Atmos. Sci. 2016, 73, 4487–4506. [Google Scholar] [CrossRef]
- Zhang, D.L.; Chen, H. Importance of the upper-level warm core in the rapid intensification of a tropical cyclone. Geophys. Res. Lett. 2012, 39, L02806. [Google Scholar] [CrossRef]
- Stern, D.; Zhang, F. How does the eye warm? Part I: A potential temperature budget analysis of an idealized tropical cyclone. J. Atmos. Sci. 2013, 70, 73–89. [Google Scholar] [CrossRef]
- Moon, Z.; Kieu, C. Impacts of the lower stratosphere on the development of intense tropical cyclones. Atmosphere 2017, 8, 128. [Google Scholar] [CrossRef]
- Winterbottom, H.R.; Xiao, Q. An intercomparison of GPS RO retrievals with colocated analysis and in situ observations within tropical cyclones. Adv. Meteorol. 2010, 2010. [Google Scholar] [CrossRef]
- Vergados, P.; Luo, Z.J.; Emanuel, K.; Mannucci, A.J. Observational tests of hurricane intensity estimations using GPS radio occultations. J. Geophys. Res. Atmos. 2014, 119, 1936–1948. [Google Scholar] [CrossRef]
- Rivoire, L.; Birner, T.; Knaff, J. Evolution of the upper-level thermal structure in tropical cyclones. Geophys. Res. Lett. 2016, 43, 10530–10537. [Google Scholar] [CrossRef]
- Liou, Y.A.; Liu, J.C.; Chane Ming, F.; Hong, J.S.; Huang, C.Y.; Chiang, P.K.; Jolivet, S. Remote sensing of typhoons in the western Pacific Ocean. In Remote Sensing of the Asian Seas; Barale, V., Gade, M., Eds.; Springer: New York, NY, USA, 2019; pp. 251–267. [Google Scholar]
- Kim, S.Y.; Chun, H.Y.; Baik, J.J. A numerical study of gravity waves induced by convection associated with Typhoon Rusa. Geophys. Res. Lett. 2005, 32, L24816. [Google Scholar] [CrossRef]
- Kim, S.Y.; Chun, H.Y. Impact of typhoon-generated gravity waves in the typhoon development. Geophys. Res. Lett. 2011, 38, L01806. [Google Scholar] [CrossRef]
- Pfister, L.; Chan, K.R.; Bui, T.P.; Bowen, S.; Legg, M.; Gary, B.; Kelly, K.; Proffitt, M.; Starr, W. Gravity waves generated by a tropical cyclone during the STEP tropical field program: A Case Study. J. Geophys. Res. 1993, 98, 8611–8638. [Google Scholar] [CrossRef]
- Dhaka, S.K.; Takahashi, M.; Shibagaki, Y.; Yamanaka, M.D.; Fukao, S. Gravity wave generation in the lower stratosphere due to passage of the Typhoon 9426 (Orchid) observed by the MU radar at Shigaraki (34.85° N, 136.10° E). J. Geophys. Res. 2003, 108, 4595. [Google Scholar] [CrossRef]
- Kim, S.Y.; Chun, H.Y.; Wu, D.L. A study on stratospheric gravity waves generated by Typhoon Ewiniar: numerical simulations and satellite observations. J. Geophys. Res. 2009, 114, D22104. [Google Scholar] [CrossRef]
- Bindu, H.H.; Ratnam, M.V.; Yesubabu, V.; Rao, T.N.; Kesarkar, A.; Naidu, C.V. Characteristics of cyclone generated gravity waves observed using assimilated WRF model simulations over Bay of Bengal. Atmos. Res. 2016, 180, 178–188. [Google Scholar] [CrossRef]
- Sato, K. Small-scale wind disturbances observed by the MU radar during the passage of Typhoon Kelly. J. Atmos. Sci. 1993, 50, 518–537. [Google Scholar] [CrossRef]
- Sato, T.; Ao, N.; Yamamoto, M.; Fukao, S.; Tsuda, T.; Kato, S. A typhoon observed with the MU radar. Mon. Weath. Rev. 1991, 119, 755–768. [Google Scholar] [CrossRef]
- Wu, J.F.; Xue, X.H.; Liu, H.L.; Dou, X.K.; Chen, T. Assessment of the simulation of gravity waves generation by a tropical cyclone in the high-resolution WACCM and the WRF. J Adv. Model. Earth Syst. 2018, 10. [Google Scholar] [CrossRef]






| Region | Heights (km) | Number | λv (km) | λh (km) | T (h) | Phi (°) | Fup (%) | d (%) |
|---|---|---|---|---|---|---|---|---|
| 0 | 8–15 | 196 | 1.6 | 31 | 1.7 | 272 | 49 | 10 |
| 18–25 | 3.5 | 250 | 5.8 | 86 | 57 | 40 | ||
| 1 | 8–15 | 56 | 1.4 | 110 | 6.7 | 261 | 39 | 49 |
| 2 | 42 | 1.8 | 110 | 5.8 | 285 | 42 | 46 | |
| 3 | 49 | 1.8 | 79 | 3.9 | 64 | 54 | 30 | |
| 4 | 49 | 1.5 | 95 | 5.3 | 264 | 54 | 32 | |
| 1 | 18–25 | 56 | 3.5 (1.4) | 340 (140) | 7.4 | 62 | 60 | 38 |
| 2 | 42 | 3.5 | 220 | 4.8 | 68 | 62 | 72 | |
| 3 | 49 | 3.5 | 230 | 6.5 | 105 | 62 | 70 | |
| 4 | 49 | 3.5 | 290 | 5.3 | 109 | 53 | 16 | |
| A | 3–10 | 30 | 1.8 | 130 | 6.7 | 177 | 59 | 41 |
| 8–15 | 1.6 | 24 | 1.2 | 142 | 51 | 25 | ||
| 18–25 | 3.5 | 140 | 3.1 | 92 | 58 | 78 | ||
| 18–25 | 143 | 3.5 | 120 | 2.6 | 92 | 57 | 81 | |
| C | 3–10 | 54 | 2.4 | 310 | 11 | 220 | 62 | 36 |
| 8–15 | 1.8 | 53 | 2.5 | 154 | 45 | 62 | ||
| 18–25 | 3.5 | 150 | 3.5 | 106 | 60 | 89 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chane Ming, F.; Jolivet, S.; Liou, Y.-A.; Jégou, F.; Mekies, D.; Hong, J.-S. Elliptical Structures of Gravity Waves Produced by Typhoon Soudelor in 2015 near Taiwan. Atmosphere 2019, 10, 260. https://doi.org/10.3390/atmos10050260
Chane Ming F, Jolivet S, Liou Y-A, Jégou F, Mekies D, Hong J-S. Elliptical Structures of Gravity Waves Produced by Typhoon Soudelor in 2015 near Taiwan. Atmosphere. 2019; 10(5):260. https://doi.org/10.3390/atmos10050260
Chicago/Turabian StyleChane Ming, Fabrice, Samuel Jolivet, Yuei-An Liou, Fabrice Jégou, Dominique Mekies, and Jing-Shan Hong. 2019. "Elliptical Structures of Gravity Waves Produced by Typhoon Soudelor in 2015 near Taiwan" Atmosphere 10, no. 5: 260. https://doi.org/10.3390/atmos10050260
APA StyleChane Ming, F., Jolivet, S., Liou, Y.-A., Jégou, F., Mekies, D., & Hong, J.-S. (2019). Elliptical Structures of Gravity Waves Produced by Typhoon Soudelor in 2015 near Taiwan. Atmosphere, 10(5), 260. https://doi.org/10.3390/atmos10050260







