Selection of an Optimal Distribution Curve for Non-Stationary Flood Series
Abstract
1. Introduction
2. Study Area and Data
3. Methodology
3.1. Probability Distribution
3.2. Trend Model
3.3. Parameter Estimation
3.4. Selection of Optimal Model
4. Results
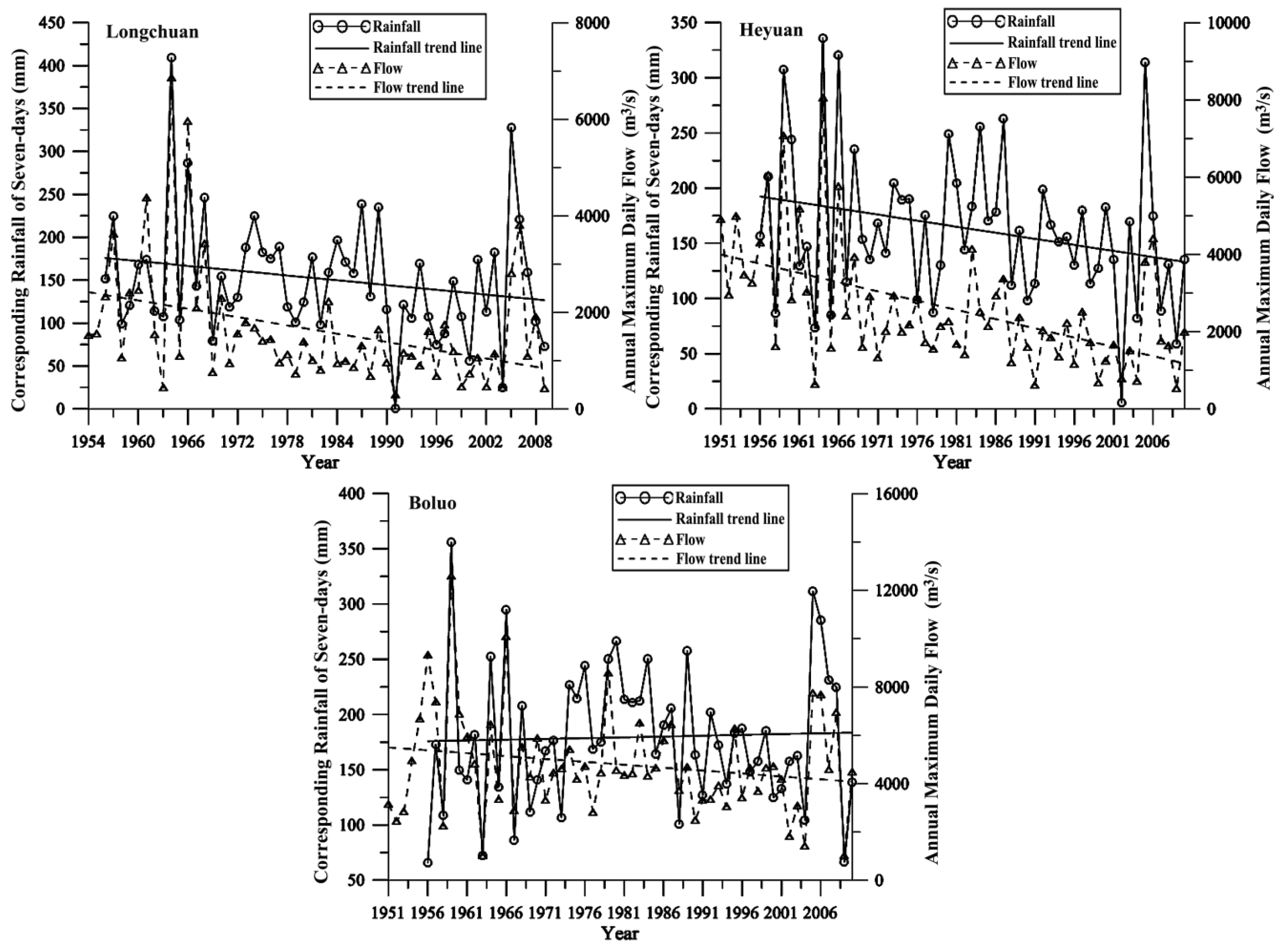
4.1. Non-Stationarity Detection in Flood Series
4.2. Selection of Optimal TVM Model
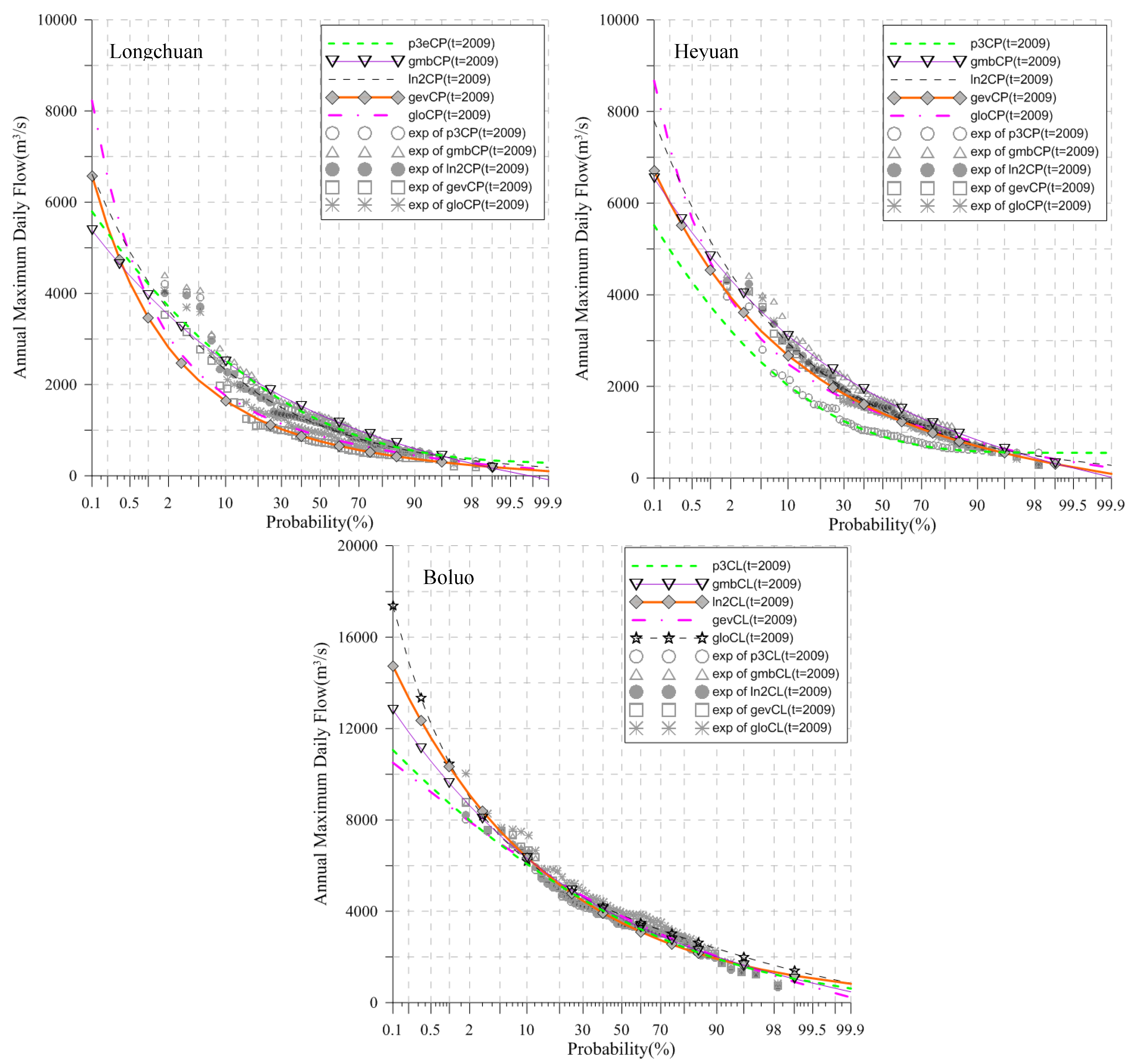
4.2.1. Selection of Optimal Distribution
4.2.2. Selection of Optimal Trend Model
4.3. Comparison of Return Period Obtained from TVM Model and Traditional Methods
5. Discussion
6. Conclusions
Author Contributions
Funding
Acknowledgment
Conflicts of Interest
References
- Liu, J.; Wang, S.-Y.; Li, D.-M. The analysis of the impact of land-use changes on flood exposure of wuhan in yangtze river basin, China. Water Resour. Manag. 2014, 28, 2507–2522. [Google Scholar] [CrossRef]
- Schiermeier, Q. Increased flood risk linked to global warming. Nature 2011, 470, 315. [Google Scholar] [CrossRef] [PubMed]
- Barnett, T.P.; Pierce, D.W.; Hidalgo, H.G.; Bonfils, C.; Santer, B.D.; Das, T.; Bala, G.; Wood, A.W.; Nozawa, T.; Mirin, A.A.; et al. Human-induced changes in the hydrology of the western united states. Science 2008, 319, 1080–1083. [Google Scholar] [CrossRef] [PubMed]
- Chen, X.; Tu, X.; Xie, P.; Li, Y. Progresses in the research of human induced variability of hydrological elements. Adv. Earth Sci. 2010, 25, 800–811. [Google Scholar]
- Milly, P.C.D.; Betancourt, J.; Falkenmark, M.; Hirsch, R.M.; Kundzewicz, Z.W.; Lettenmaier, D.P.; Stouffer, R.J. Climate change. Stationarity is dead: Whither water management? Science 2008, 319, 573–574. [Google Scholar] [CrossRef] [PubMed]
- Xiong, L.; Du, T.; Xu, C.-Y.; Guo, S.; Jiang, C.; Gippel, C.J. Non-stationary annual maximum flood frequency analysis using the norming constants method to consider non-stationarity in the annual daily flow series. Water Resour. Manag. 2015, 29, 3615–3633. [Google Scholar] [CrossRef]
- Hassanzadeh, Y.; Abdi, A.; Talatahari, S.; Singh, V.P. Meta-heuristic algorithms for hydrologic frequency analysis. Water Resour. Manag. 2011, 25, 1855–1879. [Google Scholar] [CrossRef]
- Khaliq, M.N.; Ouarda, T.B.M.J.; Ondo, J.C.; Gachon, P.; Bobée, B. Frequency analysis of a sequence of dependent and/or non-stationary hydro-meteorological observations: A review. J. Hydrol. 2006, 329, 534–552. [Google Scholar] [CrossRef]
- De Paola, F.; Giugni, M.; Pugliese, F. A harmony-based calibration tool for urban drainage systems. Proc. Instit. Civ. Eng.-Water Manag. 2018, 171, 30–41. [Google Scholar] [CrossRef]
- Hounkpè, J.; Diekkrüger, B.; Badou, D.; Afouda, A. Non-stationary flood frequency analysis in the ouémé river basin, benin republic. Hydrology 2015, 2, 210–229. [Google Scholar] [CrossRef]
- Singh, V.P.; Wang, S.X.; Zhang, L. Frequency analysis of nonidentically distributed hydrologic flood data. J. Hydrol. 2005, 307, 175–195. [Google Scholar] [CrossRef]
- Strupczewski, W.G.; Singh, V.P.; Feluch, W. Non-stationary approach to at-site flood frequency modelling i. Maximum likelihood estimation. J. Hydrol. 2001, 248, 123–142. [Google Scholar] [CrossRef]
- Cunderlik, J.M.; Burn, D.H. Non-stationary pooled frequency analysis. J. Hydrol. 2003, 276, 210–223. [Google Scholar] [CrossRef]
- Eum, H.-I.; Vasan, A.; Simonovic, S.P. Integrated reservoir management system for flood risk assessment under climate change. Water Resour. Manag. 2012, 26, 3785–3802. [Google Scholar] [CrossRef]
- Zeng, H.; Feng, P.; Li, X. Reservoir flood routing considering the non-stationarity of flood series in north China. Water Resour. Manag. 2014, 28, 4273–4287. [Google Scholar] [CrossRef]
- Wei, Q.; Sun, C.; Wu, G.; Pan, L. Haihe river discharge to bohai bay, north China: Trends, climate, and human activities. Hydrol. Res. 2017, 48, 1058–1070. [Google Scholar] [CrossRef]
- Zhang, Q.; Gu, X.; Singh, V.P.; Xiao, M.; Chen, X. Evaluation of flood frequency under non-stationarity resulting from climate indices and reservoir indices in the east river basin, China. J. Hydrol. 2015, 527, 565–575. [Google Scholar] [CrossRef]
- Chen, P.-C.; Wang, Y.-H.; You, G.J.-Y.; Wei, C.-C. Comparison of methods for non-stationary hydrologic frequency analysis: Case study using annual maximum daily precipitation in taiwan. J. Hydrol. 2017, 545, 197–211. [Google Scholar] [CrossRef]
- Diehl, T.; Potter, K.W. Mixed flood distributions in wisconsin. In Hydrologic Frequency Modeling; Singh, V.P., Ed.; D. Reidel Publishing Company: Dordrecht, The Netherlands, 1987; pp. 213–226. [Google Scholar]
- Katz, R.W.; Parlange, M.B.; Naveau, P. Statistics of extremes in hydrology. Adv. Water Resour. 2002, 25, 1287–1304. [Google Scholar] [CrossRef]
- Xiong, L.; Jiang, C.; Du, T. Statistical attribution analysis of the nonstationarity of the annual runoff series of the weihe river. Water Sci. Technol. 2014, 70, 939–946. [Google Scholar] [CrossRef]
- Vogel, R.M.; Yaindl, C.; Walter, M. Nonstationarity: Flood magnification and recurrence reduction factors in the united states. J. Am. Water Resour. Assoc. 2011, 47, 464–474. [Google Scholar] [CrossRef]
- Villarini, G.; Smith, J.A.; Serinaldi, F.; Bales, J.; Bates, P.D.; Krajewski, W.F. Flood frequency analysis for nonstationary annual peak records in an urban drainage basin. Adv. Water Resour. 2009, 32, 1255–1266. [Google Scholar] [CrossRef]
- Cox, D.R.; Isham, V.S.; Northrop, P.J. Floods: Some probabilistic and statistical approaches. Philos. Trans. Ser. A 2002, 360, 1389–1408. [Google Scholar] [CrossRef] [PubMed]
- Vasiliades, L.; Galiatsatou, P.; Loukas, A. Nonstationary frequency analysis of annual maximum rainfall using climate covariates. Water Resour. Manag. 2015, 29, 339–358. [Google Scholar] [CrossRef]
- Tu, X.; Chen, X.; Zhang, Q. Spatial-temporal variability patterns of regional runoffs series and their root cause analysis, guangdong province. J. Lake Sci. 2011, 23, 104–111. [Google Scholar]
- Yang, L.; Scheffran, J.; Qin, H.; You, Q. Climate-related flood risks and urban responses in the pearl river delta, China. Reg. Environ. Chang. 2015, 15, 379–391. [Google Scholar] [CrossRef]
- Yang, L.E.; Chan, F.K.S.; Scheffran, J. Climate change, water management and stakeholder analysis in the dongjiang river basin in south China. Int. J. Water Resour. Dev. 2018, 34, 166–191. [Google Scholar] [CrossRef]
- Liu, F.; Yuan, L.; Yang, Q.; Ou, S.; Xie, L.; Cui, X. Hydrological responses to the combined influence of diverse human activities in the pearl river delta, China. Catena 2014, 113, 41–55. [Google Scholar] [CrossRef]
- Zhang, Q.; Gu, X.; Singh, V.P.; Xiao, M.; Xu, C.-Y. Stationarity of annual flood peaks during 1951–2010 in the pearl river basin, China. J. Hydrol. 2014, 519, 3263–3274. [Google Scholar] [CrossRef]
- Zhou, Y.; Lai, C.; Wang, Z.; Chen, X.; Zeng, Z.; Chen, J.; Bai, X. Quantitative evaluation of the impact of climate change and human activity on runoff change in the dongjiang river basin, China. Water 2018, 10, 571. [Google Scholar] [CrossRef]
- Yan, L.; Xiong, L.; Guo, S.; Xu, C.-Y.; Xia, J.; Du, T. Comparison of four nonstationary hydrologic design methods for changing environment. J. Hydrol. 2017, 551, 132–150. [Google Scholar] [CrossRef]
- Malamud, B.D.; Turcotte, D.L. The applicability of power-law frequency statistics to floods. J. Hydrol. 2006, 322, 168–180. [Google Scholar] [CrossRef]
- Hosking, J.R.M.; Wallis, J.R. Regional Frequency Analysis: An Approach Based on L-Moments; Cambridge University Press: New York, NY, USA, 1997; p. 15. [Google Scholar]
- Rasmussen, P.F.; Gautam, N. Alternative pwm-estimators of the gumbel distribution. J. Hydrol. 2003, 280, 265–271. [Google Scholar] [CrossRef]
- Mohammadpour, O.; Hassanzadeh, Y.; Khodadadi, A.; Saghafian, B. Selecting the best flood flow frequency model using multi-criteria group decision-making. Water Resour. Manag. 2014, 28, 3957–3974. [Google Scholar] [CrossRef]
- Yan, L.; Xiong, L.; Liu, D.; Hu, T.; Xu, C.-Y. Frequency analysis of nonstationary annual maximum flood series using the time-varying two-component mixture distributions. Hydrol. Process. 2017, 31, 69–89. [Google Scholar] [CrossRef]
- Rao, A.; Hamed, K.H. Flood Frequency Analysis; CRC Press: Boca Raton, FL, USA, 2000; pp. 10–12. [Google Scholar]
- Chen, X.; Shao, Q.; Xu, C.-Y.; Zhang, J.; Zhang, L.; Ye, C. Comparative study on the selection criteria for fitting flood frequency distribution models with emphasis on upper-tail behavior. Water 2017, 9, 320. [Google Scholar] [CrossRef]
- Yue, S.; Pilon, P.; Cavadias, G. Power of the mann–kendall and spearman’s rho tests for detecting monotonic trends in hydrological series. J. Hydrol. 2002, 259, 254–271. [Google Scholar] [CrossRef]
- Tucker, C.J.; Pinzon, J.E.; Brown, M.E.; Slayback, D.A.; Pak, E.W.; Mahoney, R.; Vermote, E.F.; El Saleous, N. An extended avhrr 8-km ndvi dataset compatible with modis and spot vegetation ndvi data. Int. J. Remote Sens. 2005, 26, 4485–4498. [Google Scholar] [CrossRef]
- Adlouni, S.E.; Bobée, B.; Ouarda, T.B.M.J. On the tails of extreme event distributions in hydrology. J. Hydrol. 2008, 355, 16–33. [Google Scholar] [CrossRef]
- Miller, J.D.; Kjeldsen, T.R.; Hannaford, J.; Morris, D.G. A hydrological assessment of the november 2009 floods in cumbria, uk. Hydrol. Res. 2013, 44, 180–197. [Google Scholar] [CrossRef]
- Ho, M.; Lall, U.; Allaire, M.; Devineni, N.; Kwon, H.H.; Pal, I.; Raff, D.; Wegner, D. The future role of dams in the united states of america. Water Resour. Res. 2017, 53, 982–998. [Google Scholar] [CrossRef]
- Liu, X.; Li, X.; Liu, L.; He, J.; Ai, B. An Innovative Method to Classify Remote-Sensing Images Using Ant Colony Optimization. Ieee Trans. Geosci. Remote Sens. 2008, 46, 4198–4208. [Google Scholar] [CrossRef]


| No. | Dam or Reservoir | River | Storage Capacity (mm) | Drainage Area (Km2) | Precipitations (mm) | Construction Period | Post-Dam Period | Stations Affected by Reservoirs |
|---|---|---|---|---|---|---|---|---|
| 1 | Fengshuba | Mainstream of East River | 366.7 | 5150 | 1584 | 1970–1974 | 1975–2009 | Longchuan, Heyuan, Boluo |
| 2 | Xinfengjiang | Mainstream of East River | 2421.6 | 5740 | 1774 | 1958–1962 | 1963–2009 | Heyuan, Boluo |
| 3 | Baipenzhu | Tributary of East River | 1425.2 | 856 | 1800 | 1959–1985 | 1986–2008 | Boluo |
| Distributions Curve | Probability Density Function | Relationships of Parameters and First Two Order Moments |
|---|---|---|
| P3 | ||
| GMB | ≈ 0.5772 | |
| LN2 | ||
| GEV | When k > 0, ; k < 0, | |
| GLO | When k > 0, ; k < 0, |
| Trend Model | Expressions of m and σ | Increase the Number of Parameters |
|---|---|---|
| AL | , | 1 |
| AP | , | 2 |
| BL | , | 1 |
| BP | , | 2 |
| CL | , | 1 |
| CP | , | 2 |
| DL | , | 2 |
| Hydrology Factors | Longchuan | Heyuan | Boluo |
|---|---|---|---|
| Rainfall | −1.51 | −1.66 | 0.13 |
| Flood Flow | −3.18 | −4.20 | −2.07 |
| NDVI | −0.84 | −1.59 | −1.87 |
| Station | Models | P3 | GMB | LN2 | GEV | GLO |
|---|---|---|---|---|---|---|
| Longchuan | S | 922.91 | 929.85 | 919.78 | 931.85 | 921.16 |
| AL | 967.64 | 935.06 | 916.17 | 971.20 | 920.17 | |
| AP | 927.06 | 942.68 | 915.48 | 949.12 | 949.16 | |
| BL | 937.52 | 937.99 | 921.20 | 937.28 | 951.26 | |
| BP | 942.02 | 959.21 | 925.19 | 993.95 | 963.78 | |
| CL | 915.96 | 920.79 | 912.24 | 917.98 | 917.83 | |
| CP | 912.29 | 913.41 | 910.35 | 917.02 | 917.08 | |
| DL | 936.18 | 970.27 | 913.86 | 976.37 | 956.49 | |
| Heyuan | S | 973.91 | 977.34 | 974.06 | 979.34 | 977.70 |
| AL | 999.86 | 979.69 | 984.38 | 975.14 | 977.12 | |
| AP | 961.82 | 989.61 | 965.56 | 980.24 | 971.54 | |
| BL | 993.84 | 986.11 | 987.88 | 979.38 | 982.98 | |
| BP | 978.18 | 987.86 | 979.76 | 986.22 | 984.92 | |
| CL | 961.58 | 961.16 | 962.20 | 964.50 | 969.09 | |
| CP | 960.12 | 959.05 | 962.01 | 960.65 | 968.62 | |
| DL | 988.55 | 976.81 | 969.74 | 969.31 | 1132.17 | |
| Boluo | S | 1019.10 | 1016.79 | 1021.36 | 1018.27 | 1017.31 |
| AL | 1017.97 | 1016.16 | 1021.29 | 1016.97 | 1023.94 | |
| AP | 1020.00 | 1017.34 | 1033.19 | 1021.44 | 1037.68 | |
| BL | 1021.13 | 1018.76 | 1023.52 | 1020.23 | 1022.15 | |
| BP | 1017.91 | 1016.85 | 1021.81 | 1016.26 | 1028.93 | |
| CL | 1016.91 | 1014.46 | 1019.27 | 1015.39 | 1020.39 | |
| CP | 1016.93 | 1016.43 | 1021.19 | 1017.26 | 1021.45 | |
| DL | 1018.40 | 1016.47 | 1021.22 | 1034.64 | 1050.87 |
| Station | Models | |||||||
|---|---|---|---|---|---|---|---|---|
| Longchuan | LN2AL | 1892.55 | −8.21 | — | 1110.57 | — | — | — |
| LN2AP | 2295.07 | −32.16 | 0.26 | 1119.22 | — | — | — | |
| LN2BL | 1692.87 | — | — | 1139.82 | 3.08 | — | — | |
| LN2BP | 1844.35 | — | — | 1423.57 | −1.74 | 0.03 | — | |
| LN2CL | 2288.90 | −23.36 | — | — | — | — | 0.66 | |
| LN2CP | 3131.67 | −93.81 | 1.10 | — | — | — | 0.63 | |
| LN2DL | 2286.70 | −23.33 | — | 1388.50 | −11.86 | — | — | |
| Heyuan | GMBAL | 2795.37 | −4.84 | — | 1288.05 | — | — | — |
| GMBAP | 4473.59 | −5.77 | -0.80 | 2277.80 | — | — | — | |
| GMBBL | 3063.94 | — | — | 1561.10 | 0.04 | — | — | |
| GMBBP | 2443.78 | — | — | 1090.45 | 7.51 | −0.09 | — | |
| GMBCL | 3898.94 | −44.41 | — | — | — | — | 0.51 | |
| GMBCP | 4731.66 | −119.83 | 1.21 | — | — | — | 0.51 | |
| GMBDL | 2804.29 | −7.25 | — | 1464.08 | −1.97 | — | — | |
| Boluo | GMBAL | 5456.37 | −18.99 | — | 2241.31 | — | — | — |
| GMBAP | 5030.22 | 31.34 | −0.92 | 2203.54 | — | — | — | |
| GMBBL | 4959.84 | — | — | 2275.73 | 0.66 | — | — | |
| GMBBP | 4838.27 | — | — | 2277.63 | −28.21 | 0.56 | — | |
| GMBCL | 5976.34 | −36.31 | — | — | — | — | 0.45 | |
| GMBCP | 5793.74 | −45.20 | 0.31 | — | — | — | 0.45 | |
| GMBDL | 6163.79 | −40.82 | — | 2801.16 | −17.63 | — | — |
| Station | Model | T = 10 | T = 20 | T = 30 | T = 50 | T = 100 |
|---|---|---|---|---|---|---|
| Longchuan | LN2 | 3043 | 3858 | 4365 | 5038 | 6020 |
| LN2CP (2009) | 2370 | 2926 | 3264 | 3707 | 4342 | |
| Difference degree (%) | 28.36 | 31.85 | 33.70 | 35.89 | 38.65 | |
| Heyuan | GMB | 4611 | 5460 | 5949 | 6560 | 7383 |
| GMBCP (2009) | 3009 | 3524 | 3821 | 4191 | 4691 | |
| Difference degree (%) | 53.24 | 54.94 | 55.69 | 56.53 | 57.39 | |
| Boluo | GMB | 7889 | 9170 | 9906 | 10827 | 12070 |
| GMBCL (2009) | 6258 | 7255 | 7828 | 8544 | 9510 | |
| Difference degree (%) | 26.06 | 26.40 | 26.55 | 26.72 | 26.92 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, X.; Ye, C.; Zhang, J.; Xu, C.; Zhang, L.; Tang, Y. Selection of an Optimal Distribution Curve for Non-Stationary Flood Series. Atmosphere 2019, 10, 31. https://doi.org/10.3390/atmos10010031
Chen X, Ye C, Zhang J, Xu C, Zhang L, Tang Y. Selection of an Optimal Distribution Curve for Non-Stationary Flood Series. Atmosphere. 2019; 10(1):31. https://doi.org/10.3390/atmos10010031
Chicago/Turabian StyleChen, Xiaohong, Changqing Ye, Jiaming Zhang, Chongyu Xu, Lijuan Zhang, and Yihan Tang. 2019. "Selection of an Optimal Distribution Curve for Non-Stationary Flood Series" Atmosphere 10, no. 1: 31. https://doi.org/10.3390/atmos10010031
APA StyleChen, X., Ye, C., Zhang, J., Xu, C., Zhang, L., & Tang, Y. (2019). Selection of an Optimal Distribution Curve for Non-Stationary Flood Series. Atmosphere, 10(1), 31. https://doi.org/10.3390/atmos10010031







