Correlation of Plasma Membrane Microviscosity and Cell Stiffness Revealed via Fluorescence-Lifetime Imaging and Atomic Force Microscopy
Abstract
1. Introduction
2. Materials and Methods
2.1. Cell Cultures
2.2. Measuring Membrane Microviscosity via FLIM Using a Molecular Rotor
2.3. Mechanical Measurements with AFM
2.4. Confocal Microscopy
2.5. ToF-SIMS
2.6. Statistics
3. Results
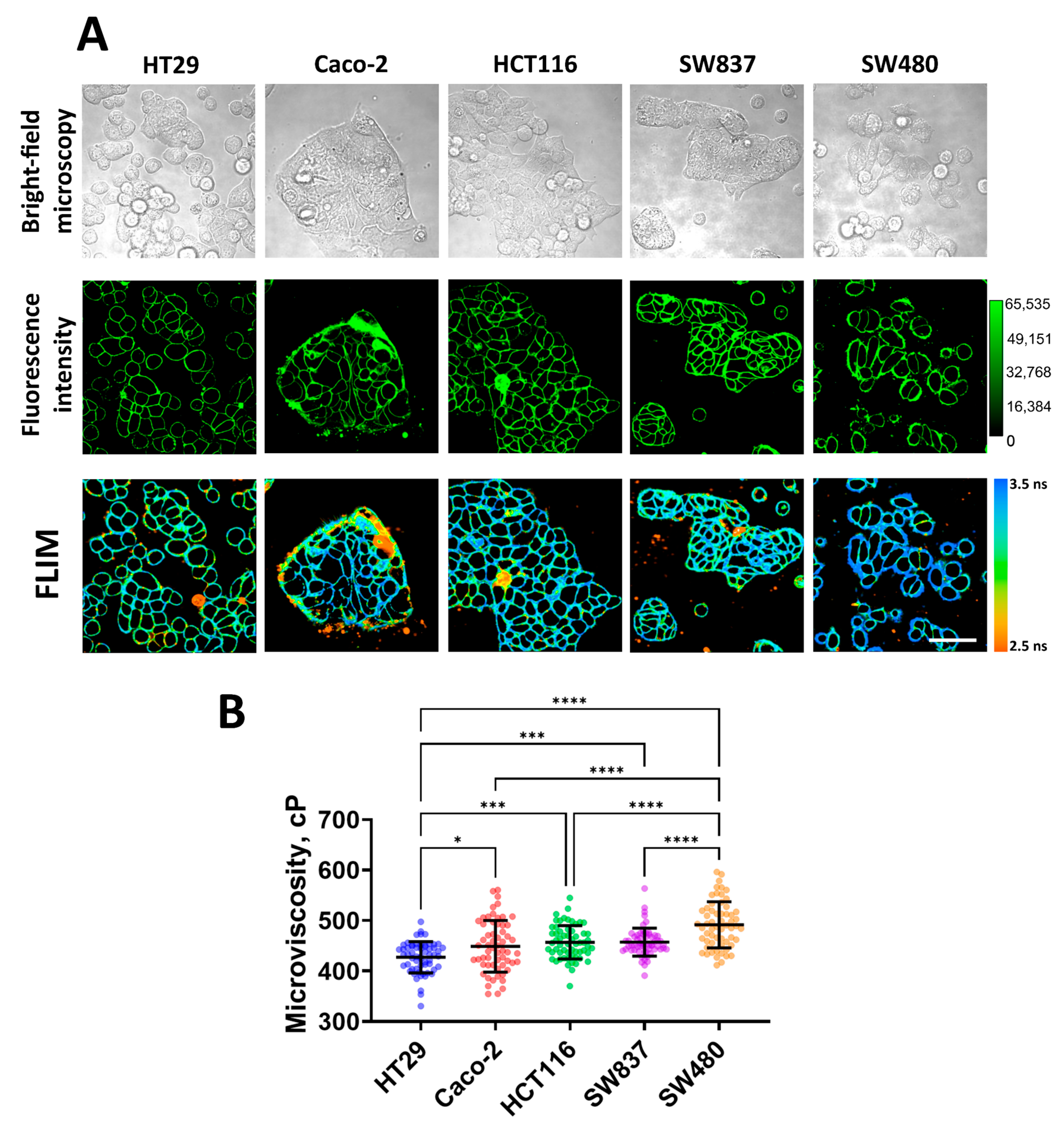
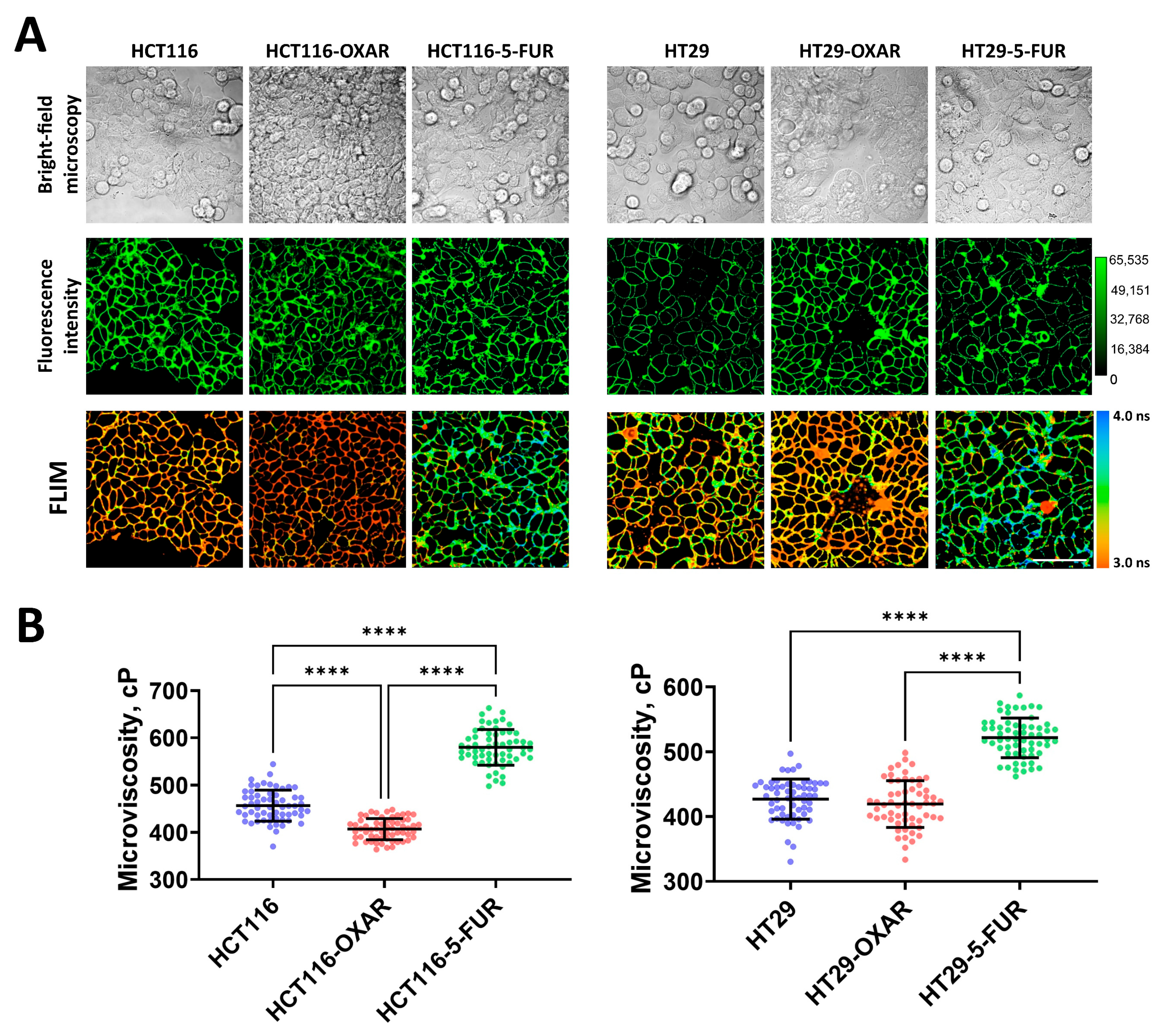
3.1. Measurement of Membrane Microviscosity of Colorectal Cancer Cell Lines via FLIM
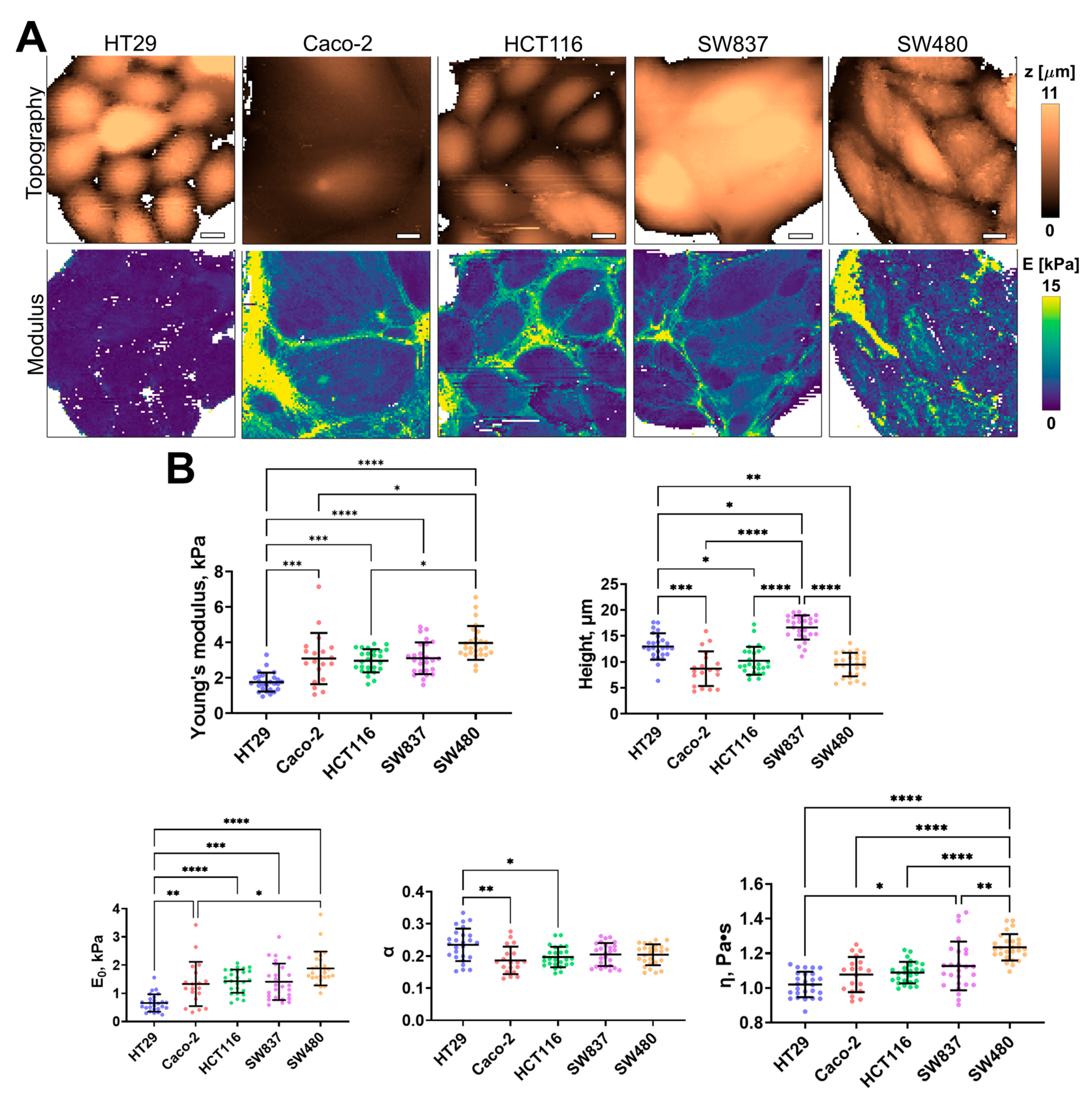
3.2. Assessment of Mechanical Properties of Colorectal Cancer Cell Lines via AFM
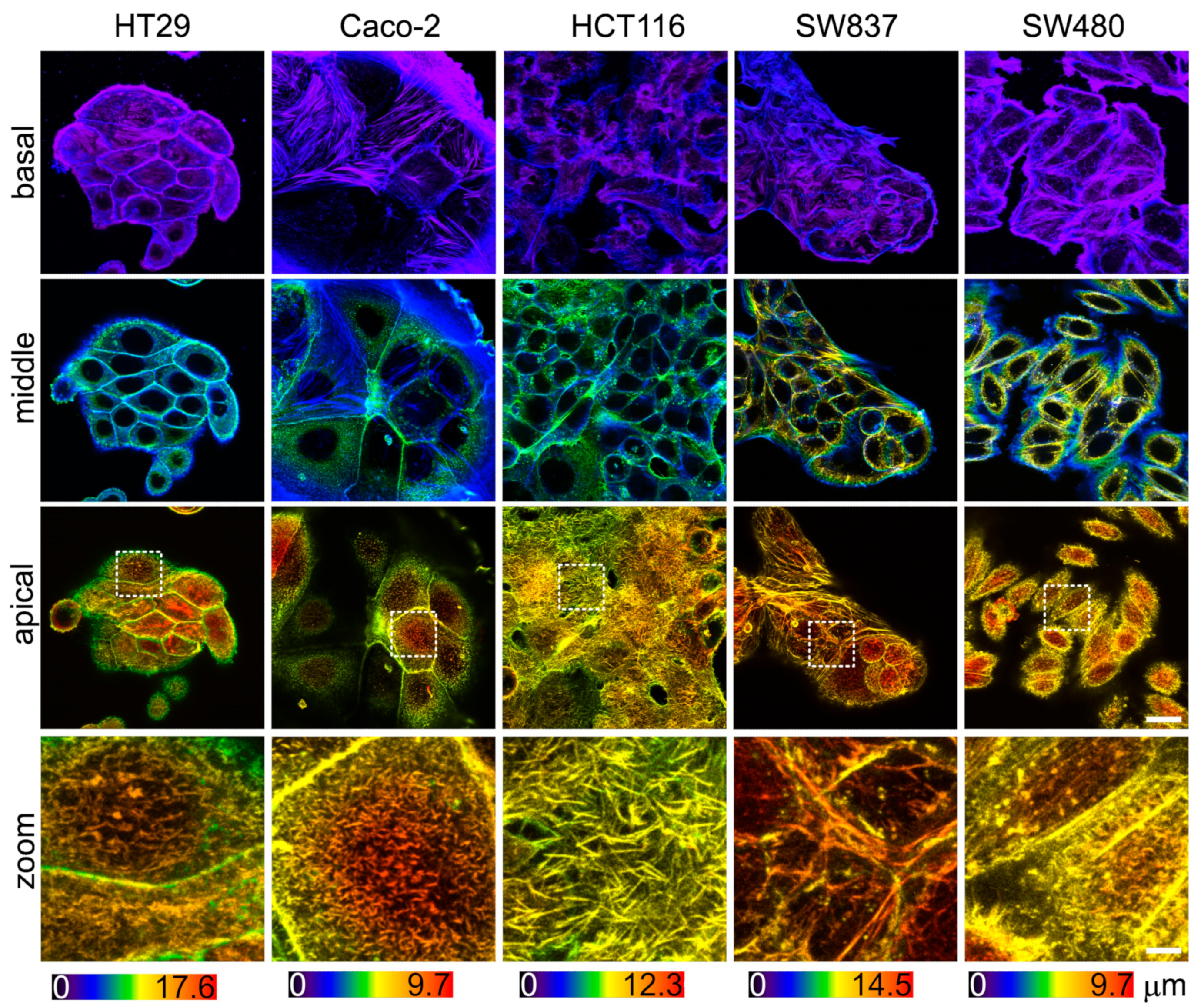
3.3. Actin Cytoskeleton of Colorectal Cancer Cell Lines Visualized via Confocal Microscopy
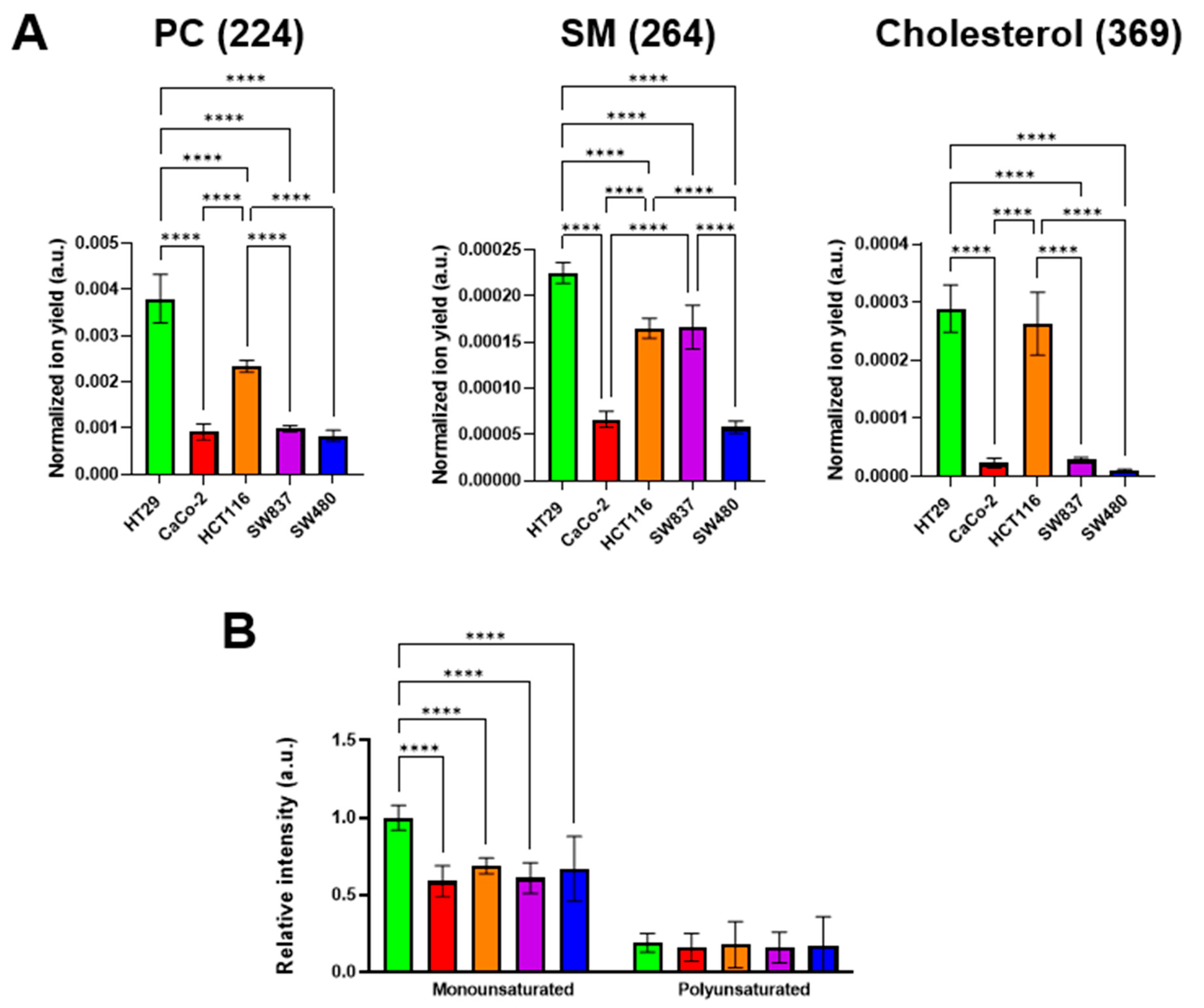
3.4. Assessment of Lipid Profile of Colorectal Cancer Cell Lines via ToF-SIMS
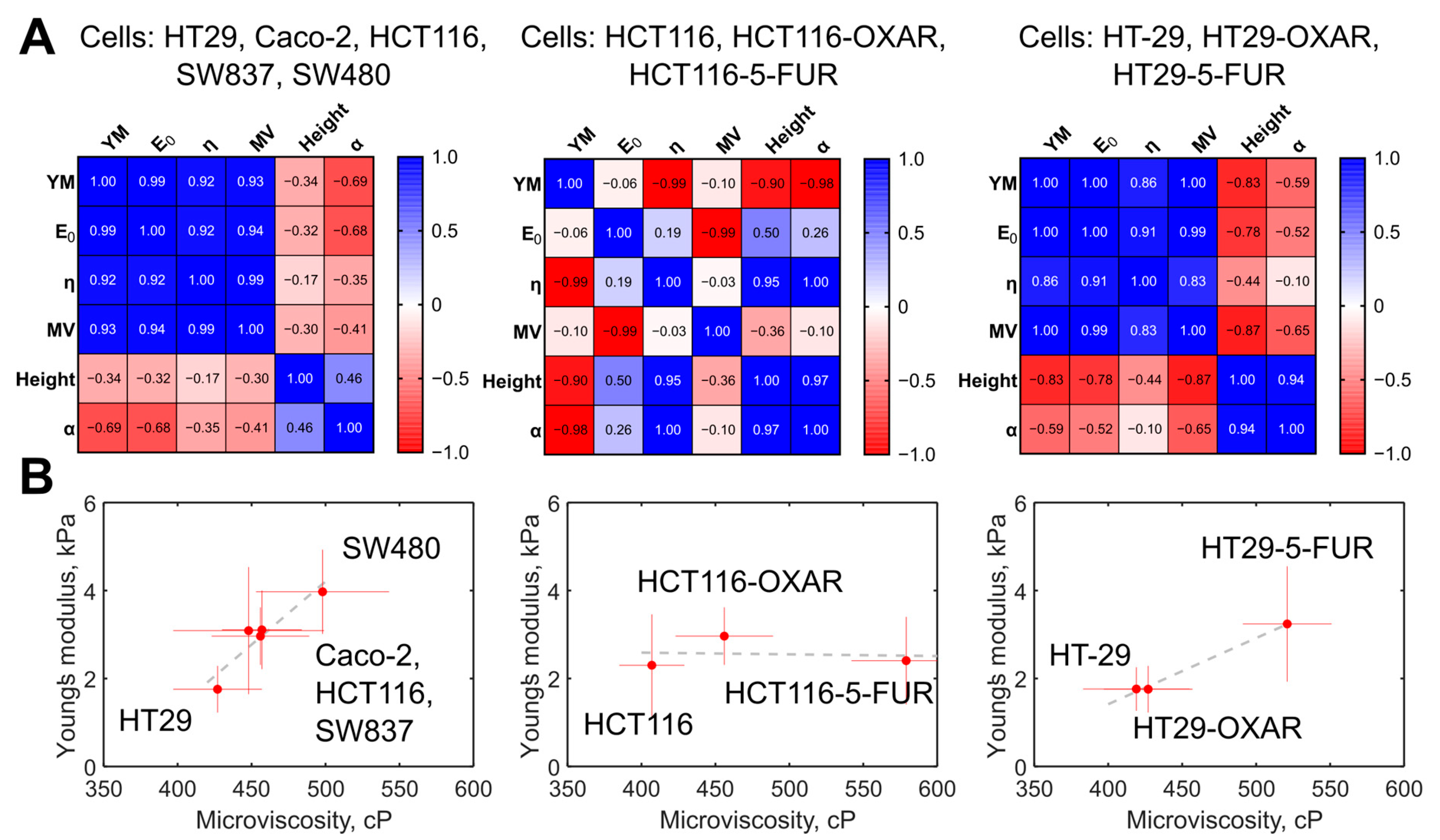
3.5. Comparison of Membrane Microviscosity and Cell Mechanical Properties of Colorectal Cancer Cell Lines
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lamparter, L.; Galic, M. Cellular Membranes, a Versatile Adaptive Composite Material. Front. Cell Dev. Biol. 2020, 8, 684. [Google Scholar] [CrossRef] [PubMed]
- Blanchard, G.J.; Busik, J.V. Interplay between Endothelial Cell Cytoskeletal Rigidity and Plasma Membrane Fluidity. Biophys. J. 2017, 112, 831–833. [Google Scholar] [CrossRef]
- Liebman, C.; McColloch, A.; Rabiei, M.; Bowling, A.; Cho, M. Mechanics of the Cell: Interaction Mechanisms and Mechanobiological Models. In Current Topics in Membranes; Elsevier Inc.: Amsterdam, The Netherlands, 2020; Volume 86, pp. 143–184. ISBN 978-0-12-821021-5. [Google Scholar]
- Galie, P.A.; Georges, P.C.; Janmey, P.A. How Do Cells Stiffen? Biochem. J. 2022, 479, 1825–1842. [Google Scholar] [CrossRef] [PubMed]
- Petersen, N.O.; McConnaughey, W.B.; Elson, E.L. Dependence of Locally Measured Cellular Deformability on Position on the Cell, Temperature, and Cytochalasin B. Proc. Natl. Acad. Sci. USA 1982, 79, 5327–5331. [Google Scholar] [CrossRef] [PubMed]
- Puig-De-Morales, M.; Grabulosa, M.; Alcaraz, J.; Mullol, J.; Maksym, G.N.; Fredberg, J.J.; Navajas, D. Measurement of Cell Microrheology by Magnetic Twisting Cytometry with Frequency Domain Demodulation. J. Appl. Physiol. 2001, 91, 1152–1159. [Google Scholar] [CrossRef] [PubMed]
- Qian, L.; Zhao, H. Nanoindentation of Soft Biological Materials. Micromachines 2018, 9, 654. [Google Scholar] [CrossRef] [PubMed]
- Pietuch, A.; Brückner, B.R.; Janshoff, A. Membrane Tension Homeostasis of Epithelial Cells through Surface Area Regulation in Response to Osmotic Stress. Biochim. Biophys. Acta-Mol. Cell Res. 2013, 1833, 712–722. [Google Scholar] [CrossRef]
- Heidemann, S.R.; Wirtz, D. Towards a Regional Approach to Cell Mechanics. Trends Cell Biol. 2004, 14, 160–166. [Google Scholar] [CrossRef]
- Dai, J.; Sheetz, M.P. Membrane Tether Formation from Blebbing Cells. Biophys. J. 1999, 77, 3363–3370. [Google Scholar] [CrossRef]
- Ayala, Y.A.; Pontes, B.; Hissa, B.; Monteiro, A.C.M.; Farina, M.; Moura-Neto, V.; Viana, N.B.; Nussenzveig, H.M. Effects of Cytoskeletal Drugs on Actin Cortex Elasticity. Exp. Cell Res. 2017, 351, 173–181. [Google Scholar] [CrossRef]
- Schneider, D.; Baronsky, T.; Pietuch, A.; Rother, J.; Oelkers, M.; Fichtner, D.; Wedlich, D.; Janshoff, A. Tension Monitoring during Epithelial-to-Mesenchymal Transition Links the Switch of Phenotype to Expression of Moesin and Cadherins in NMuMG Cells. PLoS ONE 2013, 8, e80068. [Google Scholar] [CrossRef] [PubMed]
- Köster, D.V. Pulling of Tethers from the Cell Plasma Membrane Using Optical Tweezers. In Caveolae; Blouin, C.M., Ed.; Methods in Molecular Biology; Springer: New York, NY, USA, 2020; Volume 2169, pp. 167–174. ISBN 978-1-07-160731-2. [Google Scholar]
- Pontes, B.; Viana, N.B.; Salgado, L.T.; Farina, M.; Neto, V.M.; Nussenzveig, H.M. Cell Cytoskeleton and Tether Extraction. Biophys. J. 2011, 101, 43–52. [Google Scholar] [CrossRef] [PubMed]
- Shi, Z.; Graber, Z.T.; Baumgart, T.; Stone, H.A.; Cohen, A.E. Cell Membranes Resist Flow. Cell 2018, 175, 1769–1779.e13. [Google Scholar] [CrossRef] [PubMed]
- Groves, J.T. Membrane Mechanics in Living Cells. Dev. Cell 2019, 48, 15–16. [Google Scholar] [CrossRef] [PubMed]
- Kelkar, M.; Bohec, P.; Charras, G. Mechanics of the Cellular Actin Cortex: From Signalling to Shape Change. Curr. Opin. Cell Biol. 2020, 66, 69–78. [Google Scholar] [CrossRef] [PubMed]
- Diz-Muñoz, A.; Weiner, O.D.; Fletcher, D.A. In Pursuit of the Mechanics That Shape Cell Surfaces. Nat. Phys. 2018, 14, 648–652. [Google Scholar] [CrossRef] [PubMed]
- Cordes, A.; Witt, H.; Gallemí-Pérez, A.; Brückner, B.; Grimm, F.; Vache, M.; Oswald, T.; Bodenschatz, J.; Flormann, D.; Lautenschläger, F.; et al. Prestress and Area Compressibility of Actin Cortices Determine the Viscoelastic Response of Living Cells. Phys. Rev. Lett. 2020, 125, 068101. [Google Scholar] [CrossRef]
- Efremov, Y.M.; Dokrunova, A.A.; Efremenko, A.V.; Kirpichnikov, M.P.; Shaitan, K.V.; Sokolova, O.S. Distinct Impact of Targeted Actin Cytoskeleton Reorganization on Mechanical Properties of Normal and Malignant Cells. Biochim. Biophys. Acta-Mol. Cell Res. 2015, 1853, 3117–3125. [Google Scholar] [CrossRef]
- Kwon, S.; Kim, K.S. Qualitative Analysis of Contribution of Intracellular Skeletal Changes to Cellular Elasticity. Cell. Mol. Life Sci. 2020, 77, 1345–1355. [Google Scholar] [CrossRef]
- Vakhrusheva, A.V.; Murashko, A.V.; Trifonova, E.S.; Efremov, Y.M.; Timashev, P.S.; Sokolova, O.S. Role of Actin-Binding Proteins in the Regulation of Cellular Mechanics. Eur. J. Cell Biol. 2022, 101, 151241. [Google Scholar] [CrossRef]
- Subczynski, W.K.; Pasenkiewicz-Gierula, M.; Widomska, J.; Mainali, L.; Raguz, M. High Cholesterol/Low Cholesterol: Effects in Biological Membranes: A Review. Cell Biochem. Biophys. 2017, 75, 369–385. [Google Scholar] [CrossRef]
- Doole, F.T.; Kumarage, T.; Ashkar, R.; Brown, M.F. Cholesterol Stiffening of Lipid Membranes. J. Membr. Biol. 2022, 255, 385–405. [Google Scholar] [CrossRef]
- Yang, X.; Sheng, W.; Sun, G.Y.; Lee, J.C.-M. Effects of Fatty Acid Unsaturation Numbers on Membrane Fluidity and α-Secretase-Dependent Amyloid Precursor Protein Processing. Neurochem. Int. 2011, 58, 321–329. [Google Scholar] [CrossRef] [PubMed]
- Holthuis, J.C.M.; Luberto, C. Tales and Mysteries of the Enigmatic Sphingomyelin Synthase Family. In Sphingolipids as Signaling and Regulatory Molecules; Chalfant, C., Poeta, M.D., Eds.; Advances in Experimental Medicine and Biology; Springer: New York, NY, USA, 2010; Volume 688, pp. 72–85. ISBN 978-1-4419-6740-4. [Google Scholar]
- Sitarska, E.; Diz-Muñoz, A. Pay Attention to Membrane Tension: Mechanobiology of the Cell Surface. Curr. Opin. Cell Biol. 2020, 66, 11–18. [Google Scholar] [CrossRef] [PubMed]
- Zemła, J.; Danilkiewicz, J.; Orzechowska, B.; Pabijan, J.; Seweryn, S.; Lekka, M. Atomic Force Microscopy as a Tool for Assessing the Cellular Elasticity and Adhesiveness to Identify Cancer Cells and Tissues. Semin. Cell Dev. Biol. 2018, 73, 115–124. [Google Scholar] [CrossRef] [PubMed]
- Lekka, M.; Pogoda, K.; Gostek, J.; Klymenko, O.; Prauzner-Bechcicki, S.; Wiltowska-Zuber, J.; Jaczewska, J.; Lekki, J.; Stachura, Z. Cancer Cell Recognition—Mechanical Phenotype. Micron 2012, 43, 1259–1266. [Google Scholar] [CrossRef] [PubMed]
- Li, Q.S.; Lee, G.Y.H.; Ong, C.N.; Lim, C.T. AFM Indentation Study of Breast Cancer Cells. Biochem. Biophys. Res. Commun. 2008, 374, 609–613. [Google Scholar] [CrossRef] [PubMed]
- Zeisig, R.; Koklič, T.; Wiesner, B.; Fichtner, I.; Sentjurč, M. Increase in Fluidity in the Membrane of MT3 Breast Cancer Cells Correlates with Enhanced Cell Adhesion In Vitro and Increased Lung Metastasis in NOD/SCID Mice. Arch. Biochem. Biophys. 2007, 459, 98–106. [Google Scholar] [CrossRef] [PubMed]
- Li, Q.; Zhu, W.; Gong, S.; Jiang, S.; Feng, G. Selective Visualization of Tumor Cell Membranes and Tumors with a Viscosity-Sensitive Plasma Membrane Probe. Anal. Chem. 2023, 95, 7254–7261. [Google Scholar] [CrossRef]
- Bompard, J.; Rosso, A.; Brizuela, L.; Mebarek, S.; Blum, L.J.; Trunfio-Sfarghiu, A.-M.; Lollo, G.; Granjon, T.; Girard-Egrot, A.; Maniti, O. Membrane Fluidity as a New Means to Selectively Target Cancer Cells with Fusogenic Lipid Carriers. Langmuir 2020, 36, 5134–5144. [Google Scholar] [CrossRef]
- Szlasa, W.; Zendran, I.; Zalesińska, A.; Tarek, M.; Kulbacka, J. Lipid Composition of the Cancer Cell Membrane. J. Bioenerg. Biomembr. 2020, 52, 321–342. [Google Scholar] [CrossRef] [PubMed]
- Nakazawa, I.; Iwaizumi, M. A Correlation between Cancer Metastases and the Fluidity of Cancer Cell Membrane. Tohoku J. Exp. Med. 1982, 137, 325–328. [Google Scholar] [CrossRef] [PubMed]
- Shimolina, L.E.; Gulin, A.A.; Paez-Perez, M.; López-Duarte, I.; Druzhkova, I.N.; Lukina, M.M.; Gubina, M.V.; Brooks, N.J.; Zagaynova, E.V.; Kuimova, M.K.; et al. Mapping Cisplatin-Induced Viscosity Alterations in Cancer Cells Using Molecular Rotor and Fluorescence Lifetime Imaging Microscopy. J. Biomed. Opt. 2020, 25, 126004. [Google Scholar] [CrossRef] [PubMed]
- Dallas, N.A.; Xia, L.; Fan, F.; Gray, M.J.; Gaur, P.; Van Buren, G.; Samuel, S.; Kim, M.P.; Lim, S.J.; Ellis, L.M. Chemoresistant Colorectal Cancer Cells, the Cancer Stem Cell Phenotype, and Increased Sensitivity to Insulin-like Growth Factor-I Receptor Inhibition. Cancer Res. 2009, 69, 1951–1957. [Google Scholar] [CrossRef]
- Shimolina, L.; Gulin, A.; Ignatova, N.; Druzhkova, I.; Gubina, M.; Lukina, M.; Snopova, L.; Zagaynova, E.; Kuimova, M.K.; Shirmanova, M. The Role of Plasma Membrane Viscosity in the Response and Resistance of Cancer Cells to Oxaliplatin. Cancers 2021, 13, 6165. [Google Scholar] [CrossRef] [PubMed]
- Shimolina, L.; Gulin, A.; Khlynova, A.; Ignatova, N.; Druzhkova, I.; Gubina, M.; Zagaynova, E.; Kuimova, M.K.; Shirmanova, M. Development of Resistance to 5-Fluorouracil Affects Membrane Viscosity and Lipid Composition of Cancer Cells. Methods Appl. Fluoresc. 2022, 10, 044008. [Google Scholar] [CrossRef] [PubMed]
- Dent, M.R.; López-Duarte, I.; Dickson, C.J.; Geoghegan, N.D.; Cooper, J.M.; Gould, I.R.; Krams, R.; Bull, J.A.; Brooks, N.J.; Kuimova, M.K. Imaging Phase Separation in Model Lipid Membranes through the Use of BODIPY Based Molecular Rotors. Phys. Chem. Chem. Phys. 2015, 17, 18393–18402. [Google Scholar] [CrossRef] [PubMed]
- Shimolina, L.E.; Izquierdo, M.A.; López-Duarte, I.; Bull, J.A.; Shirmanova, M.V.; Klapshina, L.G.; Zagaynova, E.V.; Kuimova, M.K. Imaging Tumor Microscopic Viscosity In Vivo Using Molecular Rotors. Sci. Rep. 2017, 7, 41097. [Google Scholar] [CrossRef]
- Shimolina, L.; Lukina, M.; Shcheslavskiy, V.; Elagin, V.; Dudenkova, V.; Ignatova, N.; Kuimova, M.K.; Shirmanova, M. Probing Metabolism and Viscosity of Cancer Cells Using Fluorescence Lifetime Imaging Microscopy. JoVE 2021, 173, 62708. [Google Scholar] [CrossRef]
- Hosny, N.A.; Mohamedi, G.; Rademeyer, P.; Owen, J.; Wu, Y.; Tang, M.-X.; Eckersley, R.J.; Stride, E.; Kuimova, M.K. Mapping Microbubble Viscosity Using Fluorescence Lifetime Imaging of Molecular Rotors. Proc. Natl. Acad. Sci. USA 2013, 110, 9225–9230. [Google Scholar] [CrossRef]
- Schillers, H.; Rianna, C.; Schäpe, J.; Luque, T.; Doschke, H.; Wälte, M.; Uriarte, J.J.; Campillo, N.; Michanetzis, G.P.A.; Bobrowska, J.; et al. Standardized Nanomechanical Atomic Force Microscopy Procedure (SNAP) for Measuring Soft and Biological Samples. Sci. Rep. 2017, 7, 5117. [Google Scholar] [CrossRef] [PubMed]
- Efremov, Y.M.; Shpichka, A.I.; Kotova, S.L.; Timashev, P.S. Viscoelastic Mapping of Cells Based on Fast Force Volume and PeakForce Tapping. Soft Matter 2019, 15, 5455–5463. [Google Scholar] [CrossRef] [PubMed]
- Efremov, Y.M.; Wang, W.-H.; Hardy, S.D.; Geahlen, R.L.; Raman, A. Measuring Nanoscale Viscoelastic Parameters of Cells Directly from AFM Force-Displacement Curves. Sci. Rep. 2017, 7, 1541. [Google Scholar] [CrossRef] [PubMed]
- Ting, T.C.T. The Contact Stresses between a Rigid Indenter and a Viscoelastic Half-Space. J. Appl. Mech. 1966, 33, 845–854. [Google Scholar] [CrossRef]
- Garcia, P.D.; Garcia, R. Determination of the Elastic Moduli of a Single Cell Cultured on a Rigid Support by Force Microscopy. Biophys. J. 2018, 114, 2923–2932. [Google Scholar] [CrossRef] [PubMed]
- Kollmannsberger, P.; Fabry, B. Active Soft Glassy Rheology of Adherent Cells. Soft Matter 2009, 5, 1771–1774. [Google Scholar] [CrossRef]
- Efremov, Y.M.; Okajima, T.; Raman, A. Measuring Viscoelasticity of Soft Biological Samples Using Atomic Force Microscopy. Soft Matter 2020, 16, 64–81. [Google Scholar] [CrossRef]
- Fabry, B.; Maksym, G.; Butler, J.; Glogauer, M.; Navajas, D.; Taback, N.; Millet, E.; Fredberg, J. Time Scale and Other Invariants of Integrative Mechanical Behavior in Living Cells. Phys. Rev. E 2003, 68, 041914. [Google Scholar] [CrossRef]
- Kollmannsberger, P.; Mierke, C.T.; Fabry, B. Nonlinear Viscoelasticity of Adherent Cells Is Controlled by Cytoskeletal Tension. Soft Matter 2011, 7, 3127. [Google Scholar] [CrossRef]
- Sanchez, J.G.; Espinosa, F.M.; Miguez, R.; Garcia, R. The Viscoelasticity of Adherent Cells Follows a Single Power-Law with Distinct Local Variations within a Single Cell and across Cell Lines. Nanoscale 2021, 13, 16339–16348. [Google Scholar] [CrossRef]
- Paez-Perez, M.; Kuimova, M. Molecular Rotors: Fluorescent Sensors for Microviscosity and Conformation of Biomolecules. Angew. Chem. Int. Ed. 2023, e202311233. [Google Scholar] [CrossRef]
- Druzhkova, I.; Shirmanova, M.; Ignatova, N.; Dudenkova, V.; Lukina, M.; Zagaynova, E.; Safina, D.; Kostrov, S.; Didych, D.; Kuzmich, A.; et al. Expression of EMT-Related Genes in Hybrid E/M Colorectal Cancer Cells Determines Fibroblast Activation and Collagen Remodeling. Int. J. Mol. Sci. 2020, 21, 8119. [Google Scholar] [CrossRef] [PubMed]
- Yubero, M.L.; Kosaka, P.M.; San Paulo, Á.; Malumbres, M.; Calleja, M.; Tamayo, J. Effects of Energy Metabolism on the Mechanical Properties of Breast Cancer Cells. Commun. Biol. 2020, 3, 590. [Google Scholar] [CrossRef] [PubMed]
- Flormann, D.A.D.; Anton, C.; Pohland, M.O.; Bautz, Y.; Kaub, K.; Terriac, E.; Schäffer, T.E.; Rheinlaender, J.; Janshoff, A.; Ott, A.; et al. Oscillatory Microrheology, Creep Compliance and Stress Relaxation of Biological Cells Reveal Strong Correlations as Probed by Atomic Force Microscopy. Front. Phys. 2021, 9, 711860. [Google Scholar] [CrossRef]
- Lin, H.H.; Lin, H.K.; Lin, I.H.; Chiou, Y.W.; Chen, H.W.; Liu, C.Y.; Harn, H.I.C.; Chiu, W.T.; Wang, Y.K.; Shen, M.R.; et al. Mechanical Phenotype of Cancer Cells: Cell Softening and Loss of Stiffness Sensing. Oncotarget 2015, 6, 20946–20958. [Google Scholar] [CrossRef] [PubMed]
- Hąc-Wydro, K.; Wydro, P. The Influence of Fatty Acids on Model Cholesterol/Phospholipid Membranes. Chem. Phys. Lipids 2007, 150, 66–81. [Google Scholar] [CrossRef] [PubMed]
- Efremov, Y.M.; Velay-Lizancos, M.; Weaver, C.J.; Athamneh, A.I.; Zavattieri, P.D.; Suter, D.M.; Raman, A. Anisotropy vs. Isotropy in Living Cell Indentation with AFM. Sci. Rep. 2019, 9, 5757. [Google Scholar] [CrossRef]
- Al-Rekabi, Z.; Haase, K.; Pelling, A.E. Microtubules Mediate Changes in Membrane Cortical Elasticity during Contractile Activation. Exp. Cell Res. 2014, 322, 21–29. [Google Scholar] [CrossRef]
- Kihara, T.; Haghparast, S.M.A.; Shimizu, Y.; Yuba, S.; Miyake, J. Physical Properties of Mesenchymal Stem Cells Are Coordinated by the Perinuclear Actin Cap. Biochem. Biophys. Res. Commun. 2011, 409, 1–6. [Google Scholar] [CrossRef]
- Alibert, C.; Goud, B.; Manneville, J.-B. Are Cancer Cells Really Softer than Normal Cells? Biol. Cell 2017, 109, 167–189. [Google Scholar] [CrossRef]
- Li, M.; Dang, D.; Liu, L.; Xi, N.; Wang, Y. Atomic Force Microscopy in Characterizing Cell Mechanics for Biomedical Applications: A Review. IEEE Trans. NanoBiosci. 2017, 16, 523–540. [Google Scholar] [CrossRef] [PubMed]
- Swaminathan, V.; Mythreye, K.; O’Brien, E.T.; Berchuck, A.; Blobe, G.C.; Superfine, R. Mechanical Stiffness Grades Metastatic Potential in Patient Tumor Cells and in Cancer Cell Lines. Cancer Res. 2011, 71, 5075–5080. [Google Scholar] [CrossRef] [PubMed]
- Xu, W.; Mezencev, R.; Kim, B.; Wang, L.; McDonald, J.; Sulchek, T. Cell Stiffness Is a Biomarker of the Metastatic Potential of Ovarian Cancer Cells. PLoS ONE 2012, 7, e46609. [Google Scholar] [CrossRef] [PubMed]
- Watanabe, T.; Kuramochi, H.; Takahashi, A.; Imai, K.; Katsuta, N.; Nakayama, T.; Fujiki, H.; Suganuma, M. Higher Cell Stiffness Indicating Lower Metastatic Potential in B16 Melanoma Cell Variants and in (−)-Epigallocatechin Gallate-Treated Cells. J. Cancer Res. Clin. Oncol. 2012, 138, 859–866. [Google Scholar] [CrossRef] [PubMed]
- Molter, C.W.; Muszynski, E.F.; Tao, Y.; Trivedi, T.; Clouvel, A.; Ehrlicher, A.J. Prostate Cancer Cells of Increasing Metastatic Potential Exhibit Diverse Contractile Forces, Cell Stiffness, and Motility in a Microenvironment Stiffness-Dependent Manner. Front. Cell Dev. Biol. 2022, 10, 932510. [Google Scholar] [CrossRef] [PubMed]
- Staunton, J.R.; Doss, B.L.; Lindsay, S.; Ros, R. Correlating Confocal Microscopy and Atomic Force Indentation Reveals Metastatic Cancer Cells Stiffen during Invasion into Collagen I Matrices. Sci. Rep. 2016, 6, 19686. [Google Scholar] [CrossRef]
- Zhang, G.; Long, M.; Wu, Z.-Z.; Yu, W.-Q. Mechanical Properties of Hepatocellular Carcinoma Cells. World J. Gastroenterol. 2002, 8, 243–246. [Google Scholar] [CrossRef]
- Faria, E.C.; Ma, N.; Gazi, E.; Gardner, P.; Brown, M.; Clarke, N.W.; Snook, R.D. Measurement of Elastic Properties of Prostate Cancer Cells Using AFM. Analyst 2008, 133, 1498–1500. [Google Scholar] [CrossRef]
- Hanahan, D.; Weinberg, R. A Hallmarks of Cancer: The next Generation. Cell 2011, 144, 646–674. [Google Scholar] [CrossRef]
- Kaur, J.; Sanyal, S.N. Alterations in Membrane Fluidity and Dynamics in Experimental Colon Cancer and Its Chemoprevention by Diclofenac. Mol. Cell Biochem. 2010, 341, 99–108. [Google Scholar] [CrossRef]
- Angelucci, C.; Maulucci, G.; Colabianchi, A.; Iacopino, F.; D’Alessio, A.; Maiorana, A.; Palmieri, V.; Papi, M.; De Spirito, M.; Di Leone, A.; et al. Stearoyl-CoA Desaturase 1 and Paracrine Diffusible Signals Have a Major Role in the Promotion of Breast Cancer Cell Migration Induced by Cancer-Associated Fibroblasts. Br. J. Cancer 2015, 112, 1675–1686. [Google Scholar] [CrossRef]
- Angelucci, C.; Maulucci, G.; Lama, G.; Proietti, G.; Colabianchi, A.; Papi, M.; Maiorana, A.; De Spirito, M.; Micera, A.; Balzamino, O.B.; et al. Epithelial-Stromal Interactions in Human Breast Cancer: Effects on Adhesion, Plasma Membrane Fluidity and Migration Speed and Directness. PLoS ONE 2012, 7, e50804. [Google Scholar] [CrossRef]
- Tsai, J.; Kam, L.C. Lateral Mobility of E-Cadherin Enhances Rac1 Response in Epithelial Cells. Cell. Mol. Bioeng. 2010, 3, 84–90. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Tabdanov, E.; Borghi, N.; Brochard-Wyart, F.; Dufour, S.; Thiery, J.-P. Role of E-Cadherin in Membrane-Cortex Interaction Probed by Nanotube Extrusion. Biophys. J. 2009, 96, 2457–2465. [Google Scholar] [CrossRef] [PubMed]
- Ozawa, M.; Kemler, R. The Membrane-Proximal Region of the E-Cadherin Cytoplasmic Domain Prevents Dimerization and Negatively Regulates Adhesion Activity. J. Cell Biol. 1998, 142, 1605–1613. [Google Scholar] [CrossRef] [PubMed]
- Mohammadalipour, A.; Showalter, C.A.; Muturi, H.T.; Farnoud, A.M.; Najjar, S.M.; Burdick, M.M. Cholesterol Depletion Decreases Adhesion of Non-Small Cell Lung Cancer Cells to E-Selectin. Am. J. Physiol.-Cell Physiol. 2023, 325, C471–C482. [Google Scholar] [CrossRef] [PubMed]
- Mierke, C.T. The Integrin Alphav Beta3 Increases Cellular Stiffness and Cytoskeletal Remodeling Dynamics to Facilitate Cancer Cell Invasion. New J. Phys. 2013, 15, 015003. [Google Scholar] [CrossRef][Green Version]
- Gopalakrishna, P.; Chaubey, S.K.; Manogaran, P.S.; Pande, G. Modulation of Alpha5beta1 Integrin Functions by the Phospholipid and Cholesterol Contents of Cell Membranes. J. Cell Biochem. 2000, 77, 517–528. [Google Scholar] [CrossRef]
- Ohmori, H.; Fujii, K.; Kadochi, Y.; Mori, S.; Nishiguchi, Y.; Fujiwara, R.; Kishi, S.; Sasaki, T.; Kuniyasu, H. Elaidic Acid, a Trans-Fatty Acid, Enhances the Metastasis of Colorectal Cancer Cells. Pathobiology 2017, 84, 144–151. [Google Scholar] [CrossRef]
- Kishi, S.; Fujiwara-Tani, R.; Luo, Y.; Kawahara, I.; Goto, K.; Fujii, K.; Ohmori, H.; Nakashima, C.; Sasaki, T.; Kuniyasu, H. Pro-metastatic Signaling of the Trans Fatty Acid Elaidic Acid Is Associated with Lipid Rafts. Oncol. Lett. 2018, 15, 4423–4426. [Google Scholar] [CrossRef]
- Pakiet, A.; Sikora, K.; Kobiela, J.; Rostkowska, O.; Mika, A.; Sledzinski, T. Alterations in Complex Lipids in Tumor Tissue of Patients with Colorectal Cancer. Lipids Health Dis. 2021, 20, 85. [Google Scholar] [CrossRef] [PubMed]
- Mika, A.; Pakiet, A.; Czumaj, A.; Kaczynski, Z.; Liakh, I.; Kobiela, J.; Perdyan, A.; Adrych, K.; Makarewicz, W.; Sledzinski, T. Decreased Triacylglycerol Content and Elevated Contents of Cell Membrane Lipids in Colorectal Cancer Tissue: A Lipidomic Study. J. Clin. Med. 2020, 9, 1095. [Google Scholar] [CrossRef] [PubMed]
- Casares, D.; Escribá, P.V.; Rosselló, C.A. Membrane Lipid Composition: Effect on Membrane and Organelle Structure, Function and Compartmentalization and Therapeutic Avenues. Int. J. Mol. Sci. 2019, 20, 2167. [Google Scholar] [CrossRef] [PubMed]
- Martinac, B.; Nikolaev, Y.A.; Silvani, G.; Bavi, N.; Romanov, V.; Nakayama, Y.; Martinac, A.D.; Rohde, P.; Bavi, O.; Cox, C.D. Cell Membrane Mechanics and Mechanosensory Transduction. In Current Topics in Membranes; Elsevier: Amsterdam, The Netherlands, 2020; Volume 86, pp. 83–141. ISBN 978-0-12-821021-5. [Google Scholar]
- Sheetz, M.P. Cell Control by Membrane–Cytoskeleton Adhesion. Nat. Rev. Mol. Cell Biol. 2001, 2, 392–396. [Google Scholar] [CrossRef] [PubMed]
- Paraschiv, A.; Lagny, T.J.; Campos, C.V.; Coudrier, E.; Bassereau, P.; Šarić, A. Influence of Membrane-Cortex Linkers on the Extrusion of Membrane Tubes. Biophys. J. 2021, 120, 598–606. [Google Scholar] [CrossRef] [PubMed]
- Guo, H.-L.; Liu, C.-X.; Duan, J.-F.; Jiang, Y.-Q.; Han, X.-H.; Li, Z.-L.; Cheng, B.-Y.; Zhang, D.-Z. Mechanical Properties of Breast Cancer Cell Membrane Studied with Optical Tweezers. Chin. Phys. Lett. 2004, 21, 2543–2546. [Google Scholar] [CrossRef]
- Lu, T.; Lee, C.-H.; Anvari, B. Morphological Characteristics, Hemoglobin Content, and Membrane Mechanical Properties of Red Blood Cell Delivery Systems. ACS Appl. Mater. Interfaces 2022, 14, 18219–18232. [Google Scholar] [CrossRef]
- Campillo, C.; Sens, P.; Köster, D.; Pontani, L.-L.; Lévy, D.; Bassereau, P.; Nassoy, P.; Sykes, C. Unexpected Membrane Dynamics Unveiled by Membrane Nanotube Extrusion. Biophys. J. 2013, 104, 1248–1256. [Google Scholar] [CrossRef]
- Li, X.; Chen, Z.; Li, Y.; Liang, H.; Wang, H.; Li, M. Optical Tweezers Study of Membrane Fluidity in Small Cell Lung Cancer Cells. Opt. Express 2021, 29, 11976. [Google Scholar] [CrossRef]
- Khatibzadeh, N.; Gupta, S.; Farrell, B.; Brownell, W.E.; Anvari, B. Effects of Cholesterol on Nano-Mechanical Properties of the Living Cell Plasma Membrane. Soft Matter 2012, 8, 8350. [Google Scholar] [CrossRef]
- Bisaria, A.; Hayer, A.; Garbett, D.; Cohen, D.; Meyer, T. Membrane-Proximal F-Actin Restricts Local Membrane Protrusions and Directs Cell Migration. Science 2020, 368, 1205–1210. [Google Scholar] [CrossRef] [PubMed]
- Adrien, V.; Rayan, G.; Astafyeva, K.; Broutin, I.; Picard, M.; Fuchs, P.; Urbach, W.; Taulier, N. How to Best Estimate the Viscosity of Lipid Bilayers. Biophys. Chem. 2022, 281, 106732. [Google Scholar] [CrossRef] [PubMed]
- Silva, P.M.; Da Silva, I.V.; Sarmento, M.J.; Silva, Í.C.; Carvalho, F.A.; Soveral, G.; Santos, N.C. Aquaporin-3 and Aquaporin-5 Facilitate Migration and Cell–Cell Adhesion in Pancreatic Cancer by Modulating Cell Biomechanical Properties. Cells 2022, 11, 1308. [Google Scholar] [CrossRef] [PubMed]
- Kosheleva, N.V.; Efremov, Y.M.; Koteneva, P.I.; Ilina, I.V.; Zurina, I.M.; Bikmulina, P.Y.; Shpichka, A.I.; Timashev, P.S. Building a Tissue: Mesenchymal and Epithelial Cell Spheroids Mechanical Properties at Micro- and Nanoscale. Acta Biomater. 2023, 165, 140–152. [Google Scholar] [CrossRef] [PubMed]
- Kashirina, A.S.; López-Duarte, I.; Kubánková, M.; Gulin, A.A.; Dudenkova, V.V.; Rodimova, S.A.; Torgomyan, H.G.; Zagaynova, E.V.; Meleshina, A.V.; Kuimova, M.K. Monitoring Membrane Viscosity in Differentiating Stem Cells Using BODIPY-Based Molecular Rotors and FLIM. Sci. Rep. 2020, 10, 14063. [Google Scholar] [CrossRef] [PubMed]
- Larson, R.E.; Higdon, J.J.L. Microscopic Flow near the Surface of Two-Dimensional Porous Media. Part 1. Axial Flow. J. Fluid. Mech. 1986, 166, 449. [Google Scholar] [CrossRef]
- Itoh, T.; Tsujita, K. Exploring Membrane Mechanics: The Role of Membrane-Cortex Attachment in Cell Dynamics. Curr. Opin. Cell Biol. 2023, 81, 102173. [Google Scholar] [CrossRef]
- Sliogeryte, K.; Thorpe, S.D.; Lee, D.A.; Botto, L.; Knight, M.M. Stem Cell Differentiation Increases Membrane-Actin Adhesion Regulating Cell Blebability, Migration and Mechanics. Sci. Rep. 2014, 4, 7307. [Google Scholar] [CrossRef]
- Song, Y.; Ma, X.; Zhang, M.; Wang, M.; Wang, G.; Ye, Y.; Xia, W. Ezrin Mediates Invasion and Metastasis in Tumorigenesis: A Review. Front. Cell Dev. Biol. 2020, 8, 588801. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Efremov, Y.M.; Shimolina, L.; Gulin, A.; Ignatova, N.; Gubina, M.; Kuimova, M.K.; Timashev, P.S.; Shirmanova, M.V. Correlation of Plasma Membrane Microviscosity and Cell Stiffness Revealed via Fluorescence-Lifetime Imaging and Atomic Force Microscopy. Cells 2023, 12, 2583. https://doi.org/10.3390/cells12212583
Efremov YM, Shimolina L, Gulin A, Ignatova N, Gubina M, Kuimova MK, Timashev PS, Shirmanova MV. Correlation of Plasma Membrane Microviscosity and Cell Stiffness Revealed via Fluorescence-Lifetime Imaging and Atomic Force Microscopy. Cells. 2023; 12(21):2583. https://doi.org/10.3390/cells12212583
Chicago/Turabian StyleEfremov, Yuri M., Liubov Shimolina, Alexander Gulin, Nadezhda Ignatova, Margarita Gubina, Marina K. Kuimova, Peter S. Timashev, and Marina V. Shirmanova. 2023. "Correlation of Plasma Membrane Microviscosity and Cell Stiffness Revealed via Fluorescence-Lifetime Imaging and Atomic Force Microscopy" Cells 12, no. 21: 2583. https://doi.org/10.3390/cells12212583
APA StyleEfremov, Y. M., Shimolina, L., Gulin, A., Ignatova, N., Gubina, M., Kuimova, M. K., Timashev, P. S., & Shirmanova, M. V. (2023). Correlation of Plasma Membrane Microviscosity and Cell Stiffness Revealed via Fluorescence-Lifetime Imaging and Atomic Force Microscopy. Cells, 12(21), 2583. https://doi.org/10.3390/cells12212583





