Collision Dynamics of Cassava Seed Stems: Analyzing Recovery Coefficients and Influential Factors for Precision Agriculture
Abstract
1. Introduction
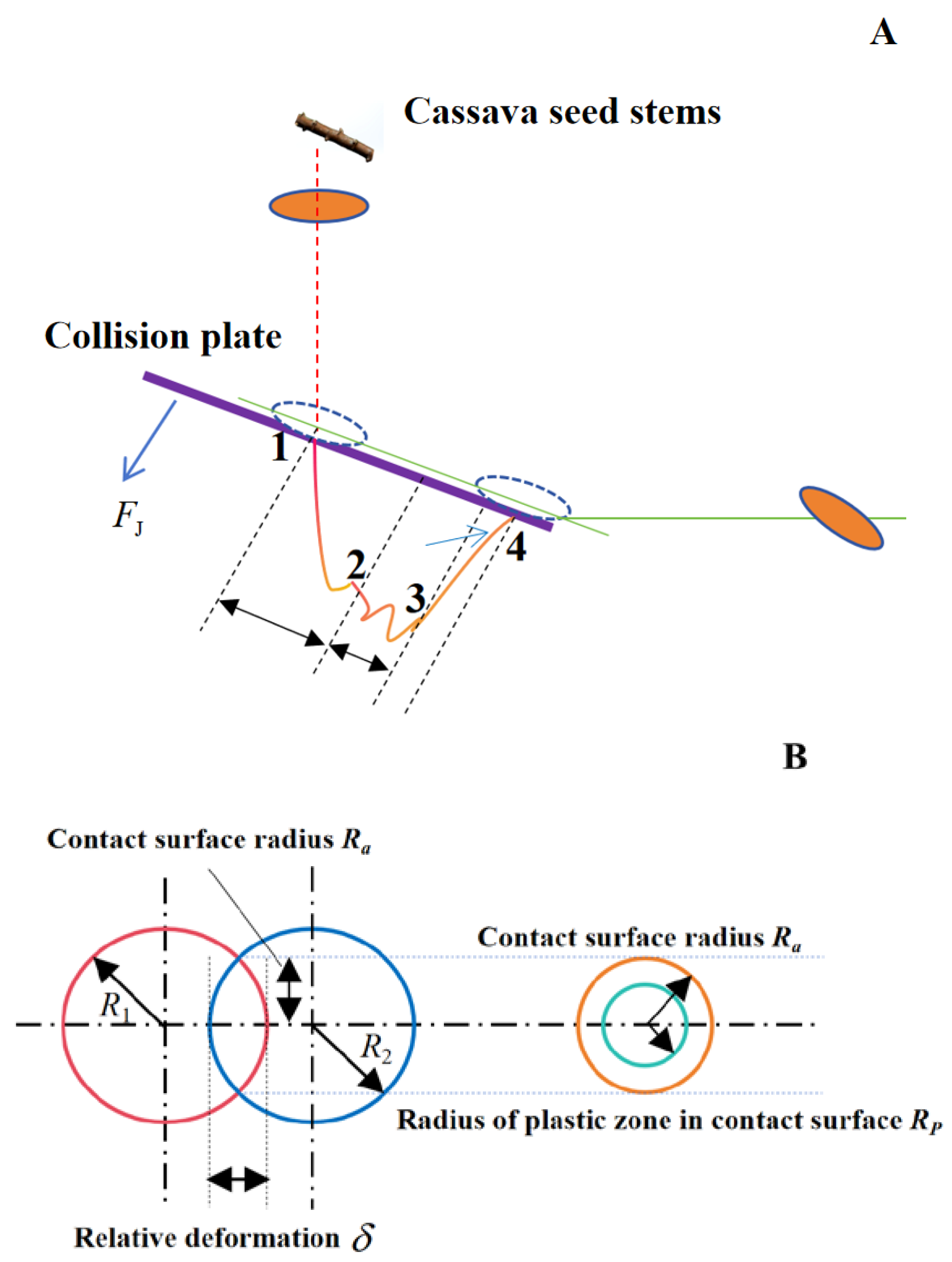
2. Analysis of Collision Contact Theory
2.1. Elastic Deformation Phase
2.2. Elastic–Plastic Deformation Stage
2.3. Resilient Recovery Phase
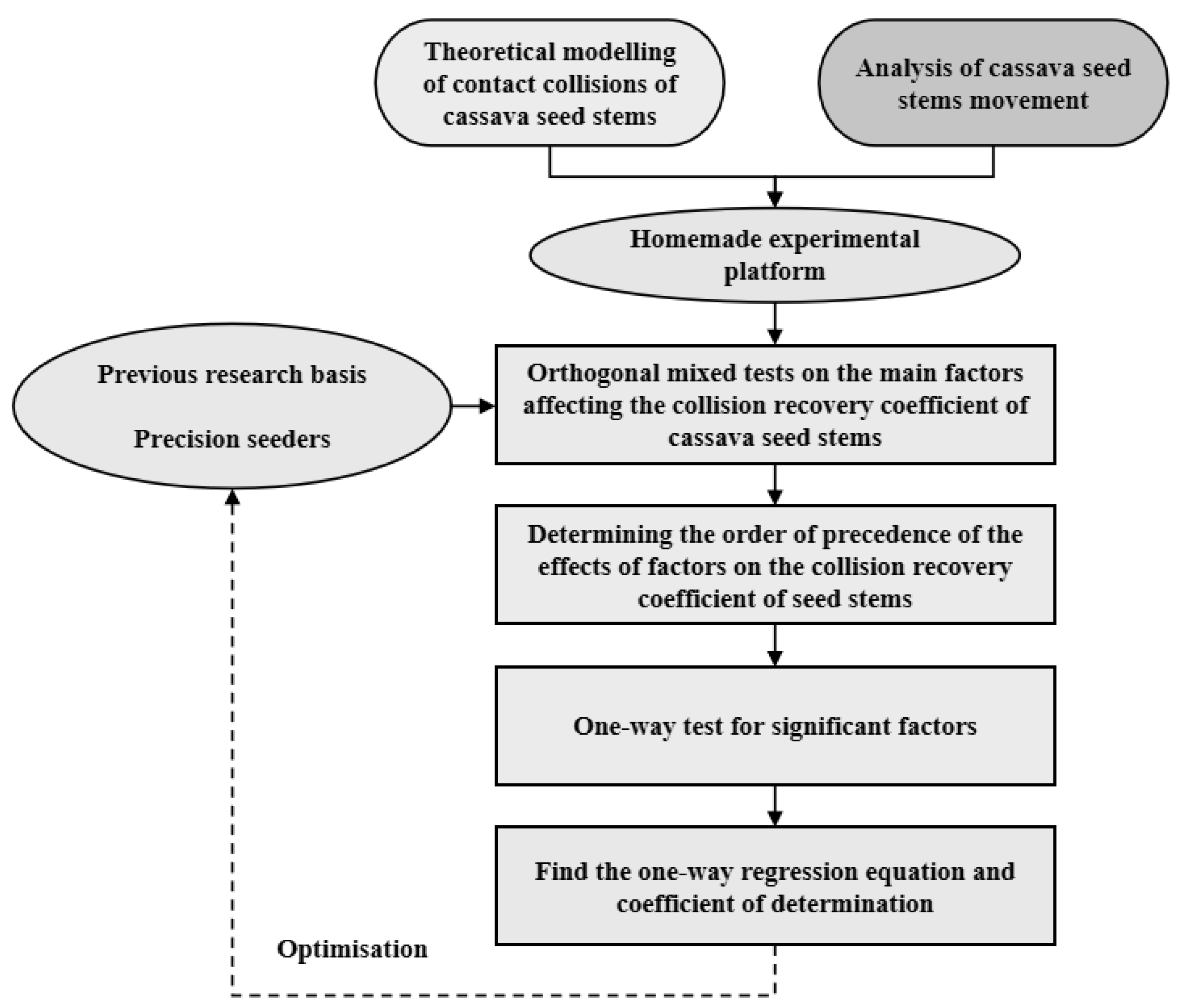
3. Test Materials and Methods
3.1. Research Framework and Hypotheses
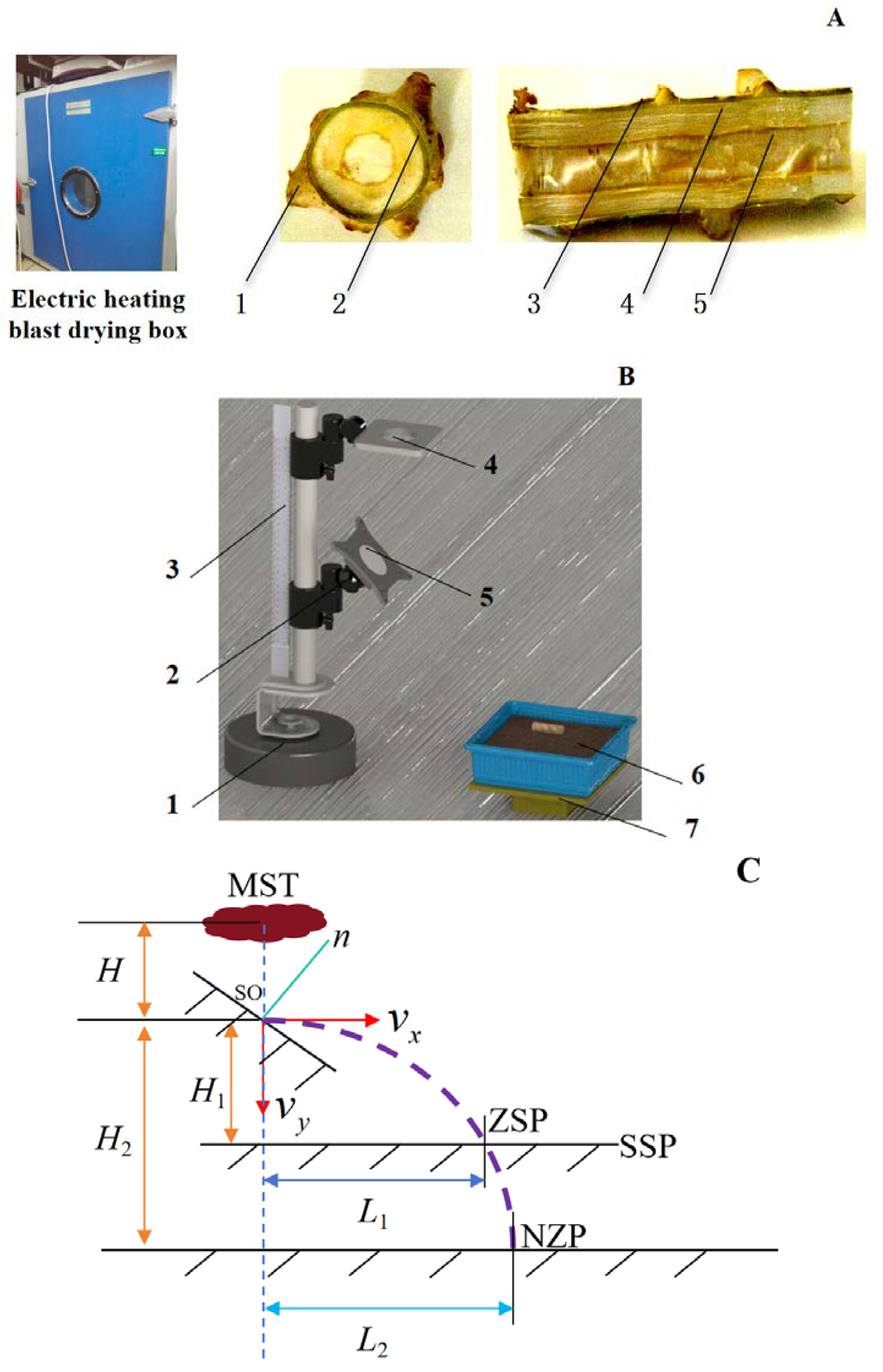
3.2. Test Materials
3.3. Test Methods
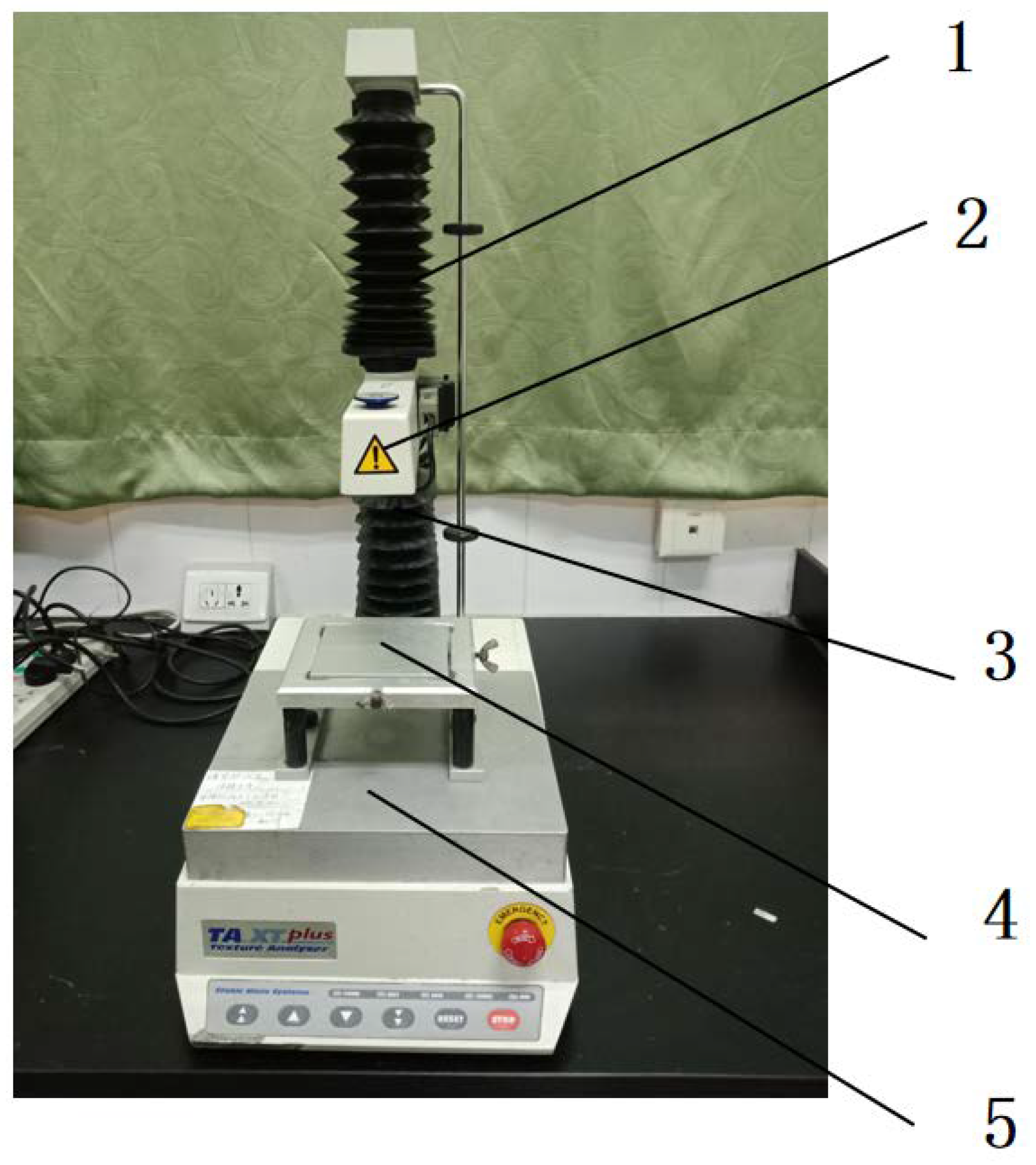
3.3.1. Mechanical Tests
3.3.2. Crash Test
3.4. Crash Test Device and Test Principle
3.5. Experimental Design
3.5.1. Orthogonal Test Factors and Levels Crash Test
3.5.2. One-Way Test Factors and Levels
4. Test Results and Analysis
4.1. Mixed Orthogonal Test Results and Analysis
4.2. Results and Analysis of the One-Way Test
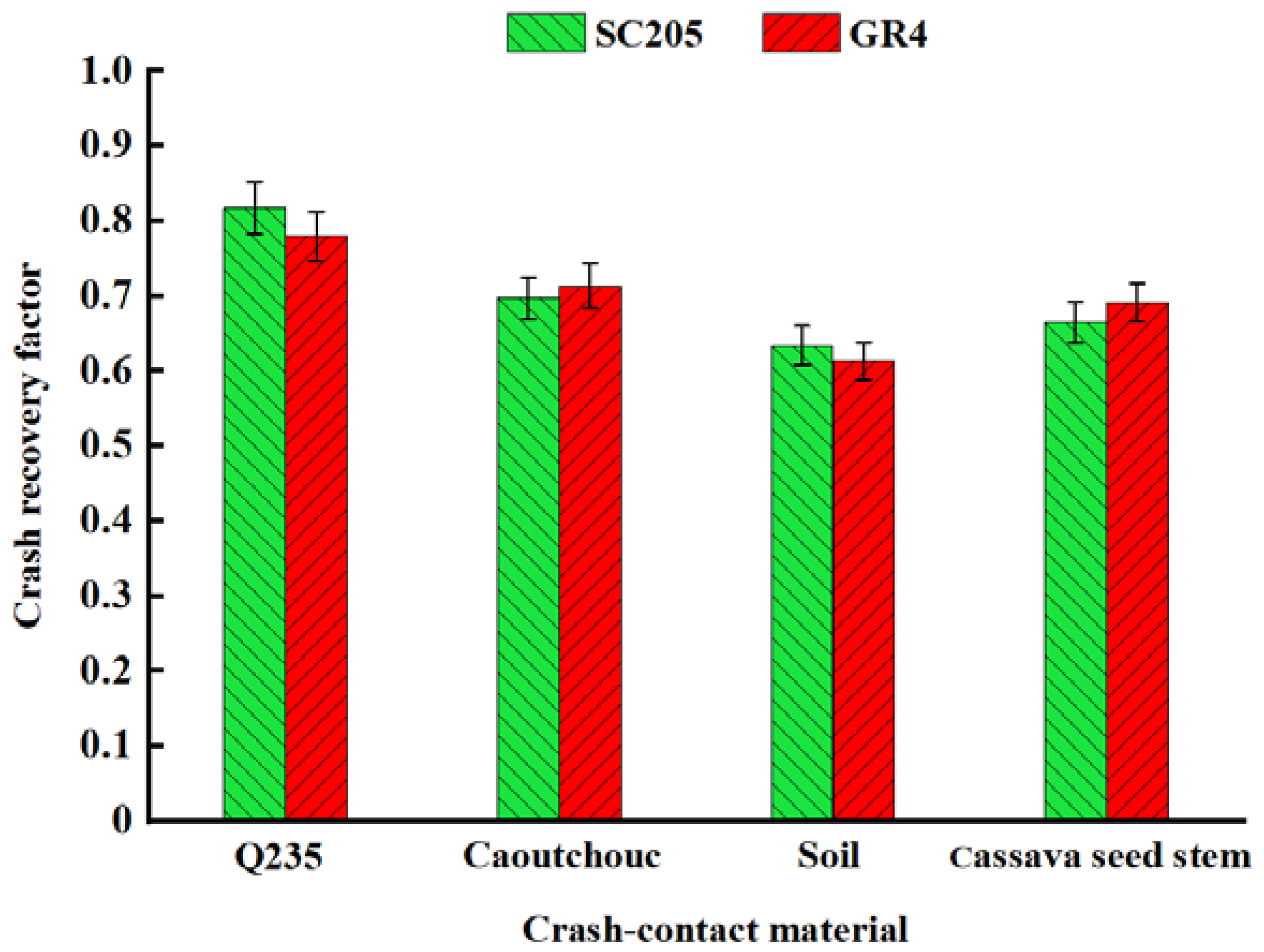
4.2.1. Effect of Collision Contact Materials on the Collision Recovery Coefficient of Seed Stems
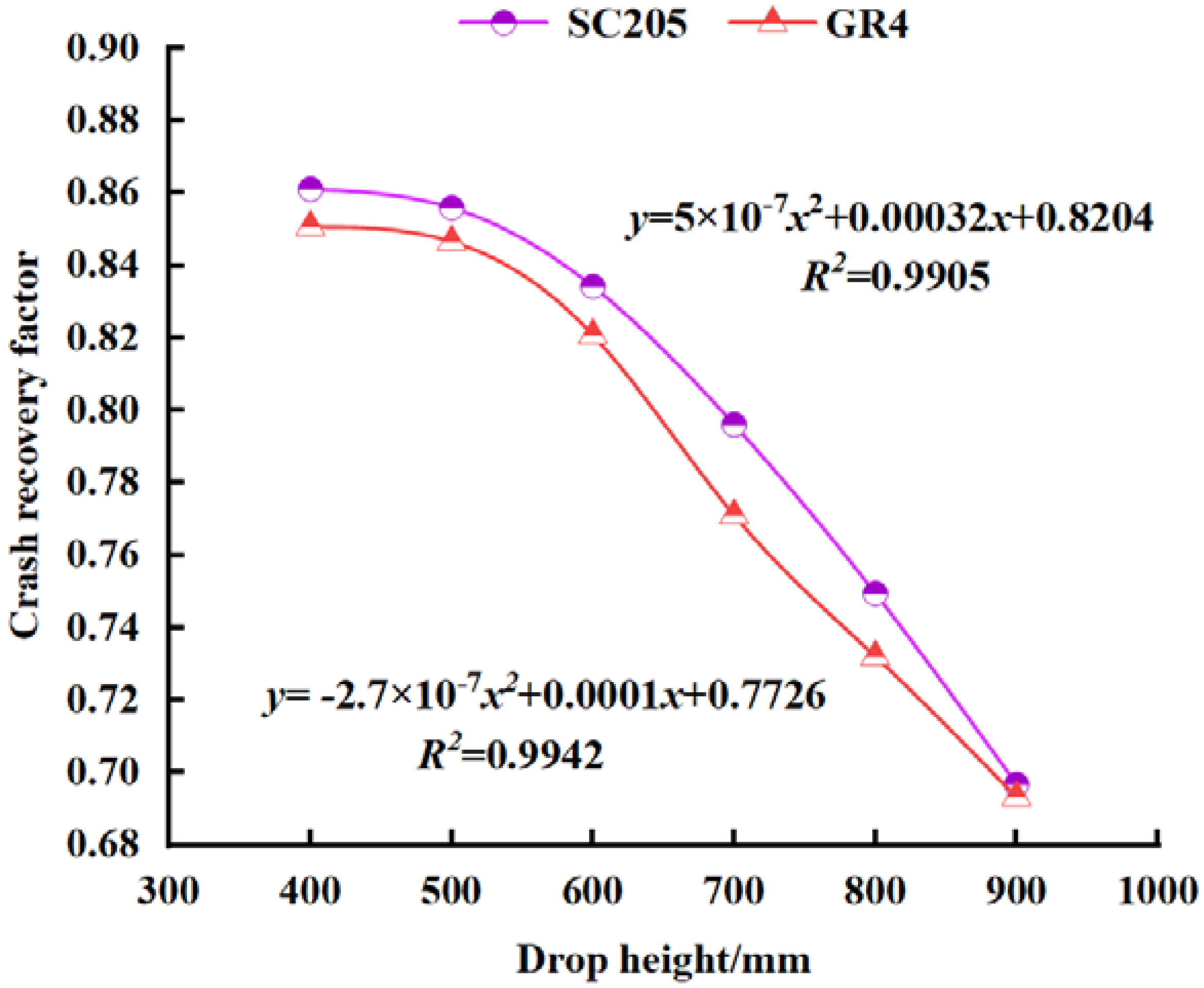
4.2.2. Effect of Fall Height on the Collision Recovery Coefficient of Seed Stems
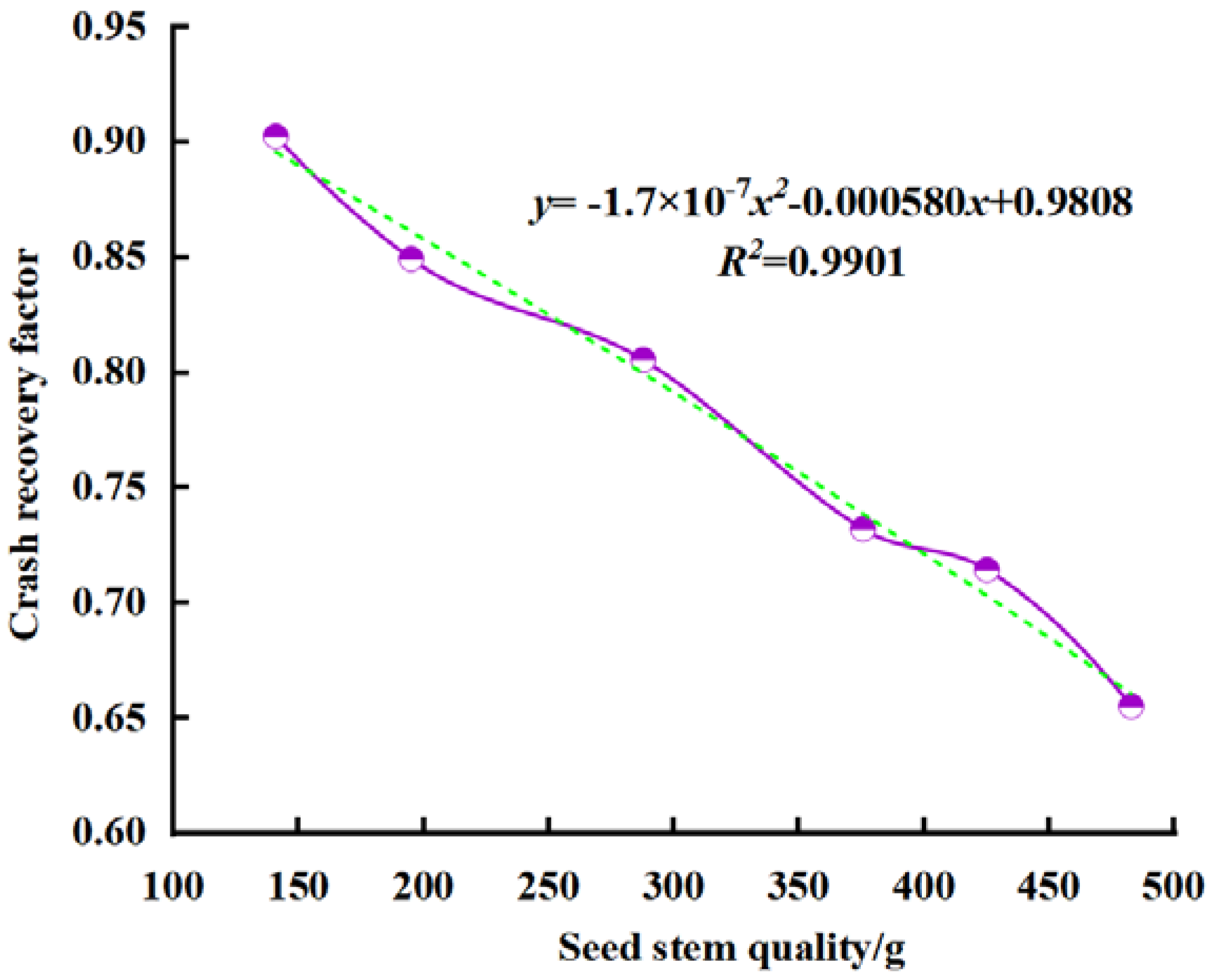
4.2.3. Effect of Cassava Seed Stem Mass on the Coefficient of Recovery of Seed Stem Collisions
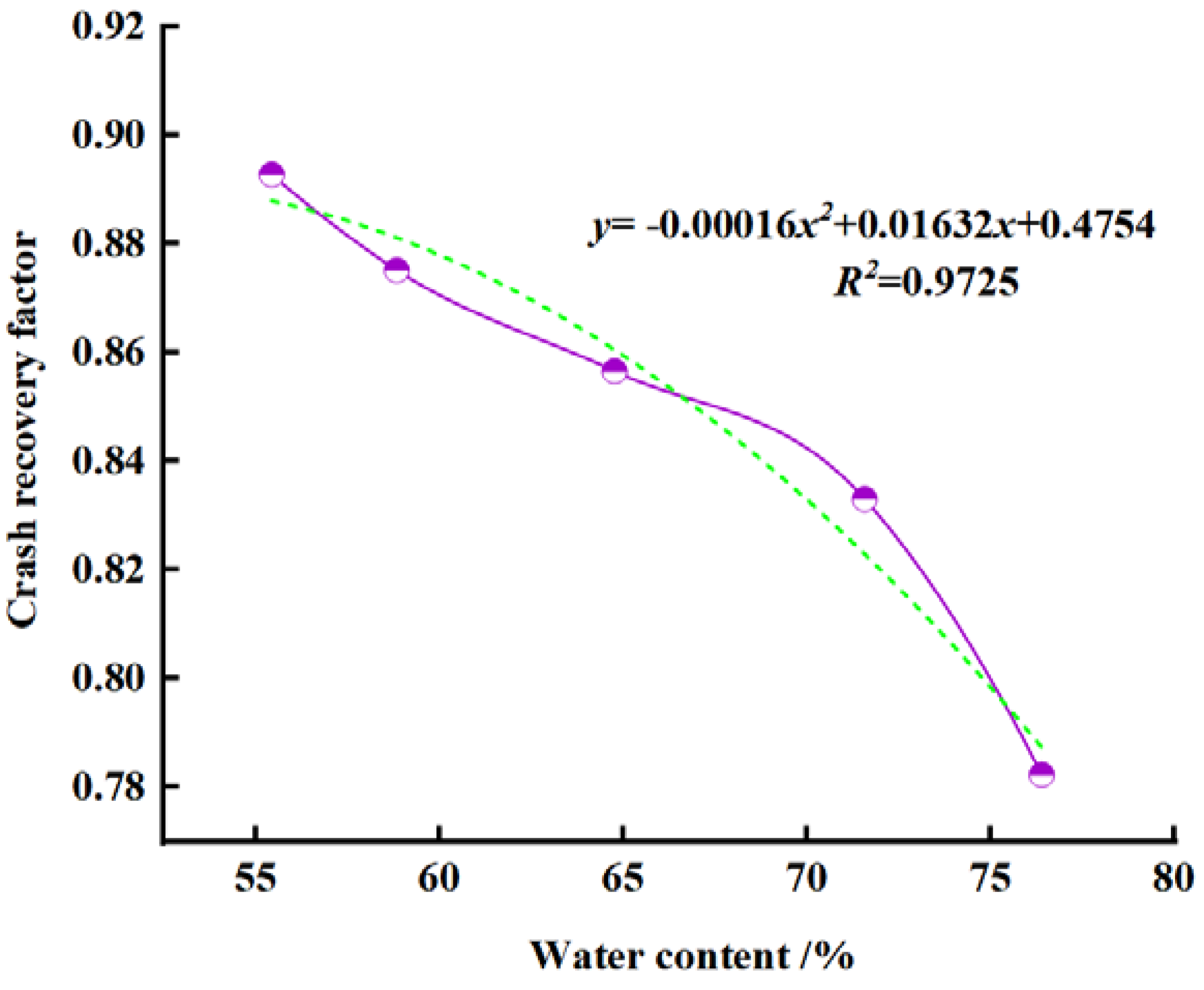
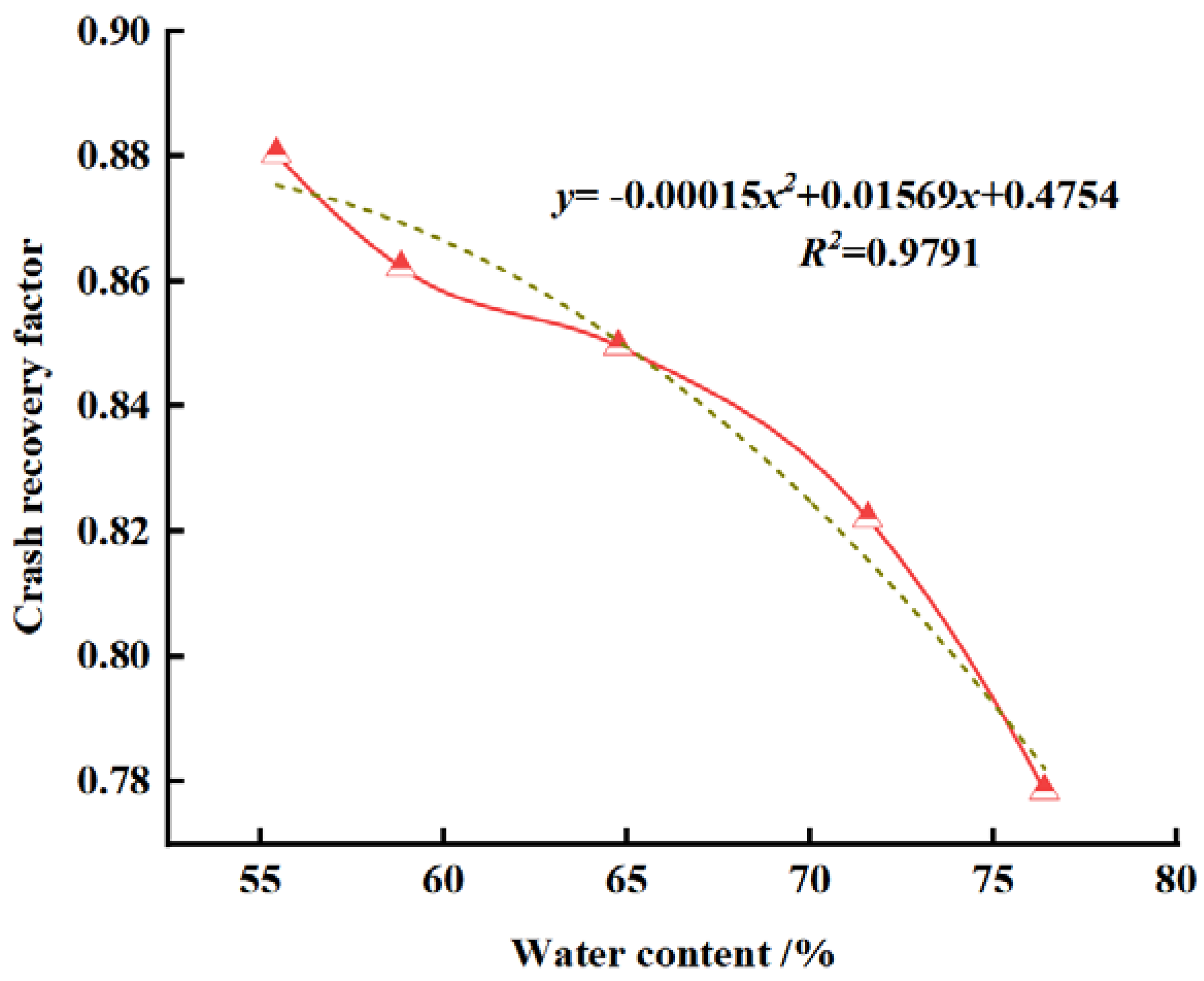
4.2.4. Effect of Water Content on the Collision Recovery Coefficient of Seed Stems
4.2.5. Influence of Seed Stem Fall Direction and Species on Collision Recovery Coefficients
5. Discussion
5.1. Material Type
5.2. Water Content
5.3. Drop Height
5.4. Seed Stem Mass
5.5. Limitations of This Study and Directions for Future Studies
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Chen, L.; Chen, R.; Atwa, E.M.; Mabrouk, M.; Jiang, H.; Mou, X.; Ma, X. Nutritional Quality Assessment of Miscellaneous Cassava Tubers Using Principal Component Analysis and Cluster Analysis. Foods 2024, 13, 1861. [Google Scholar] [CrossRef] [PubMed]
- Lu, S.; Lei, K.; Wei, L.; Ma, C.; Xu, C.; Chen, R. Climate suitability analysis and high yield cultivation techniques of cassava in Guangxi. Agric. Technol. 2021, 41, 57–59. [Google Scholar] [CrossRef]
- Cao, S.; Chen, J.; Huang, F.; Yan, H.; Wei, C.; Li, F.; Lu, L.; Qin, X.; Chen, H.; Li, H. Current situation analysis and development suggestions of cassava industry in Guangxi. J. South. Agric. 2021, 52, 1468–1476. [Google Scholar] [CrossRef]
- Lungkapin, J.; Salokhe, V.; Kalsirisilp, R.; Nakashima, H. Development of a stem cutting unit for a cassava planter. Agric. Eng. Int. 2007, 9, 1–16. Available online: https://api.semanticscholar.org/CorpusID:54547530 (accessed on 1 November 2024).
- Chen, L.; Li, K.; Mou, X.; Liu, Z.; Jiang, H.; Mabrouk, M.; Pan, J.; Atwa, E.M. Evaluating the Impact of Moisture Content and Loading Orientation on the Geometrical Characteristics and Mechanical Behavior of Cassava Tubers. Agronomy 2024, 14, 2254. [Google Scholar] [CrossRef]
- Lungkapin, J.; Salokhe, V.; Kalsirisilp, R.; Nakashima, H. Design and development of a cassava planter. Trans. ASABE 2009, 52, 393–399. [Google Scholar] [CrossRef]
- Li, G.; Zhou, S.; Deng, G.; Zheng, S.; He, F.; Cui, Z.; Dai, Y.; Li, L.; Yan, B.; Qin, S.; et al. Research Progress on Mechanization Technology and Equipment for Cassava Field Production. Agriculture 2024, 14, 1926. [Google Scholar] [CrossRef]
- Zeng, B.; Ye, C.; Lu, H. Research and design of 2CMS-2 cassava combine planter. Guangxi Agric. Mech. 2011, 06, 21–22. [Google Scholar] [CrossRef]
- Mou, X.; Chen, L.; Ma, X.; Xue, J.; Xiang, J. Design and Experiment of Spoon Chain Seed Metering Mechanism of Precutting Vibration Seed Feeding Cassava Planter. Trans. Chin. Soc. Agric. Mach. 2023, 54, 20–31. [Google Scholar]
- Chen, L.; Xue, J.; Mou, X.; Ma, X.; Xiang, J. Design and experiments of the stepped vibration seed dispersal mechanism for pre-cut cassava planters. Trans. CSAE 2022, 38, 27–37. [Google Scholar] [CrossRef]
- GB/T 6973-2005; Testing Methods of Single Seed Drills (Precision Drills), the Standardization Administration of the People’s Republic of China. China Standards Press: Beijing, China, 2005.
- Yang, W.; Cai, G.; Yang, J.; Huang, Y. Mechanical and mathematical model analysis of uprooted force on cassava storage root. Trans. CSAE 2011, 27, 95–100. [Google Scholar] [CrossRef]
- China Academy of Agricultural Mechanization. Agricultural Machinery Design Manual; Machinery Industry Press: Beijing, China, 1988. [Google Scholar]
- Yang, W.; Yang, J.; Zheng, X.; Wang, Q.; Jia, F.; Zhao, J. Experiment on mechanical properties of cassava. Trans. CSAE 2011, 27, 50–54. Available online: http://www.tcsae.org/article/id/20111411 (accessed on 5 November 2024).
- Peng, C.; Zhou, T.; Song, S.; Fang, Q.; Zhu, H.; Sun, S. Measurement and Analysis of Restitution Coefficient of Black Soldier Fly Larvae in Collision Models Based on Hertz Contact Theory. Trans. Chin. Soc. Agric. Mach. 2021, 52, 125–134. [Google Scholar] [CrossRef]
- Lv, Z.; Wang, W.; Yang, D.; He, X.; Qu, Z.; Jing, W.; Mei, Y.; Huang, H.; Su, B.; Zhuang, Y. Optimal Design and Tests of a Pulsating Roll-Cleaning Device for Tiger Nuts. Agriculture 2024, 14, 1673. [Google Scholar] [CrossRef]
- Zhang, L.; Wang, X.; Chen, J.; Wang, H.; Cao, Y. Analysis and Optimization of Low-Resistance Animal Bionic Subsoiling Shovel Based on EDEM. Agriculture 2024, 14, 2046. [Google Scholar] [CrossRef]
- Wang, L.; Liu, T.; Feng, X.; Gao, Y.; Wang, B.; Zhang, S. Research progress of the restitution coefficients of collision of particles in agricultural and food fields. Trans. Chin. Soc. Agric. Eng. 2021, 37, 313–322. [Google Scholar] [CrossRef]
- Yang, Y.; Hou, J.; Bai, J.; Yao, E.; He, T.; Li, J. Determination and analysis of collision recovery coefficient of typical castor capsules. J. China Agric. Univ. 2019, 24, 138–148. [Google Scholar] [CrossRef]
- Wang, L.; Wu, B.; Wu, Z.; Li, R.; Feng, X. Experimental determination of the coefficient of restitution of particle-particle collision for frozen maize grains. Powder Technol. 2018, 338, 263–273. [Google Scholar] [CrossRef]
- Feng, B.; Sun, W.; Sun, B.; Zhang, T.; Wu, J.; Shi, L. Determination of restitution coefficient of potato tubers collision in harvest and analysis of its influence factors. Trans. Chin. Soc. Agric. Eng. 2017, 33, 50–57. [Google Scholar] [CrossRef]
- Yang, J.; Liu, G.; Fu, H.; Du, W.; Wu, Z. Experimental platform for determining the recovery coefficient of collision between unstructured agricultural bulk materials. Exp. Technol. Manag. 2022, 39, 109–114. [Google Scholar] [CrossRef]
- Chen, D.; Yang, Z.; La, G.; Yuan, C.; Wang, H.; Wang, C.; Sun, G. Determination of collision recovery coefficient in harvesting of Hibiscus sabdariffa. Agric. Eng. 2021, 11, 82–88. [Google Scholar] [CrossRef]
- Liu, Y.; Huang, X.; Ma, L.; Zong, W.; Li, M.; Tang, C. Determination of three-dimensional collision restitution coefficient of oil sunflower grain by high-speed photography. Trans. Chin. Soc. Agric. Eng. 2020, 36, 44–53. [Google Scholar] [CrossRef]
- Du, Y.; Wang, S. Dissipation modeling of elastic-plastic positive collision of two particles. J. Mech. Eng. 2009, 45, 149–156. [Google Scholar] [CrossRef]
- Chang, B.; Zhu, Y.; Jin, G.; Song, Y.; Li, X.; Lu, C. Impact Motion Analysis and Optimization of Redundant Serial Robot Considering Collision. Trans. Chin. Soc. Agric. Mach. 2019, 50, 413–420. [Google Scholar] [CrossRef]
- Liu, G.; Han, X.; Wang, Z.; Wang, K.; Zhang, Z.; Duan, Z. Viscosity Reduction and Drag Reduction Performance Analysis of Bionic Excavator Buckets Based on Discrete Element Method. Biomimetics 2024, 9, 686. [Google Scholar] [CrossRef]
- Du, X.; Liu, J.; Zhao, Y.; Zhang, C.; Zhang, X.; Wang, Y. Design and Test of Discrete Element-Based Separation Roller Potato–Soil Separation Device. Agriculture 2024, 14, 1053. [Google Scholar] [CrossRef]
- Liu, C.; Wang, C.; Song, J.; Du, X.; Zhang, F. Design and seed-filling test of cell-type precision seed-metering device with vibration technology. Trans. Chin. Soc. Agric. Mach. 2018, 49, 108–115. [Google Scholar] [CrossRef]
- Zheng, Z.; Li, D.; Qiu, X.; Zhu, X.; Ma, P.; Zhang, D. Numerical Analysis of Effect of Impacting Velocity on Diesel Droplet Impacting on Inclined Surface. Trans. Chin. Soc. Agric. Mach. 2016, 47, 317–324. [Google Scholar] [CrossRef]
- Zhang, S.; Fu, J.; Zhang, R.; Zhang, Y.; Yuan, H. Experimental Study on the Mechanical Properties of Friction, Collision and Compression of Tiger Nut Tubers. Agriculture 2022, 12, 65. [Google Scholar] [CrossRef]
- Zhang, B. Principles of Sowing Machinery Design; Machinery Industry Press: Beijing, China, 1982. [Google Scholar]
- Campbell, C.M.; Fulton, J.P.; Mcdonald, T.P.; Wood, C.W.; Zech, W.C.; Srivastava, P. Spinner-disc technology to enhance the application of poultry litter. Appl. Eng. Agric. 2020, 26, 759–767. [Google Scholar] [CrossRef]
- Li, M.; Liu, R.; Zhong, Y.; Li, X. Current progress on the associative nitrogen fixation in sugarcane and its application potentials. Acta Microbiol. Sin. 2021, 61, 564–579. [Google Scholar] [CrossRef]
- Liu, J.; Jing, Y.; Lai, Y.; Shi, J.; Li, M. Discussion on the Unqualified Factors of Pinelliae Rhizoma. J. Chin. Med. Mater. 2019, 42, 1261–1266. [Google Scholar] [CrossRef]
- Cui, Z.; Deng, G.; Li, G.; Liu, Z.; Zheng, S.; Li, L. Design of Cassava Stalk Cutter for Cassava Planting Machine. J. Agric. Mech. Res. 2017, 39, 144–148. [Google Scholar] [CrossRef]
- Roger, C.B. Impact testing of potato harvesting equipment. Am. Potato J. 1993, 70, 243–256. [Google Scholar] [CrossRef]
- Huang, T.; Wu, B.; Li, L.; Zuo, T.; Xie, F. Construction of impact mechanics model and experimental study on impact damage of potato tuber. INMATEH—Agric. Eng. 2022, 66, 139–146. [Google Scholar] [CrossRef]
- Huang, X.; Zha, X.; Pan, M.; Zong, W.; Chen, H. Measurement and analysis of rapeseeds’restitution coefficient in point-to-plate collision model. Trans. Chin. Soc. Agric. Eng. 2014, 30, 22–29. [Google Scholar] [CrossRef]
- Lu, Y.; Wu, N.; Wang, B.; Yu, Z.; Lin, D. Measurement and analysis of peanuts’restitution coefficient inpointto plate collision mode. J. China Agric. Univ. 2016, 21, 111–118. [Google Scholar] [CrossRef]
- Wang, C.; Li, Y.; Ma, L.; Ma, Z. Experimental study on measurement of restitution coefficient of wheat seeds in collision models. Trans. Chin. Soc. Agric. Eng. 2012, 28, 274–279. [Google Scholar] [CrossRef]
- Szwed, G.; Lukaszuk, J. Effect of rapeseed and wheat kernel moisture on impact damage. Int. Agrophys. 2007, 21, 299–304. [Google Scholar] [CrossRef]
- Wojtkowski, M.; Pecen, J.; Horabik, J.; Molenda, M. Rapeseed impact against a flat surface: Physical testing and DEM simulation with two contact models. Powder Technol. 2010, 198, 61–68. [Google Scholar] [CrossRef]
- Meng, J.; Wang, C.; Xie, S.; Deng, W.; Qi, S. Measurement test analysis of potato restitution coefficient. J. China Agric. Univ. 2017, 22, 93–100. [Google Scholar] [CrossRef]


| Material Type | Density/kg/m3 | Modulus of Elasticity/MPa | Poisson’s Ratio | Thickness/mm |
|---|---|---|---|---|
| Q235 | 7860 | 212,000 | 0.29 | 10 |
| Caoutchouc | 1000 | 100 | 0.30 | 5 |
| Soil | 2100 | 35 | 0.47 | / |
| SC205 | 1530 | 6.84 | 0.51 | / |
| GR 4 | 1223 | 6.84 | 0.51 | / |
| Level | Material Type X1 | Water Content X2/% | Drop Height X3/mm | Wood Seed Stem Mass X4/g | Fall Direction X5 | Variety X6 |
|---|---|---|---|---|---|---|
| 1 | Q235 | 64.77 | 550 | 237.66 | Axially | SC205 |
| 2 | Caoutchouc | 67.31 | 650 | 176.14 | Radial | GR4 |
| 3 | Sandy loam | 71.57 | 750 | 199.44 | / | / |
| 4 | Seed stem | 76.39 | 850 | 150.89 | / | / |
| No. | Material Type X1 | Moisture Content X2/% | Drop Height X3/mm | Cassava Seed Stem Mass X4/g | Fall Direction X5 | Cassava Variety X6 | Blank Column | Horizontal Velocity (m/s) | Vertical Partial Velocity (m/s) | Crash Recovery Factor |
|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 1(Q235) | 1 | 1 | 1 | 1 | 1 | 1 | 1.7355 | −1.2747 | 0.9168 |
| 2 | 1(Q235) | 2 | 2 | 2 | 1 | 2 | 2 | 1.7656 | −1.3776 | 0.8806 |
| 3 | 1(Q235) | 3 | 3 | 3 | 2 | 1 | 2 | 2.1976 | −1.0925 | 0.8581 |
| 4 | 1(Q235) | 4 | 4 | 4 | 2 | 2 | 1 | 1.8023 | −1.4670 | 0.8010 |
| 5 | 2(Caoutchouc) | 1 | 2 | 3 | 2 | 2 | 1 | 1.4987 | −1.1464 | 0.7411 |
| 6 | 2(Caoutchouc) | 2 | 1 | 4 | 1 | 1 | 2 | 1.3446 | −0.9418 | 0.6964 |
| 7 | 2(Caoutchouc) | 3 | 4 | 1 | 1 | 2 | 2 | 1.7962 | −1.4617 | 0.7982 |
| 8 | 2(Caoutchouc) | 4 | 3 | 2 | 1 | 1 | 1 | 1.6244 | −1.2306 | 0.7446 |
| 9 | 3(Sandy loam) | 1 | 3 | 4 | 1 | 2 | 2 | 1.4013 | −0.8868 | 0.5968 |
| 10 | 3(Sandy loam) | 2 | 4 | 3 | 1 | 1 | 1 | 1.3511 | −0.9260 | 0.5579 |
| 11 | 3(Sandy loam) | 3 | 1 | 2 | 2 | 2 | 1 | 1.1975 | −1.1286 | 0.7085 |
| 12 | 3(Sandy loam) | 4 | 2 | 1 | 2 | 1 | 2 | 1.2097 | −1.1401 | 0.6583 |
| 13 | 4(Seed stem) | 1 | 4 | 2 | 2 | 1 | 2 | 1.2097 | −1.1401 | 0.6583 |
| 14 | 4(Seed stem) | 2 | 3 | 1 | 2 | 2 | 1 | 1.4371 | −1.3092 | 0.6728 |
| 15 | 4(Seed stem) | 3 | 2 | 4 | 1 | 1 | 1 | 1.5290 | −1.2066 | 0.7135 |
| 16 | 4(Seed stem) | 4 | 1 | 3 | 1 | 2 | 2 | 1.5532 | −1.0864 | 0.7395 |
| K1 | 3.4385 | 2.9095 | 3.0796 | 3.0688 | 5.9683 | 5.8024 | 5.8809 | 3.4385 | 2.9095 | 3.0796 |
| K2 | 2.9563 | 2.8244 | 2.9955 | 2.9825 | 5.8017 | 5.9676 | 5.8891 | 2.9563 | 2.8244 | 2.9955 |
| K3 | 2.4975 | 3.0803 | 2.8890 | 2.9090 | / | / | / | 2.4975 | 3.0803 | 2.8890 |
| K4 | 2.8777 | 2.9558 | 2.8059 | 2.8097 | / | / | / | 2.8777 | 2.9558 | 2.8059 |
| k1 | 0.859625 | 0.727375 | 0.769900 | 0.767200 | 0.7460375 | 0.72530 | 0.7351125 | 0.859625 | 0.727375 | 0.769900 |
| k2 | 0.739075 | 0.706100 | 0.748875 | 0.745625 | 0.7252125 | 0.74595 | 0.7361375 | 0.739075 | 0.706100 | 0.748875 |
| k3 | 0.624375 | 0.770075 | 0.722250 | 0.727250 | / | / | / | 0.624375 | 0.770075 | 0.722250 |
| k4 | 0.719425 | 0.73895 | 0.701475 | 0.702425 | / | / | / | 0.719425 | 0.738950 | 0.701475 |
| R | 0.235250 | 0.063975 | 0.068425 | 0.064775 | 0.0208250 | 0.02065 | / | / | / | / |
| Ranking of factors | Crash contact material > Drop height > Seed stem mass > Water content > Drop direction > Seed stem variety | |||||||||
| Source of Variance | Sum of Squared Deviations | Degrees of Freedom | Mean Square | F-Value | Significance |
|---|---|---|---|---|---|
| Material type | 0.110626 | 3 | 0.036875 | 2926.043 | * |
| Water content | 0.008656 | 3 | 0.002885 | 228.958 | * |
| Drop height | 0.010377 | 3 | 0.003459 | 274.476 | * |
| Cassava seed stem mass | 0.008705 | 3 | 0.002902 | 230.253 | * |
| Direction of fall | 0.001612 | 1 | 0.001612 | 127.913 | |
| Cassava varieties | 0.001832 | 1 | 0.001832 | 145.355 | |
| Inaccuracies | 0.000013 | 1 | 0.000013 | ||
| Aggregate | 8.933047 | 16 |
| Number of Tests | Drop Height/mm | /mm | /mm | Horizontal Partial Velocity (m/s) | Vertical Partial Velocity (m/s) | Crash Recovery Factor |
|---|---|---|---|---|---|---|
| 1 | 400 | 358 | 420 | 1.2335 | −1.1778 | 0.8612 |
| 2 | 500 | 414 | 486 | 1.4283 | −1.2514 | 0.8560 |
| 3 | 600 | 379 | 445 | 1.3076 | −1.5533 | 0.8343 |
| 4 | 700 | 471 | 553 | 1.6242 | −1.3250 | 0.7962 |
| 5 | 800 | 513 | 602 | 1.7691 | −1.1991 | 0.7496 |
| 6 | 900 | 585 | 686 | 2.0173 | −0.9095 | 0.6969 |
| Number of Tests | Drop Height/mm | /mm | /mm | Horizontal Partial Velocity (m/s) | Vertical Partial Velocity (m/s) | Crash Recovery Factor |
|---|---|---|---|---|---|---|
| 1 | 400 | 324 | 381 | 1.1187 | −1.2635 | 0.8508 |
| 2 | 500 | 405 | 475 | 1.3975 | −1.2532 | 0.8467 |
| 3 | 600 | 460 | 540 | 1.5876 | −1.2276 | 0.8209 |
| 4 | 700 | 528 | 620 | 1.8223 | −1.0344 | 0.7712 |
| 5 | 800 | 503 | 590 | 1.7357 | −1.1638 | 0.7322 |
| 6 | 900 | 573 | 672 | 1.9761 | −0.9360 | 0.6934 |
| Number of Tests | Cassava Seed Stem Mass/g | /mm | /mm | Horizontal Partial Velocity (m/s) | Vertical Partial Velocity (m/s) | Crash Recovery Factor |
|---|---|---|---|---|---|---|
| 1 | 140.82 | 617 | 724 | 2.1284 | −1.3324 | 0.9026 |
| 2 | 194.94 | 596 | 699 | 2.0551 | −1.2021 | 0.8495 |
| 3 | 287.72 | 542 | 635 | 1.8675 | −1.2212 | 0.8056 |
| 4 | 375.56 | 499 | 585 | 1.7208 | −1.0866 | 0.7322 |
| 5 | 425.21 | 486 | 570 | 1.6759 | −1.0637 | 0.7145 |
| 6 | 482.91 | 395 | 463 | 1.3607 | −1.1517 | 0.6553 |
| Number of Tests | Cassava Seed Stem Mass/g | /mm | /mm | Horizontal Partial Velocity (m/s) | Vertical Partial Velocity (m/s) | Crash Recovery Factor |
|---|---|---|---|---|---|---|
| 1 | 140.82 | 587 | 689 | 2.0258 | −1.3922 | 0.8915 |
| 2 | 194.94 | 515 | 604 | 1.7752 | −1.4028 | 0.8289 |
| 3 | 287.72 | 550 | 646 | 1.8996 | −1.1421 | 0.7933 |
| 4 | 375.56 | 486 | 570 | 1.6753 | −1.1025 | 0.7245 |
| 5 | 425.21 | 441 | 517 | 1.5212 | −1.1810 | 0.7048 |
| 6 | 482.91 | 433 | 508 | 1.4916 | −0.9846 | 0.6458 |
| Number of Tests | Water Content/% | /mm | /mm | Horizontal Partial Velocity (m/s) | Vertical Partial Velocity (m/s) | Crash Recovery Factor |
|---|---|---|---|---|---|---|
| 1 | 55.44 | 597 | 700 | 2.0573 | −1.3658 | 0.8928 |
| 2 | 58.84 | 564 | 661 | 1.9434 | −1.4120 | 0.8752 |
| 3 | 64.77 | 533 | 626 | 1.8388 | −1.4453 | 0.8566 |
| 4 | 71.57 | 526 | 617 | 1.8144 | −1.3797 | 0.8331 |
| 5 | 76.39 | 514 | 603 | 1.7731 | −1.2265 | 0.7824 |
| Number of Tests | Water Content/% | /mm | /mm | Horizontal Partial Velocity (m/s) | Vertical Partial Velocity (m/s) | Crash Recovery Factor |
|---|---|---|---|---|---|---|
| 1 | 55.44 | 554 | 650 | 1.9114 | −1.4640 | 0.8804 |
| 2 | 58.84 | 541 | 635 | 1.8673 | −1.4388 | 0.8623 |
| 3 | 64.77 | 537 | 630 | 1.8517 | −1.4062 | 0.8497 |
| 4 | 71.57 | 512 | 601 | 1.7668 | −1.3851 | 0.8221 |
| 5 | 76.39 | 510 | 598 | 1.7587 | −1.2266 | 0.7786 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mou, X.; Chen, R.; Chen, L.; Lan, Y.; Atwa, E.M.; Mabrouk, M.; Jiang, H.; Zhang, P. Collision Dynamics of Cassava Seed Stems: Analyzing Recovery Coefficients and Influential Factors for Precision Agriculture. Agronomy 2025, 15, 805. https://doi.org/10.3390/agronomy15040805
Mou X, Chen R, Chen L, Lan Y, Atwa EM, Mabrouk M, Jiang H, Zhang P. Collision Dynamics of Cassava Seed Stems: Analyzing Recovery Coefficients and Influential Factors for Precision Agriculture. Agronomy. 2025; 15(4):805. https://doi.org/10.3390/agronomy15040805
Chicago/Turabian StyleMou, Xiangwei, Rui Chen, Lintao Chen, Ying Lan, Elsayed M. Atwa, Mahmoud Mabrouk, Huanyu Jiang, and Peng Zhang. 2025. "Collision Dynamics of Cassava Seed Stems: Analyzing Recovery Coefficients and Influential Factors for Precision Agriculture" Agronomy 15, no. 4: 805. https://doi.org/10.3390/agronomy15040805
APA StyleMou, X., Chen, R., Chen, L., Lan, Y., Atwa, E. M., Mabrouk, M., Jiang, H., & Zhang, P. (2025). Collision Dynamics of Cassava Seed Stems: Analyzing Recovery Coefficients and Influential Factors for Precision Agriculture. Agronomy, 15(4), 805. https://doi.org/10.3390/agronomy15040805







