Investigating the Flexural Properties of 3D-Printed Nylon CF12 with Respect to the Correlation Between Loading and Layering Directions
Abstract
1. Introduction
1.1. Pole Vaulting—Basement
1.2. State of the Art
2. Materials and Methods
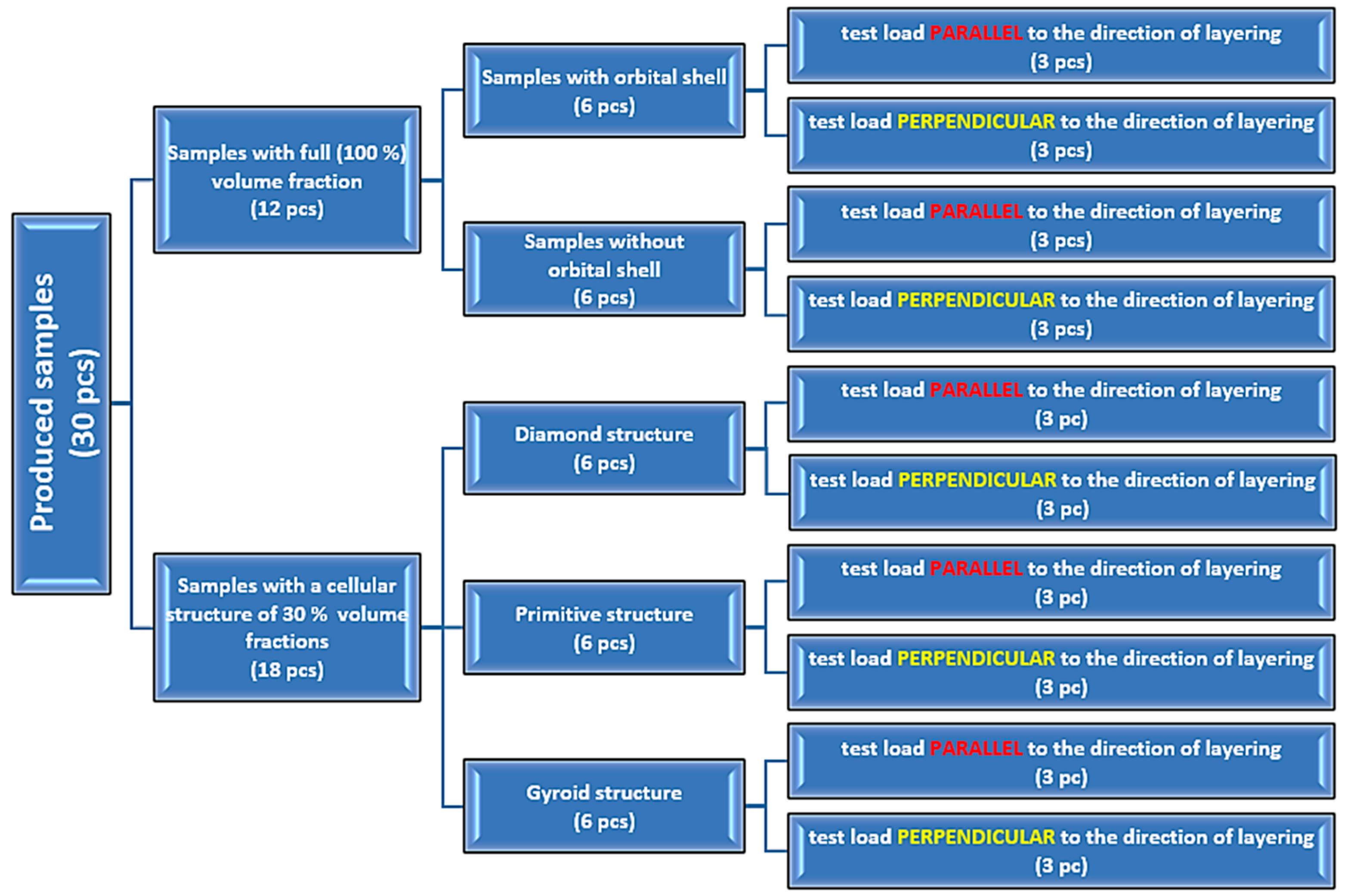
2.1. Samples—Specification and Production
2.1.1. Material of Samples
2.1.2. Design of Samples
2.1.3. Production of Samples
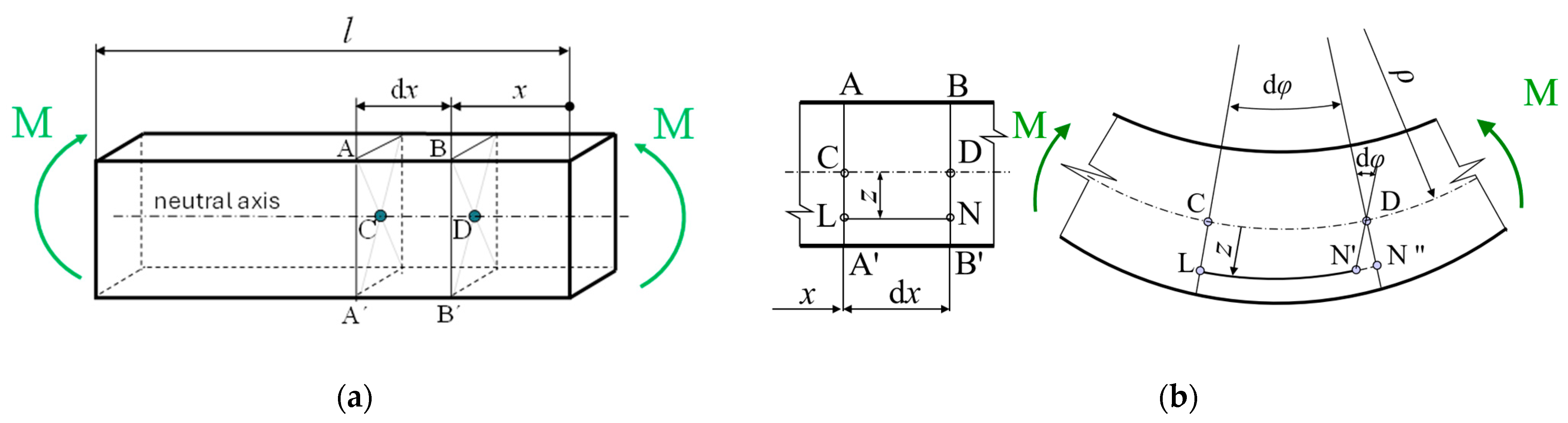
2.2. Testing and Evaluation Methodology
2.2.1. Experimental Testing
2.2.2. Data Processing
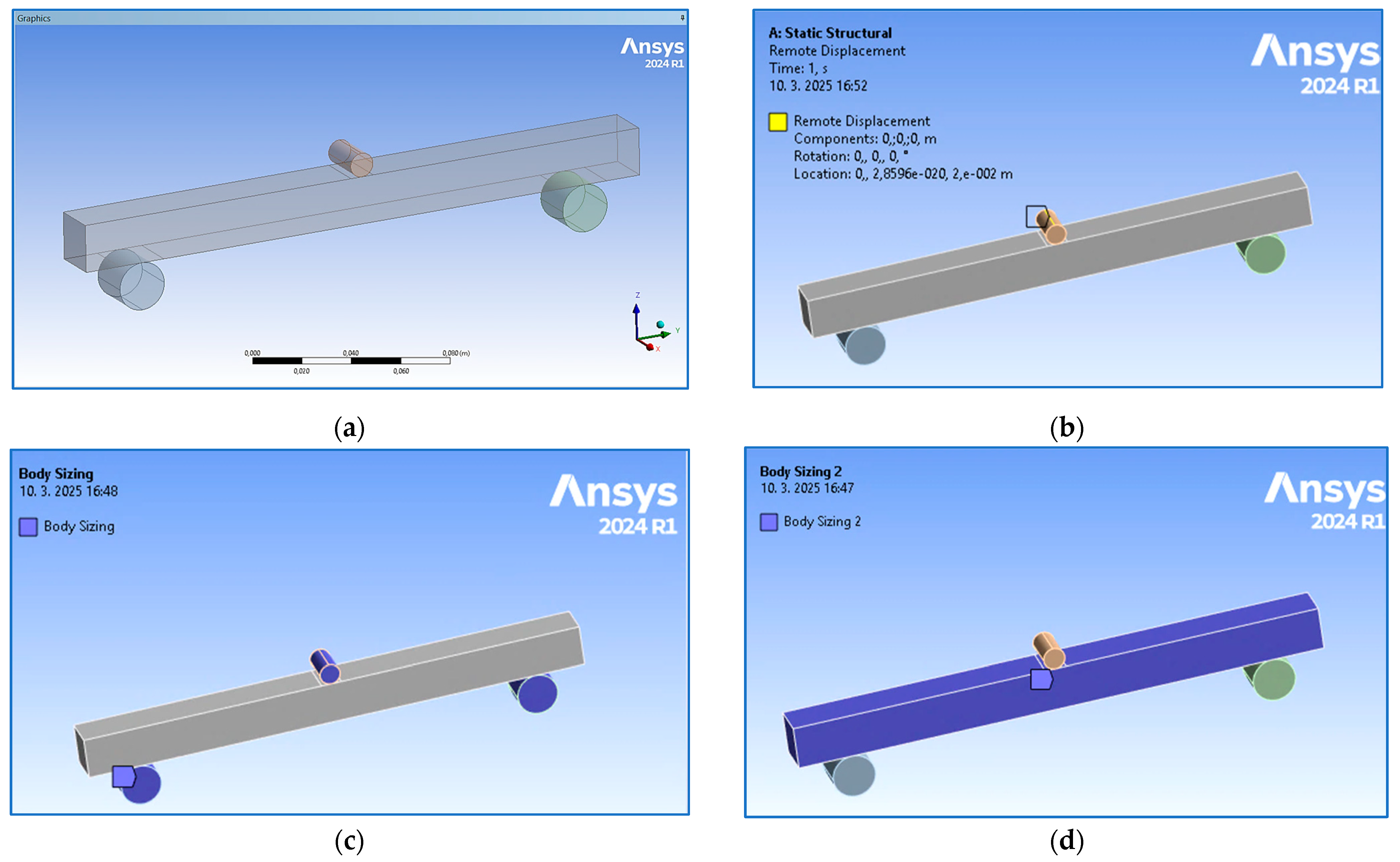
2.2.3. Numerical Analysis
3. Results and Discussion
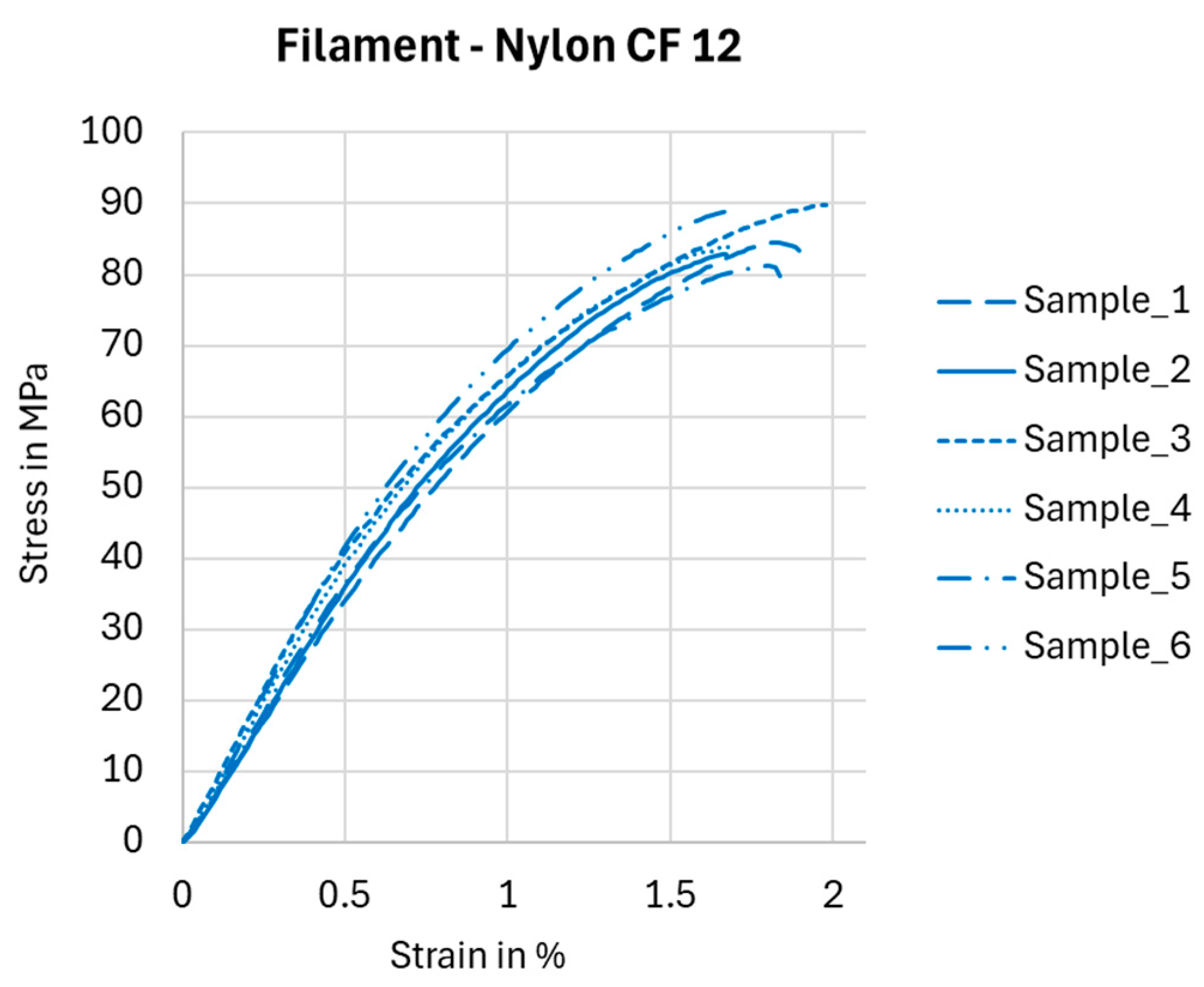
3.1. Preliminary Tests—Experimental Verification of Nylon CF12 Filament Tensile Properties
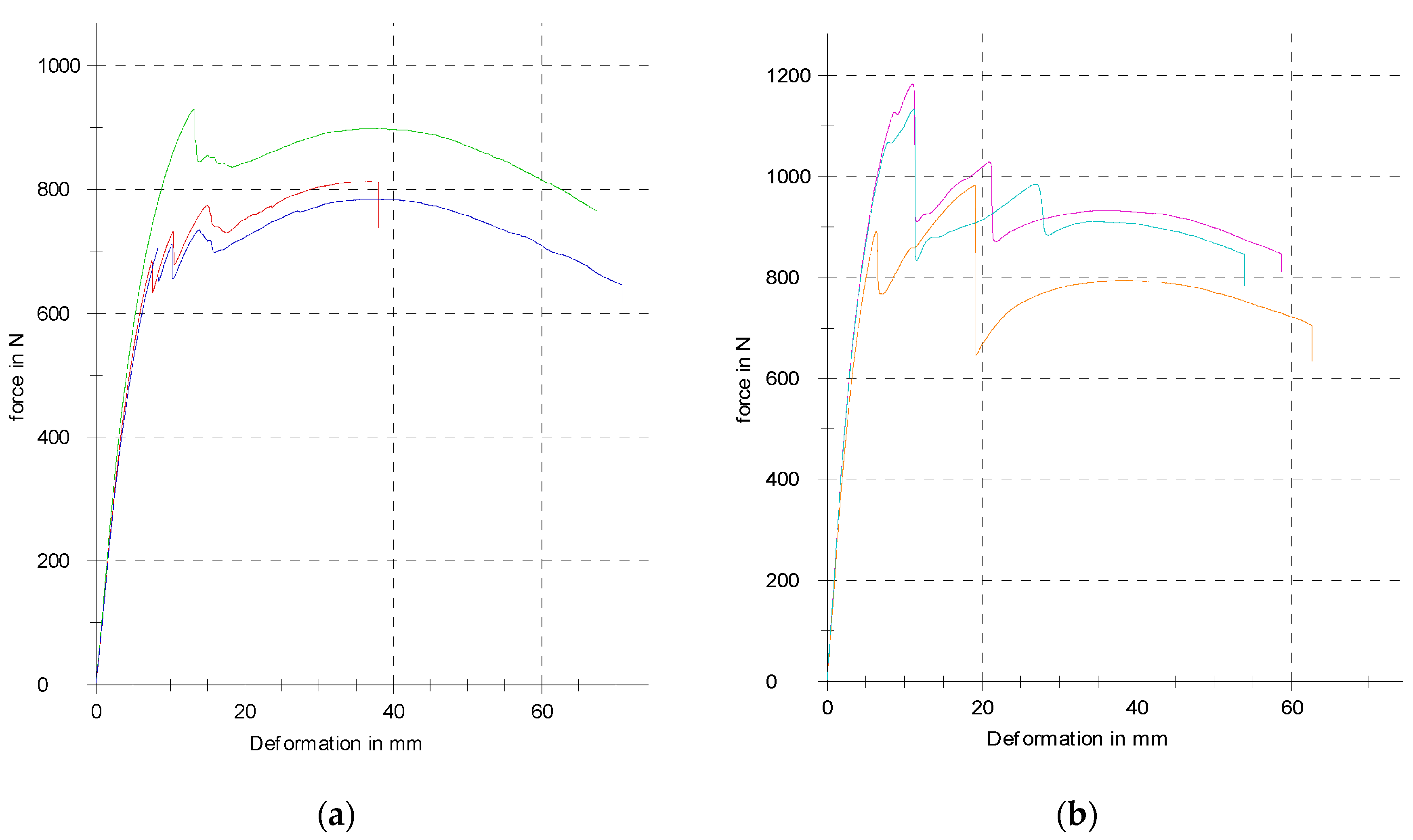
3.2. Research into the Effect of Layering Orientation on the Flexural Properties of 3D-Printed Samples with an Orbital Shell
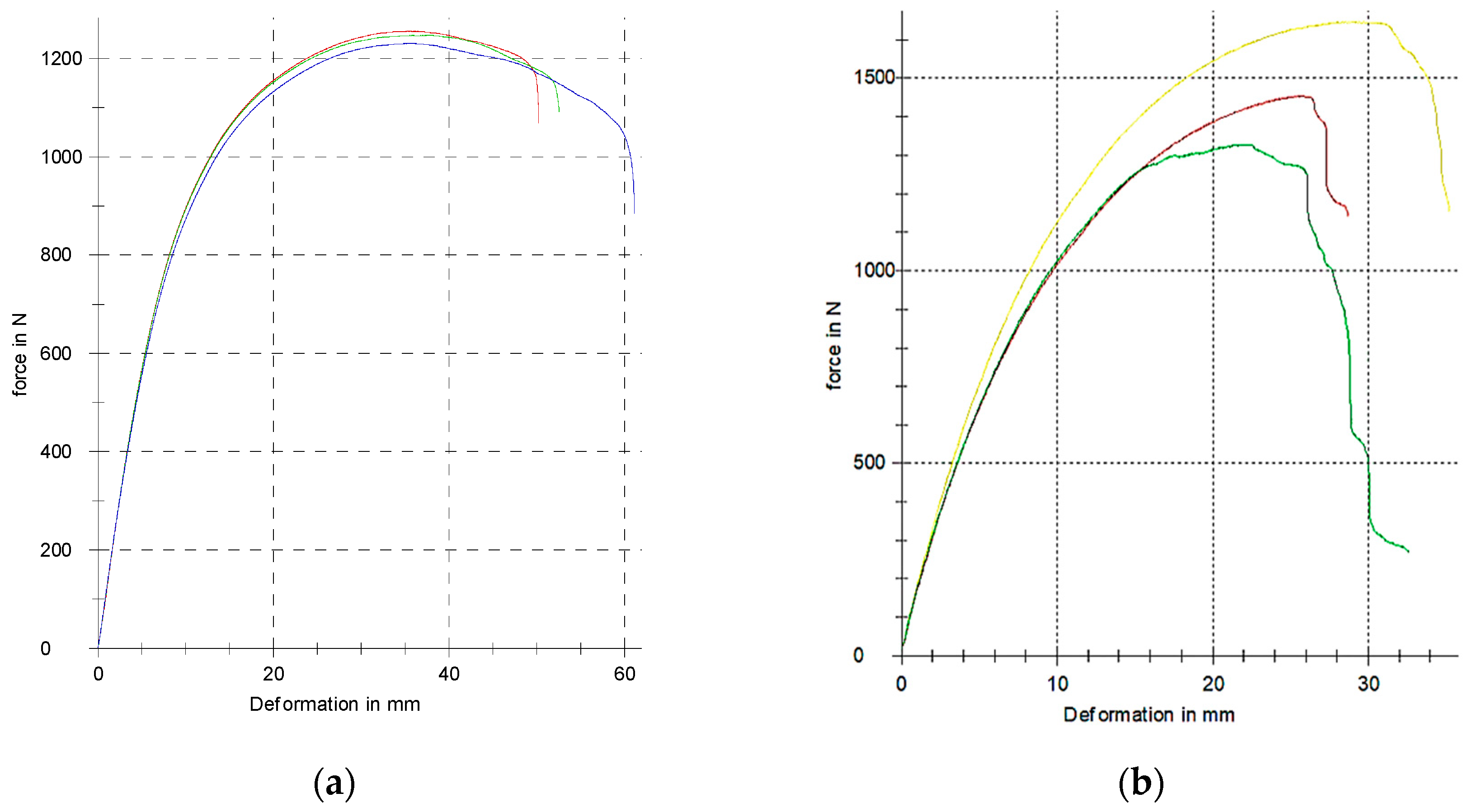
3.3. Research into the Effect of Layering Direction on the Flexural Properties of a 3D-Printed Full-Volume Body Without an Orbital Shell
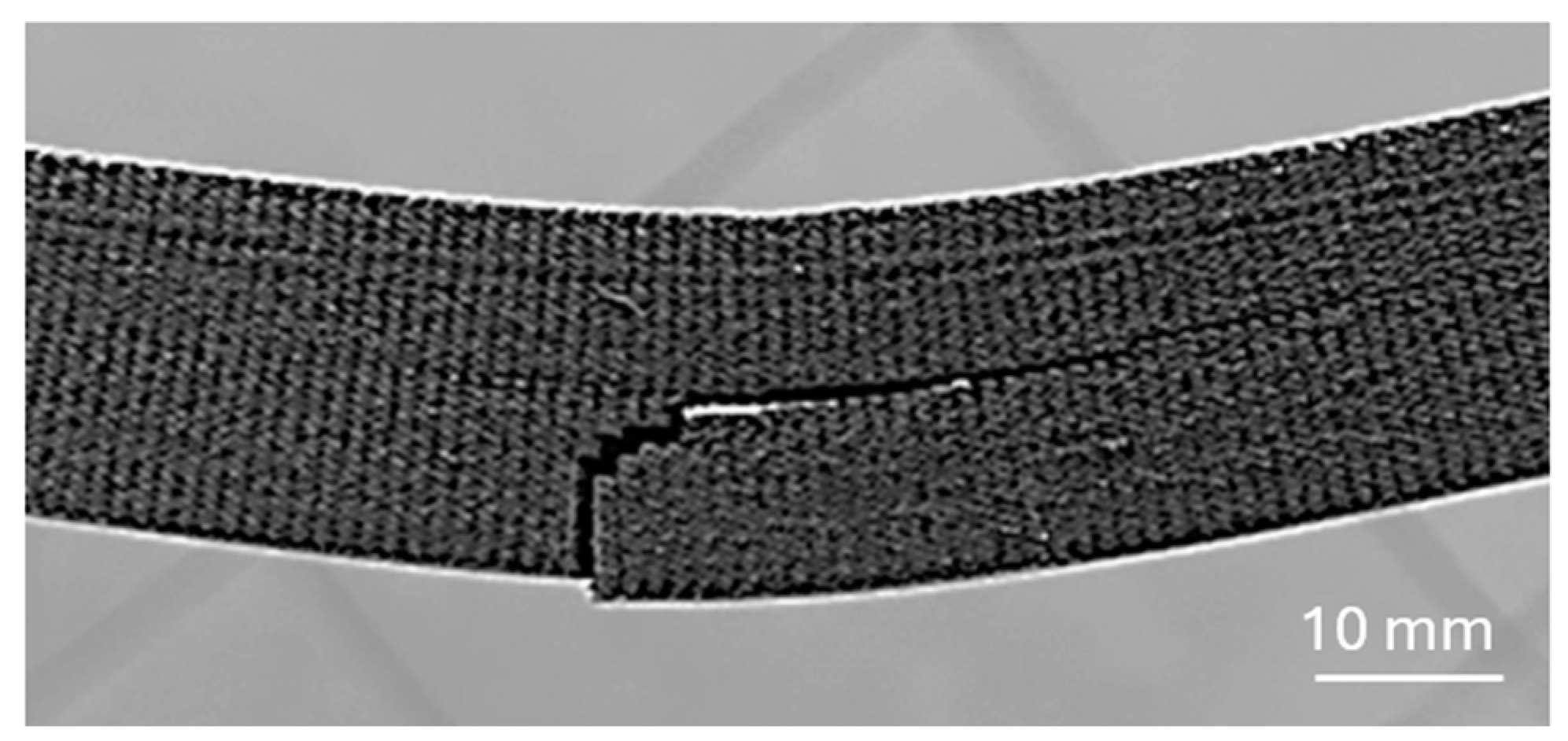
3.3.1. Experimental Testing
3.3.2. Numerical Analysis
3.4. Research into the Effect of Layering Direction on Flexural Properties of 3D-Printed Porous Samples with Cellular Structures
4. Conclusions
- (1)
- The flexural properties of a 3D-printed full-volume beam made of nylon CF12 by FFF are significantly influenced by the direction of loading relative to the layering, as well as by printing strategy. The highest maximum loads (1527 ± 136 N) were recorded for the beam without a shell at a perpendicular orientation; however, at this orientation lower forces were measured in the elastic region than for the parallel orientation, at which higher deformations were also measured. The results therefore indicate a more brittle behavior of the perpendicularly oriented specimens.
- (2)
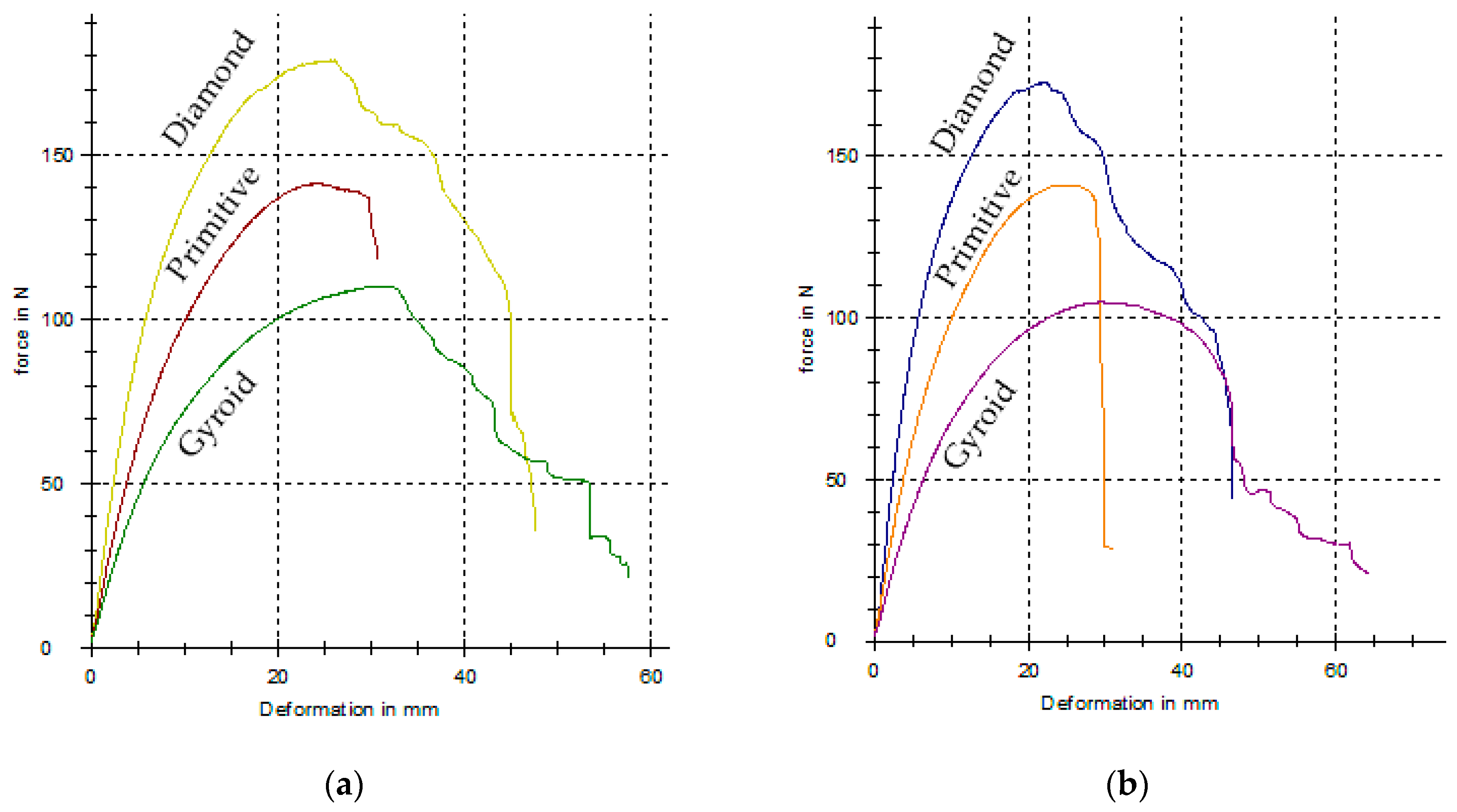
- In the case of porous beams with a cellular structure of 30% of the volume fraction, the differences in properties are very small. This is probably related not only to the adhesive forces between successive layers, but also to the layer formation strategy, i.e., the way in which the trajectories of the deposited material are generated. The best flexural properties were shown by the Diamond structure, followed by the Primitive structure, and the worst properties among those studied were achieved by the Gyroid structure.
- (3)
- There is a very good agreement between the experimentally and numerically achieved results within the linear behavior of the full-volume beam without an orbital shell.
- (4)
- The differences between the material properties listed in the datasheets and achieved within the research have been identified. This indicates that the properties of new bodies manufactured by 3D-printing using the FFF technique should first be verified experimentally; this is also due to the great diversity of materials, production conditions, and processing and testing parameters, which subsequently need to be taken into account in numerical analysis.
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Grbović, A.; Kastratović, G.; Božić, Ž.; Božić, I.; Obradović, A.; Sedmak, A.; Sedmak, S. Experimental and numerical evaluation of fracture characteristics of composite material used in the aircraft engine cover manufacturing. Eng. Fail. Anal. 2022, 137, 106286. [Google Scholar] [CrossRef]
- Bellini, C.; Borrelli, R.; Di Caprio, F.; Di Cocco, V.; Franchitti, S.; Iacoviello, F.; Mocanu, L.P.; Sorrentino, L. Manufacturing process effect on the bending characteristics of titanium-lattice/FRP hybrid structures. Procedia Struct. Integr. 2022, 42, 196–201. [Google Scholar] [CrossRef]
- Mitrovic, N.; Milosevic, M.; Momcilovic, N.; Petrovic, A.; Miskovic, Z.; Sedmak, A.; Popovic, P. Local Strain and Stress Analysis of Globe Valve Housing Subjected to External Axial Loading. In Key Engineering Materials; Trans Tech Publications Ltd.: Zurich, Switzerland, 2014; Volume 586, pp. 214–217. [Google Scholar]
- Kadkhodapour, J.; Montazerian, H.; Darabi, A.C.; Anaraki, A.P.; Ahmadi, S.M.; Zadpoor, A.A.; Schmauder, S. Failure mechanisms of additively manufactured porous biomaterials. J. Mech. Behav. Biomed. Mater. 2015, 50, 180–191. [Google Scholar] [CrossRef]
- Tkac, J.; Samborski, S.; Monkova, K.; Debski, H. Analysis of mechanical properties of a lattice structure produced with the additive technology. Compos. Struct. 2020, 242, 112138. [Google Scholar] [CrossRef]
- Adil, S.; Lazoglu, I. A review on additive manufacturing of carbon fiber-reinforced polymers: Current methods, materials, mechanical properties, applications and challenges. J. Appl. Polym. Sci. 2023, 140, e53476. [Google Scholar] [CrossRef]
- Dickson, A.N.; Abourayana, H.M.; Dowling, D.P. 3D Printing of Fibre-Reinforced Thermoplastic Composites Using Fused Filament Fabrication—A Review. Polymers 2020, 12, 2188. [Google Scholar] [CrossRef] [PubMed]
- Guo, K.; Ren, Y.; Han, G.; Xie, T.; Jiang, H. Hygrothermal aging and durability prediction of 3D-printed hybrid fiber composites with continuous carbon/Kevlar-fiber and short carbon-fiber. Eng. Fail. Anal. 2025, 167 Pt A, 108958. [Google Scholar] [CrossRef]
- Liam, T. Mondo Duplantis: Breaking down the Biomechanics Behind the Best-Ever Pole Vaulter. Available online: https://www.nytimes.com/athletic/5646961/2024/08/05/mondo-duplantis-biomechanics-pole-vault/ (accessed on 10 August 2024).
- Makaruk, H.; Porter, M.; Starzak, M.; Szymczak, E. An examination of approach run kinematics in track and field jumping events. Polish J. Sport Tour. 2016, 23, 82–87. [Google Scholar] [CrossRef]
- Linthorne, N.P. Energy loss in the pole vault take-off and the advantage of the flexible pole. Sports Eng. 2000, 3, 205–218. [Google Scholar] [CrossRef]
- Heck, A.; Uylings, P. Fierljeppen: Pole vaulting for distance. Phys. Educ. 2020, 55, 045007. [Google Scholar] [CrossRef]
- Chau, S.; Mukherjee, R. Kinetic to Potential Energy Transformation Using a Spring as an Intermediary: Application to the Pole Vault Problem. J. Appl. Mech. 2019. [Google Scholar] [CrossRef]
- Frere, J.; Sanchez, H.; Homo, S.; Rabita, G.; Morin, J.B.; Cassirame, J. Influence of pole carriage on sprint mechanical properties during pole vault run-up. Comput. Methods Biomech. Biomed. Engin. 2017, 20, 83–84. [Google Scholar] [CrossRef]
- Schade, F.; Arampatzis, A.; Bruggemann, G.-P. Reproducibility of energy parameters in the pole vault. J. Biomech. 2006, 39, 1464–1471. [Google Scholar] [CrossRef] [PubMed]
- Georgia Gwinnett College. The Future of Pole Vaulting; Technical Report; Georgia Gwinnett College: Lawrenceville, GA, USA, 2017. [Google Scholar]
- Morlier, J.; Mesnard, M.; Cid, M. Pole-vaulting: Identification of the pole local bending rigidities by an updating technique. J. Appl. Biomech. 2008, 24, 140–148. [Google Scholar] [CrossRef]
- Moore, A.; Hubbard, M. Modeling and real-time strain measurement of the non-uniform vaulting pole. In The Engineering of Sport 5; Hubbard, M., Mehta, R.D., Pallis, J.M., Eds.; Central Plain Books Manufacturing: Winfield, KS, USA, 2004; Volume 1, pp. 312–318. [Google Scholar]
- Xu, R.; Huang, Y.; Lin, Y.; Bai, B.; Huang, T. In-plane flexural behaviour and failure prediction of carbon fibre-reinforced aluminium laminates. J. Reinf. Plast. Compos. 2017, 36, 1384–1399. [Google Scholar] [CrossRef]
- De Toro, E.V.; Sobrino, J.C.; Martínez, A.M.; Eguía, V.M. Analysis of the influence of the variables of the fused deposition modeling (FDM) process on the mechanical properties of a carbon fiber-reinforced polyamide. Procedia Manuf. 2019, 41, 731–738. [Google Scholar] [CrossRef]
- Christodoulou, T.I.; Alexopoulou, V.E.; Markopoulos, A.P. An experimental investigation of the mechanical properties of fused filament fabricated nylon-carbon fiber composites. Cut. Tools Technol. Syst. 2024, 100, 148–167. [Google Scholar] [CrossRef]
- Bellini, C.; Di Cocco, V.; Iacoviello, F.; Sorrentino, L. Comparison between long and short beam flexure of a carbon fibre based FML. Procedia Struct. Integr. 2020, 26, 120–128. [Google Scholar] [CrossRef]
- Majko, J.; Handrik, M.; Vaško, M.; Piroh, O.; Vaško, A. Effect of v-notch on impact toughness of fibre reinforced laminates produced by fff method. Eng. Fail. Anal. 2024, 165, 108717. [Google Scholar] [CrossRef]
- Khosravani, M.R.; Reinicke, T. Effects of fiber on the fracture behavior of 3D-printed fiber reinforced nylon. Procedia Struct. Integr. 2022, 35, 59–65. [Google Scholar] [CrossRef]
- Lawcock, G.; Ye, L.; Mai, Y.; Sun, C. Effects of fibre/matrix adhesion on carbon-fibre-reinforced metal laminates—I. Compos. Sci. Technol. 1998, 57, 1609–1619. [Google Scholar] [CrossRef]
- FDM Nylon 12 Carbon Fiber Data Sheet; 2018 Stratasys Ltd. Available online: https://www.stratasys.com/siteassets/materials/materials-catalog/fdm-materials/nylon-12cf/mds_fdm_nylon-12cf_0921a.pdf?v=48e2e4 (accessed on 10 August 2024).
- Thompson, M.K.; Moroni, G.; Vaneker, T.; Fadel, G.; Campbell, R.I.; Gibson, I.; Bernard, A.; Schulz, J.; Graf, P.; Ahuja, B.; et al. Design for Additive Manufacturing: Trends, opportunities, considerations, and constraints. CIRP Ann. 2016, 65, 737–760. [Google Scholar] [CrossRef]
- Kinik, D.; Gánovská, B.; Hloch, S.; Monka, P.; Monková, K.; Hutyrová, Z. On-line monitoring of technological process of material abrasive water jet cutting. Teh. Vjesn.—Tech. Gaz. 2015, 22, 351–357. [Google Scholar] [CrossRef]
- Monkova, K.; Monka, P.P.; Vodilka, A. Comparison of the Bending Behavior of Cylindrically Shaped Lattice Specimens with Radially and Orthogonally Arranged Cells Made of ABS. Polymers 2024, 16, 979. [Google Scholar] [CrossRef]
- Monkova, K.; Monka, P.P.; Žaludek, M.; Beňo, P.; Hricová, R.; Šmeringaiová, A. Study of the Bending Behaviour of the Neovius Porous Structure Made Additively from Aluminium Alloy. Aerospace 2023, 10, 361. [Google Scholar] [CrossRef]
- Gado, M.G.; Al-Ketan, O.; Aziz, M.; Al-Rub, R.A.; Ookawara, S. Triply Periodic Minimal Surface Structures: Design, Fabrication, 3D Printing Techniques, State-of-the-Art Studies, and Prospective Thermal Applications for Efficient Energy Utilization. Energy Technol. 2024, 12, 2301287. [Google Scholar] [CrossRef]
- Korol, M.; Török, J.; Monka, P.P.; Baron, P.; Mrugalska, B.; Monkova, K. Researching on the Effect of Input Parameters on the Quality and Manufacturability of 3D-Printed Cellular Samples from Nylon 12 CF in Synergy with Testing Their Behavior in Bending. Polymers 2024, 16, 1429. [Google Scholar] [CrossRef]
- ISO 178:2019; Plastics Determination of Flexural Properties. ISO: Geneva, Switzerland, 2019.
- Korol, M. Research on the Adaptation of the Characteristics of Cellular Structures for the Distribution of Stress in the Components. Ph.D. Thesis, Faculty of Manufacturing Technologies with a seat in Presov, Technical University in Kosice, Kosice, Slovakia, 2024. [Google Scholar]
- Kahraman, M.F.; İriç, S.; Genel, K. Comparative failure behavior of metal honeycomb structures under bending: A finite element-based study. Eng. Fail. Anal. 2024, 157, 107963. [Google Scholar] [CrossRef]
- Heo, J.; Cho, N.-K.; Kim, D.K. Enhanced energy absorption and reusability of 3D printed continuous carbon fibre reinforced honeycomb beams under three-point bending loads. Eng. Struct. 2025, 329, 119877. [Google Scholar] [CrossRef]
- Oberlercher, H.; Heim, R.; Laux, M.; Berndt, A.; Becker, C.; Amancio-Filho, S.T.; Riemelmoser, F.O. Additive manufacturing of continuous carbon fiber reinforced polyamide 6: The effect of process parameters on the microstructure and mechanical properties. Procedia Struct. Integr. 2021, 34, 111–120. [Google Scholar] [CrossRef]
- ASTM D638; Standard Test Method for Tensile Properties of Plastics. ASTM International: West Conshohocken, PA, USA, 2022.
- Data-Sheet-EN-A4-FDM-Nylon-12CF-1; 2019 Ricoh Europe PLC. Available online: https://3d.ricoh.com/wp-content/uploads/2019/10/Ricoh-TDS-FDM-Nylon-12CF-Web-Final.pdf (accessed on 10 August 2024).
- MAKERBOT NYLON 12 Carbon Fiber, Data Sheet. Available online: https://asset.dynamism.com/media/catalog/product/pdf/MakerBot_Nylon_12_Carbon_Fiber_TDS.pdf (accessed on 10 August 2024).
- Vanca, J.; Monkova, K.; Žaludek, M.; Monka, P.P.; Koroľ, M.; Kozak, D.; Beno, P.; Ferroudji, F. Investigation of the Influence of Orientation on the Tensile Properties of 3D Printed Samples with Gyroid Structure. In Proceedings of the 2022 13th International Conference on Mechanical and Aerospace Engineering (ICMAE), Bratislava, Slovakia, 20–22 July 2022; pp. 526–531. [Google Scholar]
- Monkova, K.; Monka, P.P.; Koroľ, M.; Torok, J.; Beňo, P. The Effect of a Face Wall on a Cellular Structure During Bending. In Smart Cities: Importance of Management and Innovations for Sustainable Development. Mobility IoT 2023. EAI/Springer Innovations in Communication and Computing; Springer: Cham, Switzerland, 2024. [Google Scholar] [CrossRef]
- Khosravani, M.R.; Soltani, P.; Reinicke, T. Failure and fracture in adhesively bonded 3D-printed joints: An overview on the current trends. Eng. Fail. Anal. 2023, 153, 107574. [Google Scholar] [CrossRef]
- Rajput, M.S.; Pathak, H. Crack growth in sandwich-structured foam core graphite epoxy laminate composite using a phase-field modelling approach. Forces Mech. 2024, 16, 100284. [Google Scholar] [CrossRef]


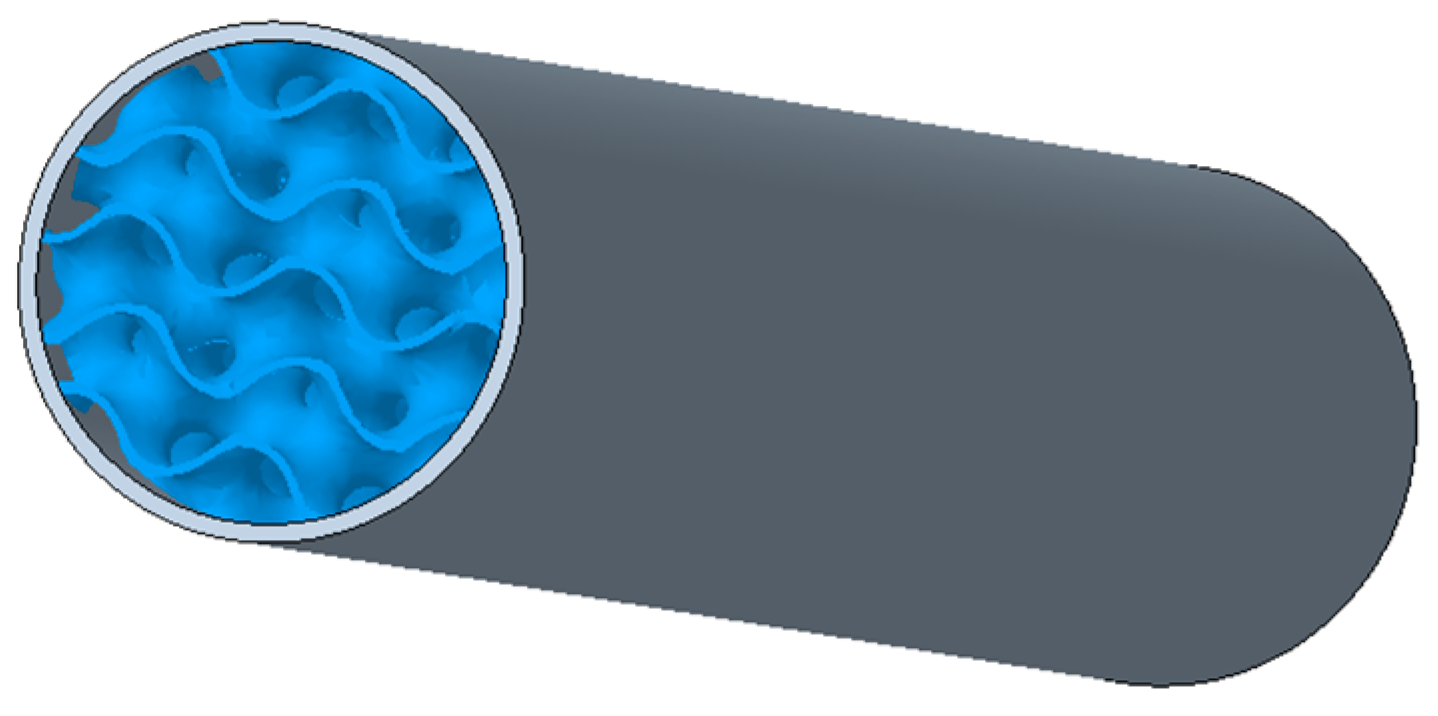






| Type of Structure | Equation [31] | Structure Visualization | |
|---|---|---|---|
| Schwarz Diamond | sin(x)sin(y)sin(z) + sin(x)cos(y)cos(z) + + cos(x)sin(y)cos(z) + cos(x) cos(y)sin(z) = 0 | (6) |  |
| Schwarz Primitive | cos(x) + cos(y) + cos(z) = 0 | (7) |  |
| Schoen Gyroid | sin(x)cos(y) + sin(y)cos(z) + sin(z)cos(x) = 0 | (8) |  |
| Description | Value | Unit | 3D-Printer—MakerBot Method X 3D |
|---|---|---|---|
| Nozzle diameter | 0.4 | mm |  |
| Basement and workspace temperatures | 65 | °C | |
| Print speed | 35 | mm/s | |
| Layer thickness | 0.15 | mm | |
| Heat treatment (post-processing) 1 | 80 | °C |
| Orientation of the Sample with Respect to the Layering | Elastic Force | Deflection at Elastic Force | Maximal Force | Deflection at Max Force | Flexural Modulus | Yield Strength | Total Absorbed Energy |
|---|---|---|---|---|---|---|---|
| (N) | (mm) | (N) | (mm) | (MPa) | (MPa) | (J) | |
| Parallel | 590 ± 23 | 5.7 ± 0.4 | 845 ± 82 | 14.1 ± 0.6 | 1293 ± 84 | 22.12 ± 0.7 | 40.12 ± 2.5 |
| Perpendicular | 780 ± 34 | 4.5 ± 0.3 | 1037 ± 141 | 9.5 ± 1.5 | 2167 ± 127 | 29.25 ± 1.3 | 52.07 ± 3.4 |
| Orientation of the Sample with Respect to the Layering | Elastic Force | Deflection at Elastic Force | Maximal Force | Deflection at Max Force | Flexural Modulus | Yield Strength | Total Absorbed Energy |
|---|---|---|---|---|---|---|---|
| (N) | (mm) | (N) | (mm) | (MPa) | (MPa) | (J) | |
| Parallel | 737 ± 15 | 7.1 ± 0.4 * | 1240 ± 45 | 36.3 ± 0.7 | 1297 ± 35 | 27.64 ± 0.5 ** | 57.35 ± 4.0 |
| Perpendicular | 635 ± 24 | 5.6 ± 0.5 | 1527 ± 136 | 26.9 ± 3.5 | 1044 ± 41 | 23.81 ± 0.9 | 37.33 ± 3.1 |
| Sample’s Orientation | Flexural Modulus (MPa) | Deflection at Elastic Force (mm) | Flexural Yield Strength (MPa) | Loading Force (N) | |||
|---|---|---|---|---|---|---|---|
| Measured | FEA | Calculated | FEA | Measured | FEA | ||
| Parallel | 1297 | 7.1 | 7.119 * | 27.64 | 27.787 ** | 737 | 364.93 *** |
| Perpendicular | 1044 | 5.6 | 5.621 | 23.81 | 24.133 | 635 | 312.97 |
| Type of Structure | Orientation of the Sample with Respect to the Layering | Elastic Force | Deflection at Elastic Force | Maximal Force | Deflection at Max Force | Yield Strength | Total Absorbed Energy |
|---|---|---|---|---|---|---|---|
| (N) | (mm) | (N) | (mm) | (MPa) | (J) | ||
| Diamond | Parallel | 108 ± 5.2 | 6.5 ± 0.3 | 179 ± 6.6 | 25.6 ± 1.2 | 25.1 | 6.41 ± 0.8 |
| Perpendicular | 126 ± 6.3 | 8.4 ± 0.4 | 173 ± 7.1 | 22.2 ± 1.0 | 27.0 | 5.90 ± 0.7 | |
| Primitive | Parallel | 86 ± 3.8 | 7.8 ± 0.3 | 141 ± 5.5 | 23.9 ± 1.1 | 16.0 | 3.27 ± 0.3 |
| Perpendicular | 83 ± 4.0 | 7.4 ± 0.3 | 141 ± 5.0 | 23.9 ± 1.0 | 15.7 | 3.17 ± 0.3 | |
| Gyroid | Parallel | 64 ± 3.2 | 8.1 ± 0.3 | 110 ± 4.6 | 31.7 ± 1.7 | 14.6 | 4.31 ± 0.4 |
| Perpendicular | 67 ± 2.7 | 9.8 ± 0.4 | 105 ± 4.1 | 29.4 ± 1.6 | 12.8 | 4.51 ± 0.5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Monkova, K.; Monka, P.P.; Burgerova, J.; Szabo, G. Investigating the Flexural Properties of 3D-Printed Nylon CF12 with Respect to the Correlation Between Loading and Layering Directions. Polymers 2025, 17, 788. https://doi.org/10.3390/polym17060788
Monkova K, Monka PP, Burgerova J, Szabo G. Investigating the Flexural Properties of 3D-Printed Nylon CF12 with Respect to the Correlation Between Loading and Layering Directions. Polymers. 2025; 17(6):788. https://doi.org/10.3390/polym17060788
Chicago/Turabian StyleMonkova, Katarina, Peter Pavol Monka, Jana Burgerova, and Gyula Szabo. 2025. "Investigating the Flexural Properties of 3D-Printed Nylon CF12 with Respect to the Correlation Between Loading and Layering Directions" Polymers 17, no. 6: 788. https://doi.org/10.3390/polym17060788
APA StyleMonkova, K., Monka, P. P., Burgerova, J., & Szabo, G. (2025). Investigating the Flexural Properties of 3D-Printed Nylon CF12 with Respect to the Correlation Between Loading and Layering Directions. Polymers, 17(6), 788. https://doi.org/10.3390/polym17060788









