Analysis of Residual Stress at the Interface of Epoxy-Resin/Silicon-Wafer Composites During Thermal Aging
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Sample Preparation
2.2.1. Preparation of Epoxy-Resin Specimens (EP-Ss)
2.2.2. Preprocessing of Silicon Wafers
2.2.3. Preparation of EP-SWs
2.3. Thermal Aging Process
2.4. Characterization and Measurement
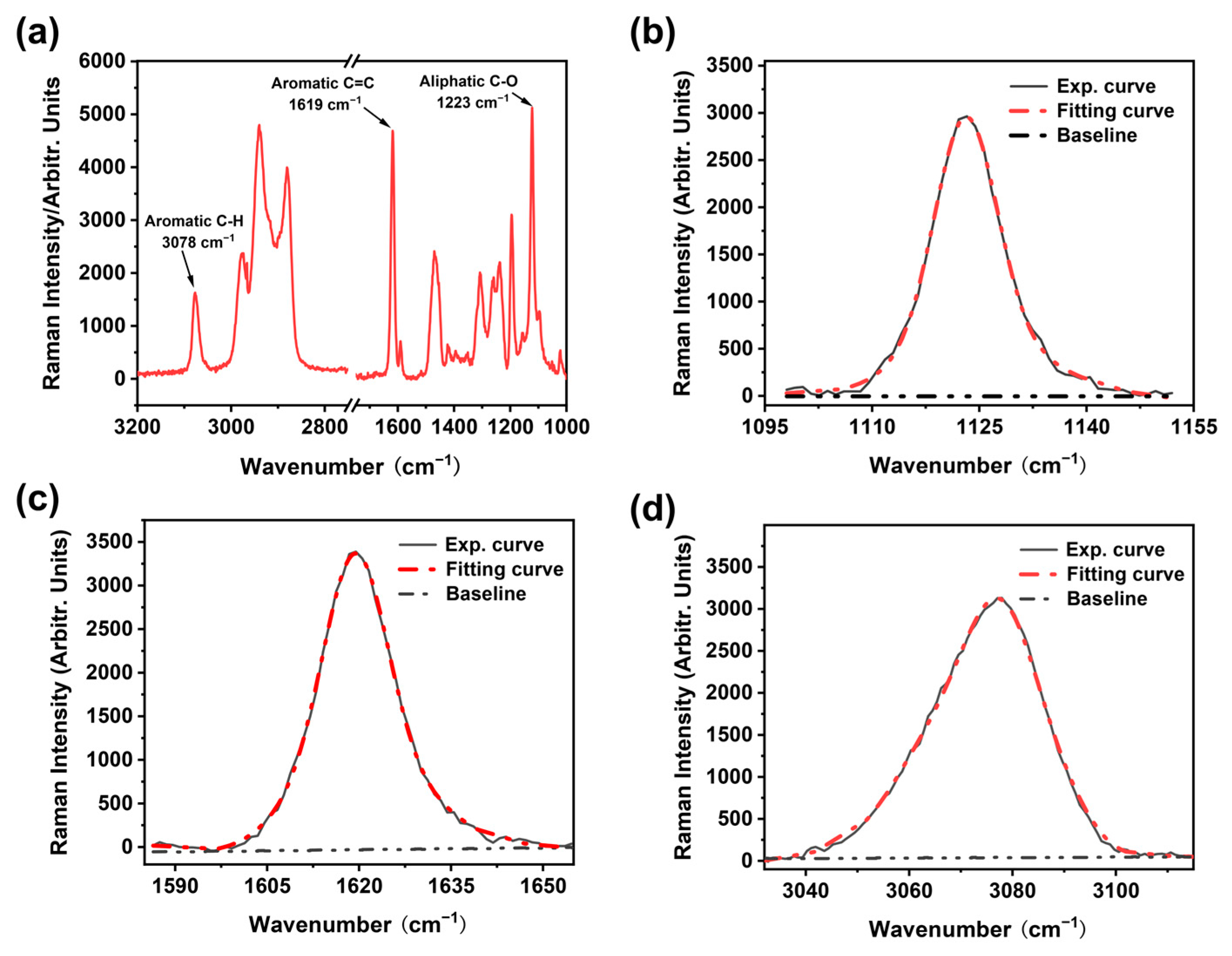
2.4.1. RS Measurement
Chemical Structure Analysis
Glass Transition Measurement
Micromorphology Characterization
3. Results and Discussion
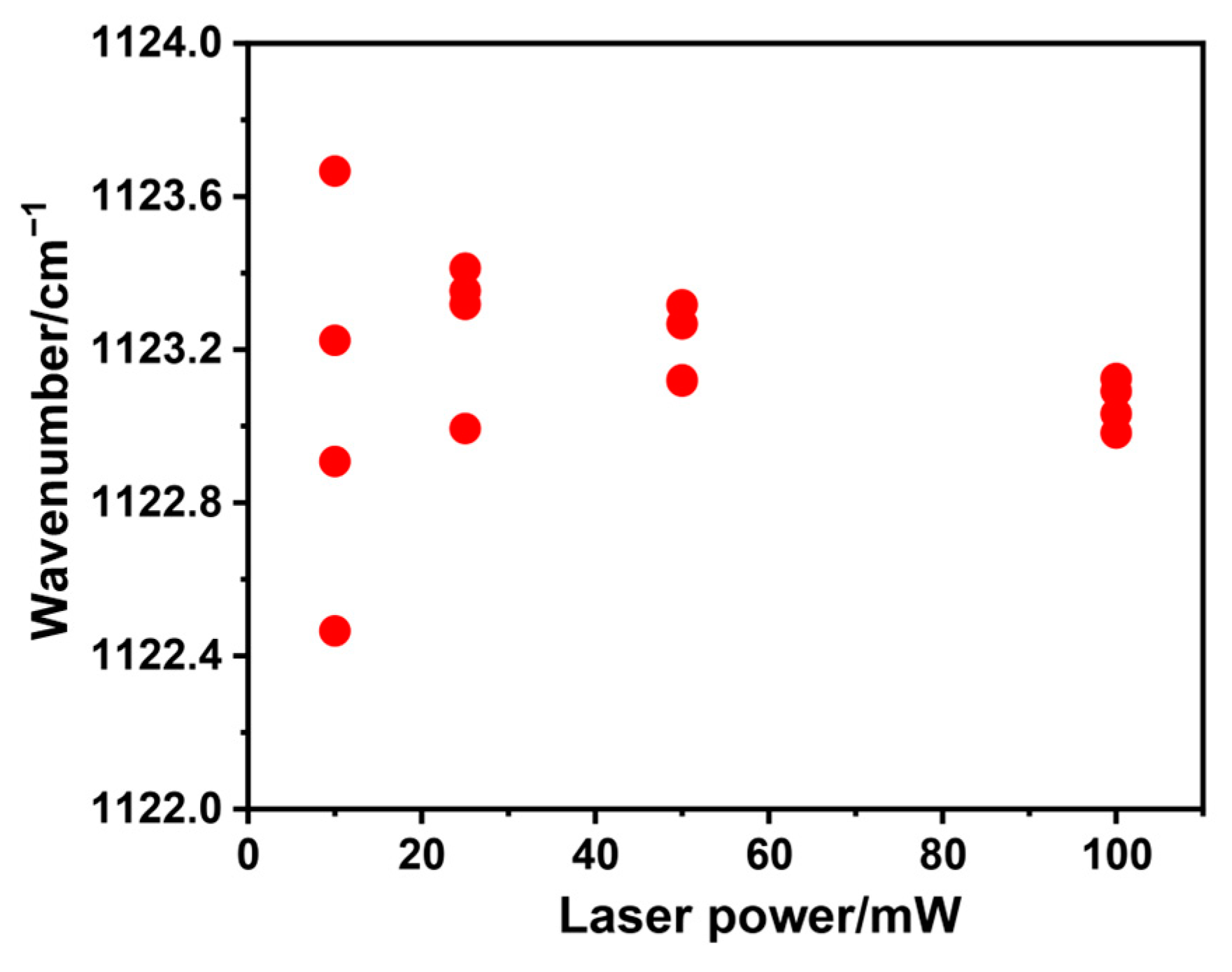
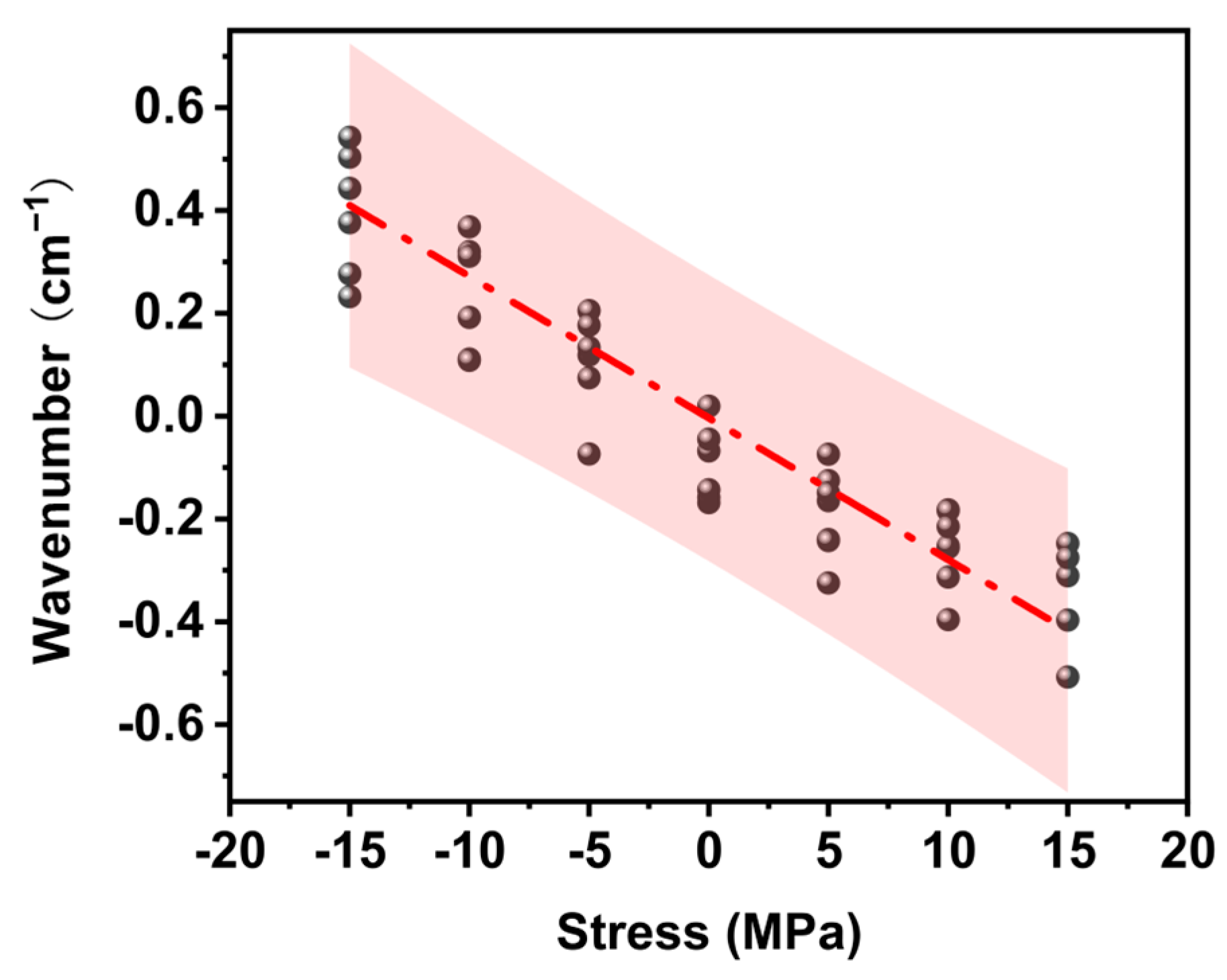
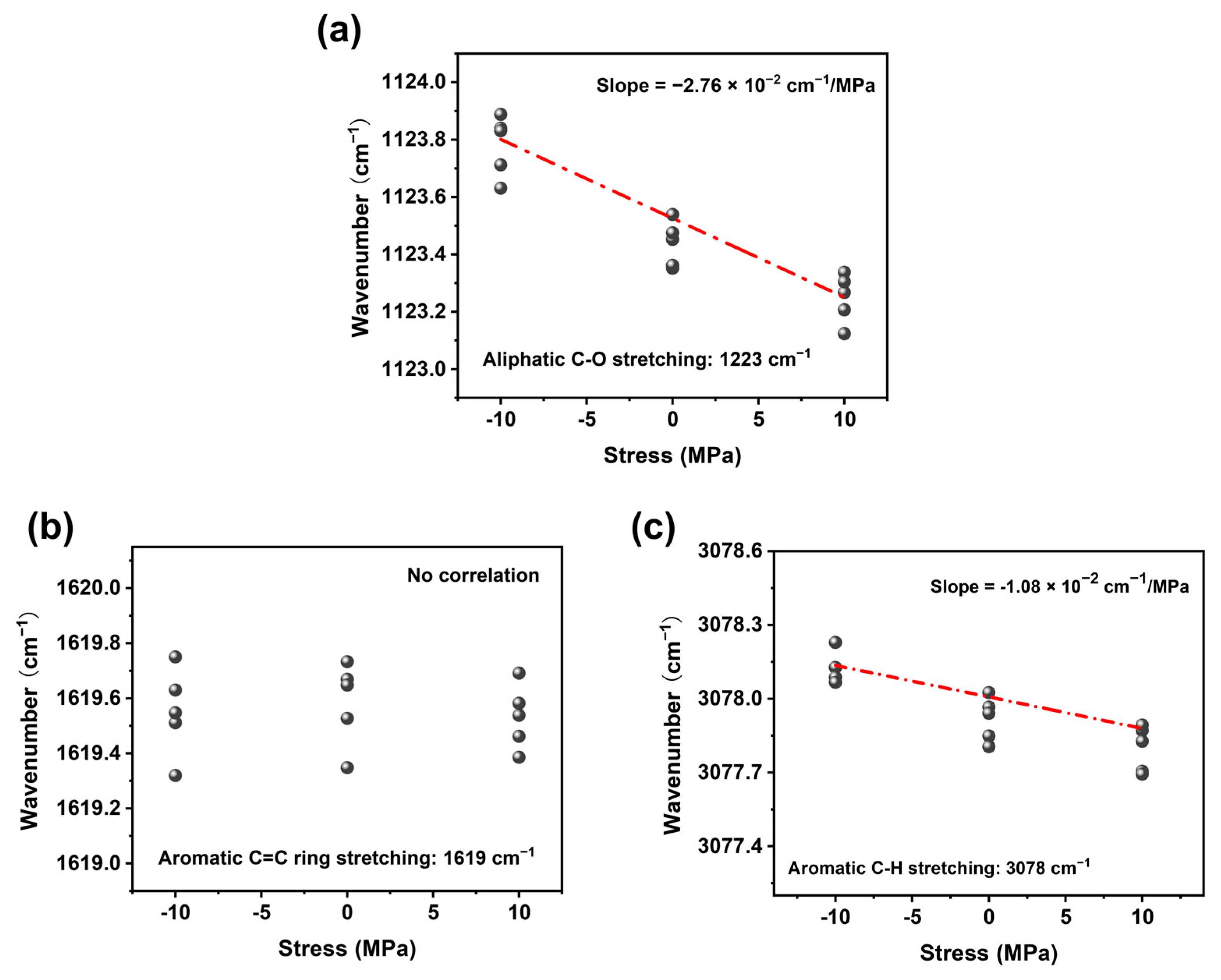
3.1. Relationship Between Peak Shift and Stress
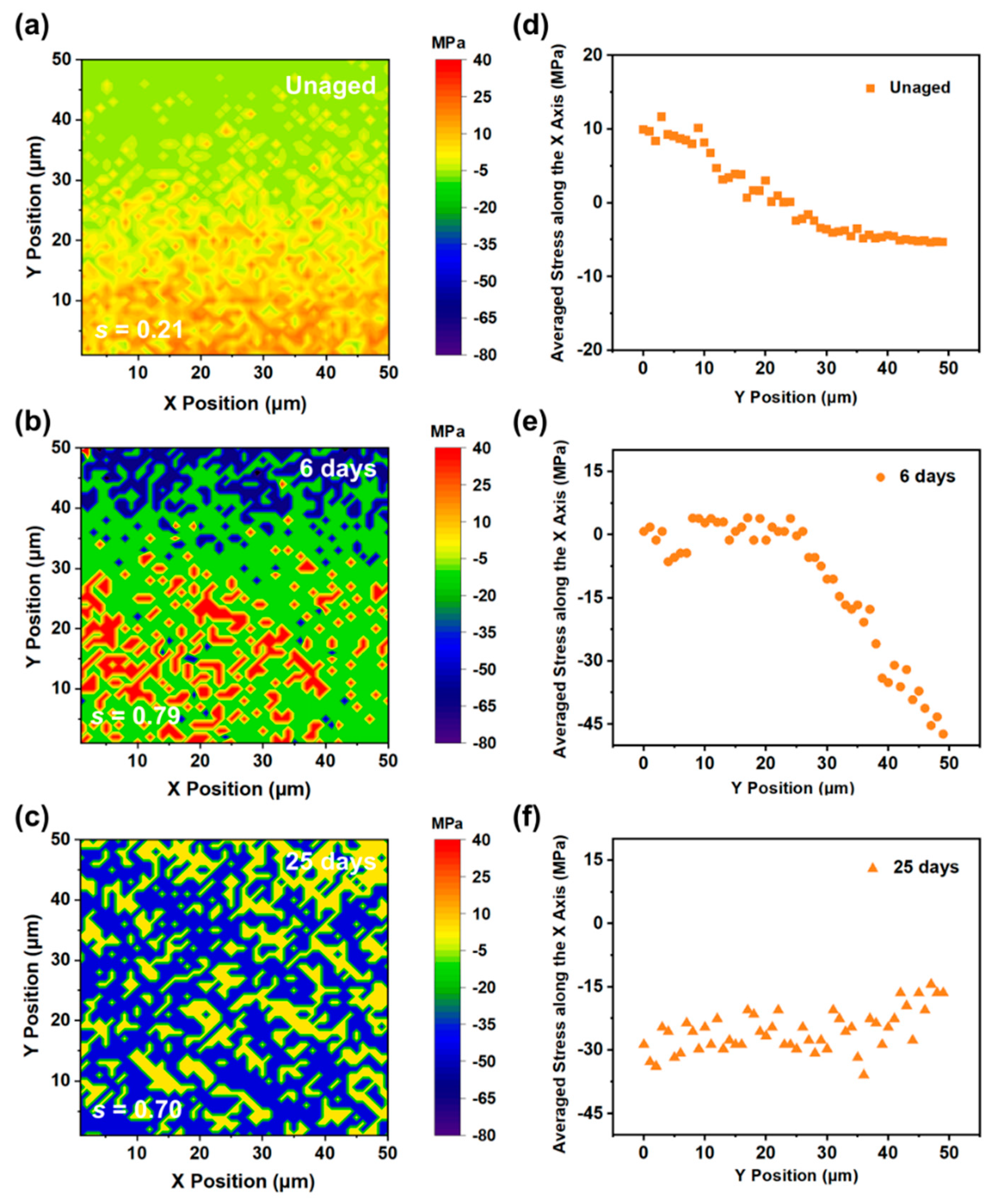
3.2. Imaging of RS Distribution
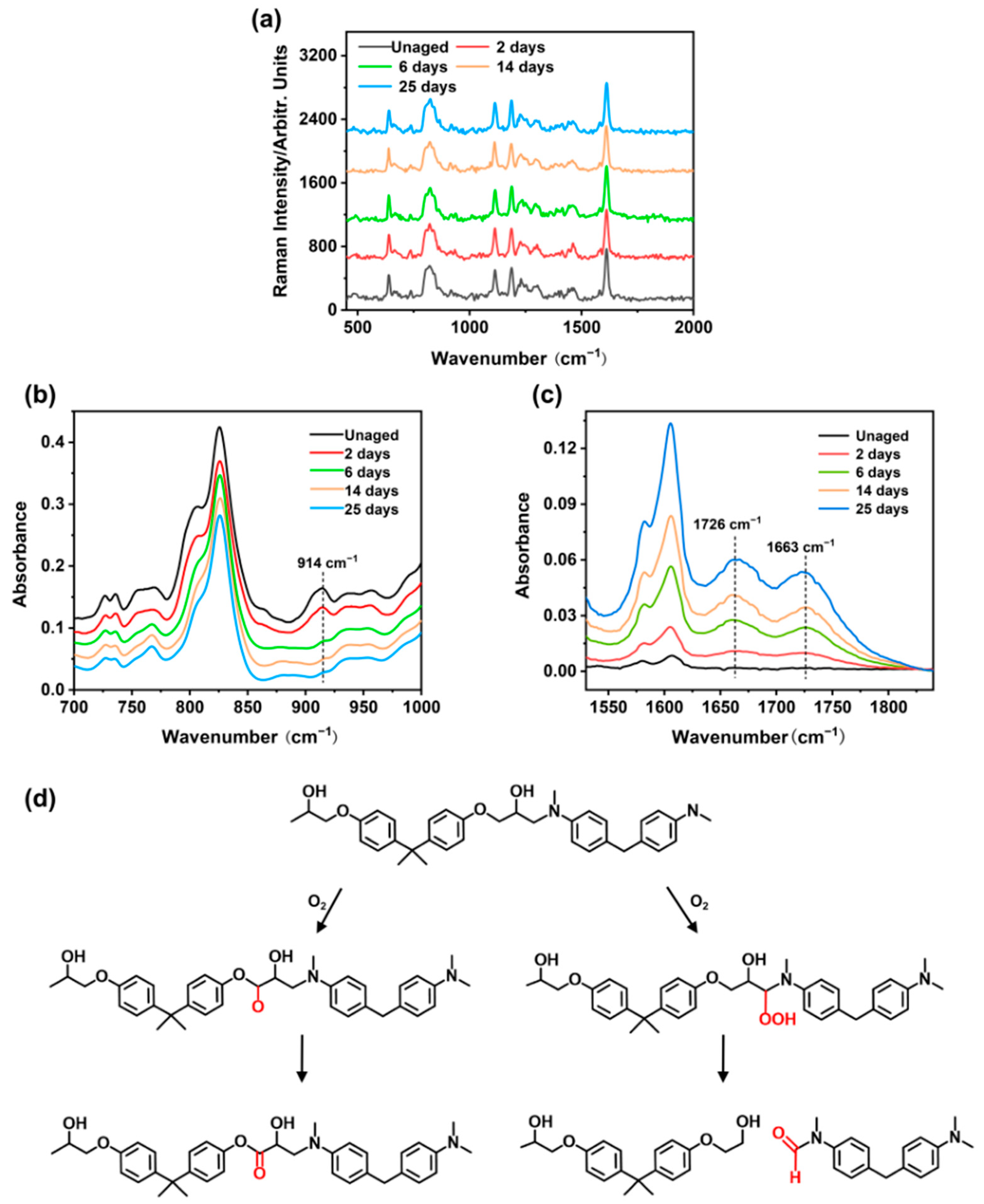
3.3. Thermal Aging Analysis
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Liu, J.; Zhang, L.; Shun, W.; Dai, J.; Peng, Y.; Liu, X. Recent development on bio-based thermosetting resins. J. Polym. Sci. 2021, 59, 1474–1490. [Google Scholar] [CrossRef]
- Zhang, Y.; Liu, X.; Wan, M.; Zhu, Y.; Zhang, K. Recent development of functional bio-based epoxy resins. Molecules 2024, 29, 4428. [Google Scholar] [CrossRef]
- Amirbeygi, H.; Khosravi, H.; Tohidlou, E. Reinforcing effects of aminosilane-functionalized graphene on the tribological and mechanical behaviors of epoxy nanocomposites. J. Appl. Polym. Sci. 2019, 136, 47410. [Google Scholar] [CrossRef]
- Mostovoi, A.S.; Kurbatova, E.A. Controlling the properties of epoxy composites filled with brick dust. Russ. J. Appl. Chem. 2017, 90, 246–256. [Google Scholar] [CrossRef]
- Zhang, Y.; Peng, C.; Wang, G.; Huang, X.; Yu, Y. Hydroxyl-free epoxy thermoplastics from active esters with low dielectric constant and water sorption. ACS Appl. Electron. Mater. 2024, 6, 4578–4586. [Google Scholar] [CrossRef]
- Capricho, J.C.; Fox, B.; Hameed, N. Multifunctionality in epoxy resins. Polym. Rev. 2020, 60, 1–41. [Google Scholar] [CrossRef]
- Ellis, B. Introduction to the chemistry, synthesis, manufacture and characterization of epoxy resins. In Chemistry and Technology of Epoxy Resins; Ellis, B., Ed.; Springer: Dordrecht, The Netherlands, 1993; pp. 1–36. [Google Scholar]
- Sui, H.; An, D.; Yang, K.; Zhang, Z.; Zhang, X.; Zhou, F.; Li, J.; Wu, K. Anomalous residual stress behavior and corresponding dielectric properties of epoxy resin induced by physical aging. IEEE Trans. Dielectr. Electr. Insul. 2023, 30, 1787–1794. [Google Scholar] [CrossRef]
- Bauhofer, A.A.; Krödel, S.; Rys, J.; Bilal, O.R.; Constantinescu, A.; Daraio, C. Harnessing photochemical shrinkage in direct laser writing for shape morphing of polymer sheets. Adv. Mater. 2017, 29, 1703024. [Google Scholar] [CrossRef]
- Tian, W.; Chen, X.; Zhang, G.; Chen, Y.; Luo, J. Delamination of plasticized devices in dynamic service environments. Micromachines 2024, 15, 376. [Google Scholar] [CrossRef]
- Pan, C.-T.; Wang, S.-Y.; Yen, C.-K.; Ho, C.-K.; Yen, J.-F.; Chen, S.-W.; Fu, F.-R.; Lin, Y.-T.; Lin, C.-H.; Kumar, A.; et al. Study on delamination between polymer materials and metals in IC packaging process. Polymers 2019, 11, 940. [Google Scholar] [CrossRef] [PubMed]
- Liu, L.; Li, E.; Luo, X. Study on delamination variation trend of two plastic packaging devices in combination reliability test. In Proceedings of the 2017 18th International Conference on Electronic Packaging Technology (ICEPT), Harbin, China, 16–19 August 2017; pp. 231–234. [Google Scholar]
- Wang, Y.; Ding, Y.; Yin, Y. Reliability of wide band gap power electronic semiconductor and packaging: A review. Energies 2022, 15, 6670. [Google Scholar] [CrossRef]
- Watanabe, Y.; Yamaguchi, H.; Enomoto, T.; Ogawa, K.; Kobayashi, T.; Omiya, M. Evaluation of thermal fatigue crack propagation in underfill resin materials for electronic packages. Fatigue Fract. Eng. Mater. Struct. 2022, 45, 1349–1360. [Google Scholar] [CrossRef]
- Li, M.; Min, Z.; Shi, Z.; Wang, Q.; Huang, W.; Shao, K. Research on the thermal oxygen aging mechanism of the composite curing system epoxy asphalt with different curing agent proportions on the molecular scale. J. Appl. Polym. Sci. 2023, 140, e54308. [Google Scholar] [CrossRef]
- Izumi, A.; Kakara, T.; Otsuki, M.W.; Shudo, Y.; Koganezawa, T.; Shibayama, M. In situ residual stress analysis in a phenolic resin and copper composite material during curing. Polymer 2019, 182, 121857. [Google Scholar] [CrossRef]
- Sham, M.-L.; Kim, J.-K. Evolution of residual stresses in modified epoxy resins for electronic packaging applications. Compos. Part A 2004, 35, 537–546. [Google Scholar] [CrossRef]
- Kravchenko, O.G.; Li, C.; Strachan, A.; Kravchenko, S.G.; Pipes, R.B. Prediction of the chemical and thermal shrinkage in a thermoset polymer. Compos. Part A 2014, 66, 35–43. [Google Scholar] [CrossRef]
- Middleton, J.; Hoffman, J.; Burks, B.; Predecki, P.; Kumosa, M. Aging of a polymer core composite conductor: Mechanical properties and residual stresses. Compos. Part A 2015, 69, 159–167. [Google Scholar] [CrossRef]
- Cysne Barbosa, A.P.; Fulco, A.P.P.; Guerra, E.S.S.; Arakaki, F.K.; Tosatto, M.; Costa, M.C.B.; Melo, J.D.D. Accelerated aging effects on carbon fiber/epoxy composites. Compos. Part B 2017, 110, 298–306. [Google Scholar] [CrossRef]
- Sim, K.-B.; Lee, T.-H.; Han, G.-Y.; Kim, H.-J. Thermal expansion and mechanical properties of urethane-modified epoxy bonded CFRP/steel joints at low and high temperatures for automotive. Compos. Struct. 2023, 322, 117426. [Google Scholar] [CrossRef]
- Nakamura, H.; Sato, C.; Ikegami, K. Internal stress induced during curing process of adhesively bonded parts of resin and metal. Zairyo 1997, 46, 820–825. [Google Scholar]
- Abiko, K.; Kato, Y.; Hohjo, H.; Kishida, Y.; Sudo, E. Raman imaging of residual stress distribution in epoxy resin and metal interface. J. Raman Spectrosc. 2020, 51, 193–200. [Google Scholar] [CrossRef]
- Wu, K.; An, D.; Zhang, Z.; Zhao, G.; Cui, C.; Zhou, F.; Li, J. Relief of residual stress in bulk thermosets in the glassy state by local bond exchange. Macromol. Rapid Commun. 2024, 45, 2300735. [Google Scholar] [CrossRef]
- Gouadec, G.; Colomban, P. Raman Spectroscopy of nanomaterials: How spectra relate to disorder, particle size and mechanical properties. Prog. Cryst. Growth Charact. Mater. 2007, 53, 1–56. [Google Scholar] [CrossRef]
- Deng, Y.; Wang, Q.; Ma, J.; Oh, J.T.; Chen, Z. The combined impact of voids and thermal aging on the mechanical reliability of epoxy resin evaluated by statistical analysis. Polym. Degrad. Stab. 2023, 215, 110455. [Google Scholar] [CrossRef]
- Morsch, S.; Liu, Y.; Lyon, S.B.; Gibbon, S.R.; Gabriele, B.; Malanin, M.; Eichhorn, K.J. Examining the early stages of thermal oxidative degradation in epoxyamine resins. Polym. Degrad. Stab. 2020, 186, 108030. [Google Scholar] [CrossRef]
- Ng, J.X.Y.; Cao, Q.; Golovanevskiy, V.; Farhat, H.; Iannuzzi, M.; Pojtanabuntoeng, T. A structured approach to the determination of residual stresses in an epoxy-amine coating and the influence of thermal ageing on the thermomechanical properties, residual stresses and the corresponding failure modes of coating. Prog. Org. Coat. 2024, 186, 108030. [Google Scholar] [CrossRef]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, J.; Chen, F.; Liu, J.; Chen, R.; Liu, P.; Zhao, H.; Zhao, Z. Analysis of Residual Stress at the Interface of Epoxy-Resin/Silicon-Wafer Composites During Thermal Aging. Polymers 2025, 17, 50. https://doi.org/10.3390/polym17010050
Wu J, Chen F, Liu J, Chen R, Liu P, Zhao H, Zhao Z. Analysis of Residual Stress at the Interface of Epoxy-Resin/Silicon-Wafer Composites During Thermal Aging. Polymers. 2025; 17(1):50. https://doi.org/10.3390/polym17010050
Chicago/Turabian StyleWu, Jianyu, Fangzhou Chen, Jiahao Liu, Rui Chen, Peijiang Liu, Hao Zhao, and Zhenbo Zhao. 2025. "Analysis of Residual Stress at the Interface of Epoxy-Resin/Silicon-Wafer Composites During Thermal Aging" Polymers 17, no. 1: 50. https://doi.org/10.3390/polym17010050
APA StyleWu, J., Chen, F., Liu, J., Chen, R., Liu, P., Zhao, H., & Zhao, Z. (2025). Analysis of Residual Stress at the Interface of Epoxy-Resin/Silicon-Wafer Composites During Thermal Aging. Polymers, 17(1), 50. https://doi.org/10.3390/polym17010050






