Fracture Toughness, Radiation Hardness, and Processibility of Polymers for Superconducting Magnets
Abstract
1. Introduction
2. Materials and Methods
2.1. The Samples
2.1.1. CTD101K
2.1.2. POLAB Mix (CTD101K+DY040)
2.1.3. CEA Mix
2.1.4. MSUT
2.1.5. Araldite F
2.1.6. MY750
2.1.7. Mix61
2.1.8. Polyurethane
2.1.9. PMMA and PC
2.2. Fracture Toughness Measurements
2.3. Dynamic Mechanical Analysis (DMA)
2.4. Viscosity Measurements
2.5. Gamma Irradiation
2.6. Proton Irradiation
3. Results
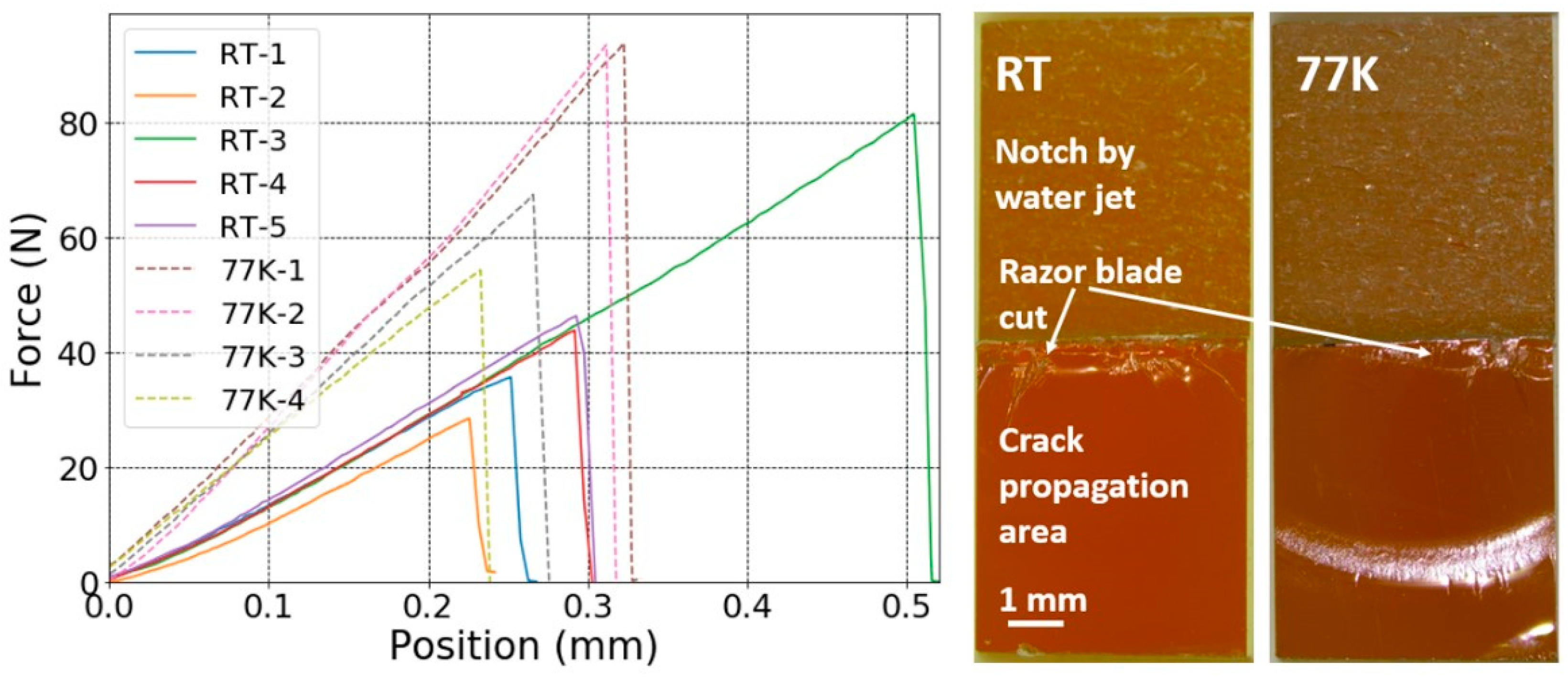
3.1. RT and 77 K Fracture Toughness of CTD101K with Different Flexibiliser Content
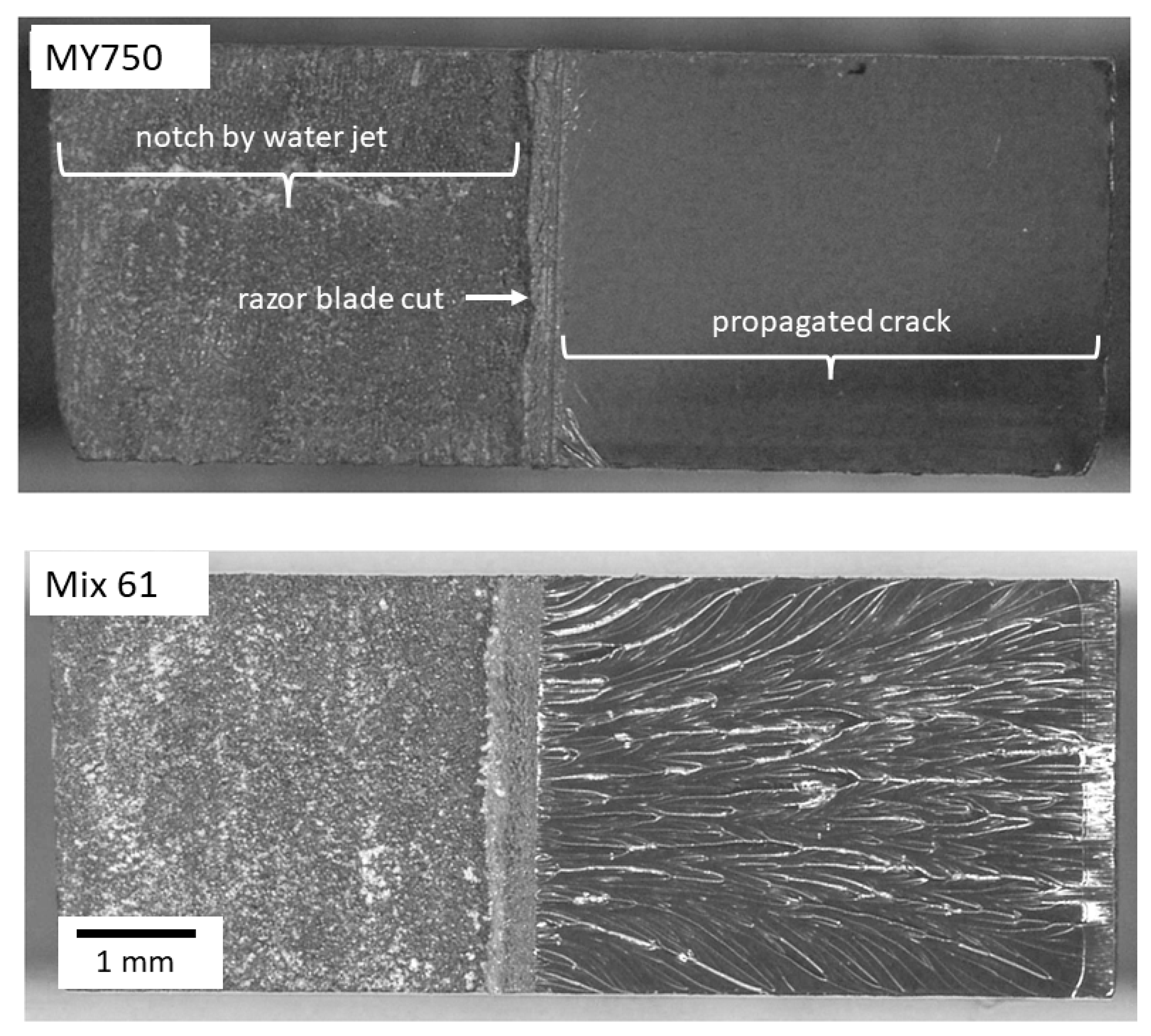
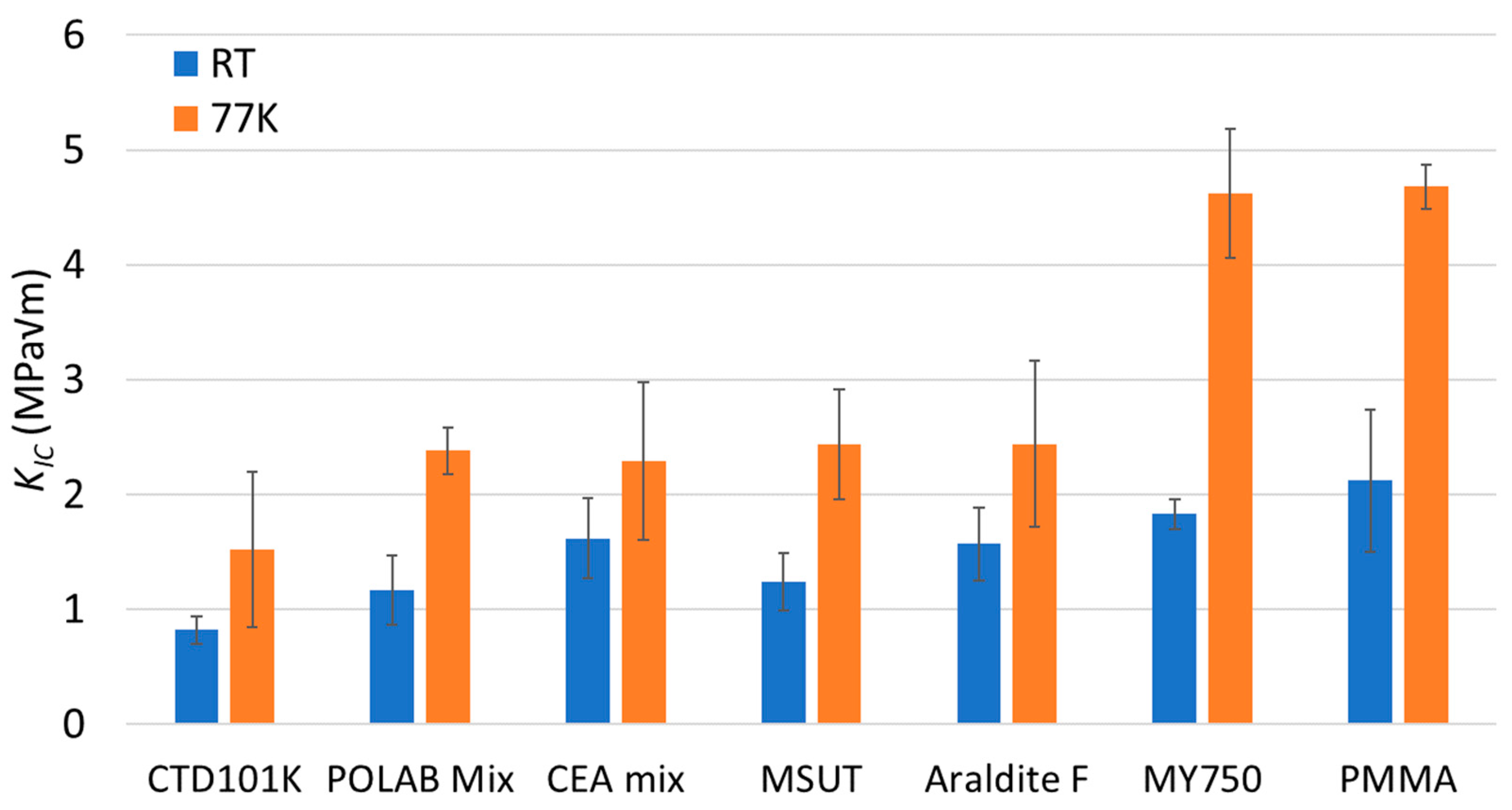
3.2. RT and 77 K Fracture Toughness of the Polymers Exhibiting Linear Elastic Behaviours and Unstable Crack Propagation
3.3. Fracture Toughness of Polyurethane and PC
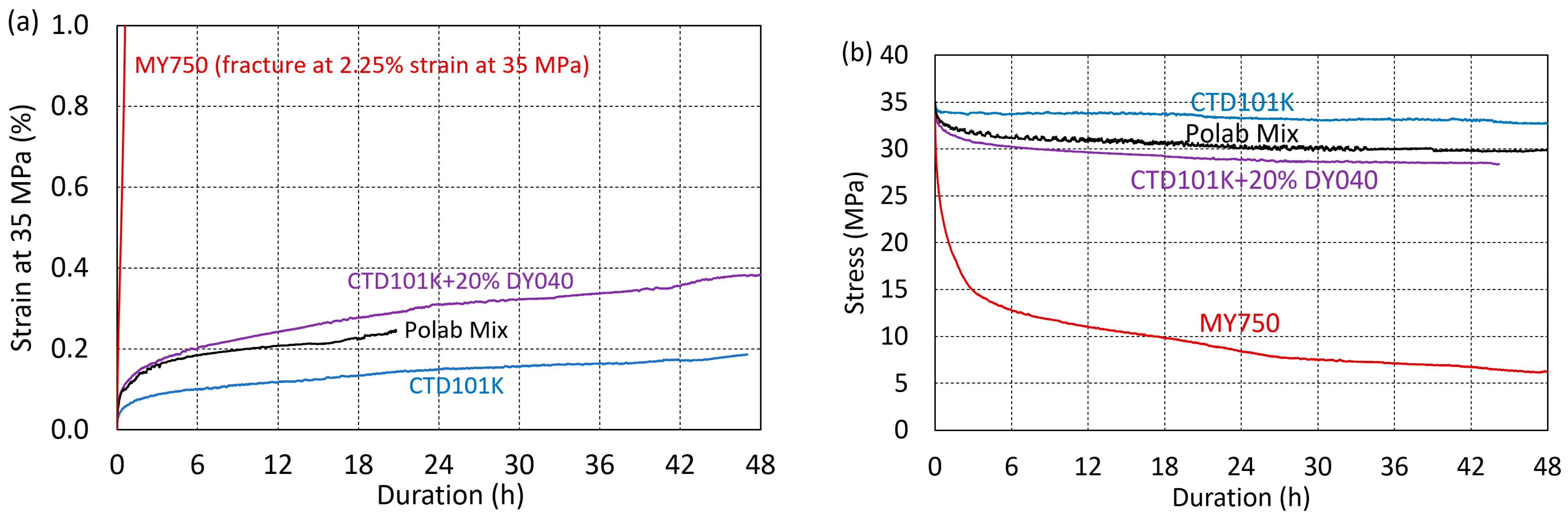
3.4. RT Tensile Stress–Strain Behaviour, Creep, and Stress Relaxation of CTD101K and POLAB Mix
3.5. Viscosity of the Uncured Epoxy-Resin Mix
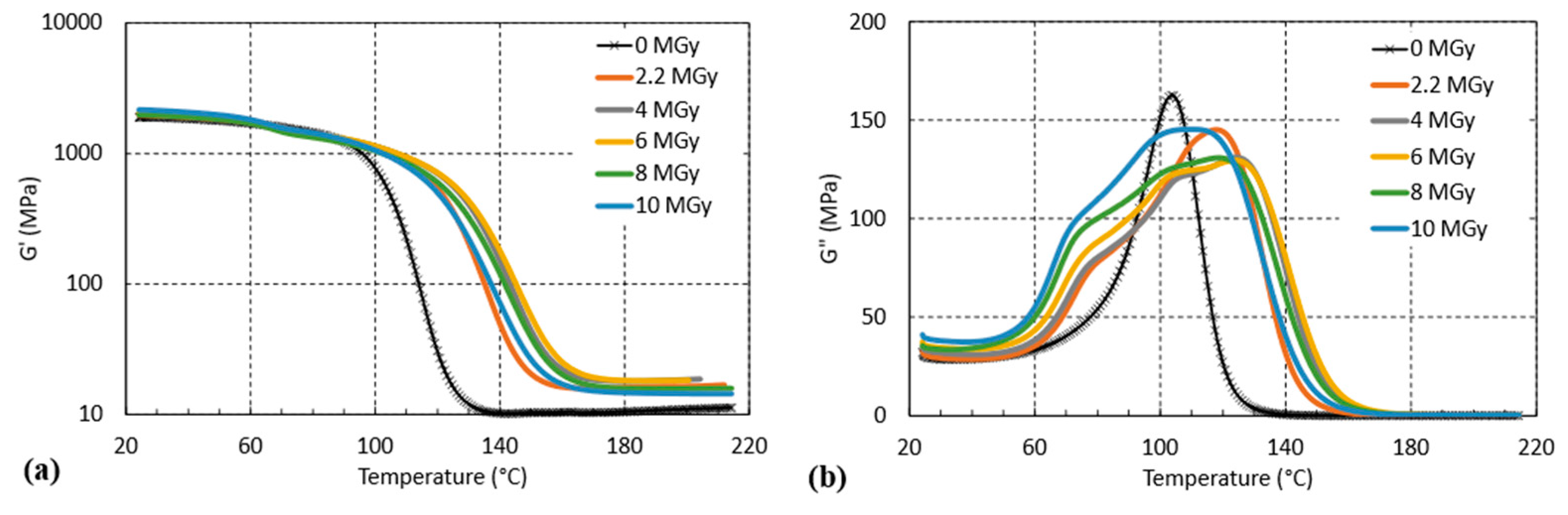
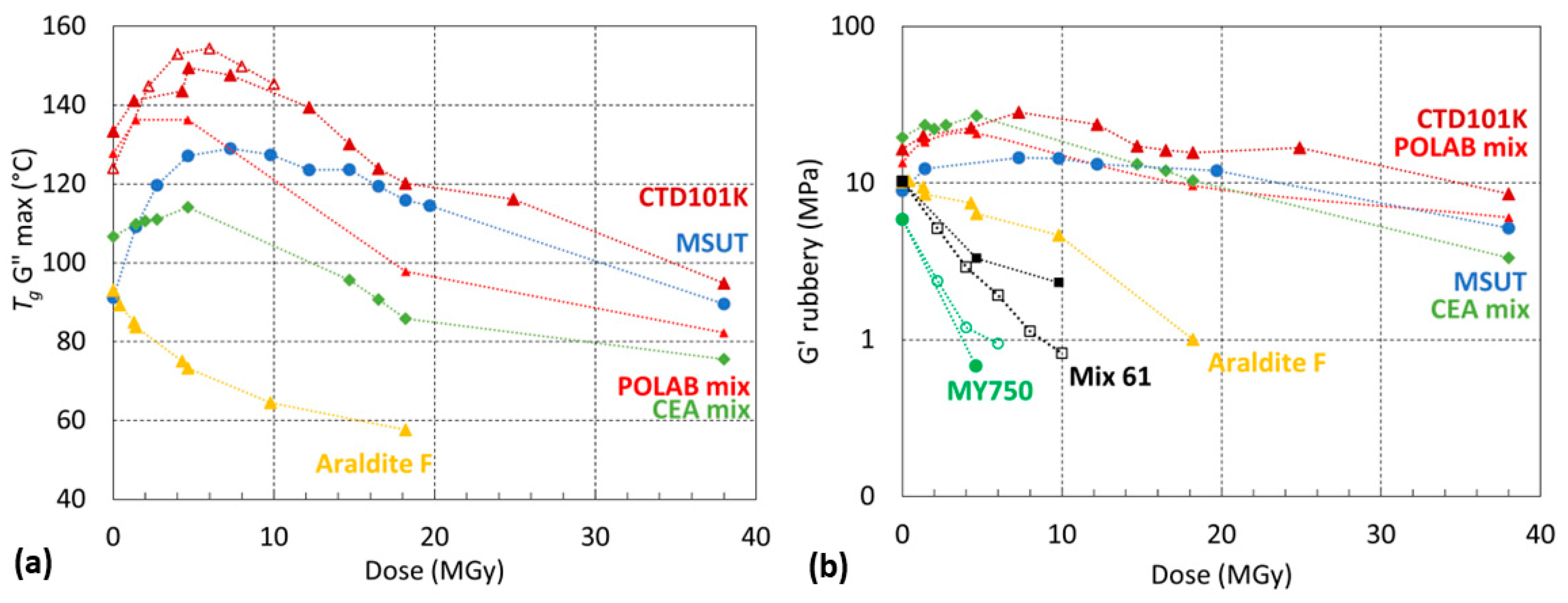
3.6. Radiation-Induced Changes of Viscoelastic Properties
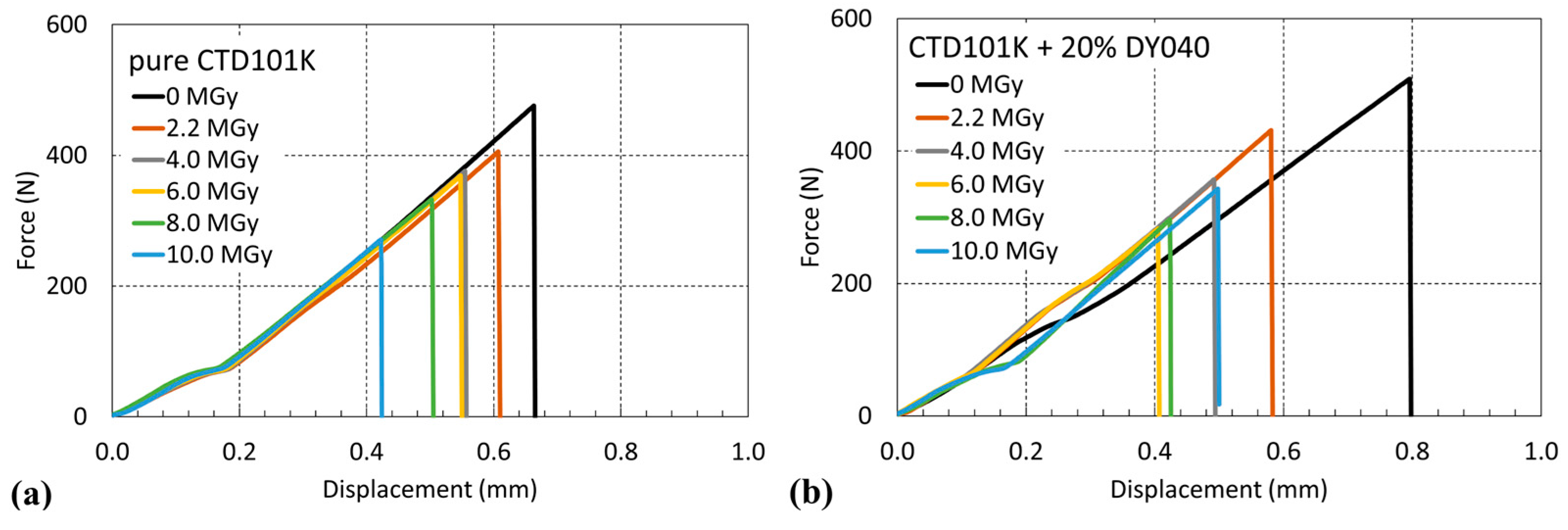
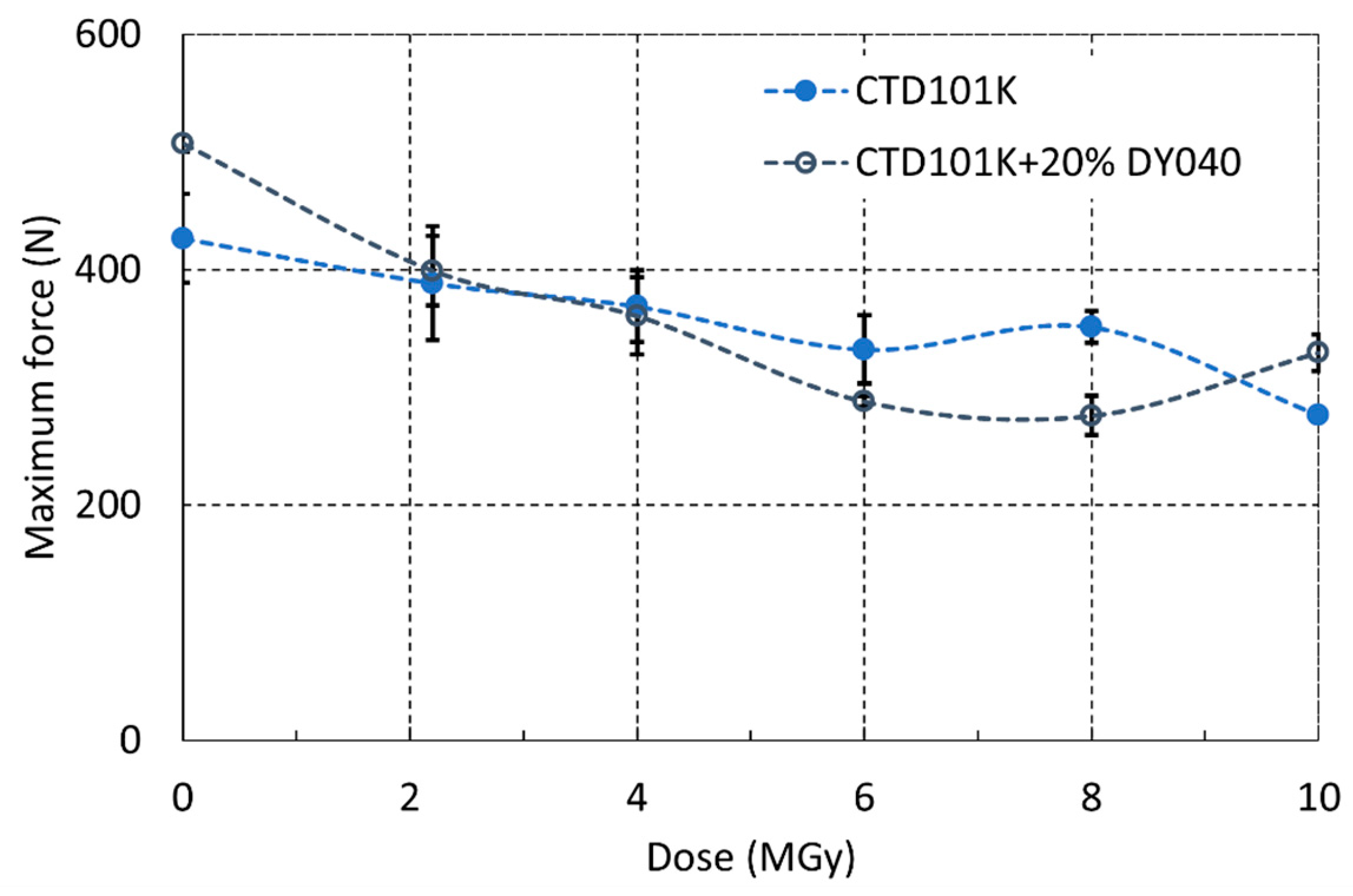
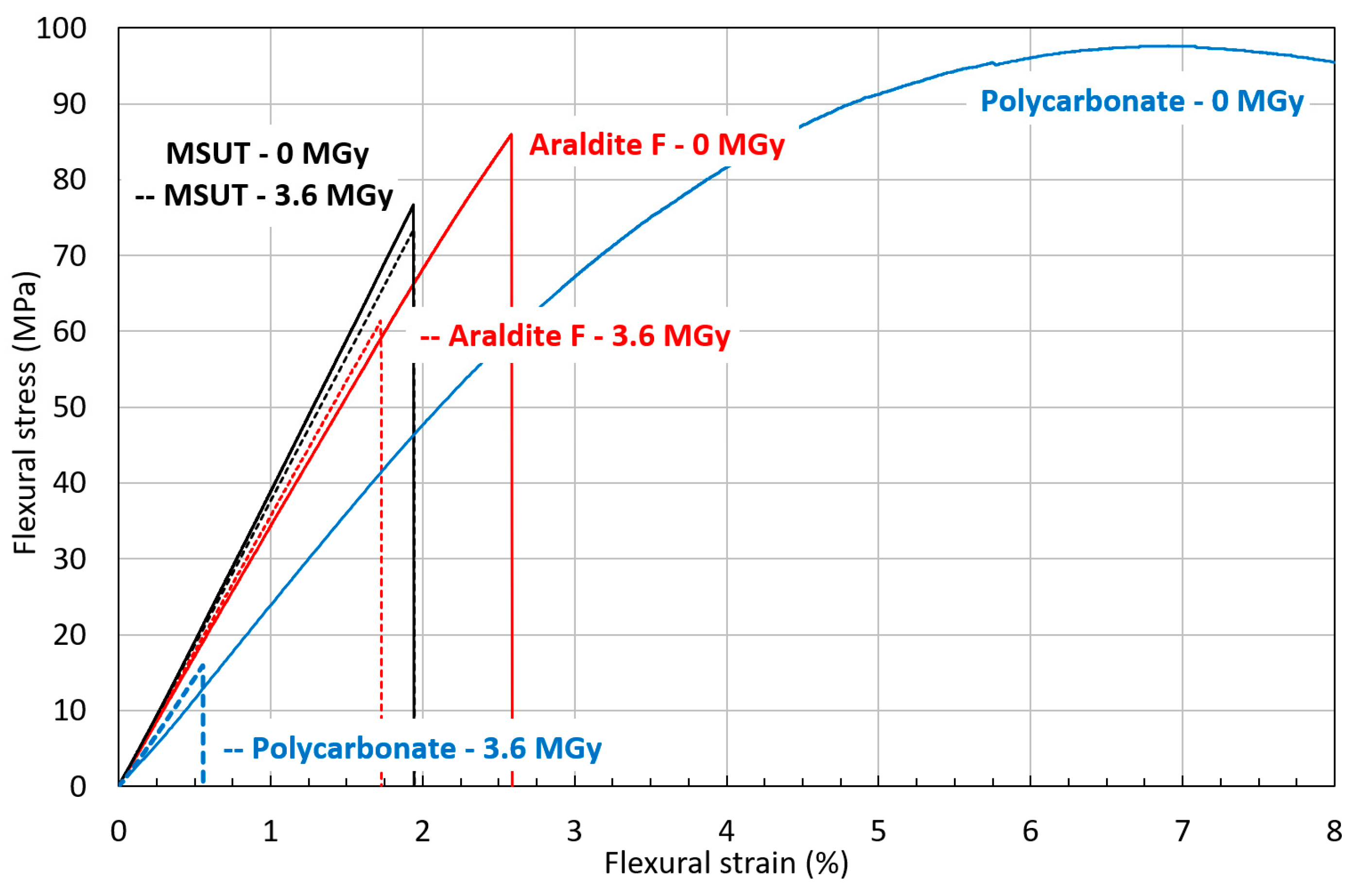
3.7. Radiation-Induced Changes of RT Mechanical Properties
4. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Rossi, L.; Brüning, O. The High Luminosity Large Hadron Collider: The New Machine for Illuminating the Mysteries of Universe. Adv. Ser. High Energy Phys. 2015, 24. [Google Scholar] [CrossRef]
- Bottura, L.; de Rijk, G.; Rossi, L.; Tedesco, E. Advanced Accelerator Magnets for Upgrading the LHC. IEEE Trans. Appl. Supercond. 2015, 25, 4002107. [Google Scholar] [CrossRef]
- Todesco, E.; Bajas, H.; Bajko, M.; Ballarino, A.; Bermudez, S.I.; Bordini, B.; Bottura, L.; De Rijk, G.; Devred, A.; Ramos, D.D.; et al. The High Luminosity LHC Interaction Region Magnets Towards Series Production. Supercond. Sci. Technol. 2021, 34, 053001. [Google Scholar] [CrossRef]
- Benedikt, M.; Blondel, A.; Janot, P.; Mangano, M.; Zimmermann, F. Future circular colliders succeeding the LHC. Nat. Phys. 2020, 16, 402–407. [Google Scholar] [CrossRef]
- Abada, A.; Abbrescia, M.; AbdusSalam, S.S.; Abdyukhanov, I.; Fernandez, J.A.; Abramov, A.; Aburaia, M.; Acar, A.O.; Adzic, P.R.; Agrawal, P.; et al. FCC-hh: The Hadron Collider. Eur. Phys. J. Spec. Top. 2019, 228, 755–1107. [Google Scholar] [CrossRef]
- Accettura, C.; Adams, D.; Agarwal, R.; Ahdida, C.; Aimè, C.; Amapane, N.; Amorim, D.; Andreetto, P.; Anulli, F.; Appleby, R.; et al. Towards a Muon Collider. Eur. Phys. J. C 2023, 83, 864. [Google Scholar] [CrossRef]
- Devred, A.; Bredy, P.; Durante, M.; Gourdin, C.; Rey, J.M.; Reytier, M. Insulation Systems for Nb3Sn Accelerator Magnet Coils Fabricated by the “Wind and React” Technique. In Advances in Cryogenic Engineering Materials; Balachandran, U.B., Hartwig, K.T., Gubser, D.U., Bardos, V.A., Eds.; Springer: Boston, UK, 2000; pp. 143–150. [Google Scholar] [CrossRef]
- Ebermann, P.; Bernardi, J.; Fleiter, J.; Lackner, F.; Meuter, F.; Pieler, M.; Scheuerlein, C.; Schoerling, D.; Wolf, F.; Ballarino, A.; et al. Irreversible degradation of Nb3Sn Rutherford cables due to transversal compression stress at room temperature. Supercond. Sci. Technol. 2018, 31, 065009. [Google Scholar] [CrossRef]
- Dotsenko, V.I.; Kislyak, I.F. Effect of Epoxy Debonding and Cracking on Stability of Superconducting Composites. Cryogenics 1991, 31, 906–912. [Google Scholar] [CrossRef]
- Maeda, H.; Iwasa, Y. Heat Generation from Epoxy Cracks and Bond Failures. Cryogenics 1982, 22, 473–476. [Google Scholar] [CrossRef]
- Stoynev, S.; Riemer, K.; Zlobin, A.V.; Ambrosio, G.; Ferracin, P.; Sabbi, G.L.; Wanderer, P. Analysis of Nb3Sn Accelerator Magnet Training. IEEE Trans. Appl. Supercond. 2019, 29, 7–12. [Google Scholar] [CrossRef]
- Iwasa, Y. Experimental and Theoretical Investigation of Mechanical Disturbances in Epoxy-Impregnated Superconducting Coils. 1. General Introduction. Cryogenics 1985, 25, 304–306. [Google Scholar] [CrossRef]
- Daly, M.; Auchmann, B.; Hug, C.; Sidorov, S.; Otten, S.; Kario, A.; Dhallé, M.; Ten Kate, H. BOX: An Efficient Benchmark Facility for the Study and Mitigation of Interface-Induced training in Accelerator Type High-Field Superconducting Magnets. Supercond. Sci. Technol. 2021, 34, 115008. [Google Scholar] [CrossRef]
- Daly, M.; Auchmann, B.; Brem, A.; Hug, C.; Sidorov, S.; Otten, S.; Dhallé, M.; Guo, Z.; Kario, A.; Ten Kate, H. Improved Training in Paraffin-Wax Impregnated Nb3Sn Rutherford Cables Demonstrated in BOX Samples. Supercond. Sci. Technol. 2022, 35, 055014. [Google Scholar] [CrossRef]
- Mousavi, S.R.; Estaji, S.; Paydayesh, A.; Arjmand, M.; Jafari, S.H.; Nouranian, S.; Khonakdar, H.A. A Review of Recent Progress in Improving the Fracture Toughness of Epoxy-Based Composites Using Carbonaceous Nanofillers. Polym. Compos. 2022, 43, 1871–1886. [Google Scholar] [CrossRef]
- Lee, B.S.; Kim, D.L.; Choi, Y.S.; Yang, H.S.; Yoo, J.S. Effect of Epoxy Cracking on Initial Quench Behavior About High Field Superconducting Magnet. Progr. Supercond. Cryog./J. Korea Inst. Appl. Supercond. Cryog. 2006, 8, 26–29. [Google Scholar] [CrossRef]
- Hübner, F.; Hoffmann, M.; Sommer, N.; Altstaedt, V.; Scherer, A.; Dickhut, T.; Ruckdaeschel, H. Temperature-Dependent Fracture Behavior of Towpreg Epoxy Resins for Cryogenic Liquid Hydrogen Composite Vessels: The Influence of Polysiloxane Tougheners on the Resin Yield Behavior. Polym. Test. 2022, 113, 107678. [Google Scholar] [CrossRef]
- Gul, W.; Xia, Y.E.; Gérard, P.; Ha, S.K. Characterization of Polymeric Composites for Hydrogen Tank. Polymers 2023, 15, 3716. [Google Scholar] [CrossRef]
- Cai, H.; Hu, J.; Wang, Y.; Wang, J. Liquid Oxygen Compatibility and Toughness of Epoxy Resin Modified by a Novel Hyperbranched Polysiloxane. Mater. Res. Express 2019, 6, 085338. [Google Scholar] [CrossRef]
- Evans, D. Resins for superconducting magnet construction—An overview of requirements, processing and properties. IOP Conf. Ser. Mater. Sci. Eng. 2020, 756, 012003. [Google Scholar] [CrossRef]
- Axensalva, J.; Lackner, F.; Gauthier, R. Vacuum Pressure Impregnation Setup at CERN for Nb3Sn Coils. IEEE Trans. Appl. Supercond. 2020, 30, 4003204. [Google Scholar] [CrossRef]
- Tavlet, M.; Fontaine, A.; Schönbacher, H. Compilation of Radiation Damage Test Data, 2nd ed.; Ser. CERN Yellow Reports: Monographs; CERN: Geneva, Switzerland, 1998; Available online: http://cds.cern.ch/record/357576 (accessed on 31 January 2024).
- Parragh, D.M.; Scheuerlein, C.; Piccin, R.; Ravotti, F.; Pezzullo, G.; Ternova, D.; Taborelli, M.; Lehner, M.; Eisterer, M. Irradiation Induced Aging of Epoxy Resins for Impregnation of Superconducting Magnet Coils. IEEE Trans. Appl. Supercond. 2024, 34, 7800107. [Google Scholar] [CrossRef]
- Hill, A.J.; Heater, K.J.; Agrawal, C.M. The Effects of Physical Aging in Polycarbonate. J. Polym. Sci. Part B Polym. Phys. 1990, 28, 387–405. [Google Scholar] [CrossRef]
- Fabian, P.E.; Munshi, N.A.; Denis, R.J. Highly Radiation-Resistant Vacuum Impregnation Resin Systems for Fusion Magnet Insulation. AIP Conf. Proc. 2002, 614, 295–304. [Google Scholar] [CrossRef]
- Brem, A.; Gold, B.J.; Auchmann, B.; Tommasini, D.; Tervoort, T.A. Elasticity, Plasticity and Fracture Toughness at Ambient and Cryogenic Temperatures of Epoxy Systems Used for the Impregnation of High-Field Superconducting Magnets. Cryogenics 2021, 115, 103260. [Google Scholar] [CrossRef]
- Araldite DY 040 Flexibilizer. Product Information of Samaro Company. Available online: https://www.samaro.fr/en/product/araldite-dy-040-flexibilizer (accessed on 3 June 2023).
- Araldite CY 192-1 100 pbw/Aradur HY 918-1 100 pbw Impregnation Resin System; Technical data sheet of Huntsman company; Huntsman Company: The Woodlands, TA, USA, 2014.
- Rifflet, J.M.; Durante, M.; Segreti, M. Nb3Sn Quadrupole Development at CEA/Saclay. In Proceedings of the WAMSDO 2008, Geneva, Switzerland, 19–23 May 2008; CERN: Zurich, Switzerland, 2008; p. 131. [Google Scholar]
- den Ouden, A.; Wessel, S.; Krooshoop, E.; ten Kate, H. Application of Nb3Sn Superconductors in High Field Accelerator Magnets. IEEE Trans. Appl. Supercond. 1997, 7, 733–738. [Google Scholar] [CrossRef]
- Araldite Casting Resin System. Technical Data Sheet of Huntsman Company. Available online: https://www.gluespec.com/Materials/SpecSheet/484420d9-3d7b-479d-a0cd-4c3204a86877 (accessed on 3 June 2023).
- Araldite, F. HY 905, DY 040, DY 061. Technical Data Sheet of Vantico Company. Available online: https://www.chemcenters.com/images/suppliers/169257/Araldite%20F,%20HY%20905,%20DY%20040,%20DY%20061.pdf (accessed on 3 June 2023).
- Brennan, A.B.; Miller, T.M.; Arnold, J.J.; Huang, K.V.; Gephart, N.L.; Markewicz, W.D. Thermomechanical Properties of a Toughened Epoxy for Impregnating Superconducting Magnets. Cryogenics 1995, 35, 783–785. [Google Scholar] [CrossRef]
- SikaBiresin® RE 700-04 POLYOL/SikaBiresin® RE 106 ISOCYANATE*; Sika Product Data Sheet; Sika: Singapore, 2023.
- ISO 1183-1; Plastics—Methods for Determining the Density of Non-Cellular Plastics—Part 1: Immersion Method, Liquid Pycnometer Method and Titration Method. ISO: Geneva, Switzerland, 2019.
- ISO 2039-1; Plastics—Determination of Hardness—Part 1: Ball Indentation Method. ISO: Geneva, Switzerland, 2001.
- ISO 527; Plastics—Determination of Tensile Properties. ISO: Geneva, Switzerland, 2019.
- ISO 48-4; Rubber, Vulcanized or Thermoplastic—Determination of Hardness—Part 4: Indentation Hardness by Durometer Method (Shore Hardness). ISO: Geneva, Switzerland, 2018.
- ISO 13586; Plastics—Determination of Fracture Toughness (GIC and KIC)—Linear Elastic Fracture Mechanics (LEFM) Approach. ISO: Geneva, Switzerland, 2018.
- Grellmann, W.; Lach, R.; Seidler, S. Experimental determination of geometry-independent fracture mechanics values J, CTOD and K for polymers. Int. J. Fract. Lett. Fract. Micromech. 2002, 118, L9–L14. [Google Scholar] [CrossRef]
- ASTM D4065; Standard Practice for Plastics: Dynamic Mechanical Properties: Determination and Report of Procedures. ASTM: West Conshohocken, PA, USA, 2020.
- ASTM D7028; Standard Test Method for Glass Transition Temperature (DMA Tg) of Polymer Matrix Composites by Dynamic Mechanical Analysis (DMA). ASTM: West Conshohocken, PA, USA, 2008.
- Flory, P.J. Molecular Theory of Rubber Elasticity. Polymer 1979, 20, 1317–1320. [Google Scholar] [CrossRef]
- Chen, T.T. Quantifying Polymer Crosslinking Density Using Rheology and DMA. TA Instruments. Available online: https://www.tainstruments.com/pdf/literature/RH102.pdf (accessed on 31 January 2024).
- Hill, L.W. Calculation of crosslink density in short chain networks. Prog. Org. Coat. 1997, 31, 235–243. [Google Scholar] [CrossRef]
- Ravotti, F. Dosimetry techniques and radiation test facilities for total ionizing dose testing. IEEE Trans. Nucl. Sci. 2018, 65, 1440–1464. [Google Scholar] [CrossRef]
- Parragh, D.M.; Scheuerlein, C.; Martin, N.; Piccin, R.; Ravotti, F.; Pezzullo, G.; Koettig, T.; Lellinger, D. Effect of Irradiation Environment and Temperature on Aging of Epoxy Resins for Superconducting Magnets. Polymers 2024, 16, 407. [Google Scholar] [CrossRef] [PubMed]
- ISO 14130; Fibre-Reinforced Plastic Composites—Determination of Apparent Interlaminar Shear Strength by Short-Beam Method. Available online: https://www.iso.org/standard/23642.html (accessed on 3 June 2023).
- Evans, D.; Crook, M.A. Irradiation of Plastics: Damage and Gas Evolution. MRS Bull. 1997, 22, 36–40. [Google Scholar] [CrossRef]
- Olejnik, T.A. Red 4034 Perspex dosimeters in industrial radiation sterilization process control. Radiat. Phys. Chem. 1979, 14, 431–447. [Google Scholar] [CrossRef]
- ISO/ASTM 51276; Standard Practice for Use of a Polymethylmethacrylate Dosimetry System. ISO: Geneva, Switzerland; ASTM: West Conshohocken, PA, USA, 2019.
- Grellmann, W.; Che, M. Assessment of Temperature-dependent fracture behaviour with different fracture mechanics concepts on example of unoriented and cold-rolled polypropylene. J. Appl. Polym. Sci. 1997, 66, 1237–1249. [Google Scholar] [CrossRef]
- Zotti, A.; Zuppolini, S.; Zarrelli, M.; Borriello, A. Fracture Toughening Mechanisms in Epoxy Adhesives. In Adhesives—Applications and Properties; Rudawska, A., Ed.; IntechOpen: London, UK, 2016. [Google Scholar] [CrossRef]
- Wu, T.; Guo, F.L.; Hu, J.M.; Li, Y.Q.; Fu, Y.T.; Fu, S.Y. Investigation of Polyurethane Toughened Epoxy Resins for Composite Cryotank Applications. Part I: Phase Separation Phenomenon and Cryogenic Mechanical Behaviors. Compos. Commun. 2022, 35, 101278. [Google Scholar] [CrossRef]
- Zhao, X.; Li, Y.; Chen, W.; Li, S.; Zhao, Y.; Du, S. Improved Fracture Toughness of Epoxy Resin Reinforced with Polyamide 6/Graphene Oxide Nanocomposites Prepared via in situ Polymerization. Compos. Sci. Technol. 2019, 171, 180–189. [Google Scholar] [CrossRef]
- Domun, N.; Hadavinia, H.; Zhang, T.; Sainsbury, T.; Liaghat, G.H.; Vahid, S. Improving the Fracture Toughness and the Strength of Epoxy Using Nanomaterials—A Review of the Current Status. Nanoscale 2015, 7, 10294–10329. [Google Scholar] [CrossRef] [PubMed]
- Haziot, A.; Dallocchio, A.; Devred, A.; Foussat, A.; Gentini, L.; Kirby, G.; Mangiarotti, F.J.; Pentella, M.; Petrone, C.; Pincot, F.O.; et al. Curved-Canted-Cosine-Theta (CCCT) Dipole Prototype Development at CERN. IEEE Trans. Appl. Supercond. 2024, 34, 4002608. [Google Scholar] [CrossRef]
- Evans, D.; Zhang, Z. The work of fracture of epoxide resins at temperatures to 4 K. Adv. Cry. Eng. 2000, 46, 235–242. [Google Scholar] [CrossRef]







| Material | ρ (g/cm3) | HB (MPa) | E (GPa) | σy (MPa) | σM (MPa) | εB (%) | Tg (°C) | α (×10−6 K−1) | ||
|---|---|---|---|---|---|---|---|---|---|---|
| G′onset | G″max | tan δmax | ||||||||
| CTD101K | 1.22 | 217 ± 5 | 3.53 ± 0.08 | - | 46 ± 3.2 | 1.3 ± 0.1 | 118 | 122 | 140 | 60 |
| POLAB Mix | 1.21 | 199 ± 8 | 3.07 ± 0.03 | - | 45 ± 3.9 | 1.6 ± 0.2 | 124 | 128 | 140 | 68 |
| CEA mix | 1.27 | 213 ± 14 | 3.86 ± 0.13 | - | 53 ± 5.5 | 1.5 ± 0.2 | 100 | 107 | 113 | 56 |
| MSUT | 1.23 | 245 ± 12 | 3.59 ± 0.07 | - | 45 ± 5.6 | 1.3 ± 0.2 | 88 | 91 | 107 | 60 |
| Araldite F | 1.22 | 199 ± 5 | 3.30 ± 0.06 | 54 | 59 ± 5.7 | 2.0 ± 0.3 | 91 | 93 | 105 | 55 |
| MY750 | 1.15 | 126 ± 4 | 3.05 ± 0.07 | 46 | 60 ± 0.4 | 2.8 ± 0.1 | 49 | 51 | 55 | 62 |
| Mix61 | 1.14 | 47.5 ± 2 | 0.67 ± 0.07 | 13 | 20 ± 0.4 | 19 ± 0.4 | −38 * | n.m. | 73 | 144 |
| Polyurethane | 1.13 | ** | ~0.08 | n.m. | ~2.4 | ~30 | −16 * | −11 * | 1.0 * | n.m. |
| PMMA | 1.2 | 202 ± 5 | 3.25 ± 0.14 | 51 | 61 ± 2.0 | 2.3 ± 0.1 | 115 | 118 | 133 | n.m. |
| PC | 1.20 | 127 ± 3 | 2.35 | 34 | 60 | ~5 | 150 | 152 | 158 | 63 |
| Material | σy, σM (MPa) | KQ (MPa√m) | GQ (kJ/m2) | h (mm) | a (mm) | w–a (mm) | |
|---|---|---|---|---|---|---|---|
| RT | |||||||
| CTD101K | 46 | 0.82 ± 0.12 | 0.45 ± 0.10 | 4.03 | 5.50 | 4.54 | 0.8 |
| POLAB Mix | 45 | 1.17 ± 0.30 | 0.65 ± 0.32 | 3.95 | 5.26 | 4.83 | 1.7 |
| CTD101K+20% DY040 | 40 | 1.28 ± 0.47 | 0.79 ± 0.58 | 4.22 | 5.28 | 4.79 | 2.6 |
| CEA mix | 53 | 1.62 ± 0.35 | 1.05 ± 0.40 | 3.83 | 4.31 | 5.78 | 2.3 |
| MSUT | 46 | 1.24 ± 0.25 | 0.63 ± 0.26 | 3.93 | 5.25 | 4.84 | 1.8 |
| Araldite F | 54 | 1.57 ± 0.32 | 1.14 ± 0.50 | 3.89 | 5.13 | 4.96 | 2.1 |
| MY750 | 46 | 1.83 ± 0.13 | 1.91 ± 0.30 | 4.00 | 5.48 | 4.56 | 4.0 |
| PMMA | 51 | 2.12 ± 0.62 | 1.50 ± 0.56 | 3.69 | 5.69 | 4.35 | 4.3 |
| Mix 61 | 13 | 1.17 ± 0.05 | 1.52 ± 0.13 | 3.90 | 4.60 | 5.36 | 20 # |
| PC | 54 | 3.51 ± 0.41 | 9.12 ± 2.49 | 3.97 | 5.56 | 4.64 | 27 # |
| 77 K | |||||||
| CTD101K | 100 * | 1.52 ± 0.68 | 0.72 ± 0.46 | 4.00 | 5.34 | 4.70 | 0.6 |
| POLAB Mix | 100 * | 2.33 ± 0.20 | 1.33 ± 0.23 | 3.96 | 5.82 | 4.28 | 1.4 |
| CTD101K+20% DY040 | 100 * | 2.43 ± 0.27 | 1.21 ± 0.28 | 4.39 | 5.31 | 4.74 | 1.5 |
| CEA mix | 100 * | 2.29 ± 0.69 | 1.40 ± 0.64 | 4.02 | 5.16 | 5.02 | 1.3 |
| MSUT | 100 * | 2.44 ± 0.48 | 1.34 ± 0.43 | 3.97 | 5.33 | 4.75 | 1.5 |
| Araldite F | 100 * | 2.44 ± 0.72 | 1.89 ± 0.50 | 3.87 | 5.43 | 4.65 | 1.5 |
| MY750 | 100 * | 4.62 ± 0.56 | 4.28 ± 1.08 | 3.94 | 5.29 | 4.75 | 5.3 |
| PMMA | 100 * | 4.68 ± 0.19 | 3.90 ± 0.45 | 3.69 | 5.49 | 4.59 | 5.5 |
| PC | 100 * | 6.81 ± 0.26 | 12.2 ± 0.79 | 3.87 | 5.61 | 4.57 | 12 # |
| Polyurethane | 100 * | 7.01 ± 1.29 | 8.72 ± 1.95 | 3.96 | 5.83 | 4.24 | 12 # |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gaarud, A.; Scheuerlein, C.; Parragh, D.M.; Clement, S.; Bertsch, J.; Urscheler, C.; Piccin, R.; Ravotti, F.; Pezzullo, G.; Lach, R. Fracture Toughness, Radiation Hardness, and Processibility of Polymers for Superconducting Magnets. Polymers 2024, 16, 1287. https://doi.org/10.3390/polym16091287
Gaarud A, Scheuerlein C, Parragh DM, Clement S, Bertsch J, Urscheler C, Piccin R, Ravotti F, Pezzullo G, Lach R. Fracture Toughness, Radiation Hardness, and Processibility of Polymers for Superconducting Magnets. Polymers. 2024; 16(9):1287. https://doi.org/10.3390/polym16091287
Chicago/Turabian StyleGaarud, Anders, Christian Scheuerlein, David Mate Parragh, Sébastien Clement, Jacob Bertsch, Cedric Urscheler, Roland Piccin, Federico Ravotti, Giuseppe Pezzullo, and Ralf Lach. 2024. "Fracture Toughness, Radiation Hardness, and Processibility of Polymers for Superconducting Magnets" Polymers 16, no. 9: 1287. https://doi.org/10.3390/polym16091287
APA StyleGaarud, A., Scheuerlein, C., Parragh, D. M., Clement, S., Bertsch, J., Urscheler, C., Piccin, R., Ravotti, F., Pezzullo, G., & Lach, R. (2024). Fracture Toughness, Radiation Hardness, and Processibility of Polymers for Superconducting Magnets. Polymers, 16(9), 1287. https://doi.org/10.3390/polym16091287







