Investigation of Additive-Manufactured Carbon Fiber-Reinforced Polyethylene Terephthalate Honeycomb for Application as Non-Pneumatic Tire Support Structure
Abstract
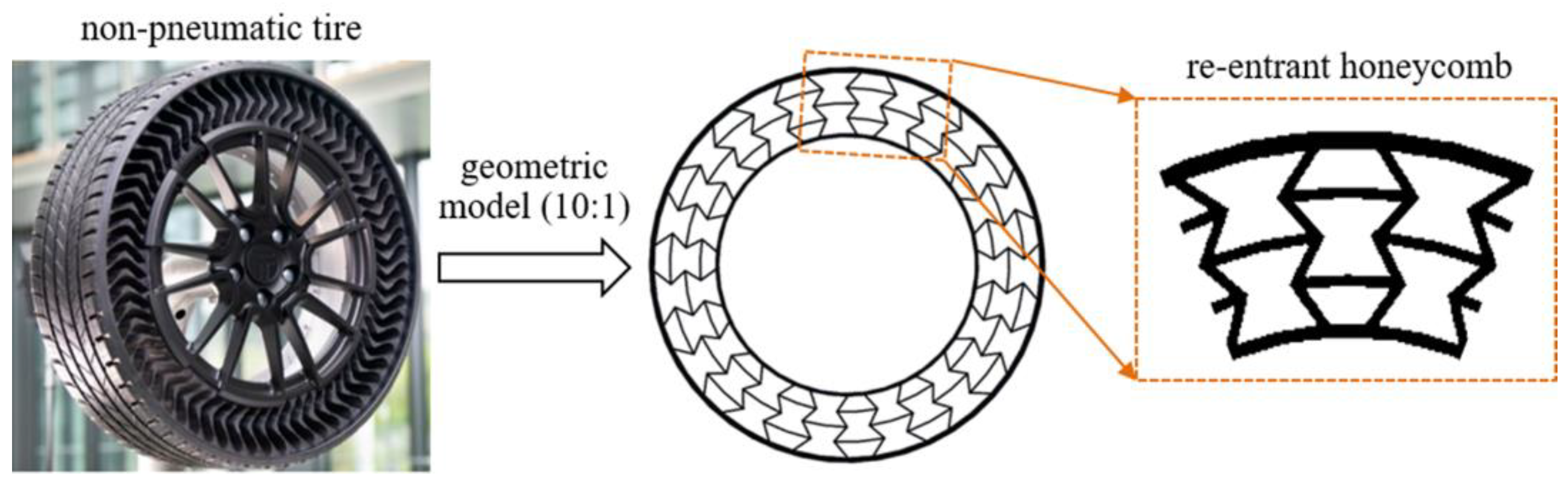
1. Introduction
2. Materials and Experimental Methods
2.1. Materials
2.2. Three-Dimentional Printing of PET/CF Samples
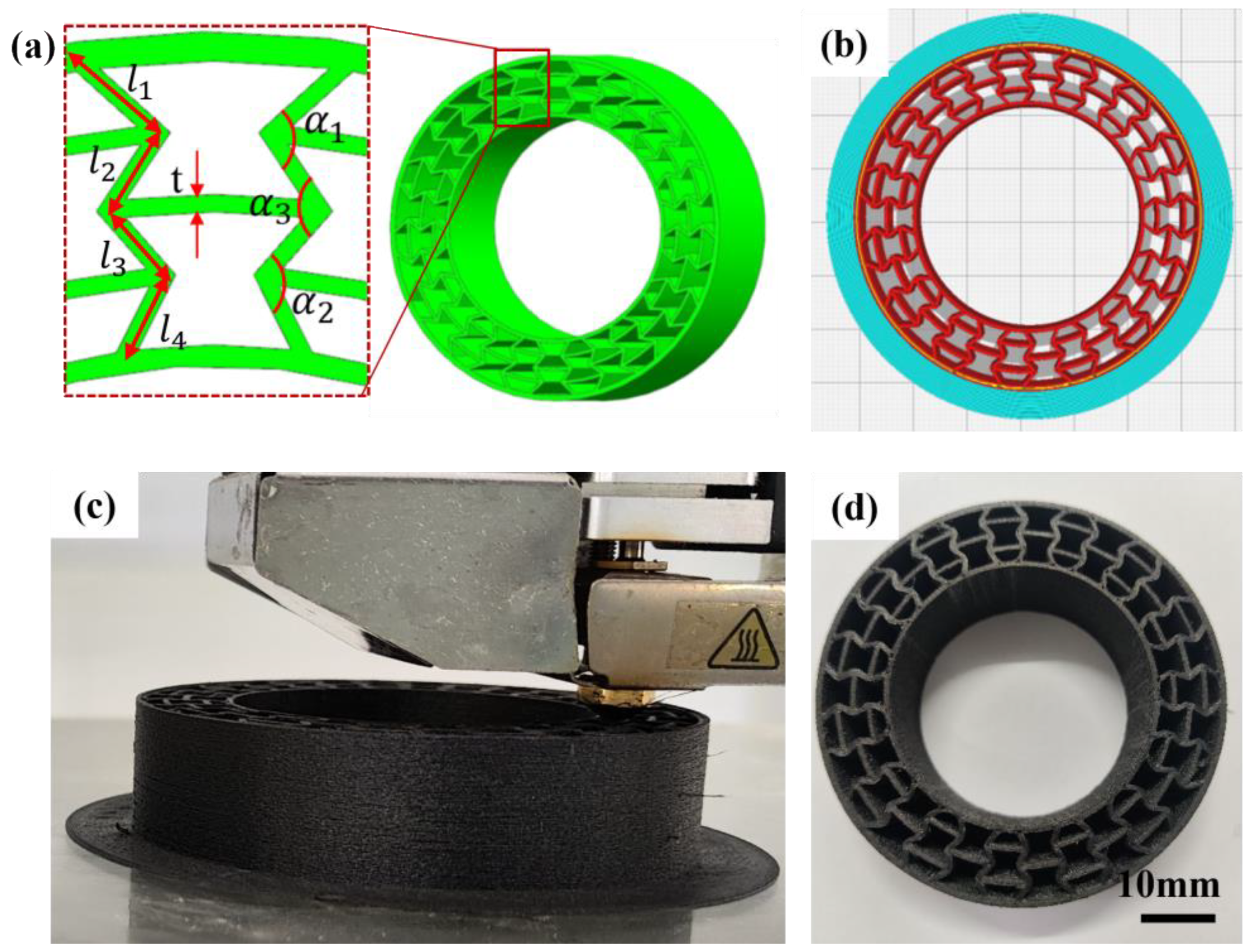
2.3. Design of the Testing Specimens and Honeycomb Structures
2.4. Characterization
3. Experimental Results and Discussion
3.1. Thermal, Rheological and Mechanical Properties of PET/CF Filaments
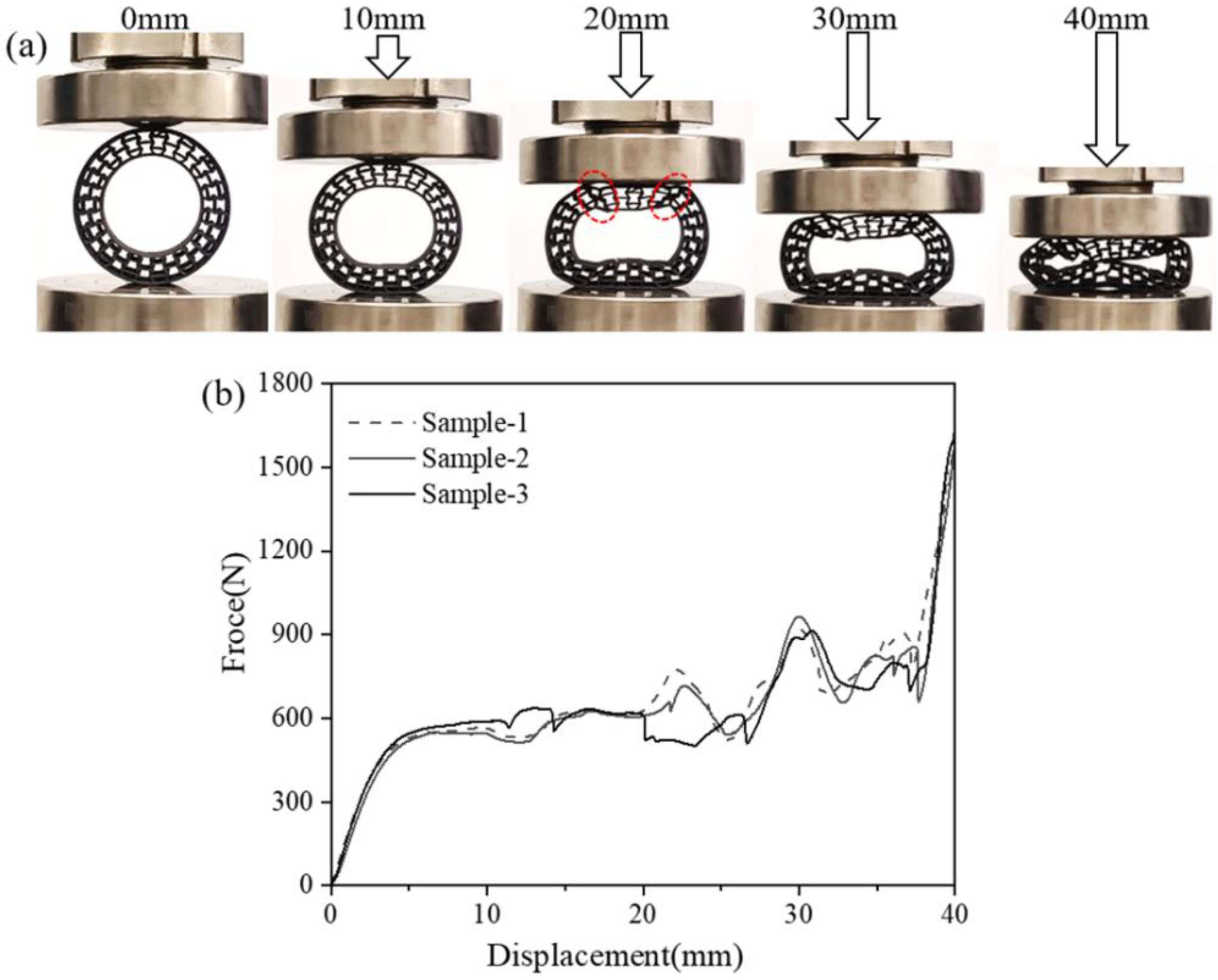
3.2. Three-Dimensional Printing of Deformable Re-Entrant Body and Compressive Performance
4. Numerical Modelling
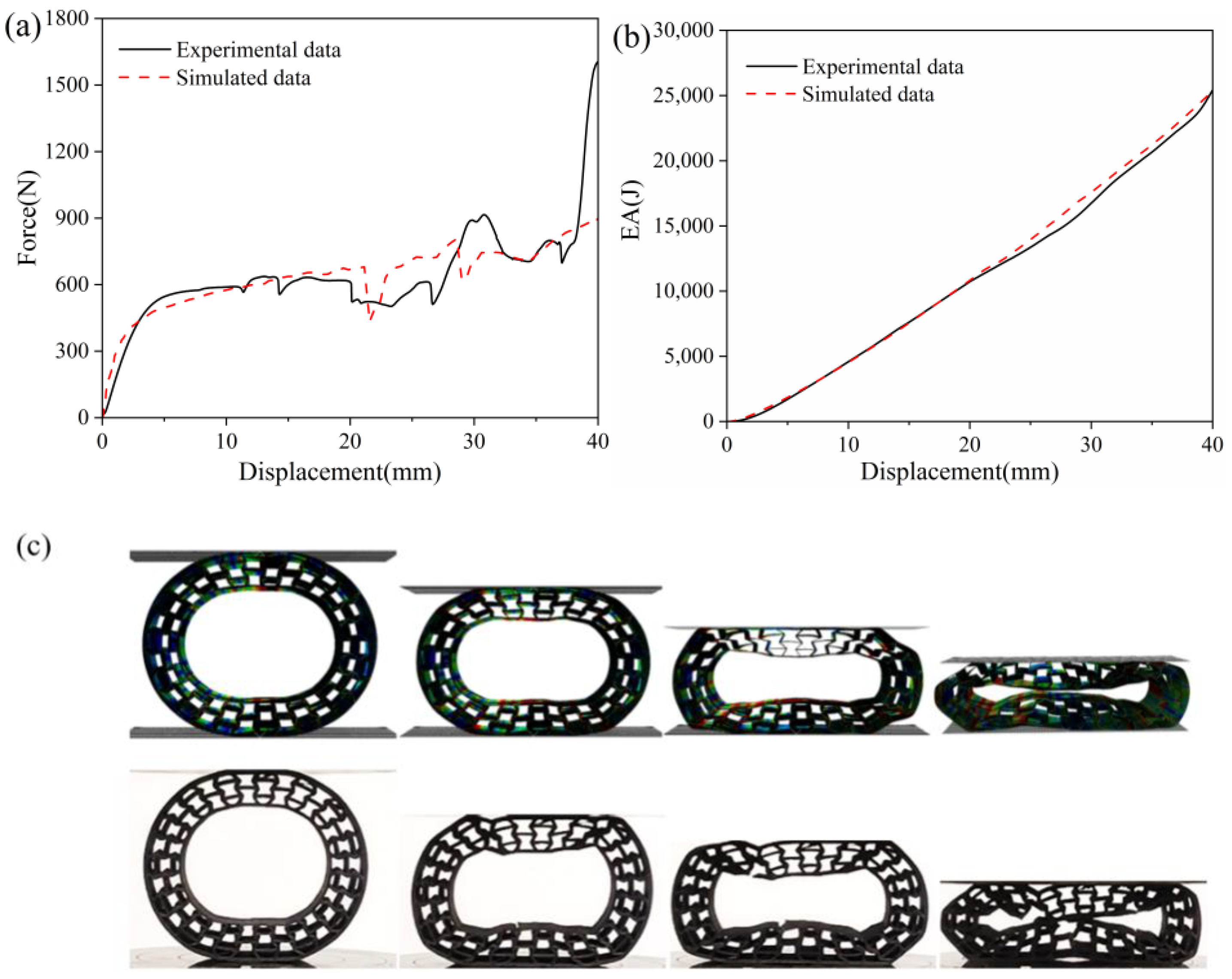
4.1. Validation of Simulation and Experimental Data of 3D Printing of Deformable PET/CF Re-Entrant Honeycomb
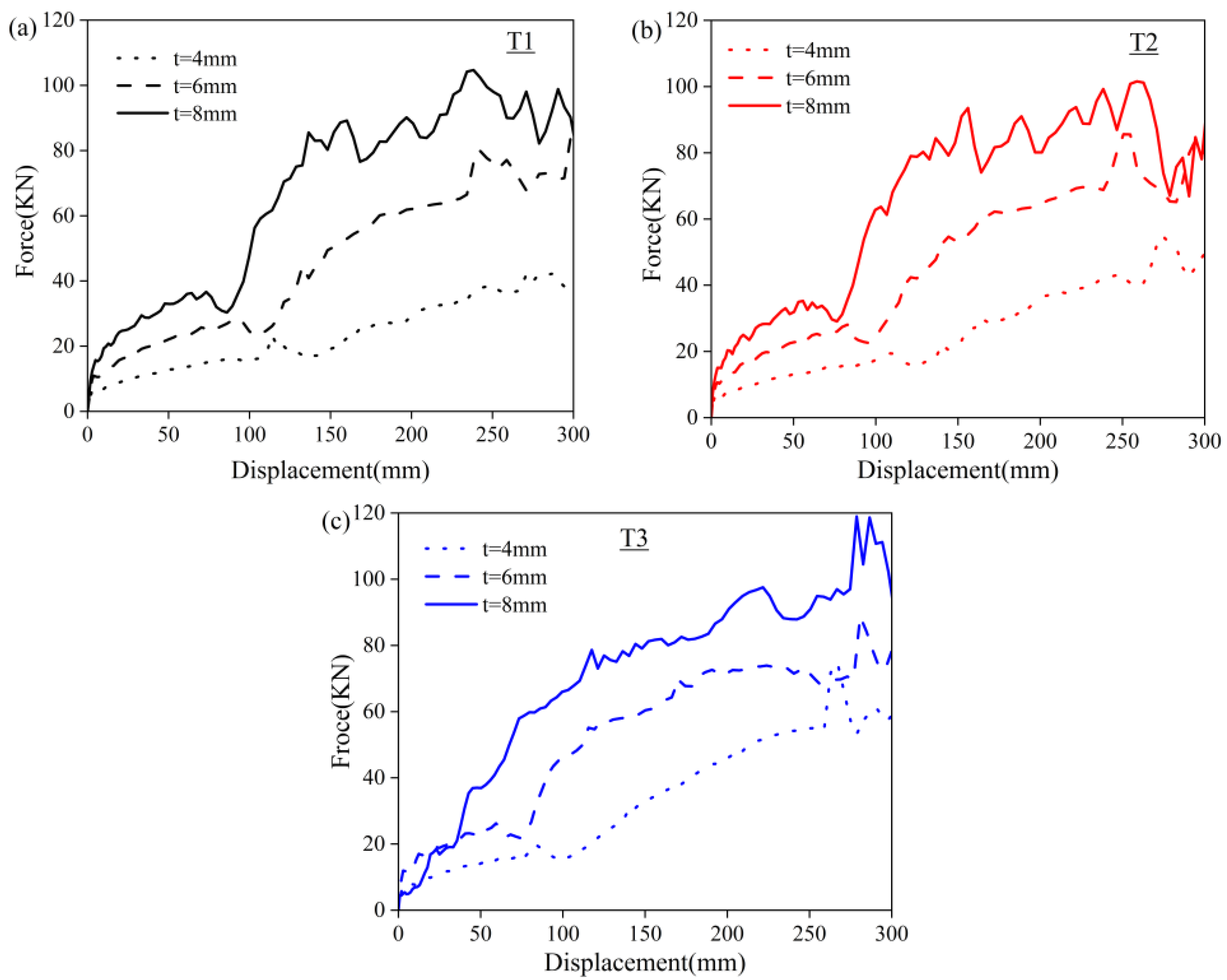
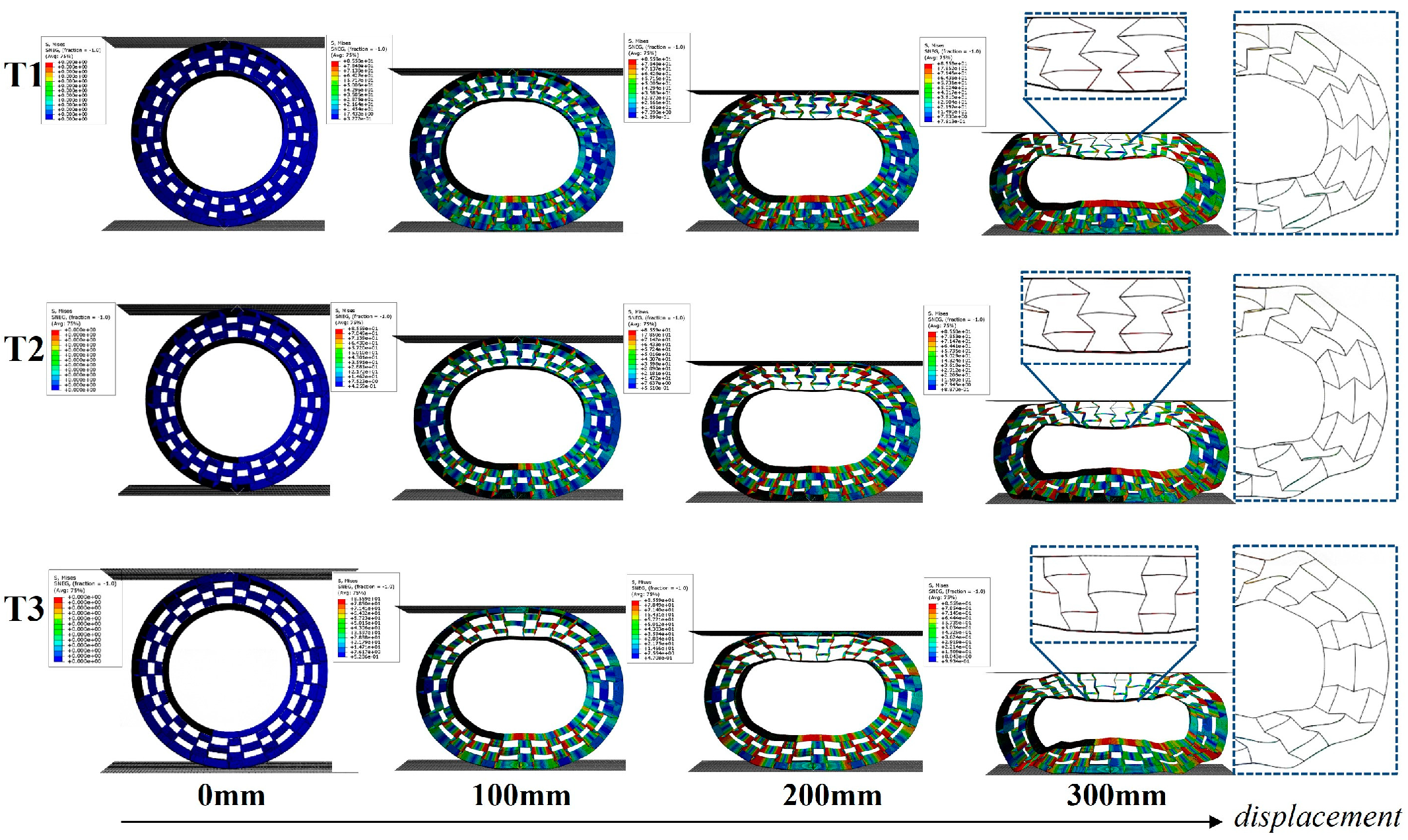
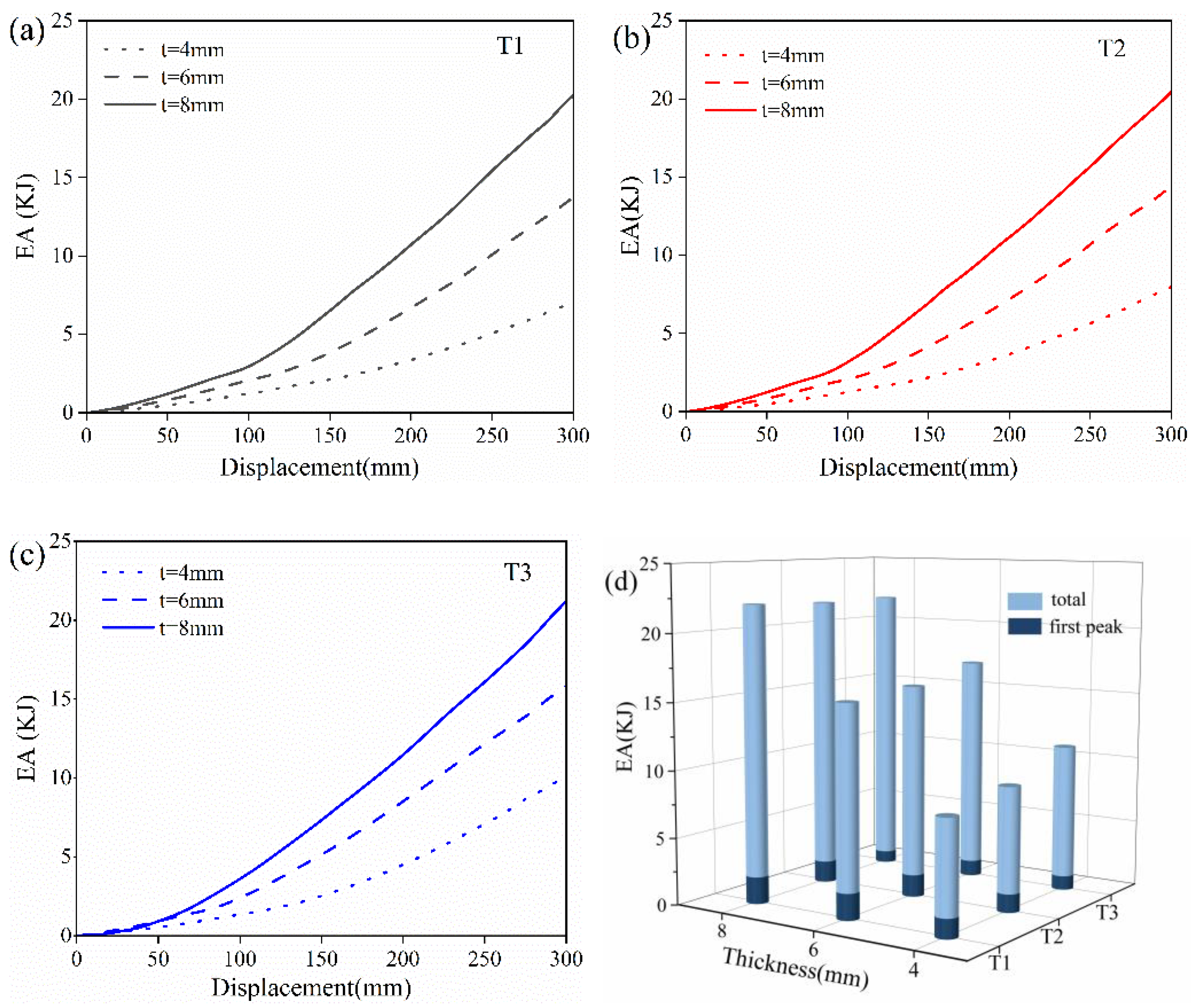
4.2. Influence of Geometric Parameters on the Compressive Behavior of 3D-Printed PET Re-Entrant Samples
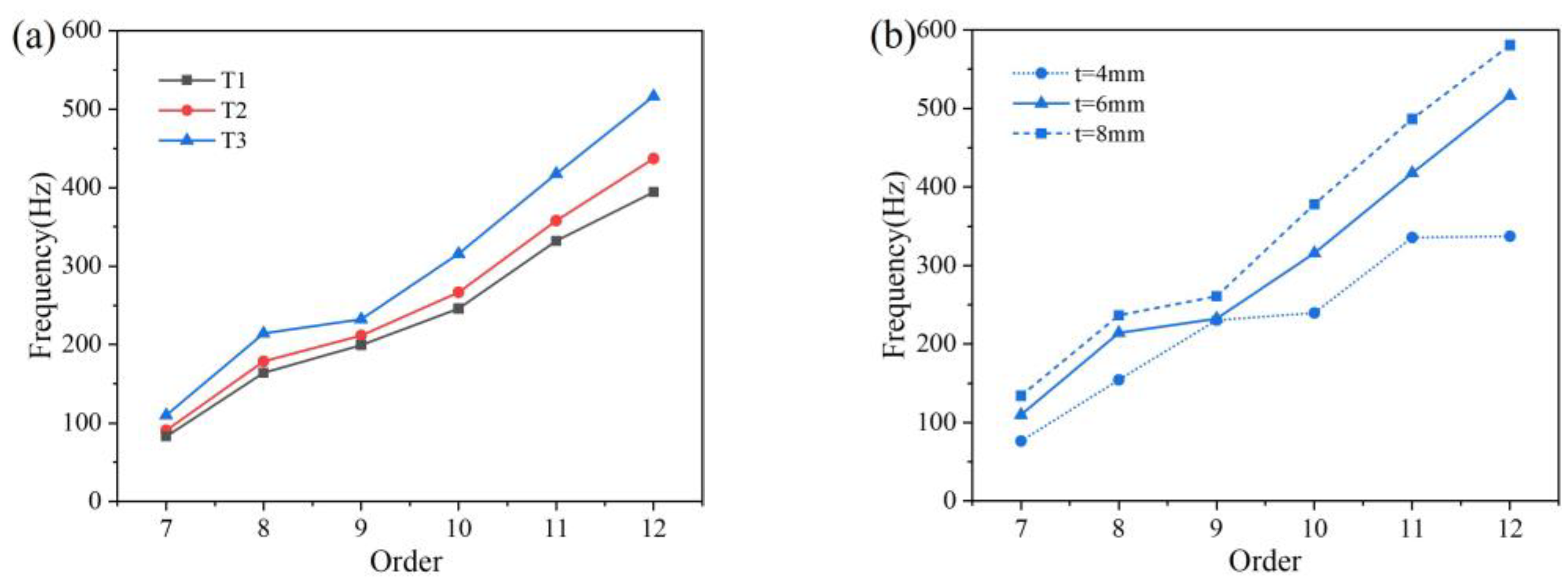
4.3. Free Vibration Condition for NPT Deformable PET/CF Re-Entrant Structures
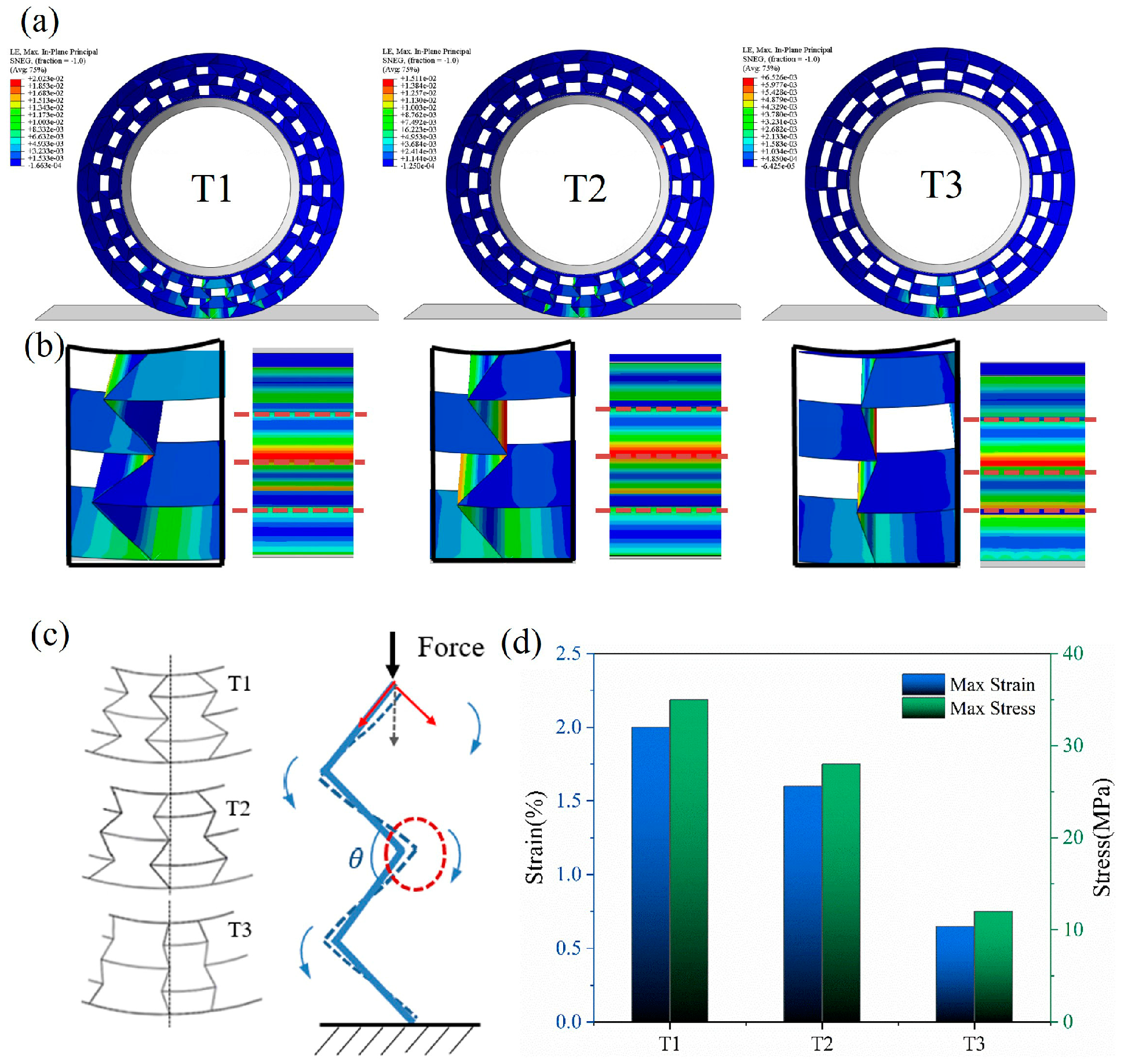
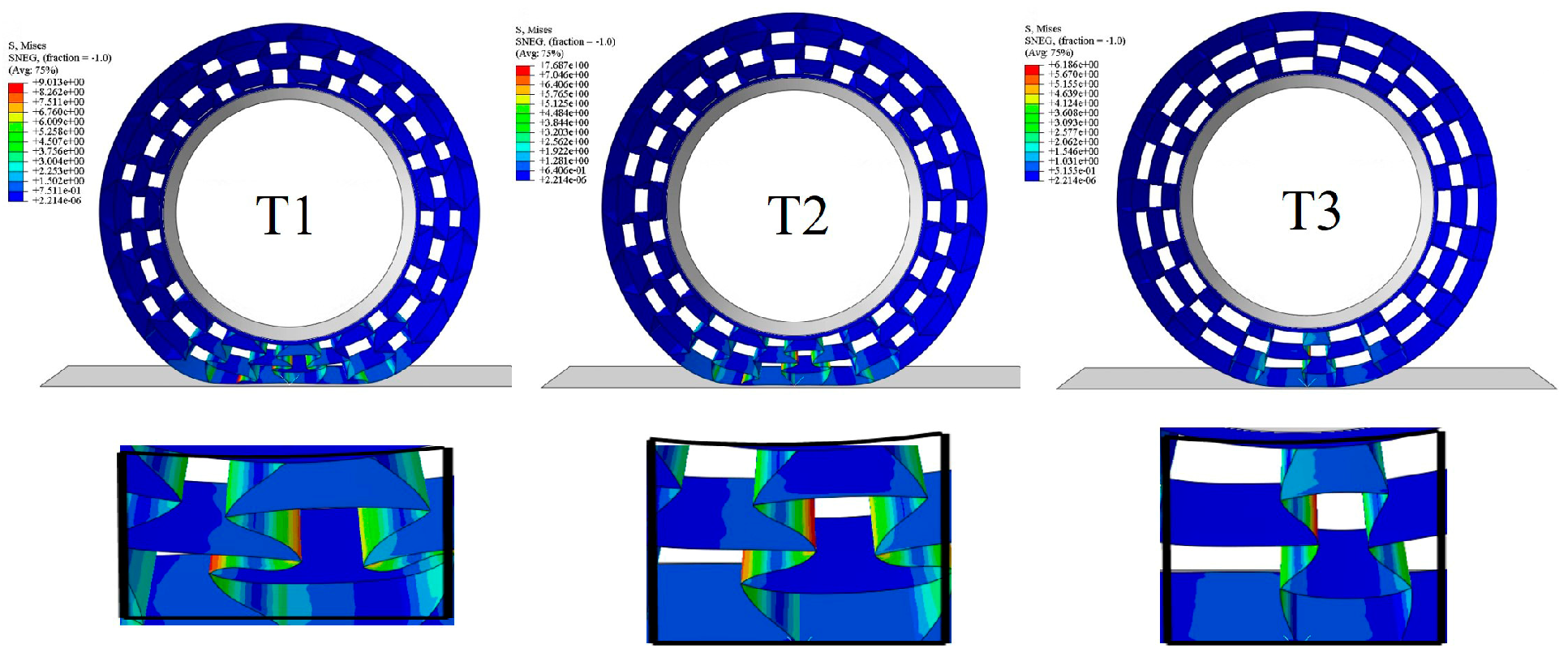
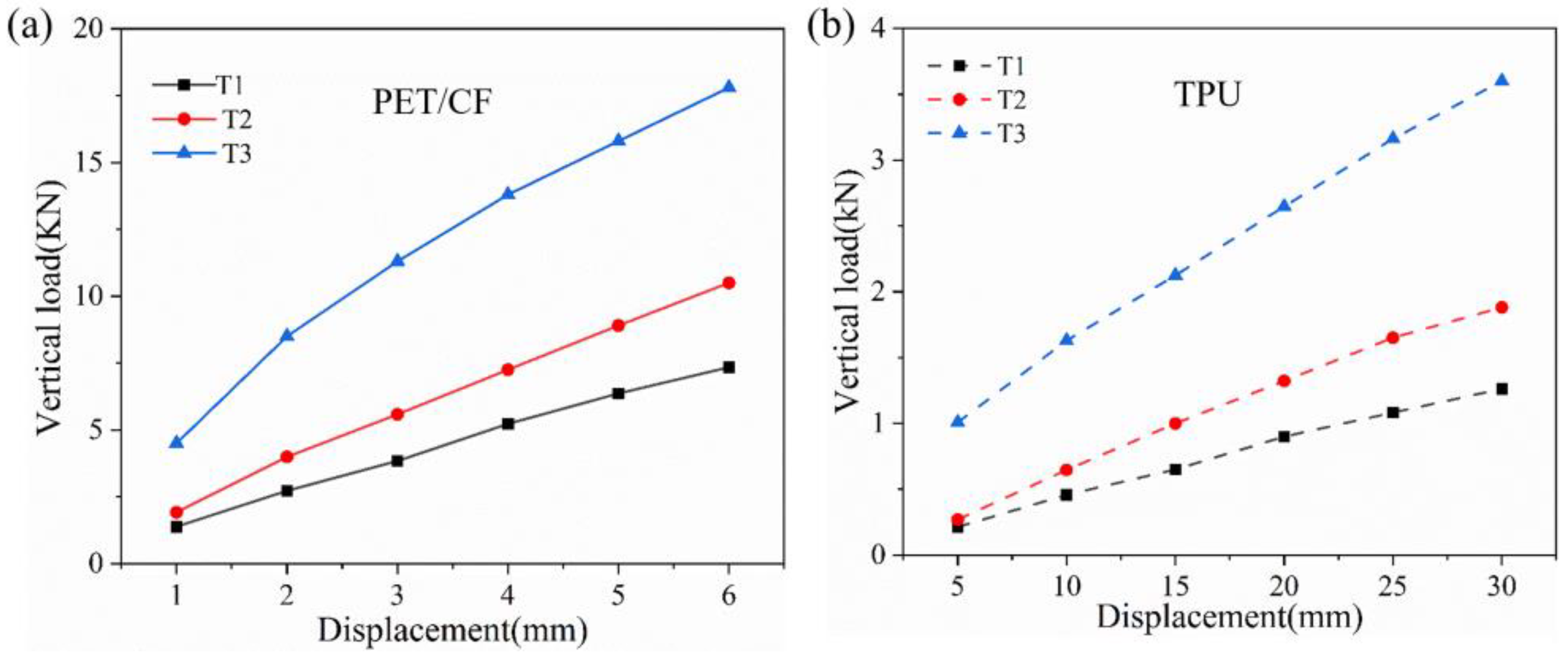
4.4. Analysis of Static Load of Non-Pneumatic Tire with Rim Support Structure
5. Conclusions
- (1)
- The PET/CF proved to possess excellent mechanical properties and good 3D printability. The PET/CF auxetic honeycomb applied as a support structure in an NPT represents a convenient option from geometric design to prototype fabrication.
- (2)
- The quasi-static compression experiment showed that the deformation mode and force displacement results were in good agreement with the actual data, which could be used to predict the compression performance of the structure. In the basic mechanics simulation, the compression performance and natural frequency of the honeycomb structure were positively correlated with the element angle and the wall thickness. The high support force initially showed the bearing potential of the structure.
- (3)
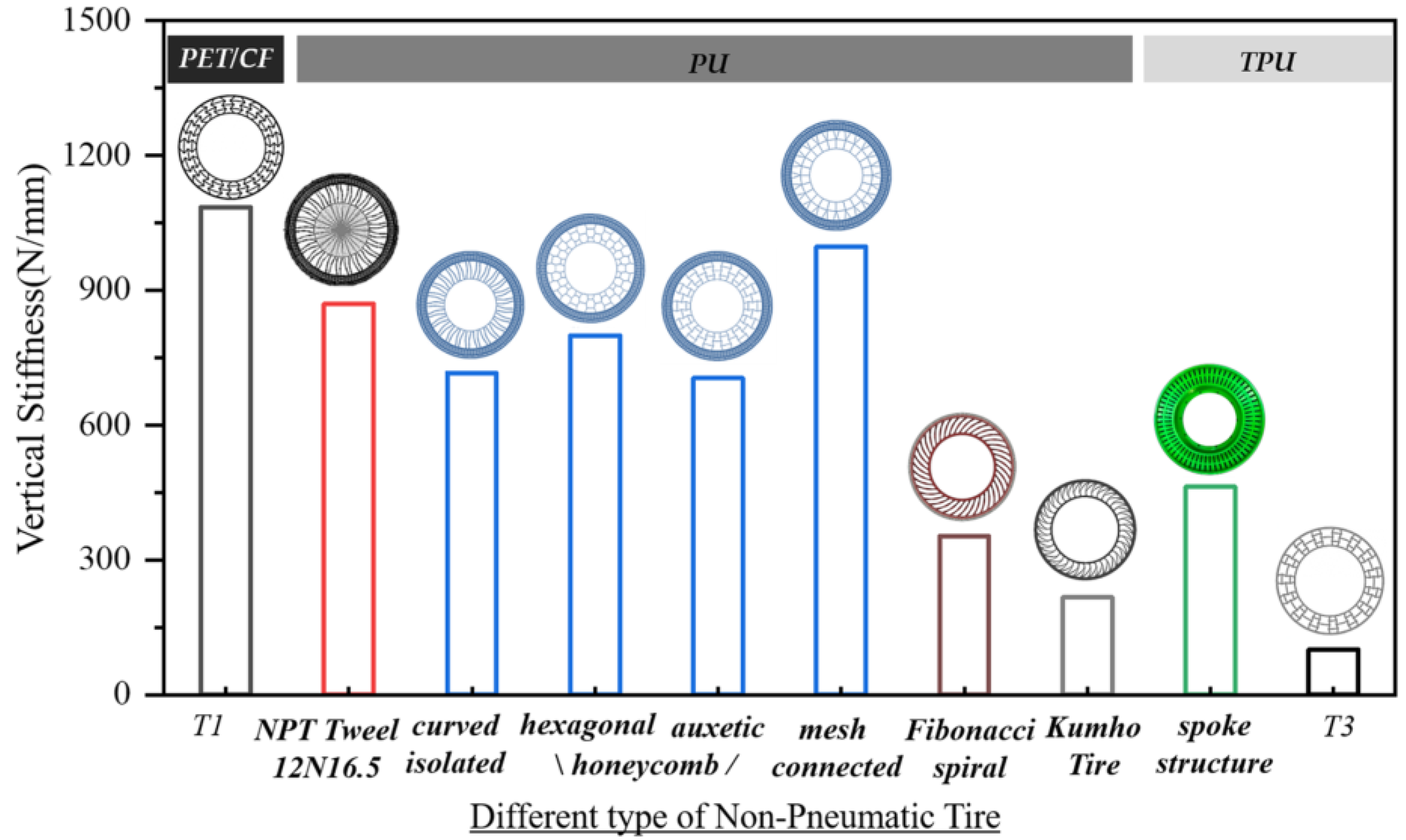
- The support structure of the PET/CF-based NPT had both a high modulus of material and the flexibility of a honeycomb. By changing the honeycomb angle, the deformation of the structure can be regulated on a large scale. High vertical stiffness ensures the stability of the tire. In comparison, it also exhibits superior load-carrying capacity to the TPU-based NPT counterparts.
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Xiong, Y.; Yang, X.G. A review on in-tire sensor systems for tire-road interaction studies. Sens. Rev. 2018, 38, 231–238. [Google Scholar] [CrossRef]
- Deng, S.; Chen, R.X.; Duan, S.Y.; Jia, Q.X.; Hao, X.M.; Zhang, L.Q. Research progress on sustainability of key tire materials. SusMat 2022, 3, 581–608. [Google Scholar] [CrossRef]
- Sienkiewicz, M.; Janik, H.; Borzedowska-Labuda, K.; Kucinska-Lipka, J. Environmentally friendly polymer-rubber composites obtained from waste tyres: A review. J. Cleaner Prod. 2022, 147, 560–571. [Google Scholar] [CrossRef]
- Rugsaj, R.; Suvanjumrat, C. Study of geometric effects on non-pneumatic tire spoke structures using finite element method. Mech. Based Des. Struct. Mach. 2020, 50, 2379–2399. [Google Scholar] [CrossRef]
- Zheng, Z.; Rakheja, S.; Sedaghati, R. Multi-axis stiffness and road contact characteristics of honeycomb wheels: A parametric analysis using Taguchi method. Compos. Struct. 2022, 279, 114735. [Google Scholar] [CrossRef]
- Deng, Y.J.; Wang, Z.Y.; Shen, H.; Gong, J.J.; Xiao, Z. A comprehensive review on non-pneumatic tyre research. Mater. Des. 2023, 227, 111742. [Google Scholar] [CrossRef]
- Bras, B.; Cobert, A. Life-cycle environmental impact of Michelin Tweel tire for passenger vehicles. SAE Int. J. Passen. Car. 2011, 4, 32–43. [Google Scholar] [CrossRef]
- Sassi, S.; Ebrahemi, M.; Mozien, M.A.; Hadary, Y.E. New design of flat-proof nonpneumatic tire. Int. J. Mech. Syst. Eng. 2016, 2, 114. [Google Scholar] [CrossRef] [PubMed]
- Yin, H.F.; Zhang, W.Z.; Zhu, L.C.; Meng, F.B.; Liu, J.E.; Wen, G.L. Review on lattice structures for energy absorption properties. Compos. Struct. 2023, 304, 116397. [Google Scholar] [CrossRef]
- Chandrasekaran, N.K.; Arunachalam, V. State-of-the-art review on honeycomb sandwich composite structures with an emphasis on filler materials. Polym. Compos. 2021, 42, 5011–5020. [Google Scholar] [CrossRef]
- Zang, L.G.; Wang, X.Y.; Yan, P.W.; Zhao, Z.D. Structural design and characteristics of a non-pneumatic tire with honeycomb structure. Mech. Adv. Mater. Struct. 2022, 22, 4066–4073. [Google Scholar] [CrossRef]
- Ganniari-Papageorgiou, E.; Chatzistergos, P.; Wang, X.X. The Influence of the Honeycomb Design Parameters on the Mechanical Behavior of Non-Pneumatic Tires. Int. J. Appl. Mech. 2020, 12, 2050024. [Google Scholar] [CrossRef]
- Zhu, L.L.; Xu, T.; Liu, X.Y.; Wu, M.Q.; Zhou, X.H.; Gao, F. Test and Simulation Study on the Static Load and Pure Longitudinal Slip Characteristics of Non-Pneumatic Tire. Machines 2023, 11, 86. [Google Scholar] [CrossRef]
- Wu, T.Y.; Li, M.X.; Zhu, X.L.; Lu, X.F. Research on non-pneumatic tire with gradient anti-tetrachiral structures. Mech. Adv. Mater. Struct. 2020, 28, 2351–2359. [Google Scholar] [CrossRef]
- Kim, H.S.; Kim, D.; Choi, J.W.; Park, S.H. High Stability in Compressive and Shear Behavior of Airless Tire Using Primitive TPMS-Based Cylindrical Spoke. Int. J. Precis. Eng. Man-Gt. 2023, 1–16. [Google Scholar] [CrossRef]
- Liang, C.; Wei, W.; Mousavi, H.; Chen, K.; Asafo-Duho, B.; Wang, G.L. Investigation on static grounding analysis model of non-pneumatic tire with nonlinear spokes. AIP Adv. 2022, 11, 105303. [Google Scholar] [CrossRef]
- Gasmi, A.; Joseph, P.F.; Rhyne, T.B.; Cron, S.M. Development of a two-dimensional model of a compliant non-pneumatic tire. Int. J. Solids Struct. 2012, 49, 1723–1740. [Google Scholar] [CrossRef]
- Jang, I.G.; Sung, Y.H.; Yoo, E.J.; Kwak, B.M. Pattern design of a non-pneumatic tyre for stiffness using topology optimization. Eng. Optim. 2012, 44, 119–131. [Google Scholar] [CrossRef]
- Kucewicz, M.; Baranowski, P.; Malachowski, J. Airless tire conceptions modeling and simulations. In Proceedings of the 13th International Scientific Conference: Computer Aided Engineering, Polanica Zdroj, Poland, 22–25 June 2016; pp. 293–301. [Google Scholar] [CrossRef]
- Aboul-Yazid, A.M.; Emam, M.A.A.; Shaaban, S.; El-Nashar, M. Effect of spokes structures on characteristics performance of non-pneumatic tires. Int. J. Automot. Mech. Eng. 2015, 11, 2212–2223. [Google Scholar] [CrossRef]
- Rugsaj, R.; Suvanjumrat, C. Proper radial spokes of nonpneumatic tire for vertical load supporting by finite element analysis. Int. J. Automot. Technol. 2019, 20, 801–812. [Google Scholar] [CrossRef]
- Li, Y.; Sun, H.J.; Song, J.L.; Zhang, S.D.; Han, S.L. Topological structure and experimental investigation of a novel whole tire bead. Mater. Des. 2021, 203, 109592. [Google Scholar] [CrossRef]
- Guessasma, S.; Belhabib, S.; Bassir, D.; Nouri, H.; Gomes, S. On the Mechanical Behaviour of Biosourced Cellular Polymer Manufactured Using Fused Deposition Modelling. Polymers 2020, 12, 2651. [Google Scholar] [CrossRef]
- Jafferson, J.M.; Sharma, H. Design of 3D printable airless tyres using NTopology. Mater. Today Proc. 2021, 46, 1147–1160. [Google Scholar] [CrossRef]
- Dezianian, S.; Azadi, M. Multi-Material Metamaterial Topology Optimization to Minimize the Compliance and the Constraint of Weight: Application of Non-Pneumatic Tire Additive-Manufactured with PLA/TPU Polymers. Polymers 2023, 15, 1927. [Google Scholar] [CrossRef]
- Wang, J.; Yang, B.; Lin, X.; Gao, L.; Liu, T.; Lu, Y.L.; Wang, R.G. Research of TPU Materials for 3D Printing Aiming at Non-Pneumatic Tires by FDM Method. Polymers 2020, 12, 2492. [Google Scholar] [CrossRef]
- Wang, K.; Wang, D.P.; Liu, Y.S.; Gao, H.J.; Yang, C.X.; Peng, Y. Path Planning and Bending Behaviors of 3D Printed Continuous Carbon Fiber Reinforced Polymer Honeycomb Structures. Polymers 2022, 15, 4485. [Google Scholar] [CrossRef]
- Ju, J.Y.; Summers, J.D. Compliant hexagonal periodic lattice structures having both high shear strength and high shear strain. Mater. Des. 2011, 32, 512–524. [Google Scholar] [CrossRef]
- Bekas, D.G.; Hou, Y.; Liu, Y.; Panesar, A. 3D printing to enable multifunctionality in polymer-based composites: A review. Compos. B Eng. 2019, 179, 107540. [Google Scholar] [CrossRef]
- Sang, L.; Han, S.F.; Peng, X.S.; Jian, X.G.; Wang, J.Y. Development of 3D-printed basalt fiber reinforced thermoplastic honeycombs with enhanced compressive mechanical properties. Compos. Part A Appl. Sci. Manuf. 2019, 125, 105508. [Google Scholar] [CrossRef]
- Qin, D.X.; Sang, L.; Zhang, Z.H.; Lai, S.Y.; Zhao, Y.P. Compression Performance and Deformation Behavior of 3D-Printed PLA-Based Lattice Structures. Polymers 2022, 14, 1062. [Google Scholar] [CrossRef]
- Hou, W.B.; He, P.; Yang, Y.; Sang, L. Crashworthiness optimization of crash box with 3D-printed lattice structures. Int. J. Mech. Sci. 2023, 247, 108198. [Google Scholar] [CrossRef]
- Wang, H.; Lu, Z.X.; Yang, Z.Y.; Li, X. A novel re-entrant auxetic honeycomb with enhanced in-plane impact resistance. Compos. Struct. 2019, 208, 758–770. [Google Scholar] [CrossRef]
- Choudhry, N.K.; Panda, B.; Kumar, S. In-plane energy absorption characteristics of a modified re-entrant auxetic structure fabricated via 3D printing. Compos. B Eng. 2022, 228, 109437. [Google Scholar] [CrossRef]
- Quan, C.; Han, B.; Hou, Z.H.; Zhang, Q.; Tian, X.Y.; Lu, T.J. 3d printed continuous fiber reinforced composite auxetic honeycomb structures. Compos. Part B Eng. 2020, 187, 107858. [Google Scholar] [CrossRef]
- He, P.; Wang, S.W.; Zhang, M.M.; Sang, L.; Tong, L.Y.; Hou, W.B. Compression performance of 3D-printed thermoplastic auxetic structures. Thin Wall Struct. 2024, 197, 111558. [Google Scholar] [CrossRef]
- Ju, J.; Kim, D.M.; Kim, K. Flexible cellular solid spokes of a non-pneumatic tire. Compos. Struct. 2012, 94, 2285–2295. [Google Scholar] [CrossRef]
- ISO 527; Plastics_Determination of Tensile Properties. ISO: Geneva, Switzerland, 2019.
- ISO 178; Plastics_Determination of Flexural Properties. ISO: Geneva, Switzerland, 2019.
- ISO 604; Plastics_Determination of Compressive Properties. ISO: Geneva, Switzerland, 2002.
- Guo, Z.C.; Peng, X.S.; Sang, L. Enhancement of Mechanical Properties of PA6 Blending with Talcum for Fused Deposition Modeling. Macromol. Mater. Eng. 2020, 305, 2000355. [Google Scholar] [CrossRef]
- Chapa, A.; Cuan-Urquizo, E.; Urbina-Coronado, P.; Roman-Flores, A. Experimental characterization of the mechanical properties of 3D printed TPU auxetic cellular materials under cyclic compressive loadings. Rapid Prototyp. J. 2023, 39, 1800–1813. [Google Scholar] [CrossRef]
- Fu, H.X.; Chen, X.X.; Zhao, Q.; Xiao, Z.; Liang, X.M. Fatigue life prediction and influencing factors analysis of mesh flexible spoke non-pneumatic tire. Adv. Mech. Eng. 2021, 13, 1–10. [Google Scholar] [CrossRef]
- Platek, P.; Rajkowski, K.; Cieplak, K.; Sarzynski, M.; Malachowski, J.; Wozniak, R.; Janiszewski, J. Deformation Process of 3D Printed Structures Made from Flexible Material with Different Values of Relative Density. Polymers 2020, 12, 2120. [Google Scholar] [CrossRef]
- Sim, J.; Hong, J.; Cho, I.; Lee, J. Analysis of Vertical Stiffness Characteristics Based on Spoke Shape of Non-Pneumatic Tire. Appl. Sci. 2021, 11, 2369. [Google Scholar] [CrossRef]
- Rugsaj, R.; Suvanjumrat, C. Mechanical characteristics of airless tyre by laboratory testing. In Proceedings of the International Conference on Materials Research and Innovation (ICMARI), Bangkok, Thailand, 16–18 December 2019. [Google Scholar] [CrossRef]
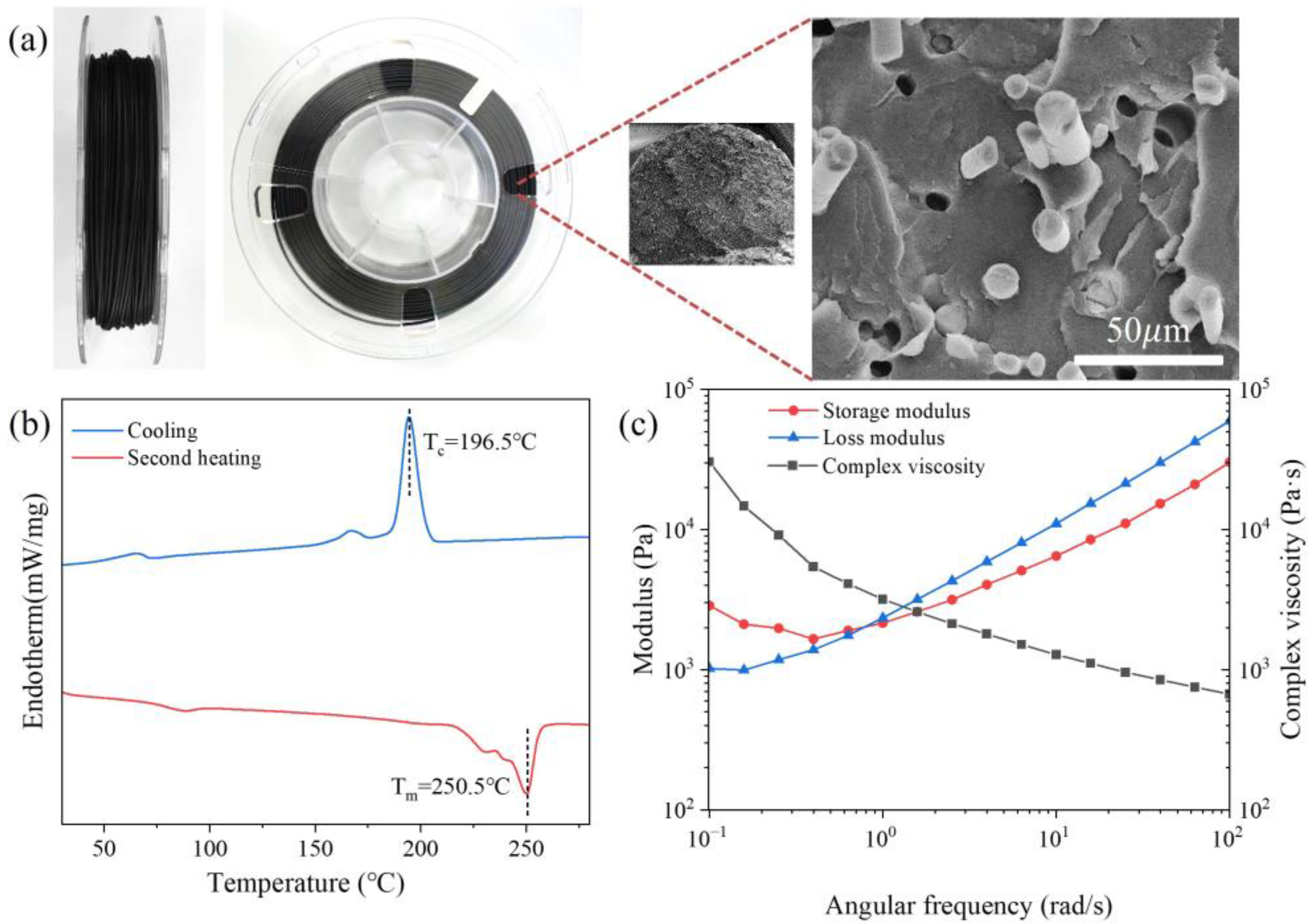

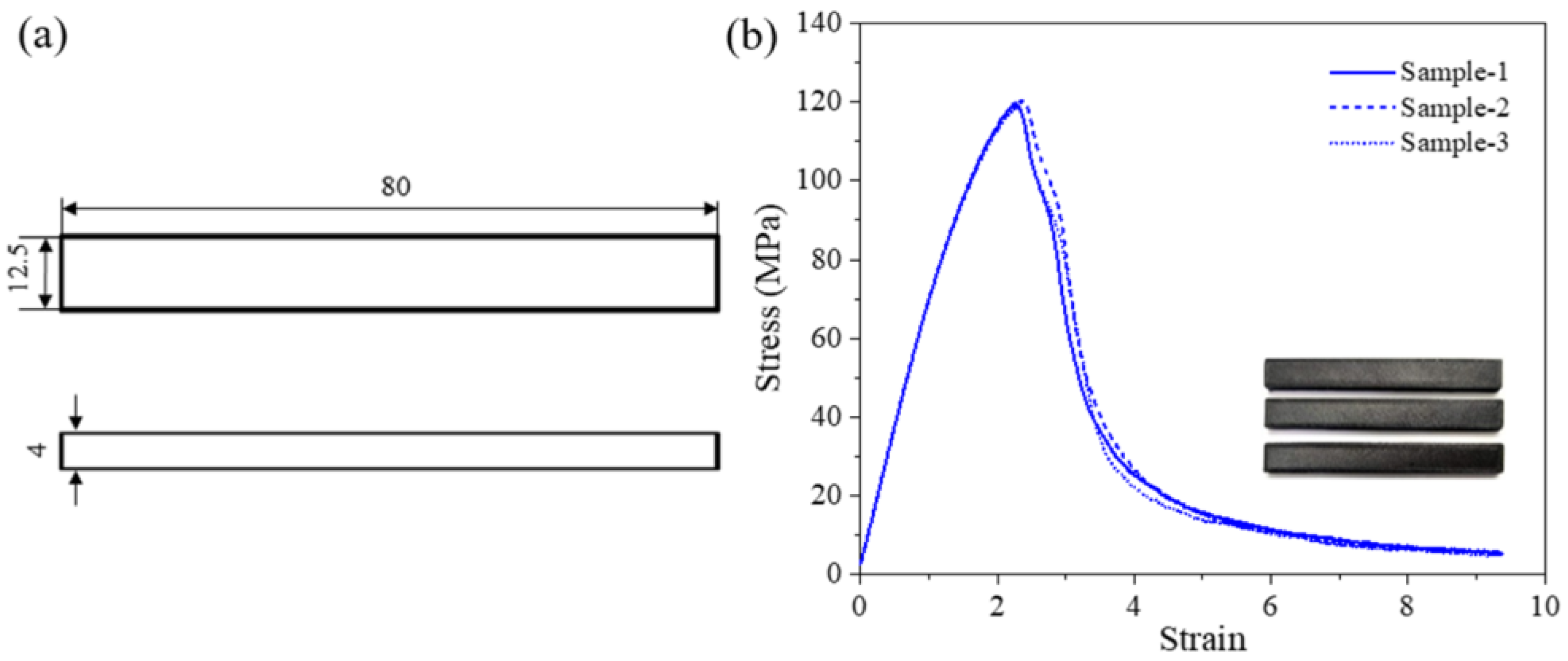




| Sample | 1 | 2 | 3 | Mean | SD |
|---|---|---|---|---|---|
| Tensile modulus (MPa) | 3473.1 | 3391.6 | 3462.2 | 3442.3 | 44.2 |
| Tensile strength (MPa) | 82.5 | 82.2 | 76.9 | 80.5 | 3.2 |
| Flexural modulus (MPa) | 6840 | 6803.5 | 7130.5 | 6924.7 | 179.2 |
| Flexural strength (MPa) | 120.3 | 119.5 | 118.4 | 119.4 | 1.0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, S.; He, P.; Geng, Q.; Huang, H.; Sang, L.; Yao, Z. Investigation of Additive-Manufactured Carbon Fiber-Reinforced Polyethylene Terephthalate Honeycomb for Application as Non-Pneumatic Tire Support Structure. Polymers 2024, 16, 1091. https://doi.org/10.3390/polym16081091
Wang S, He P, Geng Q, Huang H, Sang L, Yao Z. Investigation of Additive-Manufactured Carbon Fiber-Reinforced Polyethylene Terephthalate Honeycomb for Application as Non-Pneumatic Tire Support Structure. Polymers. 2024; 16(8):1091. https://doi.org/10.3390/polym16081091
Chicago/Turabian StyleWang, Siwen, Pan He, Quanqiang Geng, Hui Huang, Lin Sang, and Zaiqi Yao. 2024. "Investigation of Additive-Manufactured Carbon Fiber-Reinforced Polyethylene Terephthalate Honeycomb for Application as Non-Pneumatic Tire Support Structure" Polymers 16, no. 8: 1091. https://doi.org/10.3390/polym16081091
APA StyleWang, S., He, P., Geng, Q., Huang, H., Sang, L., & Yao, Z. (2024). Investigation of Additive-Manufactured Carbon Fiber-Reinforced Polyethylene Terephthalate Honeycomb for Application as Non-Pneumatic Tire Support Structure. Polymers, 16(8), 1091. https://doi.org/10.3390/polym16081091







