Numerical Simulation of Flow Characteristics for Supercritical CO2-Sprayed Polyurethane Resin
Abstract
1. Introduction
2. Experimental Section
3. Numerical Methodology
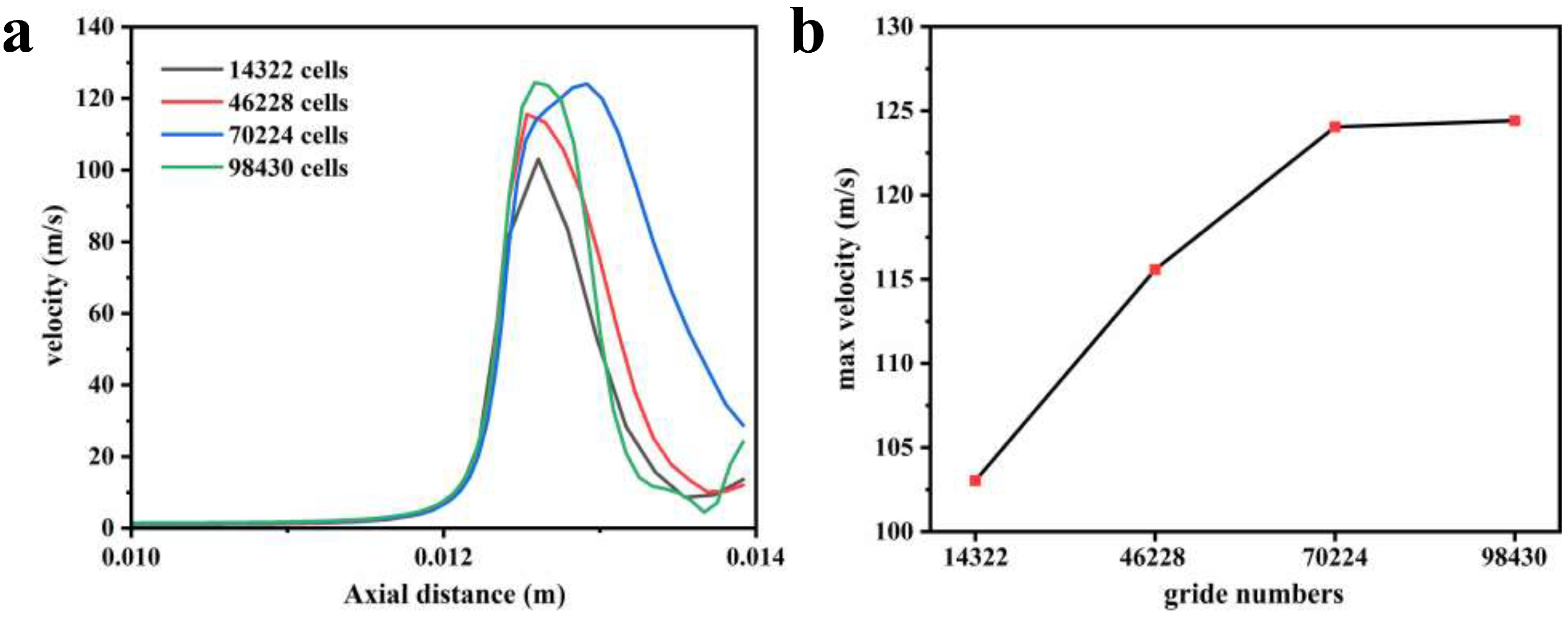
3.1. Geometry and Computational Domains
3.2. Numerical Calculation Method
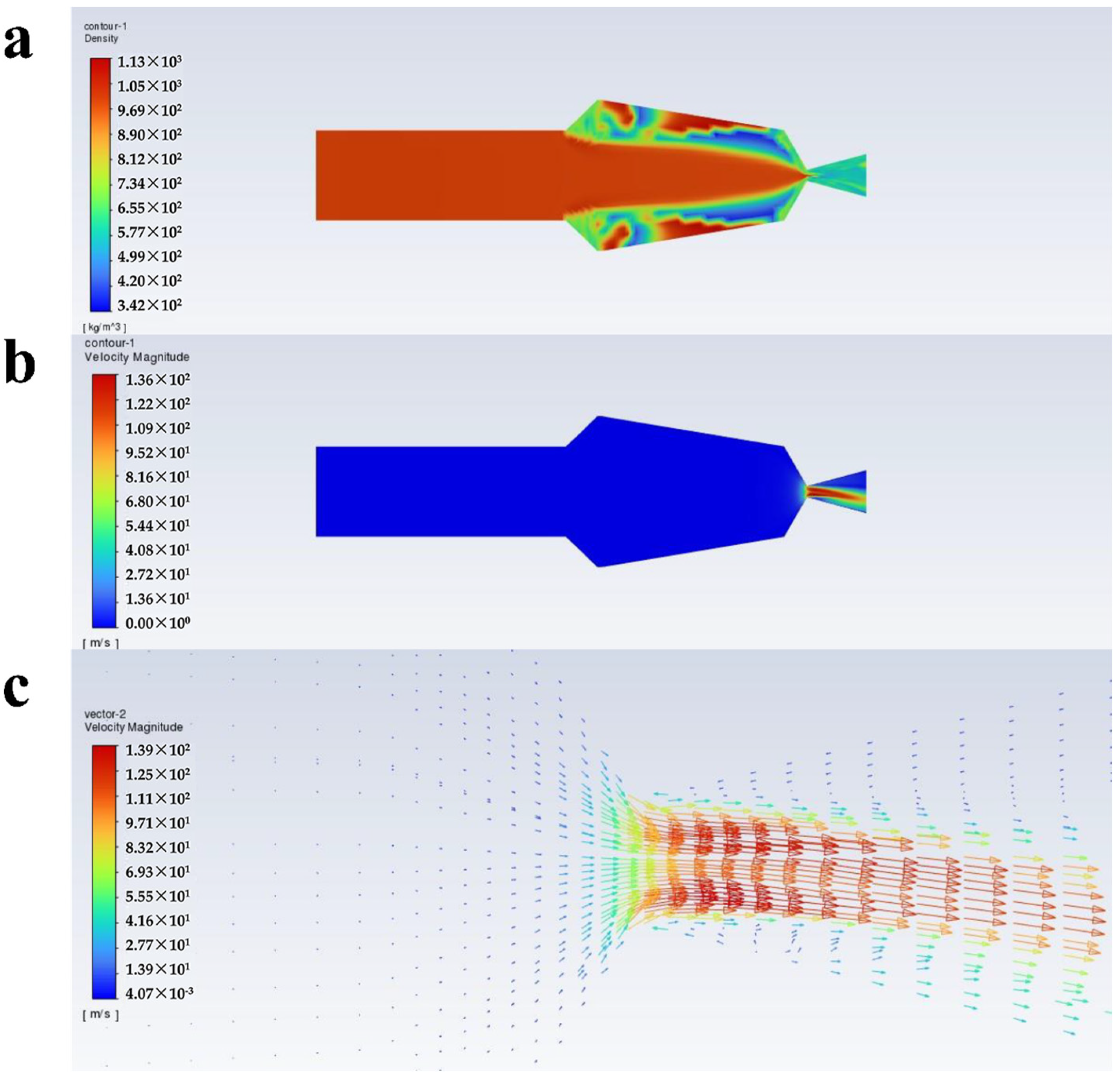
4. Results and Discussions
5. Conclusions
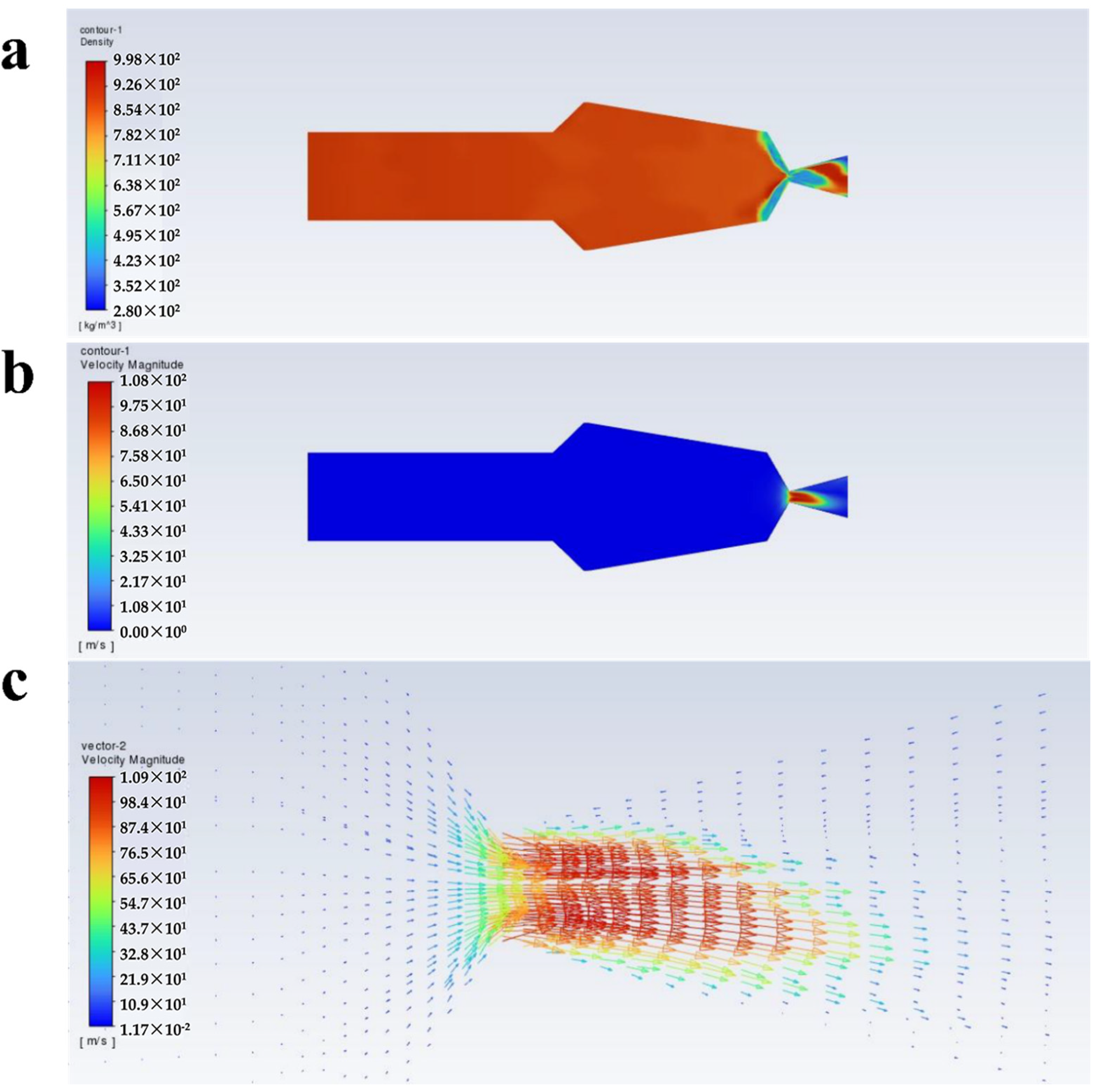
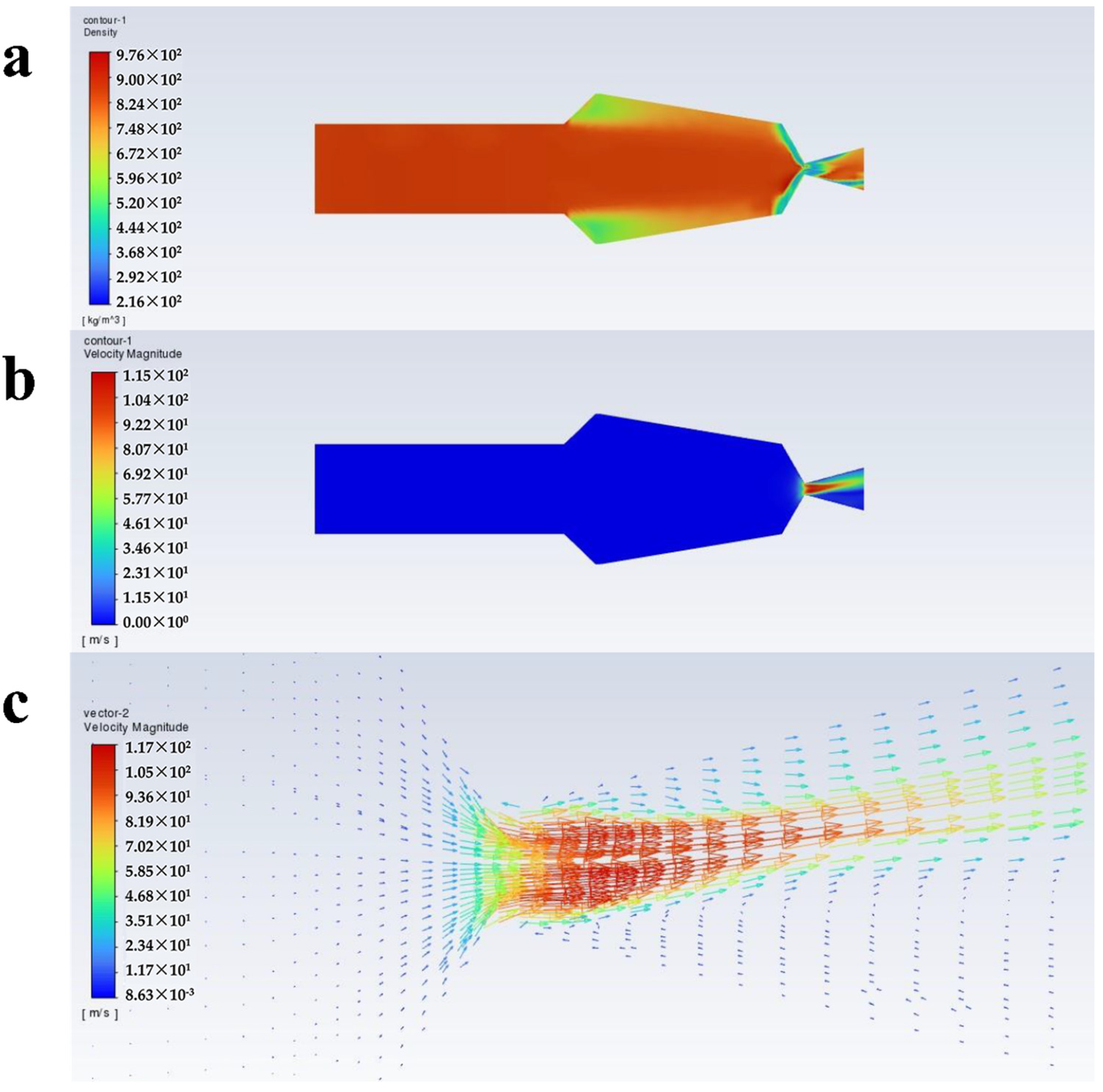
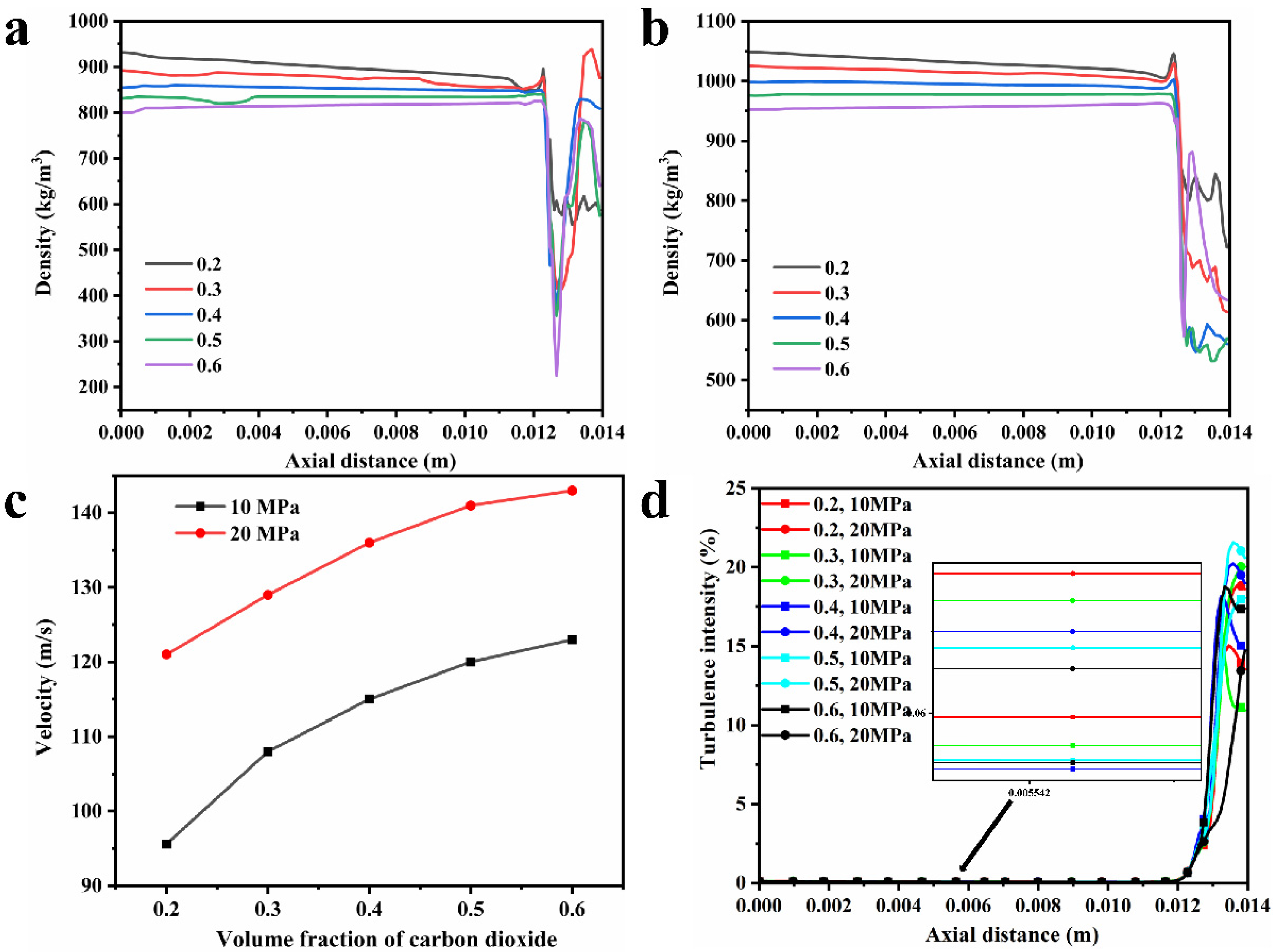
- At an inlet pressure of 10 MPa, as the volume fraction of scCO2 increases from 0.2 to 0.6, the velocity of the paint through the throat section is 95.6 m/s, 108 m/s, 115 m/s, 120 m/s, and 123 m/s. In the other cases with an inlet pressure of 20 MPa, the velocity is 121 m/s, 129 m/s, 136 m/s, 141 m/s and 143 m/s. There is a decreased tendency for the velocity from the throat section to the nozzle outlet. Near the nozzle, the density becomes inhomogeneous and the turbulence intensity increases.
- The velocity vector plots under the ten conditions do not show any obvious backflow, which is conducive to the improvement of the atomization effect of the paint with scCO2 as the solvent, and contributes to improving the spraying quality.
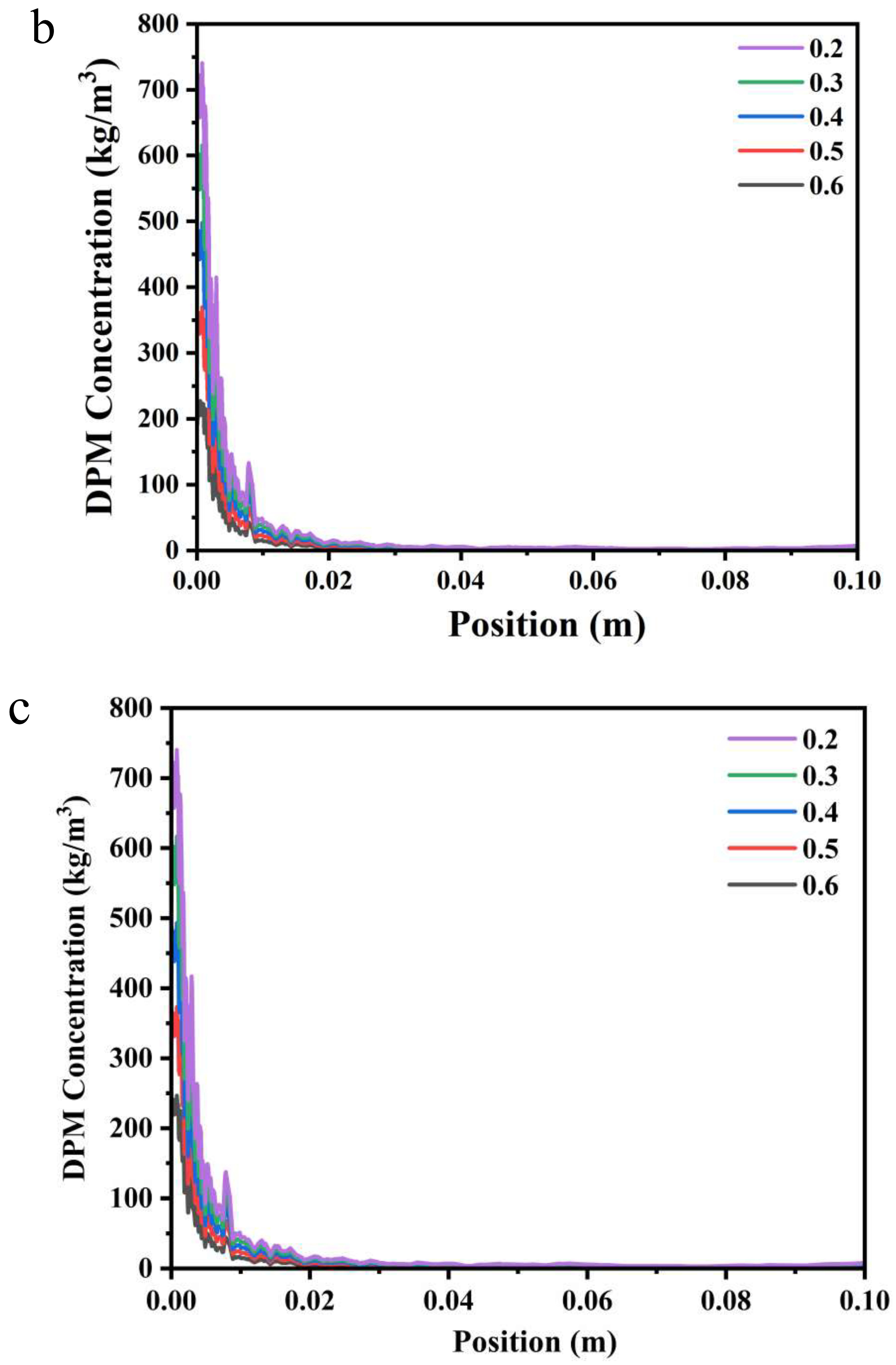
- At the same inlet pressure, systems with a large scCO2 volume percentage have a higher DPM concentration near the shaft compared to coatings with a small scCO2 volume fraction. For systems with the same scCO2 volume fraction, the DPM concentration at 10 MPa inlet pressure is slightly greater than that at 20 MPa inlet pressure.
- The higher inlet pressure results in better atomization of the paint, smaller particles, and more quantities. While a larger carbon dioxide volume fraction will further reduce the droplet size, the total number of particles is small because the polyurethane coating content is too low. Based on the consideration of the atomization effect and actual needs, it is recommended to use 0.4 volume fraction and 20 MPa inlet pressure for spray painting in industry.
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Kausar, A. Estimation of thermo-mechanical and fire resistance profile of epoxy coated polyurethane/fullerene composite films. Fuller. Nanotub. Carbon Nanostruct. 2016, 24, 391–399. [Google Scholar] [CrossRef]
- Kausar, A. Polyurethane Composite Foams in High-Performance Applications: A Review. Polym.-Plast. Technol. Eng. 2017, 57, 346–369. [Google Scholar] [CrossRef]
- Jena, K.K.; Sahoo, S.; Narayan, R.; Aminabhavi, T.M.; Raju, K. Novel hyperbranched waterborne polyurethane-urea/silica hybrid coatings and their characterizations. Polym. Int. 2011, 60, 1504–1513. [Google Scholar] [CrossRef]
- Patel, R.H.; Patel, K.S. Synthesis of flame retardant polyester-urethanes and their applications in nanoclay composites and coatings. Polym. Int. 2014, 63, 529–536. [Google Scholar] [CrossRef]
- Kausar, A. Polyurethane nanocomposite coatings: State of the art and perspectives. Polym. Int. 2018, 67, 1470–1477. [Google Scholar] [CrossRef]
- Sun, Z.; Fan, H.; Chen, Y.; Huang, J. Synthesis of self-matting waterborne polyurethane coatings with excellent transmittance. Polym. Int. 2018, 67, 78–84. [Google Scholar] [CrossRef]
- Toader, G.; Diacon, A.; Axinte, S.M.; Mocanu, A.; Rusen, E. State-of-the-Art Polyurea Coatings: Synthesis Aspects, Structure–Properties Relationship, and Nanocomposites for Ballistic Protection Applications. Polymers 2024, 16, 454. [Google Scholar] [CrossRef] [PubMed]
- Bakhshandeh, E.; Sobhani, S.; Jafari, R.; Momen, G. New insights into tailoring physicochemical properties for optimizing the anti-icing behavior of polyurethane coatings. J. Appl. Polym. Sci. 2023, 140, e54610. [Google Scholar] [CrossRef]
- Luo, H.; Wei, H.; Wang, Z.; Li, H.; Chen, Y.; Xiang, J.; Fan, H. Fabrication of UV-curable waterborne polyurethane coatings with self-cleaning, anti-graffiti performance, and corrosion resistance. Colloids Surf. A Physicochem. Eng. Asp. 2023, 676, 132177. [Google Scholar] [CrossRef]
- Yu, C.; Wang, Z.; Fei, G.; Zhang, X.; de Luna, M.S.; Lavorgna, M.; Xia, H. Robust self-healing waterborne polyurethane coatings via dynamic covalent Diels-Alder bonds for corrosion protection. J. Polym. Sci. 2024, 62, 815–825. [Google Scholar] [CrossRef]
- Petrovicova, E.; Schadler, L.S. Thermal spraying of polymers. Int. Mater. Rev. 2013, 47, 169–190. [Google Scholar] [CrossRef]
- Koivuluoto, H. A Review of Thermally Sprayed Polymer Coatings. J. Therm. Spray Technol. 2022, 31, 1750–1764. [Google Scholar] [CrossRef]
- Chi, H.; Chen, P.; Cao, L.; Wu, X.; Wang, J. Characterization and adsorptive properties of cross-linked poly (1-vinylimidazole)-iron (III) complex synthesized in supercritical carbon dioxide. e-Polymers 2016, 16, 403–410. [Google Scholar] [CrossRef]
- Zhou, Y.; Tian, Y.; Peng, X. Applications and Challenges of Supercritical Foaming Technology. Polymers 2023, 15, 402. [Google Scholar] [CrossRef]
- Haurat, M.; Anguy, Y.; Gaborieau, C.; Aubert, G.; Aymonier, C.; Dumon, M. High-pressure drop rates in solid-state batch one-step scCO2 foaming of acrylic polymers: A way to stabilize the structure of micro-nano foams. Chem. Eng. Sci. 2023, 281, 119099. [Google Scholar] [CrossRef]
- Khalati, E.; Oinas, P.; Favén, L. Techno-economic and safety assessment of supercritical CO2 extraction of essential oils and extracts. J. CO2 Util. 2023, 74, 102547. [Google Scholar] [CrossRef]
- Wang, W.; Cao, L.; Wang, J.; Zhao, J.; Wu, X.; Hao, Y. Characterization and adsorptive properties of poly(1-vinylimidazole)/silica nanocomposites synthesized in supercritical carbon dioxide. e-Polymers 2015, 15, 245–254. [Google Scholar] [CrossRef]
- Olin, P.; Hyll, C.; Ovaskainen, L.; Ruda, M.; Schmidt, O.; Turner, C.; Wågberg, L. Development of a Semicontinuous Spray Process for the Production of Superhydrophobic Coatings from Supercritical Carbon Dioxide Solutions. Ind. Eng. Chem. Res. 2015, 54, 1059–1067. [Google Scholar] [CrossRef]
- Zurita, I.; Saleha, M.; Mohamed Faris, L.; Kai Xin, S.; Muhamamd Rezal Kamel, A.; Nizam, T. CFD Simulation Using ANSYS FLUENT of Jet Nozzle of Ethanol at Temperature of 360 K. J. Adv. Res. Fluid Mech. Therm. Sci. 2022, 96, 168–178. [Google Scholar] [CrossRef]
- Feng, H.; Xu, P.; Yang, S.; Zheng, Y.; Li, W.; Liu, W.; Zhao, H.; Jiang, S. Back pressure generated by downwash and crosswind on spatial atomization characteristics during UAV spraying: CFD analysis and verification. Pest Manag. Sci. 2023, 80, 1348–1360. [Google Scholar] [CrossRef]
- Wang, J.; Wu, J.; Yuan, S.; Yan, W.-C. CFD simulation of ultrasonic atomization pyrolysis reactor: The influence of droplet behaviors and solvent evaporation. Int. J. Chem. React. Eng. 2021, 19, 167–178. [Google Scholar] [CrossRef]
- Yu, M.; Sediq, A.S.; Zhang, S.; Nejadnik, M.R.; Every, H.A.; Jiskoot, W.; Witkamp, G.-J. Towards the development of a supercritical carbon dioxide spray process to coat solid protein particles. J. Supercrit. Fluids 2018, 141, 49–59. [Google Scholar] [CrossRef]
- Hao, J.; Whitaker, M.J.; Wong, B.; Serhatkulu, G.; Shakesheff, K.M.; Howdle, S.M. Plasticization and spraying of poly (DL-lactic acid) using supercritical carbon dioxide: Control of particle size. J. Pharm. Sci. 2004, 93, 1083–1090. [Google Scholar] [CrossRef] [PubMed]
- Kim, K.D.; Jin, D.H.; Choi, Y.C. Numerical simulation on the generation of ultrasound and formation of water fog in the ultrasonic gas atomizer. Ultrasonics 2020, 102, 105851. [Google Scholar] [CrossRef]
- Stieger, S.; Mitsoulis, E.; Walluch, M.; Ebner, C.; Kerschbaumer, R.C.; Haselmann, M.; Mostafaiyan, M.; Kämpfe, M.; Kühnert, I.; Wießner, S.; et al. On the Influence of Viscoelastic Modeling in Fluid Flow Simulations of Gum Acrylonitrile Butadiene Rubber. Polymers 2021, 13, 2323. [Google Scholar] [CrossRef]
- Du, X.; Mutsuda, H.; Wasada, Y.; Nakashima, T. Hybrid simulation of dissipative particle dynamics and computational fluid dynamics for friction drag reduction of polymer coatings. Ocean Eng. 2023, 285, 115415. [Google Scholar] [CrossRef]
- Zhang, Z.; Meng, T.L.; Lee, C.J.J.; Wei, F.; Ba, T.; Zhang, Z.-Q.; Pan, J. Experimental and Numerical Study of Al2219 Powders Deposition on Al2219-T6 Substrate by Cold Spray: Effects of Spray Angle, Traverse Speed, and Standoff Distance. Materials 2023, 16, 5240. [Google Scholar] [CrossRef]
- HG/T 2454-2014; Solvent-Thinned Polyurethane Coatings (Two-Component). Ministry of Industry and Information Technology of the People’s Republic of China: Beijing, China, 2014.
- Wu, Y. Study on the Flow of Supercritical CO2 Spraying. Master’s Thesis, Fuzhou University, Fuzhou, China, 2010. [Google Scholar]
- Lupoi, R.; O’Neill, W. Powder stream characteristics in cold spray nozzles. Surf. Coat. Technol. 2011, 206, 1069–1076. [Google Scholar] [CrossRef]
- Sova, A.; Okunkova, A.; Grigoriev, S.; Smurov, I. Velocity of the Particles Accelerated by a Cold Spray Micronozzle: Experimental Measurements and Numerical Simulation. J. Therm. Spray Technol. 2012, 22, 75–80. [Google Scholar] [CrossRef]
- Lupoi, R. Current design and performance of cold spray nozzles: Experimental and numerical observations on deposition efficiency and particle velocity. Surf. Eng. 2013, 30, 316–322. [Google Scholar] [CrossRef]
- Jiang, X.; Siamas, G.A.; Jagus, K.; Karayiannis, T.G. Physical modelling and advanced simulations of gas–liquid two-phase jet flows in atomization and sprays. Prog. Energy Combust. Sci. 2010, 36, 131–167. [Google Scholar] [CrossRef]
- Champagne, V.K.; Helfritch, D.J.; Dinavahi, S.P.G.; Leyman, P.F. Theoretical and Experimental Particle Velocity in Cold Spray. J. Therm. Spray Technol. 2010, 20, 425–431. [Google Scholar] [CrossRef]
- Singhal, C.; Murtaza, Q.; Parvej. Simulation of Critical Velocity of Cold Spray Process with Different Turbulence Models. Mater. Today Proc. 2018, 5, 17371–17379. [Google Scholar] [CrossRef]
- Ansys Inc. Ansys Fluent Users Guide 2020; Ansys Inc.: Canonsburg, PA, USA, 2020. [Google Scholar]
- Gao, H.; Li, X.; Xue, J.; Bai, H.; He, X.; Liu, F. A modification to the WAVE breakup model for evaporating diesel spray. Appl. Therm. Eng. 2016, 108, 555–566. [Google Scholar] [CrossRef]
- Tonelli, C.; Ajroldi, G. New fluoro-modified thermoplastic polyurethanes. J. Appl. Polym. Sci. 2003, 87, 2279–2294. [Google Scholar] [CrossRef]
- Xia, Y.; Khezzar, L.; Alshehhi, M.; Hardalupas, Y. Droplet size and velocity characteristics of water-air impinging jet atomizer. Int. J. Multiph. Flow 2017, 94, 31–43. [Google Scholar] [CrossRef]
- Vardaman, N.J.; Agrawal, A.K. Computational Analysis of Two-Phase Mixing Inside A Twin-Fluid, Fuel-Flexible Atomizer. In Proceedings of the ASME Turbo Expo 2017: Turbomachinery Technical Conference and Exposition, Charlotte, NC, USA, 26–30 June 2017; Volume 3. [Google Scholar]
- Zhang, H.; Zhang, L.; Zhang, H.; Wu, J.; An, X.; Yang, D. Fibre bridging and nozzle clogging in 3D printing of discontinuous carbon fibre-reinforced polymer composites: Coupled CFD-DEM modelling. Int. J. Adv. Manuf. Technol. 2021, 117, 3549–3562. [Google Scholar] [CrossRef]

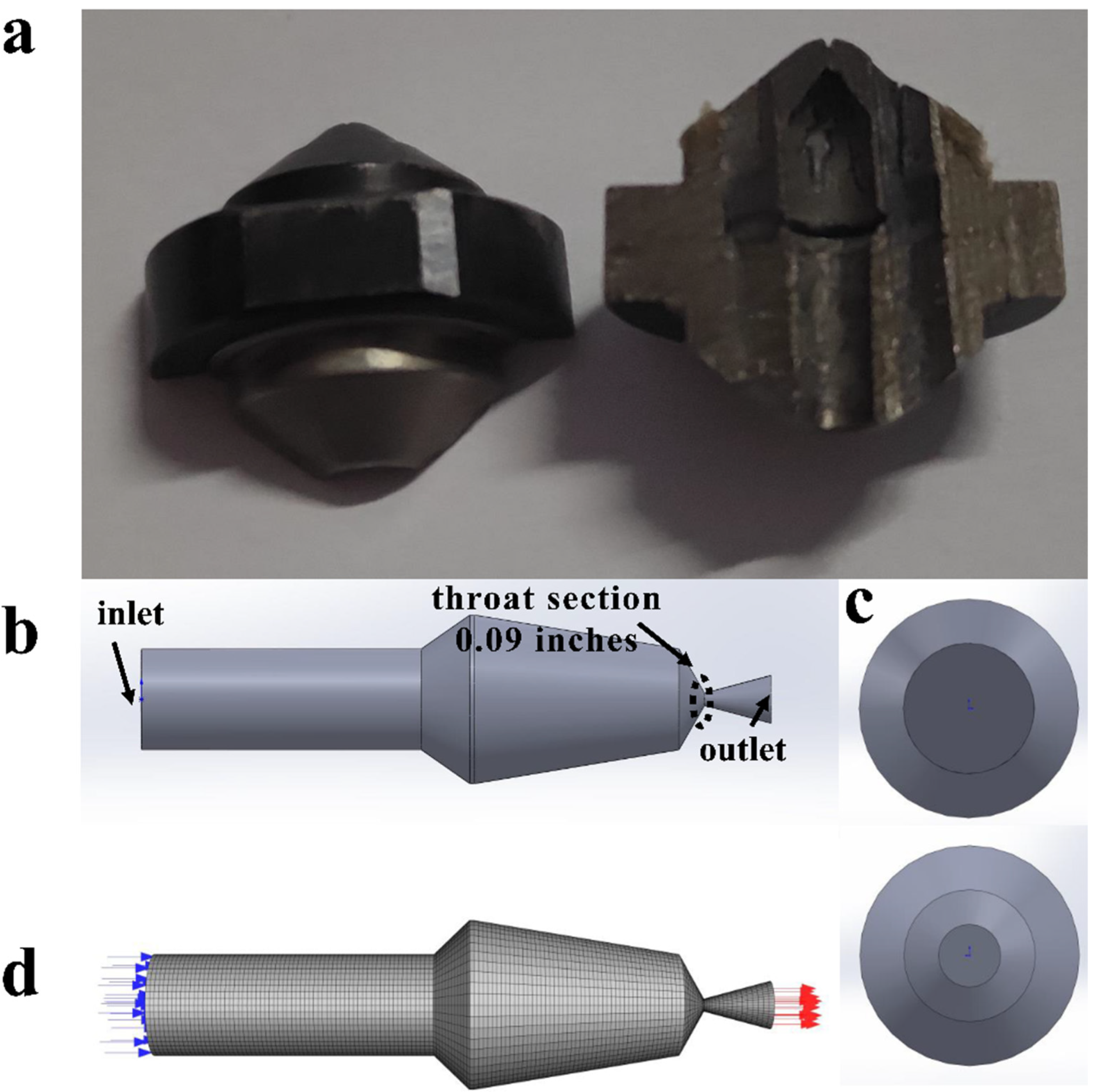



| Test Items | Technical Requirements | Test Result |
|---|---|---|
| Pencil scratch hardness test | ≥F | HB |
| Bending test (mm) | 2 | 4 |
| Impact resistance (cm) | 50 | 50 |
| Cross cut test (spacing 3 mm), level | ≤1 | 1 |
| Pull-off test (MPa) | ≥4 | 14 (12.1~15.7), 100%B |
| Condition | Case I | Case II | Case III | Case IV | Case V | Case VI | Case VII | Case VIII | Case IX | Case X |
|---|---|---|---|---|---|---|---|---|---|---|
| Driving gas pressure (MPa) | 10 | 20 | 10 | 20 | 10 | 20 | 10 | 20 | 10 | 20 |
| Driving gas temperature (K) | 330 | 330 | 330 | 330 | 330 | 330 | 330 | 330 | 330 | 330 |
| Volume fraction of scCO2 | 0.2 | 0.2 | 0.3 | 0.3 | 0.4 | 0.4 | 0.5 | 0.5 | 0.6 | 0.6 |
| Maximum Particle Diameter, 10−5 (m) | Minimum Particle Diameter, 10−5 (m) | Overall Mean Diameter, 10−5 (m) | |
|---|---|---|---|
| Case I | 4.3 | 2.2 | 3.7 |
| Case II | 2.8 | 1.4 | 2.3 |
| Case III | 3.5 | 1.7 | 3.0 |
| Case IV | 2.6 | 1.2 | 2.1 |
| Case V | 3.3 | 1.5 | 2.7 |
| Case VI | 2.4 | 1.1 | 1.9 |
| Case VII | 3.1 | 1.2 | 2.4 |
| Case VIII | 2.4 | 0.9 | 1.8 |
| Case IX | 3.0 | 1.2 | 2.3 |
| Case X | 2.3 | 0.9 | 1.7 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, C.; Zhang, C.; Xiang, M.; Chen, Q.; Luo, Z.; Luo, Y. Numerical Simulation of Flow Characteristics for Supercritical CO2-Sprayed Polyurethane Resin. Polymers 2024, 16, 940. https://doi.org/10.3390/polym16070940
Li C, Zhang C, Xiang M, Chen Q, Luo Z, Luo Y. Numerical Simulation of Flow Characteristics for Supercritical CO2-Sprayed Polyurethane Resin. Polymers. 2024; 16(7):940. https://doi.org/10.3390/polym16070940
Chicago/Turabian StyleLi, Chichao, Chengrui Zhang, Minghua Xiang, Qing Chen, Zhenyang Luo, and Yanlong Luo. 2024. "Numerical Simulation of Flow Characteristics for Supercritical CO2-Sprayed Polyurethane Resin" Polymers 16, no. 7: 940. https://doi.org/10.3390/polym16070940
APA StyleLi, C., Zhang, C., Xiang, M., Chen, Q., Luo, Z., & Luo, Y. (2024). Numerical Simulation of Flow Characteristics for Supercritical CO2-Sprayed Polyurethane Resin. Polymers, 16(7), 940. https://doi.org/10.3390/polym16070940







