Relaxation Phenomena in Low-Density and High-Density Polyethylene
Abstract
1. Introduction
2. Experimental
2.1. Materials
2.2. Methods
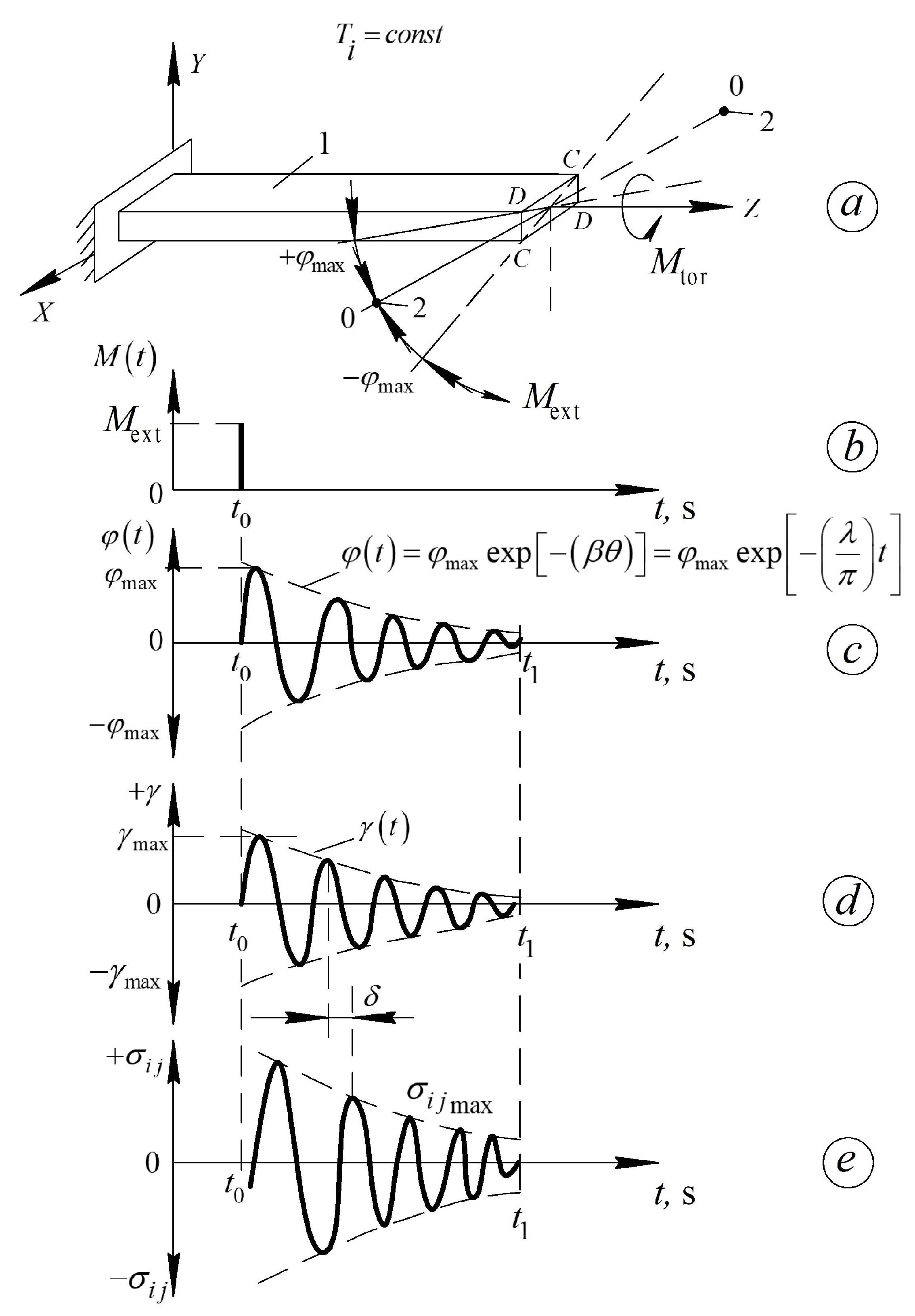
- The tested sample 1 (Figure 3a) is rigidly fixed in an immovable clamp, which, together with the sample, is placed in the thermocryochamber of the device. The research can be conducted either in an isothermal mode () or with a constant rate of temperature change .
- For , the external torque is removed, i.e., (Figure 3b), and the tested sample 1 (Figure 3a) begins to undergo a free damped torsional oscillation process around the longitudinal axis Z (Figure 3c). The presence of internal friction in the material of the tested sample leads to a decrease in the amplitude of the oscillatory process in all subsequent periods of this oscillation (Figure 3c). The envelope curve (dashed line in Figure 3c–e) is described by an equation, where is the logarithmic decrement of this process, characterizing the rate of damping, and, consequently, the internal friction, i.e., the dissipation (conversion) of part of the energy from the external impact (after the sample is brought into a nonequilibrium mechanical and thermodynamic state—presence of the angle в at time into thermal energy irreversibly dissipated within the volume of the tested sample).
- Changes in temperature and the subsequent equivalent impact on the tested sample lead to changes in the rate of the oscillatory process and, consequently, in the logarithmic decrement , the period of oscillations, and the frequency of oscillations. This allows the construction of experimental curves for the internal friction spectra and the temperature dependence of the frequency .
- The torsional oscillations arising in the tested sample induce shear strain across the cross-section of the sample (Figure 3d), which is in phase with the twisting angle throughout the entire time span of the oscillatory process (Figure 3c,d). Just like the logarithmic decrement , the period of oscillations, and the frequency of oscillations, the shear strain also depends on temperature.
- The occurrence of deformations across the sample’s cross-section is caused by shear stresses in the tested sample. A phase shift arises between the deformation and the stress which, like the logarithmic decrement , the period of oscillations, and the frequency of oscillations, depends on temperature. This phase shift angle characterizes the degree of dissipation of part of the external energy within the volume of the tested system and defines the relationship between the frequency of oscillations and the complex modulus of elasticity of the sample material in the tested system. Specifically, it determines the connection between the loss modulus (logarithmic decrement )—the imaginary part of the complex shear modulus—and the shear modulus —the real part of the complex shear modulus. This, in turn, allows for the calculation of the shear modulus defect for each local dissipative process detected in the spectrum and the temperature dependence of the frequency of the oscillatory process over the entire temperature range of the study.
3. Results and Discussion
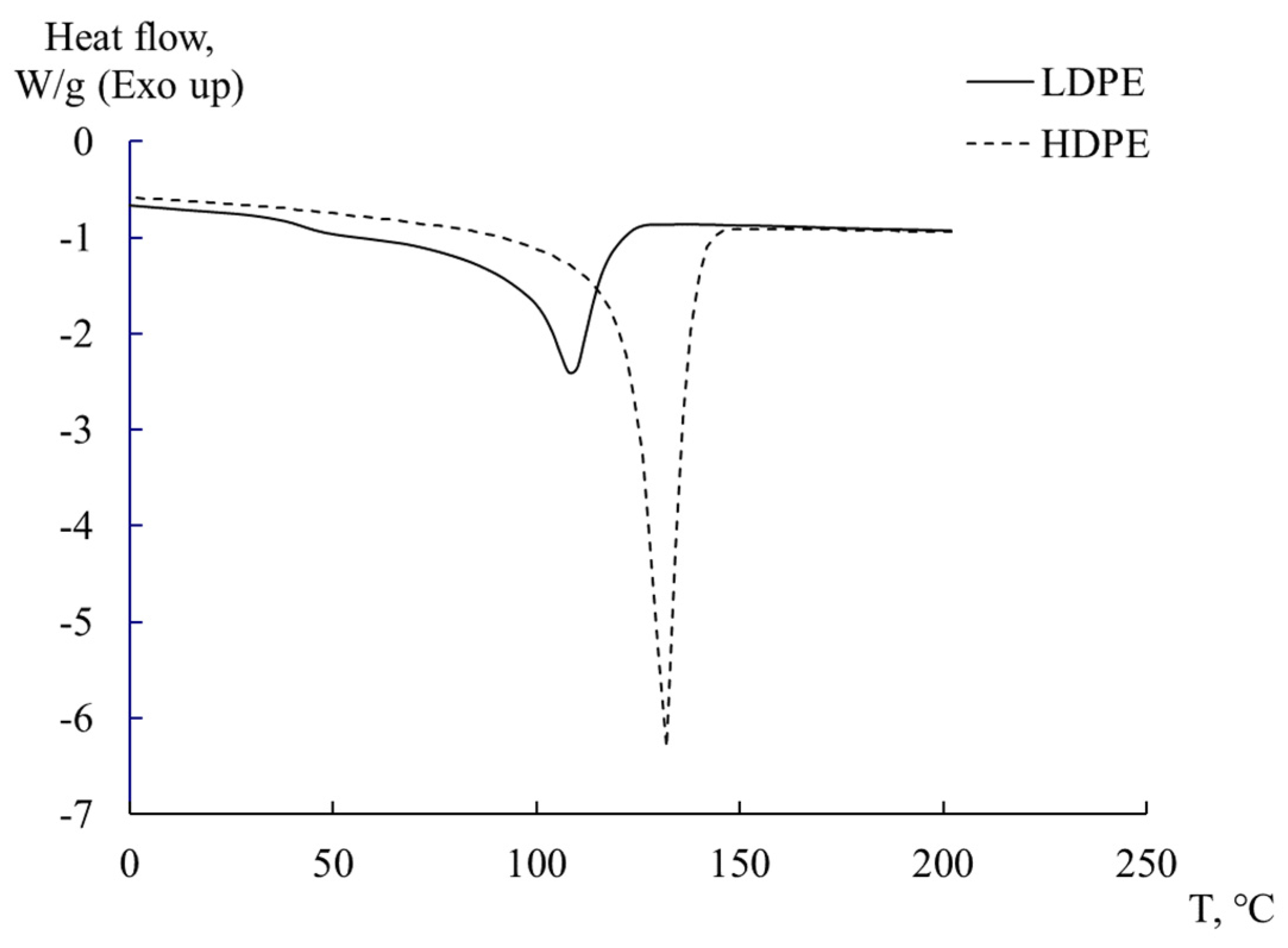
3.1. Investigation Using Differential Scanning Calorimetry (DSC)
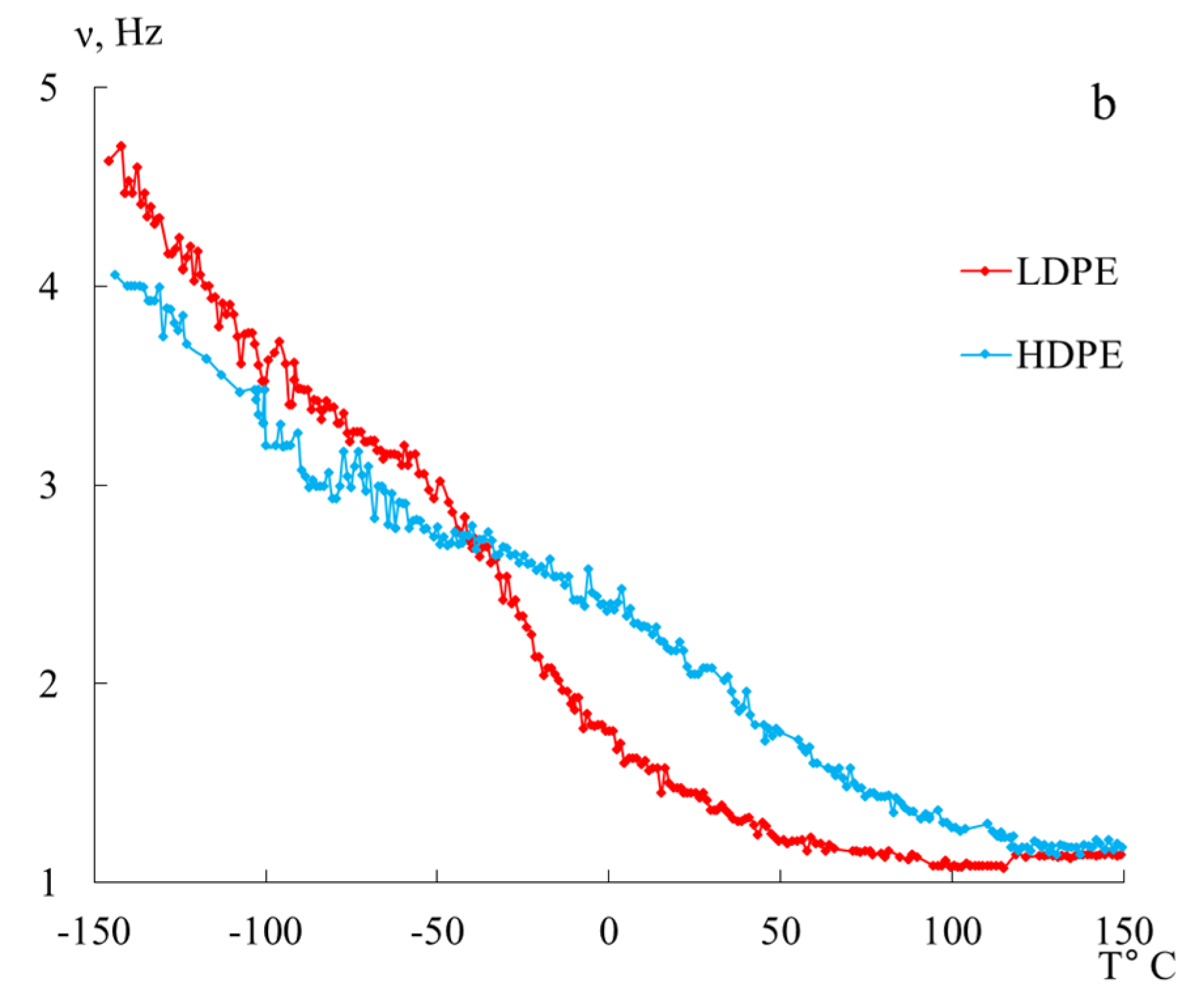
3.2. Investigation Using Relaxation Spectroscopy
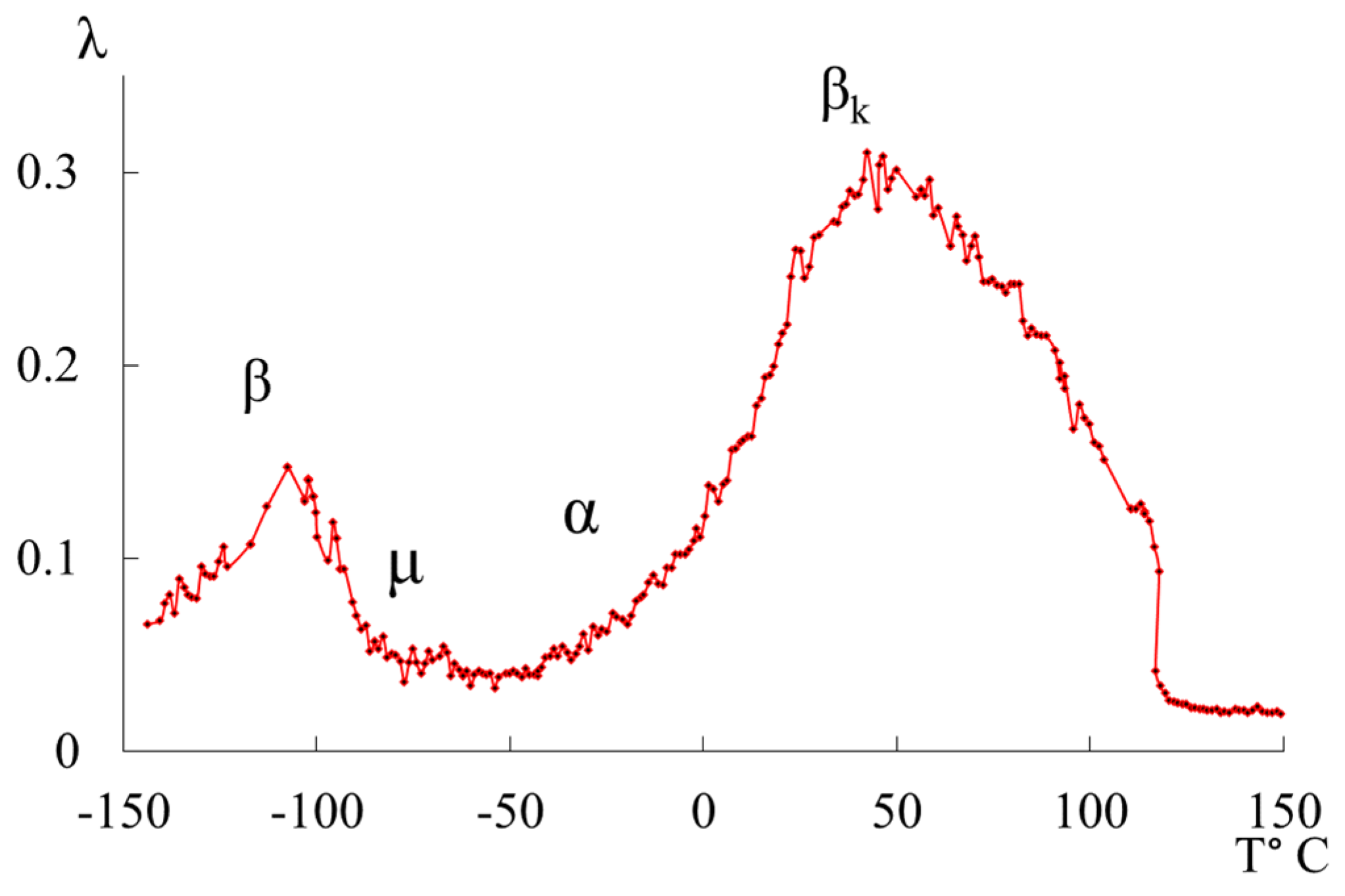
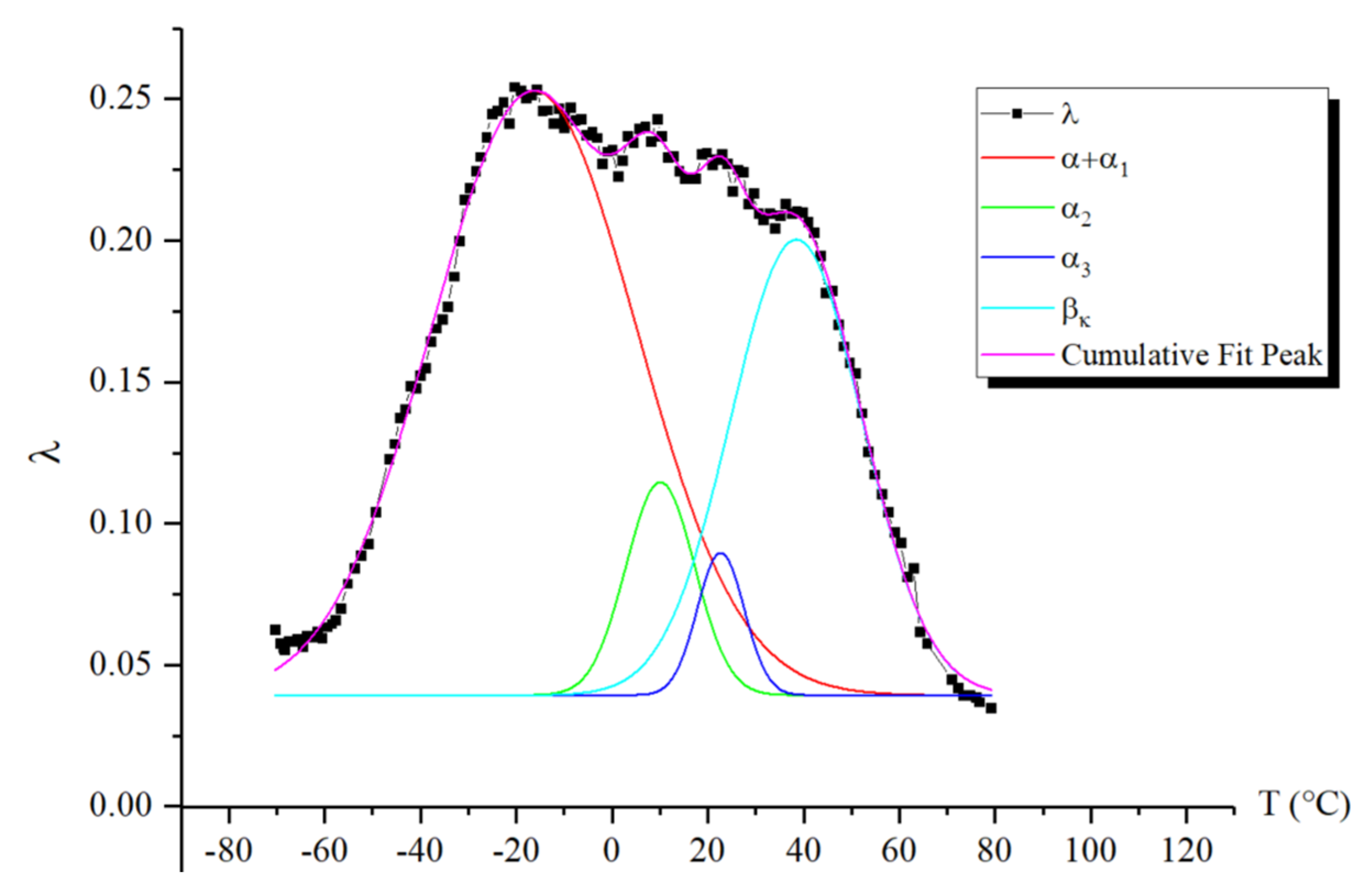
3.2.1. Segmental Mobility of Polyethylene (-Relaxation Process)
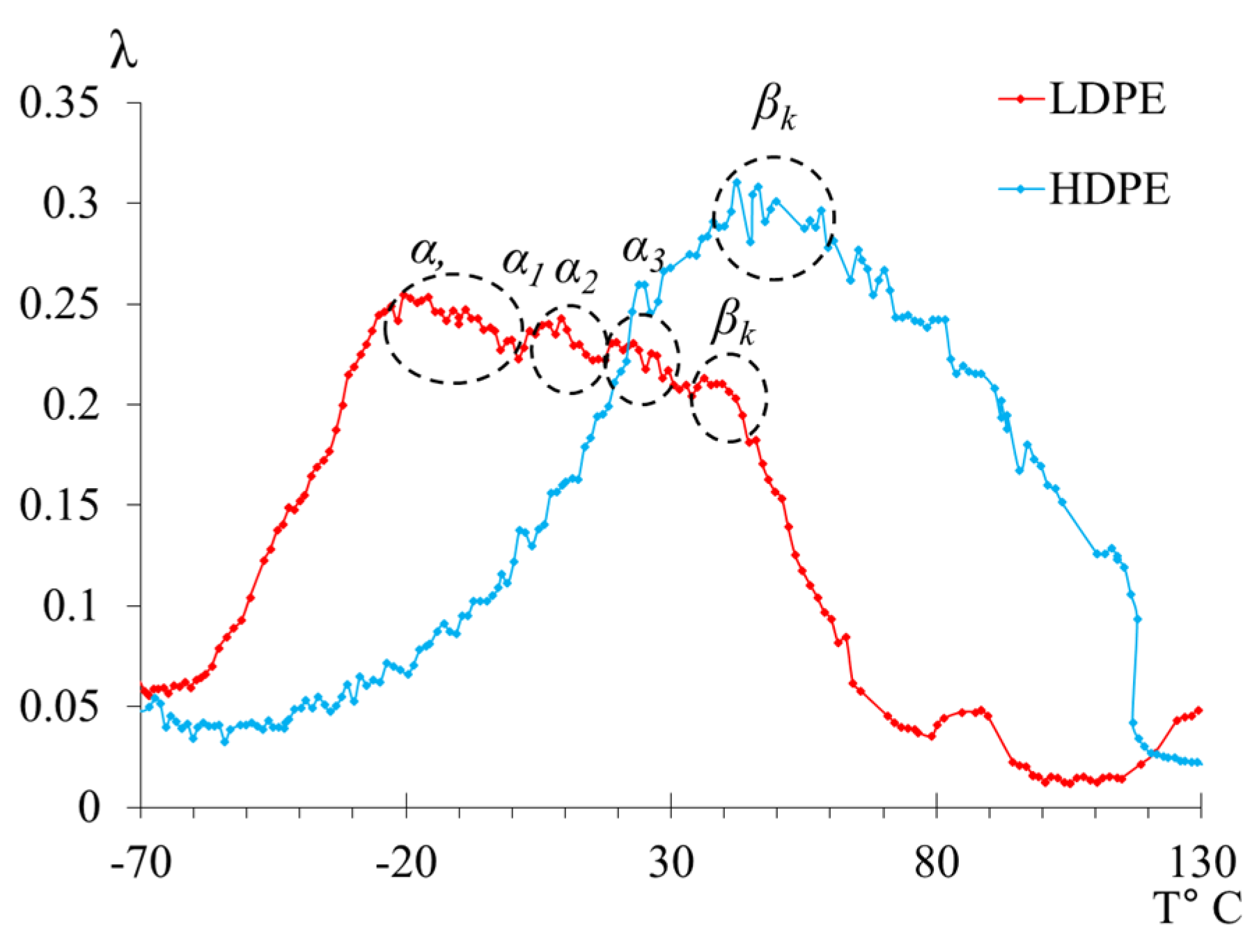
3.2.2. High-Temperature Transition (-Relaxation Process)
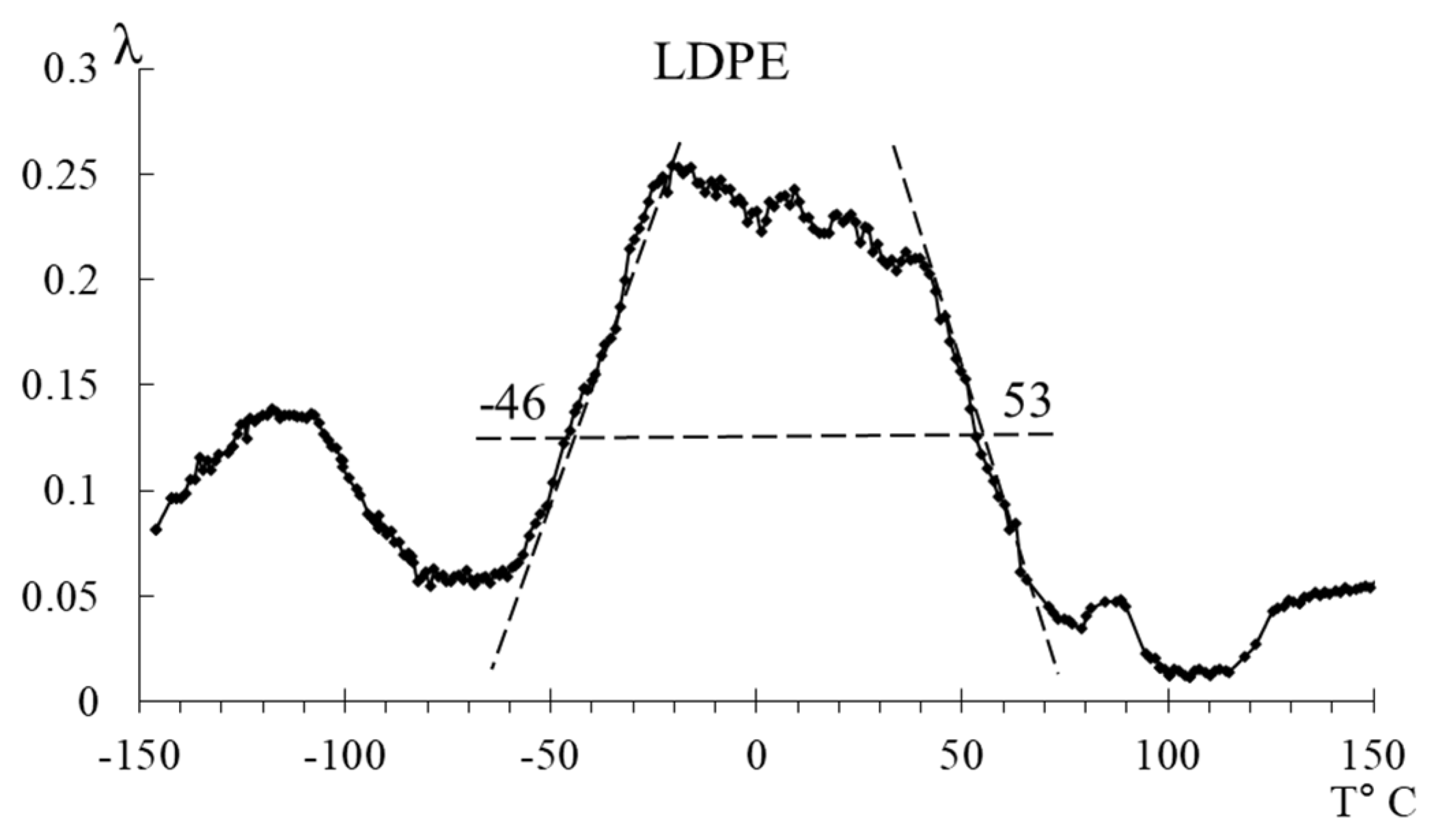
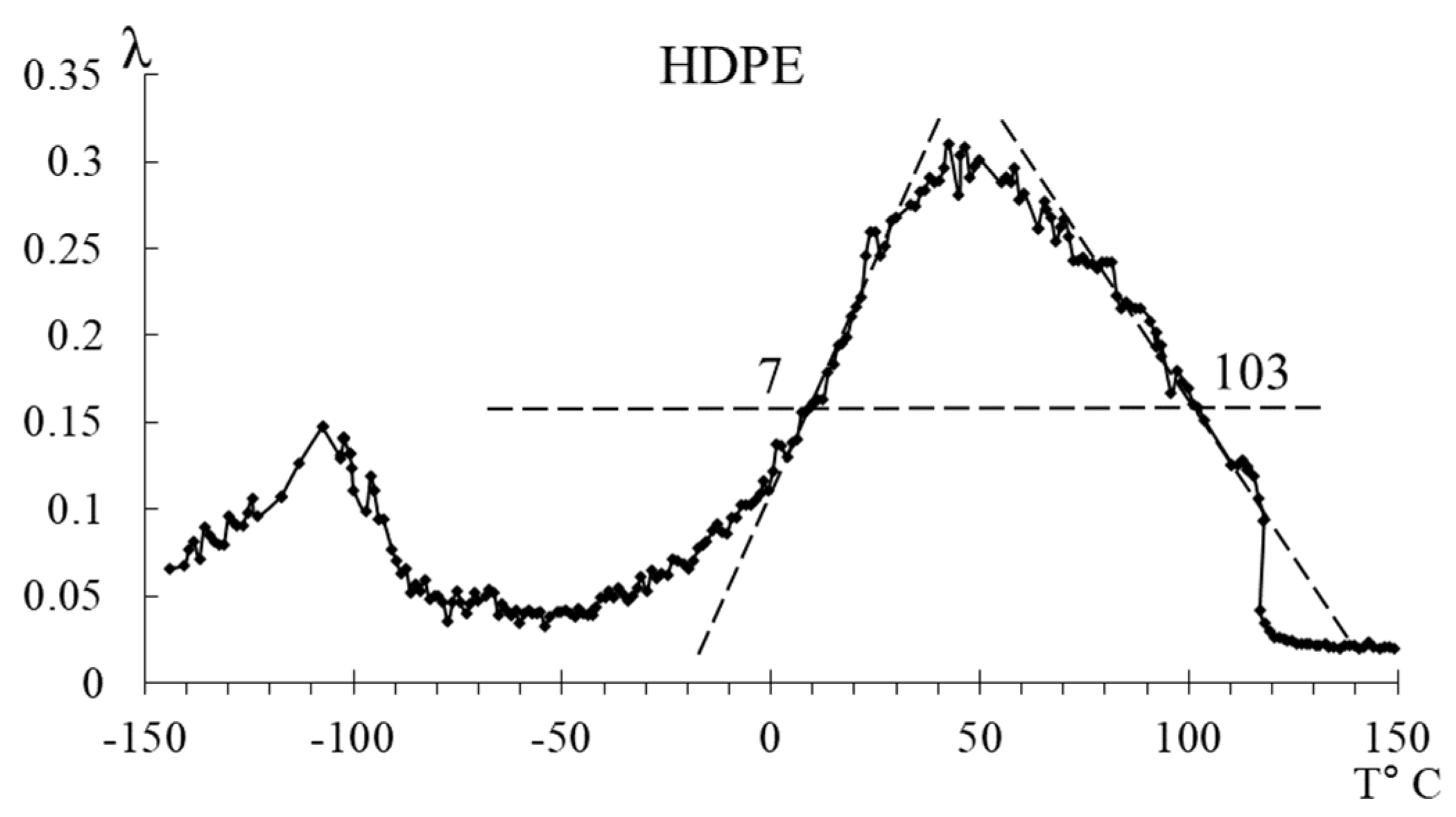
3.2.3. The Width of the Peaks of Dissipative Losses
4. Conclusions
- It has been established that the internal friction spectra may exhibit either two or three intense local dissipative processes, depending on the density of the polyethylene system under investigation (HDPE and LDPE), as well as several weakly intense dissipative processes located in different temperature ranges of this spectrum. It has been demonstrated that these loss peaks, in turn, represent a combination of superimposed dissipative processes, which is manifested in the splitting of these loss peaks into their components.
- It has been demonstrated that the temperature dependence of the frequency of the oscillatory process induced in the investigated sample exhibits anomalous frequency changes, allowing for the determination of the magnitude and sign of the modulus defect and the establishment of the mechanism of internal friction for each dissipative process. It has been found that LDPE has a lower capacity for elastic resistance to external influences across the entire temperature range. The most significant changes are observed in the temperature region corresponding to the manifestation of dissipative processes.
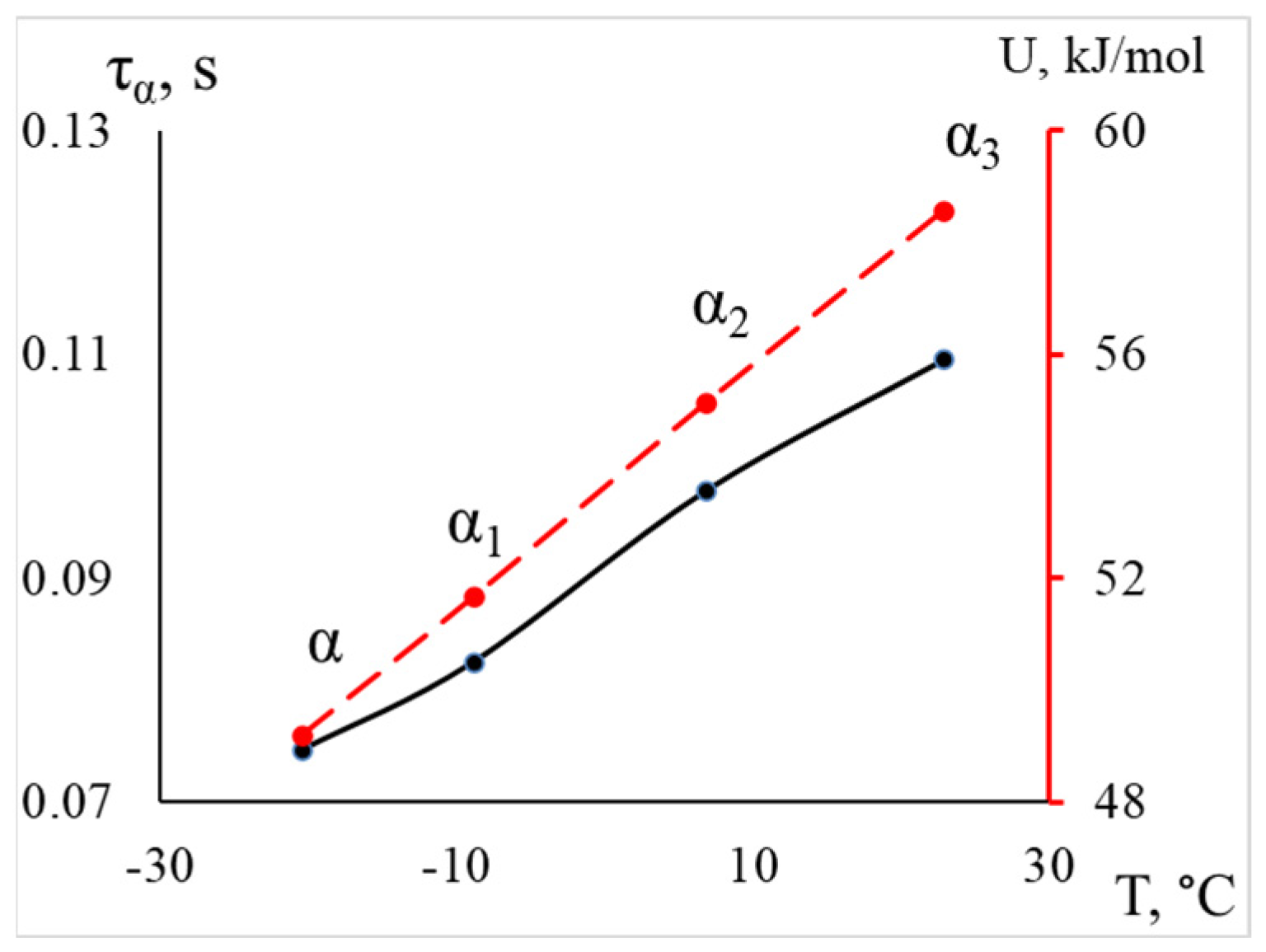
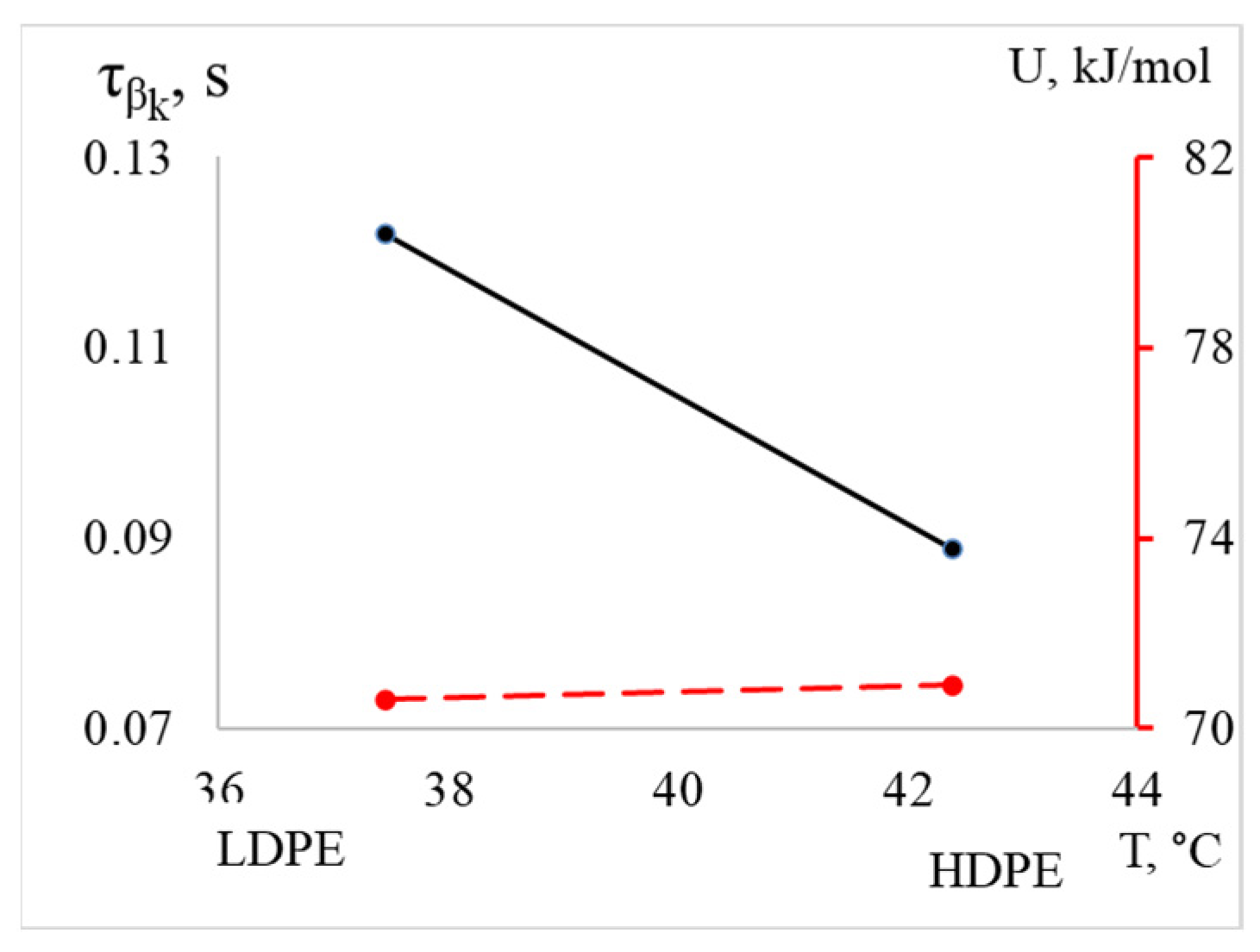
- In the internal friction spectra of LDPE in the temperature range from −50 °C to +50 °C, distinct loss peaks are clearly observed, whereas, for HDPE, these peaks are absorbed by the low-temperature branch of the process. As the temperature increases, there is an observed rise in the values of activation energy and relaxation times of these processes, which is associated with the arrangement of the responding subsystems and their topology. The relaxation microinhomogeneity in this temperature range is higher for HDPE, indicating a greater diversity of structural–kinetic subsystems responsible for the manifestation of dissipative processes within this temperature range.
- For the first time, all physical and mechanical characteristics (the defect modulus characterizing the region of local inelasticity under each peak of dissipative losses; the intensity of dissipative losses; the width of the temperature interval; and the temperature of the maximum value of the loss peak) as well as the physicochemical characteristics (activation energy, discrete relaxation time, and degree of relaxation microinhomogeneity) have been calculated based on experimentally obtained dependencies of internal friction spectra and temperature–frequency dependencies.
- It has been established that the nature of the occurrence of each of the high-intensity local dissipative processes observed in the internal friction spectrum is relaxation-based.
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Paulik, C.; Spiegel, G.; Jeremic, D. Bimodal Polyethylene: Controlling Polymer Properties by Molecular Design. In Multimodal Polymers with Supported Catalysts; Springer International Publishing: Cham, Switzerland, 2019; pp. 243–265. [Google Scholar]
- Salakhov, I.I.; Chalykh, A.E.; Shaidullin, N.M.; Shapagin, A.V.; Budylin, N.Y.; Khasbiullin, R.R.; Nifant’ev, I.E.; Gerasimov, V.K. Phase Equilibria and Interdiffusion in Bimodal High-Density Polyethylene (HDPE) and Linear Low-Density Polyethylene (LLDPE) Based Compositions. Polymers 2021, 13, 811. [Google Scholar] [CrossRef] [PubMed]
- Jeremic, D. Polyethylene. In Ullmann’s Encyclopedia of Industrial Chemistry; Wiley-VCH Verlag GmbH & Co. KGaA: Weinheim, Germany, 2014; pp. 1–42. [Google Scholar] [CrossRef]
- Ney Zo Technological and Performance Properties of Nanomodified Polyethylene Dis. Ph.D. Tech. Sciences: 05.17.06.—Russian Chemical-Technological University Named After D.I. Mendeleev 2017, 163. Available online: https://www.dissercat.com/content/tekhnologicheskie-i-ekspluatatsionnye-svoistva-nanomodifitsirovannogo-polietilena (accessed on 10 June 2023). (In Russian).
- Typology of Low-Density Polyethylene. Available online: https://studbooks.net/1965247/tovarovedenie/tipologiya_polietilena_nizkoy_plotnosti (accessed on 3 November 2023). (In Russian).
- Kireev, V.V. High Molecular Compounds. In 2 Parts. Textbook; Yurait: Moscow, Russia, 2016; p. 336. (In Russian) [Google Scholar]
- Preparation, Properties and Applications of Polyethylene. Available online: https://studentopedia.ru/tovarovedenie/poluchenie--svojstva-i-oblasti-primeneniya-polietilena---usovershenstvovanie-tehnologii-polucheniya.html (accessed on 3 November 2023). (In Russian).
- Askadsky, A.A.; Popova, M.N.; Kondrashchenko, V.I. Physico-Chemistry of Polymer Materials and Methods of Their Research: Educational Publication; Askadsky, A.A., Ed.; Publishing House ASV: Moscow, Russia, 2015; p. 408. (In Russian) [Google Scholar]
- Lomovskoy, V.A. Physico-chemical mechanics and internal friction as a theoretical and experimental basis for the synthesis of new materials. In XX Mendeleev Congress on General and Applied Chemistry; Ural Branch of the Russian Academy of Sciences: Ekaterinburg, Russia, 2016; p. 248. (In Russian) [Google Scholar]
- Lomovskoy, V.A.; Shatokhina, S.A.; Chalykh, A.E.; Matveev, V.V. Spectra of Internal Friction in Polyethylene. Polymers 2022, 14, 675. [Google Scholar] [CrossRef] [PubMed]
- Li, K.; Xiang, D.; Lei, X. Green and Self-lubricating Polyoxymethylene Composites Filled with Low-density Polyethylene and Rice Husk Flour. J. Appl. Polym. Sci. 2008, 108, 2778–2786. [Google Scholar] [CrossRef]
- Brown, N.; Ward, I.M. The Influence of Morphology and Molecular Weight on Ductile-Brittle Transitions in Linear Polyethylene. J. Mater. Sci. 1983, 18, 1405–1420. [Google Scholar] [CrossRef]
- Lu, X.; Qian, R.; Brown, N. The Effect of Crystallinity on Fracture and Yielding of Polyethylenes. Polymer 1995, 36, 4239–4244. [Google Scholar] [CrossRef]
- Kitao, K. A Study of Brittle-ductile Transition in Polyethylene. Polym. Eng. Sci. 1997, 37, 777–788. [Google Scholar] [CrossRef]
- Bershtein, V.A.; Yegorov, V.M.; Marikhin, V.A.; Myasnikova, L.P. Specific Features of Molecular Motion in Lamellar Polyethylene between 100 and 400 K. Polym. Sci. USSR 1985, 27, 864–874. [Google Scholar] [CrossRef]
- Handbook of Thermoplastics; Olabisi, O., Adewale, K., Eds.; CRC Press: Boca Raton, FL, USA, 2016; ISBN 9780429101625. [Google Scholar]
- Shalyapin, S.V.; Gvozdev, I.V.; Simonov-Emel’yanov, I.D. A Study of Stress Relaxation Processes in Crosslinked Polyethylene. Int. Polym. Sci. Technol. 2018, 41, 19–23. [Google Scholar] [CrossRef]
- Rebinder, P.A. Surface Phenomena in Disperse Systems. Physical and Chemical Mechanics; Selected Works; Science: Moscow, Russia, 1979; p. 384. (In Russian) [Google Scholar]
- Salakhov, I.I.; Shaidullin, N.M.; Chalykh, A.E.; Matsko, M.A.; Shapagin, A.V.; Batyrshin, A.Z.; Shandryuk, G.A.; Nifant’ev, I.E. Low-Temperature Mechanical Properties of High-Density and Low-Density Polyethylene and Their Blends. Polymers 2021, 13, 1821. [Google Scholar] [CrossRef]
- Patel, R.M. Polyethylene. In Multilayer Flexible Packaging; Elsevier: Amsterdam, The Netherlands, 2016; pp. 17–34. [Google Scholar]
- Lomovskoy, V.A.; Chugunov, Y.V.; Shatokhina, S.A. Methodology for the Study of Internal Friction in the Mode of Free Damped Oscillatory Process (Part 1). Nauchnoe Priborostr. Sci. Instrum. 2023, 33, 60–71. [Google Scholar]
- Lomovskoy, V.A.; Chugunov, Y.V.; Shatokhina, S.A. Methodology for the Study of Internal Friction in the Mode of Free Damped Oscillatory Process. Part 2. Theoretical Analysis of Experimental Results. Nauchnoe Priborostr. Sci. Instrum. 2024, 34, 3–18. [Google Scholar]
- Bartenev, G.M.; Barteneva, A.G. Relaxation Properties of Polymers; Chemistry: Moscow, Russia, 1992; p. 384. (In Russian) [Google Scholar]
- Lomovskoy, V.A. Problems of structure formation in dispersed systems. In Scientific Publication Modern Problems of Physical Chemistry; Ed. House “Border”: Moscow, Russia, 2005; pp. 193–209. (In Russian) [Google Scholar]
- Lomovskoy, V.A. Internal Friction Spectra and Dissipative Mobility of Elements of Aggregate and Modifying Subsystems. Mater. Sci. 2007, 2, 3–10. Available online: https://www.elibrary.ru/item.asp?id=12533415 (accessed on 10 June 2024). (In Russian).
- Shut, N.I.; Bartenev, G.M.; Kaspersky, A.V. Relaxation Transitions in Polyethylene According to Structural and Mechanical Relaxation Data. Acta Polym. 1989, 40, 529–532. [Google Scholar] [CrossRef]
- Bartenev, G.M.; Aliguliyev, R.M. Relaxational Spectrometry of Low Density Polyethylene. Polym. Sci. USSR 1982, 24, 2106–2114. [Google Scholar] [CrossRef]
- Bugaev, N.M.; Gorshkov, A.A.; Lomovskoy, V.A.; Fomkina, Z.I. Nature and possible mechanisms of dissipative loss background on internal friction spectra of amorphous polymeric materials. In Abstracts of the XXVI International Conference “Mathematical and Computer Modeling in Mechanics of Deformable Media and Constructions”; “Aysing” FARMindex Publishing House: Saint-Petersburg, Russia, 2015; pp. 346–348. (In Russian) [Google Scholar]
- Basset, D.C. Principles of Polymer Morphology; Cambridge University Press: New York, NY, USA, 1981; p. 251. [Google Scholar]
- Lebedev, I.I. “Molecular Mobility in Surface Nanolayers of Polymers: PhD Dissertation in Physical and Mathematical Sciences: 01.04.07.”—Ioffe Physical Technical Institute, RAS, 2011; 191p. Available online: https://www.dissercat.com/content/molekulyarnaya-podvizhnost-v-pripoverkhnostnykh-nanosloyakh-polimerov (accessed on 10 June 2023).
- Askadsky, A.A. Deformation of Polymers; Chemistry: Moscow, Russia, 1973; p. 440. (In Russian) [Google Scholar]
- Askadsky, A.A.; Khokhlov, A.R. Introduction to the Physical Chemistry of Polymers; Scientific World: Moscow, Russia, 2009; p. 380. (In Russian) [Google Scholar]
- Kerber, M.L.; Bukanov, A.M.; Wolfson, S.I.; Gorbunova, I.Y.; Kandyrin, L.B.; Sirota, A.G.; Sheryshev, M.A. Physical and Chemical Processes in Polymer Processing; Scientific Foundations and Technologies: St. Petersburg, Russia, 2013; p. 320. (In Russian) [Google Scholar]
- Shevchenko, A.A. Physical Chemistry and Mechanics of Composite Materials: A Textbook for Universities; CSB “Profession”: St. Petersburg, Russia, 2010; p. 224. (In Russian) [Google Scholar]
- Askadskii, A.A. Lectures on the Physico-Chemistry of Polymers; Nova Science Pub Inc: New York, NY, USA, 2003. [Google Scholar]
- Tager, A.A. Physical Chemistry of Polymers; Scientific World: Moscow, Russia, 2007; p. 573. (In Russian) [Google Scholar]
- Blanter, M.S.; Golovin, I.S.; Neuhäuser, H.; Sinning, H.-R. Introduction to Internal Friction: Terms and Definitions. In Internal Friction in Metallic Materials; Springer: Berlin/Heidelberg, Germany, 2007; Volume 90, pp. 1–10. ISBN 978-3-540-68757-3. [Google Scholar]
- Zinoviev, P.A.; Ermakov, Y.N. Energy Dissipation in Composite Materials; Routledge: New York, NY, USA, 2018; ISBN 9780203757529. [Google Scholar]
- Lomovskoy, V.A.; Mazurina, S.A.; Simonov-Emel’yanov, I.D.; Kiselev, M.R.; Konstantinov, N.Y. Relaxation Spectroscopy of Polyethylenes of Different Molecular Weight. Inorg. Mater. Appl. Res. 2019, 10, 174–183. [Google Scholar] [CrossRef]
- Tarago, S.; Calleja, D.; Guzman, G.M. Anales de Física/Serie B. Aplicaciones Métodos Instrumentos 1986, 82, 26–34. [Google Scholar]
- Khanna Yash, P.; Turi Edith, A.; Taylor Thomas, J.; Vickroy Virgil, V.; Abbott Richard, F. Dynamic Mechanical Relaxations in Polyethylene. Macromolecules 1985, 18, 1302–1309. [Google Scholar] [CrossRef]
- Bartenev, G.M.; Aliguliyev, R.M.; Khiteyeva, D.M. Relaxation Transitions in Polyethylene. Polym. Sci. USSR 1981, 23, 2183–2193. [Google Scholar] [CrossRef]
- Andrianova, G.P. Physicochemistry of Polyolefins; Chemistry: Moscow, Russia, 1974. (In Russian) [Google Scholar]
- Semchikov, Y.D. Macromolecular Compounds: Textbook. For Students Institutions of Higher Education Prof. Education, 5th ed.; Semchikov, Y.D., Ed.; Publishing Center “Academy”: Moscow, Russia, 2010; p. 368. (In Russian) [Google Scholar]
- Lomovskoj, V.A.; Abaturova, N.A.; Barteneva, A.G.; Galushko, T.B.; Lomovskaja, N.J.; Sakov, D.M.; Saunin, E.I.; Khlebnikova, O.A. Metering Converter 2015. Available online: https://yandex.ru/patents/doc/RU2568963C1_20151120 (accessed on 10 July 2024).
- Lomovskoy, V.A. The Device for Researching of Local Dissipative Processes in Solid Materials of Various Chemical Origin, Composition and Structure. Nauchnoe Priborostr. 2019, 29, 033–046. [Google Scholar] [CrossRef]
- Lomovskoy, V.A.; Shatokhina, S.A.; Simonov-Emel’yanov, I.D. Phenomenological Description of the “Structure–Property” Relation for Epoxy Oligomer Hardeners on the Basis of Internal Friction Spectra. Colloid J. 2024, 86, 418–430. [Google Scholar] [CrossRef]
- Bershtein, V.A.; Egorov, V.M. Differential Scanning Calorimetry in the Physical Chemistry of Polymers; Chemistry: Leningrad, Russia, 1990; p. 256. (In Russian) [Google Scholar]
- Sitnikova, V.E. Methods of Thermal Analysis. Workshop; Sitnikova, V.E., Ponomareva, A.A., Uspenskaya, M.V., Eds.; ITMO University: St. Petersburg, Russia, 2021; p. 152. (In Russian) [Google Scholar]
- Marikhin, V.A.; Bershtein, V.A.; Egorov, V.M.; Myasnikova, L.P. Thermodynamic Characteristics of Lamellas and Their Surfaces in Bulk Polyethylene According from Differential Scanning Calorimetry Data. Polym. Sci. USSR 1986, 28, 2207–2215. [Google Scholar] [CrossRef]



| PE Grade | LDPE 10803-020 | HDPE 277-73 |
|---|---|---|
| Density at 23 °C, g/cm3 | 0.920 | 0.957 |
| Melt Flow Rate, g/10 min 190 °C/5.0 kg 190 °C/2.16 kg | ||
| - | 17.0–25.0 | |
| 2 | - | |
| DSC Melting Point, °C | 105 | 131 |
| ∆Hm, J/g | 73.5 | 157.1 |
| Degree of crystallinity, χ, % | 25 | 54 |
| PE Grade | Tmax (K) | Tmax (°C) | U, kJ/mol | Nature of Process | |||
|---|---|---|---|---|---|---|---|
| -process | Chain unit Relaxation mechanism of internal friction | ||||||
| LDPE | 157 | −116 | 0.134 | 3.94 | 34.3 | 0.040 | |
| HDPE | 165 | −107 | 0.148 | 3.47 | 36.3 | 0.046 | |
| -process | Chain segment Relaxation mechanism of internal friction | ||||||
| LDPE | 253 | −20 | 0.254 | 2.13 | 49.2 | 0.075 | |
| HDPE | - | - | - | - | - | - | |
| -process | |||||||
| LDPE | 264 | −9 | 0.247 | 1.93 | 51.7 | 0.083 | |
| HDPE | - | - | - | - | - | - | |
| -process | |||||||
| LDPE | 280 | 7 | 0.240 | 1.63 | 55.1 | 0.098 | |
| HDPE | - | - | - | - | - | - | |
| -process | |||||||
| LDPE | 296 | 23 | 0.231 | 1.45 | 58.6 | 0.110 | |
| HDPE | - | - | - | - | - | - | |
| -process | Passing chains + oscillations of chain sections in the crystalline phase Relaxation mechanism of internal friction | ||||||
| LDPE | 310 | 37 | 0.210 | 1.31 | 70.6 | 0.122 | |
| HDPE | 315 | 42 | 0.310 | 1.79 | 70.9 | 0.089 | |
| PE Grade | Tmax (°C) | The Range of Frequency Variation, Hz. | Shear Modulus Defect | ||
|---|---|---|---|---|---|
| -process | |||||
| LDPE | −146 | −93 | 4.63 | 3.41 | 0.460 |
| HDPE | −144 | −85 | 4.06 | 2.99 | 0.455 |
| -process | |||||
| LDPE | −60 | 22 | 3.15 | 1.13 | 0.871 |
| HDPE | −26 | 97 | 2.69 | 1.18 | 0.809 |
| PE Grade | Tmax (°C) | ΔT, °C | ||||
|---|---|---|---|---|---|---|
| LDPE | −46 | 53 | 13.344 | 0.033 | 13.331 | 99 |
| HDPE | 7 | 103 | 84.334 | 0.001 | 84.333 | 96 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lomovskoy, V.A.; Shatokhina, S.A. Relaxation Phenomena in Low-Density and High-Density Polyethylene. Polymers 2024, 16, 3510. https://doi.org/10.3390/polym16243510
Lomovskoy VA, Shatokhina SA. Relaxation Phenomena in Low-Density and High-Density Polyethylene. Polymers. 2024; 16(24):3510. https://doi.org/10.3390/polym16243510
Chicago/Turabian StyleLomovskoy, Viktor A., and Svetlana A. Shatokhina. 2024. "Relaxation Phenomena in Low-Density and High-Density Polyethylene" Polymers 16, no. 24: 3510. https://doi.org/10.3390/polym16243510
APA StyleLomovskoy, V. A., & Shatokhina, S. A. (2024). Relaxation Phenomena in Low-Density and High-Density Polyethylene. Polymers, 16(24), 3510. https://doi.org/10.3390/polym16243510







