Birefringence in Injection-Molded Cyclic Olefin Copolymer Substrates and Its Impact on Integrated Photonic Structures
Abstract
1. Introduction
2. Materials and Methods
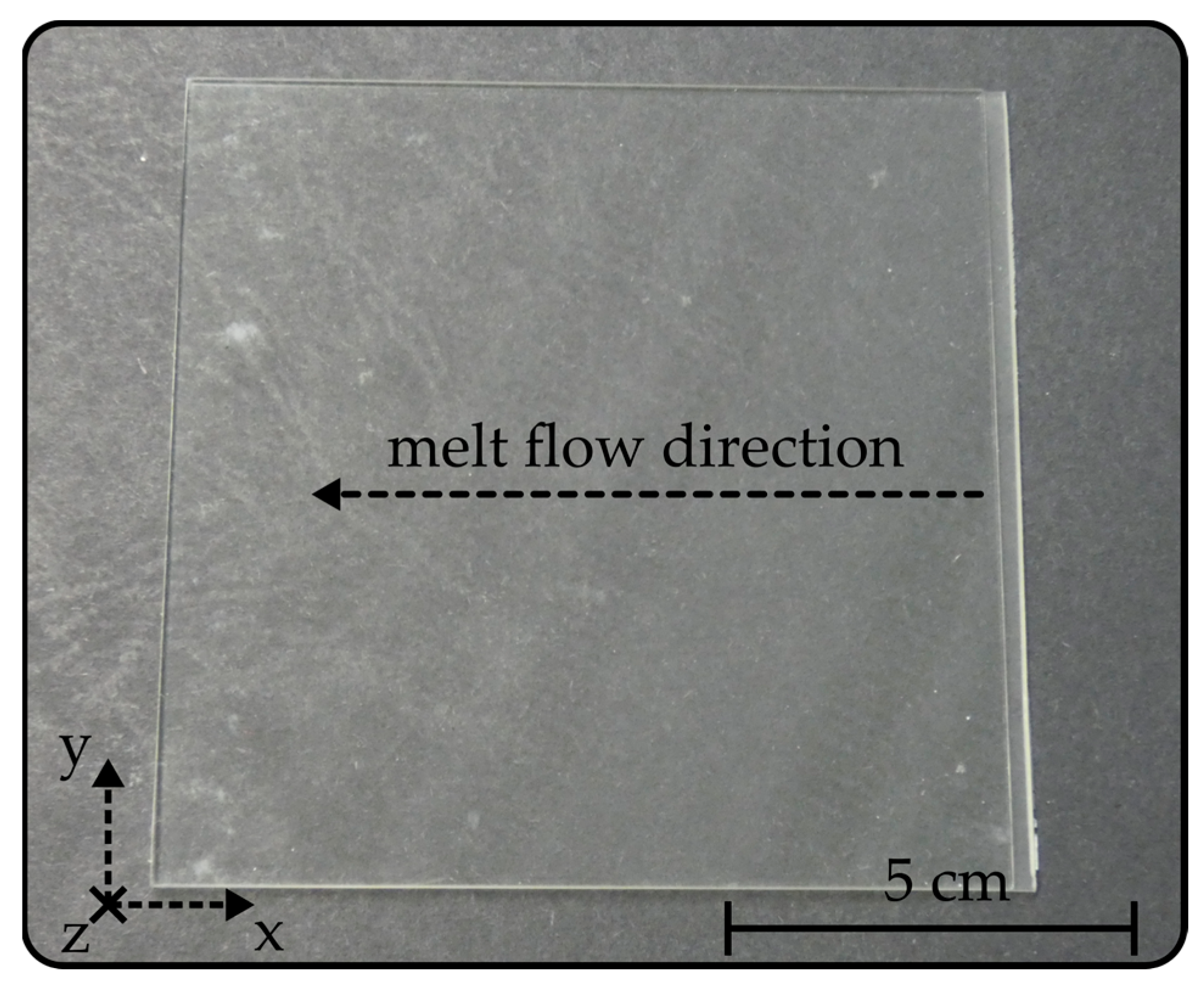
2.1. Injection-Molded Cyclic Olefin Copolymer and Sample Preparation
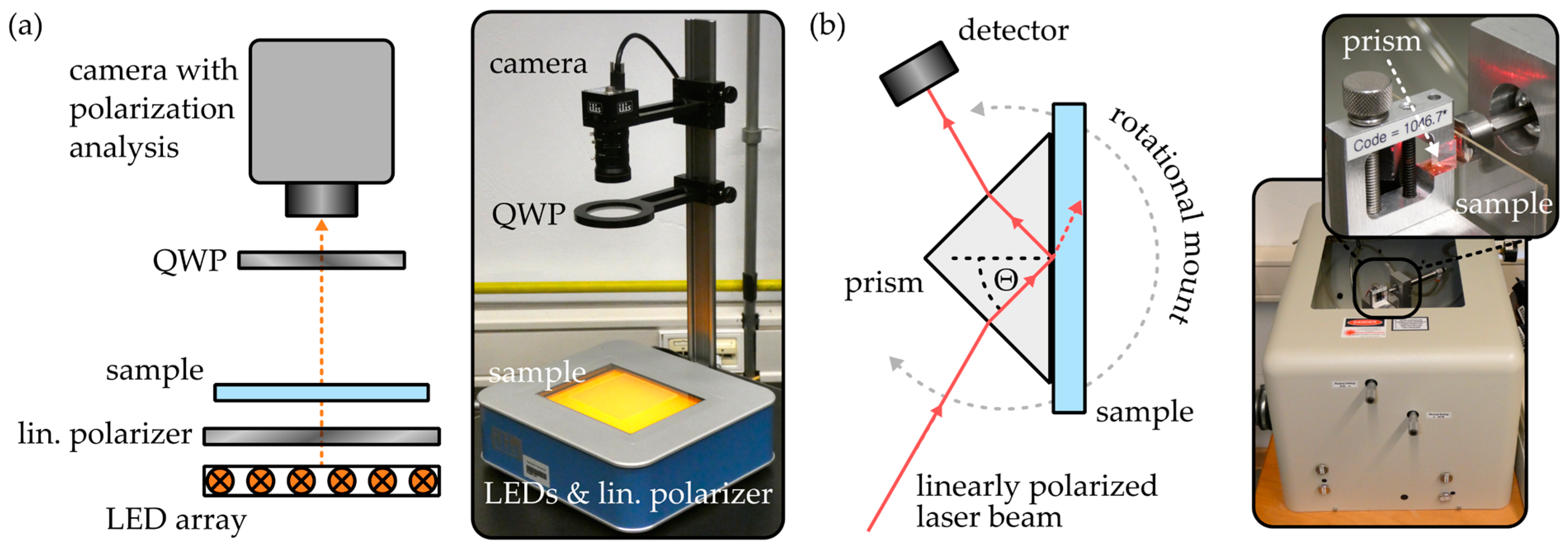
2.2. Birefringence Characterization
2.3. Mechanical Characterization
2.4. Integrated Photonic Structures
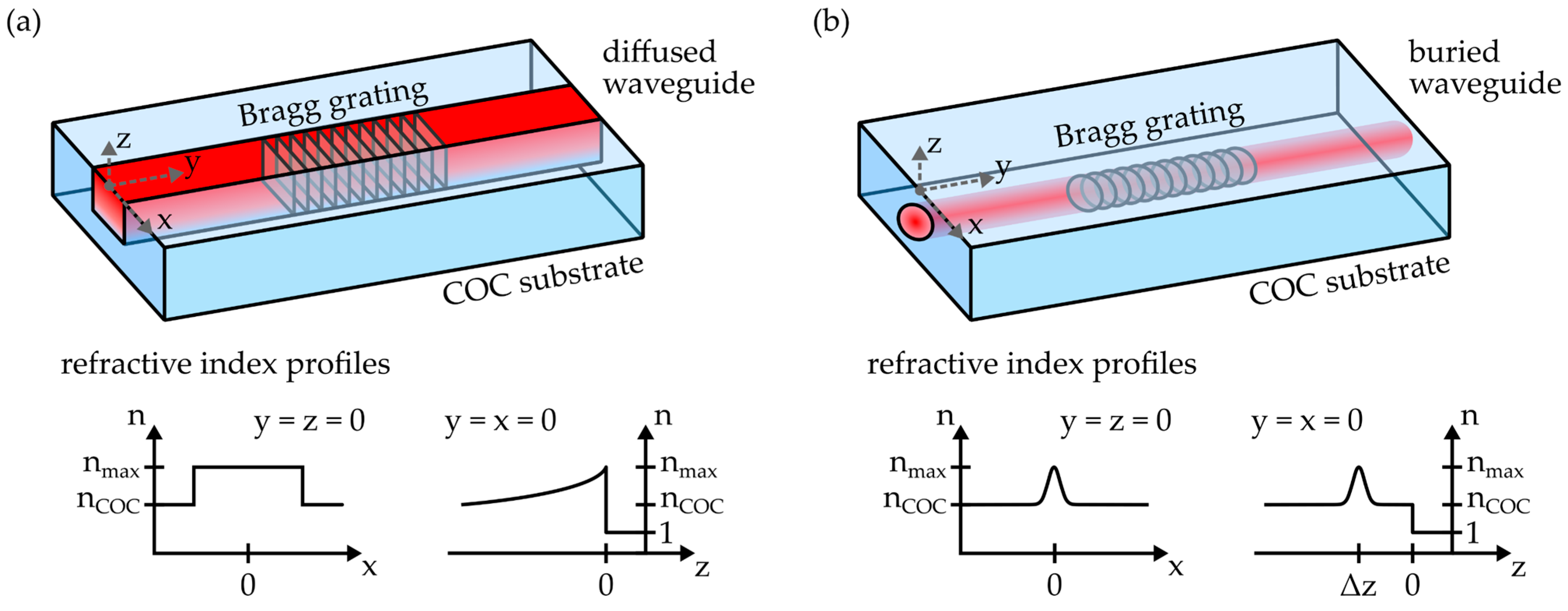
2.4.1. Fabrication and Working Principle
2.4.2. Analysis of Integrated Waveguides and Bragg Gratings
3. Results and Discussion
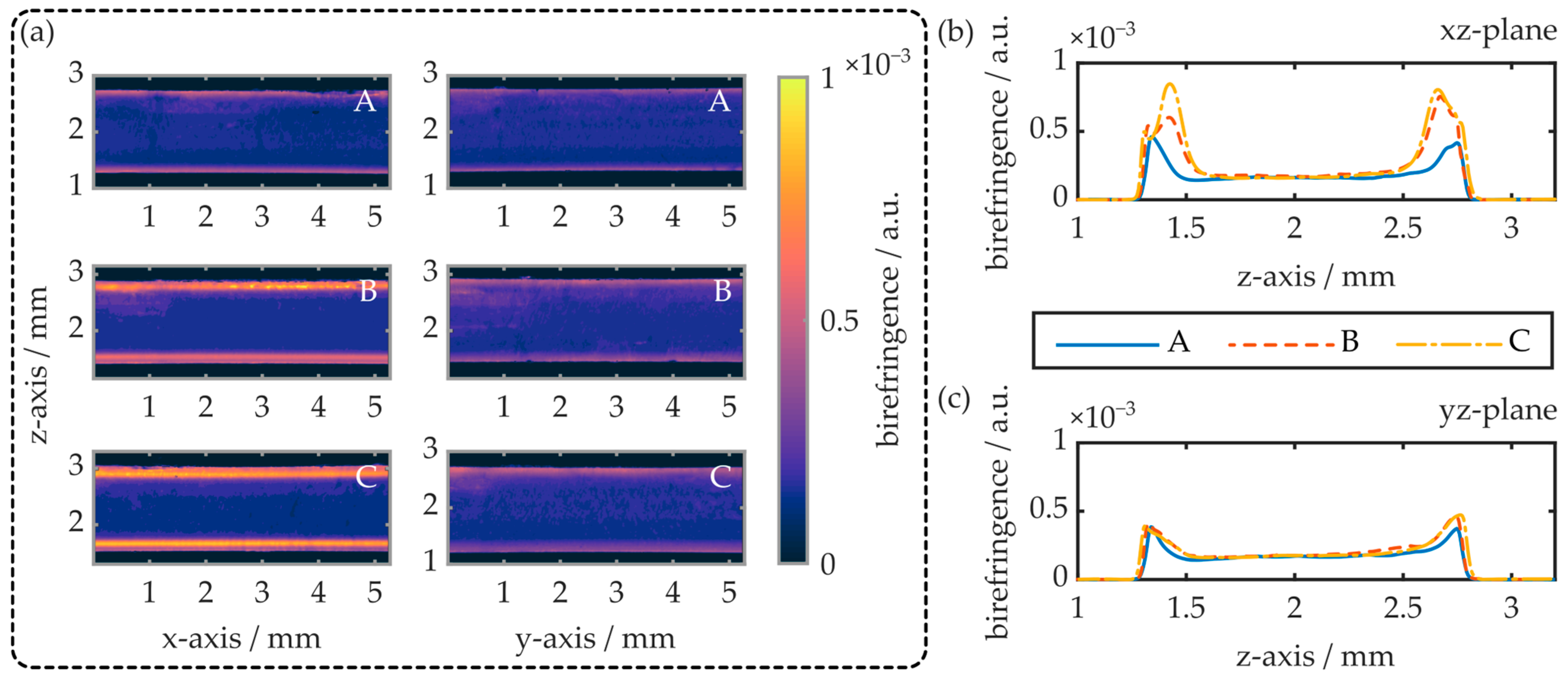
3.1. Analysis of Three-Dimensional Birefringence
3.2. Impact on Mechanical Properties
3.3. Impact on the Properties of Near-Surface Integrated Waveguides and Bragg Gratings
3.4. Buried Waveguides and Bragg Gratings
3.5. Guidelines for the Fabrication of Photonic Structures in Injection-Molded Cyclic Olefin Copolymer Substrates
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bundgaard, F.; Perozziello, G.; Geschke, O. Rapid prototyping tools and methods for all-Topas® cyclic olefin copolymer fluidic microsystems. Proc. Inst. Mech. Eng. Part C 2006, 220, 1625–1632. [Google Scholar] [CrossRef]
- Hunsperger, R.G. Integrated Optics: Theory and Technology, 6th ed.; Springer: New York, NY, USA, 2009; ISBN 9780387897745. [Google Scholar]
- Hu, J.; Li, L.; Lin, H.; Zhang, P.; Zhou, W.; Ma, Z. Flexible integrated photonics: Where materials, mechanics and optics meet. Opt. Mater. Express 2013, 3, 1313. [Google Scholar] [CrossRef]
- Ma, H.; Jen, A.-Y.; Dalton, L.R. Polymer-Based Optical Waveguides: Materials, Processing, and Devices. Adv. Mater. 2002, 14, 1339–1365. [Google Scholar] [CrossRef]
- Wypych, G. Handbook of Polymers; ChemTec Publishing: Toronto, ON, Canada, 2012; ISBN 9781895198478. [Google Scholar]
- Yuan, W.; Khan, L.; Webb, D.J.; Kalli, K.; Rasmussen, H.K.; Stefani, A.; Bang, O. Humidity insensitive TOPAS polymer fiber Bragg grating sensor. Opt. Express. 2011, 19, 19731–19739. [Google Scholar] [CrossRef] [PubMed]
- TOPAS Advanced Polymers GmbH. TOPAS®COC: Cyclic Olefin Copolymer. Available online: https://topas.com/wp-content/uploads/2023/05/TOPAS_Product-Brochure.pdf (accessed on 29 December 2023).
- Khanarian, G. Optical properties of cyclic olefin copolymers. Opt. Eng. 2001, 40, 1024–1029. [Google Scholar] [CrossRef]
- Rosenberger, M.; Kefer, S.; Girschikofsky, M.; Roth, G.-L.; Hessler, S.; Belle, S.; Schmauss, B.; Hellmann, R. High-temperature stable and sterilizable waveguide Bragg grating in planar cyclo-olefin copolymer. Opt. Lett. 2018, 43, 3321–3324. [Google Scholar] [CrossRef]
- Rosenberger, M.; Pauer, H.; Girschikofsky, M.; Woern, H.; Schmauss, B.; Hellmann, R. Flexible Polymer Shape Sensor Based on Planar Waveguide Bragg Gratings. IEEE Photon. Tech. Lett. 2016, 28, 1898–1901. [Google Scholar] [CrossRef]
- Roth, G.-L.; Kefer, S.; Hessler, S.; Esen, C.; Hellmann, R. Integration of Microfluidic and Photonic Components within Transparent Cyclic Olefin Copolymers by Using fs Laser. JLMN 2021, 16. [Google Scholar] [CrossRef]
- Kefer, S.; Bischoff, K.; Roth, G.-L.; Haubner, J.; Schmauss, B.; Hellmann, R. Tunable Bulk Polymer Planar Bragg Gratings Electrified via Femtosecond Laser Reductive Sintering of CuO Nanoparticles. Adv. Opt. Mater. 2021, 9, 2002203. [Google Scholar] [CrossRef]
- Kefer, S.; Dai, J.; Yang, M.; Schmauss, B.; Hellmann, R. Hypersensitive H2 Sensor Based on Polymer Planar Bragg Gratings Coated with Pt-Loaded WO3-SiO2. Opt. Lett. 2020, 45, 3601–3604. [Google Scholar] [CrossRef]
- Kefer, S.; Pape, D.; Roth, G.-L.; Hessler, S.; Schmauss, B.; Hellmann, R. Micromilling-assisted fabrication of monolithic polymer ridge-type waveguides with integrated photonic sensing structures. Opt. Mater. Express 2021, 11, 2389–2400. [Google Scholar] [CrossRef]
- Nunes, P.S.; Ohlsson, P.D.; Ordeig, O.; Kutter, J.P. Cyclic olefin polymers: Emerging materials for lab-on-a-chip applications. Microfluid. Nanofluid. 2010, 9, 145–161. [Google Scholar] [CrossRef]
- Kashyap, R. Fiber Bragg Gratings, 2nd ed.; Academic Press: London, UK, 2010; ISBN 978-0-12-372579-0. [Google Scholar]
- Rosenberger, M.; Koller, G.; Belle, S.; Schmauss, B.; Hellmann, R. Planar Bragg grating in bulk polymethylmethacrylate. Opt. Express. 2012, 20, 27288–27296. [Google Scholar] [CrossRef] [PubMed]
- Rosenberger, M.; Schmauss, B.; Hellmann, R. Influence of the UV dosage on planar Bragg gratings in cyclo-olefin copolymer substrates. Opt. Mater. Express 2016, 6, 2118. [Google Scholar] [CrossRef]
- Roth, G.-L.; Hessler, S.; Kefer, S.; Girschikofsky, M.; Esen, C.; Hellmann, R. Femtosecond laser inscription of waveguides and Bragg gratings in transparent cyclic olefin copolymers. Opt. Express 2020, 28, 18077–18084. [Google Scholar] [CrossRef] [PubMed]
- Rosato, D.V.; Rosato, D.V.; Rosato, M.G. Injection Molding Handbook; Springer: Boston, MA, USA, 2000; ISBN 978-1-4613-7077-2. [Google Scholar]
- Sastri, V.R. Plastics in Medical Devices: Properties, Requirements, and Applications, 2nd ed.; Elsevier Science: Burlington, MA, USA, 2013; ISBN 978-1-4557-3201-2. [Google Scholar]
- Harper, C.A. (Ed.) Modern Plastics Handbook; McGraw-Hill: New York, NY, USA, 2000; ISBN 0-07-026714-6. [Google Scholar]
- Tadmor, Z. Molecular orientation in injection molding. J. Appl. Polym. Sci. 1974, 18, 1753–1772. [Google Scholar] [CrossRef]
- Isayev, A.I. Orientation development in the injection molding of amorphous polymers. Polym. Eng. Sci. 1983, 23, 271–284. [Google Scholar] [CrossRef]
- Han, C.D.; Villamizar, C.A. Development of stress birefringence and flow patterns during mold fillin and cooling. Polym. Eng. Sci. 1978, 18, 173–179. [Google Scholar] [CrossRef]
- Chien, R.-D.; Chen, C.-S.; Chen, S.-C. Effect of moulding conditions on the tensile strength of cyclic olefin copolymers (COC) injection moulded parts. Plast. Rubber Compos. 2005, 34, 70–75. [Google Scholar] [CrossRef]
- Theocaris, P.S. Matrix Theory of Photoelasticity; Springer: Berlin/Heidelberg, Germany, 1979; ISBN 9783662158074. [Google Scholar]
- ISO 527-2:2012; Plastics—Determination of Tensile Properties—Part 2: Test Conditions for Moulding and Extrusion Plastics (527-2:2012). ISO: Geneve, Switzerland, 2012.
- Meyers, M.A.; Chawla, K.K. Mechanical Behavior of Materials, 2nd ed.; Cambridge University Press: Cambridge, UK, 2009; ISBN 978-0-511-45557-5. [Google Scholar]
- Menard, K.P. Dynamic Mechanical Analysis: A Practical Introduction; CRC Press: Boca Raton, FL, USA, 1999; ISBN 0-8493-8688-8. [Google Scholar]
- Ferry, J.D. Viscoelastic Properties of Polymers, 3rd ed.; John Wiley & Sons: New York, NY, USA; Chichester, UK; Brisbane, Australia; Toronto, ON, Canada; Singapore, 1980; ISBN 0-471-04894-1. [Google Scholar]
- You, K.Y. (Ed.) Emerging Waveguide Technology; IntechOpen: London, UK, 2018; ISBN 978-1-78923-492-3. [Google Scholar]
- Hessler, S.; Rosenberger, M.; Schmauss, B.; Hellmann, R. Two-dimensional interferometric characterization of laser-induced refractive index profiles in bulk Topas polymer. Opt. Mater. 2018, 75, 230–235. [Google Scholar] [CrossRef]
- TOPAS Advanced Polymers GmbH. TOPAS® 6017S-04 Technical Data Sheet. Available online: https://topas.com/wp-content/uploads/2023/05/TDS_6017S-04_english-units.pdf (accessed on 29 December 2023).
- Agha, A.; Waheed, W.; Alamoodi, N.; Mathew, B.; Alnaimat, F.; Abu-Nada, E.; Abderrahmane, A.; Alazzam, A. A Review of Cyclic Olefin Copolymer Applications in Microfluidics and Microdevices. Macro Mater. Eng. 2022, 307, 2200053. [Google Scholar] [CrossRef]
- Leech, P.W.; Zhang, X.; Zhu, Y. Effect of norbornene content on deformation properties and hot embossing of cyclic olefin copolymers. J. Mater. Sci. 2010, 45, 5364–5369. [Google Scholar] [CrossRef]
- Gopanna, A.; Thomas, S.P.; Rajan, K.P.; Rajan, R.; Rainosalo, E.; Zavašnik, J.; Chavali, M. Investigation of mechanical, dynamic mechanical, rheological and morphological properties of blends based on polypropylene (PP) and cyclic olefin copolymer (COC). Eur. Polym. J. 2018, 108, 439–451. [Google Scholar] [CrossRef]
- Nakade, K.; Nagai, Y.; Ohishi, F. Photodegradation of some ethylene–norbornene random copolymers. Polym. Degrad. Stab. 2010, 95, 2654–2658. [Google Scholar] [CrossRef]
- Wochnowski, C.; Metev, S.; Sepold, G. UV–laser-assisted modification of the optical properties of polymethylmethacrylate. Appl. Surf. Sci. 2000, 154–155, 706–711. [Google Scholar] [CrossRef]
- Roth, B.; Zhou, M.-Y.; Drummer, D. Influence of the Mold Temperature and Part Thickness on the Replication Quality and Molecular Orientation in Compression Injection Molding of Polystyrene. Int. Polym. Process. 2019, 34, 425–433. [Google Scholar] [CrossRef]






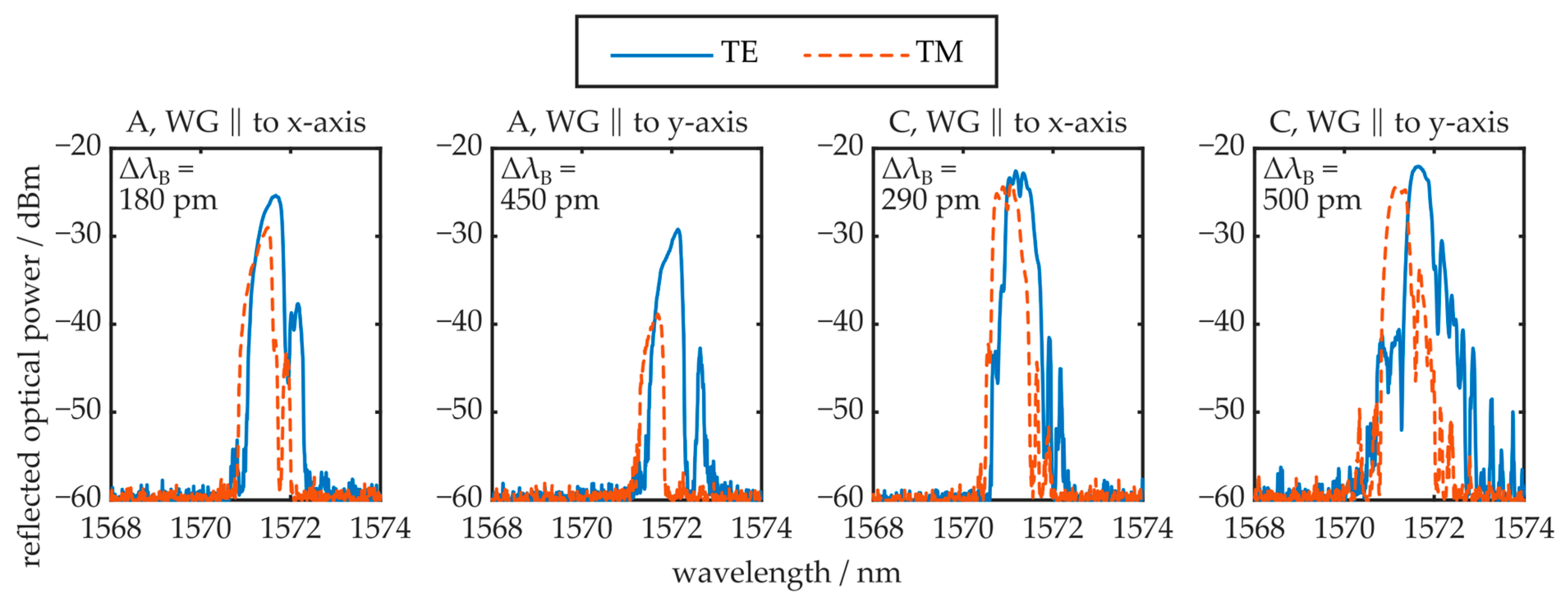

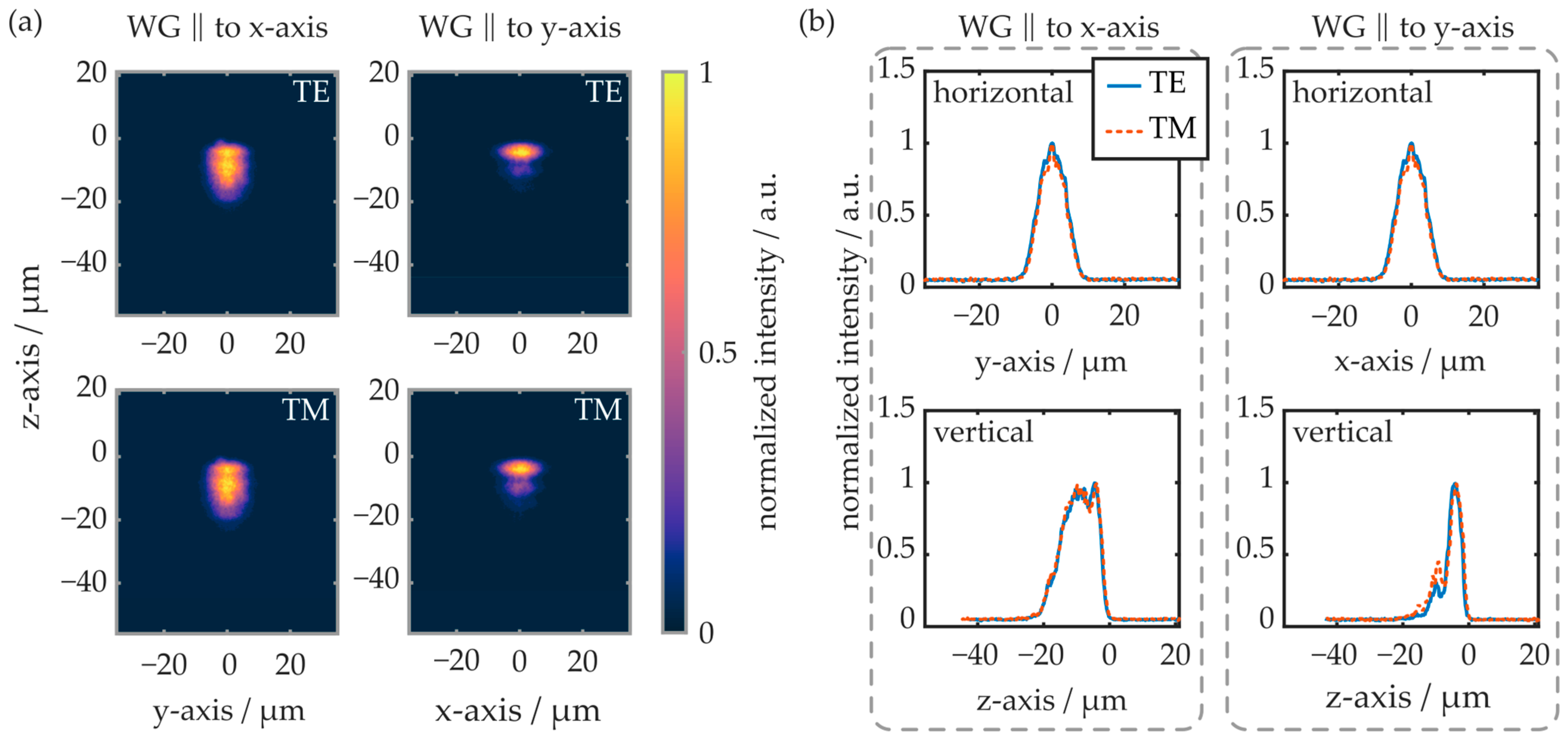
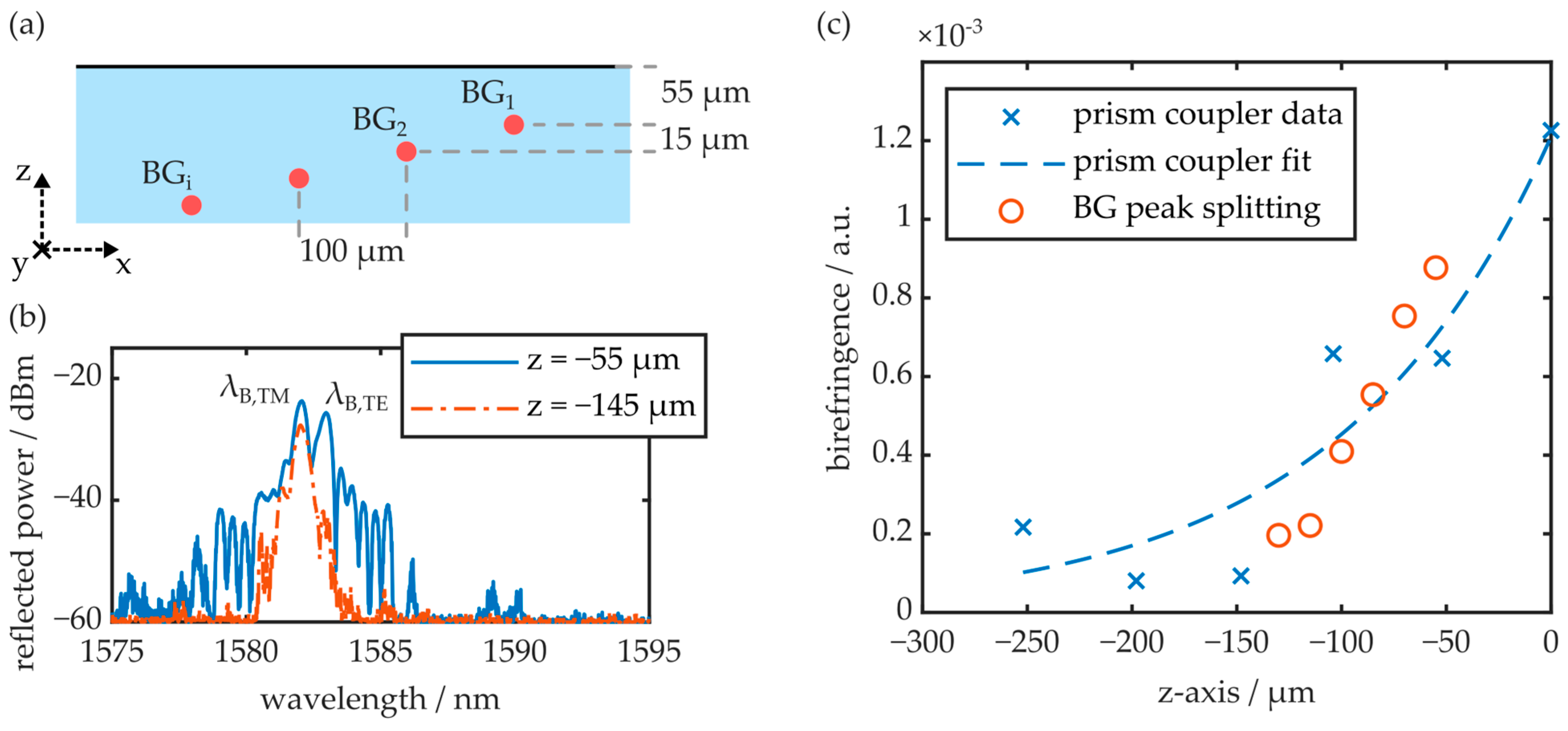
| Parameter | Waveguide | Bragg Grating |
|---|---|---|
| wavelength/nm | 514 | |
| pulse energy/nJ | 330 | |
| pulse duration/fs | 450 | |
| repetition rate/kHz | 25 | 0.5 |
| translation speed/mm × s−1 | 15 | 0.52 |
| focal cross-section/µm | 15 × 1.5 1 | |
| Position | Surface Birefringence/a.u. | |
|---|---|---|
| xz-Plane | yz-Plane | |
| A | 6 × 10−4 | 4 × 10−4 |
| B | 7 × 10−4 | 5 × 10−4 |
| C | 10 × 10−4 | 4 × 10−4 |
| Position | Average Value (1σ Standard Deviation) | ||
|---|---|---|---|
| Tensile Modulus/MPa | Tensile Strength/MPa | Elongation at Break/% | |
| A | 1991 (24) | 56 (0.5) | 3.4 (7 × 10−2) |
| B | 2011 (5) | 58 (0.9) | 3.5 (6 × 10−2) |
| C | 2040 (32) | 56 (1.1) | 3.3 (10 × 10−2) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kefer, S.; Limbach, T.; Pape, N.; Klamt, K.; Schmauss, B.; Hellmann, R. Birefringence in Injection-Molded Cyclic Olefin Copolymer Substrates and Its Impact on Integrated Photonic Structures. Polymers 2024, 16, 168. https://doi.org/10.3390/polym16020168
Kefer S, Limbach T, Pape N, Klamt K, Schmauss B, Hellmann R. Birefringence in Injection-Molded Cyclic Olefin Copolymer Substrates and Its Impact on Integrated Photonic Structures. Polymers. 2024; 16(2):168. https://doi.org/10.3390/polym16020168
Chicago/Turabian StyleKefer, Stefan, Tobias Limbach, Natalie Pape, Kathrin Klamt, Bernhard Schmauss, and Ralf Hellmann. 2024. "Birefringence in Injection-Molded Cyclic Olefin Copolymer Substrates and Its Impact on Integrated Photonic Structures" Polymers 16, no. 2: 168. https://doi.org/10.3390/polym16020168
APA StyleKefer, S., Limbach, T., Pape, N., Klamt, K., Schmauss, B., & Hellmann, R. (2024). Birefringence in Injection-Molded Cyclic Olefin Copolymer Substrates and Its Impact on Integrated Photonic Structures. Polymers, 16(2), 168. https://doi.org/10.3390/polym16020168







