Robust Strain Sensor with High Sensitivity Based on Polymer-Encapsulated Microfiber Mach–Zehnder Interferometer
Abstract
1. Introduction
2. Theory and Sensor Fabrication
2.1. Theory
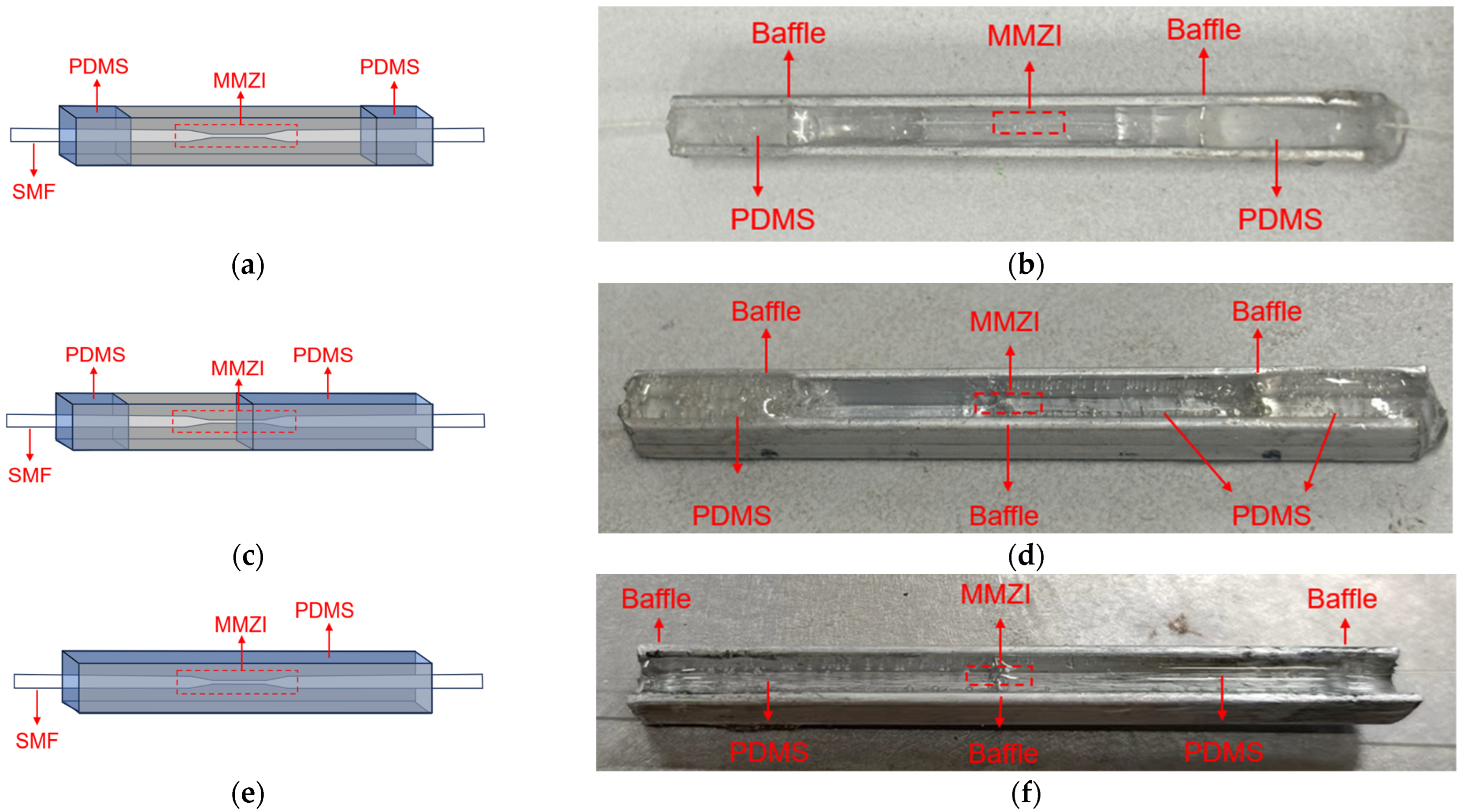
2.2. Sensor Fabrication
3. Experimental Results
4. Discussion
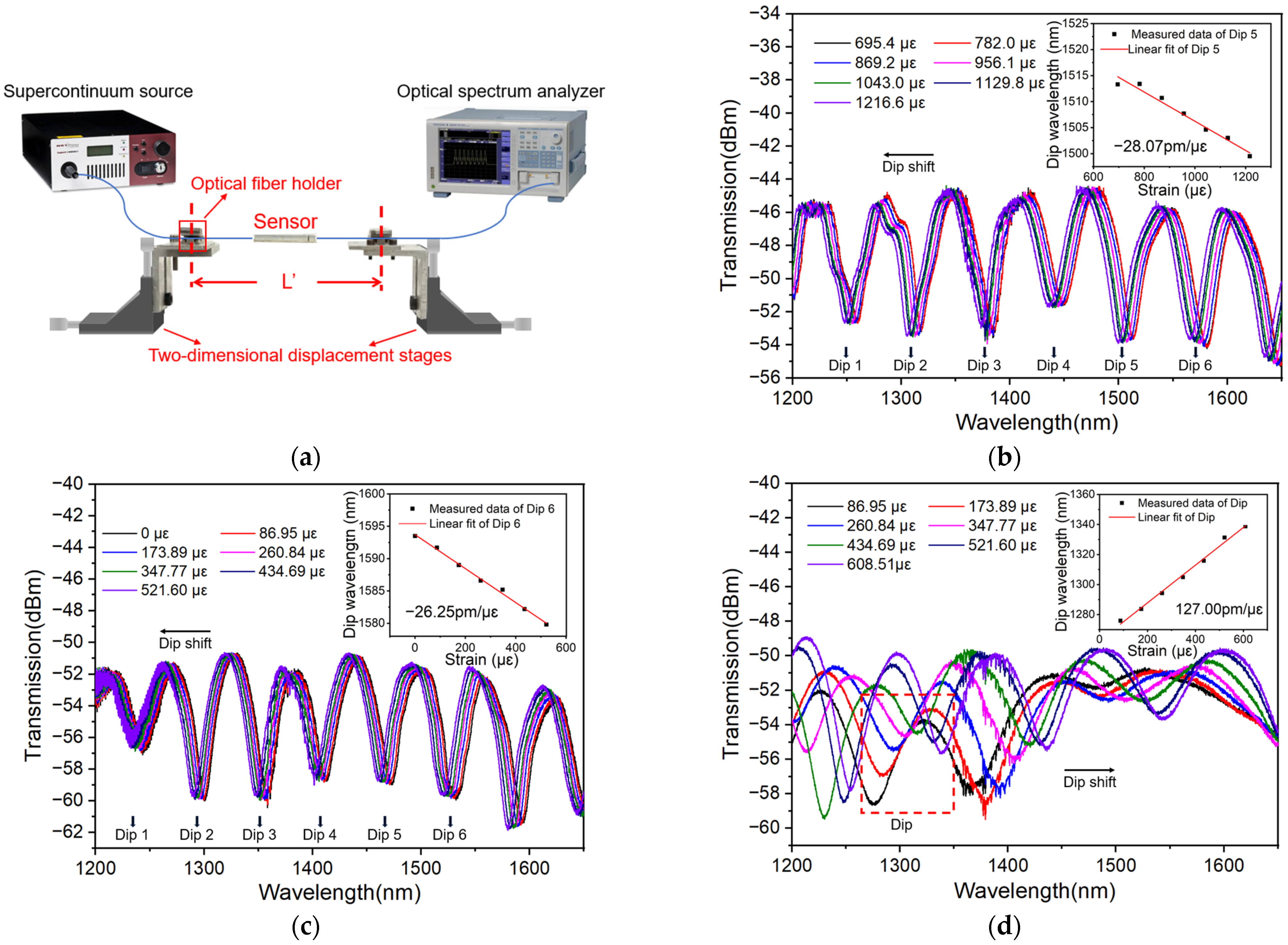
4.1. Dependence of Sensitivity on Probing Wavelength
4.2. Effect of Encapsulation Length on Sensitivity
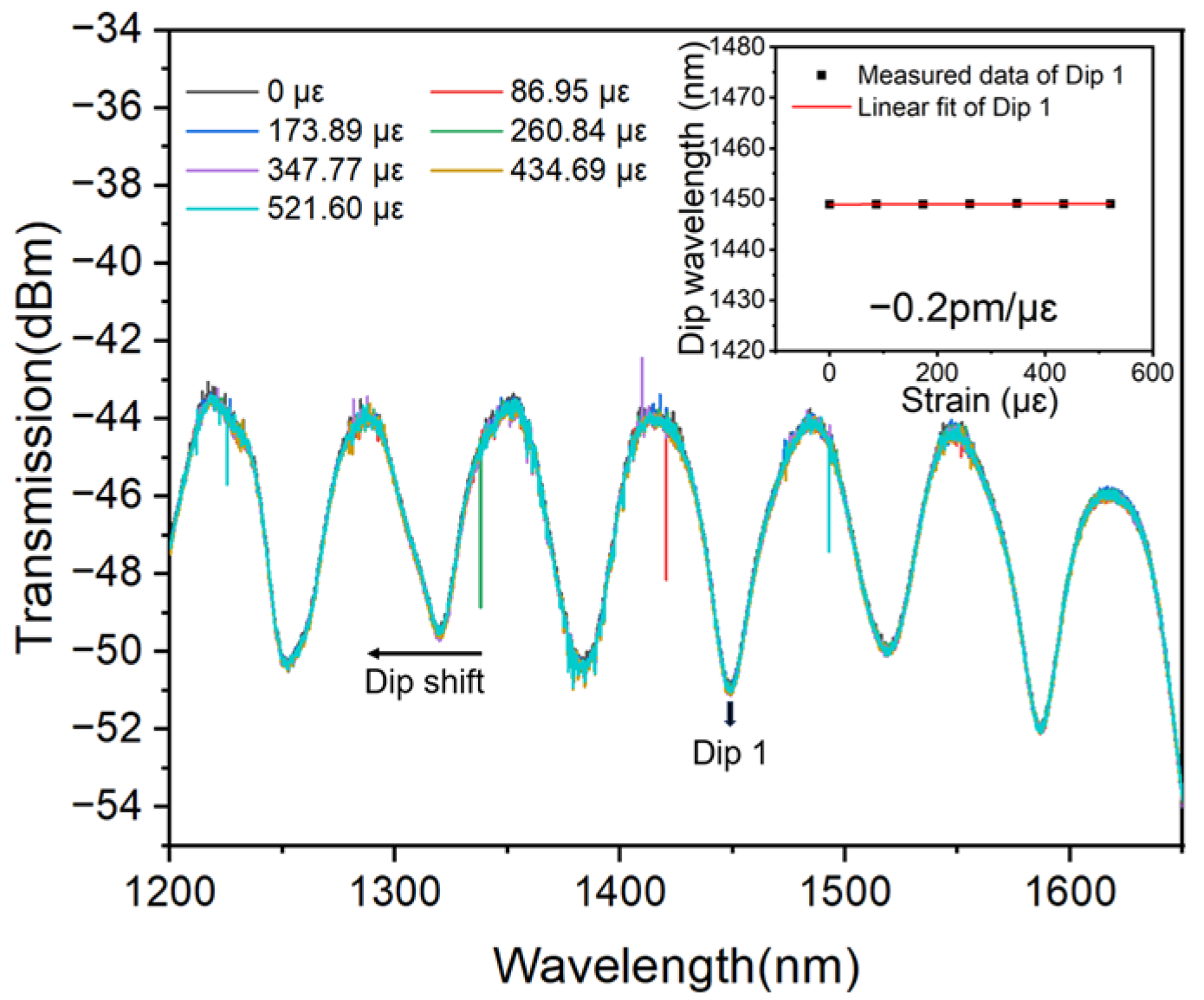
4.3. Effect of Polymer Used in Encapsulation on Sensitivity
4.4. Dependence of Strain Sensitivity on Temperature
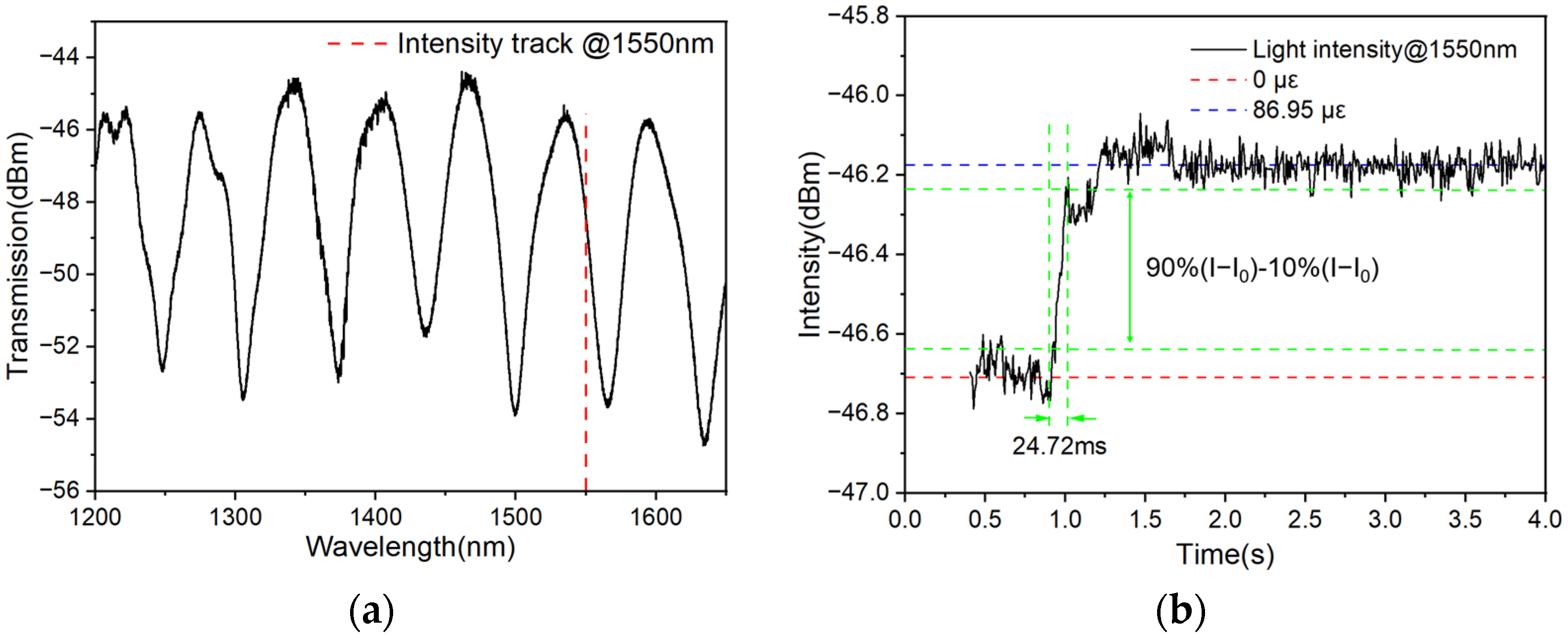
4.5. Response Time
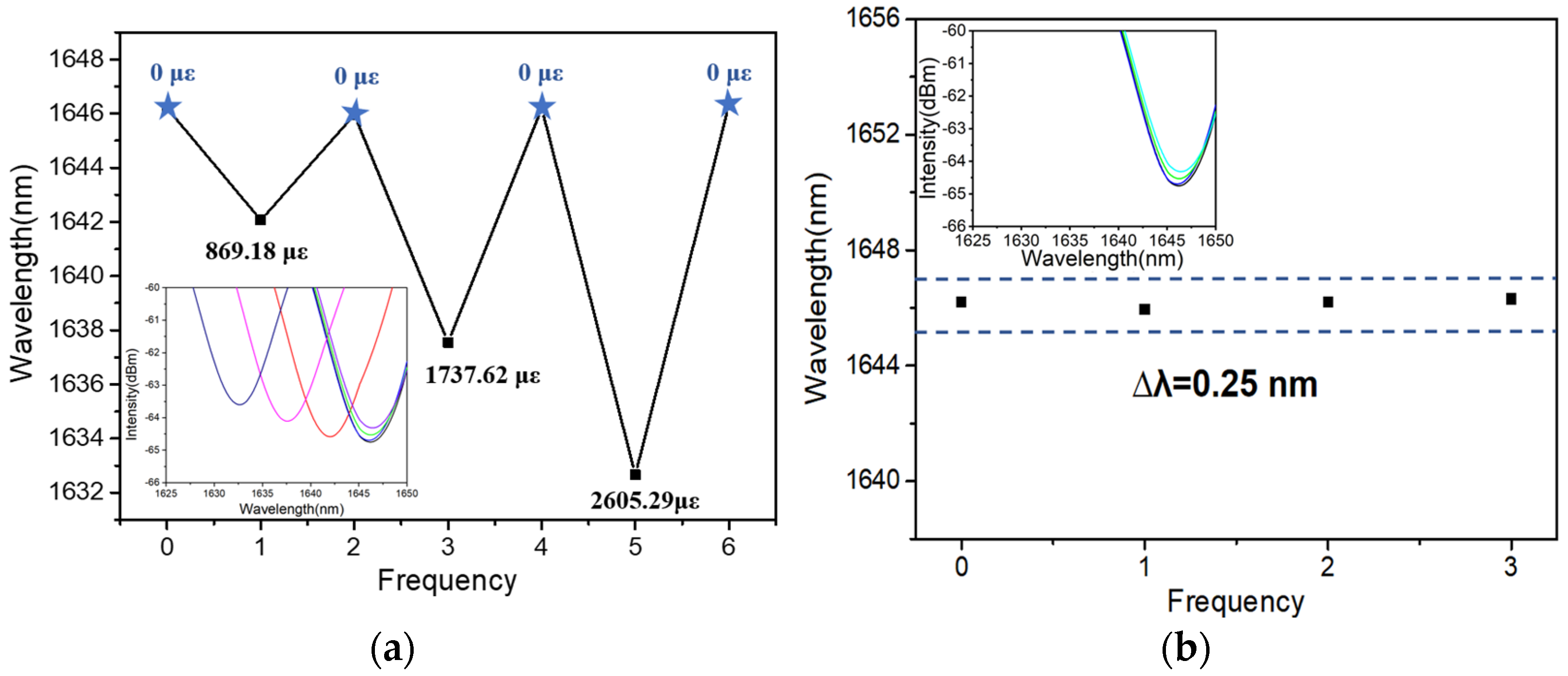
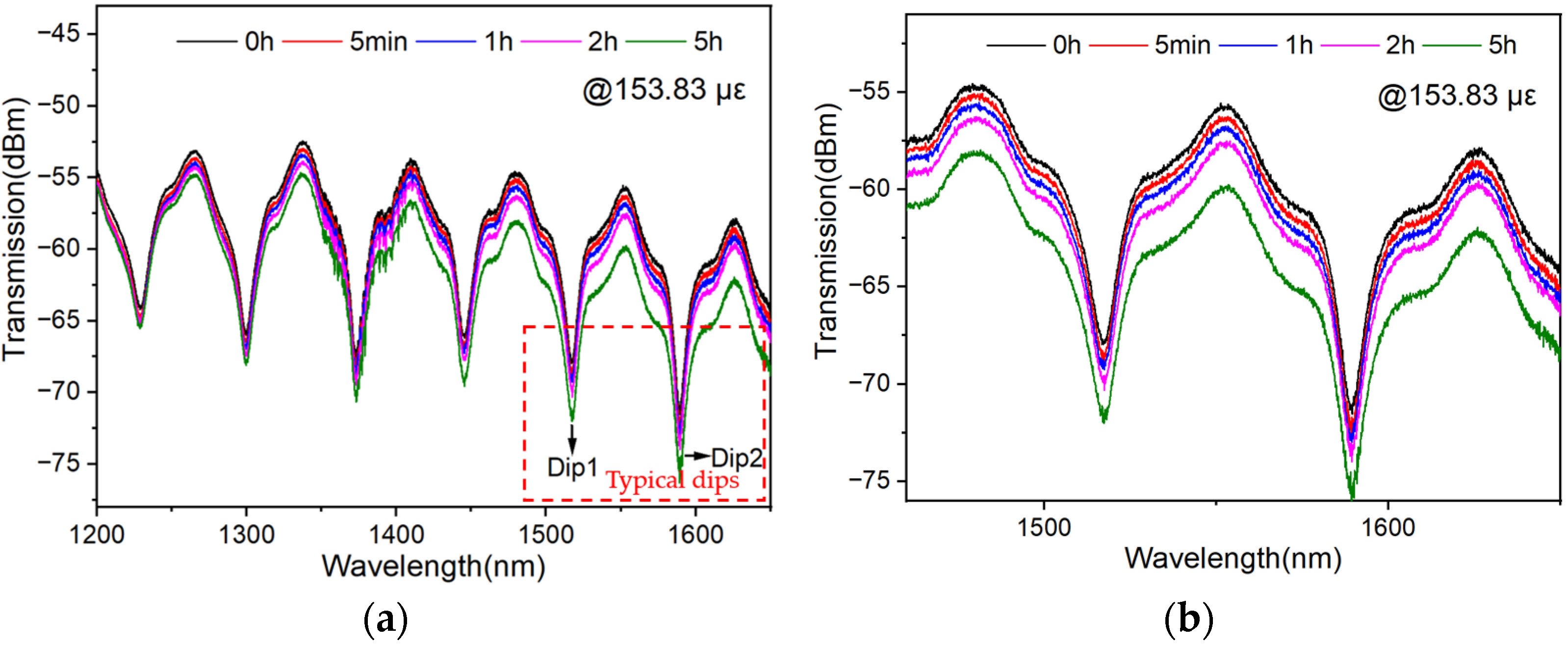
4.6. Recoverability and Repeatability
4.7. Comparison with Other Strain Optical Fiber Sensors
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kaur, G.; Kaler, R.S.; Kwatra, N. Experiment on a highly sensitive fiber Bragg grating optical sensor to monitor strain and corrosion in civil structures. J. Opt. Technol. 2018, 85, 36–41. [Google Scholar] [CrossRef]
- Wang, H.; Xiang, P.; Jiang, L. Optical fiber sensor based in-field structural performance monitoring of multilayered asphalt pavement. J. Light. Technol. 2018, 36, 3624–3632. [Google Scholar] [CrossRef]
- Ren, L.; Jia, Z.G.; Li, H.N.; Song, G. Design and experimental study on FBG hoop-strain sensor in pipeline monitoring. Opt. Fiber Technol. 2014, 20, 15–23. [Google Scholar] [CrossRef]
- Li, N.; Li, J.; Tao, C.; Cheng, J.; Jiang, X.; Zhang, J.; Mao, L.; Xiao, J.; Zhao, Y. Intensity-modulated optical fiber sensor for static strain and vibration monitoring. In Proceedings of the Advanced Sensor Systems and Applications IX, Hangzhou, China, 21–22 October 2019. [Google Scholar]
- Chen, M.; He, Y.; Liang, H.; Zhou, H.; Wang, X.; Heng, X.; Zhang, Z.; Gan, J.; Yang, Z. Stretchable and strain-decoupled fluorescent optical fiber sensor for body temperature and movement monitoring. ACS Photonics 2022, 9, 1415–1424. [Google Scholar] [CrossRef]
- Wu, L.; Shi, X.; Das, P.; Wu, Z.S. Recent application progress and key challenges of biomass-derived carbons in resistive strain/pressure sensor. Sci. China Mater. 2023, 66, 1702–1718. [Google Scholar] [CrossRef]
- Nesser, H.; Lubineau, G. Strain sensing by electrical capacitive variation: From stretchable materials to electronic interfaces. Adv. Electron. Mater. 2021, 7, 2100190. [Google Scholar] [CrossRef]
- Reder, C.; Loidl, D.; Puchegger, S.; Gitschthaler, D.; Peterlik, H.; Kromp, K.; Khatibi, G.; Betzwar-Kotas, A.; Zimprich, P.; Weiss, B. Non-contacting strain measurements of ceramic and carbon single fibres by using the laser-speckle method. Compos. Part A Appl. Sci. Manuf. 2003, 34, 1029–1033. [Google Scholar] [CrossRef]
- Choi, W.Y.; Jo, H.G.; Kwon, S.W.; Kim, Y.H.; Pyun, J.Y.; Park, K.K. Multipoint-detection strain sensor with a single electrode using optical ultrasound generated by carbon nanotubes. IEEE Sens. J. 2019, 19, 3877. [Google Scholar] [CrossRef]
- Min, R.; Ortega, B.; Broadway, C.; Hu, X.; Caucheteur, C.; Bang, O.; Antunes, P.; Marques, C. Microstructured PMMA POF chirped Bragg gratings for strain sensing. Opt. Fiber Technol. 2018, 45, 330–335. [Google Scholar] [CrossRef]
- Broadway, C.; Min, R.; Leal-Junior, A.G.; Marques, C.; Caucheteur, C. Toward commercial polymer fiber Bragg grating sensors: Review and applications. J. Light. Technol. 2019, 37, 2605–2615. [Google Scholar] [CrossRef]
- Liu, Z.; Shen, C.; Xiao, Y.; Gong, J.; Wang, Y.; Lang, T.T.; Zhang, Y.; Xu, W.; Zhang, T.; Jing, Z.; et al. Sensitivity-enhanced strain sensor based on thin-core fiber modal interferometer interacted with tilted fiber Bragg grating. IEEE Sens. J. 2018, 19, 1802–1806. [Google Scholar] [CrossRef]
- Yoon, M.S.; Park, S.; Han, Y.G. Simultaneous measurement of strain and temperature by using a micro-tapered fiber grating. J. Light. Technol. 2012, 30, 1156–1160. [Google Scholar] [CrossRef]
- Patrick, H.J.; Vohra, S.T. Fiber Bragg grating with long-period fiber grating superstructure for simultaneous strain and temperature measurement. EWOFS 1998, 3483, 264–267. [Google Scholar]
- Liao, C.; Wang, D.; Wang, Y. Microfiber in-line Mach–Zehnder interferometer for strain sensing. Opt. Lett. 2013, 38, 757–759. [Google Scholar] [CrossRef] [PubMed]
- Zhang, H.; Wu, Z.; Shum, P.P.; Dinh, X.Q.; Low, C.W.; Xu, Z.; Wang, R.; Shao, X.; Fu, S.; Tong, W.; et al. Highly sensitive strain sensor based on helical structure combined with Mach-Zehnder interferometer in multicore fiber. Sci. Rep. 2017, 1, 46633. [Google Scholar] [CrossRef]
- Tian, K.; Zhang, M.; Farrell, G.; Wang, R.; Lewis, E.; Wang, P. Highly sensitive strain sensor based on composite interference established within S-tapered multimode fiber structure. Opt. Express 2018, 26, 33982–33992. [Google Scholar] [CrossRef]
- Xing, R.; Dong, C.; Wang, Z.; Wu, Y.; Yang, Y.; Jian, S. Simultaneous strain and temperature sensor based on polarization maintaining fiber and multimode fiber. Opt. Laser Technol. 2018, 102, 17–21. [Google Scholar] [CrossRef]
- Wang, S.; Ma, Y.; Chen, W.; Wang, S.; Yi, Y.; Li, X.; Lu, C.; Zhang, S.; Geng, T.; Sun, W.; et al. Ultrasensitive strain sensor based on Mach-Zehnder interferometer with bent structures. J. Light. Technol. 2021, 21, 6958–6967. [Google Scholar] [CrossRef]
- Zhang, Y.; Wu, Y.; Han, Y.; Wu, J.; Ren, J.; Mao, Y.; Mao, B.; Liu, B. High sensitivity strain sensor based on a novel offset-core single micro-tapered fiber-optic Mach-Zehnder interferometer. Opt. Fiber Technol. 2022, 73, 103012. [Google Scholar] [CrossRef]
- Tian, J.; Jiao, Y.; Fu, Q.; Ji, S.; Li, Z.; Quan, M.; Yao, Y. A Fabry-Perot interferometer strain sensor based on concave-core photonic crystal fiber. J. Light. Technol. 2018, 36, 1952–1958. [Google Scholar] [CrossRef]
- Yang, S.; Zhang, Q.; Li, X.; Wang, Q.; Li, Y. Optical fiber strain sensor with high and tunable sensitivity. Rev. Sci. Instrum. 2023, 94, 1–4. [Google Scholar] [CrossRef] [PubMed]
- Belal, M.; Cho, Y.T.; Ibsen, M.; Newson, T.P. A temperature-compensated high spatial resolution distributed strain sensor. Meas. Sci. Technol. 2010, 21, 015204. [Google Scholar] [CrossRef]
- Liu, Z.; Zhang, Y.; Wang, Z.; Zhang, Y.; Zhao, E.; Zhou, A.; Yuan, L. A hybrid Michelson-FP interference fiber sensor. In Proceedings of the 24th International Conference on Optical Fiber Sensors, Curitiba, Brazil, 28 September–2 October 2015. [Google Scholar]
- Shin, J.C.; Kwak, W.G.; Han, Y.G. Temperature-insensitive microfiber Mach–Zehnder interferometer for absolute strain measurement. J. Light. Technol. 2016, 34, 4579–4583. [Google Scholar] [CrossRef]
- Li, W.; Hu, Z.F.; Li, X.Y.; Fang, W.; Guo, X.; Tong, L.M.; Lou, J.Y. High-sensitivity microfiber strain and force sensors. Opt. Commun. 2014, 314, 28–30. [Google Scholar] [CrossRef]
- Li, C.; Ning, T.; Li, J.; Zhang, C.; Zhang, C.; Lin, H.; Pei, L. Fiber-optic laser sensor based on all-fiber multipath Mach–Zehnder interferometer. IEEE Photonics Technol. Lett. 2016, 28, 1908–1911. [Google Scholar] [CrossRef]
- Wang, J.; Liao, Y.P.; Wang, S.S.; Wang, X. Ultrasensitive optical sensing in aqueous solution based on microfiber modal interferometer. Opt. Express 2018, 26, 24843–24853. [Google Scholar] [CrossRef]
- Liu, T.Q.; Wang, J.; Liao, Y.P.; Yang, L.; Wang, S.S. Splicing point tapered fiber Mach–Zehnder interferometer for simultaneous measurement of temperature and salinity in seawater. Opt. Express 2019, 27, 23905–23918. [Google Scholar] [CrossRef]
- Wen, J.H.; Wang, J.; Yang, L.; Hou, Y.F.; Huo, D.H.; Cai, E.L.; Xiao, Y.X.; Wang, S.S. Response time of microfiber temperature sensor in liquid environment. IEEE Sens. J. 2020, 20, 6400–6407. [Google Scholar] [CrossRef]
- Wu, Y.; Zhang, Y.; Wu, J.; Yuan, P. Temperature-insensitive fiber optic Fabry-Perot interferometer based on special air cavity for transverse load and strain measurements. Opt. Express 2017, 25, 9443–9448. [Google Scholar] [CrossRef]
- Zhao, Y.; Chen, M.Q.; Peng, Y. Tapered hollow-core fiber air-microbubble Fabry–Perot interferometer for high sensitivity strain measurement. Adv. Mater. 2018, 5, 1800886. [Google Scholar] [CrossRef]
- Jauregui-Vazquez, D.; Korterik, J.P.; Osornio-Martinez, C.E.; Estudillo-Ayala, J.M.; Offerhaus, H.L.; Alvarez-Chavez, J.A. A strain reflection-based fiber optic sensor using thin core and standard single-mode fibers. Opt. Commun. 2022, 522, 128659. [Google Scholar] [CrossRef]
- Tong, C.; Yuan, C.; Meng, W.; Pan, Q.; Sun, H.; Zheng, J.; Zhang, T.; Fan, Y.; Yuan, L.; Geng, T. All optical fiber sensor based on asymmetric staggered taper and optical fiber bitaper structure. IEEE Photonics Technol. Lett. 2023, 35, 1002–1005. [Google Scholar] [CrossRef]






| Encapsulations | Sensitivity Sε (pm/με) and Central Wavelength λc (nm) | ||||||
|---|---|---|---|---|---|---|---|
| Sε/λc | Dip 1 | Dip 2 | Dip 3 | Dip 4 | Dip 5 | Dip 6 | |
| MMZI half-covered by PDMS | Sε | −20.95 | −21.26 | −22.43 | −24.20 | −24.20 | −26.25 |
| λc | 1295.69 | 1353.40 | 1407.09 | 1466.71 | 1525.60 | 1586.86 | |
| MMZI encapsulated at two ends by PDMS | Sε | −23.26 | −25.52 | −22.98 | −27.42 | −28.07 | −30.99 |
| λc | 1254.61 | 1313.47 | 1379.67 | 1443.73 | 1507.46 | 1574.44 | |
| Encapsulation Length (Left and Right) | Strain Sensitivity (pm/με) |
|---|---|
| 1 cm, 1 cm | −90.82 ± 0.47 |
| 1 cm, 2 cm | −68.57 ± 0.62 |
| 2 cm, 2 cm | −63.04 ± 0.76 |
| 2.5 cm, 2.5 cm (Full-covered) | 84.48 ± 1.32 |
| Sensor Structure | Sensitivity | Robustness | Cost | Fabrication | Detection Complexity | Year | |
|---|---|---|---|---|---|---|---|
| FBG | FBG | 0.90 pm/µε | High | Medium | Difficult | Simple | 2018 [10] |
| MZI | MZI | −61.80 pm/µε | Low | High | Easy | Simple | 2017 [16] |
| MZI with MMF | −103.80 pm/µε | Medium | Medium | Easy | Simple | 2018 [17] | |
| MZI | 165.00 pm/µε | Medium | Low | Medium | Simple | 2021 [19] | |
| MZI | −34.61 pm/µε | Low | Low | Easy | Simple | 2022 [20] | |
| MMZI | −20.95~127.00 pm/µε | High | Low | Easy | Simple | This work | |
| MMZI | 4.84 pm/µε | Low | Low | Easy | Simple | 2014 [26] | |
| FPI | FPI with PCF | 31.58 pm/µε | Low | High | Difficult | Medium | 2018 [21] |
| FPI with SMF | 1932.00 pm/µε | High | Low | Easy | Medium | 2023 [22] | |
| FPI with HCF | 3.29 pm/µε | Medium | Medium | Medium | Medium | 2017 [31] | |
| FPI with HCF | 8.62 pm/µε | Low | Medium | Difficult | Medium | 2018 [32] | |
| Hybrid | TCF and SMF | 2.40 pm/µε | Medium | Medium | Easy | Medium | 2022 [33] |
| PCF and SMF | −71.92 pm/µε | Low | Medium | Medium | Simple | 2023 [34] | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xiao, B.; Zhuang, F.; Wang, J.; Yao, Z.; Wang, S. Robust Strain Sensor with High Sensitivity Based on Polymer-Encapsulated Microfiber Mach–Zehnder Interferometer. Polymers 2024, 16, 2810. https://doi.org/10.3390/polym16192810
Xiao B, Zhuang F, Wang J, Yao Z, Wang S. Robust Strain Sensor with High Sensitivity Based on Polymer-Encapsulated Microfiber Mach–Zehnder Interferometer. Polymers. 2024; 16(19):2810. https://doi.org/10.3390/polym16192810
Chicago/Turabian StyleXiao, Bin, Funa Zhuang, Jing Wang, Zhongyu Yao, and Shanshan Wang. 2024. "Robust Strain Sensor with High Sensitivity Based on Polymer-Encapsulated Microfiber Mach–Zehnder Interferometer" Polymers 16, no. 19: 2810. https://doi.org/10.3390/polym16192810
APA StyleXiao, B., Zhuang, F., Wang, J., Yao, Z., & Wang, S. (2024). Robust Strain Sensor with High Sensitivity Based on Polymer-Encapsulated Microfiber Mach–Zehnder Interferometer. Polymers, 16(19), 2810. https://doi.org/10.3390/polym16192810






