High-Quality Foaming and Weight Reduction in Microcellular-Injection-Molded Polycarbonate Using Supercritical Fluid Carbon Dioxide under Gas Counter Pressure
Abstract
1. Introduction
2. Materials and Methods
2.1. Foaming Materials and Mold Design
2.2. The MuCell® Machine and Gas Counter Pressure System
2.3. The Electronic Balancer
2.4. The Scanning Electron Microscope
2.5. Experimental Parameters
2.6. Cell Size and Density Measurements
2.7. Gray Relational Analysis
- 1.
- Data normalization:
- 2.
- The gray relational coefficient:
- 3.
- Calculate the GRG:
3. Results and Discussion
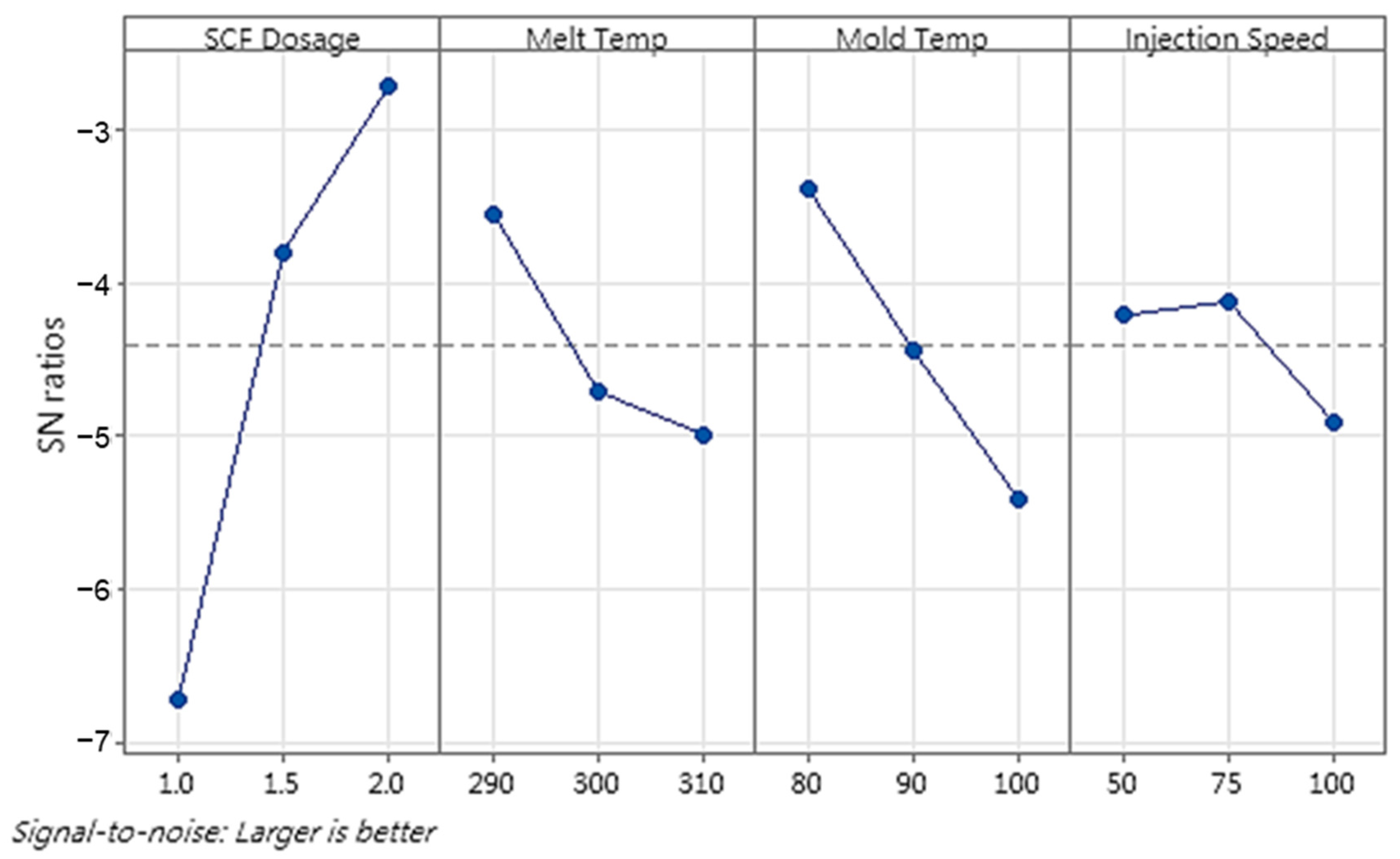
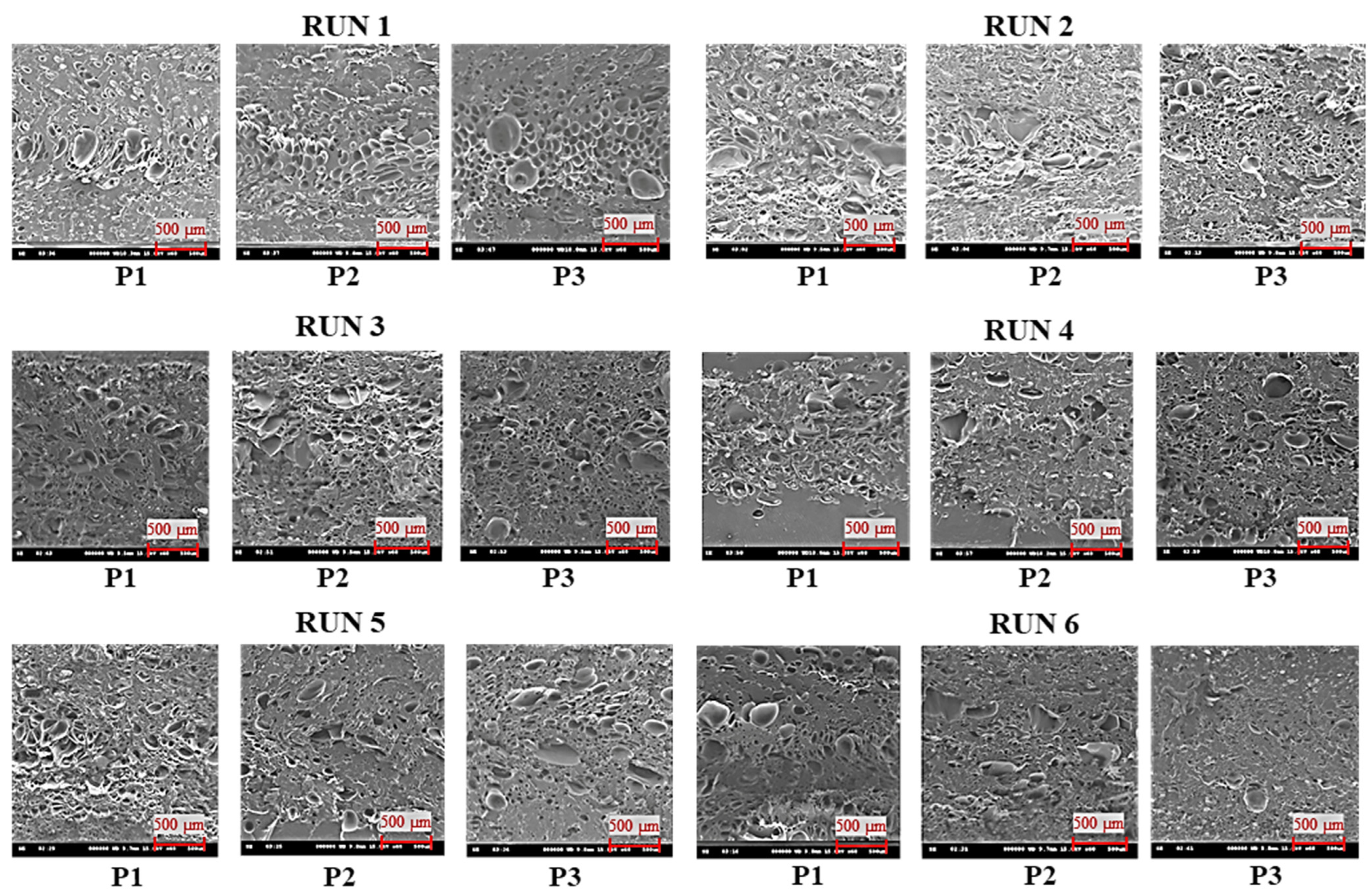
3.1. First Stage of the Experiment (without GCP)
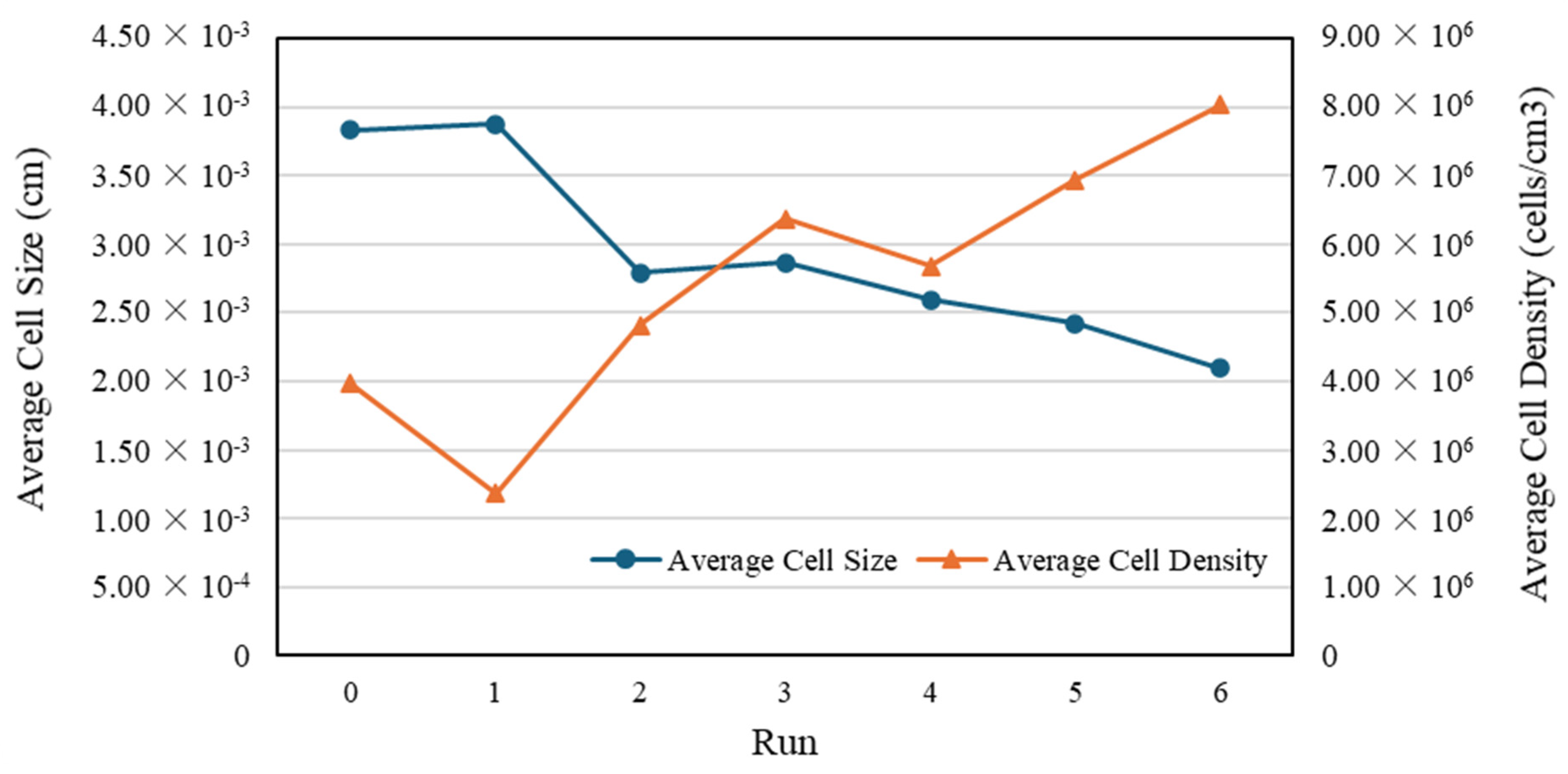
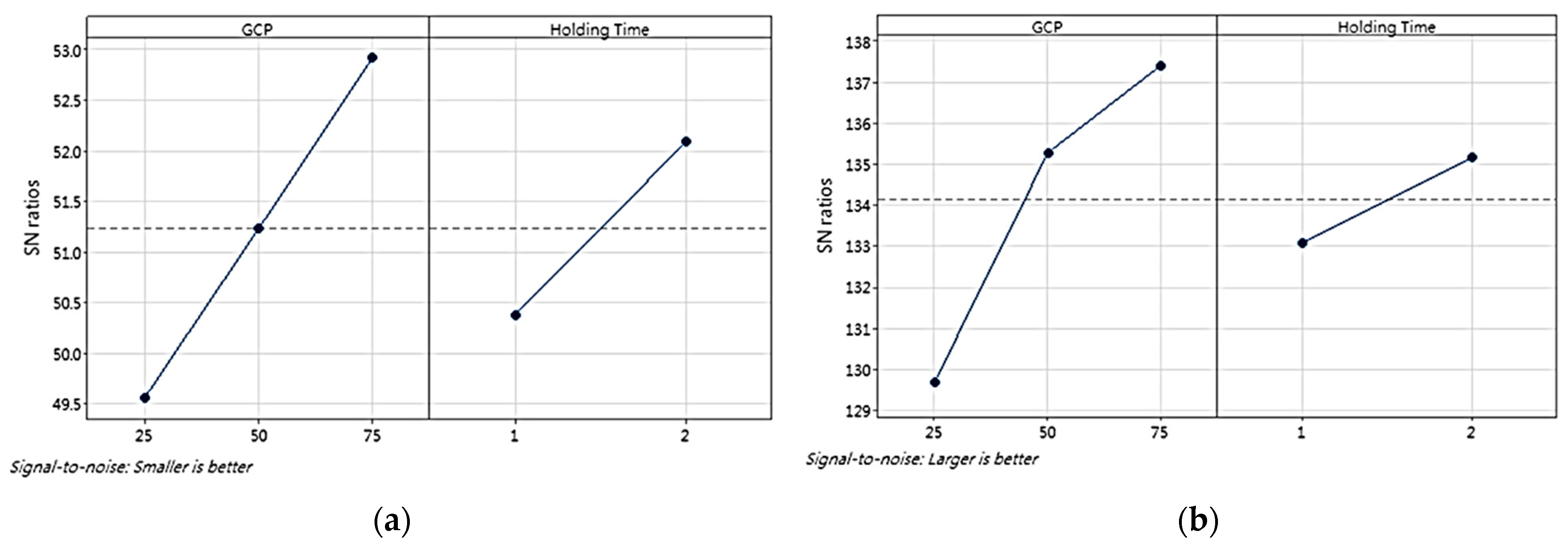
3.2. Second Stage of the Experiment (with GCP)
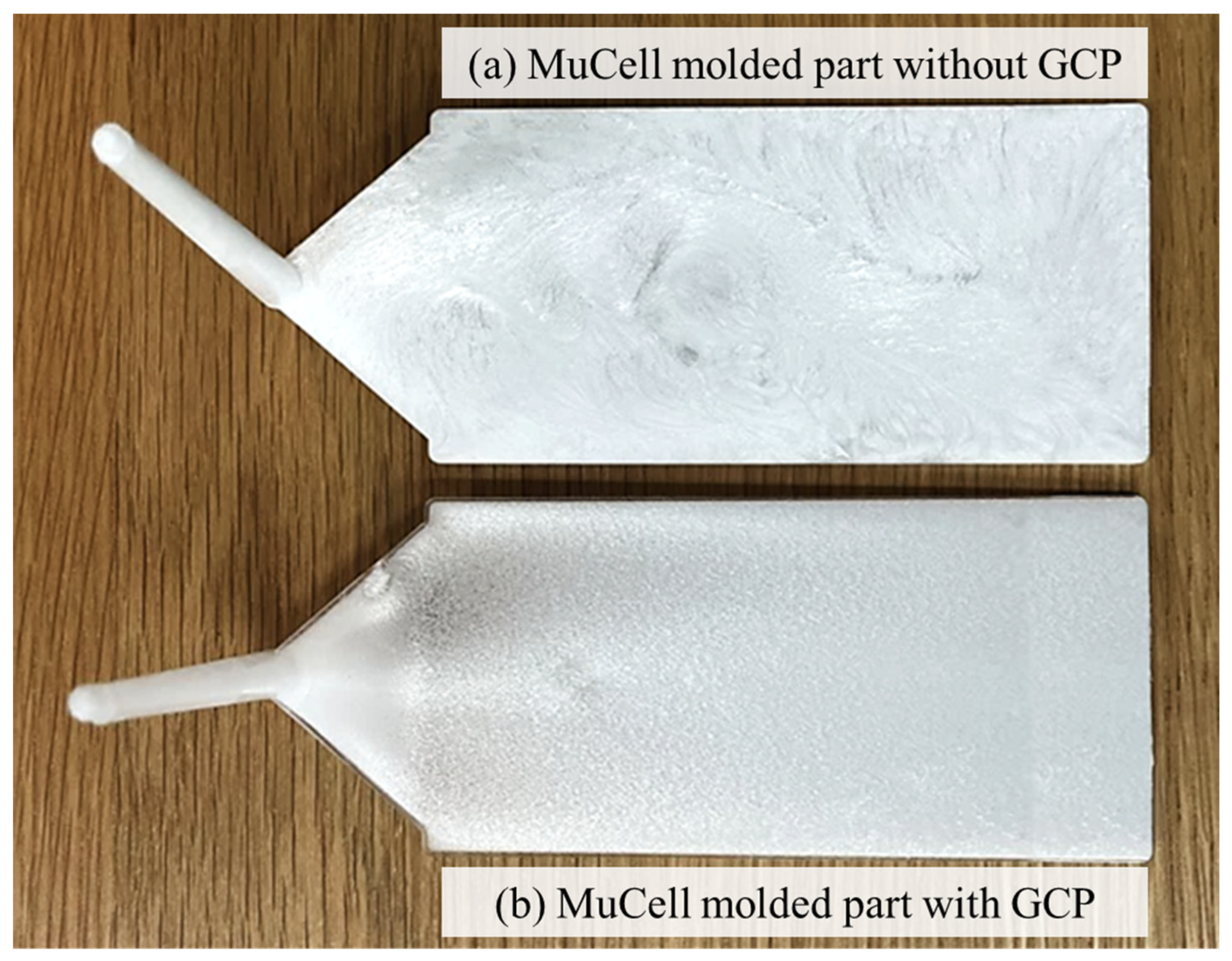
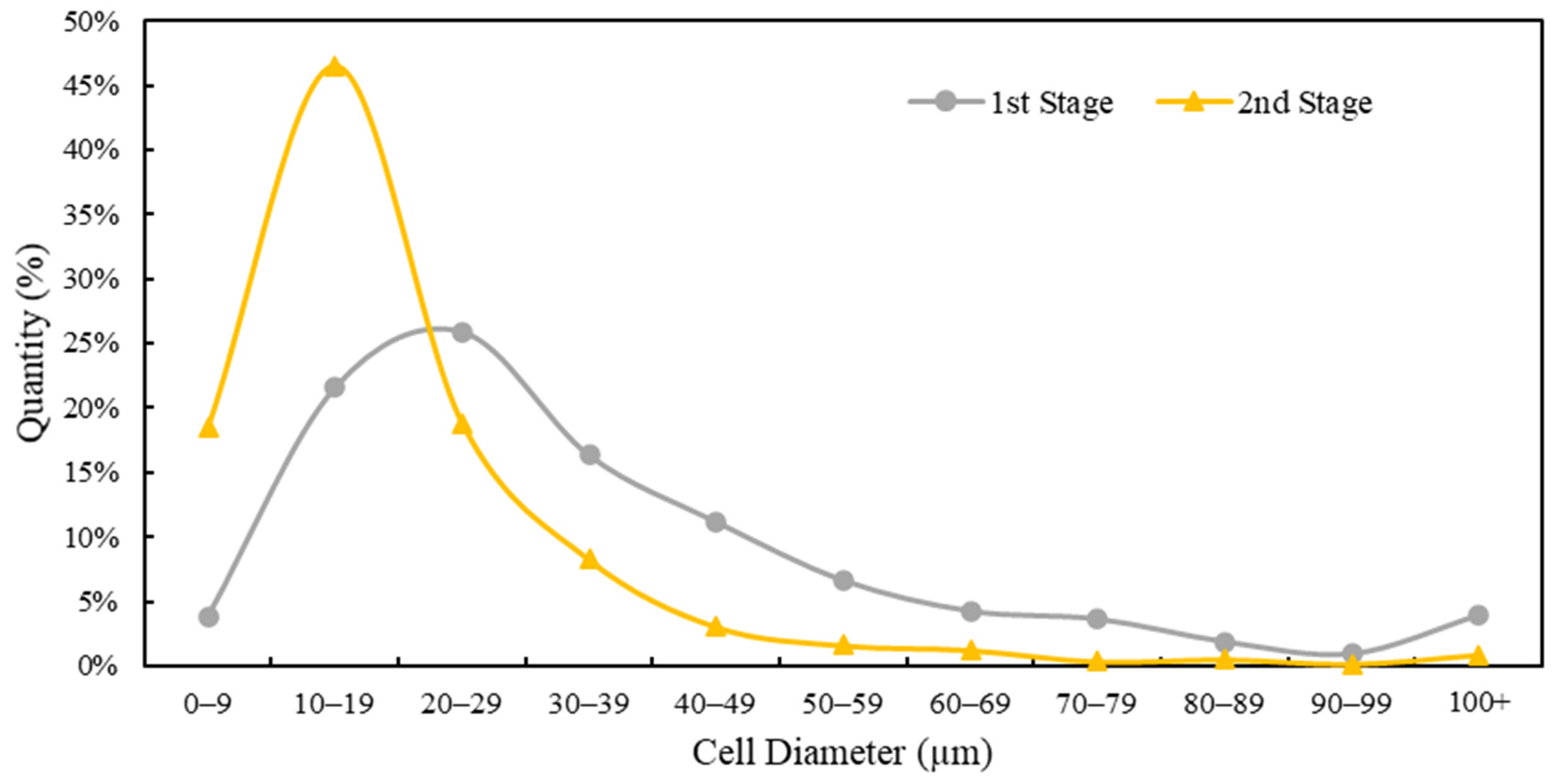
3.3. Comparison of the Results from the First and Second Stages of the Experiments
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Jiang, J.; Li, Z.; Yang, H.; Wang, X.; Li, Q.; Turng, L.-S. Microcellular injection molding of polymers: A review of process know-how, emerging technologies, and future directions. Curr. Opin. Chem. Eng. 2021, 33, 100694. [Google Scholar] [CrossRef]
- Chen, S.-C.; Lee, K.-H.; Chang, C.-W.; Hsu, T.-J.; Feng, C.-T. Using gas counter pressure and combined technologies for microcellular injection molding of thermoplastic polyurethane to achieve high foaming qualities and weight reduction. Polymers 2022, 14, 2017. [Google Scholar] [CrossRef]
- Gómez-Monterde, J.; Hain, J.; Sánchez-Soto, M.; Maspoch, M.L. Microcellular injection moulding: A comparison between MuCell process and the novel micro-foaming technology IQ foam. J. Mater. Process. Technol. 2018, 268, 162–170. [Google Scholar] [CrossRef]
- Dong, G.; Zhao, G.; Guan, Y.; Li, A.; Wang, X. Formation mechanism and structural characteristics of unfoamed skin layer in microcellular injection-molded parts. J. Cell. Plast. 2016, 52, 419–439. [Google Scholar] [CrossRef]
- Elduque, D.; Clavería, I.; Fernández, Á.; Javierre, C.; Pina, C.; Santolaria, J. Methodology to analyze the influence of microcellular injection molding on mechanical properties with samples obtained directly of an industrial component. Polym. Polym. Compos. 2014, 22, 743–752. [Google Scholar] [CrossRef]
- Wu, H.; Zhao, G.; Wang, G.; Zhang, W.; Li, Y. A new core-back foam injection molding method with chemical blowing agents. Mater. Des. 2018, 144, 331–342. [Google Scholar] [CrossRef]
- Elduque, D.; Clavería, I.; Fernández, Á.; Javierre, C.; Pina, C.; Santolaria, J. Analysis of the influence of microcellular injection molding on the environmental impact of an industrial component. Adv. Mech. Eng. 2014, 6, 793269. [Google Scholar] [CrossRef]
- Suhartono, E.; Chen, S.-C.; Lee, K.-H.; Wang, K.-J. Improvements on the tensile properties of microcellular injection molded parts using microcellular co-injection molding with the material combinations of PP and PP-GF. Int. J. Plast. Technol. 2017, 21, 351–369. [Google Scholar] [CrossRef]
- Turng, L.-S.; Kharbas, H. Development of a Hybrid Solid-Microcellular Co-injection Molding Process. Int. Polym. Proc. 2004, 19, 77–86. [Google Scholar] [CrossRef]
- Chen, S.; Hsu, P.; Hwang, S. The effects of gas counter pressure and mold temperature variation on the surface quality and morphology of the microcellular polystyrene foams. J. Appl. Polym. Sci. 2013, 127, 4769–4776. [Google Scholar] [CrossRef]
- Chen, S.-C.; Liao, W.-H.; Chien, R.-D. Structure and mechanical properties of polystyrene foams made through microcellular injection molding via control mechanisms of gas counter pressure and mold temperature. Int. Commun. Heat Mass Transf. 2012, 39, 1125–1131. [Google Scholar] [CrossRef]
- Chen, S.-C.; Chang, C.-W.; Tseng, C.-Y.; Shen, E.-N.; Feng, C.-T. Using p(Pressure)-t(temperature) path to control the foaming cell sizes in microcellular injection molding process. Polymers 2021, 13, 1843. [Google Scholar] [CrossRef] [PubMed]
- Sun, X.; Turng, L.-S.; Gorton, P.J.; Nigam, P.; Buell, S. Novel injection molding foaming approaches using gas-laden pellets with N2,CO2, and N2 + CO2 as the blowing agents. Polym. Eng. Sci. 2014, 54, 899–913. [Google Scholar] [CrossRef]
- Leicher, S.; Will, J.; Haugen, H.; Wintermantel, E. MuCell® technology for injection molding: A processing method for polyether-urethane scaffolds. J. Mater. Sci. 2005, 40, 4613–4618. [Google Scholar] [CrossRef]
- Lee, J.; Turng, L.; Dougherty, E.; Gorton, P. Novel foam injection molding technology using carbon dioxide-laden pellets. Polym. Eng. Sci. 2011, 51, 2295–2303. [Google Scholar] [CrossRef]
- Wang, L.; Hikima, Y.; Ohshima, M.; Yusa, A.; Yamamoto, S.; Goto, H. Development of a simplified foam injection molding technique and its application to the production of high void fraction polypropylene foams. Ind. Eng. Chem. Res. 2017, 56, 13734–13742. [Google Scholar] [CrossRef]
- Yeh, S.-K.; Liu, Y.-C.; Chu, C.-C.; Chang, K.-C.; Wang, S.-F. Mechanical properties of microcellular and nanocellular thermoplastic polyurethane nanocomposite foams created using supercritical carbon dioxide. Ind. Eng. Chem. Res. 2017, 56, 8499–8507. [Google Scholar] [CrossRef]
- Ding, Y.; Hassan, M.H.; Bakker, O.; Hinduja, S.; Bártolo, P. A review on microcellular injection moulding. Materials 2021, 14, 4209. [Google Scholar] [CrossRef]
- Ding, Y.; Vyas, C.; Bakker, O.; Hinduja, S.; Bartolo, P. Modelling and simulation of MuCell®: The effect of key processing parameters on cell size and weight reduction. Polymers 2022, 14, 4215. [Google Scholar] [CrossRef]
- Ren, J.; Lin, L.; Jiang, J.; Li, Q.; Hwang, S.S. Effect of Gas Counter Pressure on the Surface Roughness, Morphology, and Tensile Strength between Microcellular and Conventional Injection-Molded PP Parts. Polymers 2022, 14, 1078. [Google Scholar] [CrossRef]
- Lee, J.W.S.; Lee, R.E.; Wang, J.; Jung, P.U.; Park, C.B. Study of the foaming mechanisms associated with gas counter pressure and mold opening using the pressure profiles. Chem. Eng. Sci. 2017, 167, 105–119. [Google Scholar] [CrossRef]
- Kaewmesri, W.; Rachtanapun, P.; Pumchusak, J. Effect of Solvent Plasticization on Polypropylene Microcellular Foaming Process and Foam Characteristics. J. Appl. Polym. Sci. 2008, 107, 63–70. [Google Scholar] [CrossRef]
- Chen, S.C.; Yang, J.P.; Hwang, J.S.; Chung, M.H. Effects of process conditions on the mechanical properties of microcellular injection molded polycarbonate parts. J. Reinf. Plast. Compos. 2008, 27, 153–165. [Google Scholar] [CrossRef]
- Ishikawa, T.; Taki, K.; Ohshima, M. Visual Observation and Numerical Studies of N2 vs. CO2 Foaming Behavior in Core-Back Foam Injection Molding Takeshi. Polym. Eng. Sci. 2012, 52, 875–883. [Google Scholar] [CrossRef]
- Mi, H.-Y.; Jing, X.; Peng, J.; Turng, L.-S.; Peng, X.-F. Influence and prediction of processing parameters on the properties of microcellular injection molded thermoplastic polyurethane based on an orthogonal array test. J. Cell. Plast. 2013, 49, 439–458. [Google Scholar] [CrossRef]
- Xi, Z.; Chen, J.; Liu, T.; Zhao, L.; Turng, L.-S. Experiment and simulation of foaming injection molding of polypropylene/nano-calcium carbonate composites by supercritical carbon dioxide. Chin. J. Chem. Eng. 2015, 24, 180–189. [Google Scholar] [CrossRef]
- Wang, L.; Wakatsuki, Y.; Hikima, Y.; Ohshima, M.; Yusa, A.; Uezono, H.; Naitou, A. Preparation of microcellular injection-molded foams using different types of low-pressure gases via a new foam injection molding technology. Ind. Eng. Chem. Res. 2019, 58, 17824–17832. [Google Scholar] [CrossRef]
- Zhao, J.; Qiao, Y.; Wang, G.; Wang, C.; Park, C.B. Lightweight and tough PP/talc composite foam with bimodal nanoporous structure achieved by microcellular injection molding. Mater. Des. 2020, 195, 109051. [Google Scholar] [CrossRef]
- Kumar, V.; Suh, N.P. A process for making microcellular parts. Polym. Eng. Sci. 1990, 30, 1323–1329. [Google Scholar] [CrossRef]
- Haq, A.N.; Marimuthu, P.; Jeyapaul, R. Multi response optimization of machining parameters of drilling Al/SiC metal matrix composite using grey relational analysis in the Taguchi method. Int. J. Adv. Manuf. Technol. 2008, 37, 250–255. [Google Scholar] [CrossRef]
- Reglero Ruiz, J.A.; Vincent, M.; Agassant, J.-F.; Claverie, A.; Huck, S. Morphological analysis of microcellular PP produced in a core-back injection process using chemical blowing agents and gas counter pressure. Polym. Eng. Sci. 2015, 55, 2465–2473. [Google Scholar] [CrossRef]
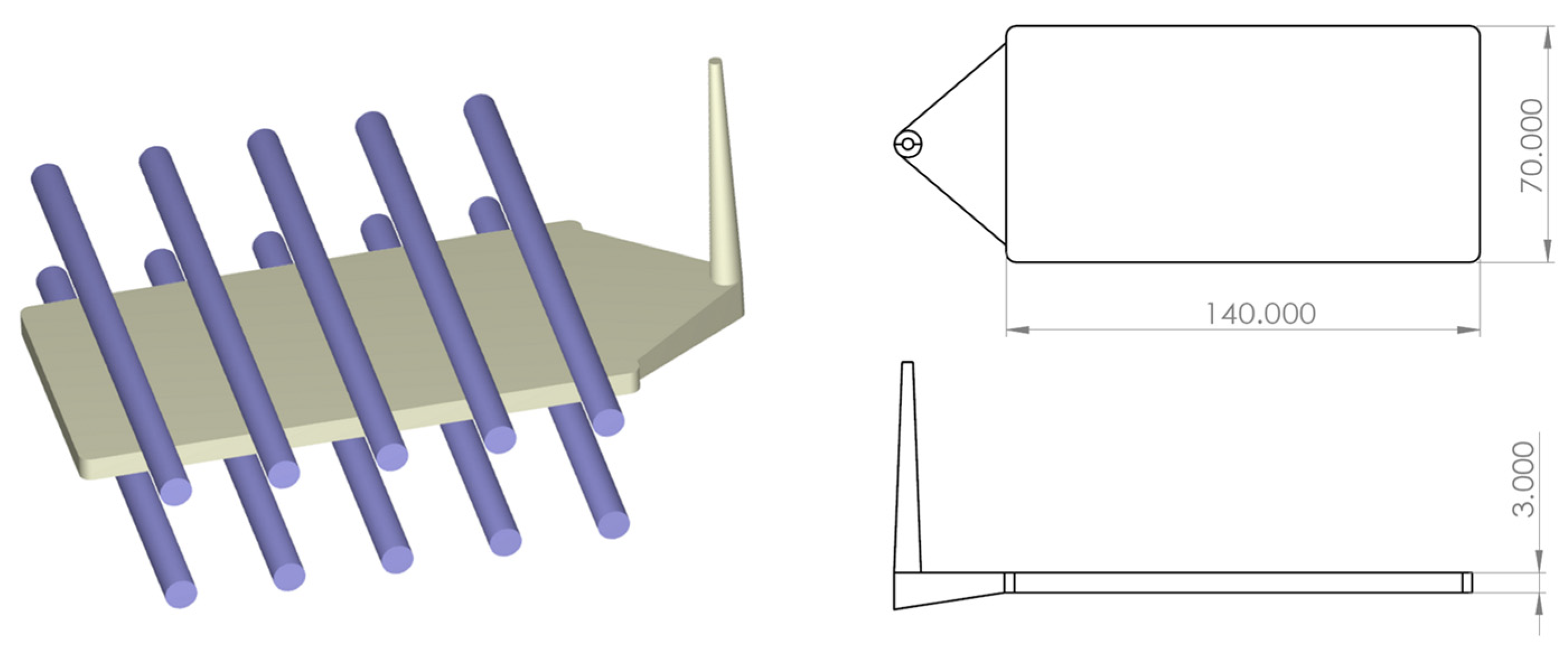
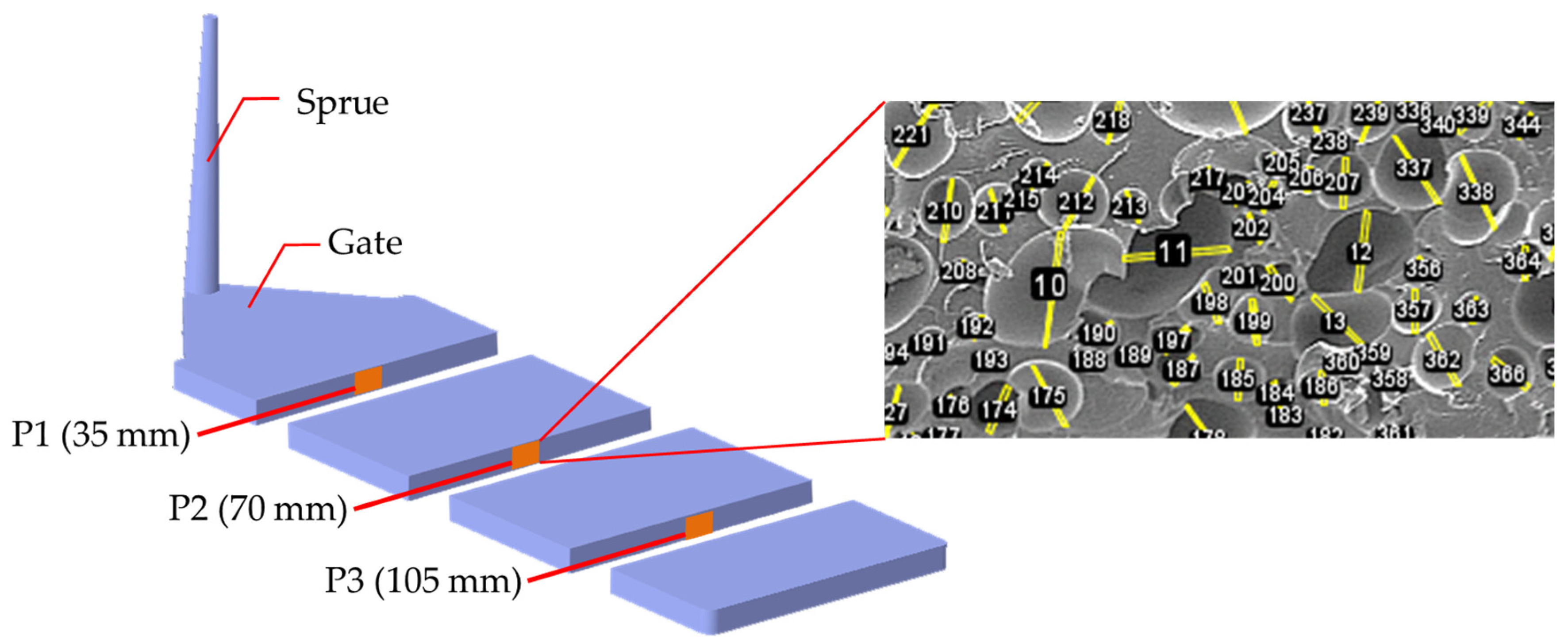


| Research | Blowing Agent | Material | Advanced Features | Processing Parameters | Cell Size (µm) | Weight Reduction |
|---|---|---|---|---|---|---|
| Kaewmesri et al. [22] | CO2 | PP | foaming temperature | 3–8 | ||
| Chen et al. [23] | N2 | PC | melt and mold temperature, microcellular plasticizing pressure, injection rate, SCF level, shot size, part thickness | Basic cell size | 40% | |
| Ishikawa et al. [24] | N2, CO2 | PP | core-back | core-back rate, gas type, gas injection time, gas flow rate | 100–400 | |
| Mi et al. [25] | CO2 | TPU | melt temperature, SCF content, injection volume and speed | 190 | 30% | |
| Sun et al. [13] | N2, CO2 | LDPE, HDPS, PP | SCF-laden pellet | gas type, gas content | 30–120 | 15–18% |
| Xi et al. [26] | CO2 | iPP and iPP/nano-CaCO3 | injection speed, mold temperature, gas content | 25–50 | 15% | |
| Wang et al. [16] | N2, CO2 | PP | RIC-FIM II | delivery pressure, void fraction, gas type | N2: 15–30 CO2: 15–20 | |
| Yeh et al. [17] | CO2 | TPU | foaming temperature, clay content | 0.45 | ||
| Wang et al. [27] | N2, CO2, Ar, He | PP | New FIM, low pressure, core-back | dwelling time, gas blowing agent, core-back distance | N2: 28 CO2: 14 Ar: 18 He: 32 | |
| Zhao et al. [28] | CO2 | PP/Talc | in situ fibrillation, toughening method | - | 82 | 15% |
| Run | SCF Dosage (wt%) | Melt Temperature (°C) | Mold Temperature (°C) | Injection Speed (mm/s) |
|---|---|---|---|---|
| 1 | 1 | 290 | 80 | 50 |
| 2 | 1 | 300 | 90 | 75 |
| 3 | 1 | 310 | 100 | 100 |
| 4 | 1.5 | 290 | 90 | 100 |
| 5 | 1.5 | 300 | 100 | 50 |
| 6 | 1.5 | 310 | 80 | 75 |
| 7 | 2 | 290 | 100 | 75 |
| 8 | 2 | 300 | 80 | 100 |
| 9 | 2 | 310 | 90 | 50 |
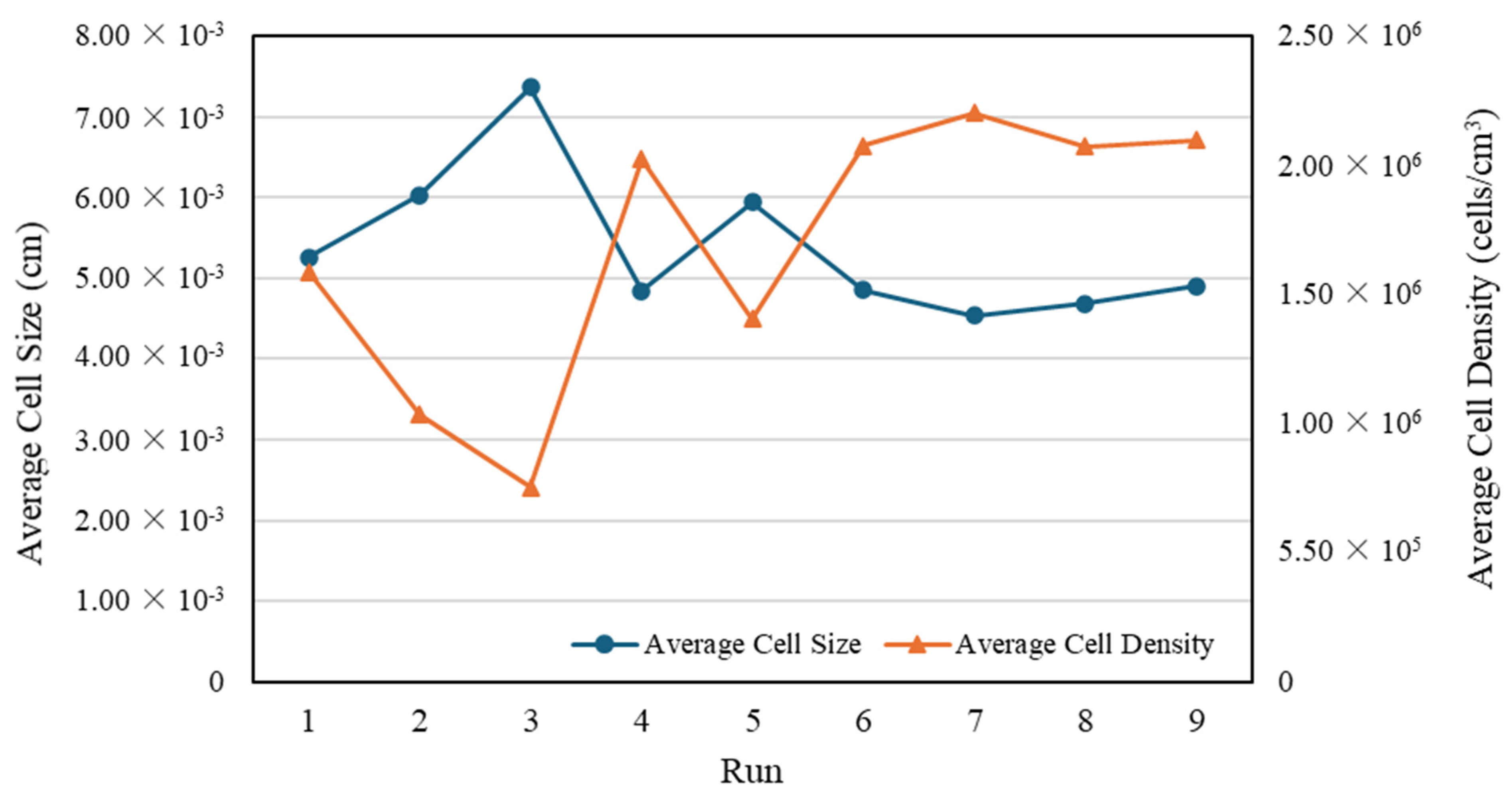
| Run | Cell Size | Cell Density | ||||||
|---|---|---|---|---|---|---|---|---|
| P1 | P2 | P3 | Average | P1 | P2 | P3 | Average | |
| 1 | 5.882 | 5.565 | 4.332 | 5.259 | 0.910 | 1.453 | 2.400 | 1.588 |
| 2 | 5.043 | 7.379 | 5.663 | 6.028 | 0.487 | 0.862 | 1.756 | 1.035 |
| 3 | 7.061 | 8.693 | 6.337 | 7.363 | 0.804 | 0.671 | 0.792 | 0.756 |
| 4 | 5.264 | 4.125 | 5.128 | 4.839 | 0.938 | 3.159 | 1.971 | 2.022 |
| 5 | 8.204 | 5.487 | 4.121 | 5.937 | 0.379 | 1.123 | 2.715 | 1.405 |
| 6 | 4.410 | 4.556 | 5.591 | 4.852 | 2.673 | 1.992 | 1.563 | 2.076 |
| 7 | 4.641 | 4.099 | 4.880 | 4.540 | 2.366 | 2.723 | 1.518 | 2.202 |
| 8 | 5.998 | 3.910 | 4.163 | 4.691 | 1.190 | 1.762 | 3.267 | 2.073 |
| 9 | 5.163 | 3.799 | 5.744 | 4.902 | 1.785 | 3.075 | 1.430 | 2.096 |
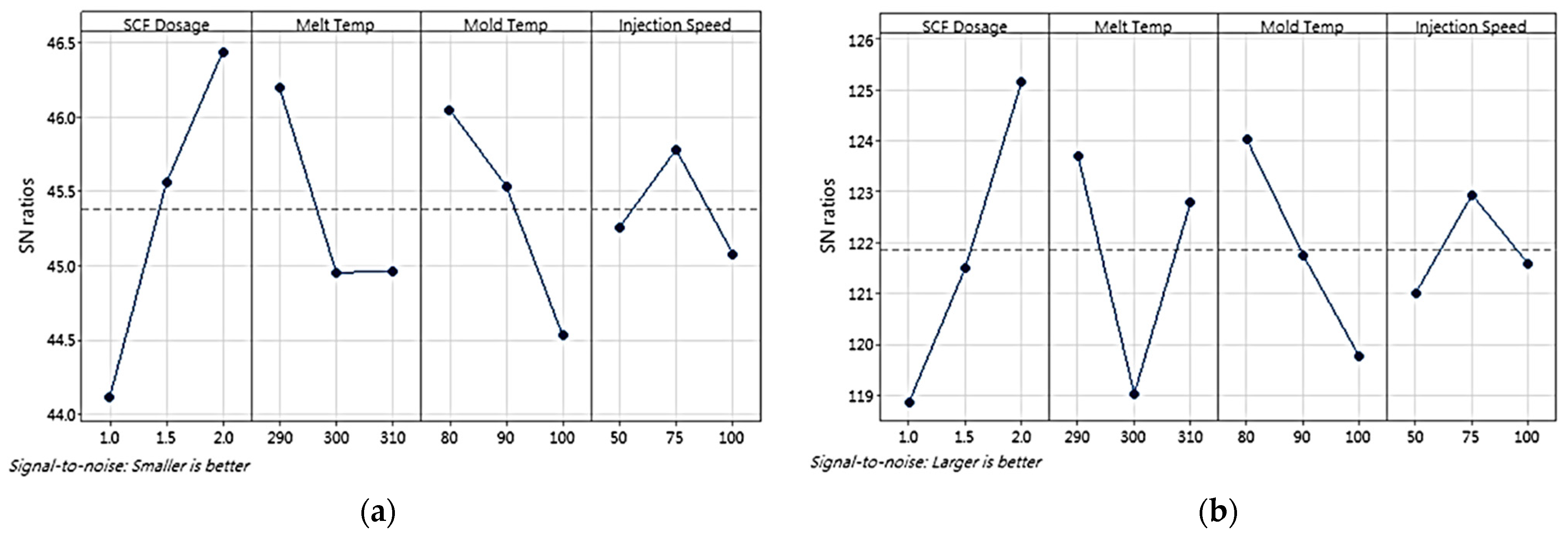
| Source | Degrees of Freedom (DFs) | Contribution | Adjusted Sums of Squares (SS) | Adjusted Mean Squares (MS) | F-Value | p-Value |
|---|---|---|---|---|---|---|
| Regression | 4 | 91.16% | 0.000006 | 0.000002 | 10.31 | 0.022 |
| SCF Dosage | 1 | 51.10% | 0.000003 | 0.000003 | 23.11 | 0.009 |
| Melt Temperature | 1 | 15.38% | 0.000001 | 0.000001 | 6.96 | 0.058 |
| Mold Temperature | 1 | 23.10% | 0.000002 | 0.000002 | 10.45 | 0.032 |
| Injection Speed | 1 | 1.58% | 0.000000 | 0.000000 | 0.71 | 0.446 |
| Error | 4 | 8.84% | 0.000001 | 0.000000 | ||
| Total | 8 | 100.00% |
| Source | DFs | Contribution | Adj. SS | Adj. MS | F-Value | p-Value |
|---|---|---|---|---|---|---|
| Regression | 4 | 87.46% | 1.95 × 1012 | 4.87 × 1011 | 6.97 | 0.043 |
| SCF Dosage | 1 | 67.06% | 1.49 × 1012 | 1.49 × 1012 | 21.39 | 0.010 |
| Melt Temperature | 1 | 5.85% | 1.30 × 1011 | 1.30 × 1011 | 1.87 | 0.244 |
| Mold Temperature | 1 | 14.12% | 3.14 × 1011 | 3.14 × 1011 | 4.50 | 0.101 |
| Injection Speed | 1 | 0.43% | 9.50 × 109 | 9.50 × 109 | 0.14 | 0.731 |
| Error | 4 | 12.54% | 2.79 × 1011 | 6.98 × 1010 | ||
| Total | 8 | 100.00% |
| Run | GRG | Rank |
|---|---|---|
| 1 | 0.587 | 6 |
| 2 | 0.459 | 8 |
| 3 | 0.363 | 9 |
| 4 | 0.672 | 5 |
| 5 | 0.570 | 7 |
| 6 | 0.704 | 3 |
| 7 | 0.745 | 2 |
| 8 | 0.752 | 1 |
| 9 | 0.699 | 4 |
| Item | Position | Run 8 | Validation Test | % Improvement |
|---|---|---|---|---|
| Cell size (cm) | P1 | 5.998 × 10−3 | 3.847 × 10−3 | 35.87% |
| P2 | 3.910 × 10−3 | 3.813 × 10−3 | 2.50% | |
| P3 | 4.163 × 10−3 | 3.848 × 10−3 | 7.58% | |
| Average | 4.691 × 10−3 | 3.836 × 10−3 | 18.23% | |
| Cell density (cells/cm3) | P1 | 1.190 × 106 | 2.661 × 106 | 123.65% |
| P2 | 1.762 × 106 | 3.791 × 106 | 115.09% | |
| P3 | 3.267 × 106 | 5.464 × 106 | 67.23% | |
| Average | 2.073 × 106 | 3.972 × 106 | 91.59% |
| Run | GCP (Bar) | Holding Time (s) |
|---|---|---|
| 1 | 25 | 1 |
| 2 | 25 | 2 |
| 3 | 50 | 1 |
| 4 | 50 | 2 |
| 5 | 75 | 1 |
| 6 | 75 | 2 |
| Run | GCP (Bar) | Holding Time (s) | Actual Weight Reduction |
|---|---|---|---|
| 0 | 0 | 0 | 35% |
| 1 | 25 | 1 | 32% |
| 2 | 25 | 2 | 28% |
| 3 | 50 | 1 | 28% |
| 4 | 50 | 2 | 22% |
| 5 | 75 | 1 | 21% |
| 6 | 75 | 2 | 17% |
| Run | Cell Size | Cell Density | ||||||
|---|---|---|---|---|---|---|---|---|
| P1 | P2 | P3 | Average | P1 | P2 | P3 | Average | |
| 1 | 2.992 | 3.648 | 4.984 | 3.875 | 3.157 | 2.363 | 1.583 | 2.368 |
| 2 | 2.815 | 2.958 | 2.607 | 2.793 | 3.785 | 4.097 | 6.574 | 4.819 |
| 3 | 2.514 | 2.989 | 3.099 | 2.867 | 6.566 | 6.210 | 6.361 | 6.379 |
| 4 | 3.099 | 2.339 | 2.340 | 2.592 | 5.865 | 4.154 | 7.028 | 5.682 |
| 5 | 2.301 | 2.205 | 2.760 | 2.422 | 7.425 | 7.236 | 6.151 | 6.937 |
| 6 | 2.055 | 2.221 | 2.006 | 2.094 | 8.204 | 7.572 | 8.351 | 8.042 |
| Source | DF | Contribution | Adj. SS | Adj. MS | F-Value | p-Value |
|---|---|---|---|---|---|---|
| Regression | 2 | 88.63% | 0.000002 | 0.000001 | 11.69 | 0.038 |
| GCP | 1 | 62.93% | 0.000001 | 0.000001 | 16.60 | 0.027 |
| Holding Time | 1 | 25.69% | 0.000000 | 0.000000 | 6.78 | 0.080 |
| Error | 3 | 11.37% | 0.000000 | 0.000000 | ||
| Total | 5 | 100.00% |
| Source | DF | Contribution | Adj. SS | Adj. MS | F-Value | p-Value |
|---|---|---|---|---|---|---|
| Regression | 2 | 85.47% | 1.65 × 1013 | 8.27 × 1012 | 8.82 | 0.055 |
| GCP | 1 | 78.43% | 1.52 × 1013 | 1.52 × 1013 | 16.19 | 0.028 |
| Holding Time | 1 | 7.04% | 1.36 × 1012 | 1.36 × 1012 | 1.45 | 0.314 |
| Error | 3 | 14.53% | 2.81 × 1012 | 9.38 × 1011 | ||
| Total | 5 | 100.00% |
| Item | Position | Optimum First Stage | Optimum Second Stage | % Improvement |
|---|---|---|---|---|
| Cell size (cm) | P1 | 3.847 × 10−3 | 2.301 × 10−3 | 40.19% |
| P2 | 3.813 × 10−3 | 2.221 × 10−3 | 41.74% | |
| P3 | 3.848 × 10−3 | 2.006 × 10−3 | 47.87% | |
| Average | 3.836 × 10−3 | 2.094 × 10−3 | 45.41% | |
| Cell density (cells/cm3) | P1 | 2.661 × 106 | 7.425 × 106 | 179.02% |
| P2 | 3.791 × 106 | 7.572 × 106 | 99.74% | |
| P3 | 5.464 × 106 | 8.351 × 106 | 52.84% | |
| Average | 3.972 × 106 | 8.042 × 106 | 102.48% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Agustion, Y.H.; Chen, S.-C.; Feng, C.-T.; Iskandar, B.P. High-Quality Foaming and Weight Reduction in Microcellular-Injection-Molded Polycarbonate Using Supercritical Fluid Carbon Dioxide under Gas Counter Pressure. Polymers 2024, 16, 2674. https://doi.org/10.3390/polym16182674
Agustion YH, Chen S-C, Feng C-T, Iskandar BP. High-Quality Foaming and Weight Reduction in Microcellular-Injection-Molded Polycarbonate Using Supercritical Fluid Carbon Dioxide under Gas Counter Pressure. Polymers. 2024; 16(18):2674. https://doi.org/10.3390/polym16182674
Chicago/Turabian StyleAgustion, Yogi Hendra, Shia-Chung Chen, Ching-Te Feng, and Bermawi Priyatna Iskandar. 2024. "High-Quality Foaming and Weight Reduction in Microcellular-Injection-Molded Polycarbonate Using Supercritical Fluid Carbon Dioxide under Gas Counter Pressure" Polymers 16, no. 18: 2674. https://doi.org/10.3390/polym16182674
APA StyleAgustion, Y. H., Chen, S.-C., Feng, C.-T., & Iskandar, B. P. (2024). High-Quality Foaming and Weight Reduction in Microcellular-Injection-Molded Polycarbonate Using Supercritical Fluid Carbon Dioxide under Gas Counter Pressure. Polymers, 16(18), 2674. https://doi.org/10.3390/polym16182674








