Flexible Positive Temperature Coefficient Composites (PVAc/EVA/GP-CNF) with Room Temperature Curie Point
Abstract
1. Introduction
2. Experimental
2.1. Materials
2.2. Sample Preparation
2.3. Characterization
3. Results and Discussion
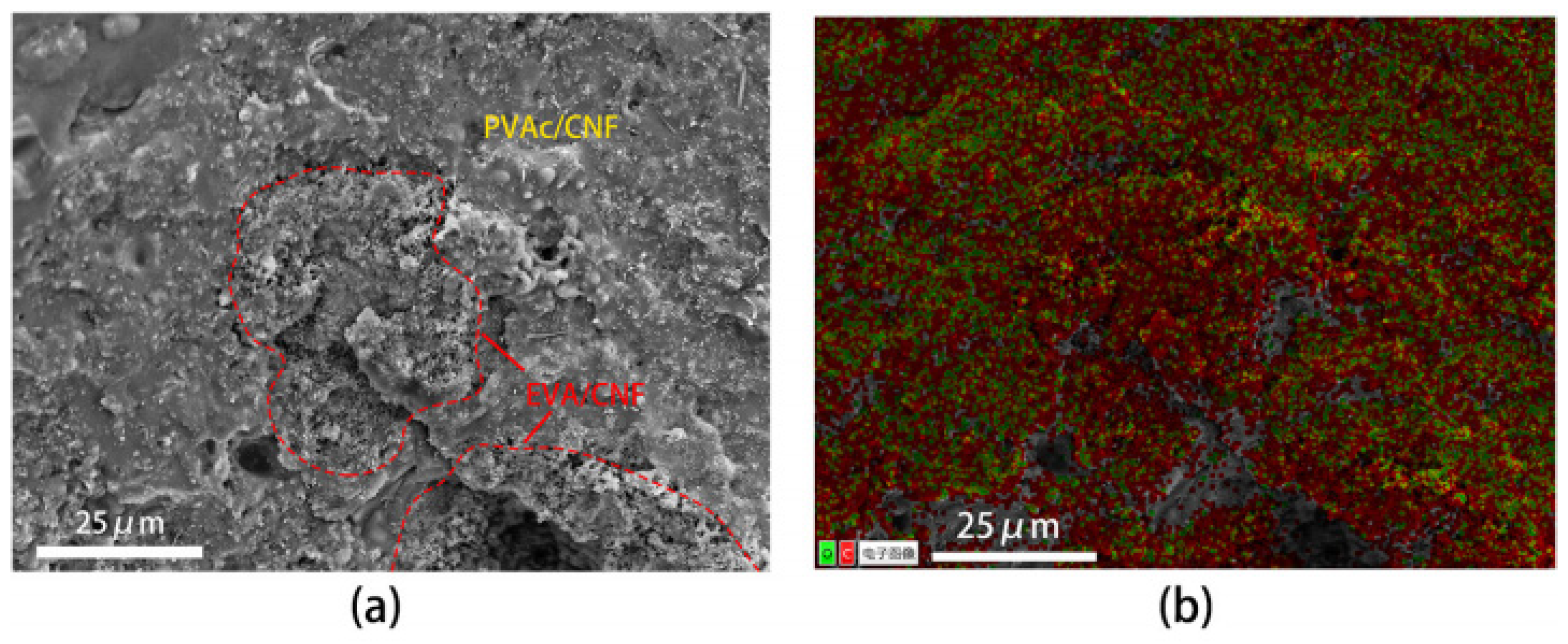
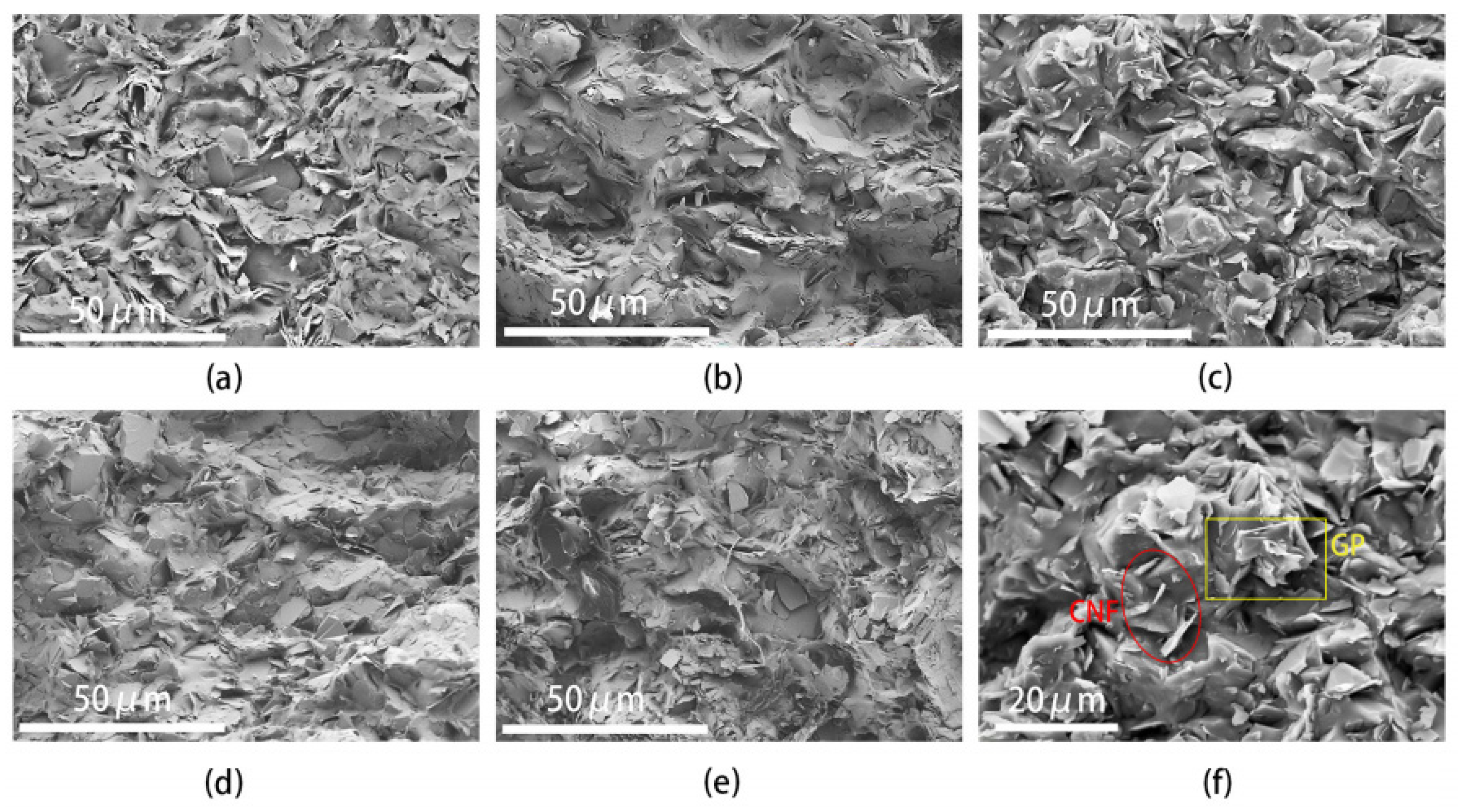
3.1. Microscopic Morphological Analysis of PVAc0.4/EVA0.6/GP-CNF Conductive Composites
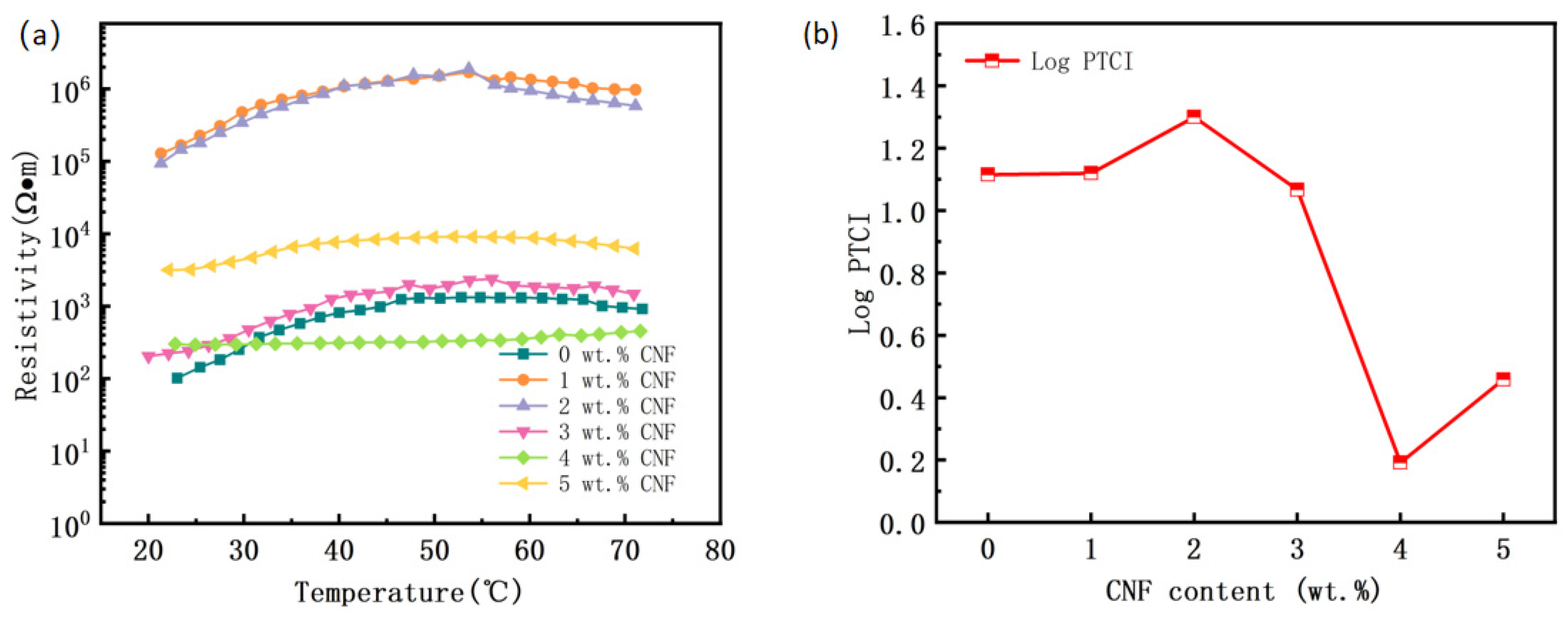
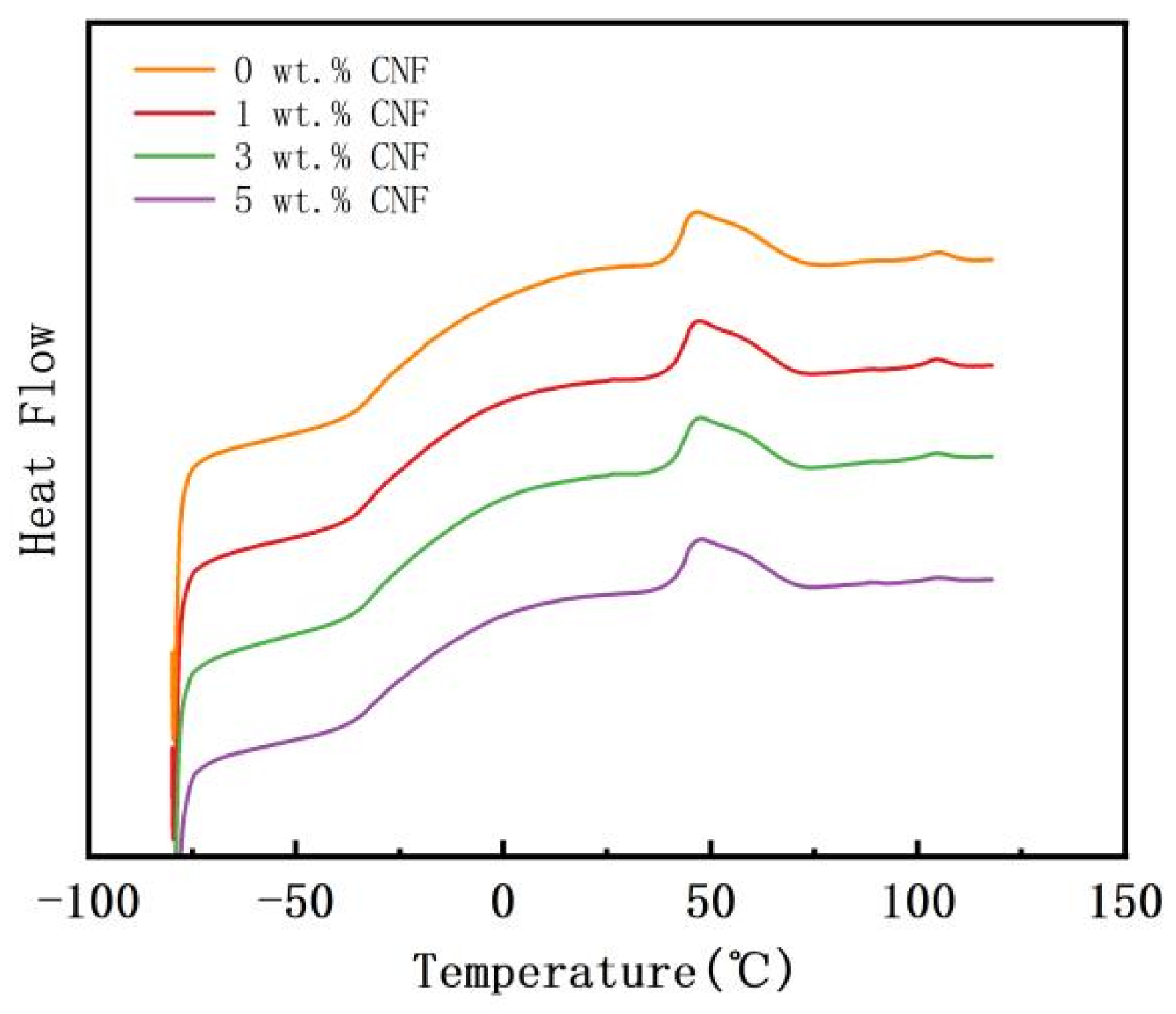
3.2. Influence of Mixed Fillers on Electrical and Thermal Resistance Properties
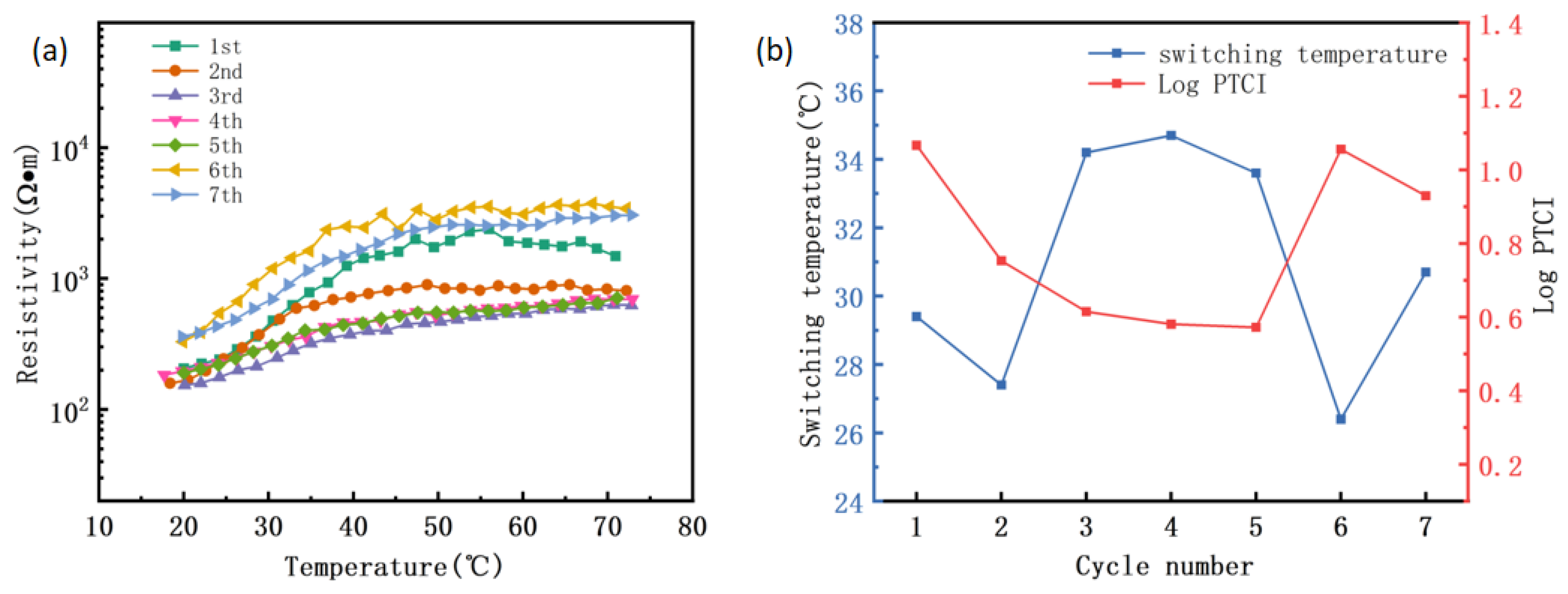
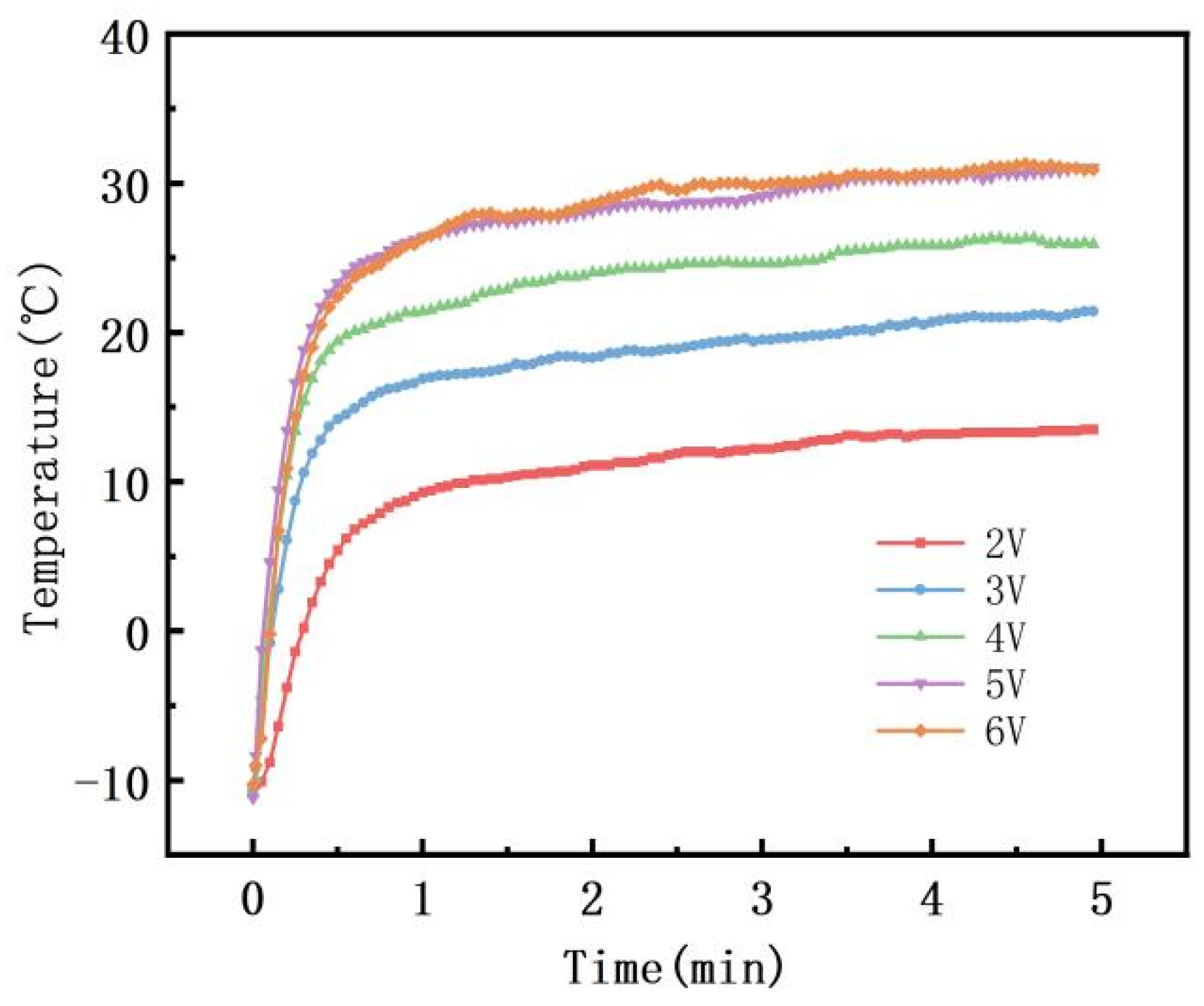
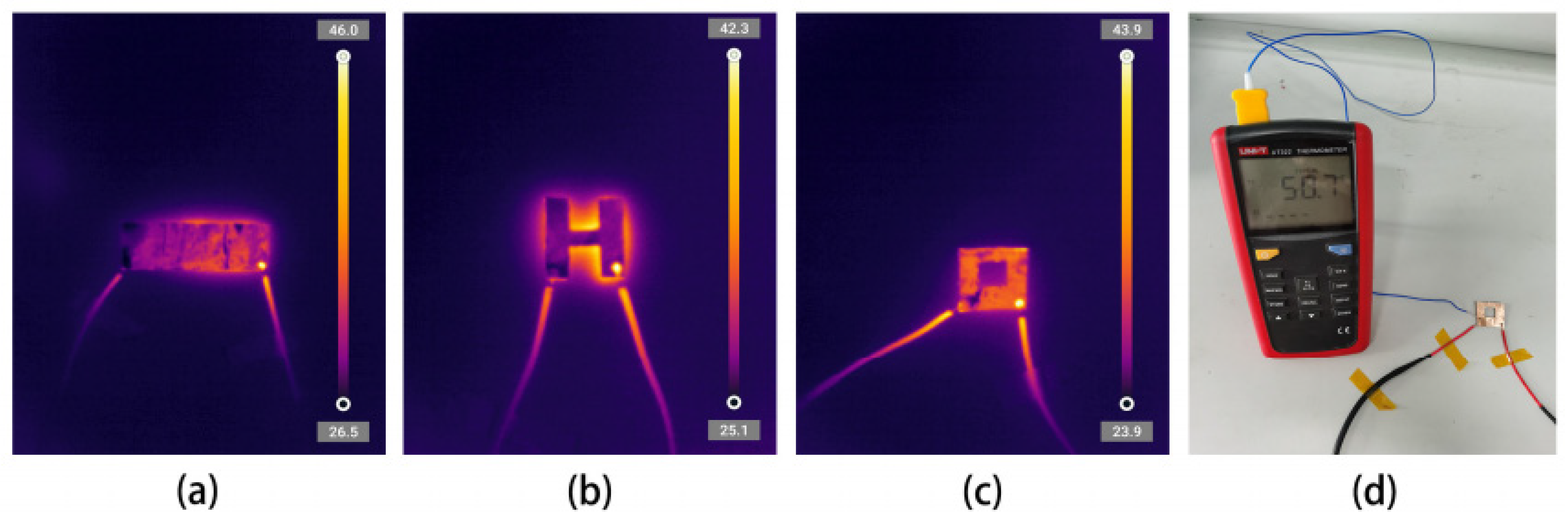
3.3. Impact of Mixed Fillers on Self-Limiting Performance
- (1)
- Room temperature ambient heating test
- (2)
- Low-temperature environment heating test
- (3)
- Breakage heating test
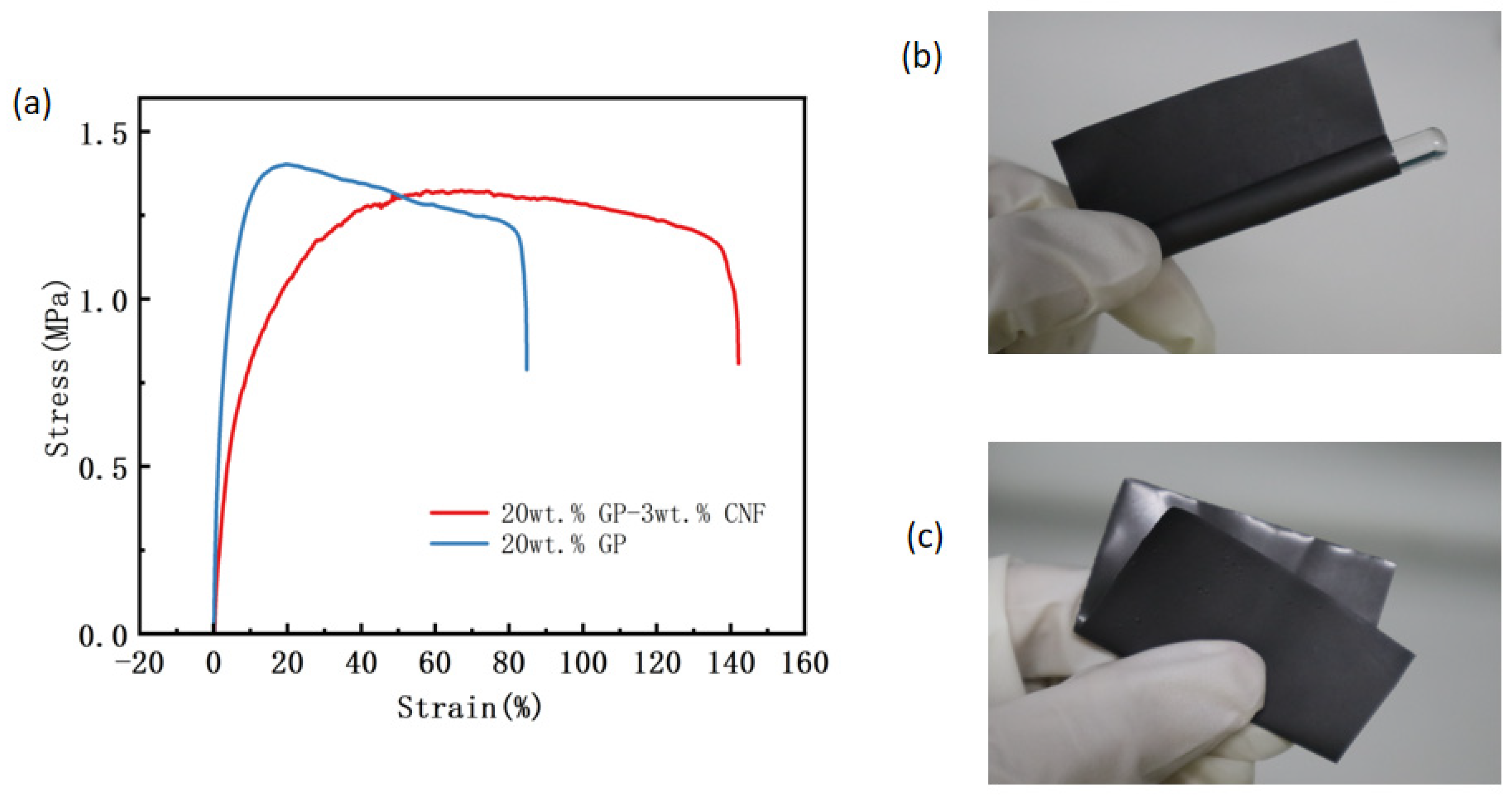
3.4. Effect of Mixed Filler on Mechanical Properties
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Liu, Y.; Zhang, H.; Porwal, H.; Busfield, J.J.; Peijs, T.; Bilotti, E. Pyroresistivity in conductive polymer composites: A perspective on recent advances and new applications. Polym. Int. 2018, 68, 299–305. [Google Scholar] [CrossRef]
- Bautista-Quijano, J.R.; Pötschke, P.; Brunig, H.; Heinrich, G. Strain sensing, electrical and mechanical properties of polycarbonate/multiwall carbon nanotubemonofilament fibers fabricated by melt spinning. Polymer 2016, 82, 181–189. [Google Scholar] [CrossRef]
- Yu, F.; Deng, H.; Zhang, Q.; Wang, K.; Zhang, C.; Chen, F.; Fu, Q. Anisotropic multilayer conductive networks in carbon nanotubes filled polyethylene/polypropylene blends obtained through high speed thin wall injection molding. Polymer 2013, 54, 6425–6436. [Google Scholar] [CrossRef]
- Kim, D.C.; Shim, H.J.; Lee, W.; Koo, J.H.; Kim, D.-H. Material-Based Approaches for the Fabrication of Stretchable Electronics. Adv. Mater. 2020, 32, e1902743. [Google Scholar] [CrossRef] [PubMed]
- Beletskii, E.; Ershov, V.; Danilov, S.; Lukyanov, D.; Alekseeva, E.; Levin, O. Resistivity-Temperature Behavior of Intrinsically Conducting Bis(3-methoxysalicylideniminato)nickel Polymer. Polymers 2021, 12, 2925. [Google Scholar] [CrossRef]
- Liu, Y.; Zhang, H.; Porwal, H.; Tu, W.; Wan, K.; Evans, J.; Newton, M.; Busfield, J.J.C.; Peijs, T.; Bilotti, E. Tailored pyroresistive performance and flexibility by introducing a secondary thermoplastic elastomeric phase into graphene nanoplatelet (GNP) filled polymer composites for self-regulating heating devices. J. Mater. Chem. C 2018, 6, 2760–2768. [Google Scholar] [CrossRef]
- Zhang, L.; Du, W.; Nautiyal, A.; Liu, Z.; Zhang, X. Recent progress on nanostructured conducting polymers and composites: Synthesis, application and future aspects. Sci. China Mater. 2018, 61, 303–352. [Google Scholar] [CrossRef]
- Feng, J.; Chan, C.-M. Positive and negative temperature coefficient effects of an alternating copolymer of tetrafluoroethylene–ethylene containing carbon black-filled HDPE particles. Polymer 2000, 41, 7279–7282. [Google Scholar] [CrossRef]
- Zhang, X.; Zheng, S.; Zheng, X.; Liu, Z.; Yang, W.; Yang, M. Distinct positive temperature coefficient effect of polymer–carbon fiber composites evaluated in terms of polymer absorption on fiber surface. Phys. Chem. Chem. Phys. 2016, 18, 8081–8087. [Google Scholar] [CrossRef]
- Xiang, Z.; Chen, T.; Li, Z.; Bian, X. Negative Temperature Coefficient of Resistivity in Lightweight Conductive Carbon Nanotube/Polymer Composites. Macromol. Mater. Eng. 2009, 294, 91–95. [Google Scholar] [CrossRef]
- Zhang, X.; Zheng, S.; Zou, H.; Zheng, X.; Liu, Z.; Yang, W.; Yang, M. Two-step positive temperature coefficient effect with favorable reproducibility achieved by specific “island-bridge” electrical conductive networks in HDPE/PVDF/CNF composite. Compos. Part A Appl. Sci. Manuf. 2017, 94, 21–31. [Google Scholar] [CrossRef]
- Zhang, R.; Tang, P.; Li, J.; Xu, D.; Bin, Y. Study on filler content dependence of the onset of positive temperature coefficient (PTC) effect of electrical resistivity for UHMWPE/LDPE/CF composites based on their DC and AC electrical behaviors. Polymer 2014, 55, 2103–2112. [Google Scholar] [CrossRef]
- Zha, J.-W.; Li, W.-K.; Liao, R.-J.; Bai, J.; Dang, Z.-M. High performance hybrid carbon fillers/binary–polymer nanocomposites with remarkably enhanced positive temperature coefficient effect of resistance. J. Mater. Chem. A 2013, 1, 843–851. [Google Scholar] [CrossRef]
- Chen, L.; Wu, X.; Zhang, X.-F.; Zhang, J.-M. Enhanced Reproducibility of Positive Temperature Coefficient Effect of CB/HDPE/PVDF Composites with the Addition of Ionic Liquid. Chin. J. Polym. Sci. 2021, 39, 228–236. [Google Scholar] [CrossRef]
- Seo, M.-K.; Rhee, K.-Y.; Park, S.-J. Influence of electro-beam irradiation on PTC/NTC behaviors of carbon blacks/HDPE conducting polymer composites. Curr. Appl. Phys. 2011, 11, 428–433. [Google Scholar] [CrossRef]
- Wang, Y.-H.; Yang, Y.-T. Three-dimensional transient cooling simulations of a portable electronic device using PCM (phase change materials) in multi-fin heat sink. Energy 2011, 36, 5214–5224. [Google Scholar] [CrossRef]
- Etminan, E.; Alimohammadi, M.; Barani, E.; Taheri, A.; Passandideh-Fard, M.; Sardarabadi, M. On the performance of an innovative electronic chipset thermal management module based on energy storage unit concept utilizing nano-additive phase change material (NPCM). J. Energy Storage 2022, 50, 104244. [Google Scholar] [CrossRef]
- Wang, R.-J.; Cheng, W.-L. A novel flexible room temperature positive temperature coefficient material for thermal management. Adv. Compos. Hybrid Mater. 2019, 2, 83–92. [Google Scholar] [CrossRef]
- Jeon, J.; Lee, H.-B.-R.; Bao, Z. Flexible Wireless Temperature Sensors Based on Ni Microparticle-Filled Binary Polymer Composites. Adv. Mater. 2013, 25, 850–855. [Google Scholar] [CrossRef]
- Lai, F.; Wang, B.; Zhang, P. Enhanced positive temperature coefficient in amorphous PS/CSPE-MWCNT composites with low percolation threshold. J. Appl. Polym. Sci. 2019, 136, 47053. [Google Scholar] [CrossRef]
- Yu, A.; Li, Q. Studies on preparation and thermal control behavior of hybrid fillers/binary-polymer composites with positive temperature coefficient characteristic. Sci. China Technol. Sci. 2021, 64, 1447–1458. [Google Scholar] [CrossRef]
- Cheng, W.-L.; Yuan, S.; Song, J.-L. Studies on preparation and adaptive thermal control performance of novel PTC (positive temperature coefficient) materials with controllable Curie temperatures. Energy 2014, 74, 447–454. [Google Scholar] [CrossRef]
- Luo, X.L.; Schubert, D.W. Positive temperature coefficient (PTC) materials based on amorphous poly (methyl methacrylate) with ultrahigh PTC intensity, tunable switching temperature and good reproducibility. Mater. Today Commun. 2022, 30, 103078. [Google Scholar] [CrossRef]
- Chen, L.; Zhang, J. Designs of conductive polymer composites with exceptional reproducibility of positive temperature coefficient effect: A review. J. Appl. Polym. Sci. 2021, 138, 49677. [Google Scholar] [CrossRef]
- Liu, P.; Liu, J.; Zhu, X.; Wu, C.; Liu, Y.; Pan, W.; Zhao, J.; Guo, X.; Liu, C.; Huang, Y.; et al. A highly adhesive flexible strain sensor based on ultra-violet adhesive filled by graphene and carbon black for wearable monitoring. Compos. Sci. Technol. 2019, 192, 107771. [Google Scholar] [CrossRef]
- Duy, L.T.; Trung, T.Q.; Hanif, A.; Siddiqui, S.; Roh, E.; Lee, W.; Lee, N.-E. A stretchable and highly sensitive chemical sensor using multilayered network of polyurethane nanofibres with self-assembled reduced graphene oxide. 2D Mater. 2017, 4, 025062. [Google Scholar] [CrossRef]
- Yokota, T.; Inoue, Y.; Terakawa, Y.; Reeder, J.; Kaltenbrunner, M.; Ware, T.; Yang, K.; Mabuchi, K.; Murakawa, T.; Sekino, M.; et al. Ultraflexible, large-area, physiological temperature sensors for multipoint measurements. Proc. Natl. Acad. Sci. USA 2015, 112, 14533–14538. [Google Scholar] [CrossRef]
- Zhang, Q.-H.; Chen, D.-J. Percolation threshold and morphology of composites of conducting carbon black/polypropylene/EVA. J. Mater. Sci. 2004, 39, 1751–1757. [Google Scholar] [CrossRef]
- Li, J.; Chang, C.; Li, X.; Li, Y.; Guan, G. A New Thermal Controlling Material with Positive Temperature Coefficient for Body Warming: Preparation and Characterization. Materials 2019, 12, 1758. [Google Scholar] [CrossRef]
- Yu, Y.; Jiang, S.; Zhou, W.; Miao, X.; Zeng, Y.; Zhang, G.; Zhang, Y.; Zhang, Q.; Zhao, H. Ultrafast room temperature wiping-rubbing fabrication of graphene nanosheets as flexible transparent conductive films with high surface stability. Appl. Phys. Lett. 2009, 101, 023119. [Google Scholar] [CrossRef]
- Jara, A.D.; Betemariam, A.; Woldetinsae, G.; Kim, J.Y. Purification, application and current market trend of natural graphite: A review. Int. J. Min. Sci. Technol. 2019, 29, 671–689. [Google Scholar] [CrossRef]
- Chehreh Chelgani, S.; Rudolph, M.; Kratzsch, R.; Sandmann, D.; Gutzmer, J. A Review of Graphite Beneficiation Techniques. Miner. Process. Extr. Met. Rev. 2016, 37, 58–68. [Google Scholar] [CrossRef]
- Zhao, Z.; Chen, H.; Liu, X.; Wang, Z.; Zhu, Y.; Zhou, Y. The development of electric heating coating with temperature controlling capability for anti-icing/de-icing. Cold Reg. Sci. Technol. 2021, 184, 103234. [Google Scholar] [CrossRef]
- Liu, K.; Xu, Z.; Mei, J.; Han, J.; Zheng, F.; Wang, H.; Huang, Y.; Wu, Q.; Qin, G.; Jiang, J.; et al. Strategies for improving positive temperature effects in conductive polymer composites—A review. J. Mater. Chem. C 2023, 11, 4966–4992. [Google Scholar] [CrossRef]
- Zha, J.-W.; Wu, D.-H.; Yang, Y.; Wu, Y.-H.; Li, R.K.Y.; Dang, Z.-M. Enhanced positive temperature coefficient behavior of the high-density polyethylene composites with multi-dimensional carbon fillers and their use for temperature-sensing resistors. RSC Adv. 2017, 7, 11338–11344. [Google Scholar] [CrossRef]
- Chen, L.; Hou, J.; Chen, Y.; Wang, H.; Duan, Y.; Zhang, J. Synergistic effect of conductive carbon black and silica particles for improving the pyroresistive properties of high density polyethylene composites. Compos. Part B Eng. 2019, 178, 107465. [Google Scholar] [CrossRef]
- Mamunya, Y.; Maruzhenko, O.; Kolisnyk, R.; Iurzhenko, M.; Pylypenko, A.; Masiuchok, O.; Godzierz, M.; Krivtsun, I.; Trzebicka, B.; Pruvost, S. Pyroresistive Properties of Composites Based on HDPE and Carbon Fillers. Polymers 2023, 15, 2105. [Google Scholar] [CrossRef] [PubMed]
- Jiang, Y.; Liu, L.; Yan, J.; Wu, Z. Room-to-low temperature thermo-mechanical behavior and corresponding constitutive model of liquid oxygen compatible epoxy composites. Compos. Sci. Technol. 2024, 245, 110357. [Google Scholar] [CrossRef]
- Li, H.; Zhang, Y.; Tai, Y.; Zhu, X.; Qi, X.; Zhou, L.; Li, Z.; Lan, H. Flexible transparent electromagnetic interference shielding films with silver mesh fabricated using electric-field-driven microscale 3D printing. Opt. Laser Technol. 2022, 148, 107717. [Google Scholar] [CrossRef]
- Deng, H.; Skipa, T.; Zhang, R.; Lellinger, D.; Bilotti, E.; Alig, I.; Peijs, T. Effect of melting and crystallization on the conductive network in conductive polymer composites. Polymer 2009, 50, 3747–3754. [Google Scholar] [CrossRef]
- Ma, L.-F.; Bao, R.-Y.; Dou, R.; Liu, Z.-Y.; Yang, W.; Xie, B.-H.; Yang, M.-B.; Fu, Q. A high-performance temperature sensitive TPV/CB elastomeric composite with balanced electrical and mechanical properties via PF-induced dynamic vulcanization. J. Mater. Chem. A 2014, 2, 16989–16996. [Google Scholar] [CrossRef]
- Xu, H.-P.; Dang, Z.-M.; Jiang, M.-J.; Yao, S.-H.; Bai, J. Enhanced dielectric properties and positive temperature coefficient effect in the binary polymer composites with surface modified carbon black. J. Mater. Chem. 2008, 18, 229–234. [Google Scholar] [CrossRef]
- Liu, Y.; Zhang, H.; Porwal, H.; Tu, W.; Evans, J.; Newton, M.; Busfield, J.J.C.; Peijs, T.; Bilotti, E. Universal Control on Pyroresistive Behavior of Flexible Self-Regulating Heating Devices. Adv. Funct. Mater. 2017, 27, 1702253. [Google Scholar] [CrossRef]
- He, X.J.; Du, J.H.; Ying, Z.; Cheng, H.M. Positive temperature coefficient effect in multiwalled carbon nanotube/high-density polyethylene composites. Appl. Phys. Lett. 2005, 86, 062112. [Google Scholar] [CrossRef]
- Göldel, A.; Marmur, A.; Kasaliwal, G.R.; Pötschke, P.; Heinrich, G. Shape-Dependent Localization of Carbon Nanotubes and Carbon Black in an Immiscible Polymer Blend during Melt Mixing. Macromolecules 2011, 44, 6094–6102. [Google Scholar] [CrossRef]
- Wu, S. Polymer Interface and Adhesion, 1st ed.; M. Dekker: New York, NY, USA, 1982. [Google Scholar]
- Sumita, M.; Sakata, K.; Asai, S.; Miyasaka, K.; Nakagawa, H. Dispersion of fillers and the electrical conductivity of polymer blends filled with carbon black. Polym. Bull. 1991, 25, 265–271. [Google Scholar] [CrossRef]
- Wu, S. Polar and Nonpolar Interactions in Adhesion. J. Adhes. 1973, 5, 39–55. [Google Scholar] [CrossRef]
- Wu, S.; Li, T.; Tong, Z.; Chao, J.; Zhai, T.; Xu, J.; Yan, T.; Wu, M.; Xu, Z.; Bao, H.; et al. High-Performance Thermally Conductive Phase Change Composites by Large-Size Oriented Graphite Sheets for Scalable Thermal Energy Harvesting. Adv. Mater. 2019, 31, e1905099. [Google Scholar] [CrossRef]
- Zhao, S.G.; Li, G.J.; Liu, H.; Dai, K.; Zheng, G.Q.; Yan, X.R.; Liu, C.; Chen, J.; Shen, C.; Guo, Z. Positive temperature coefficient (ptc) evolution of segregated structural conductive polypropylene nanocomposites with visually traceable carbon black conductive network. Adv. Mater. Interfaces 2017, 4, 10. [Google Scholar] [CrossRef]
- Dai, K.; Zhang, Y.C.; Tang, J.H.; Ji, X.; Li, Z.M. Anomalous attenuation and structural origin of positive temperature coefficient (PTC) effect in a carbon black (CB)/poly(ethylene terephthalate) (PET)/polyethylene (PE) electrically conductive microfibrillar polymer composite with a preferential CB distribution. J. Appl. Polym. Sci. 2012, 125, E561–E570. [Google Scholar]
- Yao, X.; Falzon, B.; Hawkins, S.; Tsantzalis, S. Aligned carbon nanotube webs embedded in a composite laminate: A route towards a highly tunable electro-thermal system. Carbon 2018, 129, 486–494. [Google Scholar] [CrossRef]
- Liu, X.; Chen, H.; Zhao, Z.; Yan, Y.; Zhang, D. Slippery liquid-infused porous electric heating coating for anti-icing and de-icing applications. Surf. Coatings Technol. 2019, 374, 889–896. [Google Scholar] [CrossRef]



| Materials | Total Surface Energy (mJ·m−2) | Dispersion Component (mJ·m−2) | Polar Component (mJ·m−2) |
|---|---|---|---|
| EVA (40 wt.%VA) | 35.9 | 32.5 | 3.4 |
| PVAc | 36.5 | 25.1 | 11.4 |
| Graphite | 52.8 | 41.1 | 11.7 |
| CNF | 94.7 | 92.1 | 2.6 |
| Materials | Interfacial Energy by Harmonic Mean Equation (mJ·m−2) | Interfacial Energy by Geometric Mean Equation (mJ·m−2) |
|---|---|---|
| EVA/Graphite | 5.5 | 3.0 |
| PVAc/Graphite | 3.9 | 2.0 |
| EVA/CNF | 28.6 | 15.2 |
| PVAc/CNF | 43.8 | 24.1 |
| EVA/PVAc | 5.3 | 2.8 |
| Blends | A | B | (Harmonic Mean Equation) | (Geometric Mean Equation) | Predicted Location |
|---|---|---|---|---|---|
| Graphite/PVAc/EVA | EVA | PVAc | 0.3 | 0.4 | PVAc/EVA interface |
| CNF/PVAc/EVA | EVA | PVAc | −2.9 | −3.2 | EVA |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Du, C.; Zhang, Y.; Lin, J.; Fan, G.; Zhou, C.; Yu, Y. Flexible Positive Temperature Coefficient Composites (PVAc/EVA/GP-CNF) with Room Temperature Curie Point. Polymers 2024, 16, 2028. https://doi.org/10.3390/polym16142028
Du C, Zhang Y, Lin J, Fan G, Zhou C, Yu Y. Flexible Positive Temperature Coefficient Composites (PVAc/EVA/GP-CNF) with Room Temperature Curie Point. Polymers. 2024; 16(14):2028. https://doi.org/10.3390/polym16142028
Chicago/Turabian StyleDu, Chao, Yangyang Zhang, Jiangmin Lin, Guotao Fan, Can Zhou, and Yan Yu. 2024. "Flexible Positive Temperature Coefficient Composites (PVAc/EVA/GP-CNF) with Room Temperature Curie Point" Polymers 16, no. 14: 2028. https://doi.org/10.3390/polym16142028
APA StyleDu, C., Zhang, Y., Lin, J., Fan, G., Zhou, C., & Yu, Y. (2024). Flexible Positive Temperature Coefficient Composites (PVAc/EVA/GP-CNF) with Room Temperature Curie Point. Polymers, 16(14), 2028. https://doi.org/10.3390/polym16142028







