Hemp Fiber-Reinforced Polymers Composite Jacketing Technique for Sustainable and Environment-Friendly Concrete
Abstract
1. Introduction
2. Research Significance
3. Experimental Program
3.1. Test Matrix
3.2. Properties of Materials
3.3. Construction of Square Specimens and Strengthening Process
3.4. Instrumentation and Testing Setup
4. Experimental Results
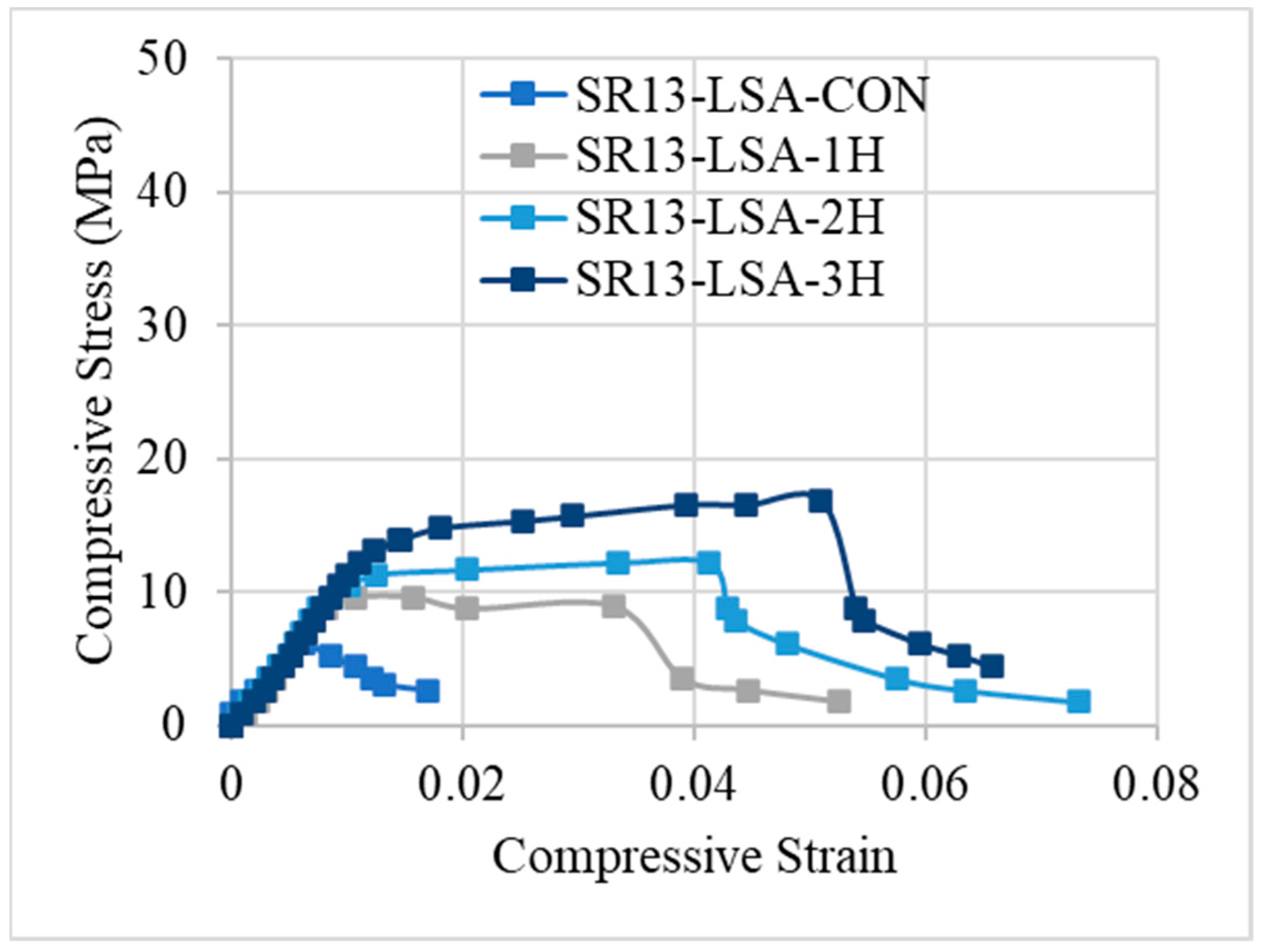
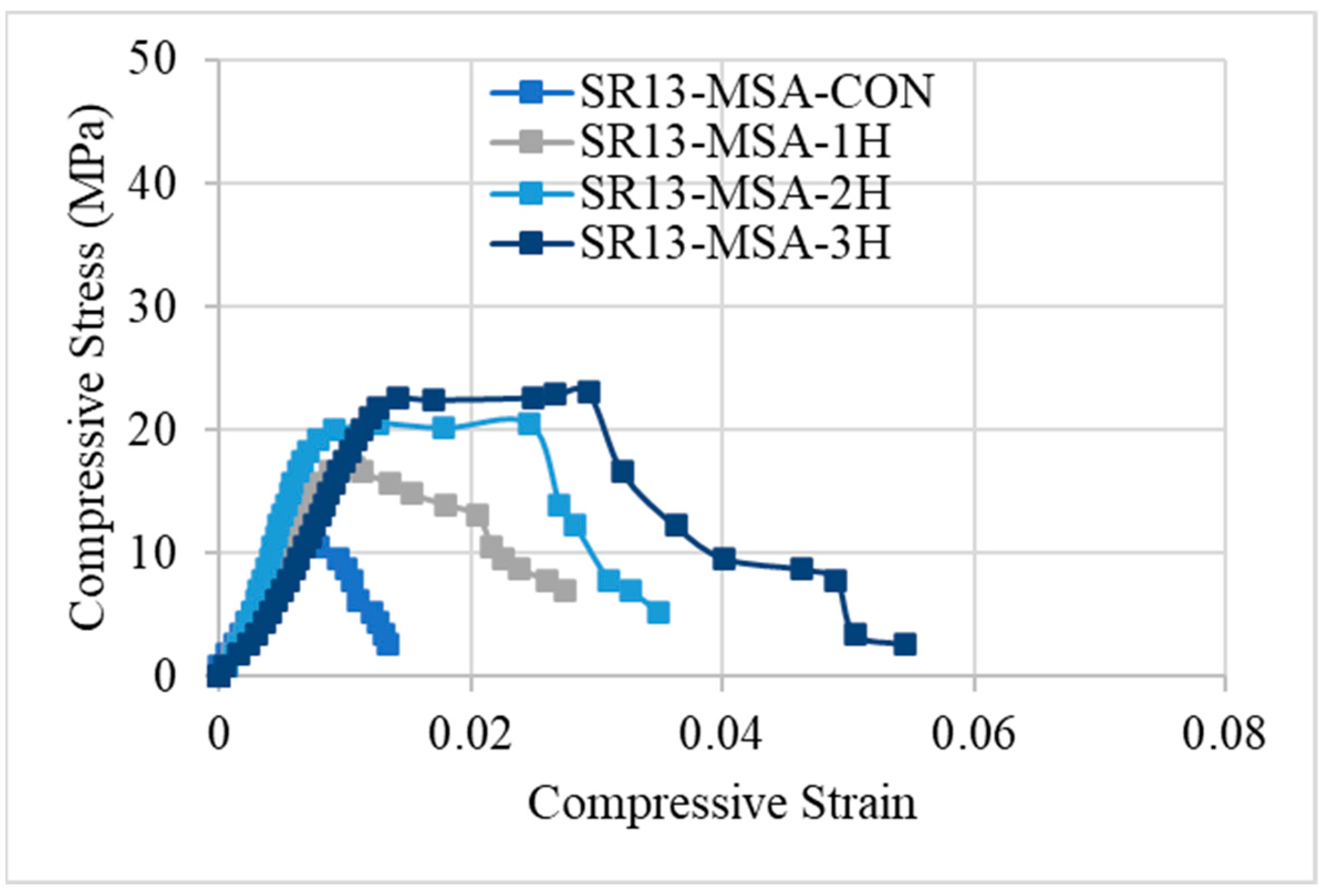
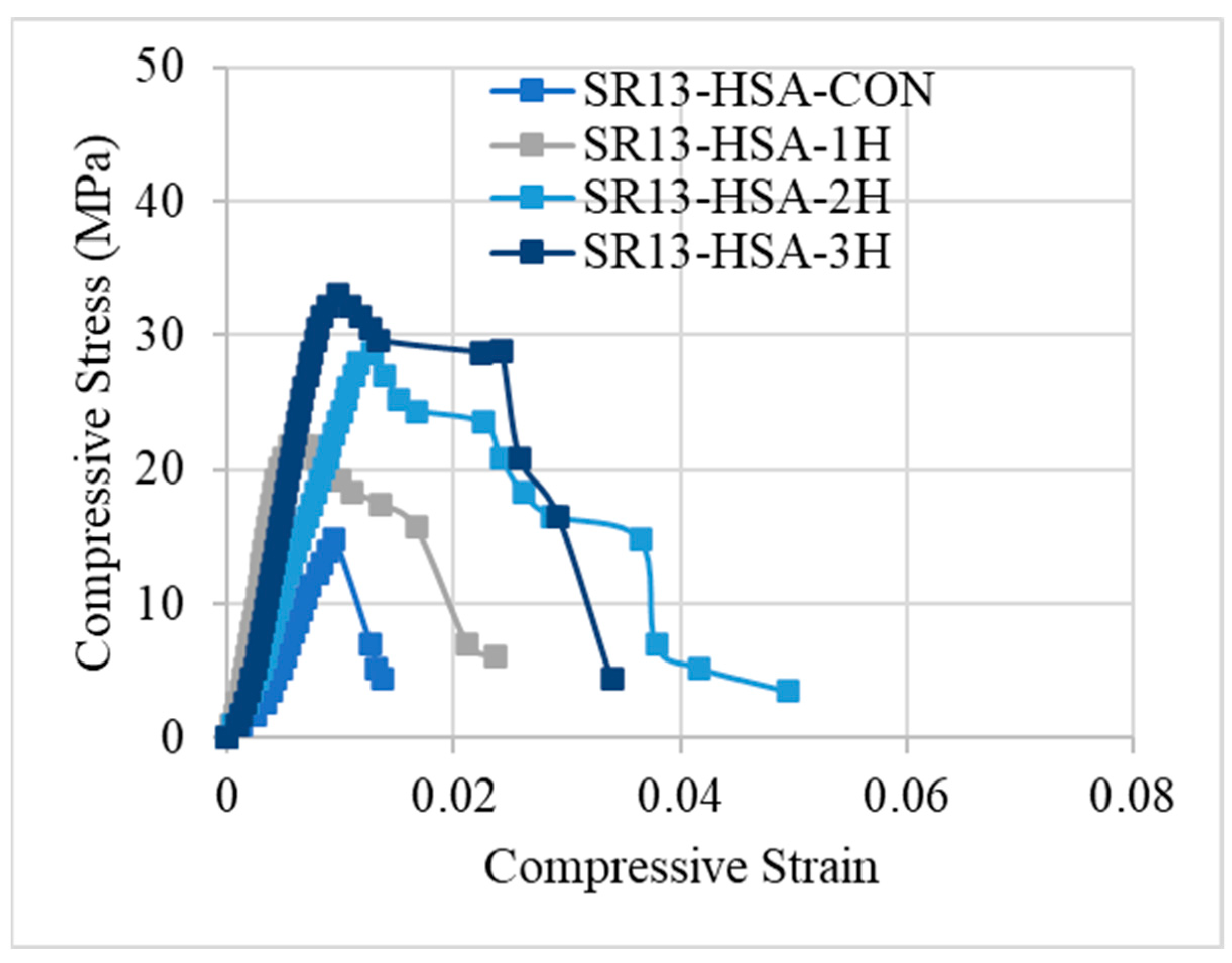
4.1. Axial Stress–Strain Response
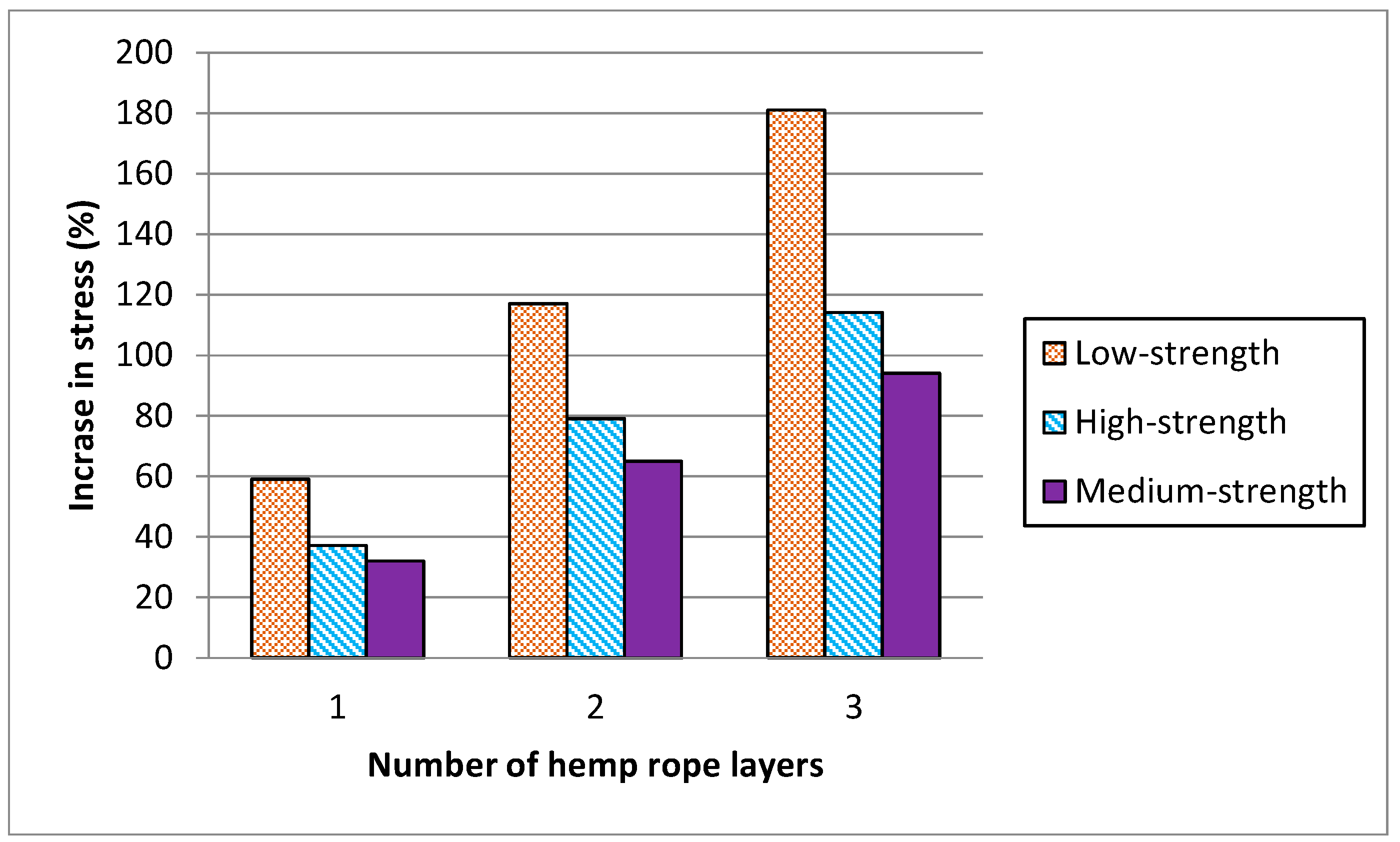
4.2. Hemp Rope Layers Effect on Low-, Medium-, and High-Strength Concrete
4.3. Failure Pattern of Specimens
5. Comparison with Analytical Models
5.1. Detail of Existing Models
5.2. Comparison of Experimental Results with Analytical Models
6. Strength Prediction by Artificial Neural Network
7. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| Type | fco (MPa) | Rupture Strength of Fiber (MPa) | Fiber Thickness | Specimen Size (mm) | (MPa) |
| Cylinder | 16.59 | 177.5 | 2.1 | 150 | 27.86 |
| Cylinder | 16.59 | 177.5 | 4.2 | 150 | 43.04 |
| Cylinder | 16.59 | 177.5 | 6.3 | 150 | 56.85 |
| Cylinder | 16.59 | 177.5 | 8.4 | 150 | 68.22 |
| Cylinder | 16.59 | 129.3 | 4.8 | 150 | 33.61 |
| Cylinder | 16.59 | 129.3 | 7.2 | 150 | 48.79 |
| Cylinder | 16.59 | 129.3 | 9.6 | 150 | 58.51 |
| Cylinder | 16.59 | 90.8 | 5.6 | 150 | 31.11 |
| Cylinder | 16.59 | 90.8 | 8.4 | 150 | 43.37 |
| Cylinder | 16.59 | 90.8 | 11.2 | 150 | 56.13 |
| Cylinder | 24.90 | 137.4 | 2.1 | 150 | 38.48 |
| Cylinder | 24.90 | 137.4 | 4.2 | 150 | 49.24 |
| Cylinder | 24.90 | 137.4 | 6.3 | 150 | 65.34 |
| Cylinder | 16.41 | 137.4 | 2.1 | 150 | 25.18 |
| Cylinder | 16.41 | 137.4 | 4.2 | 150 | 26.83 |
| Cylinder | 16.41 | 137.4 | 6.3 | 150 | 39.33 |
| Cylinder | 12.73 | 137.4 | 2.1 | 150 | 19.24 |
| Cylinder | 12.73 | 137.4 | 4.2 | 150 | 23.20 |
| Cylinder | 12.73 | 137.4 | 6.3 | 150 | 29.58 |
| Cylinder | 13.23 | 290 | 1.19 | 150 | 25.11 |
| Cylinder | 13.23 | 290 | 2.38 | 150 | 47.79 |
| Cylinder | 11.04 | 290 | 1.19 | 150 | 36.21 |
| Cylinder | 11.04 | 290 | 2.38 | 150 | 47.66 |
| Cylinder | 14.83 | 290 | 1.19 | 150 | 26.33 |
| Cylinder | 14.83 | 290 | 2.38 | 150 | 44.64 |
| Cylinder | 19.64 | 290 | 1.19 | 150 | 36.53 |
| Cylinder | 19.64 | 290 | 2.38 | 150 | 57.91 |
| Cylinder | 21.86 | 290 | 1.19 | 150 | 42.90 |
| Cylinder | 21.86 | 290 | 2.38 | 150 | 63.59 |
| Cylinder | 34.48 | 290 | 1.19 | 150 | 39.27 |
| Cylinder | 34.48 | 290 | 2.38 | 150 | 62.74 |
| Cylinder | 13.02 | 137.4 | 2.1 | 150 | 23.77 |
| Cylinder | 13.02 | 137.4 | 4.2 | 150 | 33.39 |
| Cylinder | 13.02 | 137.4 | 6.3 | 150 | 40.18 |
| Cylinder | 24.34 | 137.4 | 2.1 | 150 | 36.22 |
| Cylinder | 24.34 | 137.4 | 4.2 | 150 | 43.58 |
| Cylinder | 24.34 | 137.4 | 6.3 | 150 | 56.03 |
| Cylinder | 19.60 | 83.58 | 0.8 | 150 | 23.20 |
| Cylinder | 19.60 | 83.58 | 2.4 | 150 | 26.60 |
| Cylinder | 19.60 | 83.58 | 4 | 150 | 31.10 |
| Cylinder | 21.00 | 83.58 | 0.8 | 150 | 23.80 |
| Cylinder | 21.00 | 83.58 | 2.4 | 150 | 28.20 |
| Cylinder | 21.00 | 83.58 | 4 | 150 | 34.30 |
| Cylinder | 22.01 | 80 | 3 | 100 | 37.79 |
| Cylinder | 22.01 | 80 | 6 | 100 | 49.72 |
| Cylinder | 22.01 | 104 | 3 | 100 | 40.40 |
| Cylinder | 22.01 | 104 | 6 | 100 | 59.79 |
| Cylinder | 40.75 | 80 | 3 | 100 | 51.75 |
| Cylinder | 40.75 | 80 | 6 | 100 | 61.08 |
| Cylinder | 40.75 | 104 | 3 | 100 | 57.75 |
| Cylinder | 40.75 | 104 | 6 | 100 | 74.04 |
| Cylinder | 58.58 | 80 | 3 | 100 | 66.87 |
| Cylinder | 58.58 | 80 | 6 | 100 | 69.34 |
| Cylinder | 58.58 | 104 | 3 | 100 | 71.31 |
| Cylinder | 58.58 | 104 | 6 | 100 | 75.35 |
| Cylinder | 18.10 | 104 | 3 | 100 | 39.00 |
| Cylinder | 18.10 | 104 | 6 | 100 | 55.00 |
| Cylinder | 18.10 | 104 | 9 | 100 | 72.00 |
| Cylinder | 38.35 | 104 | 3 | 100 | 53.10 |
| Cylinder | 38.35 | 104 | 6 | 100 | 67.20 |
| Cylinder | 38.35 | 104 | 9 | 100 | 89.00 |
| Cylinder | 20.06 | 174 | 0.18 | 100 | 20.78 |
| Cylinder | 20.06 | 174 | 0.36 | 100 | 22.21 |
| Cylinder | 20.06 | 174 | 0.53 | 100 | 22.69 |
| Cylinder | 20.06 | 174 | 0.71 | 100 | 23.17 |
| Cylinder | 20.06 | 174 | 0.89 | 100 | 25.08 |
| Cylinder | 20.06 | 174 | 1.07 | 100 | 28.66 |
| Cylinder | 21.50 | 179 | 0.14 | 100 | 22.21 |
| Cylinder | 21.50 | 179 | 0.28 | 100 | 24.60 |
| Cylinder | 21.50 | 179 | 0.42 | 100 | 24.84 |
| Cylinder | 21.50 | 179 | 0.56 | 100 | 25.08 |
| Cylinder | 21.50 | 179 | 0.7 | 100 | 25.32 |
| Cylinder | 21.50 | 179 | 0.84 | 100 | 26.99 |
| Cylinder | 18.63 | 104 | 0.31 | 100 | 19.11 |
| Cylinder | 18.63 | 104 | 0.61 | 100 | 19.35 |
| Cylinder | 18.63 | 104 | 0.92 | 100 | 19.58 |
| Cylinder | 18.63 | 104 | 1.22 | 100 | 20.30 |
| Cylinder | 18.63 | 104 | 1.53 | 100 | 20.54 |
| Cylinder | 18.63 | 104 | 1.83 | 100 | 22.69 |
| Cylinder | 13.00 | 250 | 1.304 | 100 | 22.00 |
| Cylinder | 18.00 | 250 | 1.304 | 100 | 25.00 |
| Cylinder | 25.00 | 250 | 1.304 | 100 | 32.00 |
| Cylinder | 35.00 | 250 | 1.304 | 100 | 46.00 |
| SQ1 | 11.77 | 177.5 | 2.1 | 150 | 13.64 |
| SQ1 | 11.77 | 177.5 | 4.2 | 150 | 16.21 |
| SQ1 | 11.77 | 177.5 | 6.3 | 150 | 18.41 |
| SQ1 | 12.64 | 177.5 | 2.1 | 150 | 17.00 |
| SQ1 | 12.64 | 177.5 | 4.2 | 150 | 18.83 |
| SQ1 | 12.64 | 177.5 | 6.3 | 150 | 20.92 |
| SQ1 | 10.02 | 177.5 | 2.1 | 150 | 13.08 |
| SQ1 | 10.02 | 177.5 | 4.2 | 150 | 13.77 |
| SQ1 | 10.02 | 177.5 | 6.3 | 150 | 16.56 |
| SQ1 | 12.64 | 177.5 | 2.1 | 150 | 16.54 |
| SQ1 | 12.64 | 177.5 | 4.2 | 150 | 20.70 |
| SQ1 | 12.64 | 177.5 | 6.3 | 150 | 22.01 |
| SQ1 | 16.56 | 177.5 | 2.1 | 150 | 18.74 |
| SQ1 | 16.56 | 177.5 | 4.2 | 150 | 21.36 |
| SQ1 | 16.56 | 177.5 | 6.3 | 150 | 25.72 |
| SQ1 | 23.54 | 177.5 | 4.2 | 150 | 30.51 |
| SQ1 | 23.54 | 177.5 | 6.3 | 150 | 36.72 |
| SQ2 | 10.98 | 177.5 | 2.1 | 150 | 14.38 |
| SQ2 | 10.98 | 177.5 | 4.2 | 150 | 18.04 |
| SQ2 | 10.98 | 177.5 | 6.3 | 150 | 21.79 |
| SQ2 | 14.17 | 177.5 | 2.1 | 150 | 19.40 |
| SQ2 | 14.17 | 177.5 | 4.2 | 150 | 20.81 |
| SQ2 | 14.17 | 177.5 | 6.3 | 150 | 27.46 |
| SQ2 | 11.55 | 177.5 | 2.1 | 150 | 17.65 |
| SQ2 | 11.55 | 177.5 | 4.2 | 150 | 20.49 |
| SQ2 | 11.55 | 177.5 | 6.3 | 150 | 24.15 |
| SQ2 | 18.61 | 177.5 | 2.1 | 150 | 20.05 |
| SQ2 | 18.61 | 177.5 | 4.2 | 150 | 21.14 |
| SQ2 | 18.61 | 177.5 | 6.3 | 150 | 23.10 |
| SQ2 | 21.79 | 177.5 | 2.1 | 150 | 25.45 |
| SQ2 | 21.79 | 177.5 | 4.2 | 150 | 25.89 |
| SQ2 | 21.79 | 177.5 | 6.3 | 150 | 28.77 |
| SQ2 | 20.05 | 177.5 | 2.1 | 150 | 28.33 |
| SQ2 | 20.05 | 177.5 | 4.2 | 150 | 32.04 |
| SQ2 | 20.05 | 177.5 | 6.3 | 150 | 30.18 |
| SQ3 | 11.33 | 177.5 | 2.1 | 150 | 15.69 |
| SQ3 | 11.33 | 177.5 | 4.2 | 150 | 19.40 |
| SQ3 | 11.33 | 177.5 | 6.3 | 150 | 23.86 |
| SQ3 | 15.52 | 177.5 | 2.1 | 150 | 23.62 |
| SQ3 | 15.52 | 177.5 | 4.2 | 150 | 28.24 |
| SQ3 | 15.52 | 177.5 | 6.3 | 150 | 32.60 |
| SQ3 | 10.55 | 177.5 | 2.1 | 150 | 16.74 |
| SQ3 | 10.55 | 177.5 | 4.2 | 150 | 21.23 |
| SQ3 | 10.55 | 177.5 | 6.3 | 150 | 26.59 |
| SQ3 | 13.51 | 177.5 | 2.1 | 150 | 17.87 |
| SQ3 | 13.51 | 177.5 | 4.2 | 150 | 22.01 |
| SQ3 | 13.51 | 177.5 | 6.3 | 150 | 24.84 |
| SQ3 | 16.13 | 177.5 | 2.1 | 150 | 22.23 |
| SQ3 | 16.13 | 177.5 | 4.2 | 150 | 26.59 |
| SQ3 | 16.13 | 177.5 | 6.3 | 150 | 27.46 |
| SQ3 | 23.54 | 177.5 | 2.1 | 150 | 25.61 |
| SQ3 | 23.54 | 177.5 | 4.2 | 150 | 29.64 |
| SQ3 | 23.54 | 177.5 | 6.3 | 150 | 31.82 |
| SQ1 | 16.10 | 177.5 | 2.1 | 150 | 22.00 |
| SQ1 | 16.10 | 177.5 | 4.2 | 150 | 27.40 |
| SQ1 | 16.10 | 177.5 | 6.3 | 150 | 33.00 |
| SQ2 | 15.40 | 177.5 | 2.1 | 150 | 24.00 |
| SQ2 | 15.40 | 177.5 | 4.2 | 150 | 31.60 |
| SQ2 | 15.40 | 177.5 | 6.3 | 150 | 38.00 |
| SQ3 | 14.90 | 177.5 | 2.1 | 150 | 26.00 |
| SQ3 | 14.90 | 177.5 | 4.2 | 150 | 34.50 |
| SQ3 | 14.90 | 177.5 | 6.3 | 150 | 42.00 |
| SQ2 | 28.00 | 177.5 | 2.1 | 150 | 33.33 |
| SQ2 | 28.00 | 177.5 | 4.2 | 150 | 39.11 |
| SQ2 | 28.00 | 177.5 | 6.3 | 150 | 42.67 |
| SQ2 | 18.22 | 177.5 | 2.1 | 150 | 21.16 |
| SQ2 | 18.22 | 177.5 | 4.2 | 150 | 23.11 |
| SQ2 | 18.22 | 177.5 | 6.3 | 150 | 24.89 |
| SQ2 | 17.78 | 177.5 | 2.1 | 150 | 21.56 |
| SQ2 | 17.78 | 177.5 | 4.2 | 150 | 23.11 |
| SQ2 | 17.78 | 177.5 | 6.3 | 150 | 24.44 |
| Note: SQ1: Square specimen with corner radii = 0 mm, SQ2: Square specimen with corner radii = 13 mm, SQ3: Square specimen with corner radii = 26 mm. | |||||
References
- Zabalza Bribián, I.; Valero Capilla, A.; Aranda Usón, A. Life cycle assessment of building materials: Comparative analysis of energy and environmental impacts and evaluation of the eco-efficiency improvement potential. Build. Environ. 2011, 46, 1133–1140. [Google Scholar] [CrossRef]
- Xiao, J.; Li, W.; Fan, Y.; Huang, X. An overview of study on recycled aggregate concrete in China (1996–2011). Constr. Build. Mater. 2012, 31, 364–383. [Google Scholar] [CrossRef]
- Zhu, L.; Zhu, Z. Reuse of Clay Brick Waste in Mortar and Concrete. Adv. Mater. Sci. Eng. 2020, 2020, 6326178. [Google Scholar] [CrossRef]
- Manfredi, S.; Pant, R.; Pennington, D.W.; Versmann, A. Supporting environmentally sound decisions for waste management with LCT and LCA. Int. J. Life Cycle Assess. 2011, 16, 937–939. [Google Scholar] [CrossRef]
- Hamad, B.S.; Dawi, A.H. Sustainable normal and high strength recycled aggregate concretes using crushed tested cylinders as coarse aggregates. Case Stud. Constr. Mater. 2017, 7, 228–239. [Google Scholar] [CrossRef]
- Ohemeng, E.A.; Ekolu, S.O. Comparative analysis on costs and benefits of producing natural and recycled concrete aggregates: A South African case study. Case Stud. Constr. Mater. 2020, 13, e00450. [Google Scholar] [CrossRef]
- Debieb, F.; Kenai, S. The use of coarse and fine crushed bricks as aggregate in concrete. Constr. Build. Mater. 2008, 22, 886–893. [Google Scholar] [CrossRef]
- Kox, S.; Vanroelen, G.; Van Herck, J.; de Krem, H.; Vandoren, B. Experimental evaluation of the high-grade properties of recycled concrete aggregates and their application in concrete road pavement construction. Case Stud. Constr. Mater. 2019, 11, e00282. [Google Scholar] [CrossRef]
- Yang, Y.F.; Ma, G.L. Experimental behaviour of recycled aggregate concrete filled stainless steel tube stub columns and beams. Thin-Walled Struct. 2013, 66, 62–75. [Google Scholar] [CrossRef]
- Nováková, I.; Mikulica, K. Properties of Concrete with Partial Replacement of Natural Aggregate by Recycled Concrete Aggregates from Precast Production. Procedia Eng. 2016, 151, 360–367. [Google Scholar] [CrossRef]
- Yang, J.; Du, Q.; Bao, Y. Concrete with recycled concrete aggregate and crushed clay bricks. Constr. Build. Mater. 2011, 25, 1935–1945. [Google Scholar] [CrossRef]
- Tabsh, S.W.; Abdelfatah, A.S. Influence of recycled concrete aggregates on strength properties of concrete. Constr. Build. Mater. 2009, 23, 1163–1167. [Google Scholar] [CrossRef]
- McKee, T.B.; Doesken, N.J.; Kleist, J. Analysis of Standardized Precipitation Index (SPI) data for drought assessment. Water 1993, 26, 1–72. [Google Scholar]
- González, J.S.; Gayarre, F.L.; Pérez, C.L.C.; Ros, P.S.; López, M.A.S. Influence of recycled brick aggregates on properties of structural concrete for manufacturing precast prestressed beams. Constr. Build. Mater. 2017, 149, 507–514. [Google Scholar] [CrossRef]
- Cachim, P.B. Mechanical properties of brick aggregate concrete. Constr. Build. Mater. 2009, 23, 1292–1297. [Google Scholar] [CrossRef]
- Medina, C.; Zhu, W.; Howind, T.; Sánchez De Rojas, M.I.; Frías, M. Influence of mixed recycled aggregate on the physical–mechanical properties of recycled concrete. J. Clean. Prod. 2014, 68, 216–225. [Google Scholar] [CrossRef]
- Jiang, T.; Wang, X.M.; Zhang, W.P.; Chen, G.M.; Lin, Z.H. Behavior of FRP-Confined Recycled Brick Aggregate Concrete under Monotonic Compression. J. Compos. Constr. 2020, 24, 1–14. [Google Scholar] [CrossRef]
- Khalaf, F.M.; DeVenny, A.S. Recycling of Demolished Masonry Rubble as Coarse Aggregate in Concrete: Review. J. Mater. Civ. Eng. 2004, 16, 331–340. [Google Scholar] [CrossRef]
- Smith, S.T.; Teng, J.G. FRP-strengthened RC beams. I: Review of debonding strength models. Eng. Struct. 2002, 24, 385–395. [Google Scholar] [CrossRef]
- Liang, J.; Lin, S.; Ahmed, M. Axial behavior of carbon fiber-reinforced polymer–confined recycled aggregate concrete-filled steel tube slender square columns. Adv. Struct. Eng. 2021, 24, 3507–3518. [Google Scholar] [CrossRef]
- Chen, G.M.; He, Y.H.; Jiang, T.; Lin, C.J. Behavior of CFRP-confined recycled aggregate concrete under axial compression. Constr. Build. Mater. 2016, 111, 85–97. [Google Scholar] [CrossRef]
- Chaiyasarn, K.; Hussain, Q.; Joyklad, P.; Rodsin, K. New hybrid basalt/E-glass FRP jacketing for enhanced confinement of recycled aggregate concrete with clay brick aggregate. Case Stud. Constr. Mater. 2021, 14, e00507. [Google Scholar] [CrossRef]
- Yinh, S.; Hussain, Q.; Joyklad, P.; Chaimahawan, P.; Rattanapitikon, W.; Limkatanyu, S.; Pimanmas, A. Strengthening effect of natural fiber reinforced polymer composites (NFRP) on concrete. Case Stud. Constr. Mater. 2021, 15, e00653. [Google Scholar] [CrossRef]
- Sen, T.; Jagannatha Reddy, H.N. Efficacy of bio derived jute FRP composite based technique for shear strength retrofitting of reinforced concrete beams and its comparative analysis with carbon and glass FRP shear retrofitting schemes. Sustain. Cities Soc. 2014, 13, 105–124. [Google Scholar] [CrossRef]
- Li, Y.; Mai, Y.W.; Ye, L. Sisal fiber and its composites: A review of recent developments. Compos. Sci. Technol. 2000, 60, 2037–2055. [Google Scholar] [CrossRef]
- Alam, M.A.; Al Riyami, K. Shear strengthening of reinforced concrete beam using natural fiber reinforced polymer laminates. Constr. Build. Mater. 2018, 162, 683–696. [Google Scholar] [CrossRef]
- Bambach, M.R. Compression strength of natural fiber composite plates and sections of flax, jute and hemp. Thin-Walled Struct. 2017, 119, 103–113. [Google Scholar] [CrossRef]
- Hussain, Q.; Ruangrassamee, A.; Tangtermsirikul, S.; Joyklad, P. Behavior of concrete confined with epoxy bonded fiber ropes under axial load. Constr. Build. Mater. 2020, 263, 120093. [Google Scholar] [CrossRef]
- Fragoudakis, R.; Gallagher, J.A.; Kim, V. A Computational Analysis of the Energy Harvested by Gfrp and Nfrp Laminated Beams Under Cyclic Loading. Procedia Eng. 2017, 200, 221–228. [Google Scholar] [CrossRef]
- Ghalieh, L.; Awwad, E.; Saad, G.; Khatib, H.; Mabsout, M. Concrete Columns Wrapped with Hemp Fiber Reinforced Polymer—An Experimental Study. Procedia Eng. 2017, 200, 440–447. [Google Scholar] [CrossRef]
- Hussain, Q.; Ruangrassamee, A.; Tangtermsirikul, S.; Joyklad, P.; Wijeyewickrema, A.C. Low-Cost Fiber Rope Reinforced Polymer (FRRP) Confinement of Square Columns with Different Corner Radii. Buildings 2021, 11, 355. [Google Scholar] [CrossRef]
- ASTM C1314-21; Standard Test Method For Compressive Strength of Masonry Prisms. ASTM International: West Conshohocken, PA, USA, 2022.
- ASTM C140/C140M-22a; Standard Test Methods for Sampling and Testing Concrete Masonry Units and Related Units. ASTM International: West Conshohocken, PA, USA, 2022.
- ASTM A931-18; Standard Test Method for Tension Testing of Wire Ropes and Strand. ASTM International: West Conshohocken, PA, USA, 2018.
- ASTM E8/E8M-13; Standard Test Methods for Tension Testing of Metallic Materials. ASTM International: West Conshohocken PA, USA, 2013.
- Joyklad, P.; Nawaz, A.; Hussain, Q. Effect of Fired Clay Brick Aggregates on Mechanical Properties of concrete. Suranaree J. Sci. Technol. 2018, 25, 349. [Google Scholar]
- Pimanmas, A.; Hussain, Q.; Panyasirikhunawut, A.; Rattanapitikon, W. Axial strength and deformability of concrete confined with natural fiber-reinforced polymers. Mag. Concr. Res. 2019, 71, 55–70. [Google Scholar] [CrossRef]
- Rodsin, K. Confinement effects of glass FRP on circular concrete columns made with crushed fired clay bricks as coarse aggregates. Case Stud. Constr. Mater. 2021, 15, e00609. [Google Scholar] [CrossRef]
- Di Schino, A.; Corradi, M. Construction and building materials. AIMS Mater. Sci. 2020, 7, 157–159. [Google Scholar] [CrossRef]
- Qi, Y.; Xie, L.; Bai, Y.; Liu, W.; Fang, H. Axial Compression Behaviours of Pultruded GFRP-Wood Composite Columns. Sensors 2019, 19, 755. [Google Scholar] [CrossRef] [PubMed]
- Cusson, D.; Paultre, P. Stress-strain model for confined high-strength concrete. J. Struct. Eng. 1995, 121, 468–477. [Google Scholar] [CrossRef]
- Légeron, F.; Paultre, P. Uniaxial Confinement Model for Normal- and High-Strength Concrete Columns. J. Struct. Eng. 2003, 129, 241–252. [Google Scholar] [CrossRef]
- Shehata, I.A.E.M.; Carneiro, L.A.V.; Shehata, L.C.D. Strength of short concrete columns confined with CFRP sheets. Mater. Struct. 2001, 35, 50–58. [Google Scholar] [CrossRef]
- Triantafillou, T.C.; Papanicolaou, C.G.; Zissimopoulos, P.; Laourdekis, T. Concrete confinement with textile-reinforced mortar jackets. ACI Struct. J. 2006, 103, 28–37. [Google Scholar]
- Wang, Z.; Wang, D.; Smith, S.T.; Lu, D. CFRP-Confined Square RC Columns. I: Experimental Investigation. J. Compos. Constr. 2012, 16, 150–160. [Google Scholar] [CrossRef]
- Akiyama, M.; Suzuki, M.; Frangopol, D.M. Stress-averaged strain model for confined high-strength concrete. ACI Struct. J. 2010, 107, 179–188. [Google Scholar] [CrossRef]
- Naderpour, H.; Kheyroddin, A.; Ghodrati Amiri, G. Prediction of FRP-confined compressive strength of concrete using artificial neural networks. Compos. Struct. 2010, 92, 2817–2829. [Google Scholar] [CrossRef]
- Cascardi, A.; Micelli, F.; Aiello, M.A. An Artificial Neural Networks model for the prediction of the compressive strength of FRP-confined concrete circular columns. Eng. Struct. 2017, 140, 199–208. [Google Scholar] [CrossRef]
- Ahmad, A.; Chaiyasarn, K.; Farooq, F.; Suparp, S.; Aslam, F. Compressive strength prediction via gene expression programming (GEP) and artificial neural network (ANN) for concrete containing RCA. Buildings 2021, 11, 324. [Google Scholar] [CrossRef]
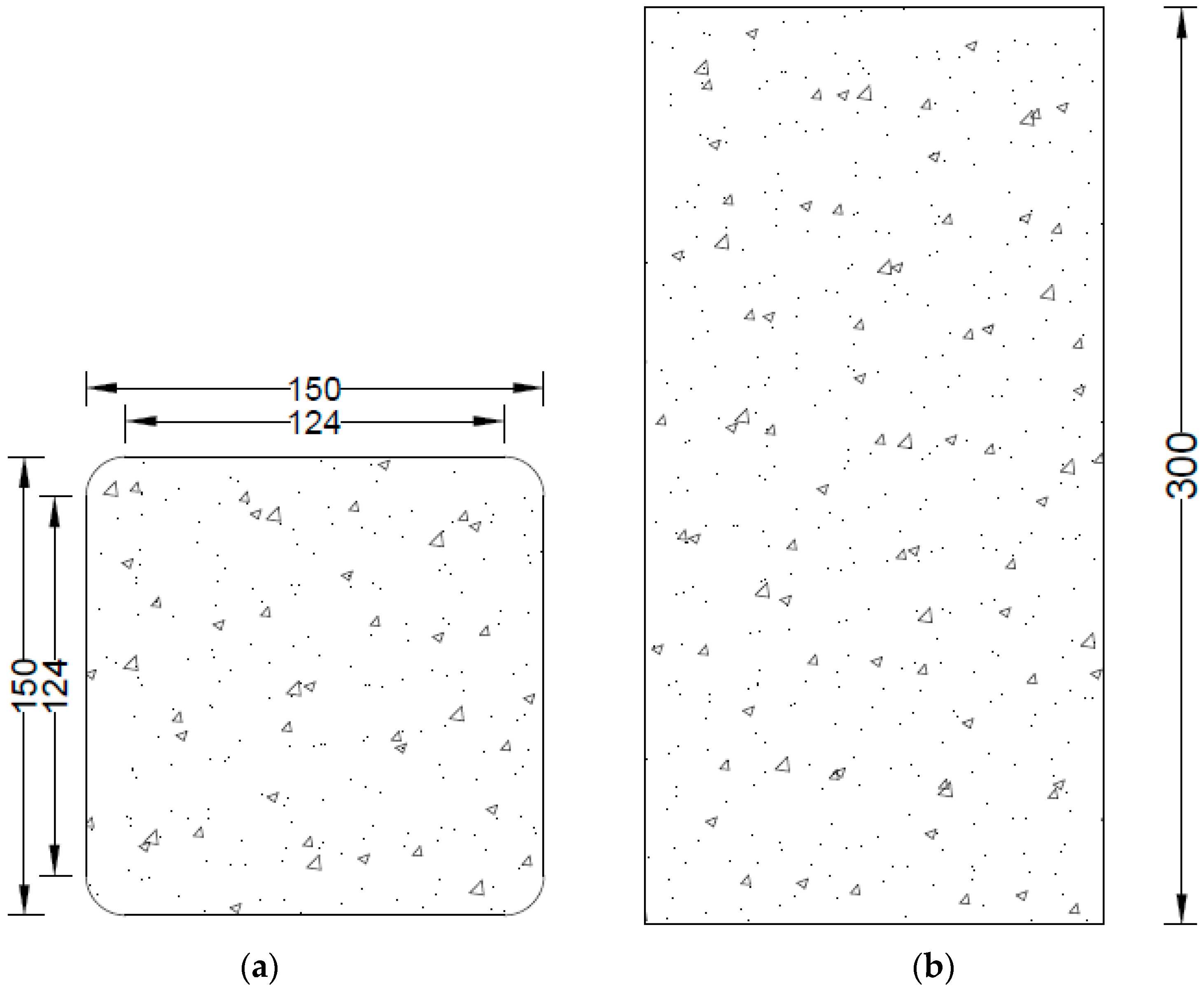








| Group | Specimen Name | Replacement Level of CBA | No. of Layer | No. of Specimen |
|---|---|---|---|---|
| Group 1 | SR13-LS-CBA-CON | 50% | None | 2 |
| SR13-LS-CBA-1H | 50% | 1 | 2 | |
| SR13-LS-CBA-2H | 50% | 2 | 2 | |
| SR13-LS-CBA-3H | 50% | 3 | 2 | |
| Group 1 | SR13-MS-CBA-CON | 50% | None | 2 |
| SR13-MS-CBA-1H | 50% | 1 | 2 | |
| SR13-MS-CBA-2H | 50% | 2 | 2 | |
| SR13-MS-CBA-3H | 50% | 3 | 2 | |
| Group 3 | SR13-HS-CBA-CON | 50% | None | 2 |
| SR13-HS-CBA-1H | 50% | 1 | 2 | |
| SR13-HS-CBA-2H | 50% | 2 | 2 | |
| SR13-HS-CBA-3H | 50% | 3 | 2 |
| Mix Material | Quantity Required in kg for 1 m3 of Concrete | ||
|---|---|---|---|
| Low Strength | Medium Strength | High Strength | |
| Cement | 242 | 343 | 444 |
| Coarse aggregates | 605 | 554 | 504 |
| Fine aggregates | 726 | 665 | 605 |
| Fired-clay solid brick aggregates | 605 | 554 | 504 |
| Property | Value |
|---|---|
| Ultimate tensile strength (MPa) | 137.4 |
| Nominal diameter (mm) | 2.1 |
| Strain (%) | 3.5 |
| Specimen Name | Peak Stress (MPa) | Increase (%) | Ultimate Strain | Increase (%) |
|---|---|---|---|---|
| Low-strength concrete | ||||
| SR13-LS-CBA-CON | 6.13 | --- | 0.0075 | --- |
| SR13-LS-CBA-1H | 9.78 | 59 | 0.0153 | 104 |
| SR13-LS-CBA-2H | 13.33 | 117 | 0.0403 | 438 |
| SR13-LS-CBA-3H | 17.24 | 181 | 0.0498 | 564 |
| Medium-strength concrete | ||||
| SR13-MS-CBA-CON | 12.44 | --- | 0.0067 | --- |
| SR13-MS-CBA-1H | 17.07 | 37 | 0.0132 | 98 |
| SR13-MS-CBA-2H | 22.22 | 79 | 0.0237 | 256 |
| SR13-MS-CBA-3H | 26.67 | 114 | 0.0333 | 400 |
| High-strength concrete | ||||
| SR13-HS-CBA-CON | 15.11 | --- | 0.0060 | --- |
| SR13-HS-CBA-1H | 20.00 | 32 | 0.0117 | 94 |
| SR13-HS-CBA-2H | 24.89 | 65 | 0.0138 | 131 |
| SR13-HS-CBA-3H | 29.33 | 94 | 0.0175 | 192 |
| Study | Stress Equation | Strain Equation |
|---|---|---|
| Legeron and Paultre 2003 [42] | ||
| Triantafillou et al. 2006 [44] | ||
| Akiyama et al. 2010 [46] | ||
| Shehata et al. 2002 [43] | ||
| Cusson and Paultre 1995 [41] | ||
| Wang et al. 2012 [45] |
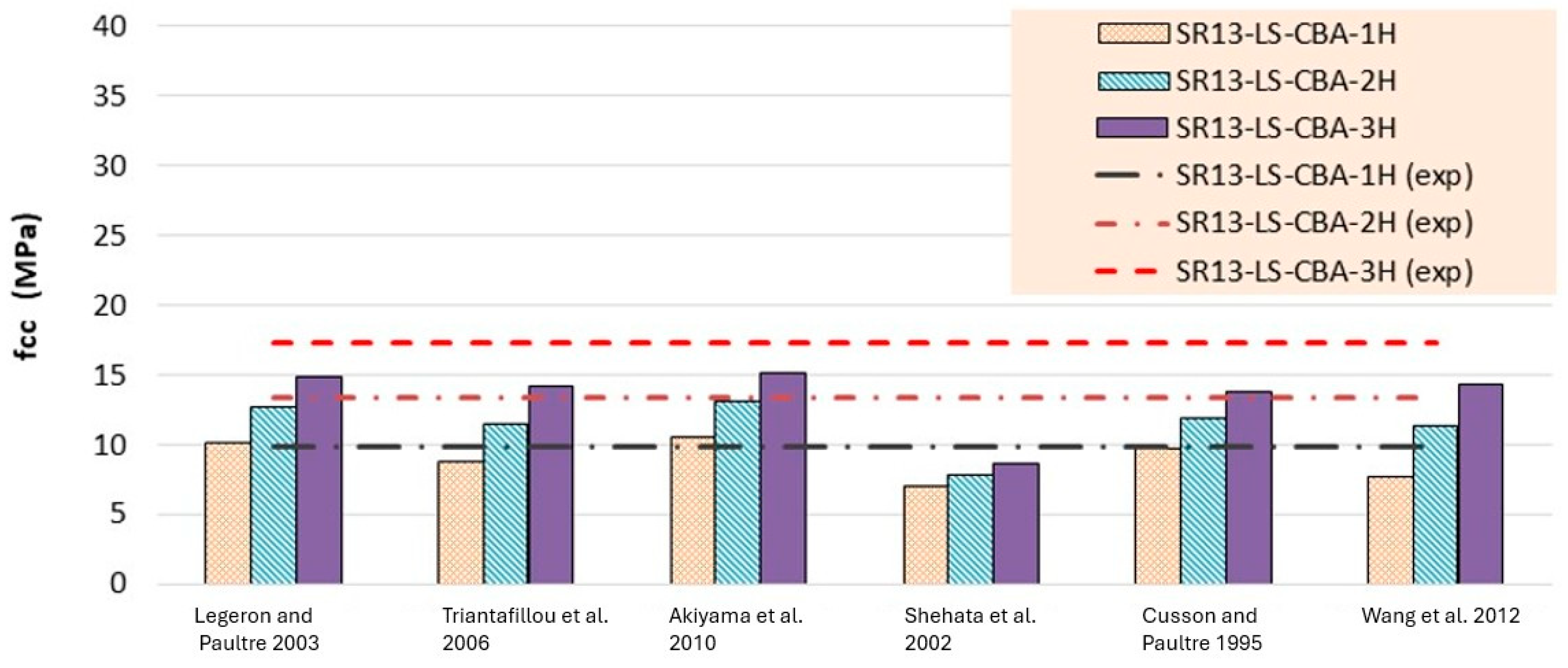
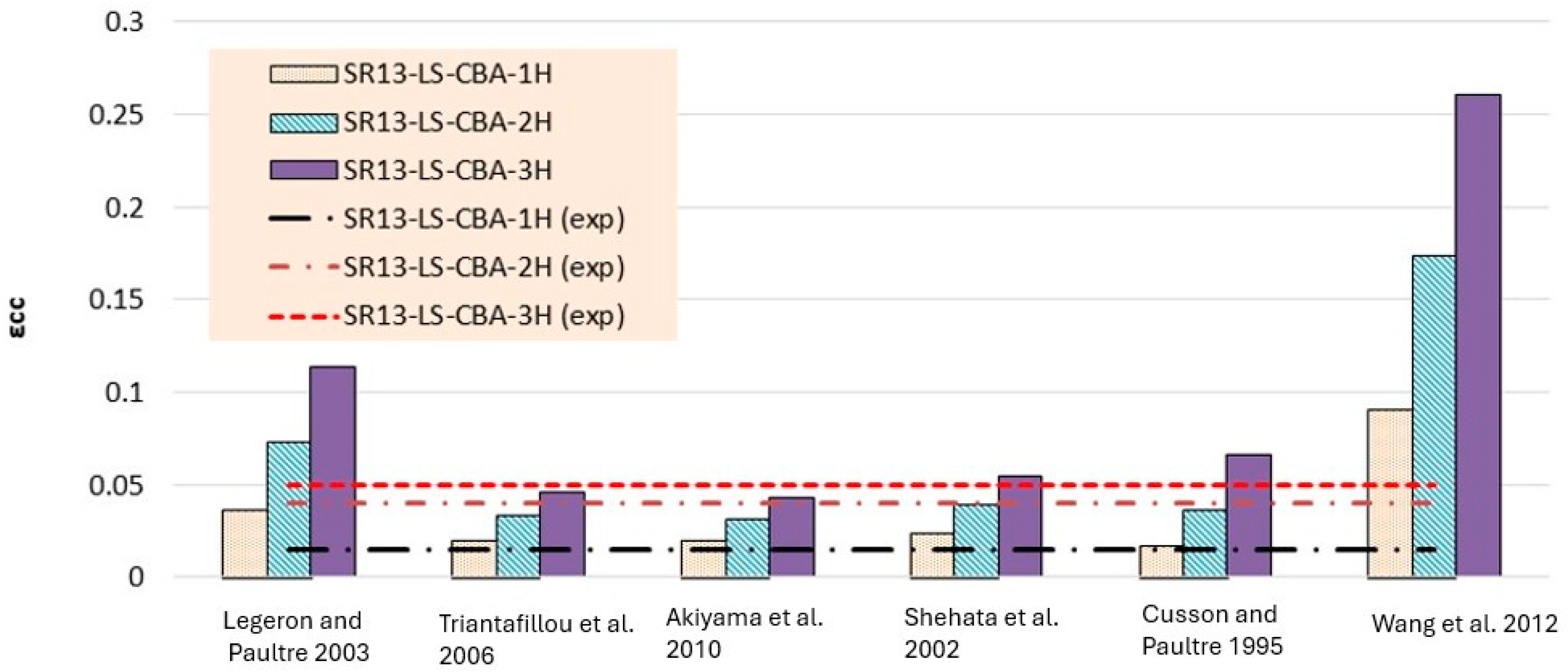
| Study | Specimen | fcc (exp) MPa | fcc (theo) MPa | εcc (exp) | εcc (theo) |
|---|---|---|---|---|---|
| Legeron and Paultre 2003 [42] | SR13-LS-CBA-1H | 9.78 | 10.15 | 0.0153 | 0.0359 |
| SR13-LS-CBA-2H | 13.33 | 12.67 | 0.0403 | 0.0728 | |
| SR13-LS-CBA-3H | 17.24 | 14.81 | 0.0498 | 0.1137 | |
| Triantafillou et al. 2006 [44] | SR13-LS-CBA-1H | 9.78 | 8.81 | 0.0153 | 0.0203 |
| SR13-LS-CBA-2H | 13.33 | 11.50 | 0.0403 | 0.0332 | |
| SR13-LS-CBA-3H | 17.24 | 14.18 | 0.0498 | 0.0461 | |
| Akiyama et al. 2010 [46] | SR13-LS-CBA-1H | 9.78 | 10.57 | 0.0153 | 0.0195 |
| SR13-LS-CBA-2H | 13.33 | 13.08 | 0.0403 | 0.0315 | |
| SR13-LS-CBA-3H | 17.24 | 15.17 | 0.0498 | 0.0435 | |
| Shehata et al. 2002 [43] | SR13-LS-CBA-1H | 9.78 | 6.95 | 0.0153 | 0.0233 |
| SR13-LS-CBA-2H | 13.33 | 7.76 | 0.0403 | 0.0392 | |
| SR13-LS-CBA-3H | 17.24 | 8.58 | 0.0498 | 0.0551 | |
| Cusson and Paultre 1995 [41] | SR13-LS-CBA-1H | 9.78 | 9.65 | 0.0153 | 0.0165 |
| SR13-LS-CBA-2H | 13.33 | 11.85 | 0.0403 | 0.0367 | |
| SR13-LS-CBA-3H | 17.24 | 13.72 | 0.0498 | 0.0658 | |
| Wang et al. 2012 [45] | SR13-LS-CBA-1H | 9.78 | 7.73 | 0.0153 | 0.0907 |
| SR13-LS-CBA-2H | 13.33 | 11.36 | 0.0403 | 0.1740 | |
| SR13-LS-CBA-3H | 17.24 | 14.36 | 0.0498 | 0.2605 |
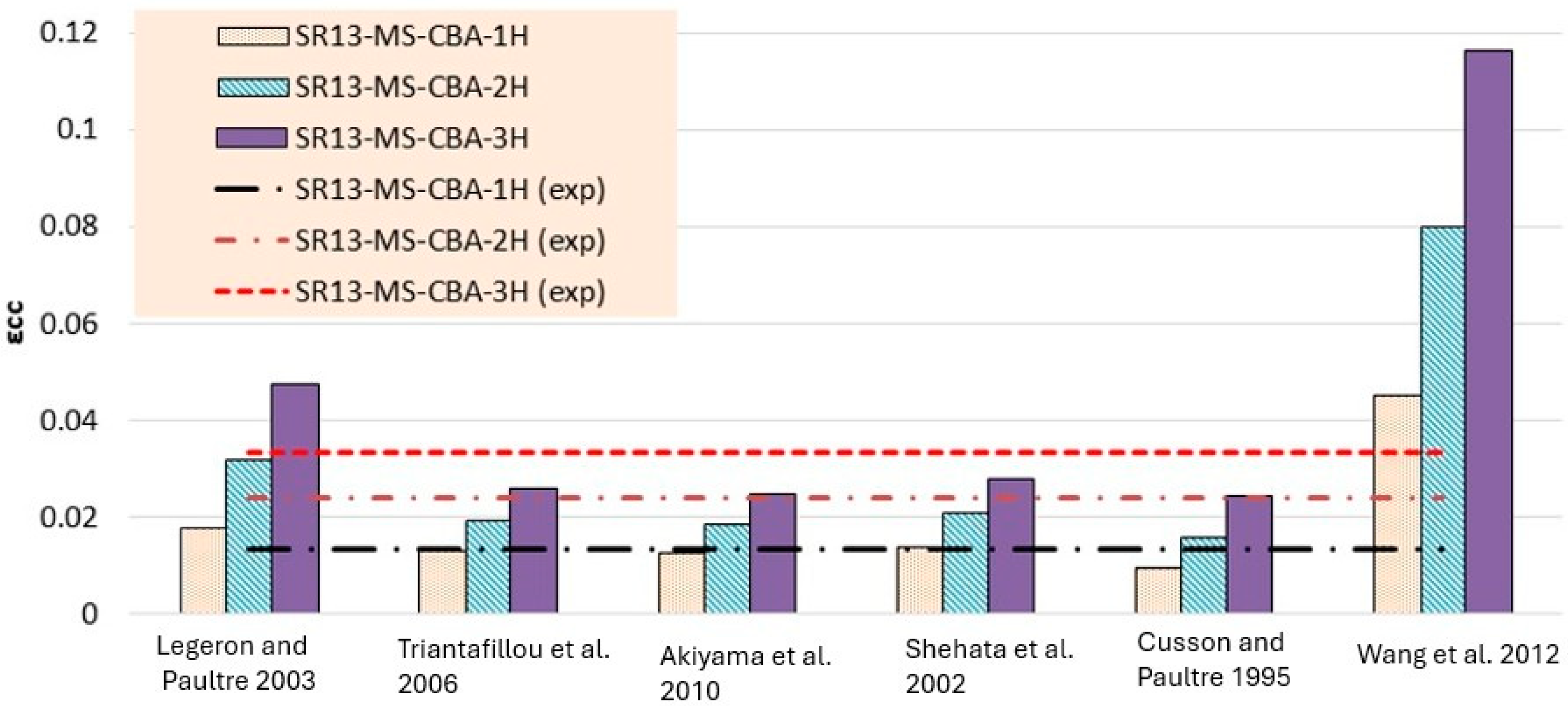
| Study | Specimen | fcc (exp) MPa | fcc (theo) MPa | εcc (exp) | εcc (theo) |
|---|---|---|---|---|---|
| Legeron and Paultre 2003 [42] | SR13-MS-CBA-1H | 17.07 | 17.41 | 0.0132 | 0.0175 |
| SR13-MS-CBA-2H | 22.22 | 20.52 | 0.0237 | 0.0316 | |
| SR13-MS-CBA-3H | 26.67 | 23.18 | 0.0333 | 0.0473 | |
| Triantafillou et al. 2006 [44] | SR13-MS-CBA-1H | 17.07 | 15.12 | 0.0132 | 0.0130 |
| SR13-MS-CBA-2H | 22.22 | 17.81 | 0.0237 | 0.0193 | |
| SR13-MS-CBA-3H | 26.67 | 20.50 | 0.0333 | 0.0257 | |
| Akiyama et al. 2010 [46] | SR13-MS-CBA-1H | 17.07 | 18.14 | 0.0132 | 0.0126 |
| SR13-MS-CBA-2H | 22.22 | 21.36 | 0.0237 | 0.0185 | |
| SR13-MS-CBA-3H | 26.67 | 24.04 | 0.0333 | 0.0245 | |
| Shehata et al. 2002 [43] | SR13-MS-CBA-1H | 17.07 | 13.26 | 0.0132 | 0.0137 |
| SR13-MS-CBA-2H | 22.22 | 14.08 | 0.0237 | 0.0207 | |
| SR13-MS-CBA-3H | 26.67 | 14.89 | 0.0333 | 0.0277 | |
| Cusson and Paultre 1995 [41] | SR13-MS-CBA-1H | 17.07 | 16.80 | 0.0132 | 0.0094 |
| SR13-MS-CBA-2H | 22.22 | 19.51 | 0.0237 | 0.0155 | |
| SR13-MS-CBA-3H | 26.67 | 21.83 | 0.0333 | 0.0242 | |
| Wang et al. 2012 [45] | SR13-MS-CBA-1H | 17.07 | 10.88 | 0.0132 | 0.0451 |
| SR13-MS-CBA-2H | 22.22 | 15.56 | 0.0237 | 0.0800 | |
| SR13-MS-CBA-3H | 26.67 | 19.43 | 0.0333 | 0.1162 |
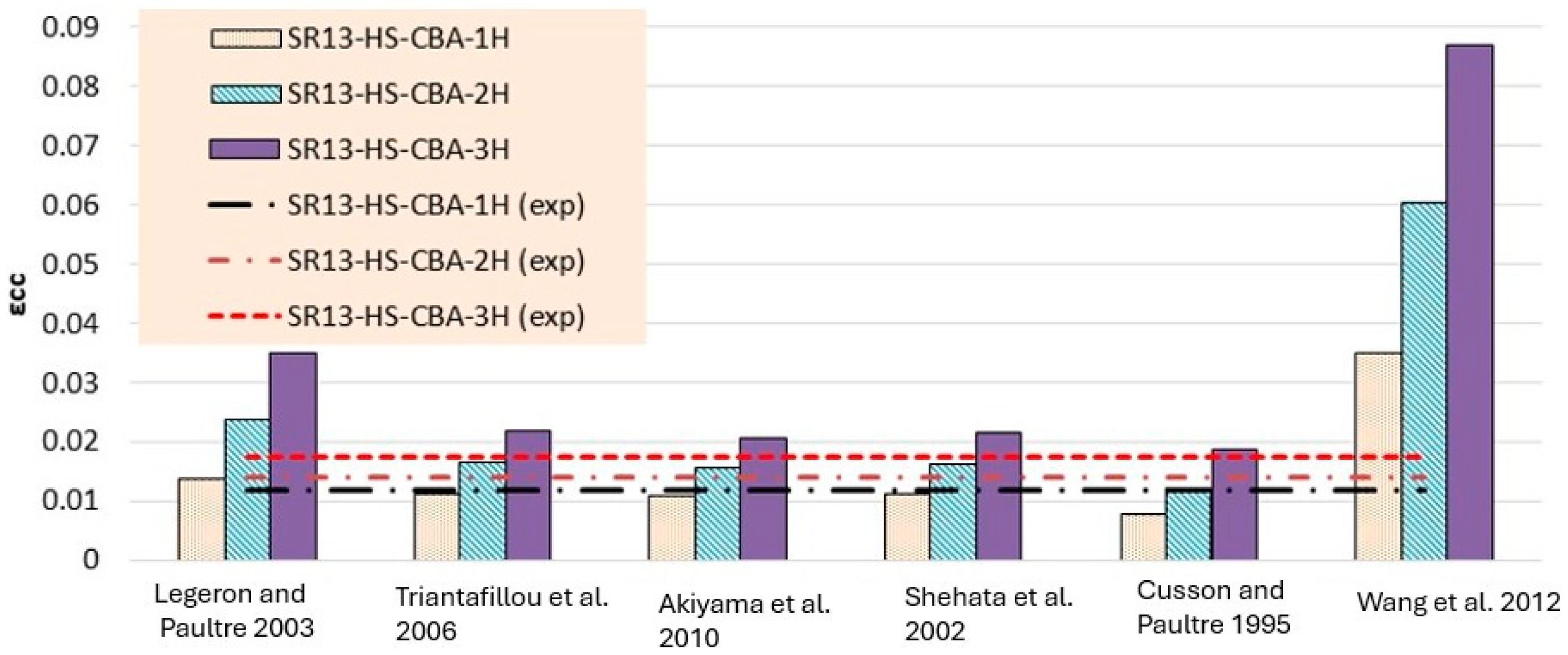
| Study | Specimen | fcc (exp) MPa | fcc (theo) MPa | εcc (exp) | εcc (theo) |
|---|---|---|---|---|---|
| Legeron and Paultre 2003 [42] | SR13-HS-CBA-1H | 20.00 | 20.38 | 0.0117 | 0.0137 |
| SR13-HS-CBA-2H | 24.89 | 23.68 | 0.0138 | 0.0237 | |
| SR13-HS-CBA-3H | 29.33 | 26.49 | 0.0175 | 0.0348 | |
| Triantafillou et al. 2006 [44] | SR13-HS-CBA-1H | 20.00 | 17.79 | 0.0117 | 0.0112 |
| SR13-HS-CBA-2H | 24.89 | 20.48 | 0.0138 | 0.0164 | |
| SR13-HS-CBA-3H | 29.33 | 23.16 | 0.0175 | 0.0217 | |
| Akiyama et al. 2010 [46] | SR13-HS-CBA-1H | 20.00 | 21.21 | 0.0117 | 0.0109 |
| SR13-HS-CBA-2H | 24.89 | 24.67 | 0.0138 | 0.0157 | |
| SR13-HS-CBA-3H | 29.33 | 27.53 | 0.0175 | 0.0206 | |
| Shehata et al. 2002 [43] | SR13-HS-CBA-1H | 20.00 | 15.93 | 0.0117 | 0.0112 |
| SR13-HS-CBA-2H | 24.89 | 16.74 | 0.0138 | 0.0163 | |
| SR13-HS-CBA-3H | 29.33 | 17.56 | 0.0175 | 0.0215 | |
| Cusson and Paultre 1995 [41] | SR13-HS-CBA-1H | 20.00 | 19.73 | 0.0117 | 0.0079 |
| SR13-HS-CBA-2H | 24.89 | 22.61 | 0.0138 | 0.0123 | |
| SR13-HS-CBA-3H | 29.33 | 25.07 | 0.0175 | 0.0186 | |
| Wang et al. 2012 [45] | SR13-HS-CBA-1H | 20.00 | 12.02 | 0.0117 | 0.0351 |
| SR13-HS-CBA-2H | 24.89 | 17.04 | 0.0138 | 0.0605 | |
| SR13-HS-CBA-3H | 29.33 | 21.19 | 0.0175 | 0.0868 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Saingam, P.; Hussain, Q.; Sua-iam, G.; Nawaz, A.; Ejaz, A. Hemp Fiber-Reinforced Polymers Composite Jacketing Technique for Sustainable and Environment-Friendly Concrete. Polymers 2024, 16, 1774. https://doi.org/10.3390/polym16131774
Saingam P, Hussain Q, Sua-iam G, Nawaz A, Ejaz A. Hemp Fiber-Reinforced Polymers Composite Jacketing Technique for Sustainable and Environment-Friendly Concrete. Polymers. 2024; 16(13):1774. https://doi.org/10.3390/polym16131774
Chicago/Turabian StyleSaingam, Panumas, Qudeer Hussain, Gritsada Sua-iam, Adnan Nawaz, and Ali Ejaz. 2024. "Hemp Fiber-Reinforced Polymers Composite Jacketing Technique for Sustainable and Environment-Friendly Concrete" Polymers 16, no. 13: 1774. https://doi.org/10.3390/polym16131774
APA StyleSaingam, P., Hussain, Q., Sua-iam, G., Nawaz, A., & Ejaz, A. (2024). Hemp Fiber-Reinforced Polymers Composite Jacketing Technique for Sustainable and Environment-Friendly Concrete. Polymers, 16(13), 1774. https://doi.org/10.3390/polym16131774