Improved Mechanical Performance in FDM Cellular Frame Structures through Partial Incorporation of Faces
Abstract
1. Introduction
2. Materials and Methods
2.1. Material, Lattice Design, and Fabrication
2.2. Lattice Compression Testing
2.3. Density of the Lattice
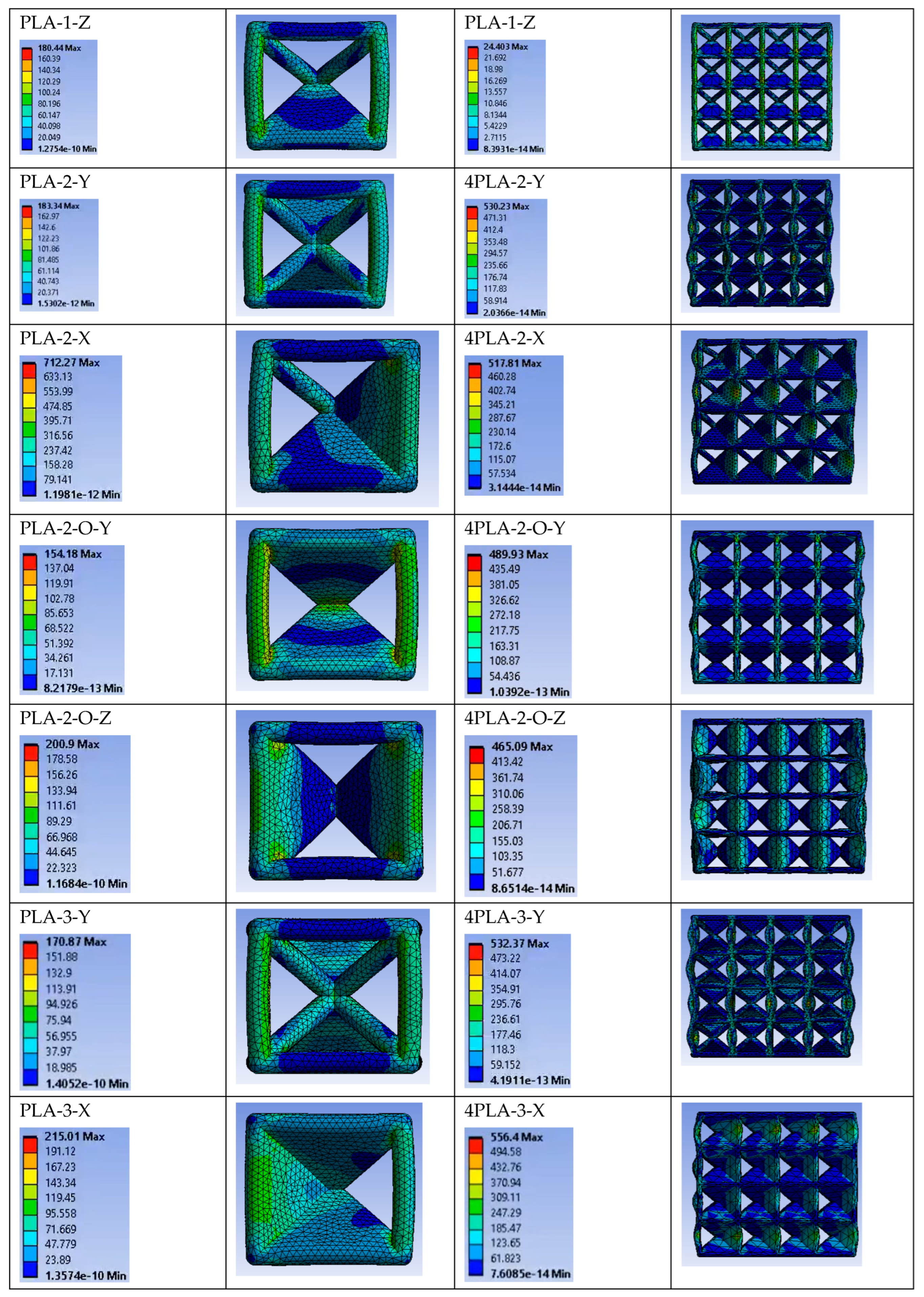
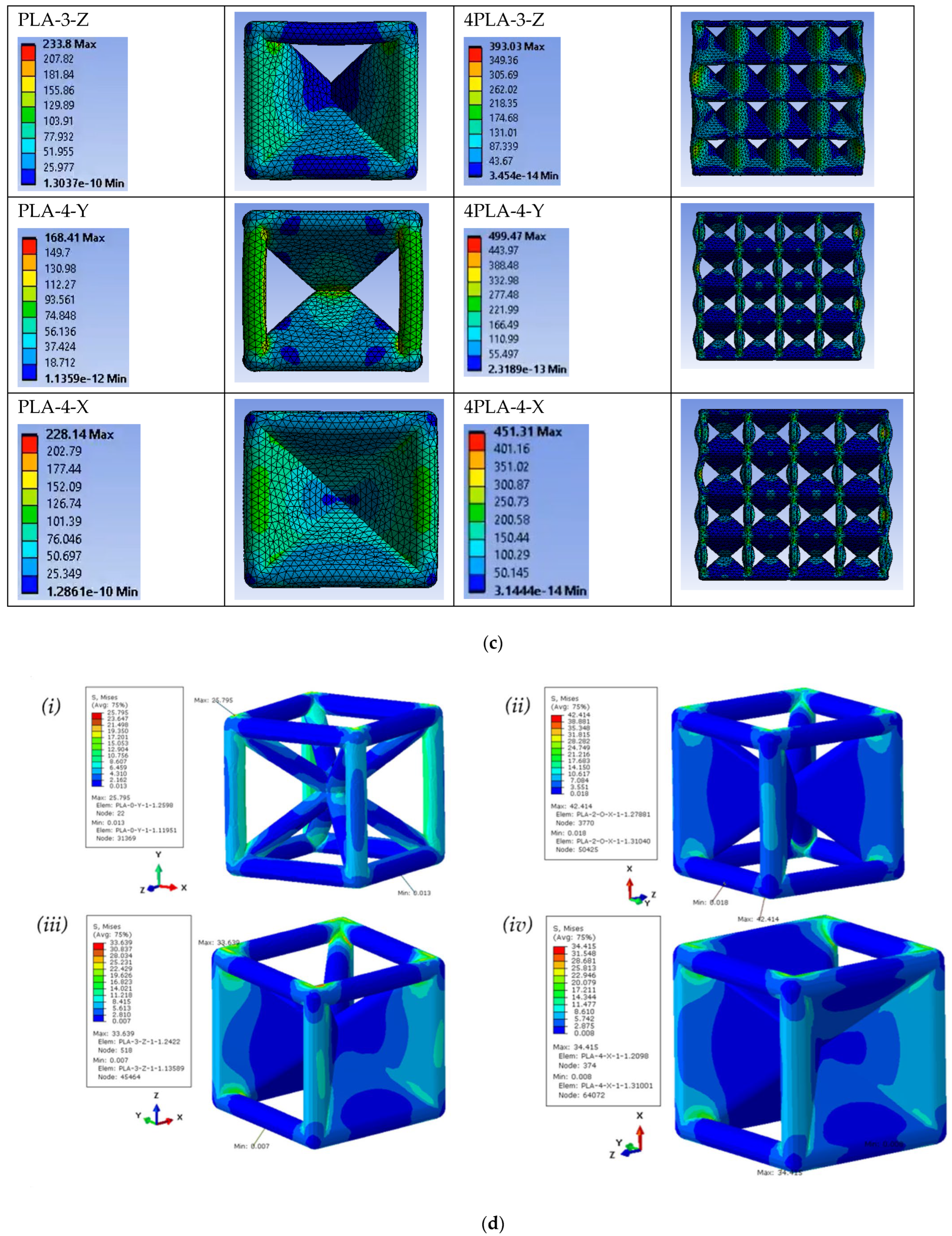
2.4. Finite Element Analysis Boundary Conditions
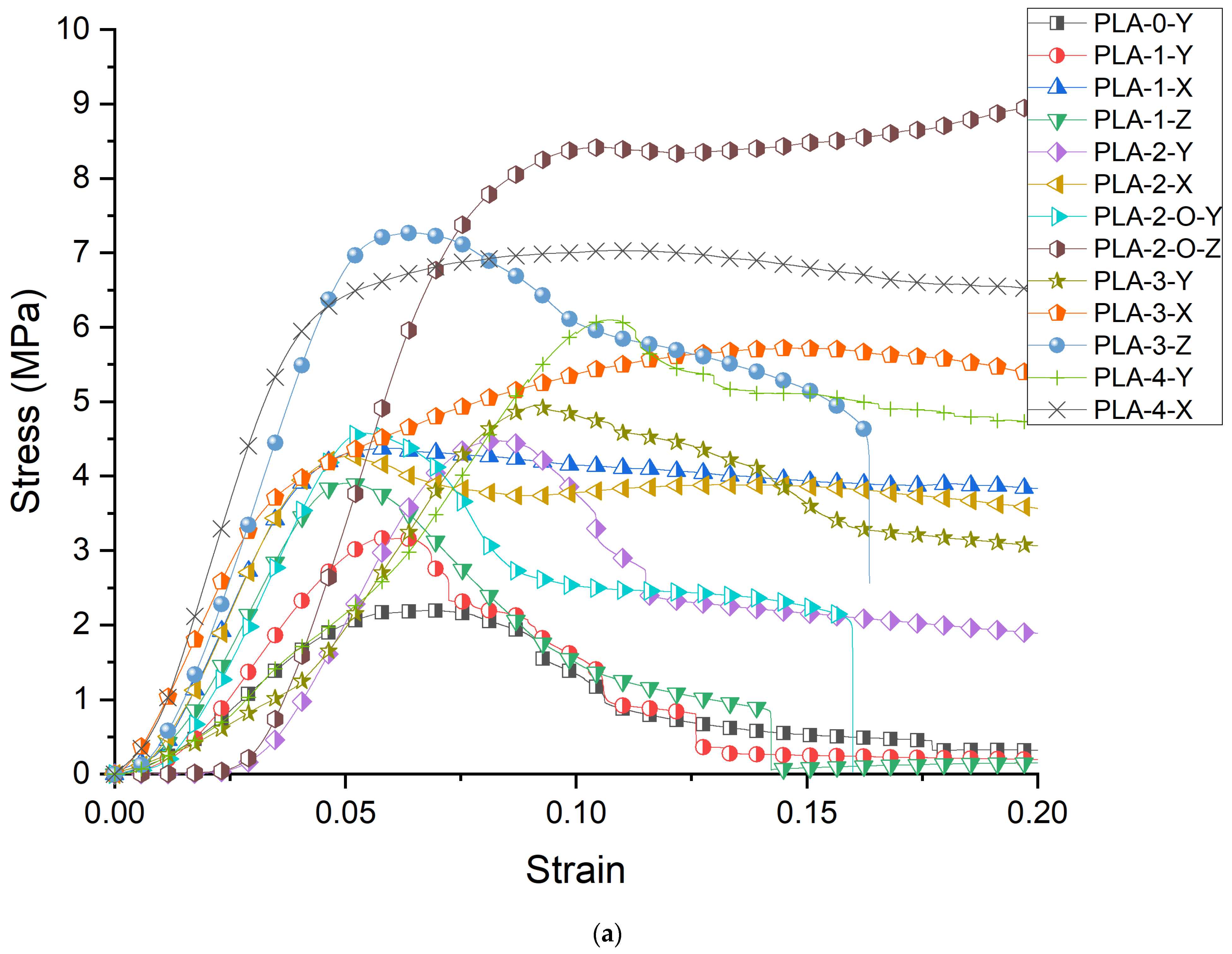
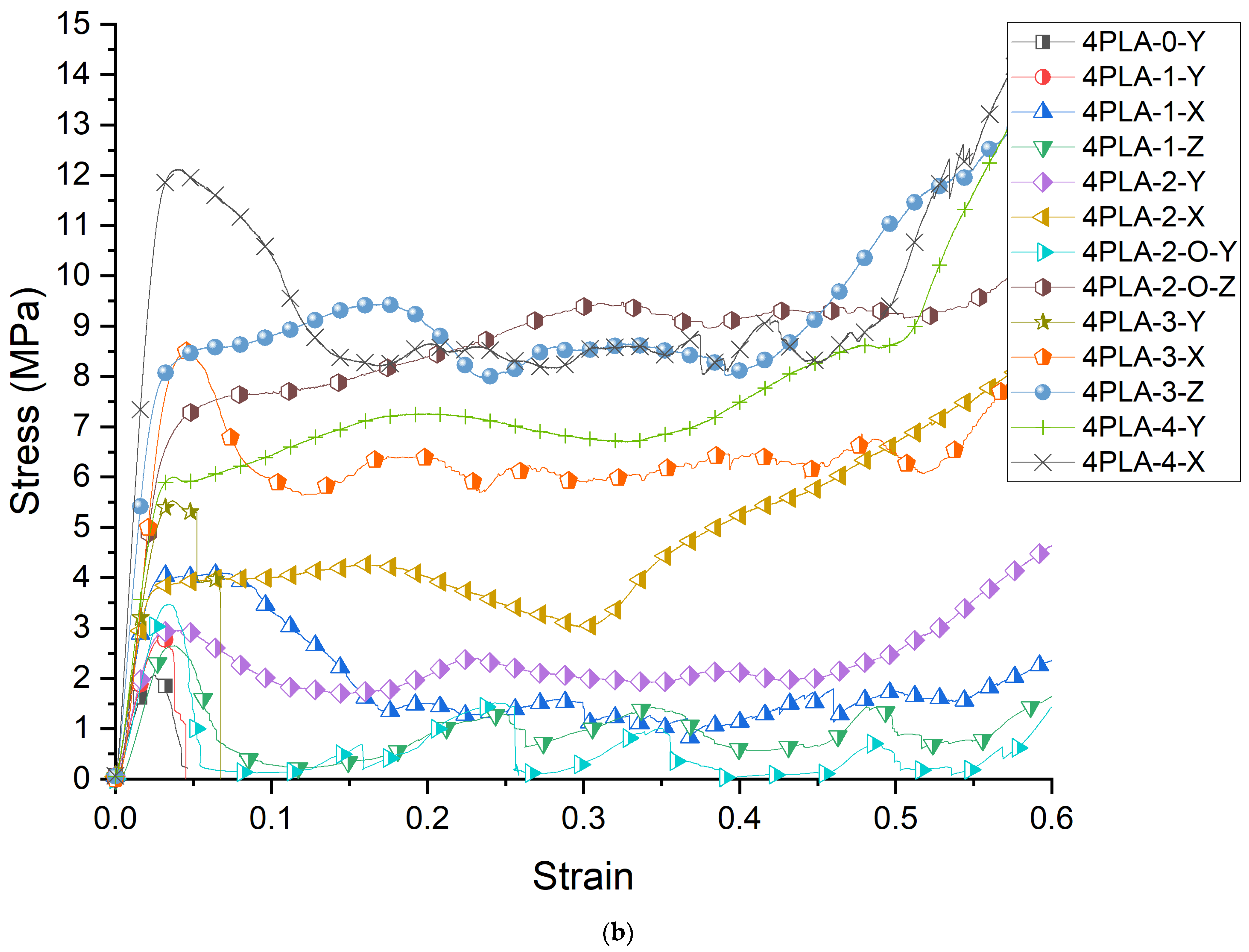
3. Results and Discussion
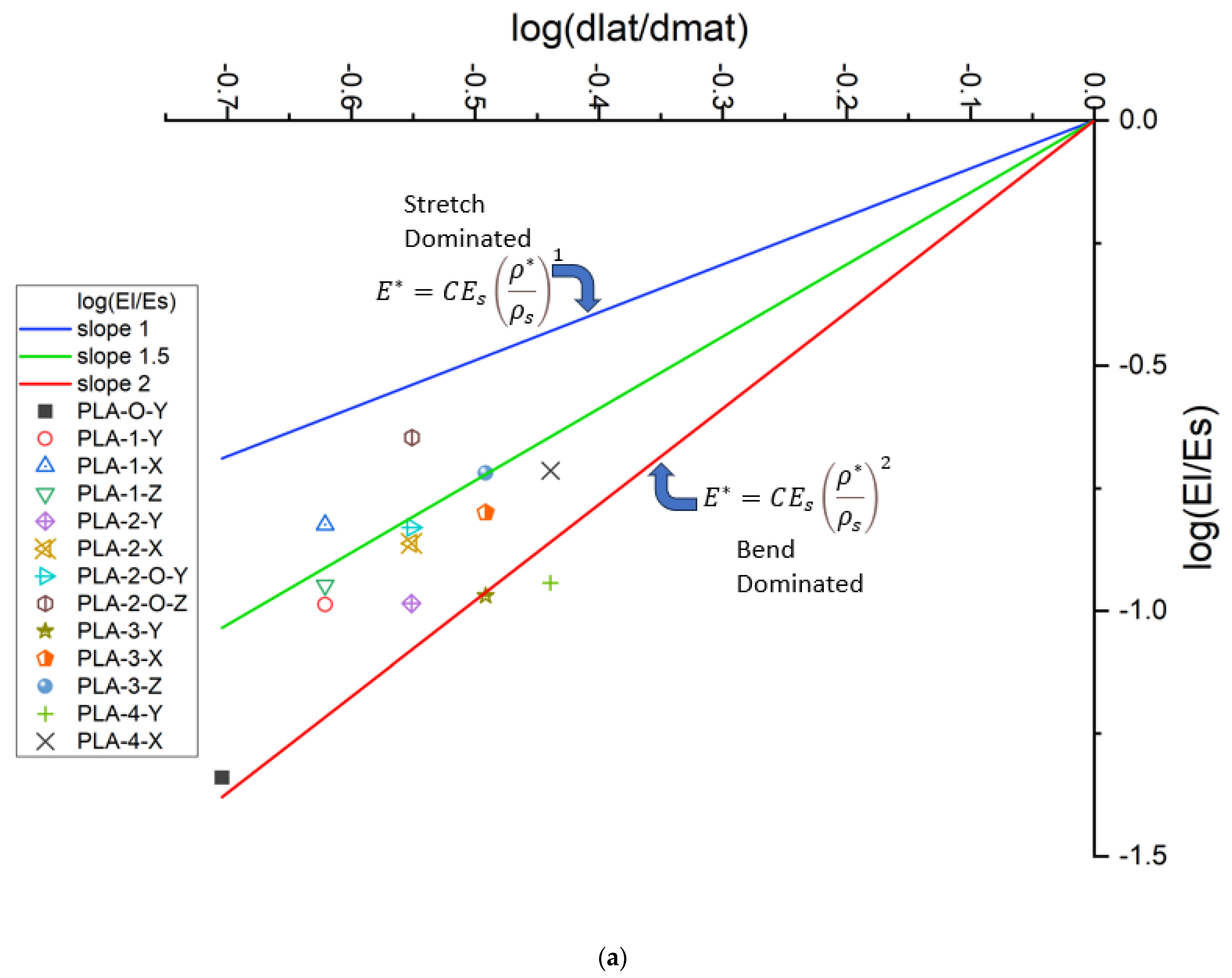
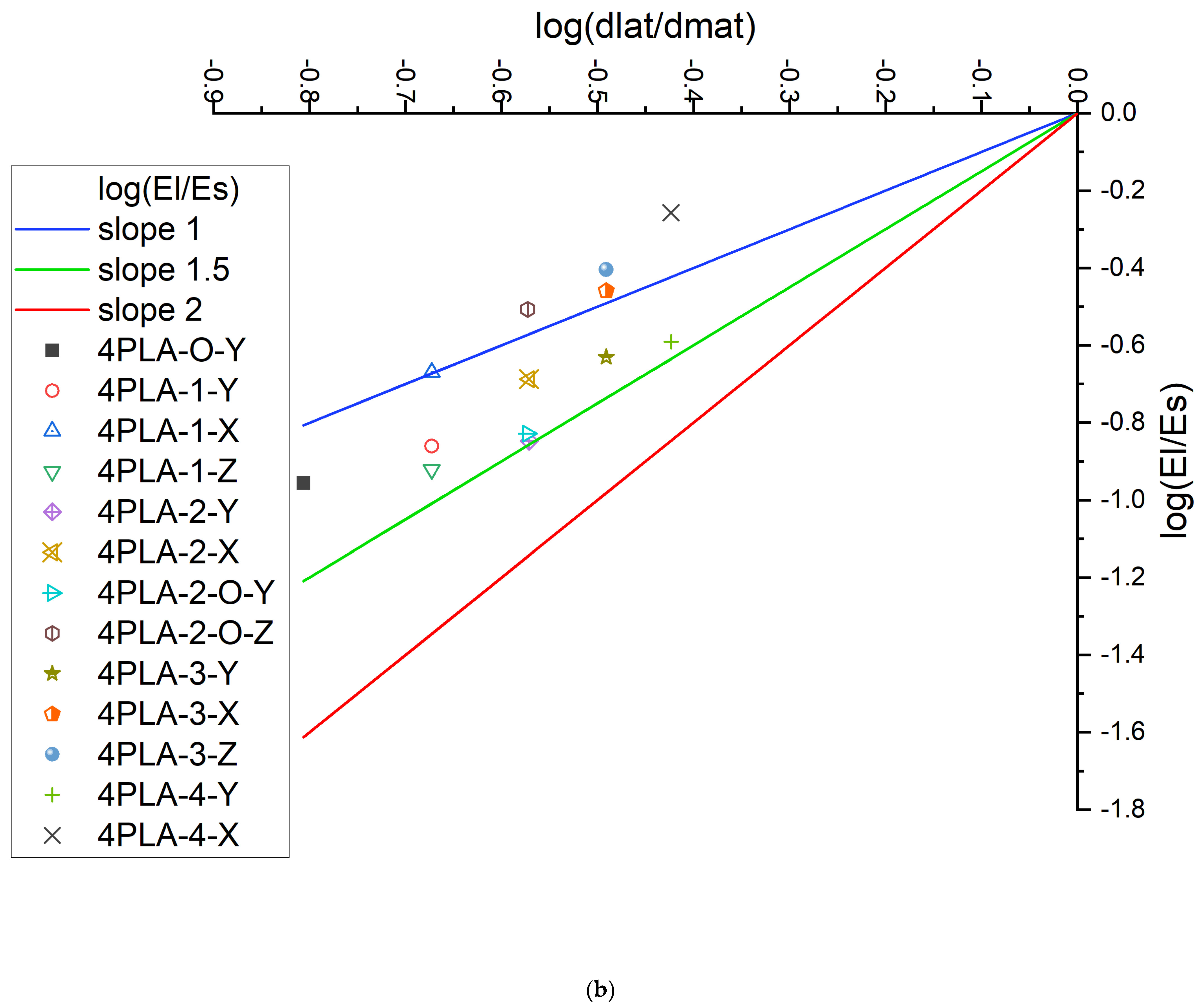
3.1. Effect of the Addition of Faces on the Unit Cell
3.2. Energy Effects
3.3. Transformation in Deformation Mechanism from Addition of Plates
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Gibson, L.J.; Ashby, M.F. Cellular Solids: Structure and Properties; Cambridge University Press: Cambridge, UK, 1997. [Google Scholar]
- Lim, J.-H.; Kang, K.-J. Mechanical behavior of sandwich panels with tetrahedral and Kagome truss cores fabricated from wires. Int. J. Solids Struct. 2006, 43, 5228–5246. [Google Scholar] [CrossRef]
- Bonatti, C.; Mohr, D. Large deformation response of additively-manufactured FCC metamaterials: From octet truss lattices towards continuous shell mesostructures. Int. J. Plast. 2017, 92, 122–147. [Google Scholar] [CrossRef]
- Maconachie, T.; Leary, M.; Lozanovski, B.; Zhang, X.; Qian, M.; Faruque, O.; Brandt, M. SLM lattice structures: Properties, performance applications and challenges. Mater. Des. 2019, 183, 108137. [Google Scholar] [CrossRef]
- Tancogne-Dejean, T.; Diamantopoulou, M.; Gorji, M.B.; Bonatti, C.; Mohr, D. 3D Plate-Lattices: An Emerging Class of Low-Density Metamaterial Exhibiting Optimal Isotropic Stiffness. Adv. Mater. 2018, 30, 1803334. [Google Scholar] [CrossRef] [PubMed]
- Bitzer, T. Honeycomb Technology; Springer: Dordrecht, The Netherlands, 1997. [Google Scholar] [CrossRef]
- Oluwabunmi, K.; D’souza, N.A.; Zhao, W.; Choi, T.-Y.; Theyson, T. Compostable, fully biobased foams using PLA and micro cellulose for zero energy buildings. Sci. Rep. 2020, 10, 17771. [Google Scholar] [CrossRef] [PubMed]
- Ashby, M.F. The properties of foams and lattices. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2006, 364, 15–30. [Google Scholar] [CrossRef]
- Deshpande, V.S.; Fleck, N.A.; Ashby, M.F. Effective properties of the octet-truss lattice material. J. Mech. Phys. Solids 2001, 49, 1747–1769. [Google Scholar] [CrossRef]
- Pellegrino, S.; Calladine, C. Matrix analysis of statically and kinematically indeterminate frameworks. Int. J. Solids Struct. 1986, 22, 409–428. [Google Scholar] [CrossRef]
- Deshpande, V.S.; Ashby, M.F.; Fleck, N.A. Foam topology: Bending versus stretching dominated architectures. Acta Mater. 2001, 49, 1035–1040. [Google Scholar] [CrossRef]
- Meza, L.R.; Das, S.; Greer, J.R. Strong, lightweight, and recoverable three-dimensional ceramic nanolattices. Science 2014, 345, 1322–1326. [Google Scholar] [CrossRef]
- Evans, A. Lightweight Materials and Structures. MRS Bull. 2001, 26, 790–797. [Google Scholar] [CrossRef]
- Tancogne-Dejean, T.; Mohr, D. Elastically-isotropic elementary cubic lattices composed of tailored hollow beams. Extrem. Mech. Lett. 2018, 22, 13–18. [Google Scholar] [CrossRef]
- Xu, S.; Shen, J.; Zhou, S.; Huang, X.; Xie, Y.M. Design of lattice structures with controlled anisotropy. Mater. Des. 2016, 93, 443–447. [Google Scholar] [CrossRef]
- Messner, M.C. Optimal lattice-structured materials. J. Mech. Phys. Solids 2016, 96, 162–183. [Google Scholar] [CrossRef]
- Tancogne-Dejean, T.; Mohr, D. Elastically-isotropic truss lattice materials of reduced plastic anisotropy. Int. J. Solids Struct. 2018, 138, 24–39. [Google Scholar] [CrossRef]
- Gurtner, G.; Durand, M. Stiffest elastic networks. Proc. R. Soc. A Math. Phys. Eng. Sci. 2014, 470, 20130611. [Google Scholar] [CrossRef]
- Liu, Z.; Zhao, R.; Tao, C.; Wang, Y.; Liang, X. Mechanical Performance of a Node-Reinforced Body-Centered Cubic Lattice Structure: An Equal-Strength Concept Design. Aerospace 2023, 11, 4. [Google Scholar] [CrossRef]
- Wang, P.; Yang, F.; Zhao, J. Compression behaviors and mechanical properties of modified face-centered cubic lattice structures under quasi-static and high-speed loading. Materials 2022, 15, 1949. [Google Scholar] [CrossRef]
- Li, B.; Shen, C. Solid Stress-Distribution-Oriented Design and Topology Optimization of 3D-Printed Heterogeneous Lattice Structures with Light Weight and High Specific Rigidity. Polymers 2022, 14, 2807. [Google Scholar] [CrossRef]
- Lohmuller, P.; Favre, J.; Piotrowski, B.; Kenzari, S.; Laheurte, P. Stress Concentration and Mechanical Strength of Cubic Lattice Architectures. Materials 2018, 11, 1146. [Google Scholar] [CrossRef]
- Boniotti, L.; Foletti, S.; Beretta, S.; Patriarca, L. Analysis of strain and stress concentrations in micro-lattice structures manufactured by SLM. Rapid Prototyp. J. 2020, 26, 370–380. [Google Scholar] [CrossRef]
- Berger, J.B.; Wadley, H.N.G.; McMeeking, R.M. Mechanical metamaterials at the theoretical limit of isotropic elastic stiffness. Nature 2017, 543, 533–537. [Google Scholar] [CrossRef] [PubMed]
- Medori, E. Mechanical Behavior of FDM Printed Lattice Structures with Potential for Biomedical Application. Master’s Thesis, Norwegian University of Science and Technology, Trondheim, Norway, 2021. [Google Scholar]
- ASTM D638-14; Standard Test Method for Tensile Properties of Plastics. ASTM International: West Conshohocken, PA, USA, 2014.
- ASTM D695-15; Standard Test Method for Compressive Properties of Rigid Plastics. ASTM International: West Conshohocken, PA, USA, 2015.
- ASTM D790-17; Standard Test Methods for Flexural Properties of Unreinforced and Reinforced Plastics and Electrical Insulating Materials. Annual book of ASTM Standards. ASTM International: West Conshohocken, PA, USA, 1997.
- Righetti, M.C.; Gazzano, M.; Di Lorenzo, M.L.; Androsch, R. Enthalpy of melting of α′- and α-crystals of poly(l-lactic acid). Eur. Polym. J. 2015, 70, 215–220. [Google Scholar] [CrossRef]
- Vakharia, V.S.; Kuentz, L.; Salem, A.; Halbig, M.C.; Salem, J.A.; Singh, M. Additive Manufacturing and Characterization of Metal Particulate Reinforced Polylactic Acid (PLA) Polymer Composites. Polymers 2021, 13, 3545. [Google Scholar] [CrossRef] [PubMed]
- Abbot, D.; Kallon, D.; Anghel, C.; Dube, P. Finite Element Analysis of 3D Printed Model via Compression Tests. Procedia Manuf. 2019, 35, 164–173. [Google Scholar] [CrossRef]
- Mishra, A.K.; Chavan, H.; Kumar, A. Effect of material variation on the uniaxial compression behavior of FDM manufactured polymeric TPMS lattice materials. Mater. Today Proc. 2021, 46, 7752–7759. [Google Scholar] [CrossRef]
- Wang, P.; Yang, F.; Li, P.; Zheng, B.; Fan, H. Design and additive manufacturing of a modified face-centered cubic lattice with enhanced energy absorption capability. Extrem. Mech. Lett. 2021, 47, 101358. [Google Scholar] [CrossRef]
- Maskery, I.; Aboulkhair, N.; Aremu, A.; Tuck, C.; Ashcroft, I. Compressive failure modes and energy absorption in additively manufactured double gyroid lattices. Addit. Manuf. 2017, 16, 24–29. [Google Scholar] [CrossRef]
- Li, Q.M.; Magkiriadis, I.; Harrigan, J.J. Compressive strain at the onset of densification of cellular solids. J. Cell. Plast. 2006, 42, 371–392. [Google Scholar] [CrossRef]






| Lattice Types | Center of Mass | X Orientation | Y Orientation | Z Orientation |
|---|---|---|---|---|
| PLA-0 | (0, 0, 0) |  |  |  |
| PLA-1 | (0.58856, 0, 0) | 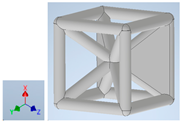 |  |  |
| PLA-2 | (0.50247, 0, −0.50224) |  | 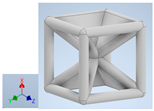 |  |
| PLA-2-O | (0, 0, 0) | 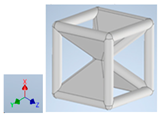 |  | 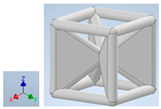 |
| PLA-3 | (0, 0, −0.43692) | 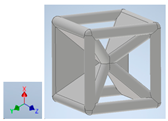 | 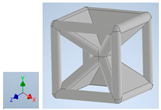 |  |
| PLA-4 | (0, 0, 0) | 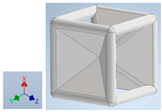 |  | 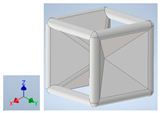 |
| Modulus (MPa) | Yield Point (MPa) | Strain to Failure | |
|---|---|---|---|
| Flexural Modulus | 2031.69 ± 39.35 | 43.60 ± 8.07 | 1.54% |
| Tensile tests | 1036.83 ± 35.69 | 34.84 ± 2.69 | 4.50% |
| Compression tests | 903.84 ± 61.33 | 56.60 ± 2.99 | No Failure |
| Lattice Types | Mass Moment of Inertia (kg.m2) | Density-Lattice (kg/m3) | Modulus (MPa) | % Change in Modulus with Respect to Open Cell | Simulation Modulus (Mpa) | Sp. Modulus Mpa-m3/kg | % Change in Sp. Modulus with Respect to Open Cell | Yield Strength (MPa) | % Change in Yield Strength with Respect to Open Cell | Sp. Yield Strength*103 Mpa-m3/kg | % Change in Sp. Yield Strength with Respect to Open Cell | Truncated EA (MJ/m3) | % Change in Truncated EA Strength with Respect to Open Cell | Truncated SEA (J/g) | % Change in Truncated SEA Strength with Respect to Open Cell | EA (MJ/m3) | % Change in EA Strength with Respect to Open Cell | SEA (J/g) | % Change in SEA Strength with Respect to Open Cell |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| PLA-O-Y | 9.70 × 10−09 | 229.12 | 41.34 ± 10.42 | 0.00 | 62.25 | 0.29 | 0.00 | 2.14 ± 0.39 | 0.00 | 9.20 | 0.00 | 0.19 | 0.00 | 0.82 | 0.00 | 0.80 | 0.00 | 3.48 | 0.00 |
| PLA-1-Y | 1.05 × 10−08 | 277.67 | 93.10 ± 7.22 | 125.21 | 73.31 | 0.29 | 0.18 | 1.96 ± 0.41 | −8.41 | 12.40 | 34.78 | 0.22 | 17.12 | 0.79 | −3.36 | 0.97 | 21.25 | 3.49 | 0.05 |
| PLA-1-X | 1.12 × 10−08 | 277.67 | 135.35 ± 6.47 | 227.41 | 106.32 | 0.51 | 76.46 | 4.67 ± 0.52 | 118.22 | 16.38 | 77.99 | 0.56 | 200.05 | 2.03 | 147.59 | 1.08 | 35.00 | 3.89 | 11.40 |
| PLA-1-Z | 1.06 × 10−08 | 277.67 | 101.88 ± 8.63 | 146.44 | 63.41 | 0.37 | 28.43 | 3.68 ± 0.17 | 71.96 | 13.26 | 44.13 | 0.28 | 48.01 | 1 | 22.13 | 0.48 | −40.00 | 1.73 | −50.49 |
| PLA-2-Y | 1.14 × 10−08 | 325.89 | 93.53 ± 11.65 | 126.25 | 83.00 | 0.32 | 12.72 | 2.97 ± 0.39 | 38.79 | 13.18 | 43.24 | 0.36 | 91.54 | 1.1 | 34.67 | 1.22 | 52.50 | 3.74 | 7.22 |
| PLA-2-X | 1.21 × 10−08 | 325.89 | 124.10 ± 37.52 | 200.19 | 118.99 | 0.50 | 73.58 | 5.64 ± 0.07 | 163.55 | 13.00 | 41.35 | 0.53 | 184.63 | 1.64 | 100.12 | 1.14 | 42.50 | 3.49 | 0.19 |
| PLA-2-O-Y | 1.16 × 10−08 | 326.22 | 133.66 ± 1.73 | 223.32 | 72.78 | 0.42 | 47.02 | 4.93 ± 0.22 | 130.37 | 13.92 | 51.29 | 0.41 | 118.80 | 1.26 | 53.67 | 0.39 | −51.25 | 1.19 | −65.76 |
| PLA-2-O-Z | 1.29 × 10−08 | 326.22 | 203.92 ± 5.51 | 393.28 | 113.34 | 0.63 | 120.38 | 8.84 ± 0.25 | 313.08 | 23.35 | 153.78 | 0.88 | 368.25 | 2.69 | 228.88 | 13.82 | 1627.50 | 42.37 | 1113.32 |
| PLA-3-Y | 1.25 × 10−08 | 374.10 | 97.00 ± 12.60 | 134.64 | 99.93 | 0.27 | −5.90 | 3.34 ± 0.40 | 56.07 | 13.08 | 42.23 | 0.49 | 158.92 | 1.3 | 58.58 | 1.88 | 135.00 | 5.01 | 43.93 |
| PLA-3-X | 1.30 × 10−08 | 374.10 | 143.48 ± 6.78 | 247.07 | 108.33 | 0.47 | 64.64 | 7.19 ± 0.31 | 235.98 | 11.77 | 27.97 | 0.71 | 276.63 | 1.89 | 130.67 | 0.90 | 12.50 | 2.40 | −31.10 |
| PLA-3-Z | 1.38 × 10−08 | 374.10 | 172.63 ± 9.04 | 317.59 | 158.19 | 0.50 | 73.25 | 7.40 ± 0.22 | 245.79 | 19.78 | 115.00 | 0.82 | 337.50 | 2.19 | 167.95 | 1.49 | 86.25 | 3.98 | 14.07 |
| PLA-4-Y | 1.35 × 10−08 | 421.99 | 103.00 ±34.88 | 149.15 | 117.09 | 0.30 | 5.79 | 4.53 ± 0.40 | 111.68 | 14.55 | 58.17 | 0.57 | 204.76 | 1.35 | 65.47 | 2.83 | 253.75 | 6.71 | 92.07 |
| PLA-4-X | 1.47 × 10−08 | 421.99 | 174.37 ± 34.85 | 321.79 | 194.09 | 0.46 | 60.55 | 7.15 ± 0.62 | 234.11 | 16.02 | 74.12 | 0.93 | 395.01 | 2.2 | 168.77 | 1.72 | 115.00 | 4.07 | 16.74 |
| 4PLA-O-Y | 3.58 × 10−06 | 181.20 | 100.13 ± 1.80 | 0.00 | 41.12 | 0.55 | 0.00 | 1.98 ± 0.06 | 0.00 | 10.92 | 0.00 | 0.06 | 0.00 | 0.32 | 0.00 | 0.06 | 0.00 | 0.35 | 0.00 |
| 4PLA-1-Y | 4.69 × 10−06 | 246.45 | 124.41 ± 0.36 | 24.25 | 47.29 | 0.50 | −8.65 | 2.72 ± 0.06 | 37.75 | 11.06 | 1.28 | 0.08 | 37.54 | 0.32 | 1.12 | 0.07 | 16.67 | 0.30 | −14.22 |
| 4PLA-1-X | 4.73 × 10−06 | 246.45 | 193.50 ± 3.13 | 93.25 | 79.38 | 0.79 | 42.08 | 4.02 ± 0.04 | 103.04 | 16.30 | 49.28 | 0.13 | 133.49 | 0.55 | 71.67 | 1.23 | 1950.00 | 5.00 | 1407.22 |
| 4PLA-1-Z | 4.70 × 10−06 | 246.45 | 108.42 ± 2.58 | 8.28 | 71.51 | 0.44 | −20.39 | 2.63 ± 0.02 | 33.06 | 10.68 | −2.17 | 0.07 | 26.33 | 0.3 | −7.12 | 0.65 | 983.33 | 2.64 | 696.50 |
| 4PLA-2-Y | 5.79 × 10−06 | 311.26 | 128.29 ± 3.92 | 28.12 | 59.70 | 0.41 | −25.41 | 2.85 ± 0.17 | 44.26 | 9.17 | −16.02 | 0.10 | 67.81 | 0.31 | −2.31 | 1.56 | 2500.00 | 5.03 | 1413.56 |
| 4PLA-2-X | 5.85 × 10−06 | 311.26 | 185.54 ± 11.42 | 85.30 | 91.13 | 0.60 | 7.87 | 3.66 ± 0.14 | 85.03 | 11.76 | 7.71 | 0.13 | 129.89 | 0.43 | 33.83 | 3.04 | 4966.00 | 9.77 | 2849.50 |
| 4PLA-2-O-Y | 5.80 × 10−06 | 310.25 | 134.25 ± 4.65 | 34.08 | 83.98 | 0.43 | −21.69 | 3.41 ± 0.06 | 72.43 | 10.99 | 0.71 | 0.10 | 74.10 | 0.32 | 1.68 | 0.52 | 766.00 | 1.66 | 406.17 |
| 4PLA-2-O-Z | 5.88 × 10−06 | 310.25 | 281.27 ± 9.64 | 180.90 | 126.73 | 0.91 | 64.06 | 7.60 ± 0.03 | 284.18 | 24.49 | 124.38 | 0.20 | 245.08 | 0.64 | 101.54 | 5.54 | 9133.00 | 17.85 | 5292.61 |
| 4PLA-3-Y | 5.80 × 10−06 | 374.63 | 211.60 ± 5.96 | 111.33 | 77.96 | 0.56 | 2.21 | 5.43 ± 0.17 | 174.41 | 14.49 | 32.72 | 0.17 | 193.03 | 0.45 | 41.73 | 0.78 | 1200.00 | 2.09 | 528.78 |
| 4PLA-3-X | 5.80 × 10−06 | 374.63 | 313.98 ± 13.32 | 213.57 | 125.34 | 0.84 | 51.67 | 8.25 ± 0.23 | 316.92 | 22.01 | 101.66 | 0.22 | 283.95 | 0.59 | 85.71 | 3.91 | 6416.67 | 10.44 | 3051.95 |
| 4PLA-3-Z | 5.88 × 10−06 | 374.63 | 356.72 ± 14.32 | 256.26 | 141.03 | 0.95 | 72.31 | 8.26 ± 0.07 | 317.71 | 22.06 | 102.03 | 0.27 | 360.73 | 0.71 | 122.84 | 5.88 | 9700.00 | 15.68 | 4640.02 |
| 4PLA-4-Y | 9.70 × 10−06 | 437.55 | 231.85 ± 24.11 | 131.55 | 100.32 | 0.53 | −4.11 | 5.41 ± 0.52 | 173.27 | 12.35 | 13.17 | 0.19 | 221.56 | 0.42 | 33.16 | 5.02 | 8266.67 | 11.48 | 3364.79 |
| 4PLA-4-X | 1.00 × 10−05 | 437.55 | 500.11 ± 8.99 | 399.46 | 199.70 | 1.14 | 106.84 | 11.87 ± 0.25 | 500.26 | 27.14 | 148.58 | 0.38 | 553.08 | 0.86 | 170.45 | 5.77 | 9516.67 | 13.18 | 3882.44 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ghosh, M.; D’Souza, N.A. Improved Mechanical Performance in FDM Cellular Frame Structures through Partial Incorporation of Faces. Polymers 2024, 16, 1340. https://doi.org/10.3390/polym16101340
Ghosh M, D’Souza NA. Improved Mechanical Performance in FDM Cellular Frame Structures through Partial Incorporation of Faces. Polymers. 2024; 16(10):1340. https://doi.org/10.3390/polym16101340
Chicago/Turabian StyleGhosh, Mahan, and Nandika Anne D’Souza. 2024. "Improved Mechanical Performance in FDM Cellular Frame Structures through Partial Incorporation of Faces" Polymers 16, no. 10: 1340. https://doi.org/10.3390/polym16101340
APA StyleGhosh, M., & D’Souza, N. A. (2024). Improved Mechanical Performance in FDM Cellular Frame Structures through Partial Incorporation of Faces. Polymers, 16(10), 1340. https://doi.org/10.3390/polym16101340







