Statistical Modeling and Optimization of Electrospinning for Improved Morphology and Enhanced β-Phase in Polyvinylidene Fluoride Nanofibers
Abstract
:1. Introduction
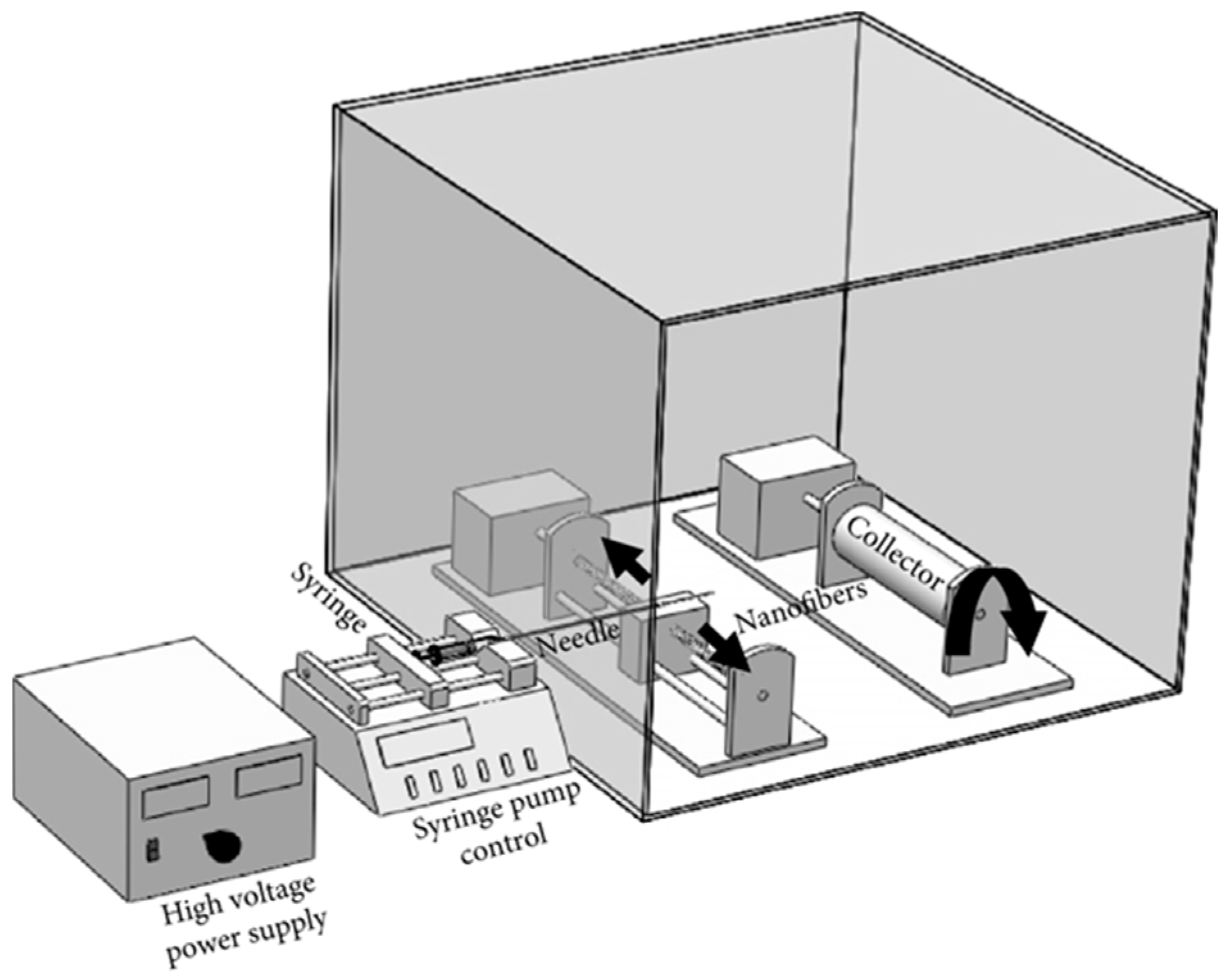
2. Materials and Sample Preparation
Characterization
3. Results and Discussion
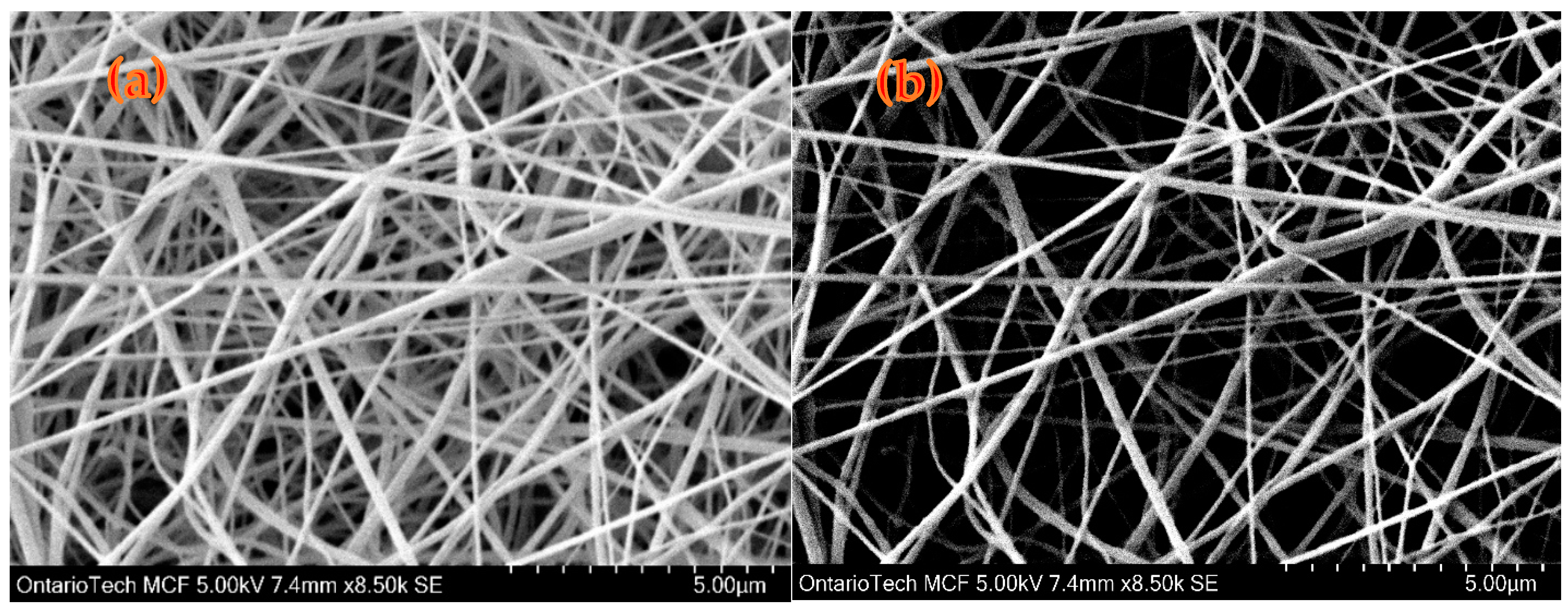
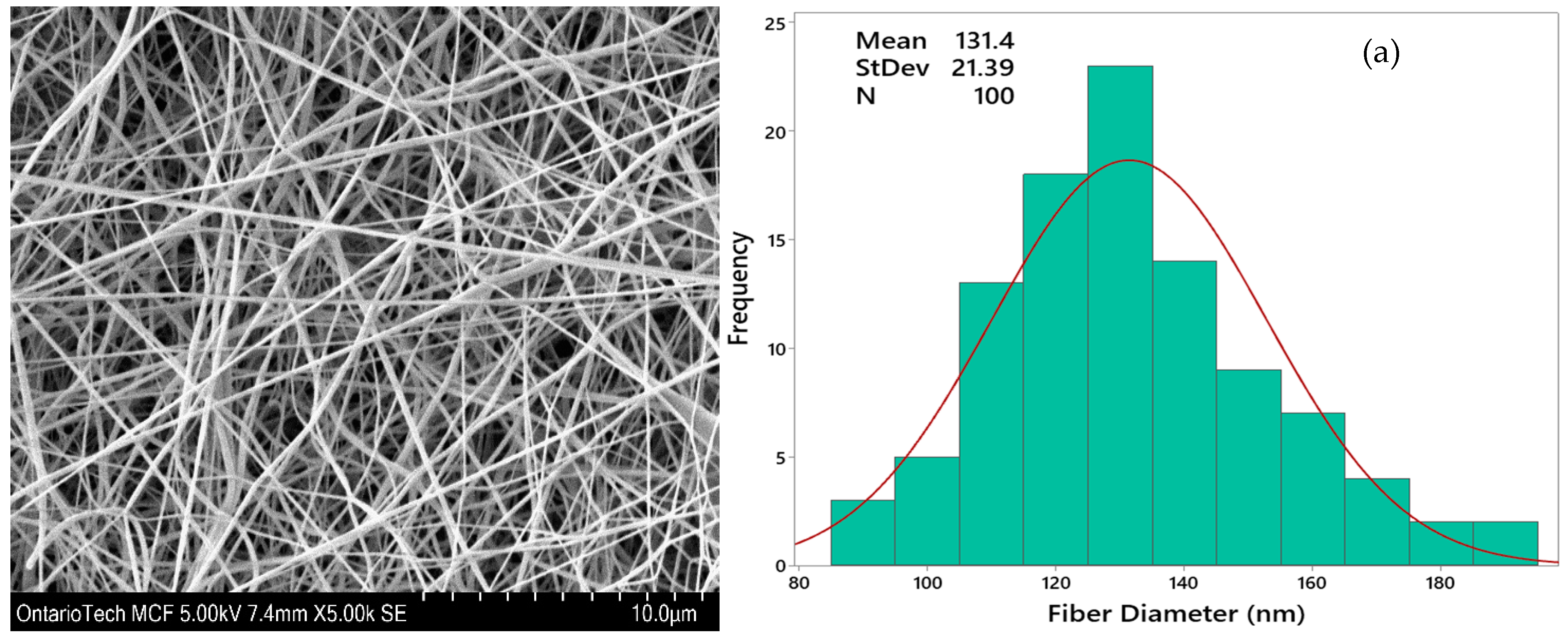
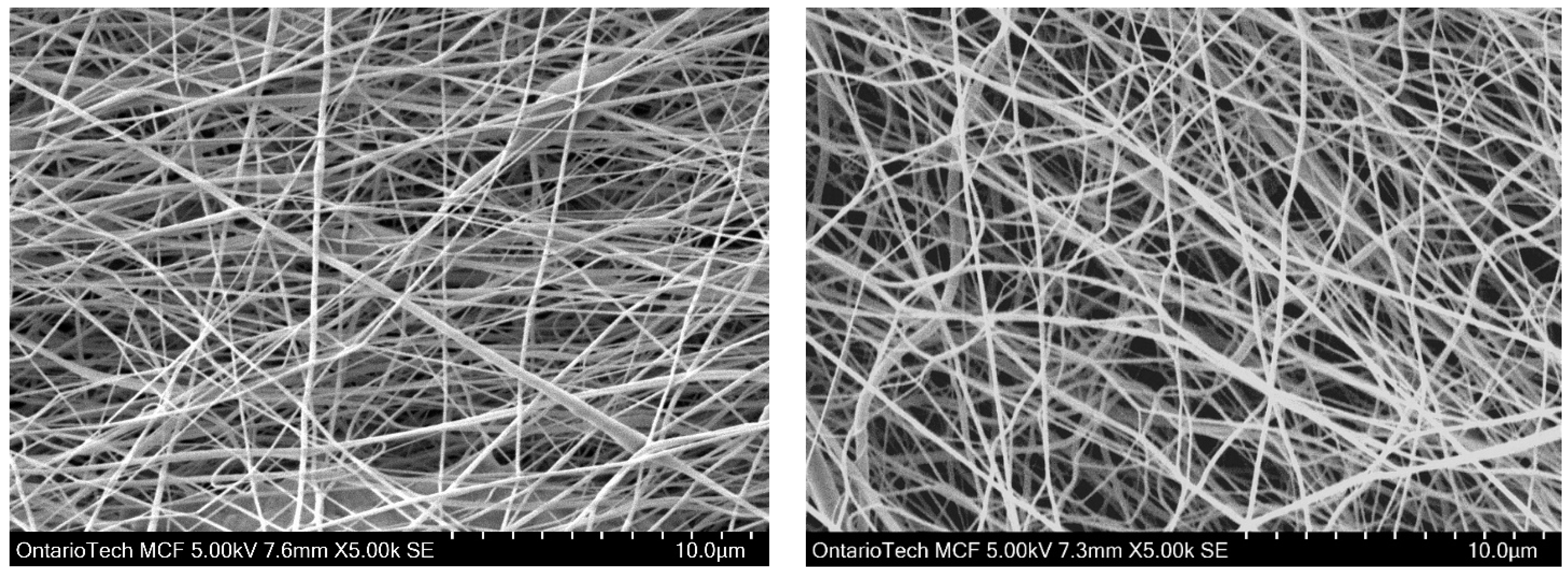
3.1. Morphology of PVDF Nanofibers
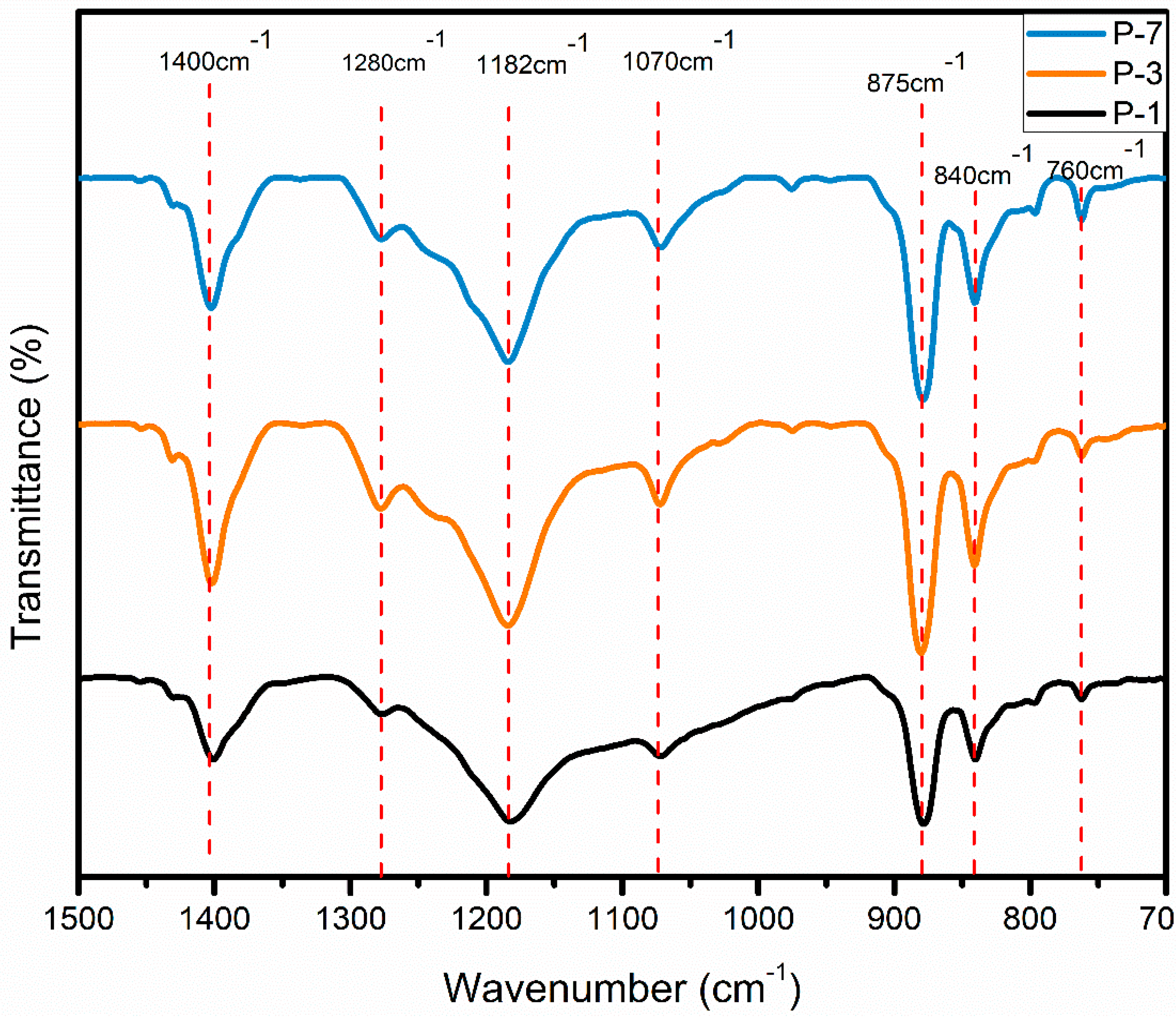
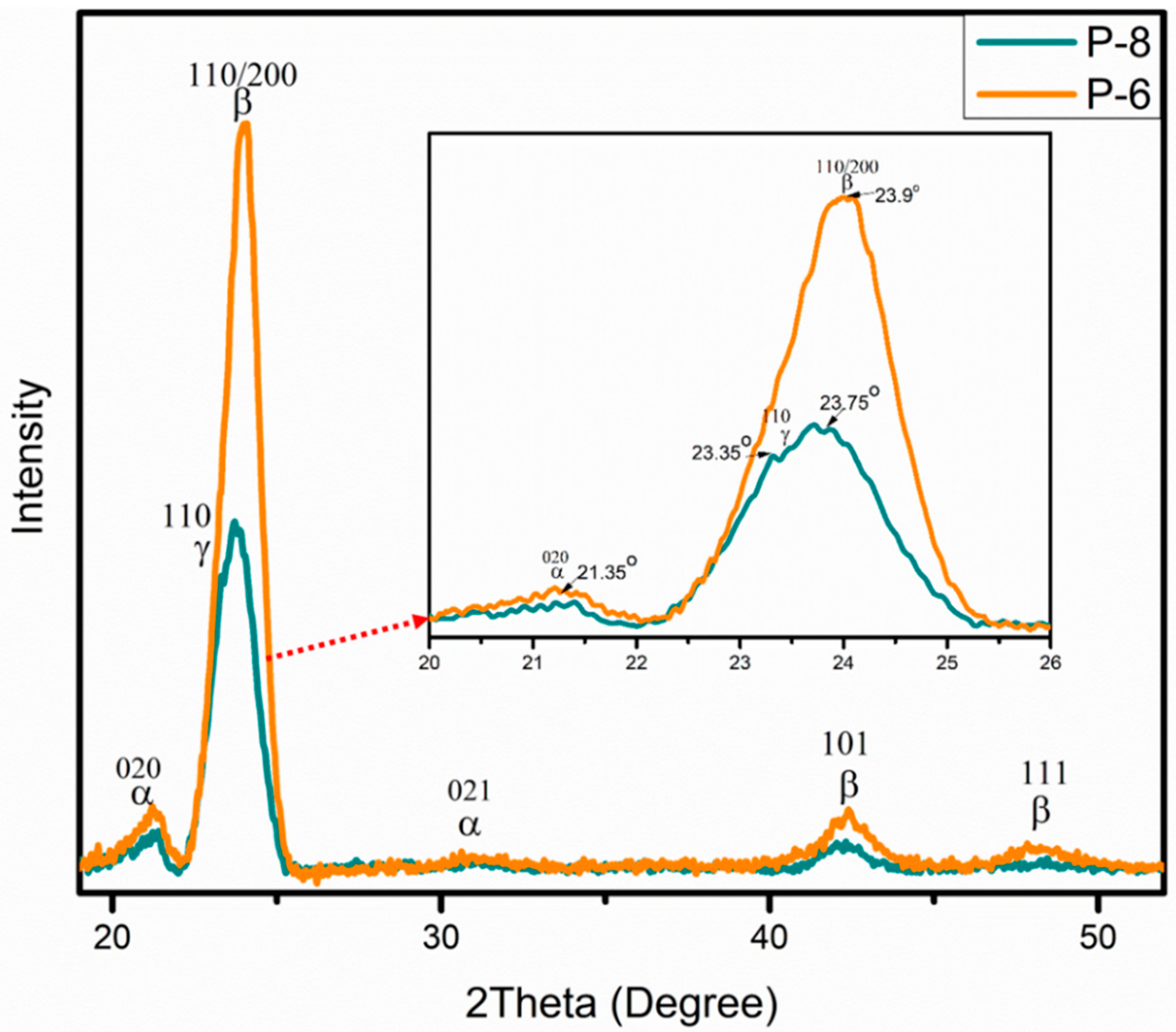
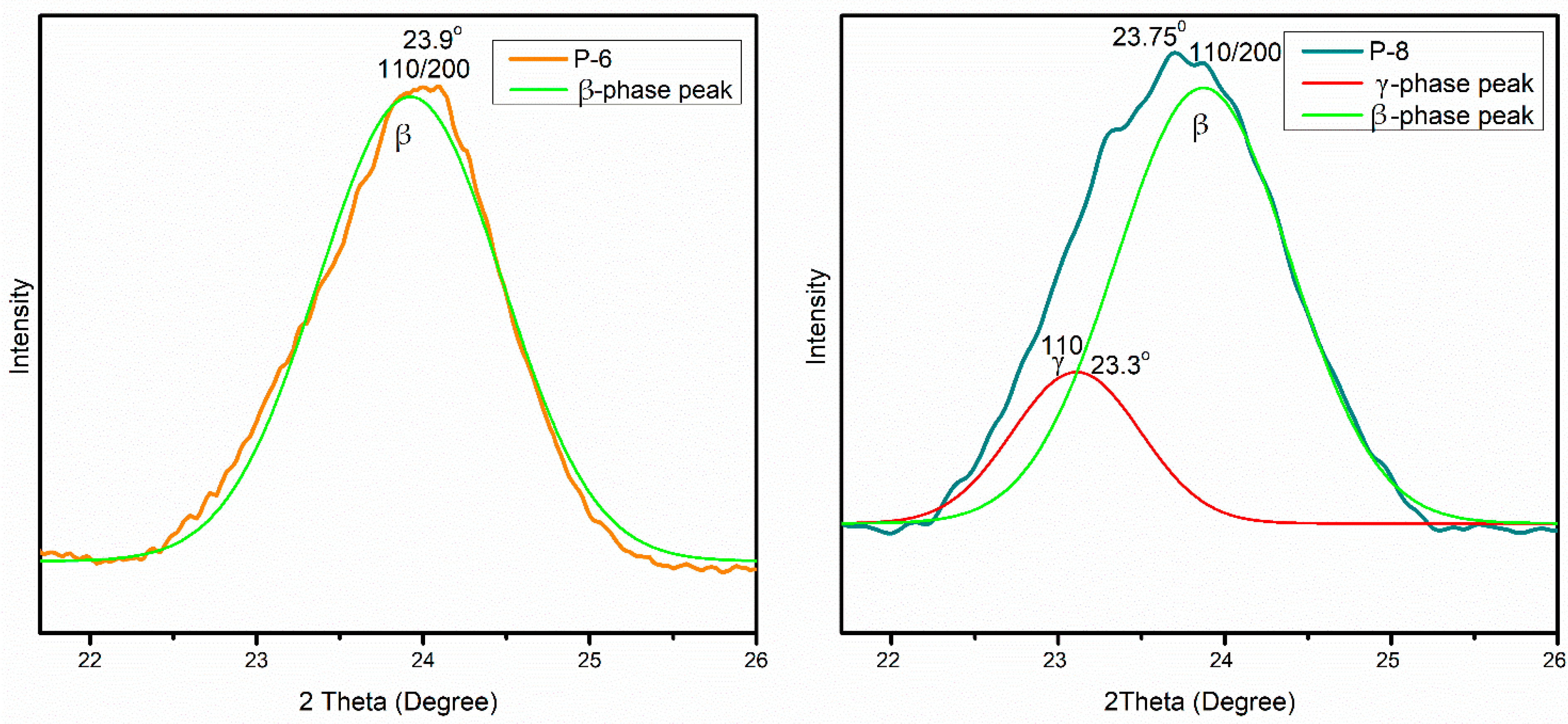
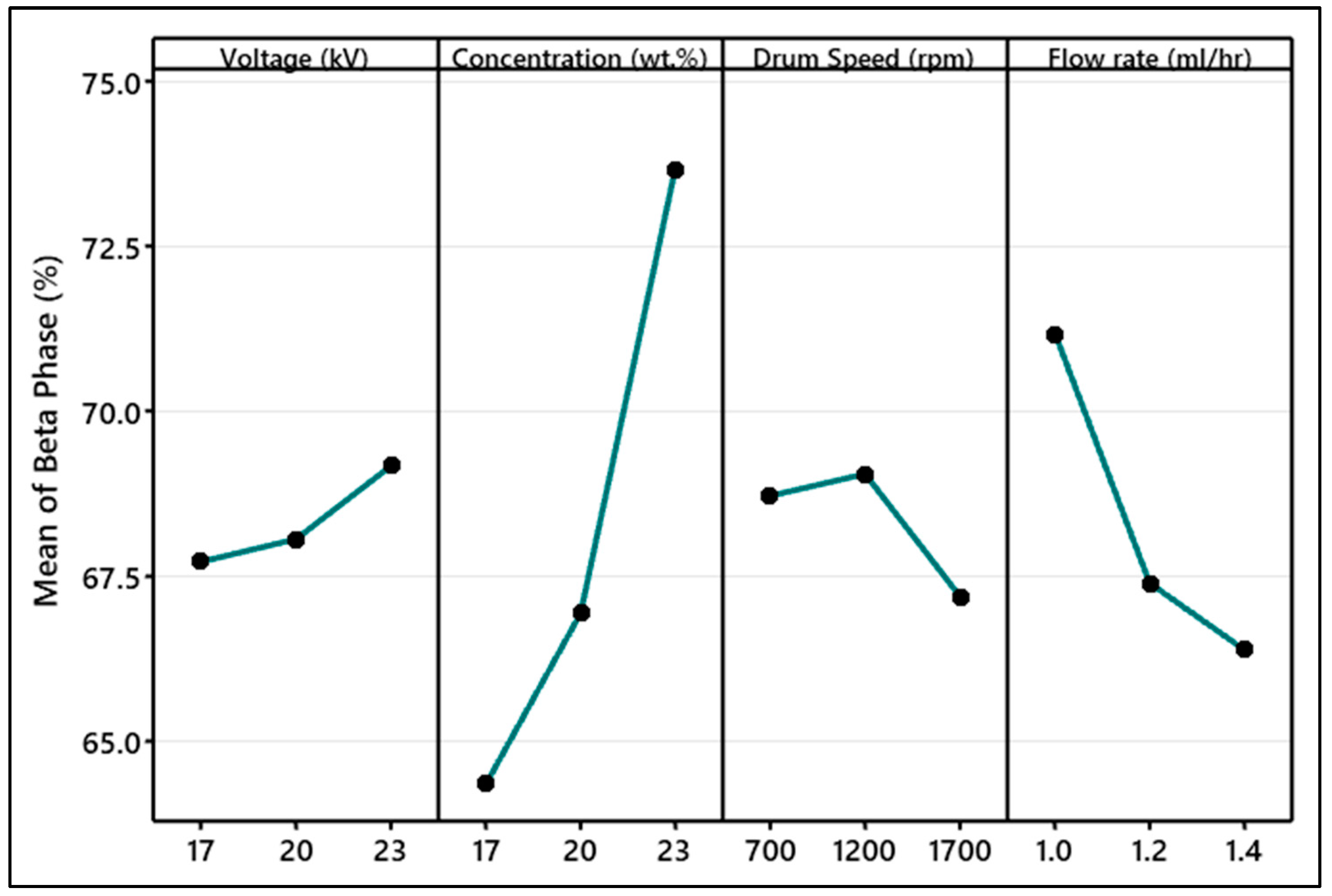
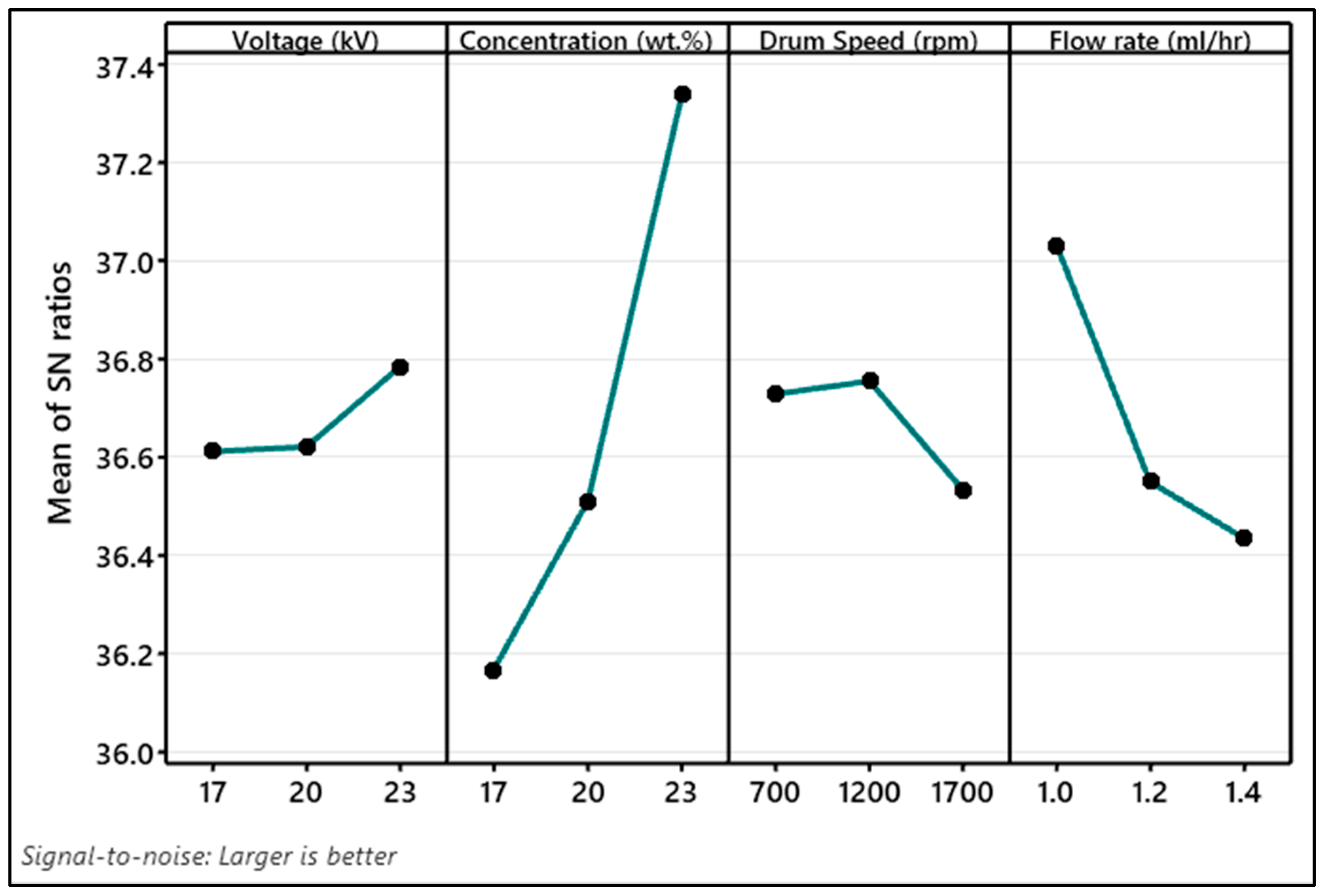
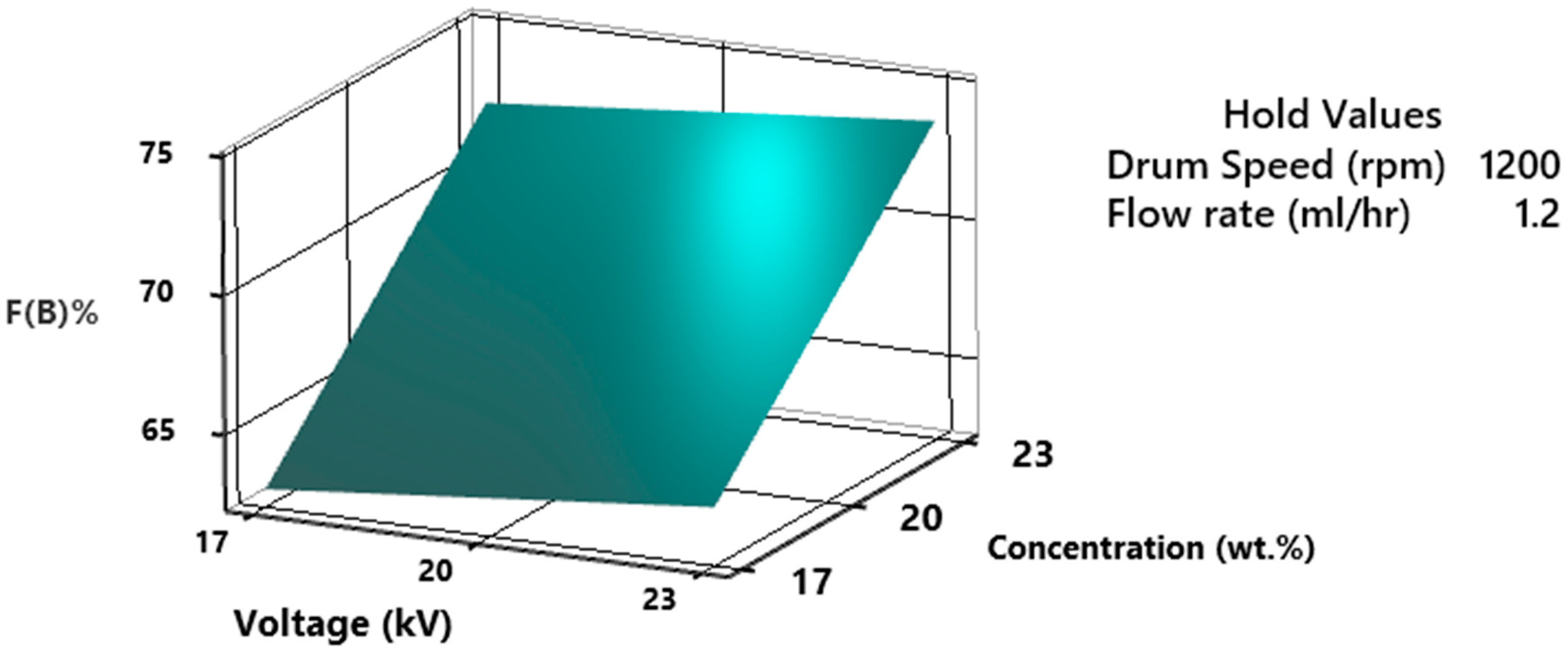
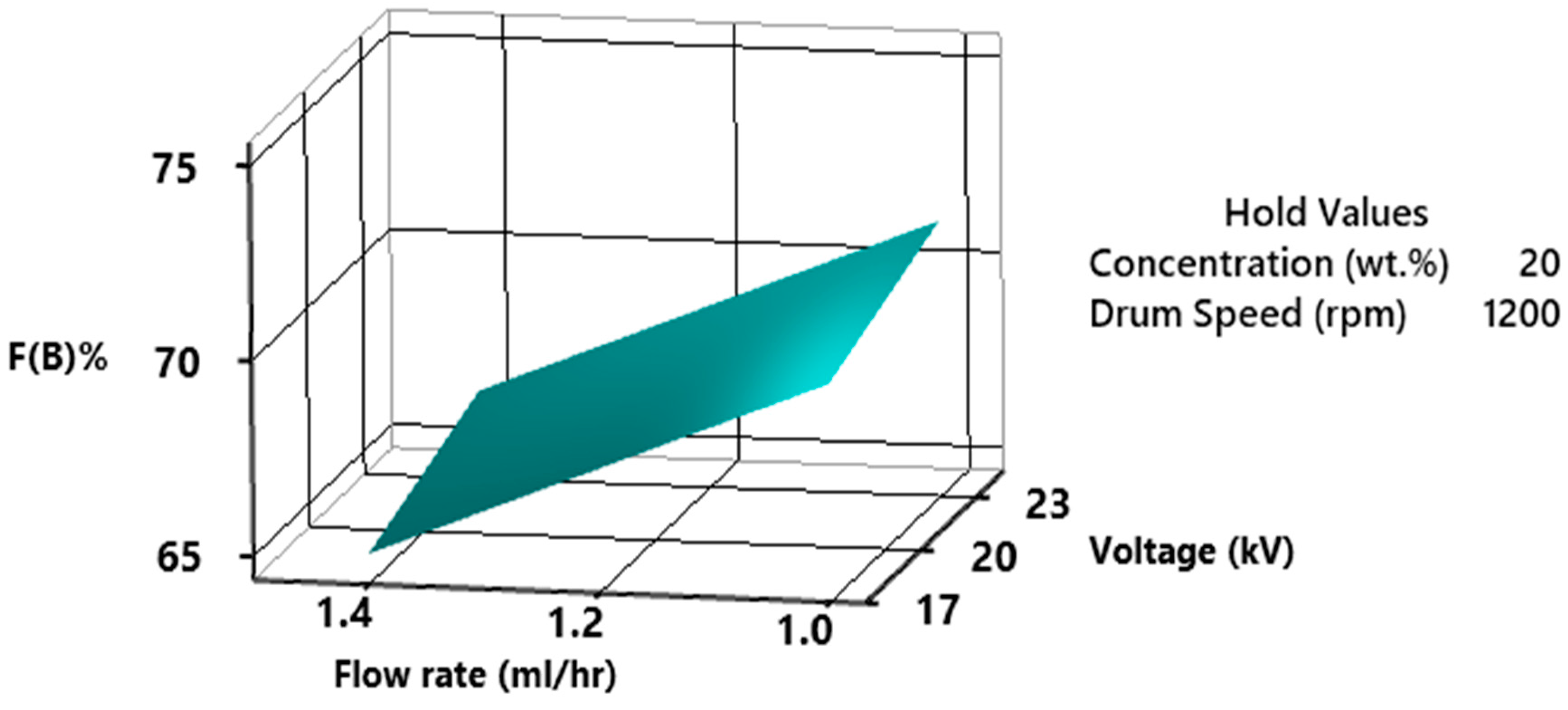
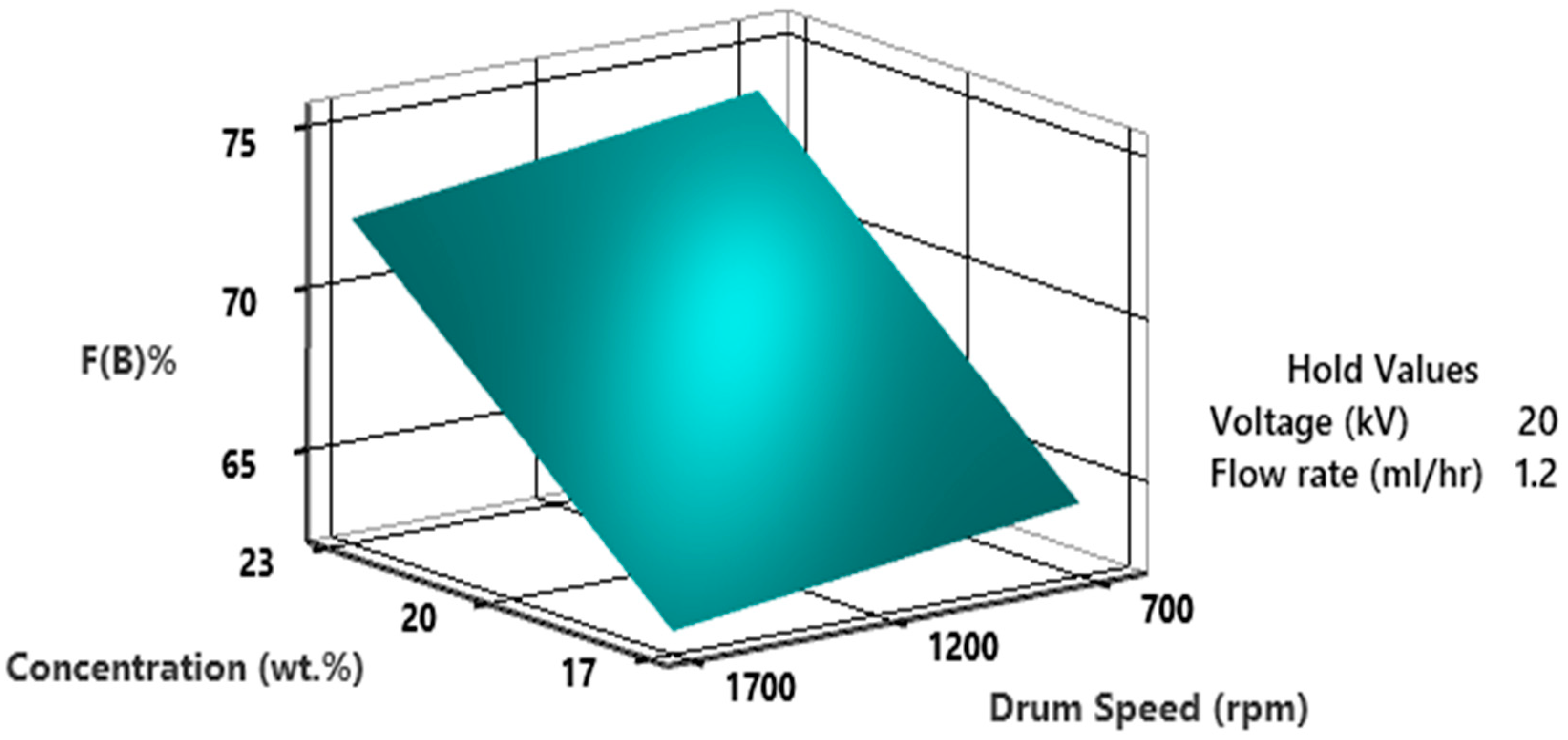
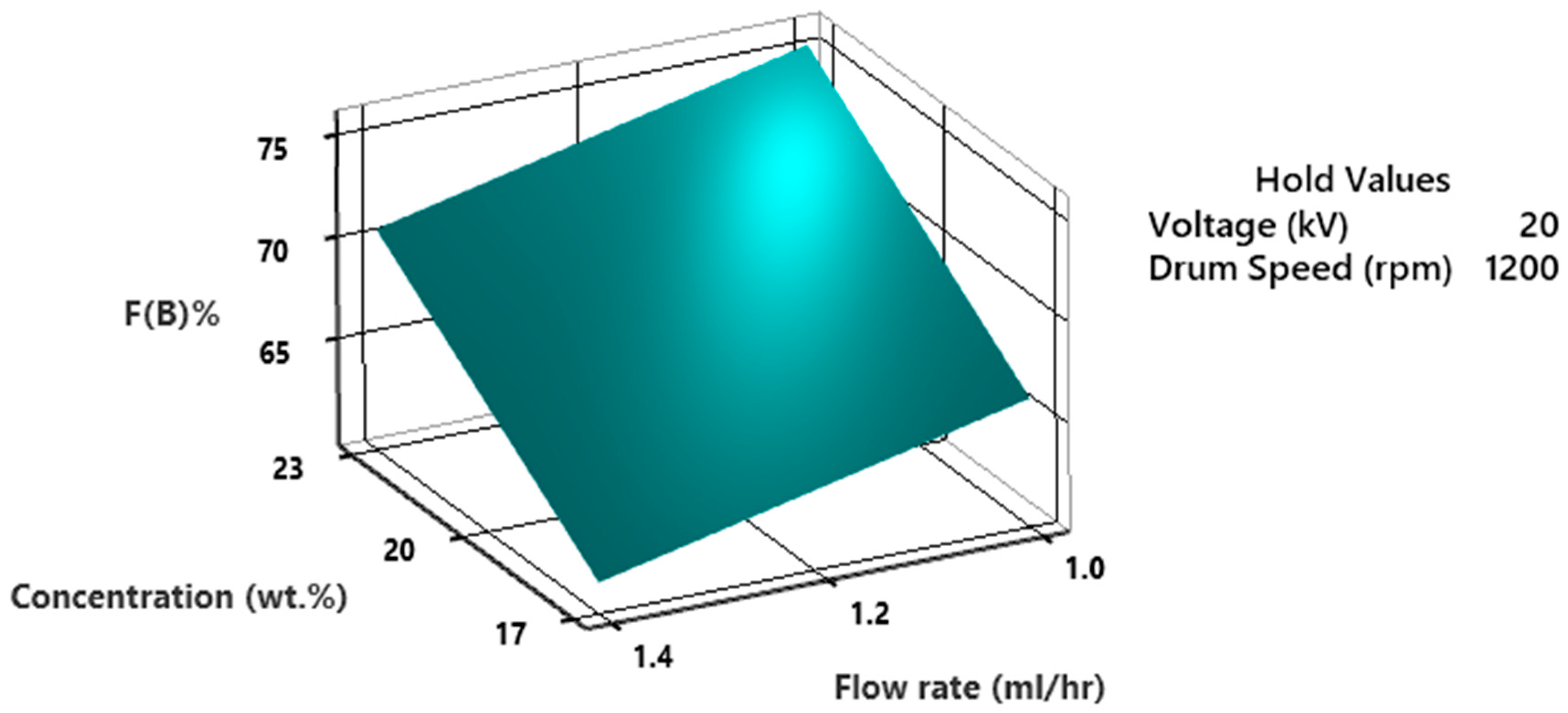
3.2. β-Phase Fraction
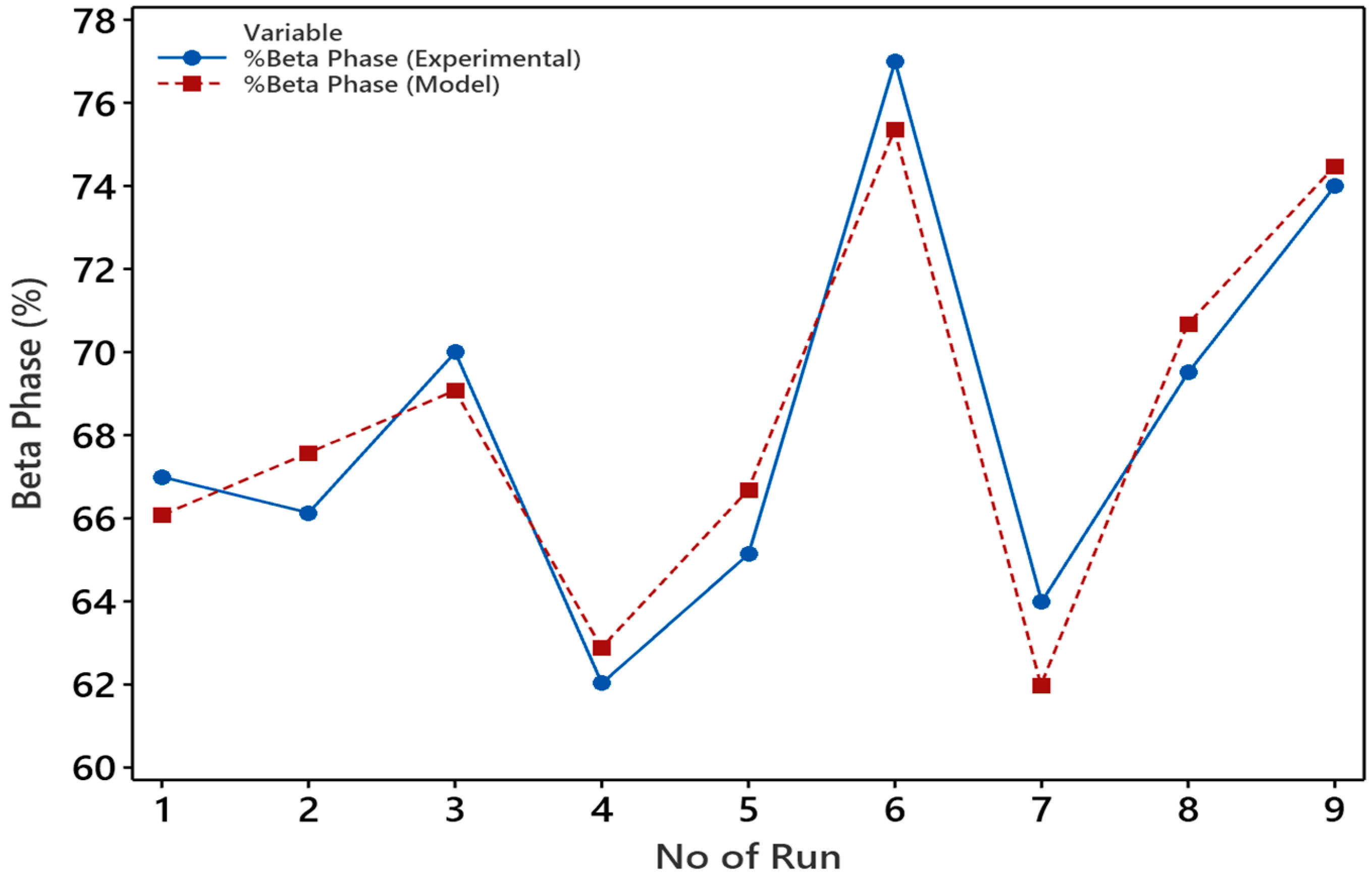
3.3. Model Fitting and Validation
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Schutte, J.; Yuan, X.; Dirven, S.; Potgieter, J. The opportunity of electrospinning as a form of additive manufacturing in biotechnology. In Proceedings of the 2017 24th International Conference on Mechatronics and Machine Vision in Practice (M2VIP), Auckland, New Zealand, 21–23 November 2017; pp. 1–6. [Google Scholar] [CrossRef]
- Ding, Y.; Onyilagha, O.; Zhu, Z. 9—Electrospun nanofibers for tactile sensors. In Functional Tactile Sensors; Zhou, Y., Chou, H.-H., Eds.; Woodhead Publishing: Sawston, CA, USA, 2021; pp. 159–196. [Google Scholar] [CrossRef]
- Wan, X.; Cong, H.; Jiang, G.; Liang, X.; Liu, L.; He, H. A review on PVDF nanofibers in textiles for flexible piezoelectric sensors. ACS Appl. Nano Mater. 2023, 6, 1522–1540. [Google Scholar] [CrossRef]
- Liu, F.; Hashim, N.A.; Liu, Y.; Abed, M.M.; Li, K. Progress in the production and modification of PVDF membranes. J. Membr. Sci. 2011, 375, 1–27. [Google Scholar] [CrossRef]
- Meng, N.; Mao, R.; Tu, W.; Zhu, X.; Wilson, R.M.; Bilotti, E.; Reece, M.J. Processing and characterization of free standing highly oriented ferroelectric polymer films with remarkably low coercive field and high remnant polarization. Polymer 2016, 100, 69–76. [Google Scholar] [CrossRef]
- Ribeiro, C.; Costa, C.M.; Correia, D.M.; Nunes-Pereira, J.; Oliveira, J.; Martins, P.; Gonçalves, R.; Cardoso, V.F.; Lanceros-Méndez, S. Electroactive poly (vinylidene fluoride)-based structures for advanced applications. Nat. Protoc. 2018, 13, 681–704. [Google Scholar] [CrossRef] [PubMed]
- Meng, N.; Ren, X.; Santagiuliana, G.; Ventura, L.; Zhang, H.; Wu, J.; Yan, H.; Reece, M.J.; Bilotti, E. Ultrahigh β-phase content poly(vinylidene fluoride) with relaxor-like ferroelectricity for high energy density capacitors. Nat. Commun. 2019, 10, 4535. [Google Scholar] [CrossRef]
- Mohammadpourfazeli, S.; Arash, S.; Ansari, A.; Yang, S.; Mallick, K.; Bagherzadeh, R. Future prospects and recent developments of polyvinylidene fluoride (PVDF) piezoelectric polymer; fabrication methods, structure, and electro-mechanical properties. RSC Adv. 2023, 13, 370–387. [Google Scholar] [CrossRef]
- Tanaka, Y.; Nguyen, D.P.; Fukuda, T.; Sano, A. Wearable skin vibration sensor using a PVDF film. In Proceedings of the 2015 IEEE World Haptics Conference (WHC), Evanston, IL, USA, 22–26 June 2015; pp. 146–151. [Google Scholar] [CrossRef]
- Seminara, L.; Pinna, L.; Valle, M.; Basiricò, L.; Loi, A.; Cosseddu, P.; Bonfiglio, A.; Ascia, A.; Biso, M.; Ansaldo, A.; et al. Piezoelectric Polymer Transducer Arrays for Flexible Tactile Sensors. IEEE Sens. J. 2013, 13, 4022–4029. [Google Scholar] [CrossRef]
- Lang, C.; Fang, J.; Shao, H.; Ding, X.; Lin, T. High-sensitivity acoustic sensors from nanofibre webs. Nat. Commun. 2016, 7, 11108. [Google Scholar] [CrossRef] [PubMed]
- Singh, R.K.; Lye, S.W.; Miao, J. PVDF Nanofiber Sensor for Vibration Measurement in a String. Sensors 2019, 19, 3739. [Google Scholar] [CrossRef] [PubMed]
- Zampetti, E.; Bearzotti, A.; Macagnano, A. Flexible Piezoelectric Transducer Based on Electrospun PVDF Nanofibers for Sensing Applications. Procedia Eng. 2014, 87, 1509–1512. [Google Scholar] [CrossRef]
- Parker, A.; Ueda, A.; Marvinney, C.; Hargrove, S.; Williams, F.; Mu, R. Structural and thermal treatment evaluation of electrospun PVDF nanofibers for sensors. J. Polym. Sci. Appl. 2018, 2, 1–4. [Google Scholar]
- Li, D.; Xia, Y. Electrospinning of nanofibers: Reinventing the wheel? Adv. Mater. 2004, 16, 1151–1170. [Google Scholar] [CrossRef]
- Agarwal, S.; Greiner, A.; Wendorff, J.H. Electrospinning of manmade and biopolymer nanofibers—Progress in techniques, materials, and applications. Adv. Funct. Mater. 2009, 19, 2863–2879. [Google Scholar] [CrossRef]
- He, Z.; Rault, F.; Lewandowski, M.; Mohsenzadeh, E.; Salaün, F. Electrospun PVDF Nanofibers for Piezoelectric Applications: A Review of the Influence of Electrospinning Parameters on the β Phase and Crystallinity Enhancement. Polymers 2021, 13, 174. [Google Scholar] [CrossRef]
- Gao, K.; Hu, X.; Dai, C.; Yi, T. Crystal structures of electrospun PVDF membranes and its separator application for rechargeable lithium metal cells. Mater. Sci. Eng. B 2006, 131, 100–105. [Google Scholar] [CrossRef]
- Damaraju, S.M.; Wu, S.; Jaffe, M.; Arinzeh, T.L. Structural changes in PVDF fibers due to electrospinning and its effect on biological function. Biomed. Mater. 2013, 8, 045007. [Google Scholar] [CrossRef]
- Jiyong, H.; Yinda, Z.; Hele, Z.; Yuanyuan, G.; Xudong, Y. Mixed effect of main electrospinning parameters on the β-phase crystallinity of electrospun PVDF nanofibers. Smart Mater. Struct. 2017, 26, 085019. [Google Scholar] [CrossRef]
- Zhu, G.; Zhao, L.; Zhu, L.; Deng, X.; Chen, W. Effect of experimental parameters on nanofiber diameter from electrospinning with wire electrodes. IOP Conf. Ser. Mater. Sci. Eng. 2017, 230, 012043. [Google Scholar] [CrossRef]
- Zhao, Z.; Li, J.; Yuan, X.; Li, X.; Zhang, Y.; Sheng, J. Preparation and properties of electrospun poly (vinylidene fluoride) membranes. J. Appl. Polym. Sci. 2005, 97, 466–474. [Google Scholar] [CrossRef]
- Shao, H.; Fang, J.; Wang, H.; Lin, T. Effect of electrospinning parameters and polymer concentrations on mechanical-to-electrical energy conversion of randomly-oriented electrospun poly(vinylidene fluoride) nanofiber mats. RSC Adv. 2015, 5, 14345–14350. [Google Scholar] [CrossRef]
- Gee, S.; Johnson, B.; Smith, A.L. Optimizing electrospinning parameters for piezoelectric PVDF nanofiber membranes. J. Membr. Sci. 2018, 563, 804–812. [Google Scholar] [CrossRef]
- Zaarour, B.; Liu, W. Enhanced piezoelectric performance of electrospun PVDF nanofibers by regulating the solvent systems. J. Eng. Fibers Fabr. 2022, 17, 15589250221125437. [Google Scholar] [CrossRef]
- Mokhtari, F.; Shamshirsaz, M.; Latifi, M. Investigation of Β phase formation in piezoelectric response of electrospun polyvinylidene fluoride nanofibers: LiCl additive and increasing fibers tension. Polym. Eng. Sci. 2016, 56, 61–70. [Google Scholar] [CrossRef]
- Utkarsh; Hegab, H.; Tariq, M.; Syed, N.A.; Rizvi, G.; Pop-Iliev, R. Towards Analysis and Optimization of Electrospun PVP (Polyvinylpyrrolidone) Nanofibers. Adv. Polym. Technol. 2020, 2020, 4090747. [Google Scholar] [CrossRef]
- Zhu, R.; Wang, Z. 9—Piezoelectric one- to two-dimensional nanomaterials for vibration energy harvesting devices. In Emerging 2D Materials and Devices for the Internet of Things; Tao, L., Akinwande, D., Eds.; Elsevier: Amsterdam, The Netherlands, 2020; pp. 221–241. [Google Scholar] [CrossRef]
- Jian, H.Y.; Fridrikh, S.V.; Rutledge, G.C. The role of elasticity in the formation of electrospun fibers. Polymer 2006, 47, 4789–4797. [Google Scholar]
- Zong, X.; Kim, K.; Fang, D.; Ran, S.; Hsiao, B.S.; Chu, B. Structure and process relationship of electrospun bioabsorbable nanofiber membranes. Polymer 2002, 43, 4403–4412. [Google Scholar] [CrossRef]
- Chen, X.; Zhang, Y.; He, X.; Li, H.; Wei, B.; Yang, W. Electrospinning on a plucked string. J. Mater. Sci. 2019, 54, 901–910. [Google Scholar] [CrossRef]
- Gonzalez, E.; Barquero, A.; Muñoz-Sanchez, B.; Paulis, M.; Leiza, J.R. Green Electrospinning of Polymer Latexes: A Systematic Study of the Effect of Latex Properties on Fiber Morphology. Nanomaterials 2021, 11, 706. [Google Scholar] [CrossRef]
- Greiner, A.; Wendorff, J.H. Electrospinning: A fascinating method for the preparation of ultrathin fibers. Angew. Chem. Int. Ed. 2007, 46, 5670–5703. [Google Scholar] [CrossRef]
- Navaie, F.; Esmaeilnezhad, E.; Choi, H.-J. Effect of Rheological Properties of Polymer Solution on Polymer Flooding Characteristics. Polymers 2022, 14, 5555. [Google Scholar] [CrossRef]
- Nezarati, R.M.; Eifert, M.B.; Cosgriff-Hernandez, E. Effects of humidity and solution viscosity on electrospun fiber morphology. Tissue Eng. Part C Methods 2013, 19, 810–819. [Google Scholar] [CrossRef] [PubMed]
- Demir, M.M.; Yilgor, I.; Yilgor, E.; Erman, B. Electrospinning of polyurethane fibers. Polymer 2002, 43, 3303–3309. [Google Scholar] [CrossRef]
- Deitzel, J.M.; Kleinmeyer, J.; Harris, D.; Tan, N.B. The effect of processing variables on the morphology of electrospun nanofibers and textiles. Polymer 2001, 42, 261–272. [Google Scholar] [CrossRef]
- Teo, W.-E. Electrospinning Parameters. Electrospintech. Available online: http://electrospintech.com/voltage.html (accessed on 7 October 2023).
- Chowdhury, M.; Stylios, G. Effect of experimental parameters on the morphology of electrospun Nylon 6 fibres. Int. J. Basic Appl. Sci. 2010, 10, 70–78. [Google Scholar]
- Wang, C.; Chien, H.-S.; Yan, K.-W.; Hung, C.-L.; Hung, K.-L.; Tsai, S.-J.; Jhang, H.-J. Correlation between processing parameters and microstructure of electrospun poly (D, L-lactic acid) nanofibers. Polymer 2009, 50, 6100–6110. [Google Scholar] [CrossRef]
- Zargham, S.; Bazgir, S.; Tavakoli, A.; Rashidi, A.S.; Damerchely, R. The effect of flow rate on morphology and deposition area of electrospun nylon 6 nanofiber. J. Eng. Fibers Fabr. 2012, 7, 155892501200700414. [Google Scholar] [CrossRef]
- Becker, A.; Zernetsch, H.; Mueller, M.; Glasmacher, B. A novel coaxial nozzle for in-process adjustment of electrospun scaffolds’ fiber diameter: Electrospun mats and their dependence on process parameters. Curr. Dir. Biomed. Eng. 2015, 1, 104–107. [Google Scholar] [CrossRef]
- Li, Y.; Liao, C.; Tjong, S.C. Electrospun Polyvinylidene Fluoride-Based Fibrous Scaffolds with Piezoelectric Characteristics for Bone and Neural Tissue Engineering. Nanomaterials 2019, 9, 952. [Google Scholar] [CrossRef]
- Kalimuldina, G.; Turdakyn, N.; Abay, I.; Medeubayev, A.; Nurpeissova, A.; Adair, D.; Bakenov, Z. A review of piezoelectric PVDF film by electrospinning and its applications. Sensors 2020, 20, 5214. [Google Scholar] [CrossRef]
- Singh, R.K.; Lye, S.W.; Miao, J. Holistic investigation of the electrospinning parameters for high percentage of β-phase in PVDF nanofibers. Polymer 2021, 214, 123366. [Google Scholar] [CrossRef]
- Kim, K.W.; Lee, K.H.; Khil, M.S.; Ho, Y.S.; Kim, H.Y. The effect of molecular weight and the linear velocity of drum surface on the properties of electrospun poly (ethylene terephthalate) nonwovens. Fibers Polym. 2004, 5, 122–127. [Google Scholar] [CrossRef]
- Oflaz, K.; Özaytekin, İ. Analysis of electrospinning and additive effect on β phase content of electrospun PVDF nanofiber mats for piezoelectric energy harvester nanogenerators. Smart Mater. Struct. 2022, 31, 105022. [Google Scholar] [CrossRef]
- Abolhasani, M.M.; Azimi, S.; Fashandi, H. Enhanced ferroelectric properties of electrospun poly (vinylidene fluoride) nanofibers by adjusting processing parameters. RSC Adv. 2015, 5, 61277–61283. [Google Scholar] [CrossRef]
- Ribeiro, C.; Sencadas, V.; Ribelles, J.L.G.; Lanceros-Méndez, S. Influence of processing conditions on polymorphism and nanofiber morphology of electroactive poly (vinylidene fluoride) electrospun membranes. Soft Mater. 2010, 8, 274–287. [Google Scholar] [CrossRef]
- Ghafari, E.; Jiang, X.; Lu, N. Surface morphology and beta-phase formation of single polyvinylidene fluoride (PVDF) composite nanofibers. Adv. Compos. Hybrid Mater. 2018, 1, 332–340. [Google Scholar] [CrossRef]





| Unit | Level 1 | Level 2 | Level 3 | ||
|---|---|---|---|---|---|
| Control Factors | Voltage | kV | 17 | 20 | 23 |
| Polymer Concentration | wt.% | 17 | 20 | 23 | |
| Rotational Speed of Drum | RPM | 700 | 1200 | 1700 | |
| Solution Flow Rate | mL/h | 1 | 1.2 | 1.4 | |
| Sample Name | Voltage (kV) | Concentration (wt.%) | Speed of the Collector Drum (rpm) | Flow Rate (mL/h) |
|---|---|---|---|---|
| P-1 | 17 | 17 | 700 | 1.0 |
| P-2 | 17 | 20 | 1200 | 1.2 |
| P-3 | 17 | 23 | 1700 | 1.4 |
| P-4 | 20 | 17 | 1700 | 1.2 |
| P-5 | 20 | 20 | 700 | 1.4 |
| P-6 | 20 | 23 | 1200 | 1.0 |
| P-7 | 23 | 17 | 1200 | 1.4 |
| P-8 | 23 | 20 | 1700 | 1.0 |
| P-9 | 23 | 23 | 700 | 1.2 |
| Sample | Solution Concentration | Electrical Conductivity mS/cm |
|---|---|---|
| 1 | 17 wt.% PVDF | 1.723 |
| 2 | 20 wt.% PVDF | 1.531 |
| 3 | 23 wt.% PVDF | 1.165 |
| Frequency | Assignment |
|---|---|
| 1400 cm−1 | C-H bending due to methylene in aliphatic |
| 1390 cm−1 | C-CH2 rocking |
| 1280 cm−1 | In the β-phase, C-F2 out-of-plane deformation |
| 1230 cm−1 | In the γ-phase, C-F2 stretching |
| 1182, 1172 cm−1 | C-H rocking |
| 1090, 1070 cm−1 | C-H in the plane and out of plane deformation |
| 875 cm−1 | Amorphous phase in PVDF (C-H) |
| 840 cm−1 | In the β-phase, C-H2 rocking |
| 833 cm−1 | Shoulder, due to γ-phase |
| 760 and/or 660 cm−1 | In the α-phase, due to C-F2 |
| Electrospinning Parameters | Fiber Diameter | β-Phase Fraction | Ref. |
|---|---|---|---|
| 17–23 kV voltage, 1–1.4 mL/h flowrate, 17–23 wt.% of PVDF in DMF solvent, 700 rpm to 1700 rpm drum speed, 13 cm Needle tip to collector drum distance, 0.26 mmOD needle size. | 120–150 nm | 65–75% | This work |
| 12–30 kV, 20 wt% PVDF in 1:1 ratio DMAC:Acetone solvent mixture, 0.5 mL/h, flat plate collector at a distance of 20 cm. | 151–295 nm Increasing the voltage causes a reduction in fiber diameter. | 65–72% Increasing the voltage causes an increase in β-phase. | [19] |
| 14–18 kV voltage, flow rate in a range of 0.5–3 mL/h, 10 wt.% PVDF in 4/6 DMF:Acetone solvent mixture. 0.3–1 needle OD, Collector drum at a distance of 15 cm and 300 rpm. | Not studied | 70–78% | [20] |
| 16–26 wt.% PVDF in DMF/acetone (v/v 4/6), 1 mL/h flow rate, 9–21 kV voltage, Drum speed = 100 rpm, 9–21 cm needle to collector distance. | 0.3–0.8 μm | 75–85% | [23] |
| 20 wt.% PVDF in DMF/Acetone (1:1, 1:3), 13 and 21 kV voltage, 0.5 and 1 mL/h flow rate, needle to collector distance = 17 cm, 0.75 mm ID of the needle. | 300–400 nm | 0.6–0.8 fraction | [48] |
| 20 wt.% PVDF in DMF, Varying voltage from 15–30 kV, needle Id 250 μm at 4 mL/h, collector drum at 15 cm distance from needle. | 403–495 nm High fiber diameter at low voltage and high flow rate. | Increasing the voltage causes the reduction in β-phase from 85–80%. | [49] |
| 12 wt.% PVDF in DMF:Acetone (100:0, 80:20, 60:40), 0.43 ID of the needle, 250 rpm collector drum placed at a distance of 10 and 16 cm from the needle tip, voltage varied in a range of 9–14 kV, flow rate varied between 0.7 to 0.5 mL/h | 495 nm | 80% β-phase when electrospun with pure DMF, an increase in the voltage results in an increase in β-phase from 67–72% and increasing the TCD results in higher β-phase. The trend in flow rate was not readily evident. | [24] |
| Concetration varied from 16–26 wt.% in DMF:Acetone mixture at 4/6 v/v, 1 mL/h, drum speed of 100 rpm, applied voltage 15 kV; spinning distance 15 cm; nanofiber mat thickness 70 mm | 0.2–0.8 μm Increasing the concentration increased fiber diameter. | Increasing the concentration to 20 wt.% gives a higher β-phase (85%) and further increases concentration to 26 wt.% resulted in a lower β-phase fraction (80%). | [23] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tariq, A.; Behravesh, A.H.; Utkarsh; Rizvi, G. Statistical Modeling and Optimization of Electrospinning for Improved Morphology and Enhanced β-Phase in Polyvinylidene Fluoride Nanofibers. Polymers 2023, 15, 4344. https://doi.org/10.3390/polym15224344
Tariq A, Behravesh AH, Utkarsh, Rizvi G. Statistical Modeling and Optimization of Electrospinning for Improved Morphology and Enhanced β-Phase in Polyvinylidene Fluoride Nanofibers. Polymers. 2023; 15(22):4344. https://doi.org/10.3390/polym15224344
Chicago/Turabian StyleTariq, Asra, Amir H. Behravesh, Utkarsh, and Ghaus Rizvi. 2023. "Statistical Modeling and Optimization of Electrospinning for Improved Morphology and Enhanced β-Phase in Polyvinylidene Fluoride Nanofibers" Polymers 15, no. 22: 4344. https://doi.org/10.3390/polym15224344
APA StyleTariq, A., Behravesh, A. H., Utkarsh, & Rizvi, G. (2023). Statistical Modeling and Optimization of Electrospinning for Improved Morphology and Enhanced β-Phase in Polyvinylidene Fluoride Nanofibers. Polymers, 15(22), 4344. https://doi.org/10.3390/polym15224344






