Effect of Process Parameters on the Crystallinity and Geometric Quality of Injection Molded Polymer Gears and the Resulting Stress State during Gear Operation
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
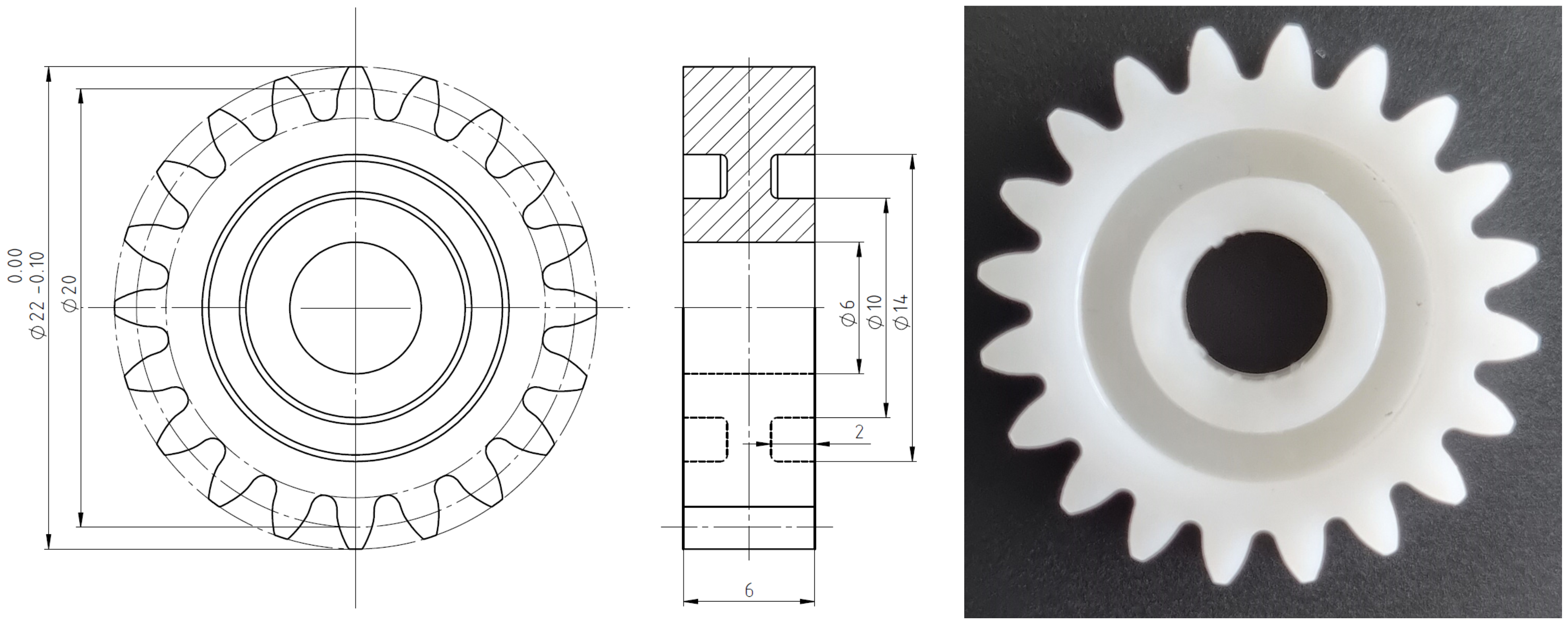
2.2. Sample Preparation
2.3. Crystallinity Measurement
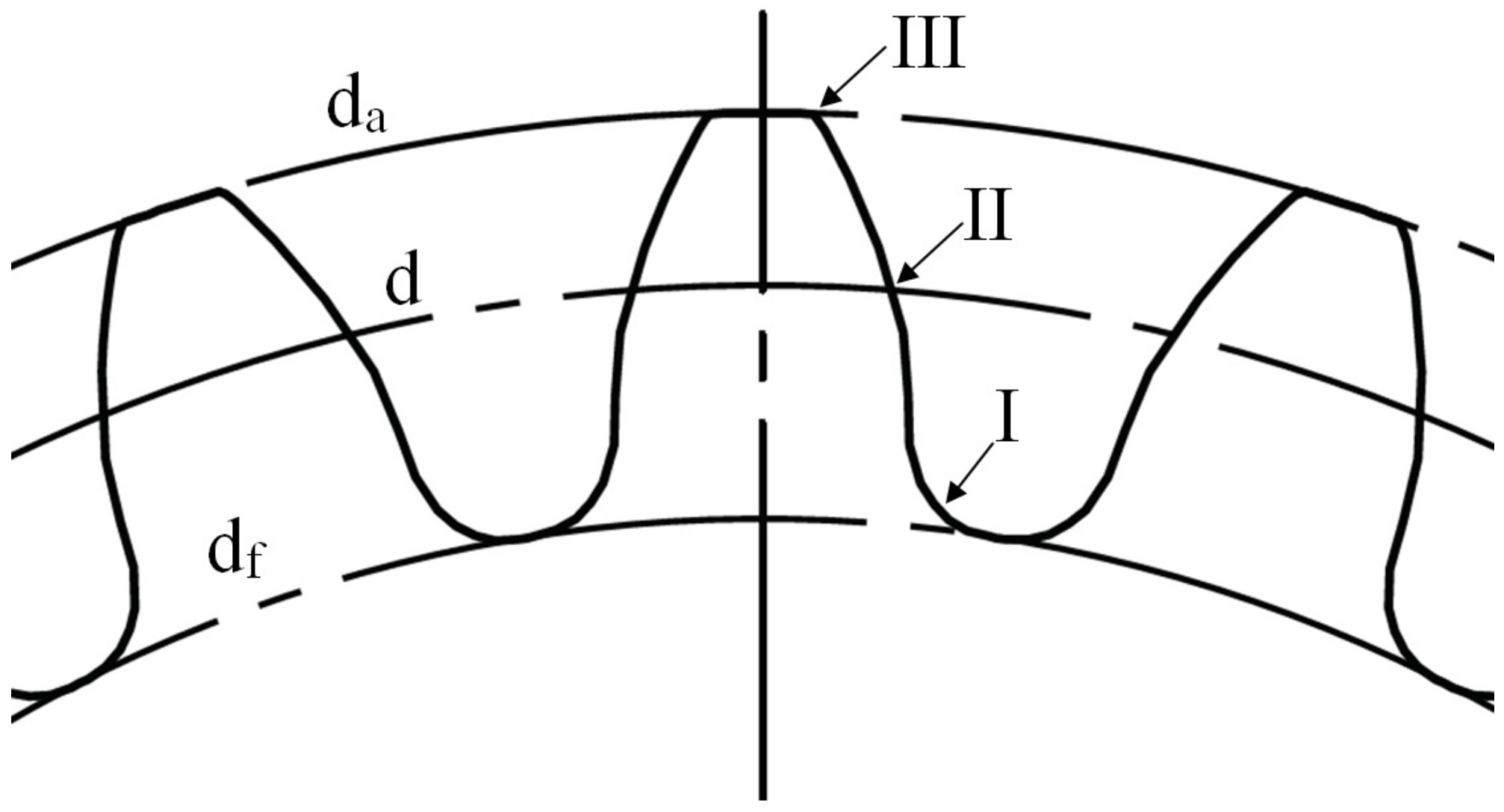
2.4. Gear Quality Measurements
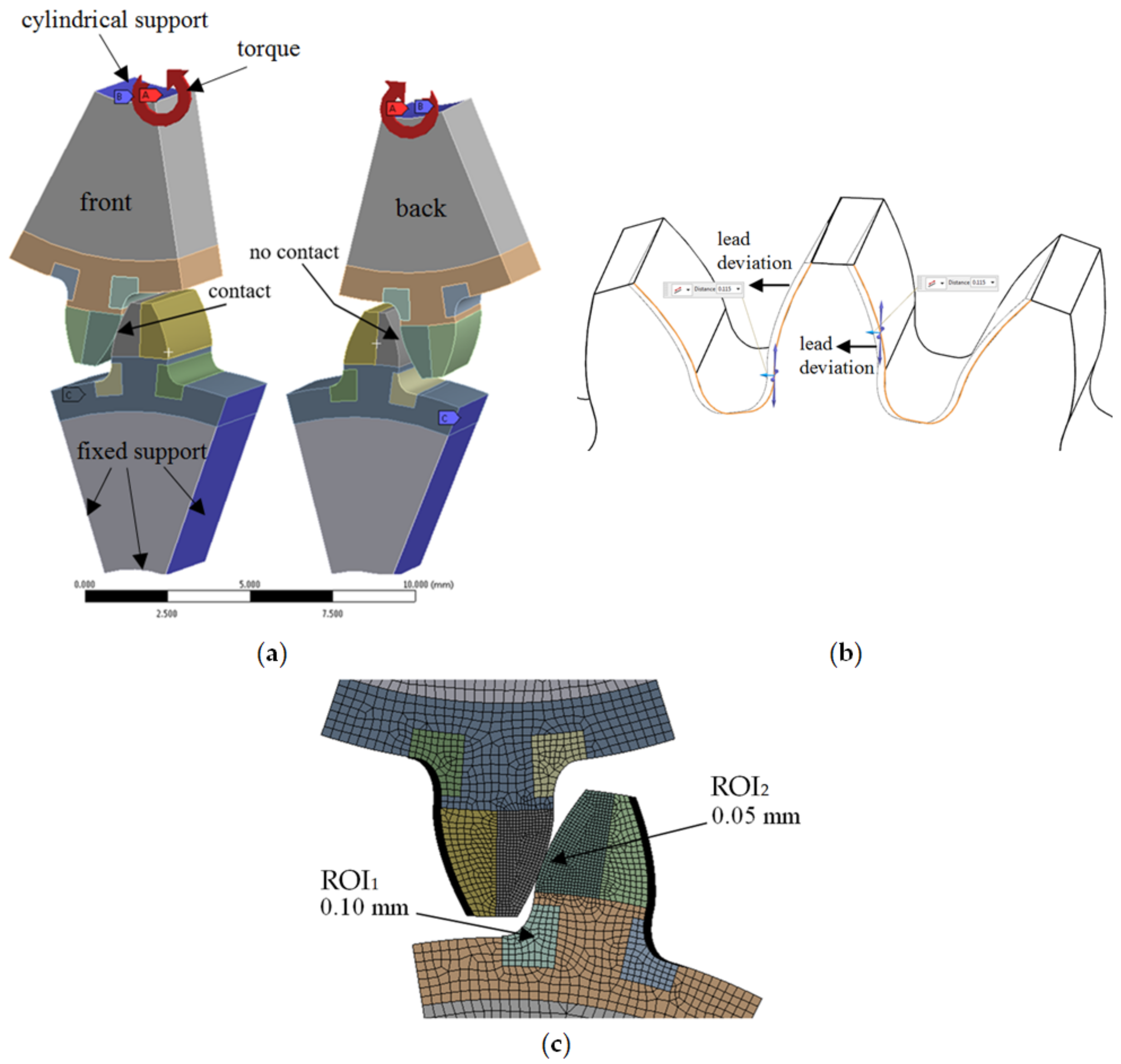
2.5. Stress Evaluation
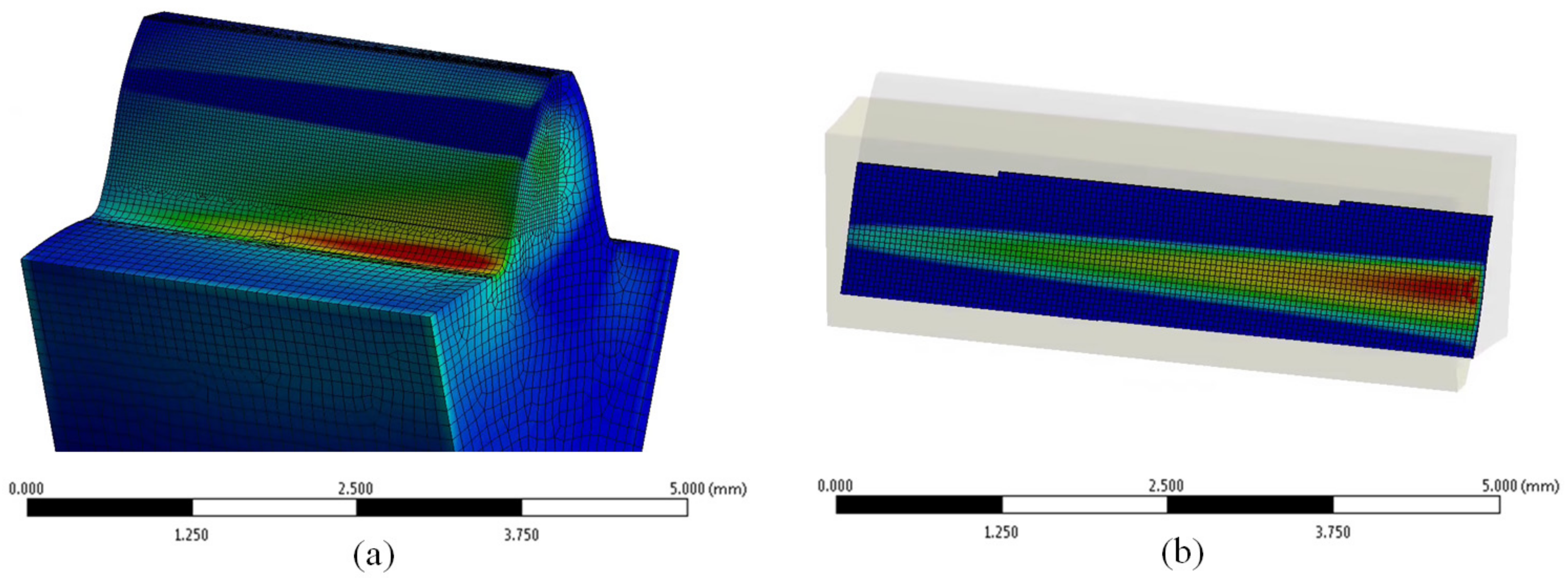
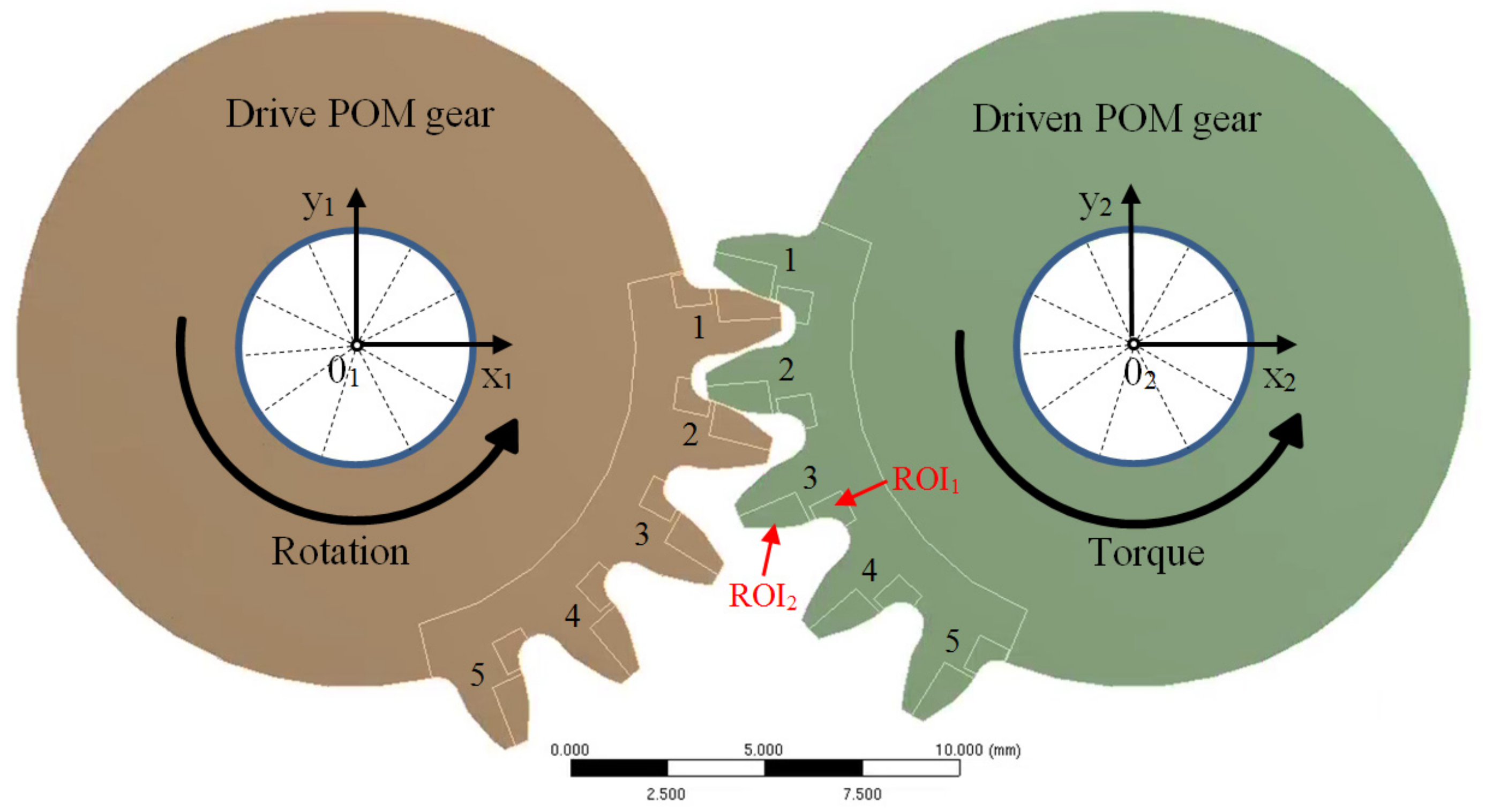
2.5.1. The Effect of Lead Quality (Distribution of Load across the Tooth’s Width)
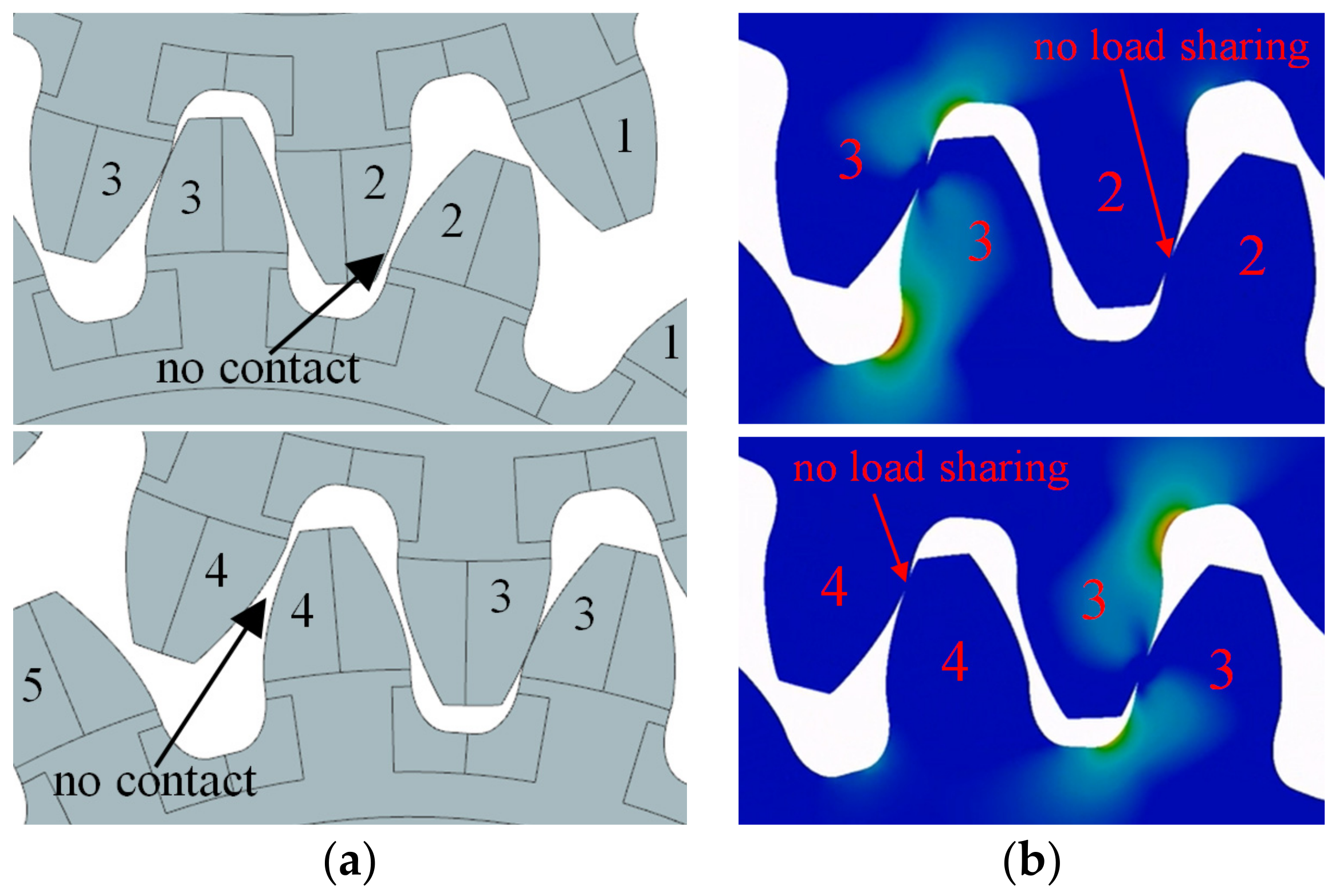
2.5.2. The Effect of Pitch Quality (LOAD Sharing among the Teeth)
3. Results
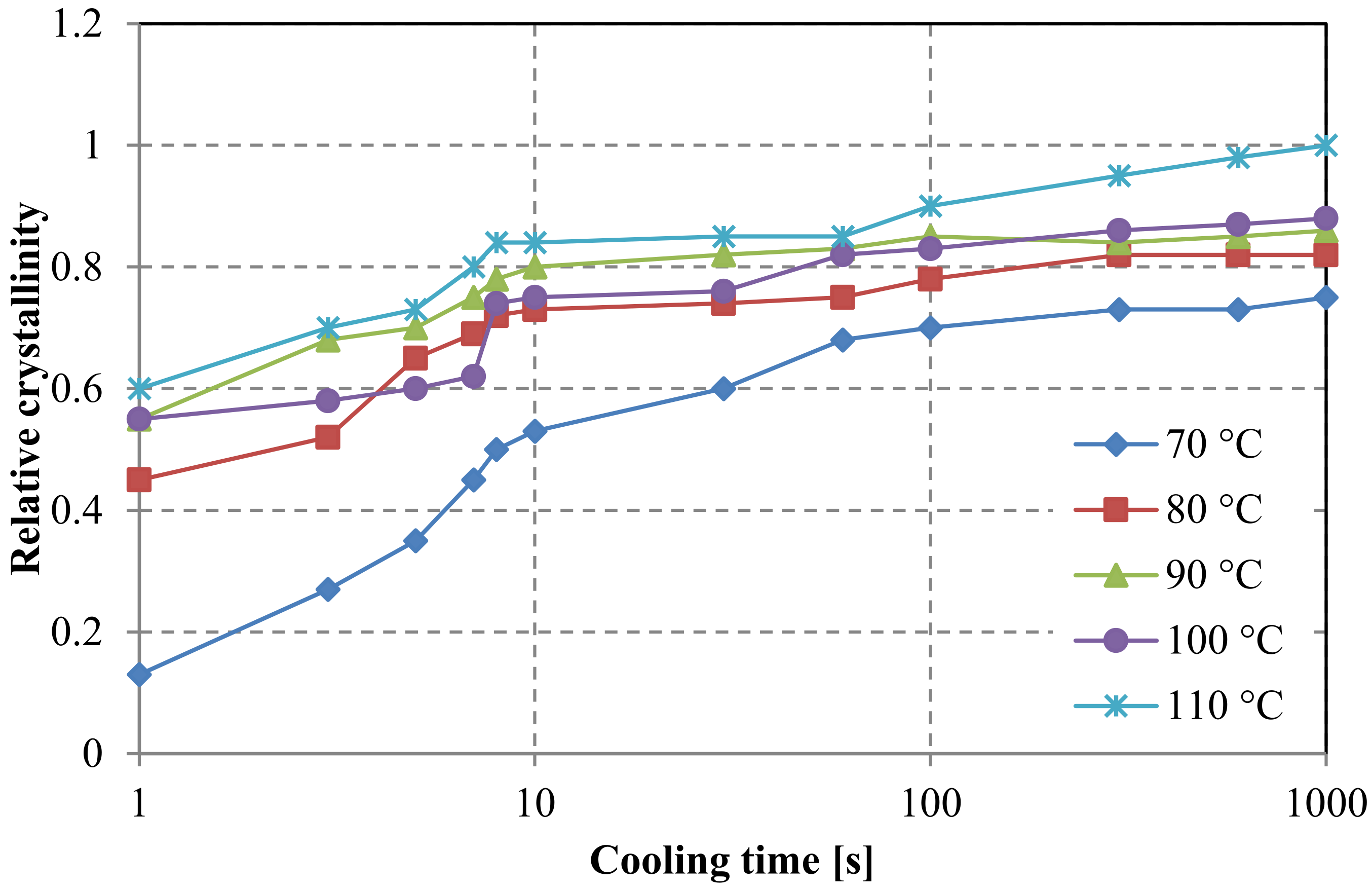
3.1. Crystallinity Measurements
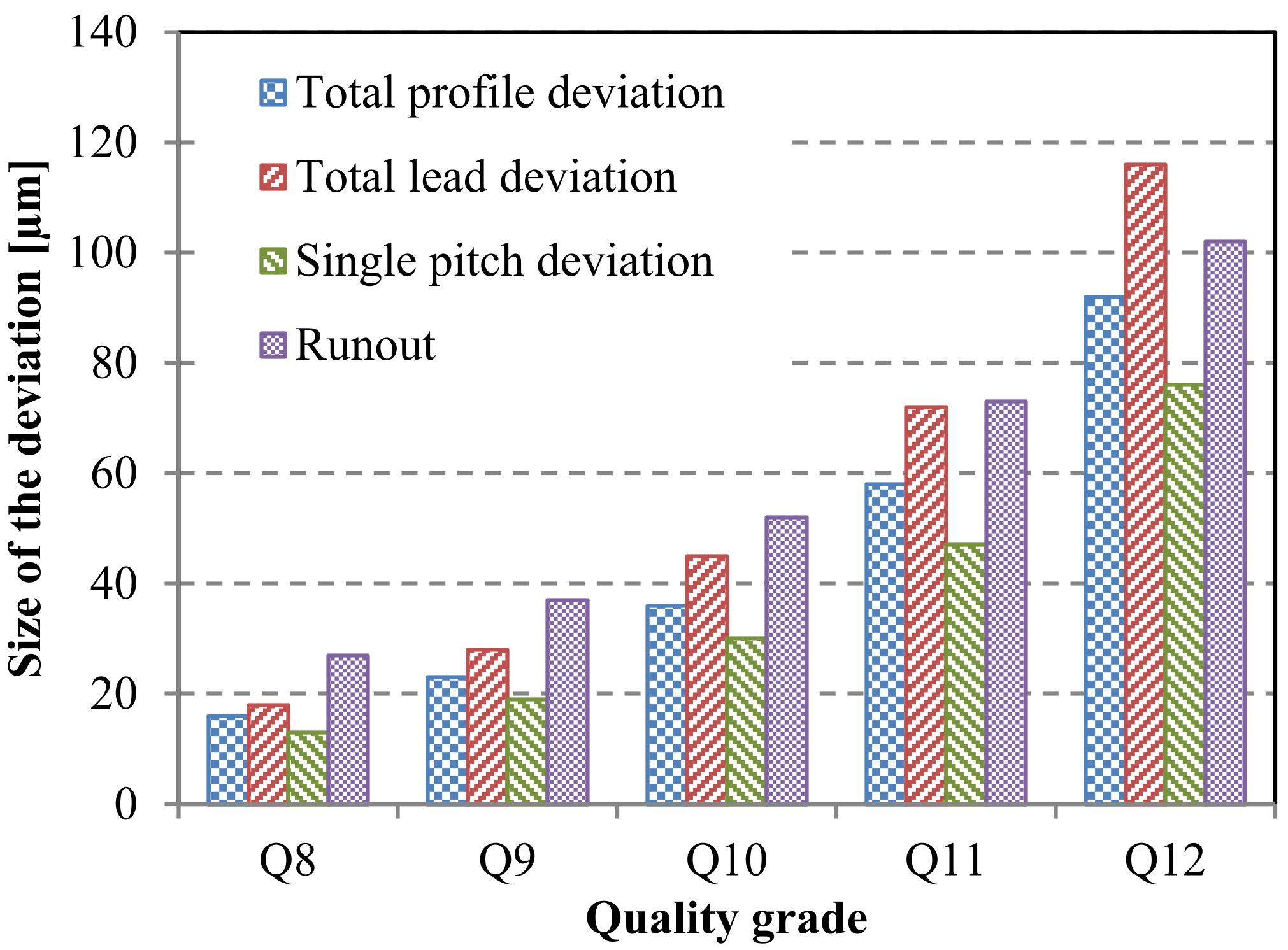
3.2. Gear Quality Measurements
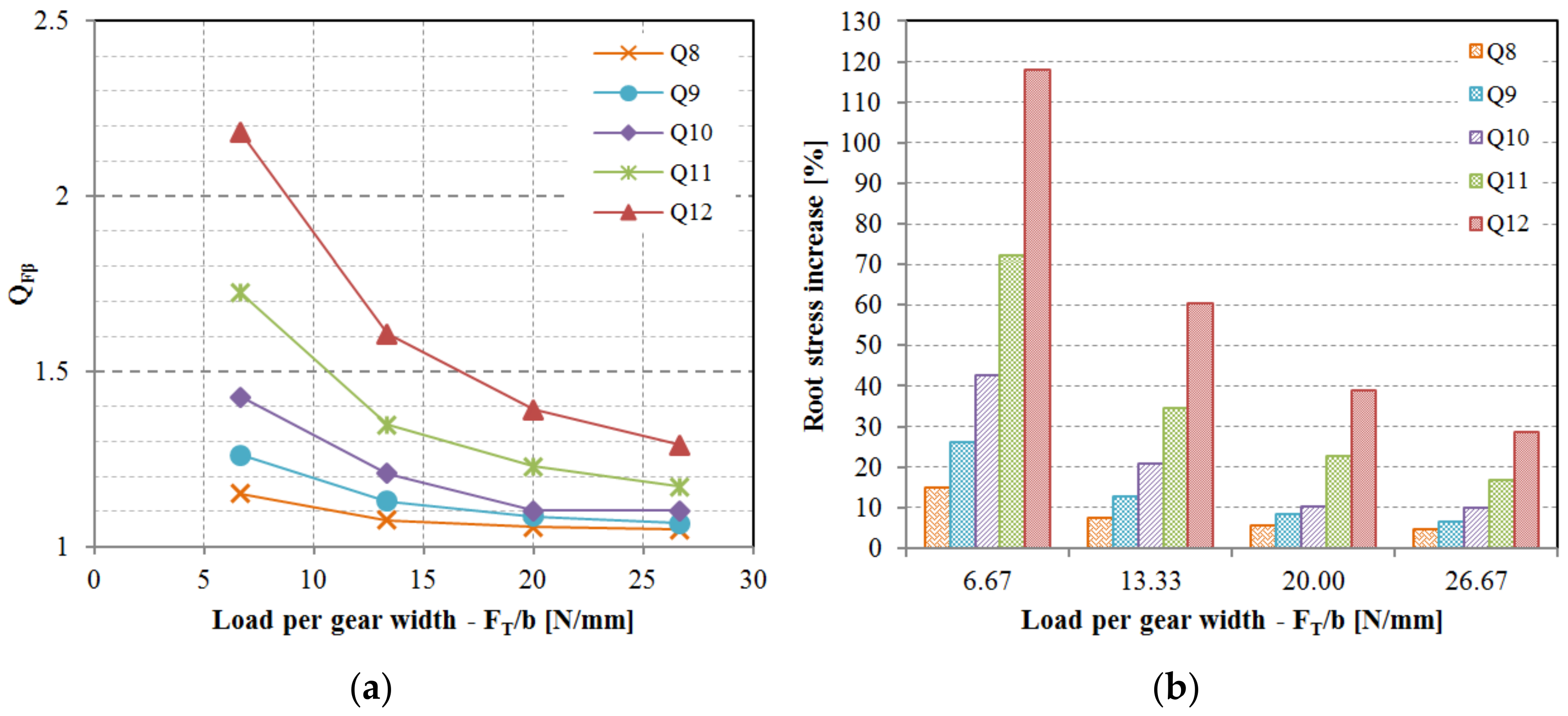
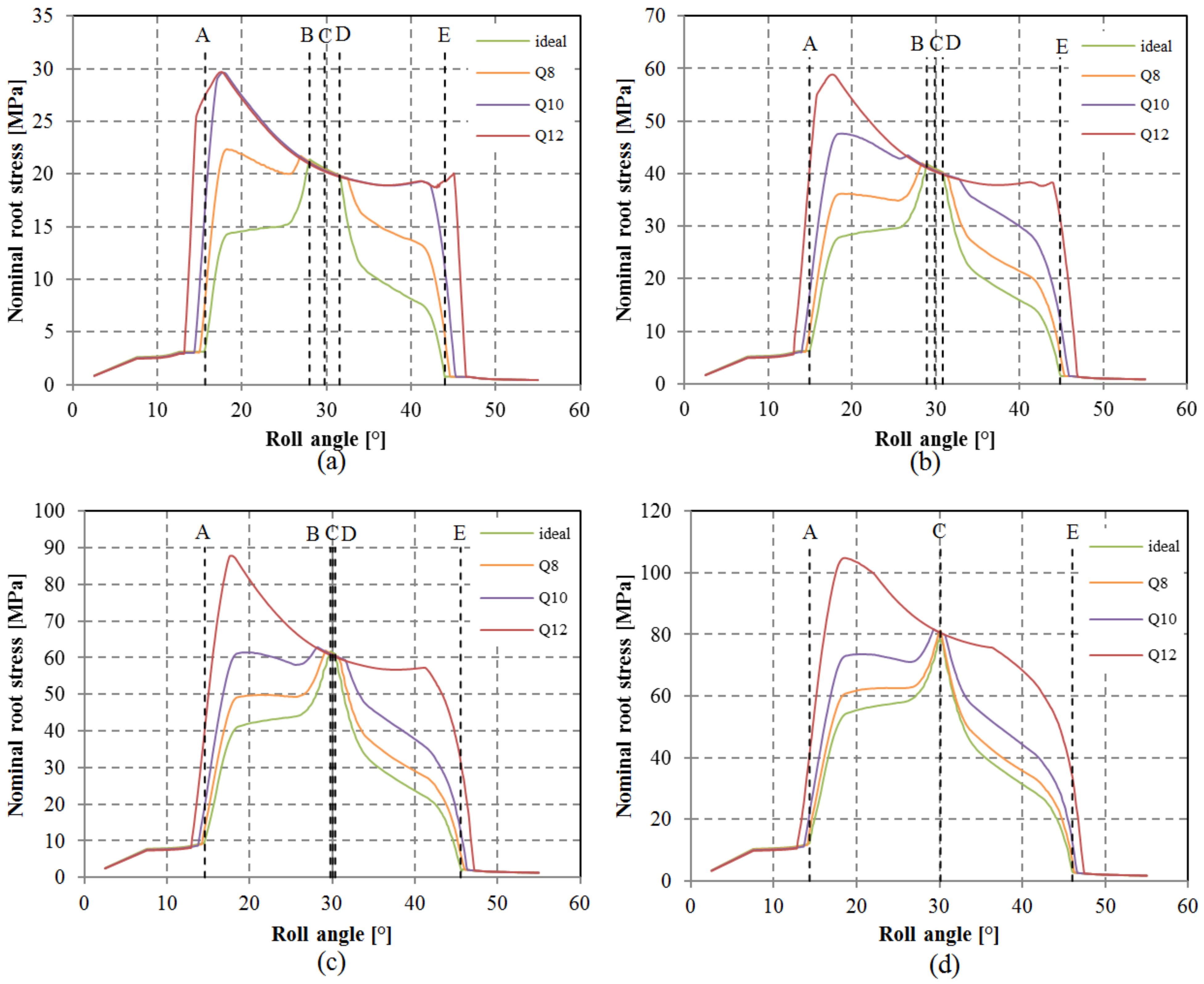
3.3. The Influence of Lead Deviation on the Stress Condition in the Gear
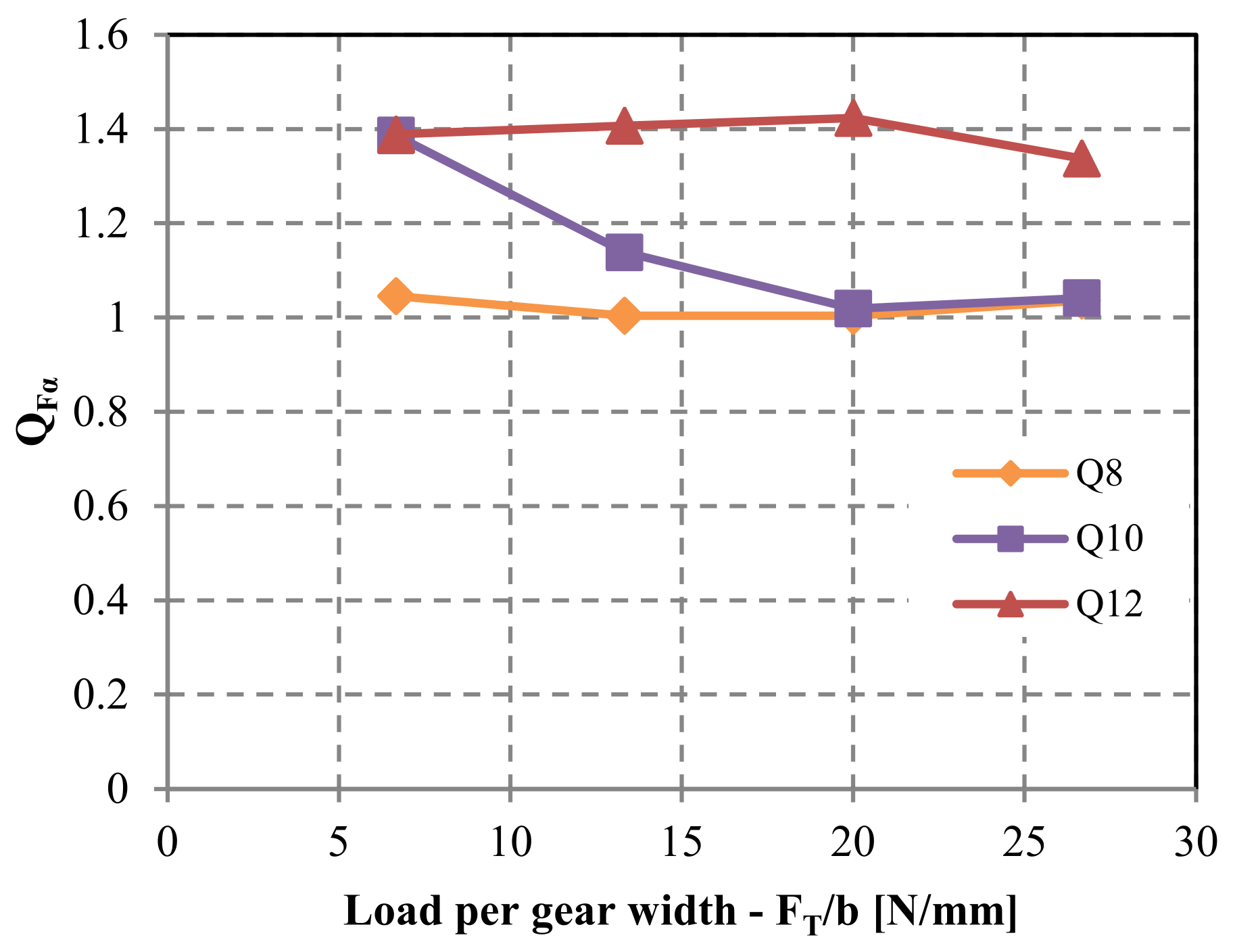
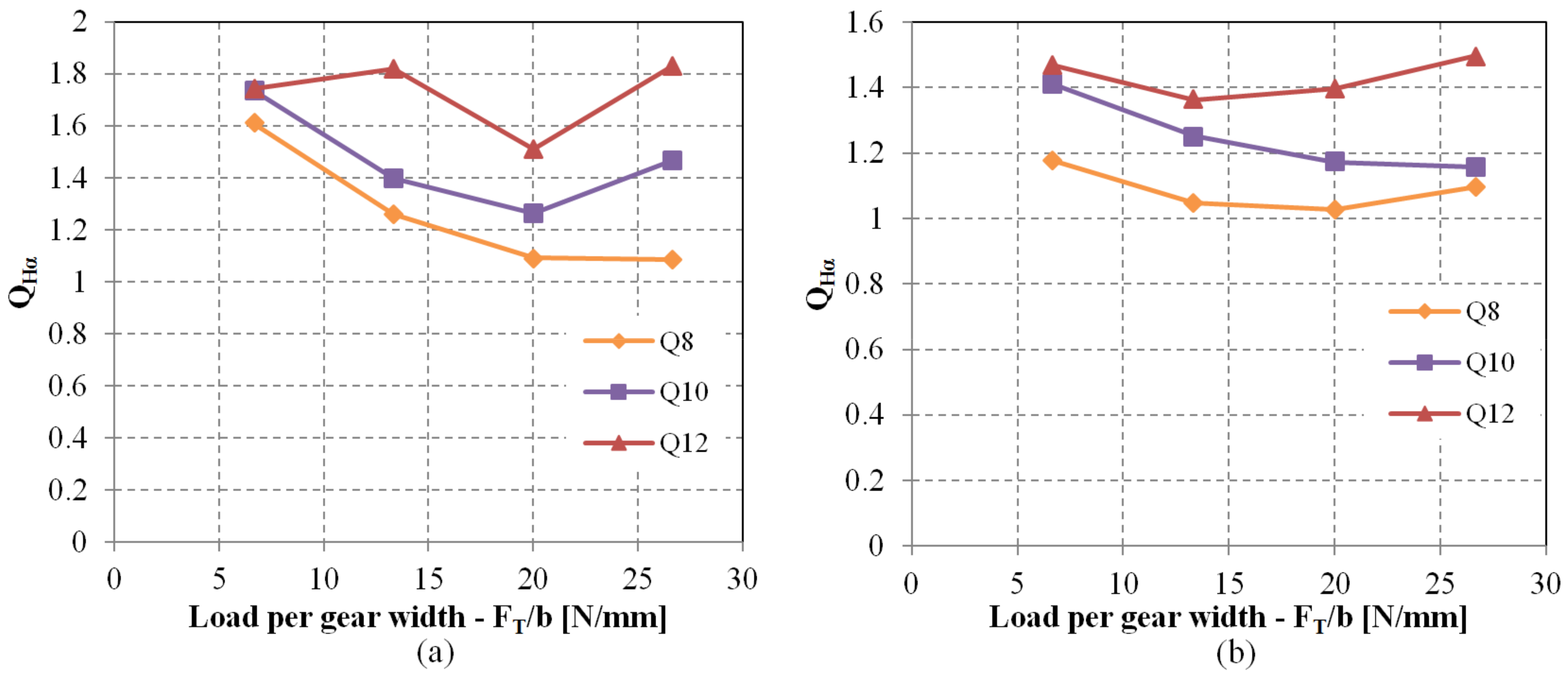
3.4. The Influence of Pitch Deviation on the Stress Condition in the Gear
4. Limits of the Study
5. Conclusions
6. Future Research
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| mm | face width | |
| mm | reference diameter | |
| mm | tip diameter | |
| mm | root diameter | |
| N | tangential load | |
| mm | normal module | |
| root stress | ||
| - | application factor | |
| - | dynamic factor | |
| - | face load factor for tooth-root stress | |
| - | transverse load factor for tooth-root stress | |
| - | ctor for tooth-root load | |
| - | form factor | |
| - | stress correction factor (notch effect) | |
| - | contact-ratio factor for root stress | |
| - | helix-angle factor for root stress | |
| flank pressure | ||
| - | face load factor for flank pressure | |
| - | transverse load factor for flank pressure | |
| - | elasticity factor | |
| - | zone factor | |
| - | contact-ratio factor for flank pressure | |
| - | helix-angle factor for flank pressure | |
| Q | - | quality grade |
| - | effect of lead deviation on the contact pressure | |
| - | contact pressure calculated for the analyzed gear-quality grade | |
| - | contact pressure calculated for the theoretical gear geometry | |
| - | effect of lead deviation on the root stress | |
| - | root stress calculated for the analyzed gear-quality grade | |
| - | root stress calculated for the theoretical gear geometry | |
| - | effect of pitch deviation on the root stress | |
| - | effect of pitch deviation on the contact pressure | |
| Ai | - | initial point of tooth contact |
| B | - | lowest point of single-tooth contact (LPSTC) for the drive gear and the highest point of single tooth contact (HPSTC) for the driven gear |
| C | - | pitch point (kinematic point) |
| D | - | highest point of single-tooth contact (HPSTC) for the drive gear and the lowest point of single tooth contact (LPSTC) for the driven gear |
| Ei | - | end point of tooth contact |
| µm | total profile deviation | |
| µm | profile form deviation | |
| µm | profile slope deviation | |
| µm | total helix deviation | |
| µm | helix form deviation | |
| µm | helix slope deviation | |
| µm | single pitch deviation | |
| µm | total cumulative pitch deviation | |
| µm | runout | |
| - | overall quality grade of the measured gear | |
| POM | - | poly-oxy-methylene |
| PA | - | polyamide |
| PEEK | - | poly-ether-ether-ketone |
| DSC | - | differential scanning calorimetry |
| PC | - | polycarbonate |
References
- Hoskins, T.J.; Dearn, K.D.; Kukureka, S.N.; Walton, D. Acoustic noise from polymer gears—A tribological investigation. Mater. Des. 2011, 32, 3509–3515. [Google Scholar] [CrossRef]
- Lu, Z.; Liu, H.; Wei, P.; Zhu, C.; Xin, D.; Shen, Y. The effect of injection molding lunker defect on the durability performance of polymer gears. Int. J. Mech. Sci. 2020, 180, 105665. [Google Scholar] [CrossRef]
- Mao, K.; Chetwynd, D.G.; Millson, M. A new method for testing polymer gear wear rate and performance. Polym. Test. 2020, 82, 106323. [Google Scholar] [CrossRef]
- Mao, K.; Greenwood, D.; Ramakrishnan, R.; Goodship, V.; Shrouti, C.; Chetwynd, D.; Langlois, P. The wear resistance improvement of fibre reinforced polymer composite gears. In Proceedings of the 24th International Conference on Wear of Materials, Miami, FL, USA, 14–18 April 2019; Volume 426–427, pp. 1033–1039. [Google Scholar] [CrossRef]
- Lu, Z.; Liu, H.; Zhu, C.; Song, H.; Yu, G. Identification of failure modes of a PEEK-steel gear pair under lubrication. Int. J. Fatigue 2019, 125, 342–348. [Google Scholar] [CrossRef]
- VDI 2736; Blatt 2, Thermoplastische Zahnräder, Stirngetriebe, Tragfähigkeitsberechnung. VDI Richtlinien: Berlin, Germany, 2014.
- DIN 3990; Calculation of Load Capacity of Cylindrical Gears. German National Standard: Berlin, Germany, 1987.
- Hasl, C.; Liu, H.; Oster, P.; Tobie, T.; Stahl, K. Forschungsstelle fuer Zahnraeder und Getriebebau (Gear Research Centre), Method for calculating the tooth root stress of plastic spur gears meshing with steel gears under consideration of deflection-induced load sharing. Mech. Mach. Theory 2017, 111, 152–163. [Google Scholar]
- Tavčar, J.; Grkman, G.; Duhovnik, J. Accelerated lifetime testing of reinforced polymer gears. J. Adv. Mech. Des. Syst. Manuf. 2018, 12, JAMDSM0006. [Google Scholar] [CrossRef]
- Mohsenzadeh, R.; Soudmand, B.H.; Shelesh-Nezhad, K. Load-bearing analysis of polymer nanocomposite gears using a temperature-based step loading technique: Experimental and numerical study. Wear 2023, 514–515, 204595. [Google Scholar] [CrossRef]
- Lu, Z.; Li, Z.; Liu, H.; Wei, P.; Xia, B.; Ye, M. An accelerated fatigue test method of polymer gears based on thermostress conversion relation. Eng. Fract. Mech. 2022, 266, 108388. [Google Scholar] [CrossRef]
- Tavčar, J.; Černe, B.; Duhovnik, J.; Zorko, D. A multicriteria function for polymer gear design optimization. J. Comput. Des. Eng. 2021, 8, 581–599. [Google Scholar] [CrossRef]
- Urbas, U.; Zorko, D.; Vukašinović, N. Machine learning based nominal root stress calculation model for gears with a progressive curved path of contact. Mech. Mach. Theory 2021, 165, 104430. [Google Scholar] [CrossRef]
- Jia, C.; Wei, P.; Lu, Z.; Ye, M.; Zhu, R.; Liu, H. A novel prediction approach of polymer gear contact fatigue based on a WGAN-XGBoost model. Fatigue Fract. Eng. Mater. Struct. 2023, 46, 2272–2283. [Google Scholar] [CrossRef]
- Trobentar, B.; Kulovec, S.; Hlebanja, G.; Glodež, S. Experimental failure analysis of S-polymer gears. Eng. Fail. Anal. 2020, 111, 104496. [Google Scholar] [CrossRef]
- Trobentar, B.; Hriberšek, M.; Kulovec, S.; Glodež, S.; Belšak, A. Noise Evaluation of S-Polymer Gears. Polymers 2022, 14, 438. [Google Scholar] [CrossRef]
- Koide, T.; Yukawa, T.; Takami, S.; Ueda, A.; Moriwaki, I.; Tamura, A.; Hongu, J. Tooth surface temperature and power transmission efficiency of plastic sine-curve gear. J. Adv. Mech. Des. Syst. Manuf. 2017, 11, JAMDSM0082. [Google Scholar] [CrossRef][Green Version]
- Luo, S.; Wu, Y.; Wang, J. The generation principle and mathematical models of a novel cosine gear drive. Mech. Mach. Theory 2008, 43, 1543–1556. [Google Scholar] [CrossRef]
- Chakroun, A.E.; Hammami, C.; Hammami, A.; De-Juan, A.; Chaari, F.; Fernandez, A.; Viadero, F.; Haddar, M. Gear mesh stiffness of polymer-metal spur gear system using generalized Maxwell model. Mech. Mach. Theory 2022, 175, 104934. [Google Scholar] [CrossRef]
- Hribersek, M.; Erjavec, M.; Hlebanja, G.; Kulovec, S. Durability testing and characterization of POM gears. Eng. Fail. Anal. 2021, 124, 105377. [Google Scholar] [CrossRef]
- Hriberšek, M.; Kulovec, S. Study of the glass fibres and internal lubricants influence in a polyamide 66 matrix on the wear evolution of polyacetal and polyamide 66 based gears in a meshing process. Eng. Fail. Anal. 2022, 134, 106071. [Google Scholar] [CrossRef]
- Feulner, R.W. Verschleiß Trocken Laufender Kunststoffgetriebe: Kennwertermittlung und Auslegung; Univ. Erlangen-Nürnberg, Lehrstuhl für Kunststofftechnik: Erlangen, Germany, 2008; Available online: https://books.google.si/books?id=i1xqPgAACAAJ (accessed on 15 July 2023).
- Zorko, D.; Tavčar, J.; Šturm, R.; Bergant, Z. Investigation of the durability and performance of autoclave-cured, woven carbon fiber-reinforced polymer composite gears in mesh with a steel pinion. Compos. Struct. 2021, 273, 114250. [Google Scholar] [CrossRef]
- Černe, B.; Petkovšek, M. High-speed camera-based optical measurement methods for in-mesh tooth deflection analysis of thermoplastic spur gears. Mater. Des. 2022, 223, 111184. [Google Scholar] [CrossRef]
- Letzelter, E.; Guingand, M.; de Vaujany, J.-P.; Schlosser, P. A new experimental approach for measuring thermal behaviour in the case of nylon 6/6 cylindrical gears. Polym. Test. 2010, 29, 1041–1051. [Google Scholar] [CrossRef]
- Roda-Casanova, V.; Sanchez-Marin, F. A 2D finite element based approach to predict the temperature field in polymer spur gear transmissions. Mech. Mach. Theory 2019, 133, 195–210. [Google Scholar] [CrossRef]
- Černe, B.; Petkovšek, M.; Duhovnik, J.; Tavčar, J. Thermo-mechanical modeling of polymer spur gears with experimental validation using high-speed infrared thermography. Mech. Mach. Theory 2020, 146, 103734. [Google Scholar] [CrossRef]
- Fernandes, C.M.C.G.; Rocha, D.M.P.; Martins, R.C.; Magalhães, L.; Seabra, J.H.O. Finite element method model to predict bulk and flash temperatures on polymer gears. Tribol. Int. 2018, 120, 255–268. [Google Scholar] [CrossRef]
- Düzcükoğlu, H. PA 66 spur gear durability improvement with tooth width modification. Mater. Des. 2009, 30, 1060–1067. [Google Scholar] [CrossRef]
- Kim, C.H. Durability improvement method for plastic spur gears. Tribol. Int. 2006, 39, 1454–1461. [Google Scholar] [CrossRef]
- Fernandes, C.M.C.G.; Rocha, D.M.P.; Martins, R.C.; Magalhães, L.; Seabra, J.H.O. Hybrid Polymer Gear Concepts to Improve Thermal Behavior. J. Tribol. 2019, 141, 032201. [Google Scholar] [CrossRef]
- Hooton, J.D.S.; Gonçalves, D.E.P.; Fernandes, C.M.C.G. Experiments and finite element analysis on a hybrid polymer gear rack. Mech. Mach. Theory 2023, 186, 105363. [Google Scholar] [CrossRef]
- Okubo, H.; Kobayashi, K.; Iba, D.; Moriwaki, I.; Yao, S.; Sasaki, S. Time-resolved ex situ Raman/FT-IR spectroscopic study of structural changes in polymeric gears during operation: Towards the development of operando spectroscopic systems for polymer gears. Polym. Test. 2022, 113, 107675. [Google Scholar] [CrossRef]
- Zorko, D.; Duhovnik, J.; Tavčar, J. Tooth bending strength of gears with a progressive curved path of contact. J. Comput. Des. Eng. 2021, 8, 1037–1058. [Google Scholar] [CrossRef]
- Hakimian, E.; Sulong, A.B. Analysis of warpage and shrinkage properties of injection-molded micro gears polymer composites using numerical simulations assisted by the Taguchi method. Mater. Des. 2012, 42, 62–71. [Google Scholar] [CrossRef]
- Mehat, N.M.; Kamaruddin, S.; Othman, A.R. Modeling and Analysis of Injection Moulding Process Parameters for Plastic Gear Industry Application. ISRN Ind. Eng. 2013, 2013, 1–10. [Google Scholar] [CrossRef]
- Kenda, J.; Duhovnik, J.; Tavčar, J.; Kopač, J. Abrasive flow machining applied to plastic gear matrix polishing. Int. J. Adv. Manuf. Technol. 2014, 71, 141–151. [Google Scholar] [CrossRef]
- ISO 1328-1:2013; Cylindrical Gears—ISO System of Flank Tolerance Classification—Part 1: Definitions and Allowable Values of Deviations Relevant to Flanks of Gear Teeth. International Standard Organization: Geneva, Switzerland, 2013.
- ISO 1328-2:1997; Cylindrical Gears—ISO System of Accuracy—Part 2: Definitions and Allowable Values of Deviations Relevant to Radial Composite Deviations and Runout Information. International Standard Organization: Geneva, Switzerland, 1997.
- DIN 3961/62:1978; Tolerances for Cylindrical Gear Teeth. German National Standard: Berlin, Germany, 1978.
- Wang, S.; Zhou, Y.; Tang, J.; Tang, K.; Li, Z. Digital tooth contact analysis of face gear drives with an accurate measurement model of face gear tooth surface inspected by CMMs. Mech. Mach. Theory 2022, 167, 104498. [Google Scholar] [CrossRef]
- Lin, H.; Keller, F.; Stein, M. Influence and compensation of CMM geometric errors on 3D gear measurements. Measurement 2020, 151, 107110. [Google Scholar] [CrossRef]
- Cacho, R.A.; Mazo, J.S.; Arteta, M.P. Verification Methods for Micro Gears. Analysis of Double Flank Roll Testing Applied to Micro Gears. Procedia Eng. 2013, 63, 142–150. [Google Scholar] [CrossRef][Green Version]
- Tang, J.; Yang, B.; Shi, Z. Influence on center distance by measurement force in double-flank gear rolling test. Measurement 2021, 168, 108321. [Google Scholar] [CrossRef]
- Urbas, U.; Zorko, D.; Černe, B.; Tavčar, J.; Vukašinović, N. A method for enhanced polymer spur gear inspection based on 3D optical metrology. Measurement 2021, 169, 108584. [Google Scholar] [CrossRef]
- Urbas, U.; Hrga, T.; Povh, J.; Vukašinović, N. Novel alignment method for optical 3D gear metrology of spur gears with a plain borehole. Measurement 2022, 192, 110839. [Google Scholar] [CrossRef]
- Zengeya, P.; Mao, K.; Goodship, V. The effects of cooling rate (mould temperature) on HDPE gears produced through injection moulding. Wear 2023, 530–531, 205000. [Google Scholar] [CrossRef]
- ISO 527:2019; Plastics—Determination of Tensile Properties. International Standard Organization: Geneva, Switzerland, 2019.
- ISO 11357-1:2016; Plastics—Differential Scanning Calorimetry (DSC). International Standard Organization: Geneva, Switzerland, 2016.
- DIN 53765; Testing of Plastics and Elastomeres; Thermal Analysis; DSC-Method. German National Standard: Berlin, Germany, 1994.
- ISO 1183:2019; Plastics—Methods for Determining the Density of Non-Cellular Plastics. International Standard Organization: Geneva, Switzerland, 2019.
- Drummer, D.; Gierl, B. Possibilities and Potential of Plastics in Gear Applications. In Proceedings of the International Conference on Gears 2015, Garching, Germany, 5–7 October 2015; pp. 1095–1104. [Google Scholar]
- Zorko, D. Investigation on the high-cycle tooth bending fatigue and thermo-mechanical behavior of polymer gears with a progressive curved path of contact. Int. J. Fatigue 2021, 151, 106394. [Google Scholar] [CrossRef]
- Zorko, D.; Demšar, I.; Tavčar, J. An investigation on the potential of bio-based polymers for use in polymer gear transmissions. Polym. Test. 2021, 93, 106994. [Google Scholar] [CrossRef]
- ISO 53:1998; Cylindrical Gears for General and Heavy Engineering—Standard Basic Rack Tooth Profile. International Standard Organization: Geneva, Switzerland, 1998.
- ISO 6336; Calculation of Load Capacity of Spur and Helical Gears, Parts 1–6. International Standard Organization: Geneva, Switzerland, 2006.
- Černe, B.; Lorber, R.; Duhovnik, J.; Tavčar, J. Influence of temperature- and strain rate-dependent viscoplastic properties of polyoxymethylene on the thermo-mechanical response of a steel-polyoxymethylene spur gear pair. Mater. Today Commun. 2020, 25, 101078. [Google Scholar] [CrossRef]





| Parameter | Value |
|---|---|
| Melt temperature range [°C] | 210–220 |
| Melt temperature optimum [°C] | 215 |
| Mold temperature range [°C] | 80–100 |
| Mold temperature optimum [°C] | 90 |
| Drying time, dehumidified dryer [h] | 2–4 |
| Drying temperature [°C] | 80 |
| Processing moisture content [%] | <0.2 |
| Hold pressure range [MPa] | −110 |
| Parameter | Standard | Unit | Value |
|---|---|---|---|
| Elastic modulus (23 °C) | ISO 527 [48] | MPa | 2900 |
| Yield stress (23 °C) | ISO 527 | MPa | 71 |
| Melting temperature | ISO 11357 [49] | 178 °C | |
| Glass transition temperature | DIN53765 [50] | −35 °C | |
| Density | ISO 1183 [51] | 1.42 g/cm3 |
| Nr. | Melt Temperature [°C] | Mold Temperature [°C] | Cooling Time [s] | Packing Pressure [MPa] | Packing Time [s] |
|---|---|---|---|---|---|
| Classical injection molding | |||||
| C1 | 210 | 90 | 30 | 80 | 5 |
| C2 | 210 | 90 | 50 | 80 | 7 |
| C3 | 210 | 130 | 30 | 120 | 5 |
| C4 | 210 | 130 | 50 | 120 | 7 |
| C5 | 220 | 90 | 50 | 100 | 5 |
| C6 | 220 | 90 | 30 | 100 | 6 |
| C7 | 220 | 130 | 50 | 80 | 5 |
| C8 | 220 | 130 | 30 | 80 | 6 |
| Variotherm | |||||
| V1 | 210 | 90 | 50 | 80 | 7 |
| V2 | 215 | 110 | 30 | 80 | 6 |
| V3 | 220 | 90 | 30 | 100 | 7 |
| Parameter | Value |
|---|---|
| Profile (ISO 53 [55]) | A |
| Normal module [mm] | 1 |
| Number of teeth [/] | 20 |
| Face width [mm] | 6 |
| Reference diameter [mm] | 20 |
| Tip diameter [mm] | 22 |
| Profile shift [/] | 0 |
| Pressure angle [°] | 20 |
| Helix angle [°] | 0 |
| Param. | C1 | C2 | C3 | C4 | C5 | C6 | C7 | C8 | V1 | V2 | V3 |
|---|---|---|---|---|---|---|---|---|---|---|---|
| 9/9 | 9/9 | 12/12 | 10/10 | 8/8 | 8/9 | 11/11 | 12/12 | 8/8 | 11/11 | 11/11 | |
| 7/6 | 9/9 | 9/8 | 7/6 | 8/8 | 10/9 | 8/8 | 8/9 | 6/5 | 7/7 | 6/7 | |
| 10/10 | 9/9 | 12/12 | 11/11 | 9/8 | 9/10 | 12/12 | 12/12 | 8/9 | 12/12 | 12/12 | |
| 7/7 | 8/8 | 9/8 | 11/11 | 8/8 | 9/9 | 8/8 | 9/9 | 6/6 | 5/7 | 6/6 | |
| 4/6 | 7/8 | 6/4 | 8/8 | 7/7 | 9/10 | 6/6 | 6/7 | 2/3 | 4/6 | 5/5 | |
| 8/8 | 8/9 | 10/9 | 12/12 | 9/9 | 9/9 | 9/9 | 10/10 | 7/7 | 7/8 | 7/6 | |
| 5/6 | 7/8 | 7/6 | 10/10 | 7/7 | 10/10 | 6/5 | 7/7 | 6/6 | 8/8 | 8/8 | |
| 8/8 | 11/11 | 10/9 | 12/12 | 10/10 | 9/9 | 7/7 | 10/10 | 9/10 | 11/11 | 11/11 | |
| 9 | 12 | 11 | 12 | 10 | 12 | 9 | 11 | 10 | 12 | 12 | |
| 10 | 12 | 12 | 12 | 10 | 12 | 12 | 12 | 10 | 12 | 12 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zorko, D. Effect of Process Parameters on the Crystallinity and Geometric Quality of Injection Molded Polymer Gears and the Resulting Stress State during Gear Operation. Polymers 2023, 15, 4118. https://doi.org/10.3390/polym15204118
Zorko D. Effect of Process Parameters on the Crystallinity and Geometric Quality of Injection Molded Polymer Gears and the Resulting Stress State during Gear Operation. Polymers. 2023; 15(20):4118. https://doi.org/10.3390/polym15204118
Chicago/Turabian StyleZorko, Damijan. 2023. "Effect of Process Parameters on the Crystallinity and Geometric Quality of Injection Molded Polymer Gears and the Resulting Stress State during Gear Operation" Polymers 15, no. 20: 4118. https://doi.org/10.3390/polym15204118
APA StyleZorko, D. (2023). Effect of Process Parameters on the Crystallinity and Geometric Quality of Injection Molded Polymer Gears and the Resulting Stress State during Gear Operation. Polymers, 15(20), 4118. https://doi.org/10.3390/polym15204118






