Understanding the Behavior of Sodium Polyacrylate in Suspensions of Silica and Monovalent Salts
Abstract
:1. Introduction
2. Experimental Methodology
2.1. Materials
2.2. Rheology
3. Computer Simulation
3.1. Molecules and Surfaces
3.2. Force Field
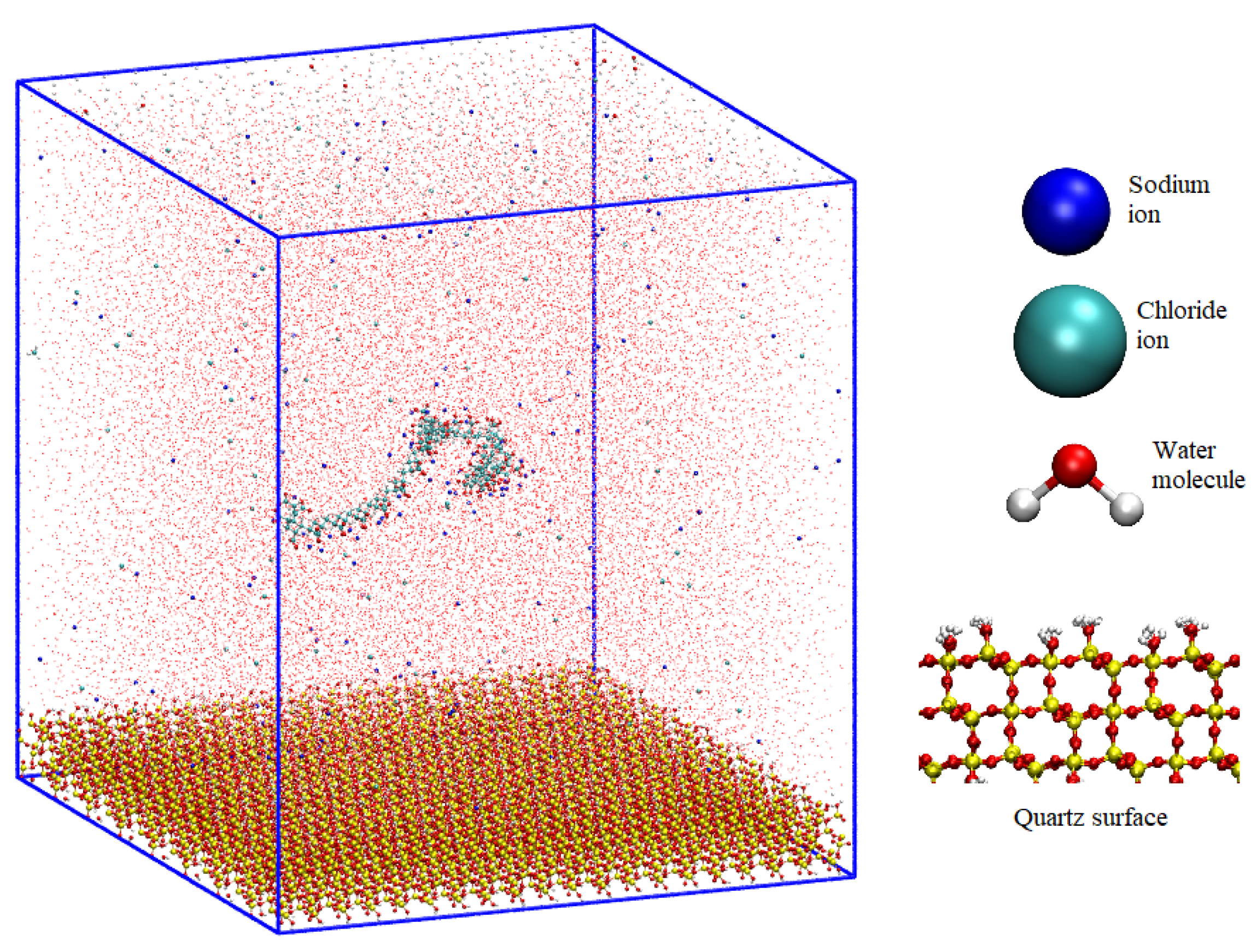
3.3. Initial Setup
- (1)
- Once the quartz surface with dimensions (Sx × Sy × Sz) was constructed, the dimensions Sx and Sy were employed to define the LX and LY of the simulation box. Subsequently, dimension Lz was established to encompass both the surface and an aqueous solution containing the polymer. Lz was set at 12 nm. Consequently, the simulation box dimensions were (LX, LY, LZ) = 9.832 × 11.056 × 12.000 nm³. The selection of these dimensions is critical to prevent interactions between the polymer and its periodic images.
- (2)
- The polymer was placed in the previously generated space, positioned 5 nm above the surface along the z-direction. This polymer consisted of 48 monomers and had an initial length of 12 nm. Although this length is comparable to the box size, it substantially reduced to less than 5 nm during equilibration stages.
- (3)
- Ions corresponding to the salts investigated in this study were randomly distributed within the space occupied by the flocculant, maintaining a separation of 1 nm between them and the polymer. The concentrations utilized in this investigation were 0.001, 0.01, and 0.1 moles/liter. The total number of cations and anions was determined based on the salt concentration and the counterions necessary to neutralize both the quartz surface and the PA.
- (4)
- Finally, water from an equilibrated configuration at 300 K was introduced, and this configuration was replicated throughout the box until completion. Water molecules that coincided with the preexisting elements in the box were removed. The elimination criterion was based on less than 0.3 nm between the water molecules and other atoms (the initial setup can be seen in Figure 1).
3.4. Molecular Simulation
- Minimization of forces in the initial configuration. This step is essential since the initial configuration stems from stable yet vacant configurations, which necessarily alter upon their assembly. For quartz, solely hydrogen atoms were allowed movement.
- NVT-equilibration simulation for 0.1 ns. This simulation was conducted at 300 K, with the positions of the polymer and ions held fixed, while only water molecules exhibited motion.
- NVT-equilibration simulation with annealing for 1 ns. During this simulation, the temperature rapidly increased from 300 to 450 K within 0.0001 ns, followed by a 0.5 ns maintenance at the elevated temperature and then a return to 300 K over 0.5 ns. This annealing process enhanced polymer fluidization in the liquid phase, achieving a stable configuration.
- NPT-equilibration simulation for 2 ns. Conducted at 300 K and 1 bar pressure, adjustments to the simulation box were confined to the z-direction to relax the pressure to the predefined value.
- Lastly, an NVT-production simulation spanning 100 ns captured the time evolution of the PA’s interaction with the quartz surface under varying salt concentrations. Six repetitions were performed to mitigate statistical error.
3.5. Data Processing
4. Results
4.1. Rheological Behavior
- (i)
- An enlargement in cation size precipitated an elevation in yield stress due to the fortification of interparticle bonding. Considering that quartz lends itself to a conceptualization akin to “breaker” salts in certain aspects, it is foreseeable that pulps experience a more pronounced loss of fluidity in the presence of such salts. This observation aligns with the findings reported by Jeldres et al. [57], who proffered an explanation informed by electrostatic and structural considerations of water behavior to characterize rheological conduct.
- (ii)
- Sodium polyacrylate evinced heightened effectiveness in tandem with smaller salts. The augmented effectiveness can be attributed to its superior adeptness in traversing the hydrated layer enveloping surfaces, particularly in scenarios where the ionic milieu comprises “maker” salts.
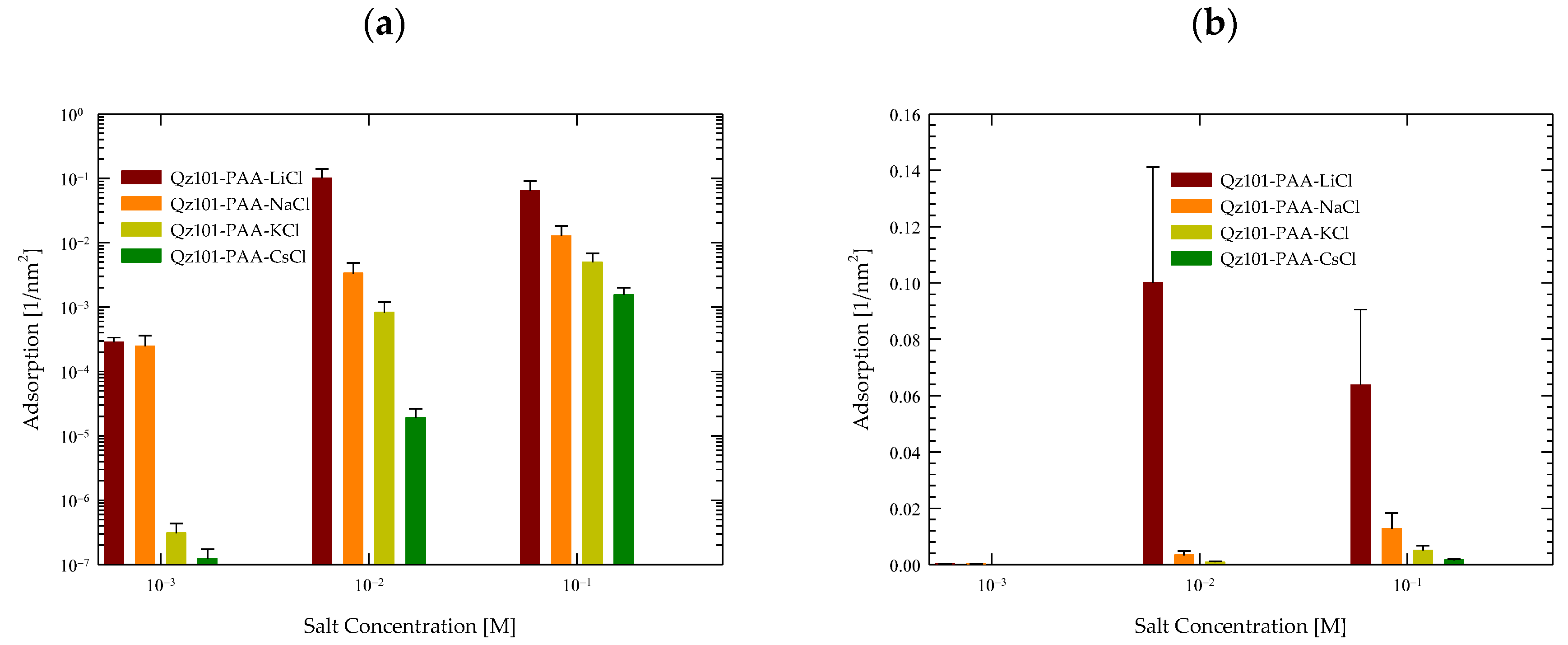
4.2. Polymer Adsorption
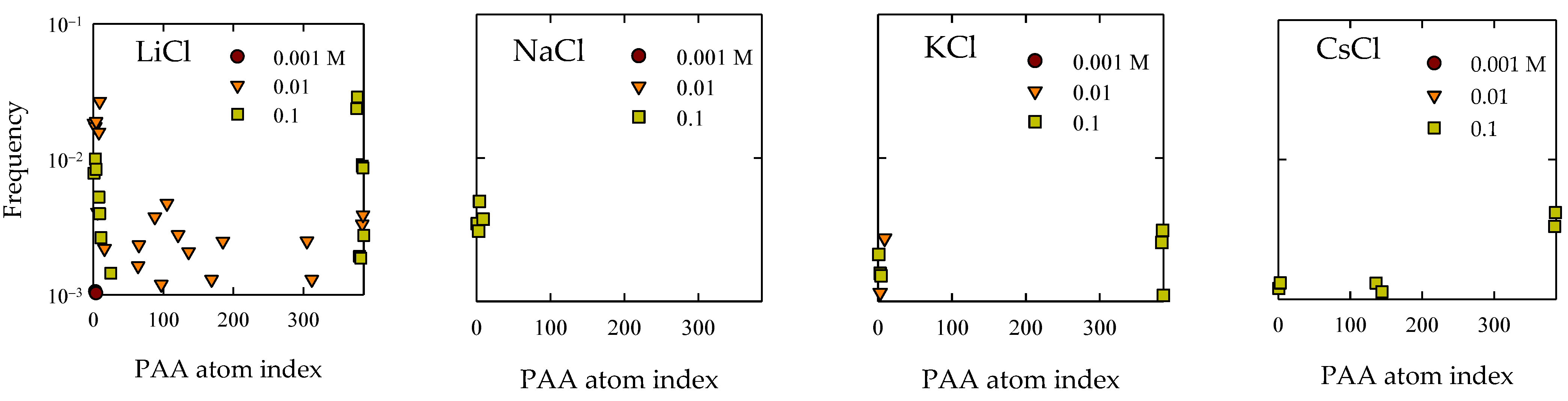
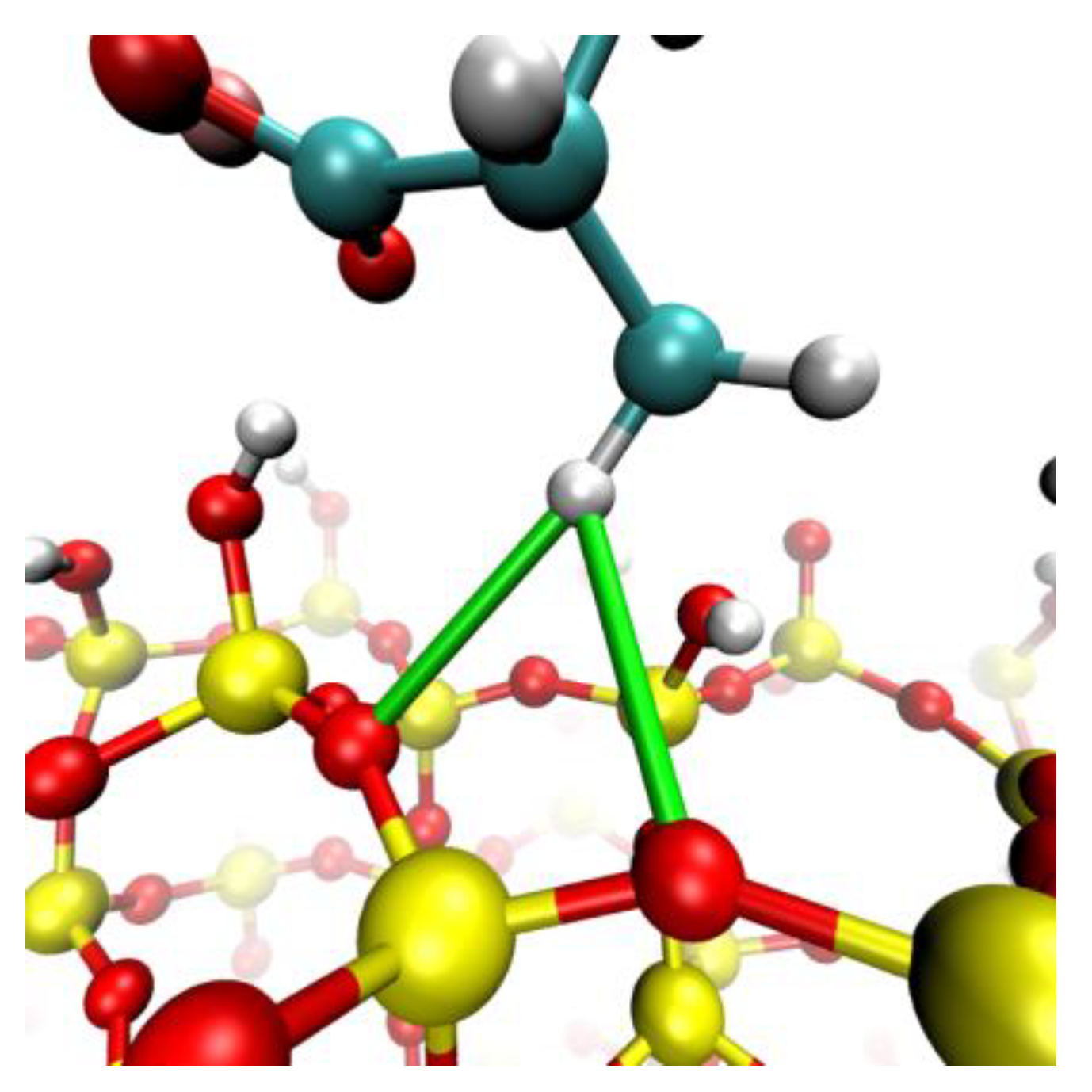
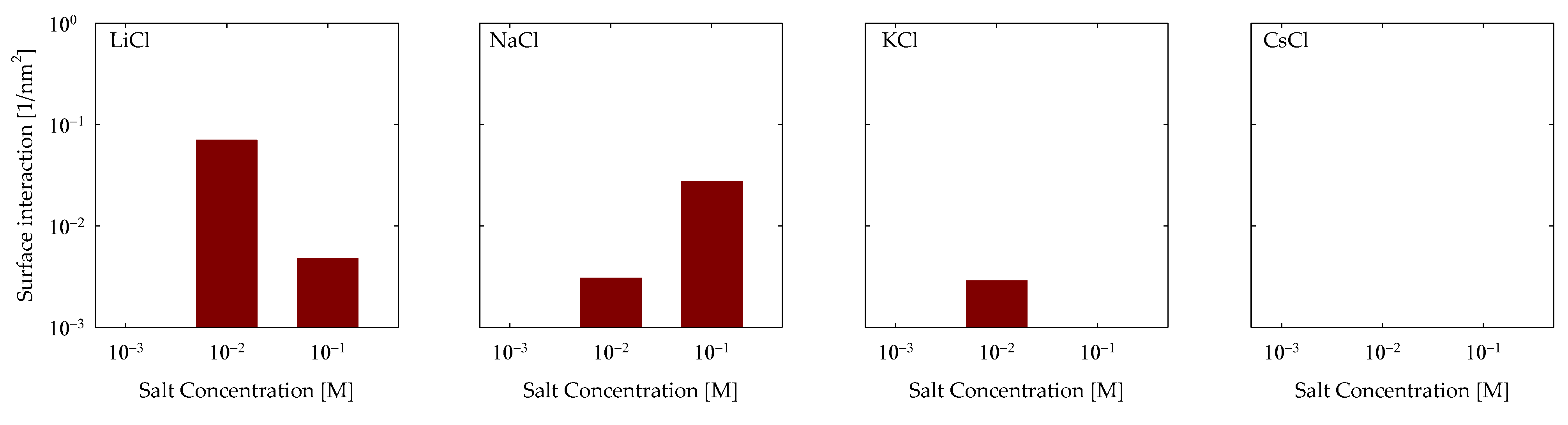
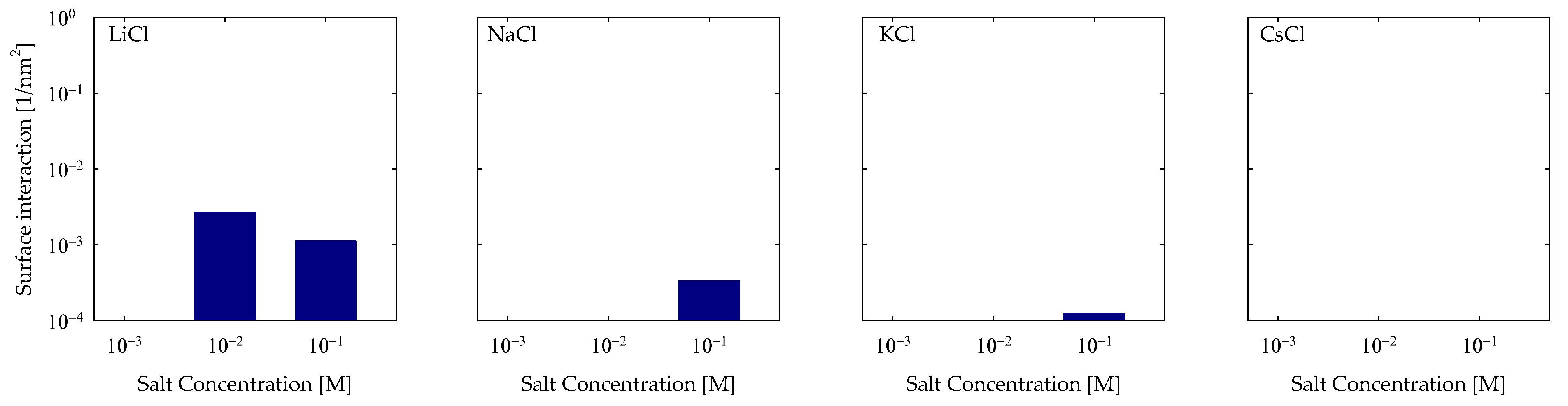
4.3. Polymer Interaction
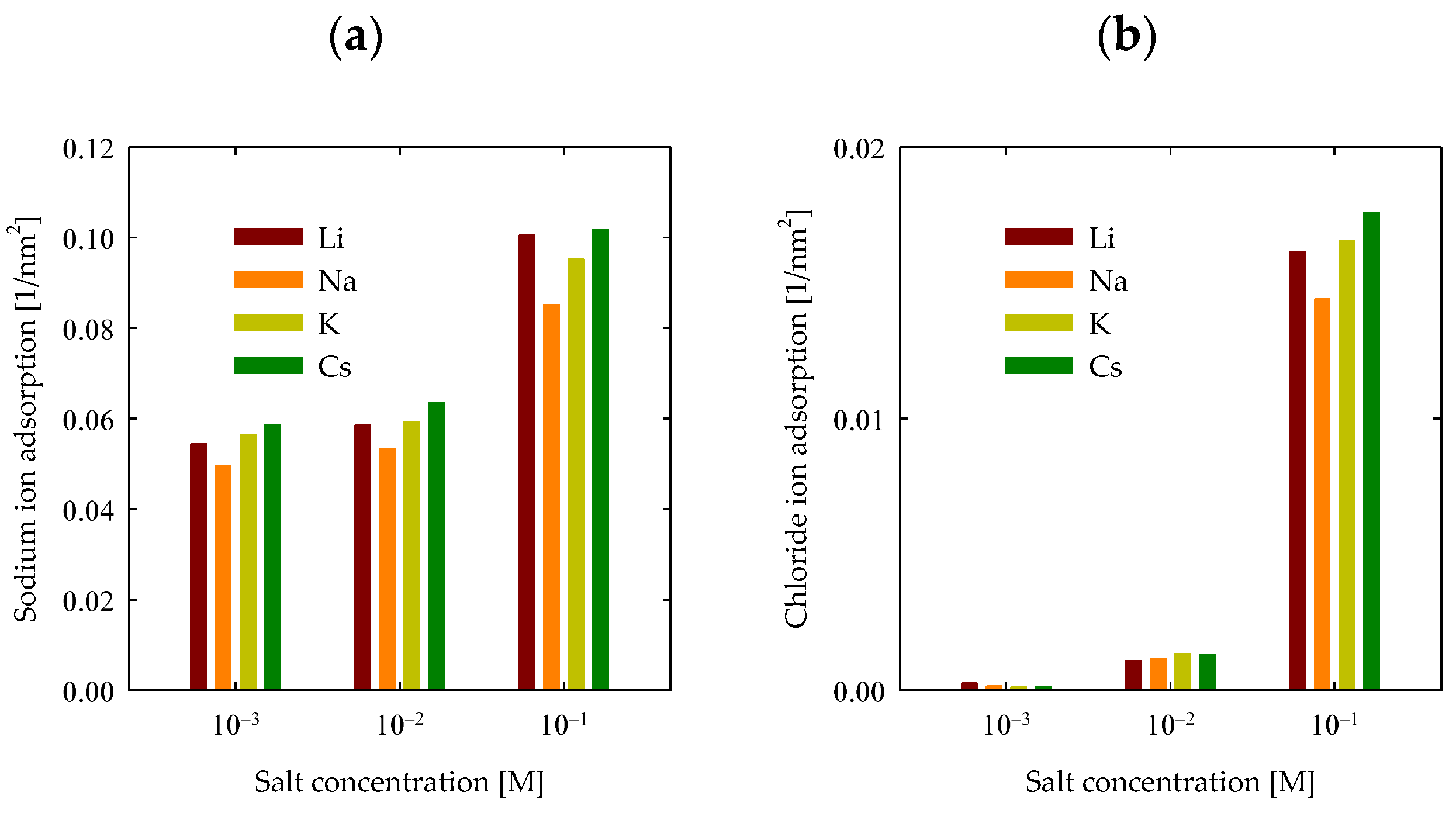
4.4. Ion Adsorption
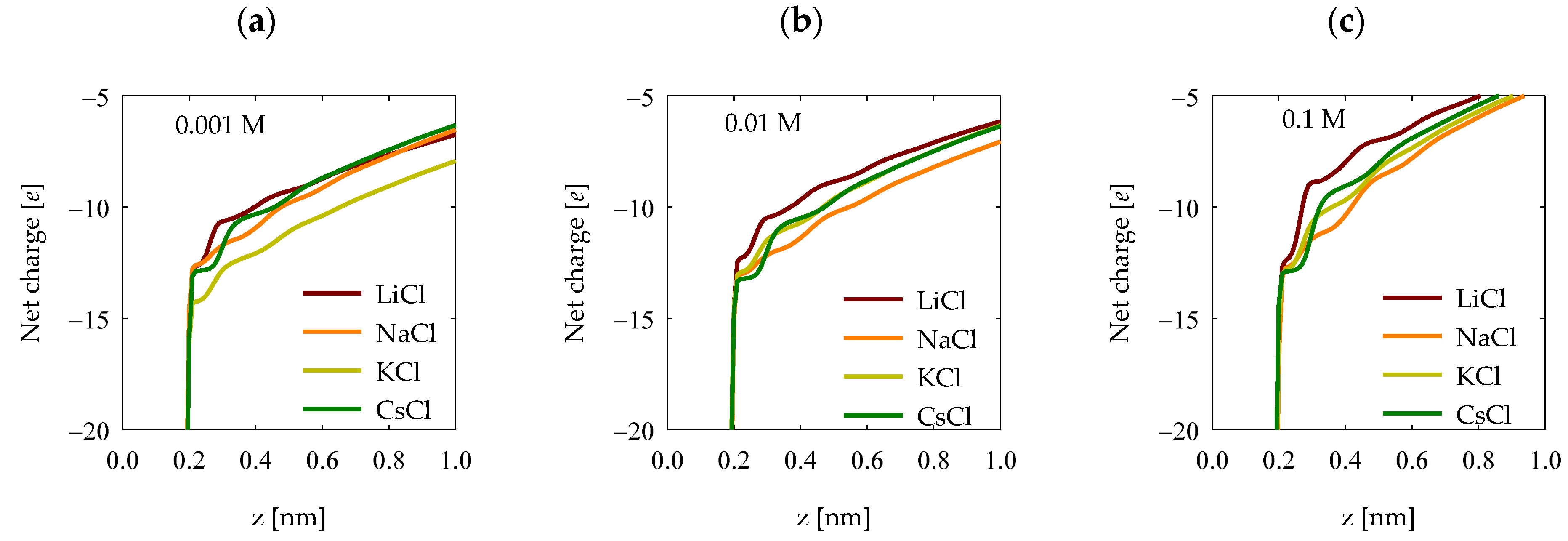
4.5. Net Charge
5. Conclusions
- It was confirmed that the yield stress is directly affected by the presence of cations following a specific sequence: Cs > K > Na > Li. This suggests that the adsorbed cations of the “breaker” type have a higher affinity for the quartz surface without the presence of polyacrylate. This observation is related to the weak charge density on the quartz surface, which predisposes it to interact more favorably with low charge density breaking cations.
- The addition of PAA was shown to reduce the yield stress in all cases studied, which indicates its leading role as a dispersant for quartz particles. However, it has been observed that this reduction is more marked in the presence of Li and Na compared to K and Cs. This implies that PA exerts a stronger effect in the presence of Li and Na, suggesting that “maker” ions with PA can overcome the breaker-adsorbed ions in quartz.
- Molecular dynamics simulations supported the experimental results, revealing that the adsorption of the PA polymer on quartz follows the sequence Li > Na > K > Cs. Furthermore, it was observed that higher salt concentrations increase the adsorption of PA on the quartz surface. These results align with the experimental findings, indicating that the presence of cations facilitates PA adsorption.
- Molecular interactions revealed that the formation of cationic bridges was more pronounced on Li and Na, and these bridges contribute to the formation of hydrogen bonds and hydrophobic bridges, increasing adsorption stability on Li and Na. In contrast, the interactions are weak in the cases of K and Cs.
- These results highlight the utility of computational simulations to support experimental observations and provide a deeper understanding of the optimal conditions for admixture rheology modifications in mining processes.
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lewis, J.A. Organic Processing Additives. Encycl. Mater. Sci. Technol. 2001, 6556–6560. [Google Scholar] [CrossRef]
- Loiseau, J.; Doërr, N.; Suau, J.M.; Egraz, J.B.; Llauro, M.F.; Ladavière, C.; Claverie, J. Synthesis and characterization of poly(acrylic acid) produced by RAFT polymerization. Application as a very efficient dispersant of CaCO3, Kaolin, and TiO2. Macromolecules 2003, 36, 3066–3077. [Google Scholar] [CrossRef]
- Brusotti, G.; Calleri, E.; Milanese, C.; Catenacci, L.; Marrubini, G.; Sorrenti, M.; Girella, A.; Massolini, G.; Tripodo, G. Rational design of functionalized polyacrylate-based high internal phase emulsion materials for analytical and biomedical uses. Polym. Chem. 2016, 7, 7436–7445. [Google Scholar] [CrossRef]
- Sabatini, V.; Pellicano, L.; Farina, H.; Pargoletti, E.; Annunziata, L.; Ortenzi, M.A.; Stori, A.; Cappelletti, G. Design of new polyacrylate microcapsules to modify the water-soluble active substances release. Polymers 2021, 13, 809. [Google Scholar] [CrossRef]
- Sternberg, M.; Hershberger, D. Separation of proteins with polyacrylic acids. BBA-Protein Struct. 1974, 342, 195–206. [Google Scholar] [CrossRef]
- Vaz, F.A.S.; De Castro, P.M.; Molina, C.; Ribeiro, S.J.L.; Polachini, F.C.; Messaddeq, Y.; Nunes, A.P.; De Oliveira, M.A.L. External polyacrylate-coating as alternative material for preparation of photopolymerized sol-gel monolithic column. Talanta 2008, 76, 226–229. [Google Scholar] [CrossRef]
- Li, H.; Zhou, J.; Yu, J.; Zhao, J. Light-activated cellulose nanocrystals/fluorinated polyacrylate-based waterborne coating: Facile preparation, mechanical and self-healing behavior. Int. J. Biol. Macromol. 2023, 249, 126062. [Google Scholar] [CrossRef]
- Komaba, S.; Okushi, K.; Ozeki, T.; Yui, H.; Katayama, Y.; Miura, T.; Saito, T.; Groult, H. Polyacrylate modifier for graphite anode of lithiumion batteries. Electrochem. Solid-State Lett. 2009, 12, A107. [Google Scholar] [CrossRef]
- Ma, X. Effect of a low-molecular-weight polyacrylic acid on the coagulation of kaolinite particles. Int. J. Miner. Process. 2011, 99, 17–20. [Google Scholar] [CrossRef]
- Sahi, A.; Mahboub, K.; El Belem, T.; Maqsoud, A.; Mbonimpa, M. Dewatering of mine tailings slurries using superabsorbent polymers (SAPs) reclaimed from industrial reject of baby diapers: A preliminary study. Minerals 2019, 9, 785. [Google Scholar] [CrossRef]
- Jeldres, M.; Robles, P.; Toledo, P.G.; Saldaña, M.; Quezada, L.; Jeldres, R.I. Improved dispersion of clay-rich tailings in seawater using sodium polyacrylate. Colloids Surf. A Physicochem. Eng. Asp. 2021, 612, 126015. [Google Scholar] [CrossRef]
- Robles, P.; Piceros, E.; Leiva, W.H.; Valenzuela, J.; Toro, N.; Jeldres, R.I. Analysis of sodium polyacrylate as a rheological modifier for kaolin suspensions in seawater. Appl. Clay Sci. 2019, 183, 105328. [Google Scholar] [CrossRef]
- Quezada, G.R.; Piceros, E.; Robles, P.; Moraga, C.; Gálvez, E.; Nieto, S.; Jeldres, R.I. Polyacrylic Acid to Improve Flotation Tailings Management: Understanding the Chemical Interactions through Molecular Dynamics. Metals 2021, 11, 987. [Google Scholar] [CrossRef]
- Cisternas, L.A.; Gálvez, E.D. The Use of Seawater in Mining; Taylor & Francis: Abingdon, UK, 2018; Volume 39, pp. 18–33. [Google Scholar]
- Liu, D.; Edraki, M.; Fawell, P.; Berry, L. Improved water recovery: A review of clay-rich tailings and saline water interactions. Powder Technol. 2020, 364, 604–621. [Google Scholar]
- Northey, S.A.; Mudd, G.M.; Werner, T.T.; Jowitt, S.M.; Haque, N.; Yellishetty, M.; Weng, Z. The exposure of global base metal resources to water criticality, scarcity and climate change. Glob. Environ. Chang. 2017, 44, 109–124. [Google Scholar] [CrossRef]
- Ozboyaci, M.; Kokh, D.B.; Corni, S.; Wade, R.C. Modeling and simulation of protein-surface interactions: Achievements and challenges. Q. Rev. Biophys. 2016, 49, e4. [Google Scholar] [CrossRef]
- Tong, Z.; Xie, Y.; Zhang, Y. Molecular dynamics simulation on the interaction between polymer inhibitors and β-dicalcium silicate surface. J. Mol. Liq. 2018, 259, 65–75. [Google Scholar] [CrossRef]
- Zuo, Z.; Liang, L.; Bao, Q.; Yan, P.; Jin, X.; Yang, Y. Molecular Dynamics Calculation on the Adhesive Interaction Between the Polytetrafluoroethylene Transfer Film and Iron Surface. Front. Chem. 2021, 9, 740447. [Google Scholar] [CrossRef]
- Wang, R.; Klein, M.L.; Carnevale, V.; Borguet, E. Investigations of water/oxide interfaces by molecular dynamics simulations. Wiley Interdiscip. Rev. Comput. Mol. Sci. 2021, 11, e1537. [Google Scholar] [CrossRef]
- Butenuth, A.; Moras, G.; Schneider, J.; Koleini, M.; Köppen, S.; Meißner, R.; Wright, L.B.; Walsh, T.R.; Ciacchi, L.C. Ab initio derived force-field parameters for molecular dynamics simulations of deprotonated amorphous-SiO2/water interfaces. Phys. Status Solidi (B) Basic Res. 2012, 249, 292–305. [Google Scholar] [CrossRef]
- Emami, F.S.; Puddu, V.; Berry, R.J.; Varshney, V.; Patwardhan, S.V.; Perry, C.C.; Heinz, H. Force field and a surface model database for silica to simulate interfacial properties in atomic resolution. Chem. Mater. 2014, 26, 2647–2658. [Google Scholar] [CrossRef]
- Schlegel, M.L.; Nagy, K.L.; Fenter, P.; Sturchio, N.C. Structures of quartz (1010)- and (1011)-water interfaces determined by X-ray reflectivity and atomic force microscopy of natural growth surfaces. Geochim. Cosmochim. Acta 2002, 66, 3037–3054. [Google Scholar] [CrossRef]
- Skelton, A.A.; Fenter, P.; Kubicki, J.D.; Wesolowski, D.J.; Cummings, P.T. Simulations of the quartz(1011)/water interface: A comparison of classical force fields, Ab initio molecular dynamics, and X-ray reflectivity experiments. J. Phys. Chem. C 2011, 115, 2076–2088. [Google Scholar] [CrossRef]
- Brkljača, Z.; Namjesnik, D.; Lützenkirchen, J.; Předota, M.; Preočanin, T. Quartz/Aqueous Electrolyte Solution Interface: Molecular Dynamic Simulation and Interfacial Potential Measurements. J. Phys. Chem. C 2018, 122, 24025–24036. [Google Scholar] [CrossRef]
- DelloStritto, M.J.; Kubicki, J.D.; Sofo, J.O. Effect of Ions on H-Bond Structure and Dynamics at the Quartz(101)-Water Interface. Langmuir 2016, 32, 11353–11365. [Google Scholar] [CrossRef] [PubMed]
- Franks, G.V. Zeta potentials and yield stresses of silica suspensions in concentrated monovalent electrolytes: Isoelectric point shift and additional attraction. J. Colloid Interface Sci. 2002, 249, 44–51. [Google Scholar] [CrossRef]
- Kroutil, O.; Chval, Z.; Skelton, A.A.; Předota, M. Computer simulations of quartz (101)-water interface over a range of pH values. J. Phys. Chem. C 2015, 119, 9274–9286. [Google Scholar] [CrossRef]
- Porus, M.; Labbez, C.; Maroni, P.; Borkovec, M. Adsorption of monovalent and divalent cations on planar water-silica interfaces studied by optical reflectivity and Monte Carlo simulations. J. Chem. Phys. 2011, 135, 064701. [Google Scholar] [CrossRef]
- Quezada, G.R.; Rozas, R.E.; Toledo, P.G. Molecular Dynamics Simulations of Quartz (101)-Water and Corundum (001)-Water Interfaces: Effect of Surface Charge and Ions on Cation Adsorption, Water Orientation, and Surface Charge Reversal. J. Phys. Chem. C 2017, 121, 25271–25282. [Google Scholar] [CrossRef]
- Duan, H.; Liu, W.; Wang, X.; Liu, W.; Zhang, X. Effect of secondary amino on the adsorption of N-Dodecylethylenediamine on quartz surface: A molecular dynamics study. Powder Technol. 2019, 351, 46–53. [Google Scholar] [CrossRef]
- Li, L.; Hao, H.; Yuan, Z.; Liu, J. Molecular dynamics simulation of siderite-hematite-quartz flotation with sodium oleate. Appl. Surf. Sci. 2017, 419, 557–563. [Google Scholar] [CrossRef]
- Quezada, G.R.; Jeldres, R.I.; Fawell, P.D.; Toledo, P.G. Use of molecular dynamics to study the conformation of an anionic polyelectrolyte in saline medium and its adsorption on a quartz surface. Miner. Eng. 2018, 129, 102–105. [Google Scholar] [CrossRef]
- Xu, Y.; Liu, Y.L.; Liu, G.S. Molecular dynamics simulation of primary ammonium ions with different alkyl chains on the muscovite (001) surface. Int. J. Miner. Process. 2015, 145, 48–56. [Google Scholar] [CrossRef]
- Jeldres, M.; Toro, N.; Gallegos, S.; Robles, P.; Salazar, I.; Fawell, P.D.; Jeldres, R.I. Reducing Magnesium within Seawater Used in Mineral Processing to Improve Water Recovery and Rheological Properties When Dewatering Clay-Based Tailings. Polymers 2022, 14, 339. [Google Scholar] [CrossRef]
- Quezada, G.R.; Toro, N.; Saavedra, J.; Robles, P.; Salazar, I.; Navarra, A.; Jeldres, R.I. Molecular Dynamics Study of the Conformation, Ion Adsorption, Diffusion and Water Structure of Soluble Polymers in Saline Solutions. Polymers 2021, 13, 3550. [Google Scholar] [CrossRef]
- Mintis, D.G.; Alexiou, T.S.; Mavrantzas, V.G. Effect of pH and Molecular Length on the Structure and Dynamics of Linear and Short-Chain Branched Poly(ethylene imine) in Dilute Solution: Scaling Laws from Detailed Molecular Dynamics Simulations. J. Phys. Chem. B 2020, 124, 6154–6169. [Google Scholar] [CrossRef]
- Mintis, D.G.; Mavrantzas, V.G. Effect of pH and Molecular Length on the Structure and Dynamics of Short Poly(acrylic acid) in Dilute Solution: Detailed Molecular Dynamics Study. J. Phys. Chem. B 2019, 123, 4204–4219. [Google Scholar] [CrossRef]
- Wang, J.; Wang, W.; Kollman, P.A.; Case, D.A. Automatic atom type and bond type perception in molecular mechanical calculations. J. Mol. Graph. Model. 2006, 25, 247–260. [Google Scholar] [CrossRef]
- Wang, J.; Wolf, R.M.; Caldwell, J.W.; Kollman, P.A.; Case, D.A. Development and testing of a general Amber force field. J. Comput. Chem. 2004, 25, 1157–1174. [Google Scholar] [CrossRef]
- Cornell, W.D.; Cieplak, P.; Bayly, C.I.; Kollman, P.A. Application of RESP charges to calculate conformational energies, hydrogen bond energies, and free energies of solvation. J. Am. Chem. Soc. 2002, 115, 9620–9631. [Google Scholar] [CrossRef]
- Dupradeau, F.-Y.; Pigache, A.; Zaffran, T.; Savineau, C.; Lelong, R.; Grivel, N.; Lelong, D.; Rosanski, W.; Cieplak, P. The R.E.D. Tools: Advances in RESP and ESP charge derivation and force field library building. Phys. Chem. Chem. Phys. 2010, 12, 7821. [Google Scholar] [CrossRef] [PubMed]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Mennucci, B.; Petersson, G.A.; et al. Gaussian 09, Revision D.01 2013. Available online: https://silo.tips/download/gaussian-09-revision-d01-release-notes-4-june-2013 (accessed on 1 September 2023).
- Li, P.; Song, L.F.; Merz, K.M. Systematic parameterization of monovalent ions employing the nonbonded model. J. Chem. Theory Comput. 2015, 11, 1645–1657. [Google Scholar] [CrossRef] [PubMed]
- Berendsen, H.J.C.; Grigera, J.R.; Straatsma, T.P. The missing term in effective pair potentials. J. Phys. Chem. 1987, 91, 6269–6271. [Google Scholar] [CrossRef]
- Miyamoto, S.; Kollman, P.A. Settle: An analytical version of the SHAKE and RATTLE algorithm for rigid water models. J. Comput. Chem. 1992, 13, 952–962. [Google Scholar] [CrossRef]
- Cygan, R.T.; Liang, J.J.; Kalinichev, A.G. Molecular models of hydroxide, oxyhydroxide, and clay phases and the development of a general force field. J. Phys. Chem. B 2004, 108, 1255–1266. [Google Scholar] [CrossRef]
- Zhang, C.; Xu, Z.; Hu, Y.; He, J.; Tian, M.; Zhou, J.; Zhou, Q.; Chen, S.; Chen, D.; Sun, W. Novel Insights into the Hydroxylation Behaviors of α-Quartz (101) Surface and its Effects on the Adsorption of Sodium Oleate. Minerals 2019, 9, 450. [Google Scholar] [CrossRef]
- Abraham, M.J.; Murtola, T.; Schulz, R.; Páll, S.; Smith, J.C.; Hess, B.; Lindah, E. Gromacs: High performance molecular simulations through multi-level parallelism from laptops to supercomputers. SoftwareX 2015, 1–2, 19–25. [Google Scholar] [CrossRef]
- Sousa da Silva, A.W.; Vranken, W.F. ACPYPE—AnteChamber PYthon Parser interfacE. BMC Res. Notes 2012, 5, 367. [Google Scholar] [CrossRef]
- Hess, B.; Bekker, H.; Berendsen, H.J.C.; Fraaije, J.G.E.M. LINCS: A Linear Constraint Solver for molecular simulations. J. Comput. Chem. 1997, 18, 1463–1472. [Google Scholar] [CrossRef]
- Hoover, W.G. Canonical dynamics: Equilibrium phase-space distributions. Phys. Rev. A 1985, 31, 1695. [Google Scholar] [CrossRef]
- Nosé, S. A unified formulation of the constant temperature molecular dynamics methods. J. Chem. Phys. 1984, 81, 511–519. [Google Scholar] [CrossRef]
- Parrinello, M.; Rahman, A. Polymorphic transitions in single crystals: A new molecular dynamics method. J. Appl. Phys. 1981, 52, 7182–7190. [Google Scholar] [CrossRef]
- Darden, T.; York, D.; Pedersen, L. Particle mesh Ewald: An N·log(N) method for Ewald sums in large systems. J. Chem. Phys. 1993, 98, 10089–10092. [Google Scholar] [CrossRef]
- Quezada, G.R.; Piceros, E.; Saavedra, J.H.; Robles, P.; Jeldres, R.I. Polymer affinity with quartz (1 0 1) surface in saline solutions: A molecular dynamics study. Miner. Eng. 2022, 186, 107750. [Google Scholar] [CrossRef]
- Jeldres, R.I.; Piceros, E.C.; Leiva, W.H.; Toledo, P.G.; Quezada, G.R.; Robles, P.A.; Valenzuela, J. Analysis of silica pulp viscoelasticity in saline media: The effect of cation size. Minerals 2019, 9, 216. [Google Scholar] [CrossRef]
- Quezada, G.R.; Toledo, P.G. Complexation of Alkali and Alkaline-Earth Metal Cations at Spodumene-Saltwater Interfaces by Molecular Simulation: Impact on Oleate Adsorption. Minerals 2020, 11, 12. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Quezada, G.R.; Retamal, F.; Jeldres, M.; Jeldres, R.I. Understanding the Behavior of Sodium Polyacrylate in Suspensions of Silica and Monovalent Salts. Polymers 2023, 15, 3861. https://doi.org/10.3390/polym15193861
Quezada GR, Retamal F, Jeldres M, Jeldres RI. Understanding the Behavior of Sodium Polyacrylate in Suspensions of Silica and Monovalent Salts. Polymers. 2023; 15(19):3861. https://doi.org/10.3390/polym15193861
Chicago/Turabian StyleQuezada, Gonzalo R., Francisco Retamal, Matías Jeldres, and Ricardo I. Jeldres. 2023. "Understanding the Behavior of Sodium Polyacrylate in Suspensions of Silica and Monovalent Salts" Polymers 15, no. 19: 3861. https://doi.org/10.3390/polym15193861
APA StyleQuezada, G. R., Retamal, F., Jeldres, M., & Jeldres, R. I. (2023). Understanding the Behavior of Sodium Polyacrylate in Suspensions of Silica and Monovalent Salts. Polymers, 15(19), 3861. https://doi.org/10.3390/polym15193861








