The Development of Efficient Contaminated Polymer Materials Shredding in Recycling Processes
Abstract
1. Introduction
2. Recycling Engineering and Shredding
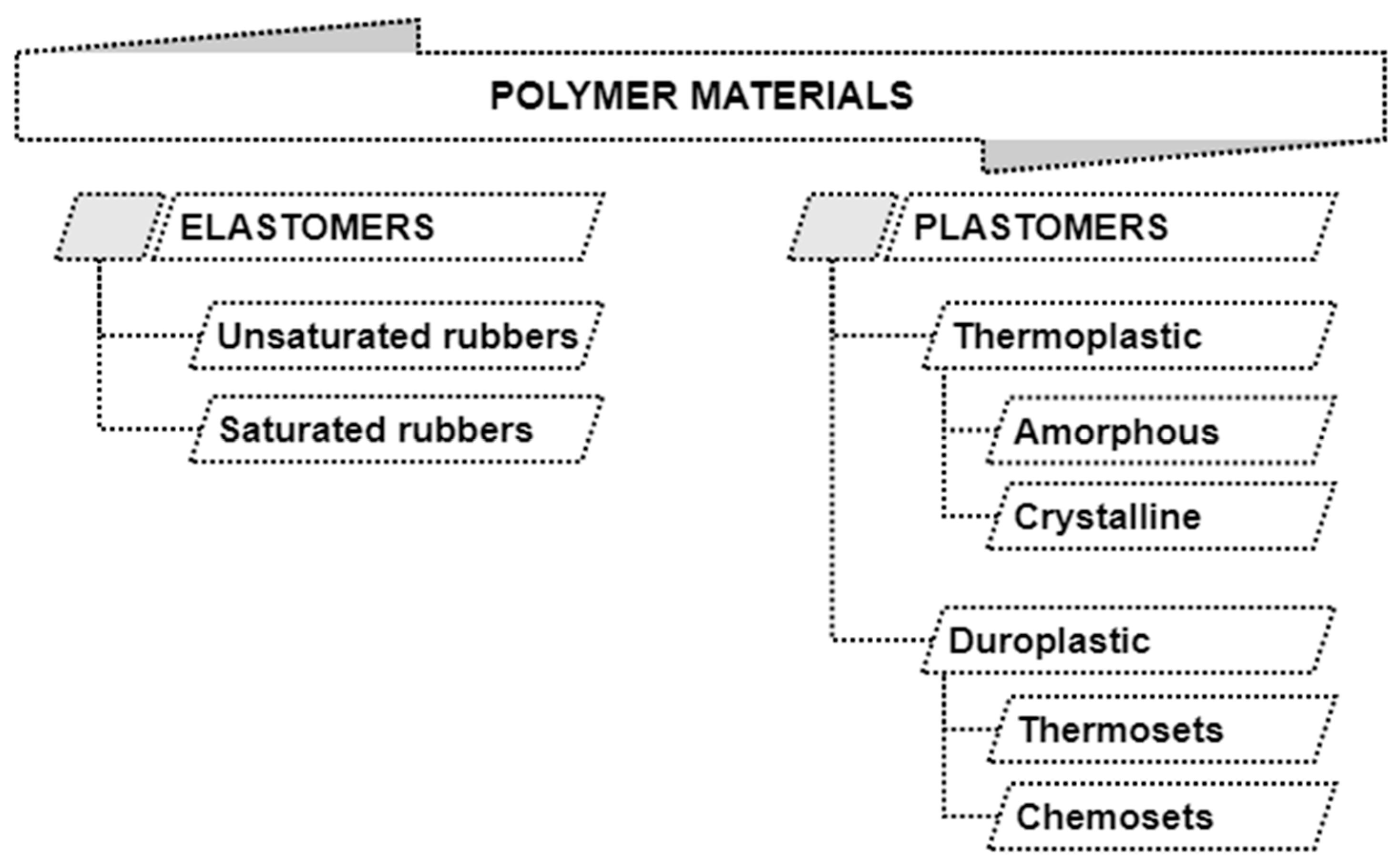
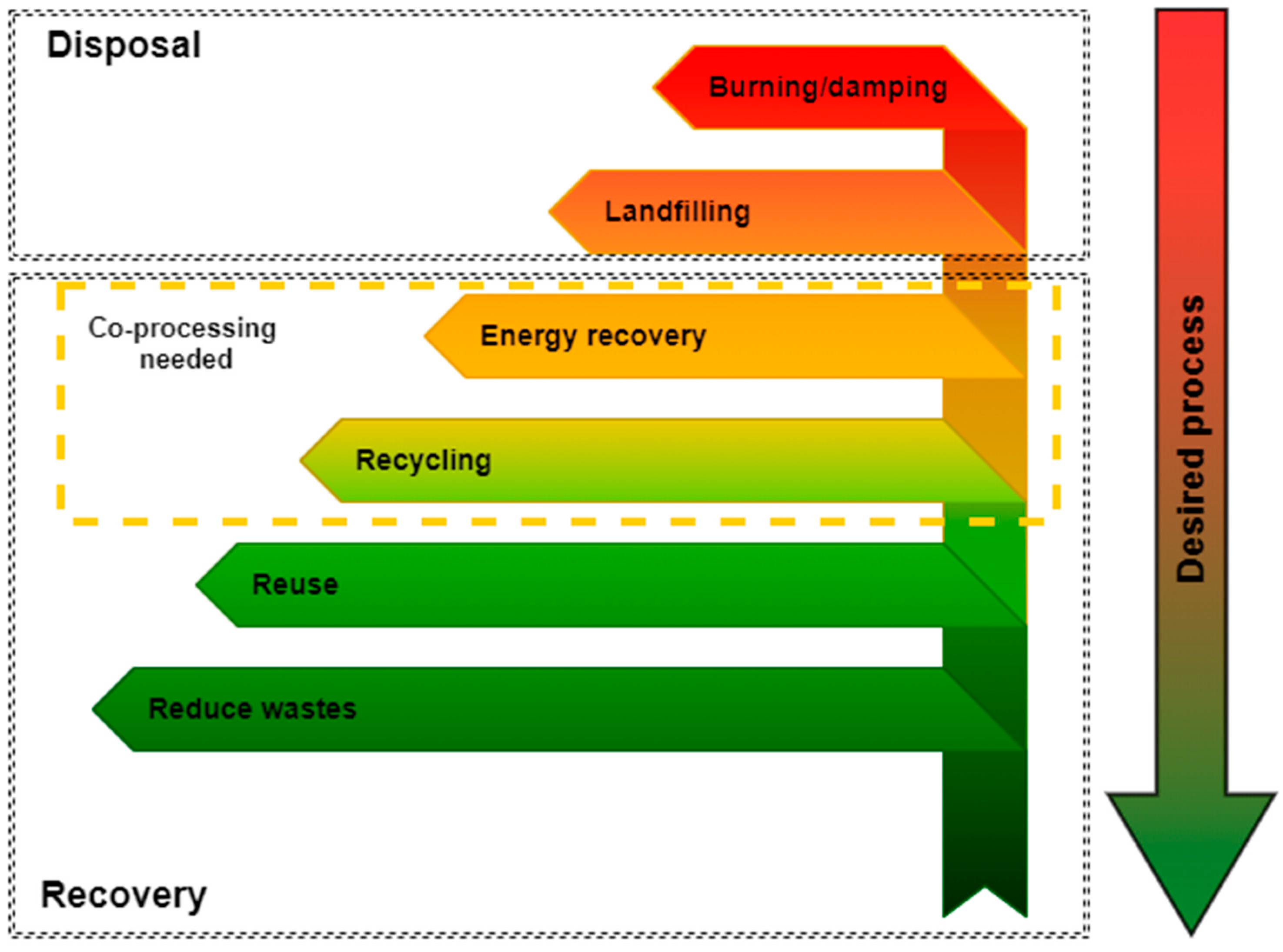
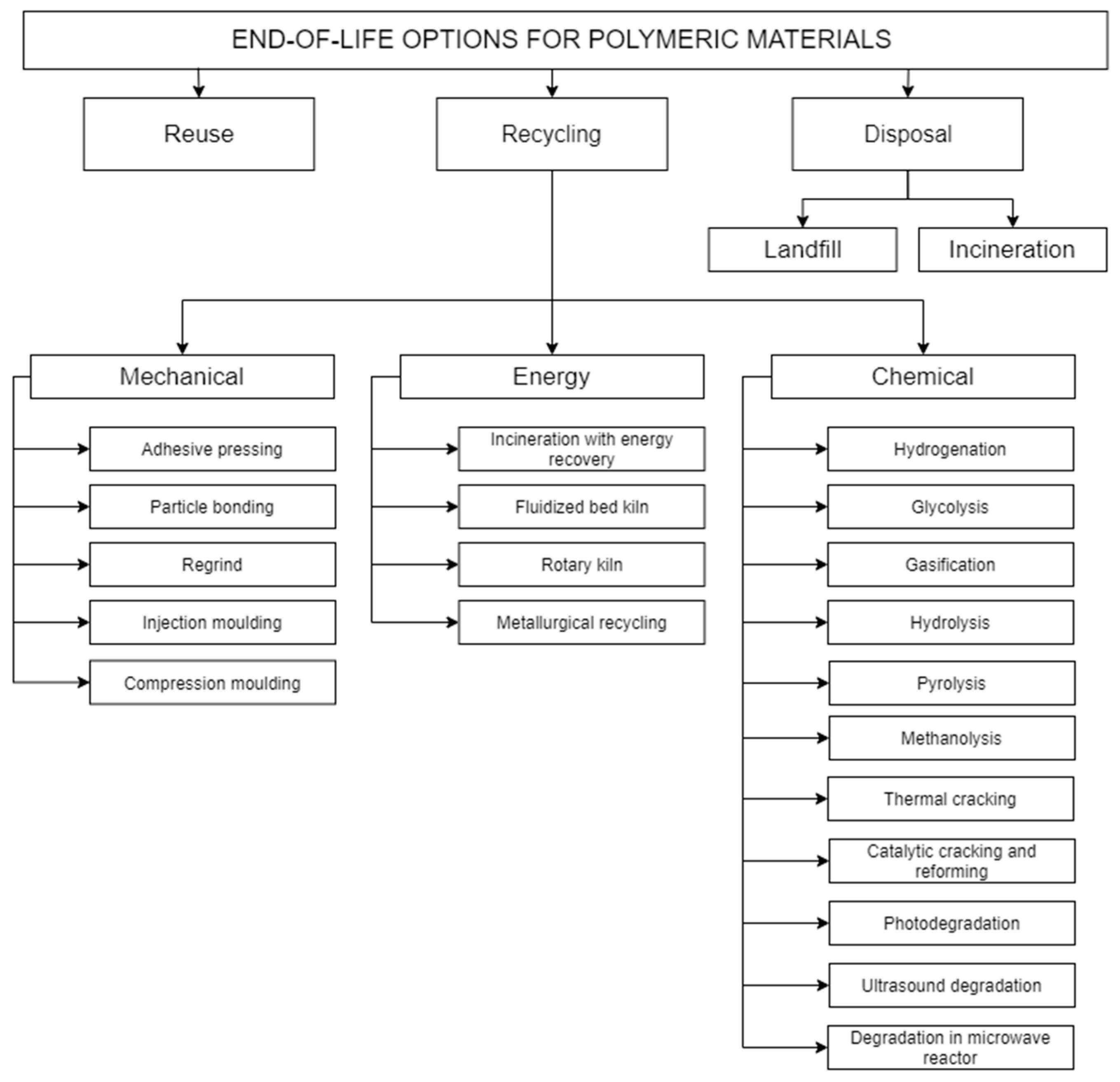
2.1. End-Of-Life Options for Contaminated Polymeric Materials
- Waste Framework Directive (Directive 2008/98/EC on waste) [29],
- Council Directive 1999/31/EC of 26 April 1999 on the landfill of waste [56],
- Directive (EU) 2018/852 Of The European Parliament and of The Council of 30 May 2018 amending Directive 94/62/EC on packaging and packaging waste (Text with EEA relevance) [57],
- Directive 2000/53/EC of the European Parliament and of the Council of 18 September 2000 on end-of-life vehicles [58],
- Directive 2012/19/EU of the European Parliament and of the Council of 4 July 2012 on waste electrical and electronic equipment (WEEE) [59].
- Reduce the impact of disturbances in polymer materials from operation, affecting the initial size of the object.
- Reduce the impact of changes in object parameters on its output size.
- Modify the static and dynamic properties of the object
2.1.1. Reuse
2.1.2. Recycling
2.1.3. Disposal
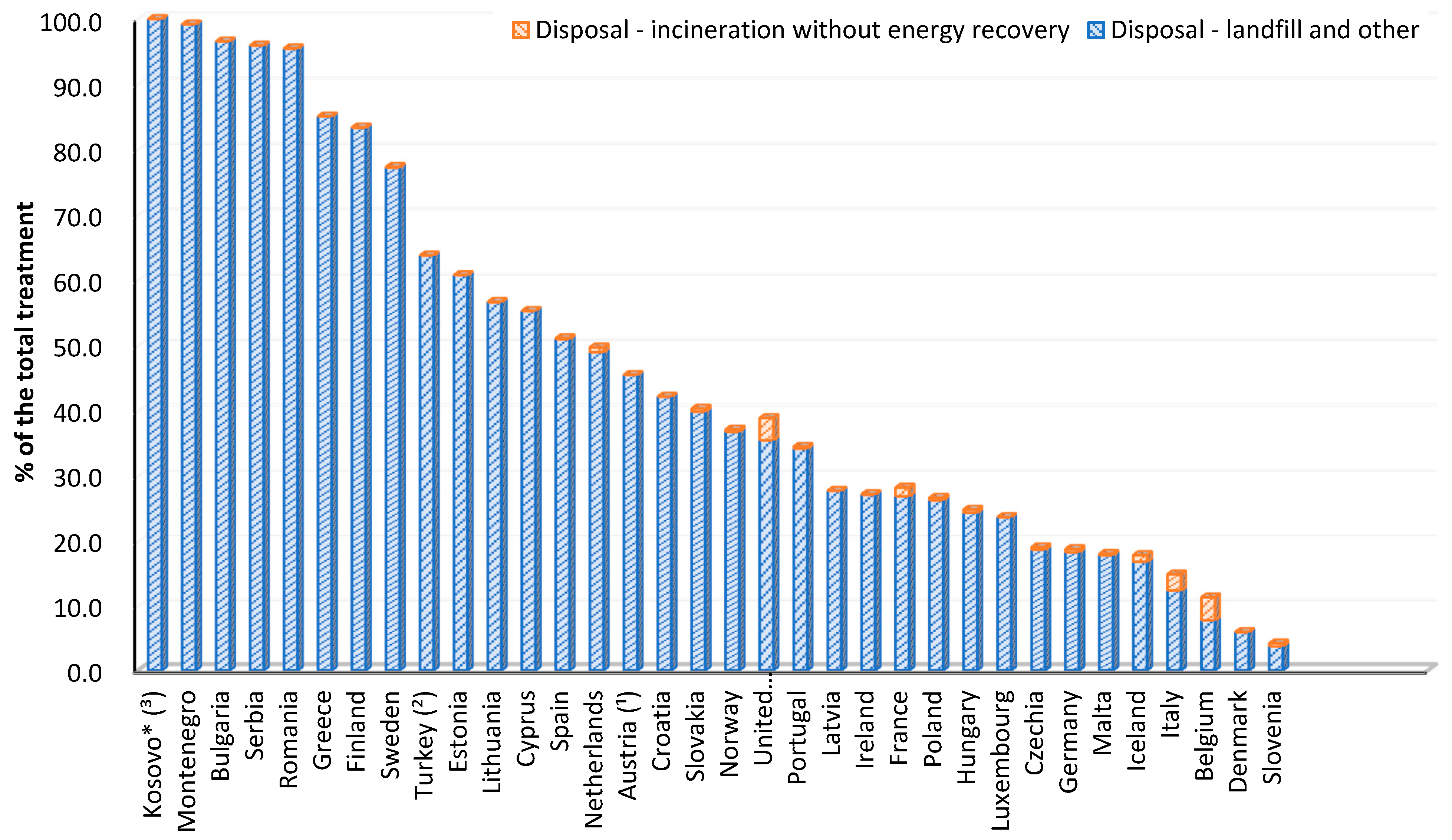
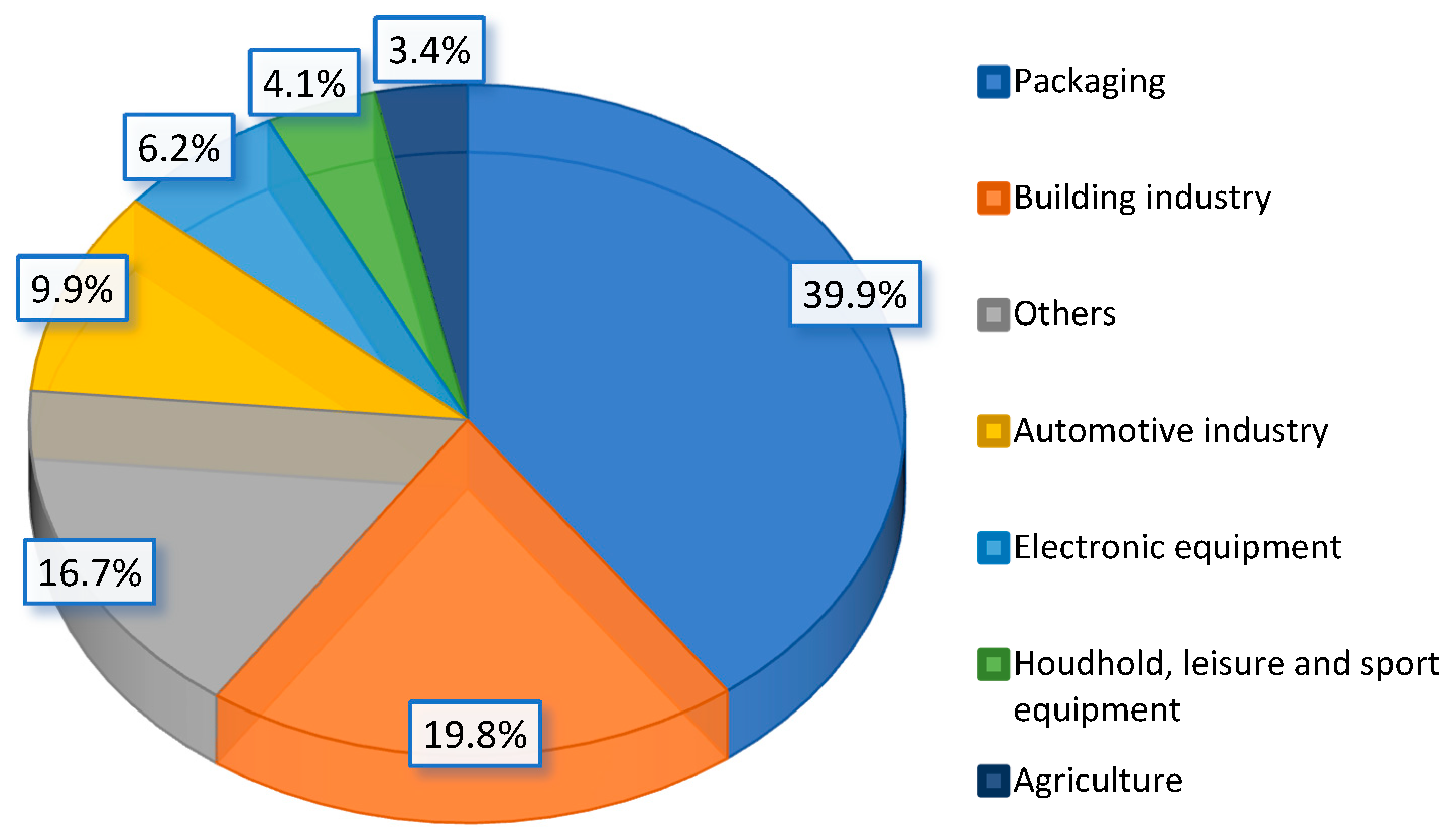
2.2. End-Of-Life Contaminated Polymer Material Potential and Scale
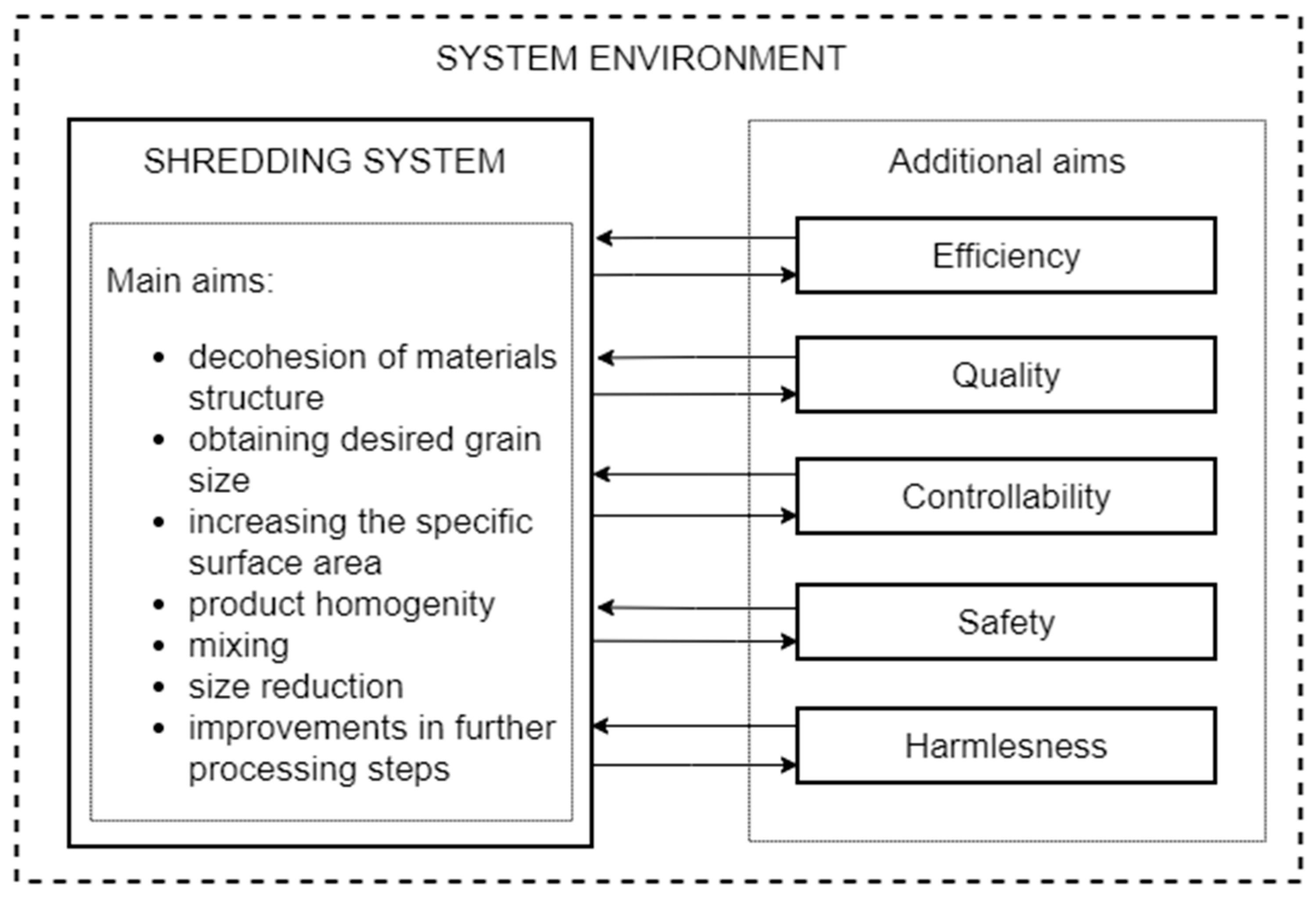
2.3. Importance of the Shredding Process in Recycling Engineering
3. Development of Shredding in Recycling
- Creative action (creation) understood as basics of shredding, creation of knowledge, resulting from system development, optimization, modernization, and innovation,
- Optimal parameters/processes/products—coming into possession of the process, machine (construction) design, or system condition taking into account the criteria enabling a rational assessment of condition,
- Modernization—intentional actions undertaken on the level of the technical system and the border zone; these actions aim to reduce the harmfulness of technology in the wider aspect considering improvement, restoration, and strengthening the environment properties.
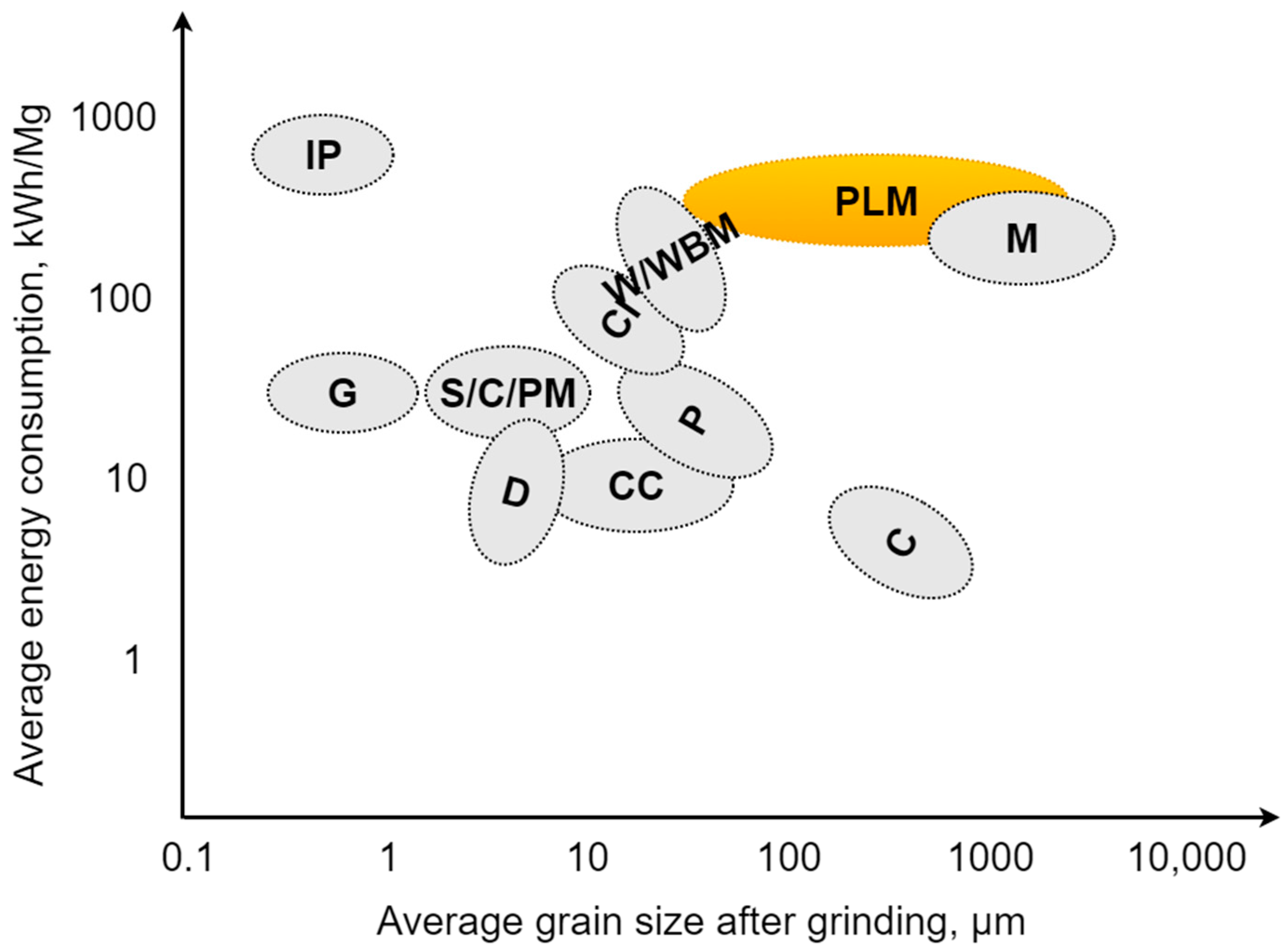
3.1. Types of Grinding
3.2. Quasi-Cutting Phenomenon
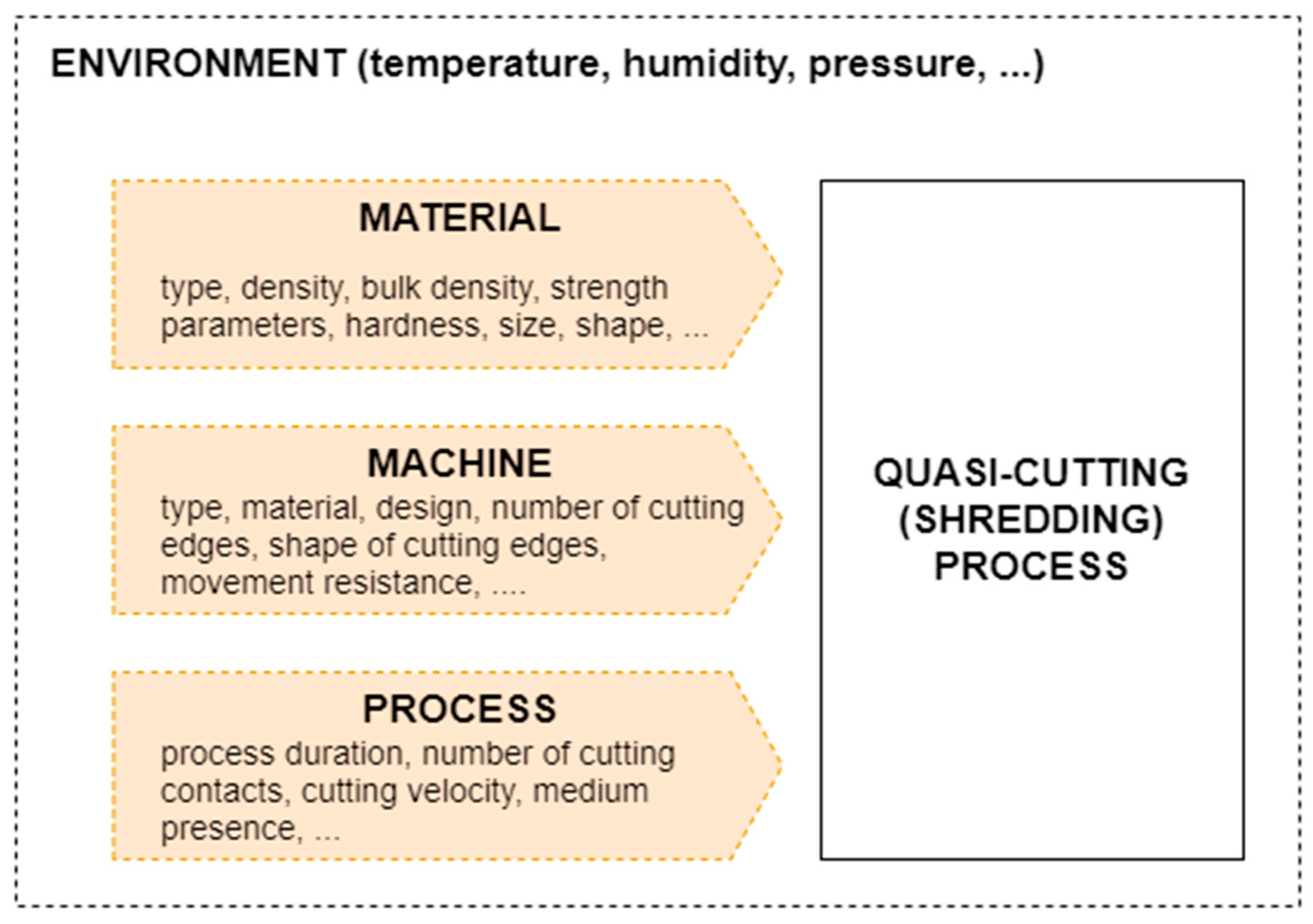
3.3. Factors Affecting the Quasi-Cutting
3.3.1. Material Factors and Disturbances d(m)
- Total energy of fracture propagation,
- Crack (cutting) stress,
- Crack (cutting) resistance,
- Load during collision and cutting,
- Collision duration,
- Performance ratio of ground product incineration,
- Relation of dimensions before and after the shredding process,
- Increase of specific surface area.
3.3.2. Factors and Disturbances d(mp) in Relation to the Grinding Machine and the Grinding Process
- The rake angle of the cutting edge on the material—it affects, inter alia, the size reduction ratio and energy consumption,
- The way of feeding the batch material to the shredding chamber (gravitational, forced)—it primarily affects the efficiency of the process; forcing the feeding speed may increase or decrease the efficiency depending on the relationship between the feeding speed and the processing capacity of the machine,
- The number of times the material is shredded and the number of contacts between the material and the cutting edges—increasing the number of contacts between the cutting edges and the material increases the particles size reduction ratio; unfortunately, it also increases energy demand,
- Geometry of the cutting edges and design of the entire cutting unit—primarily affects the efficiency and size reduction ratio,
- The material flow index for adjacent pairs of the cutting holes edges affects the efficiency; poorly selected relations of the hole sizes in the cutting discs may cause the material throttling and lower efficiency due to disturbances in the flow of particles in the shredding chamber,
- The size of the working gap between the cooperating edges primarily determines the size reduction ratio: the smaller the gap, the smaller the particles that can be obtained.
3.4. Quasi-Cutting Research of Contaminated Polymer Materials
3.5. Research in Machine (Shredder) Conditions
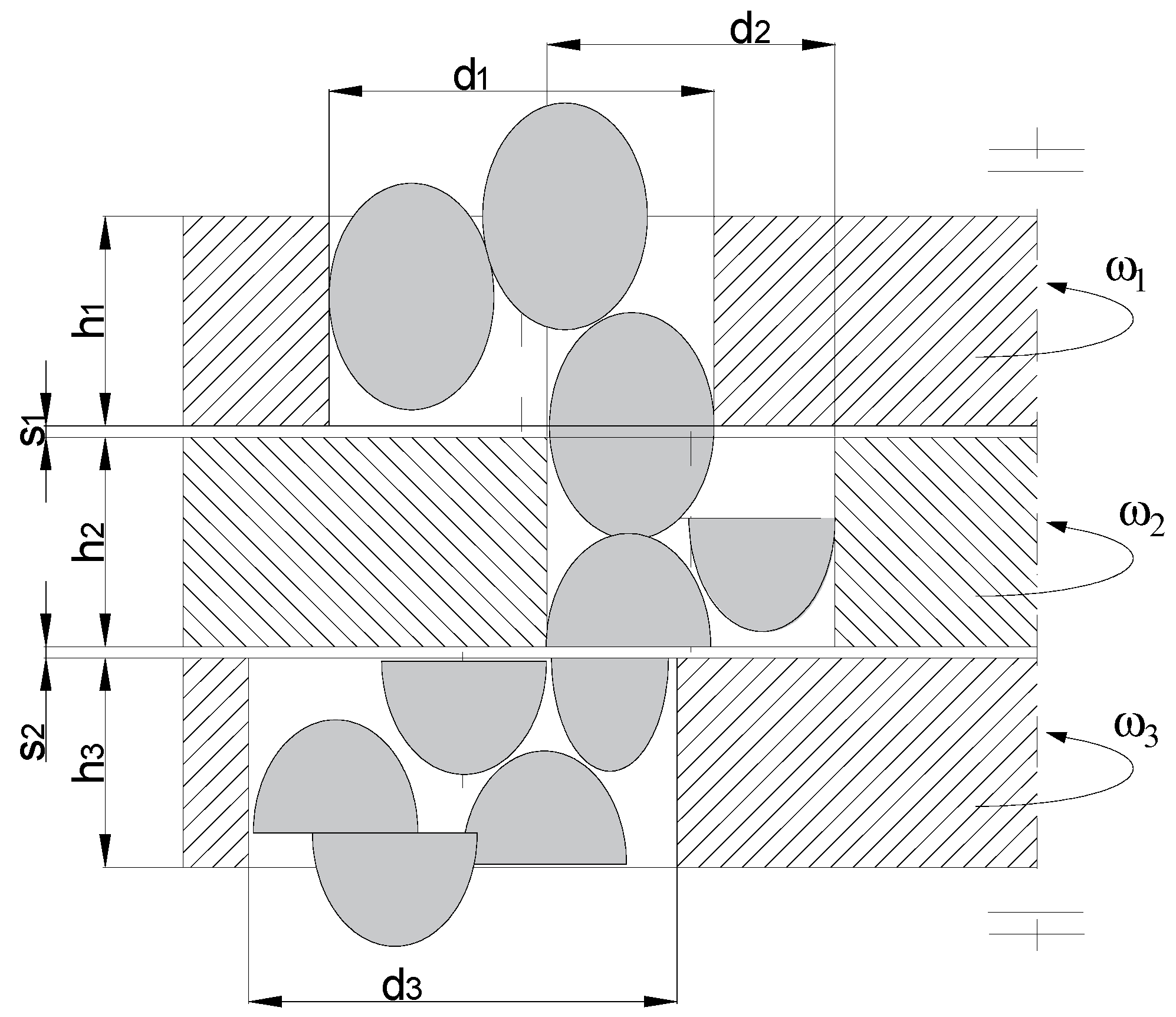
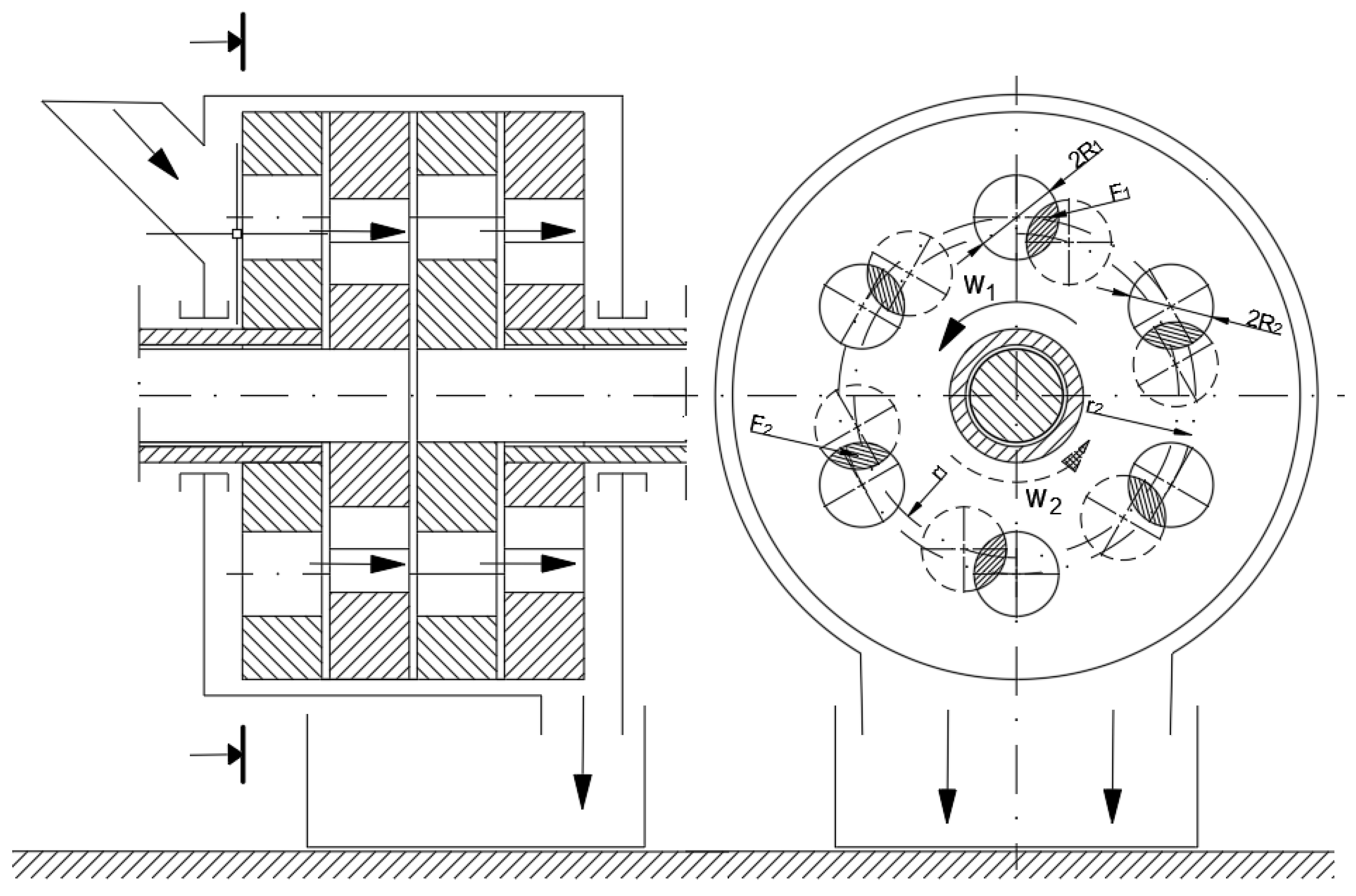
3.5.1. Quasi-Cutting Machines
3.5.2. Shredding Area
3.5.3. Quasi-Cutting Process Indicators
- Material,
- Machine,
- Process.
- Product (properties such as particle size distribution, specific surface area, bulk density, viscosity, etc.),
- Process (energy, technology, quality),
- Shredding environment (generated noise, emissions of solid, liquid and gaseous waste, generated vibrations, etc.).
- Pr—power consumed for grinding process [kW],
- Wfq—efficiency of obtaining material with desired dimensions [kg∙h−1].
- VotwT1—volume of grains introduced into the holes in the first disc, m3,
- ω1—angular velocity of the first disc, rad·s−1,
- δ—hole filling factor, –,
- t—time, s.
- Dmax—arithmetic mean of the diameters of the largest grains of the feed,
- dmax—arithmetic mean of the diameters of the largest grains of the grinding product.
4. Recycling Potential in the Context of Shredding
- Current executive possibilities, πO(t),
- Volume of operation used actively, usefully MO(t),
- Theoretical possibilities and operational needs, ε,
4.1. Material and Technical Potentials (PE(t); PT(t))
- TT—all technical potential intended for polymer materials recycling,
- MT(t)—number of machines (shredders) taking part in recycling,
- εT—theoretical technical possibilities,
- πT—real technical possibilities of machines,
- The construction with its substantial scope includes also the destruction, = 1
- The building and machine are constructed according to the construction, = 1
- Use, operation, resistance, and durability are adequate, = 1.
- EE—amount of useful energy and matter introduced into the system,
- ME(t)—material and energy resources used in the processing,
- εE—theoretical material and energy possibilities,
- πE—real, useful material and energy possibilities.
- Only renewable energy is used in processing, = 1,
- The risk of depletion of water and food resources is minimized, = 1,
- Processes are carried out in a sufficiently short time, = 1,
- Maximum security of the processing is ensured, = 1,
- Waste energy and waste (solid, liquid, gases) are minimized, = 1.
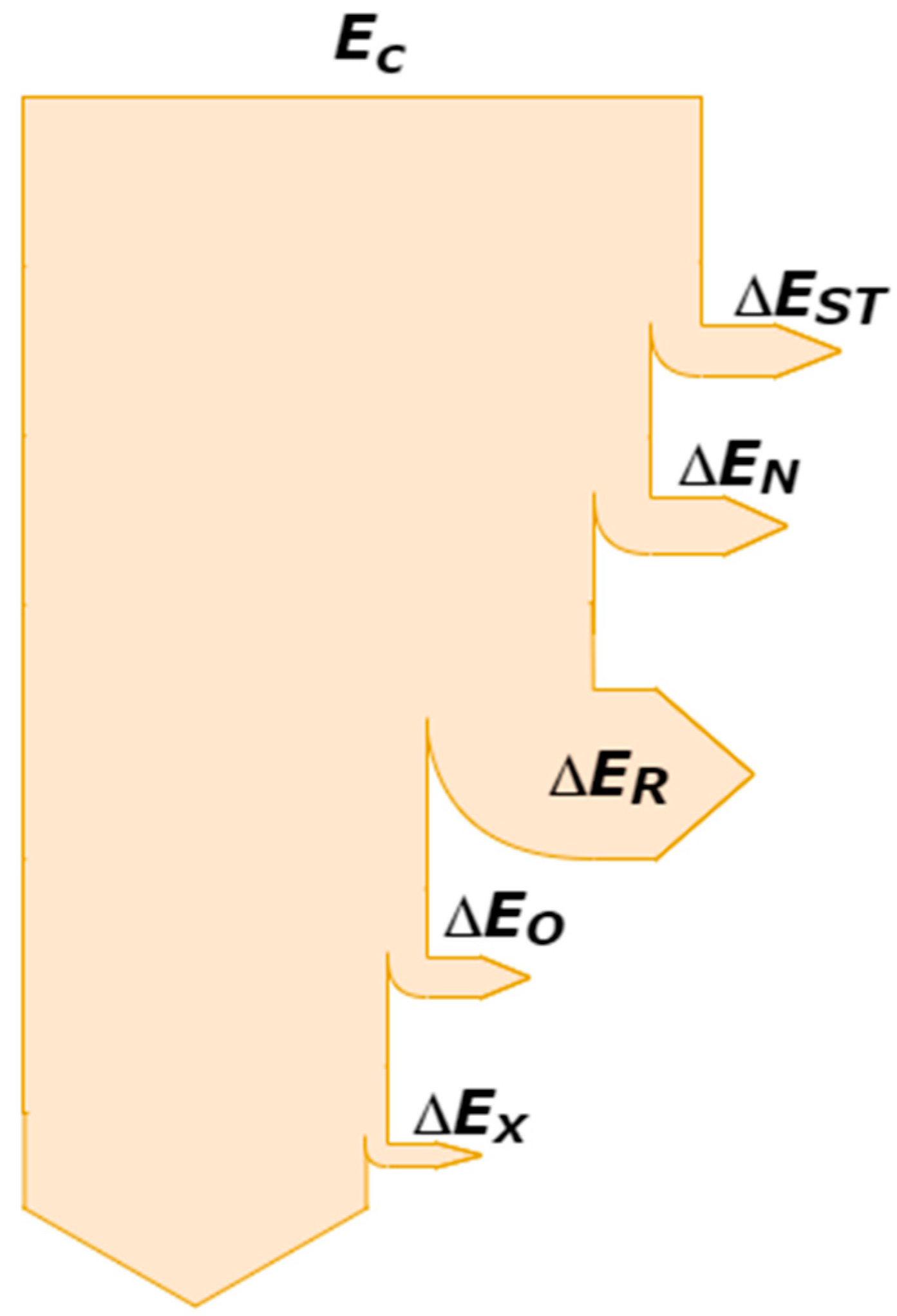
4.2. Integrated Efficiency
4.3. Control and Human Potentials (PS(t), PL(t))
- The design features of the shredding unit (for instance, the common area of the edges of two holes (Sc,ST)),
- Grain density and volume in the grinding chamber (ρm,Vg),
- Rotational, angular, and linear speed of a grinding component and time (respectively n,ω,v,Θ,ti) [148].
- SS—possible control information stream,
- MS(t)—a stream of used information,
- εS—theoretical possibilities and needs of information and decision systems,
- πS—instantaneous actual stream of control information.
- —information reaching and leaving the system ensure the implementation of autonomous, integral, and reliable operation,
- —the control system automatically neutralizes the negative effects of processing,
- —the control system is adapted to self-control and self-diagnosis within a predetermined tolerance field for effective system operation.
- LL—number of people scheduled to carry out activities in the recycling (shredding) operations,
- ML(t)—the number of people involved (taking part) in the recycling (shredding) operations,
- εL—theoretical human capabilities,
- πL—the real value of human creativity and responsibility.
- Motivation to carry out entrusted tasks, when , one can talk about the ideal state of full motivation and full human commitment in the task,
- Knowledge about the entrusted task, when —full (complete) knowledge about the task,
- Access to self-improvement channels, when —open (full) access to self-improvement channels,
- Development of the market of goods and services adequate to meet human needs, when –, the market of goods and services is able to satisfy all needs of individuals.
5. Summary and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kruszelnicka, W. A New Model for Environmental Assessment of the Comminution Process in the Chain of Biomass Energy Processing. Energies 2020, 13, 330. [Google Scholar] [CrossRef]
- Kruszelnicka, W.; Kasner, R.; Bałdowska-Witos, P.; Flizikowski, J.; Tomporowski, A. The Integrated Energy Consumption Index for Energy Biomass Grinding Technology Assessment. Energies 2020, 13, 1417. [Google Scholar] [CrossRef]
- Zając, G.; Węgrzyn, A. Analysis of Work Parameters Changes of Diesel Engine Powered with Diesel Fuel and Faee Blends. Eksploat. Niezawodn. Maint. Reliab. 2008, 17–24. [Google Scholar]
- Marczuk, A.; Misztal, W.; Savinykh, P.; Turubanov, N.; Isupov, A.; Zyryanov, D. Improving Efficiency of Horizontal Ribbon Mixer by Optimizing Its Constructional and Operational Parameters. Eksploat. Niezawodn. Maint. Reliab. 2019, 21, 220–225. [Google Scholar] [CrossRef]
- Hejft, R.; Obidziński, S. Ciśnieniowa Aglomeracja Materiałów Roślinnych-Innowacje Technologiczno-Techniczne. Część 1. J. Res. Appl. Agric. Eng. 2012, 57, 63–65. [Google Scholar]
- Ignatyev, I.A.; Thielemans, W.; Vander Beke, B. Recycling of Polymers: A Review. ChemSusChem 2014, 7, 1579–1593. [Google Scholar] [CrossRef]
- Flizikowski, J.; Piasecka, I.; Kruszelnicka, W.; Tomporowski, A.; Mroziński, A. Destruction Assessment of Wind Power Plastics Blade. Polimery 2018, 63, 381–386. [Google Scholar] [CrossRef]
- Ratner, S.; Gomonov, K.; Revinova, S.; Lazanyuk, I. Eco-Design of Energy Production Systems: The Problem of Renewable Energy Capacity Recycling. Appl. Sci. 2020, 10, 4339. [Google Scholar] [CrossRef]
- Grigore, M.E. Methods of Recycling, Properties and Applications of Recycled Thermoplastic Polymers. Recycling 2017, 2, 24. [Google Scholar] [CrossRef]
- Hamad, K.; Kaseem, M.; Deri, F. Recycling of Waste from Polymer Materials: An Overview of the Recent Works. Polym. Degrad. Stab. 2013, 98, 2801–2812. [Google Scholar] [CrossRef]
- Mannheim, V.; Fehér, Z.S.; Siménfalvi, Z. Innovative solutions for the building industry to improve sustainability performance with Life Cycle Assessment modelling. In Solutions for Sustainable Development; Taylor & Francis Group: London, UK, 2019. [Google Scholar]
- Smyth, K.; Christie, N.; Burdon, D.; Atkins, J.P.; Barnes, R.; Elliott, M. Renewables-to-Reefs?—Decommissioning Options for the Offshore Wind Power Industry. Mar. Pollut. Bull. 2015, 90, 247–258. [Google Scholar] [CrossRef] [PubMed]
- Piasecka, I.; Tomporowski, A.; Flizikowski, J.; Kruszelnicka, W.; Kasner, R.; Mroziński, A. Life Cycle Analysis of Ecological Impacts of an Offshore and a Land-Based Wind Power Plant. Appl. Sci. 2019, 9, 231. [Google Scholar] [CrossRef]
- Piasecka, I.; Bałdowska-Witos, P.; Piotrowska, K.; Tomporowski, A. Eco-Energetical Life Cycle Assessment of Materials and Components of Photovoltaic Power Plant. Energies 2020, 13, 1385. [Google Scholar] [CrossRef]
- Cherrington, R.; Goodship, V.; Meredith, J.; Wood, B.M.; Coles, S.R.; Vuillaume, A.; Feito-Boirac, A.; Spee, F.; Kirwan, K. Producer Responsibility: Defining the Incentive for Recycling Composite Wind Turbine Blades in Europe. Energy Policy 2012, 47, 13–21. [Google Scholar] [CrossRef]
- Larsen, K. Recycling Wind Turbine Blades. Renew. Energy Focus 2009, 9, 70–73. [Google Scholar] [CrossRef]
- Lunardi, M.M.; Alvarez-Gaitan, J.P.; Bilbao, J.I.; Corkish, R. A Review of Recycling Processes for Photovoltaic Modules. Sol. Panels Photovolt. Mater. 2018. [Google Scholar] [CrossRef]
- Komoto, K.; Lee, J.-S.; Zhang, J.; Ravikumar, D.; Sinha, P.; Wade, A.; Heath, G.A. End-of-Life Management of Photovoltaic Panels: Trends in PV Module Recycling Technologies; No. NREL/TP-6A20-73847; International Energy Agency: USA, 2018; p. 1561523. [Google Scholar]
- Navajas, A.; Uriarte, L.; Gandía, L.M. Application of Eco-Design and Life Cycle Assessment Standards for Environmental Impact Reduction of an Industrial Product. Sustainability 2017, 9, 1724. [Google Scholar] [CrossRef]
- Lewandowska, A.; Matuszak-Flejszman, A. Eco-Design as a Normative Element of Environmental Management Systems—the Context of the Revised ISO 14001:2015. Int. J. Life Cycle Assess. 2014, 19, 1794–1798. [Google Scholar] [CrossRef]
- Bałdowska-Witos, P.; Kruszelnicka, W.; Kasner, R.; Tomporowski, A.; Flizikowski, J.; Kłos, Z.; Piotrowska, K.; Markowska, K. Application of LCA Method for Assessment of Environmental Impacts of a Polylactide (PLA) Bottle Shaping. Polymers 2020, 12, 388. [Google Scholar] [CrossRef]
- Piotrowska, K.; Kruszelnicka, W.; Bałdowska-Witos, P.; Kasner, R.; Rudnicki, J.; Tomporowski, A.; Flizikowski, J.; Opielak, M. Assessment of the Environmental Impact of a Car Tire throughout Its Lifecycle Using the LCA Method. Materials 2019, 12, 4177. [Google Scholar] [CrossRef] [PubMed]
- Kasner, R.; Kruszelnicka, W.; Bałdowska-Witos, P.; Flizikowski, J.; Tomporowski, A. Sustainable Wind Power Plant Modernization. Energies 2020, 13, 1461. [Google Scholar] [CrossRef]
- Beauson, J.; Bech, J.I.; Brøndsted, P. Composite Recycling: Characterizing End of Life Wind Turbine Blade Material. In Proceedings of the 19th International Conference on Composite Materials (ICCM 2013), Montreal, QC, Canada, 28 July–2 August 2013. [Google Scholar]
- Tomporowski, A.; Flizikowski, J.; Wełnowski, J.; Najzarek, Z.; Topoliński, T.; Kruszelnicka, W.; Piasecka, I.; Śmigiel, S. Regeneration of Rubber Waste Using an Intelligent Grinding System. Przem. Chem. 2018, 97, 1659–1665. [Google Scholar] [CrossRef]
- Hocine, L.; Mounia Samira, K. Optimal PV Panel’s End-Life Assessment Based on the Supervision of Their Own Aging Evolution and Waste Management Forecasting. Sol. Energy 2019, 191, 227–234. [Google Scholar] [CrossRef]
- Aoudia, K.; Azem, S.; Aït Hocine, N.; Gratton, M.; Pettarin, V.; Seghar, S. Recycling of Waste Tire Rubber: Microwave Devulcanization and Incorporation in a Thermoset Resin. Waste Manag. 2017, 60, 471–481. [Google Scholar] [CrossRef] [PubMed]
- Chowdhury, M.S.; Rahman, K.S.; Chowdhury, T.; Nuthammachot, N.; Techato, K.; Akhtaruzzaman, M.; Tiong, S.K.; Sopian, K.; Amin, N. An Overview of Solar Photovoltaic Panels’ End-of-Life Material Recycling. Energy Strategy Rev. 2020, 27, 100431. [Google Scholar] [CrossRef]
- Directive 2008/98/EC on Waste (Waste Framework Directive)—Environment—European Commission. Available online: https://ec.europa.eu/environment/waste/framework/ (accessed on 29 July 2020).
- Malinauskaite, J.; Jouhara, H.; Spencer, N. Waste Prevention and Technologies. Eur. Energy Environ. Law Rev. 2017, 26, 66–80. [Google Scholar]
- Hopewell, J.; Dvorak, R.; Kosior, E. Plastics Recycling: Challenges and Opportunities. Philos. Trans. R. Soc. B Biol. Sci. 2009, 364, 2115–2126. [Google Scholar] [CrossRef]
- Kruszelnicka, W.; Baldowska-Witos, P.; Kasner, R.; Flizikowski, J.; Tomporowski, A.; Rudnicki, J. Evaluation of emissivity and environmental safety of biomass grinders drive. Przemysl Chem. 2019, 98, 1494–1498. [Google Scholar] [CrossRef]
- Flizikowski, J.; Tomporowski, A.; Kasner, R.; Mroziński, A.; Kruszelnicka, W. Machinery Life Cycle Efficiency Models for Their Sustainable Development. Syst. Saf. Hum. Tech. Facil. Environ. 2019, 1, 363–370. [Google Scholar] [CrossRef]
- Mannheim, V. Empirical and Scale-up Modeling in Stirred Ball Mills. Chem. Eng. Res. Des. 2011, 89, 405–409. [Google Scholar] [CrossRef]
- Flizikowski, J.B.; Mrozinski, A.; Tomporowski, A. Active Monitoring as Cognitive Control of Grinders Design. AIP Conf. Proc. 2017, 1822, 20006. [Google Scholar]
- Macko, M. Size reduction by grinding as an important stage in recycling. In Post-Consumer Waste Recycling and Optimal Production; IntechOpen Limited: London, UK, 2012; pp. 273–294. [Google Scholar]
- Macko, M.; Tyszczuk, K.; Śmigielski, G.; Flizikowski, J.; Mroziński, A. The Use of CAD Applications in the Design of Shredders for Polymers. MATEC Web Conf. 2018, 157, 2027. [Google Scholar] [CrossRef][Green Version]
- Benabderrahmane, A.; Zeghloul, T.; Aksa, W.; Tilmatine, A.; Medles, K.; Dascalescu, L. Shredding as Simultaneous Size-Reduction and Tribo-Charging Operation for Improved Performances of an Electrostatic Separation Process for Granular Plastic Wastes. Part. Sci. Technol. 2019, 1–8. [Google Scholar] [CrossRef]
- Głogowska, K.; Rozpędowski, J. Examination of Shredding Process Parameters and the Properties of Recyclate. Adv. Sci. Technol. Res. J. 2016, 10, 176–179. [Google Scholar] [CrossRef]
- Bochat, A.; Bogusz, M.; Kalwaj, J. Kierunki Rozowju Rozdrabniaczy Bijakowych Ziarna Zbóż; Politechnika Białostocka: Białystok, Poland, 1998. [Google Scholar]
- Zastempowski, M.; Bochat, A. Innovative Constructions of Cutting and Grinding Assemblies of Agricultural Machinery; Choteborsky, R., Kovar, S., Krepcik, V., Herak, D., Eds.; Czech University Life Sciences Prague: Prague, Czech Republic, 2016; pp. 726–735. ISBN 978-80-213-2683-5. [Google Scholar]
- Zastempowski, M.; Bochat, A. Modeling of Cutting Process by the Shear-Finger Cutting Block. Appl. Eng. Agric. 2014, 30, 347–353. [Google Scholar]
- Bembenek, M. Badania i Perspektywy Nowych Obszarów Stosowania Pras Walcowych. Przem. Chem. 2017, 96, 1845–1847. [Google Scholar] [CrossRef]
- Bembenek, M.; Wdaniec, P. Wpływ Rodzaju Kruszarki Oraz Parametrów Jej Pracy Na Suchą Granulację Proszków. Przem. Chem. 2019, 98, 310–313. [Google Scholar] [CrossRef]
- Flizikowski, J. Rozdrabnianie Tworzyw Sztucznych; Wydawnictwa Akademii Techniczno-Rolniczej: Bydgoszcz, Poland, 1998. [Google Scholar]
- Tomporowski, A. Rozdrabnianie Alternatywnych Nośników Energii; Wydawnictwa Uczelniane Uniwersytetu Technologiczno-Przyrodniczego w Bydgoszczy: Bydgoszcz, Poland, 2018. [Google Scholar]
- Bieliński, M.; Flizikowski, J. Rozdrabnianie Materiałów Pokonstrukcyjnych z Tworzyw Polimerowych. In Proceedings of the II Środkowo-Europejska Konferencja: Recykling Materiałów Polimerowych Nauka-Przemysł, Toruń, Poland, 12–14 November 2003; Wydawnictwo UMK: Toruń, Poland, 2003; p. 5. [Google Scholar]
- Bösch, D. Comminution—An Essential Component of Waste Treatment; TK Verlag Karl Thomé-Kozmiensky: Neuruppin, Germany, 2011. [Google Scholar]
- Macko, M.; Mroziński, A.; Flizikowski, J. Design and Utility of Specialist Comminution Set-Up for Plastics and Organic Materials. In Proceedings of the ASME 2011 International Mechanical Engineering Congress and Exposition, Denver, CO, USA, 11–17 November 2011; pp. 397–402. [Google Scholar]
- Flizikowski, J. Comminution of plastics in the recycling process. Polimery 1999, 44, 397–402. [Google Scholar] [CrossRef]
- Macko, M.; Flizikowski, J. The Method of the Selection of Comminution Design for Non-Brittle Materials | AIChE. In Proceedings of the 2010 AIChE Annual Meeting, Salt Lake City, UT, USA, 7–12 November 2010; p. 392e. [Google Scholar]
- Thi, B.N.V.; Vu-Khanh, T.; Lara, J. Effect of Friction on Cut Resistance of Polymers. J. Thermoplast. Compos. Mater. 2005, 18, 23–35. [Google Scholar] [CrossRef]
- Nowosielski, R.; Kania, A.; Spilka, M. Recycling as an Important Element of Engineering Design. J. Achiev. Mater. Manuf. Eng. 2010, 42, 8. [Google Scholar]
- Huisman, J.; Stevels, A.; Baldé, K.; Magalini, F.; Kuehr, R. Chapter 5—Implementation road map and conditions for success. In Waste Electrical and Electronic Equipment (WEEE) Handbook, 2nd ed.; Goodship, V., Stevels, A., Huisman, J., Eds.; Woodhead Publishing Series in Electronic and Optical Materials; Woodhead Publishing: Sawston, Cambridge, UK, 2019; pp. 143–184. ISBN 978-0-08-102158-3. [Google Scholar]
- Petrakli, F.; Gkika, A.; Bonou, A.; Karayannis, P.; Koumoulos, E.P.; Semitekolos, D.; Trompeta, A.-F.; Rocha, N.; Santos, R.M.; Simmonds, G.; et al. End-of-Life Recycling Options of (Nano)Enhanced CFRP Composite Prototypes Waste—A Life Cycle Perspective. Polymers 2020, 12, 2129. [Google Scholar] [CrossRef] [PubMed]
- Council Directive 1999/31/EC of 26 April 1999 on the Landfill of Waste; The Council of The European Union, Publication Office of the European Union: Luxemburg, 1999.
- Directive (EU) 2018/852 of the European Parliament and of the Council of 30 May 2018 Amending Directive 94/62/EC on Packaging and Packaging Waste (Text with EEA Relevance); 14.6.2018, L150; Official Journal of the European Union: Luxemburg, 2018; pp. 109–140.
- EUR-Lex—32000L0053—EN—EUR-Lex. Available online: https://eur-lex.europa.eu/legal-content/EN/ALL/?uri=celex%3A32000L0053 (accessed on 9 November 2020).
- Directive 2012/19/EU of the European Parliament and of the Council of 4 July 2012 on Waste Electrical and Electronic Equipment (WEEE) Text with EEA Relevance; 24.7.2012, L.197; Official Journal of the European Union: Luxemburg, 2012; pp. 38–71.
- Maris, E.; Froelich, D.; Aoussat, A.; Naffrechoux, E. Chapter 27—From Recycling to Eco-design. In Handbook of Recycling; Worrell, E., Reuter, M.A., Eds.; Elsevier: Boston, MA, USA, 2014; pp. 421–427. ISBN 978-0-12-396459-5. [Google Scholar]
- Pires, A.; Martinho, G.; Rodrigues, S.; Gomes, M.I. Prevention and Reuse: Waste Hierarchy Steps Before Waste Collection. In Sustainable Solid Waste Collection and Management; Pires, A., Martinho, G., Rodrigues, S., Gomes, M.I., Eds.; Springer International Publishing: Cham, Switzerland, 2019; pp. 13–23. ISBN 978-3-319-93200-2. [Google Scholar]
- Pires, A.; Martinho, G.; Rodrigues, S.; Gomes, M.I. Sustainable Solid Waste Collection and Management; Springer International Publishing: Cham, Switzerland, 2019; ISBN 978-3-319-93199-9. [Google Scholar]
- Chandrappa, R.; Brown, J. Solid Waste Management: Principles and Practice; Environmental Science; Springer: Berlin/Heidelberg, Germany, 2012; ISBN 978-3-642-28680-3. [Google Scholar]
- Schyns, Z.O.G.; Shaver, M.P. Mechanical Recycling of Packaging Plastics: A Review. Macromol. Rapid Commun. 2021, 42, 2000415. [Google Scholar] [CrossRef] [PubMed]
- Solis, M.; Silveira, S. Technologies for Chemical Recycling of Household Plastics—A Technical Review and TRL Assessment. Waste Manag. 2020, 105, 128–138. [Google Scholar] [CrossRef] [PubMed]
- Payne, J.; McKeown, P.; Jones, M.D. A Circular Economy Approach to Plastic Waste. Polym. Degrad. Stab. 2019, 165, 170–181. [Google Scholar] [CrossRef]
- Ludwig, C.; Hellweg, S.; Stucki, S. (Eds.) Municipal Solid Waste Management: Strategies and Technologies for Sustainable Solutions; Springer: Berlin/Heidelberg, Germany, 2003; ISBN 978-3-642-62898-6. [Google Scholar]
- McCarthy, B.E.C. Developments in the End-of-Life Management of Plastics; IIIEE, Lund University: Lund, Sweden, 2005. [Google Scholar]
- IJgosse, J. Waste Incineration and Informal Livelihoods: A Technical Guide on Waste-to-Energy Initiatives; WIEGO Technical Brief No. 11; WIEGO: Manchester, UK, 2019. [Google Scholar]
- Flizikowski, J.B. Intelligent Grinding System. Inż. Ap. Chem. 2011, 50, 22–23. [Google Scholar]
- Flizikowski, J. Levels of Intelligent Grinding System. Inż. Apar. Chem. 2011, 50, 24–26. [Google Scholar]
- Kruszelnicka, W.; Flizikowski, J.; Tomporowski, A. Auto-Monitoring System of Grainy Biomass Comminution Technology. IOP Conf. Ser. Mater. Sci. Eng. 2018, 393, 12076. [Google Scholar] [CrossRef]
- Ragaert, K.; Delva, L.; Van Geem, K. Mechanical and Chemical Recycling of Solid Plastic Waste. Waste Manag. 2017, 69, 24–58. [Google Scholar] [CrossRef]
- D20 Committee. Guide for Waste Reduction, Resource Recovery, and Use of Recycled Polymeric Materials and Products; ASTM International: West Conshohocken, PA, USA, 2006. [Google Scholar]
- Chemical Recycling | Plastics Recyclers Europe. Available online: https://www.plasticsrecyclers.eu/chemical-recycling (accessed on 9 November 2020).
- Devasahayam, S.; Bhaskar Raju, G.; Mustansar Hussain, C. Utilization and Recycling of End of Life Plastics for Sustainable and Clean Industrial Processes Including the Iron and Steel Industry. Mater. Sci. Energy Technol. 2019, 2, 634–646. [Google Scholar] [CrossRef]
- Waste Statistics—Statistics Explained. Available online: https://ec.europa.eu/eurostat/statistics-explained/index.php/Waste_statistics#Waste_treatment (accessed on 15 November 2020).
- PlasticsEurope. Plastics—The Facts 2019. An Analysis of European Plastics Production, Demand and Waste Data; PlasticsEurope: Wemmel, Belgium, 2019. [Google Scholar]
- Saldívar-Guerra, E.; Vivaldo-Lima, E. (Eds.) Handbook of Polymer Synthesis, Characterization, and Processing: Saldívar-Guerra/Handbook of Polymer Synthesis, Characterization, and Processing; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2013; ISBN 978-1-118-48079-3. [Google Scholar]
- Żuchowska, D. Polimery Konstrukcyjne; WNT: Warsaw, Poland, 2000.
- Worrell, E.; Reuter, M. (Eds.) Handbook of Recycling; Elsevier: Amsterdam, The Netherlands, 2014; ISBN 978-0-12-396459-5. [Google Scholar]
- PlasticsEurope AISBL. The Circular Economy for Plastics. A European Overview; PlasticsEurope AISBL: Brussels, Belgium, 2019. [Google Scholar]
- P2. Recyclability of Products—OECD. Available online: https://www.oecd.org/innovation/green/toolkit/p2recyclabilityofproducts.htm (accessed on 16 September 2020).
- WRAP. Recyclability Efficiency Metric; Banbury: Oxon, UK, 2008. [Google Scholar]
- I3. Recycled/Reused Content of Non-Energy Material Inputs—OECD. Available online: https://www.oecd.org/innovation/green/toolkit/i3recycledreusedcontentofnon-energymaterialinputs.htm (accessed on 17 September 2020).
- Flizikowski, J.; Bieliński, M. Ekologiczna Niezawodnośc Potencjałów Rozdrabniania; Wydawnictwo Uczelniane Akademii Techniczno-Rolniczej w Bydgoszczy: Bydgoszcz, Poland, 1994. [Google Scholar]
- Godlewska, J. Recovery and Recycling of Waste Tires in Poland. Procedia Eng. 2017, 182, 229–234. [Google Scholar] [CrossRef]
- Piasecka, I.; Tomporowski, A.; Piotrowska, K. Environmental analysis of post-use management of car tires. Przemysl. Chem. 2018, 97, 1649–1653. [Google Scholar] [CrossRef]
- Ramos, G.; Alguacil, F.J.; López, F.A. The Recycling of End-of-Life Tyres. Technological Review. Rev. Metal. 2011, 47, 273–284. [Google Scholar] [CrossRef]
- Piasecka, I.; Bałdowska-Witos, P.; Flizikowski, J.; Piotrowska, K.; Tomporowski, A. Control the System and Environment of Post-Production Wind Turbine Blade Waste Using Life Cycle Models. Part 1. Environmental Transformation Models. Polymers 2020, 12, 1828. [Google Scholar] [CrossRef]
- Chen, J.; Wang, J.; Ni, A. Recycling and Reuse of Composite Materials for Wind Turbine Blades: An Overview. J. Reinf. Plast. Compos. 2019, 38, 567–577. [Google Scholar] [CrossRef]
- The Plastics Industry Trade Association. Automotive Recycling. Devalued Is Now Revalued; SPI, The Plastics Industry Trade Association: Washington, DC, USA, 2016.
- Schönmayr, D. Automotive Recycling, Plastics, and Sustainability; Springer International Publishing: Cham, Switzerland, 2017; ISBN 978-3-319-57399-1. [Google Scholar]
- Stagner, J.A.; Sagan, B.; Tam, E.K. Using Sieving and Pretreatment to Separate Plastics during End-of-Life Vehicle Recycling. Waste Manag. Res. 2013. [Google Scholar] [CrossRef] [PubMed]
- Johnson, N.W. Review of Existing Eco-Efficient Comminution Devices. In The Coalition for Eco-Efficient Comminution (CEEC) Review Paper; CEEC International Ltd.: Melbourne, Australia, 2006. [Google Scholar]
- Salman, A.D.; Ghadiri, M.; Hounslow, M. Particle Breakage; Elsevier: Amsterdam, The Netherlands, 2007; ISBN 978-0-08-055346-7. [Google Scholar]
- Eisenlauer, M.; Teipel, U. Comminution of Biogenic Materials. Can. J. Chem. Eng. 2017, 95, 1236–1244. [Google Scholar] [CrossRef]
- Flizikowski, J.; Bielinski, K. Technology and Energy Sources Monitoring: Control, Efficiency, and Optimization; IGI Global: Hershey, PA, USA, 2012; ISBN 978-1-4666-2664-5. [Google Scholar]
- Brożek, M.; Naziemiec, Z. Analiza mechaniki procesu rozdrabniania surowców mineralnych w kruszarkach i wysokociśnieniowych prasach walcowych. Gospod. Surowcami Miner. 2012, 28, 139–153. [Google Scholar]
- Pahl, M.H.; Brundiek, H. (Eds.) Zerkleinerungstechnik; Praxiswissen Verfahrenstechnik Mechanische Verfahrenstechnik, 2. Aufl.; Fachbuchverl. [u.a.]: Leipzig, Germany, 1993; ISBN 978-3-88585-682-5. [Google Scholar]
- Rumpf, H. Die Einzelkornzerkleinerung als Grundlage einer technischen Zerkleinerungswissenschaft. Chem. Ing. Tech. 1965, 37, 187–202. [Google Scholar] [CrossRef]
- Koch, R.; Naworyta, A. Procesy Mechaniczne w Inżynierii Chemicznej; WNT: Warszawa, Poland, 1998; ISBN 83-204-2262-0.
- Macko, M.; Flizikowski, J. Podatność energetyczna poużytkowych tworzyw polimerowych. Czas. Tech. Mech. 2009, 106, 205–209. [Google Scholar]
- Tomporowski, A.; Flizikowski, J.; Kasner, R.; Kruszelnicka, W.; Bałdowska-Witos, P. Pat.235318: Grinder for Spatial Products from Polymer Plastics; 08/2020 WUP; The Patent Office of the Republic of Poland: Warsaw, Poland, 2020. [Google Scholar]
- Kwiatkowski, S.P.; Zawiślak, K.; Sobczak, P. Ocena Procesu Rozdrabniania Ziarna Jęczmienia Rozdrabniaczami Bijakowymi. Acta Sci. Pol. Tech. Agrar. 2012, 11, 65–72. [Google Scholar]
- Kruszelnicka, W. Analiza Procesu Wielotarczowego Rozdrabniania Biomasy w Ujęciu Energochlonności i Emisji CO2. Rozprawa Doktorska; Uniwersytet Technologiczno-Przyrodniczy im. J. J. Śniadeckich w Bydgoszczy: Bydgoszcz, Poland, 2019. [Google Scholar]
- Flizikowski, J.; Macko, M.; Mroziński, A. Implementacje Mechatroniczne w Rozdrabniaczu do Materiałów Nie-Kruchych. Available online: https://www.logistyka.net.pl/bank-wiedzy/item/83330-implementacje-mechatroniczne-w-rozdrabniaczu-do-materialow-nie-kruchych (accessed on 15 November 2020).
- Woldt, D.; Schubert, G.; Jäckel, H.-G. Size Reduction by Means of Low-Speed Rotary Shears. Int. J. Miner. Process. 2004, 74, S405–S415. [Google Scholar] [CrossRef]
- Bieliński, M. Materiałowa i Przetwórcza Charakterystyka Wybranych Termoplastycznych Tworzyw Wtórnych; Wydaw. Uczelniane Akademii Techniczno-Rolniczej: Bydgoszcz, Poland, 1998. [Google Scholar]
- Bauer, W. Untersuchung des Einzelzerkleinerungsvorganges in Schneidmühlen am Beispiel von Polypropylen; VDI-Verlag: Düsseldorf, Germany, 2001; ISBN 978-3-18-369403-7. [Google Scholar]
- Sadkiewicz, J.; Flizikowski, J. Konstrukcyjne Podwyższanie Wydajności Wielotarczowych Rozdrabniaczy Ziaren Zbóż. Inż. Przetwórstwa Spoż. 2014, 3, 20–24. [Google Scholar]
- Al-Zubiedy, A.A.A. Rozdrabniacze Wielotarczowe: Badania i Stabilizacja Produktu; Wydawnictwo Politechniki Częstochowskiej: Częstochowa, Poland, 2006. [Google Scholar]
- Adamski, M.; Wtorek, K.; Adamczak, W.; Mioduszewska, N.; Osuch, E.; Osuch, A.; Dworecki, Z.; Niedbała, G.; Szaferski, W.; Mitkowski, P. Analiza możliwości zastosowania rozdrabniacza wielotarczowego do przetwarzania ubocznych produktów z produkcji roślinnej. In Aktualne Problemy Inżynierii Biosystemów; Uniwersytet Przyrodniczy w Poznaniu: Poznań, Poland, 2016; pp. 241–258. ISBN 978-83-7160-827-8. [Google Scholar]
- Tomporowski, A. Studium Efektywności Napędu i Rozwiązań Innowacyjnych Konstrukcji Wielotarczowych Rozdrabniaczy Ziaren Biomasy; Lubelskie Towarzystwo Naukowe: Lublin, Poland, 2011. [Google Scholar]
- Baláž, P. High-energy milling. In Mechanochemistry in Nanoscience and Minerals Engineering; Springer: Berlin/Heidelberg, Germany, 2008; pp. 103–132. [Google Scholar]
- Zhu, J.Y.; Wang, G.S.; Pan, X.J.; Gleisner, R. Specific Surface to Evaluate the Efficiencies of Milling and Pretreatment of Wood for Enzymatic Saccharification. Chem. Eng. Sci. 2009, 64, 474–485. [Google Scholar] [CrossRef]
- Ghorbani, Z.; Masoumi, A.A.; Hemmat, A.; Seifi, M.R. Prediction of Specific Energy Consumption in Milling Process Using Some Physical and Mechanical Properties of Lfalfa Grind. Aust. J. Crop Sci. 2013, 7, 1449. [Google Scholar]
- Goyal, S.K.; Jogdand, S.V.; Agrawal, A.K. Energy Use Pattern in Rice Milling Industries—A Critical Appraisal. J. Food Sci. Technol. 2014, 51, 2907–2916. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Nnaemeka, N.; MacManus, N. Measurement of Energy Requirements for Size Reduction of Palm Kernel and Groundnut Shells for Downstream Bioenergy Generation. J. Eng. Technol. Res. 2016, 8, 47–57. [Google Scholar] [CrossRef][Green Version]
- Bieliński, M. Techniki Porowania Tworzyw Termoplastycznych; Wydawnictwa Uczelniane Akademii Techniczno-Rolniczej: Bydgoszcz, Poland, 2004; ISBN 978-83-89334-86-2. [Google Scholar]
- Wandrasz, J.; Hryb, W. Badanie wpływu charakterystyki odpadów na efektywność pracy młyna nożowego. Arch. Gospod. Odpad. Ochr. Śr. 2009, 11, 55–62. [Google Scholar]
- Giętka, T.; Wojciechowska, E.; Bieliński, M. Badanie Charakterystyk Materiałów Polimerowych. Inż. Apar. Chem. 2005, Nr 1–2, 50–51. [Google Scholar]
- Bieliński, M.; Flizikowski, J. Kształtowanie cech geometrycznych granulatu tworzyw polimerowych. In Przetwórstwo Materiałów Polimerowych; Wydawnictwo Politechniki Częstochowskiej: Częstochowa, Poland, 2008; pp. 23–28. [Google Scholar]
- Pepliński, K.; Bieliński, M. Właściwości Przetwórcze i Użytkowe Pojemników Wytwarzanych w Procesie Wytłaczania z Rozdmuchiwaniem w Zmiennych Warunkach Przetwórstwa—Ocena Wydajności i Jakości Procesu. Polimery 2009, 54, 448–456. [Google Scholar]
- Flizikowski, J.; Bieliński, K.; Nowicki, M. Research and Development of Plastic Wastes Grinding in Recycling. Inż. Ap. Chem. 2012, 51, 216–218. [Google Scholar]
- Balani, K.; Verma, V.; Agarwal, A.; Naryan, R. Physical, Thermal, and Mechanical Properties of Polymers. In Biosurfaces: A Materials Science and Engineering Perspective; John Wiley & Sons, Ltd.: Hoboken, NJ, USA, 2015; pp. 329–344. ISBN 978-1-118-95062-3. [Google Scholar]
- Bieliński, M.; Muzyczuk, P. Ocena Wytrzymałości Na Ściskanie Próbek Porowatego Polipropylenu. Polimery 2011, 56, 309–316. [Google Scholar]
- Mroziński, A.; Flizikowski, J.; Macko, M. Inżynieria Rozdrabniania Biomasy; Grafpol: Wrocław, Poland, 2016; ISBN 978-83-64423-35-2. [Google Scholar]
- Spagnoli, A.; Brighenti, R.; Terzano, M.; Artoni, F.; Ståhle, P. Cutting Resistance of Polymeric Materials: Experimental and Theoretical Investigation. Procedia Struct. Integr. 2018, 13, 137–142. [Google Scholar] [CrossRef]
- Mechanical Behavior of Polymers | MATSE 81: Materials in Today’s World. Available online: https://www.e-education.psu.edu/matse81/node/2109 (accessed on 16 September 2020).
- Hussein, M. Effects of Strain Rate and Temperature on the Mechanical Behavior of Carbon Black Reinforced Elastomers Based on Butyl Rubber and High Molecular Weight Polyethylene. Results Phys. 2018, 9, 511–517. [Google Scholar] [CrossRef]
- Millar, T.M.; Patel, Y.; Wang, H.; Chang, L.; Balint, D.S.; Williams, J.G. An Investigation of Cutting Resistance in Stretched Polymer Films. Procedia Struct. Integr. 2016, 2, 190–196. [Google Scholar] [CrossRef][Green Version]
- Macko, M. Wpływ Cech Konstrukcyjnych Zespołu Wielotarczowego Na Charakterystyki Użytkowe Procesu Rozdrabniania Rurowych Recyklatów Tworzyw Sztucznych. Rozprawa Doktorska; Akademia Techniczno-Rolnicza w Bydgoszczy: Bydgoszcz, Poland, 2000. [Google Scholar]
- Flizikowski, J.; Macko, M. Competitive Design of Shredder for Plastic in Recycling. In Tools and Methods of Competitive Engineering; IOS Press: Amsterdam, The Netherlands, 2004; pp. 1147–1148. Volume 1 and 2. [Google Scholar]
- Lake, G.J.; Yeoh, O.H. Measurement of Rubber Cutting Resistance in the Absence of Friction. Int. J. Fract. 1978, 14, 509–526. [Google Scholar] [CrossRef]
- Flizikowski, J. Pat.176538 Apparatus for Disintegrating Grainy Materials; 06/1999 WUP; The Patent Office of the Republic of Poland: Warsaw, Poland, 1999. [Google Scholar]
- Grellmann, W.; Seidler, S. (Eds.) Deformation and Fracture Behaviour of Polymers; Engineering Materials; Springer: Berlin/Heidelberg, Germany, 2001; ISBN 978-3-540-41247-2. [Google Scholar]
- Arola, D.; Ramulu, M. Orthogonal Cutting of Fiber-Reinforced Composites: A Finite Element Analysis. Int. J. Mech. Sci. 1997, 39, 597–613. [Google Scholar] [CrossRef]
- Vogel, L.; Peukert, W. From Single Particle Impact Behaviour to Modelling of Impact Mills. Chem. Eng. Sci. 2005, 60, 5164–5176. [Google Scholar] [CrossRef]
- Wüstenberg, D.; Kasper, J. Required Energy and Structural Breakdown at the Process of Dynamic Cutting—Comminution of Polypropylene and Aluminium. Int. J. Miner. Process. 2004, 74, S417–S424. [Google Scholar] [CrossRef]
- Macko, M.; Tyszczuk, K.; Śmigielski, G.; Mroziński, A. Utility of an Unitary-Shredding Method to Evaluate the Conditions and Selection of Constructional Features during Grinding. MATEC Web Conf. 2018, 157, 5016. [Google Scholar] [CrossRef]
- Macko, M. Badania oraz analiza sił i odkształceń w rozdrabnianiu jednokrotnym. Inż. Apar. Chem. 2011, 50, 47–48. [Google Scholar]
- Warguła, Ł.; Kukla, M.; Krawiec, P.; Wieczorek, B. Reduction in Operating Costs and Environmental Impact Consisting in the Modernization of the Low-Power Cylindrical Wood Chipper Power Unit by Using Alternative Fuel. Energies 2020, 13, 2995. [Google Scholar] [CrossRef]
- Warguła, Ł.; Kukla, M.; Krawiec, P.; Wieczorek, B. Impact of Number of Operators and Distance to Branch Piles on Woodchipper Operation. Forests 2020, 11, 598. [Google Scholar] [CrossRef]
- Warguła, Ł.; Krawiec, P.; Waluś, K.J.; Kukla, M. Fuel Consumption Test Results for a Self-Adaptive, Maintenance-Free Wood Chipper Drive Control System. Appl. Sci. 2020, 10, 2727. [Google Scholar] [CrossRef]
- Knosala, R. Zastosowania Metod Sztucznej Inteligencji w Inżynierii Produkcji; Wydawnictwa Naukowo-Techniczne: Warsaw, Poland, 2002; ISBN 978-83-204-2767-7. [Google Scholar]
- Sidor, J. Modelowanie Procesu Rozdrabniania Materiału w Młynach Wibracyjnych. Inż. Apar. Chem. 2006, Nr 4s, 129–130. [Google Scholar]
- Flizikowski, J.; Tamporowski, A. Motional Characteristics of Grains in the Multihole Space of a Multi-Disc Grinder. J. Pol. CIMAC 2012, 7, 59–67. [Google Scholar]
- Tomporowski, A. Stream of Efficiency of Rice Grains Multi-Disc Grinding. Eksploat. Niezawodn. Maint. Reliab. 2012, 14, 150–153. [Google Scholar]
- Tomporowski, A.; Opielak, M. Structural Features versus Multi-Hole Grinding Efficiency. Eksploat. Niezawodn. 2012, 14, 222–227. [Google Scholar]
- Zawada, J. Wstęp Do Mechaniki Procesów Kruszenia; Wydawnictwo Technologii Eksploatacji: Radom, Poland, 1998. [Google Scholar]
- Zuñiga, J.M.; Mantari, J.L. A Computational Methodology to Calculate the Required Power in Disc Crushers. J. Comput. Des. Eng. 2017, 4, 14–20. [Google Scholar] [CrossRef]
- Ligaj, B.; Szala, G. Obliczanie Zapotrzebowania Energii w Procesach Rozdrabniania Materiałów Ziarnistych Na Przykładzie Ziaren Zbóż. Acta Mech. Autom. 2009, 3, 97–99. [Google Scholar]
- Domek, G. Pasowo-Zębaty Napęd Rozdrabniacza Wielotarczowego. Inż. Apar. Chem. 2005, Nr 1–2, 35–36. [Google Scholar]
- Tomporowski, A.; Sykut, B.; Kowalik, K.; Antczak, M. Badanie i Analiza Energochłonności Wielotarczowego Rozdrabniacza Nowej Generacji. Inż. Apar. Chem. 2012, 51, 256–258. [Google Scholar]
- Flizikowski, J.; Bieliński, K.; Bieliński, M. Podwyższanie Energetycznej Efektywności Wielotarczowego Rozdrabniania Nasion Zbóż Na Paszę; Wydawnictwa Akademii Techniczno-Rolniczej: Bydgoszcz, Poland, 1994. [Google Scholar]
- Flizikowski, J.; Świetlicki, A. Wydajność Wielotarczowego Rozdrabniania Ziaren. Inż. Apar. Chem. 2009, 48, 45–47. [Google Scholar]
- Flizikowski, J.; Kamyk, W. Efektywność Energetyczna Specjalnego Systemu Rozdrabniania. Teka Kom. Bud. Ekspl. Masz. Elektrotech. Bud. 2008, 17–22. [Google Scholar]
- Flizikowski, J. Test Methods for the Intelligent Development of Grinding Processes. Inż. Apar. Chem. 2016, 55, 90–92. [Google Scholar]
- Flizikowski, J.; Mroziński, A.; Macko, M. Computer Aided Design of Multi-Disc Grinders. Logistyka 2010, 6, 813–822. [Google Scholar]
- Opielak, M. Investigation on the Dependence Oa a Quern Mill Output on the Size of Grinding Slot and Grain Moisture. Int. Agrophysics 1999, 13, 487–491. [Google Scholar]
- Opielak, M.; Jankowski, M.; Piasecka, I.; Tomporowski, A. Analiza Nierównomierności Rozdrabniania Biomasy. Cz. 2. Badania Maszynowe. Inż. Apar. Chem. 2013, 52, 44–46. [Google Scholar]
- Tomporowski, A.; Opielak, M.; Kowalik, K.; Sykut, B. Analiza Nierównomierności Rozdrabniania Biomasy. Część I: Podstawy Teoretyczne. Inż. Apar. Chem. 2013, 52, 42–43. [Google Scholar]
- Opielak, M. Rozdrabnianie Materiałów w Przemyśle Rolno-Spożywczym: Badanie Wpływu Konstrukcji Rozdrabniacza i Cech Materiału Rozdrabnianego na Jakość i Energochłonność Procesu; Wydawnictwa Uczelniane PL: Lublin, Poland, 1996; ISBN 978-83-86333-53-0. [Google Scholar]
- Dudziak, P. Badania Zintegrowanej Efektywności Procesu Rozdrabniania Materiałów Uziarnionych Naddźwiękowym Młynem Tarczowym; Uniwersytet Technologiczno-Przyrodniczy w Bydgoszczy: Bydgoszcz, Poland, 2017. [Google Scholar]
- Yıldırım, A.; Bayram, M.; Öner, M.D. Bulgur Milling Using a Helical Disc Mill. J. Food Eng. 2008, 87, 564–570. [Google Scholar] [CrossRef]
- Radziszewski, P. Energy Recovery Potential in Comminution Processes. Miner. Eng. 2013, 46–47, 83–88. [Google Scholar] [CrossRef]
- Dikici, T.; Sutcu, M. Effects of Disc Milling Parameters on the Physical Properties and Microstructural Characteristics of Ti6Al4V Powders. J. Alloys Compd. 2017, 723, 395–400. [Google Scholar] [CrossRef]
- Kruszelnicka, W.; Marczuk, A.; Kasner, R.; Bałdowska-Witos, P.; Piotrowska, K.; Flizikowski, J.; Tomporowski, A. Mechanical and Processing Properties of Rice Grains. Sustainability 2020, 12, 552. [Google Scholar] [CrossRef]
- Tomporowski, A.; Flizikowski, J.; Kruszelnicka, W. A new concept of roller-plate mills. Przem. Chem. 2017, 96, 1750–1755. [Google Scholar] [CrossRef]
- Adapa, P.; Tabil, L.; Schoenau, G. Grinding Performance and Physical Properties of Non-Treated and Steam Exploded Barley, Canola, Oat and Wheat Straw. Biomass Bioenergy 2011, 35, 549–561. [Google Scholar] [CrossRef]
- Chițoiu, M.; Voicu, G.; Moiceanu, G.; Paraschiv, G.; Dincă, M.; Vladuț, V.; Tudor, P. Energy Consumption Analysis on Energetic Plant Biomass Grinding Using Hammer Mills. UPB Sci. Bull. Ser. Mech. Eng. 2018, 80, 117–128. [Google Scholar]
- Macko, M. Konstrukcja Rozdrabniaczy Do Biomasy. Available online: http://naszaenergia.kujawsko-pomorskie.pl/fileadmin/doc/artykuly/nowe/KONSTRUKCJA_ROZDRABNIACZY_DO_BIOMASY.pdf.
- Flizikowski, J.; Sadkiewicz, J. Inżynieria Innowacji Rozdrabniaczy Ziarnistej Biomasy. Inż. Apar. Chem. 2013, 52, 36–37. [Google Scholar]
- Griffith, A.A. The Phenomena of Rupture and Flow in Solids. Philos. Trans. R. Soc. Lond. Ser. Contain. Pap. Math. Phys. Character 1921, 221, 163–198. [Google Scholar]
- Behrens, D. Einige Neuentwicklungen von Fein- und Feinstprallzerkleinerungs-maschinen. In Symposium Zerkleinern; Verlag Chemie GmbH: Weinheim, Germany, 1962; pp. 474–502. [Google Scholar]
- Rumpf, H. Physical Aspects of Comminution and New Formulation of a Law of Comminution. Powder Technol. 1973, 7, 145–159. [Google Scholar] [CrossRef]
- Rumpf, H. Problems of Scientific Development in Particle Technology, Looked upon from a Practical Point of View. Powder Technol. 1977, 18, 3–17. [Google Scholar] [CrossRef]
- Schonert, K. The Characteristics of Comminution with High Pressure Roller Mills. KONA 1991, 149–158. [Google Scholar] [CrossRef]
- Schönert, K. A First Survey of Grinding with High-Compression Roller Mills. Int. J. Miner. Process. 1988, 22, 401–412. [Google Scholar] [CrossRef]
- Schönert, K. Breakage of Spheres and Circular Discs. Powder Technol. 2004, 143–144, 2–18. [Google Scholar] [CrossRef]
- Schönert, K.; Sander, U. Shear Stresses and Material Slip in High Pressure Roller Mills. Powder Technol. 2002, 122, 136–144. [Google Scholar] [CrossRef]
- Forssberg, K.S.E.; Schönert, K. Comminution 1994; Newnes: Oxford, UK, 2012; ISBN 978-0-444-59865-3. [Google Scholar]
- Kerlin, H.P. Comminution of Coal Grains and Bulk Coal. Energy Res. Abstr. 1983, 8, 497. [Google Scholar]
- Kruszelnicka, W. Analiza Wskaźników Stosowanych Do Oceny Rozdrabniania. Inz. Apar. Chem. 2017, 56, 208–210. [Google Scholar]
- Kruszelnicka, W.; Tomporowski, A.; Flizikowski, J.; Mroziński, A. Analiza procesu rozdrabniania biomateriałów w młynie walcowym z płytą międzywalcową w ujęciu emisji CO2. Cz. 1, Składowe modelu. Przem. Chem. 2020, 99. [Google Scholar] [CrossRef]
- Hryniewicz, M.; Bembenek, M.; Janewicz, A.; Kosturkiewicz, B. Agglomeration of fine-grained materials in roll presses with asymmetrical compaction unit. Przemysl. Chem. 2015, 94, 2223–2226. [Google Scholar]
- Flizikowski, J. Rozprawa o Konstrukcji; ATR Bydgoszcz, Instytut Technologii Eksploatacji w Radomiu: Radom, Poland, 2002; ISBN 978-83-7204-280-4. [Google Scholar]
- Kasner, R. Ocena Korzyści i Nakładów Cyklu Życia Elektrowni Wiatrowej; Politechnika Poznańska: Poznań, Poland, 2016; p. 186. [Google Scholar]
- Flizikowski, J. Energy Consumption Reduction in Recycling Processes. Inż. Apar. Chem. 2012, 51, 61–62. [Google Scholar]
- Flizikowski, J. Badania i rozwój rozdrabniania materiałów polimerowych w kierunku podwyższenia energetycznej efektywności procesów recyklingu. Przetw. Tworzyw 2011, 17, 128–131. [Google Scholar]



| End-Of-Life Option | Total Amount of Waste 1, mln t | Percentage of Waste Shredded 2, % | Mass of Waste Shredded, mln t |
|---|---|---|---|
| Landfill | 7.2 | 2 | 0.144 |
| Energy Recovery | 12.4 | 20 | 2.480 |
| Recycling | 9.5 | 50 (100 3) | 4.750 (9.500) |
| Sum | 29.1 | - | 7.374 (12.124) |
| Type | Load Model | Stresses |
|---|---|---|
| Crushing |  | Compressive stresses |
| Shear |  | Shear stresses |
| Abrasion |  | Surface pressures |
| Impact |  | Surface pressures |
| Breaking |  | Bending stresses |
| Type | Grain Size Classes |
|---|---|
| coarse crushing | 50–500 mm |
| fine crushing | 5–50 mm |
| very fine crushing | 0.5–5 mm |
| coarse grinding | 0.1–1 mm |
| fine grinding | 10–150 µm |
| very fine grinding | 1–20 µm |
| colloidal grinding | 0.1–2 µm |
| Stage of the Problem | Results & Solution |
|---|---|
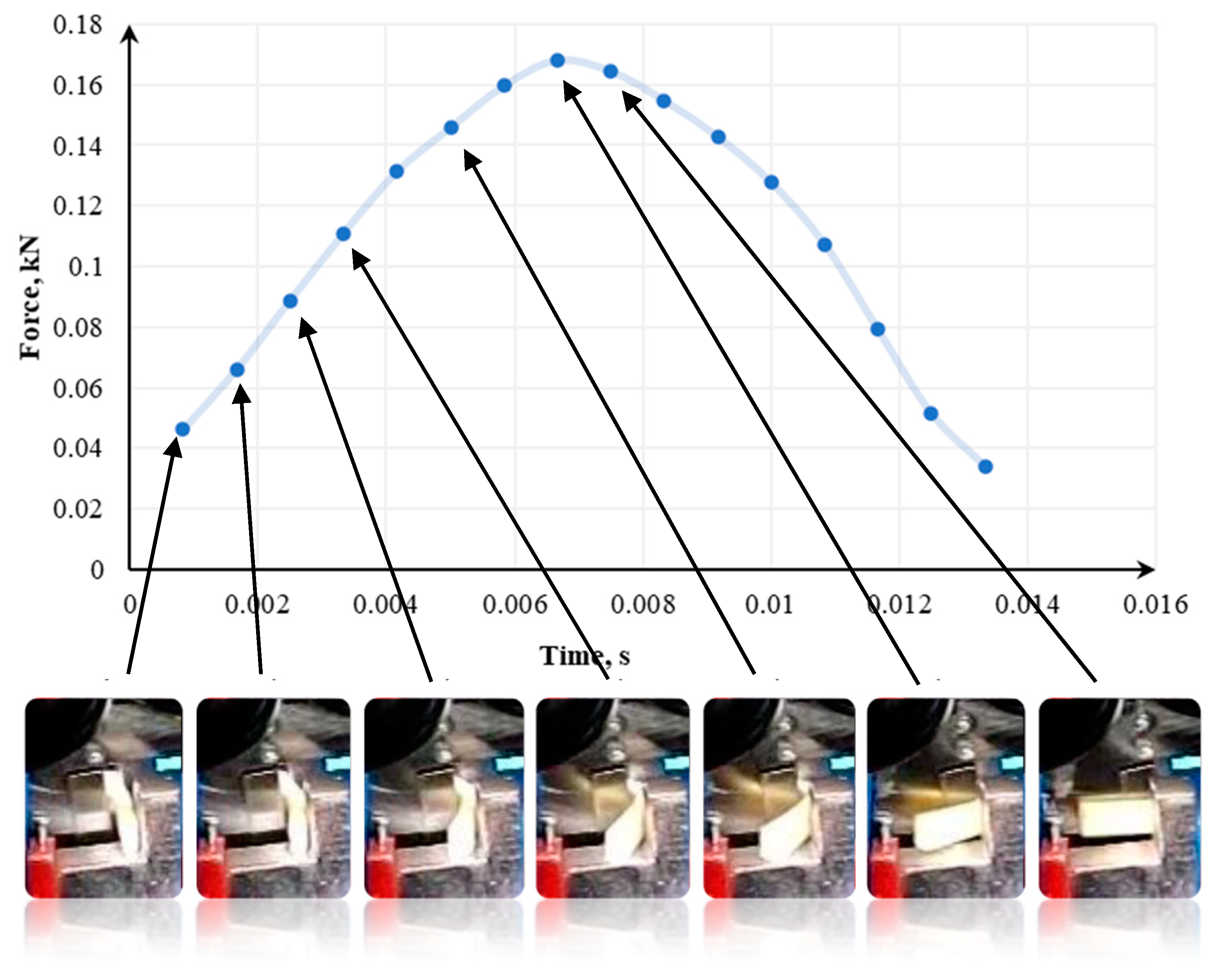
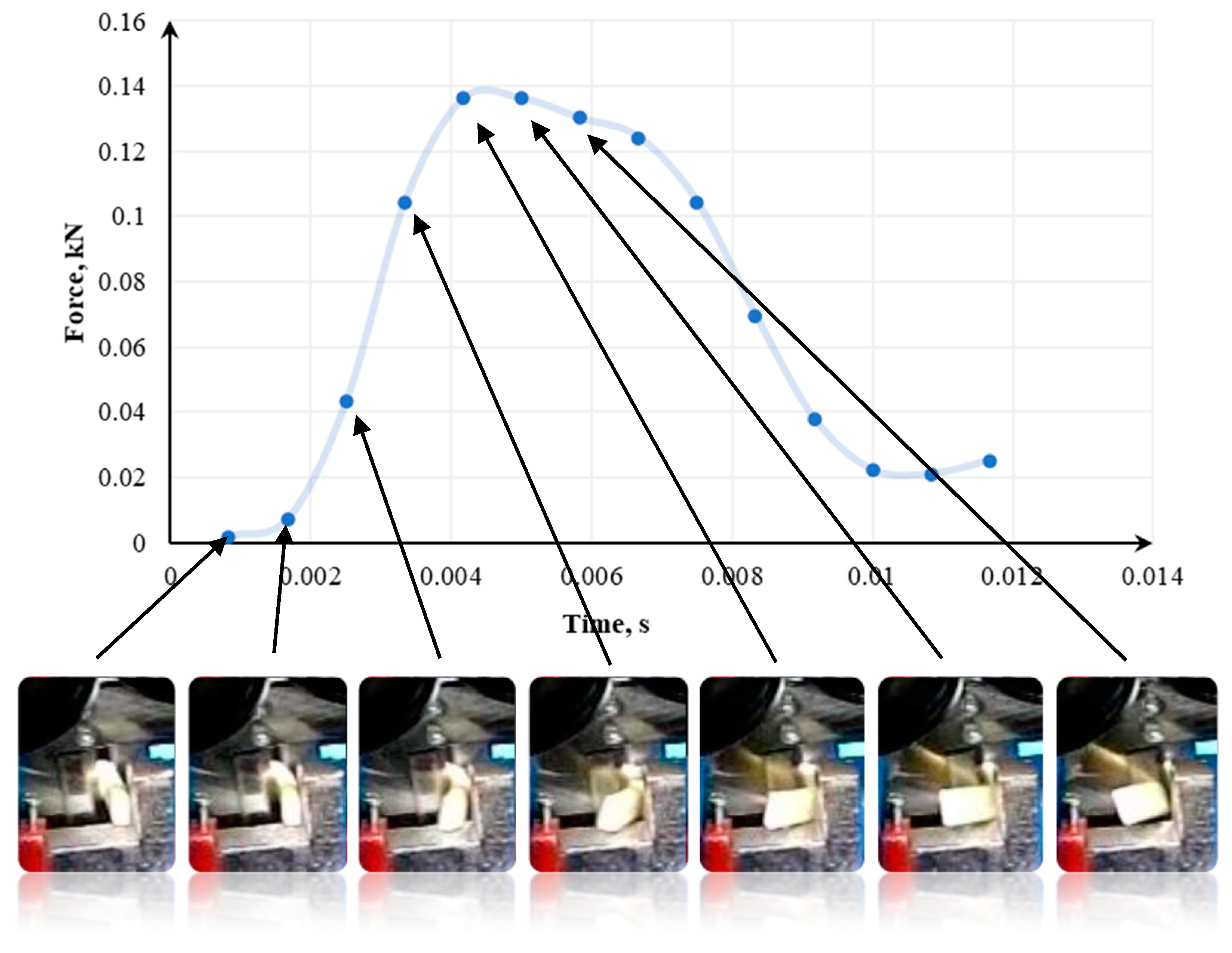
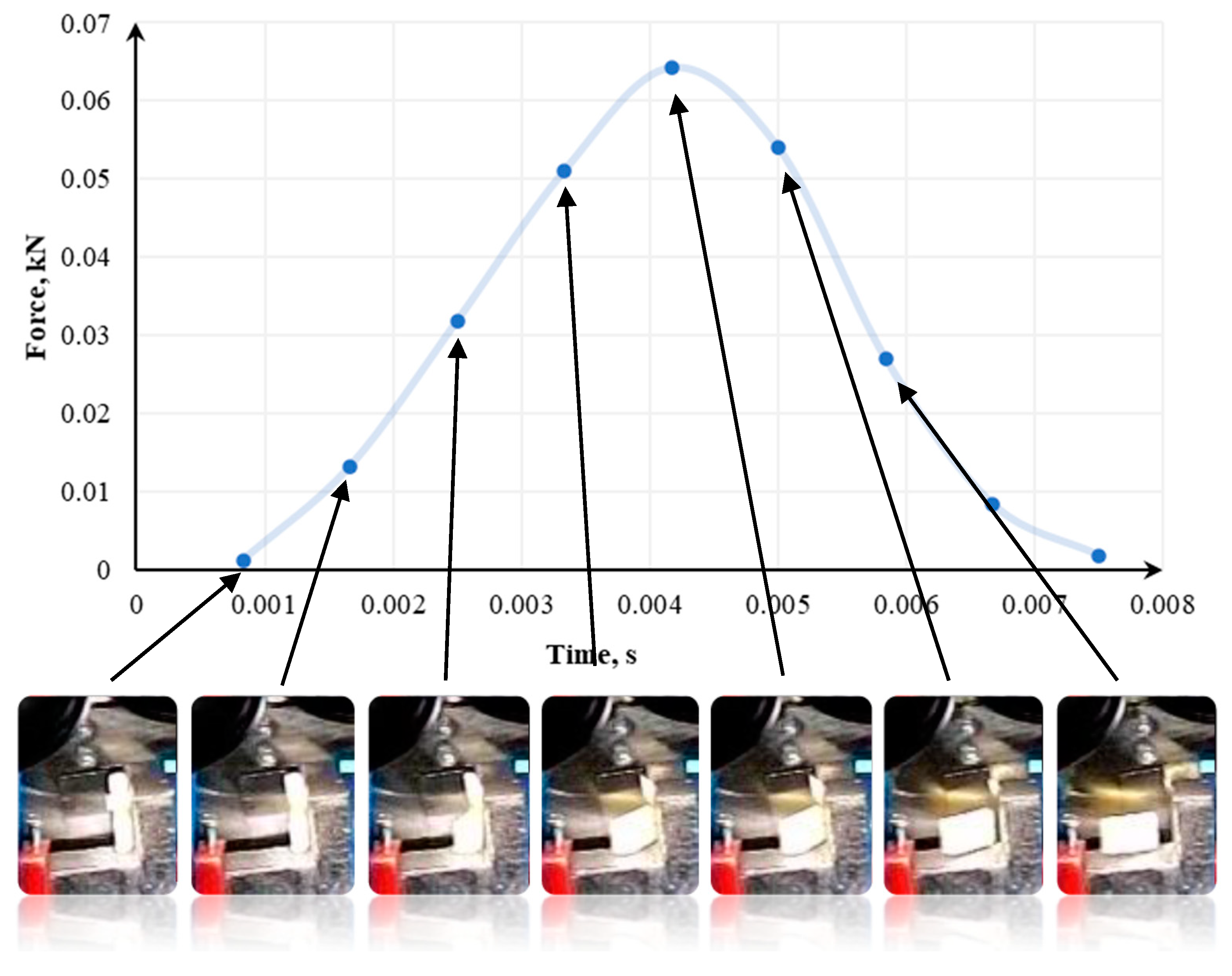
| Strength-static investigations of PVC post-use pipe carried out by INSTRON 8501—Value of energy needed to disintegration (lines blade angle β = 60°, β = 75°, β = 90°, β = 105°, β = 120°—models of deformation and loads of PVC pipe) |  P = f (Δl), p-value of force, Δl—value of displacement P = f (Δl), p-value of force, Δl—value of displacement |
| Unevenness of quasi-shear forces P and plate displacements, for different sample (disturbed) forms and settings in the instrument. Blade angle β = 90°, plastic: Low-density polyethylene (LDPE) pipe, outer diameter Dz = 40 mm, wall thickness, g = 4.3 mm, sample length lp = 50 mm, relative speed of shredding vr = 30 mm·s−1 | 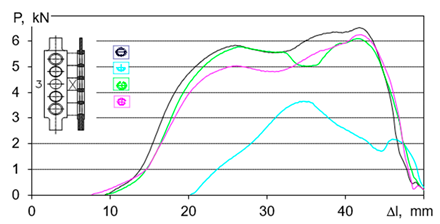 P = f (Δl), p-value of force, Δl—value of displacement P = f (Δl), p-value of force, Δl—value of displacement |
| Lp. | Research Area | Ref. |
|---|---|---|
| 1 | Determination of the dependencies and effects of the shredder design features and the charge physical and mechanical features on the grinding parameters, i.e., energy consumption and quality | [164] |
| 2 | Study of the multi-disc grinders uneven operation | [162,163] |
| 3 | Study of the grinding process efficiency in a supersonic disc grinder | [165] |
| 4 | Study of the influence of inter-disc gap size on the grinding product quality, energy consumption, and efficiency | [166] |
| 5 | Analysis of energy losses in the form of heat during grinding and the possibility of its recovery | [167] |
| 6 | Dynamic analysis of forces acting on the shredded material and the grinding disc | [152] |
| 7 | Investigation of the influence of the grinding process parameters on the product physical properties and microstructure | [168] |
| GRINDING INDICATORS | ||
|---|---|---|
| Technological | Technical | Economic |
| Size reduction ratio | Specific energy consumption | Operating costs |
| Specific surface area | Possibility of cooperation with other devices | Investment costs |
| The degree of surface growth | Effectiveness | Costs of accompanying processes |
| Total efficiency | ||
| Grain shape | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Flizikowski, J.; Kruszelnicka, W.; Macko, M. The Development of Efficient Contaminated Polymer Materials Shredding in Recycling Processes. Polymers 2021, 13, 713. https://doi.org/10.3390/polym13050713
Flizikowski J, Kruszelnicka W, Macko M. The Development of Efficient Contaminated Polymer Materials Shredding in Recycling Processes. Polymers. 2021; 13(5):713. https://doi.org/10.3390/polym13050713
Chicago/Turabian StyleFlizikowski, Józef, Weronika Kruszelnicka, and Marek Macko. 2021. "The Development of Efficient Contaminated Polymer Materials Shredding in Recycling Processes" Polymers 13, no. 5: 713. https://doi.org/10.3390/polym13050713
APA StyleFlizikowski, J., Kruszelnicka, W., & Macko, M. (2021). The Development of Efficient Contaminated Polymer Materials Shredding in Recycling Processes. Polymers, 13(5), 713. https://doi.org/10.3390/polym13050713