Effects of Large Deformation and Velocity Impacts on the Mechanical Behavior of Filled Rubber: Microstructure-Based Constitutive Modeling and Mechanical Testing
Abstract
:1. Introduction
2. Constitutive Modeling
2.1. Hyperelasticity
2.2. Hyper-Viscoelastic
3. Mechanical Testing Program
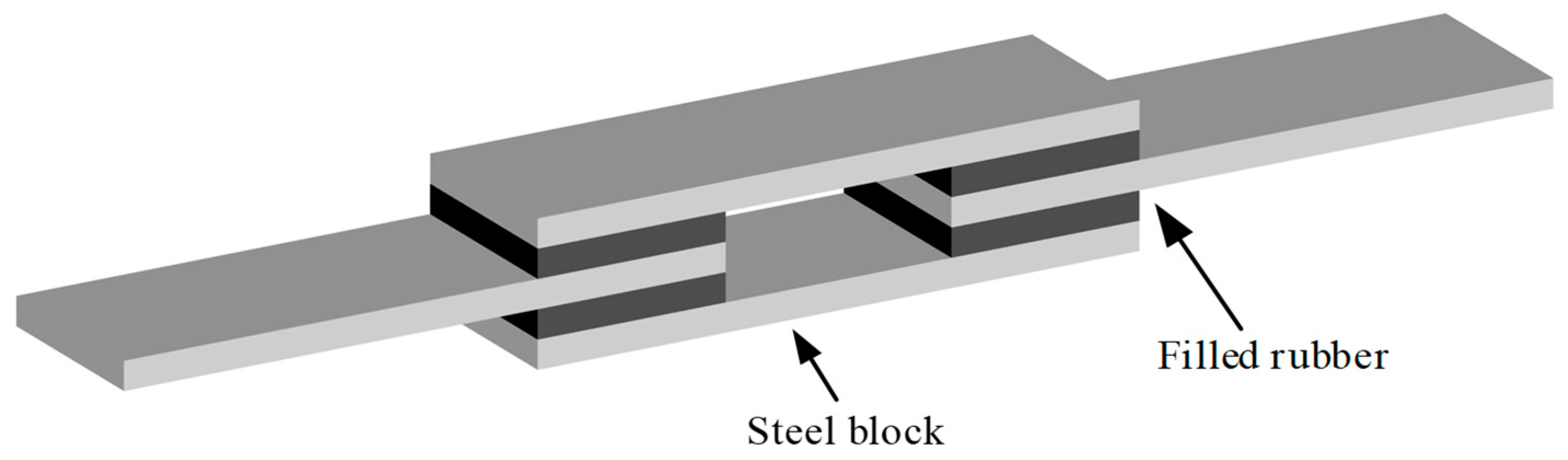
3.1. Specimen Preparation and Test Setup
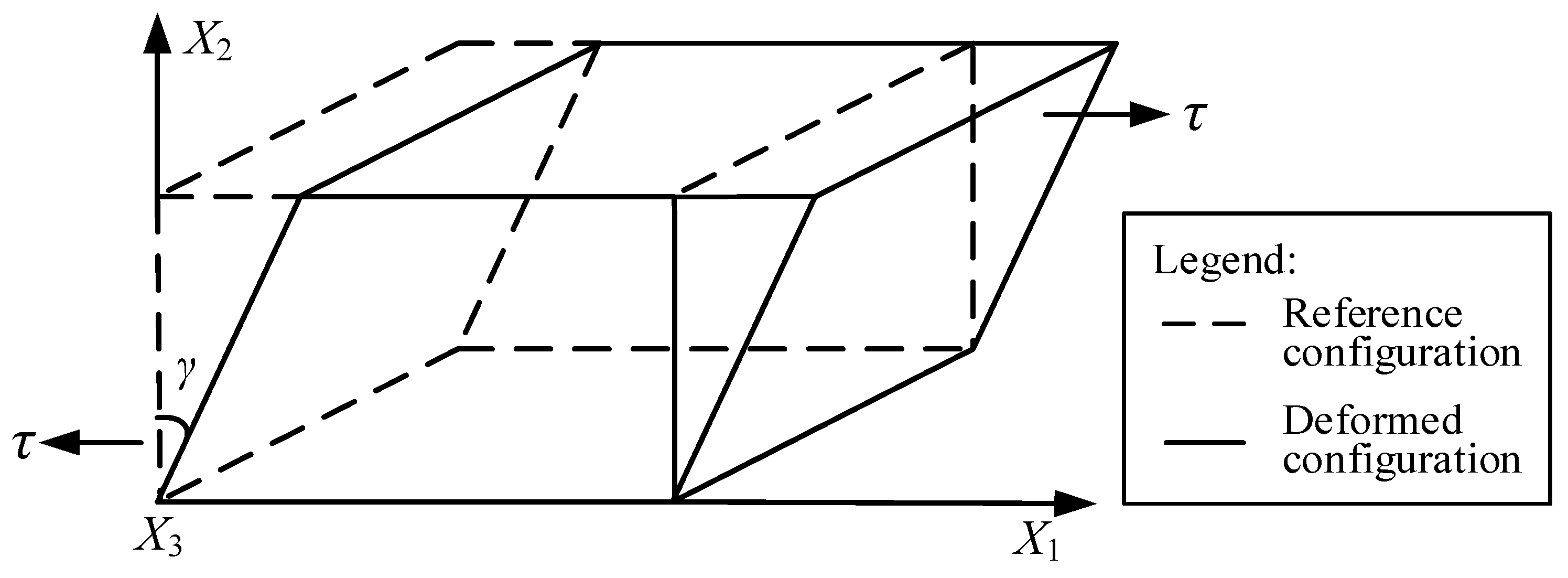
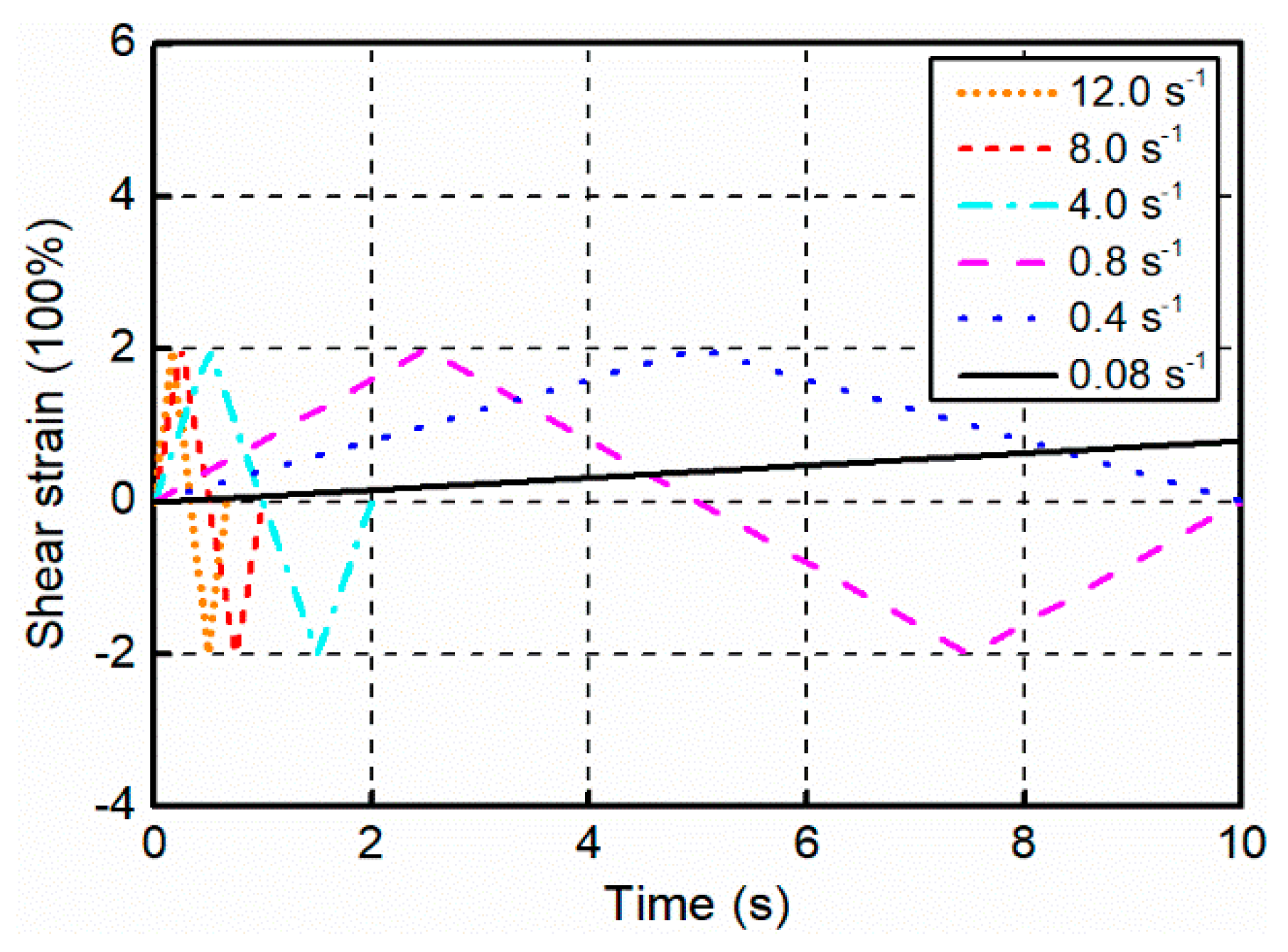
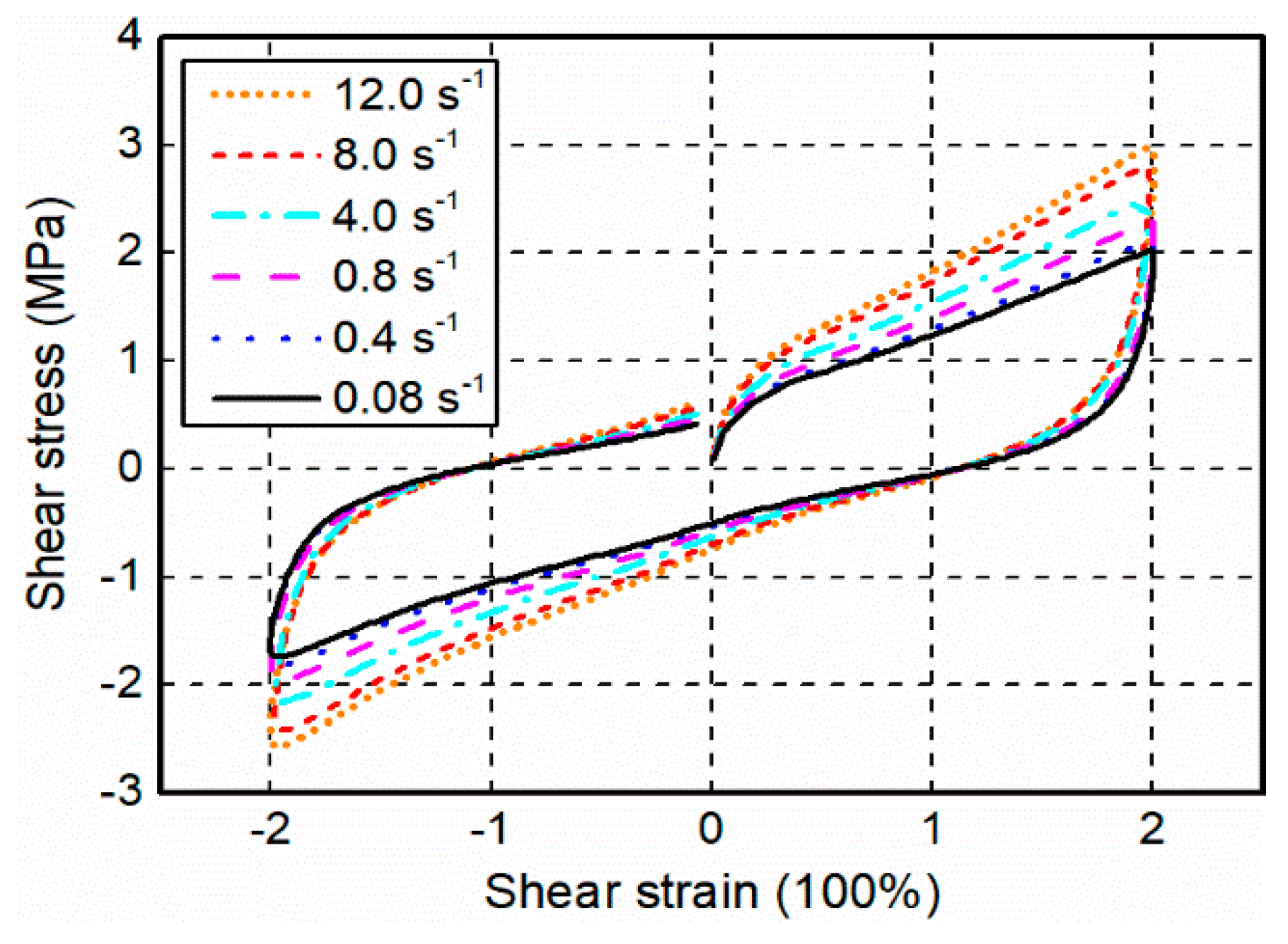
3.2. Stress Relaxation and Cyclic Shear Tests
4. Parameter Identification
4.1. Hyperelastic Parameters
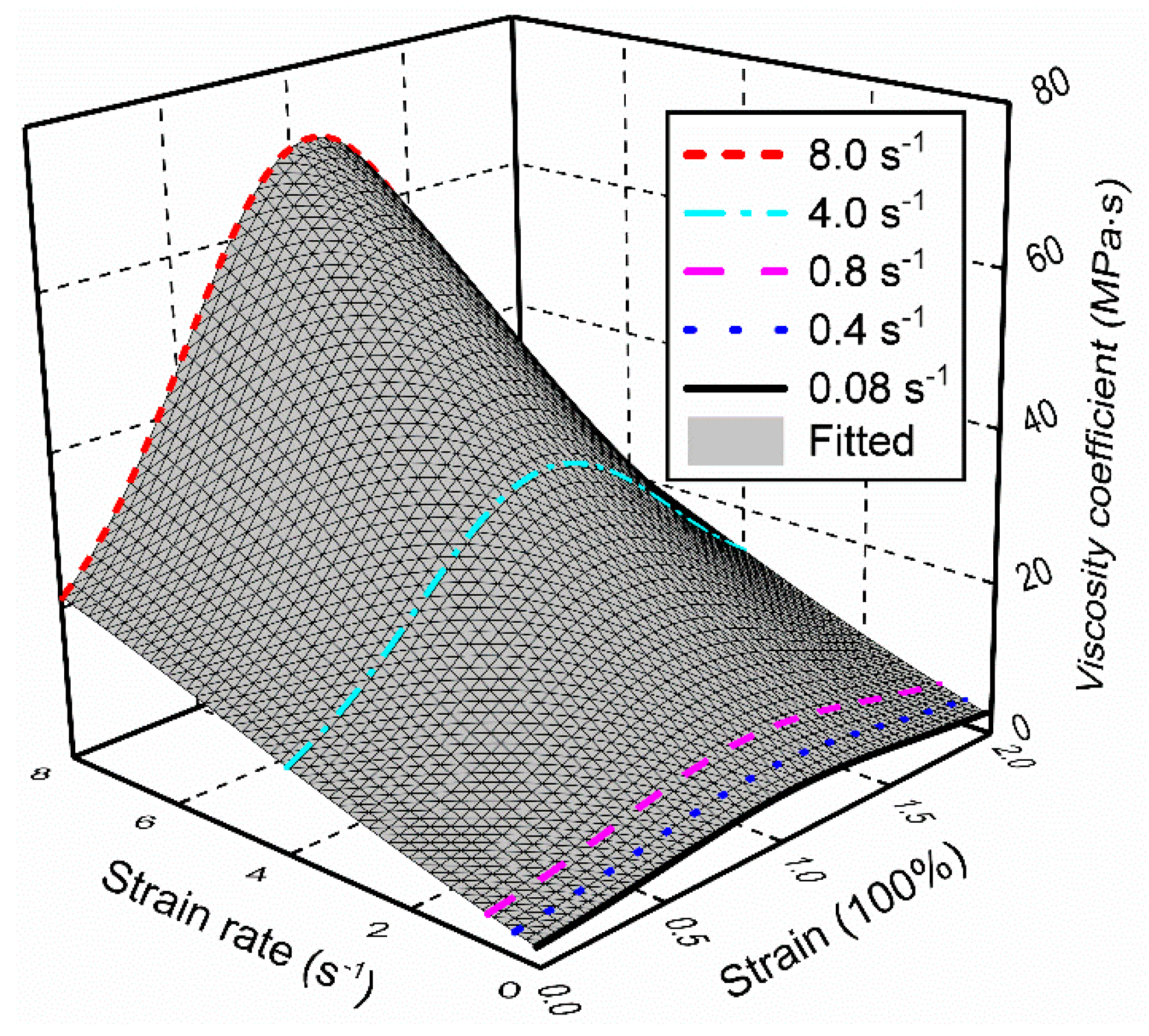
4.2. Viscoelastic Parameters
5. Model Application, Validation, and Discussion
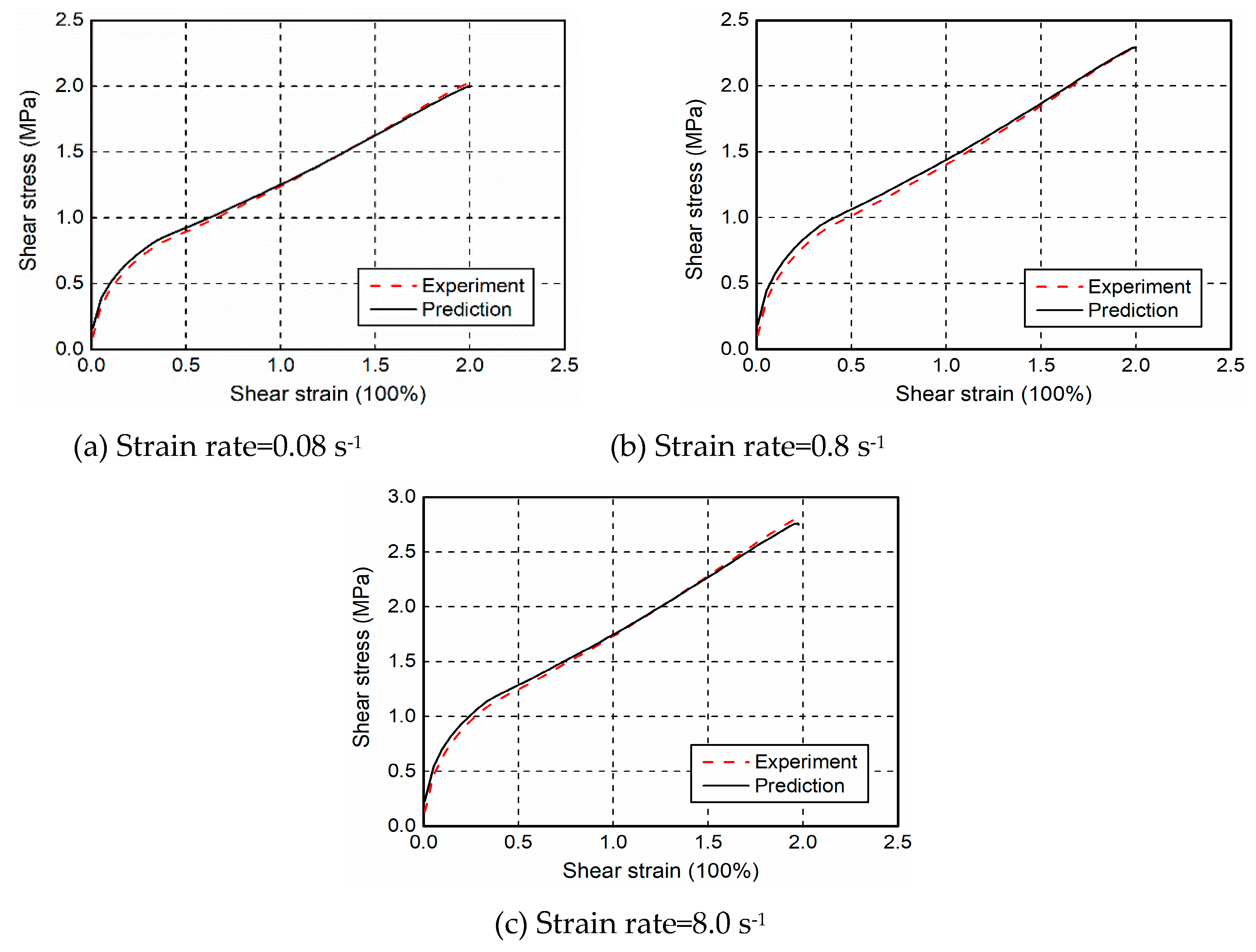
5.1. Monotonic Shear Tests and Model Predictions
5.2. Cyclic Shear Tests and Model Predictions
6. Conclusions
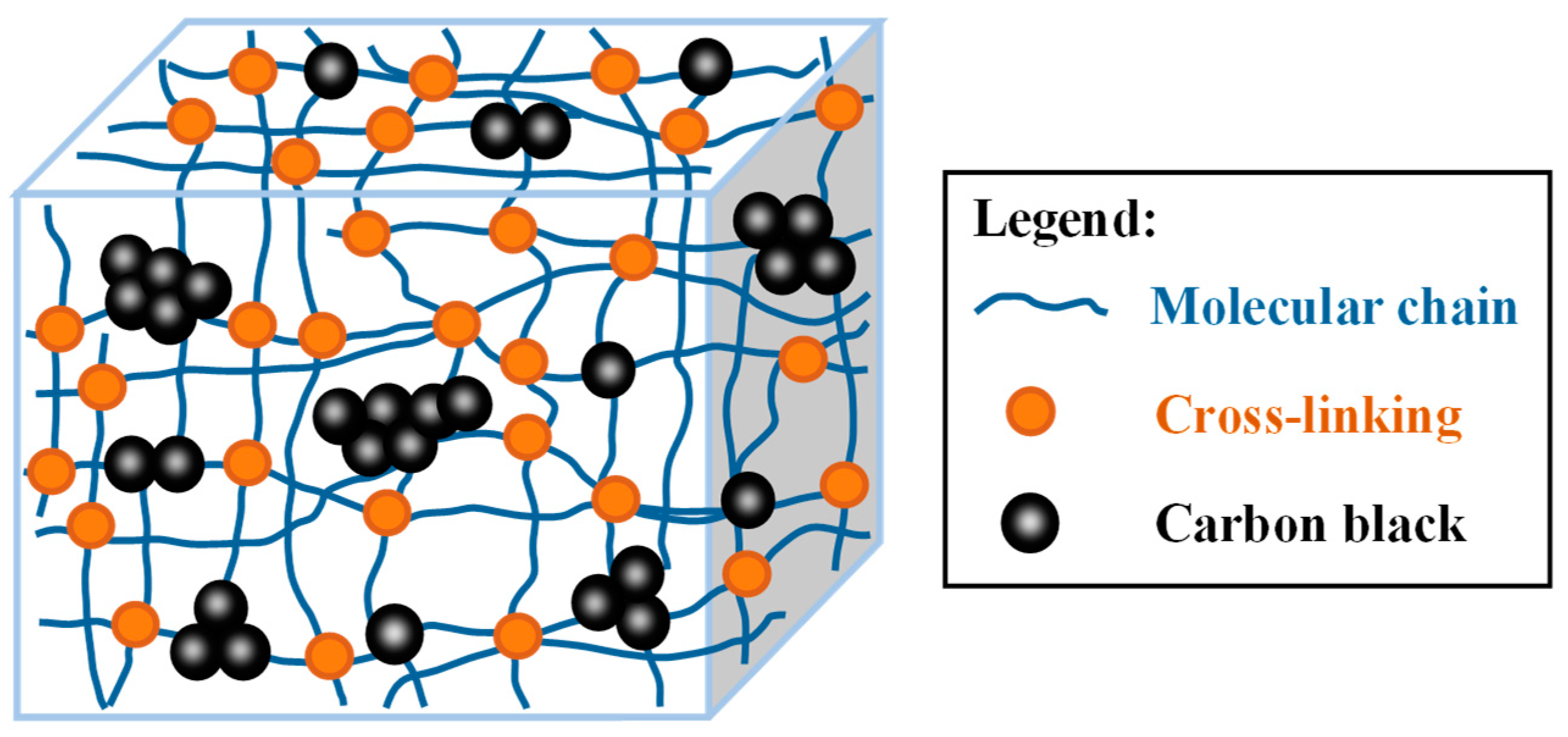
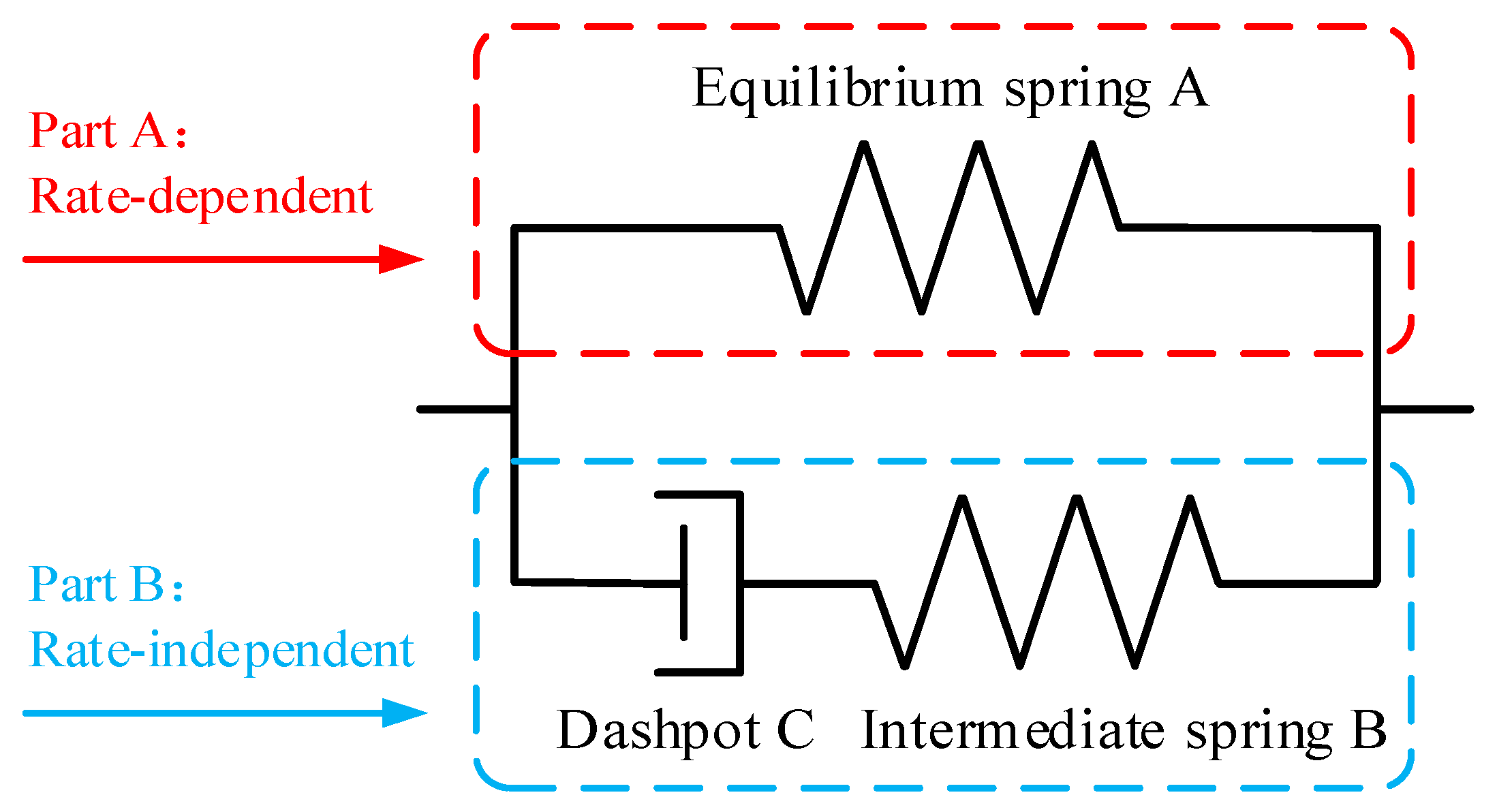
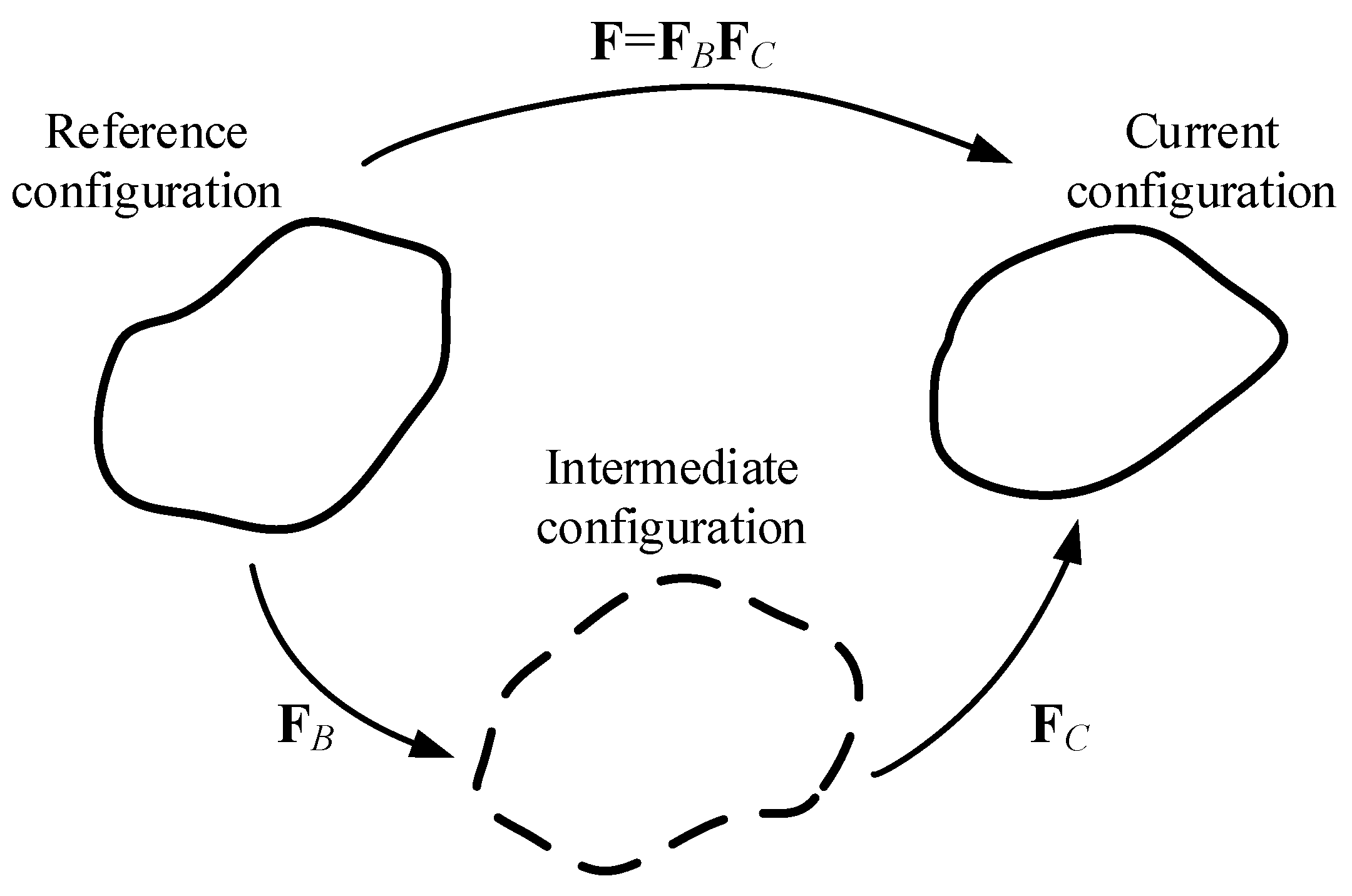
- The constitutive model comprises two parts: the first captures the equilibrium and instantaneous responses of filled rubber; the second incorporates the decomposition of the deformation gradient tensor to correlate the overstress tensor with strain rate. The proposed model covers a wide range of conditions, including small to large shear deformations as well as low to high velocity impacts that the filled rubber is expected to undergo in engineering practices.
- Considering the similar nature (i.e., nonlinear hyperelasticity) of equilibrium and instantaneous responses of filled rubber, a newly-developed polynomial strain energy function is applied for equilibrium and intermediate springs in the constitutive model. The proposed strain energy function has a relatively simple mathematical formulation and its parameters are related to the physical description of the material molecular network.
- A “Gau-Poly” function isproposed to capture the nonlinear viscosity coefficient in the constitutive model, three-dimensional plot of the experimental and fitted results of the viscosity coefficientshows that the “Gau-Poly” function has a good generalization ability to predict the variation in viscosity coefficient with extensive ranges of strains and strain rates.
- The accuracy of the proposed constitutive model was verified by comparing the experimental tests and numerical simulation. A reasonable agreement between the experimental and numerical results substantiated the validity of the proposed model. The model not only fills a theoretical gap by developing an advanced model of filled rubber, but also supplies an appropriate choice to engineers to describe the behavior of filled rubber under the considered conditions. In the future, it is expected that the model can be implemented in finite element codes as a novel user-defined model to facilitate the numerical simulation when designing structural devices that incorporate such materials.
Author Contributions
Funding
Conflicts of Interest
References
- Luo, W.; Hu, X.; Wang, C.; Li, Q. Frequency-and strain-amplitude-dependent dynamical mechanical properties and hysteresis loss of CB-filled vulcanized natural rubber. Int. J. Mech. Sci. 2010, 52, 168–174. [Google Scholar] [CrossRef]
- Medina, N.F.; Garcia, R.; Hajirasouliha, I.; Pilakoutas, K.; Guadagnini, M.; Raffoul, S. Composites with recycled rubber aggregates: Properties and opportunities in construction. Constr. Build. Mater. 2018, 188, 884–897. [Google Scholar] [CrossRef]
- Zhao, P.; Hua, X.; Wang, Y.; Zhu, J.; Wen, Q. Structure and properties of polyurethane elastomer cured in graded temperature field. Mater. Sci. Eng. A 2007, 457, 231–235. [Google Scholar] [CrossRef]
- Wei, W.; Tan, P.; Yuan, Y.; Zhu, H. Experimental and analytical investigation of the influence of compressive load on rate-dependent high-damping rubber bearings. Constr. Build. Mater. 2019, 200, 26–35. [Google Scholar] [CrossRef]
- Luo, W.; Huang, Y.; Yin, B.; Jiang, X.; Hu, X. Fatigue Life Assessment of Filled Rubber by Hysteresis Induced Self-Heating Temperature. Polymers 2020, 12, 846. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ye, N.; Zheng, J.; Ye, X.; Xue, J.; Han, D.; Xu, H.; Wang, Z.; Zhang, L. Performance enhancement of rubber composites using VOC-Free interfacial silica coupling agent. Compos. Part B Eng. 2020, 202, 108301. [Google Scholar] [CrossRef]
- Roy, K.; Debnath, S.C.; Potiyaraj, P. A critical review on the utilization of various reinforcement modifiers in filled rubber composites. J. Elastomers Plast. 2020, 52, 167–193. [Google Scholar] [CrossRef]
- Gao, Y.; Hu, F.; Wu, Y.; Liu, J.; Zhang, L. Understanding the structural evolution under the oscillatory shear field to determine the viscoelastic behavior of nanorod filled polymer nanocomposites. Comput. Mater. Sci. 2018, 142, 192–199. [Google Scholar] [CrossRef]
- Lei, M.; Xu, B.; Pei, Y.; Lu, H.; Fu, Y.Q. Micro-mechanics of nanostructured carbon/shape memory polymer hybrid thin film. Soft Matter. 2016, 12, 106–114. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Z.; Hou, S.; Mao, Y.; He, L.; Han, X. Rate-related study on the ply orientation of carbon fiber reinforced epoxy composite laminates. Int. J. Mech. Sci. 2020, 188, 105968. [Google Scholar] [CrossRef]
- Khajehsaeid, H.; Arghavani, J.; Naghdabadi, R.; Sohrabpour, S. A visco-hyperelastic constitutive model for rubber-like materials: A rate-dependent relaxation time scheme. Int. J. Eng. Sci. 2014, 79, 44–58. [Google Scholar] [CrossRef]
- Lu, X.; Li, X.; Tian, M. Preparation of high damping elastomer with broad temperature and frequency ranges based on ternary rubber blends. Polym. Adv. Technol. 2014, 25, 21–28. [Google Scholar] [CrossRef]
- Amin, A.F.M.S.; Lion, A.; Sekita, S.; Okui, Y. Nonlinear dependence of viscosity in modeling the rate-dependent response of natural and high damping rubbers in compression and shear: Experimental identification and numerical verification. International. J. Plast. 2006, 22, 1610–1657. [Google Scholar] [CrossRef]
- Lion, A. A constitutive model for carbon black filled rubber: Experimental investigations and mathematical representation. Contin. Mech. Thermodynamics 1996, 8, 153–169. [Google Scholar] [CrossRef]
- Jankowski, R. Nonlinear rate dependent model of high damping rubber bearing. Bull. Earthq. Eng. 2003, 1, 397–403. [Google Scholar] [CrossRef]
- Li, X.; Xia, Y.; Li, Z.; Xia, Y. Three-dimensional numerical simulations on the hyperelastic behavior of carbon-black particle filled rubbers under moderate finite deformation. Comput. Mater. Sci. 2012, 55, 157–165. [Google Scholar] [CrossRef]
- Gjorgjiev, I.; Garevski, M. A polynomial analytical model of rubber bearings based on series of tests. Eng. Struct. 2013, 56, 600–609. [Google Scholar] [CrossRef]
- Sahu, R.K.; Patra, K. Rate-dependent mechanical behavior of VHB 4910 elastomer. Mech. Adv. Mater. Struct. 2016, 23, 170–179. [Google Scholar] [CrossRef]
- Khajehsaeid, H. Application of fractional time derivatives in modeling the finite deformation viscoelastic behavior of carbon-black filled NR and SBR. Polym. Test. 2018, 68, 110–115. [Google Scholar] [CrossRef]
- Mokhireva, K.A.; Svistkov, A.L. A new approach to describe the elastic behavior of filled rubber-like materials under complex uniaxial loading. Int. J. Solids Struct. 2020, 202, 816–821. [Google Scholar] [CrossRef]
- Nunes, L.C.S.; Moreira, D.C. Simple shear under large deformation: Experimental and theoretical analyses. Eur. J. Mech. A/Solids. 2013, 42, 315–322. [Google Scholar] [CrossRef]
- Ghorbanoghli, A.; Narooei, K. A new hyper-viscoelastic model for investigating rate dependent mechanical behavior of dual cross link self-healing hydrogel. Int. J. Mech. Sci. 2019, 159, 278–286. [Google Scholar] [CrossRef]
- Somarathna, H.M.C.C.; Raman, S.N.; Mohotti, D.; Mutalib, A.A.; Badri, K.H. Hyper-viscoelastic constitutive models for predicting the material behavior of polyurethane under varying strain rates and uniaxial tensile loading. Constr. Build. Mater. 2020, 236, 117417. [Google Scholar] [CrossRef]
- Tomita, Y.; Lu, W.; Naito, M.; Furutani, Y. Numerical evaluation of micro-to macroscopic mechanical behavior of carbon-black-filled rubber. Int. J. Mech. Sci. 2006, 48, 108–116. [Google Scholar] [CrossRef] [Green Version]
- Bergström, J.S.; Boyce, M.C. Large strain time-dependent behavior of filled elastomers. Mech. Mater. 2000, 32, 627–644. [Google Scholar] [CrossRef]
- Pouriayevali, H.; Guo, Y.B.; Shim, V.P.W. A constitutive description of elastomer behaviour at high strain rates-A strain-dependent relaxation time approach. Int. J. Impact Eng. 2012, 47, 71–78. [Google Scholar] [CrossRef]
- Shim, J.; Mohr, D. Rate dependent finite strain constitutive model of polyurea. Int. J. Plast. 2011, 27, 868–886. [Google Scholar] [CrossRef]
- Zhou, L.; Wang, S.; Li, L.; Fu, Y. An evaluation of the Gent and Gent-Gent material models using inflation of a plane membrane. Int. J. Mech. Sci. 2018, 146, 39–48. [Google Scholar] [CrossRef]
- Bahreman, M.; Darijani, H. New polynomial strain energy function; application to rubbery circular cylinders under finite extension and torsion. J. Appl. Polym. Sci. 2015, 132, 41748. [Google Scholar] [CrossRef]
- Mansouri, M.R.; Darijani, H. Constitutive modeling of isotropic hyperelastic materials in an exponential framework using a self-contained approach. Int. J. Solids Struct. 2014, 51, 4316–4326. [Google Scholar] [CrossRef] [Green Version]
- Kawabata, S.; Matsuda, M.; Tei, K.; Kawai, H. Experimental survey of the strain energy density function of isoprene rubber vulcanizate. Macromolecules 1981, 14, 154–162. [Google Scholar] [CrossRef]
- Marckmann, G.; Verron, E. Comparison of hyperelastic models for rubber-like materials. Rubber Chem. Technol. 2006, 79, 835–858. [Google Scholar] [CrossRef] [Green Version]
- Kim, B.; Lee, S.B.; Lee, J.; Cho, S.; Park, H.; Yeom, S.; Park, S.H. A comparison among Neo-Hookean model, Mooney-Rivlin model, and Ogden model for chloroprene rubber. Int. J. Precis. Eng. Manuf. 2012, 13, 759–764. [Google Scholar] [CrossRef]
- Horgan, C.O.; Smayda, M.G. The importance of the second strain invariant in the constitutive modeling of elastomers and soft biomaterials. Mech. Mater. 2012, 51, 43–52. [Google Scholar] [CrossRef]
- Huber, N.; Tsakmakis, C. Finite deformation viscoelasticity laws. Mech. Mater. 2000, 32, 1–18. [Google Scholar] [CrossRef]
- Coleman, B.D.; Gurtin, M.E. Thermodynamics with internal state variables. J. Chem. Phys. 1967, 47, 597–613. [Google Scholar] [CrossRef]
- British Standard, ISO 1827:2007. In Determination of Shear Modulus and Adhesion to Rigid Plates—Quadruple-Shear Methods; ISO: Geneva, Switzerland, 2007.
- Yamaguchi, K.; Thomas, A.G.; Busfield, J.J.C. Stress relaxation, creep and set recovery of elastomers. Int. J. Non-Linear Mech. 2015, 68, 66–70. [Google Scholar] [CrossRef]
- Fatt, M.S.H.; Ouyang, X. Three-dimensional constitutive equations for styrene butadiene rubber at high strain rates. Mech. Mater. 2008, 40, 1–16. [Google Scholar] [CrossRef]
- Wei, W.; Yuan, Y.; Igarashi, A.; Tan, P.; Iemura, H.; Zhu, H. A generalized rate-dependent constitutive law for elastomeric bearings. Constr. Build. Mater. 2016, 106, 693–699. [Google Scholar] [CrossRef] [Green Version]




| Stress Tensor | C1 | C2 | C3 | C4 | C5 |
|---|---|---|---|---|---|
| Equilibrium stress | 0.899 | −1.146 | 0.678 | −0.127 | 0 |
| Overstress | 2.484 | −5.623 | 6.263 | −3.075 | 0.548 |
| z0 (MPa·s) | A (MPa·s) | xc | w1 | yc (s−1) | w2 (s−1) | b | c (s) | d (s2) |
|---|---|---|---|---|---|---|---|---|
| 0.346 | 1.559 | 1.090 | 0.551 | 6.607 | 6.030 | 4.194 | 3.779 | 0.052 |
| Case | Experimental Results | Model Predictions | Relative Error |
|---|---|---|---|
| Strain rate = 0.4 s−1 | 4.65 | 4.83 | +3.87% |
| Strain rate = 4.0 s−1 | 5.46 | 5.73 | +4.94% |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wei, W.; Yuan, Y.; Gao, X. Effects of Large Deformation and Velocity Impacts on the Mechanical Behavior of Filled Rubber: Microstructure-Based Constitutive Modeling and Mechanical Testing. Polymers 2020, 12, 2322. https://doi.org/10.3390/polym12102322
Wei W, Yuan Y, Gao X. Effects of Large Deformation and Velocity Impacts on the Mechanical Behavior of Filled Rubber: Microstructure-Based Constitutive Modeling and Mechanical Testing. Polymers. 2020; 12(10):2322. https://doi.org/10.3390/polym12102322
Chicago/Turabian StyleWei, Wei, Yong Yuan, and Xiaoyu Gao. 2020. "Effects of Large Deformation and Velocity Impacts on the Mechanical Behavior of Filled Rubber: Microstructure-Based Constitutive Modeling and Mechanical Testing" Polymers 12, no. 10: 2322. https://doi.org/10.3390/polym12102322
APA StyleWei, W., Yuan, Y., & Gao, X. (2020). Effects of Large Deformation and Velocity Impacts on the Mechanical Behavior of Filled Rubber: Microstructure-Based Constitutive Modeling and Mechanical Testing. Polymers, 12(10), 2322. https://doi.org/10.3390/polym12102322





