Modelling of the Polymorph Nucleation Based on Classical Nucleation Theory
Abstract
:1. Introduction
2. Theory
3. Results and Discussion
4. Conclusions
Funding
Acknowledgments
Conflicts of Interest
Notation
| = initial concentration of solutes (molecules/cm3) |
| = saturated concentration of solutes (molecules/cm3) |
| = potential energy of the solute molecules (kJ/mole) |
| = potential energy of A-type crystals (kJ/mole) |
| = potential energy of B-type crystals (kJ/mole) |
| = activation energy for the association between A-type solute clusters (kJ/mole) |
| = activation energy for the association between B-type solute clusters (kJ/mole) |
| = critical nucleus size of A-type crystals (dimensionless) |
| = critical nucleus size of B-type crystals (dimensionless) |
| = association rate constant of A-type clusters (cm3/s) |
| = association rate constant of B-type clusters (cm3/s) |
| = collision rate constant (cm3/s) |
| = dissociation frequency factor (cm3/s) |
| = dissociation rate constant of (1/s) |
| = dissociation rate coefficient of A-type clusters (1/s) |
| = dissociation rate constant of (1/s) |
| = dissociation rate coefficient of B-type clusters (1/s) |
| = total number of molecules for per unit volume, (#/cm3) |
| = total number of molecules for per unit volume, (#/cm3) |
| = molecular weight (g/mol) |
| = Avogadro number (1/mol) |
| = number concentration of A-type clusters with solute molecules (#/cm3) |
| = number concentration of B-type clusters with solute molecules (#/cm3) |
| = total number concentration of A-type clusters (#/cm3) |
| = total number concentration of B-type clusters (#/cm3) |
| = net formation rate of due to association (#/cm3-s) |
| = net formation rate of due to dissociation (#/cm3-s) |
| = net formation rate of due to association (#/cm3-s) |
| = net formation rate of due to dissociation (#/cm3-s) |
| = supersaturation based on A-type crystals, |
| (dimensionless) |
| = supersaturation based on B-type crystals, |
| (dimensionless) |
| = temperature (K) |
| = time (s) |
| = experimental induction time (s) |
| = theoretical induction time (s) |
| = volume of a solute molecule (cm3) |
| = experimental weight fraction of A-type crystals (dimensionless) |
| = theoretical weight fraction of A-type crystals (dimensionless) Greek letters |
| = interfacial energy for form A (erg/cm2) |
| = interfacial energy for form B (erg/cm2) |
| = crystal density (g/cm3) |
| = average relative deviation between the experimental and theoretical induction time (dimensionless) |
| = average relative deviation between the experimental and theoretical weight fraction of A-type or B-type crystals (dimensionless). |
Appendix A
References
- Desiraju, G.R. Crystal Engineering: The Design of Organic Solids; Elsevier: New York, NY, USA, 1989. [Google Scholar]
- Bernstein, J. Polymorphism in Molecular Crystals; Oxford University Press: Oxford, UK, 2002. [Google Scholar]
- Randolph, A.D.; Larson, M.A. Theory of Particulate Processes; Academic Press, Inc.: San Diego, CA, USA, 1988. [Google Scholar]
- Mullin, J.W. Crystallization; Butterworth-Heinemann: Oxford, UK, 1993. [Google Scholar]
- Kashchiev, D. Nucleation: Basic Theory with Applications; Butterworth-Heinemann: Oxford, UK, 2000. [Google Scholar]
- Allen, A.T.; McDonald, M.P.; Nicol, W.M.; Wood, R.M. Athermal concentration gradients in supersaturated solutions of sucrose. Nature 1972, 235, 36–37. [Google Scholar] [CrossRef]
- Larson, M.A.; Garside, J. Solute clustering in supersaturated solutions. Chem. Eng. Sci. 1986, 41, 1285–1289. [Google Scholar] [CrossRef]
- Tanaka, S.; Ito, K.; Hayakawa, R.; Ataka, M. Size and number density of precrystalline aggregates in lysozyme crystallization process. J. Chem. Phys. 1999, 111, 10330–10337. [Google Scholar] [CrossRef]
- Yau, S.T.; Vekilov, P.G. Quasi-planar nucleus structure in apoferritin crystallization. Nature 2000, 406, 494–497. [Google Scholar] [CrossRef] [PubMed]
- Chattopadhyay, S.; Erdemir, D.; Evans, J.M.B.; Ilavsky, J.; Amenitsch, H.; Segre, C.U.; Myerson, A.S. SAXS study of the nucleation of glycine crystals from a supersaturated solution. Cryst. Growth Des. 2005, 5, 523–527. [Google Scholar] [CrossRef]
- Weissbuch, I.; Lahav, M.; Leiserowitz, L. Toward stereochemical control, monitoring, and understanding of crystal nucleation. Cryst. Growth Des. 2003, 3, 125–150. [Google Scholar] [CrossRef]
- Torbeev, V.Y.; Shavit, E.; Weissbuch, I.; Leiserowitz, L.; Lahav, M. Control of crystal polymorphism by tuning the structure of auxiliary molecules as nucleation inhibitors. The β-polymorph of glycine grown in aqueous solutions. Cryst. Growth Des. 2005, 5, 2190–2196. [Google Scholar] [CrossRef]
- Van Driessche, A.E.S.; Van Gerven, N.; Bomans, P.H.H.; Joosten, R.R.M.; Friedrich, H.; Gil-Carton, D.; Sommerdijk, N.A.J.M.; Sleutel, M. Molecular nucleation mechanisms and control strategies for crystal polymorph selection. Nature 2018, 556, 89–94. [Google Scholar] [CrossRef]
- Chakraborty, D.; Bhatia, S.K. Formation and agglomeration of polymorphs in continuous precipitation. 1. Mathematical modeling. Ind. Eng. Chem. Res. 1996, 35, 1985–1994. [Google Scholar] [CrossRef]
- Ter Horst, J.H.; Kramer, H.J.M.; Jansens, P.J. A new molecular modeling approach to predict concomitant nucleation of polymorphs. Cryst. Growth Des. 2002, 2, 351–356. [Google Scholar] [CrossRef]
- Blagden, N.; Davey, R.J. Polymorph Selection: Challenges for the Future? Cryst. Growth Des. 2003, 3, 873–885. [Google Scholar] [CrossRef]
- Madras, G.; McCoy, B.J. Growth and Ripening Kinetics of Crystalline Polymorphs. Cryst. Growth Des. 2003, 3, 981–990. [Google Scholar] [CrossRef]
- Kitamura, M. Controlling factors and mechanism of polymorphic crystallization. Cryst. Growth Des. 2004, 4, 1153–1159. [Google Scholar] [CrossRef]
- Gracin, S.; Uusi-Penttila, M.; Rasmuson, A.C. Influence of ultrasound on the nucleation of polymorphs of p-aminobenzoic acid. Cryst. Growth Des. 2005, 5, 1787–1794. [Google Scholar] [CrossRef]
- Chen, S.; Xi, H.; Yu, L. Cross Nucleation between ROY Polymorphs. J. Am. Chem. Soc. 2005, 127, 17439–17444. [Google Scholar] [CrossRef] [PubMed]
- Mendez Del Rio, J.R.; Rousseau, R.W. Batch and tubular-batch crystallization of paracetamol: Crystal size distribution and polymorph formation. Cryst. Growth Des. 2006, 6, 1407–1414. [Google Scholar] [CrossRef]
- Desgranges, C.; Delhommelle, J. Polymorph selection during the crystallization of Yukawa systems. J. Chem. Phys. 2007, 126, 054501. [Google Scholar] [CrossRef]
- Barthe, S.C.; Grover, M.A.; Rousseau, R.W. Observation of polymorphic change through analysis of FBRM data: Transformation of paracetamol from form II to form I. Cryst. Growth Des. 2008, 8, 3316–3322. [Google Scholar] [CrossRef]
- Mangin, D.; Puel, F.; Veesler, S. Polymorphism in processes of crystallization in solution: A practical review. Org. Process Res. Dev. 2009, 13, 1241–1253. [Google Scholar] [CrossRef]
- Hall, V.J.; Simpson, G.J. Direct Observation of Transient Ostwald Crystallization Ordering from Racemic Serine Solutions. J. Am. Chem. Soc. 2010, 132, 13598–13599. [Google Scholar] [CrossRef]
- Ike, Y.; Hirasawa, I. Polymorph control of L-phenylalanine in cooling crystallization by ultrasonication. Chem. Eng. Technol. 2018, 41, 1093–1097. [Google Scholar] [CrossRef]
- Rimez, B.; Conte, J.; Lecomte-Norrant, E.; Cognet, P.; Gourdon, C.; Scheid, B. Continuous-flow tubular crystallization to discriminate between two competing crystal polymorphs. 2. Antisolvent crystallization. Cryst. Growth Des. 2018, 18, 6440–6447. [Google Scholar] [CrossRef]
- Narsimhan, G.; Ruckenstein, E. A new approach for the prediction of the rate of nucleation in liquids. J. Colloid Interface Sci. 1989, 128, 549–565. [Google Scholar] [CrossRef]
- Ruckenstein, E.; Karpe, P. On the enzymatic superactivity in ionic reverse micelles. J. Colloid Interface Sci. 1990, 139, 408–436. [Google Scholar] [CrossRef]
- Ruckenstein, E.; Berim, G.O. Kinetics of heterogeneous nucleation on a rough surface: Nucleation of a liquid phase in nanocavities. J. Colloid Interface Sci. 2010, 351, 277–282. [Google Scholar] [CrossRef] [PubMed]
- Hammond, R.B.; Pencheva, K.; Roberts, K.J. Simulation of energetic stability of facetted L-glutamic acid nanocrystalline clusters in relation to their polymorphic phase stability as a function of crystal size. J. Phys. Chem. B 2005, 109, 19550–19552. [Google Scholar] [CrossRef]
- Ter Horst, J.H.; Kramer, H.J.M.; Jansens, P.J. Towards a crystalline product quality prediction method by combining process modeling and molecular simulations. Chem. Eng. Technol. 2006, 29, 175–181. [Google Scholar] [CrossRef]
- Deij, M.A.; ter Horst, J.H.; Meekes, H.; Jansens, P.; Vlieg, E. Polymorph formation studied by 3D nucleation simulations. Application to a yellow isoxazolone dye, paracetamol, and L-glutamic acid. J. Phys. Chem. B 2007, 111, 1523–1530. [Google Scholar] [CrossRef]
- Lu, J.; Rohani, S. Polymorphism and crystallization of active pharmaceutical ingredients (APIs). Curr. Med. Chem. 2009, 16, 884–905. [Google Scholar] [CrossRef]
- Ni, X.; Liao, A. Effects of cooling rate and solution concentration on solution crystallization of L-glutamic acid in an oscillatory baffled crystallizer. Cryst. Growth Des. 2008, 8, 2875–2881. [Google Scholar] [CrossRef]
- Qu, H.; Alatalo, H.; Hatakka, H.; Kohonen, J.; Louhi-Kultanen, M.; Reinikainen, S.P.; Kallas, J. Raman and ATR FTIR spectroscopy in reactive crystallization: Simultaneous monitoring of solute concentration and polymorphic state of the crystals. J Cryst. Growth 2009, 311, 3466–3475. [Google Scholar] [CrossRef]
- Sun, X.; Garetz, B.A.; Myerson, A.S. Supersaturation and polarization dependence of polymorph control in the nonphotochemical laser-induced nucleation (NPLIN) of aqueous glycine solutions. Cryst. Growth Des. 2006, 6, 684–689. [Google Scholar] [CrossRef]
- Gracin, S.; Rasmuson, A.C. Polymorphism and crystallization of p-aminobenzoic acid. Cryst. Growth Des. 2004, 4, 1013–1023. [Google Scholar] [CrossRef]
- Teychene, S.; Biscans, B. Nucleation kinetics of polymorphs: Induction period and interfacial energy measurements. Cryst. Growth Des. 2008, 8, 1133–1139. [Google Scholar] [CrossRef]
- Zhu, L.; Wang, L.Y.; Sha, Z.L.; Wang, Y.F.; Yang, L.B.; Zhu, X.Y.; Du, W. Interplay between thermodynamics and kinetics on polymorphic appearance in the solution crystallization of an enantiotropic system. Cryst. Growth Des. 2017, 17, 4582–4595. [Google Scholar] [CrossRef]
- Roelands, C.P.M.; Jiang, S.; Kitamura, M.; ter Horst, J.H.; Kramer, H.J.M.; Jansens, P.J. Antisolvent crystallization of the polymorphs of L-histidine as a function of supersaturation ratio an of solvent composition. Cryst. Growth Des. 2006, 6, 955–963. [Google Scholar] [CrossRef]
- Wantha, L.; Punmalee, N.; Sawaddiphol, V.; Flood, A.E. Effect of ethanol on crystsllization of the polymorphs of L-histidine. J. Cryst. Growth 2018, 490, 65–70. [Google Scholar] [CrossRef]
- Stranski, I.N.; Totomanov, D. Rate of formation of (crystal) nuclei and the Ostwald step rule. Z. Phys. Chem. 1933, 163, 399–408. [Google Scholar]
- Tahri, Y.; Kozisek, Z.; Gagniere, E.; Chabanon, E.; Bounahmidi, T.; Mangin, D. Modeling the competition between polymorphic phases: Highlights on the effect of Ostwald ripening. Cryst. Growth Des. 2016, 16, 5689–5697. [Google Scholar] [CrossRef]
- Scholl, J.; Bonalumi, D.; Vicum, L.; Mazzotti, M. In situ monitoring and modeling of the solvent-mediated polymorphic transformation of L-glutamic acid. Cryst. Growth Des. 2006, 6, 881–891. [Google Scholar] [CrossRef]
- Kee, N.C.S.; Arendt, P.D.; Tan, R.B.H.; Braatz, R.D. Selective crystallization of the metastable anhydrate form in the enantiotropic pseudo-dimorph system of L-phenylalanine using concentration feedback control. Cryst. Growth Des. 2009, 9, 3052–3061. [Google Scholar] [CrossRef]
- Rimez, B.; Debuysschere, R.; Conte, J.; Lecomte-Norrant, E.; Gourdon, C.; Cogent, P.; Scheid, B. Continuous-flow tubular crystallization to discriminate between two competing crystal polymorphs. 1. Cooling crystallization. Cryst. Growth Des. 2018, 18, 6431–6439. [Google Scholar] [CrossRef]
- Smoluchowski, M. Versuch einer mathematischen Theorie der Koagulationskinetic kolloider Lösungen. Z. Phys. Chem. 1917, 92, 129–168. [Google Scholar]
- Farkas, L. Keimbildnngsgeschwindigkeit in übersättigten dämpfen. Z. Phys. Chem. 1927, 125, 236–242. [Google Scholar]
- Shiau, L.D.; Lu, Y.F. Modeling solute clustering in the diffusion layer around a growing crystal. J. Chem. Phys. 2009, 130, 094105. [Google Scholar] [CrossRef] [PubMed]
- Mersmann, A.; Bartosch, K. How to predict the metastable zone width. J. Cryst. Growth 1998, 183, 240–250. [Google Scholar] [CrossRef]
- Lindenberg, C.; Mazzotti, M. Effect of temperature on the nucleation kinetics of α L-glutamic acid. J. Cryst. Growth 2009, 311, 1178–1184. [Google Scholar] [CrossRef]
- Shiau, L.D.; Lu, T.S. A model for determination of the interfacial energy from the induction time or metastable zone width data based on turbidity measurements. CrystEngComm 2014, 16, 9743–9752. [Google Scholar] [CrossRef]
- Shiau, L.D. Determination of the nucleation and growth kinetics from aqueous L-glycine solutions from the turbidity induction time data. Crystals 2018, 8, 403. [Google Scholar] [CrossRef]
- Shiau, L.D. The temperature dependence of the pre-exponential factor and interfacial energy for aqueous glycine solutions based on the metastable zone width data. J. Cryst. Growth 2018, 496–497, 18–23. [Google Scholar] [CrossRef]
- Vekilov, P.G. The two-step mechanism of nucleation of crystals in solution. Nanoscale 2010, 2, 2346–2357. [Google Scholar] [CrossRef] [PubMed]
- Qian, R.Y.; Botsaris, G.D. A new mechanism for nuclei formation in suspension crystallizers: The role of interparticle forces. Chem. Eng. Sci. 1997, 52, 3429–3440. [Google Scholar] [CrossRef]
- Lee, G.W. Formation of metastable crystals from supercooled, supersaturated, and supercompressed liquids: Role of crystal-liquid interfacial free energy. Crystals 2017, 7, 326. [Google Scholar] [CrossRef]

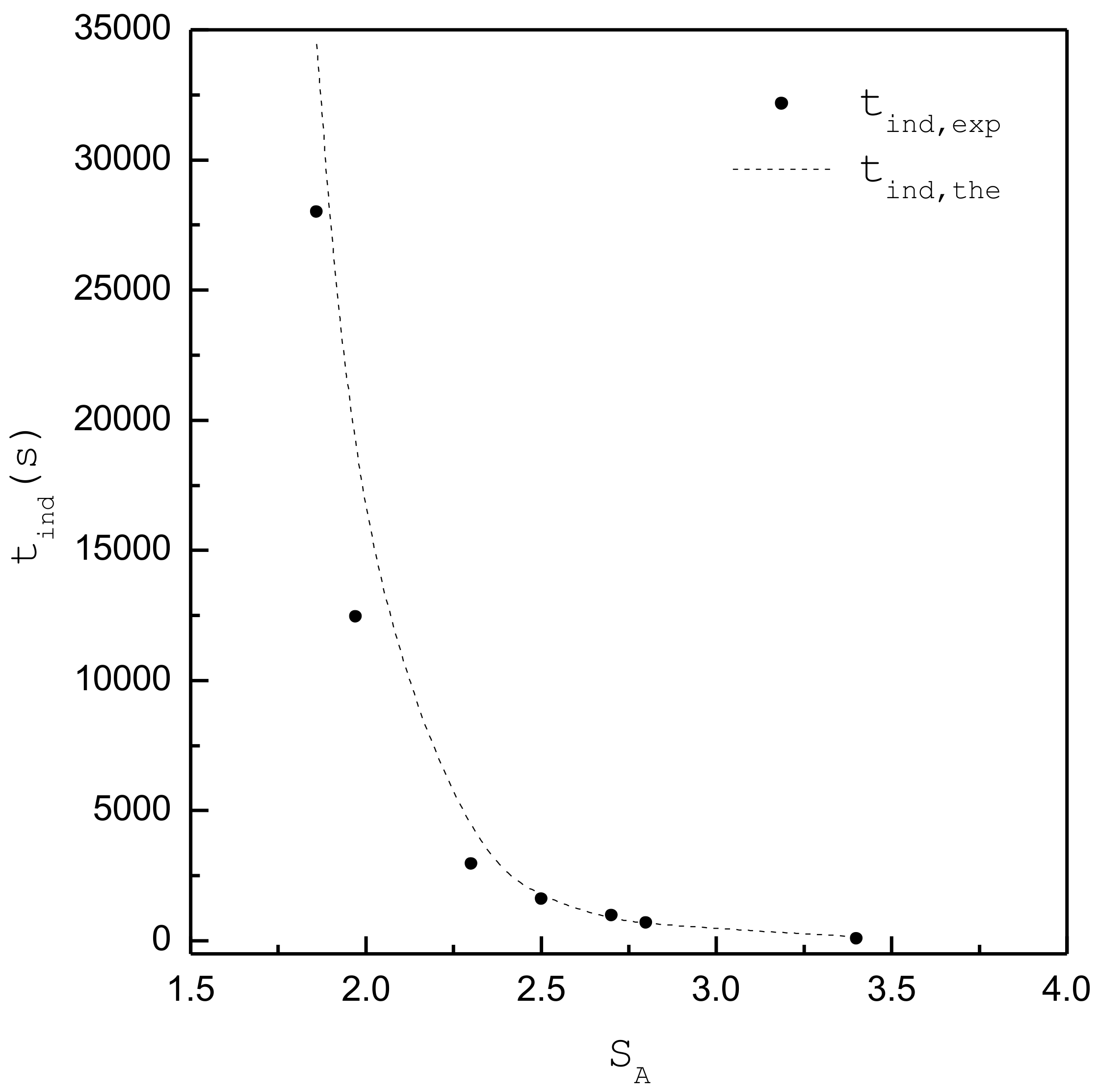
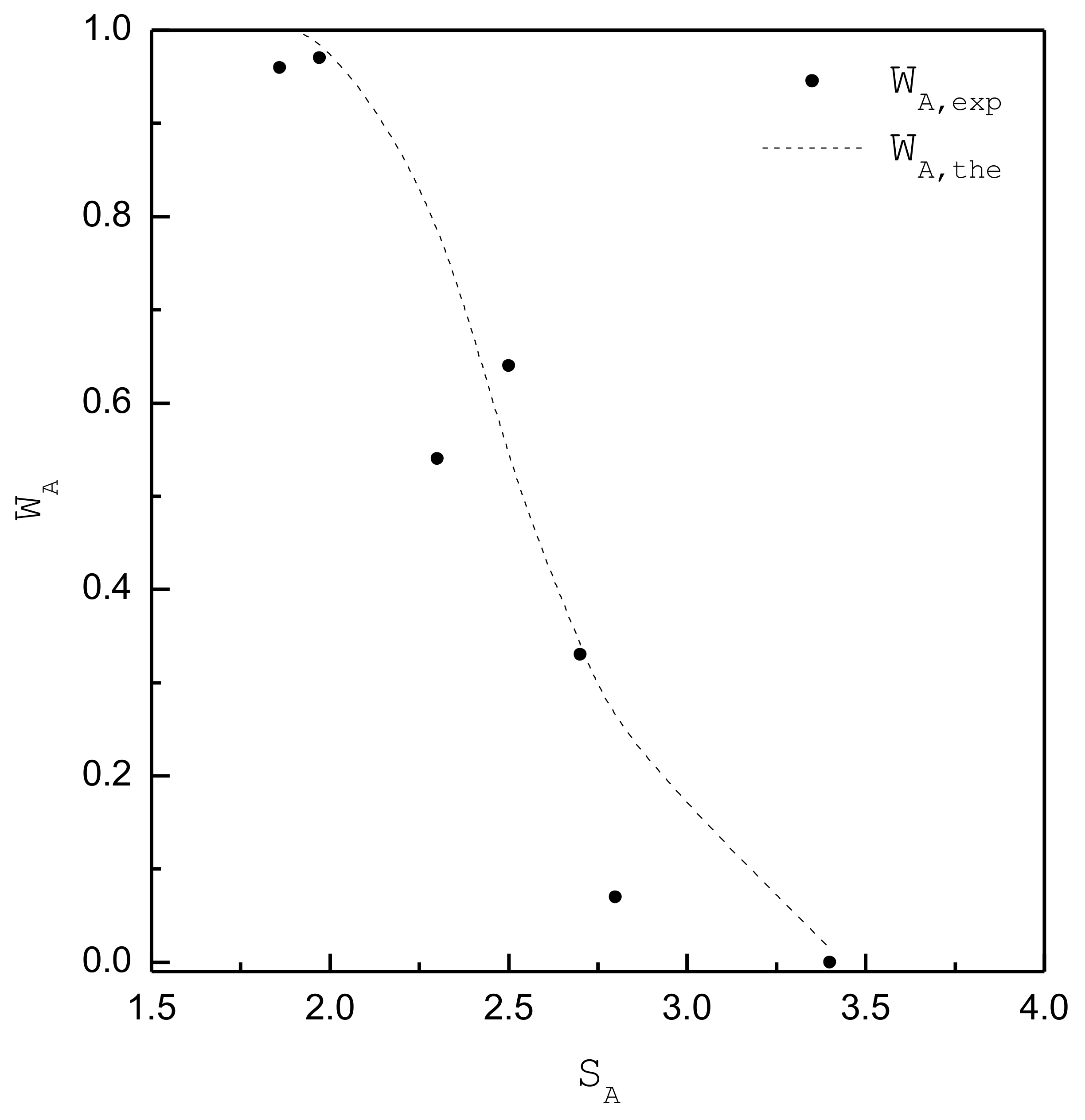
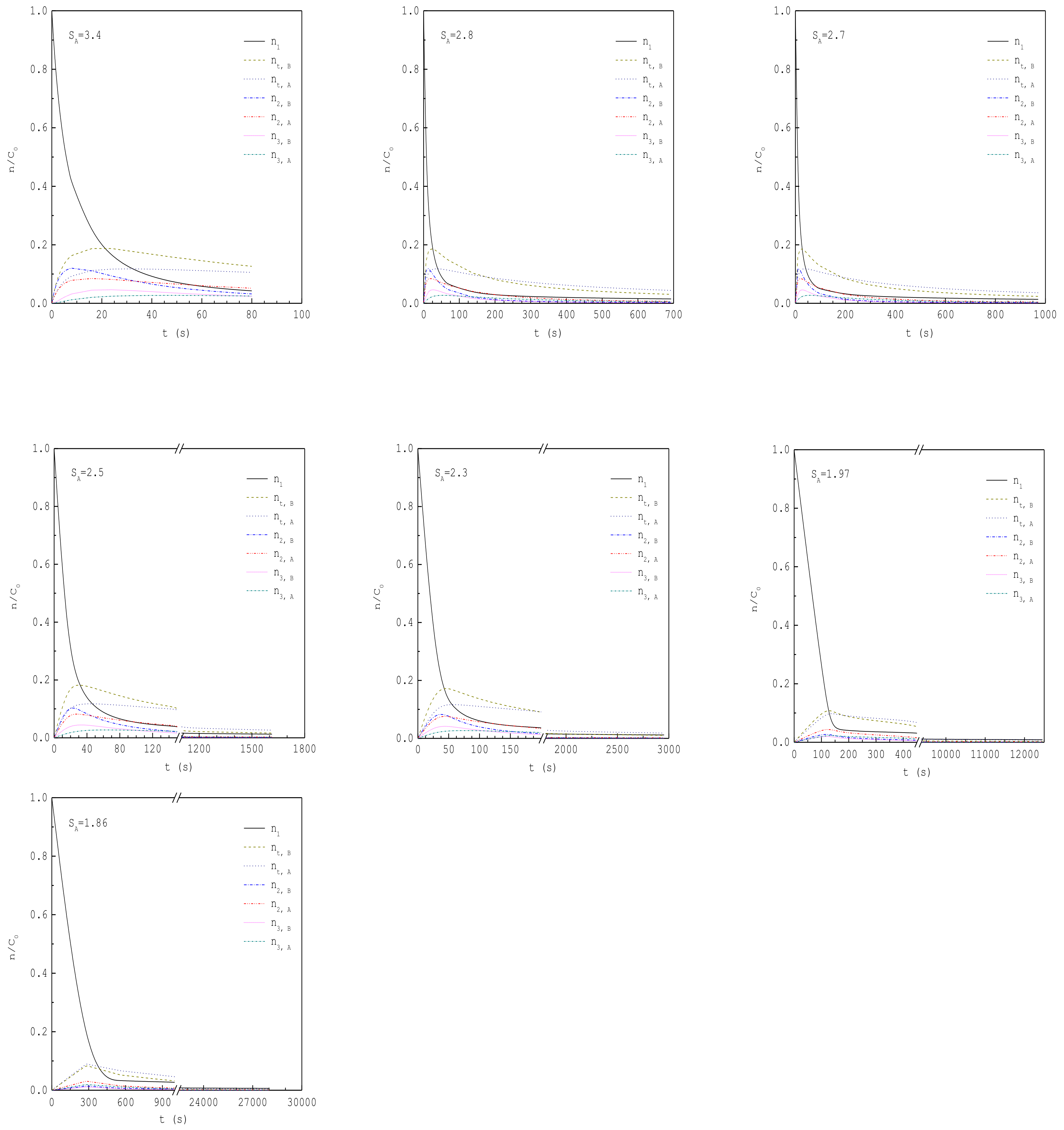
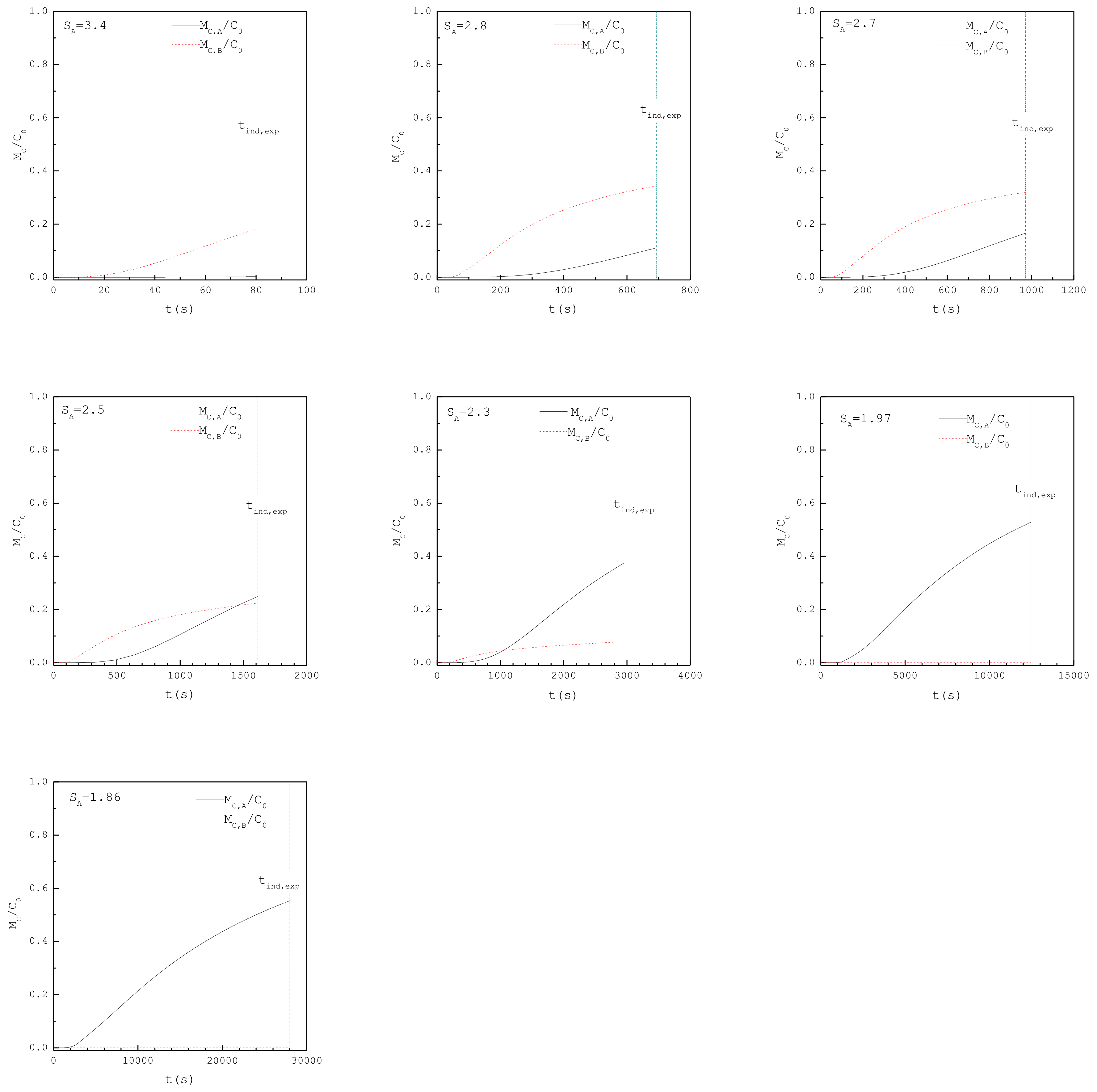
| Association and Dissociation Processes |
|---|
| Polymorphic Form | |||||||
|---|---|---|---|---|---|---|---|
| 1.86 | 1.66 | 3.94 × 1019 | 28000 | 91 | 92 | 0.96 | A |
| 1.97 | 1.76 | 4.18 × 1019 | 12456 | 70 | 67 | 0.97 | A |
| 2.3 | 2.05 | 4.88 × 1019 | 2954 | 38 | 32 | 0.54 | A+B |
| 2.5 | 2.23 | 5.30 × 1019 | 1614 | 28 | 23 | 0.64 | A+B |
| 2.7 | 2.41 | 5.72 × 1019 | 971 | 22 | 18 | 0.33 | A+B |
| 2.8 | 2.50 | 5.94 × 1019 | 693 | 20 | 16 | 0.07 | ~B |
| 3.4 | 3.03 | 7.21 × 1019 | 80 | 12 | 9 | 0 | B |
| 7.7 10−22 | 1.4 10−21 | 5.3 10−4 | 3.2 10−3 | 0.22 | 0.14 |
© 2019 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shiau, L.-D. Modelling of the Polymorph Nucleation Based on Classical Nucleation Theory. Crystals 2019, 9, 69. https://doi.org/10.3390/cryst9020069
Shiau L-D. Modelling of the Polymorph Nucleation Based on Classical Nucleation Theory. Crystals. 2019; 9(2):69. https://doi.org/10.3390/cryst9020069
Chicago/Turabian StyleShiau, Lie-Ding. 2019. "Modelling of the Polymorph Nucleation Based on Classical Nucleation Theory" Crystals 9, no. 2: 69. https://doi.org/10.3390/cryst9020069
APA StyleShiau, L.-D. (2019). Modelling of the Polymorph Nucleation Based on Classical Nucleation Theory. Crystals, 9(2), 69. https://doi.org/10.3390/cryst9020069





