Raman Spectra of Quartz and Pb4+-Doped SiO2 Crystals at Different Temperature and Pressure
Abstract
:1. Introduction
2. Materials and Methods
2.1. The Structures of Raman Crystals
2.2. Computational Methods for Raman Spectrum
3. Results
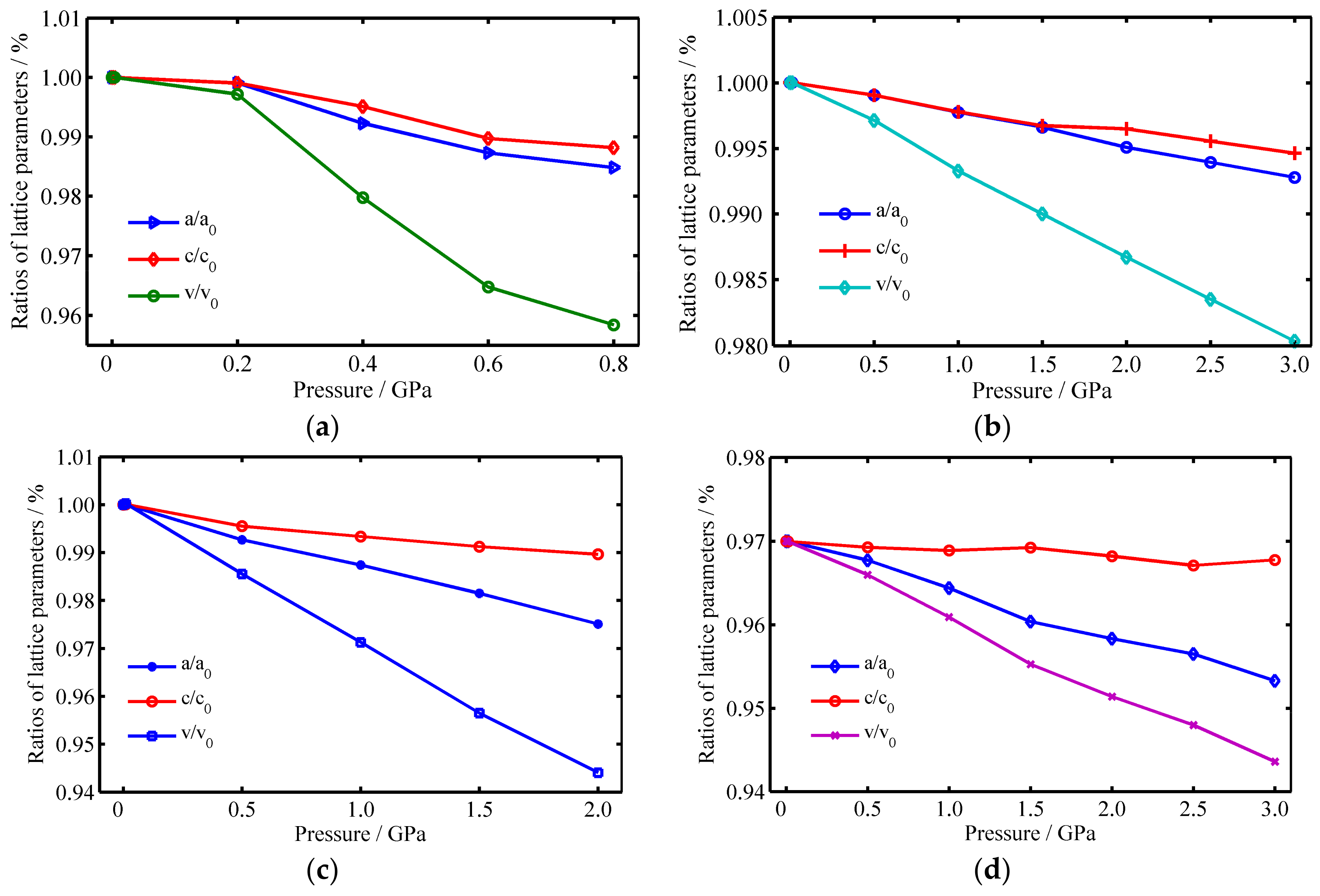
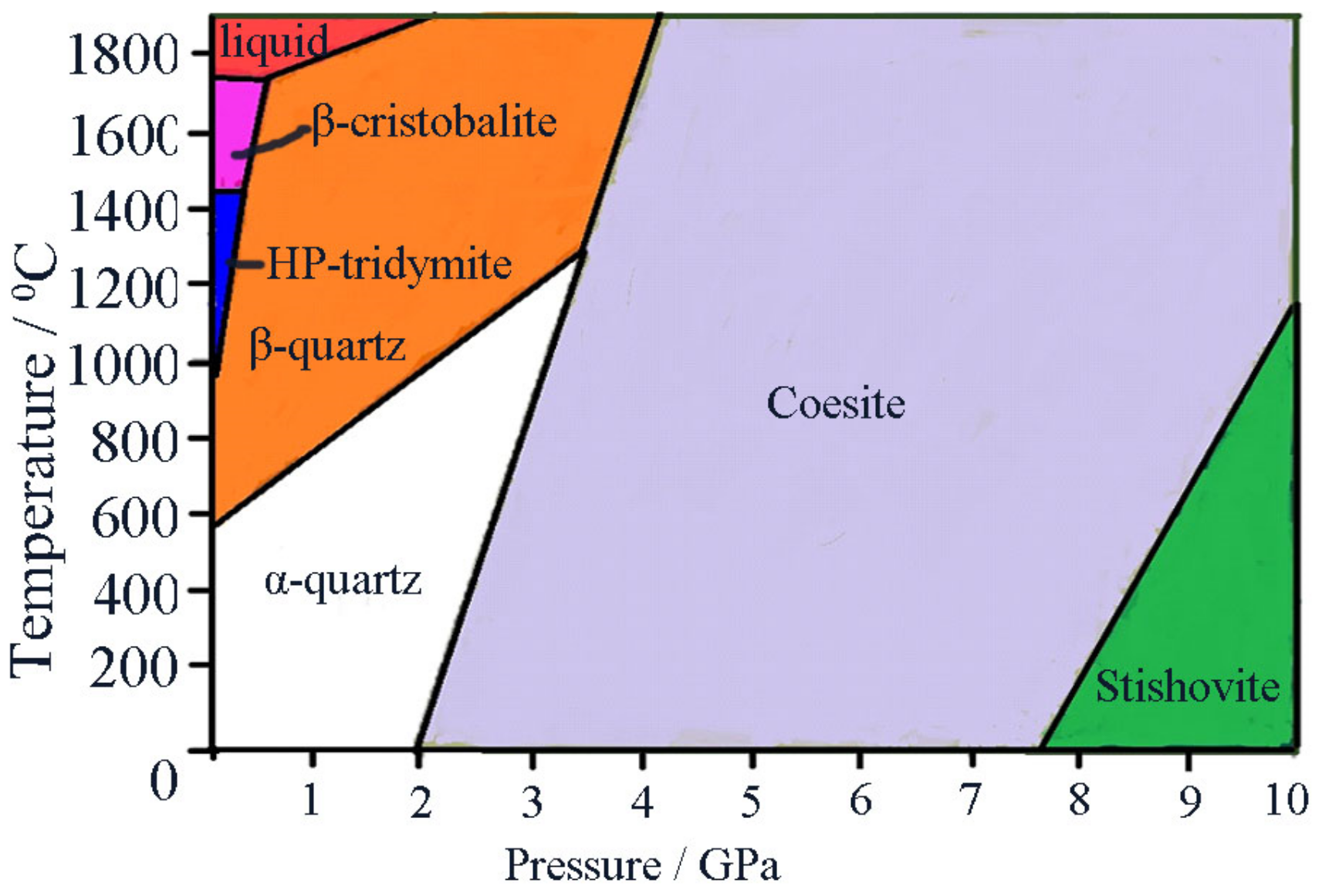
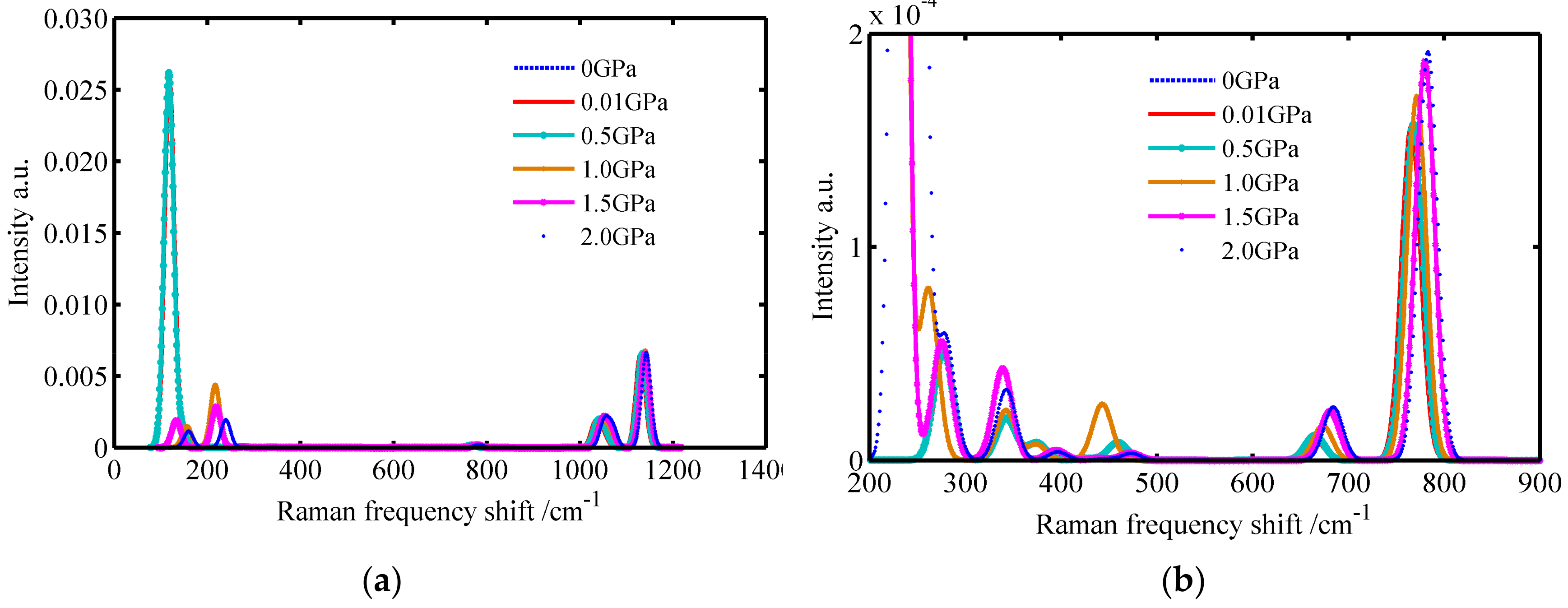
3.1. The Raman Spectrums of SiO2 Crystals
3.2. The Raman Spectrums of Pb4+-Doped SiO2 Crystals
4. Discussion and Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Dick, S.; Bell, S. Quantitative surface-enhanced Raman spectroscopy of single bases in oligodeoxynucleotides. Faraday Discuss. 2017, 37, 1012–1024. [Google Scholar] [CrossRef] [PubMed]
- Ding, S.Y.; You, E.M.; Tian, Z.Q.; Moskovits, M. Electromagnetic theories of surface-enhanced Raman spectroscopy. Chem. Soc. Rev. 2017, 46, 4042–4076. [Google Scholar] [CrossRef] [PubMed]
- Nishizawa, J.; Suto, K. Semiconductor Raman laser. J. Appl. Phys. 1980, 51, 2429–2431. [Google Scholar] [CrossRef]
- Basiev, T.T.; Zverev, P.G.; Karasik, A.Y.; Osiko, V.V.; Sobol, A.A.; Chunaev, D.S. Picosecond stimulated Raman scattering in crystals. J. Exp. Theor. Phys. 2005, 99, 934–941. [Google Scholar] [CrossRef]
- Frank, M.; Snetanin, S.N.; Jelinek, M.; Vyhlidal, D.; Shukshin, V.E.; Ivleva, L.I.; Zverev, P.G.; Kubecek, V. Efficient synchronously-pumped all-solid-state Raman laser at 1178 and 1227 nm on stretching and bending anionic group vibrations in a SrWO4 crystal with pulse shortening down to 1.4 ps. Opt. Laser Technol. 2019, 119, 105660. [Google Scholar] [CrossRef]
- Shinya, I.; Jun, S.; Tsutomu, S.; Yushi, S. Lattice dynamics and temperature dependence of the first-order Raman spectra for α-SiO2 crystals. J. Raman Spectrosc. 2003, 34, 135–141. [Google Scholar]
- Guo, J.; Ma, L. Atomic simulation of basic properties forα-SiO2 crystal. Mater. Sci. 2019, 9, 355–360. [Google Scholar]
- Krishnana, R.S. The scattering of light in fused quartz and its Raman spectrum. Proc. Math. Sci. 1953, 37, 377–384. [Google Scholar] [CrossRef]
- Nitin, R.K.; James, R.C. Structural properties of nine silica polymorphs. Phys. Rev. B 1992, 46, 1. [Google Scholar]
- Bishambhar, D.S. Calculation of the elastic constants of quartz at room temperature from the Raman effect data. Proc. Indian Acad. Sci.-Sect. A 1944, 19, 367–369. [Google Scholar]
- Jayaraman, A.; Wood, D.L.; Maines, R.G. High-Pressure Raman study of the vibrational modes in AIPO4 and SiO2 (α-quartz). Phys. Rev. Lett. 1987, 35, 8316–8321. [Google Scholar]
- Nedungadi, T.M.K. Effect of temperature on the Raman spectrum of quartz. Proc. Indian Acad. Sci.-Sect. A 1940, 11, 86–95. [Google Scholar] [CrossRef]
- Chen, W.B.; Inagawa, Y.J.; Omatsu, T.; Tateda, M.; Takeuchi, N.; Usuki, Y. Diode-Pumped, self-stimulating, passively Q-switched Nd3+: PbWO4 Raman laser. Opt. Commun. 2001, 194, 401–407. [Google Scholar] [CrossRef]
- Jia, H.X.; Ding, S.H.; Liu, J.J.; Ding, Z. Laser-diode-pumped Cr4+: YAG passively Q-switched Intracavity PbWO4 mode-locked Raman laser. Chin. J. Lasers 2014, 41, 7. [Google Scholar]
- Baidu Wenku. Available online: https://wenku.baidu.com/view/af779d3a87c24028915fc3f6.html (accessed on 25 July 2019).
- Li, Y.; Yan, S.Y.; Chen, Y. Study of quartz Raman spectrum at high pressure and room temperature. Spectrosc. Spectr. Anal. 2011, 31, 2120–2122. [Google Scholar]
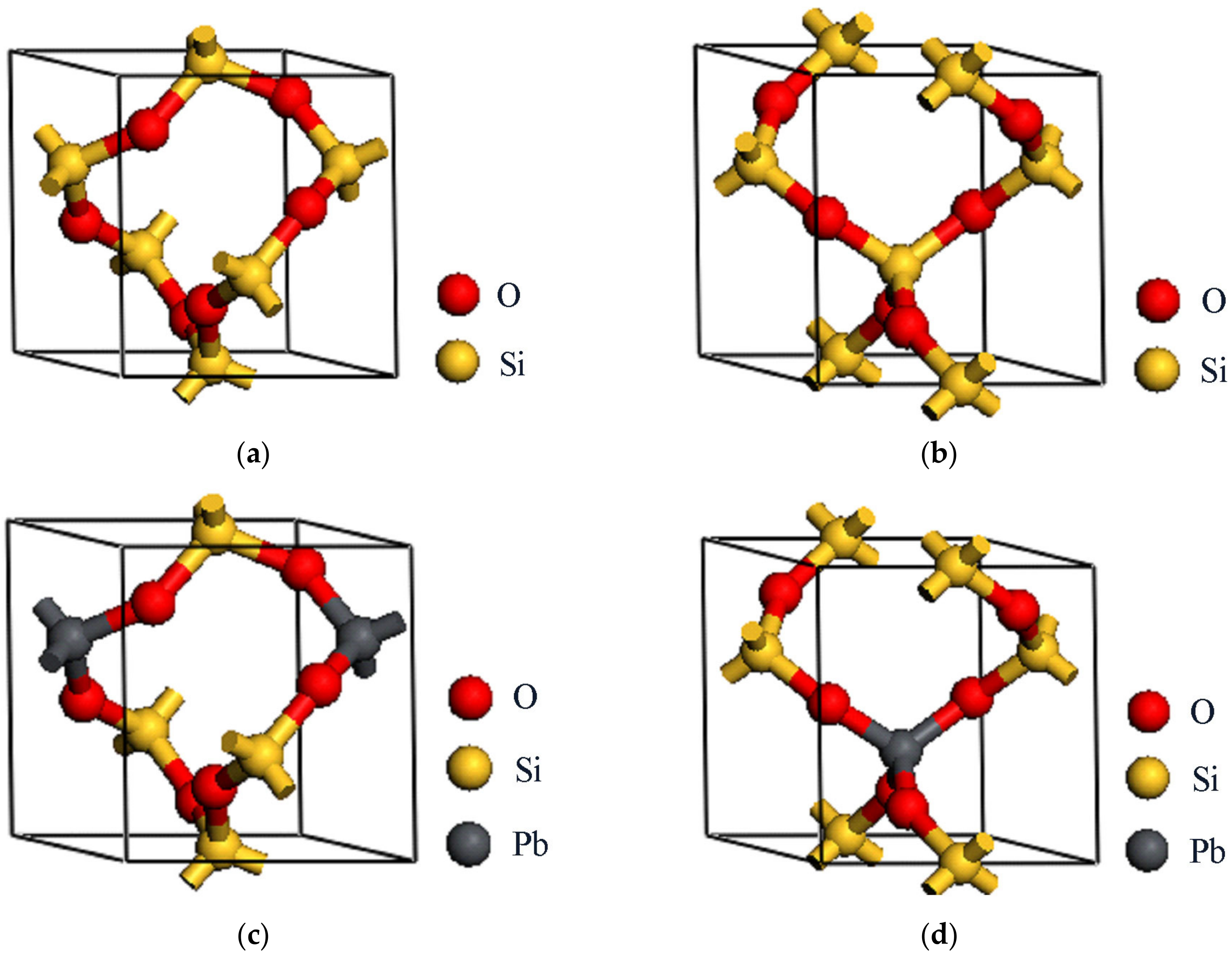
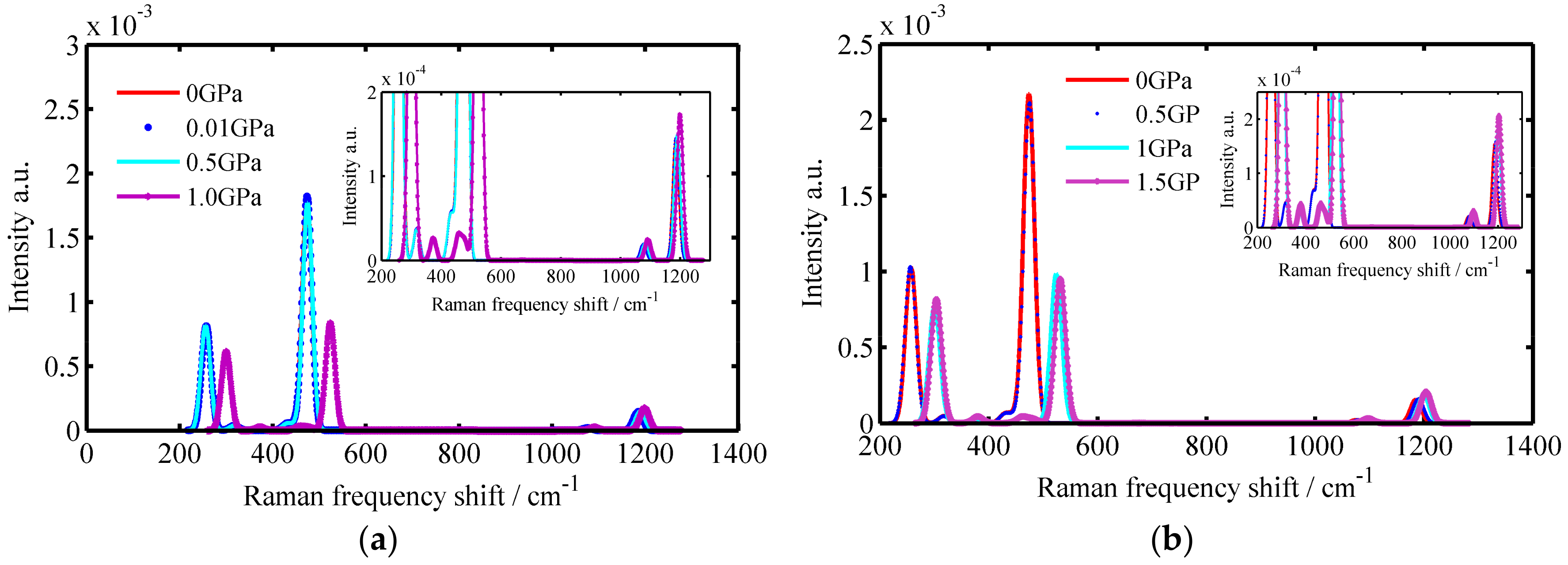
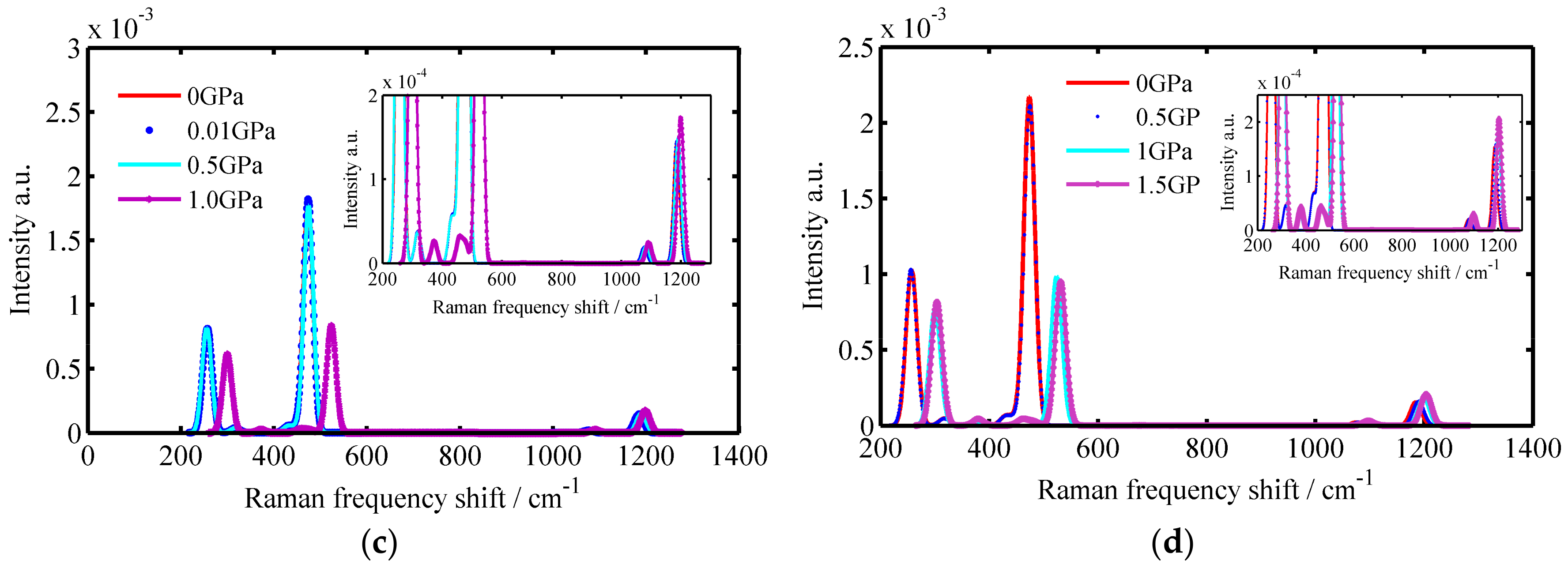
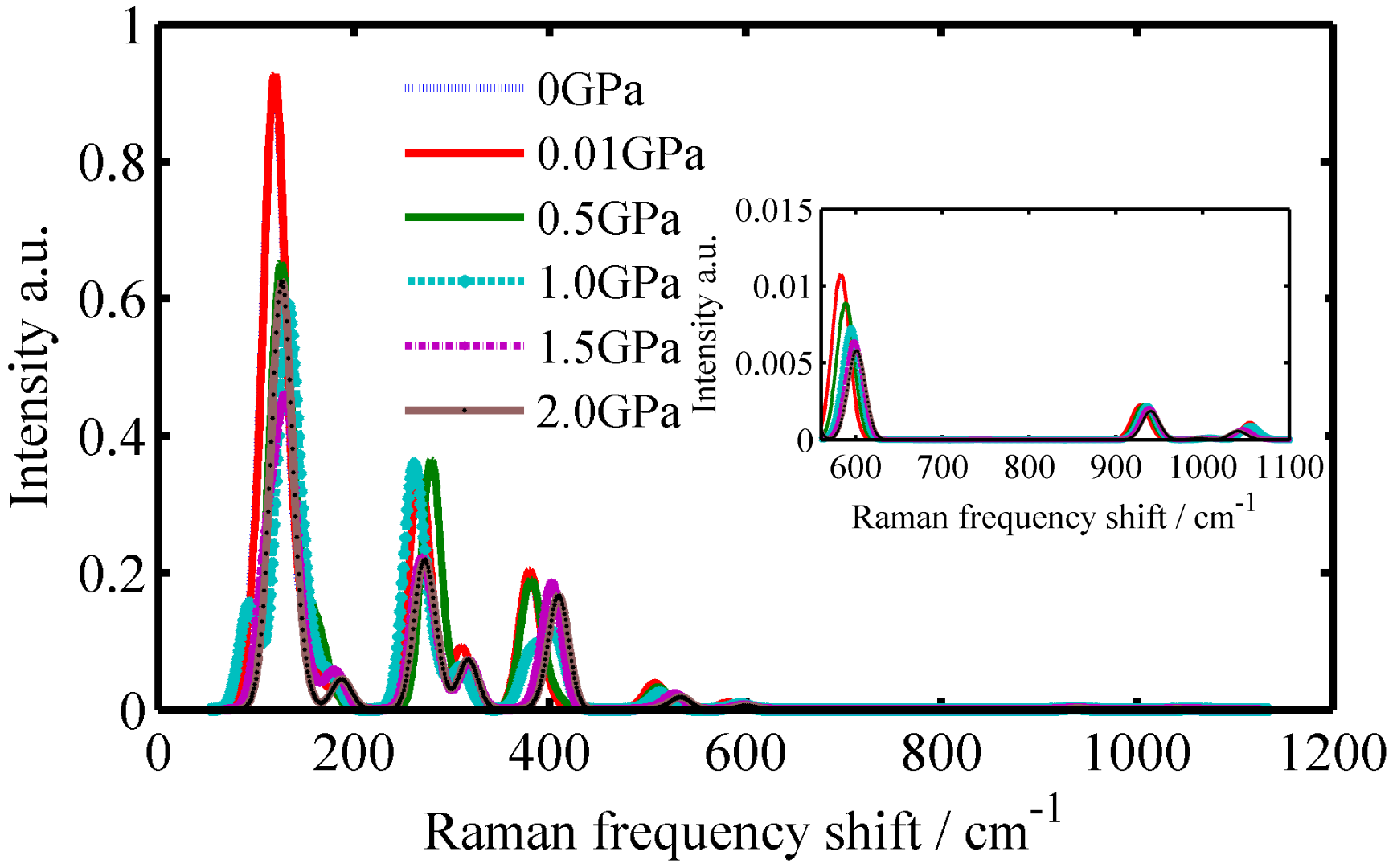
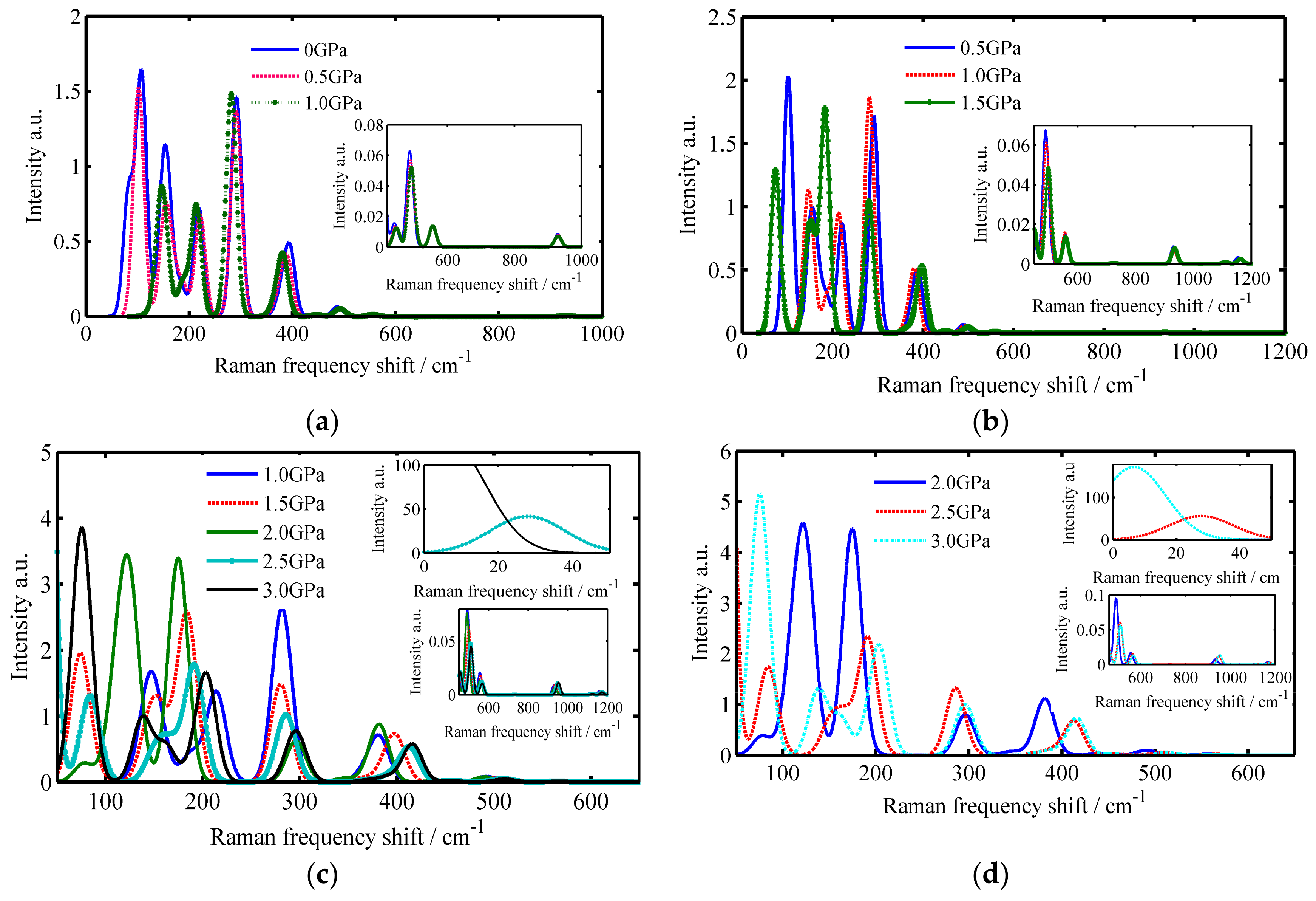
| Crystals | Temperature/K | Raman Spectrums/cm−1 |
|---|---|---|
| α-SiO2 | 127 | 117, 135, 241, 343, 686, 782, 1055, 1144 |
| β-SiO2 | 1527 | 221, 458, 1101, 1206 |
| Pb4+-doped α-SiO2 | 127 | 270, 382, 532, 600, 938, 1055 |
| Pb4+-doped β-SiO2 | 527 | 148, 107 216, 282, 381, 445, 487, 559, 930 |
| Pb4+-doped β-SiO2 | 727 | 75, 102, 145, 183, 278, 396, 446, 502, 558, 936, 1161 |
| Pb4+-doped β-SiO2 | 1127 | 8, 29, 75, 121, 176, 282, 382, 448, 492, 555, 937, 1168 |
| Pb4+-doped β-SiO2 | 1527 | 7, 28, 75, 121, 173, 295, 381,440, 492, 558, 933, 1166 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, X.; Feng, W.; Zhang, G.; Gao, Y. Raman Spectra of Quartz and Pb4+-Doped SiO2 Crystals at Different Temperature and Pressure. Crystals 2019, 9, 569. https://doi.org/10.3390/cryst9110569
Chen X, Feng W, Zhang G, Gao Y. Raman Spectra of Quartz and Pb4+-Doped SiO2 Crystals at Different Temperature and Pressure. Crystals. 2019; 9(11):569. https://doi.org/10.3390/cryst9110569
Chicago/Turabian StyleChen, Xiuyan, Wenjiang Feng, Guoying Zhang, and Yan Gao. 2019. "Raman Spectra of Quartz and Pb4+-Doped SiO2 Crystals at Different Temperature and Pressure" Crystals 9, no. 11: 569. https://doi.org/10.3390/cryst9110569
APA StyleChen, X., Feng, W., Zhang, G., & Gao, Y. (2019). Raman Spectra of Quartz and Pb4+-Doped SiO2 Crystals at Different Temperature and Pressure. Crystals, 9(11), 569. https://doi.org/10.3390/cryst9110569





