Disassembly of Faceted Macrosteps in the Step Droplet Zone in Non-Equilibrium Steady State
Abstract
:1. Introduction
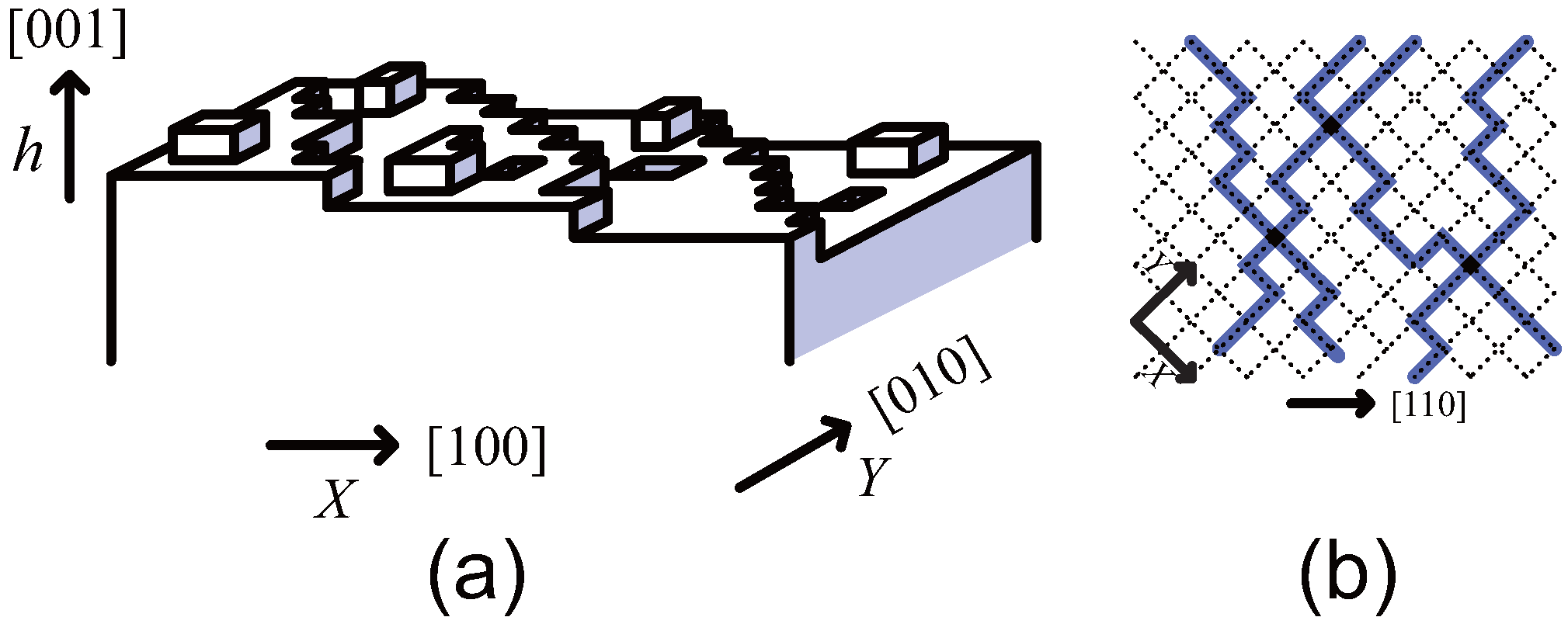
2. Restricted Solid-on-Solid Model with Point-Contact-Type Step–Step Attraction
2.1. Restricted Solid-on-Solid Model with Point-Contact-Type Step–Step Attraction
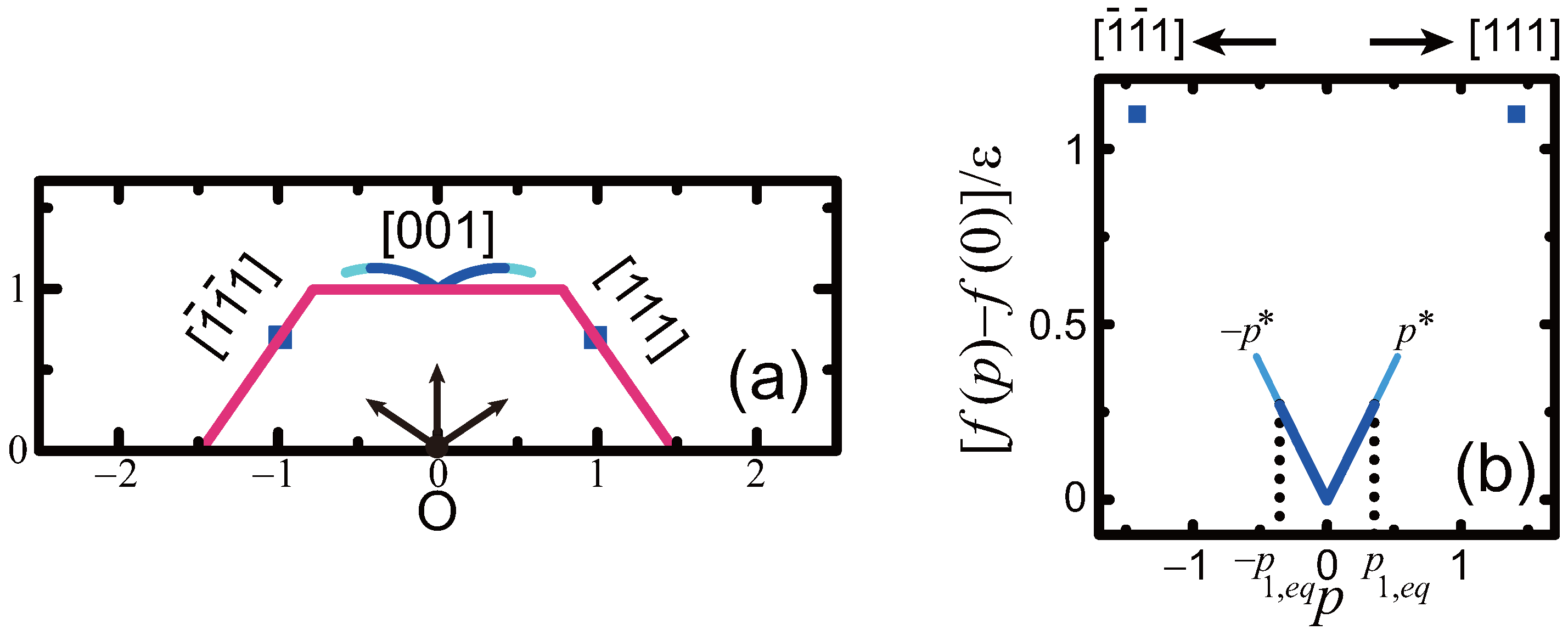
2.2. Discontinuous Surface Tension
3. Monte Carlo Results
3.1. Monte Carlo Method
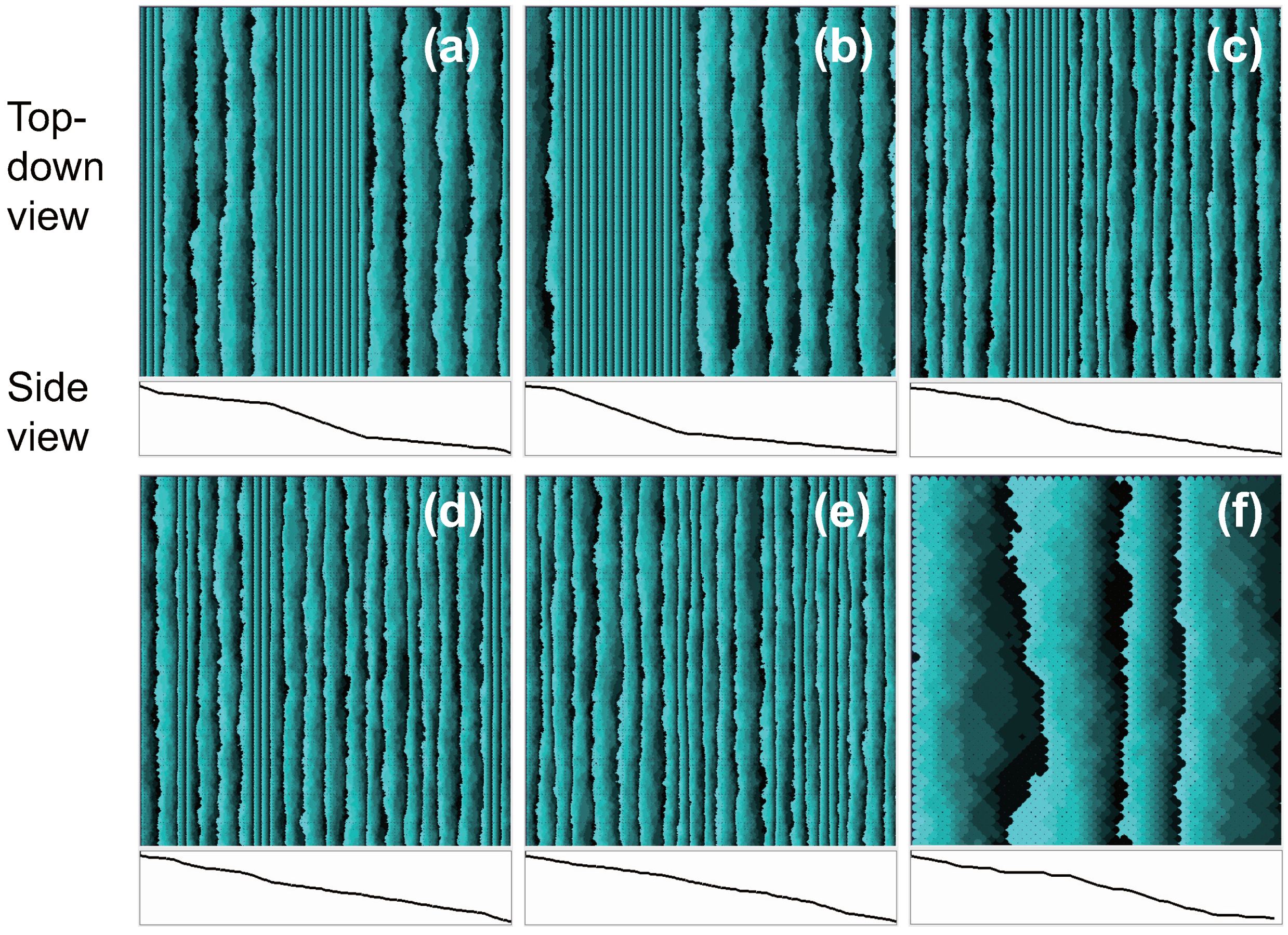
3.2. Macrostep Size and Surface Growth Rate
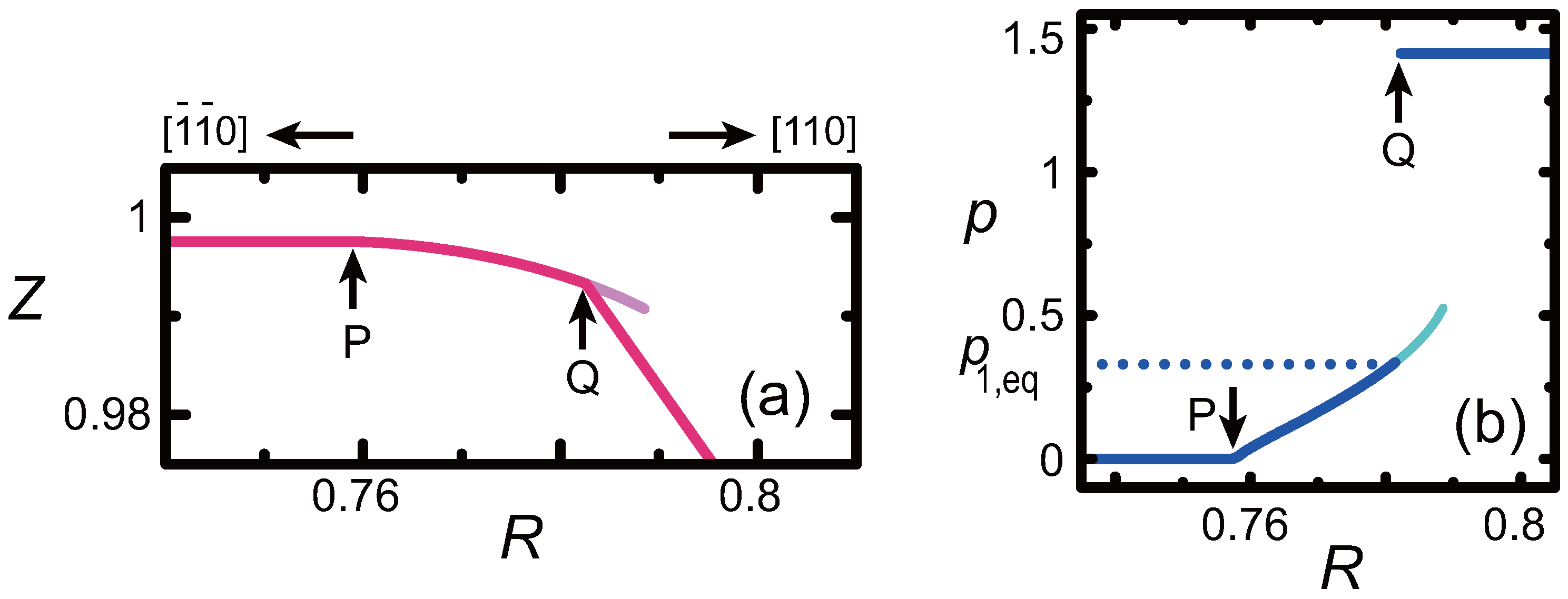
4. Detachment of Steps from Macrosteps
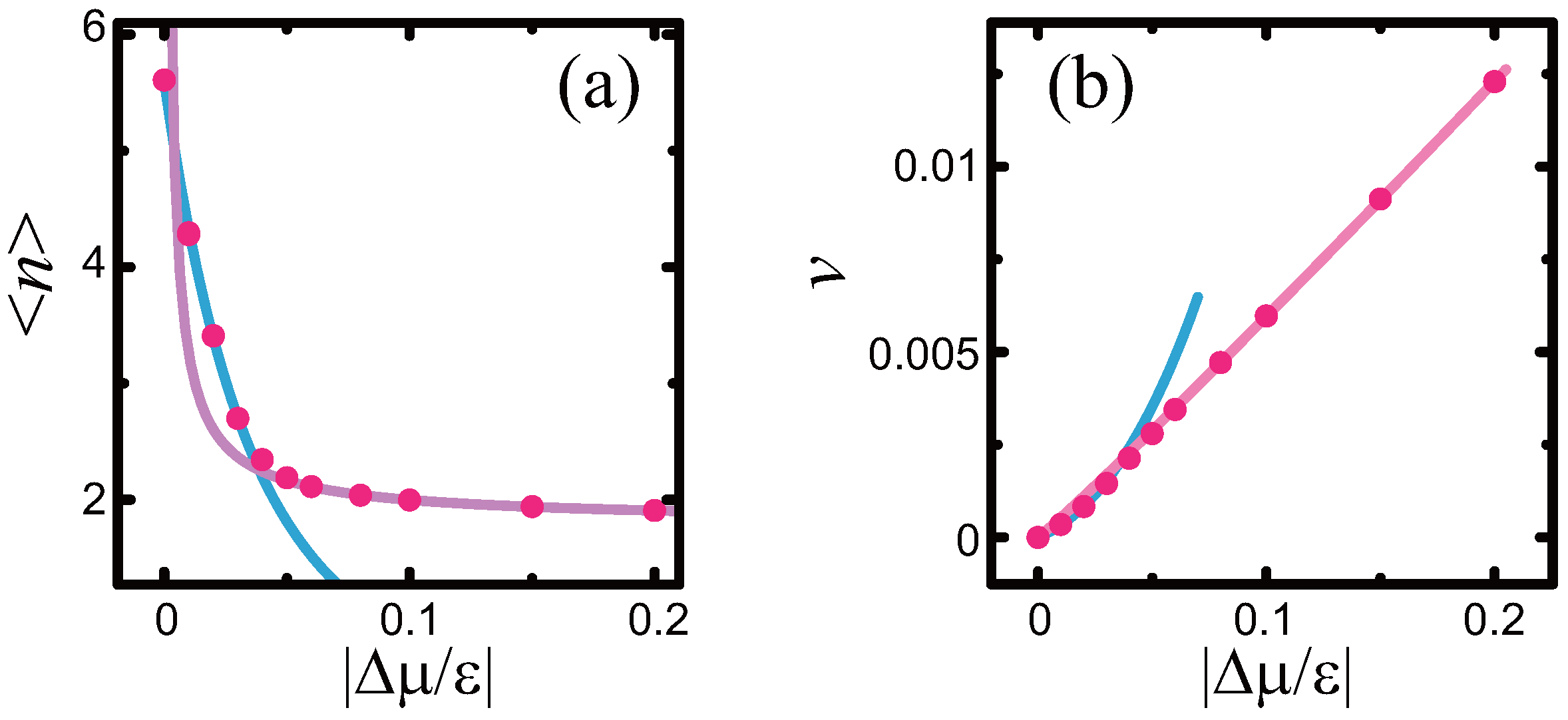
4.1. Size of a Macrostep
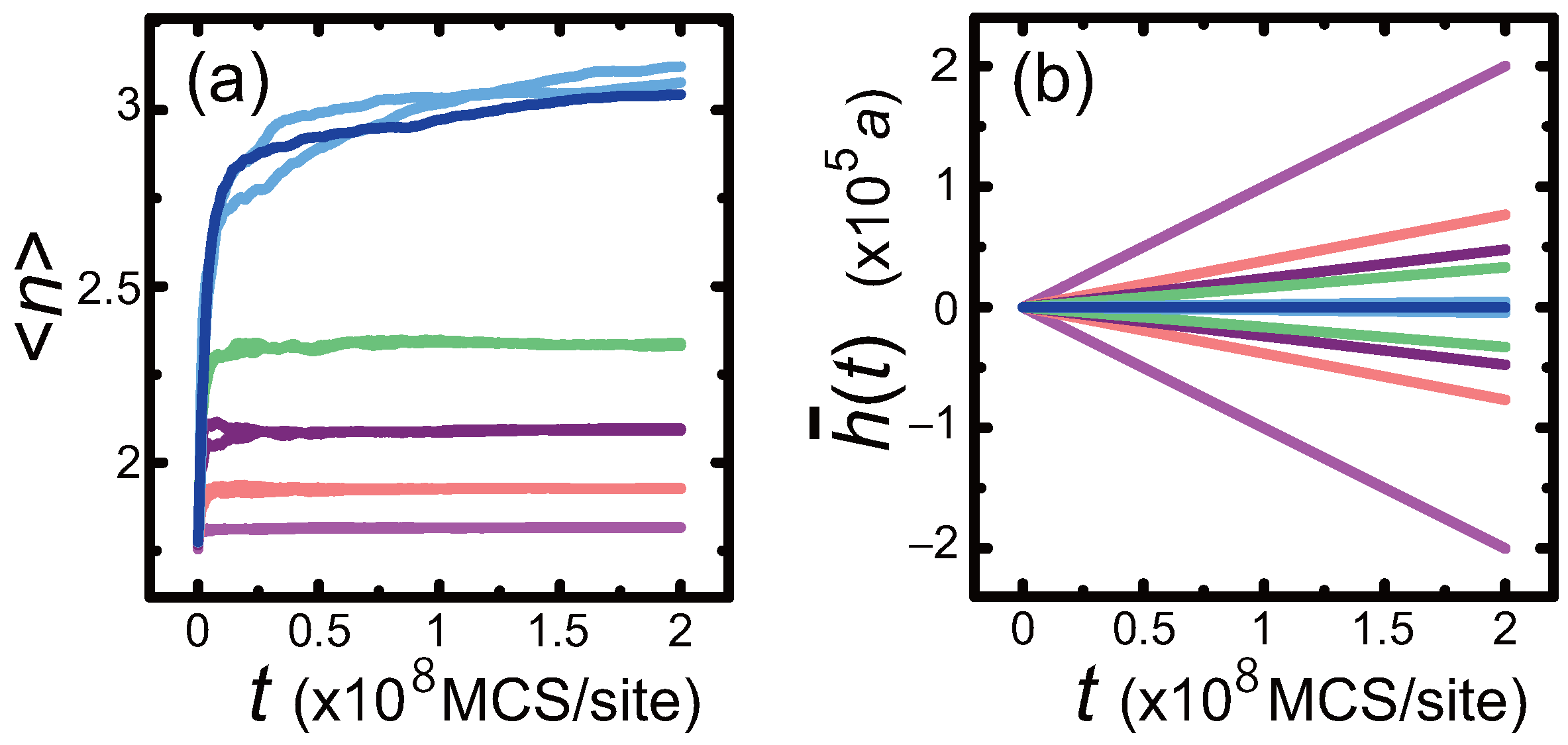
4.2. Growth Rate
5. Kinetic Roughening
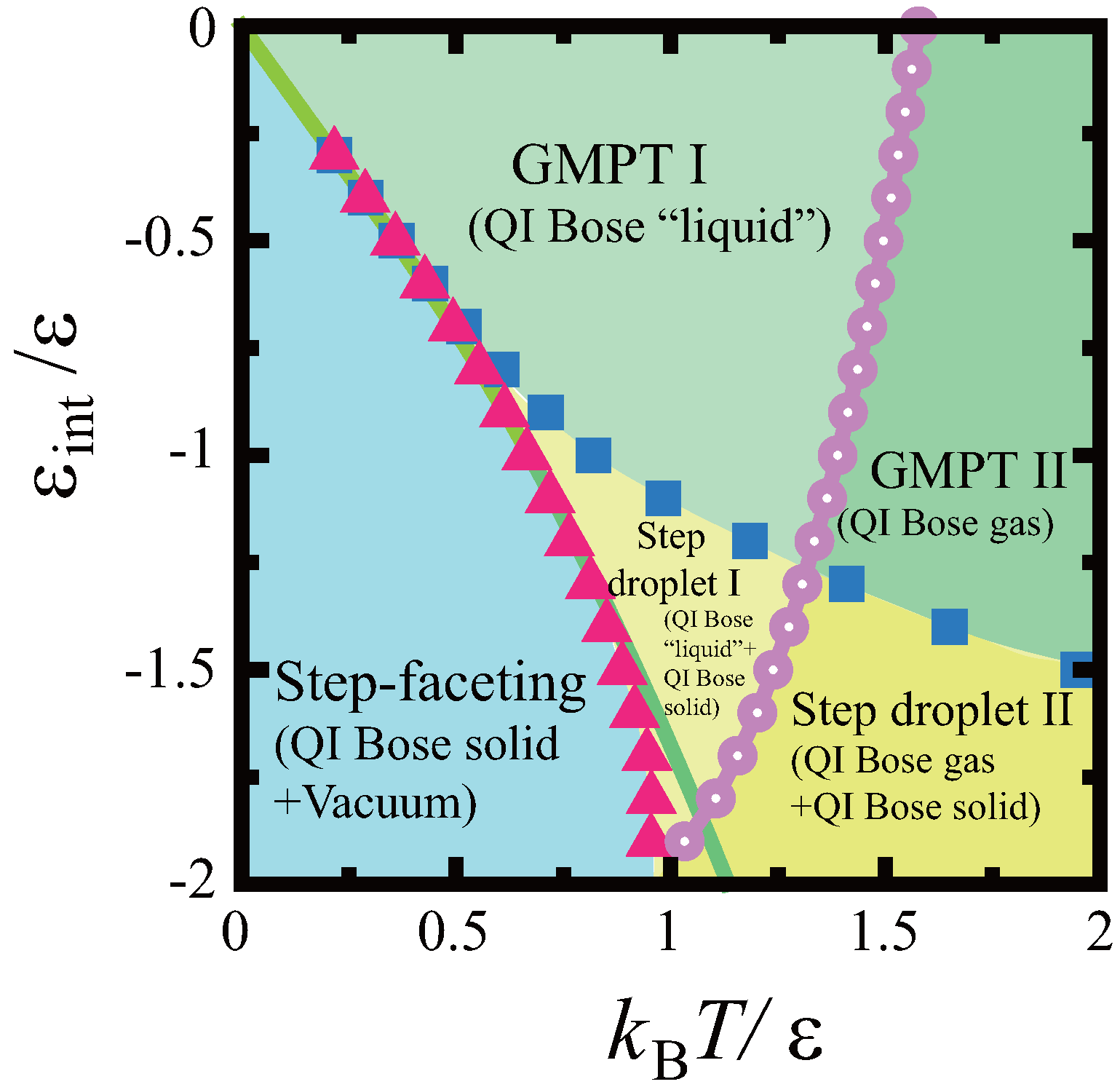
6. Discussion
7. Conclusions
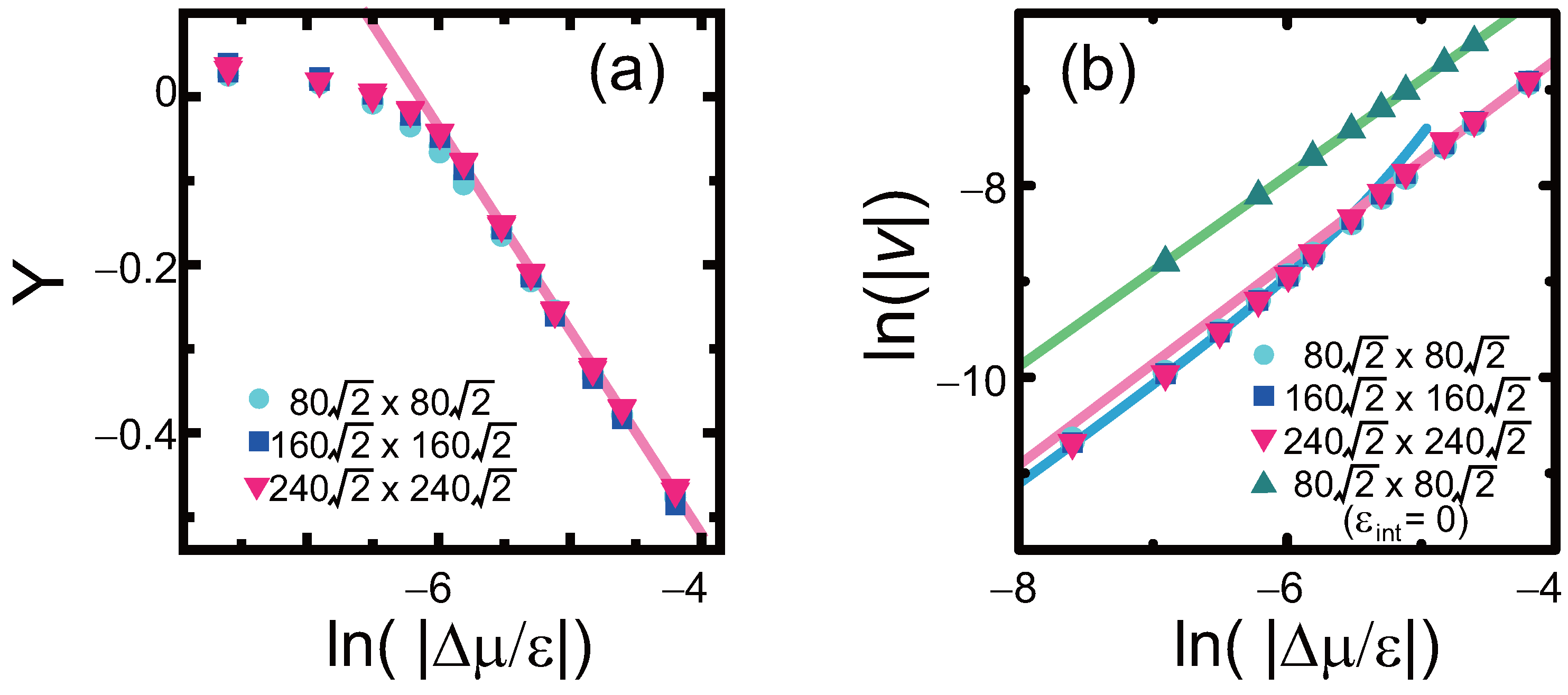
- As increases, the size of the macrostep decreases, whereas the growth rate increases.
- At small , the -dependence of and v can be explained by the attachment and detachment of elementary steps to and from the macrostep.
- When , the macrostep disassembles, and the surface roughens kinetically. is the crossover point from the two-surface coexistent state to the rough surface state.
- A scaling function was obtained with and .
- For , and both show power law behavior.
Acknowledgments
Conflicts of Interest
Abbreviations
| ECS | Equilibrium crystal shape |
| TSK | Terrace–step–kink |
| GMPT | Gruber–Mullins–Pokrovsky–Talapov |
| RSOS | Restricted solid-on-solid |
| p-RSOS | Restricted solid-on-solid with a point-contact-type step–step attraction |
| NN | Nearest-neighbor |
| NNN | Next-nearest-neighbor |
| DMRG | Density matrix renormalization group |
| 1D | One-dimensional |
| PWFRG | Product wave function renormalization group |
| MCS | Monte Carlo steps |
| 2D | Two-dimensional |
Appendix A. Anomalous Surface Tension: Density Matrix Renormalization Group Calculation
References
- Mitani, T.; Komatsu, N.; Takahashi, T.; Kato, T.; Harada, S.; Ujihara, T.; Matsumoto, Y.; Kurashige, K.; Okumura, H. Effect of aluminum addition on the surface step morphology of 4H–SiC grown from Si–Cr–C solution. J. Cryst. Growth 2015, 423, 45–49. [Google Scholar] [CrossRef]
- Cabrera, N.; Coleman, R.V. The Art and Science of Growing Crystals; Gilman, J.J., Ed.; John Wiley & Sons: New York, NY, USA; London, UK, 1963. [Google Scholar]
- Cabrera, N. The equilibrium of crystal surfaces. Surf. Sci. 1964, 2, 320–345. [Google Scholar] [CrossRef]
- Jayaprakash, C.; Rottman, C.; Saam, W.F. Simple model for crystal shapes: Step-step interactions and facet edges. Phys. Rev. B 1984, 30, 6549–6554. [Google Scholar] [CrossRef]
- Calogero, F. Solution of a three—Body problem in one dimension. J. Math. Phys. 1969, 10, 2191–2196. [Google Scholar] [CrossRef]
- Sutherland, B. Quantum Many—Body Problem in One Dimension: Ground State. J. Math. Phys. 1971, 12, 246–250. [Google Scholar] [CrossRef]
- Alerhand, O.L.; Vanderbilt, D.; Meade, R.D.; Joannopoulos, J.D. Spontaneous formation of stress domains on crystal surfaces. Phys. Rev. Lett. 1988, 61, 1973–1976. [Google Scholar] [CrossRef] [PubMed]
- Gruber, E.E.; Mullins, W.W. On the theory of anisotropy of crystalline surface tension. J. Phys. Chem. Solids 1967, 28, 875–887. [Google Scholar] [CrossRef]
- Pokrovsky, V.L.; Talapov, A.L. Ground state, spectrum, and phase diagram of two-dimensional incommensurate crystals. Phys. Rev. Lett. 1979, 42, 65–67. [Google Scholar] [CrossRef]
- Einstein, T.L. Equilibrium Shape of Crystals. In Handbook of Crystal Growth; Nishinaga, T., Ed.; Elsevier: Amsterdam, The Netherlands; Boston, MA, USA; Heidelberg, Germany; London, UK; Paris, France; Singapore; Sydney, Australia; Tokyo, Japan, 2015; Volume I, p. 216. [Google Scholar]
- Akutsu, N.; Yamamoto, T. Rough-Smooth Transition of Step and Surface. Handbook of Crystal Growth; Nishinaga, T., Ed.; Elsevier: Amsterdam, The Netherlands; Boston, MA, USA; Heidelberg, Germany; London, UK; Paris, France; Singapore; Sydney, Australia; Tokyo, Japan, 2015; Volume I, p. 265. [Google Scholar]
- Landau, L.D.; Lifshitz, E.M. Statistical Physics, 2nd ed.; Pergamon: Oxford, UK, 1968. [Google Scholar]
- Andreev, A.F. Faceting phase transitions of crystals. Sov. Phys. JETP 1981, 53, 1063–1069. [Google Scholar]
- Von Laue, M. Der Wulffsche Satz für die Gleichgewichtsform von Kristallen. Z. Kristallogr. 1944, 105, 124–133. [Google Scholar]
- Herring, C. Some theorems on the free energies of crystal surfaces. Phys. Rev. 1951, 82, 87–93. [Google Scholar] [CrossRef]
- MacKenzie, J.K.; Moore, A.J.W.; Nicholas, J.F. Bonds broken at atomically flat crystal surfaces–I: Face-centred and body-centred cubic crystals. J. Chem. Phys. Solids 1962, 23, 185–196. [Google Scholar] [CrossRef]
- Toschev, S. Crystal Growth: An Introduction; Hartman, P., Ed.; North-Holland: Amsterdam, The Netherlands; Oxford, UK, 1973; p. 328. [Google Scholar]
- Rottman, C.; Wortis, M. Equilibrium crystal shapes for lattice models with nearest- and next-nearest-neighbor interactions. Phys. Rev. B 1984, 29, 328–339. [Google Scholar] [CrossRef]
- Williams, E.D.; Bartelt, N.C. Thermodynamics of surface morphology. Science 1991, 251, 393–401. [Google Scholar] [CrossRef] [PubMed]
- Williams, E.D.; Phaneuf, R.J.; Wei, J.; Bartelt, N.C.; Einstein, T.L. Thermodynamics and statistical mechanics of the faceting of stepped Si (111). Surf. Sci. 1993, 294, 219–242, Erratum in 1994, 310, 451. [Google Scholar] [CrossRef]
- Yamamoto, T.; Akutsu, Y.; Akutsu, N. Fluctuation of a single step on the vicinal surface-universal and non-universal behaviors. J. Phys. Soc. Jpn. 1994, 63, 915–925. [Google Scholar] [CrossRef]
- Akutsu, Y.; Akutsu, N.; Yamamoto, T. Logarithmic step fluctuations in vicinal surface: A Monte Carlo study. J. Phys. Soc. Jpn. 1994, 63, 2032–2036. [Google Scholar] [CrossRef]
- Hibino, H.; Ogino, T. Transient Step Bunching on a Vicinal Si(111) Surface. Phys. Rev. Lett. 1994, 72, 657–660. [Google Scholar] [CrossRef] [PubMed]
- Ogino, T.; Hibino, H.; Homma, Y. Kinetics and Thermodynamics of Surface Steps on Semiconductors. Crit. Rev. Solid State Mater. Sci. 1999, 24, 227–263. [Google Scholar] [CrossRef]
- Song, S.; Mochrie, S.G.J. Tricriticality in the orientational phase diagram of stepped Si (113) surfaces. Phys. Rev. Lett. 1994, 73, 995–998. [Google Scholar] [CrossRef] [PubMed]
- Song, S.; Mochrie, S.G.J. Attractive step-step interactions, tricriticality, and faceting in the orientational phase diagram of silicon surfaces between [113] and [114]. Phys. Rev. B 1995, 51, 10068–10084. [Google Scholar] [CrossRef]
- Van Dijken, S.; Zandvliet, H.J.W.; Poelsema, B. Anomalous strong repulsive step-step interaction on slightly misoriented Si (113). Phys. Rev. B 1997, 55, 7864–7867. [Google Scholar] [CrossRef]
- Jeong, H.C.; Williams, E.D. Steps on surfaces: Experiment and theory. Surf. Sci. Rep. 1999, 34, 171–294. [Google Scholar] [CrossRef]
- Lassig, M. Vicinal surfaces and the Calogero-Sutherland model. Phys. Rev. Lett. 1996, 77, 526–529. [Google Scholar] [CrossRef] [PubMed]
- Bhattacharjee, S.M. Theory of tricriticality for miscut surfaces. Phys. Rev. Lett. 1996, 76, 4568–4571. [Google Scholar] [CrossRef] [PubMed]
- Shenoy, V.B.; Zhang, S.; Saam, W.F. Bunching transitions on vicinal surfaces and quantum n-mers. Phys. Rev. Lett. 1998, 81, 3475–3478. [Google Scholar] [CrossRef]
- Shenoy, V.B.; Zhang, S.; Saam, W.F. Step-bunching transitions on vicinal surfaces with attractive step interactions. Surf. Sci. 2000, 467, 58–84. [Google Scholar] [CrossRef]
- Einstein, T.L.; Richards, H.L.; Cohen, S.D.; Pierre-Louis, O.; Giesen, M. Terrace-width distributions on vicinal surfaces: Generalized Wigner surmise and extraction of step–step repulsions. Appl. Surf. Sci. 2001, 175–176, 62–68. [Google Scholar] [CrossRef]
- Akutsu, N.; Akutsu, Y.; Yamamoto, T. Stiffening transition in vicinal surfaces with adsorption. Prog. Theory Phys. 2001, 105, 361–366. [Google Scholar] [CrossRef]
- Akutsu, N.; Akutsu, Y.; Yamamoto, T. Statistical mechanics of the vicinal surfaces with adsorption. Surf. Sci. 2001, 493, 475–479. [Google Scholar] [CrossRef]
- Akutsu, N.; Akutsu, Y.; Yamamoto, T. Thermal step bunching and interstep attraction on the vicinal surface with adsorption. Phys. Rev. B 2003, 67, 125407. [Google Scholar] [CrossRef]
- Akutsu, N.; Hibino, H.; Yamamoto, T. A Lattice Model for Thermal Decoration and Step Bunching in Vicinal Surface with Sub-Monolayer Adsorbates. e-J. Surf. Sci. Nanotechnol. 2009, 7, 39–44. [Google Scholar] [CrossRef]
- Akutsu, N. Thermal step bunching on the restricted solid-on-solid model with point contact inter-step attractions. Appl. Surf. Sci. 2009, 256, 1205–1209. [Google Scholar] [CrossRef]
- Akutsu, N. Zipping process on the step bunching in the vicinal surface of the restricted solid-on-solid model with the step attraction of the point contact type. J. Cryst. Growth 2011, 318, 10–13. [Google Scholar] [CrossRef]
- Akutsu, N. Non-universal equilibrium crystal shape results from sticky steps. J. Phys. Condens. Matter 2011, 23, 485004. [Google Scholar] [CrossRef] [PubMed]
- Akutsu, N. Sticky steps inhibit step motions near equilibrium. Phys. Rev. E 2012, 86, 061604. [Google Scholar] [CrossRef] [PubMed]
- Akutsu, N. Pinning of steps near equilibrium without impurities, adsorbates, or dislocations. J. Cryst. Growth 2014, 401, 72–77. [Google Scholar] [CrossRef]
- Akutsu, N. Faceting diagram for sticky steps. AIP Adv. 2016, 6, 035301. [Google Scholar] [CrossRef]
- Akutsu, N. Effect of the roughening transition on the vicinal surface in the step droplet zone. J. Cryst. Growth 2016. [Google Scholar] [CrossRef]
- Akutsu, N. Profile of a Faceted Macrostep Caused by Anomalous Surface Tension. Adv. Condens. Matter Phys. 2017, 2021510. [Google Scholar] [CrossRef]
- White, S.R. Density matrix formulation for quantum renormalization groups. Phys. Rev. Lett. 1992, 69, 2863–2866. [Google Scholar] [CrossRef] [PubMed]
- Nishino, T. Density matrix renormalization group method for 2D classical models. J. Phys. Soc. Jpn. 1995, 64, 3598–3601. [Google Scholar] [CrossRef]
- Schollwöck, U. The density-matrix renormalization group. Rev. Mod. Phys. 2005, 77, 259–315. [Google Scholar] [CrossRef]
- Nishino, T.; Okunishi, K. Product wave function renormalization group. J. Phys. Soc. Jpn. 1995, 64, 4084–4087. [Google Scholar] [CrossRef]
- Hieida, Y.; Okunishi, K.; Akutsu, Y. Magnetization process of a one-dimensional quantum antiferromagnet: The product-wave-function renormalization group approach. Phys. Lett. A 1997, 233, 464–470. [Google Scholar] [CrossRef]
- Hieida, Y.; Okunishi, K.; Akutsu, Y. Numerical renormalization approach to two-dimensional quantum antiferromagnets with valence-bond-solid type ground state. New J. Phys. 1999, 1, 7. [Google Scholar] [CrossRef]
- Ehrlich, G.; Hudda, F.G. Atomic view of surface self-diffusion: Tungsten on tungsten. J. Chem. Phys. 1966, 44, 1039–1049. [Google Scholar] [CrossRef]
- Schwoeble, R.L.; Shipsey, E.J. Step motion on crystal surfaces. J. Appl. Phys. 1966, 37, 3682–3686. [Google Scholar] [CrossRef]
- Pimpinelli, A.; Villain, J. Physics of Crystal Growth; Cambridge University Press: Cambridge, UK, 1998. [Google Scholar]
- Misbah, C.; Pierre-Louis, O.; Saito, Y. Crystal surfaces in and out of equilibrium: A modern view. Rev. Mod. Phys. 2010, 82, 981–1040. [Google Scholar] [CrossRef]
- Mermin, N.D.; Wagner, H. Absence of ferromagnetism or antiferromagnetism in one-or two-dimensional isotropic Heisenberg models. Phys. Rev. Lett. 1966, 17, 1133–1136, Erratum in 1966, 17, 1307. [Google Scholar] [CrossRef]
- Akutsu, Y.; Akutsu, N.; Yamamoto, T. Universal jump of Gaussian curvature at the facet edge of a crystal. Phys. Rev. Lett. 1988, 61, 424–427. [Google Scholar] [CrossRef] [PubMed]
- Yamamoto, T.; Akutsu, Y.; Akutsu, N. Universal Behavior of the Equilibrium Crystal Shape near the Facet Edge. I. A Generalized Terrace-Step-Kink Model. J. Phys. Soc. Jpn. 1988, 57, 453–460. [Google Scholar] [CrossRef]
- Mikheev, L.V.; Pokrovsky, V.L. Free-fermion solution for overall equilibrium crystal shape. J. Phys. 1991, 1, 373–382. [Google Scholar] [CrossRef]
- Akutsu, Y. Exact Landau Free-Energy of Solvable N-State Vertex Model. J. Phys. Soc. Jpn. 1989, 58, 2219–2222. [Google Scholar] [CrossRef]
- Okunishi, K.; Hieida, Y.; Akutsu, Y. δ-function Bose-gas picture of S = 1 antiferromagnetic quantum spin chains near critical fields. Phys. Rev. B 1999, 59, 6806–6812. [Google Scholar] [CrossRef]
- Markov, I.V. Crystal Growth for Beginners, 2nd ed.; World Scientific: Hackensack, NJ, USA; London, UK; Singapore; Hong Kong, China, 2003. [Google Scholar]
- Müller-Krumbhaar, H.; Burkhardt, T.W.; Kroll, D.M. A generalized kinetic equation for crystal growth. J. Cryst. Growth 1977, 38, 13–22. [Google Scholar] [CrossRef]
- Enomoto, Y.; Kawasaki, K.; Ohta, T.; Ohta, S. Interface dynamics under the anisotropic surface tension. Phys. Lett. 1985, 107, 319–323. [Google Scholar] [CrossRef]
- Akutsu, N.; Akutsu, Y. Roughening, faceting and equilibrium shape of two-dimensional anisotropic interface. I. Thermodynamics of interface fluctuations and geometry of equilibrium crystal shape. J. Phys. Soc. Jpn. 1987, 56, 1443–1453. [Google Scholar] [CrossRef]
- Burton, W.K.; Cabrera, N.; Frank, F.C. The growth of crystals and the equilibrium structure of their surfaces. Philos. Trans. R. Soc. Lond. A 1951, 243, 299–358. [Google Scholar] [CrossRef]
- Krug, J.; Meakin, P. Kinetic roughening of Laplacian fronts. Phys. Rev. Lett. 1991, 66, 703–706. [Google Scholar] [CrossRef] [PubMed]
- Uwaha, M.; Saito, Y. Kinetic smoothing and roughening of a step with surface diffusion. Phys. Rev. Lett. 1992, 68, 224–227. [Google Scholar] [CrossRef] [PubMed]
- Barabasi, A.L.; Stanley, H.E. Fractal Concepts in Surface Growth; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar]
- Reichhardt, C.; Reichhardt, C.J.O. Depinning and nonequilibrium dynamic phases of particle assemblies driven over random and ordered substrates: A review. Rep. Prog. Phys. 2017, 80, 026501. [Google Scholar] [CrossRef] [PubMed]
- Jayaprakash, C.; Saam, W.F.; Teitel, S. Roughening and facet formation in crystals. Phys. Rev. Lett. 1983, 50, 2017–2020. [Google Scholar] [CrossRef]
- Cabrera, N.; Garcia, N. Roughening transition in the interface between superfluid and solid He 4. Phys. Rev. B 1982, 25, 6057–6059. [Google Scholar] [CrossRef]
- Saenz, J.; Garcia, N. Classical critical behaviour in crystal surfaces near smooth and sharp edges. Surf. Sci. 1985, 155, 24–30. [Google Scholar] [CrossRef]
- Van Beijeren, H.; Nolden, I. Structure and Dynamics of Surfaces; Schommers, W., von Blancken-Hagen, P., Eds.; Springer: Berlin/Heidelberg, Germany, 1987; Volume 2, p. 259. [Google Scholar]
- Saito, Y. Self-Consistent Calculation of Statics and Dynamics of the Roughening Transition. Z. Phys. B 1978, 32, 75–82. [Google Scholar] [CrossRef]
- Pimpinelli, A.; Tonchev, V.; Videcoq, A.; Vladimirova, M. Scaling and Universality of Self-Organized Patterns on Unstable Vicinal Surfaces. Phys. Rev. Lett. 2002, 88, 206103. [Google Scholar] [CrossRef] [PubMed]
- Krug, J.; Tonchev, V.; Stoyanov, S.; Pimpinelli, A. Scaling properties of step bunches induced by sublimation and related mechanisms. Phys. Rev. B 2005, 71, 045412. [Google Scholar] [CrossRef]
- Krasteva, A.; Popova, H.; Akutsu, N.; Tonchev, V. Time scaling relations for step bunches from models with step-step attractions (B1-type models). AIP Conf. Proc. 2016, 1722, 220015. [Google Scholar]
- Akutsu, N.; Akutsu, Y. Thermal evolution of step stiffness on the Si (001) surface: Temperature-rescaled Ising-model approach. Phys. Rev. B 1998, 57, R4233–R4236. [Google Scholar] [CrossRef]
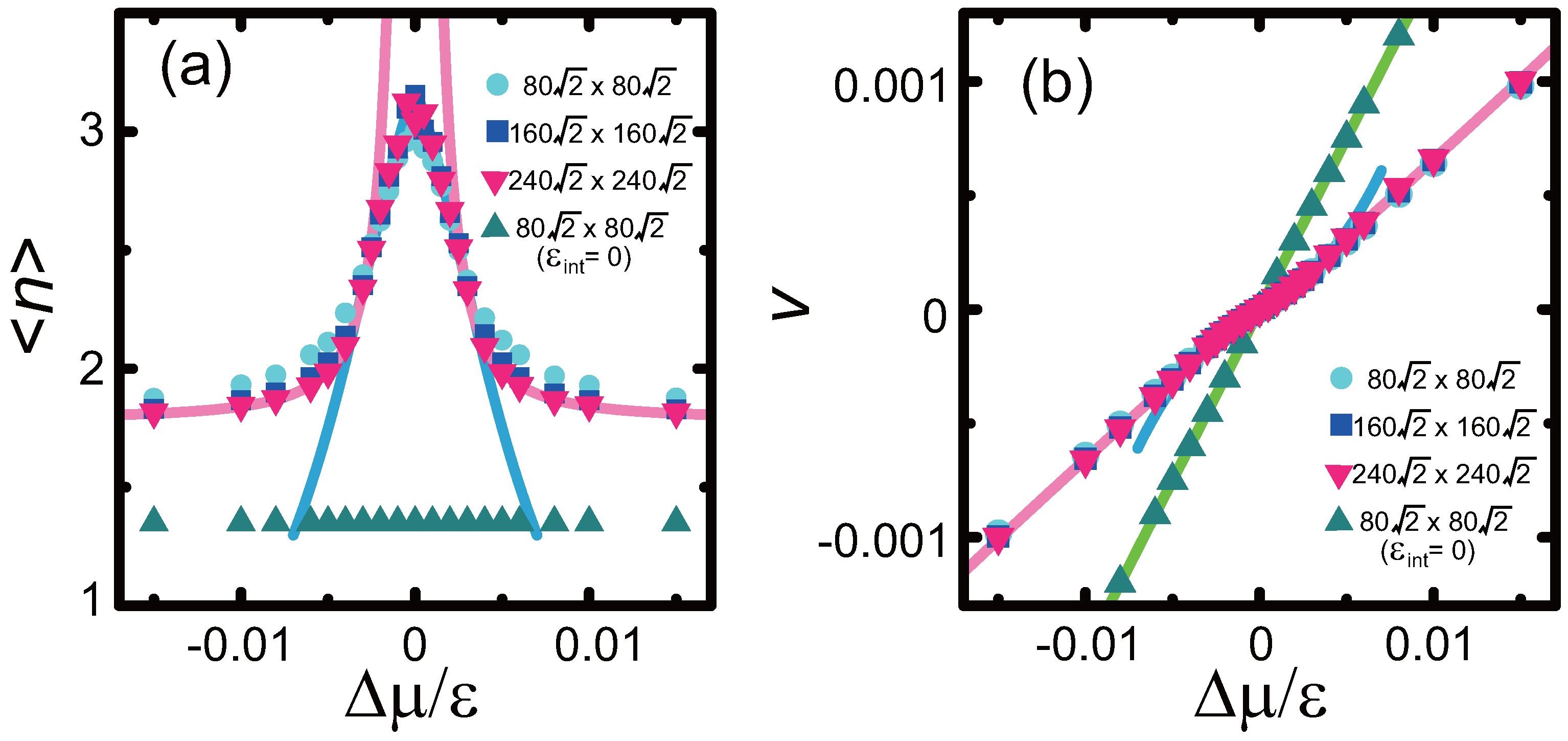
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license ( http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Akutsu, N. Disassembly of Faceted Macrosteps in the Step Droplet Zone in Non-Equilibrium Steady State. Crystals 2017, 7, 42. https://doi.org/10.3390/cryst7020042
Akutsu N. Disassembly of Faceted Macrosteps in the Step Droplet Zone in Non-Equilibrium Steady State. Crystals. 2017; 7(2):42. https://doi.org/10.3390/cryst7020042
Chicago/Turabian StyleAkutsu, Noriko. 2017. "Disassembly of Faceted Macrosteps in the Step Droplet Zone in Non-Equilibrium Steady State" Crystals 7, no. 2: 42. https://doi.org/10.3390/cryst7020042
APA StyleAkutsu, N. (2017). Disassembly of Faceted Macrosteps in the Step Droplet Zone in Non-Equilibrium Steady State. Crystals, 7(2), 42. https://doi.org/10.3390/cryst7020042





