Impact of Inter-Modular Connections on Progressive Compressive Behavior of Prefabricated Column-Supported Volumetric Modular Steel Frames
Abstract
1. Introduction
2. Experimental Testing
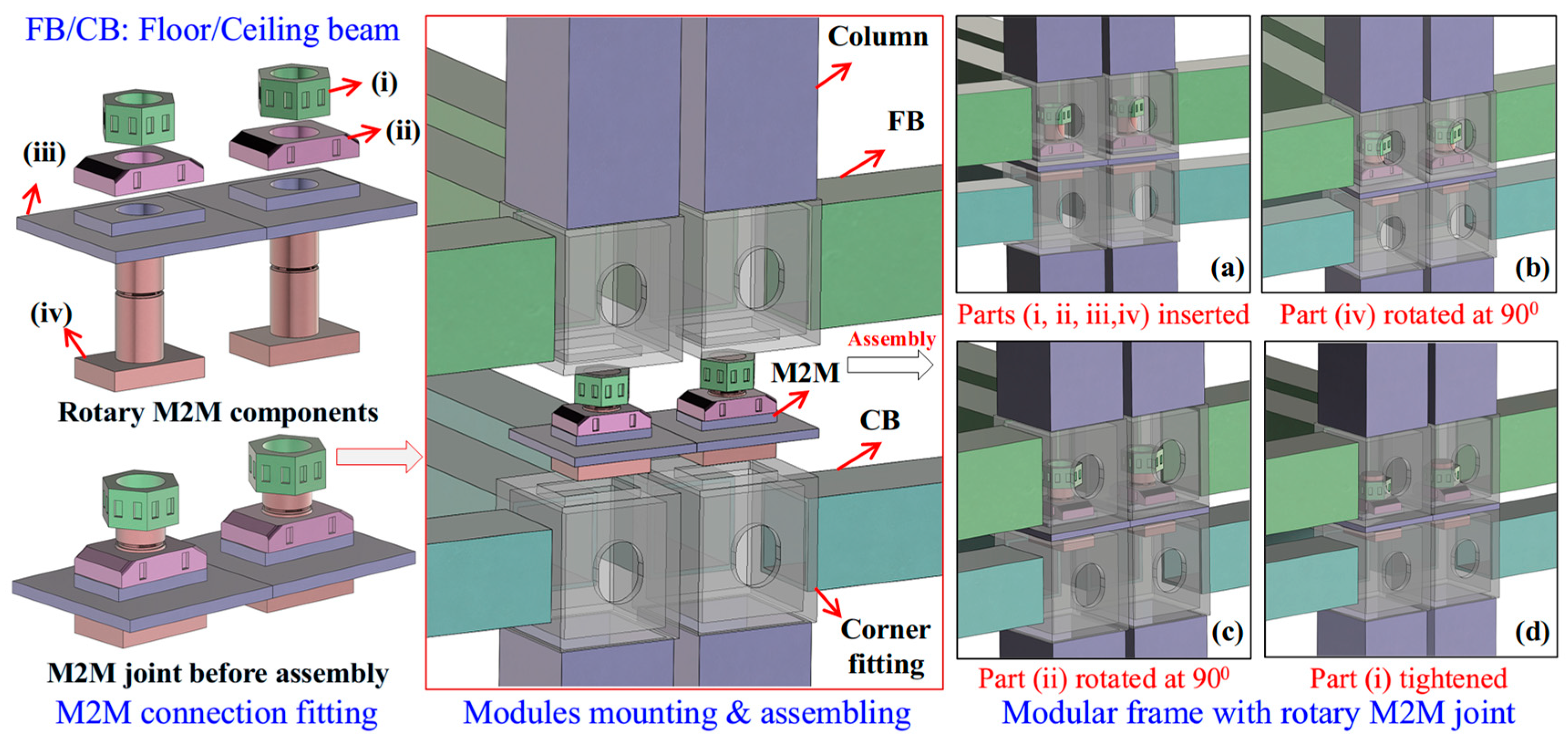
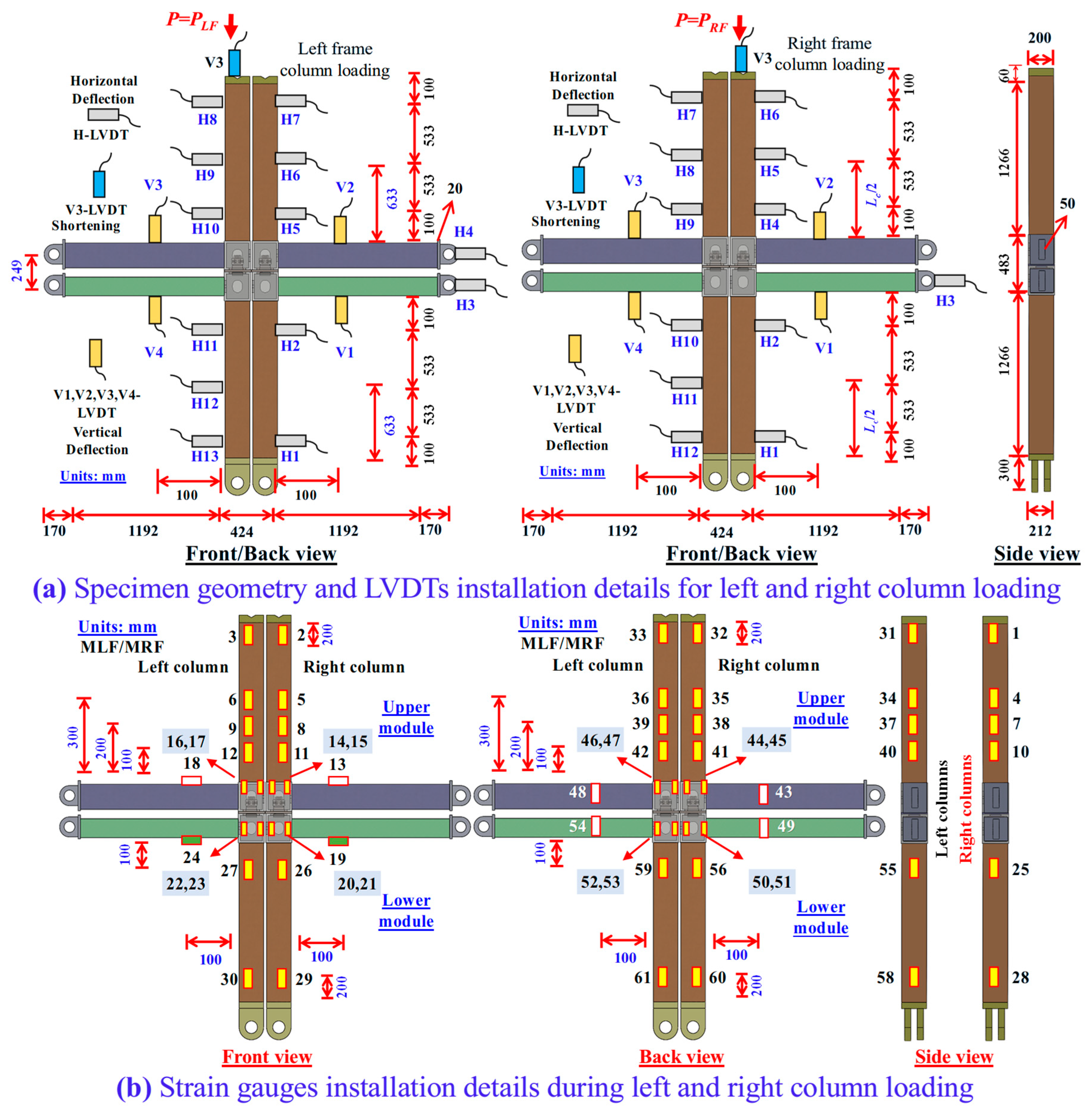
2.1. Specimen Design and Geometry
2.2. Material Testing
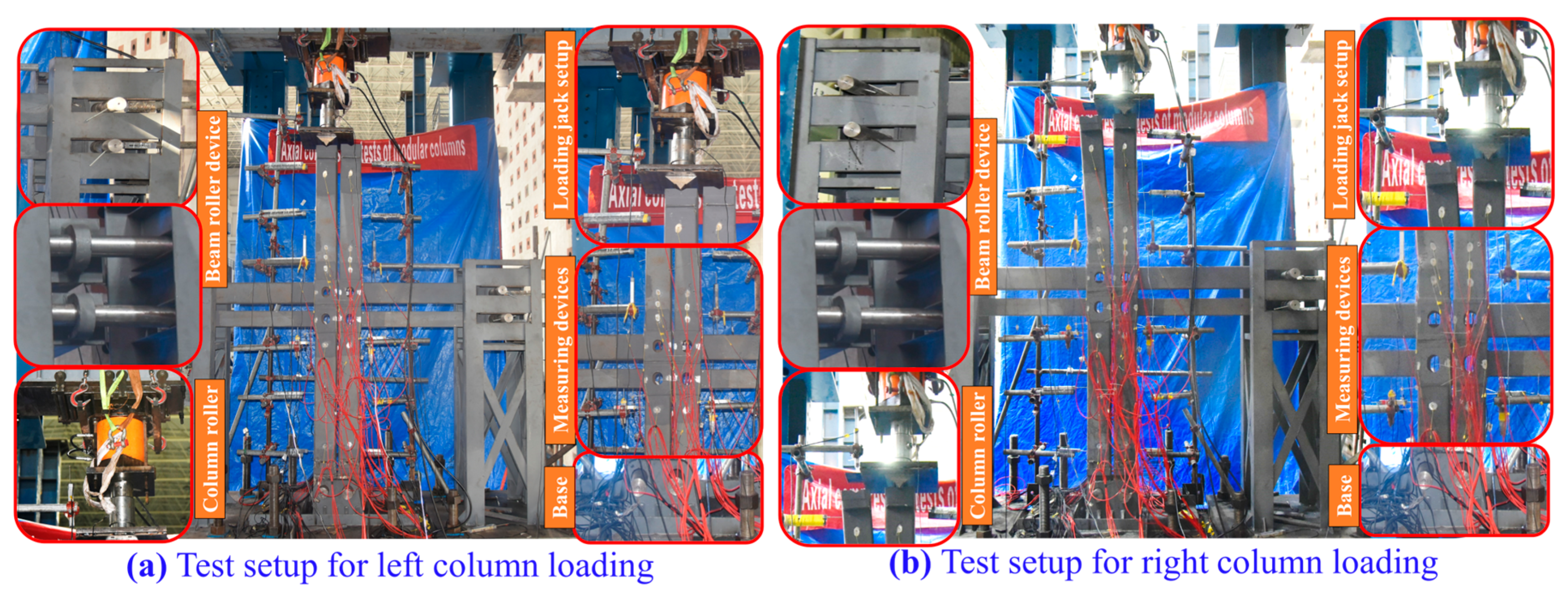
2.3. Test Setup
3. Experimental Findings
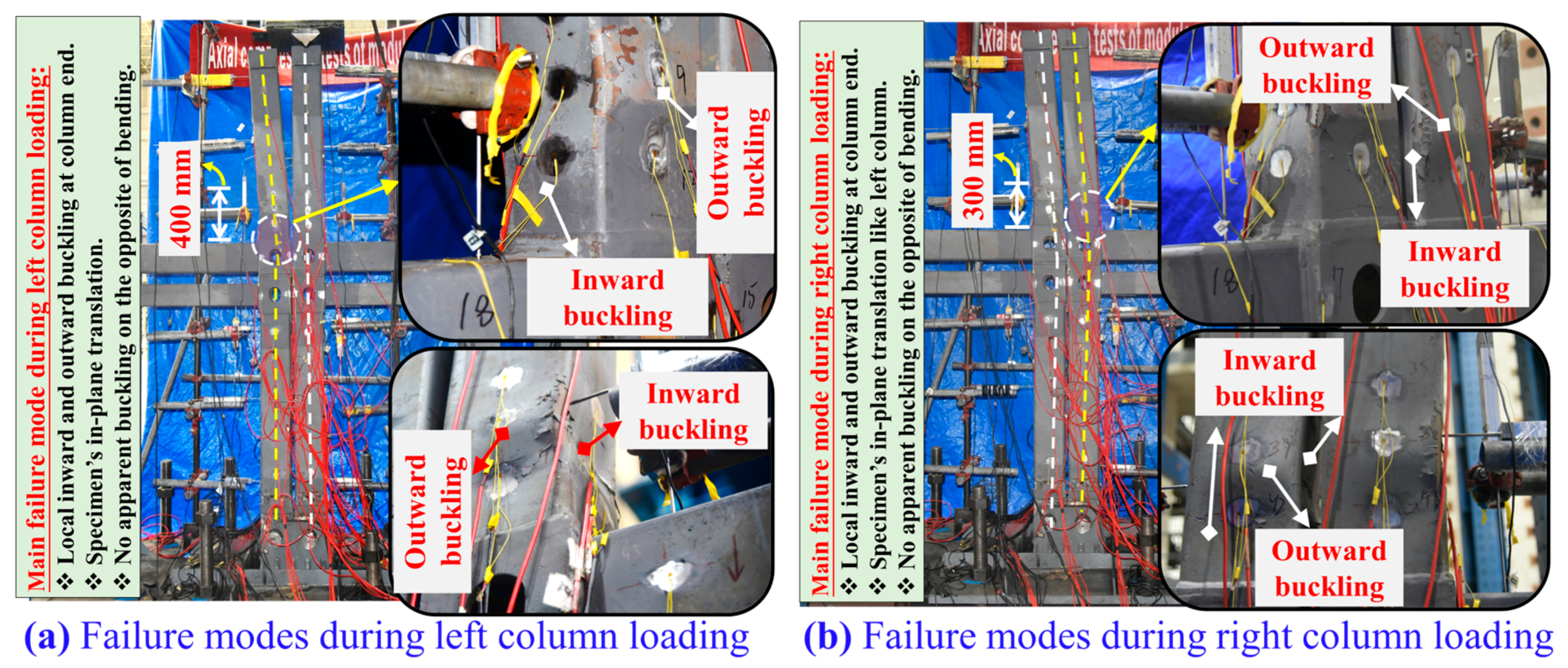
3.1. Damaging Patterns
3.2. Load and Axial Shortening Behaviors
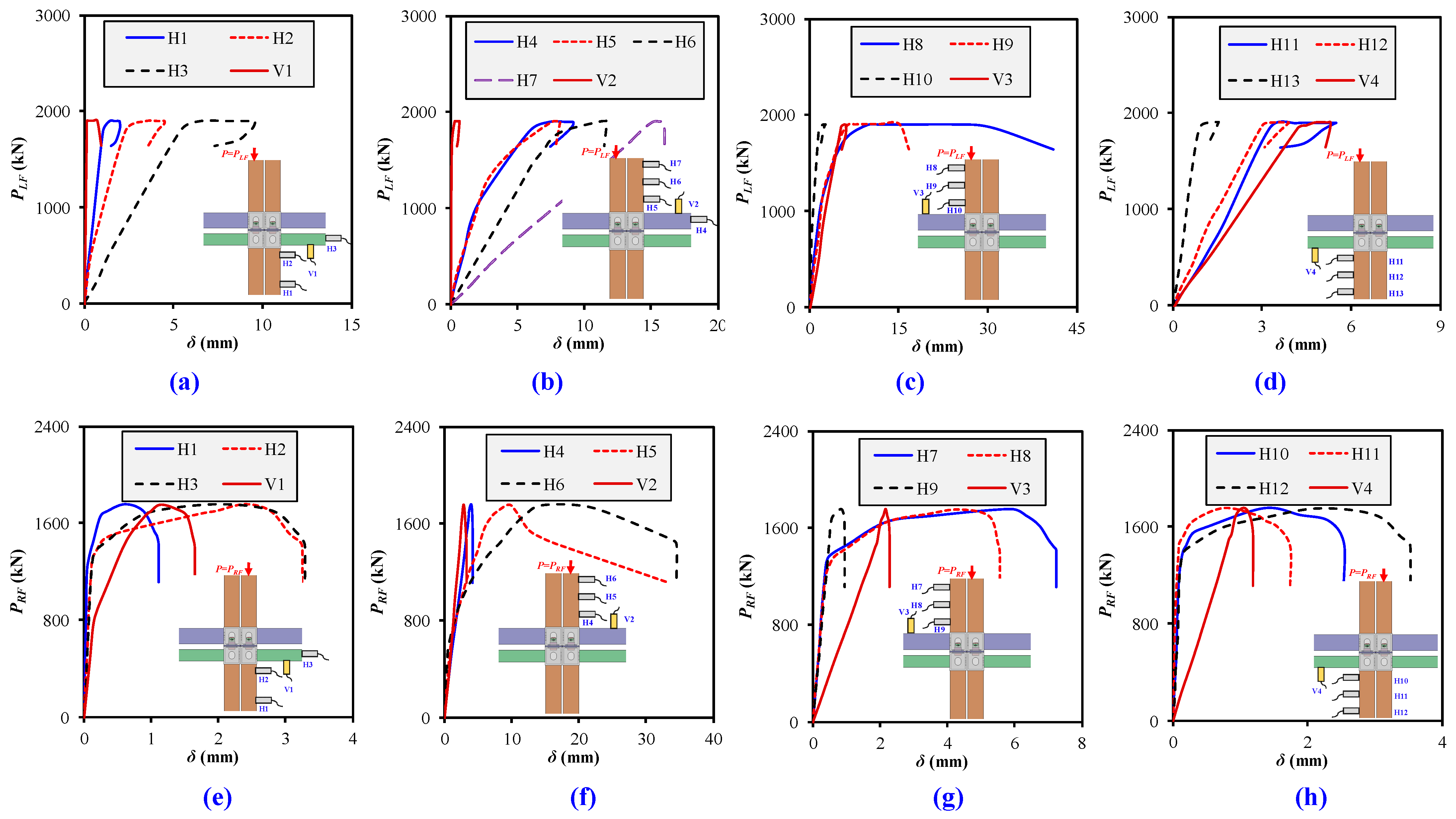
3.3. Load and Strain Behaviors
3.4. Load and Deflection Behaviors
4. Finite-Element Analysis
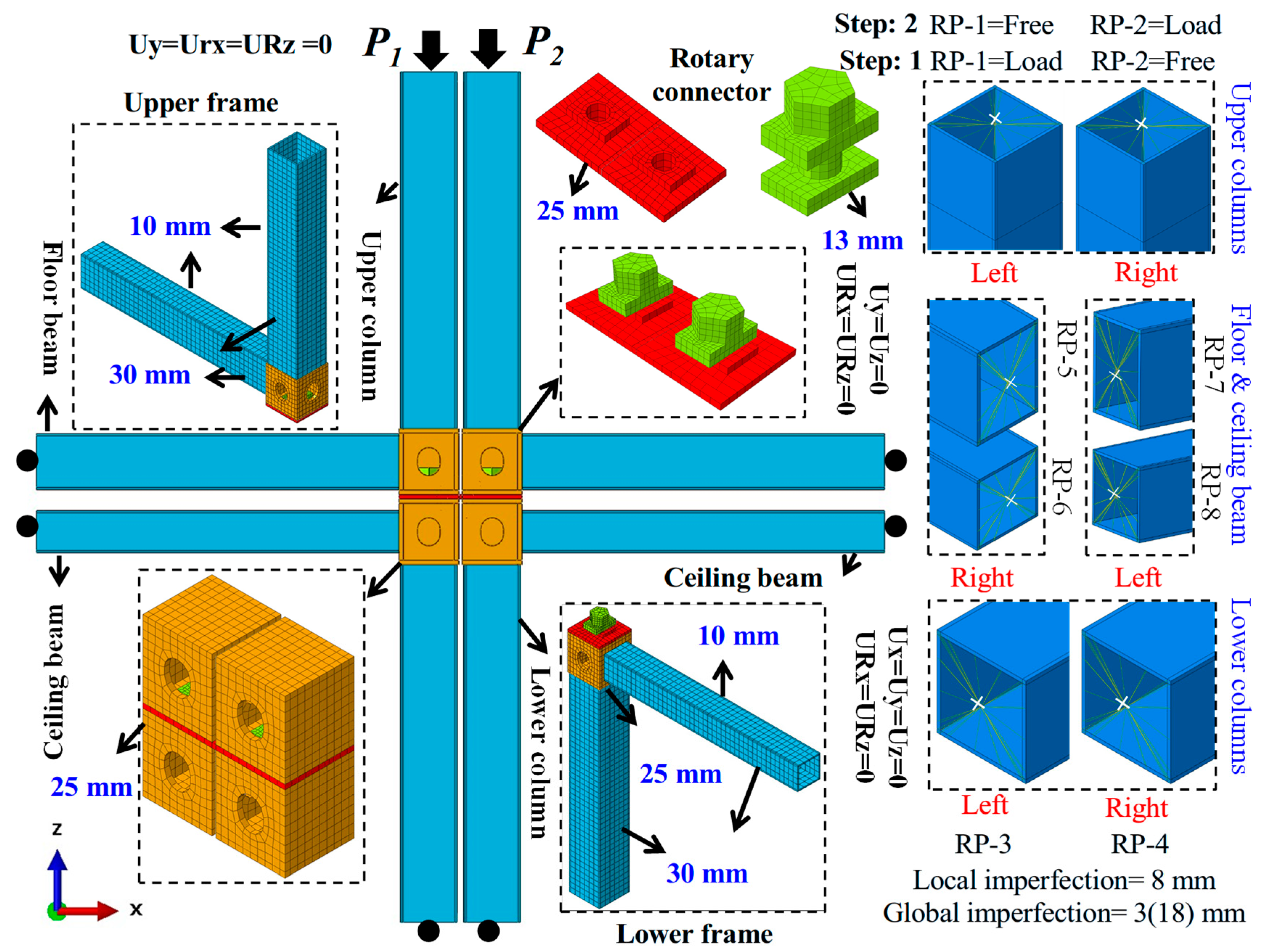
4.1. Establishment of FEMs
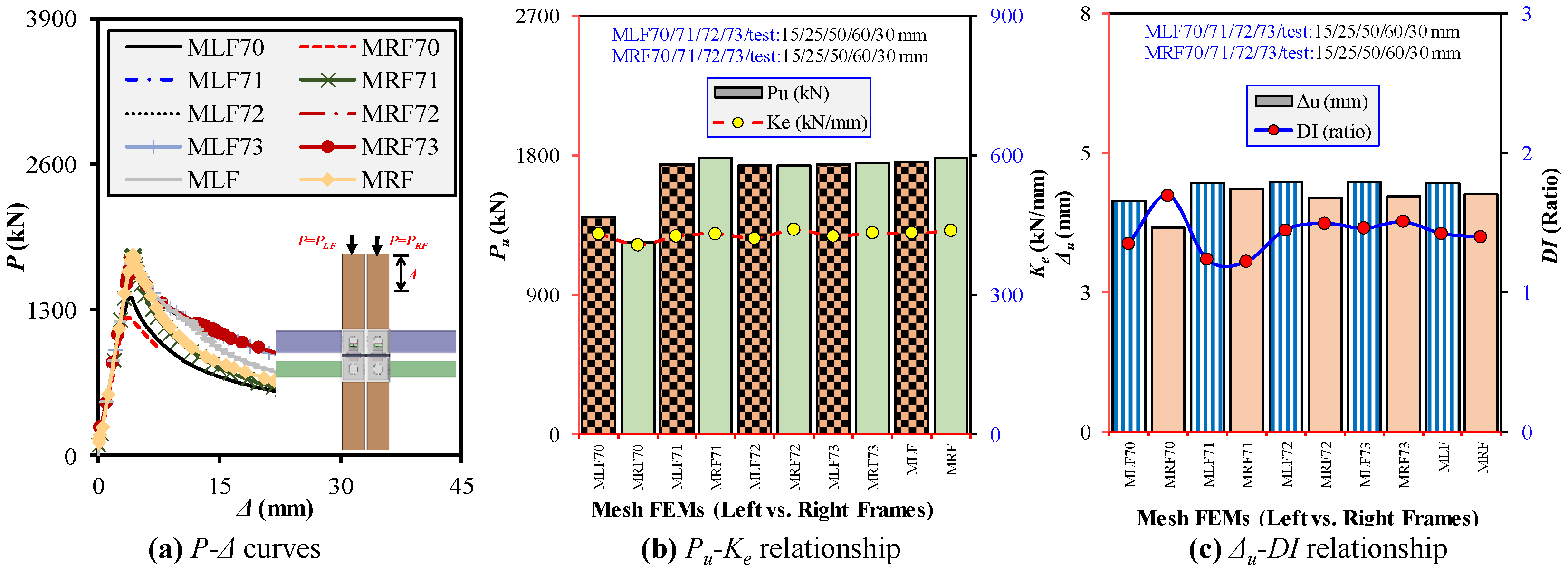
4.2. Mesh Sensitivity Analysis
4.3. Loading and Boundary Constraints
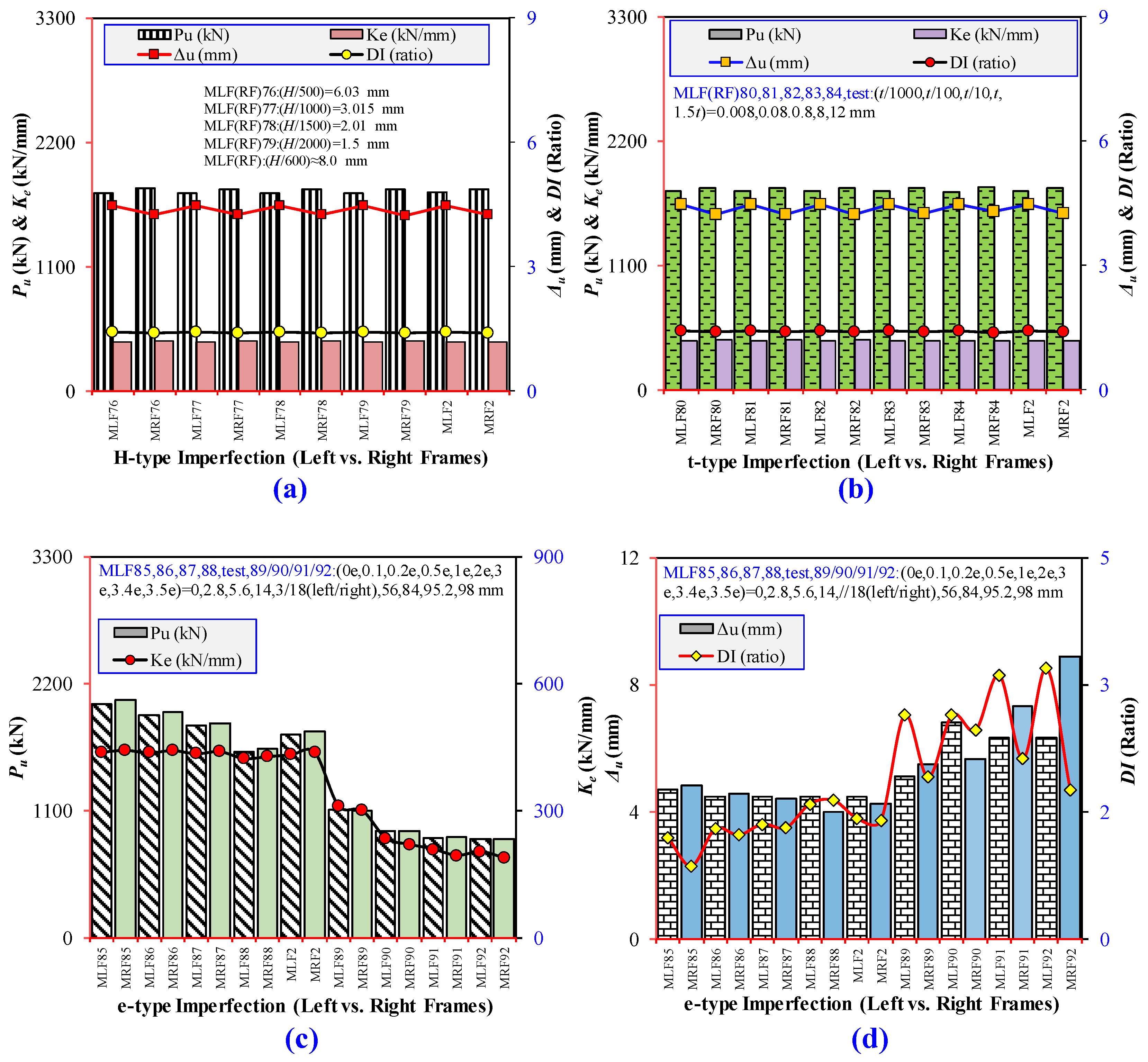
4.4. Initial Imperfections
4.5. Validations
5. Parametric Analysis
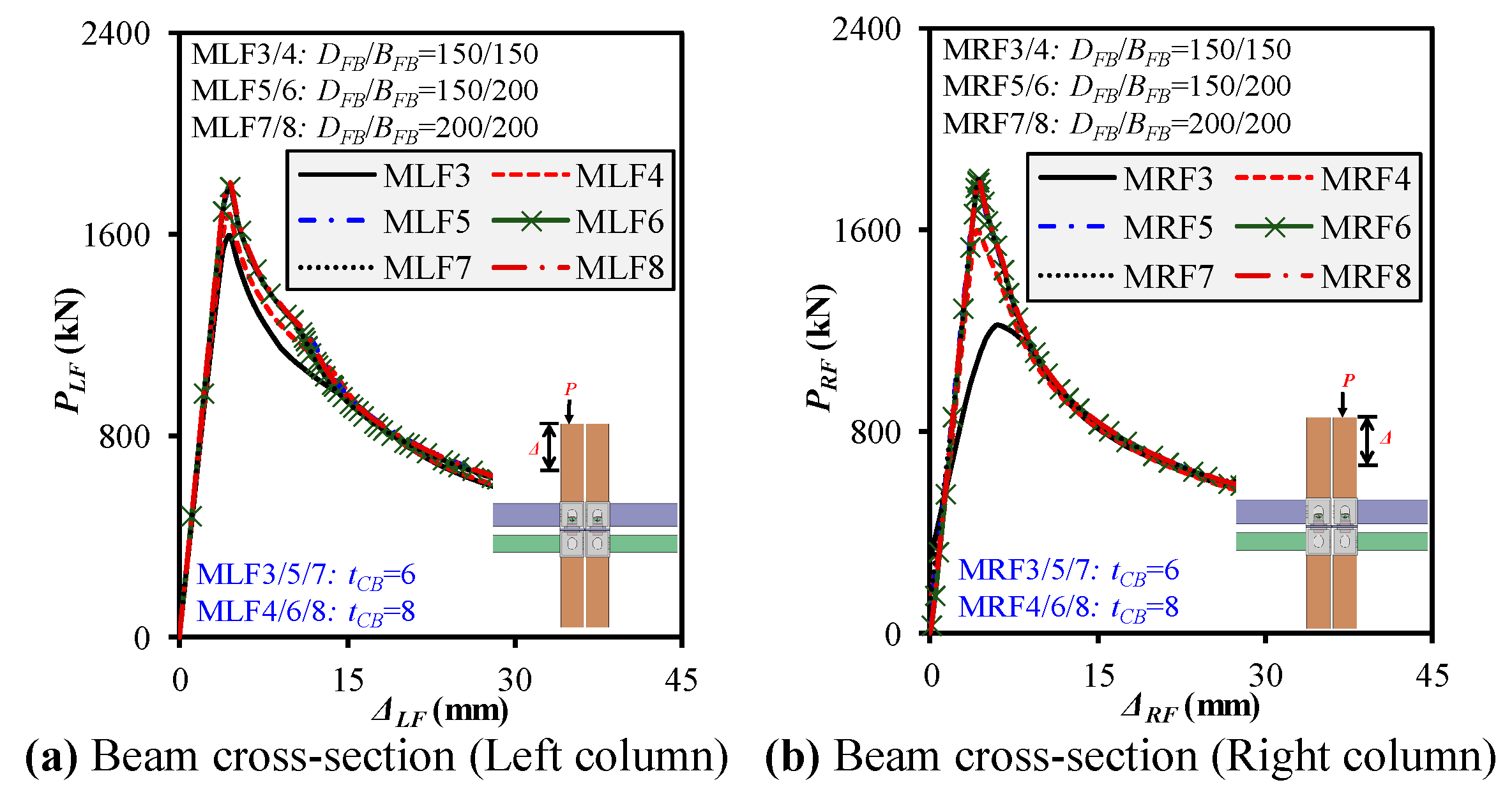
5.1. Influence of Beam Cross Sections on Progressive Compression Behavior
5.2. Influence of Beam Lengths on Progressive Behavior
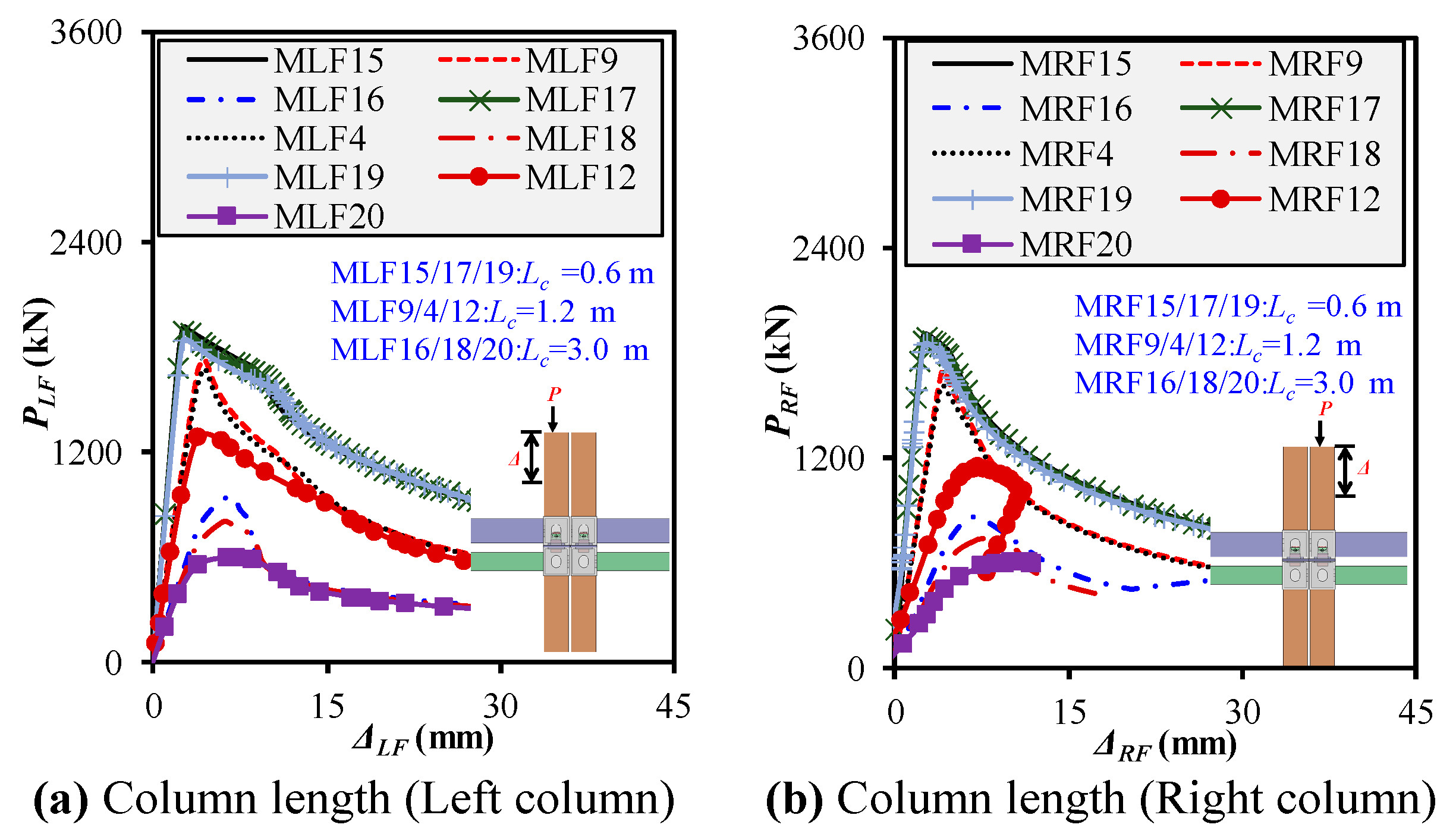
5.3. Influence of Column Length on Progressive Behavior
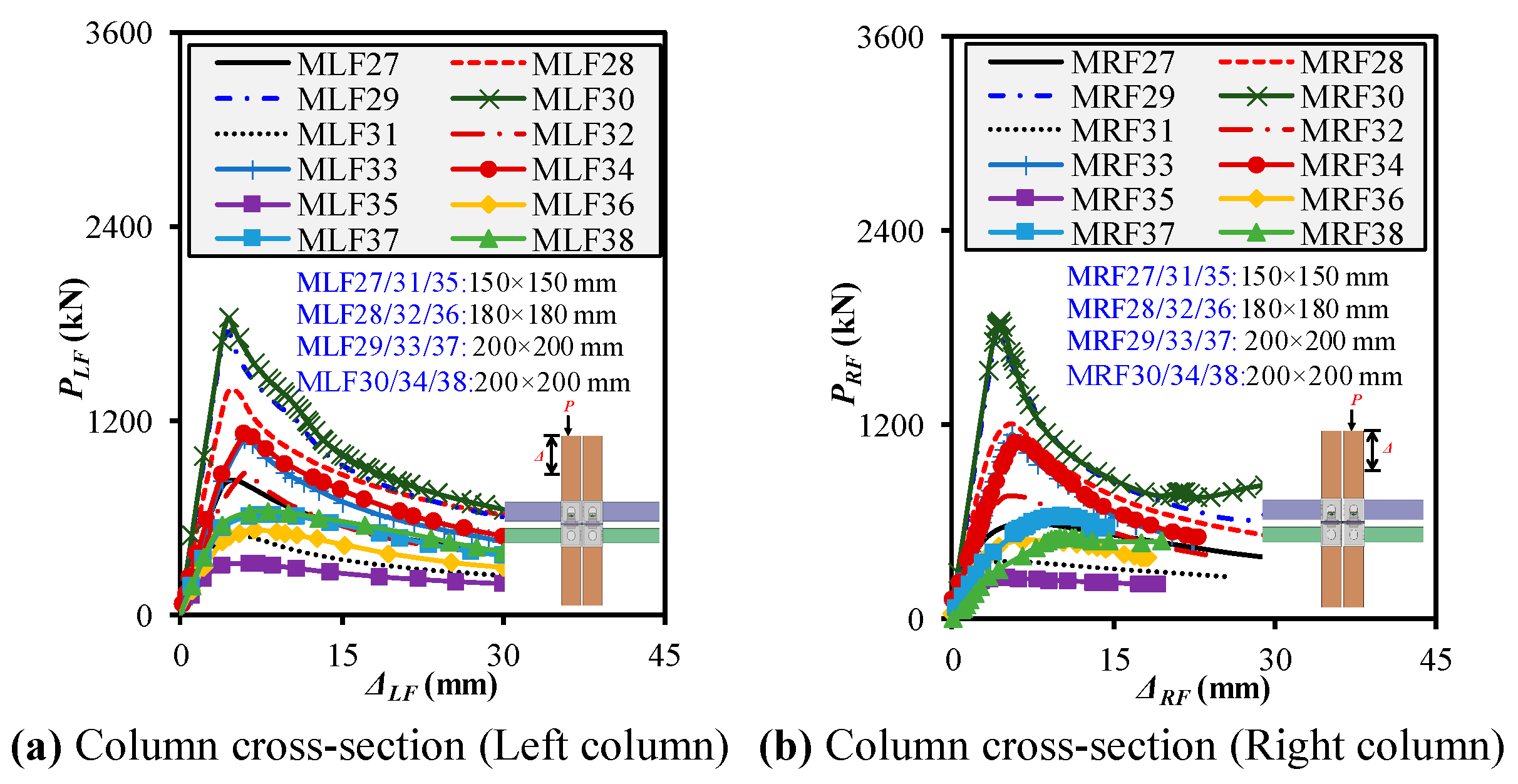
5.4. Influence of Column Cross Sections on Progressive Behavior
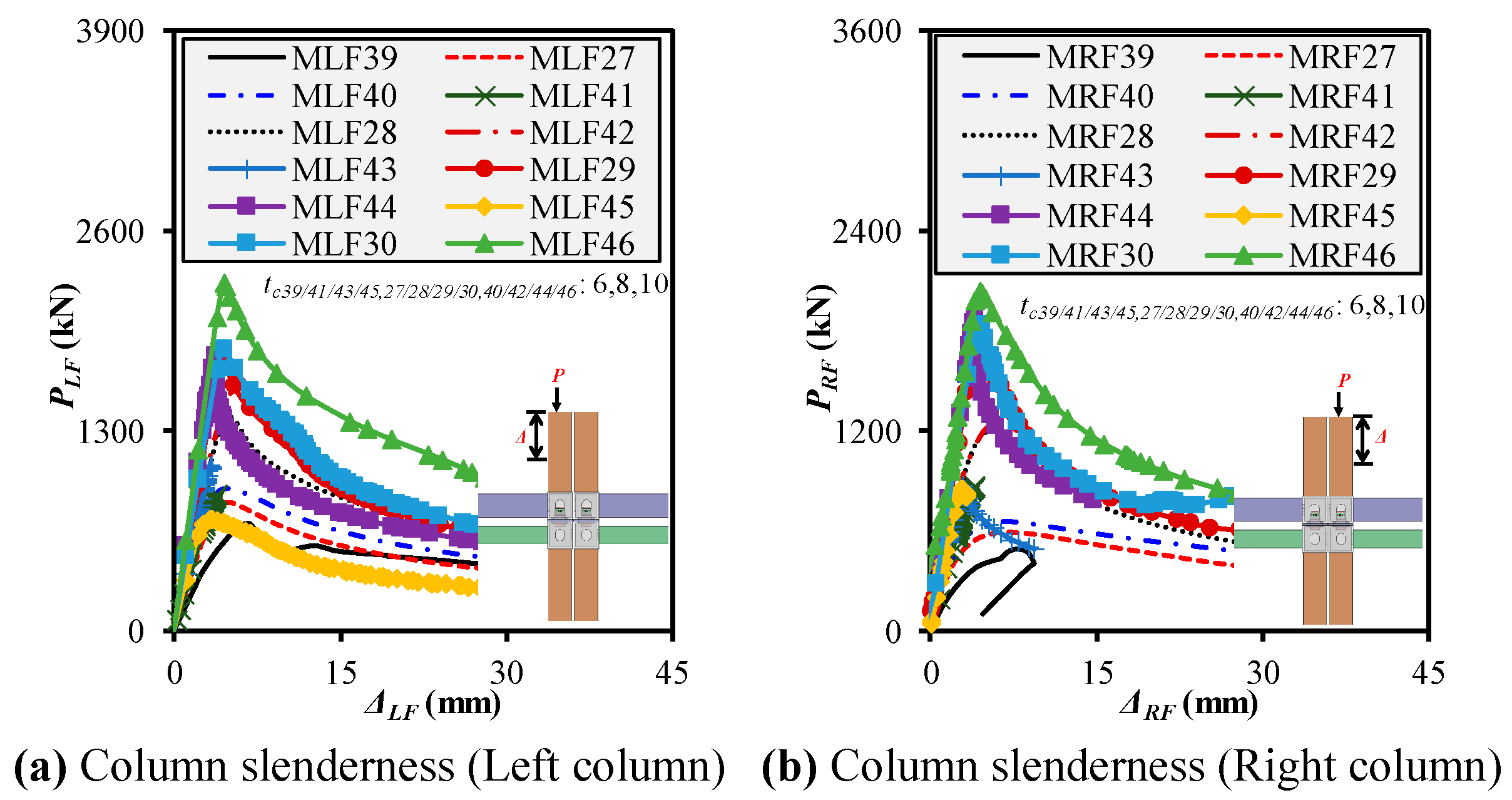
5.5. Effect of Column Slenderness on Progressive Behavior
5.6. Effect of Beam Gap on Progressive Behavior
5.7. Impact of Gap Between Adjacent Columns on Progressive Behaviors
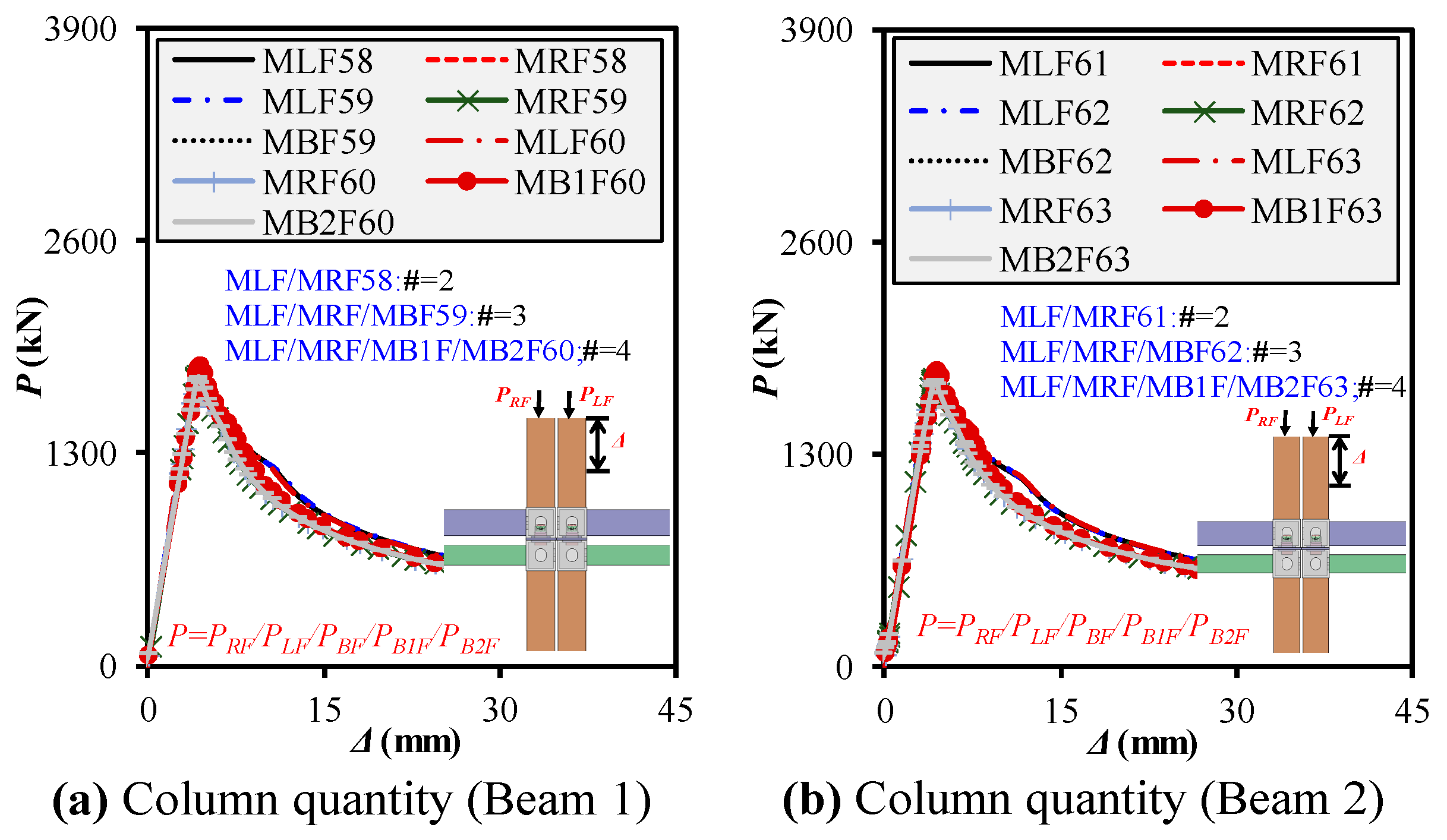
5.8. Impact of Number of Columns on Progressive Behaviors
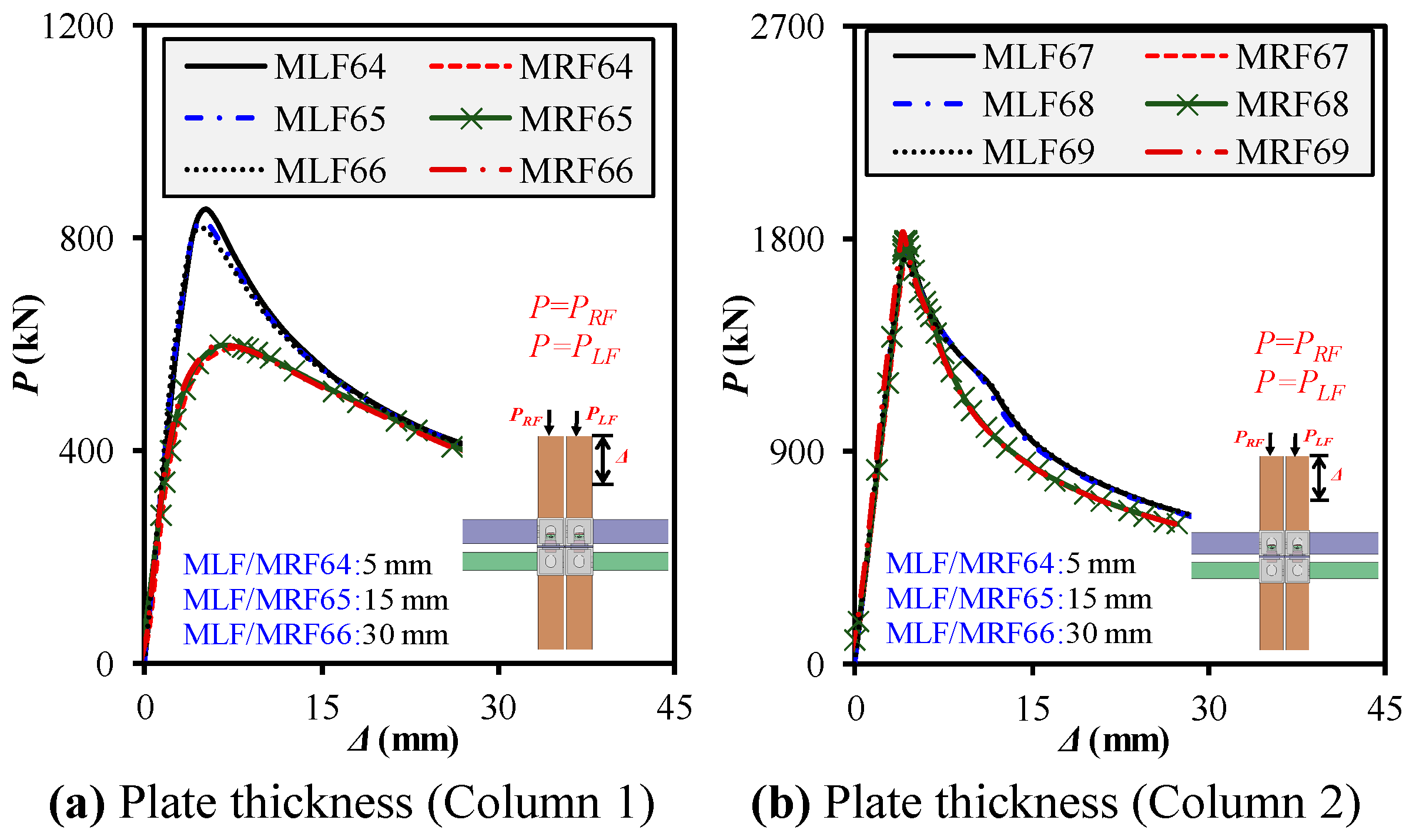
5.9. Impact of Connection Plate Thickness on Progressive Behaviors
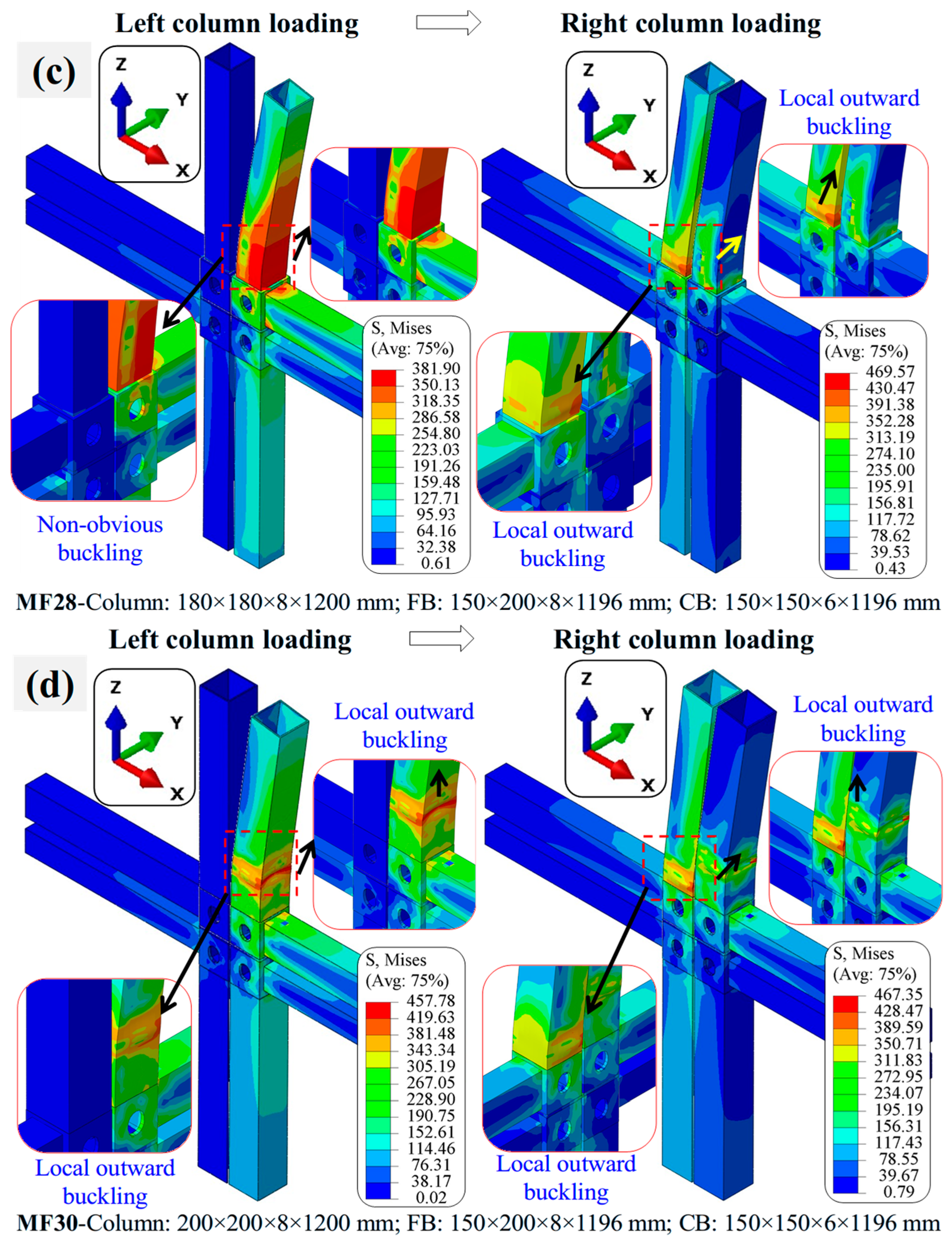
5.10. Typical Failure Modes Under Progressive Compression
6. Applicability of Conventional Steel Design Code Predictions
Validations
7. Conclusions
- Progressive failure in modular frames was predominantly governed by the upper columns, which underwent elastic-to-inelastic transition followed by local inward or outward buckling (from ultimate-to-recession phase), often near the M2M joints. Beams, lower columns, and M2M connections remained elastic, acting primarily as boundary restraints. This confirms that upper columns are the primary load-bearing elements under progressive compression.
- Consistent failure modes were characterized by the lateral sway of columns and local buckling patterns—typically forming in the same direction on opposite faces but at different heights. Buckling was primarily elastic and initiated within 400 mm of the column–M2M interface during initial (left-column) loading and shifted to elastic–plastic buckling behavior near 300 mm during right-column loading due to residual deformation. These phenomena were successfully captured in FEM simulations.
- The validated FEMs (with 30 mm element size, 8 mm local, and 3/18 mm global imperfection) accurately replicated both local and global responses under progressive loading. FEM predictions showed excellent agreement (within 2–10%) for strength and stiffness under both left- and right-column loading, confirming their suitability for modeling progressive behavior in sway-sensitive modular frames.
- Increasing the column cross section from 150 to 210 mm improved strength and stiffness by up to 121% and 96%, respectively, especially during left-column loading. Increasing the thickness from 6 to 10 mm resulted in strength and stiffness gains of over 200% and 100%, respectively. By contrast, increasing the column height to 3000 mm reduced strength by up to 54% due to elastic buckling. Due to cumulative sway effects and residual deformation, right-column loading consistently exhibited lower strength and more variable ductility. Beam/column gaps and connecting plate thickness had a minor influence (≤6%) on strength but affected ductility patterns. Increasing the number of columns provided strength gains and improved ductility up to 1.6×, enhancing redundancy and energy dissipation in progressive collapse scenarios.
- Average normalized ratios (Pu,FEM/Pu,code) were consistently below unity, which indicates that all six codes evaluated—EC3 (mean = 0.64), GB (0.66), IS (0.71), NZS (0.72), CSA (0.72), and AISC (0.72)—tended to overestimate column capacity. The Cov ranged from 0.25 to 0.27, which reflects significant scatter and underscores the inaccuracy of applying isolated-member-based predictions to sway-sensitive modular frames with semi-rigid M2M joints. The findings suggest that current code-based approaches require modification through stricter reduction factors, revised slenderness limits, or, ideally, the use of frame-level stability design methods that incorporate full-frame stiffness, rotational flexibility, and progressive failure characteristics.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
References
- Kazeem, K.O.; Olawumi, T.O.; Adam, J.J.; Lam, E.W.M. Integration of Building Services in Modular Construction: A PRISMA Approach. Appl. Sci. 2024, 14, 4151. [Google Scholar] [CrossRef]
- Gunawardena, T.; Ngo, T.; Mendis, P.; Alfano, J. Innovative Flexible Structural System Using Prefabricated Modules. J. Archit. Eng. 2016, 22, 05016003. [Google Scholar] [CrossRef]
- Wee, F.Y.Z. A Comprehensive Study on the Prefabricated Modular House. Bachelor’s Thesis, Universiti Tunku Abdul Rahman, Kampar, Malaysia, 2022. Volume 9. [Google Scholar]
- Thai, H.-T.; Ngo, T.; Uy, B. A Review on Modular Construction for High-Rise Buildings. Structures 2020, 28, 1265–1290. [Google Scholar] [CrossRef]
- Khan, K.; Chen, Z.; Liu, J.; Javed, K. State-of-the-Art on Technological Developments and Adaptability of Prefabricated Industrial Steel Buildings. Appl. Sci. 2023, 13, 685. [Google Scholar] [CrossRef]
- Ma, R.; Xia, J.; Chang, H.; Xu, B.; Zhang, L. Experimental and Numerical Investigation of Mechanical Properties on Novel Modular Connections with Superimposed Beams. Eng. Struct. 2021, 232, 111858. [Google Scholar] [CrossRef]
- Yang, C.; Xu, B.; Xia, J.; Chang, H.; Chen, X.; Ma, R. Mechanical Behaviors of Inter-Module Connections and Assembled Joints in Modular Steel Buildings: A Comprehensive Review. Buildings 2023, 13, 1727. [Google Scholar] [CrossRef]
- Habib, A.; Al Houri, A.; Alibrahim, B.; Habib, M. Behavior and Sustainability Benefits of Modular Steel Buildings. Int. J. Sustain. Eng. 2025, 18, 2459715. [Google Scholar] [CrossRef]
- Srisangeerthanan, S.; Hashemi, M.J.; Rajeev, P.; Gad, E.; Fernando, S. Review of Performance Requirements for Inter-Module Connections in Multi-Story Modular Buildings. J. Build. Eng. 2020, 28, 101087. [Google Scholar] [CrossRef]
- Chen, Z.; Wang, J.; Liu, J.; Cong, Z. Tensile and Shear Performance of Rotary Inter-Module Connection for Modular Steel Buildings. J. Constr. Steel Res. 2020, 175, 106367. [Google Scholar] [CrossRef]
- Liang, X.; Hou, C.; Peng, J. Experimental Study on Rotary Inter-Module Connections with Corrosion-Resistant Metal Materials: Under Axial Tension. Thin-Walled Struct. 2024, 205, 112507. [Google Scholar] [CrossRef]
- Peng, J.; Hou, C. Experimental and Numerical Study on a Novel Self-Locking Inter-Module Connection with Spring-Loaded Plunger Latches: Under Axial Tension. Eng. Struct. 2025, 324, 119364. [Google Scholar] [CrossRef]
- Liu, J.; Jiang, J.; Liu, Y.; Chen, Z.; Khan, K. Tensile Behavior of a Novel Self-Locking Inter-Module Connection in Modular Steel Buildings. Structures 2024, 62, 106190. [Google Scholar] [CrossRef]
- Li, T.; Tian, H.; Xiao, B.; Huang, Z.; Wang, X. Comparative Study on the Shear Resistance of L-Shaped and Square Grouted Sleeve Connections in Modular Integrated Construction. J. Constr. Steel Res. 2025, 229, 109521. [Google Scholar] [CrossRef]
- Lacey, A.W.; Chen, W.; Hao, H.; Bi, K.; Tallowin, F.J. Shear Behaviour of Post-Tensioned Inter-Module Connection for Modular Steel Buildings. J. Constr. Steel Res. 2019, 162, 105707. [Google Scholar] [CrossRef]
- Deng, E.-F.; Du, Y.-P.; Lian, J.-Y.; Zhang, Z.; Qian, H.; Zhang, J.-F.; Liu, C.-Z. Shear Behavior of the Fully Prefabricated Liftable Connection for Modular Steel Construction. J. Build. Eng. 2024, 92, 109793. [Google Scholar] [CrossRef]
- Dai, Z.; Cheong, T.Y.C.; Pang, S.D.; Liew, J.Y.R. Experimental Study of Grouted Sleeve Connections under Bending for Steel Modular Buildings. Eng. Struct. 2021, 243, 112614. [Google Scholar] [CrossRef]
- Yang, N.; Xia, J.; Chang, H.; Zhang, L. The Flexural Mechanical Response of a Novel Plug-in Self-Locking Inter-Module Connection for Modular Steel Buildings. Eng. Struct. 2024, 308, 118008. [Google Scholar] [CrossRef]
- Zhai, S.-Y.; Lyu, Y.-F.; Cao, K.; Li, G.-Q.; Wang, W.-Y.; Chen, C. Experimental-Numerical Investigation and Design of Bolted-Cover Plate Connections under Bending for Modular Steel Buildings. J. Build. Eng. 2023, 75, 107057. [Google Scholar] [CrossRef]
- Zhang, A.; Liu, J.; Chen, Z.; Chen, T. Bending Behavior of Detachable Tapered-Head Bolt Inter-Module Connection of Steel Modular Structure. J. Constr. Steel Res. 2024, 220, 108829. [Google Scholar] [CrossRef]
- Deng, E.-F.; Du, Y.-P.; Gao, J.-D.; Zhang, Z.; Wang, Y.-B. Seismic Performance of an Innovative Self-Centering and Repairable Connection with SMA Bolts in Modular Steel Construction. Thin-Walled Struct. 2024, 205, 112377. [Google Scholar] [CrossRef]
- Deng, E.-F.; Wang, Y.-H.; Zong, L.; Zhang, Z.; Zhang, J.-F. Seismic Behavior of a Novel Liftable Connection for Modular Steel Buildings: Experimental and Numerical Studies. Thin-Walled Struct. 2024, 197, 111563. [Google Scholar] [CrossRef]
- Liu, J.; Cao, X.; Yan, S.; Chen, Z.; Liu, Y.; Lu, G. Research on Seismic Behavior of Tapered-Head Bolted Inter-Module Connection of Modular Steel Buildings. Eng. Struct. 2024, 304, 117586. [Google Scholar] [CrossRef]
- Jiang, J.; Chen, Z.; Liu, Y.; Liu, X.; Lu, G.; Lin, X. Experimental and Numerical Investigation on the Seismic Behavior of a Novel Bolted Inter-Module Connection. J. Constr. Steel Res. 2025, 224, 109101. [Google Scholar] [CrossRef]
- Deng, E.-F.; Lian, J.-Y.; Zhang, Z.; Qian, H.; Zhang, G.-C.; Zhang, P.; Sheikh, S.A. Axial Mechanical Behavior of an Innovative Liftable Connection for Modular Steel Construction. Thin-Walled Struct. 2023, 182, 110256. [Google Scholar] [CrossRef]
- Shi, F.-W.; Ding, Y.; Zong, L.; Meng, X.; Chen, Y. Axial Mechanical Behavior of Innovative Inter-Module Connection for Modular Steel Constructions. J. Build. Eng. 2023, 65, 105765. [Google Scholar] [CrossRef]
- Chan, T.M.; Gardner, L. Compressive Resistance of Hot-Rolled Elliptical Hollow Sections. Eng. Struct. 2008, 30, 522–532. [Google Scholar] [CrossRef]
- Meng, X.; Gardner, L. Testing of Hot-Finished High Strength Steel SHS and RHS under Combined Compression and Bending. Thin-Walled Struct. 2020, 148, 106262. [Google Scholar] [CrossRef]
- Ma, J.-L.; Chan, T.-M.; Young, B. Experimental Investigation on Stub-Column Behavior of Cold-Formed High-Strength Steel Tubular Sections. J. Struct. Eng. 2016, 142, 04015174. [Google Scholar] [CrossRef]
- Chen, J.; Chan, T.-M.; Varma, A.H. Stub Column Behavior of Cold-Formed High-Strength Steel Circular Hollow Sections under Compression. J. Struct. Eng. 2020, 146, 04020277. [Google Scholar] [CrossRef]
- ANSI/AISC 360-16; AISC360/16 Specification for Structural Steel Buildings-An American National Standard. American Institute of Steel Construction: Chicago, IL, USA, 2016.
- EN 1993-1-1; Eurocode 3: Design of Steel Structures-Part 1-1: General Rules and Rules for Buildings. European Committee for Standardization: Brussels, Belgium, 2005; Volume 1, ISBN 0580663876, 9780580663871.
- GB50017-2017; Standard for Design of Steel Structures. National Standard of China: Beijing, China, 2017. (In Chinese)
- Mark Lawson, R.; Richards, J. Modular Design for High-Rise Buildings. Proc. Inst. Civ. Eng. Struct. Build. 2010, 163, 151–164. [Google Scholar] [CrossRef]
- Hou, J.; Wang, X.; Liu, J.; Chen, Z.; Zhong, X. Study on the Stability Bearing Capacity of Multi-Column Wall in Modular Steel Building. Eng. Struct. 2020, 214, 110648. [Google Scholar] [CrossRef]
- Khan, K.; Chen, Z.; Liu, J.; Khan, A. Numerical and Parametric Analysis on Compressive Behaviours of Continuous-Supported Wall Systems in MSB. Structures 2021, 33, 4053–4079. [Google Scholar] [CrossRef]
- Khan, K.; Chen, Z.; Liu, J.; Khan, A.; Javed, K. Axial Compression Behaviours of Tubular Sectioned C-Shape Continuous-Supported Steel Walls in MSB. J. Constr. Steel Res. 2022, 188, 107009. [Google Scholar] [CrossRef]
- Khan, K.; Chen, Z.; Liu, J.; Khan, A. Experimental and Numerical Study on Planar Multi-Column Walls Behaviours with Boundary Supports. J. Constr. Steel Res. 2021, 186, 106880. [Google Scholar] [CrossRef]
- Chen, Y.; Hou, C.; Peng, J. Stability Study on Tenon-Connected SHS and CFST Columns in Modular Construction. Steel Compos. Struct. 2019, 30, 185–199. [Google Scholar] [CrossRef]
- Khan, K.; Chen, Z.; Youssef, M.A.; Abbas, D. Numerical and Theoretical Studies on Axial Compression Performance of Modular Steel Tubular Columns Grouped with Shear-Key Connectors. Buildings 2024, 14, 2018. [Google Scholar] [CrossRef]
- Deng, E.F.; Yan, J.B.; Ding, Y.; Zong, L.; Li, Z.X.; Dai, X.M. Analytical and Numerical Studies on Steel Columns with Novel Connections in Modular Construction. Int. J. Steel Struct. 2017, 17, 1613–1626. [Google Scholar] [CrossRef]
- Khan, K.; Chen, Z.; Liu, J.; Tsavdaridis, K.D.; Poologanathan, K. Axial Compression Behaviors of Steel Shear-Keyed Tubular Columns: Numerical and Analytical Studies. J. Constr. Steel Res. 2023, 205, 107894. [Google Scholar] [CrossRef]
- Khan, K.; Chen, Z.; Liu, J.; Javed, K.; Tsavdaridis, K.D.; Poologanathan, K. Compressive Behaviors of Modular Steel Shear-Keyed Grouped Tubular Columns. J. Build. Eng. 2023, 66, 105861. [Google Scholar] [CrossRef]
- Khan, K.; Chen, Z.; Liu, J.; Javed, K. Experimental and Analytical Investigations on Compressive Behaviors of Modular Steel Shear-Keyed Tubes. Eng. Struct. 2023, 279, 115604. [Google Scholar] [CrossRef]
- Zhang, G.; Xu, L.; Xie, X.; Bi, K. Seismic Evaluation of a Self-Centering Retrofit Solution for Modular Steel Structure Connections. J. Constr. Steel Res. 2024, 218, 108701. [Google Scholar] [CrossRef]
- Xu, B.; Xia, J.; Chang, H.; Ma, R.; Zhang, L. Evaluation of Superimposed Bending Behaviour of Laminated Channel Beams in Modular Steel Buildings Subjected to Lateral Load. Thin-Walled Struct. 2022, 175, 109234. [Google Scholar] [CrossRef]
- Chen, Z.; Liu, J.; Yu, Y.; Zhou, C.; Yan, R. Experimental Study of an Innovative Modular Steel Building Connection. J. Constr. Steel Res. 2017, 139, 69–82. [Google Scholar] [CrossRef]
- Shi, F.-W.; Li, Y.-M. Innovative Inner Sleeve Composite Bolted Connections for Modular Steel Constructions: Experimental and Numerical Studies. J. Build. Eng. 2023, 64, 105624. [Google Scholar] [CrossRef]
- Hwan Doh, J.; Ho, N.M.; Miller, D.; Peters, T.; Carlson, D.; Lai, P. Steel Bracket Connection on Modular Buildings. J. Steel Struct. Constr. 2017, 2, 1000121. [Google Scholar] [CrossRef]
- Lee, S.S.; Park, K.S.; Jung, J.S.; Lee, K.S. Evaluation of the Structural Performance of a Novel Methodology for Connecting Modular Units Using Straight and Cross-Shaped Connector Plates in Modular Buildings. Appl. Sci. 2020, 10, 8186. [Google Scholar] [CrossRef]
- Lyu, Y.-F.; Li, G.-Q.; Cao, K.; Zhai, S.-Y.; Li, H.; Chen, C.; Wang, Y.-Z. Behavior of Splice Connection during Transfer of Vertical Load in Full-Scale Corner-Supported Modular Building. Eng. Struct. 2021, 230, 111698. [Google Scholar] [CrossRef]
- Lacey, A.W.; Chen, W.; Hao, H.; Bi, K. Review of Bolted Inter-Module Connections in Modular Steel Buildings. J. Build. Eng. 2019, 23, 207–219. [Google Scholar] [CrossRef]
- Annan, C.D.; Youssef, M.A.; El Naggar, M.H. Experimental Evaluation of the Seismic Performance of Modular Steel-Braced Frames. Eng. Struct. 2009, 31, 1435–1446. [Google Scholar] [CrossRef]
- Fathieh, A.; Mercan, O. Seismic Evaluation of Modular Steel Buildings. Eng. Struct. 2016, 122, 83–92. [Google Scholar] [CrossRef]
- Nadeem, G.; Safiee, N.A.; Bakar, N.A.; Karim, I.A.; Mohd Nasir, N.A. Experimental and Numerical Study of Self-Locking Adaptable Inter Connection for Modular Steel Structures. J. Build. Eng. 2023, 65, 105723. [Google Scholar] [CrossRef]
- Liu, X.; Meng, Q.; Xu, L.; Liu, Y.; Tian, X. Modular Steel Buildings Based on Self-Locking-Unlockable Connections Seismic Performance Analysis. Buildings 2025, 15, 678. [Google Scholar] [CrossRef]
- Lacey, A.W.; Chen, W.; Hao, H.; Bi, K. New Interlocking Inter-Module Connection for Modular Steel Buildings: Experimental and Numerical Studies. Eng. Struct. 2019, 198, 109465. [Google Scholar] [CrossRef]
- Lee, K.; Cho, B.H.; Rasmussen, K.J.R.; Jung, D. Experimental and Numerical Investigation of Bolt-Free Preloaded Connection for Steel-Framed Modular Buildings. J. Constr. Steel Res. 2024, 220, 108827. [Google Scholar] [CrossRef]
- Sanches, R.; Mercan, O.; Roberts, B. Experimental Investigations of Vertical Post-Tensioned Connection for Modular Steel Structures. Eng. Struct. 2018, 175, 776–789. [Google Scholar] [CrossRef]
- Liew, J.Y.R.; Chua, Y.S.; Dai, Z. Steel Concrete Composite Systems for Modular Construction of High-Rise Buildings. Structures 2019, 21, 135–149. [Google Scholar] [CrossRef]
- Kujawa, M.; Smakosz, Ł.; Iwicki, P.; Perliński, A.; Tejchman, J. Mechanical Performance Investigations of a Post-Tensioned Inter-Module Connection in Steel Buildings. Structures 2025, 71, 108187. [Google Scholar] [CrossRef]
- Chen, Z.; Liu, Y.; Zhong, X.; Liu, J. Rotational Stiffness of Inter-Module Connection in Mid-Rise Modular Steel Buildings. Eng. Struct. 2019, 196, 109273. [Google Scholar] [CrossRef]
- Khan, K.; Chen, Z.; Liu, J.; Tsavdaridis, K.D. Compressive Behaviors of Corner-Supported Modular Steel Sway Frames with Rotary Inter-Modular Connections. Thin-Walled Struct. 2023, 193, 111245. [Google Scholar] [CrossRef]
- Farajian, M.; Sharafi, P.; Eslamnia, H.; Kildashti, K.; Bai, Y. Classification of Inter-Modular Connections for Stiffness and Strength in Sway Corner-Supported Steel Modular Frames. J. Constr. Steel Res. 2022, 197, 107458. [Google Scholar] [CrossRef]
- He, X.-H.-C.; Chan, T.-M. Classification System for Inter- and Intra-Module Joints in Non-Sway Steel MiC Structures. J. Constr. Steel Res. 2023, 206, 107913. [Google Scholar] [CrossRef]
- Farajian, M.; Sharafi, P.; Eslamnia, H.; Bai, Y.; Samali, B. Classification System for Inter-Modular Connections in Non-Sway Corner-Supported Steel Modular Buildings. Structures 2023, 49, 807–825. [Google Scholar] [CrossRef]
- Zhai, X.; Zha, X.; Chen, D. Elastic Stability of Unbraced Plate-Type Modular Steel Frames with Semi-Rigid Corner Connections. J. Constr. Steel Res. 2022, 192, 107243. [Google Scholar] [CrossRef]
- Wang, Q.; Su, M. Stability Study on Sway Modular Steel Structures with Semi-Rigid Connections. Thin-Walled Struct. 2021, 161, 107529. [Google Scholar] [CrossRef]
- Alembagheri, M.; Sharafi, P.; Hajirezaei, R.; Tao, Z. Anti-Collapse Resistance Mechanisms in Corner-Supported Modular Steel Buildings. J. Constr. Steel Res. 2020, 170, 106083. [Google Scholar] [CrossRef]
- Luo, F.J.; Bai, Y.; Hou, J.; Huang, Y. Progressive Collapse Analysis and Structural Robustness of Steel-Framed Modular Buildings. Eng. Fail. Anal. 2019, 104, 643–656. [Google Scholar] [CrossRef]
- He, X.-H.-C.; Meng, G.; An, Y. Progressive Collapse Resistance of Steel Modular Integrated Construction Structures: Comparison with Steel Frame Structures and an Enhancement Method. J. Build. Eng. 2025, 104, 112382. [Google Scholar] [CrossRef]
- Chua, Y.S.; Pang, S.D.; Liew, J.Y.R.; Dai, Z. Robustness of Inter-Module Connections and Steel Modular Buildings under Column Loss Scenarios. J. Build. Eng. 2022, 47, 103888. [Google Scholar] [CrossRef]
- Peng, J.; Hou, C.; Shen, L. Progressive Collapse Analysis of Corner-Supported Composite Modular Buildings. J. Build. Eng. 2022, 48, 103977. [Google Scholar] [CrossRef]
- Yu, Z.-W.; Ma, W.-L.; Zhang, J.-Z.; Tian, Z. Comparative Study on Collapse Behavior of Modular Steel Buildings: Experiment and Analysis. Thin-Walled Struct. 2025, 206, 112695. [Google Scholar] [CrossRef]
- Shan, S.; Pan, W. Progressive Collapse Mechanisms of Multi-Story Steel-Framed Modular Structures under Module Removal Scenarios. Structures 2022, 46, 1119–1133. [Google Scholar] [CrossRef]
- Jiaxi, M. Performance Evaluation Method Research of Modular Prefabricated Steel Structure to Resist Progressive Collapse. Build. Sci. 2015, 31, 87–97. (In Chinese) [Google Scholar]
- Zhang, J.-Z.; Lin, X.; Zhang, W.-J.; Yu, Z.-W. Collapse Behavior and Resistance Mechanisms of Steel Modular Buildings with Corrugated Walls: Experimental, Numerical, and Analytical Insights. Thin-Walled Struct. 2025, 212, 113157. [Google Scholar] [CrossRef]
- NZS3404; Part 1:1997-Steel Structures Standard. ISO: Geneva, Switzerland, 1997.
- IS 801-1975; Indian Standard Code of Practice for Use of Cold-Formed Light Gauge Steel Structural Members in General Building Construction. Bureau of Indian Standards: New Delhi, India, 1975.
- IS 801-1975; Indian Standard Code of Practice for General Construction in Steel. Bureau of Indian Standards: New Delhi, India, 2007.
- CSA-S16-09; CSA Standard-Design of Steel Structures. Canadian Standards Association: Toronto, ON, Canada, 2009; p. 7.
- Han, X.-Z.; Wang, C.; He, X.-H.-C.; Hu, Y.-F.; Chan, T.-M.; Chung, K.-F. Experimental Investigation on Bolted Inter-Module Connections and Subassemblies of Steel Modular Frames. J. Constr. Steel Res. 2023, 211, 108118. [Google Scholar] [CrossRef]
- GB/T 228.1-2010; Metallic Materials-Tensile Testing-Part 1: Method of Test at Room Temperature. National Standard of China: Beijing, China, 2010. (In Chinese)
- GBT50344-2019; Technical Standards for Building Structure Inspection. National Standard of China: Beijing, China, 2019. (In Chinese)
- ABAQUS, User Manual Version 6.23; DS SIMULIA Corp.: Providence, RI, USA, 2023.
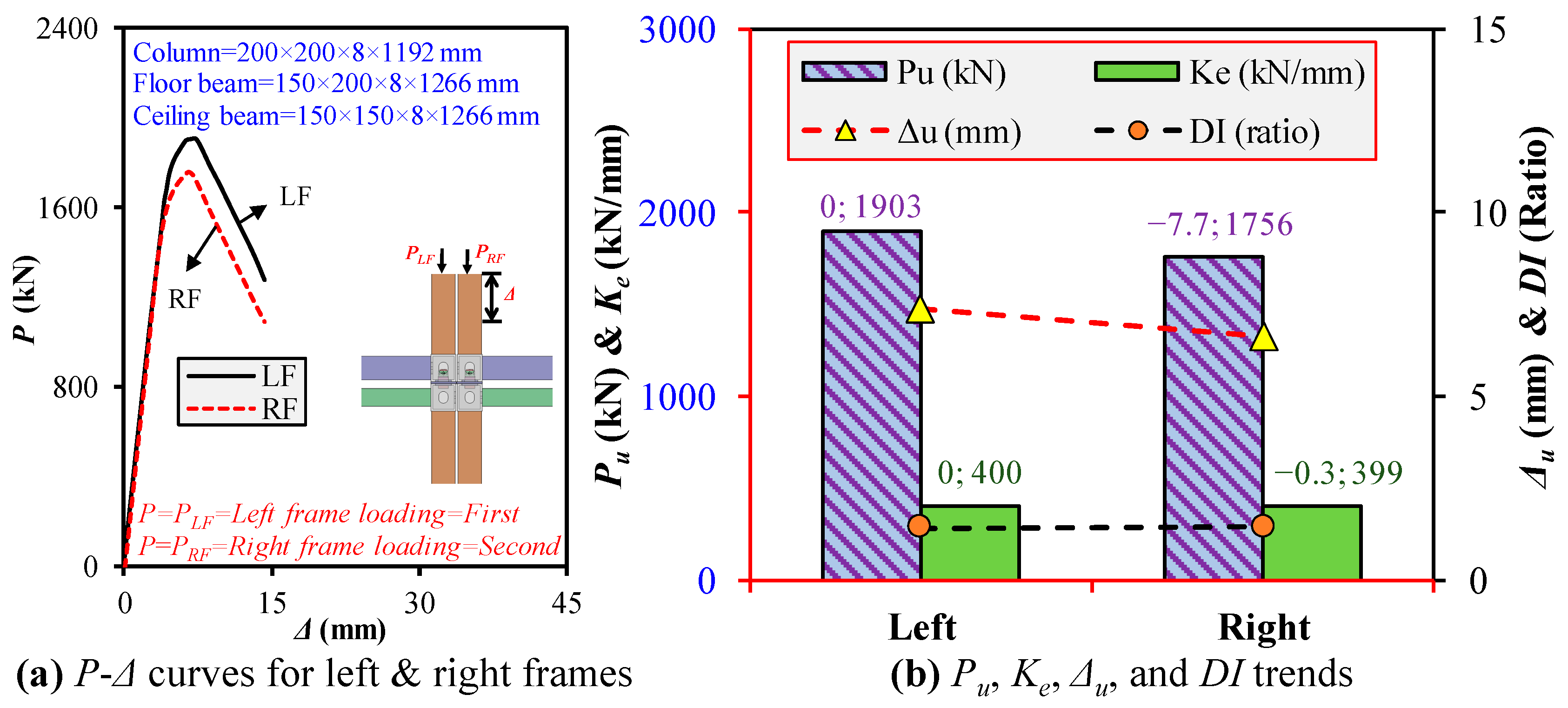




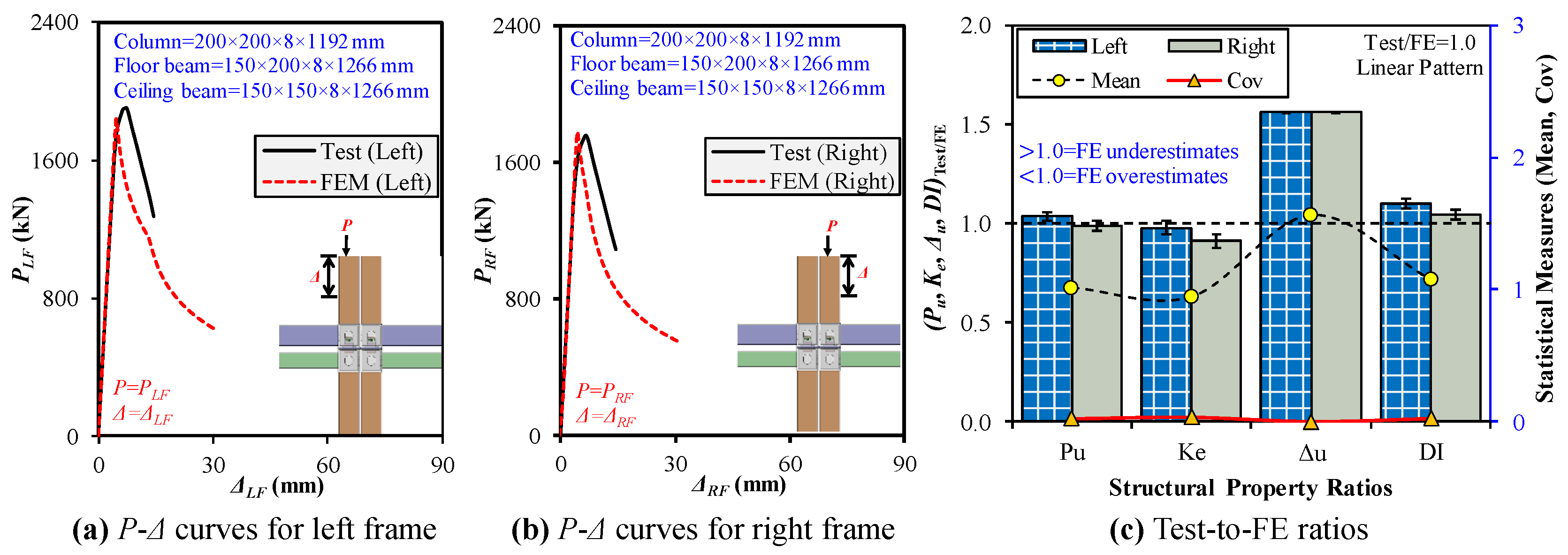






| Items | (D/B/t)c (mm) | (D/B/t)FB (mm) | (D/B/t)CB (mm) | Lc/Lb (m) | (Pu)Test/FEM (kN) | (Ke)Test/FEM (kN/mm) | (Δu)Test/FEM (mm) | (DI)Test/FEM (Ratio) |
|---|---|---|---|---|---|---|---|---|
| MLF | 200/200/8 | 150/200/8 | 150/150/8 | 1.26/1.19 | 1903/1838 | 400/409 | 7.4/4.7 | 1.5/1.3 |
| MRF | 1756/1778 | 399/438 | 6.7/4.3 | 1.5/1.4 | ||||
| 1.04 | 0.98 | 1.56 | 1.10 | |||||
| 0.99 | 0.91 | 1.56 | 1.05 | |||||
| Mean | 1.01 | 0.94 | 1.56 | 0.03 | ||||
| Cov | 0.02 | 0.04 | 0.00 | 0.02 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, K.; Khan, K.; Yang, Y.; Jiang, L.; Chen, Z. Impact of Inter-Modular Connections on Progressive Compressive Behavior of Prefabricated Column-Supported Volumetric Modular Steel Frames. Crystals 2025, 15, 413. https://doi.org/10.3390/cryst15050413
Yang K, Khan K, Yang Y, Jiang L, Chen Z. Impact of Inter-Modular Connections on Progressive Compressive Behavior of Prefabricated Column-Supported Volumetric Modular Steel Frames. Crystals. 2025; 15(5):413. https://doi.org/10.3390/cryst15050413
Chicago/Turabian StyleYang, Kejia, Kashan Khan, Yukun Yang, Lu Jiang, and Zhihua Chen. 2025. "Impact of Inter-Modular Connections on Progressive Compressive Behavior of Prefabricated Column-Supported Volumetric Modular Steel Frames" Crystals 15, no. 5: 413. https://doi.org/10.3390/cryst15050413
APA StyleYang, K., Khan, K., Yang, Y., Jiang, L., & Chen, Z. (2025). Impact of Inter-Modular Connections on Progressive Compressive Behavior of Prefabricated Column-Supported Volumetric Modular Steel Frames. Crystals, 15(5), 413. https://doi.org/10.3390/cryst15050413







