Delicate Competition Between Different Excitonic Orderings in Ta2NiSe5
Abstract
1. Introduction
2. Model and Methods
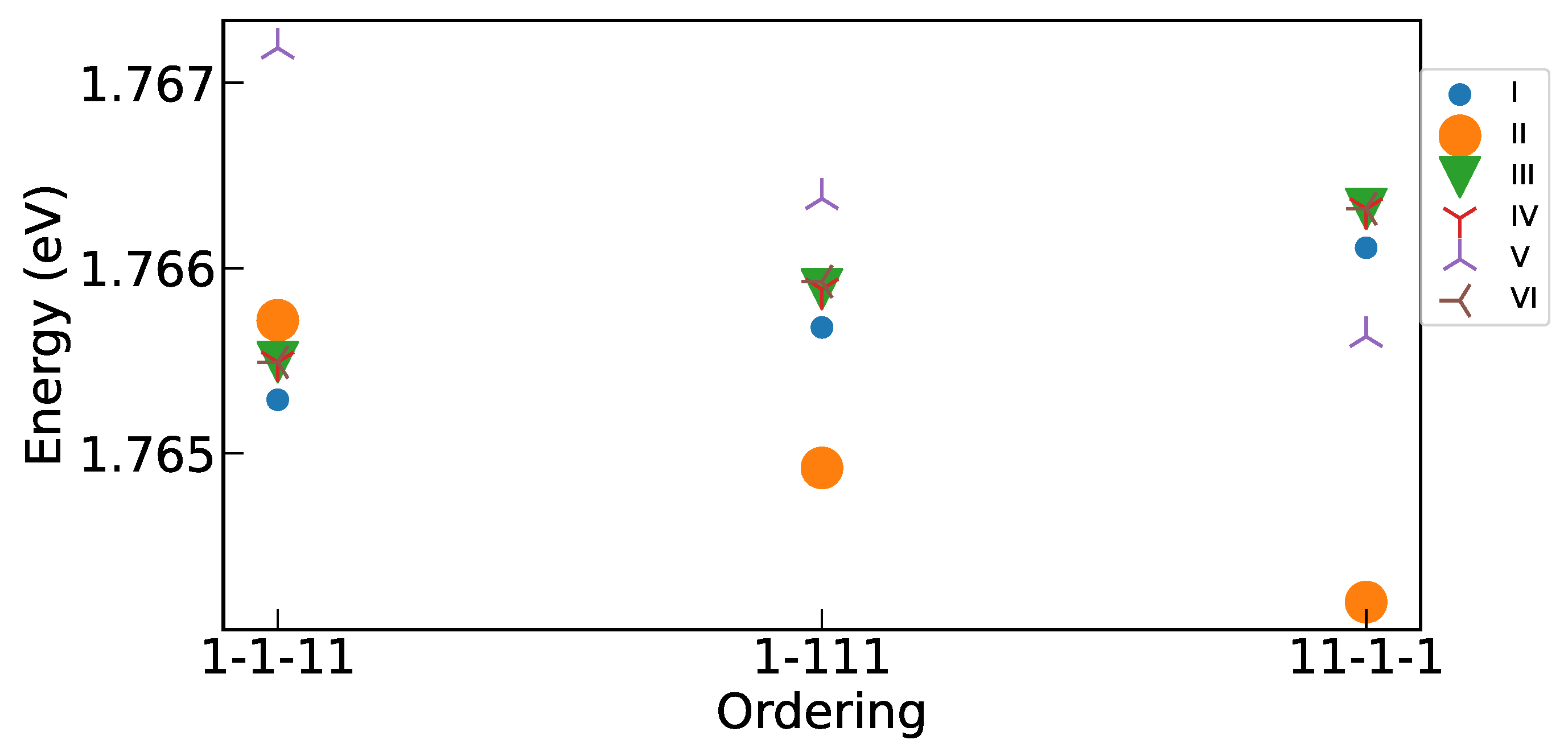
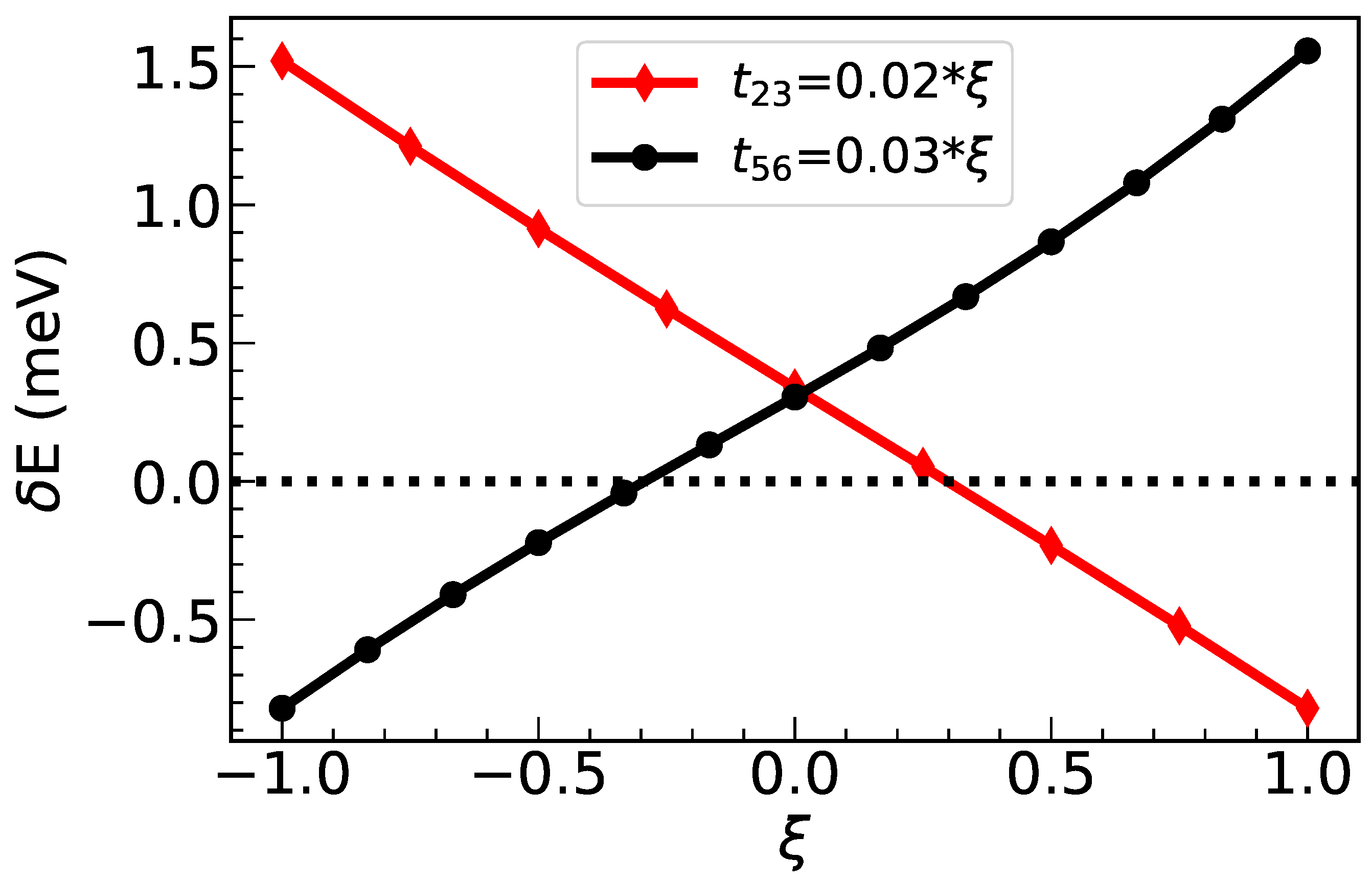
3. Results
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviation
| TNS |
References
- Sunshine, S.A.; Ibers, J.A. Structure and physical properties of the new layered ternary chalcogenides tantalum nickel sulfide (Ta2NiS5) and tantalum nickel selenide (Ta2NiSe5). Inorg. Chem. 1985, 24, 3611–3614. [Google Scholar] [CrossRef]
- Di Salvo, F.; Chen, C.; Fleming, R.; Waszczak, J.; Dunn, R.; Sunshine, S.; Ibers, J.A. Physical and structural properties of the new layered compounds Ta2NiS5 and Ta2NiSe5. J. Less Common Met. 1986, 116, 51–61. [Google Scholar] [CrossRef]
- Lu, Y.; Kono, H.; Larkin, T.; Rost, A.; Takayama, T.; Boris, A.; Keimer, B.; Takagi, H. Zero-gap semiconductor to excitonic insulator transition in Ta2NiSe5. Nat. Commun. 2017, 8, 14408. [Google Scholar] [CrossRef] [PubMed]
- Subedi, A. Orthorhombic-to-monoclinic transition in Ta2NiSe5 due to a zone-center optical phonon instability. Phys. Rev. Mater. 2020, 4, 083601. [Google Scholar] [CrossRef]
- Lee, J.; Kang, C.J.; Eom, M.J.; Kim, J.S.; Min, B.I.; Yeom, H.W. Strong interband interaction in the excitonic insulator phase of Ta2NiSe5. Phys. Rev. B 2019, 99, 075408. [Google Scholar] [CrossRef]
- Watson, M.D.; Marković, I.; Morales, E.A.; Le Fèvre, P.; Merz, M.; Haghighirad, A.A.; King, P.D. Band hybridization at the semimetal-semiconductor transition of Ta2NiSe5 enabled by mirror-symmetry breaking. Phys. Rev. Res. 2020, 2, 013236. [Google Scholar] [CrossRef]
- Wakisaka, Y.; Sudayama, T.; Takubo, K.; Mizokawa, T.; Arita, M.; Namatame, H.; Taniguchi, M.; Katayama, N.; Nohara, M.; Takagi, H. Excitonic insulator state in Ta2NiSe5 probed by photoemission spectroscopy. Phys. Rev. Lett. 2009, 103, 026402. [Google Scholar] [CrossRef]
- Wakisaka, Y.; Sudayama, T.; Takubo, K.; Mizokawa, T.; Saini, N.; Arita, M.; Namatame, H.; Taniguchi, M.; Katayama, N.; Nohara, M.; et al. Photoemission spectroscopy of Ta2NiSe5. J. Supercond. Nov. Magn. 2012, 25, 1231–1234. [Google Scholar] [CrossRef]
- Seki, K.; Wakisaka, Y.; Kaneko, T.; Toriyama, T.; Konishi, T.; Sudayama, T.; Saini, N.; Arita, M.; Namatame, H.; Taniguchi, M.; et al. Excitonic Bose–Einstein condensation in Ta2NiSe5 above room temperature. Phys. Rev. B 2014, 90, 155116. [Google Scholar] [CrossRef]
- Des Cloizeaux, J. Exciton instability and crystallographic anomalies in semiconductors. J. Phys. Chem. Solids 1965, 26, 259–266. [Google Scholar] [CrossRef]
- Jérome, D.; Rice, T.; Kohn, W. Excitonic insulator. Phys. Rev. 1967, 158, 462–475. [Google Scholar] [CrossRef]
- Kuneš, J. Excitonic condensation in systems of strongly correlated electrons. J. Phys. Condens. Matter 2015, 27, 333201. [Google Scholar] [CrossRef]
- Kaneko, T.; Toriyama, T.; Konishi, T.; Ohta, Y. Electronic structure of Ta2NiSe5 as a candidate for excitonic insulators. J. Phys. Conf. Ser. 2012, 400, 032035. [Google Scholar] [CrossRef]
- Kaneko, T.; Toriyama, T.; Konishi, T.; Ohta, Y. Orthorhombic-to-monoclinic phase transition of Ta2NiSe5 induced by the Bose–Einstein condensation of excitons. Phys. Rev. B 2013, 87, 035121. [Google Scholar] [CrossRef]
- Mor, S.; Herzog, M.; Golež, D.; Werner, P.; Eckstein, M.; Katayama, N.; Nohara, M.; Takagi, H.; Mizokawa, T.; Monney, C.; et al. Ultrafast Electronic Band Gap Control in an Excitonic Insulator. Phys. Rev. Lett. 2017, 119, 086401. [Google Scholar] [CrossRef]
- Mor, S.; Herzog, M.; Noack, J.; Katayama, N.; Nohara, M.; Takagi, H.; Trunschke, A.; Mizokawa, T.; Monney, C.; Stähler, J. Inhibition of the photoinduced structural phase transition in the excitonic insulator Ta2NiSe5. Phys. Rev. B 2018, 97, 115154. [Google Scholar] [CrossRef]
- Okazaki, K.; Ogawa, Y.; Suzuki, T.; Yamamoto, T.; Someya, T.; Michimae, S.; Watanabe, M.; Lu, Y.; Nohara, M.; Takagi, H.; et al. Photo-induced semimetallic states realised in electron–hole coupled insulators. Nat. Commun. 2018, 9, 4322. [Google Scholar] [CrossRef]
- Bretscher, H.M.; Andrich, P.; Telang, P.; Singh, A.; Harnagea, L.; Sood, A.K.; Rao, A. Ultrafast melting and recovery of collective order in the excitonic insulator Ta2NiSe5. Nat. Commun. 2021, 12, 1699. [Google Scholar] [CrossRef]
- Saha, T.; Golež, D.; De Ninno, G.; Mravlje, J.; Murakami, Y.; Ressel, B.; Stupar, M.; Ribič, P.R. Photoinduced phase transition and associated timescales in the excitonic insulator Ta2NiSe5. Phys. Rev. B 2021, 103, 144304. [Google Scholar] [CrossRef]
- Nakano, A.; Nagai, T.; Katayama, N.; Sawa, H.; Taniguchi, H.; Terasaki, I. Exciton Transport in the Electron–Hole System Ta2NiSe5. J. Phys. Soc. Jpn. 2019, 88, 113706. [Google Scholar] [CrossRef]
- Eisenstein, J. Exciton Condensation in Bilayer Quantum Hall Systems. Annu. Rev. Condens. Matter Phys. 2014, 5, 159–181. [Google Scholar] [CrossRef]
- Li, J.I.A.; Taniguchi, T.; Watanabe, K.; Hone, J.; Dean, C.R. Excitonic superfluid phase in double bilayer graphene. Nat. Phys. 2017, 13, 751–755. [Google Scholar] [CrossRef]
- Liu, X.; Watanabe, K.; Taniguchi, T.; Halperin, B.I.; Kim, P. Quantum Hall drag of exciton condensate in graphene. Nat. Phys. 2017, 13, 746–750. [Google Scholar] [CrossRef]
- Wang, Z.; Rhodes, D.A.; Watanabe, K.; Taniguchi, T.; Hone, J.C.; Shan, J.; Mak, K.F. Evidence of high-temperature exciton condensation in two-dimensional atomic double layers. Nature 2019, 574, 76–80. [Google Scholar] [CrossRef]
- Gupta, S.; Kutana, A.; Yakobson, B.I. Heterobilayers of 2D materials as a platform for excitonic superfluidity. Nat. Commun. 2020, 11, 2989. [Google Scholar] [CrossRef] [PubMed]
- Nilsson, F.; Kuisma, M.; Pakdel, S.; Thygesen, K.S. Excitonic Insulators and Superfluidity in Two-Dimensional Bilayers without External Fields. J. Phys. Chem. Lett. 2023, 14, 2277–2283. [Google Scholar] [CrossRef]
- Mazza, G.; Rösner, M.; Windgätter, L.; Latini, S.; Hübener, H.; Millis, A.J.; Rubio, A.; Georges, A. Nature of symmetry breaking at the excitonic insulator transition: Ta 2 NiSe 5. Phys. Rev. Lett. 2020, 124, 197601. [Google Scholar] [CrossRef] [PubMed]
- Windgätter, L.; Rösner, M.; Mazza, G.; Hübener, H.; Georges, A.; Millis, A.J.; Latini, S.; Rubio, A. Common microscopic origin of the phase transitions in Ta2NiS5 and the excitonic insulator candidate Ta2NiSe5. npj Comput. Mater. 2021, 7, 210. [Google Scholar] [CrossRef]
- Baldini, E.; Zong, A.; Choi, D.; Lee, C.; Michael, M.H.; Windgaetter, L.; Mazin, I.I.; Latini, S.; Azoury, D.; Lv, B.; et al. The spontaneous symmetry breaking in Ta2NiSe5 is structural in nature. Proc. Natl. Acad. Sci. 2023, 120, e2221688120. [Google Scholar] [CrossRef]
- Katsumi, K.; Alekhin, A.; Souliou, S.M.; Merz, M.; Haghighirad, A.A.; Le Tacon, M.; Houver, S.; Cazayous, M.; Sacuto, A.; Gallais, Y. Disentangling Lattice and Electronic Instabilities in the Excitonic Insulator Candidate Ta2NiSe5 by Nonequilibrium Spectroscopy. Phys. Rev. Lett. 2023, 130, 106904. [Google Scholar] [CrossRef]
- Chatterjee, B.; Mravlje, J.; Golež, D. Collective modes and Raman response in Ta2NiSe5. Phys. Rev. B 2025, 111, L121106. [Google Scholar] [CrossRef]
- Mostofi, A.A.; Yates, J.R.; Lee, Y.S.; Souza, I.; Vanderbilt, D.; Marzari, N. wannier90: A tool for obtaining maximally localised Wannier functions. Comput. Phys. Commun. 2008, 178, 685–699. [Google Scholar] [CrossRef]
- Sun, Z.; Kaneko, T.; Golež, D.; Millis, A.J. Second-order Josephson effect in excitonic insulators. Phys. Rev. Lett. 2021, 127, 127702. [Google Scholar] [CrossRef]
- Huang, J.; Jiang, B.; Yao, J.; Yan, D.; Lei, X.; Gao, J.; Guo, Z.; Jin, F.; Li, Y.; Yuan, Z.; et al. Evidence for an excitonic insulator state in Ta2Pd3Te5. Phys. Rev. X 2024, 14, 011046. [Google Scholar] [CrossRef]
- Zhang, P.; Dong, Y.; Yan, D.; Jiang, B.; Yang, T.; Li, J.; Guo, Z.; Huang, Y.; Haobo; Li, Q.; et al. Spontaneous gap opening and potential excitonic states in an ideal Dirac semimetal Ta2Pd3Te5. Phys. Rev. X 2024, 14, 011047. [Google Scholar] [CrossRef]


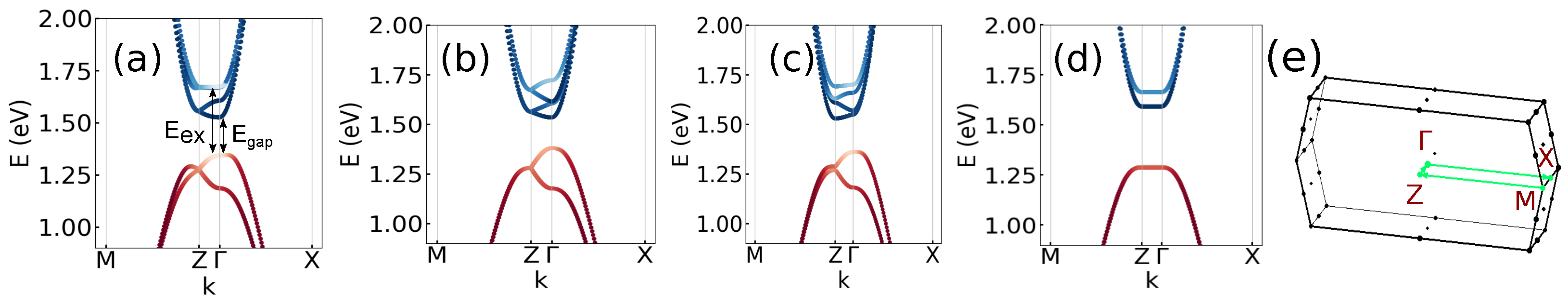

| Cases | Hopping Matrix Elements t() |
|---|---|
| Intra-chain Ta-Ta | (, 0) = (, 0) = −0.72 eV |
| (0, 0) = 1.35 eV, i = 1,...,4 | |
| Intra-chain Ni-Ni | (, 0) = (, 0) = 0.30 eV, |
| (0, 0) = −0.36 eV, i = 5, 6 | |
| Intra-chain Ta-Ni | (, 0) = (, 0) = (0) = (0) |
| = (0) = (0) = (, 0), (, 0) = −0.035 eV | |
| Inter-chain Ta-Ni | (, 0) = (, 0), (, ) = |
| (, ) = −0.04 eV, | |
| (, 0) = (, 0) = | |
| (, ) = (, ) = (, ) | |
| Inter-chain Ta-Ta | (0) = (, 0) = (, ) = |
| (0, ) = 0.02 eV | |
| Inter-chain Ni-Ni | (0) = (, 0) = (, ) = |
| (0, ) = −0.03 eV |
| Ordering | Comment | |||
|---|---|---|---|---|
| (1-1-11) | 1.765289 | 0.138 | 0.308 | |
| (11-1-1) | 1.766109 | 0.133 | 0.328 | + |
| (1-111) | 1.765679 | 0.139 | 0.340 | + |
| Config * | GS | E | Comment | ||||
|---|---|---|---|---|---|---|---|
| I | (1-1-11) | 3.9 | - | ||||
| II | (11-1-1) | 7.2 | Ta-Ta interchain | ||||
| III | (1-1-11) | 3.9 | Ta-Ni intrachain | ||||
| IV | (1-1-11) | 3.9 | Ta-Ni interchain | ||||
| V | (11-1-1) | 7.4 | Ni-Ni interchain | ||||
| VI | (1-1-11) | 4.3 | Ta-Ta, Ni-Ni interchain |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chatterjee, B.; Golež, D.; Mravlje, J. Delicate Competition Between Different Excitonic Orderings in Ta2NiSe5. Crystals 2025, 15, 414. https://doi.org/10.3390/cryst15050414
Chatterjee B, Golež D, Mravlje J. Delicate Competition Between Different Excitonic Orderings in Ta2NiSe5. Crystals. 2025; 15(5):414. https://doi.org/10.3390/cryst15050414
Chicago/Turabian StyleChatterjee, Banhi, Denis Golež, and Jernej Mravlje. 2025. "Delicate Competition Between Different Excitonic Orderings in Ta2NiSe5" Crystals 15, no. 5: 414. https://doi.org/10.3390/cryst15050414
APA StyleChatterjee, B., Golež, D., & Mravlje, J. (2025). Delicate Competition Between Different Excitonic Orderings in Ta2NiSe5. Crystals, 15(5), 414. https://doi.org/10.3390/cryst15050414






