Investigation of the Electrical Properties of Polycrystalline Crednerite CuMn1−xMgxO2 (x = 0–0.06)-Type Materials in a Low-Frequency Field
Abstract
1. Introduction
2. Materials and Methods
2.1. Synthesis Using the Hydrothermal Method
2.2. Characterization Techniques
3. Results and Discussion
3.1. X-Ray Diffraction Analysis
3.2. Raman Spectroscopy
3.3. Electrical Properties
3.3.1. Complex Impedance
3.3.2. DC and AC Conductivity
Temperature Dependence of DC Conductivity
3.3.3. Electrical Modulus
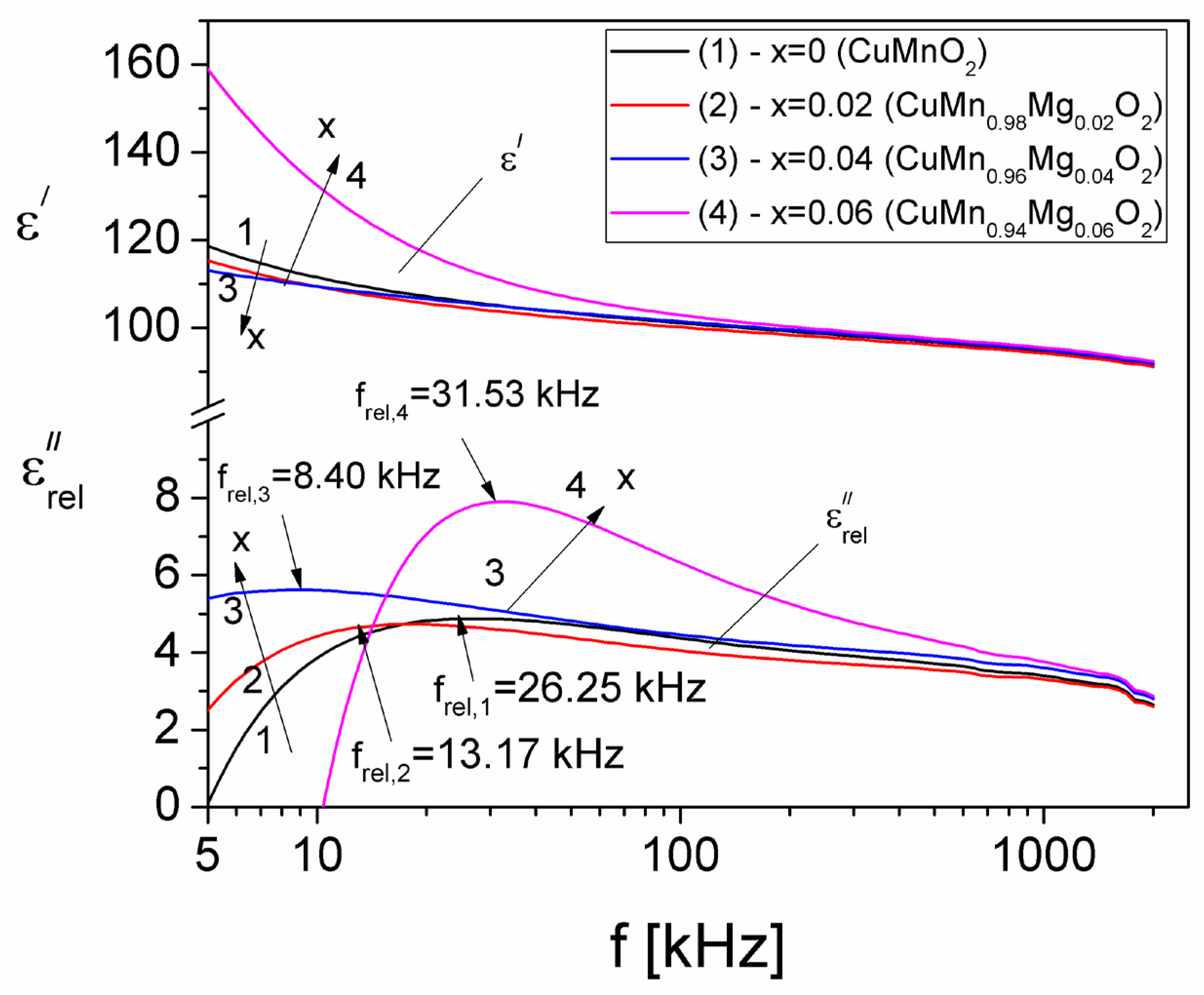
3.3.4. Complex Dielectric Permittivity
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Benreguia, N.; Abdi, A.; Mahroua, O.; Trari, M. Photoelectrochemical properties of the crednerite CuMnO2 and its application to hydrogen production and Mn+ reduction (Mn+ = Cd2+, Pd2+, Zn2+, Ni2+ and Ag+). J. Mater. Sci. Mater. Electron. 2021, 32, 10498–10509. [Google Scholar] [CrossRef]
- Williamson, B.A.D.; Buckeridge, J.; Brown, J.; Ansbro, S.; Palgrave, R.G.; Scanlon, D.O. Engineering valence band dispersion for high mobility p-type semiconductors. Chem. Mater. 2017, 29, 2402–2413. [Google Scholar] [CrossRef]
- Xiong, D.; Zeng, X.; Zhang, W.; Wang, H.; Zhao, X.; Chen, W.; Cheng, Y. Synthesis and Characterization of CuAlO2 and AgAlO2 Delafossite Oxides through Low-Temperature Hydrothermal Methods. Inorg. Chem. 2014, 53, 4106–4116. [Google Scholar] [CrossRef] [PubMed]
- Xiong, D.; Xu, Z.; Zeng, X.; Zhang, W.; Chen, W.; Xu, X.; Wang, M.; Chenget, Y.-B. Hydrothermal synthesis of ultrasmall CuCrO2 nanocrystal alternatives to NiO nanoparticles in efficient p-type dye-sensitized solar cells. J. Mater. Chem. 2012, 22, 24760–24768. [Google Scholar] [CrossRef]
- Xiong, D.; Qi, Y.; Li, X.; Liu, X.; Tao, H.; Chen, W.; Zhao, X. Hydrothermal synthesis of delafossite CuFeO2 crystals at 100 °C. RSC Adv. 2015, 5, 49280–49286. [Google Scholar] [CrossRef]
- Younsi, M.; Manaa, S. Synthesis and characterization of rich delafossite CuYO2+x application for solar energy conversion. Energy Procedia 2013, 36, 638–641. [Google Scholar] [CrossRef]
- Zhao, Q.-M.; Zhao, Z.-Y.; Liu, Q.-L.; Yao, G.-Y.; Dong, X.-D. Delafossite CuGaO2 as promising visible-light-driven photocatalyst: Synthesize, properties, and performances. J. Phys. D Appl. Phys. 2020, 53, 135102. [Google Scholar] [CrossRef]
- Hossain, S.A.; Sarkar, S.; Bose, S.; Das, P. Synthesis, characterization, and application of delafossites as catalysts for degrading organic pollutants and degradation mechanisms: A detailed insight. Surf. Interf. 2023, 42, 103281. [Google Scholar] [CrossRef]
- Zhang, Q.; Xiong, D.; Li, H.; Xia, D.; Tao, H.; Zhao, X. A facile hydrothermal route to synthesize delafossite CuMnO2 nanocrystals. J. Mater. Sci. Mater. Electron. 2015, 26, 10159–10163. [Google Scholar] [CrossRef]
- Poienar, M.; Lungu, A.; Sfirloaga, P.; Lungu, M.; Mihali, C.V.; Vlazan, P. Use of ultrasound-assisted co-precipitation route to obtain CuMnO2 semiconductor nanomaterials. Chem. Pap. 2019, 73, 1541–1546. [Google Scholar] [CrossRef]
- Kondrashev, I.D. The crystal structure and composition of crednerite, CuMnO2. Sov. Phys. Crystallogr. 1959, 3, 703–706. [Google Scholar]
- Poienar, M.; Martin, C.; Lebedev, O.I.; Maignan, A. Advantage of low-temperature hydrothermal synthesis to grow stoichiometric crednerite crystals. Solid State Sci. 2018, 80, 39–45. [Google Scholar] [CrossRef]
- Amrute, A.P.; Łodziana, Z.; Mondelli, C.; Krumeich, F.; Perez-Ramirez, J. Solid-state chemistry of cuprous delafossites: Synthesis and stability aspects. Chem. Mater. 2013, 25, 4423–4435. [Google Scholar] [CrossRef]
- Sheets, W.C.; Mugnier, E.; Barnabe, A.; Marks, T.J.; Poeppelmeier, K.R. Hydrothermal synthesis of delafossite-type oxides. Chem. Mater. 2006, 18, 7–20. [Google Scholar] [CrossRef]
- Poienar, M.; Banica, R.; Sfirloaga, P.; Ianasi, C.; Mihali, C.V.; Vlazan, P. Microwave-assisted hydrothermal synthesis and catalytic activity study of crednerite-type CuMnO2 materials. Ceram. Intern. 2018, 44, 6157–6161. [Google Scholar] [CrossRef]
- Ma, C.; Pan, J.; Chen, C.; Dong, Y.; Yao, F.; Wang, F.; Song, M. Investigation into the roles of interfacial H2O structure in catalytic oxidation of HCHO and CO over CuMnO2 catalysts. J. Environ. Sci. 2024, 137, 310–320. [Google Scholar] [CrossRef]
- Yin, C.; Zhou, S.; Zhang, K.; Bai, J.; Lv, Y.; Zhang, X.; Li, X.; Xu, A. Crednerite CuMnO2 as highly efficient Fenton-like catalysts for p-nitrophenol removal: Synergism between Cu(I) and Mn (III). J. Clean. Prod. 2021, 319, 128640. [Google Scholar] [CrossRef]
- Cheng, C. A novel hydrothermal route of preparing CuMnO2 nanoflakes and their application in Li-ion batteries and supercapacitors. APL Mater. 2023, 11, 071105. [Google Scholar] [CrossRef]
- Poienar, M.; Sfirloaga, P.; Martin, C.; Ursu, D.; Vlazan, P. Hydrothermal synthesis of crednerite CuMn1−xMxO2 (M = Mg, Al; x = 0−0.08): Structural characterization and magnetic properties. J. Mater. Sci. 2018, 53, 2389–2395. [Google Scholar] [CrossRef]
- Shukla, K.K.; Shahi, P.; Gopal, S.; Kumar, A.; Ghosh, A.K.; Singh, R.; Sharma, N.; Das, A.; Sinha, A.K.; Joshi, A.G.; et al. Magnetic and optical properties of Fe doped crednerite CuMnO2. RSC Adv. 2015, 5, 83504–83511. [Google Scholar] [CrossRef]
- Ushakov, A.V.; Streltsov, S.V.; Khomskii, D.I. Orbital structure and magnetic ordering in stoichiometric and doped crednerite CuMnO2. Phys. Rev. B 2014, 89, 024406. [Google Scholar] [CrossRef]
- Poienar, M.; Vecchini, C.; Andre, G.; Daoud-Aladine, A.; Margiolaki, I.; Maignan, A.; Lappas, A.; Chapon, L.; Hervieu, M.; Damay, F.; et al. Substitution Effect on the Interplane Coupling in Crednerite: The Cu1.04Mn0.96O2 Case. Chem. Mater. 2011, 23, 85–94. [Google Scholar] [CrossRef]
- Okuda, T.; Jufuku, N.; Hidaka, S.; Terada, N. Magnetic, transport, and thermoelectric properties of the delafossite oxides CuCr1−xMgxO2 (0 ⩽ x ⩽ 0.04). Phys. Rev. B 2005, 72, 144403. [Google Scholar] [CrossRef]
- Rattanathrum, P.; Taddee, C.; Chanlek, N.; Thongbai, P.; Kamwanna, T. Structural and physical properties of Ge-doped CuCrO2 delafossite oxide. Cer. Intern. 2017, 43, S417–S422. [Google Scholar] [CrossRef]
- Jlaiel, F.; Elkhouni, T.; Amami, M.; Strobel, P.; Ben Salah, A. Structural and physical properties of the (Ca, Mg)-doped delafossite powder CuGaO2. Mat. Res. Bull. 2013, 48, 1020–1026. [Google Scholar] [CrossRef]
- Malaescu, I.; Lungu, A.; Marin, C.N.; Sfirloaga, P.; Vlazan, P.; Brindusoiu, S.; Poienar, M. Temperature dependence of the dynamic electrical properties of Cu1+xMn1−xO2 (x=0 and 0.06) crednerite materials. Cer. Intern. 2018, 44, 11610–11616. [Google Scholar] [CrossRef]
- Mott, N.F.; Davis, E.A. Electronic Processes in Non-Crystalline Materials; Clarendon: Oxford, UK, 1979. [Google Scholar]
- Elliot, S.R. AC conduction in amorphous chalcogenide and pnictide semiconductors. Adv. Phys. 1987, 36, 135–217. [Google Scholar] [CrossRef]
- Carvajal, J.R. Recent advances in magnetic structure determination by neutron powder diffraction. Phys. B Cond. Matter. 1993, 192, 55–69. [Google Scholar] [CrossRef]
- Malaescu, I.; Ercuta, A.; Sfirloaga, P.; Poienar, M.; Vlazan, P.; Lungu, A.; Marin, C.N.; Lungu, M. Methods of morpho-structural characterization and determination of the electrical and magnetic properties of metal oxides. In The Fundamentals and Challenges of Oxide Materials; Lungu, M., Marin, C.N., Eds.; Cambridge Scholars Publishing: London, UK, 2023; Chapter 2; pp. 49–50. [Google Scholar]
- ASTM D150-98; Standard Test Methods for AC Loss Characteristics and Permittivity (Dielectric Constant) of Solid Electrical Insulation. ASTM International: West Conshohocken, PA, USA, 2017.
- Aktas, O.; Truong, K.D.; Otani, T.; Balakrishnan, G.; Clouter, M.J.; Kimura, T.; Quirion, G. Raman scattering study of delafossite magnetoelectric multiferroic compounds: CuFeO2 and CuCrO2. J. Phys. Cond. Matter 2012, 24, 036003. [Google Scholar] [CrossRef]
- Pellicer-Porres, J.; Segura, A.; Martínez, E.; Saitta, A.M.; Polian, A.; Chervin, J.C.; Canny, B. Vibrational properties of delafossite CuGaO2 at ambient and high pressures. Phys. Rev. B 2005, 72, 064301. [Google Scholar] [CrossRef]
- Pellicer-Porres, J.; Martínez-García, D.; Segura, A.; Rodríguez-Hernández, P.; Muñoz, A.; Chervin, J.C.; Garro, N.; Kim, D. Pressure and temperature dependence of the lattice dynamics of CuAlO2 investigated by Raman scattering experiments and ab initio calculations. Phys. Rev. B 2006, 74, 184301. [Google Scholar] [CrossRef]
- Benreguia, N.; Barnabé, A.; Trari, M. Preparation and characterization of the semiconductor CuMnO2 by sol-gel route. Mater. Sci. Semicond. Process. 2016, 56, 14–19. [Google Scholar] [CrossRef]
- Deng, Y.; Handoko, A.D.; Du, Y.; Xi, S.; Yeo, B.S. In Situ Raman Spectroscopy of Copper and Copper Oxide Surfaces during Electrochemical Oxygen Evolution Reaction: Identification of CuIII Oxides as Catalytically Active Species. ACS Catalysis. 2016, 6, 2473–2481. [Google Scholar] [CrossRef]
- Lalanne, M.; Demont, P.; Barnabé, A. Ac conductivity and dielectric properties of CuFe1−xCrxO2:Mg delafossite. J. Phys. D Appl. Phys. 2011, 44, 185401. [Google Scholar] [CrossRef]
- Debye, P. Polar Molecules; Chemical Catalog Company, Inc.: New York, NY, USA, 1929. [Google Scholar]
- Jonscher, A.K. Universal Relaxation Law, 1st ed; Chelsea Dielectrics Press: London, UK, 1996; pp. 198–200. [Google Scholar]
- Bessekhouad, Y.; Gabes, Y.; Bouguelia, A.; Trari, M. The physical and photo electrochemical characterization of the crednerite CuMnO2. J. Mater. Sci. 2007, 42, 6469–6476. [Google Scholar] [CrossRef]
- Chen, H.; Hsu, D. Characterization of crednerite-Cu1.1Mn0.9O2 films prepared using sol–gel processing. Appl. Surf. Sci. 2014, 290, 161–166. [Google Scholar] [CrossRef]
- Funke, K. Jump relaxation in solid electrolytes. Prog. Solid State Chem. 1993, 22, 111–195. [Google Scholar] [CrossRef]
- Malaescu, I.; Sfirloaga, P.; Marin, C.N.; Bunoiu, M.O.; Vlazan, P. Experimental Investigations on the Electrical Conductivity and Complex Dielectric Permittivity of ZnxMn1−xFe2O4 (x = 0 and 0.4) Ferrites in a Low-Frequency Field. Crystals 2024, 14, 437. [Google Scholar] [CrossRef]
- Papathanassiou, A.N. Specific feature of mixed alkali effect in the a.c. conductivity of ion-conducting glasses. Mater. Lett. 2005, 59, 1634–1635. [Google Scholar] [CrossRef]
- Papathanassiou, A.N. Novel feature of the universal power law dispersion of the ac conductivity in disordered matter. J. Non-Cryst. Solids 2006, 352, 5444–5445. [Google Scholar] [CrossRef]
- Cramer, C.; Brunklaus, S.; Ratai, E.; Gao, Y. New Mixed Alkali Effect in the ac Conductivity of Ion-Conducting Glasses. Phys. Rev. Lett. 2003, 91, 26601. [Google Scholar] [CrossRef] [PubMed]
- Lalanne, M.; Barnabé, A.; Mathieu, F.; Tailhades, P. Synthesis and thermostructural studies of a CuFe(1−x)Cr(x)O(2) delafossite solid solution with 0 < x <1. Inorg. Chem. 2009, 48, 6065–6071. [Google Scholar] [PubMed]
- Brindusoiu, S.; Poienar, M.; Marin, C.N.; Sfirloaga, P.; Vlazan, P.; Malaescu, I. The electrical conductivity of Fe3(PO4)2·8H2O materials. J. Mater. Sci. Mater. Electron. 2019, 30, 15693–15699. [Google Scholar] [CrossRef]
- Viswanathan, M.; Anil Kumar, P.S.; Bhadram, V.S.; Narayana, C.; Bera, A.K.; Yusuf, S.M. Influence of lattice distortion on the Curie temperature and spin–phonon coupling in LaMn0.5Co0.5O3. J. Phys. Condens. Matter 2010, 22, 346006. [Google Scholar] [CrossRef] [PubMed]
- Horn, T.; Deutschlander, S.; Lowen, H.; Maret, G.; Keim, P. Fluctuations of orientational order and clustering in a two-dimensional colloidal system under quenched disorder. Phys. Rev. E 2013, 88, 062305. [Google Scholar] [CrossRef] [PubMed]
- Harun, M.H.; Saion, E.; Kassim, A.; Mahmud, E.; Hussain, M.Y.; Mustafa, I.S. Dielectric Properties of Poly (vinyl alcohol)/Polypyrrole Composite Polymer Films. J. Adv. Sci. Arts. 2009, 1, 9–16. [Google Scholar]
- Wang, Y.; Liu, H.; Yan, T.; Zhao, J.; Li, J.; Guo, S.; Sun, S.; Sun, R.; Lu, Z.; Wang, D. Significantly reduced conductivity in strontium titanate-based lead-free ceramics by excess bismuth. Mater. Lett. 2022, 309, 131453. [Google Scholar] [CrossRef]
- Barsoukov, E.; Macdonald, J.R. Impedance Spectroscopy: Theory, Experiments and Applications; Wiley: New York, NY, USA, 2005. [Google Scholar]
- Iwasaki, M.; Hara, M.; Kawada, H.; Tada, H.; Ito, S. Cobalt Ion-Doped TiO2 Photocatalyst Response to Visible Light. J. Coll. Interf. Sci. 2000, 224, 202–204. [Google Scholar] [CrossRef] [PubMed]
- Scaife, B.K. Principles of Dielectrics; Clarendon Press: Oxford, UK, 1998. [Google Scholar]
- Kumar, G.; Rani, R.; Sharma, S.; Batoo, K.M.; Singh, M. Electric and dielectric study of cobalt substituted Mg–Mn nanoferrites synthesized by solution combustion technique. Ceram. Intern. 2013, 39, 4813–4818. [Google Scholar] [CrossRef]
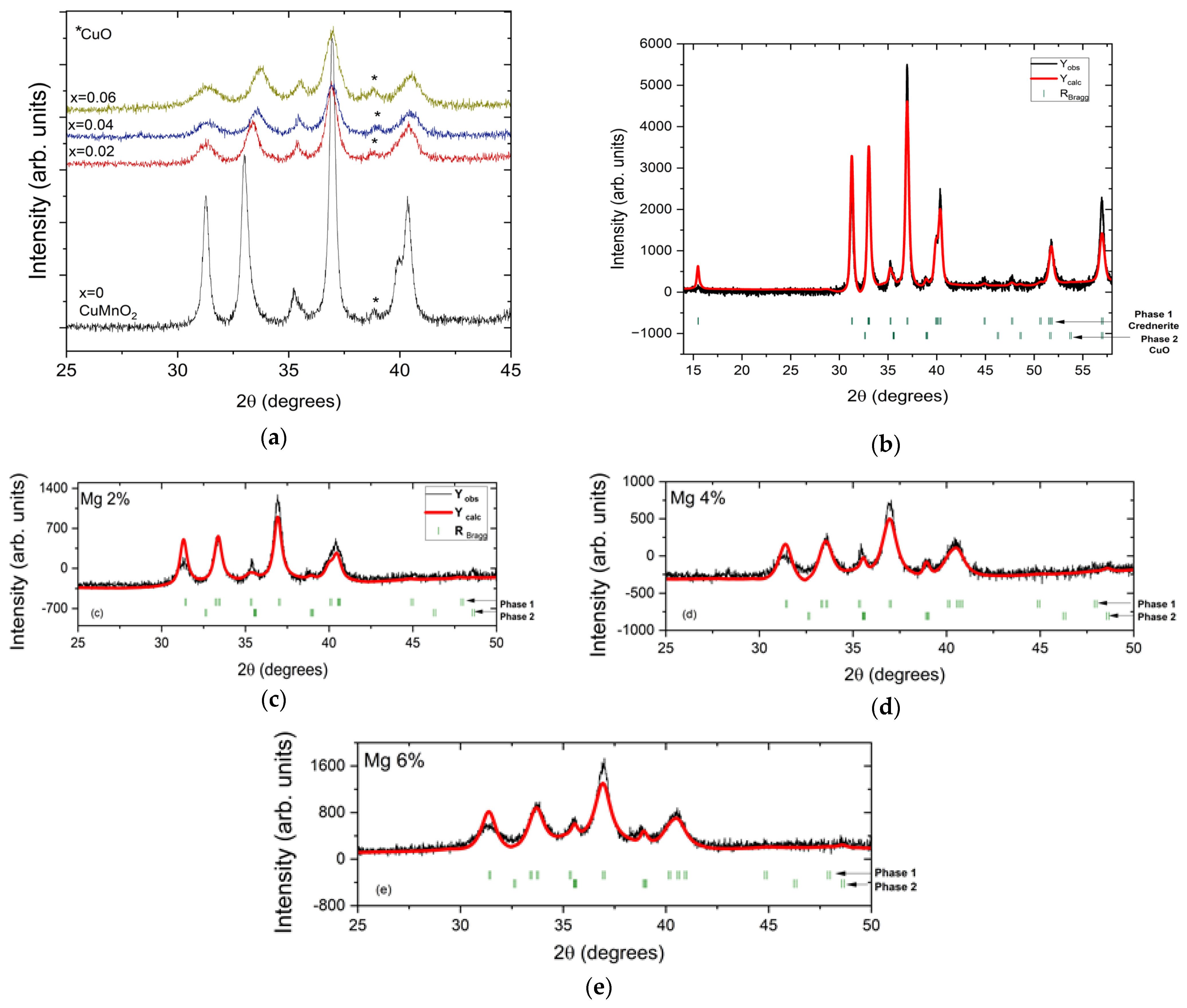
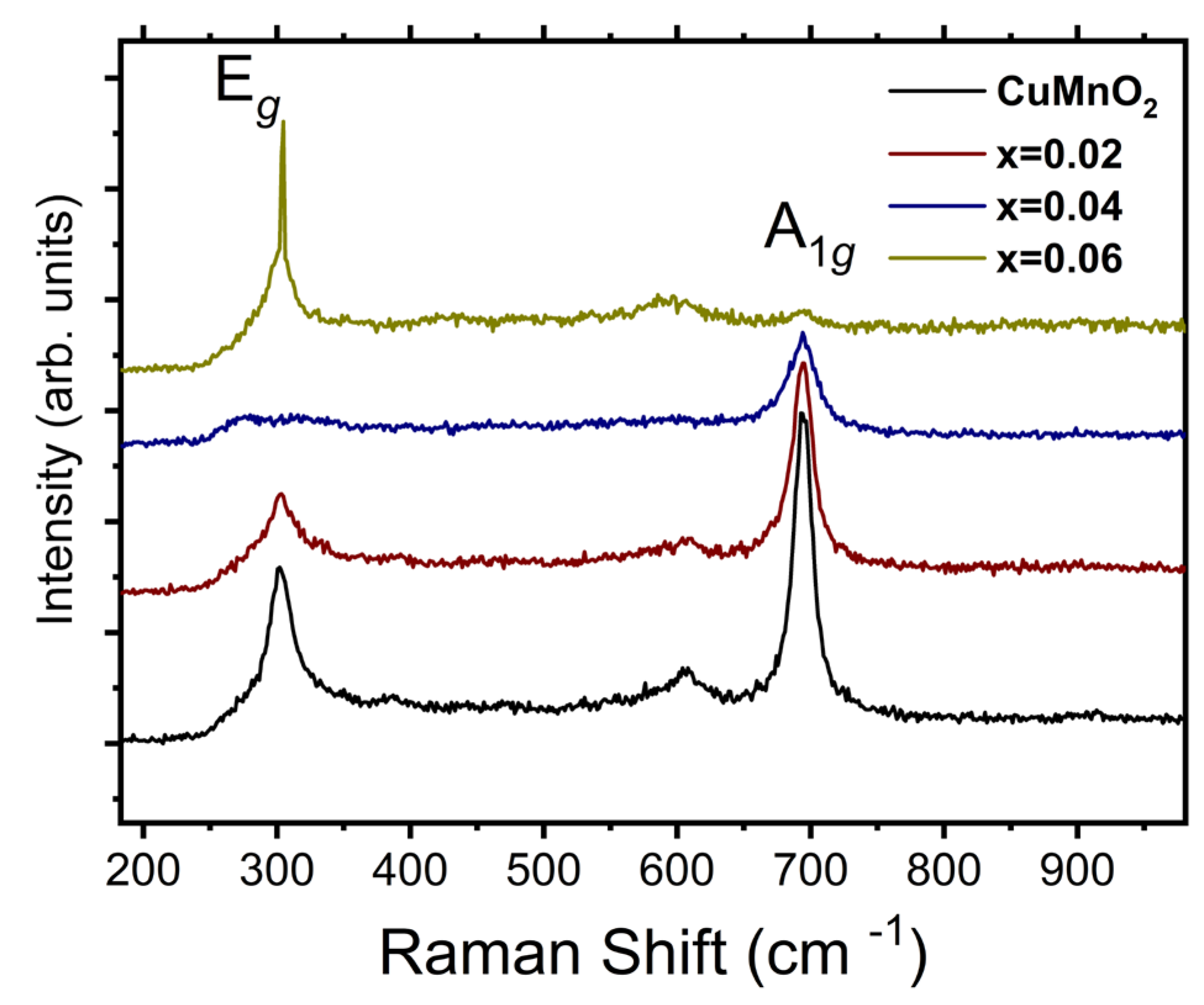


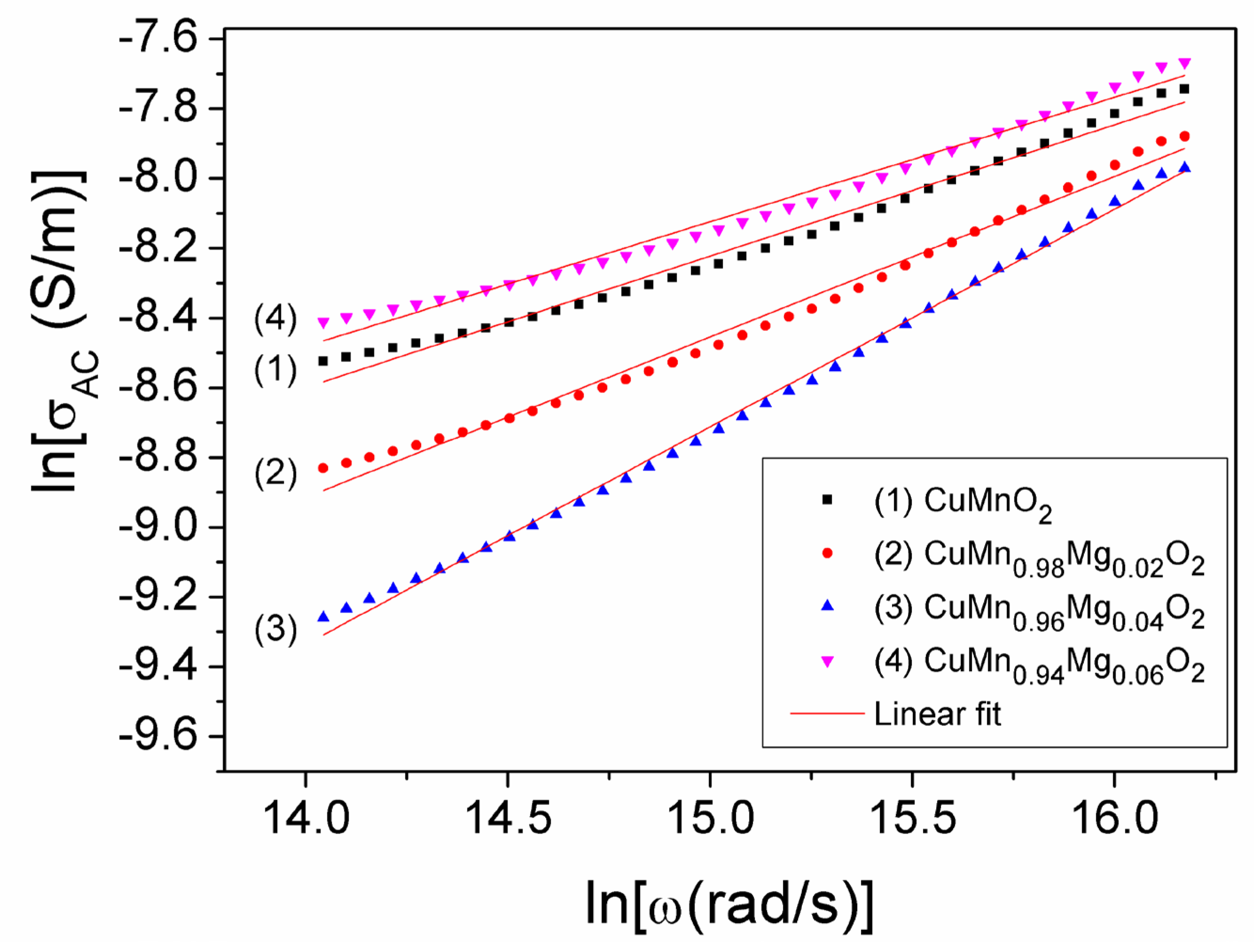
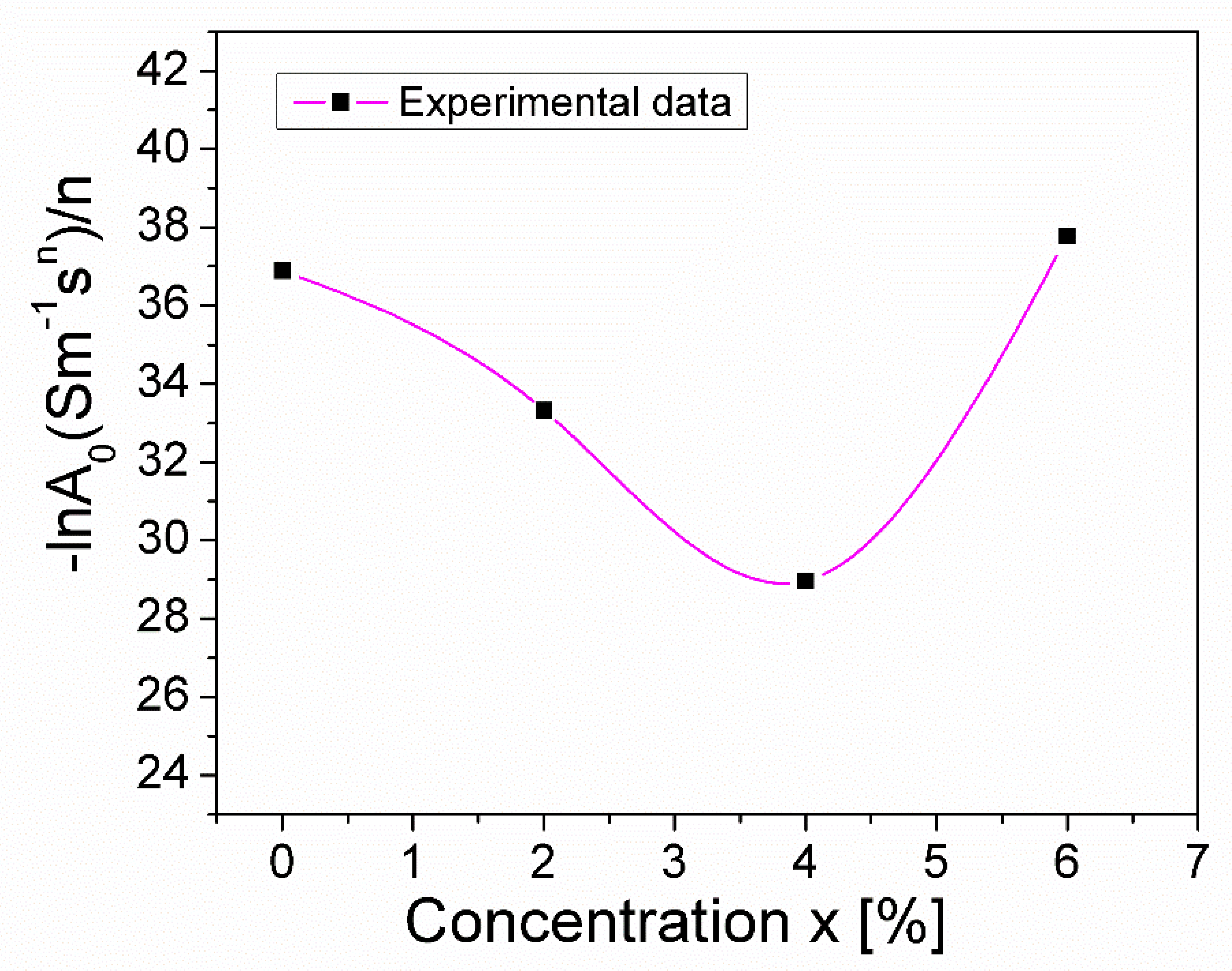
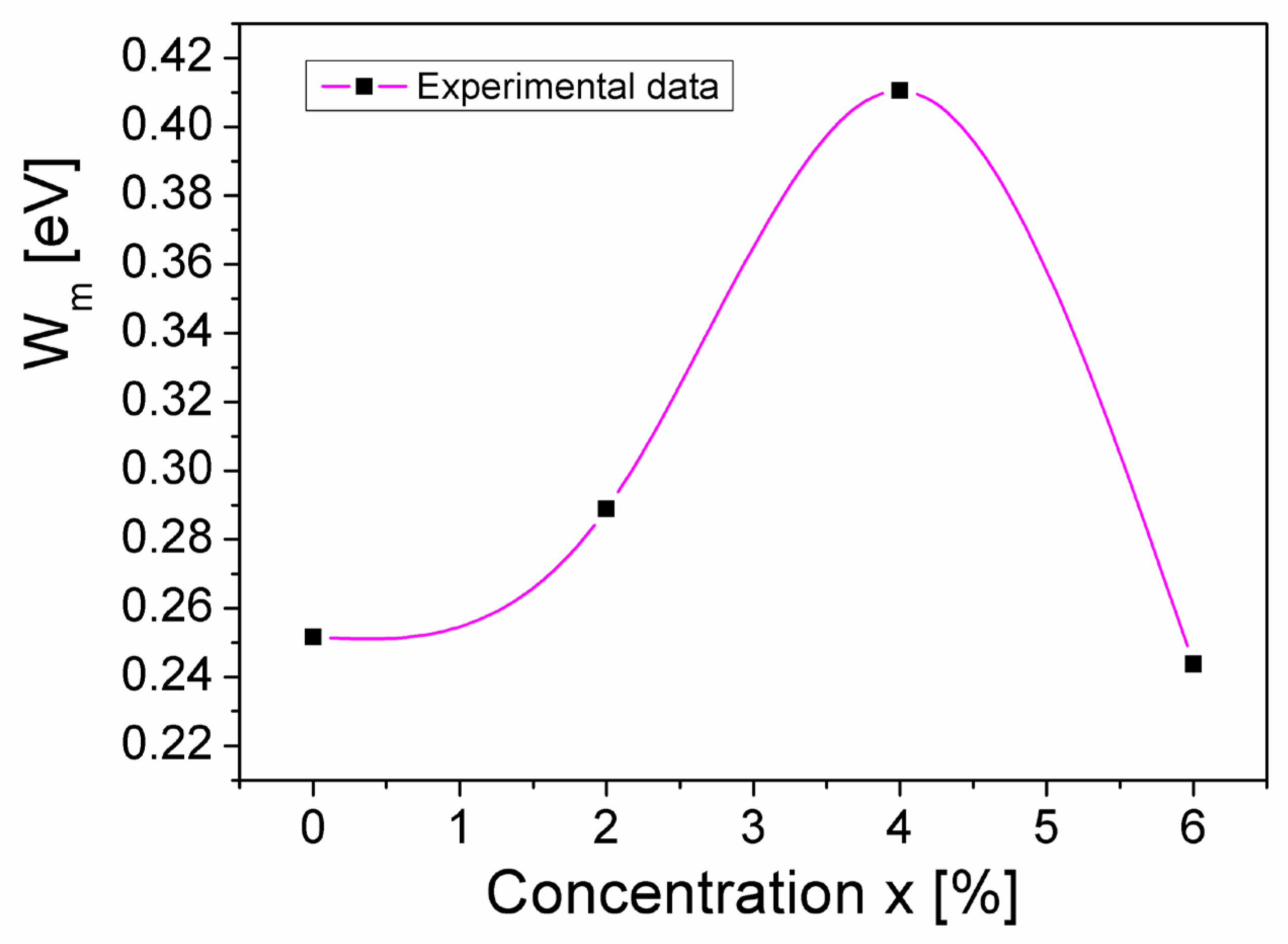
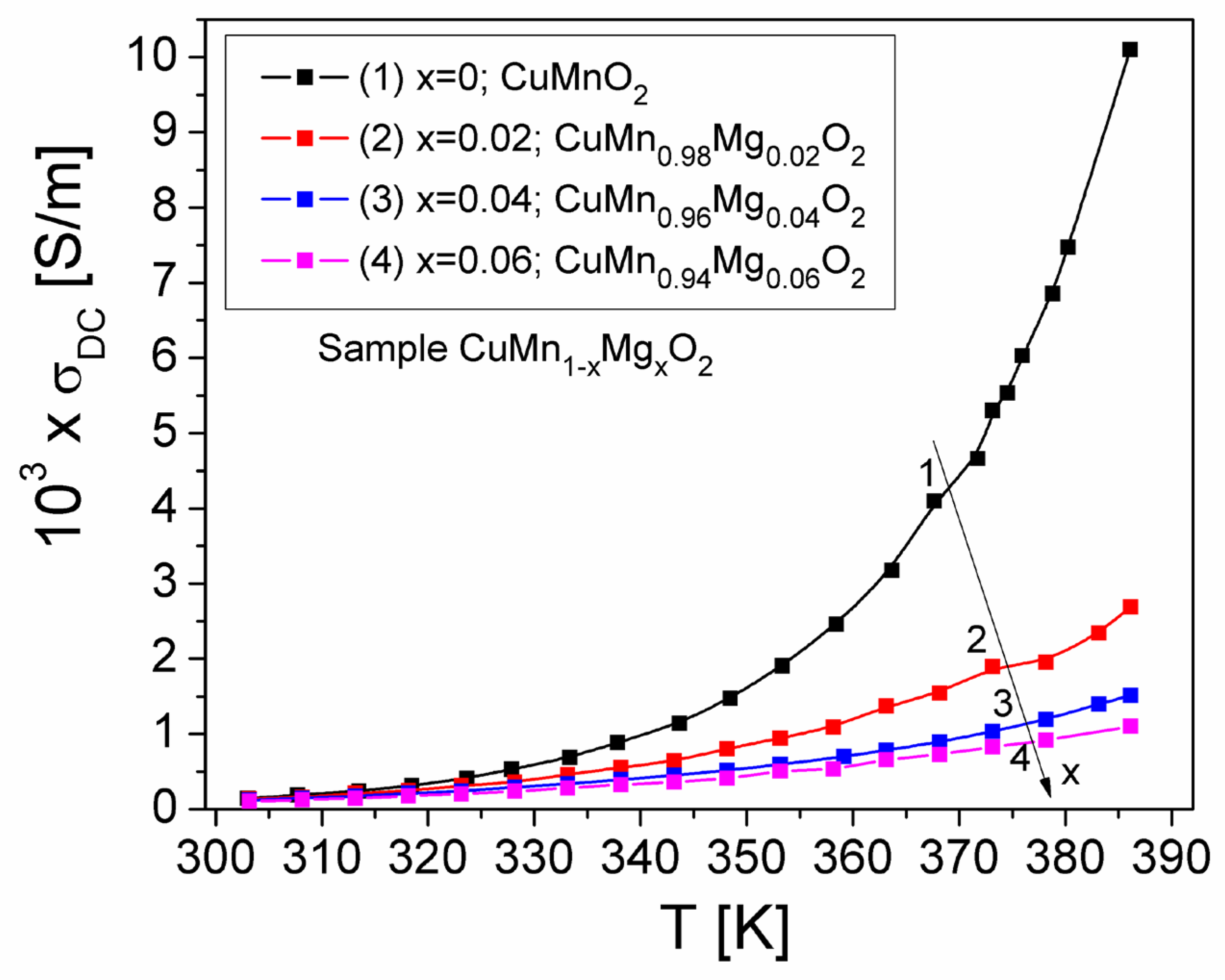

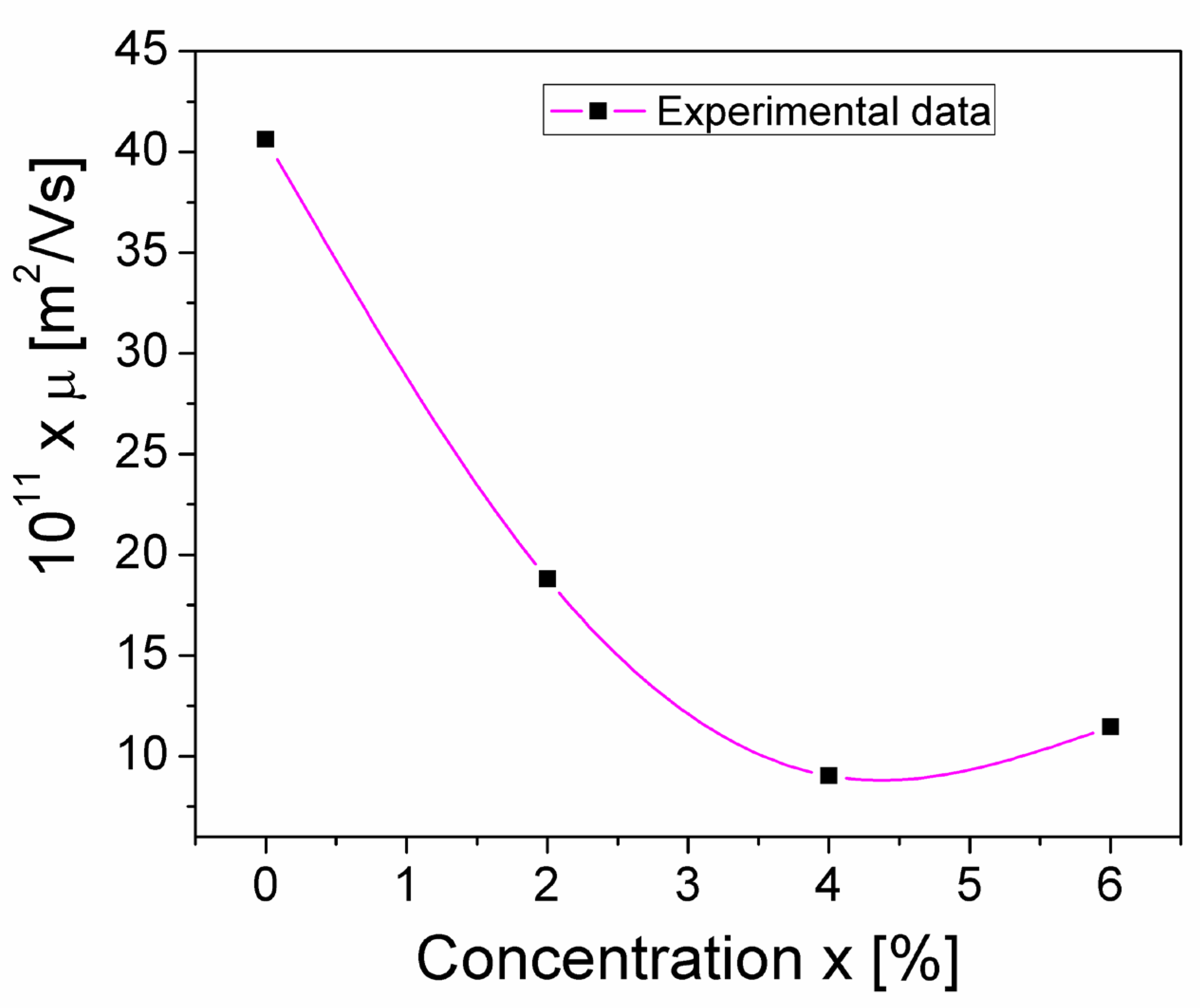

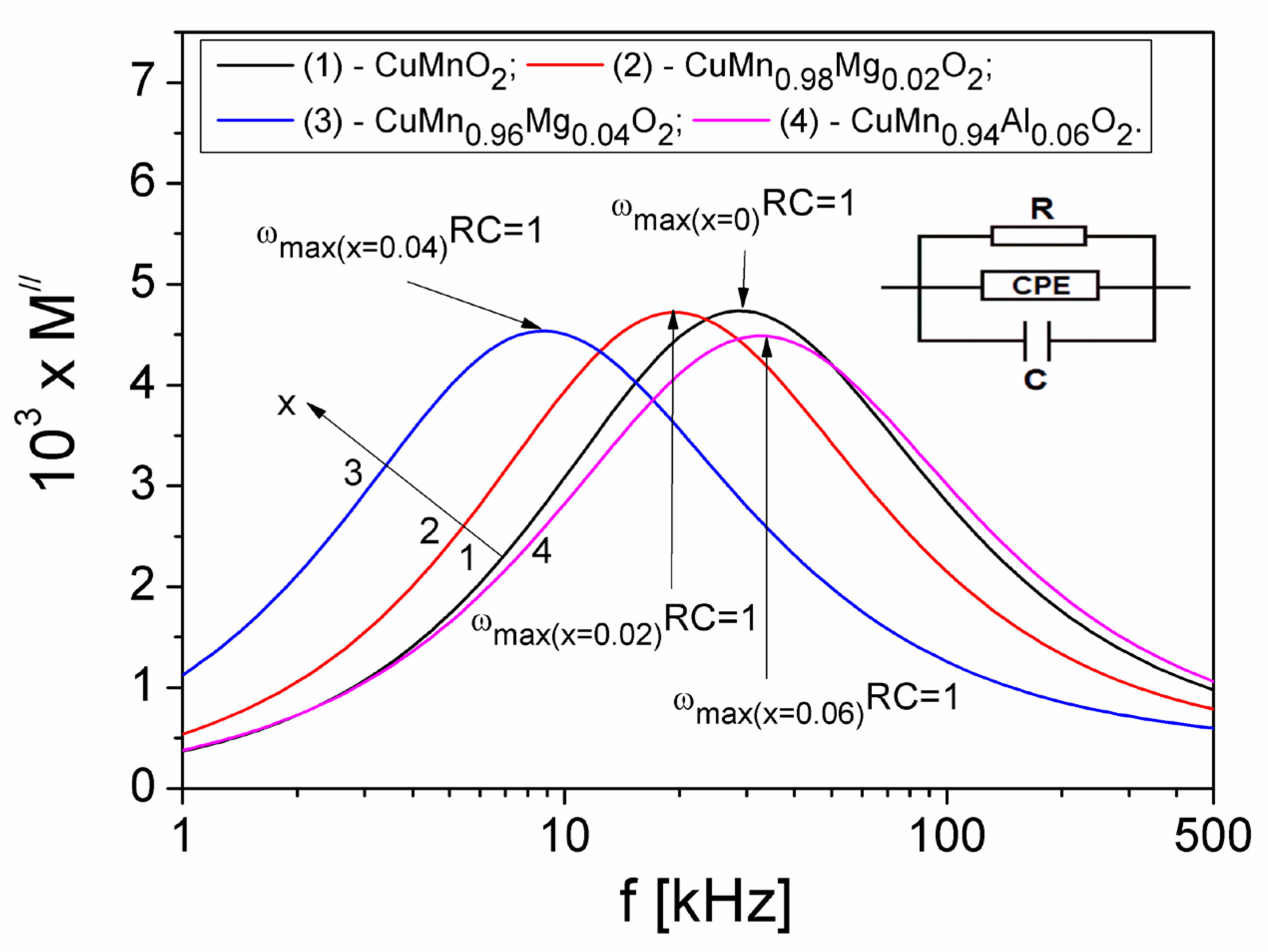

| Samples | CuMnO2 | CuMn0.98Mg0.02O2 | CuMn0.96Mg0.04O2 | CuMn0.94Mg0.06O2 |
|---|---|---|---|---|
| a (Å) | 5.5875(5) | 5.5379(1) | 5.5133(1) | 5.4921(2) |
| b (Å) | 2.8848(2) | 2.8899(6) | 2.8889(5) | 2.8928(5) |
| c (Å) | 5.8915(5) | 5.8949(2) | 5.8805(1) | 5.8859(2) |
| β (deg) | 103.969 (1) | 104.335(2) | 104.519(2) | 104.622(2) |
| Volume (Å3) | 92.157(3) | 91.406(4) | 90.671(3) | 90.485(6) |
| Crystallite size (Å) | 212 | 129 | 65 | 66 |
| CuO phase content | 1.92% | 3.66% | 4.55% | 4.09% |
| χ2 | 6.89 | 2.71 | 2.11 | 1.97 |
| RBragg | 9.62 | 6.75 | 6.87 | 5.91 |
| RF | 12.00 | 7.79 | 6.94 | 5.50 |
| Samples | CuMnO2 | CuMn0.98Mg0.02O2 | CuMn0.96Mg0.04O2 | CuMn0.94Mg0.06O2 |
|---|---|---|---|---|
| τZ [μs] | 5.88 | 8.73 | 20.15 | 6.02 |
| τM [μs] | 5.48 | 8.08 | 17.75 | 4.88 |
| τrel [μs] | 6.06 | 10.53 | 18.95 | 5.03 |
| σDC [S/m] | 15.360 × 10−5 | 10.385 × 10−5 | 4.854 × 10−5 | 16.420 × 10−5 |
| n | 0.376 | 0.461 | 0.624 | 0.357 |
| A0[Sm−1sn] | 9.48 × 10−7 | 2.13 × 10−7 | 0.141 × 10−7 | 13.97 × 10−7 |
| ωh[rad/s] | 7.434 × 105 | 6.938 × 105 | 4.867 × 105 | 6.855 × 105 |
| NC[m−3] | 2.350 × 1024 | 3.436 × 1024 | 3.344 × 1024 | 8.907 × 1024 |
| R [MΩ] | 4.151 | 6.109 | 12.892 | 3.502 |
| C [pF] | 1.320 | 1.323 | 1.377 | 1.393 |
| Q [nF∙sn−1] | 2.533 | 0.735 | 0.084 | 3.626 |
| x [%] | T [K] | N (EF) [cm−3 eV−1] | EA,cond [eV] | Rh [nm] | Wh [eV] |
|---|---|---|---|---|---|
| x = 0 | 300 | 2.313 × 1016 | 0.469 | 23.14 | 0.833 |
| 323 | 2.313 × 1016 | 0.496 | 22.71 | 0.880 | |
| 353 | 2.313 × 1016 | 0.530 | 22.21 | 0.941 | |
| x = 2% | 300 | 9.416 × 1016 | 0.330 | 16.29 | 0.586 |
| 323 | 9.416 × 1016 | 0.349 | 15.99 | 0.620 | |
| 353 | 9.416 × 1016 | 0.373 | 15.64 | 0.662 | |
| x = 4% | 300 | 2.008 × 1017 | 0.273 | 13.48 | 0.485 |
| 323 | 2.008 × 1017 | 0.289 | 13.23 | 0.513 | |
| 353 | 2.008 × 1017 | 0.309 | 12.94 | 0.548 | |
| x = 6% | 300 | 2.470 × 1017 | 0.259 | 12.80 | 0.460 |
| 323 | 2.470 × 1017 | 0.274 | 12.56 | 0.487 | |
| 353 | 2.470 × 1017 | 0.293 | 12.29 | 0.521 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Malaescu, I.; Poienar, M.; Marin, C.N. Investigation of the Electrical Properties of Polycrystalline Crednerite CuMn1−xMgxO2 (x = 0–0.06)-Type Materials in a Low-Frequency Field. Crystals 2025, 15, 184. https://doi.org/10.3390/cryst15020184
Malaescu I, Poienar M, Marin CN. Investigation of the Electrical Properties of Polycrystalline Crednerite CuMn1−xMgxO2 (x = 0–0.06)-Type Materials in a Low-Frequency Field. Crystals. 2025; 15(2):184. https://doi.org/10.3390/cryst15020184
Chicago/Turabian StyleMalaescu, Iosif, Maria Poienar, and Catalin N. Marin. 2025. "Investigation of the Electrical Properties of Polycrystalline Crednerite CuMn1−xMgxO2 (x = 0–0.06)-Type Materials in a Low-Frequency Field" Crystals 15, no. 2: 184. https://doi.org/10.3390/cryst15020184
APA StyleMalaescu, I., Poienar, M., & Marin, C. N. (2025). Investigation of the Electrical Properties of Polycrystalline Crednerite CuMn1−xMgxO2 (x = 0–0.06)-Type Materials in a Low-Frequency Field. Crystals, 15(2), 184. https://doi.org/10.3390/cryst15020184







