A Chemical Transport Method for the Synthesis of Simple and Complex Inorganic Crystals—Survey of Applications and Modeling
Abstract
1. Introduction
2. Examples of Simple and Complex Inorganic Compounds Obtained with the Assistance of the Chemical Transport Method with Emphasis on the Experimental Conditions
2.1. Germanium
2.2. Phosphorus
2.2.1. Violet Phosphorus
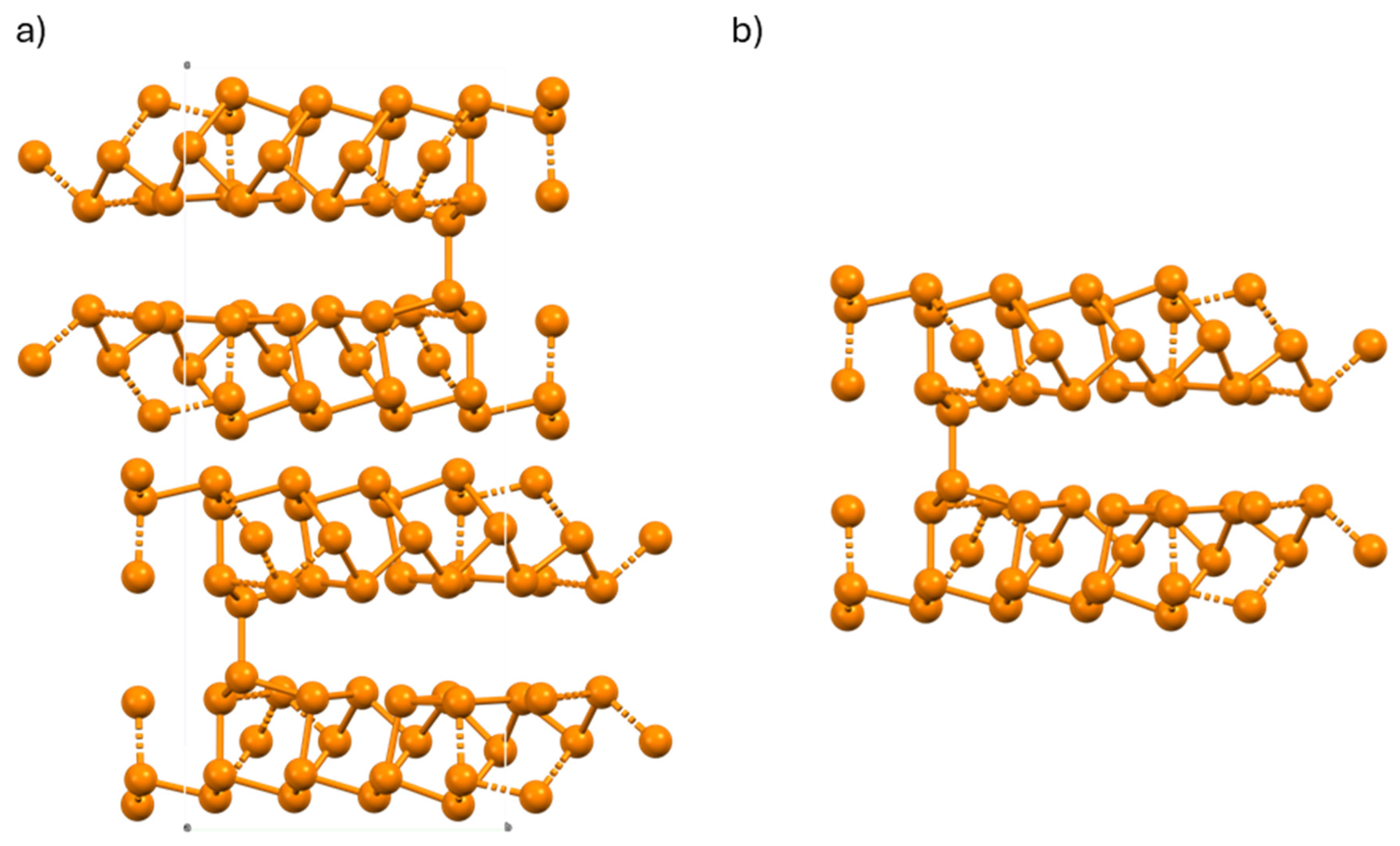
2.2.2. Orthorhombic Black Phosphorus
2.2.3. Doped Black Phosphorus
2.3. PbGa2Se4 Crystals for Photodetection Applications
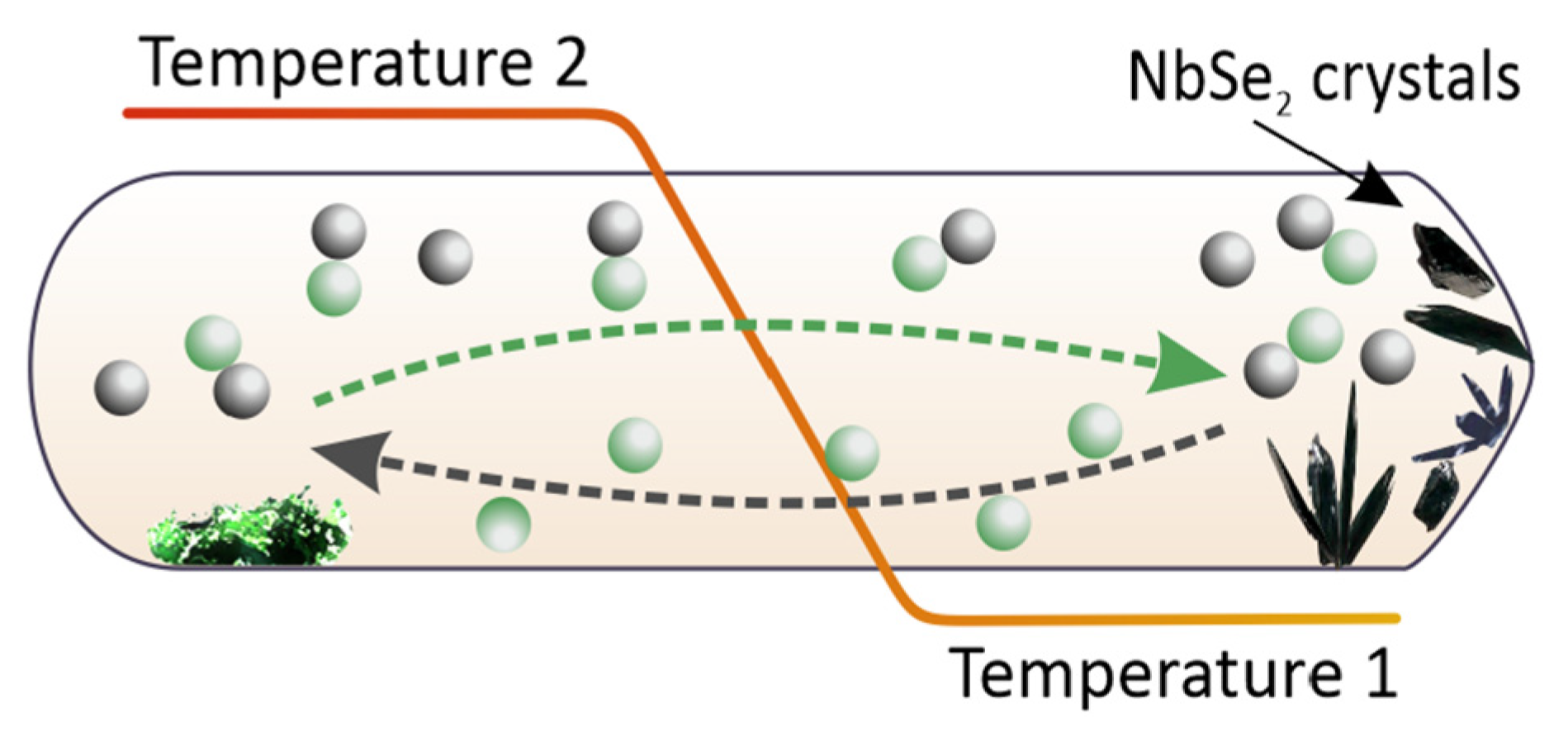
2.4. NbSe2—A Two-Dimensional Compound
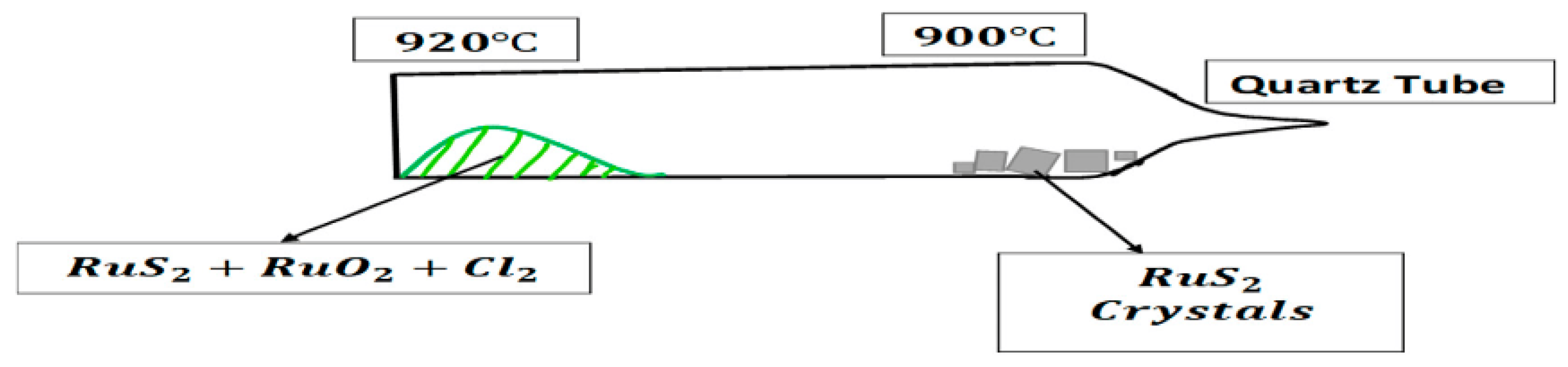
2.5. RuS2—A Two-Dimensional Compound
2.6. ZnSe Crystals Doped with Fe2+ and/or Cr2+ for Mid-IR Applications
2.7. NbCo1.1Te—A Ferromagnetic Non-Stoichiometric Intercalated Compound
2.8. CuInS2—A Semiconducting Material
2.9. Centimeter-Sized UTe2 Single Crystals
2.10. ZrSe3—A Quasi-1D van der Waals Materials
2.11. Cubic BAs
2.12. TiSe2—A Two-Dimensional Compound
2.13. ReSe2—A Two-Dimensional Compound
2.14. Ta2Pd3Se8—A One-Dimensional Compound in the Form of Nanowires
2.15. WS2-MoS2 Heterostructure
2.16. Magnetite (Fe3O4) Coated with Fe3−xSnxO4
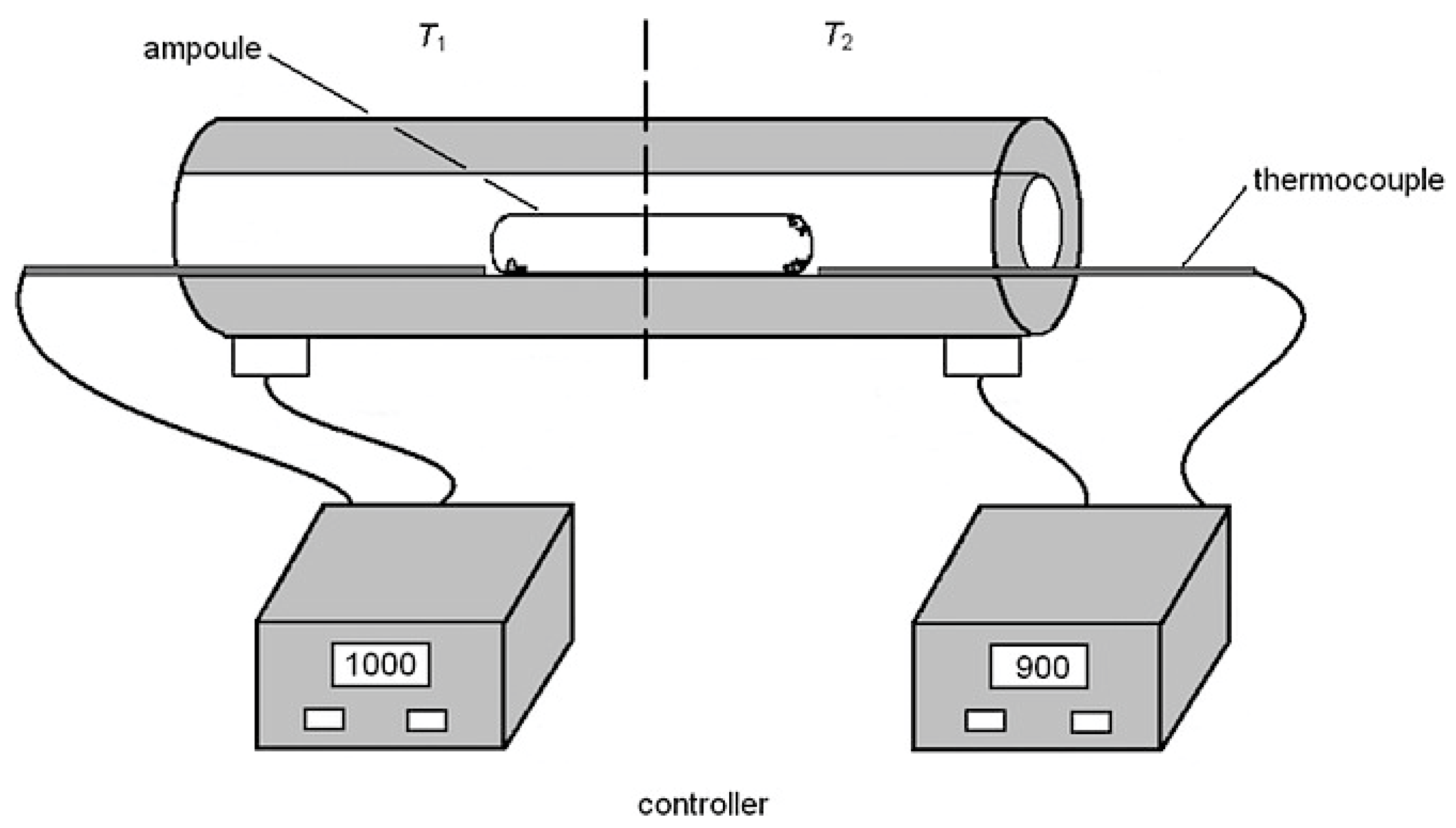
3. Mathematical and Theoretical Description of the Chemical Vapor Transport Process
3.1. Exact Analytical Description of Mass Transport Coupled with Chemical Reactions
3.2. Qualitative, Simplified Description of Chemical Vapor Transport—The Schäfer Equation
- -
- at the source, phase change of reagents from solid to gaseous or liquid phase (sometimes connected with additional chemical reaction);
- -
- liquid or gas movement;
- -
- at the sink, deposition of the solid from the gaseous or liquid phase (sometimes connected with additional chemical reaction).
3.3. Thermodynamic Modeling of the Chemical Vapor Transport
4. Conclusions
Funding
Conflicts of Interest
References
- Binnewies, M.; Glaum, R.; Schmidt, M.; Schmidt, P. Chemical Vapor Transport Reactions; De Gruyter: Berlin, Germany; Boston, MA, USA, 2012. [Google Scholar]
- Binnewies, M.; Schmidt, M.; Schmidt, P. Chemical Vapor Transport Reactions—Arguments for Choosing a Suitable Transport Agent. Z. Anorg. Allg. Chem. 2017, 643, 1295–1311. [Google Scholar] [CrossRef]
- Colibaba, G.V. Halide-Hydrogen Vapor Transport for Growth of ZnO Single Crystals with Controllable Electrical Parameters. Mater. Sci. Semicond. Process. 2016, 43, 75–81. [Google Scholar] [CrossRef]
- Heinemann, R.; Schmidt, P. Crystal Growth by Chemical Vapor Transport: Process Screening by Complementary Modeling and Experiment. Cryst. Growth Des. 2020, 20, 5986–6000. [Google Scholar] [CrossRef]
- Hansen, F.; Wels, M.; Froeschke, S.; Popov, A.; Wolf, D.; Büchner, B.; Schmidt, P.; Hampel, S. Thermodynamic Evaluation and Chemical Vapor Transport of Few-Layer WTe2. Cryst. Growth Des. 2020, 20, 7341–7349. [Google Scholar] [CrossRef]
- Zhang, L.; Gu, M.; Li, L.; Zhao, X.; Fu, C.; Liu, T.; Xu, X.; Cheng, Y.; Zhang, J. High Yield Synthesis of Violet Phosphorus Crystals. Chem. Mater. 2020, 32, 7363. [Google Scholar] [CrossRef]
- Zhang, L.; Huang, H.; Zhang, B.; Gu, M.; Zhao, D.; Zhao, X.; Li, L.; Zhou, J.; Wu, K.; Cheng, Y.; et al. CCDC 1935087: Experimental Crystal Structure Determination. Angew. Chem. Int. Ed. 2020, 59, 1074. [Google Scholar] [CrossRef]
- Macrae, C.F.; Edgington, P.R.; McCabe, P.; Pidcock, E.; Shields, G.P.; Taylor, R.; Towler, M.; van de Streek, J. Mercury: Visualization and analysis of crystal structures. J. Appl. Cryst. 2006, 39, 453–457. [Google Scholar] [CrossRef]
- Zhang, Z.; Xin, X.; Yan, Q.; Li, Q.; Yang, Y.; Ren, T.-L. Two-Step Heating Synthesis of Sub-3 Millimeter-Sized Orthorhombic Black Phosphorus Single Crystal by Chemical Vapor Transport Reaction Method. Sci. China Mater. 2016, 59, 122–134. [Google Scholar] [CrossRef]
- Fu, M.; Yang, W.; Du, K.; Li, J.; Zhang, Y.; Deng, S.; Yang, P. Fabrication of Doped Black Phosphorus Crystal by the Chemical Vapor Transport Method. Cryst. Growth Des. 2022, 22, 7168. [Google Scholar] [CrossRef]
- Zhang, Z.; Khurram, M.; Sun, Z.; Yan, Q. Uniform Tellurium Doping in Black Phosphorus Single Crystals by Chemical Vapor Transport. Inorg. Chem. 2018, 57, 4098–4103. [Google Scholar] [CrossRef]
- Ji, L.; Xiao, B.; Sun, Q.; Xu, Y.; Jie, W. Refined Synthesis and Single Crystal Growth of PbGa2Se4 by Chemical Vapor Transport Method for Photodetection. Cryst. Res. Technol. 2024, 59, 2300276. [Google Scholar] [CrossRef]
- Dimitrov, D.; Rafailov, P.; Marinova, V.; Avramova, I.; Kovacheva, D.; Dionisiev, I.; Minev, N.; Gospodinov, M. NbSe2 Crystals Growth by Bromine Transport. Coatings 2023, 13, 947. [Google Scholar] [CrossRef]
- Sai, R.; Gorochov, O.; Alghamdi, E.A.; Ezzaouia, H. Crystal Growth of RuS2 Using a Chemical Vapor Transport Technique and Its Properties. Crystals 2022, 12, 994. [Google Scholar] [CrossRef]
- Huang, W.; Tang, G.; Wang, C.; He, Y.; Jin, Z.; Du, Y.; Zhang, K.; Chen, B.; He, Z. Effect of Different Doping Concentrations on the Properties of Fe2+: ZnSe and Fe2+, Cr2+: ZnSe Crystals Grown by the Chemical Vapor Transport Method. Inorg. Chem. 2024, 63, 22605. [Google Scholar] [CrossRef]
- Feng, Y.; Shang, Z.; Wu, J.; Tan, H.; Wang, C.; Huang, L.; Li, R.; Cui, S.; Liu, Y.; Lu, Y.; et al. Crystal growth, ferromagnetism and electronic structure of van der Waals NbCo1.1Te2. J. Alloys Compd. 2025, 1010, 177844. [Google Scholar] [CrossRef]
- Giri, R.K.; Chaki, S.H.; Khimani, A.J.; Deshpande, M.P. Mechanistic insights into transport properties of chemical vapour transport grown CuInS2 single crystal. J. Alloys Compd. 2023, 959, 170487. [Google Scholar] [CrossRef]
- Yao, S.; Li, T.; Yue, C.; Xu, X.; Zhang, B.; Zhang, C. Controllable growth of centimetre-sized UTe2 single crystals by the chemical vapor transport method. CrystEngComm 2022, 24, 6262. [Google Scholar] [CrossRef]
- Xu, Y.; Guo, S.; Chen, X. Crystal Growth and Thermal Properties of Quasi-One-Dimensional van der Waals Material ZrSe3. Micromachines 2022, 13, 1994. [Google Scholar] [CrossRef] [PubMed]
- Xing, J.; Glaser, E.R.; Song, B.; Culbertson, J.C.; Freitas, J.A.; Duncan, R.A.; Nelson, K.A.; Chen, G.; Ni, N. Gas-Pressure Chemical Vapor Transport Growth of Millimeter-Sized c-BAs Single Crystals with Moderate Thermal Conductivity. Appl. Phys. Lett. 2018, 112, 241903. [Google Scholar] [CrossRef]
- Wang, J.; Zheng, H.; Xu, G.; Sun, L.; Hu, D.; Lu, Z.; Liu, L.; Zheng, J.; Tao, C.; Jiao, L. Controlled Synthesis of Two-Dimensional 1 T-TiSe2 with Charge Density Wave Transition by Chemical Vapor Transport. J. Am. Chem. Soc. 2016, 138, 16216–16219. [Google Scholar] [CrossRef] [PubMed]
- Xing, L.; Yan, X.; Zheng, J.; Xu, G.; Lu, Z.; Liu, L.; Wang, J.; Wang, P.; Pan, X.; Jiao, L. Highly Crystalline ReSe2 Atomic Layers Synthesized by Chemical Vapor Transport. InfoMat 2019, 1, 552–558. [Google Scholar] [CrossRef]
- Zhang, X.; Kang, J.; Choi, K.H.; Jeon, J.; Jeong, B.J.; Bang, H.-S.; Oh, H.-S.; Lim, J.; Park, J.-H.; Lee, J.-H.; et al. Facile High-Yield Growth of One-Dimensional van Der Waals Ta2Pd3Se8 via Chemical Vapor Transport Using PdCl2 as a Transport Agent. Chem. Mater. 2024, 36, 2533–2543. [Google Scholar] [CrossRef]
- Zhao, Y.; Jin, S. Controllable Water Vapor Assisted Chemical Vapor Transport Synthesis of WS2–MoS2 Heterostructure. ACS Mater. Lett. 2020, 2, 42–48. [Google Scholar] [CrossRef]
- Su, Z.; Zhang, Y.; Liu, B.; Chen, Y.; Li, G.; Jiang, T. Formation Mechanisms of Fe3−xSnxO4 by a Chemical Vapor Transport (CVT) Process. Sci. Rep. 2017, 7, 43463. [Google Scholar] [CrossRef] [PubMed]
- Olander, D.R. Simultaneous Mass Transfer and Equilibrium Chemical Reaction. AIChE J. 1960, 6, 233–239. [Google Scholar] [CrossRef]
- Huang, C.-J.; Kuo, C.-H. Mathematical Models for Mass Transfer Accompanied by Reversible Chemical Reaction. AIChE J. 1965, 11, 901–910. [Google Scholar] [CrossRef]
- Huang, C.-J.; Kuo, C.-H. General Mathematical Model for Mass Transfer Accompanied by Chemical Reaction. AIChE J. 1963, 9, 161–167. [Google Scholar] [CrossRef]
- Maas, U. Coupling of chemical reaction with flow and molecular transport. Appl. Math. 1995, 40, 249–266. [Google Scholar] [CrossRef]
- Greenwell, D.W.; Markham, B.L.; Rosenberger, F. Numerical modeling of diffusive physical vapor transport in cylindrical ampoules. J. Cryst. Growth 1981, 51, 413–425. [Google Scholar] [CrossRef]
- Markham, B.L.; Greenwell, D.W.; Rosenberger, F. Numerical modeling of diffusive-convective physical vapor transport in cylindrical vertical ampoules. J. Cryst. Growth 1981, 51, 426–437. [Google Scholar] [CrossRef]
- Klosse, K.; Ullersma, P. Convection in a chemical vapor transport process. J. Cryst. Growth 1973, 18, 167–174. [Google Scholar] [CrossRef]
- Klosse, K. A New Productivity Function and Stability Criterion in Chemical Vapor Transport Processes. J. Solid State Chem. 1975, 15, 105–115. [Google Scholar] [CrossRef][Green Version]
- Binnewies, M.; Glaum, R.; Schmidt, M.; Schmidt, P. Chemical Vapor Transport Reactions—A Historical Review. Z. Anorg. Allg. Chem. 2013, 639, 219–229. [Google Scholar] [CrossRef]
- Schäfer, H. Chemische Transportreaktionen. Der Transport anorganischer Stoffe uber die Gasphase und seine Anwendung. Angew. Chem. 1962, 66, 885. [Google Scholar]
- Lauck, R. Chemical vapor transport of zinc sulfide. Part I: Isotopic crystals from nearly stoichiometric vapor phase. J. Cryst. Growth 2010, 312, 3642–3649. [Google Scholar] [CrossRef]
- Kumar, O.S.; Soundeswaran, S.; Dhanasekaran, R. Thermodynamic Calculations and Growth of ZnSe Single Crystals by Chemical Vapor Transport Technique. Cryst. Growth Des. 2002, 2, 585–589. [Google Scholar] [CrossRef]
- Li, Z.; Xi, X.; Ding, B.; Li, H.; Liu, E.; Yao, Y.; Wang, W. Thermodynamics and Kinetics Synergy for Controlled Synthesis of 2D van der Waals Single-Crystal NbSe2 via Modified Chemical Vapor Transport. Cryst. Growth Des. 2020, 20, 706–712. [Google Scholar] [CrossRef]
- Grönke, M.; Pohflepp, D.; Schmidt, P.; Valldor, M.; Oswald, S.; Wolf, D.; Hao, Q.; Steiner, U.; Büchner, B.; Hampel, S. Simulation and synthesis of α-MoCl3 nanosheets on substrates by short time chemical vapor transport. Nano-Struct. Nano-Objects 2019, 19, 100324. [Google Scholar] [CrossRef]
- Grönke, M.; Buschbeck, B.; Schmidt, P.; Valldor, M.; Oswald, S.; Hao, Q.; Lubk, A.; Wolf, D.; Steiner, U.; Büchner, B.; et al. Chromium Trihalides CrX3 (X = Cl, Br, I): Direct Deposition of Micro- and Nanosheets on Substrates by Chemical Vapor Transport. Adv. Mater. Interfaces 2019, 6, 1901410. [Google Scholar] [CrossRef]
- Nowka, C.; Gellesch, M.; Borrero, J.E.H.; Partzsch, S.; Wuttke, C.; Steckel, F.; Hess, C.; Wolter, A.U.B.; Bohorquez, L.T.C.; Büchner, B.; et al. Chemical vapor transport and characterization of MnBi2Se4. J. Cryst. Growth 2017, 459, 81–86. [Google Scholar] [CrossRef]
- Available online: https://www.htw-dresden.de/luc/forschung/chemieingenieurwesen/festkoerperchemie/tragmin (accessed on 28 January 2025).

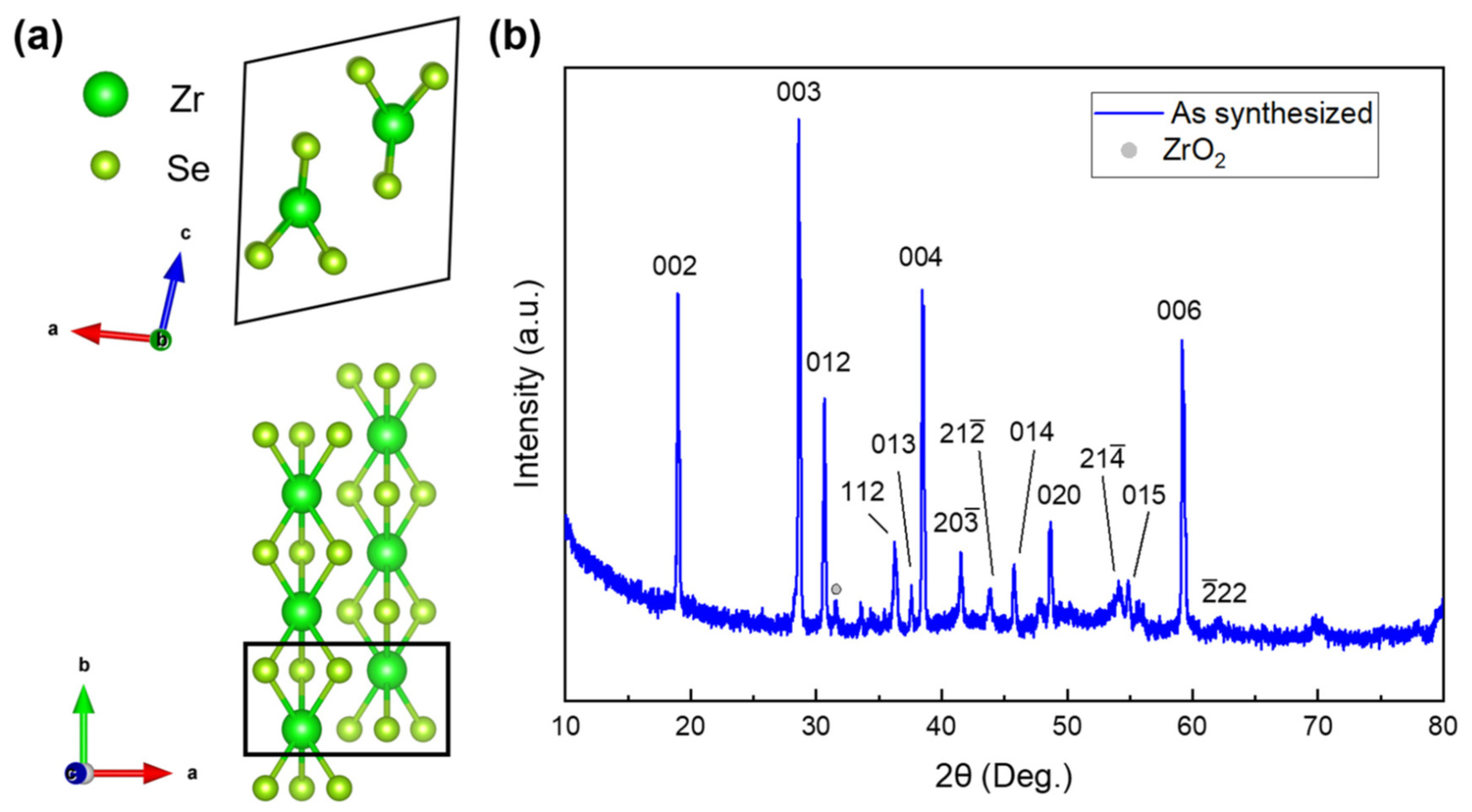

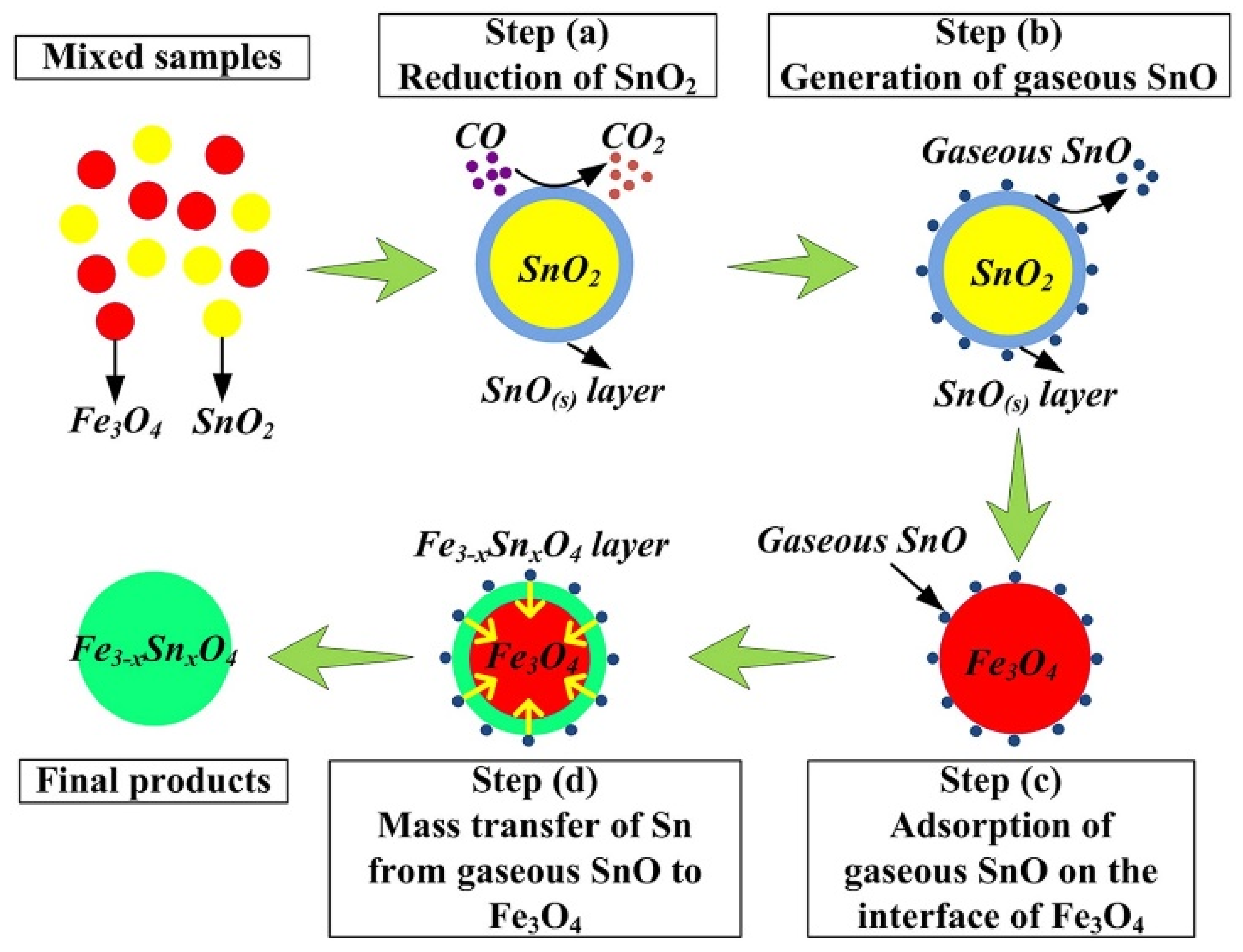
| Property | T = 303 K | T = 423 K |
|---|---|---|
| ρbulk [Ω·cm] | 16.78 | 5.26 |
| σbulk [ʊ·cm] | 5.96 × 10−2 | 0.19 |
| S [μV∙K] | −148 | −372 |
| k [W∙m−1∙K−1] | 0.37 | 2.83 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Matyszczak, G.; Krawczyk, K.; Yedzikhanau, A.; Brzozowski, M. A Chemical Transport Method for the Synthesis of Simple and Complex Inorganic Crystals—Survey of Applications and Modeling. Crystals 2025, 15, 162. https://doi.org/10.3390/cryst15020162
Matyszczak G, Krawczyk K, Yedzikhanau A, Brzozowski M. A Chemical Transport Method for the Synthesis of Simple and Complex Inorganic Crystals—Survey of Applications and Modeling. Crystals. 2025; 15(2):162. https://doi.org/10.3390/cryst15020162
Chicago/Turabian StyleMatyszczak, Grzegorz, Krzysztof Krawczyk, Albert Yedzikhanau, and Michał Brzozowski. 2025. "A Chemical Transport Method for the Synthesis of Simple and Complex Inorganic Crystals—Survey of Applications and Modeling" Crystals 15, no. 2: 162. https://doi.org/10.3390/cryst15020162
APA StyleMatyszczak, G., Krawczyk, K., Yedzikhanau, A., & Brzozowski, M. (2025). A Chemical Transport Method for the Synthesis of Simple and Complex Inorganic Crystals—Survey of Applications and Modeling. Crystals, 15(2), 162. https://doi.org/10.3390/cryst15020162







