Additive Manufacturing of High-Performance Ti-Mo Alloys Used on a Puncture Needle: The Role of Linear Energy Density in Microstructure Evolution and Mechanical Properties
Abstract
1. Introduction
2. Experimental Procedure
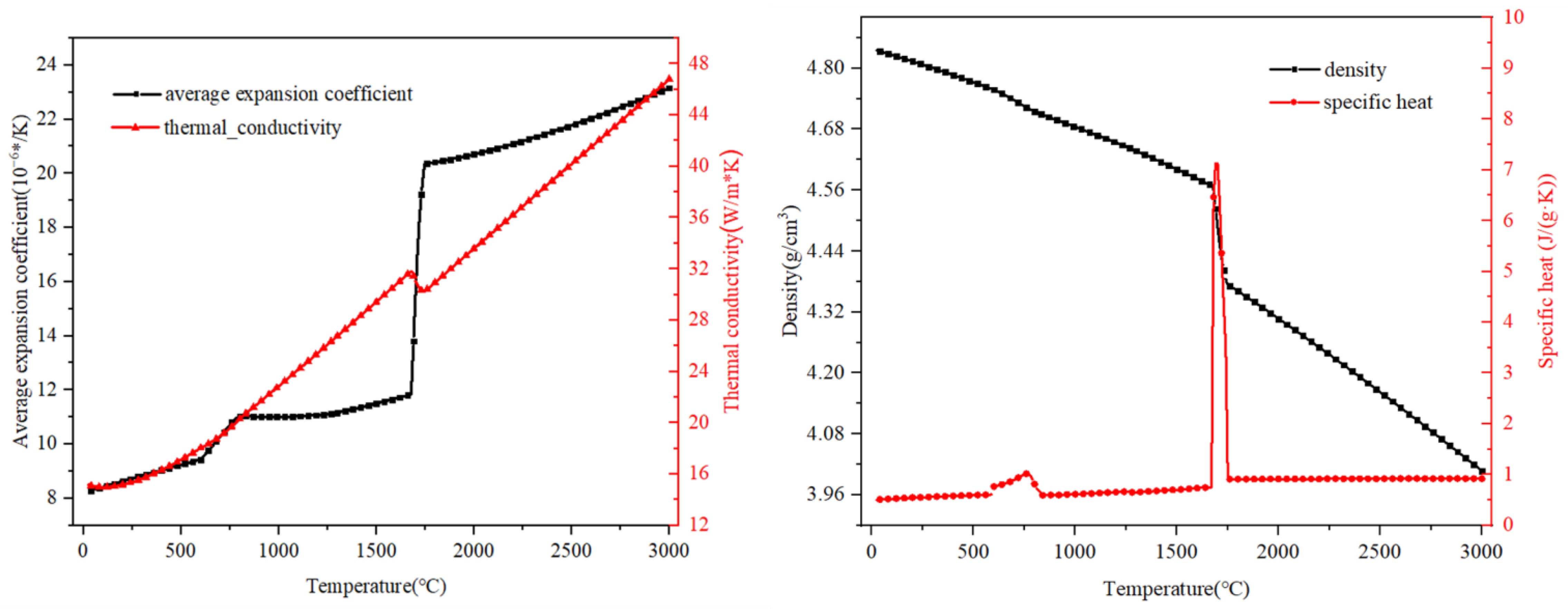
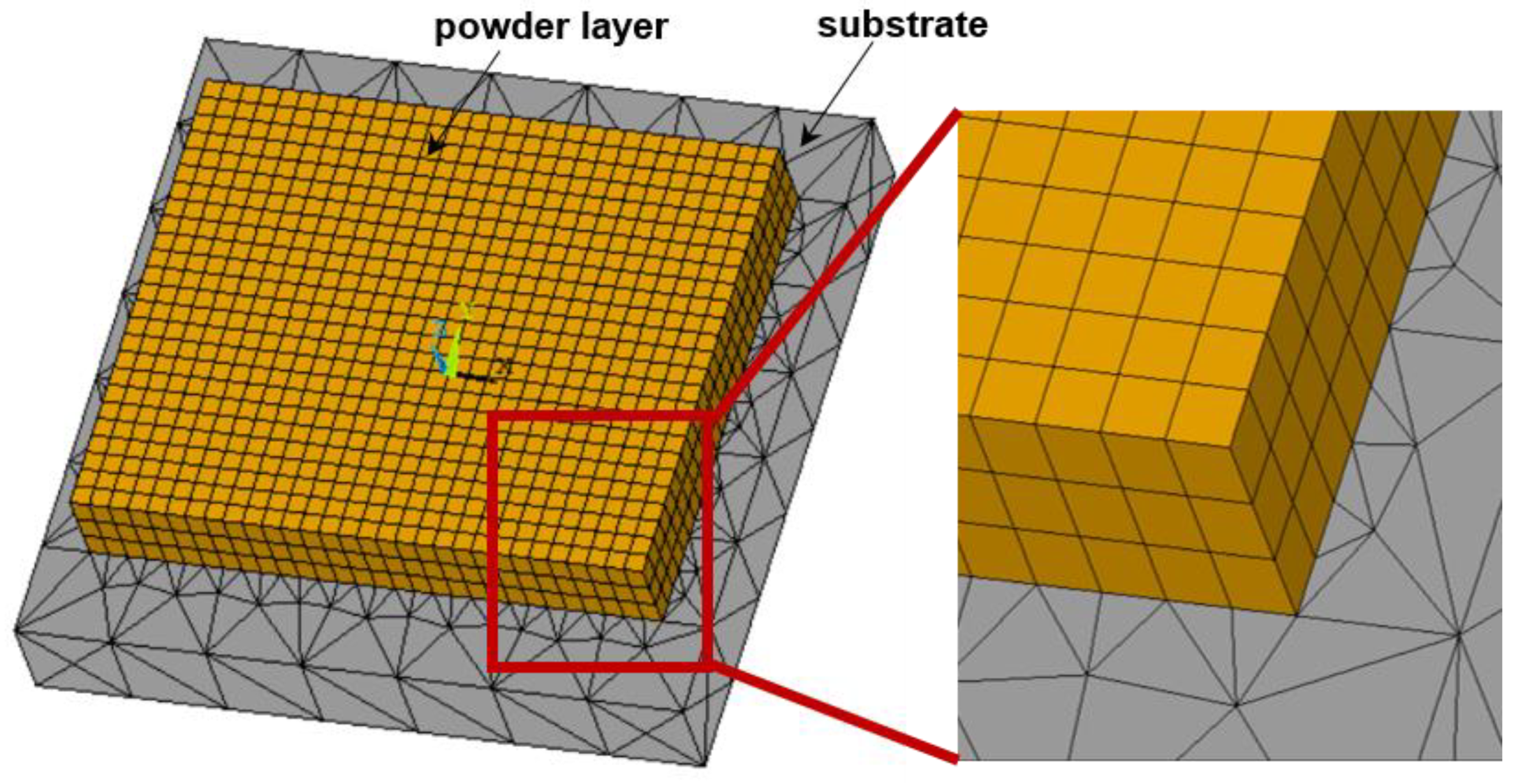
2.1. FEM Modeling for SLM
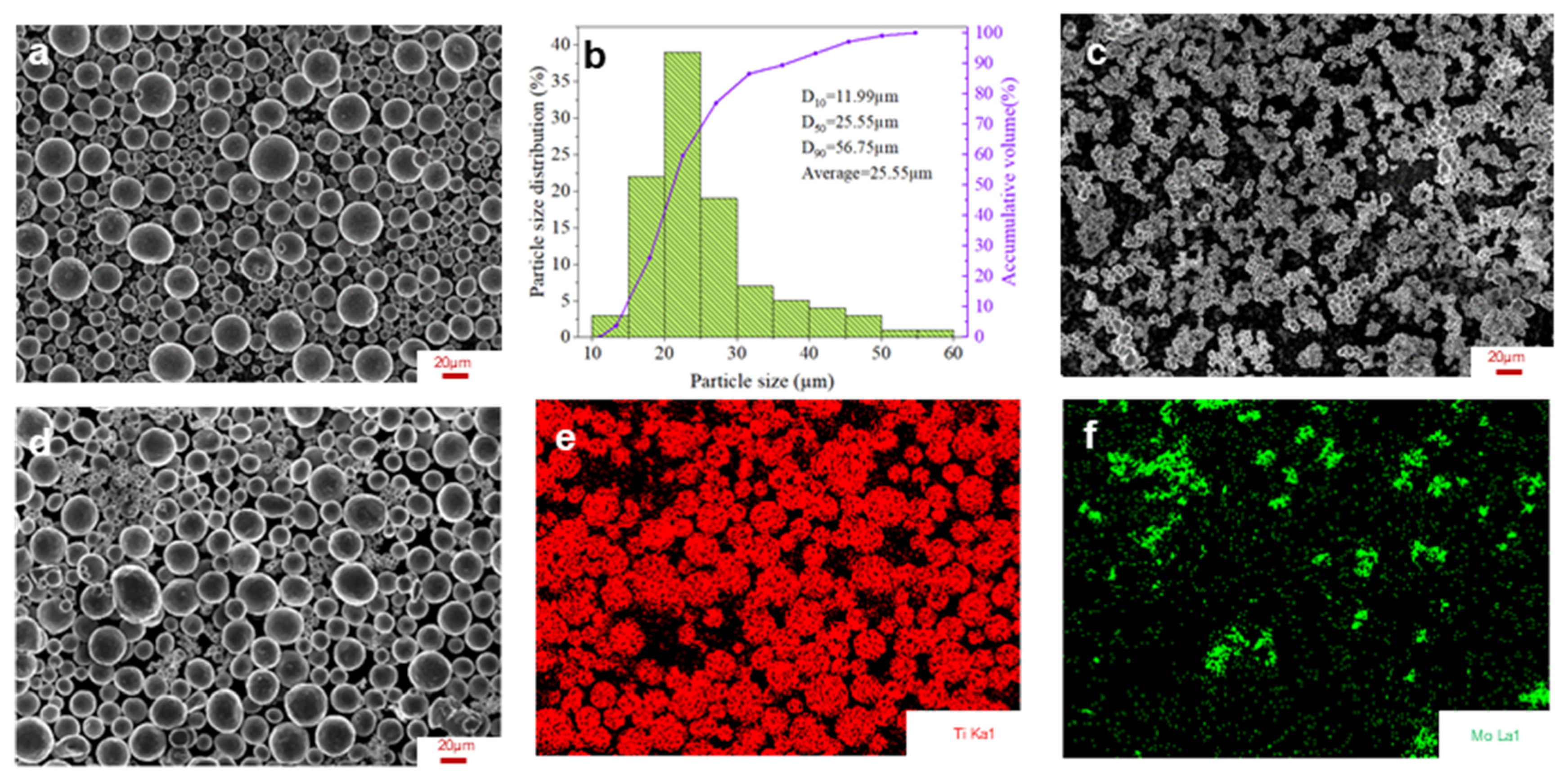
2.2. Materials Preparation
2.3. In Situ Fabrication of Ti-10Mo Samples by SLM
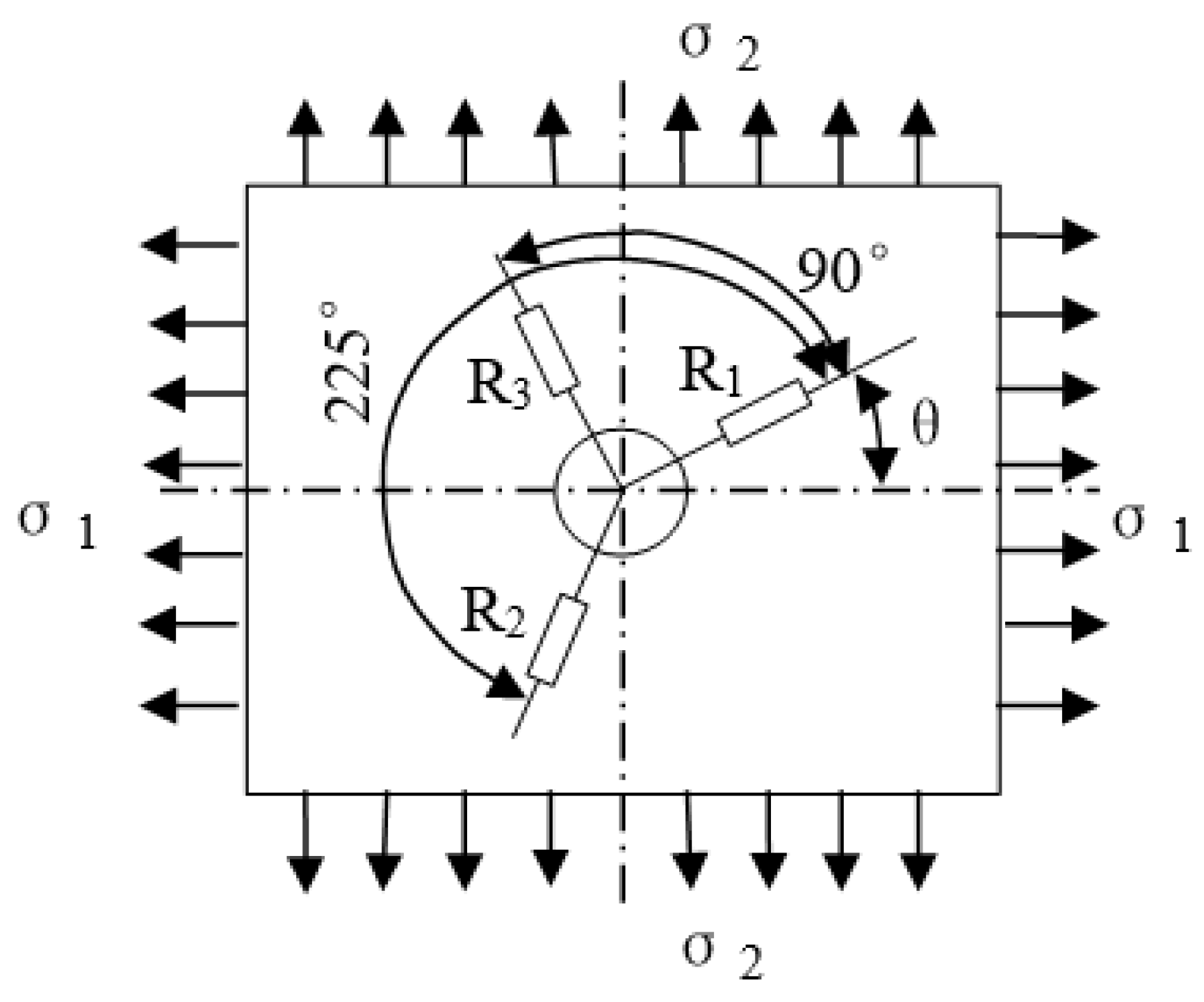
2.4. Characterizations
2.5. Mechanical Testing
3. Results and Discussion
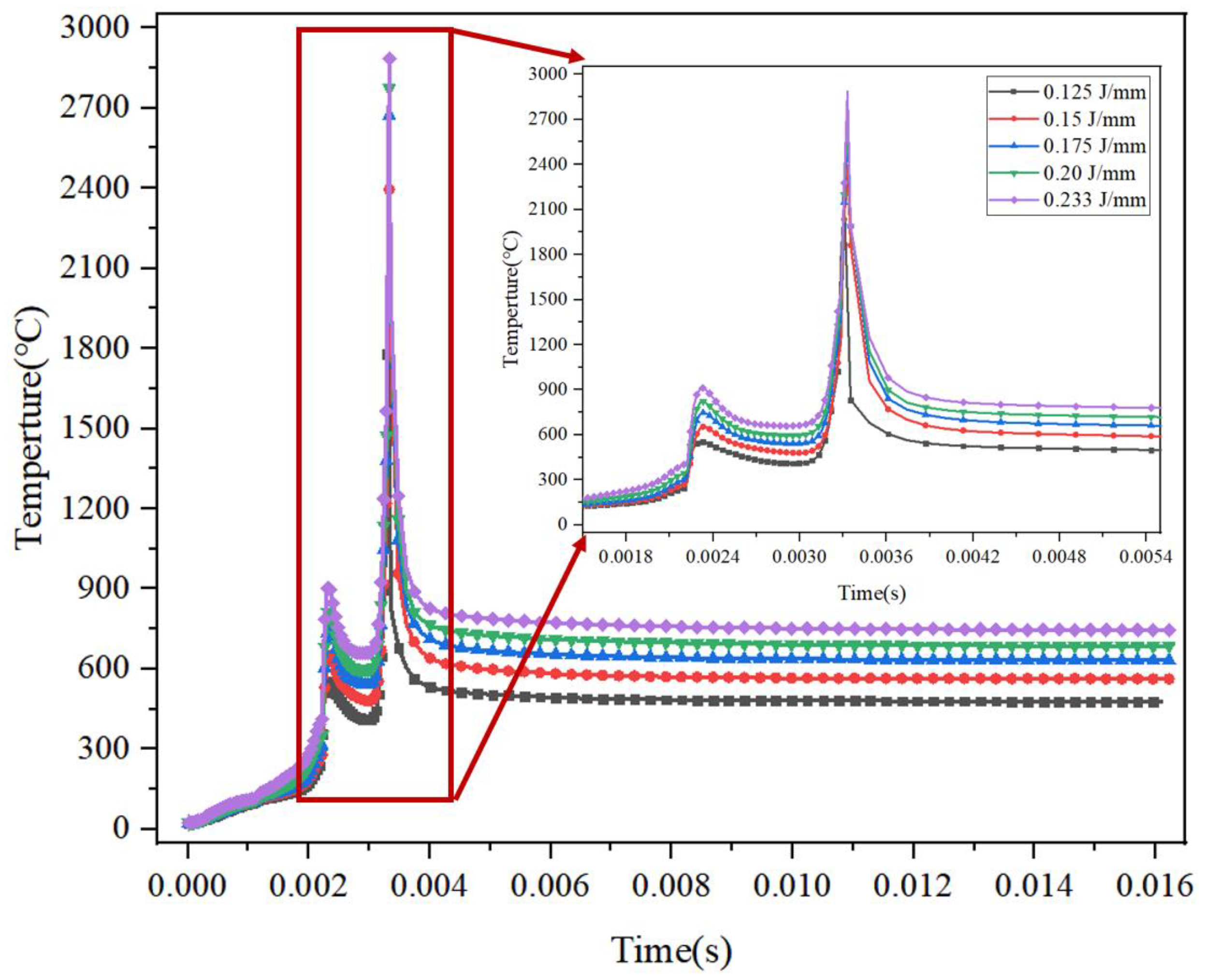
3.1. Subsection Residual Stress Evolution
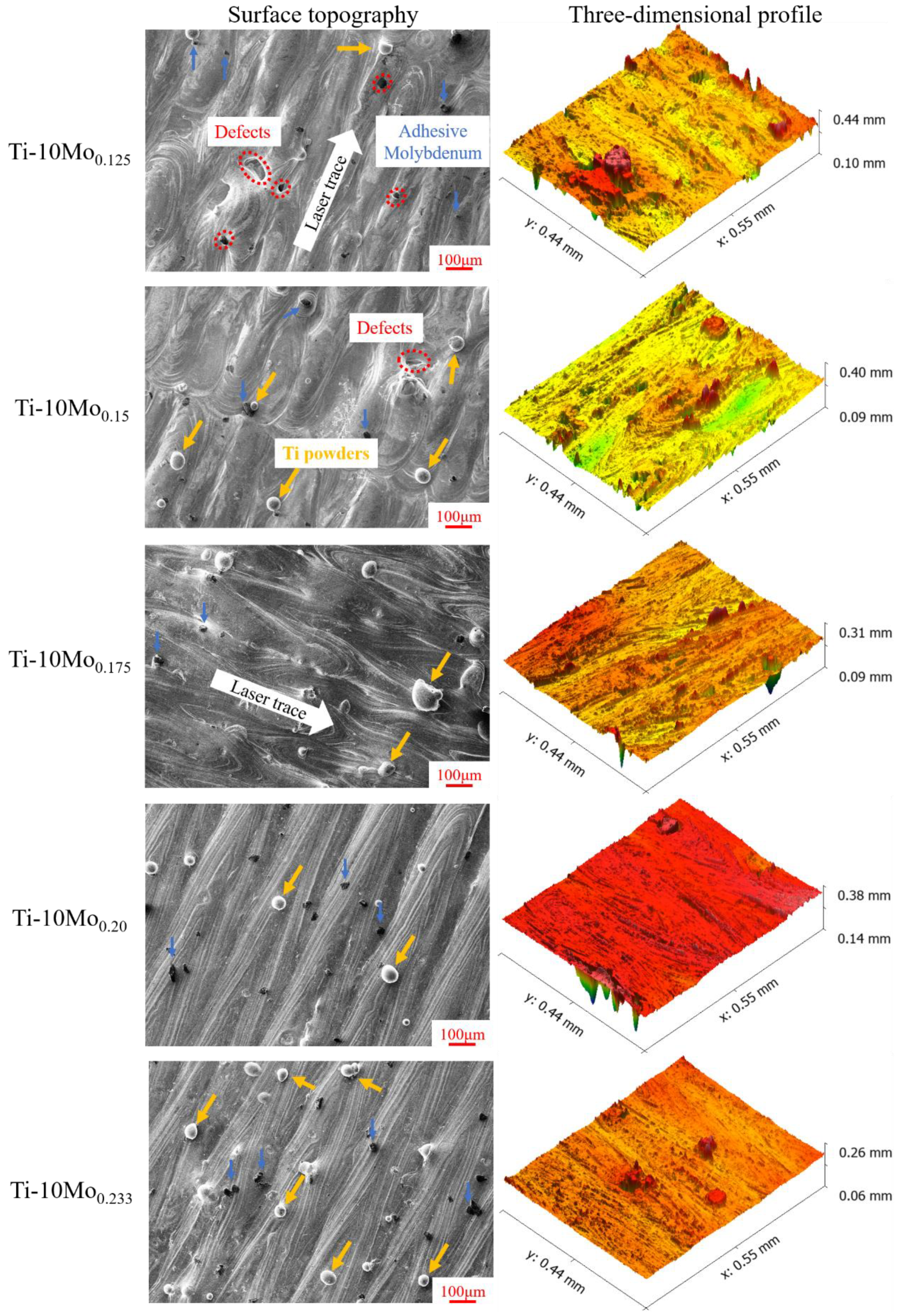
3.2. Surface Morphology
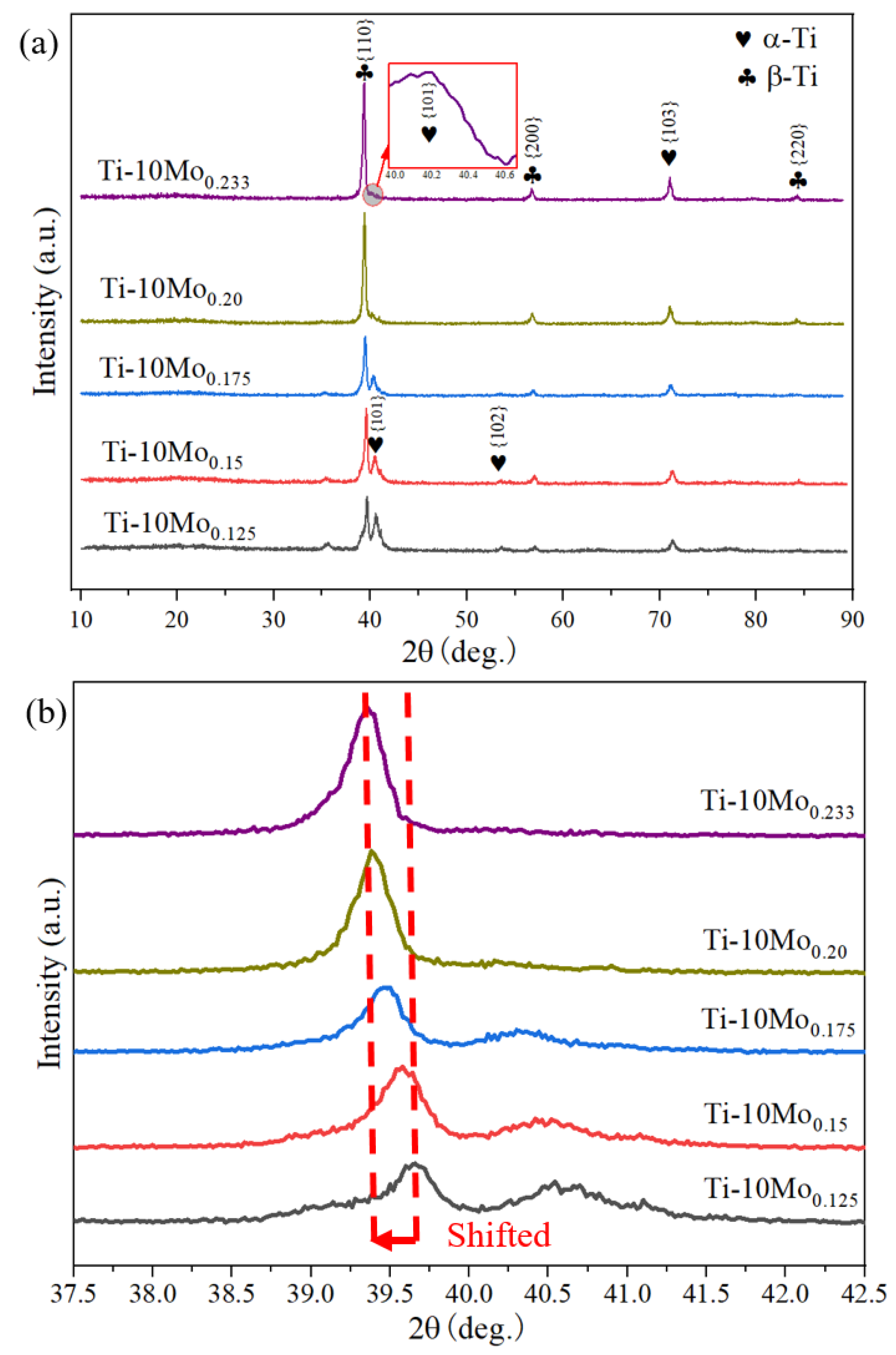
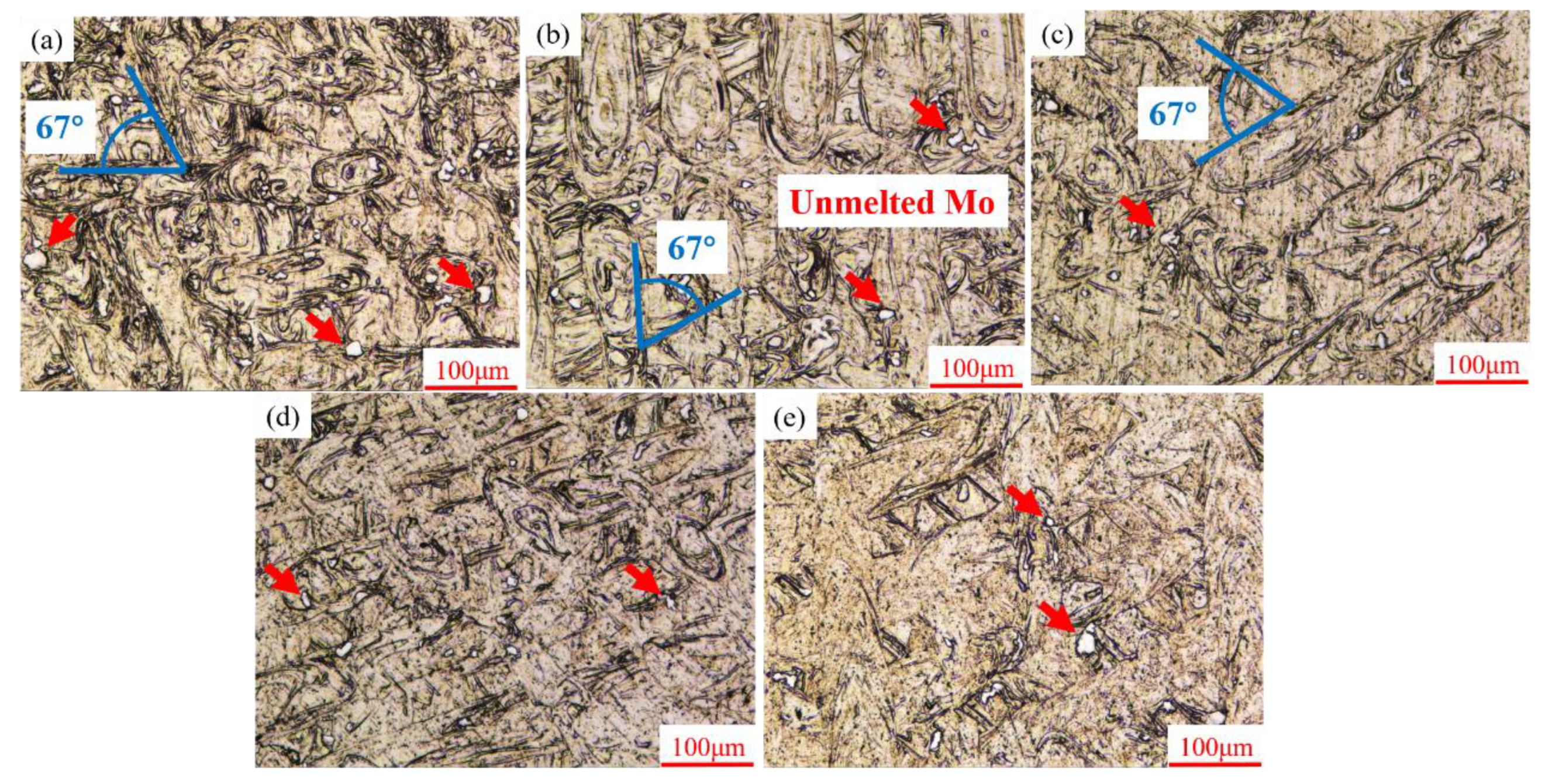
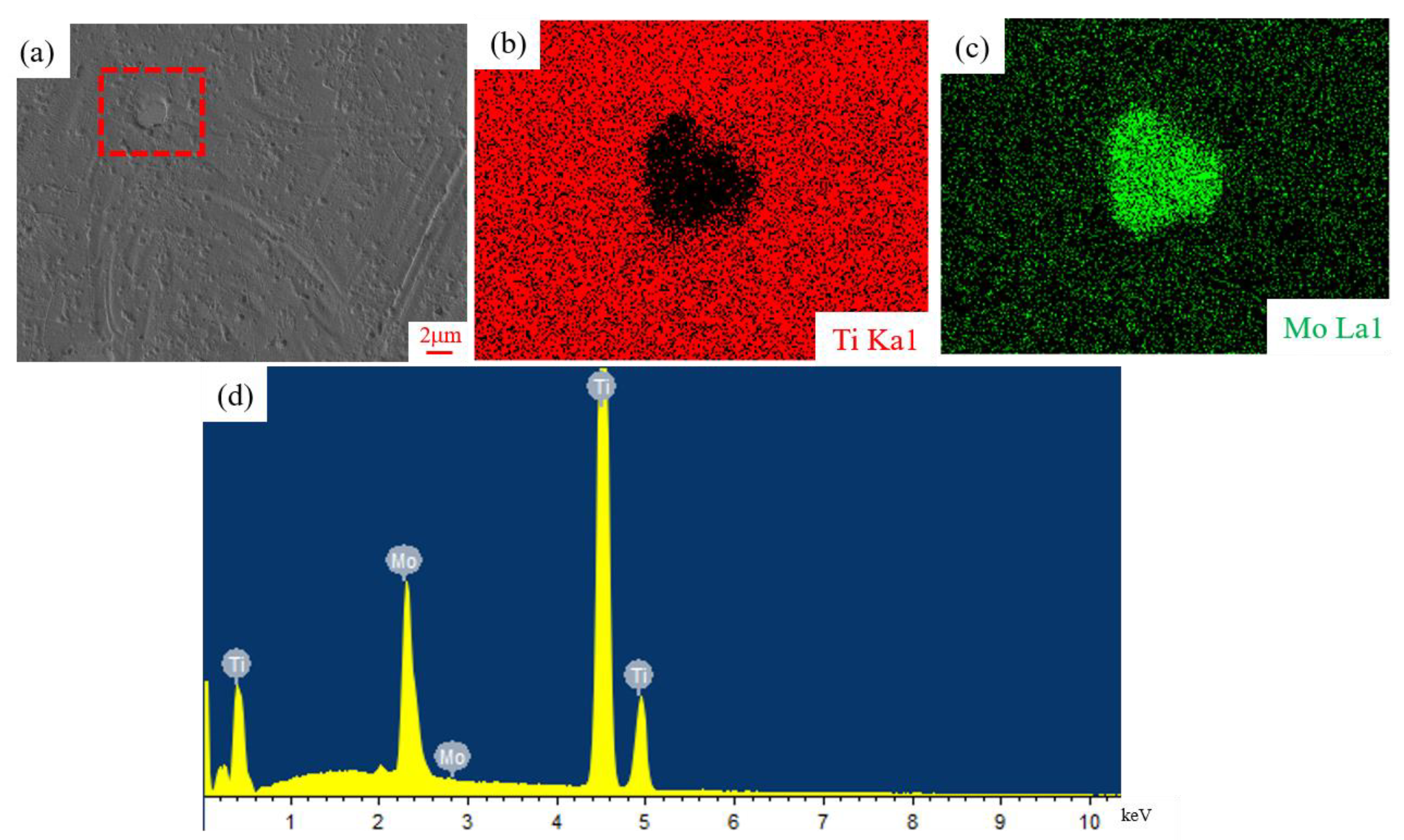
3.3. Microstructural Characterization
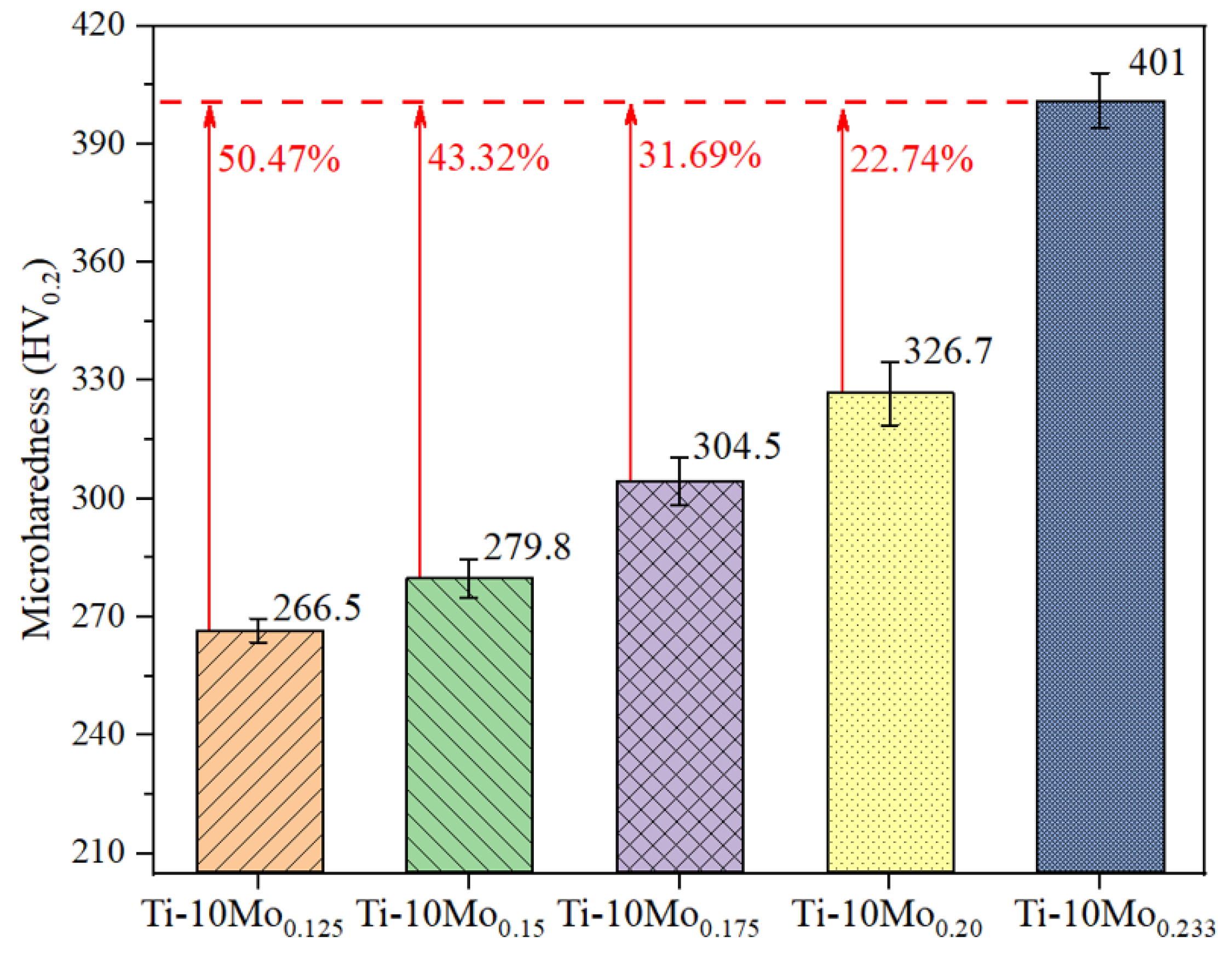
3.4. Microhardness
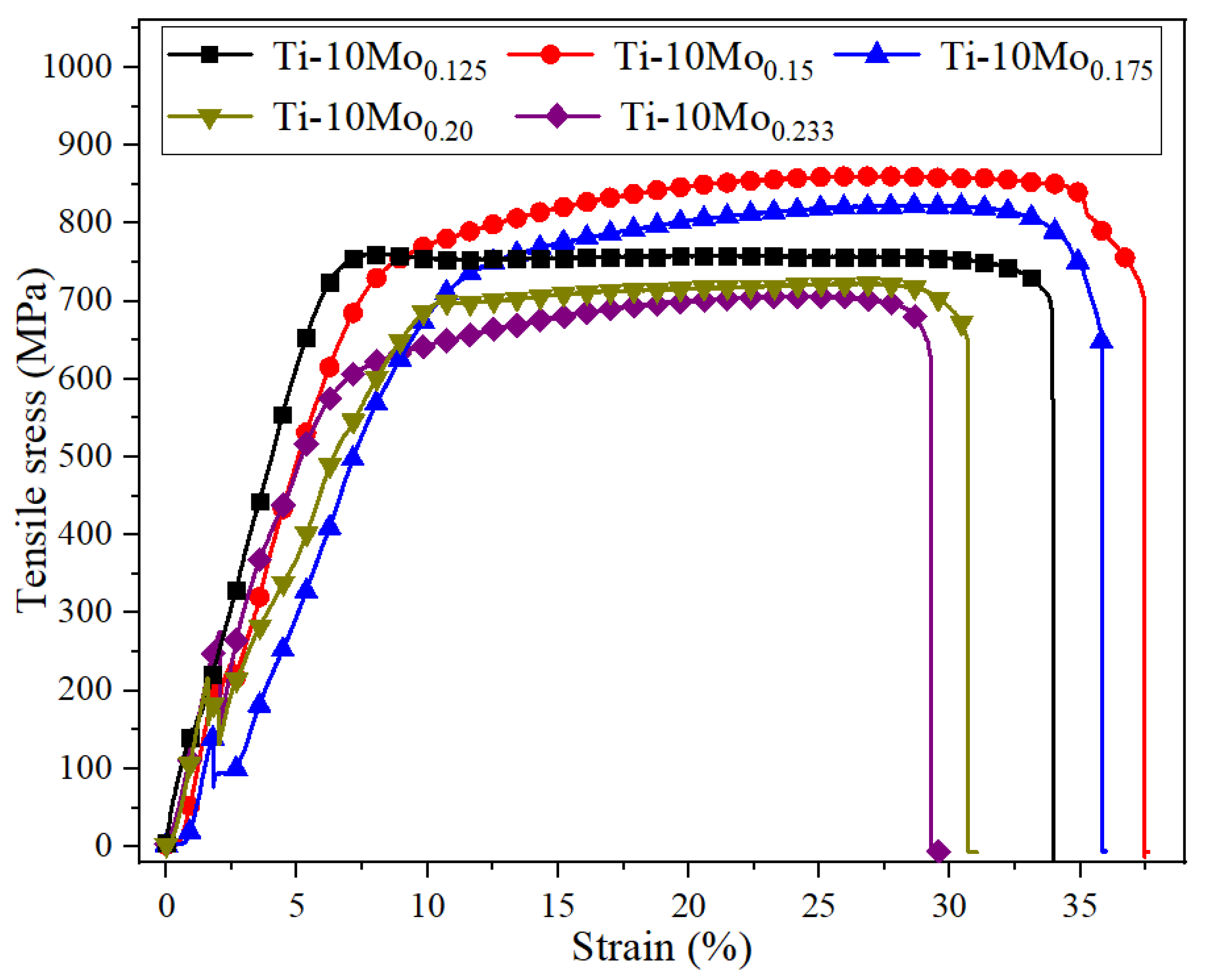
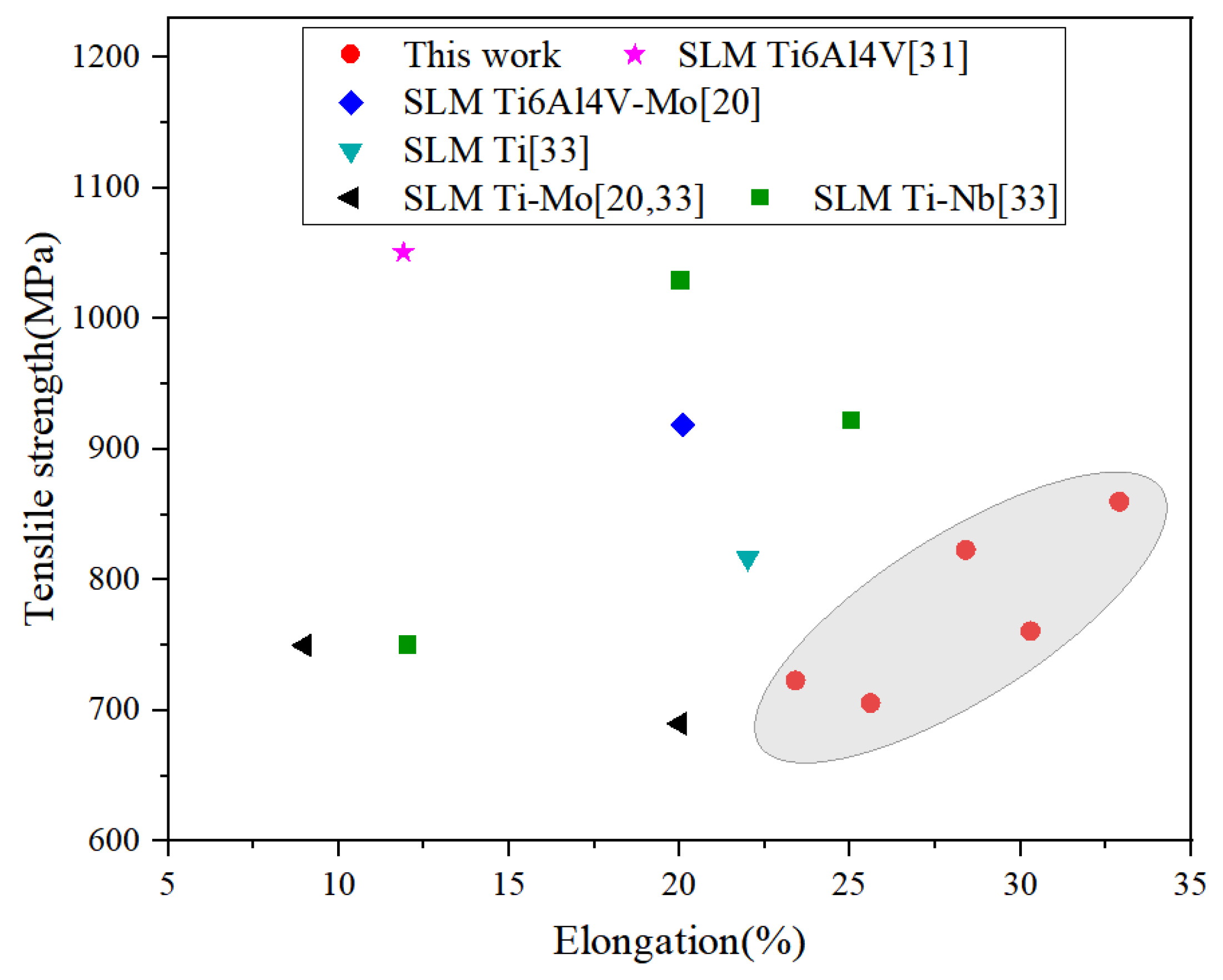
3.5. Tensile Properties
4. Conclusions
- (1)
- The residual stress in SLM-processed components is influenced by the cooling rate and temperature evolution. The maximum values of Von Mises stress increased with the higher linear energy density, and both the simulation and experimental results showed a good agreement, with an error range of 17–23%.
- (2)
- The applied linear energy density significantly influenced the densification and roughness of SLM-processed Ti-10Mo parts. As the linear energy density increased from 0.120 J/mm to 0.233 J/mm, the surface roughness decreased from 18.2 μm to 4.4 μm and relative compactness increased from 94.9% to 99.8%.
- (3)
- The SLM-processed Ti-10Mo samples present a special conchoidal two-phase structure, including hexagonal close-packed (hcp) α-Ti and body-centered cubic (bcc) β-Ti. With the linear energy density increases, the β-stabilizer Mo particle was nearly completely melted, leading to an increment in the densification.
- (4)
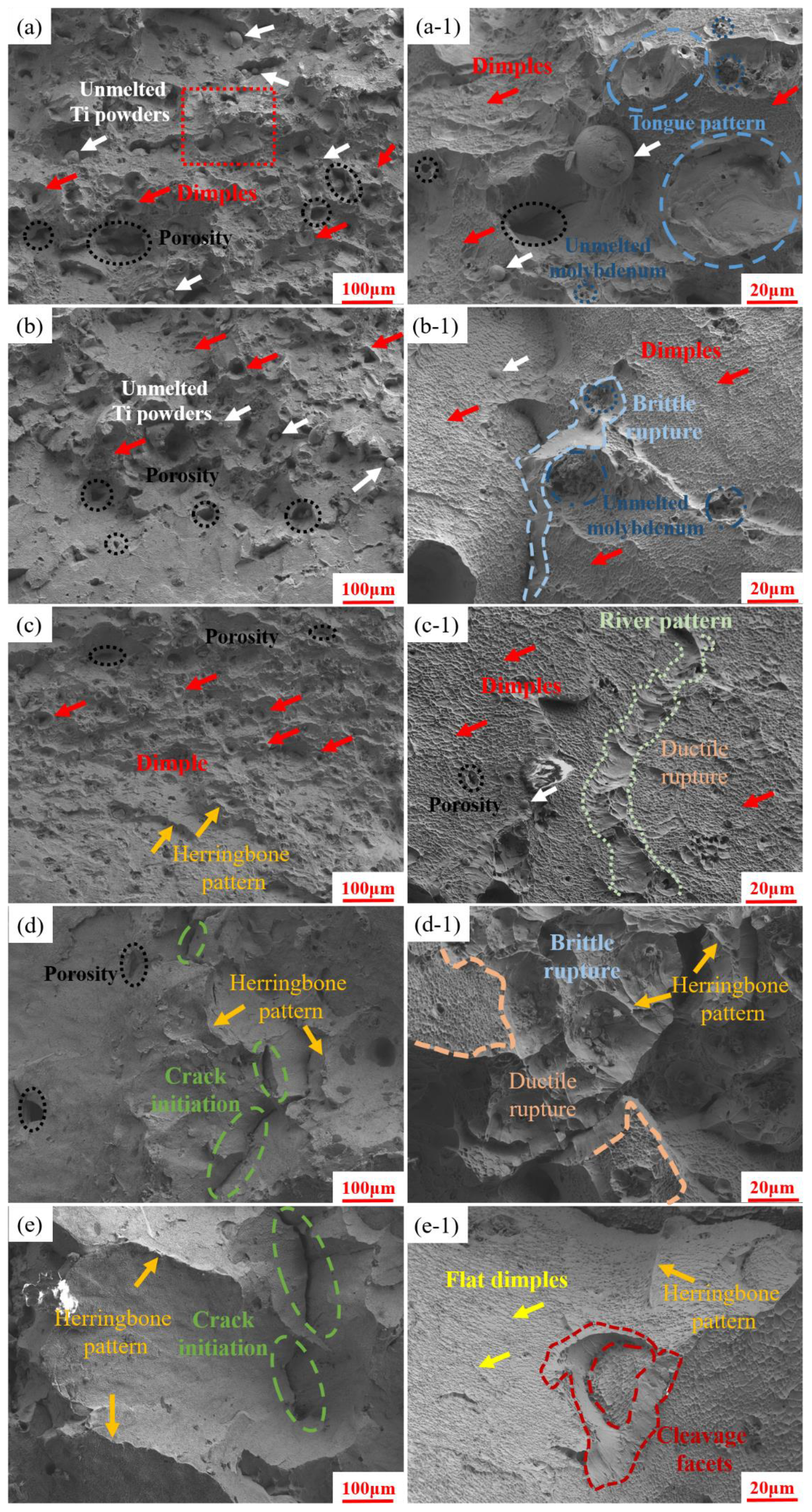
- The Ti-10Mo alloys prepared at a linear energy density of 0.15 J/mm exhibited the highest UTS and YS of 860 MPa and 752 MPa with a ductile fracture. However, when a higher linear energy density was applied, the fracture mechanism was a predominantly brittle fracture that was accompanied by a ductile fracture.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| SLM | Linear dichroism selective laser melting |
| FEM | Finite element model |
| SEM | Scanning electron microscopy |
| HDM | Hole-drilling method |
| UTS | Ultimate tensile strength |
| YS | Yield strength |
| hcp | Hexagonal tight-packed |
| bcc | Body-centered cubic |
References
- Nguyen, D.S.; Park, H.S.; Lee, C.M. Optimization of selective laser melting process parameters for Ti-6Al-4V alloy manufacturing using deep learning. J. Manuf. Process. 2020, 55, 230–235. [Google Scholar] [CrossRef]
- Carter, F.M.; Porter, C.; Kozjek, D.; Shimoyoshi, K.; Fujishima, M.; Irino, N.; Cao, J. Machine learning guided adaptive laser power control in selective laser melting for pore reduction. CIRP Ann. Manuf. Technol. 2024, 73, 149–152. [Google Scholar] [CrossRef]
- Liu, S.J.; Lee, M.; Choi, C.; Shin, K. Effect of additive manufacturing of SUS316L using selective laser melting. J. Mater. Res. Technol. 2023, 24, 9824–9833. [Google Scholar] [CrossRef]
- Lu, S.L.; Zhang, Z.J.; Liu, R.; Qu, Z.; Li, S.J.; Zhou, X.H.; Duan, Q.Q.; Zhang, B.N.; Zhao, X.M.; Zhao, W.; et al. Tailoring hierarchical microstructures to improve the strength and plasticity of a laser powder bed fusion additively manufactured Ti-6Al-4V alloy. Addit. Manuf. 2023, 71, 103603. [Google Scholar] [CrossRef]
- Qian, J.; Zhang, L.; Tang, S.L. Preparation of Spherical Porous and Spherical Ti6Al4V Powder by Copper-Assisted Spheroidization Method. JOM 2023, 76, 1276–1283. [Google Scholar] [CrossRef]
- Zhang, X.; Liu, G.; Yi, J.; Liu, H.X. TiN/Ti-Al-Nb composite coatings prepared by in-situ synthesis assisted laser cladding process on Ti6Al4V titanium alloy surface. Surf. Technol. 2020, 49, 61–68. [Google Scholar]
- Zhou, Y.H.; Li, W.P.; Zhang, L.; Zhou, S.; Jia, X.; Wang, D.; Yan, M. Selective laser melting of Ti-22Al-25Nb intermetallic: Significant effects of hatch distance on microstructural features and mechanical properties. J. Mater. Process. Technol. 2020, 276, 116398. [Google Scholar] [CrossRef]
- Asri, R.I.M.; Harun, W.S.W.; Samykano, M.; Lah, N.A.C.; Ghani, S.A.C.; Tarlochan, F.; Raza, M.R. Corrosion and surface modification on biocompatible metals: A review. Mater. Sci. Eng. C Mater. Biol. Appl. 2017, 77, 1261–1274. [Google Scholar] [CrossRef]
- Hanada, S.; Masahashi, N.; Semboshi, S.; Jung, T.K. Low Young’s modulus of cold groove-rolled β Ti–Nb–Sn alloys for orthopedic applications. Mater. Sci. Eng. A-Struct. Mater. Prop. Microstruct. Process. 2021, 802, 140645. [Google Scholar] [CrossRef]
- Li, S.L.; Lee, W.T.; Yeom, J.T.; Kim, J.G.; Oh, J.S.; Lee, T.; Liu, Y.; Nam, T.H. Towards bone-like elastic modulus in Tisingle bondNbsingle bondSn alloys with large recovery strain for biomedical applications. J. Alloys Compd. 2022, 925, 166724. [Google Scholar] [CrossRef]
- Shahzamanian, M.M.; Banerjee, R.; Dahotre, N.B.; Srinivasa, A.R.; Reddy, J. Analysis of stress shielding reduction in bone fracture fixation implant using functionally graded materials. Compos. Struct. 2023, 321, 117262. [Google Scholar] [CrossRef]
- Zhang, C.Y.; Zeng, C.Y.; Wang, Z.F.; Zeng, T.; Wang, Y. Optimization of stress distribution of bone-implant interface (BII). Biomater. Adv. 2023, 147, 213342. [Google Scholar] [CrossRef] [PubMed]
- Eldabah, N.M.; Shoukry, A.; Khair-Eldeen, W.; Kobayashi, S.; Gepreel, M.A.H. Design and characterization of low Young’s modulus Ti-Zr-Nb-based medium entropy alloys assisted by extreme learning machine for biomedical applications. J. Alloys Compd. 2023, 968, 171755. [Google Scholar] [CrossRef]
- Namini, A.S.; Dilawary, S.A.A.; Motallebzadeh, A.; Asl, M.S. Effect of TiB2 addition on the elevated temperature tribological behavior of spark plasma sintered Ti matrix composite. Comp. Part B Eng. 2019, 172, 271–280. [Google Scholar] [CrossRef]
- Jia, Y.J.; Han, W.Z. Beta phase controls hydride precipitation within alpha phase in dual-phase Zr-2.5Nb. Scripta. Mater. 2025, 256, 116419. [Google Scholar] [CrossRef]
- Lourenço, L.M.; Correa, R.M.; Almeida, D.S.G.; Kuroda, P.A.B.; Zambuzzi, W.F.; Grandini, C.R. Development of new β Ti-10-Mo-Mn alloys for biomedical applications. J. Alloys Compd. 2024, 1008, 176524. [Google Scholar] [CrossRef]
- Fischer, M.; Joguet, D.; Robin, G.; Peltier, L.; Laheurte, P. In situ elaboration of a binary Ti-6Nb alloy by selective laser melting of elemental titanium and niobium mixed powders. Mater. Sci. Eng. C. 2016, 62, 852–859. [Google Scholar] [CrossRef]
- Sing, S.L.; Wiria, F.E.; Yeong, W.Y. Selective laser melting of titanium alloy with 50 wt% tantalum: Effect of laser process parameters on part quality. Int. J. Refract. Met. Hard Mater. 2018, 77, 120–127. [Google Scholar] [CrossRef]
- Li, W.; Song, Y.; Qian, X.; Zhou, L.; Zhu, H.; Shen, L.; Dai, Y.; Dong, F.; Li, Y. Radiomics analysis combining gray-scale ultrasound and mammography for differentiating breast adenosis from invasive ductal carcinoma. Front. Oncol. 2024, 14, 1390342. [Google Scholar] [CrossRef]
- Vrancken, B.; Thijs, L.; Kruth, J.P.; Van Humbeeck, J. Microstructure and mechanical properties of a novel β titanium metallic composite by selective laser melting. Acta Mater. 2014, 68, 150–158. [Google Scholar] [CrossRef]
- Kahlert, M.; Wegener, T.; Laabs, L.; Vollmer, M.; Niendorf, T. Influence of Defects and Microstructure on the Thermal Expansion Behavior and the Mechanical Properties of Additively Manufactured Fe-36Ni. Materials 2024, 17, 4313. [Google Scholar] [CrossRef] [PubMed]
- Yang, Y.B.; Lin, H.; Li, Q. A computationally efficient thermo-mechanical model with temporal acceleration for prediction of residual stresses deformations in, W.A.A.M. Virtual Phys. Prototyp. 2024, 19, e2349683. [Google Scholar] [CrossRef]
- Luo, Z.B.; Zhao, Y.Y. Numerical simulation of part-level temperature fields during selective laser melting of stainless steel 316L. Int. J. Adv. Manuf. Technol. 2019, 104, 1615–1635. [Google Scholar] [CrossRef]
- Waqar, S.; Guo, K.; Sun, J. FEM analysis of thermal and residual stress profile in selective laser melting of 316L stainless steel. J. Mater. Process. 2021, 66, 81–100. [Google Scholar] [CrossRef]
- Markovic, P.; Scheel, P.; Wróbel, R.; Leinenbach, C.; Mazza, E.; Hosseini, E. Cyclic mechanical response of LPBFHastelloy X over a wide temperature strain range: Experiments modelling. Int. J. Solids Struct. 2024, 305, 113047. [Google Scholar] [CrossRef]
- Xiao, Z.X.; Chen, C.P.; Zhu, H.H.; Hu, Z.H.; Nagarajan, B.; Guo, L.B.; Zeng, X.Y. Study of residual stress in selective laser melting of, T.i.6.A.l.4.V. Mater Design. 2020, 193. [Google Scholar] [CrossRef]
- Zhang, J.; Liu, C.; Hu, Q.D.; Liu, Q.; Li, H. Residual Stress Characterization in Additively Manufactured Titanium Alloy Rod-Type Specimens Prepared by Laser Powder Bed Fusion. JOM 2024. [Google Scholar] [CrossRef]
- Chen, C.; Yin, J.; Zhu, H.; Xiao, Z.; Zhang, L.; Zeng, X. Effect of overlap rate and pattern on residual stress in selective laser melting. Int. J. Mach. Tools Manuf. 2019, 145. [Google Scholar] [CrossRef]
- Weldeanenia, G.K.; Kassegne, K.S.; Perumalla, R.J. Minimization of melt-pool field variables fluctuation during selective laser melting of Ti6Al4V alloy through computational investigation. Model. Simul. Mater. Sci. Eng. 2025, 33, 015002. [Google Scholar] [CrossRef]
- Yi, H.; Jiao, F.R.; Cao, H.J.; Zhang, J.; Zou, Y. Numerical simulations of molten pool dynamics in wire-arc directed energy deposition processes: A review. J. Manuf. Process. 2025, 134, 970–997. [Google Scholar] [CrossRef]
- Xie, F.X.; He, X.B.; Cao, S.L.; Lu, X.; Qu, X. Structural characterization and electrochemical behavior of a laser-sintered porous Ti–10Mo alloy. Corros Sci. 2013, 67, 217–224. [Google Scholar] [CrossRef]
- Kang, N.; Li, Y.; Lin, X.; Feng, E.; Huang, W. Microstructure and tensile properties of Ti-Mo alloys manufactured via using laser powder bed fusion. J. Alloys Compd. 2019, 771, 877–884. [Google Scholar] [CrossRef]
- Luo, H.Y.; Sun, X.; Xu, L.; He, W.; Liang, X. A review on stress determination and control in metal-based additive manufacturing. Theor. Appl. Mech. Lett. 2023, 13, 100396. [Google Scholar] [CrossRef]
- Arya, P.K.; Jain, N.K.; Sathiaraj, D. Microstructure mechanical properties of additively manufactured Ti6Al4VxCryNi alloy. CIRP J. Manuf. Sci. Technol. 2024, 53, 67–80. [Google Scholar] [CrossRef]


| Powder | Chemical Composition (wt.%) | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Fe | C | O | N | H | W | Si | Zr | Ni | Ti | Mo | ||
| Ti | 0.25 | 0.1 | 0.13 | 0.03 | 0.015 | / | / | / | / | / | Bal. | / |
| Mo | 0.001 | / | / | / | / | 0.002 | 0.003 | 0.002 | 0.001 | 0.001 | / | Bal. |
| Samples | Laser Power (W) | Scanning Speed (mm/s) | Laser Linear Energy Density (J/mm) |
|---|---|---|---|
| Ti-10Mo0.125 | 150 | 1200 | 0.125 |
| Ti-10Mo0.150 | 180 | 1200 | 0.15 |
| Ti-10Mo0.175 | 210 | 1200 | 0.175 |
| Ti-10Mo0.20 | 210 | 1050 | 0.20 |
| Ti-10Mo0.233 | 210 | 900 | 0.233 |
| Ti-10Mo0.125 | Ti-10Mo0.150 | Ti-10Mo0.175 | Ti-10Mo0.20 | Ti-10Mo0.233 | |
|---|---|---|---|---|---|
| Peak temperature (°C) | 2177.66 | 2394.69 | 2670.80 | 2775.45 | 2886.16 |
| Cooling rate (×106 °C·s−1) | 26.02 | 26.47 | 29.09 | 29.35 | 29.74 |
| Samples | Ti-10Mo0.125 | Ti-10Mo0.150 | Ti-10Mo0.175 | Ti-10Mo0.20 | Ti-10Mo0.233 | |
|---|---|---|---|---|---|---|
| Simulation data | Von Mises stress | +309 | +338 | +367 | +419 | +440 |
| X-direction stress | −171 | −184 | −197 | −219 | −227 | |
| Y-direction stress | −256 | −280 | −303 | −345 | −364 | |
| Z-direction stress | −99.3 | −106 | −113 | −127 | −132 | |
| Experimental results | +263 ± 10 | +286 ± 15 | +305 ± 20 | +341 ± 25 | +374 ± 25 | |
| Error (%) | 17.5 | 18.2 | 20.3 | 22.9 | 17.6 | |
| Samples | Ti-10Mo0.125 | Ti-10Mo0.15 | Ti-10Mo0.175 | Ti-10Mo0.20 | Ti-10Mo0.233 | |
|---|---|---|---|---|---|---|
| Surface Roughness | I | 18.0 | 9.9 | 8.4 | 6.3 | 5.3 |
| II | 19.4 | 10.8 | 8.0 | 6.3 | 3.8 | |
| III | 17.1 | 13.3 | 8.1 | 6.9 | 4.2 | |
| AVG. | 18.2 | 11.3 | 8.2 | 6.5 | 4.4 | |
| Relative Compactness (%) | 94.9 ± 0.5 | 97.3 ± 0.4 | 98.8 ± 0.2 | 99.5 ± 0.2 | 99.8 ± 0.1 | |
| Samples | UTS (MPa) | MS (%) | UD (mm) | YS (MPa) | YD (mm) | Elongation (%) |
|---|---|---|---|---|---|---|
| Ti-10Mo0.125 | 761 ± 18 | 34.20 | 9.576 | 730 | 1.68 | 30.3 |
| Ti-10Mo0.150 | 860 ± 15 | 37.59 | 10.526 | 752 | 1.91 | 32.9 |
| Ti-10Mo0.175 | 823 ± 15 | 35.95 | 10.066 | 714 | 2.88 | 28.4 |
| Ti-10Mo0.20 | 723 ± 10 | 31.02 | 8.686 | 685 | 2.54 | 23.4 |
| Ti-10Mo0.233 | 706 ± 10 | 29.70 | 8.316 | 573 | 1.88 | 25.6 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dai, X.; Sun, Y.; Han, J. Additive Manufacturing of High-Performance Ti-Mo Alloys Used on a Puncture Needle: The Role of Linear Energy Density in Microstructure Evolution and Mechanical Properties. Crystals 2025, 15, 149. https://doi.org/10.3390/cryst15020149
Dai X, Sun Y, Han J. Additive Manufacturing of High-Performance Ti-Mo Alloys Used on a Puncture Needle: The Role of Linear Energy Density in Microstructure Evolution and Mechanical Properties. Crystals. 2025; 15(2):149. https://doi.org/10.3390/cryst15020149
Chicago/Turabian StyleDai, Xuesong, Yue Sun, and Jitai Han. 2025. "Additive Manufacturing of High-Performance Ti-Mo Alloys Used on a Puncture Needle: The Role of Linear Energy Density in Microstructure Evolution and Mechanical Properties" Crystals 15, no. 2: 149. https://doi.org/10.3390/cryst15020149
APA StyleDai, X., Sun, Y., & Han, J. (2025). Additive Manufacturing of High-Performance Ti-Mo Alloys Used on a Puncture Needle: The Role of Linear Energy Density in Microstructure Evolution and Mechanical Properties. Crystals, 15(2), 149. https://doi.org/10.3390/cryst15020149





