A Comparison of the Hot Deformation Behavior and Constitutive Model of the GH4079 Alloy
Abstract
1. Introduction
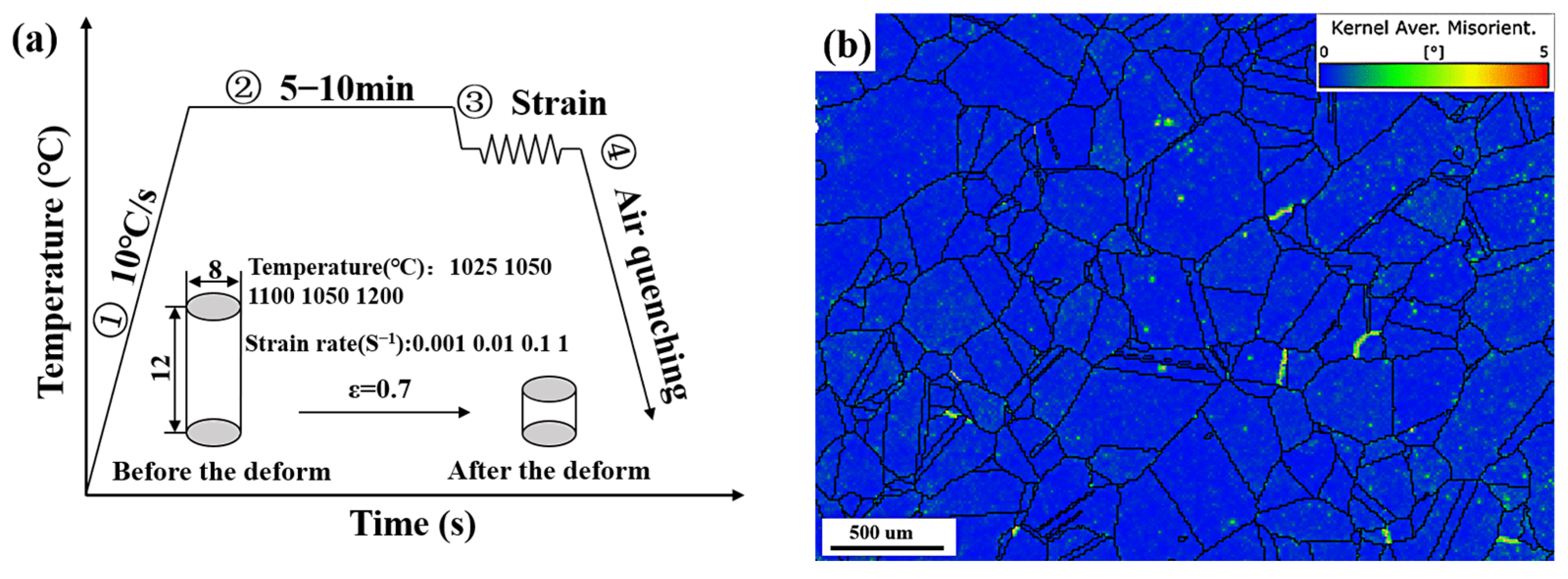
2. Experimental Materials and Methods
3. Results and Discussion
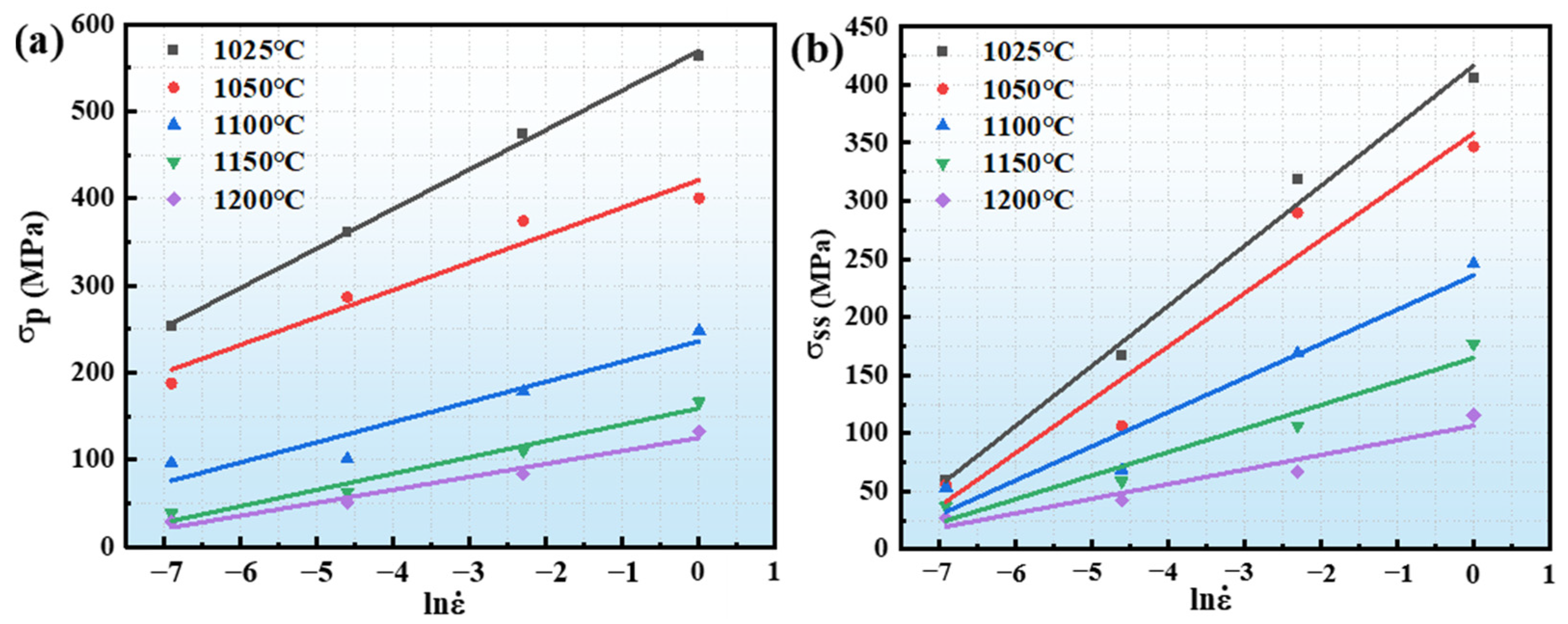
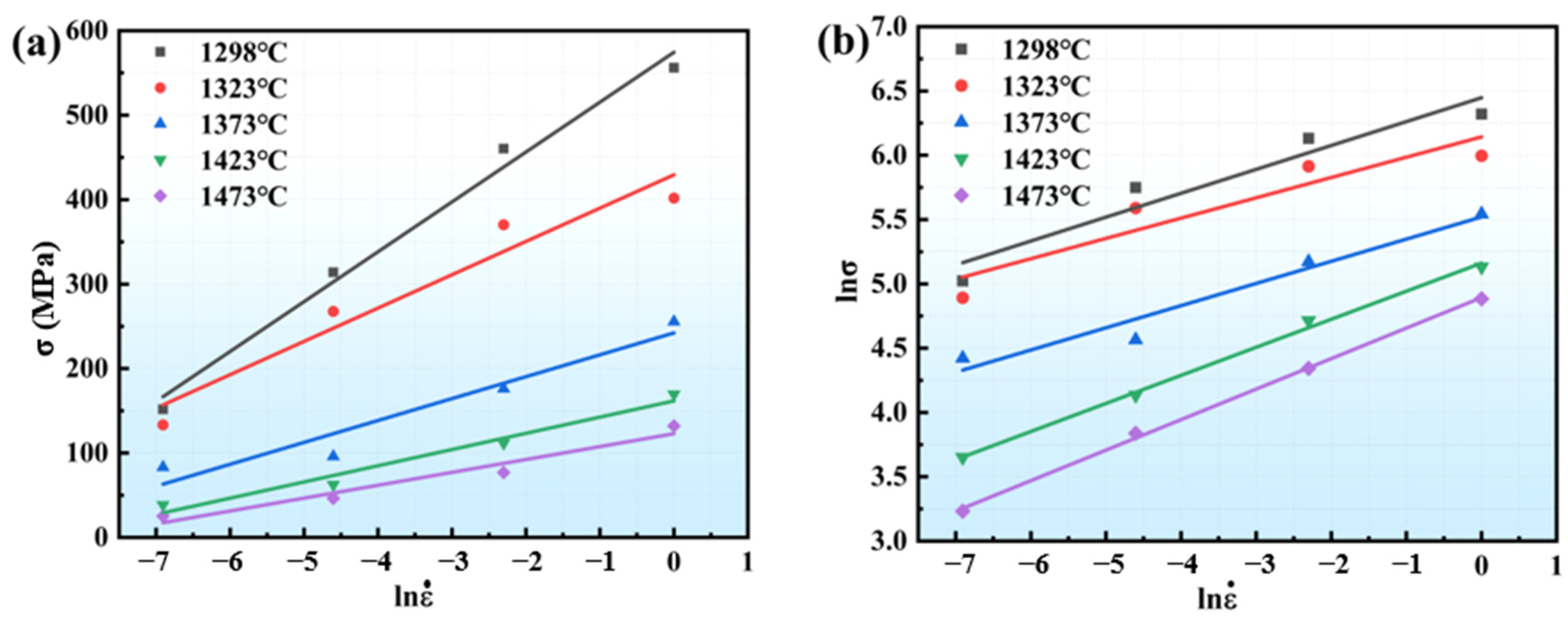
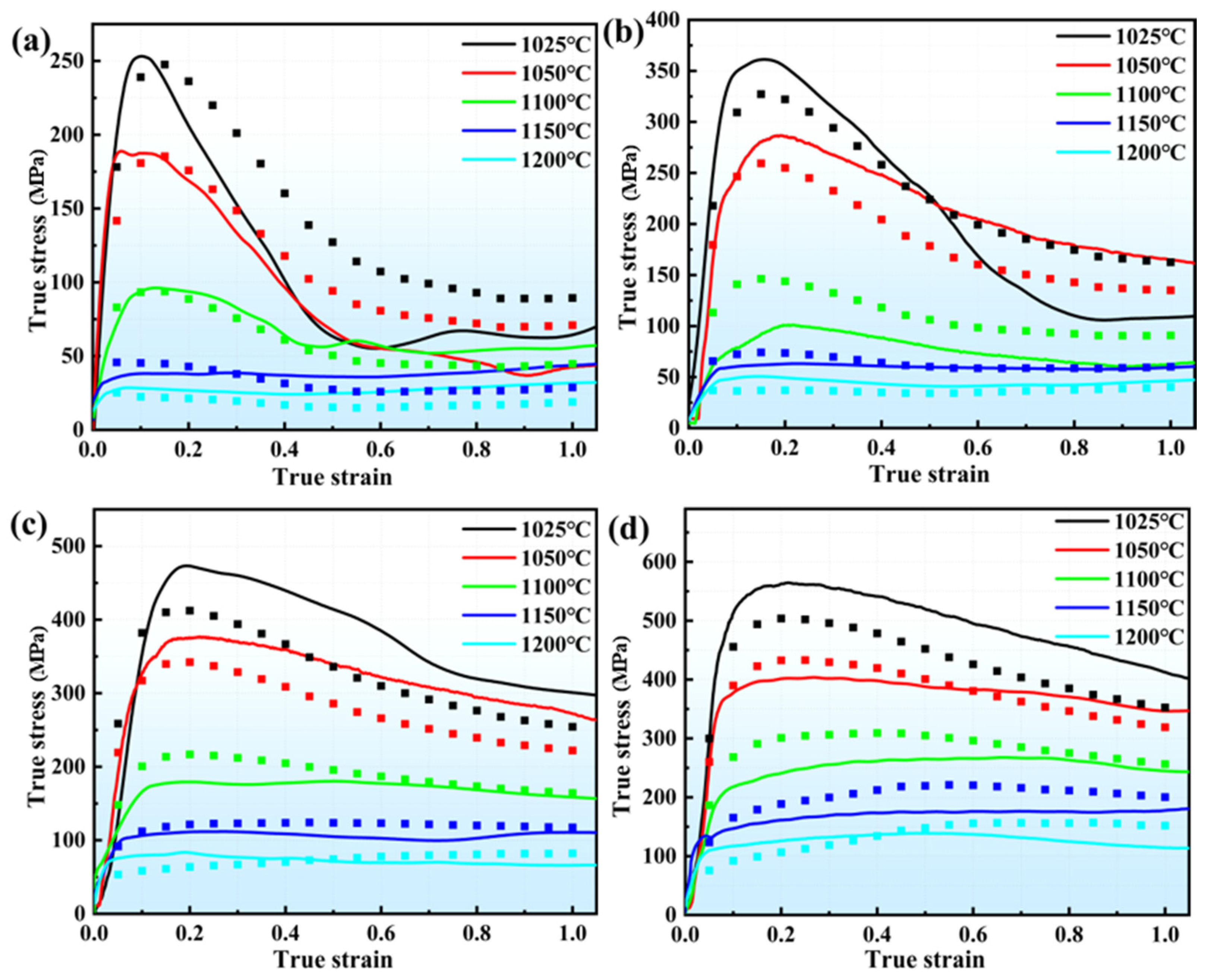
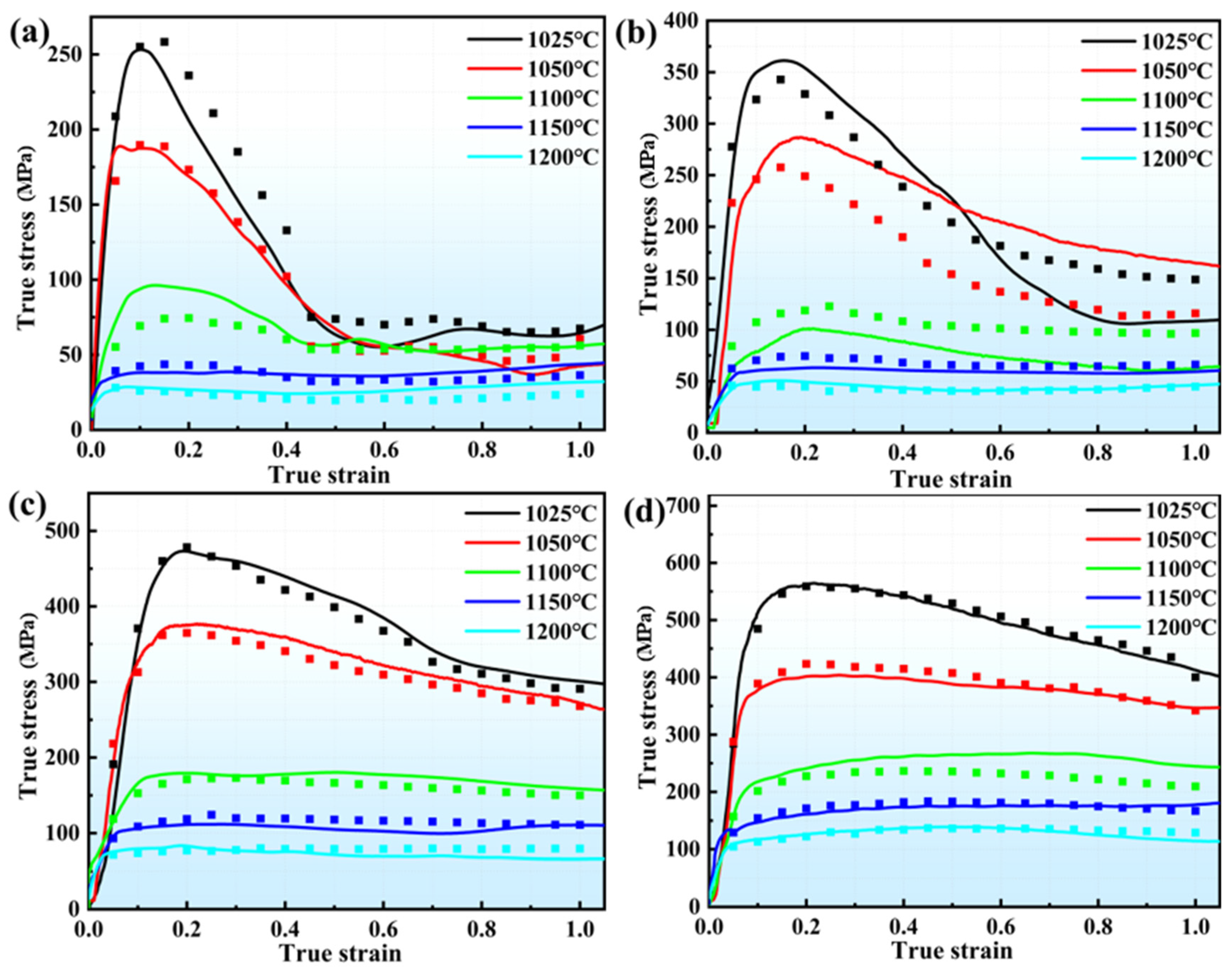
3.1. Rheological Behavior Analysis of GH4079 Alloy
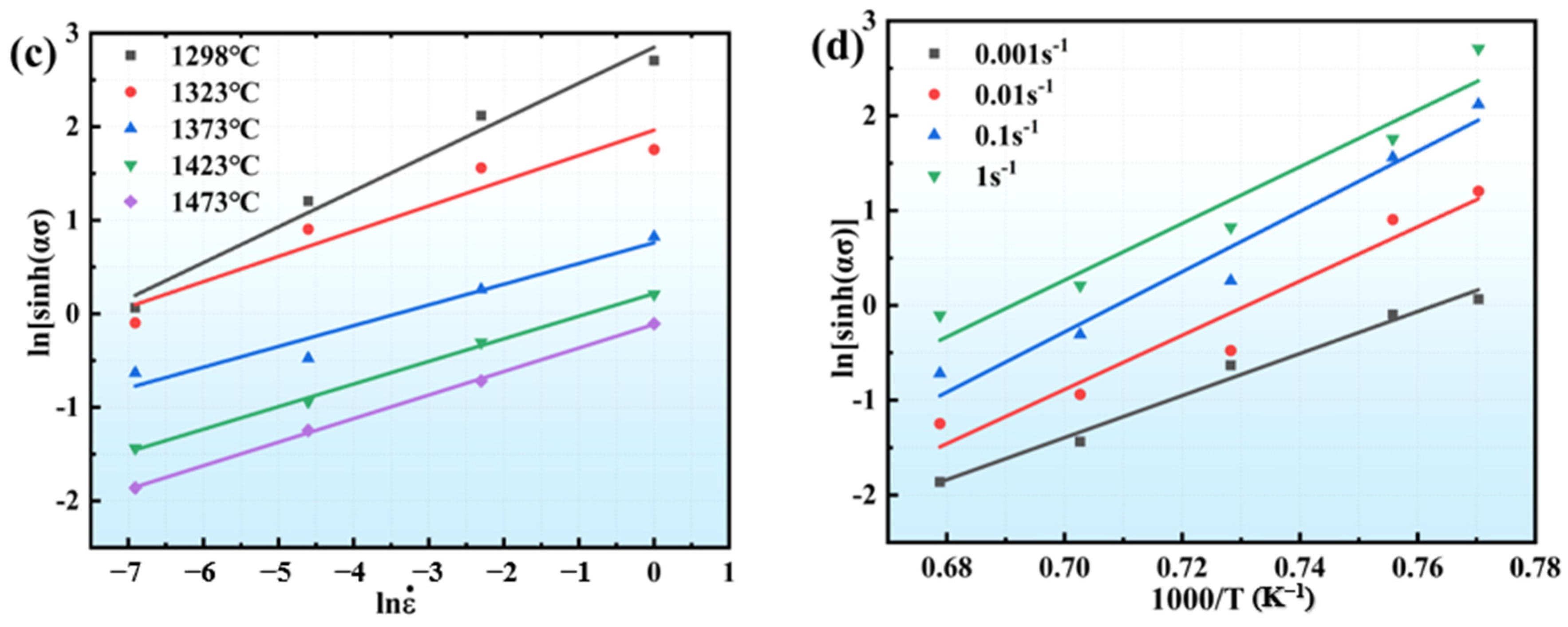
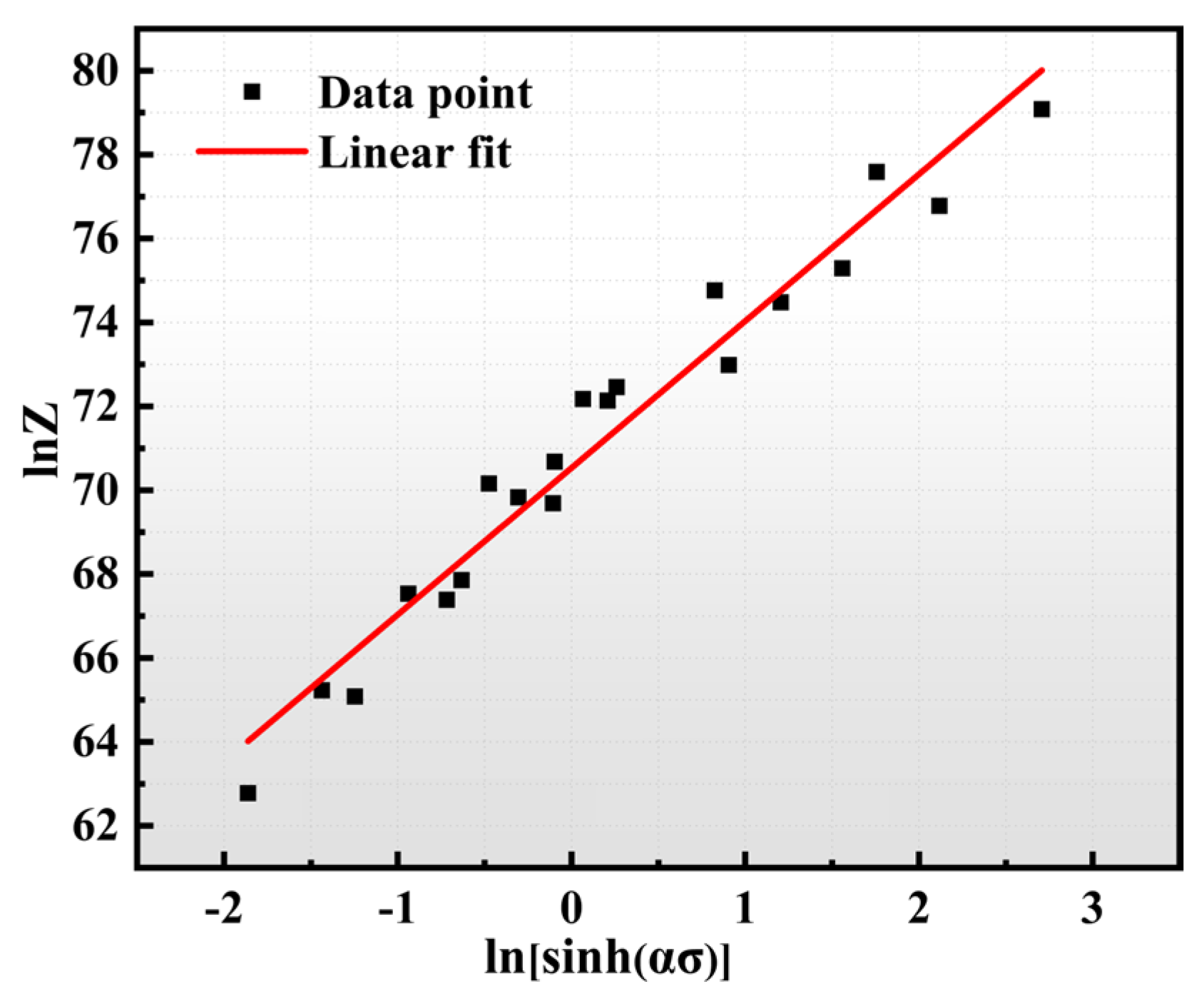
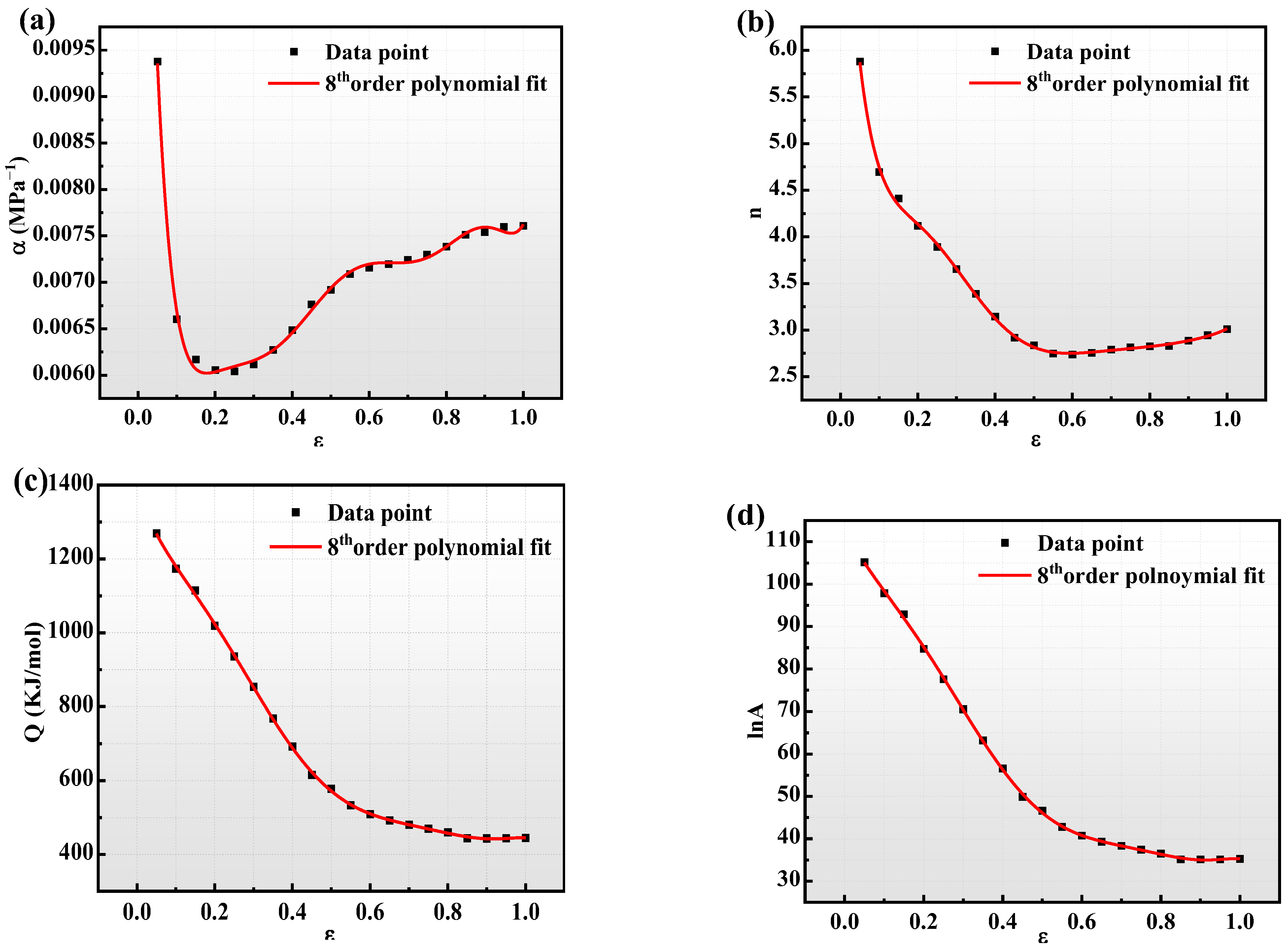
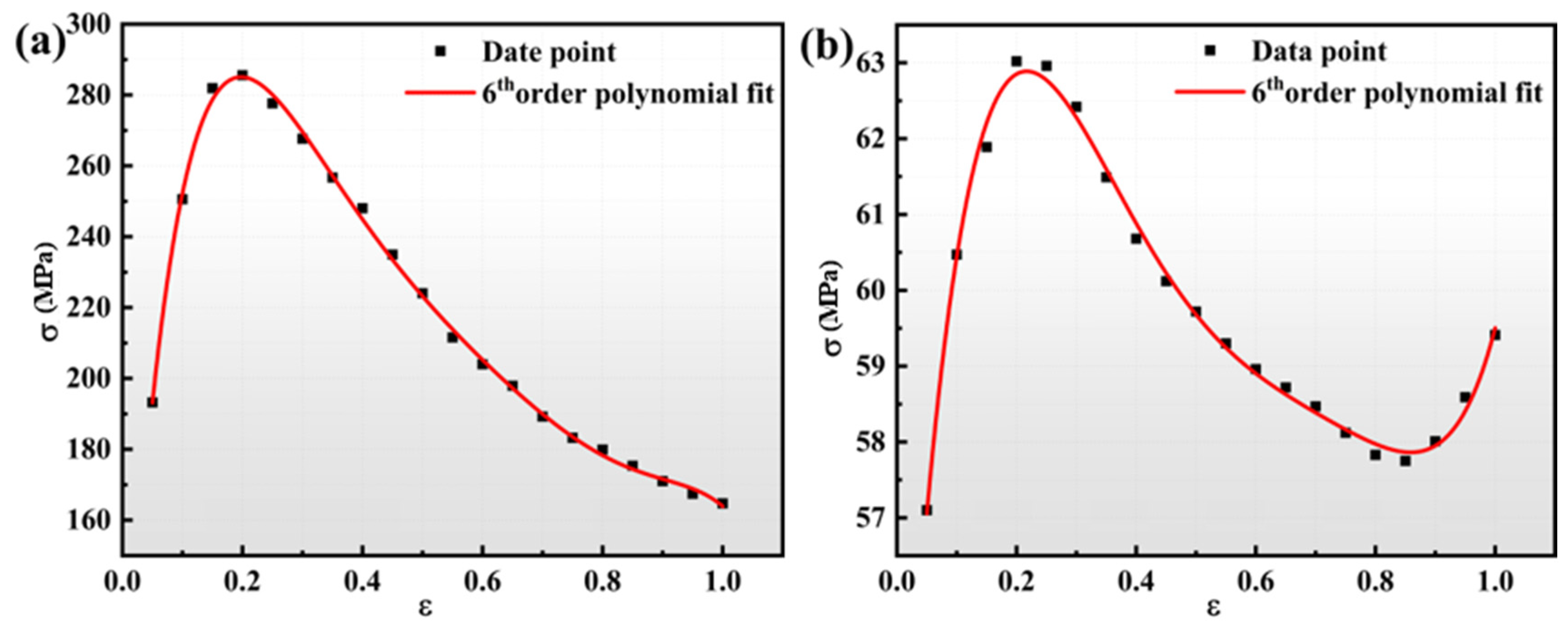
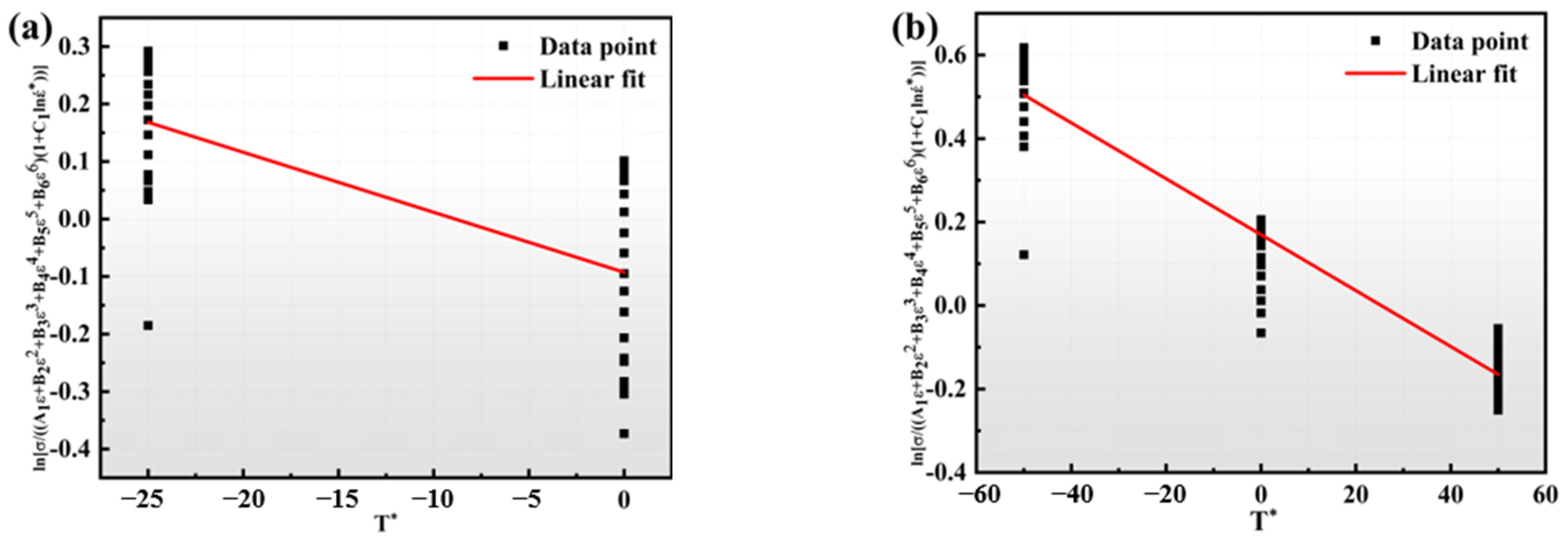
3.2. The Arrhenius Model of Strain Compensation
3.3. An Improved Johnson–Cook Model
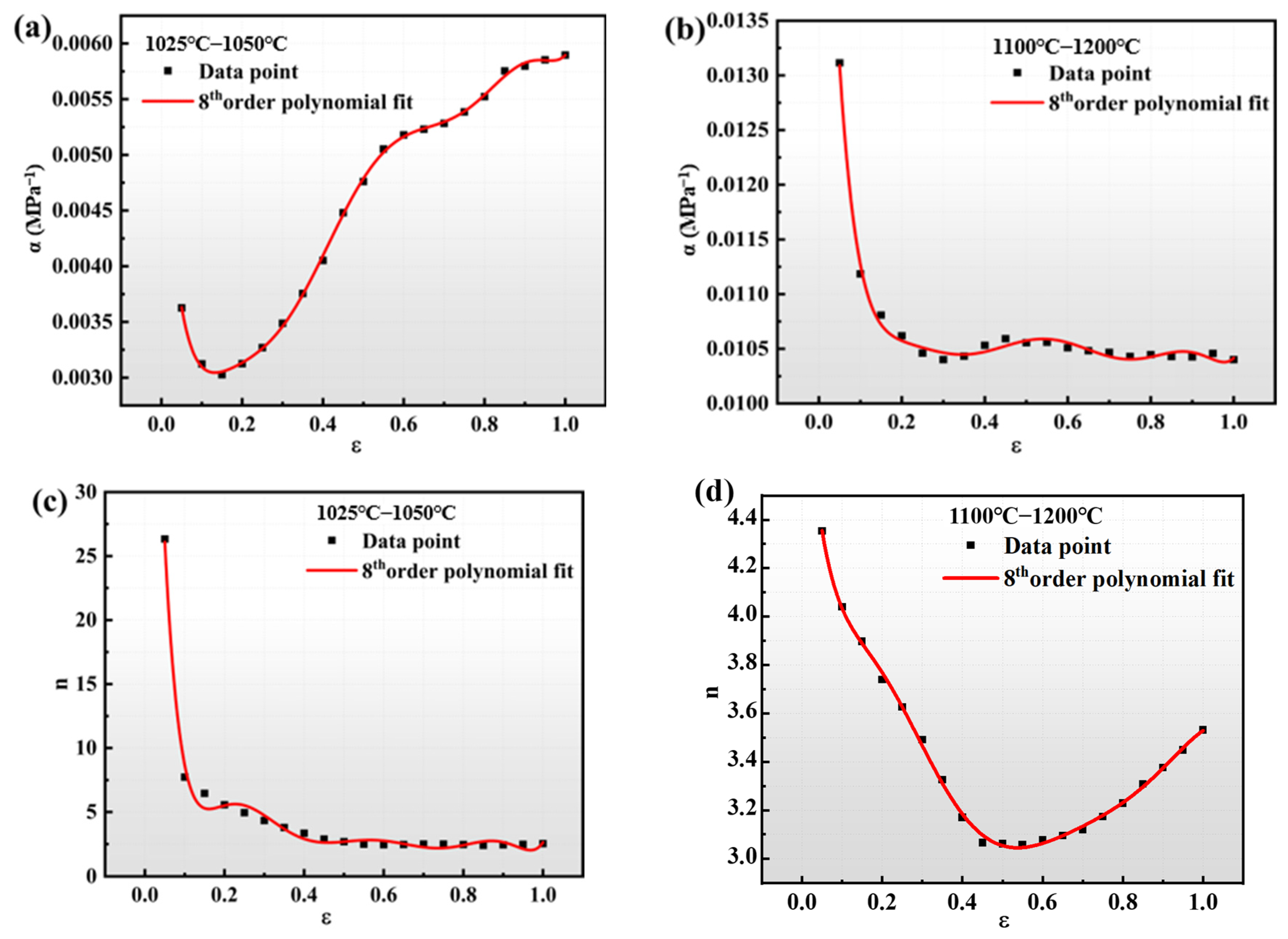
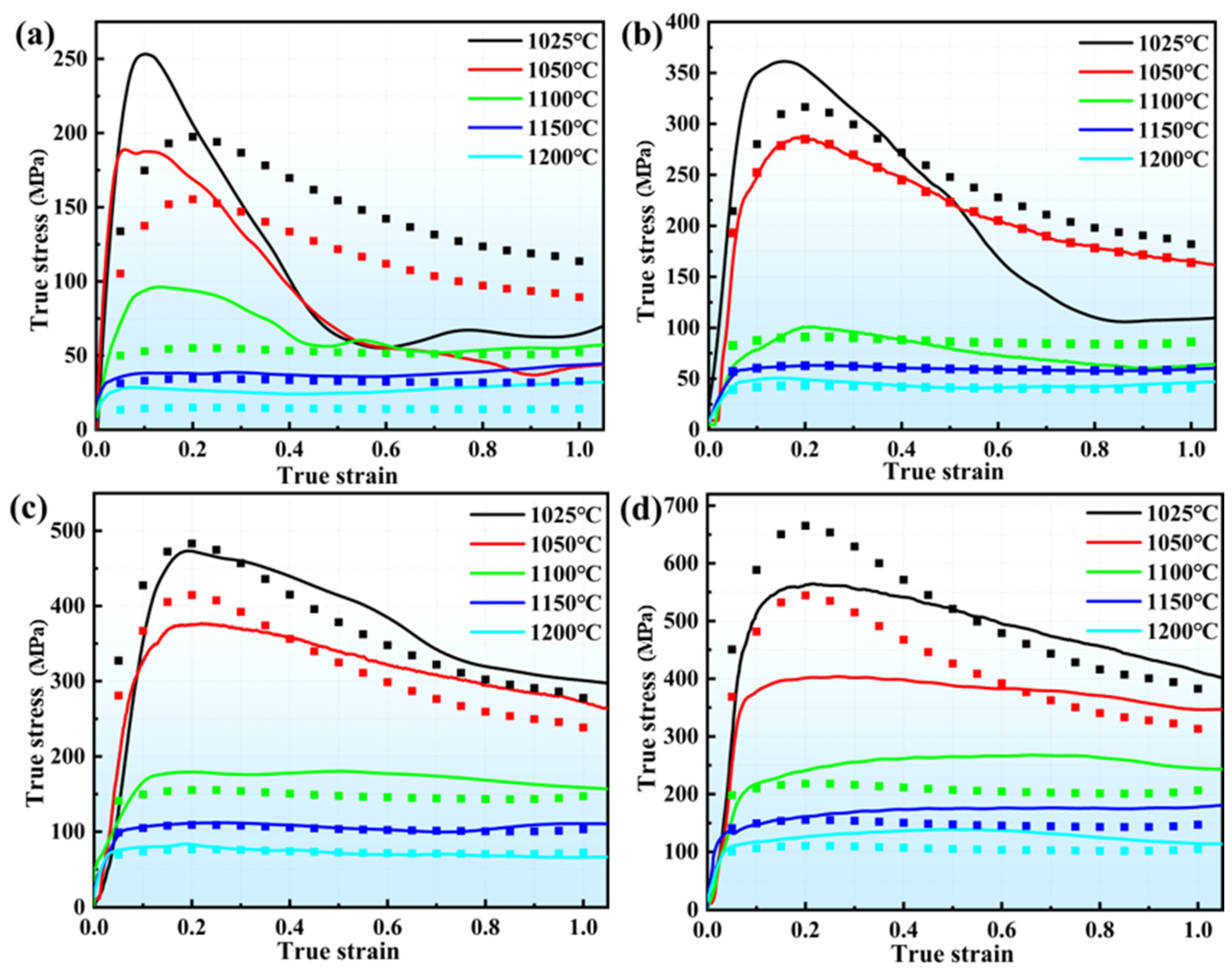
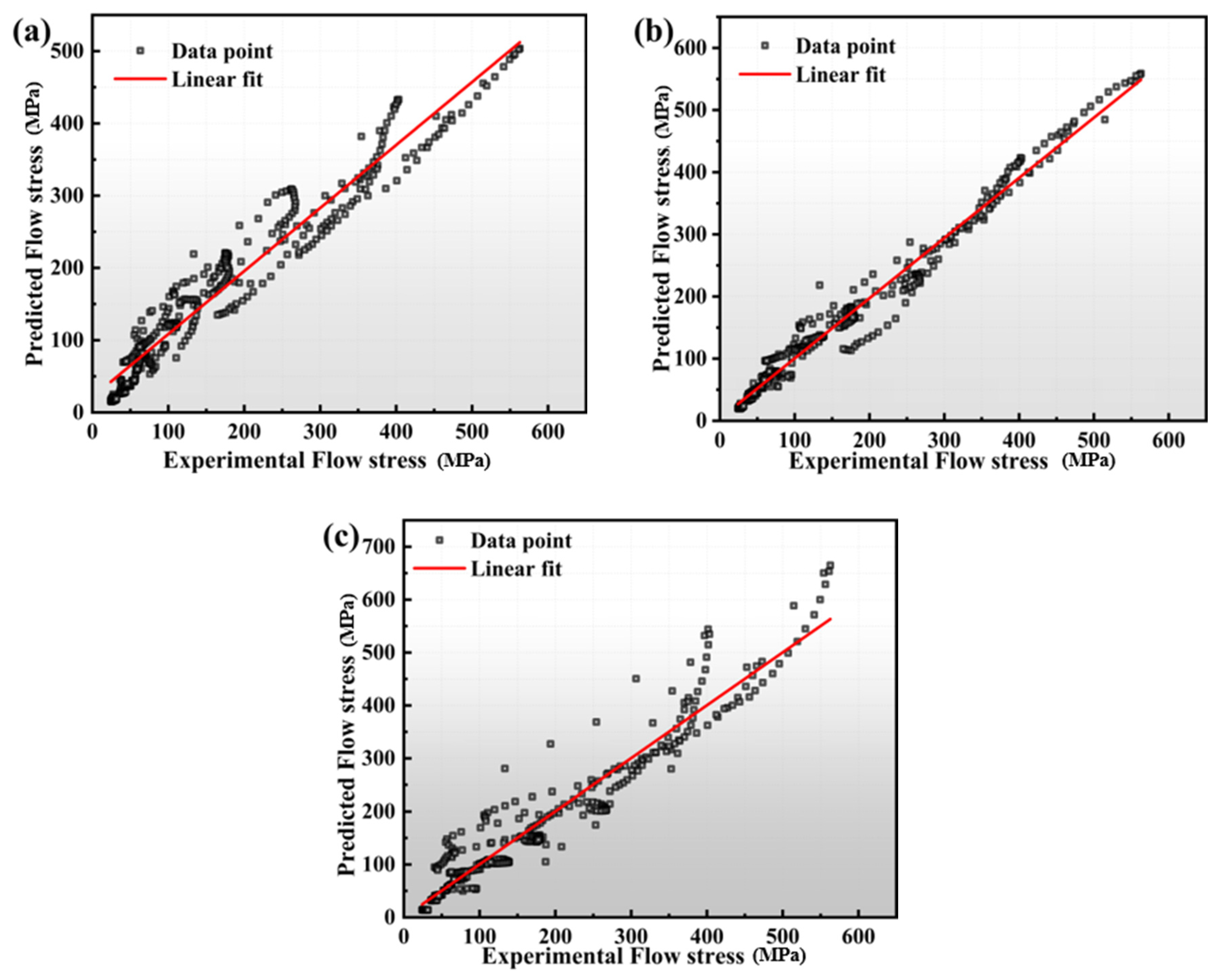
3.4. Comparison of Strain-Compensated Arrhenius Eigenmodes, Segmented Strain-Compensated Arrhenius Eigenmodes, and Improved J-C Model
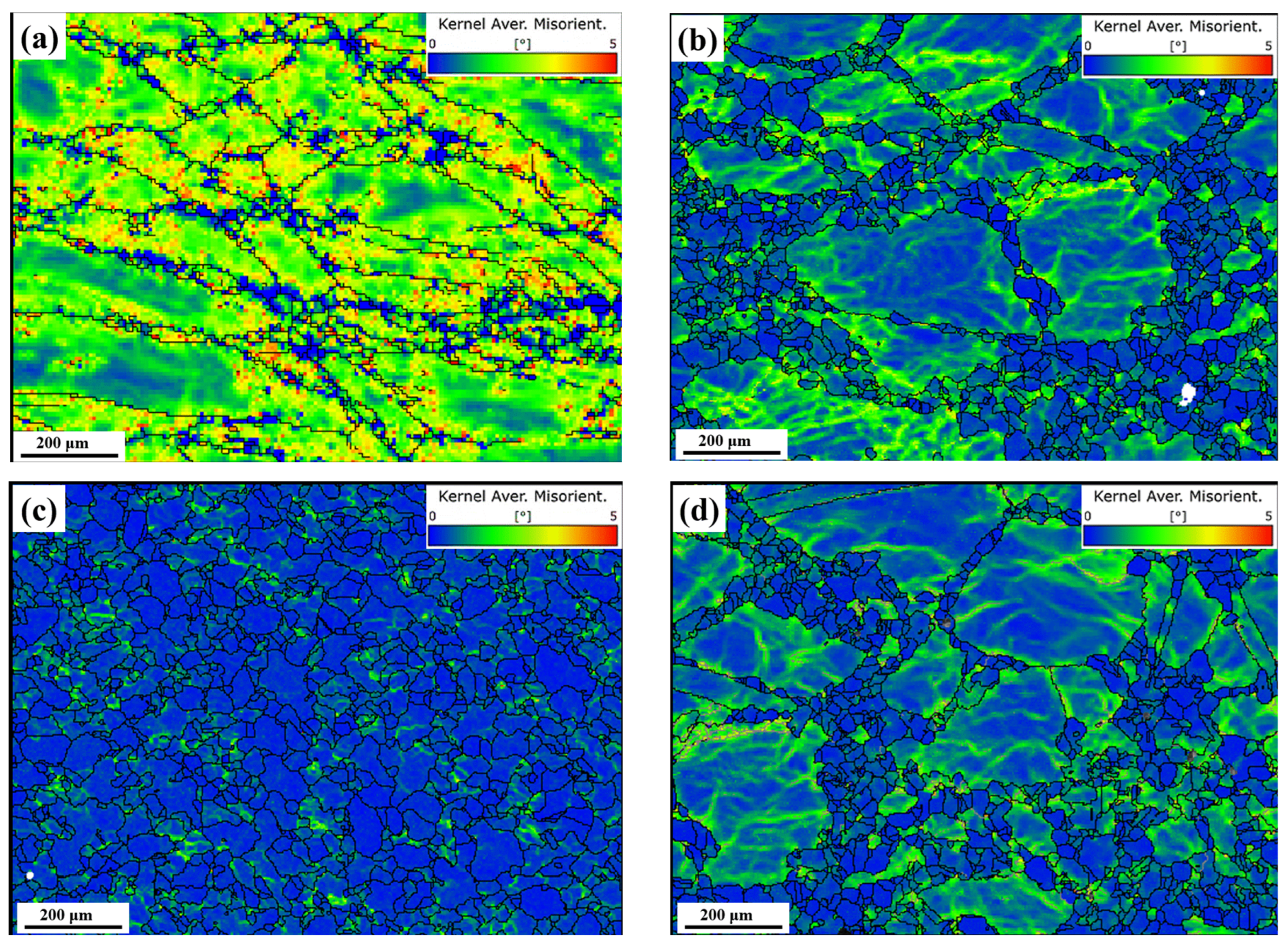
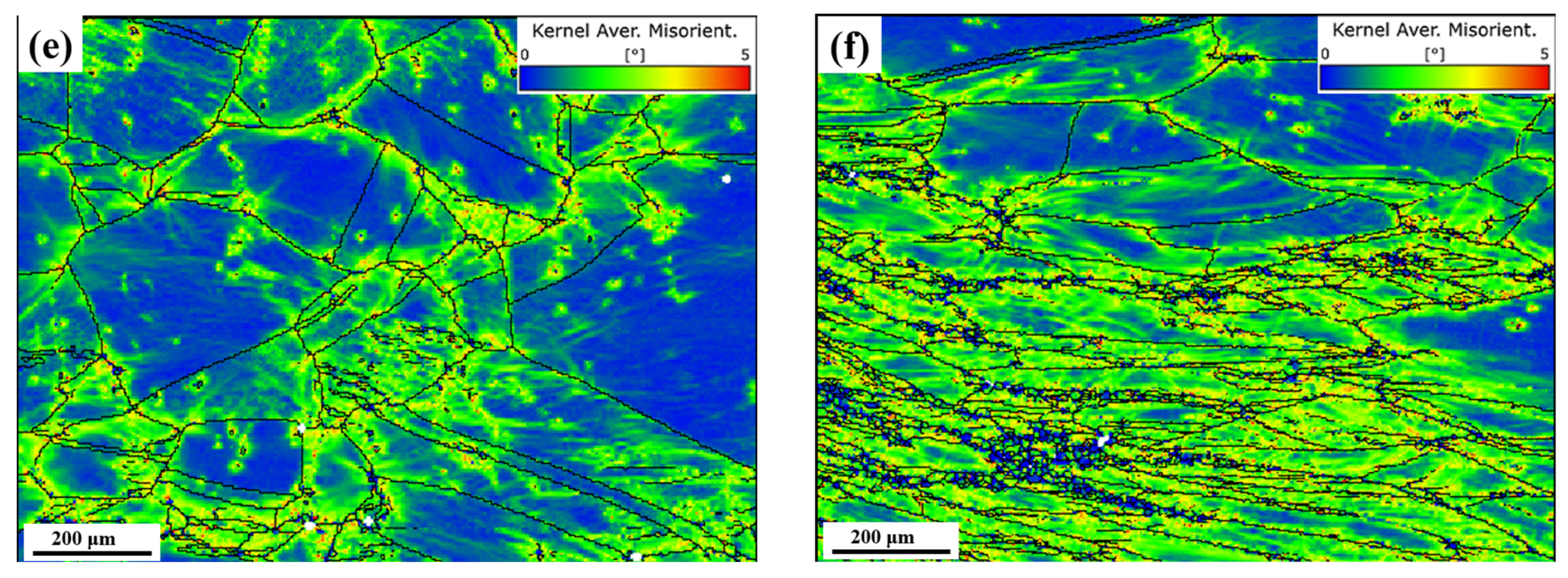
3.5. Microstructure Analysis
4. Conclusions
- In the thermal deformation experiment of alloy GH4079, the value of the flow stress is directly proportional to the deformation temperature and inversely proportional to the strain rate.
- The rheological stresses of the GH4079 alloy were computed by applying the strain-compensated Arrhenius constitutive equation, the Arrhenius constitutive equation based on the segmentation of the phase transition temperature, and the improved Johnson–Cook constitutive equation. The correlation coefficients of the three models were 0.94449, 0.9787, and 0.9274, respectively, and the AARE values were 21.09%, 10.62%, and 20.47%, respectively. Combining these three models, the Arrhenius constitutive equation based on phase transition temperature segmentation has the highest accuracy.
- The alloy identified as GH4079 demonstrates a significantly higher dislocation density during the thermal deformation process at low temperatures and high strain rates, which directly impacts the macroscopic rheological stress. Furthermore, the presence of precipitated phases plays a crucial role in influencing the rheological behavior of the material. Therefore, by integrating the Arrhenius constitutive equation based on the segmentation of the phase transition temperature with a high precision to predict the microstructural evolution of the alloy under various conditions, we can avoid cracking issues in practical applications. This approach not only enhances the material’s performance but also ensures reliability in demanding environments.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Liu, D.H.; Chai, H.R.; Yang, L.; Qiu, W.Q.; Guo, Z.H.; Wang, Z.L. Study on the dynamic recrystallization mechanisms of GH5188 superalloy during hot compression deformation. J. Alloys Compd. 2022, 895, 162565. [Google Scholar] [CrossRef]
- Miao, Q.; Ding, W.F.; Xu, J.H.; Cao, L.J.; Wang, H.C.; Yin, Z.; Dai, C.W.; Kuang, W.J. Creep feed grinding induced gradient microstructures in the superficial layer of turbine blade root of single crystal nickel-based superalloy. Int. J. Extreme Manuf. 2021, 3, 045102. [Google Scholar] [CrossRef]
- Cheng, Z.Y.; Zhao, Q.; Tao, M.J.; Du, J.J.; Huang, X.X.; Liu, C.J. Preparation of FeCoNi medium entropy al-loy from Fe3+-Co2+-Ni2+ solution system. Int. J. Min. Met. Mater. 2025, 32, 92–101. [Google Scholar] [CrossRef]
- Zhang, W.W.; Liu, X.G.; Lu, T.G.; Liu, Y.L.; Guo, Y.; Qin, H.Y.; Tian, Q. Microstructural evolution of GH4079 superalloy during hot deformation and heat treatment. J. Mater. Res. Technol. 2024, 33, 298–317. [Google Scholar] [CrossRef]
- Zhang, W.W.; Liu, X.A.; Du, Q.; Li, H.Z.; Qin, H.Y.; Tian, Q. Hot Deformation Behavior and Dynamic recrystallization mechanism transition of GH4742 Ni-Based superalloy under various deformation conditions. J. Mater. Eng. Perform. 2023, 32, 3253–3273. [Google Scholar] [CrossRef]
- Zhang, X.J.; Zhuo, G.; Zhang, H.Y.; Ma, X.; Gan, B.; Chen, L.J. Study of Processing Map of GH4079 Alloy Based on Different Instability Criteria. JOM 2024, 76, 3872–3887. [Google Scholar] [CrossRef]
- Sellars, C.M.; McTegart, W.J. On the mechanism of hot deformation. Acta Metall. 1966, 14, 1136–1138. [Google Scholar] [CrossRef]
- Johnson, G.R.; Cook, W.H. A constitutive model and data for metals subjected to large strains, high strain rates and high temperature. Eng. Fract. Mech. 1983, 21, 541–543. [Google Scholar]
- Fields, D.S.; Backofen, W.A. Determination of strain hardening characteristics by torsion testing. Proc. Am. Soc. Test Mater. 1957, 57, 1259–1272. [Google Scholar]
- Wang, H.; Qin, G.L.; Li, C.A. Modified Arrhenius constitutive model of 2219-O aluminum alloy based on hot compression with simulation verification. J. Mater. Res. Technol. 2022, 19, 3302–3320. [Google Scholar] [CrossRef]
- Harikrishna, K.; Bhowmik, A.; Davidson, M.J.; Kumar, R.; Anqi, A.E.; Rajhi, A.A.; Alamri, S.; Kumar, R. Evaluation of constitutive equations for modeling and characterization of microstructure during hot deformation of sintered Al-Zn-Mg alloy. J. Mater. Res. Technol. 2024, 28, 1523–1537. [Google Scholar] [CrossRef]
- Zhu, S.S.; Liu, J.; Deng, X. Modification of strain rate strengthening coefficient for Johnson-Cook constitutive model of Ti6Al4V alloy. Mater. Today Commun. 2021, 26, 102016. [Google Scholar] [CrossRef]
- Xie, G.L.; Yu, X.T.; Gao, Z.F.; Xue, W.L.; Zheng, L. The modified Johnson-Cook strain-stress constitutive model according to the deformation behaviors of a Ni-W-Co-C alloy. J. Mater. Res. Technol. 2022, 20, 1020–1027. [Google Scholar] [CrossRef]
- Jia, W.T.; Xu, S.; Le, Q.C.; Fu, L.; Ma, L.F.; Tang, Y. Modified Fields-Backofen model for constitutive behavior of as-cast AZ31B magnesium alloy during hot deformation. Mater. Des. 2018, 106, 120–132. [Google Scholar] [CrossRef]
- Shen, J.Y.; Hu, L.X.; Sun, Y.; Wan, Z.P.; Feng, X.Y.; Ning, Y.Q. A comparative study on artificial neural network, phenomenological-based constitutive and modified Fields-Backofen Models to predict flow stress in Ti-4Al-3V-2Mo-2Fe alloy. J. Mater. Eng. Perform. 2019, 28, 4302–4315. [Google Scholar] [CrossRef]
- Cai, Z.M.; Ji, H.C.; Pei, W.C.; Wang, B.Y.; Huang, X.M.; Li, Y.M. Constitutive equation and model validation for 33Cr23Ni8Mn3N heat-resistant steel during hot compression. Results Phys. 2019, 15, 102633. [Google Scholar] [CrossRef]
- He, A.; Xie, G.L.; Zhang, H.L.; Wang, X.T. A comparative study on Johnson-Cook, modified Johnson-Cook and Arrhenius-type constitutive models to predict the high-temperature flow stress in 20CrMo alloy steel. Mater. Des. 2013, 52, 677–685. [Google Scholar] [CrossRef]
- Song, Y.H.; Li, Y.G.; Li, H.Y.; Zhao, G.H.; Cai, Z.H.; Sun, M.X. Hot deformation and recrystallization behavior of a new nickel-base superalloy for ultra-supercritical applications. J. Mater. Res. Technol. 2022, 19, 4308–4324. [Google Scholar] [CrossRef]
- Li, Y.J.; Zhang, Y.; Chen, Z.Y.; Ji, Z.C.; Zhu, H.Y.; Sun, C.F.; Dong, W.P.; Li, X.; Sun, Y.; Yao, S. Hot deformation behavior and dynamic recrystallization of GH690 nickel-based superalloy. J. Alloys Compd. 2020, 847, 156507. [Google Scholar] [CrossRef]
- Wu, B.; Li, M.Q.; Ma, D.W. The flow behavior and constitutive equations in isothermal compression of 7050 aluminum alloy. Mater. Sci. Eng. A 2012, 542, 79–87. [Google Scholar] [CrossRef]
- Jia, D.; Sun, W.R.; Xu, D.S.; Yu, L.X.; Xin, X.; Zhang, W.H.; Qi, F. Abnormal dynamic recrystallization behavior of a nickel-based superalloy during hot deformation. J. Alloys Compd. 2019, 787, 196–205. [Google Scholar] [CrossRef]
- Huang, M.J.; Jiang, J.F.; Wang, Y.; Liu, Y.Z.; Zhang, Y.; Dong, J.; Tong, Z.Y. Unraveling hot deformation behavior and microstructure evolution, flow stress prediction of powder metallurgy BCC/B2 Al1.8CrCuFeNi2 HEA. J. Alloys Compd. 2024, 972, 172828. [Google Scholar] [CrossRef]
- Ashtiani, H.R.; Shahsavari, P. A comparative study on the phenomenological and artificial neural network models to predict hot deformation behavior of AlCuMgPb alloy. J. Alloys Compd. 2016, 687, 263–273. [Google Scholar] [CrossRef]
- Amitava, R.; Mohammad, A.; Satyabrata, D.; Rupa, D. Constitutive modeling for predicting high-temperature flow behavior in aluminum 5083 + 10 wt Pct SiCP composite. Metall. Mater. Trans. B 2019, 50, 1060–1076. [Google Scholar]
- Zhang, J.B.; Wu, C.J.; Peng, Y.Y.; Xia, X.C.; Li, J.A.; Ding, J.; Liu, C.; Chen, X.G.; Dong, J.; Liu, Y.C. Hot compression deformation behavior and processing maps of ATI 718Plus superalloy. J. Alloys Compd. 2020, 835, 155195. [Google Scholar] [CrossRef]
- Li, N.; Zhang, F.G.; Yang, Q.; Wu, Y.; Wang, M.L.; Liu, J.; Wang, L.; Chen, Z.; Wang, H.W. Microstructure and Mechanical Properties of In Situ TiB2/2024 Composites Fabricated by Powder Metallurgy. J. Mater. Eng. Perform. 2022, 31, 8775–8783. [Google Scholar] [CrossRef]
- Zhong, X.T.; Wang, L.; Huang, L.K.; Liu, F. Transition of dynamic recrystallization mechanism during hot deformation of Incoloy 028 alloy. J. Mater. Sci. Technol. 2020, 42, 241–253. [Google Scholar] [CrossRef]
- Lin, Y.C.; Wu, X.Y.; Chen, X.M.; Chen, J.; Wen, D.X.; Zhang, J.L.; Li, L.T. EBSD study of a hot deformed nickel-based superalloy. J. Alloys Compd. 2015, 640, 101–113. [Google Scholar] [CrossRef]
- Li, H.Y.; Feng, W.; Zhuang, W.H.; Hua, L. Microstructure analysis and segmented constitutive model for Ni-Cr-Co-based superalloy during hot deformation. Metals 2022, 12, 357. [Google Scholar] [CrossRef]
- Sun, B.; Zhang, T.B.; Song, L.; Zhang, L. Microstructural evolution and dynamic recrystallization of a nickel-based superalloy PM EP962NP during hot deformation at 1150 °C. J. Mater. Res. Technol. 2022, 18, 1436–1449. [Google Scholar] [CrossRef]
- Chen, X.M.; Lin, Y.C.; Wen, D.X.; Zhan, J.L.; He, M. Dynamic recrystallization behavior of a typical nickel-based superalloy during hot deformation. Mater. Des. 2014, 57, 568–577. [Google Scholar] [CrossRef]
- Mi, J.; Ma, D.Q.; Luan, S.Y.; Jin, P.P.; Chen, L.J.; Che, X.; Li, X.Q. The hot deformation behavior and microstructural evolution of as-cast Mg-2Zn-0.5Mn-0.2Ca-0.3Nd-0.7La alloy. J. Mater. Res. Technol. 2023, 22, 1533–1545. [Google Scholar] [CrossRef]
- Qin, D.H.; Wang, M.J.; Sun, C.Y.; Su, Z.X.; Qian, L.Y.; Sun, Z.H. Interaction between texture evolution and dynamic recrystallization of extruded AZ80 magnesium alloy during hot deformation. Mater. Sci. Eng. A 2020, 788, 139537. [Google Scholar] [CrossRef]
- Huang, K.; Marthinsen, K.; Zhao, Q.L.; Logé, R.E. The double-edge effect of second-phase particles on the recrystallization behavior and associated mechanical properties of metallic materials. Prog. Mater. Sci. 2018, 92, 284–359. [Google Scholar] [CrossRef]



| Component | Co | Cr | Nb | Ti | Cu | Mo | V | Fe | C | Ni |
|---|---|---|---|---|---|---|---|---|---|---|
| Content (wt%) | 15.46 | 10.05 | 8.97 | 6.18 | 4.76 | 4.11 | 0.62 | 0.3 | 0.062 | col |
| α (MPa−1) | n | Q (KJ mol−1) | lnA |
|---|---|---|---|
| C0 = 0.02 | D0 = 8.77 | E0 = 1390.47 | F0 = 112.80 |
| C1 = −0.24 | D1 = 86.36 | E1 = 3018.07 | F1 = 171.52 |
| C2 = 2.18 | D2 = 707.90 | E2 = 14,210.70 | F2 = 401.95 |
| C3 = 10.47 | D3 = 3150.32 | E3 = −60,499.50 | F3 = 1127.99 |
| C4 = 9.53 | D4 = 7933.88 | E4 = 104,325.11 | F4 = −2803.14 |
| C5 = 49.97 | D5 = 11,755.61 | E5 = 23,424.29 | F5 = 18,269.46 |
| C6 = 9.72 | D6 = 1021.31 | E6 = −129,786.29 | F6 = −31,620.63 |
| C7 = 26.76 | D7 = 4810.79 | E7 = 143,382.31 | F7 = 23,460.72 |
| C8 = 6.00 | D8 = 954.24 | E8 = −46,135.07 | F8 = −6486.37 |
| α(C0–C8) | n(D0–D8) | Q(E0–E8) | lnA(F0–F8) | ||||
|---|---|---|---|---|---|---|---|
| 1025~ 1050 °C | 1100~ 1200 °C | 1025~ 1050 °C | 1100~ 1200 °C | 1025~ 1050 °C | 1100~ 1200 °C | 1025~ 1050 °C | 1100~ 1200 °C |
| 0.006 | 0.02 | 80.06 | 5.18 | 1338.79 | 156.62 | 128.91 | 8.29 |
| 0.07 | 0.15 | 1682.94 | 25.01 | 6686.56 | 10,975.60 | 322.88 | 954.35 |
| 0.69 | 1.29 | 15,234.63 | 215.59 | −58,704.68 | −76,924.14 | −2399.62 | 6810.22 |
| −3.75 | −6.09 | −72,271.63 | −1037.04 | 37,636.11 | 284,443.42 | −11,921.3 | 25,781.30 |
| 11.94 | 17.00 | 197,440.80 | 2733.40 | 636,325.02 | −662,127.4 | 102,319.3 | −61,288.03 |
| −22.25 | −28.62 | −322,838.02 | −4119.27 | −2,195,60 | 996,362.92 | −273,86 | 93,601.33 |
| 23.79 | 28.41 | 310,878.90 | 3557.00 | −3,416,50 | −929,397.5 | 356,953.22 | −88,092.06 |
| 13.49 | −15.28 | −162,742.11 | −1637.41 | −2,143,20 | 483,704.49 | −230,762.8 | 46,071.77 |
| 3.14 | 3.43 | 35,702.95 | 311.09 | 569,531.60 | 106,662.12 | 592,557.02 | −10,185.35 |
| A1 | B1 | B2 | B3 | B4 | B5 | B6 | C1 | λ1 | λ2 | |
|---|---|---|---|---|---|---|---|---|---|---|
| Before the phase change | 84.18 | 2818.39 | 14,405.73 | 34,424.15 | 44,315.63 | 29,512.70 | 7954.10 | 0.20 | −0.004 | −1.93 |
| After the phase change | 51.42 | 139.81 | −539.83 | 1067.12 | −868.83 | 230.55 | 33.28 | 0.32 | −0.007 | 0.00013 |
| T/K | /s−1 | AARE (A) | AARE (B) | AARE (C) |
|---|---|---|---|---|
| 1298 | 0.001 | 0.48 | 0.13 | 0.78 |
| 0.01 | 0.16 | 0.19 | 0.36 | |
| 0.1 | 0.16 | 0.03 | 0.10 | |
| 1 | 0.13 | 0.02 | 0.10 | |
| 1323 | 0.001 | 0.36 | 0.10 | 0.70 |
| 0.01 | 0.44 | 0.25 | 0.01 | |
| 0.1 | 0.18 | 0.07 | 0.14 | |
| 1 | 0.05 | 0.03 | 0.17 | |
| 1373 | 0.001 | 0.12 | 0.09 | 0.18 |
| 0.01 | 0.44 | 0.37 | 0.18 | |
| 0.1 | 0.11 | 0.06 | 0.15 | |
| 1 | 0.14 | 0.11 | 0.19 | |
| 1423 | 0.001 | 0.23 | 0.11 | 0.14 |
| 0.01 | 0.06 | 0.13 | 0.01 | |
| 0.1 | 0.13 | 0.08 | 0.03 | |
| 1 | 0.19 | 0.04 | 0.13 | |
| 1473 | 0.001 | 0.33 | 0.18 | 0.48 |
| 0.01 | 0.18 | 0.04 | 0.07 | |
| 0.1 | 0.16 | 0.11 | 0.04 | |
| 1 | 0.18 | 0.04 | 0.17 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ying, W.; Hou, J.; Jiang, S.; Wang, J. A Comparison of the Hot Deformation Behavior and Constitutive Model of the GH4079 Alloy. Crystals 2025, 15, 148. https://doi.org/10.3390/cryst15020148
Ying W, Hou J, Jiang S, Wang J. A Comparison of the Hot Deformation Behavior and Constitutive Model of the GH4079 Alloy. Crystals. 2025; 15(2):148. https://doi.org/10.3390/cryst15020148
Chicago/Turabian StyleYing, Weifeng, Jia Hou, Shengnan Jiang, and Jianan Wang. 2025. "A Comparison of the Hot Deformation Behavior and Constitutive Model of the GH4079 Alloy" Crystals 15, no. 2: 148. https://doi.org/10.3390/cryst15020148
APA StyleYing, W., Hou, J., Jiang, S., & Wang, J. (2025). A Comparison of the Hot Deformation Behavior and Constitutive Model of the GH4079 Alloy. Crystals, 15(2), 148. https://doi.org/10.3390/cryst15020148





